Evaluation of Mixing Effect on Coupled Heat Release and Transfer Performance of a Novel Segregated Solid Rocket Motor
Abstract
1. Introduction
2. Formulation of the Model
2.1. Conservation Equations
2.1.1. Equations of Gas Phase
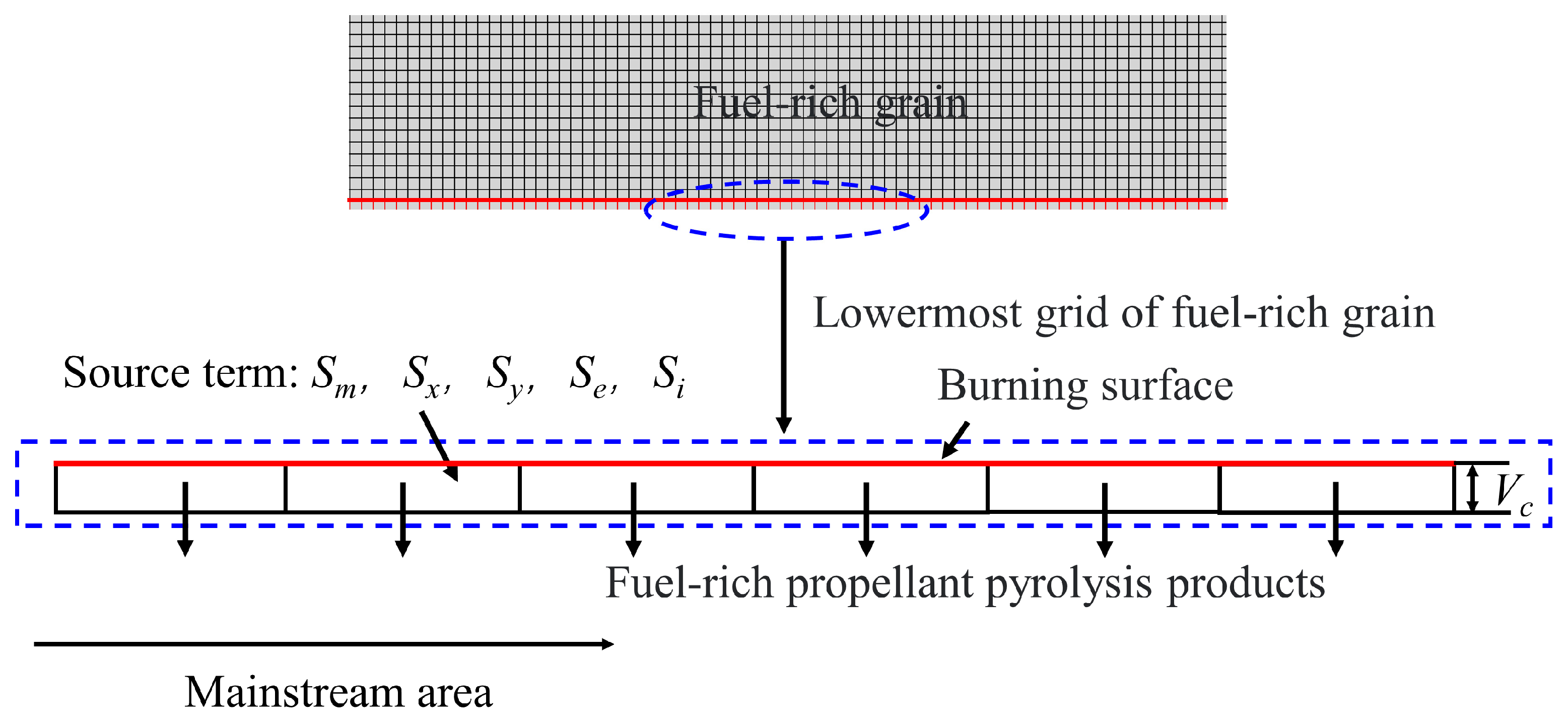
2.1.2. Gas-Solid Boundary Conditions
2.1.3. Thermal Decomposition Model of Propellant
2.1.4. Turbulence Model
2.2. Combustion Reaction Model
2.2.1. Detailed Reaction Mechanism
2.2.2. Regression Rate Model
2.3. Model Formulation and Boundary Conditions
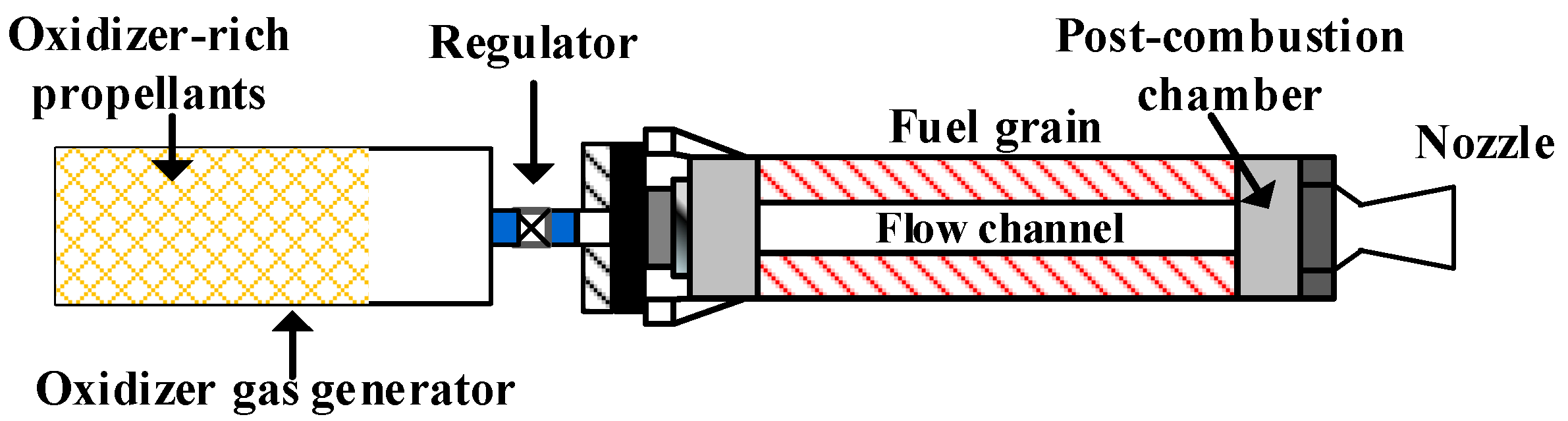
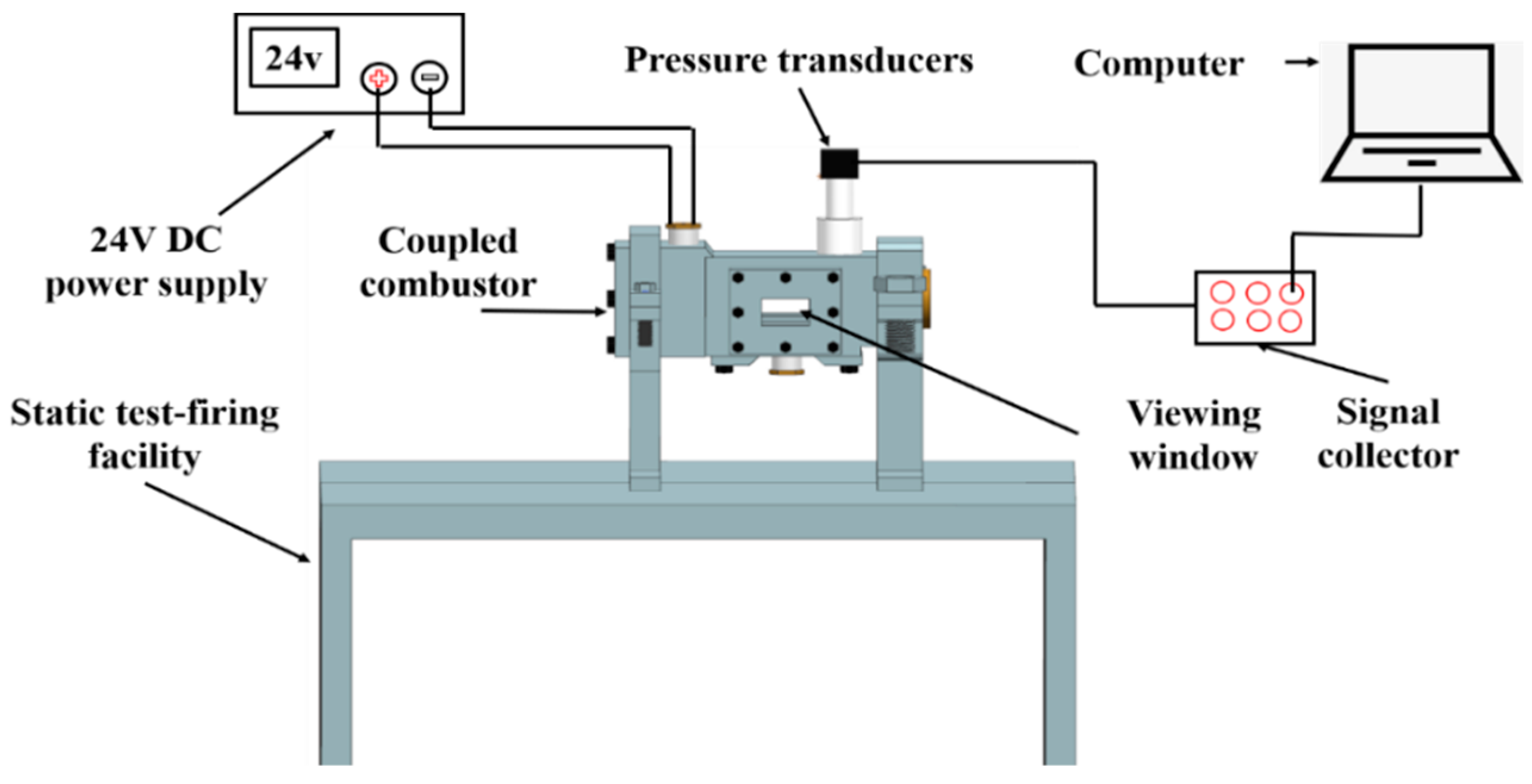
2.3.1. Physical Model of the Segregated Solid Motor
2.3.2. Boundary Conditions for Numerical Simulation
2.3.3. Cases and Conditions for Numerical Simulation
3. Results and Discussion
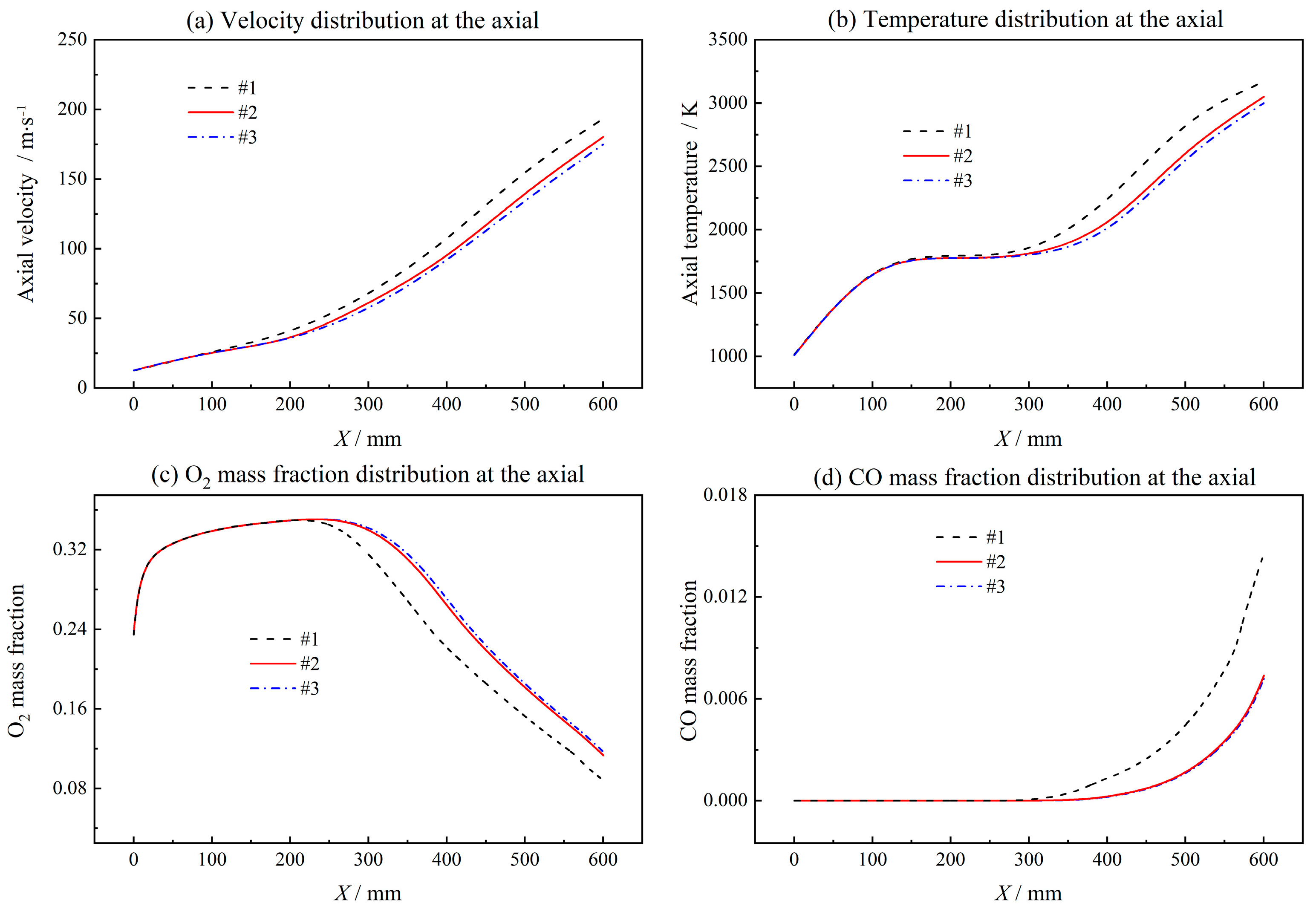
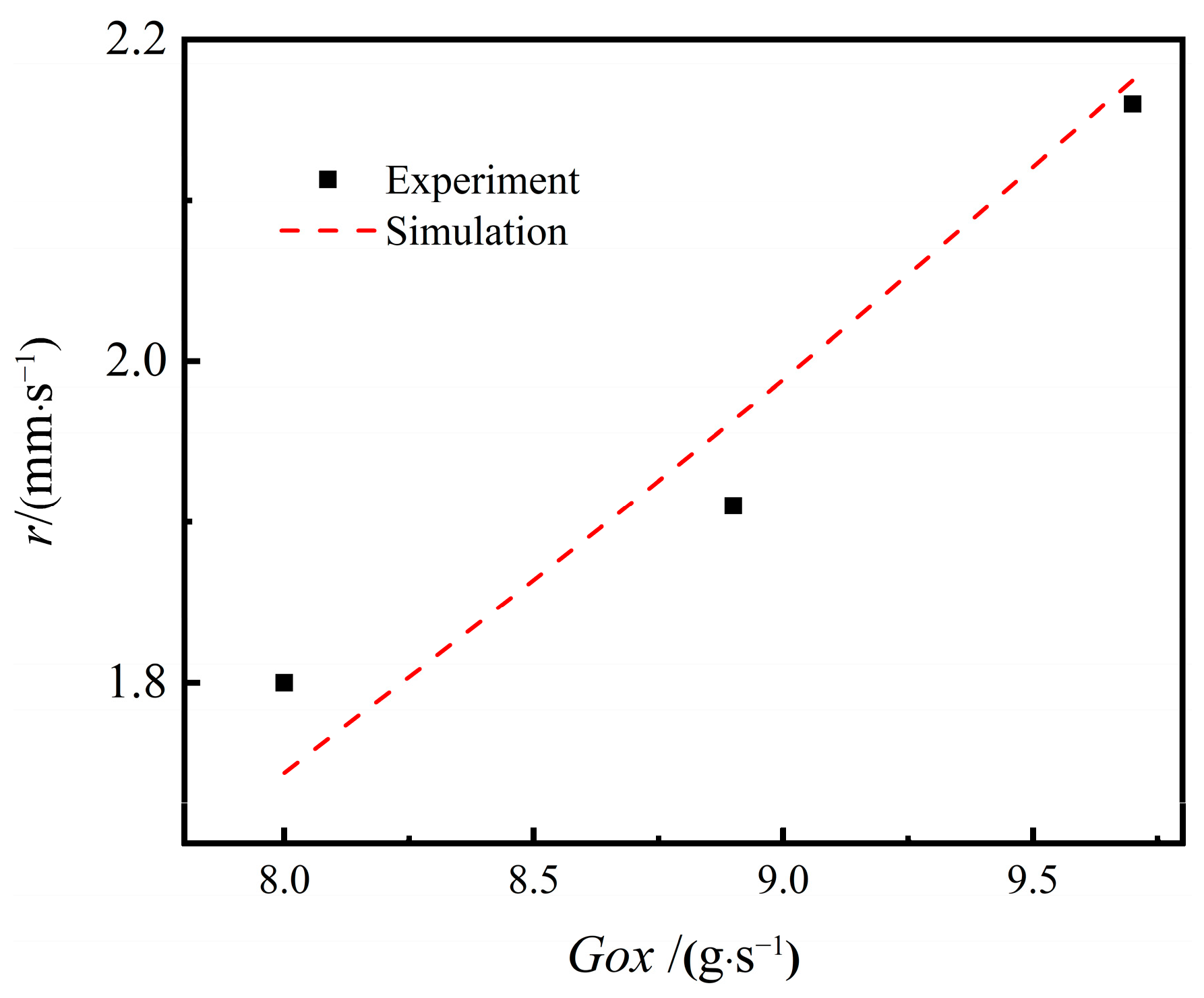
3.1. Model Verification
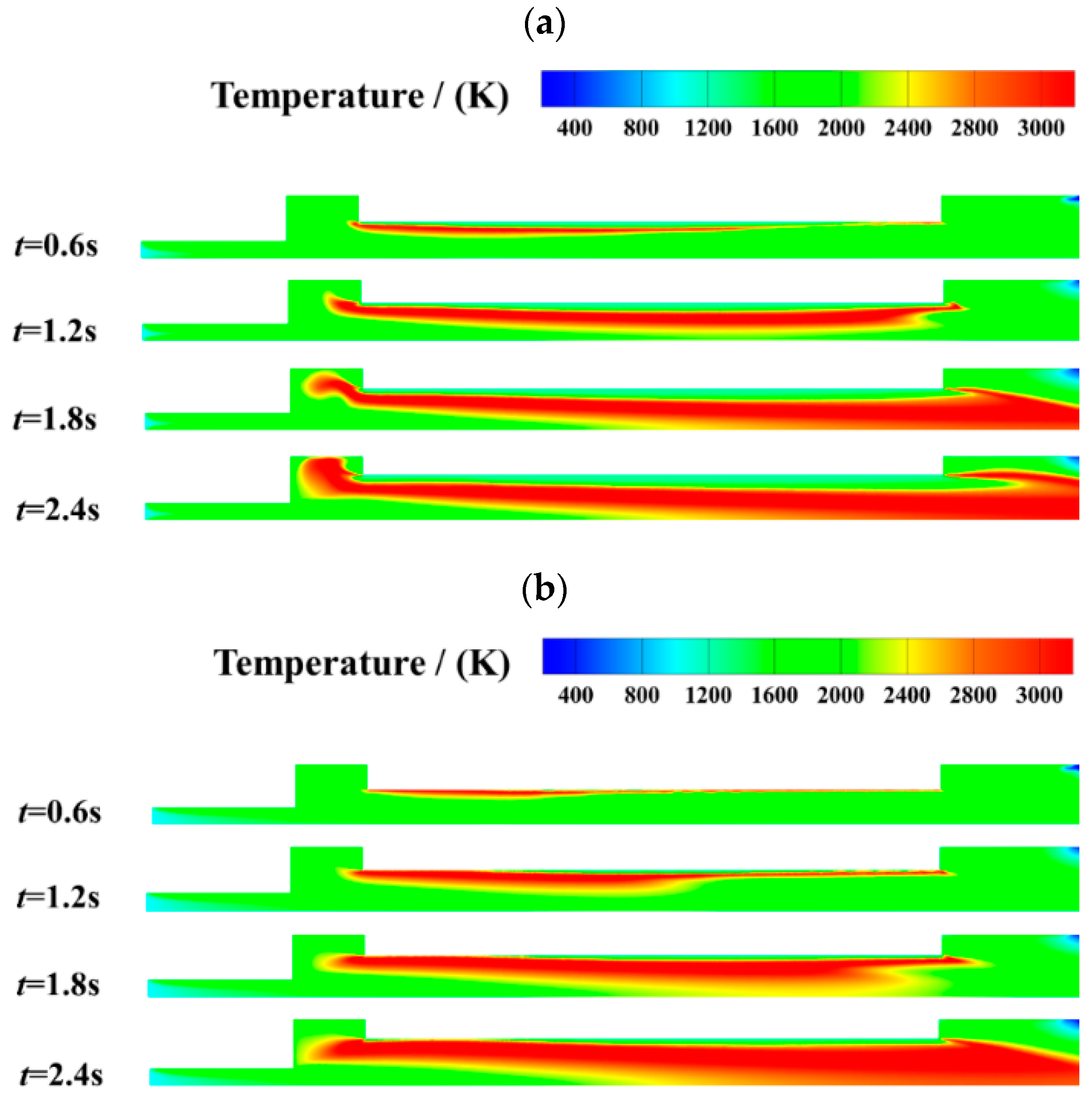
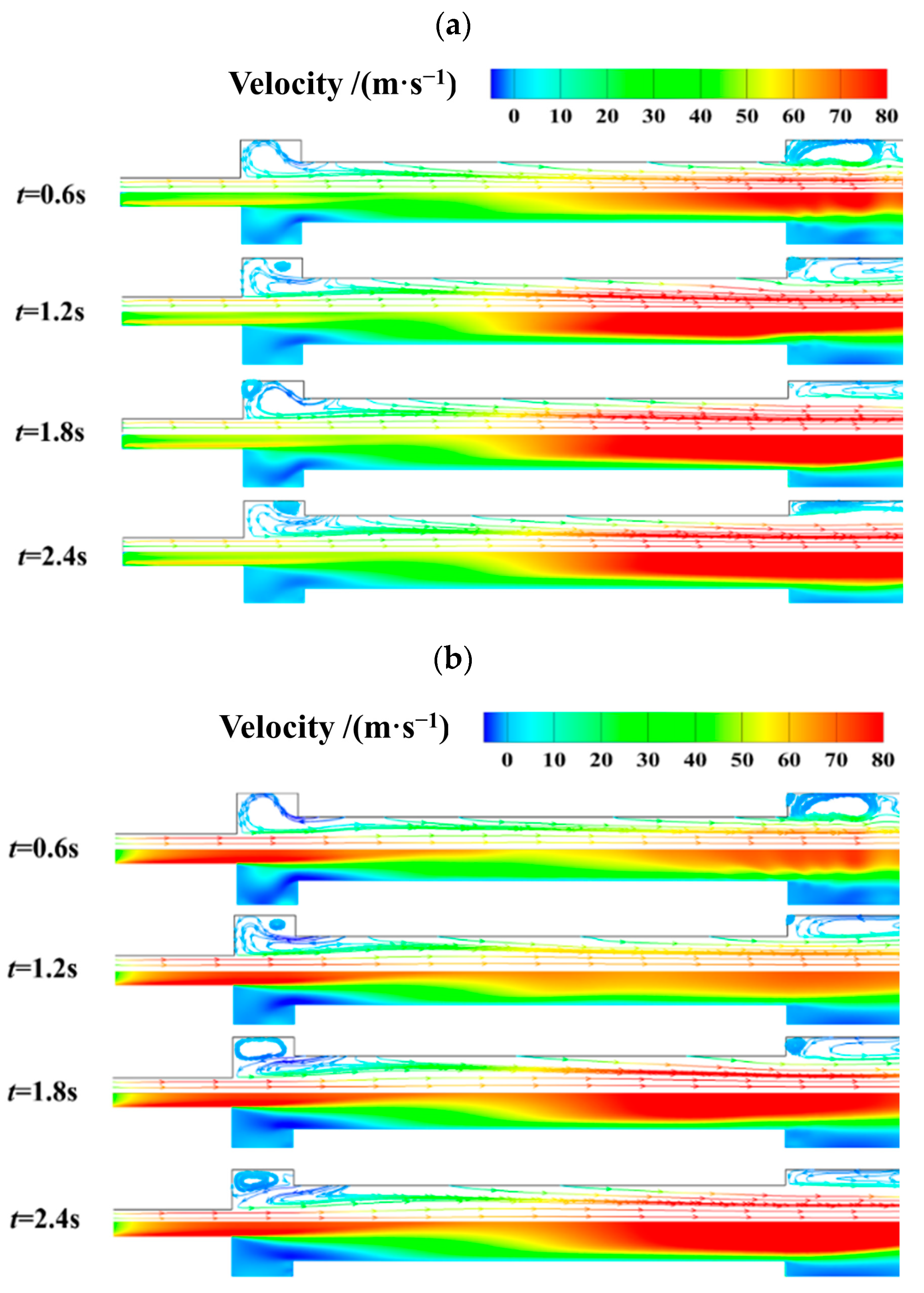
3.2. Flow and Heat Transfer Characteristics
3.3. Combustion and Heat Release Characteristics
4. Conclusions
- 1.
- Vortex structures are formed and evolved in the pre-post-combustion chambers. The formation and evolution of the vortex structures enhance the local mixing and combustion reactions through the combustion chamber. However, the non-uniformity of heat release rate distribution is also exacerbated by the re-developed vortex structures.
- 2.
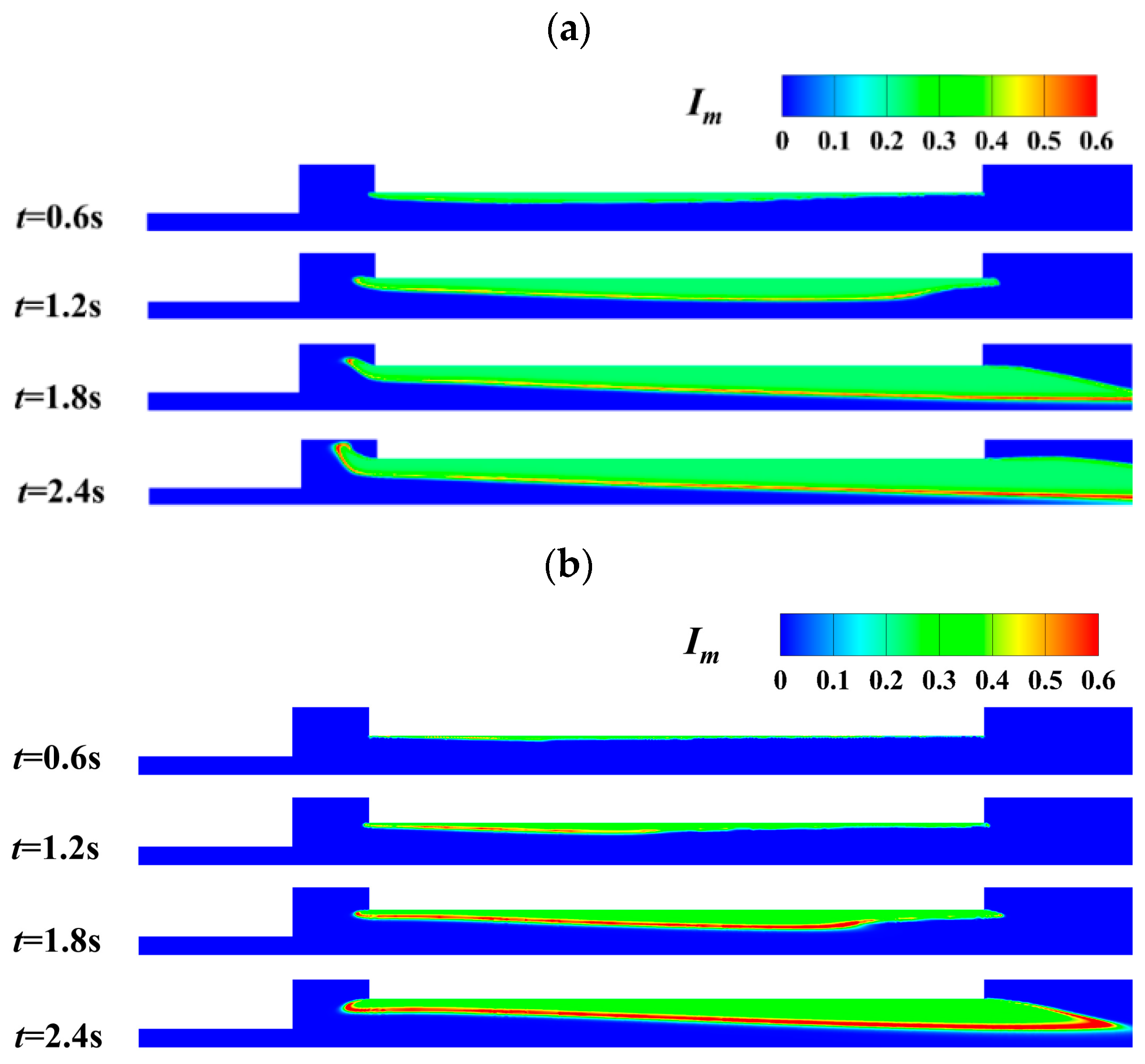
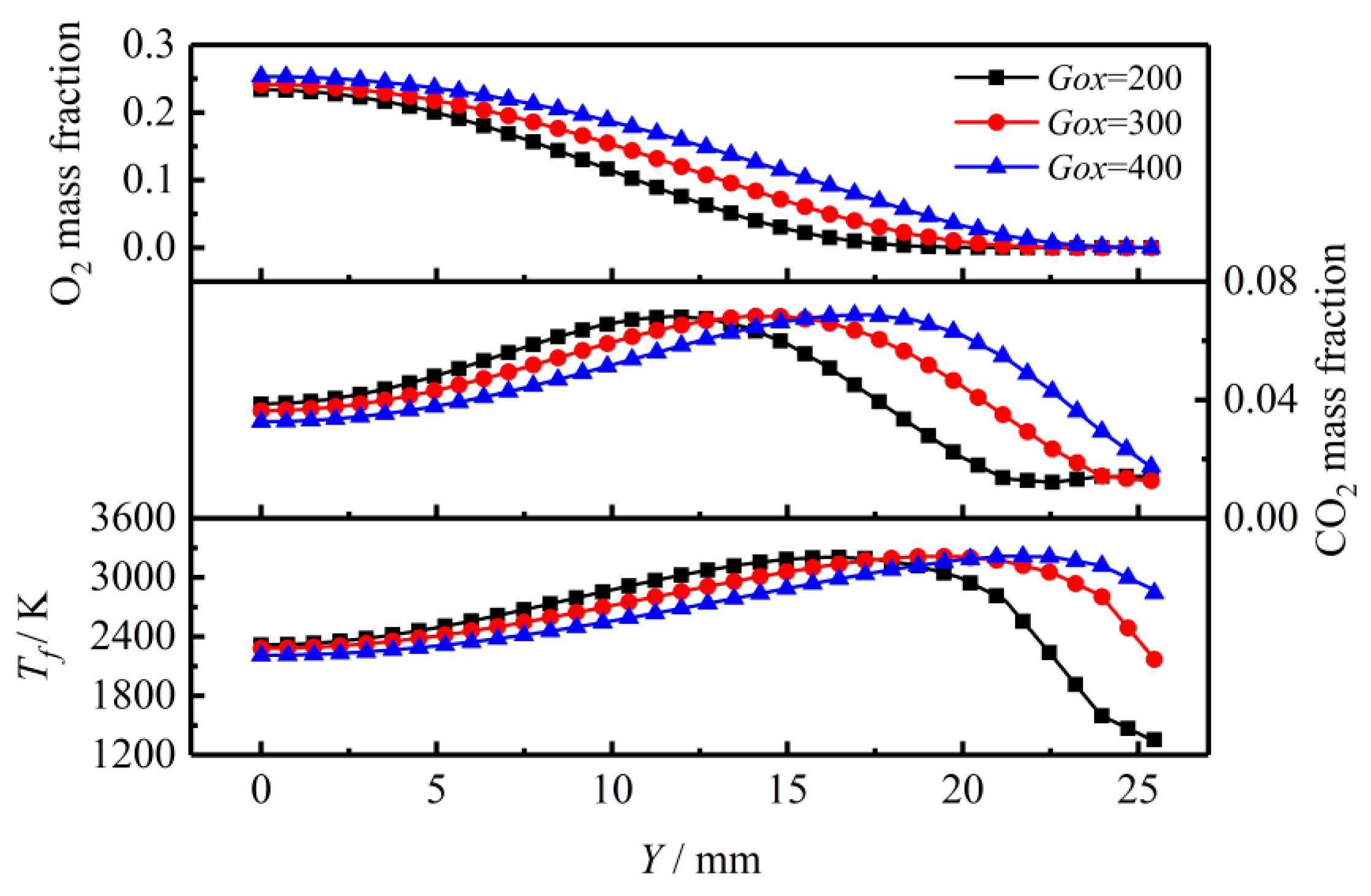
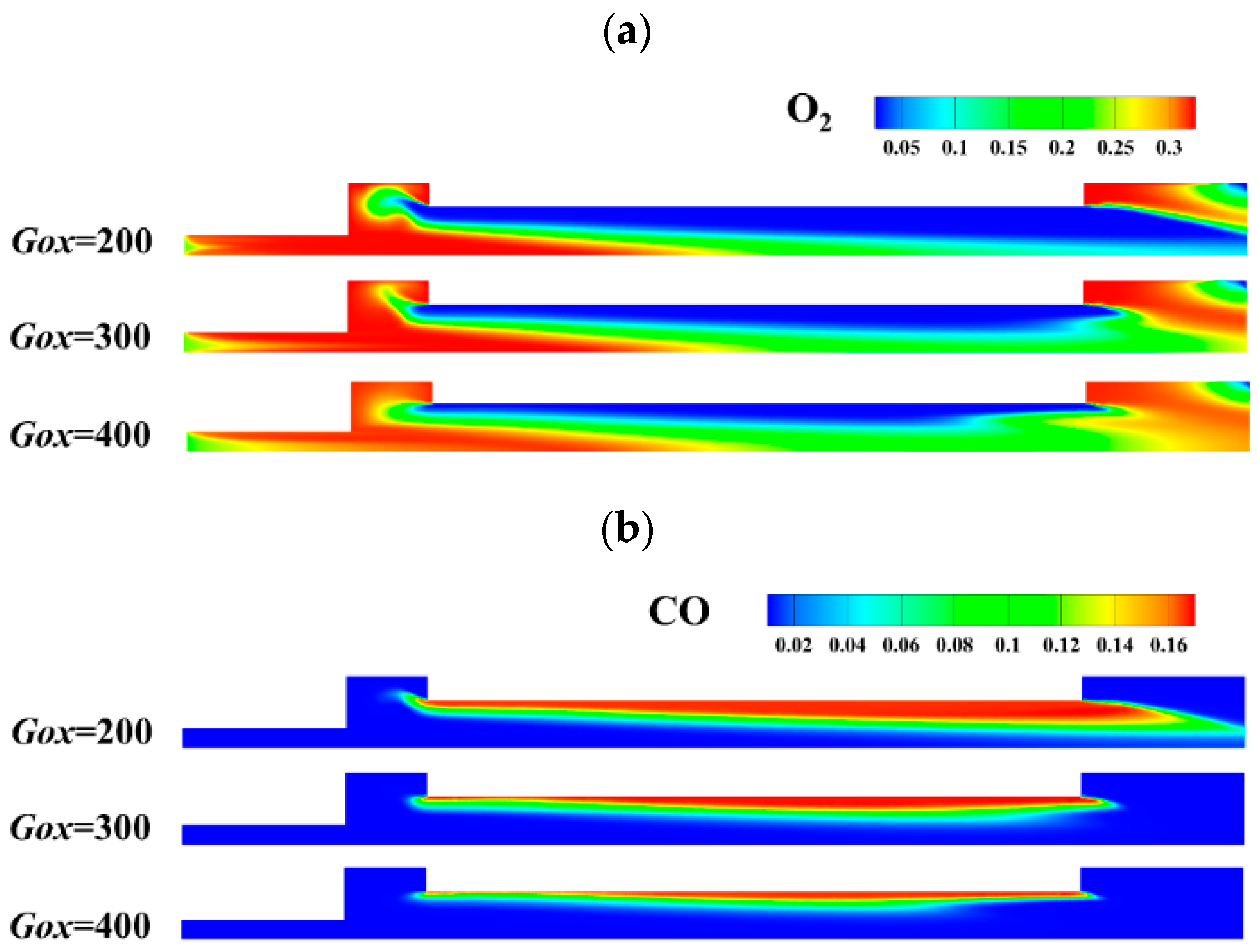
- Mixing is inhomogeneous in the coupled combustion process. There exists a narrow belt-like region with an excellent mixing effect. In the flow direction, the mixing degree increases as the mixing process is improved. With the increasing mass flux of the oxidant, the mixing in the belt-like coupled combustion region is enhanced. Coupled combustion is thus significantly strengthened. As combustion proceeds, the belt-like zone expands but moves away from the propellant grain.
- 3.
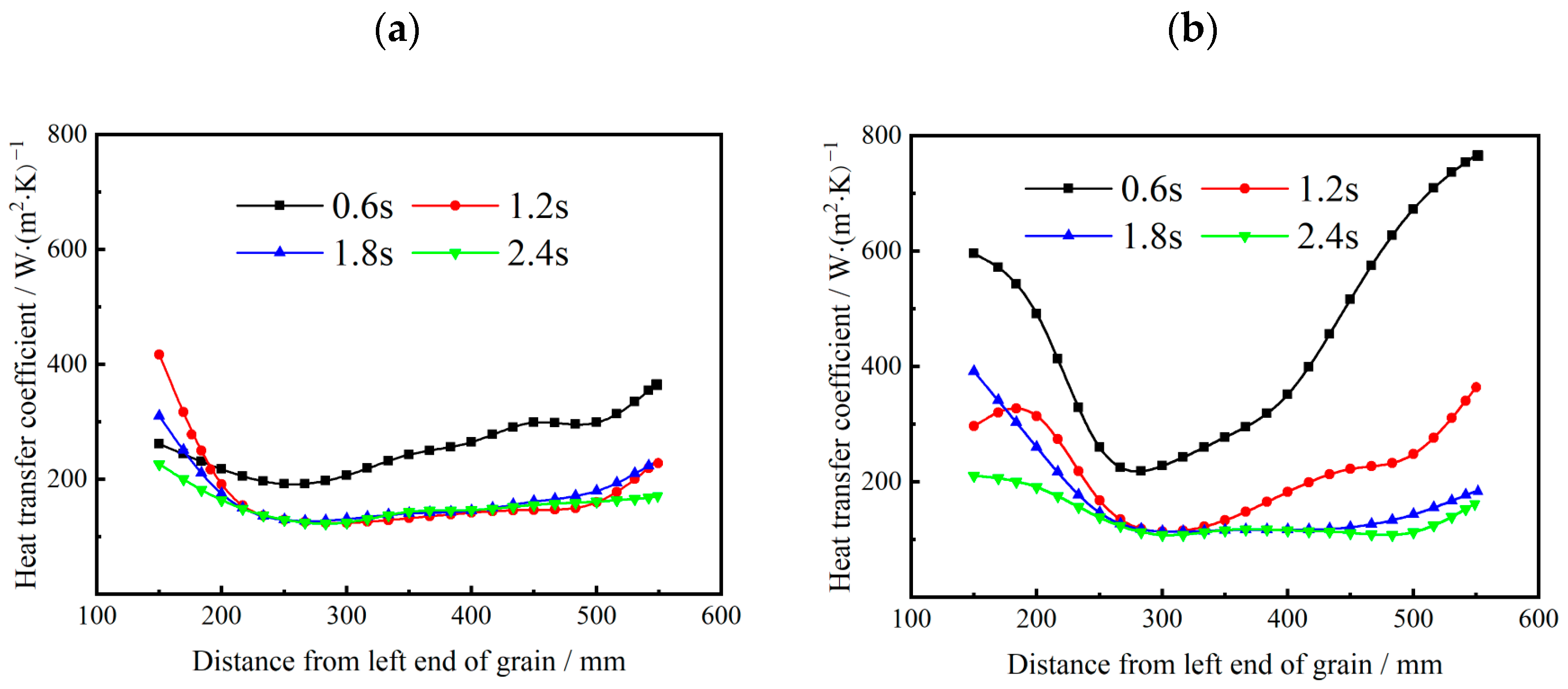
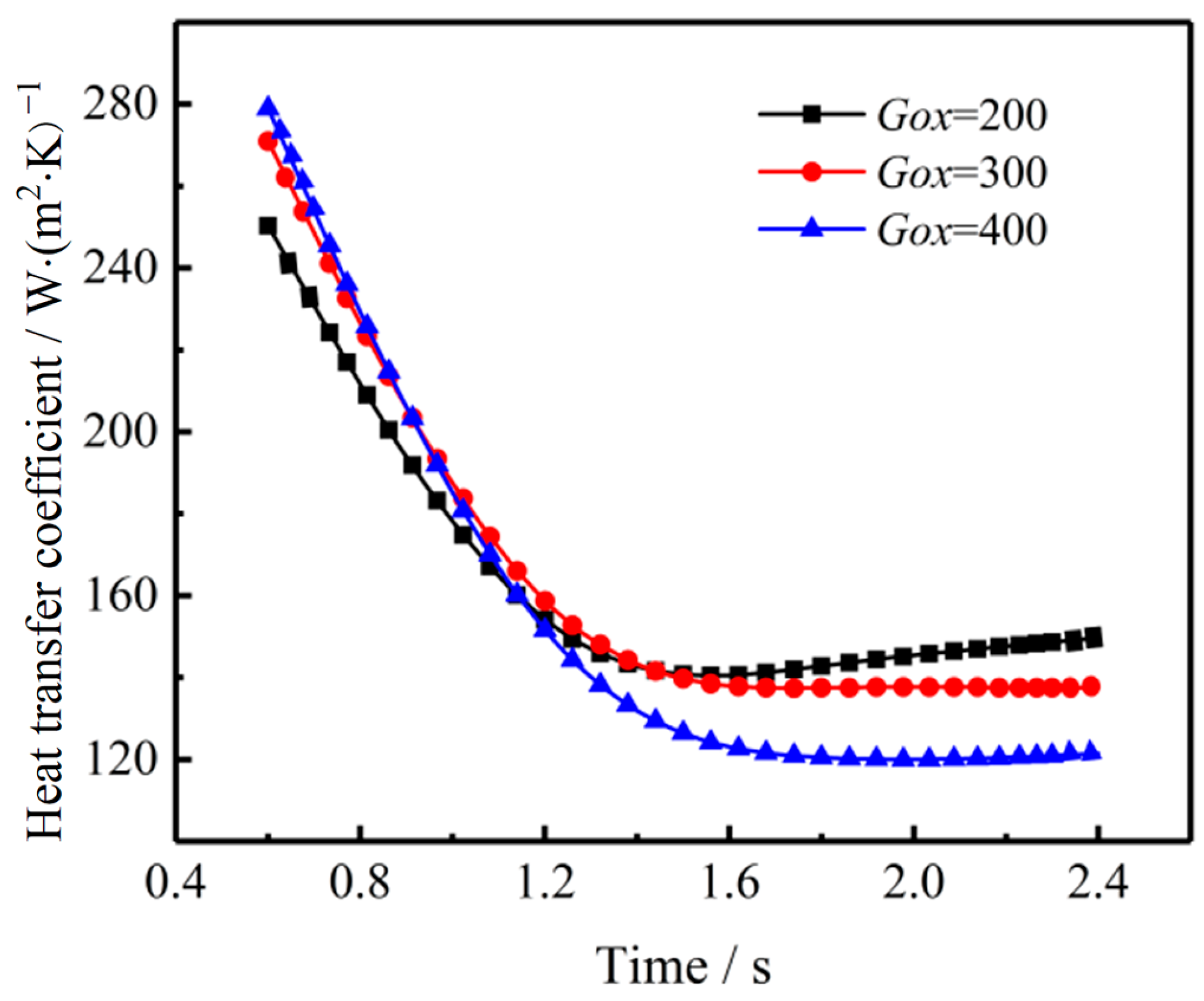
- Heat transfer coefficients at the two ends of the propellant are higher than those in the middle part of the propellant due to the vortex structures formed in the combustion chamber. The heat transfer coefficient is enhanced by increasing the mass flux of the oxidant in the initial combustion stage but is weakened in the late combustion stage. As the combustion progresses, the heat transfer coefficient decreases substantially due to decelerating reaction rate and enlarging flow channel area.
- 4.
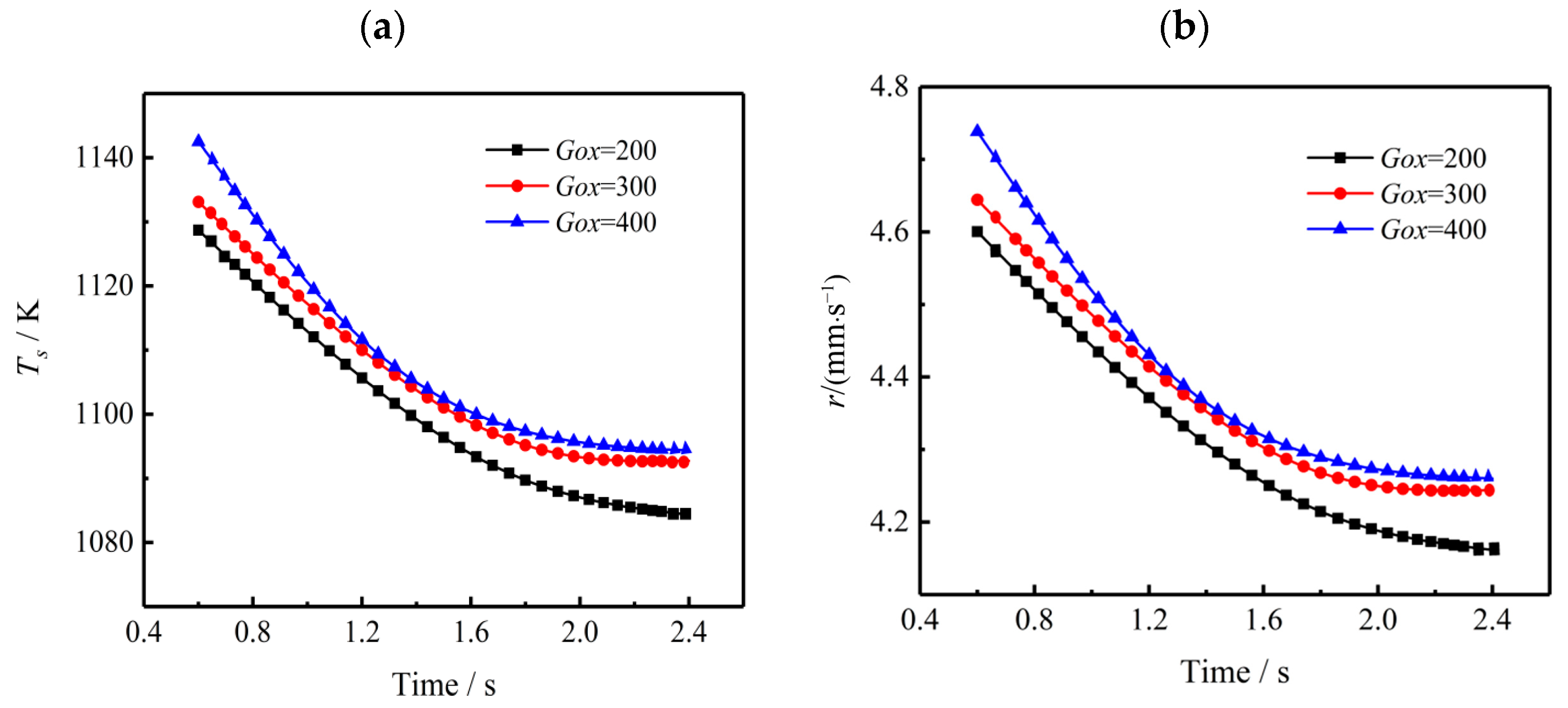
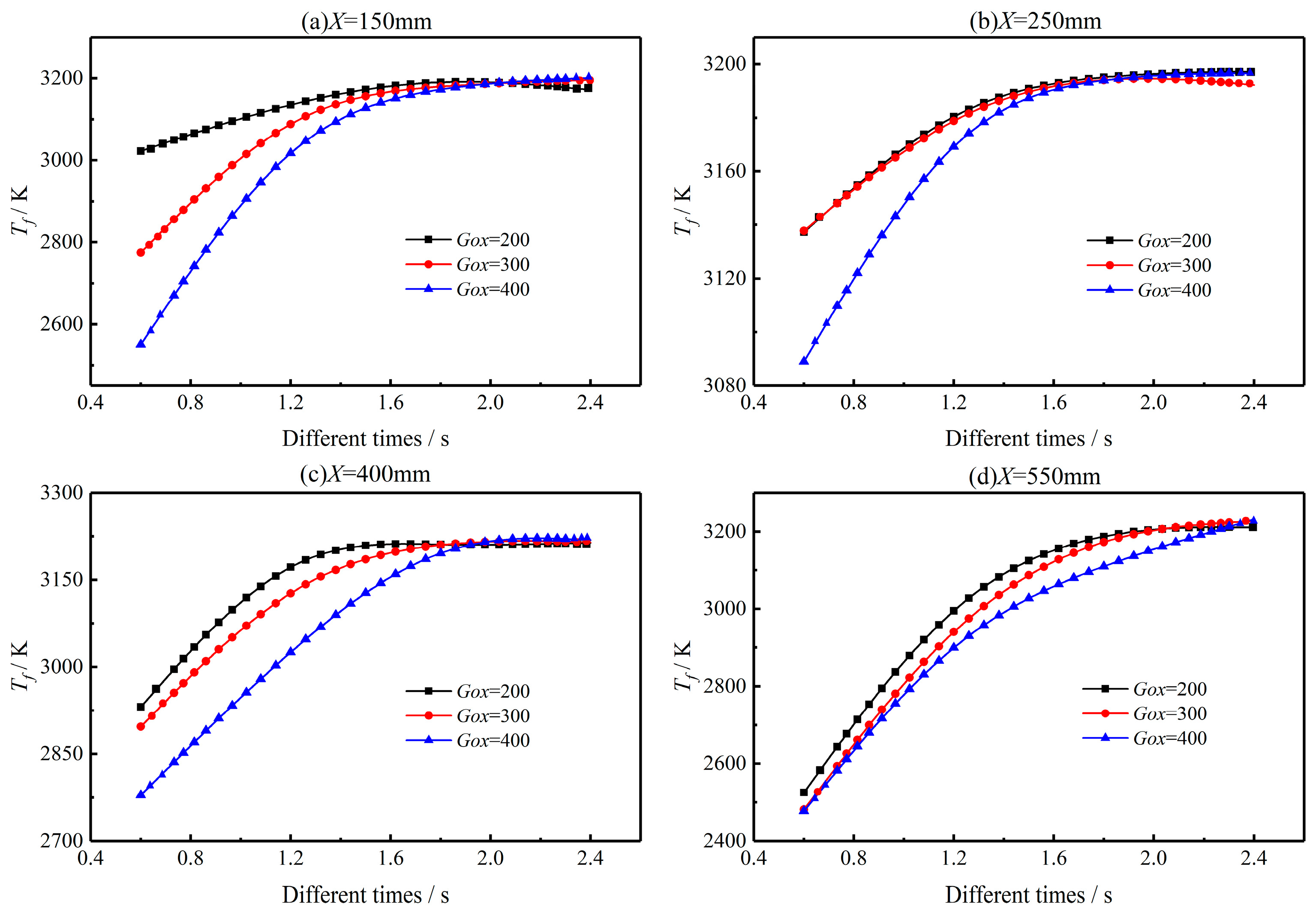
- As the combustion progresses, the temperature of the burning surface gradually decreases as the combustion reaction rate is decelerated. Increasing oxidizer mass flux leads to a slower decrease of temperature and regression rate with combustion time as mixing and combustion reaction are enhanced. Due to the entrainment effect of the vortex structure, temperature distribution along the flow direction is quite unevenly distributed.
- 5.
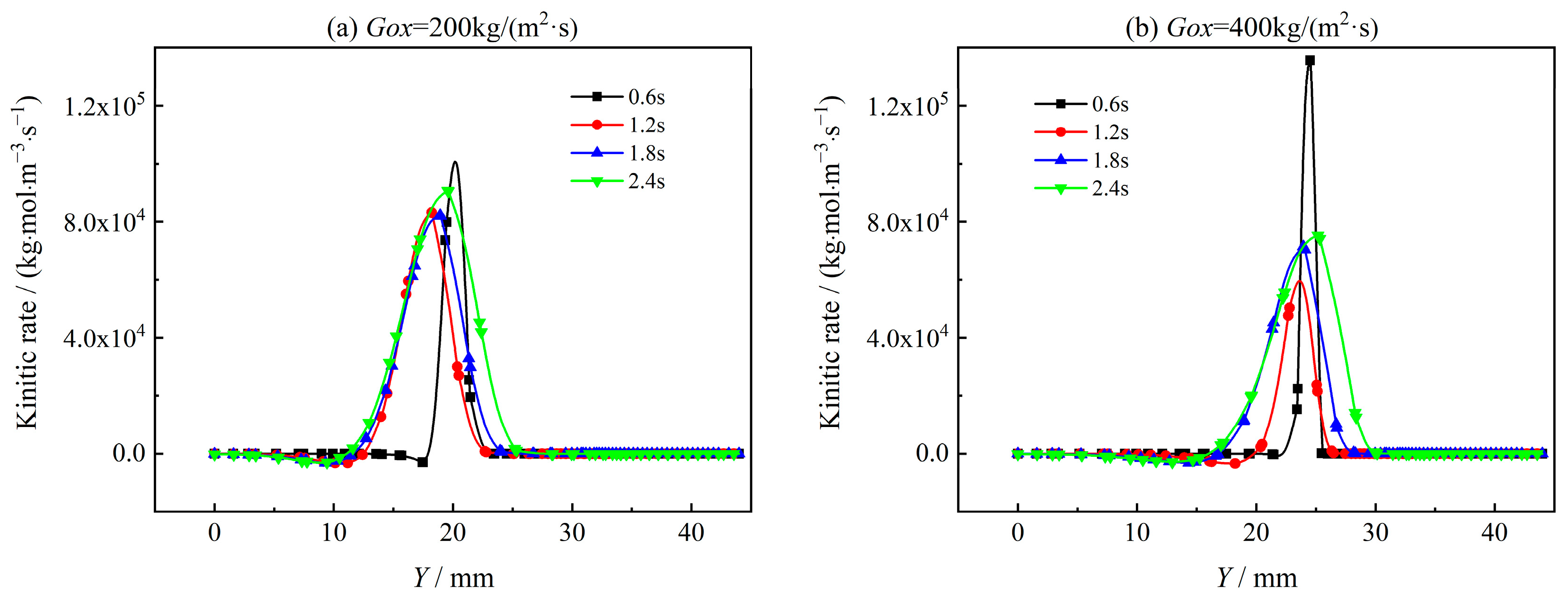
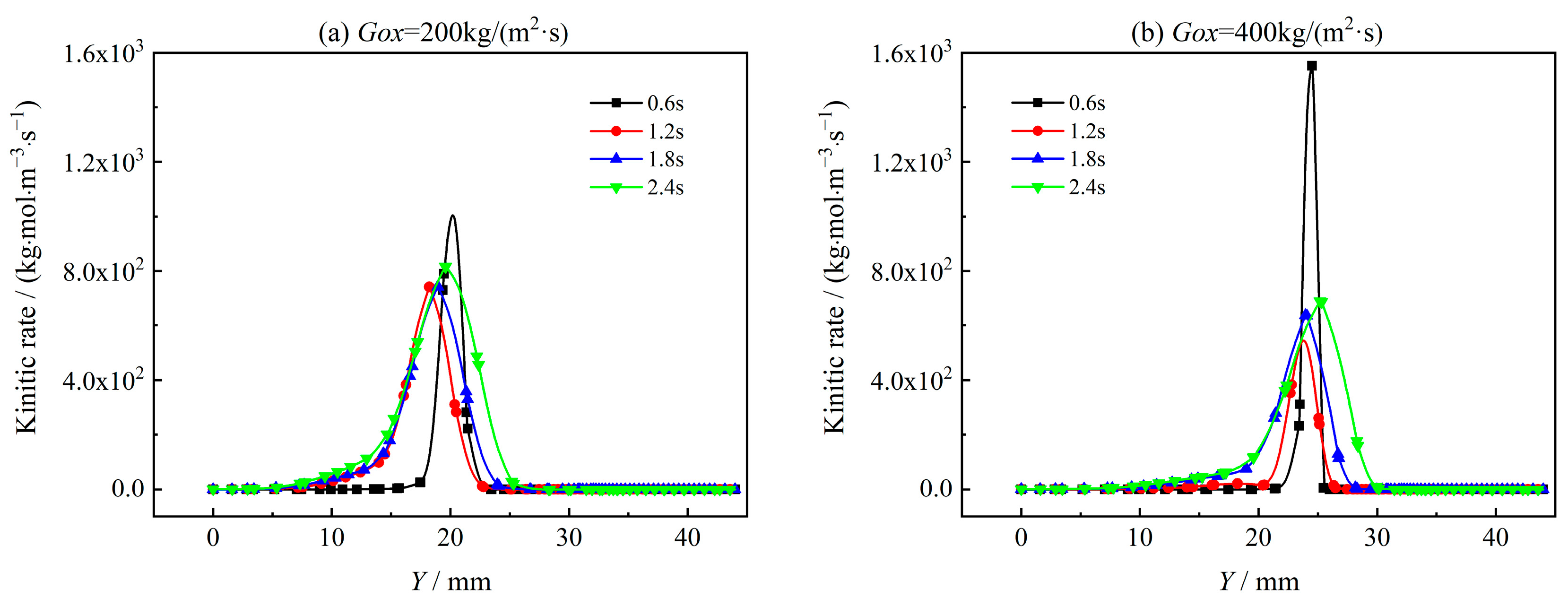
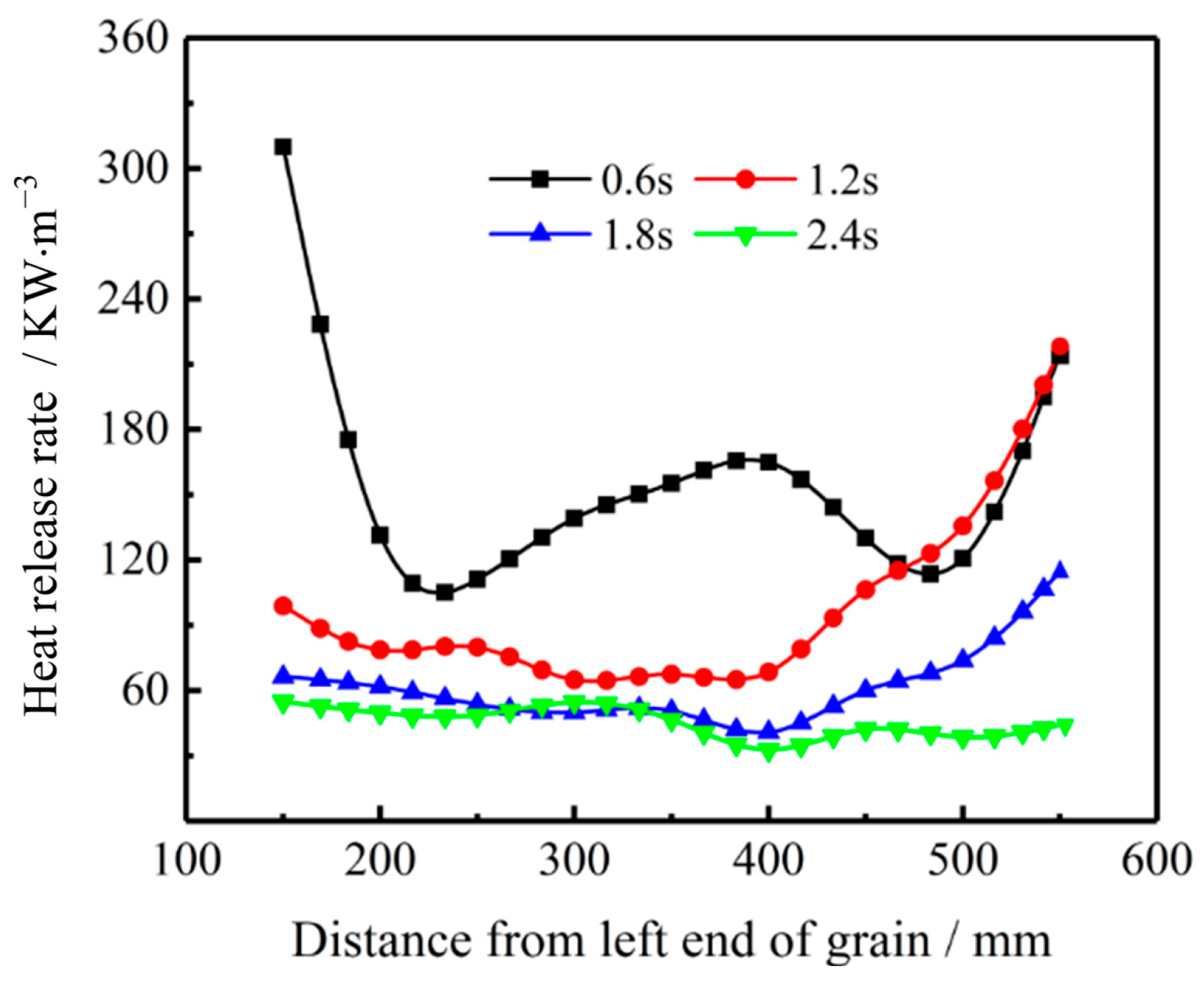
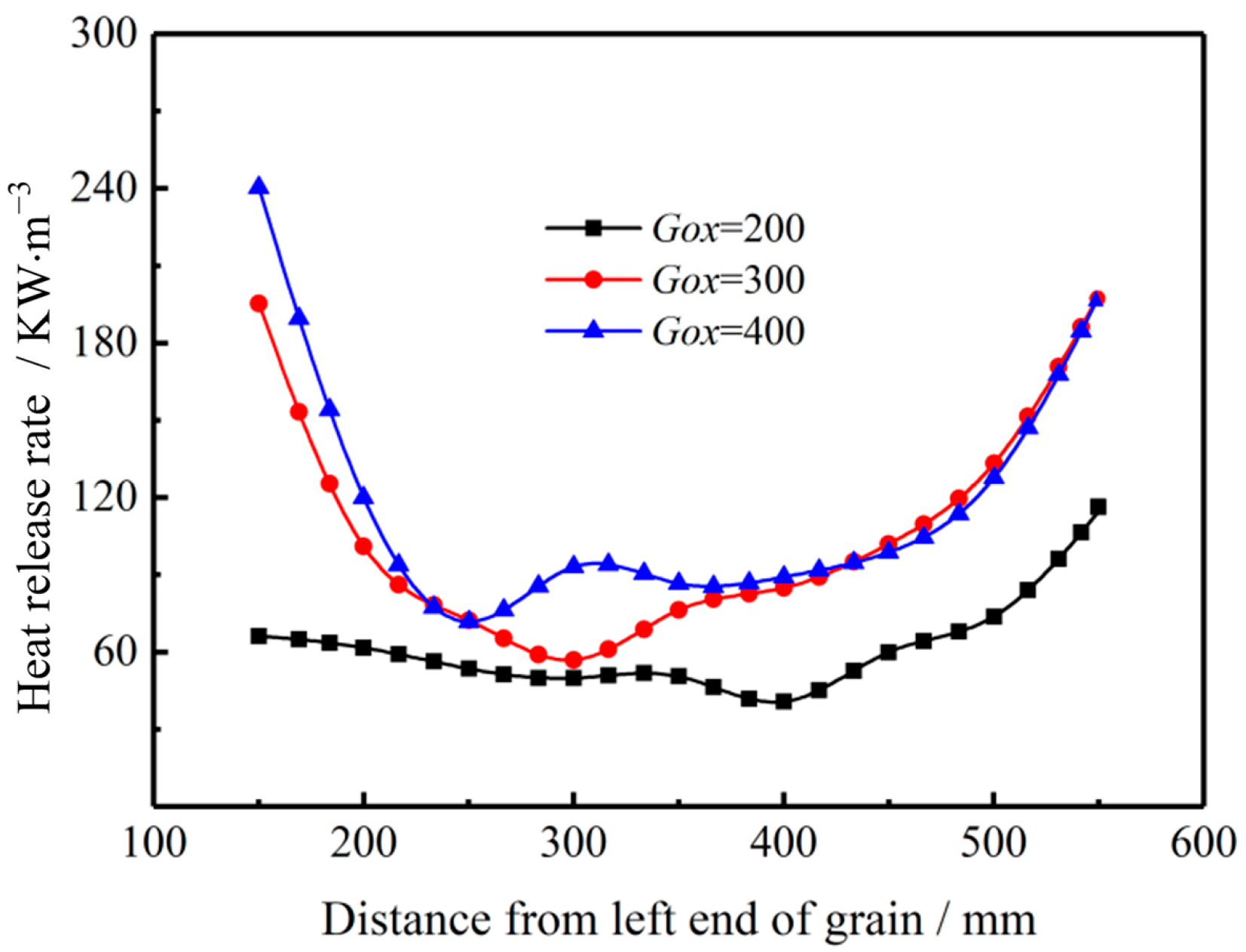
- Increasing inlet oxidizer mass flux leads to a higher combustion heat release rate as the coupled combustion reactions are accelerated by higher oxygen concentration and better mixing. Therefore, the maximum gas phase region temperature increases significantly. The heat release rate in the coupled combustion region decreases significantly as oxygen is consumed. The maximum combustion heat release rate appears near the ends of the propellant, where the existence of vortex structures enhances the mixing and combustion process.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tappan, B.C.; Dallmann, N.A.; Novak, A.M.; Lichthardt, J.P.; De, N.N.; Baca, E.V.; Seitz, D.N. High deltav solid propulsion system fort small satellites. In Proceedings of the 30th Annual AIAA/USU Conference on Small Satellites, Logan, UT, USA, 6–11 August 2016. [Google Scholar]
- Zou, X.R.; Wang, N.F.; Han, L.; Bai, T.T.; Xie, K. Numerical investigation on regression rate and thrust regulation behaviors of a combined solid rocket motor with aluminum-based fuel. Aerosp. Sci. Technol. 2021, 119, 107102. [Google Scholar] [CrossRef]
- Zhang, S. Numerical Simulation Study on Working Process and Combustion Microstructure of Solid-Liquid Hybrid Rocket Engine. Ph.D. Thesis, National University of Defense Technology, Changsha, China, 2017. [Google Scholar]
- Chiaverini, M.; Kuo, K.; Peretz, A. Heat flux and internal ballistic characterization of a hybrid rocket motor analog. In Proceedings of the 33th Joint Propulsion Conference and Exhibit, Seattle, WA, USA, 6–9 July 1997; p. 3080. [Google Scholar]
- Leccese, G.; Bianchi, D.; Nasuti, F. Numerical investigation on the role of thermal radiation in hybrid rocket fuel pyrolysis. In Proceedings of the 2018 Joint Propulsion Conference, Cincinnati, OH, USA, 9–11 July 2018; p. 4924. [Google Scholar]
- Stowe, R.; Champlain, A.D.; Mayer, A. Modelling and flow visualization of mixing in a ducted rocket combustor. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 13–15 July 1998; p. 3768. [Google Scholar]
- Jiang, Y.; Abu-Hamdeh, N.H.; Bantan, R.A.R. Mixing efficiency of hydrogen and air co-flow jets via wedge shock generator in dual-combustor ramjet. Aerosp. Sci. Technol. 2021, 116, 106846. [Google Scholar] [CrossRef]
- Zhang, Z.; Lin, X.; Wang, Z. Effects of swirl injection on the combustion of a novel composite hybrid rocket fuel grain. Acta Astronaut. 2022, 199, 174–182. [Google Scholar] [CrossRef]
- Vigot, C.; Bardelle, L.; Nadaud, L. Improvement of boron combustion in a solid-fuel ramrocket. In Proceedings of the 22th Joint Propulsion Conference, Huntsville, AL, USA, 16–18 June 1986; p. 1590. [Google Scholar]
- Evans, J.V.; Senior, W.C.B.; Gejji, R.M. Performance of a solid-fuel ramjet combustor with bypass air addition. J. Propuls. Power 2022, 39, 167–175. [Google Scholar] [CrossRef]
- Wang, S.; Liu, Y.; Wu, Y. Numerical simulation of mixing and combustion characteristics based on air turbine rocket engine rocket afterburner. Highlights Sci. Eng. Technol. 2022, 1, 163–170. [Google Scholar] [CrossRef]
- Cherng, D.L.; Yang, V.; Kuo, K. Numerical study of turbulent reacting flows in solid-propellant ducted rocket combustors. J. Propuls. Power 1989, 5, 678–685. [Google Scholar] [CrossRef]
- Li, W.; Zhao, D.; Chen, X. Numerical investigation of inlet thermodynamic conditions on solid fuel ramjet performances. Int. J. Aerosp. Eng. 2021, 2021, 8868288. [Google Scholar] [CrossRef]
- Hu, Y.P.; Liu, Y.S.; Wang, Y.J.; Zhou, B.Q.; Zheng, X.; Xue, R. Effect of the heat release on ejector-to-ramjet dynamic mode transition process in the rocket-based combined cycle engine. Int. Commun. Heat Mass Transf. 2023, 140, 106539. [Google Scholar] [CrossRef]
- Jia, E.Q.; Peng, Q.; Liu, X.; Zuo, W.; Zhao, X.; Liu, H. Numerical investigation on hydrogen/air non-premixed combustion in a three-dimensional micro combustor. Energy Convers. Manag. 2016, 124, 427–438. [Google Scholar]
- Jia, E.Q.; Peng, Q.; Zhao, X.; Zuo, W.; Zhang, Z.; Pham, M. Numerical investigation on the combustion characteristics of non-premixed hydrogen-air in a novel micro-combustor. Appl. Therm. Eng. 2017, 110, 665–677. [Google Scholar]
- Jia, E.Q.; Ding, J.; Chen, J.; Liao, G.; Zhang, F.; Luo, B. Process in micro-combustion and energy conversion of micro power system: A review. Energy Convers. Manag. 2021, 246, 114664. [Google Scholar]
- Chen, Q.; Zheng, Z.; Zhu, Z. Effects of syngas addition on combustion characteristics of gasoline surrogate fuel. ACS Omega 2023, 8, 3929–3944. [Google Scholar] [CrossRef] [PubMed]
- Wei, Z.L.; Leung, C.W.; Cheung, C.S.; Huang, Z.H. Effects of equivalence ratio, H2 and CO2 addition on the heat release characteristics of premixed laminar biogas-hydrogen flame. Int. J. Hydrogen Energy 2016, 41, 6567–6580. [Google Scholar] [CrossRef]
- Song, M.T.; Wang, C.Y.; Chang, C.C. Two-stream interaction problem and its application to mass transport. Int. J. Heat Mass Transf. 2022, 196, 123312. [Google Scholar] [CrossRef]
- Musa, O.; Xiong, C.; Zhou, C.S.; Li, W. Effect of inlet conditions on swirling turbulent reacting flows in a solid fuel ramjet engine. Appl. Therm. Eng. 2017, 113, 186–207. [Google Scholar] [CrossRef]
- Cai, G.; Zhao, Z.; Zhao, B.; Liu, Y.; Yu, N. Regression rate and combustion performance investigation on hybrid rocket motor with head-end swirl injection under high geometric swirl number. Aerosp. Sci. Technol. 2020, 103, 105922. [Google Scholar] [CrossRef]
- Tian, H.; He, L.; Zhu, H.; Wang, P.; Xu, X. Numerical and experimental investigation on hybrid rocket motor with two-hole segmented rotation grain. Aerosp. Sci. Technol. 2019, 92, 820–830. [Google Scholar] [CrossRef]
- Betelin, V.B.; Kushnirenko, A.G.; Smirnov, N.N.; Nikitin, V.F.; Tyurenkova, V.V.; Stamov, L.I. Numerical investigations of hybrid rocket engines. Acta Astronaut. 2018, 144, 363–370. [Google Scholar] [CrossRef]
- Wang, H.; Wang, Z.; Sun, M.; Qin, N. Large eddy simulation of a hydrogen-fueled scramjet combustor with dual cavity. Acta Astronaut. 2015, 108, 119–128. [Google Scholar] [CrossRef]
- Liu, S.Y.; Chen, Z.C.; Wang, L.M.; Li, Y.; Hu, S.Q. Numerical study on transient regression rate and combustion characteristics of segregated AP-based oxidizer/TAGN-based fuel. Fuel 2022, 337, 126893. [Google Scholar] [CrossRef]
- Liu, S.Y.; Zhang, Y.; Wang, L.M.; Han, L.Y.; Chen, Z.C.; Hu, S.Q. The effect of mixing and heat transfer on regression rate of TAGN-based fuel in a segregated AP/TAGN solid motor. Int. J. Therm. Sci. 2023, 186, 108133. [Google Scholar] [CrossRef]
- Shih, T.M. Numerical Heat Transfer; CRC Press: Boca Raton, FL, USA, 1984. [Google Scholar]
- Kuo, K.K.; Chiaverini, M.J. Fundamentals of Hybrid Rocket Combustion and Propulsion; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2007. [Google Scholar]
- Li, X.T.; Tian, H.; Cai, G.B. Numerical analysis of fuel regression rate distribution characteristics in hybrid rocket motors with different fuel types. Sci. China Technol. Sci. 2013, 56, 1807–1817. [Google Scholar] [CrossRef]
- Wilcox, D.C. Reassessment of the scale-determining equation for advanced turbulence models. AIAA J. 1988, 26, 1299–1310. [Google Scholar] [CrossRef]
- Wilcox, D.C. A half century historical review of the k-omega model. In Proceedings of the 29th Aerospace Sciences Meeting, Reno, NV, USA, 7–10 January 1991; p. 615. [Google Scholar]
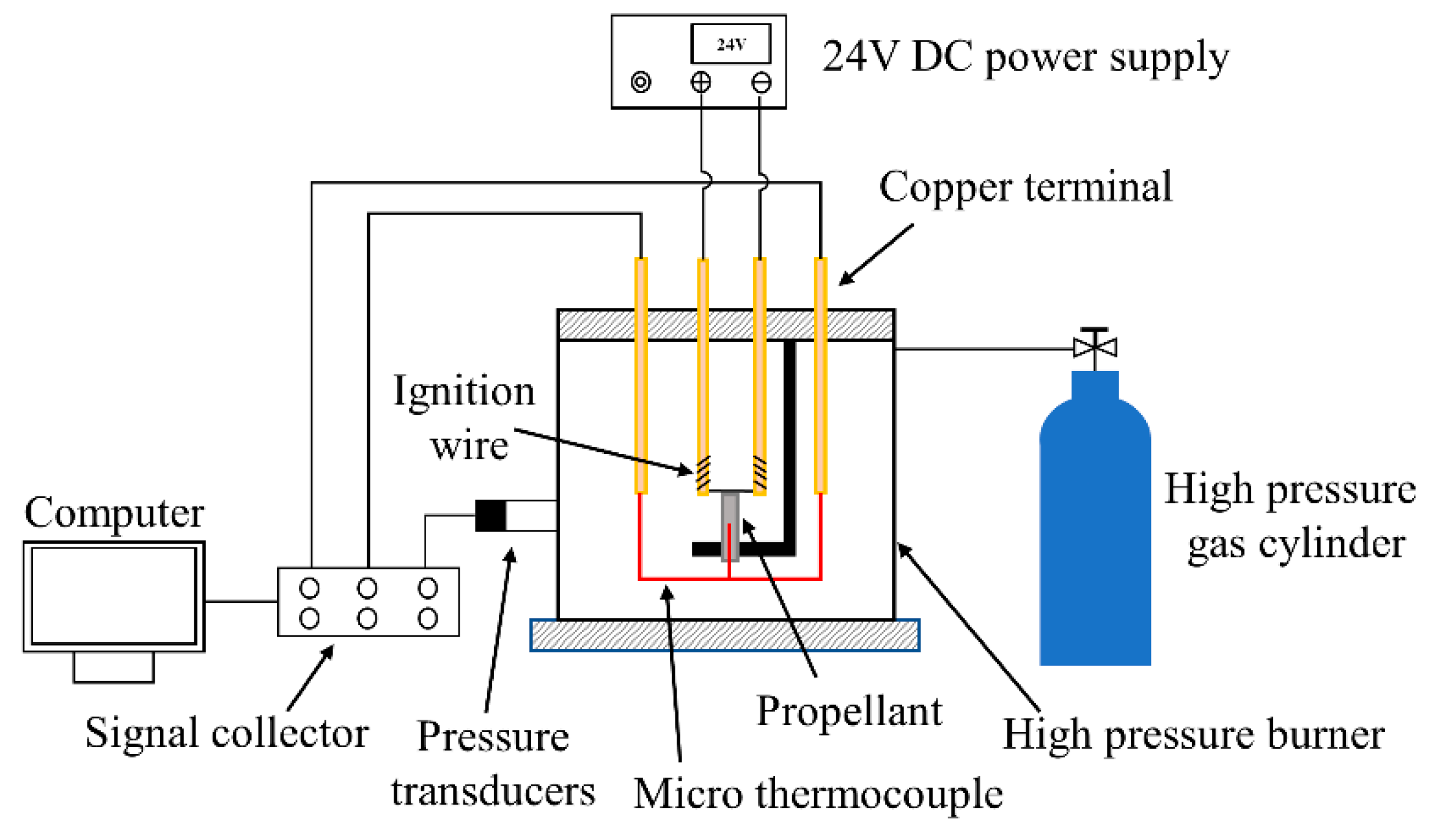
- Carro, R.; Stephens, M.; Arvanetes, J.; Powell, A.; Petersen, E.; Smith, C. High-pressure testing of composite solid propellant mixtures: Burner facility characterization. In Proceedings of the 41st AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Tucson, AZ, USA, 10–13 July 2005; p. 3617. [Google Scholar]





| Components | Mole Fraction | Components | Mole Fraction |
|---|---|---|---|
| H2O | 0.2945 | CO2 | 0.0063 |
| O2 | 0.11 | CO | 0.049 |
| HCl | 0.045 | H2 | 0.145 |
| NH3 | 0.04 | N2 | 0.1945 |
| NO2 | 0.03 | Cl2 | 0.01 |
| N2O | 0.015 | ClO2 | 0.01 |
| N2 | 0.2095 | HClO4 | 0.01 |
| ClO | 0.015 | NO | 0.005 |
| Inlet Flow Rate/(g·s−1) | Pressure/MPa | Regression Rate/(mm·s−1) | |
|---|---|---|---|
| Experiment | Simulation | ||
| 8.00 | 1.65 | 1.80 | 1.74 |
| 8.90 | 1.65 | 1.91 | 1.96 |
| 9.70 | 1.65 | 2.16 | 2.18 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Zhang, Y.; Wang, L.; Chen, Z.; Hu, S. Evaluation of Mixing Effect on Coupled Heat Release and Transfer Performance of a Novel Segregated Solid Rocket Motor. Aerospace 2024, 11, 72. https://doi.org/10.3390/aerospace11010072
Liu S, Zhang Y, Wang L, Chen Z, Hu S. Evaluation of Mixing Effect on Coupled Heat Release and Transfer Performance of a Novel Segregated Solid Rocket Motor. Aerospace. 2024; 11(1):72. https://doi.org/10.3390/aerospace11010072
Chicago/Turabian StyleLiu, Shuyuan, Yu Zhang, Limin Wang, Zhengchun Chen, and Songqi Hu. 2024. "Evaluation of Mixing Effect on Coupled Heat Release and Transfer Performance of a Novel Segregated Solid Rocket Motor" Aerospace 11, no. 1: 72. https://doi.org/10.3390/aerospace11010072
APA StyleLiu, S., Zhang, Y., Wang, L., Chen, Z., & Hu, S. (2024). Evaluation of Mixing Effect on Coupled Heat Release and Transfer Performance of a Novel Segregated Solid Rocket Motor. Aerospace, 11(1), 72. https://doi.org/10.3390/aerospace11010072





