Control of Cowl Shock/Boundary Layer Interaction in Supersonic Inlet Based on Dynamic Vortex Generator
Abstract
:1. Introduction
2. Concept of Dynamic MVG Control
3. Methodology
3.1. Description of the Model
3.2. Numerical Approach
3.2.1. Numerical Method
3.2.2. Numerical Validation
3.2.3. Quasi-Steady Verification
4. Results and Discussion
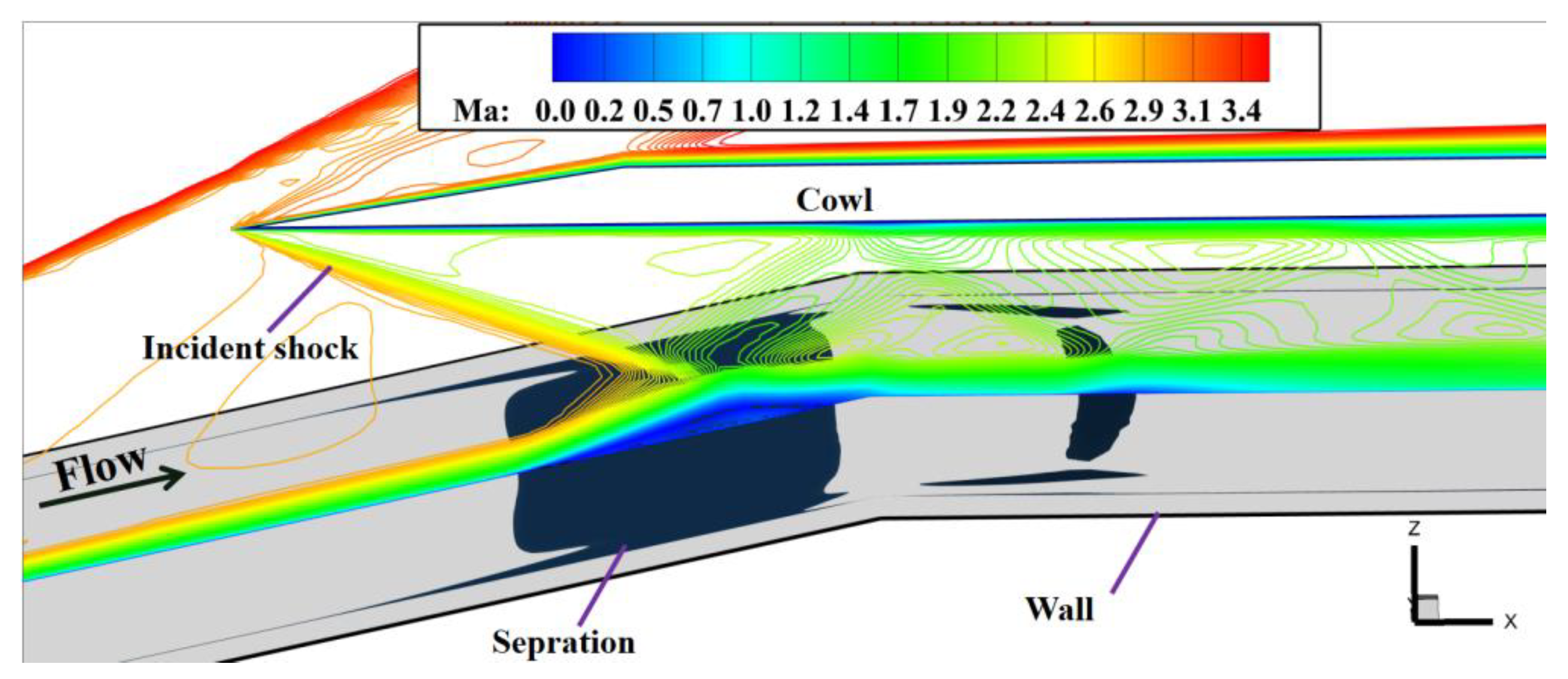
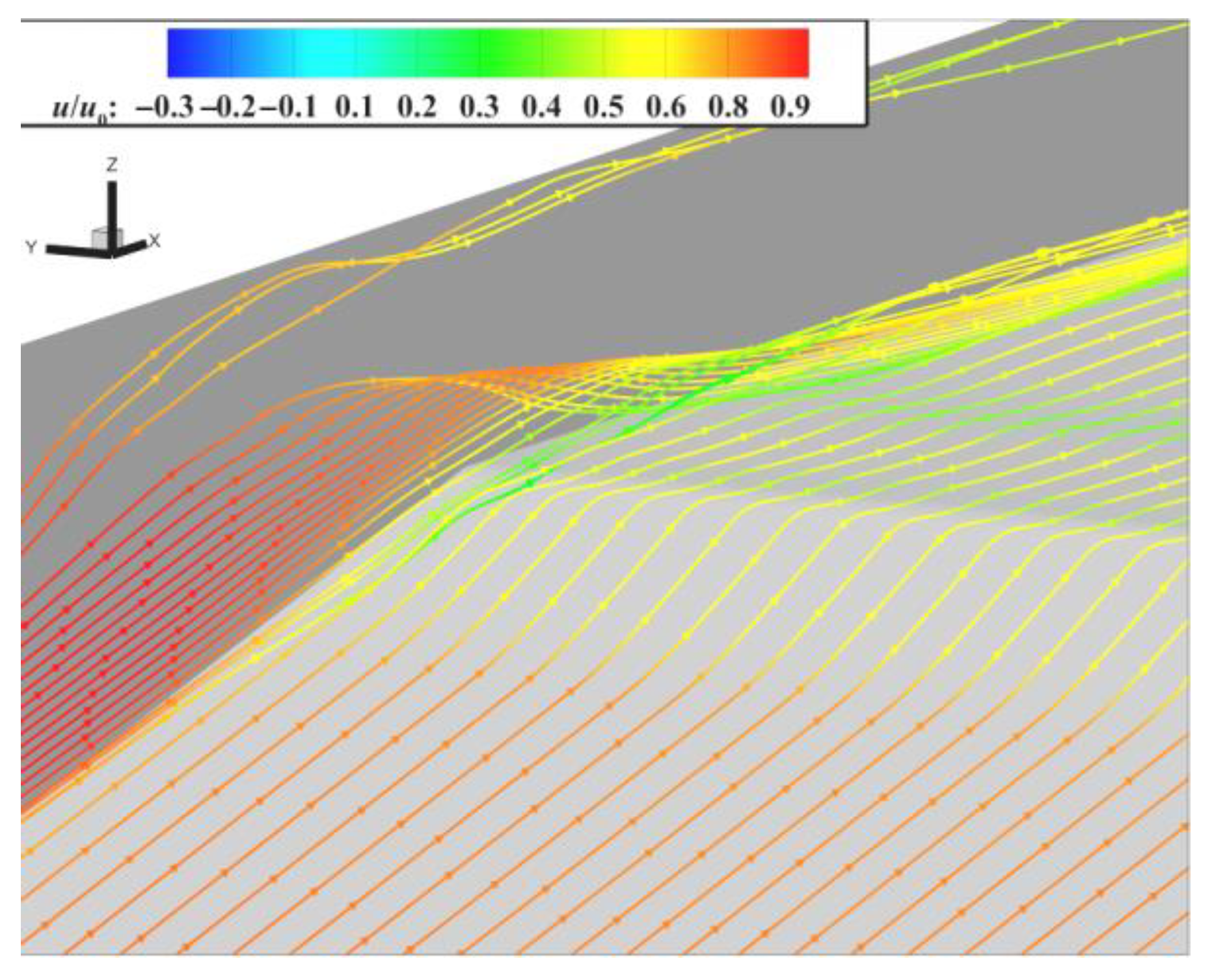
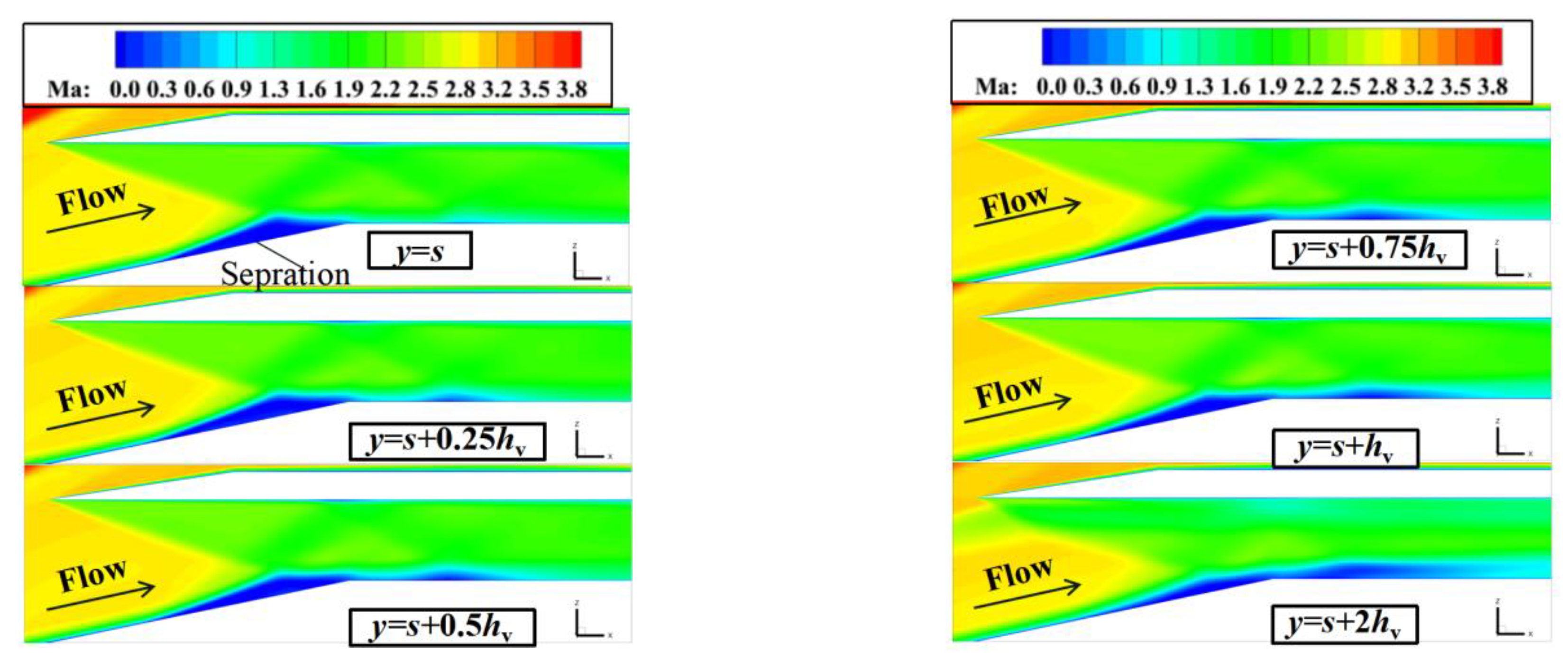
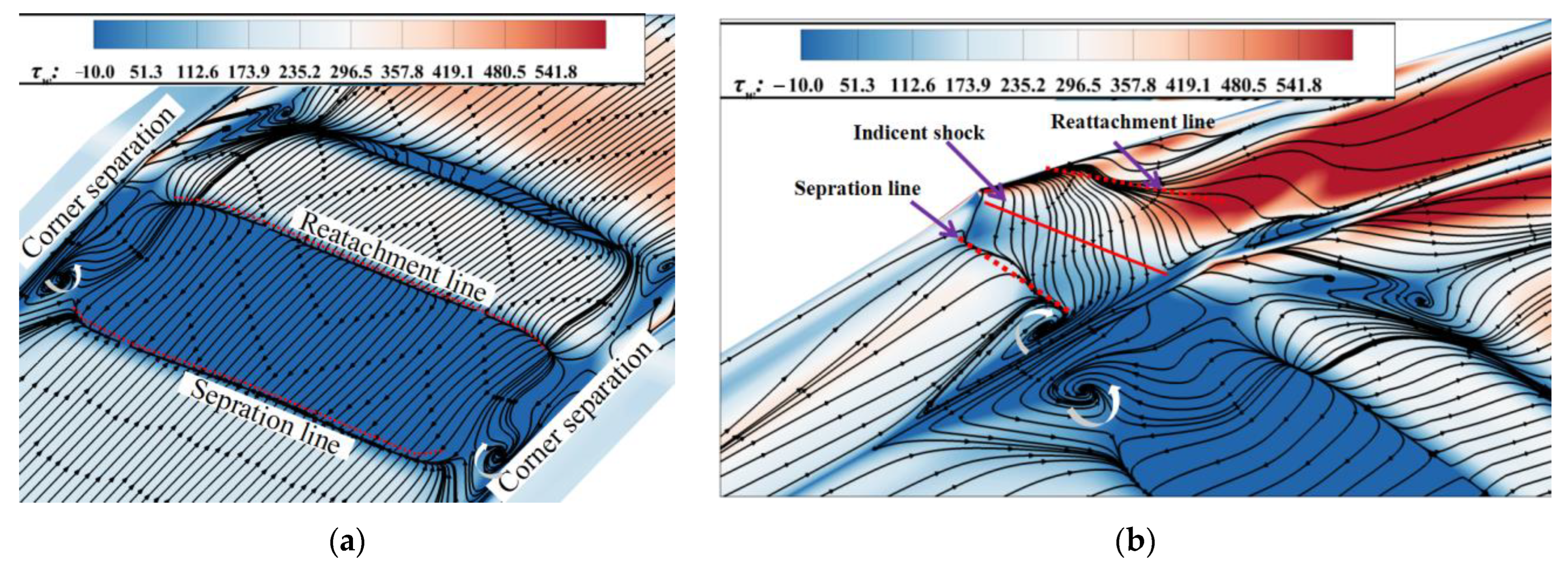
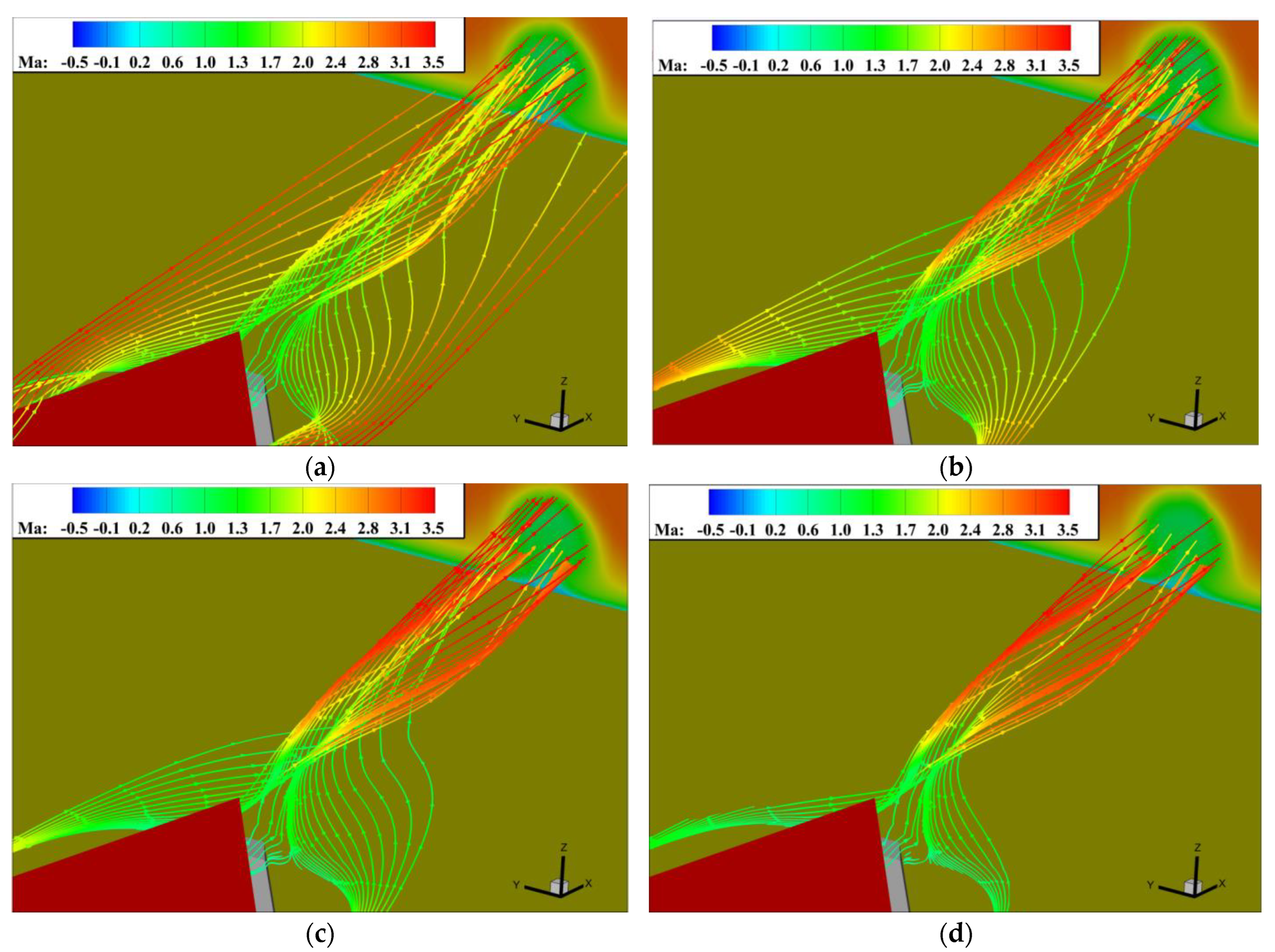
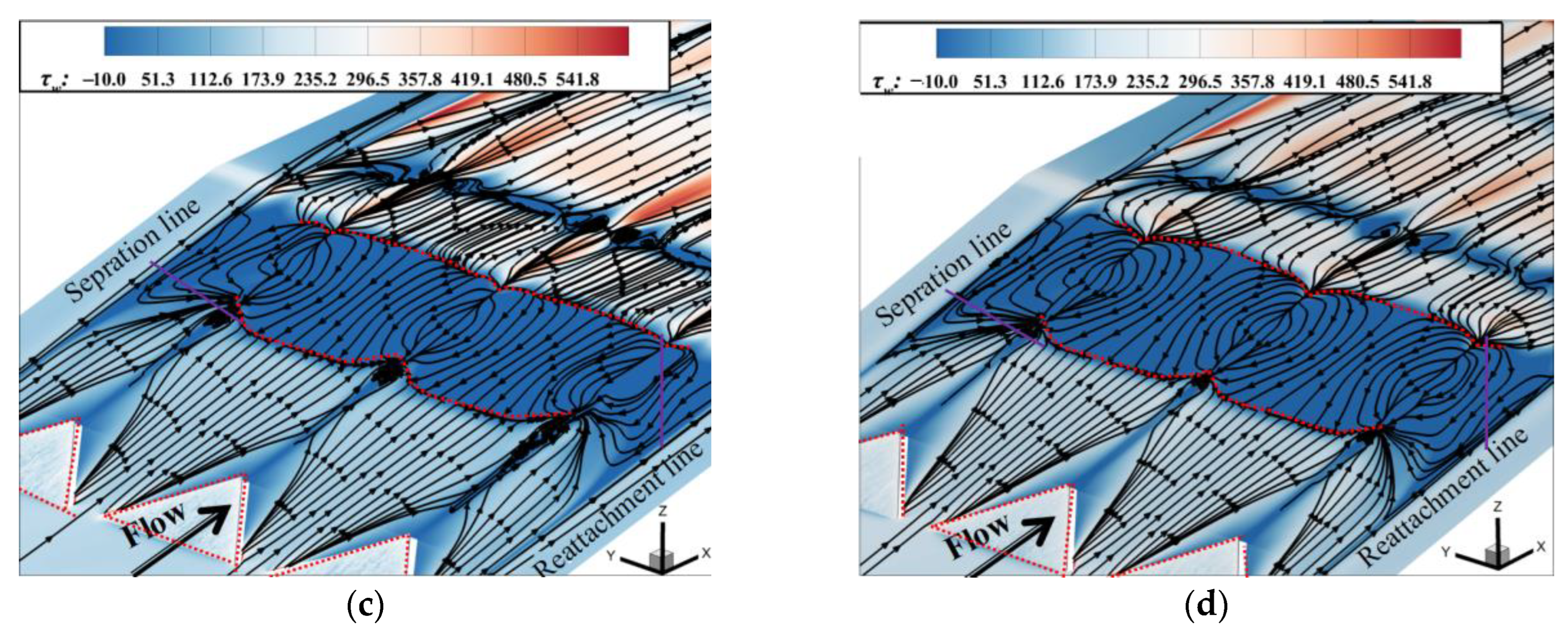
4.1. Flow Structure of SWBLI in Supersonic Inlet without Control
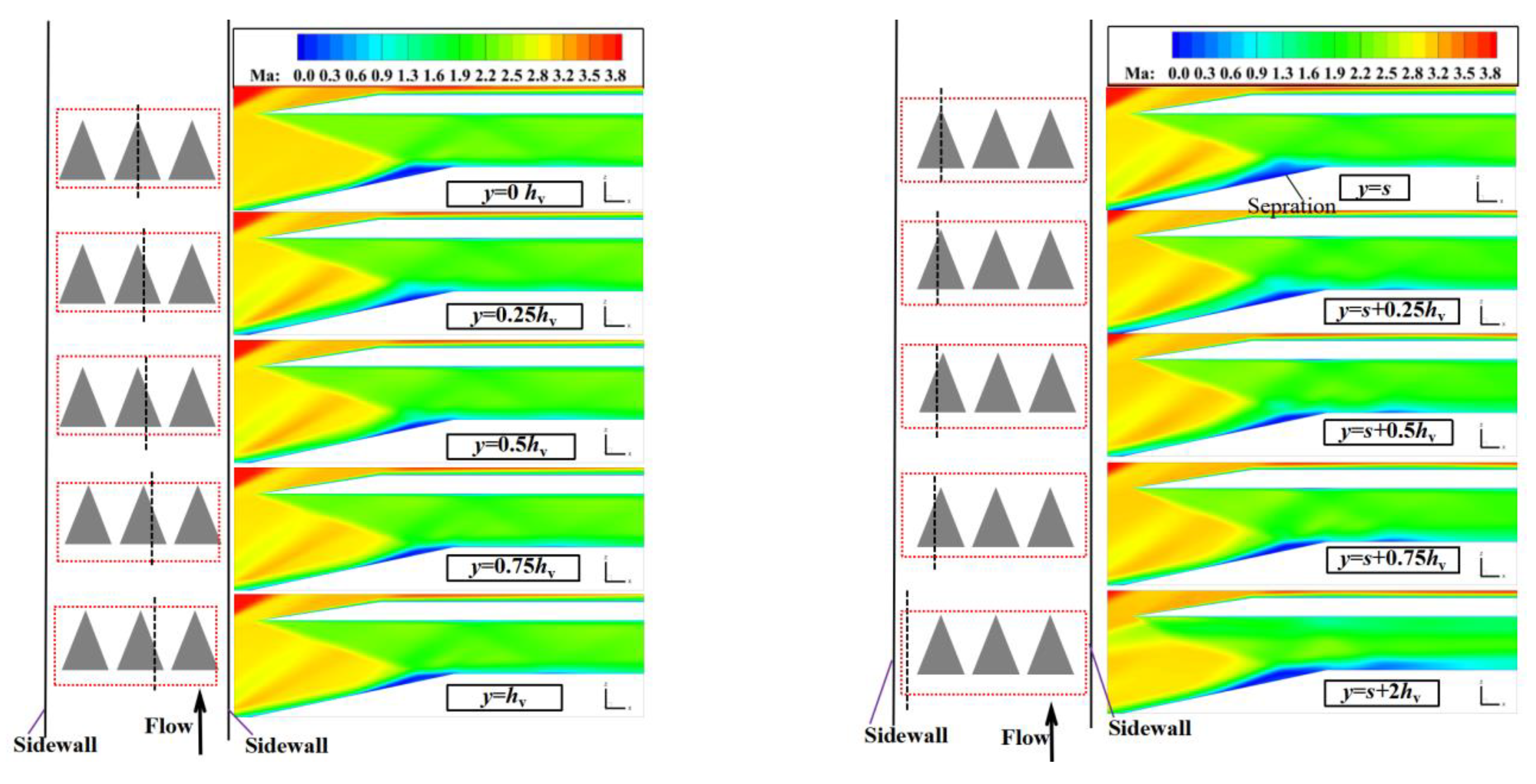
4.2. Capability of Dynamic MVG Array to Control SWBLI in Supersonic Inlet
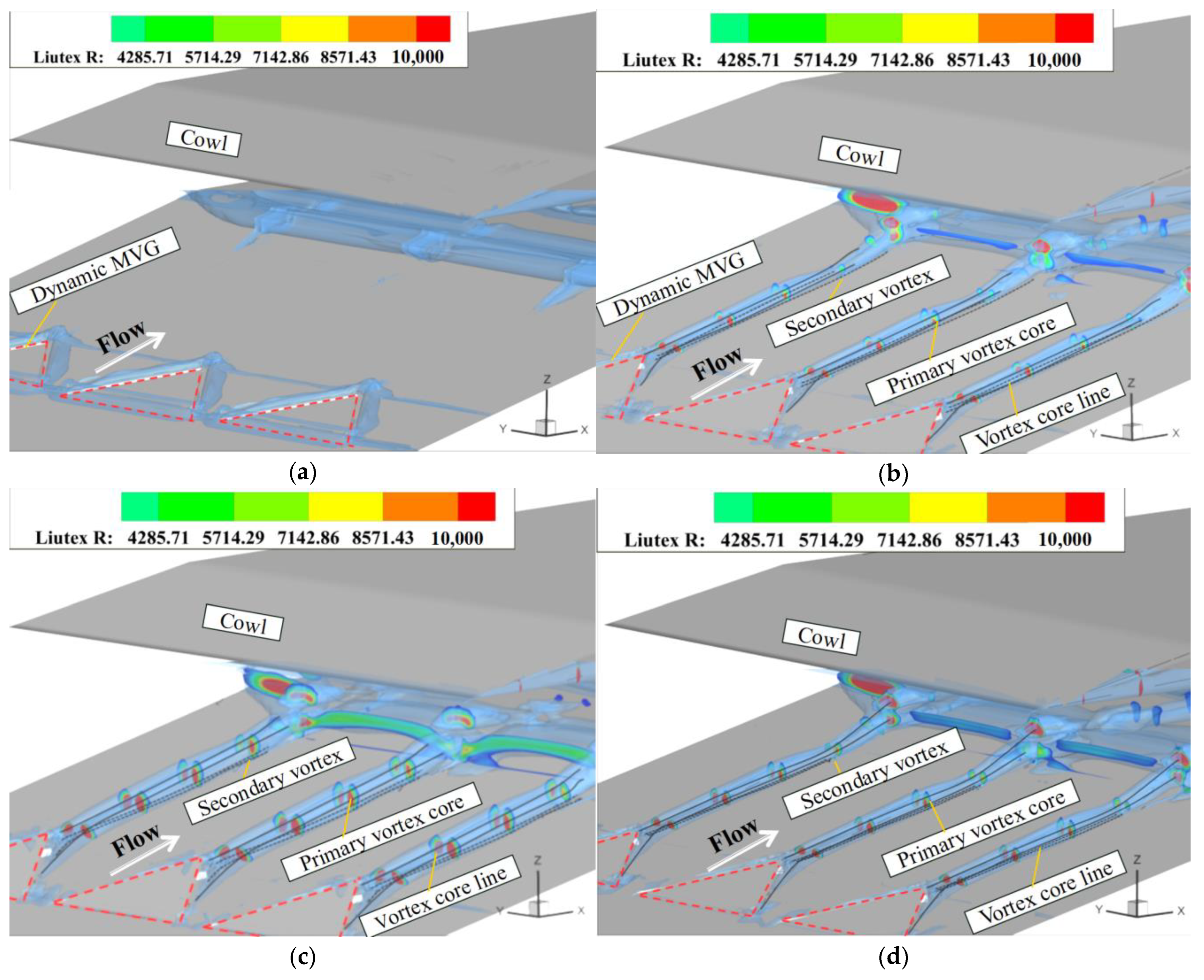
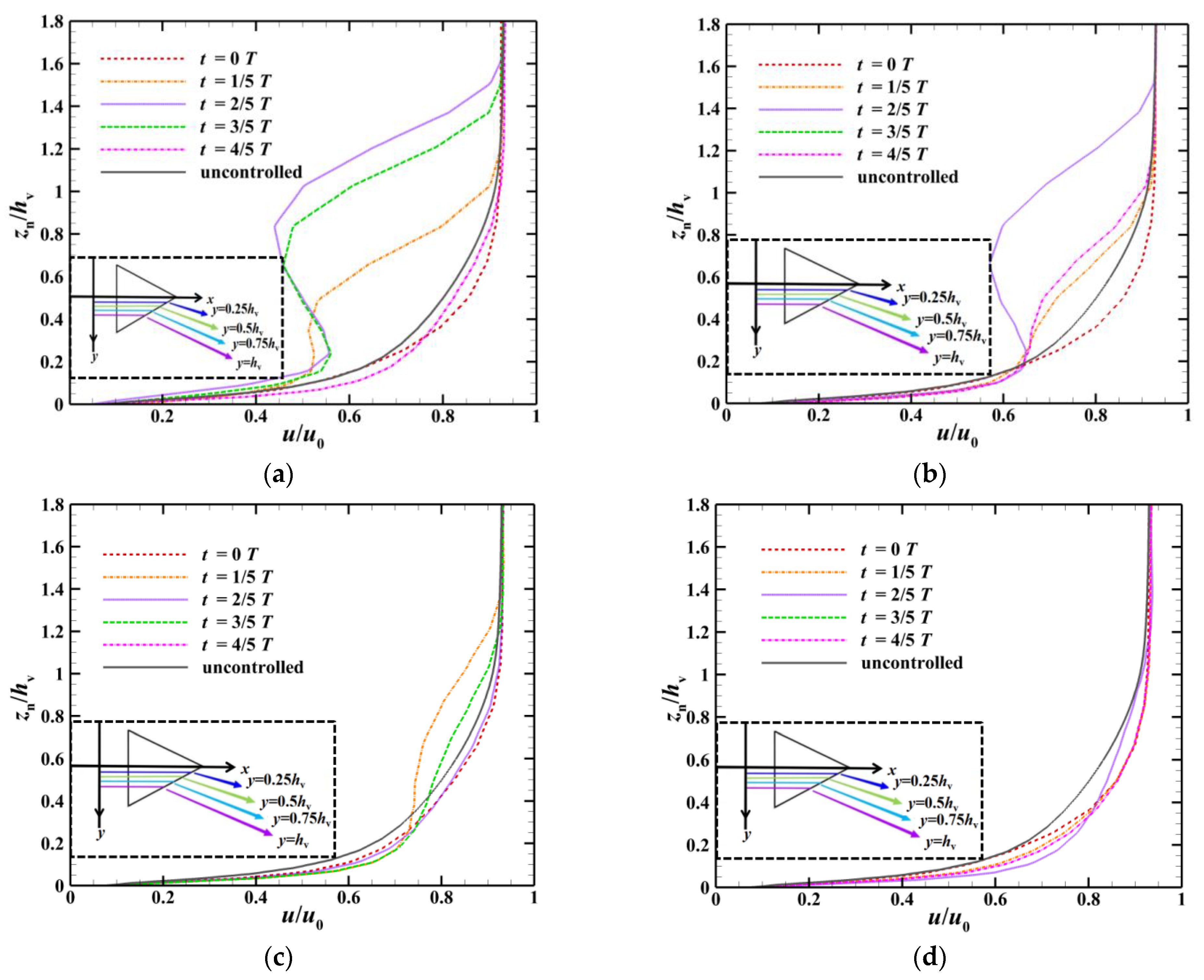
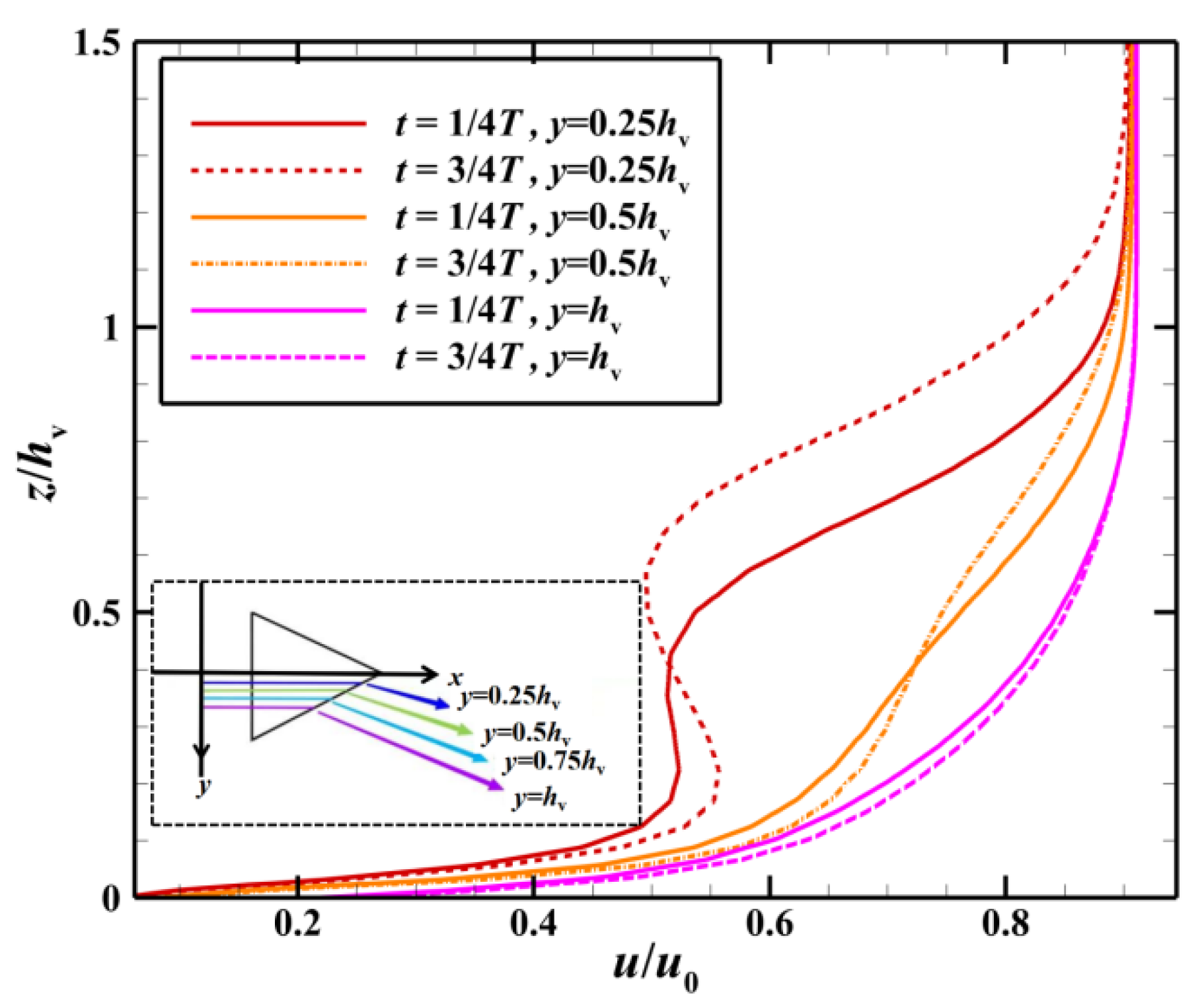
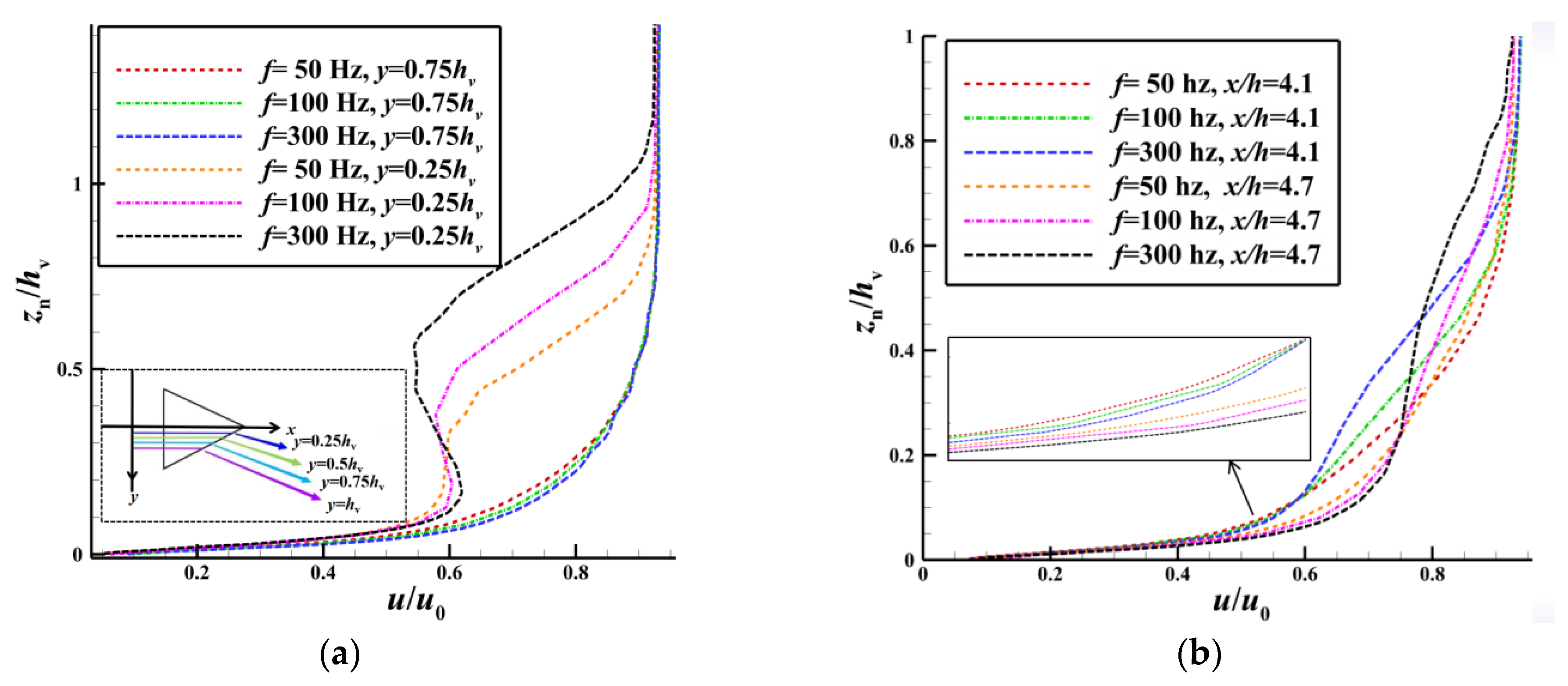
4.2.1. Effect of Dynamic MVG Array on Disturbance in Supersonic Boundary Layer
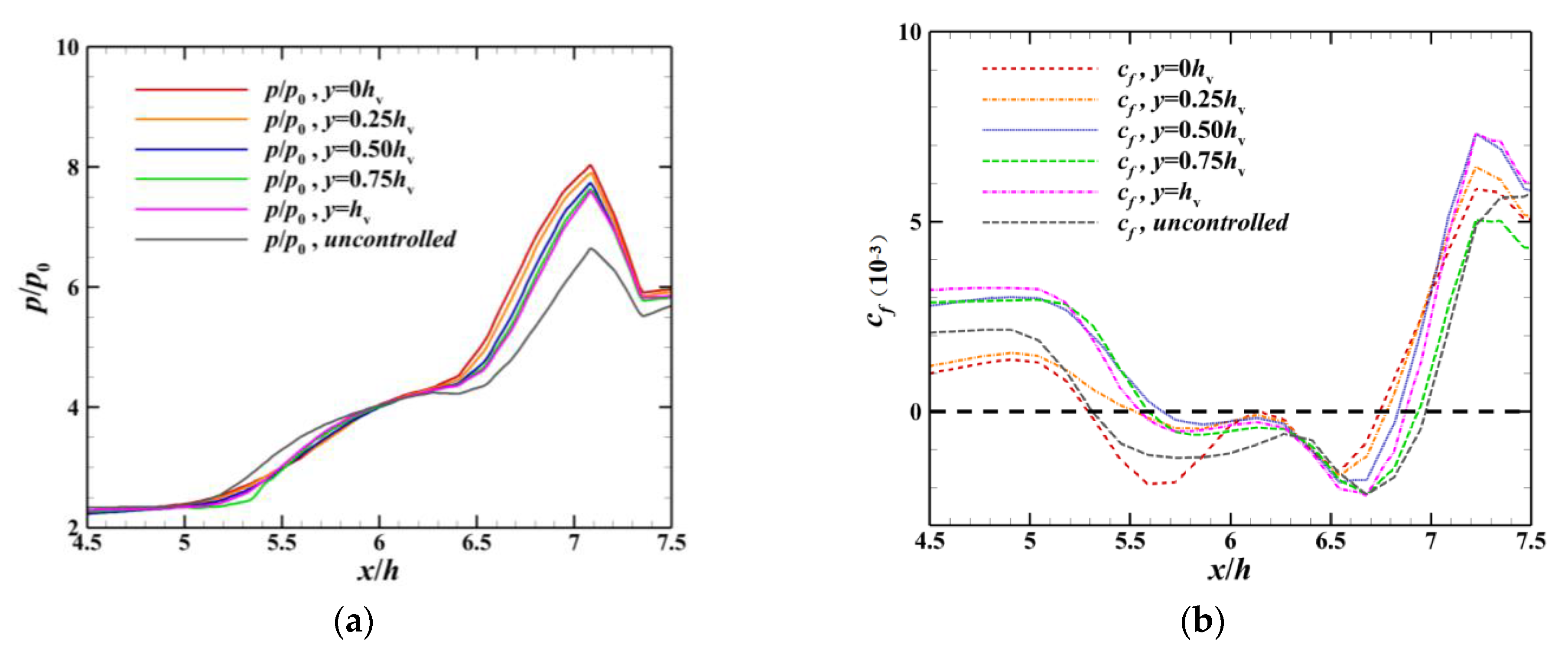
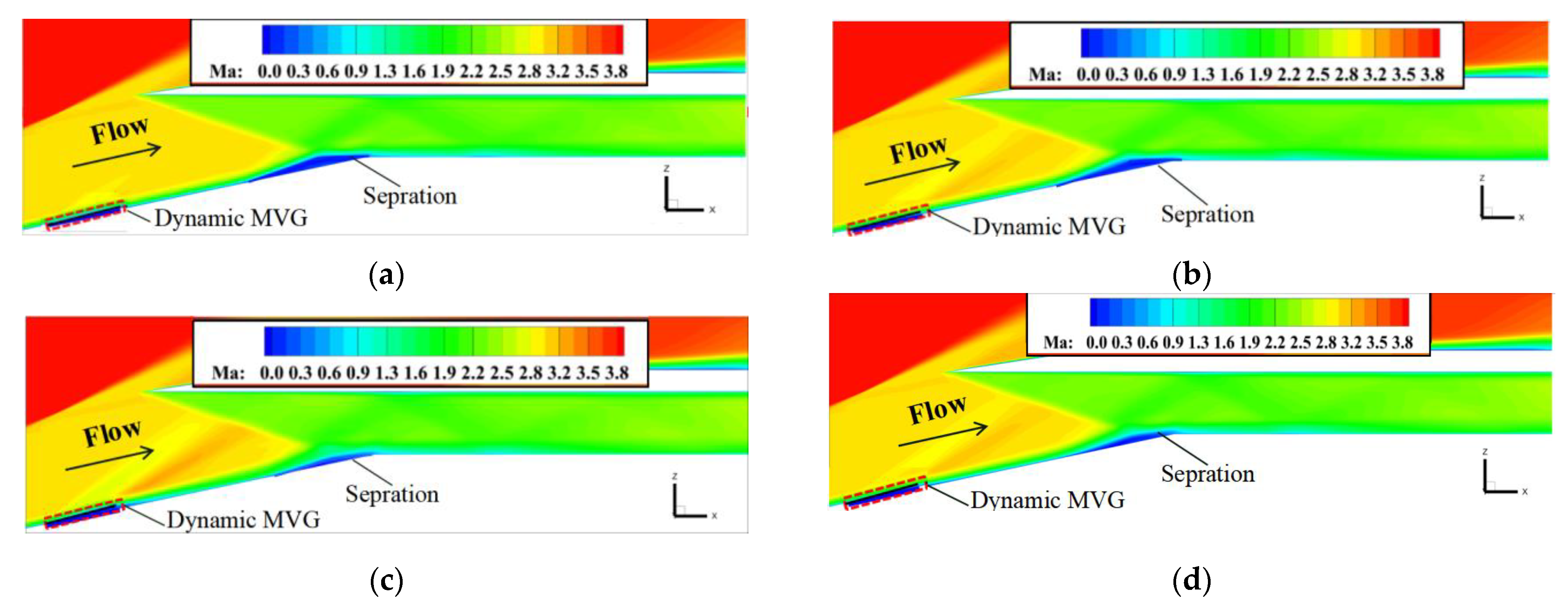
4.2.2. Effect of Dynamic MVG Array on SWBLI
4.3. Influence of Dynamic Frequency of MVG Array on its Control Effect
5. Conclusions
- (1)
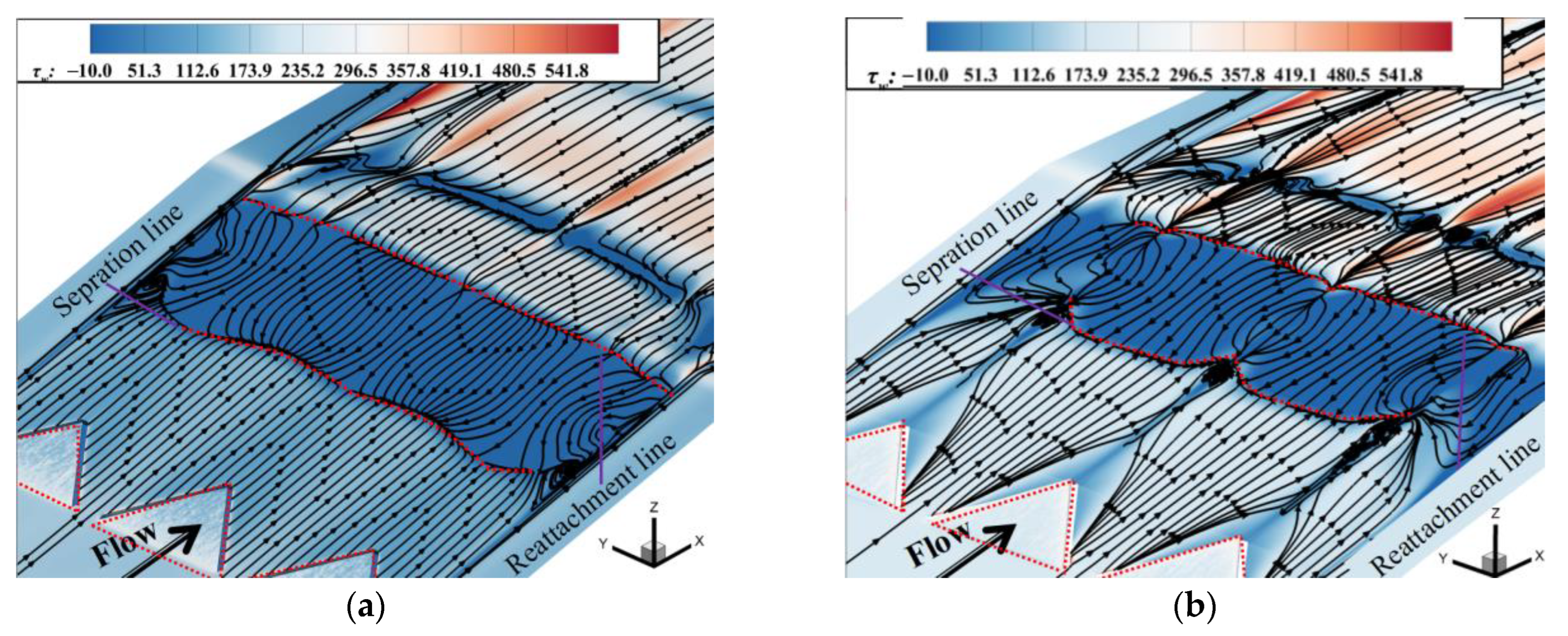
- The incident shock in the supersonic inlet imposed a strong adverse pressure gradient on the boundary layer, which led to its local thickening and separation. Due to the presence of the side wall of the inlet, vortices that intensified transverse flow were generated near the side wall, leading to a complex 3D structure of flow of the separation bubble. A large separation was formed in the middle of the bottom wall.
- (2)
- The dynamic MVGs induced a vortex structure with variable intensity in the supersonic boundary layer due to their oscillation. This enhanced the mixing of the flow of the boundary layer with the high-speed mainstream flow and caused the profile of the velocity of the separation to become fuller while enhancing the stability of the boundary layer. At the same time, the unique effects of “extrusion” and “suction” of the vortex generators during their oscillation continued to charge the airflow, further enhancing its ability to suppress the separation.
- (3)
- When flow was controlled by the array of dynamic MVGs, the height of the separation bubble in the supersonic inlet decreased more significantly than that in the absence of control. Compared with the case without control, the length of the separation in the streamwise direction decreased by up to 31.76%, and the coefficient of total pressure recovery increased by 6.4%.
- (4)
- When the frequency of the dynamic MVG was in the range of 50–300 Hz, the effect of charging the low-speed airflow near the boundary layer was enhanced as the frequency of oscillations of the vortex generators increased, and the shape factor of the boundary layer decreased by up to 30% at a frequency of 300 Hz compared with that in the absence of control.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Seddon, J.; Goldsmith, E.L. Intake Aerodynamics; AIAA Education Series; American Institute of Aeronautics and Astronautics: New York, NY, USA, 1985; pp. 1–30. [Google Scholar]
- Holden, M. Historical review of experimental studies and prediction methods to describe laminar and turbulent shock wave/boundary layer interactions in hypersonic flows. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006. [Google Scholar]
- Tan, H.-J.; Li, C.-H.; Zhang, Y.; Li, G.-S. Review of progress in shock control technology with fixed geometry. J. Propul. Technol. 2016, 37, 2001–2008. [Google Scholar]
- Van Wie, D.M. Scramjet inlets. In Scramjet Propulsion; Curran, E.T., Murthy, S.N.B., Eds.; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2000; Volume 189, pp. 447–511. [Google Scholar]
- Pan, H.-L.; Li, J.-H.; Shen, Q. Studies of turbulence/shock interaction in a scramjet inlet. J. Propul. Technol. 2013, 34, 1172–1178. [Google Scholar]
- Yuan, H.C.; Liang, D.W. Effect of suction on starting of hypersonic inlet. J. Propul. Technol. 2006, 27, 525–528. [Google Scholar]
- Yan, H.-M.; Zhong, J.-J.; Han, J.-A.; Feng, Z.-M. Research on boundary layer suction in the throat of supersonic inlet. J. Propul. Technol. 2009, 30, 175–181. [Google Scholar]
- Zhao, J.; Fan, X.-Q.; Wang, Y.; Tao, J. Classification of flow field in supersonic boundary layer bleed slot. J. Propul. Technol. 2017, 38, 2463–2470. [Google Scholar]
- Shi, X.; Lyu, M.; Zhao, Y.; Tao, S.; Hao, L.; Yuan, X. Flow control technique for shock wave/turbulent boundary layer interactions. Acta Aeronaut. Astronaut. Sin. 2022, 43, 625929. [Google Scholar]
- Wu, H.; Wang, J.; Huang, W.; Du, Z.; Yan, L. Research progress on shock wave/boundary layer interactions and flow controls induced by micro vortex generators. Acta Aeronaut. Astronaut. Sin. 2021, 42, 25371. [Google Scholar]
- Babinsky, H.; Li, Y.; Pitt Ford, C.W. Microramp control of supersonic oblique shock-wave/boundary-layer interactions. AIAA J. 2009, 47, 668–675. [Google Scholar] [CrossRef]
- Wang, B.; Liu, W.; Zhao, Y.; Fan, X.; Wang, C. Experimental investigation of the microramp based shock wave and turbulent boundary layer interaction control. Phys. Fluids 2012, 24, 055110. [Google Scholar]
- Blinde, P.L.; Humble, R.A.; van Oudheusden, B.W.; Scarano, F. Effects of micro-ramps on a shock wave/turbulent boundary layer interaction. Shock Waves 2009, 19, 507–520. [Google Scholar] [CrossRef]
- Anderson, B.H.; Tinapple, J.; Surber, L. Optimal control of shock wave turbulent boundary layer interactions using micro-array actuation. In Proceedings of the 3rd AIAA Flow Control Conference, San Francisco, CA, USA, 5–8 June 2006. [Google Scholar]
- Zhang, Y.; Tan, H.-J.; Du, M.-C.; Wang, D.-P. Control of shock/boundary-layer interaction for hypersonic inlets by highly swept microramps. J. Propul. Power 2015, 31, 133–143. [Google Scholar] [CrossRef]
- Anderson, B.H.; Mace, J.L.; Mani, M. Active “fail safe” micro-array flow control for advanced embedded propulsion systems. In Proceedings of the 47th Aerospace Sciences Meeting, sponsored by the American Institute of Aeronautics and Astronautics, Orlando, FL, USA, 5–8 January 2009. [Google Scholar]
- Wagner, J.L. Experimental Studies of Unstart Dynamics in Inlet/Isolator Configurations in a Mach 5 Flow. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, 2009. [Google Scholar]
- Giepman, R.H.M.; Schrijer, F.F.J.; Van Oudheusden, B.W. Flow control of an oblique shock wave reflection with micro-ramp vortex generators: Effects of location and size. Phys. Fluids 2014, 26, 066101. [Google Scholar] [CrossRef]
- Wang, M.G.; He, X.M.; Wang, J.J.; Zhang, Y.; Wang, K.; Tan, H.J.; Li, L.G. Shock wave/boundary layer interaction control method based on oscillating vortex generator. J. Aviat. 2023, 1–16. Available online: http://kns.cnki.net/kcms/detail/11.1929.v.20230421.1348.010.html (accessed on 14 August 2023).
- Lee, S.; Goettke, M.K.; Loth, E.; Tinapple, J.; Benek, J. Microramps upstream of an oblique-shock/boundary-layer interaction. AIAA J. 2010, 48, 104–118. [Google Scholar] [CrossRef]
- Van Leer, B. Towards the ultimate conservative difference scheme. V. A second-order sequel to Godunov’s method. J. Comput. Phys. 1979, 32, 101–136. [Google Scholar] [CrossRef]
- White, F.M. Viscous Fluid Flow; McGraw-Hill: New York, NY, USA, 1991. [Google Scholar]
- Jiao, X.; Chang, J.; Wang, Z.; Yu, D. Numerical study on hypersonic nozzleinlet starting characteristics in a shock tunnel. Acta Astronaut. 2017, 130, 167–179. [Google Scholar] [CrossRef]
- Sekar, K.R.; Karthick, S.K.; Jegadheeswaran, S.; Kannan, R. On the unsteady throttling dynamics and scaling analysis in a typical hypersonic inlet–isolator flow. Phys. Fluids 2020, 32, 126104. [Google Scholar] [CrossRef]
- Huang, H.-X.; Tan, H.-J.; Sun, S.; Ling, Y. Evolution of supersonic corner vortex in a hypersonic inlet/isolator model. Phys. Fluids 2016, 28, 126101. [Google Scholar] [CrossRef]
- Jiao, X.; Chang, J.; Wang, Z.; Yu, D. Mechanism study on local unstart of hypersonic inlet at high Mach number. AIAA J. 2015, 53, 3102–3112. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- ANSYS Inc. Fluent Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2018. [Google Scholar]
- Wilcox, D.C. Turbulence Modeling for CFD; DCW Industries: La Cañada, CA, USA, 1993. [Google Scholar]
- Li, N.; Chang, J.T.; Xu, K.J.; Yu, D.R.; Bao, W.; Song, Y.P. Prediction dynamic model of shock train with complex background waves. Phys. Fluids 2017, 29, 116103. [Google Scholar] [CrossRef]
- Jordan, C.; Edwards, J.R.; Stefanski, D.L. Evaluation of RANS closure models using LES datasets for hypersonic shock boundary layer interactions. In Proceedings of the AIAA Scitech 2021 Forum, Virtual, 11–15 January 2021. [Google Scholar]
- Bonne, N.; Brion, V.; Garnier, E.; Bur, R.; Molton, P.; Sipp, D.; Jacquin, L. Analysis of the two-dimensional dynamics of a Mach 1.6 shock wave/transitional boundary layer interaction using a RANS based resolvent approach. J. Fluid Mech. 2019, 862, 1166–1202. [Google Scholar] [CrossRef]
- Hong, W.; Kim, C. Computational study on hysteretic inlet buzz characteristics under varying mass flow conditions. AIAA J. 2014, 52, 1357–1373. [Google Scholar] [CrossRef]
- Hulme, I.; Clavelle, E.; Van der Lee, L.; Kantzas, A. CFD Modeling and Validation of Bubble Properties for a Bubbling Fluidized Bed. Ind. Eng. Chem. Res. 2005, 44, 4254–4266. [Google Scholar] [CrossRef]
- Lambourne, N.C.; Landon, R.H.; Zwaan, R.J. Compendium of Unsteady Aerodynamics Measurements; AGARD-R-702; Technical Editing and Reproduction Ltd.: London, UK, 1982. [Google Scholar]
- Giepman, R.; Srivastava, A.; Schrijer, F.; van Oudheusden, B. The effects of Mach and Reynolds number on the flow mixing properties of micro-ramp vortex generators in a supersonic boundary layer. In Proceedings of the 45th AIAA Fluid Dynamics Conference, Dallas, TX, USA, 22–26 June 2015. [Google Scholar]
- Liu, C.Q. Liutex-vortex definition and the third generation vortex recognition method. Acta Aerodyn. Sin. 2020, 38, 413–431. [Google Scholar]
- Kendall, A.; Koochesfahani, M. A method for estimating wall friction in turbulent wall-bounded flows. Exp. Fluids 2008, 44, 773–780. [Google Scholar] [CrossRef]
- Babinsky, H.; Titchener, N. Shock Boundary Layer Interaction Flow Control with Micro Vortex Generators; European Office of Aerospace Research and Development: London, UK, 2011. [Google Scholar]
- Babinsky, H.; Harvey, J.K. Shock Wave–Boundary-Layer Interactions; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
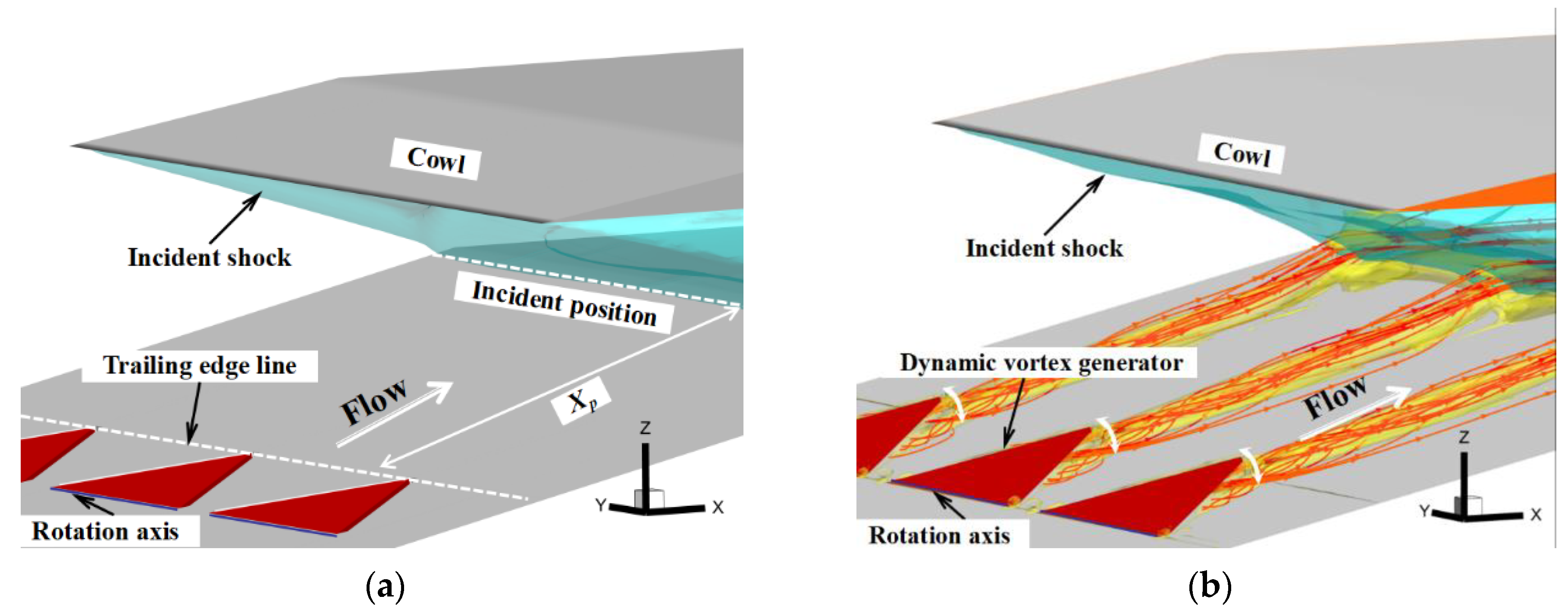
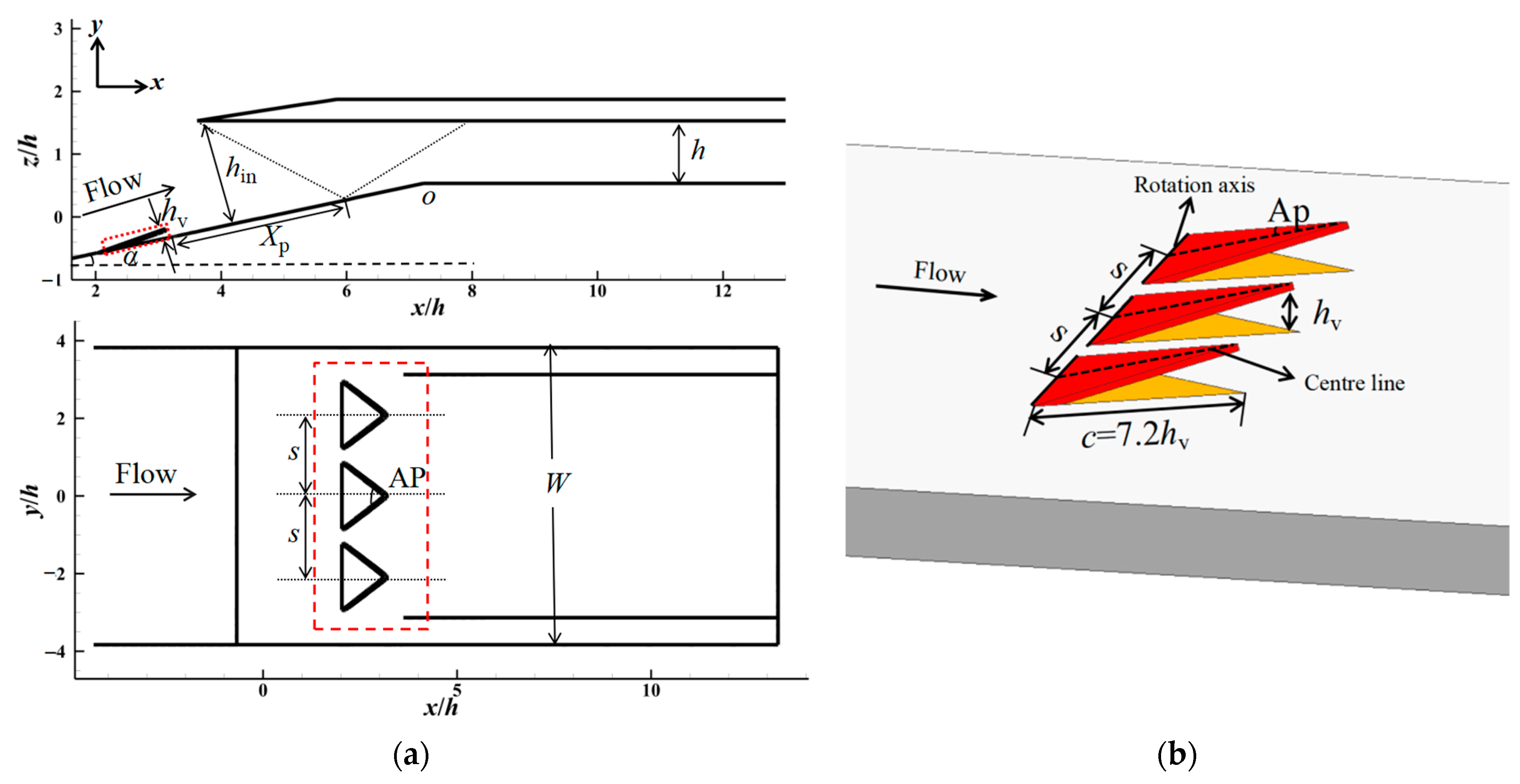
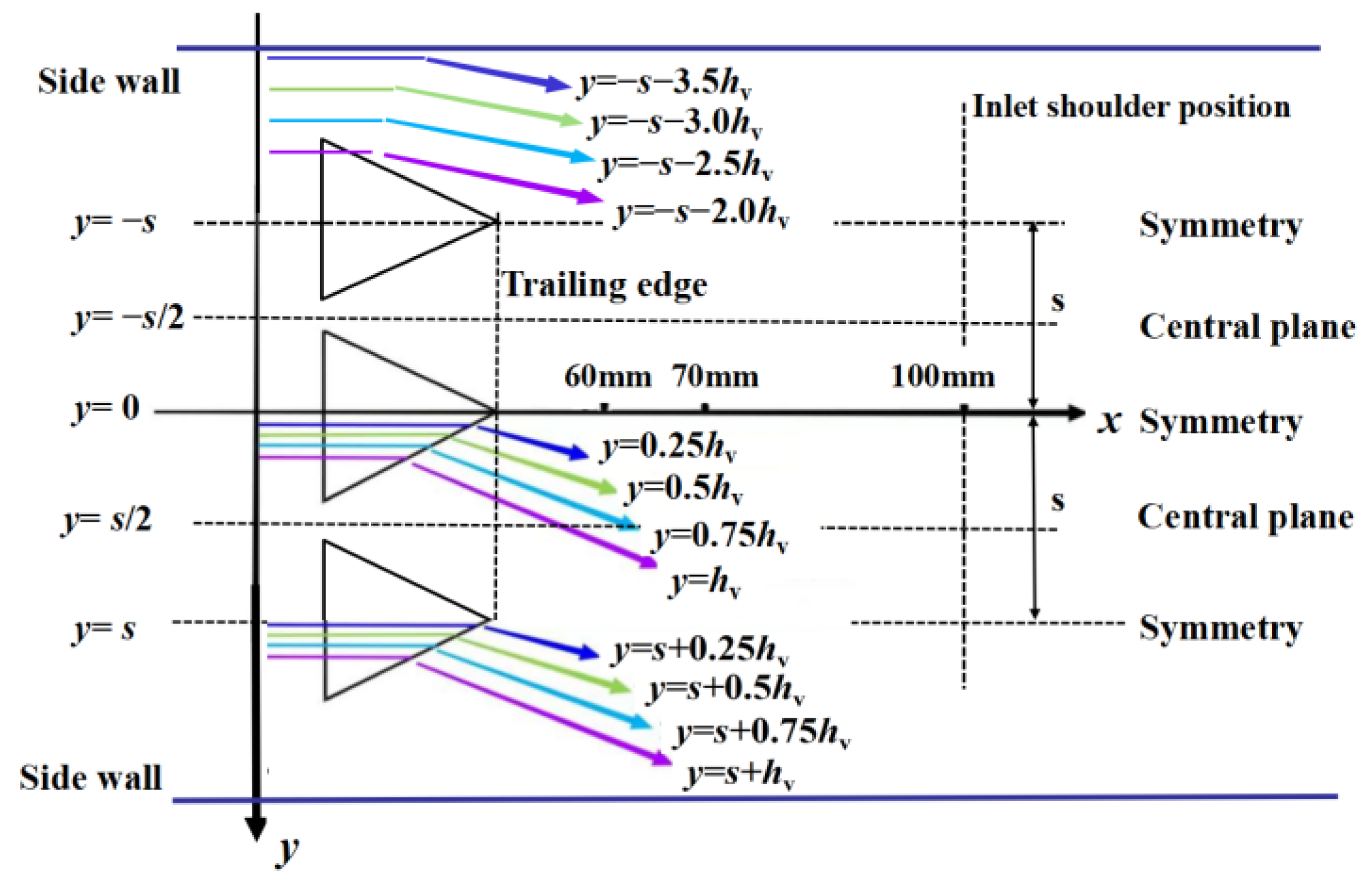
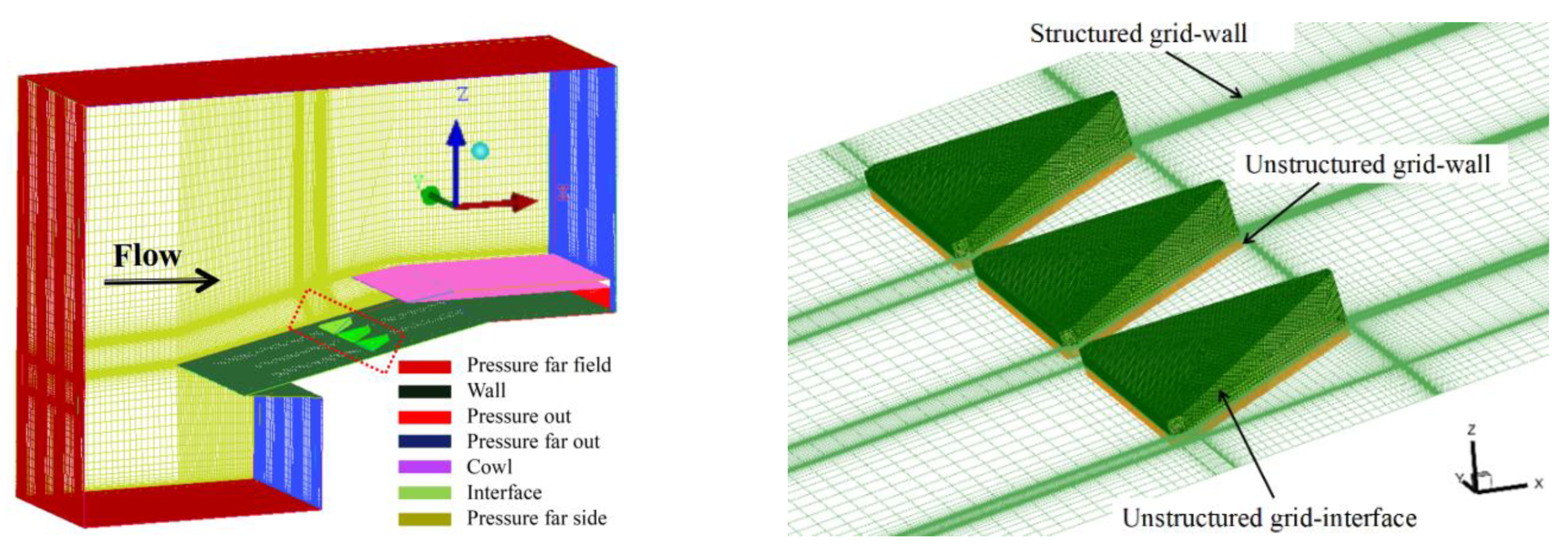
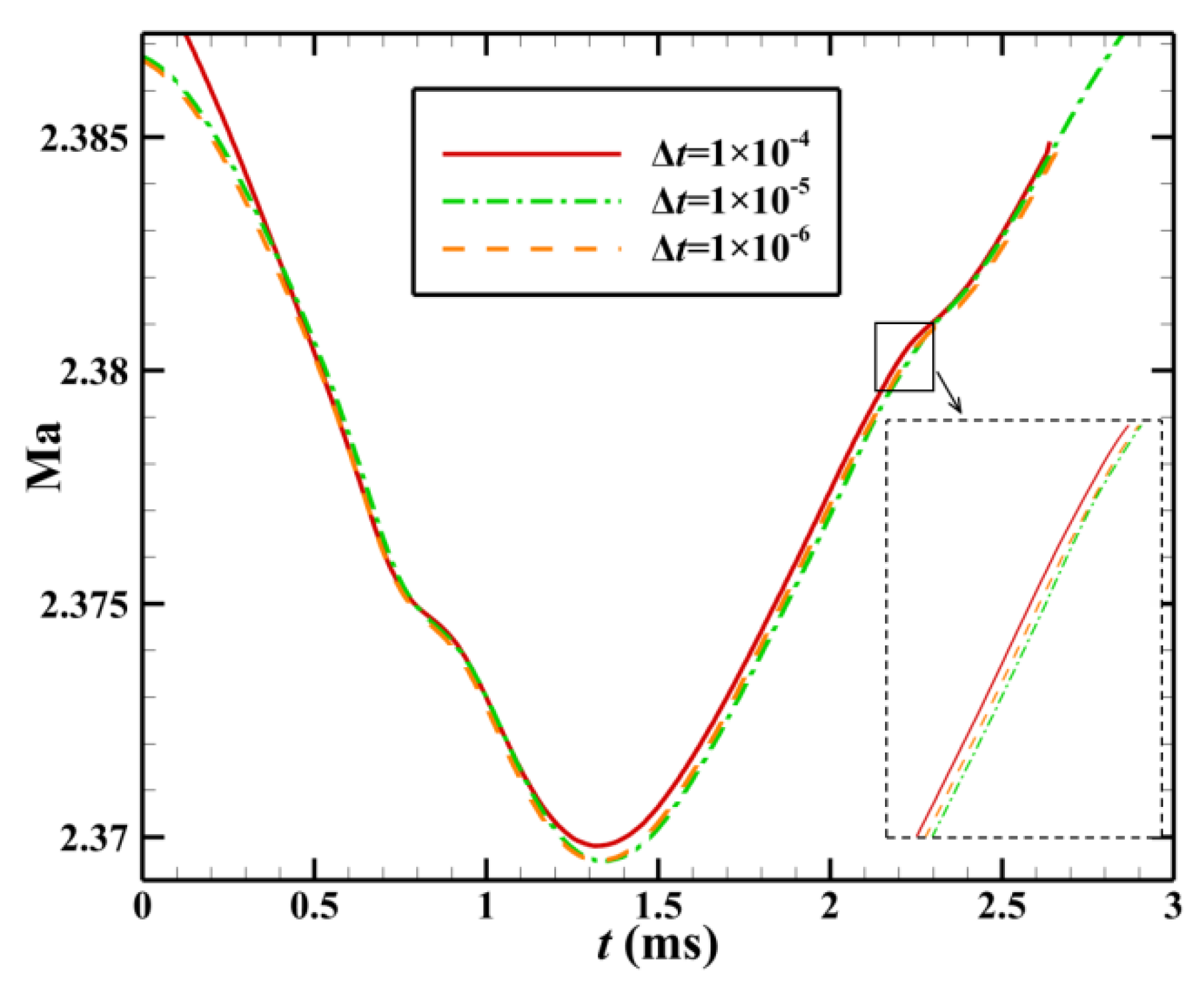
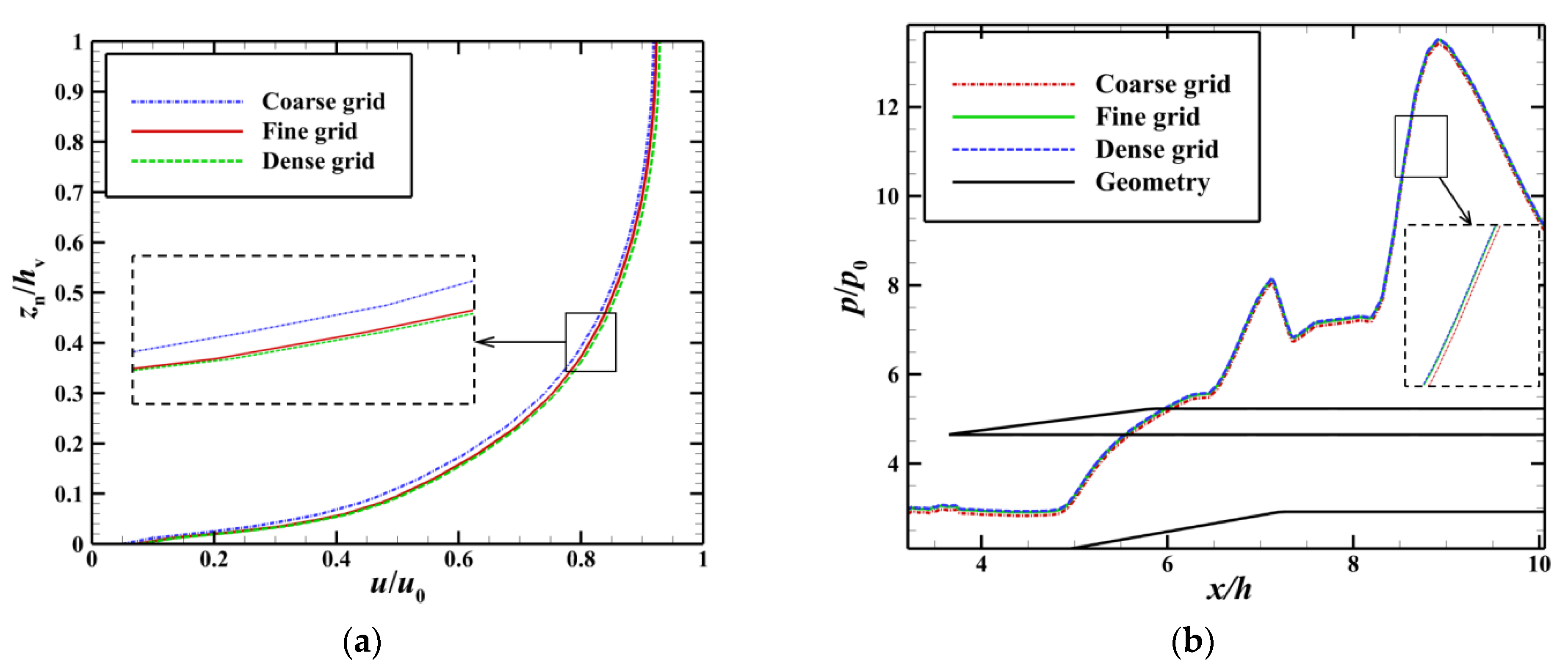

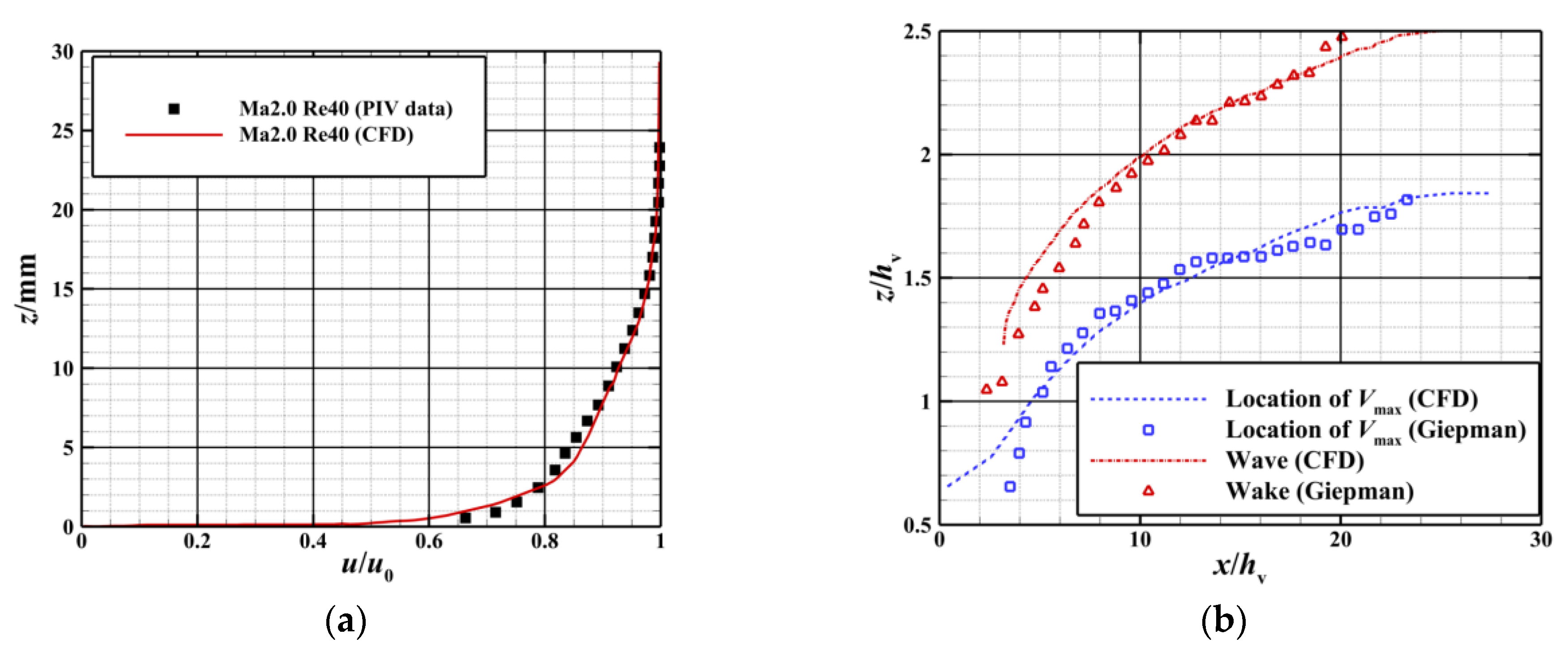
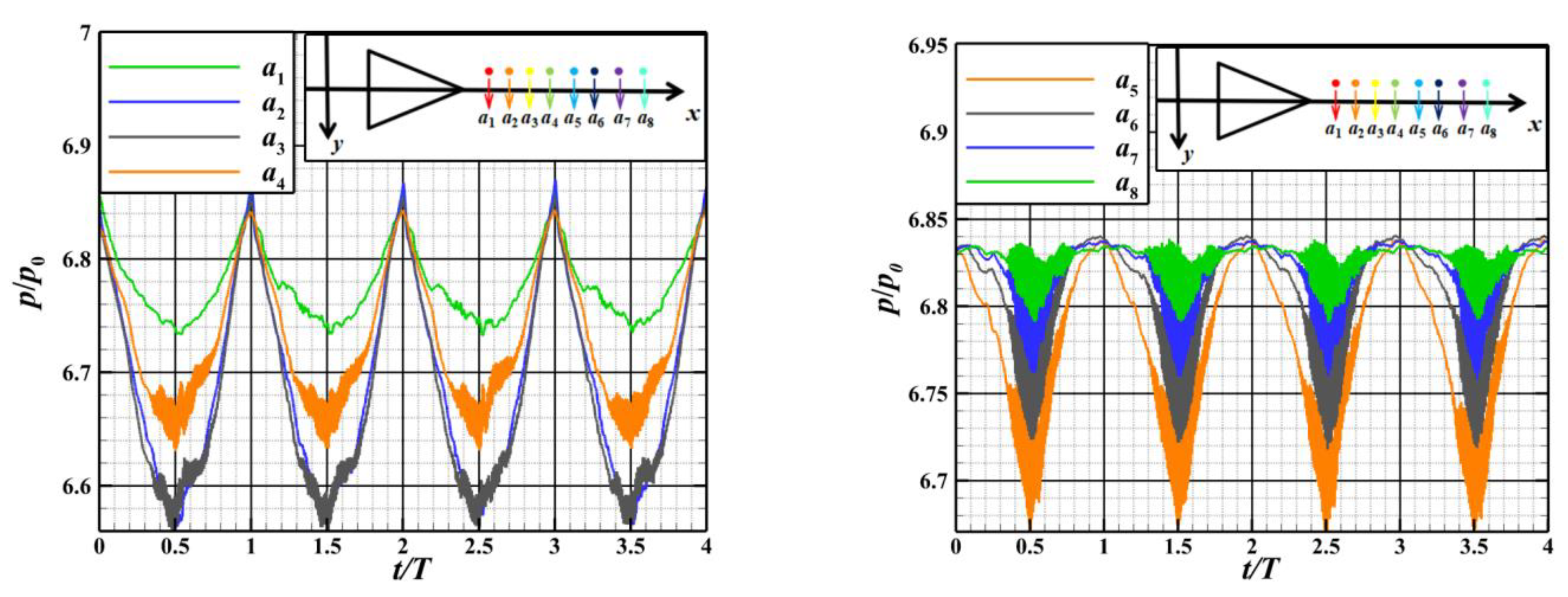



| Design Parameter | Value |
|---|---|
| h (mm) | 14.4 |
| hv (mm) | 4.0 |
| hin (mm) | 24.9 |
| W (mm) | 110.0 |
| AP | 24.0 |
| s (mm) | 30.0 |
| c (mm) | 28.8 |
| α (°) | 12.0 |
| Xp (mm) | 40 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, M.; Wang, Z.; Zhang, Y.; Cheng, D.; Tan, H.; Wang, K.; Gao, S. Control of Cowl Shock/Boundary Layer Interaction in Supersonic Inlet Based on Dynamic Vortex Generator. Aerospace 2023, 10, 729. https://doi.org/10.3390/aerospace10080729
Wang M, Wang Z, Zhang Y, Cheng D, Tan H, Wang K, Gao S. Control of Cowl Shock/Boundary Layer Interaction in Supersonic Inlet Based on Dynamic Vortex Generator. Aerospace. 2023; 10(8):729. https://doi.org/10.3390/aerospace10080729
Chicago/Turabian StyleWang, Mengge, Ziyun Wang, Yue Zhang, Daishu Cheng, Huijun Tan, Kun Wang, and Simin Gao. 2023. "Control of Cowl Shock/Boundary Layer Interaction in Supersonic Inlet Based on Dynamic Vortex Generator" Aerospace 10, no. 8: 729. https://doi.org/10.3390/aerospace10080729
APA StyleWang, M., Wang, Z., Zhang, Y., Cheng, D., Tan, H., Wang, K., & Gao, S. (2023). Control of Cowl Shock/Boundary Layer Interaction in Supersonic Inlet Based on Dynamic Vortex Generator. Aerospace, 10(8), 729. https://doi.org/10.3390/aerospace10080729








