Attitude Tracking Adaptive Control of a Geocentric Polar Displaced Solar Sail
Abstract
1. Introduction
- Although the mass of a solar sail is not too large, its special type of structure makes the overall dimensions very large, resulting in a solar sail spacecraft with a large rotational inertia.
- The flight time of a solar sail in polar displaced orbit would be very long, typically at least 2 years.
- Solar sails have the characteristics of high flexibility and low frequency and are prone to system vibration due to slight disturbances in the space environment.
2. Attitude Dynamics Model of a GPDSS
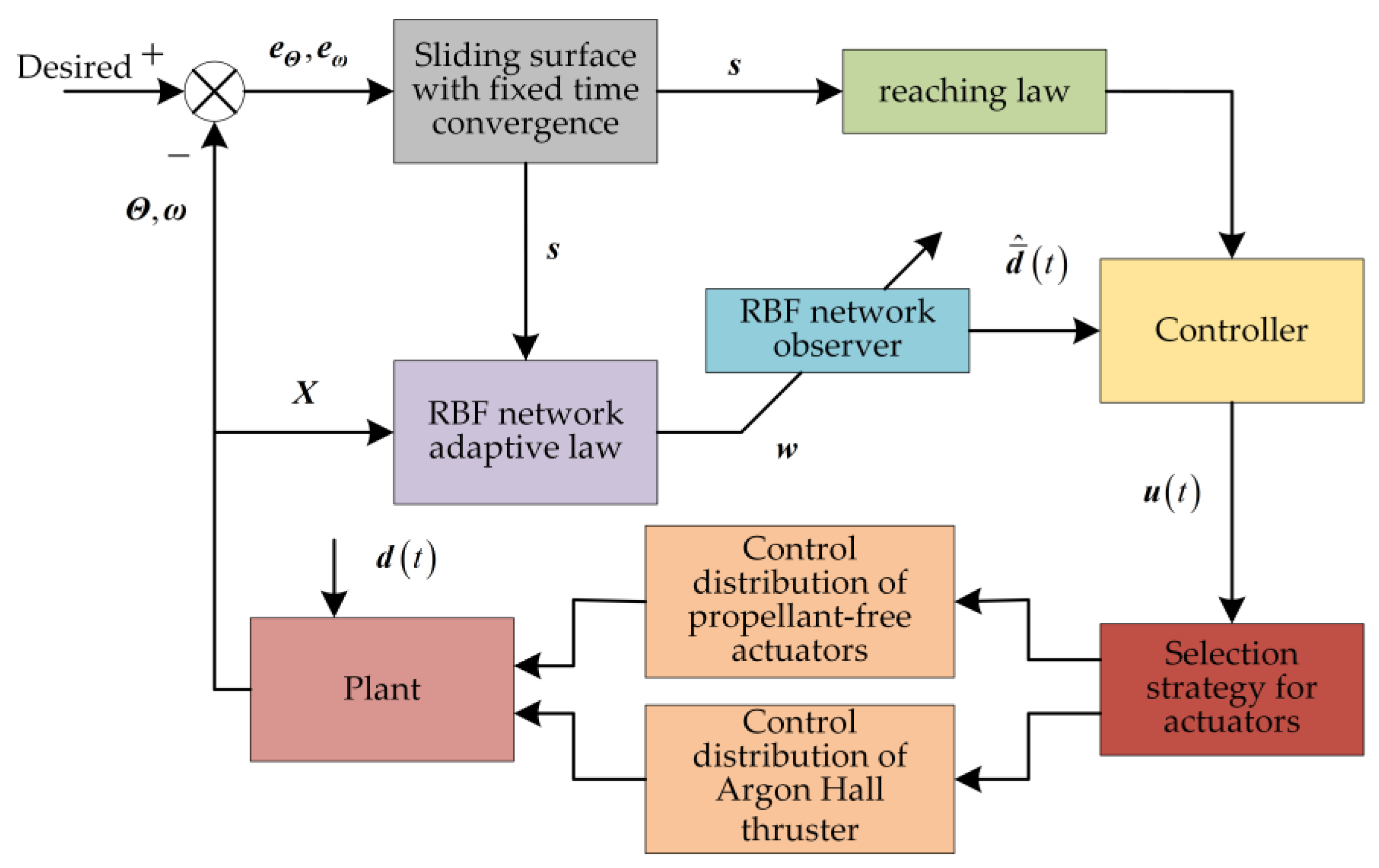
3. Attitude Tracking Controller Design
3.1. RBF Neural Network Disturbance Estimator Design

3.2. Control Law Design
3.3. Stability Analysis
- Step 1. The Lyapunov function is chosen as follows:
3.4. Control Variable Conversion
4. Simulation Results and Discussion
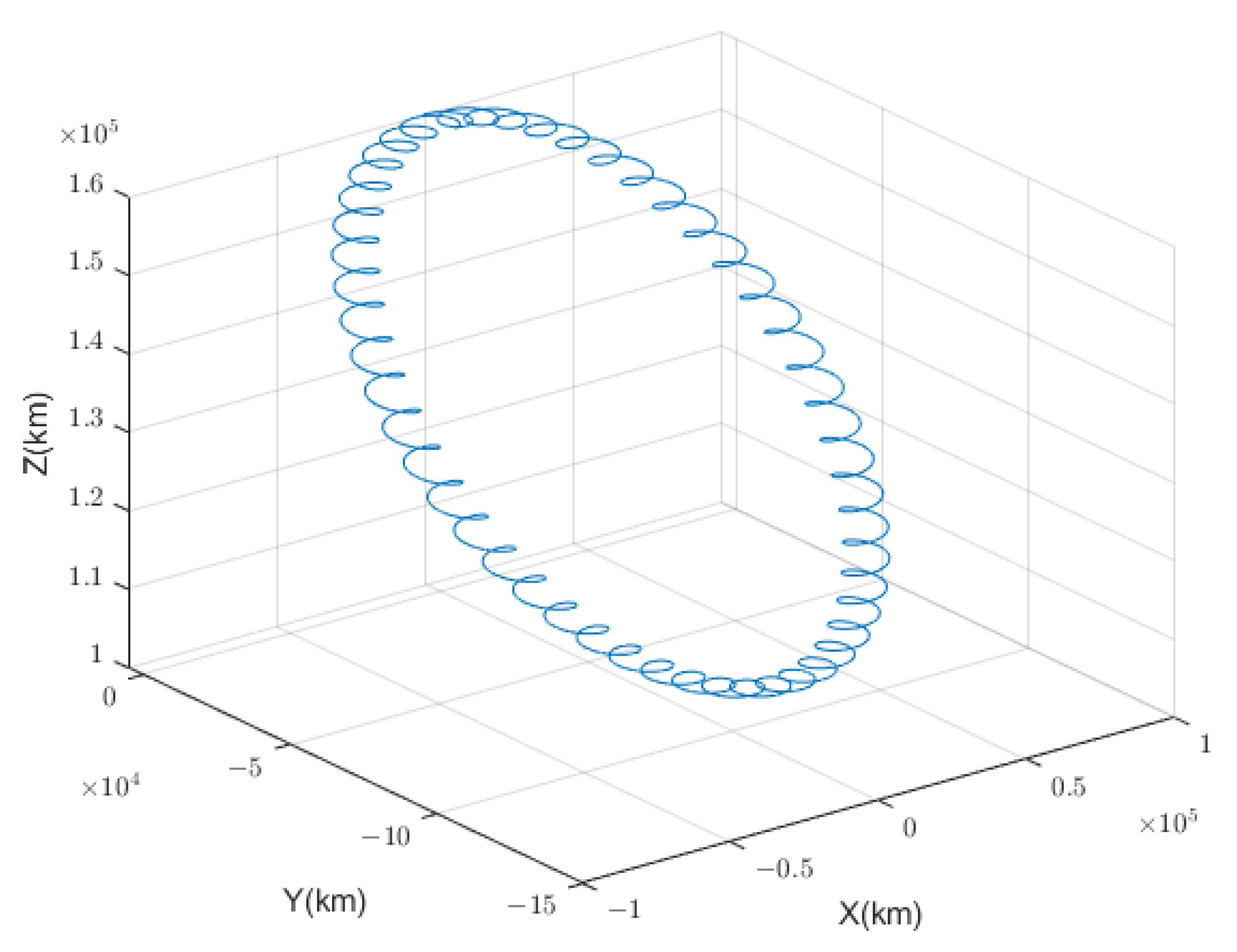
4.1. Simulation Conditions
4.2. Simulation Results and Analysis
- Case 1: there are small initial state errors.
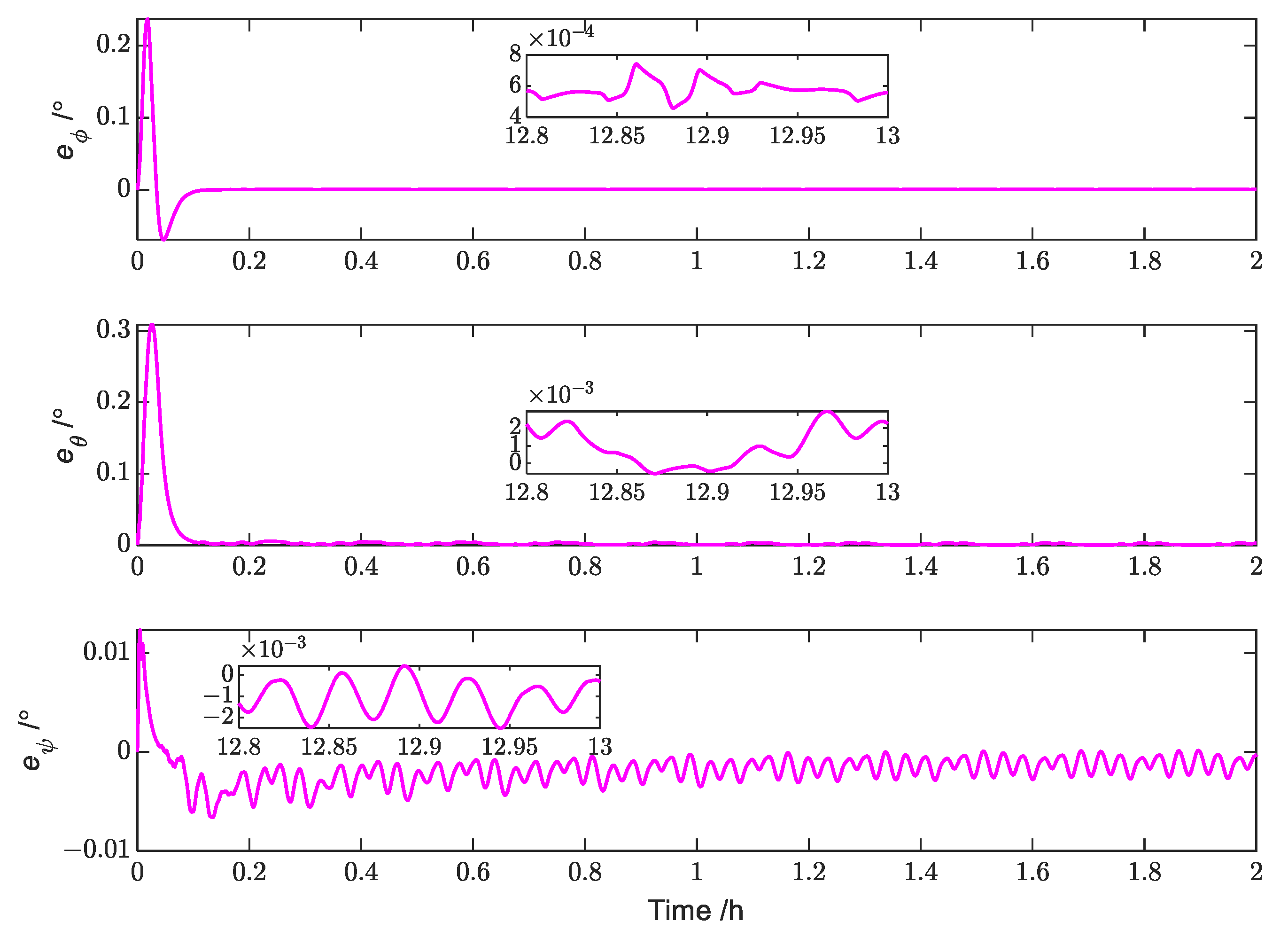


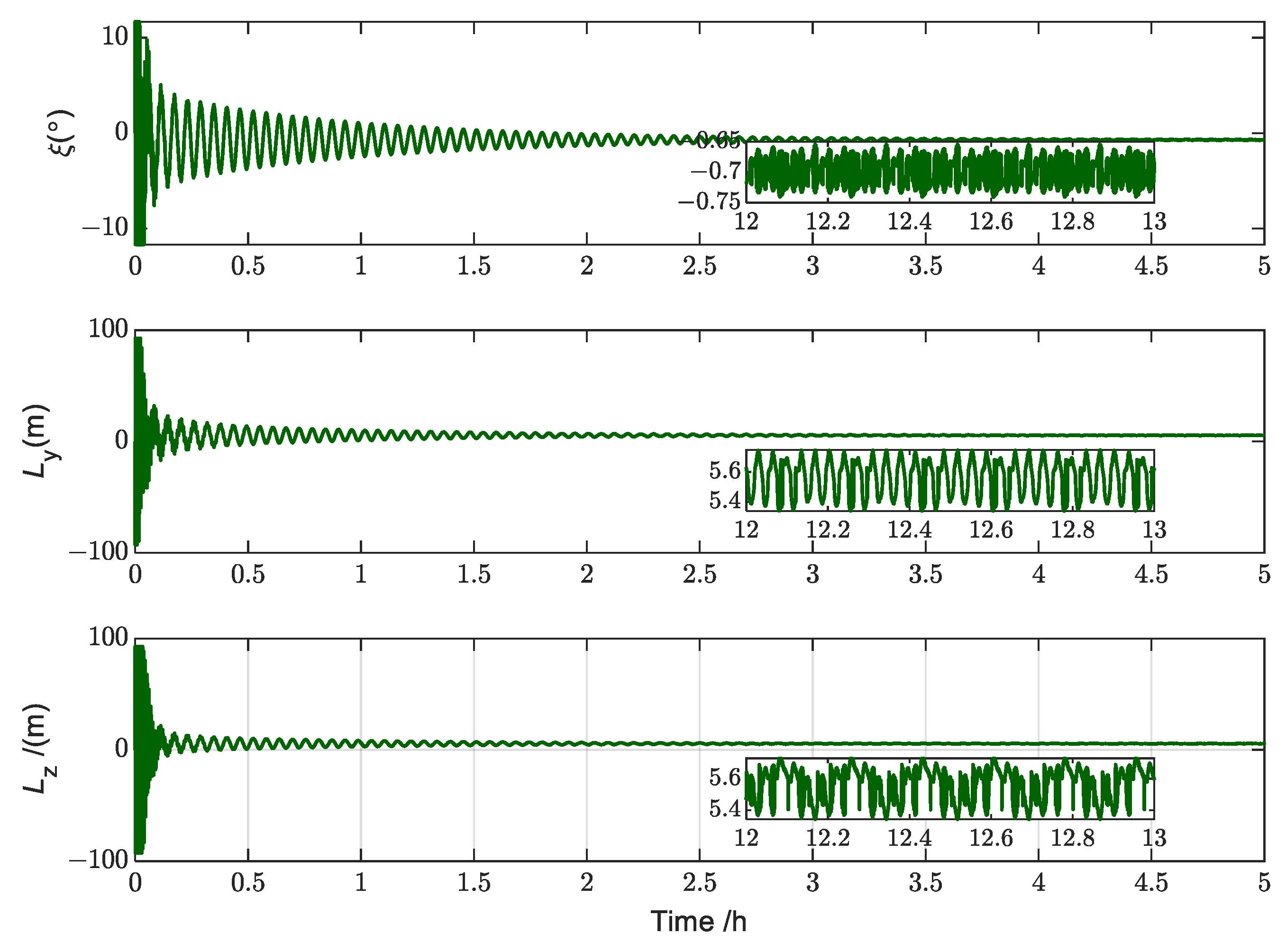



- Case 2: there are large initial state errors.
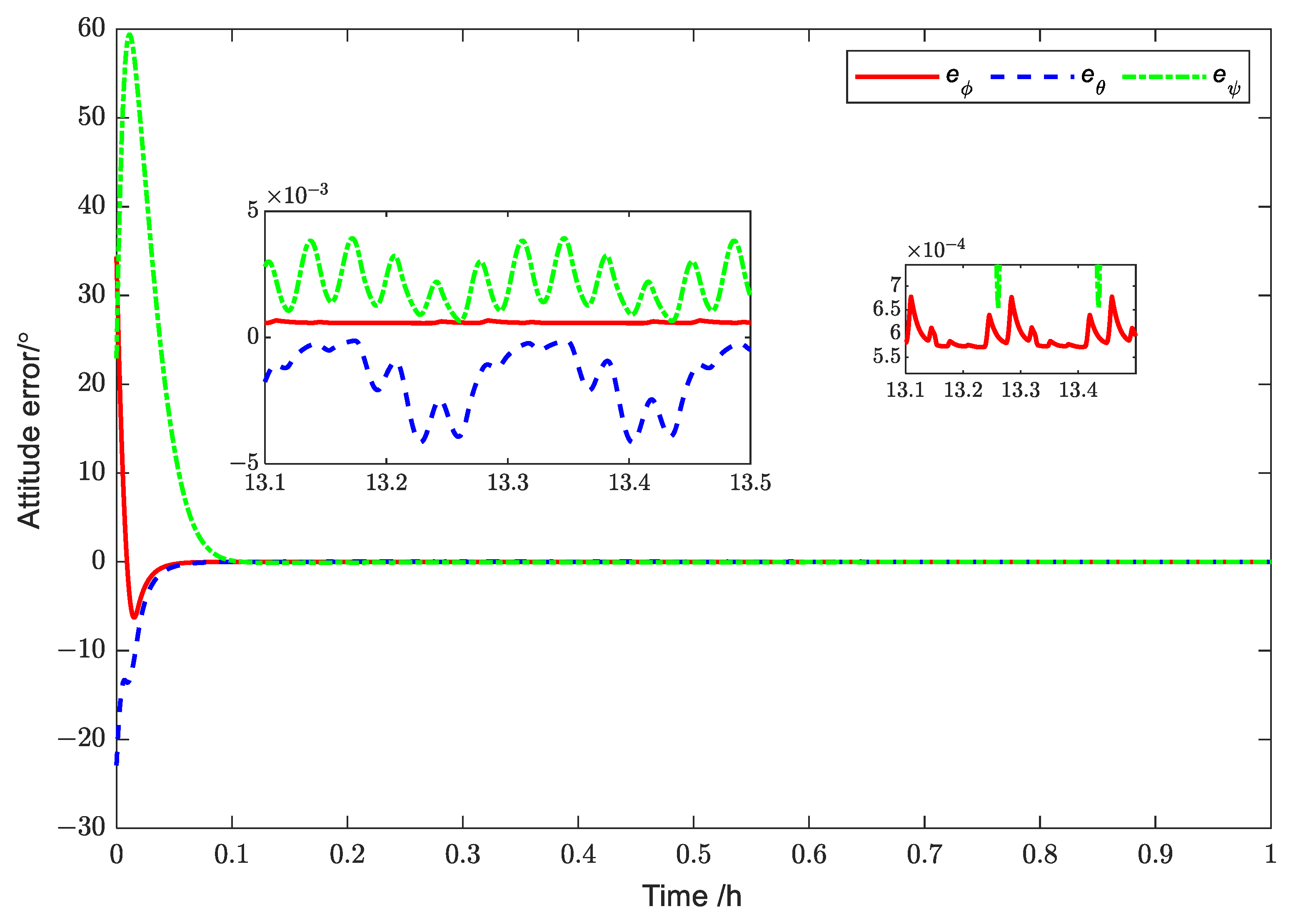

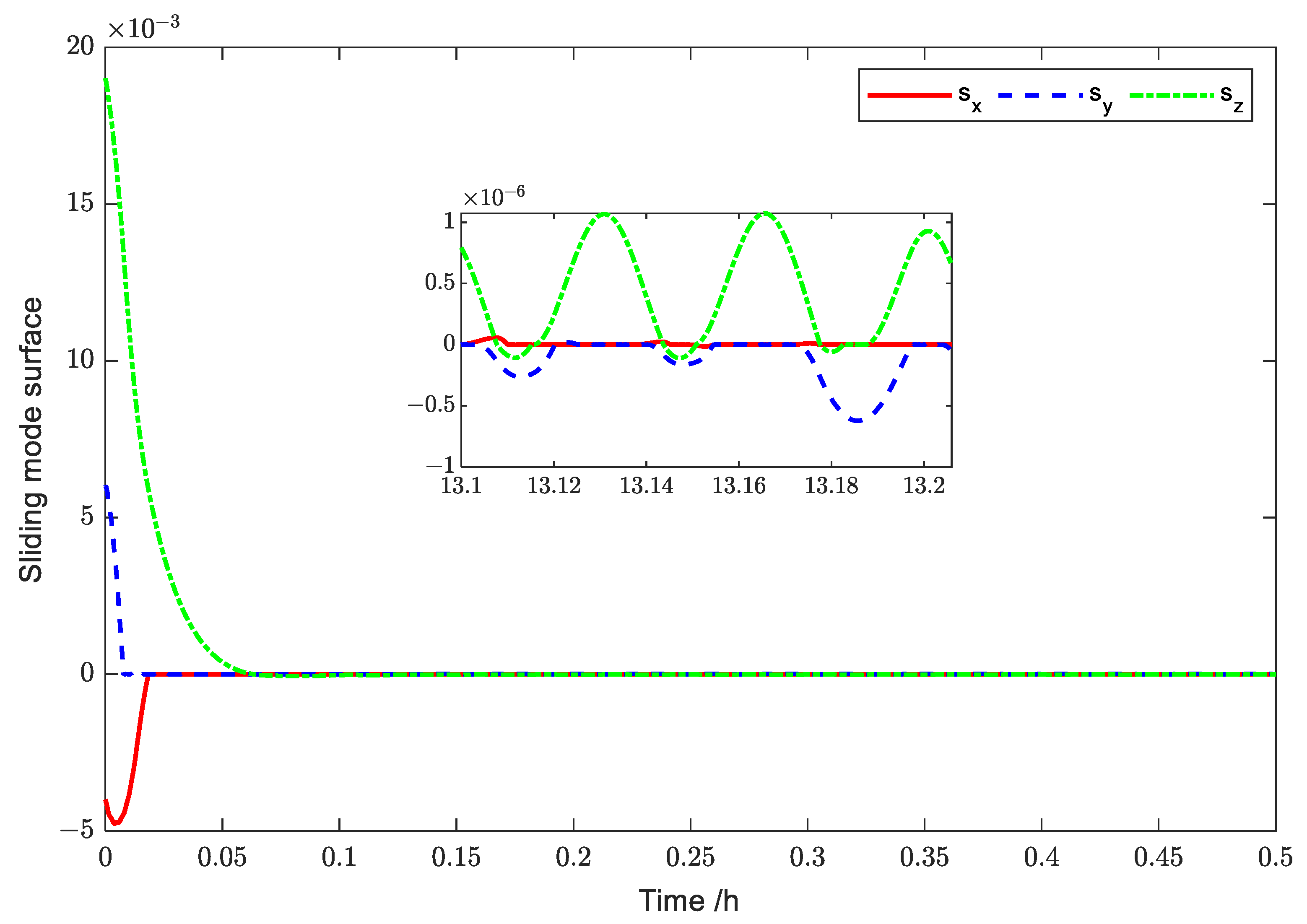
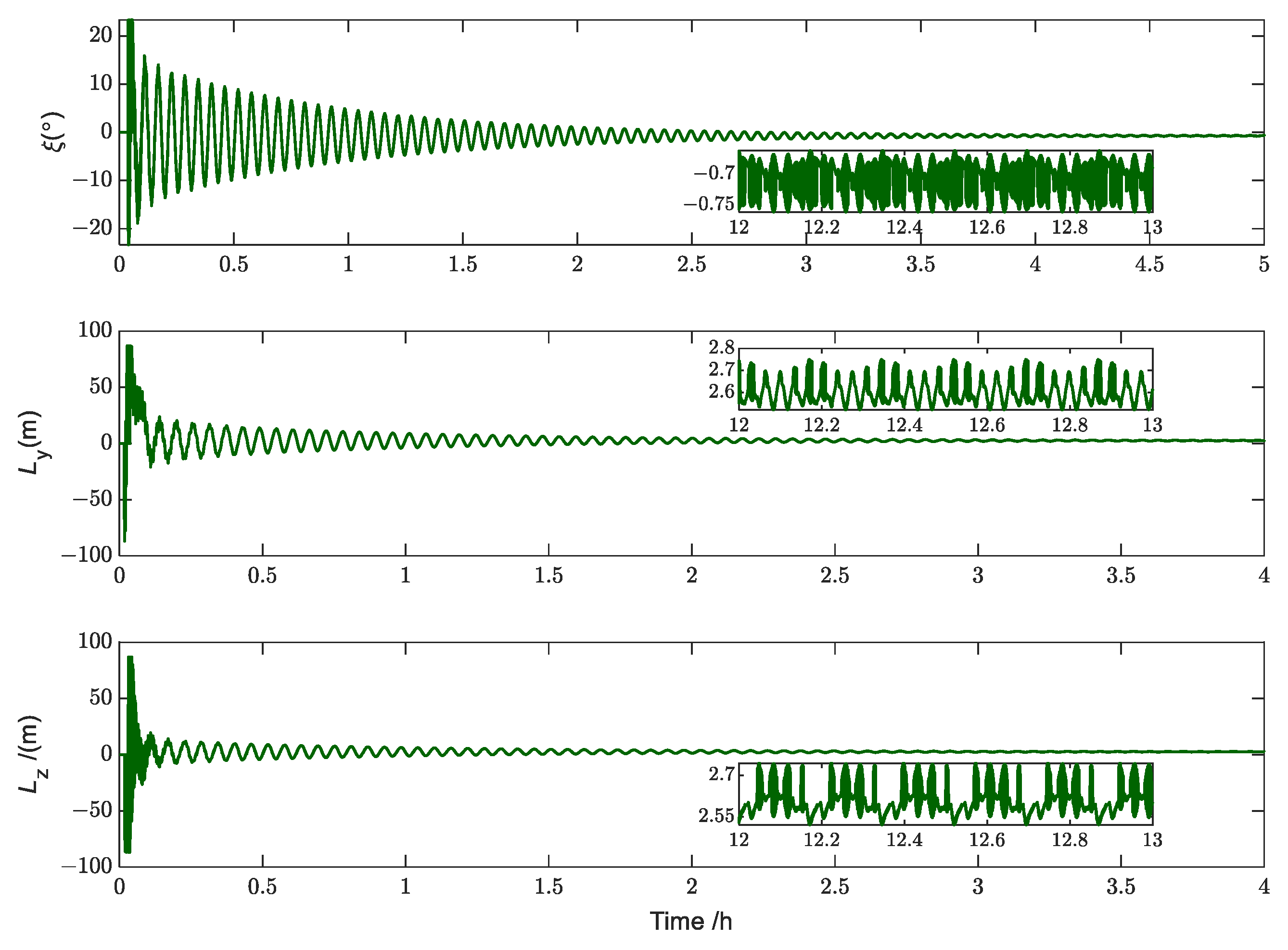


- Case 3: Comparison simulation with a controller designed from a linear sliding surface.
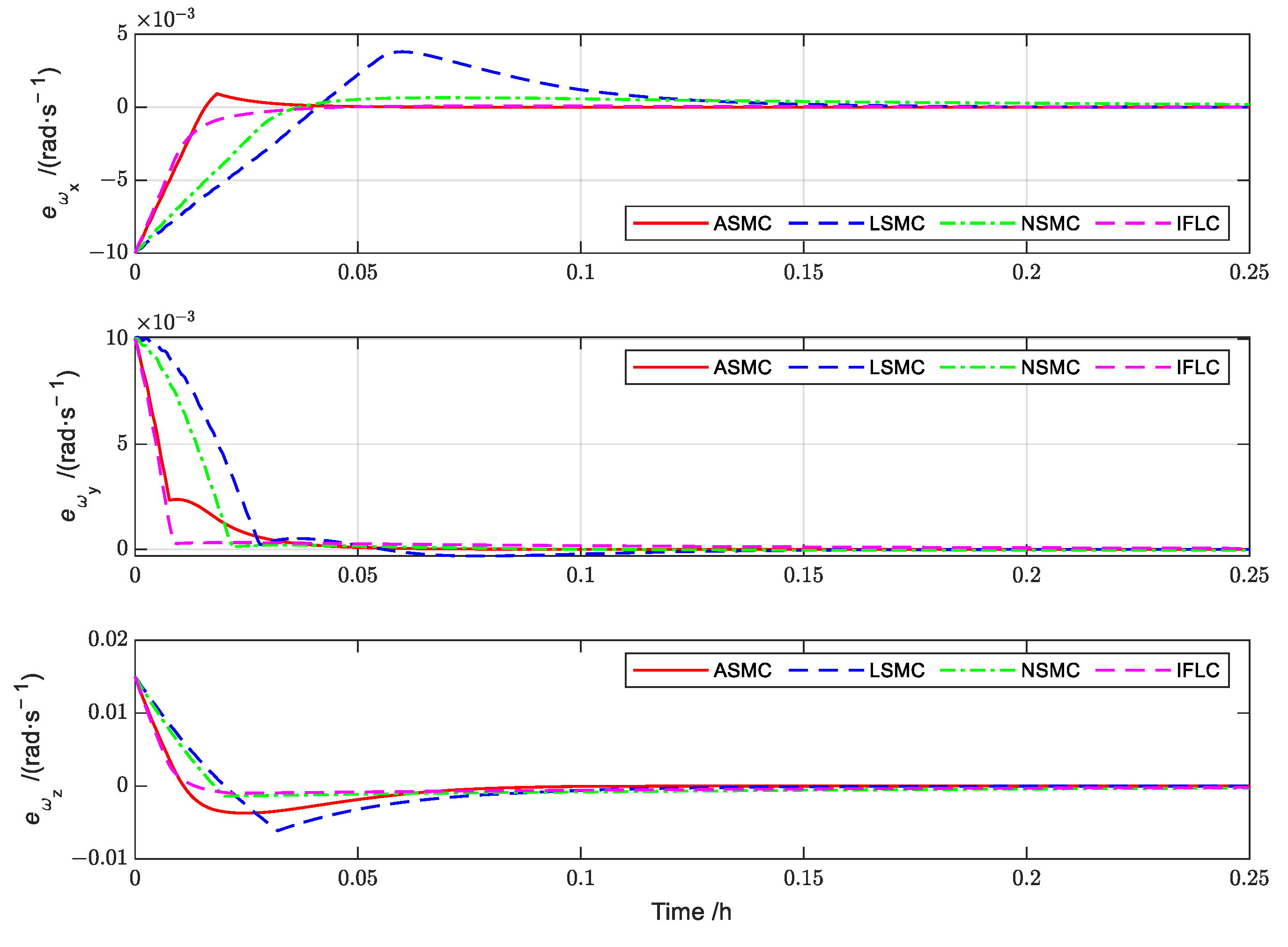
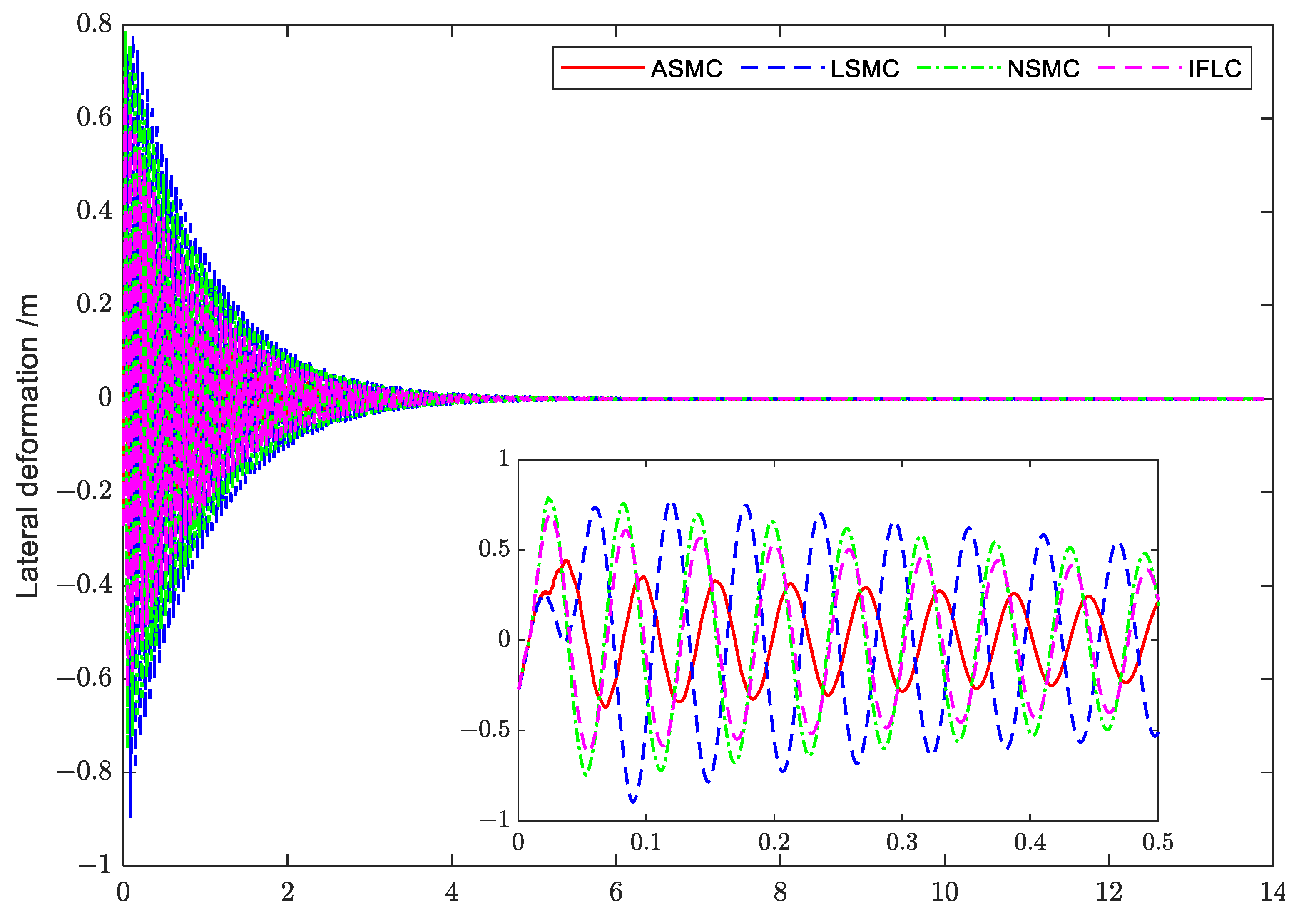
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Yang, J. Thinking on the Development of China’s Cause. Important Issues 2017, 12, 57264. [Google Scholar] [CrossRef]
- Yin, J.F.; Zhang, R.; Zhang, X.Y. Orbital Dynamic Characters and Orbital Maintenances of Pole-sitter Spacecraft. Spacecr. Eng. 2018, 27, 26–34. [Google Scholar]
- Driver, J.M. Analysis of an Arctic Polesitter. In Proceedings of the 17th Aerospace Sciences Meeting, New Orleans, LA, USA, 15–17 January 1979. [Google Scholar]
- Zhang, F.; Gong, S.P.; Gong, H.R.; Baoyin, H.X. Solar sail attitude control using shape variation of booms. Chin. J. Aeronaut. 2022, 35, 326–336. [Google Scholar] [CrossRef]
- Rong, S.Y.; Liu, J.F.; Cui, N.G. A review of solar sail spacecraft research and its key technology. Aerosp. Shanghai 2011, 28, 53–62. [Google Scholar]
- Gong, S.P.; Macdonald, M. Review on solar sail technology. Astrodynamics 2019, 3, 93–125. [Google Scholar] [CrossRef]
- Scholz, C.; Romagnoli, D.; Dachwald, B.; Theil, S. Performance analysis of an attitude control system for solar sails using sliding masses. Adv. Space Res. 2011, 48, 1822–1835. [Google Scholar] [CrossRef]
- Bolle, A.; Circi, C. Solar sail attitude control through in-plane moving masses. J. Aerospace Eng. 2008, 222, 81–94. [Google Scholar] [CrossRef]
- Romagnoli, D.; Oehlschlägel, T. High performance two degrees of freedom attitude control for solar sails. Adv. Space Res. 2011, 48, 1869–1879. [Google Scholar] [CrossRef]
- Baculi, J.; Ayoubi, M.A. Fuzzy attitude control of solar sail via linear matrix inequalities. Acta Astronaut. 2017, 138, 233–241. [Google Scholar] [CrossRef]
- Sperber, E.; Fu, B.; Ek, F.O. Large angle reorientation of a solar sail using gimballed mass control. J. Astronaut. Sci. 2016, 63, 103–123. [Google Scholar] [CrossRef]
- Gong, H.R.; Gong, S.P. Internal Torques Attitude Control for Solar Sail with Gimballed Mass. In Proceedings of the 8th International Conference on Vibration Engineering, Shanghai, China, 24 July 2021. [Google Scholar]
- Benjamin, L.D. Attitude Control and Dynamics of Solar Sails. Master’s Thesis, University of Washington, Washington, DC, USA, 2001. [Google Scholar]
- Wei, Y.H.; Zhu, M.; Peng, C.; Wang, Y. Attitude Control of Solar Sail Spacecraft with Saturation. In Proceedings of the 31st Chinese Control Conference, Heifei, China, 25–27 July 2012. [Google Scholar]
- Gong, H.R.; Gong, S.P.; Liu, D.L. Attitude dynamics and control of solar sail with multibody structure. Adv. Space Res. 2022, 69, 609–619. [Google Scholar] [CrossRef]
- Luo, T.; Yao, C.; Xu, M.; Qu, Q.Y. Attitude Dynamics and Control for a Solar Sail with Individually Controllable Elements. J. Guid. Control Dyn. 2019, 42, 1622–1629. [Google Scholar] [CrossRef]
- Wie, B.; Murphy, D. Solar-sail attitude control design for a flight validation mission. J. Spacecr. Rockets 2007, 44, 809–821. [Google Scholar] [CrossRef]
- Fu, B.; Eke, F.O. Attitude control methodology for large solar sails. J. Guid. Control Dyn. 2014, 38, 662–670. [Google Scholar] [CrossRef]
- Zhang, F.; Gong, S.P.; Baoyin, H.X. Three-Axes Attitude Control of Solar Sail Based on Shape Variation of Booms. Aerospace 2021, 8, 198. [Google Scholar] [CrossRef]
- Guerrant, D.; Lawrence, D. Tactics for Heliogyro Solar Sail Attitude Control via Blade Pitching. J. Guid. Control Dyn. 2015, 38, 1785–1799. [Google Scholar] [CrossRef]
- Liu, M.L.; Wang, Z.H.; Ikeuchi, D.; Fu, J.Y.; Wu, X.F. Design and Simulation of a Flexible Bending Actuator for Solar Sail Attitude Control. Aerospace 2021, 8, 372. [Google Scholar] [CrossRef]
- Chujo, T. Propellant-free attitude control of solar sails with variable-shape mechanisms. Acta Astronaut. 2022, 193, 182–196. [Google Scholar] [CrossRef]
- Hassanpour, S.; Damaren, C.J. Collocated attitude and vibrations control for square solar sails with tip vanes. Acta Astronaut. 2020, 166, 482–492. [Google Scholar] [CrossRef]
- Choi, M.; Damaren, C.J. Structural dynamics and attitude control of a solar sail using tip vanes. J. Spacecr. Rockets 2007, 52, 1665–1679. [Google Scholar] [CrossRef]
- Boni, L.; Bassetto, M.; Niccolai, L.; Mengali, G.; Quarta, A.A.; Circi, C.; Pellegrini, R.C.; Cavallini, E. Structural response of Helianthus solar sail during attitude maneuvers. Aerosp. Sci. Technol. 2023, 133, 108152. [Google Scholar] [CrossRef]
- Funase, R.; Shirasawa, Y.; Mimasu, Y.; Mori, O.; Tsuda, Y.; Saiki, T.; Kawaguchi, J. On-orbit verification of fuel-free attitude control system for spinning solar sail utilizing solar radiation pressure. Adv. Space Res. 2011, 48, 1740–1746. [Google Scholar] [CrossRef]
- Kikuchi, S.; Kawaguchi, J. Asteroid de-spin and deflection strategy using a solar-sail spacecraft with reflectivity control devices. Acta Astronaut. 2019, 156, 375–386. [Google Scholar] [CrossRef]
- Tsuda, Y.; Mori, O.; Funase, R.; Sawada, H.; Yamamoto, T.; Saiki, T.; Endo, T.; Yonekura, K.; Hoshino, H.; Kawaguchi, J. Achievement of IKAROS—Japanese deep space solar sail demonstration mission. Acta Astronaut. 2013, 82, 183–188. [Google Scholar] [CrossRef]
- Ullery, D.C.; Soleymani, S.; Heaton, A.; Orphee, J.; Johnson, L.; Sood, R.; Kung, P.; Kim, S.M. Strong Solar Radiation Forces from Anomalously Reflecting Metasurfaces for Solar Sail Attitude Control. Sci. Rep. 2018, 8, 10026. [Google Scholar] [CrossRef]
- Bassetto, M.; Niccolai, L.; Boni, L.; Mengali, G.; Quarta, A.A.; Circi, C.; Pizzurro, S.; Pizzarelli, M.; Pellegrini, R.; Cavallini, E. Sliding mode control for attitude maneuvers of Helianthus solar sail. Acta Astronaut. 2022, 198, 100–110. [Google Scholar] [CrossRef]
- Gong, S.P.; Li, J.F. Spin-stabilized solar sail for displaced solar orbits. Aerosp. Sci. Technol. 2014, 32, 188–199. [Google Scholar] [CrossRef]
- Gong, S.P.; Li, J.F. A new inclination cranking method for a flexible spinning solar sail. IEEE Trans. Aerosp. Electron. Syst. 2015, 51, 2680–2696. [Google Scholar] [CrossRef]
- Wang, S.B. Design and Optimization of Pole-Sitter Orbit for Hybrid Thruster Spacecraft. Master’s Thesis, Harbin Engineering University, Harbin, China, 2019. [Google Scholar]
- SpaceX Just Announced Their Hall Thruster Parameters, Actually Ar Propellant. Available online: https://m.163.com/dy/article/HUMMT1QL05119RIN.html (accessed on 22 April 2023).
- Coverstone, V.L.; Prussing, J.E. Technique for Escape from Geosynchronous Transfer Orbit Using a Solar Sail. J. Guid. Control Dyn. 2003, 26, 628–634. [Google Scholar] [CrossRef]
- Genta, G.; Brusa, E. The Parachute Sail with Hydrostatic Beam: A New Concept for Solar Sailing. Acta Astronaut. 1999, 44, 133–140. [Google Scholar] [CrossRef]
- Galhofo, D.; Silvestre, N.; de Deus, A.M.; Reis, L.; Duarte, A.P.C.; Carvalho, R. Structural behaviour of pre-tensioned solar sails. Thin Wall. Struct. 2022, 181, 110007. [Google Scholar] [CrossRef]
- Bianchi, C.; Niccolai, L.; Mengali, G.; Quarta, A.A. Collinear artificial equilibrium point maintenance with a wrinkled solar sail. Aerosp. Sci. Technol. 2021, 119, 107150. [Google Scholar] [CrossRef]
- Seefeldt, P.; Grundmann, J.T.; Hillebrandt, M.; Zander, M. Performance analysis and mission applications of a new solar sail concept based on crossed booms with tip-deployed membranes. Adv. Space Res. 2020, 67, 2736–2745. [Google Scholar] [CrossRef]
- Let the Sun Drum up Its Sails. Available online: https://www.docin.com/p-8729284.html (accessed on 22 April 2023).
- Chen, Y.C. Research on Displaced Orbit Keeping and Flexible Attitude Control for Solar Sail Spacecraft. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2020. [Google Scholar]
- Zhang, J.R. Research on Attitude-Vibration Coupled Modeling and Fault-Tolerant Control of Flexible Solar Sail Spacecraft. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2019. [Google Scholar]
- Zhang, T.F.; Chang, H.C.; Wu, Y.P.; Xiao, P.S.; Yi, N.B.; Lu, Y.H.; Ma, Y.F.; Huang, Y.; Zhao, K.; Yan, X.Q.; et al. Macroscopic and direct light propulsion of bulk graphene material. Nat. Photonics 2015, 9, 471–476. [Google Scholar] [CrossRef]
- Wu, L.P. Attitude and Orbit Control Studies for Solar Sail Spacecraft. Ph.D. Thesis, Nanjing University of Science & Technology, Nanjing, China, 2021. [Google Scholar]
- Chen, L.; Fu, X.Y.; Ramil, S.; Xu, M. Intelligent Fuzzy Control in Stabilizing Solar Sail with Individually Controllable Elements. Space Sci. Technol. 2022, 2022, 9831270. [Google Scholar] [CrossRef]
- He, T.F.; Wu, Z. Neural network disturbance observer with extended weight matrix for spacecraft disturbance attenuation. Aerosp. Sci. Technol. 2022, 126, 107572. [Google Scholar] [CrossRef]
- Liu, J.K. RBF Neural Network Control for Mechanical Systems: Design, Analysis and Matlab Simulation, 2nd ed.; Tsinghua University Press: Beijing, China, 2018; pp. 1–3. [Google Scholar]
- Zuo, Z.Y. Nonsingular fixed-time consensus tracking for second-order multi-agent networks. Automatica 2015, 54, 305–309. [Google Scholar] [CrossRef]
- Cao, L.; Xiao, B.; Golestani, M.; Rn, D.C. Faster Fixed-Time Control of Flexible Spacecraft Attitude Stabilization. IEEE Trans. Industr. Inform. 2020, 16, 1281–1290. [Google Scholar] [CrossRef]
- Yu, S.H.; Yu, X.H.; Shirinzadeh, B.; Man, Z.H. Continuous finite-time control for robotic manipulators with terminal sliding mode. Automatica 2005, 41, 1957–1964. [Google Scholar] [CrossRef]




| Parameters | Value |
|---|---|
| Length of the boom/m | 150 |
| Slider mass/kg | 5 |
| Moveable range of slider/m | ±100 |
| Maximum area of a single vane/ | 800 |
| Extendable range of a single vane/m | ±50 |
| Density of SRP/() | |
| Rotational inertia of -axis/ | 382,549.8 |
| Rotational inertia of -axis or -axis/ | 192,201.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, T.; Mu, R. Attitude Tracking Adaptive Control of a Geocentric Polar Displaced Solar Sail. Aerospace 2023, 10, 606. https://doi.org/10.3390/aerospace10070606
Zhang T, Mu R. Attitude Tracking Adaptive Control of a Geocentric Polar Displaced Solar Sail. Aerospace. 2023; 10(7):606. https://doi.org/10.3390/aerospace10070606
Chicago/Turabian StyleZhang, Tengfei, and Rongjun Mu. 2023. "Attitude Tracking Adaptive Control of a Geocentric Polar Displaced Solar Sail" Aerospace 10, no. 7: 606. https://doi.org/10.3390/aerospace10070606
APA StyleZhang, T., & Mu, R. (2023). Attitude Tracking Adaptive Control of a Geocentric Polar Displaced Solar Sail. Aerospace, 10(7), 606. https://doi.org/10.3390/aerospace10070606







