Design Investigation of Potential Long-Range Hydrogen Combustion Blended Wing Body Aircraft with Future Technologies
Abstract
1. Introduction
2. Summary of Future Airframe and Propulsion Technologies
2.1. Load Alleviation
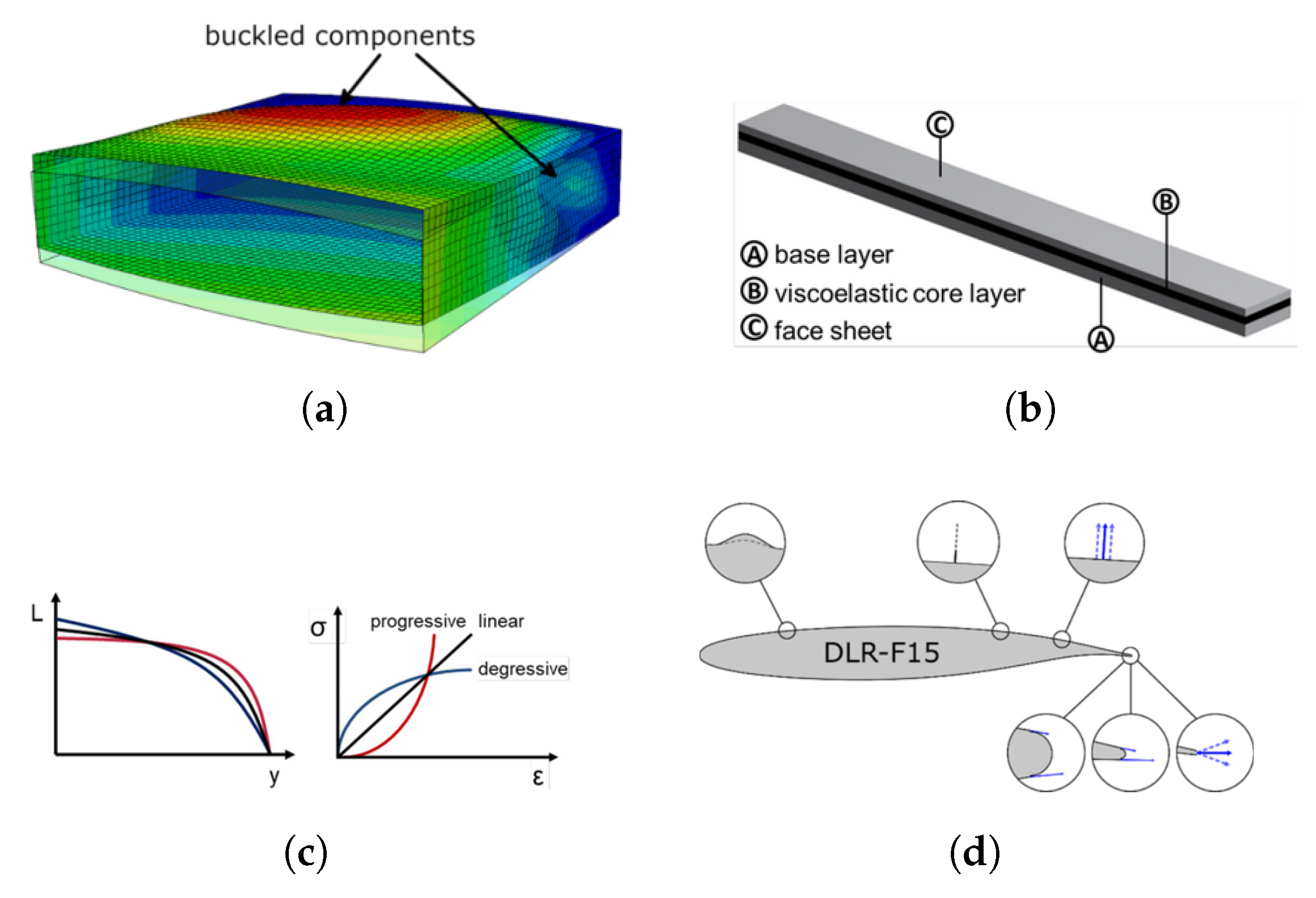
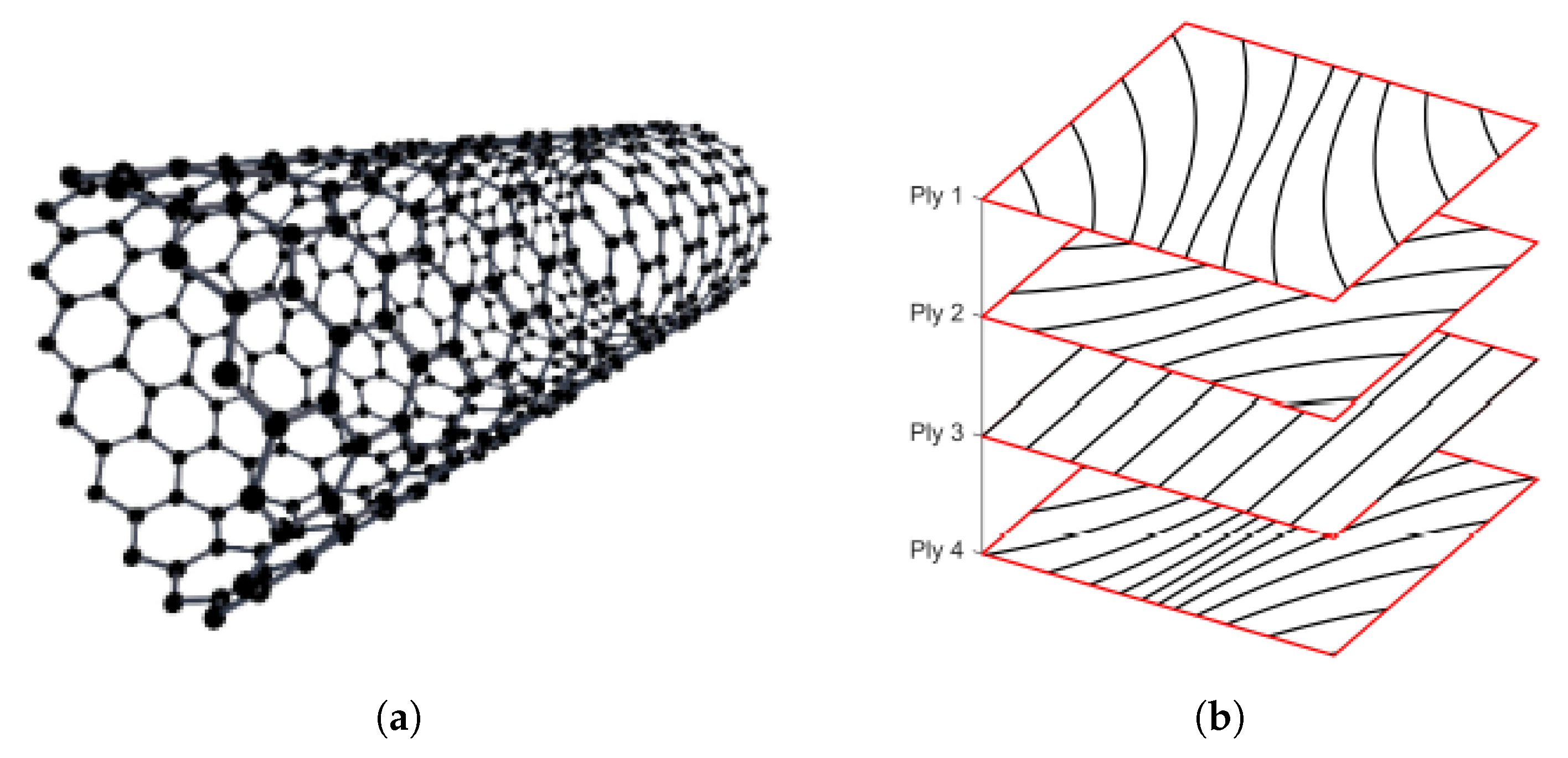
2.2. Advanced Materials
2.3. Ultra-High Bypass Ratio Turbofan Engines
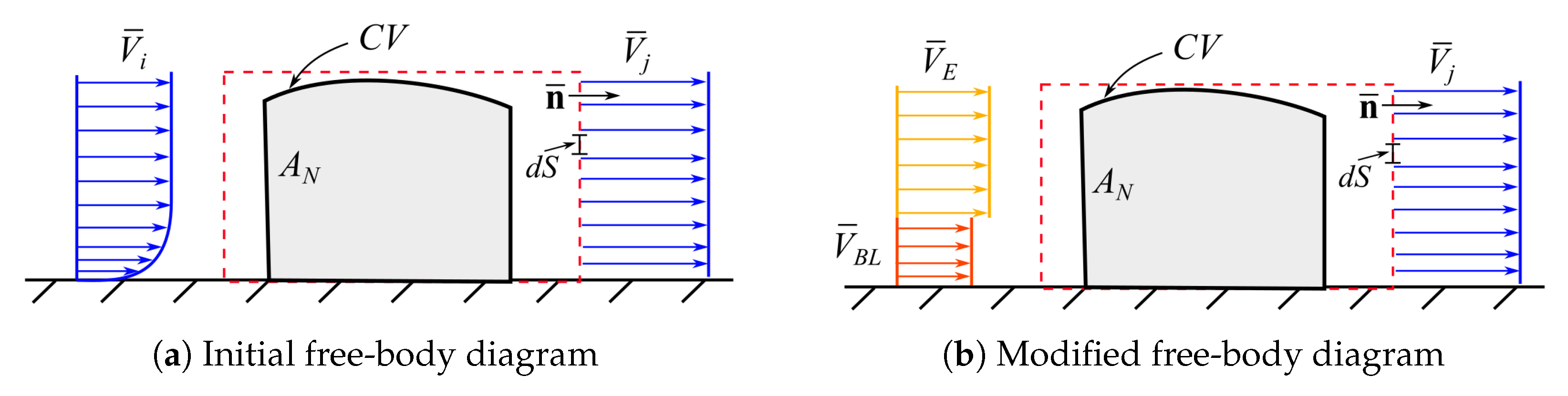
2.4. Boundary Layer Ingestion
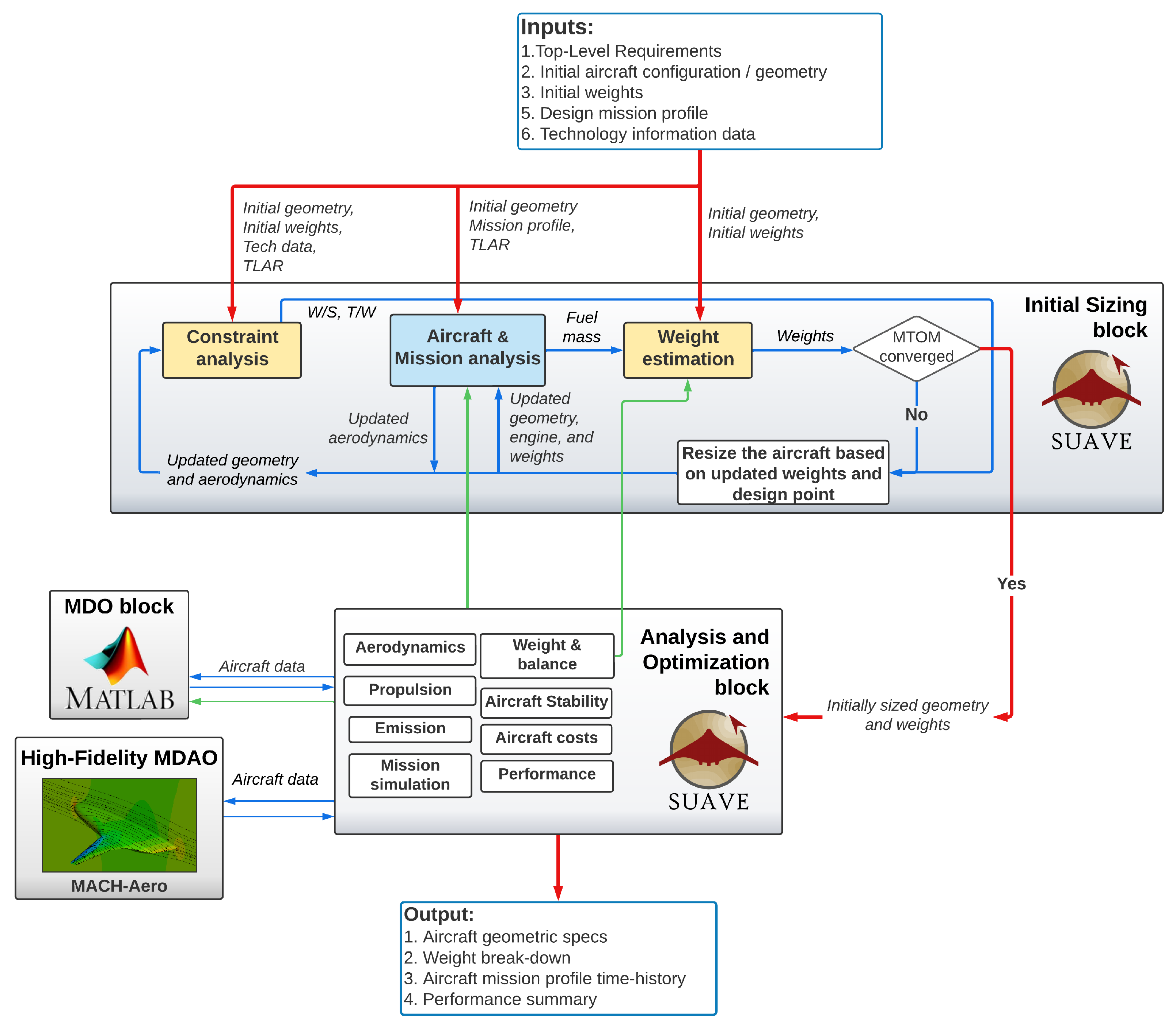
3. Initial Sizing Methodology
3.1. Constraint Analysis Module
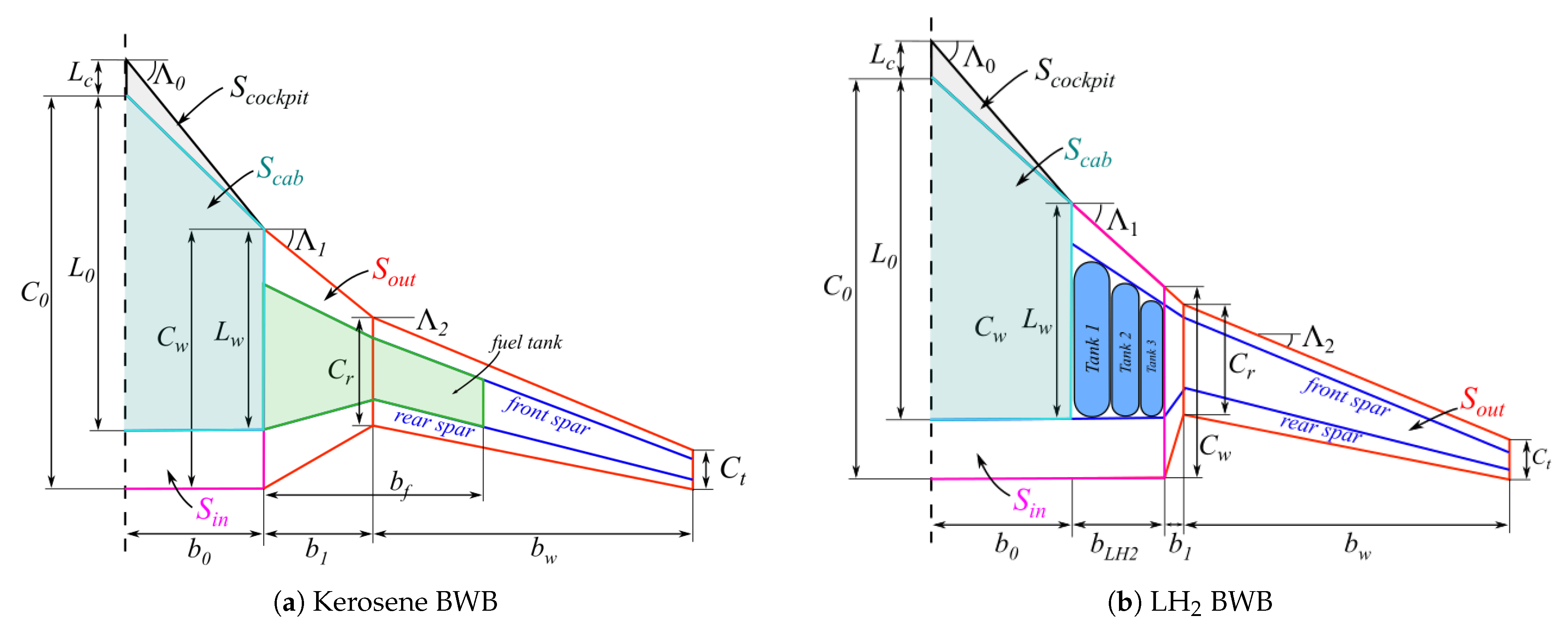
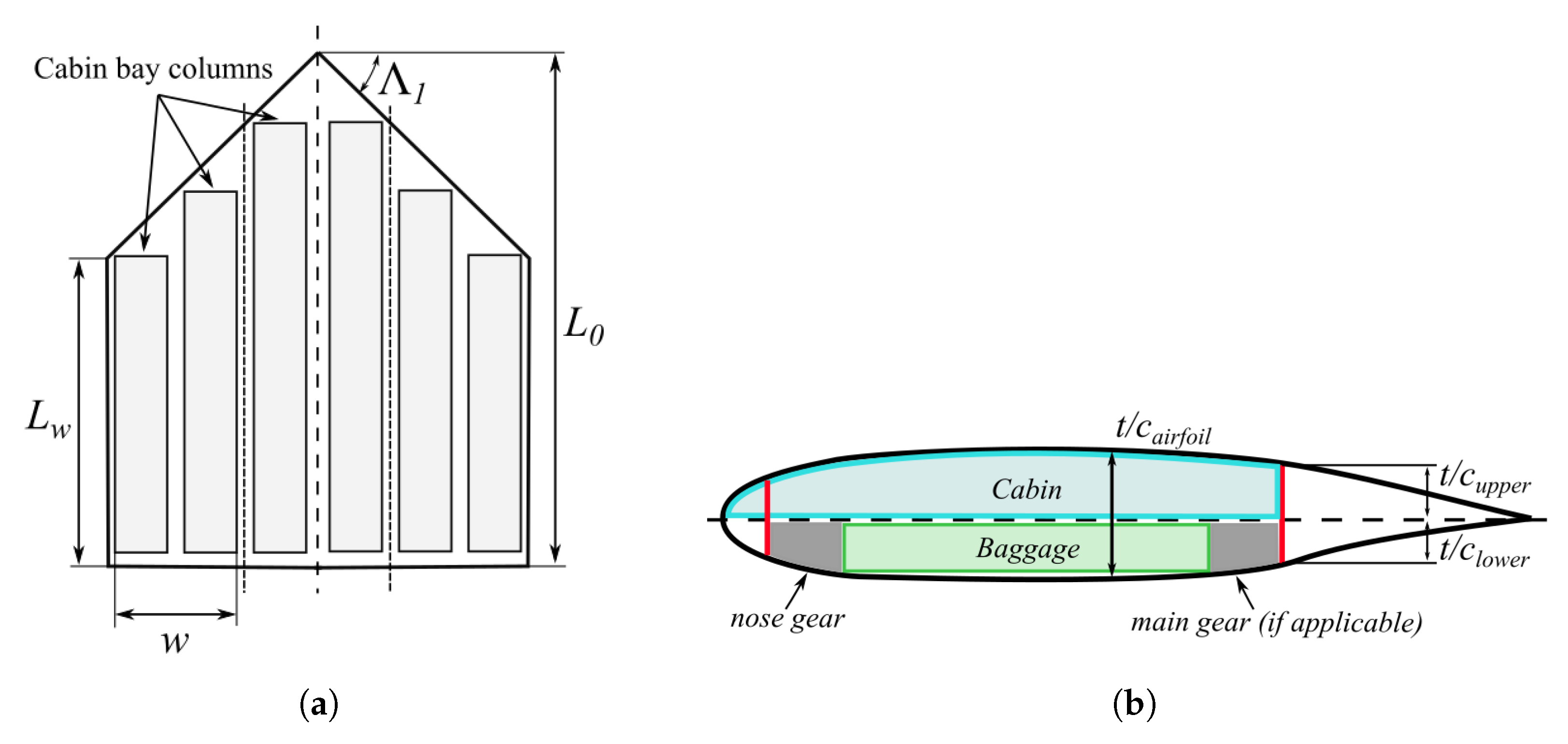
- The inner wing responsible for the cabin part and the transition portion of the outer wing segment have a similar leading edge sweep
- The inner wing trailing edge sweep angle was assumed to be equal to zero to simplify the sizing based on the aircraft cabin dimensions.
- The rear bulkhead of the cabin segment is parallel to the segment trailing edge.
3.2. Constraint Analysis
3.2.1. Aerodynamic Analysis
3.2.2. Weight and Balance Estimation
3.2.3. Propulsion
3.2.4. Aircraft Stability Analysis
3.2.5. Costs Analysis
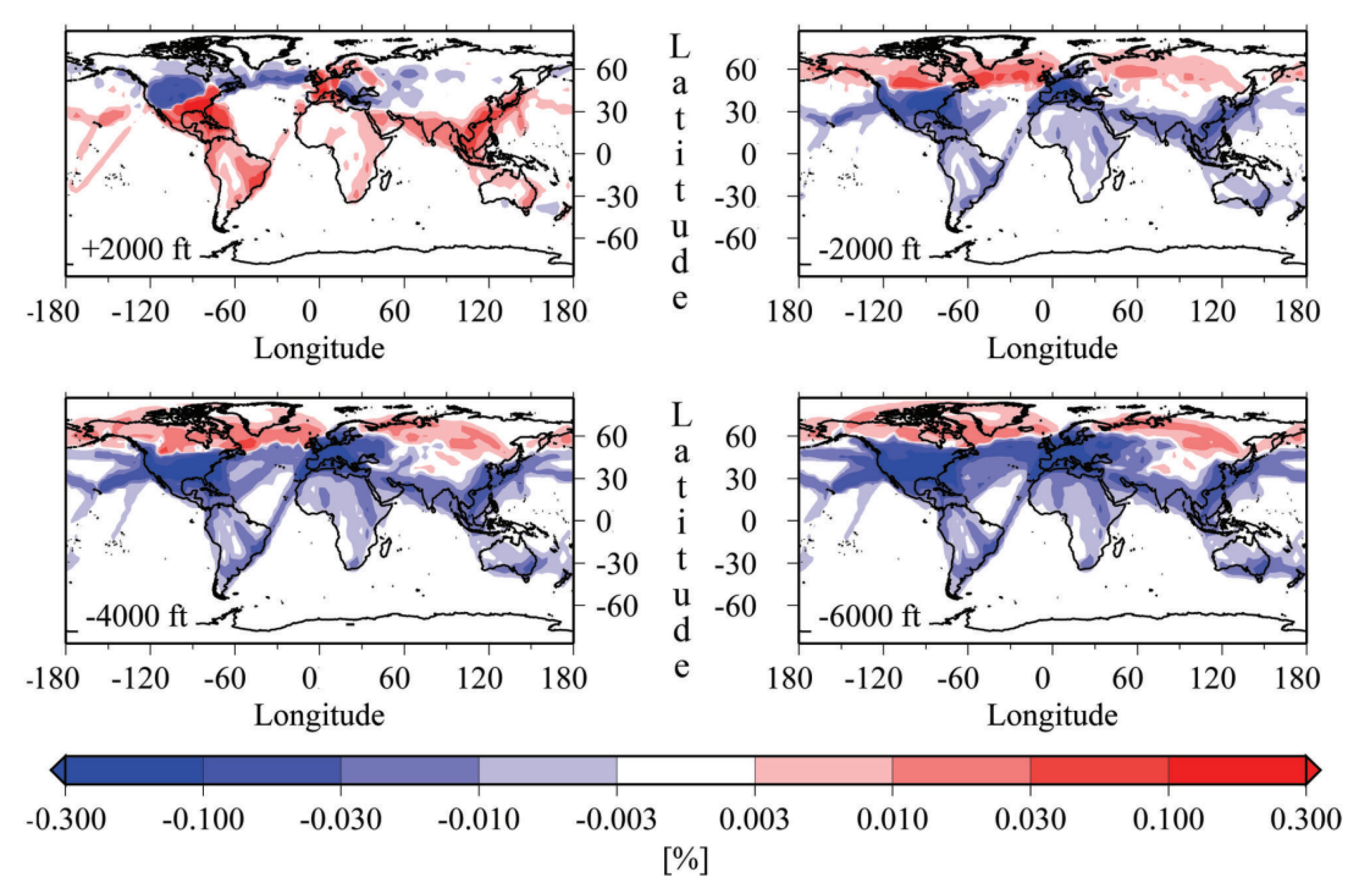
3.2.6. Emission Analysis
3.3. Analysis and Optimization Block
3.3.1. Low-Fidelity MDO Framework
3.3.2. High-Fidelity Aerodynamic Analysis
3.4. Technology Implementation
4. Comparative Study of Kerosene and LH2 BWB Aircraft
4.1. Top-Level Requirements
4.2. Design Studies
4.2.1. Concept Definition
4.2.2. Aircraft Sizing and Optimization
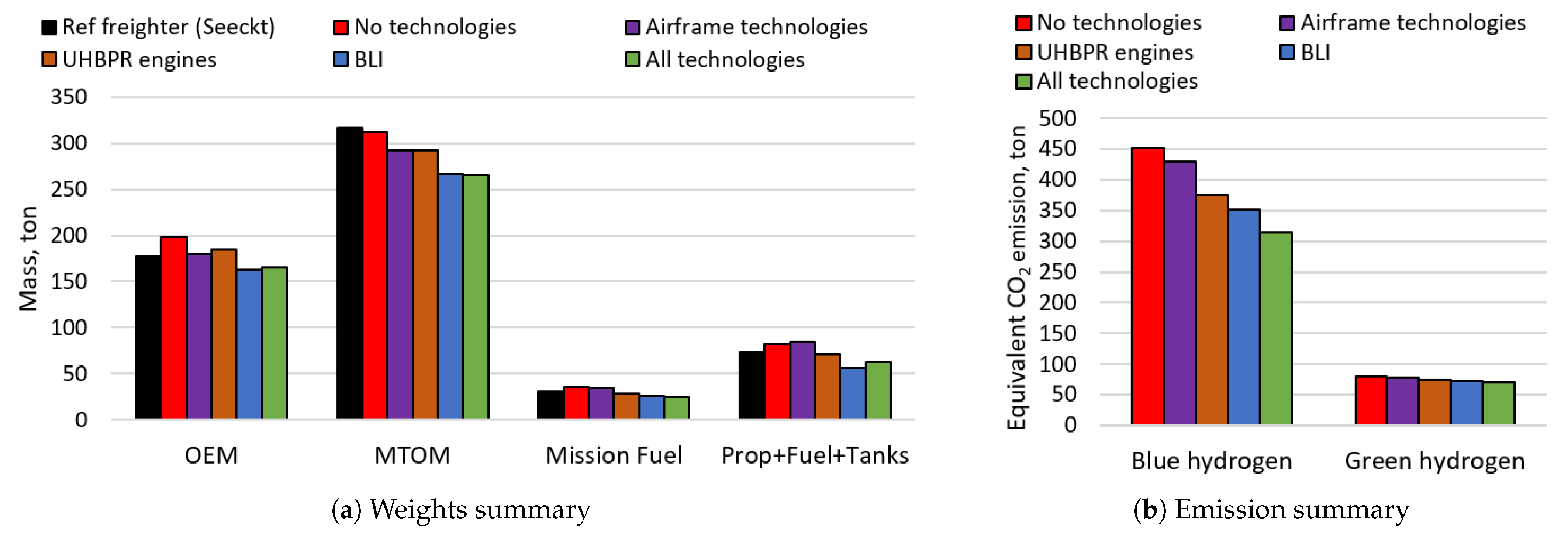
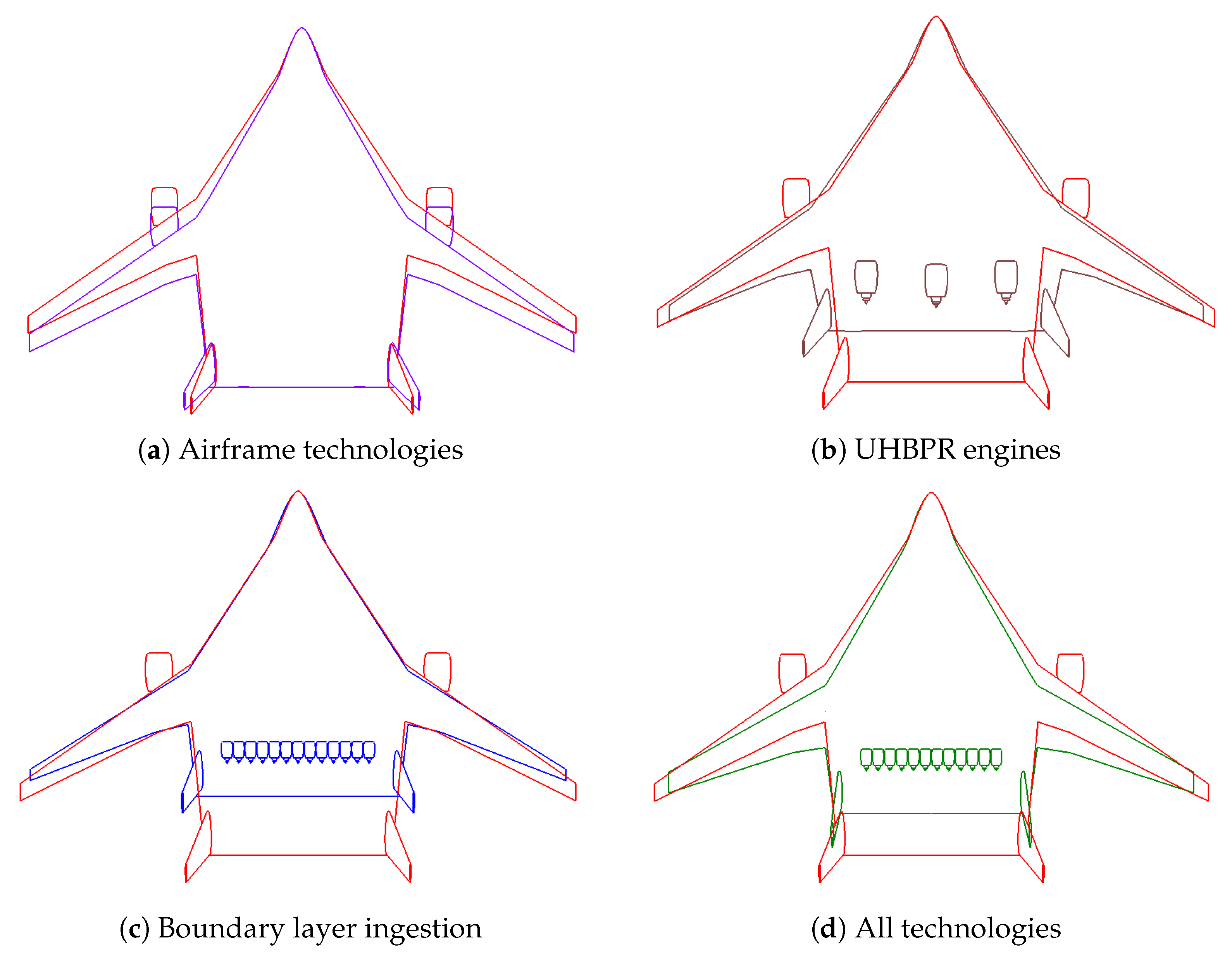
4.2.3. Technology Sensitivity of Hydrogen BWB Aircraft
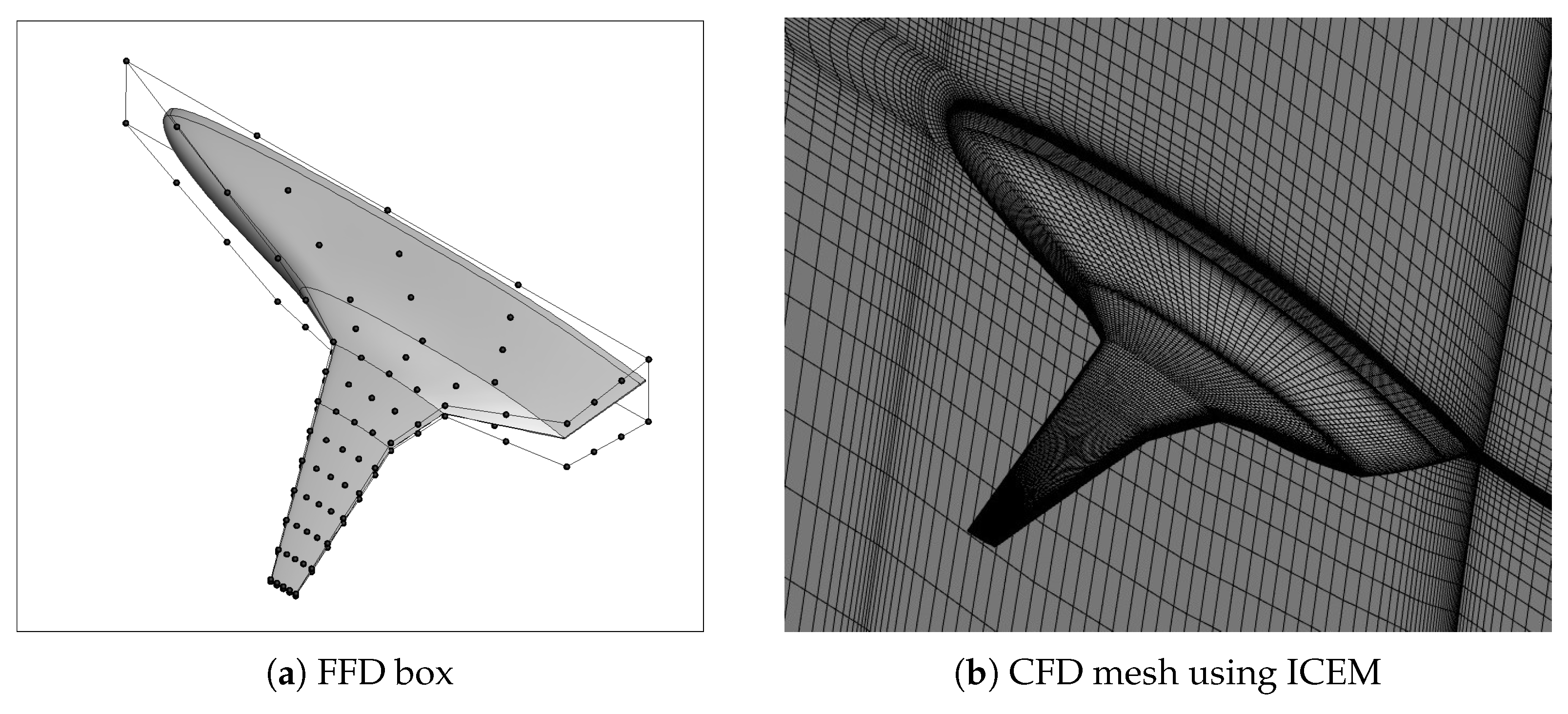
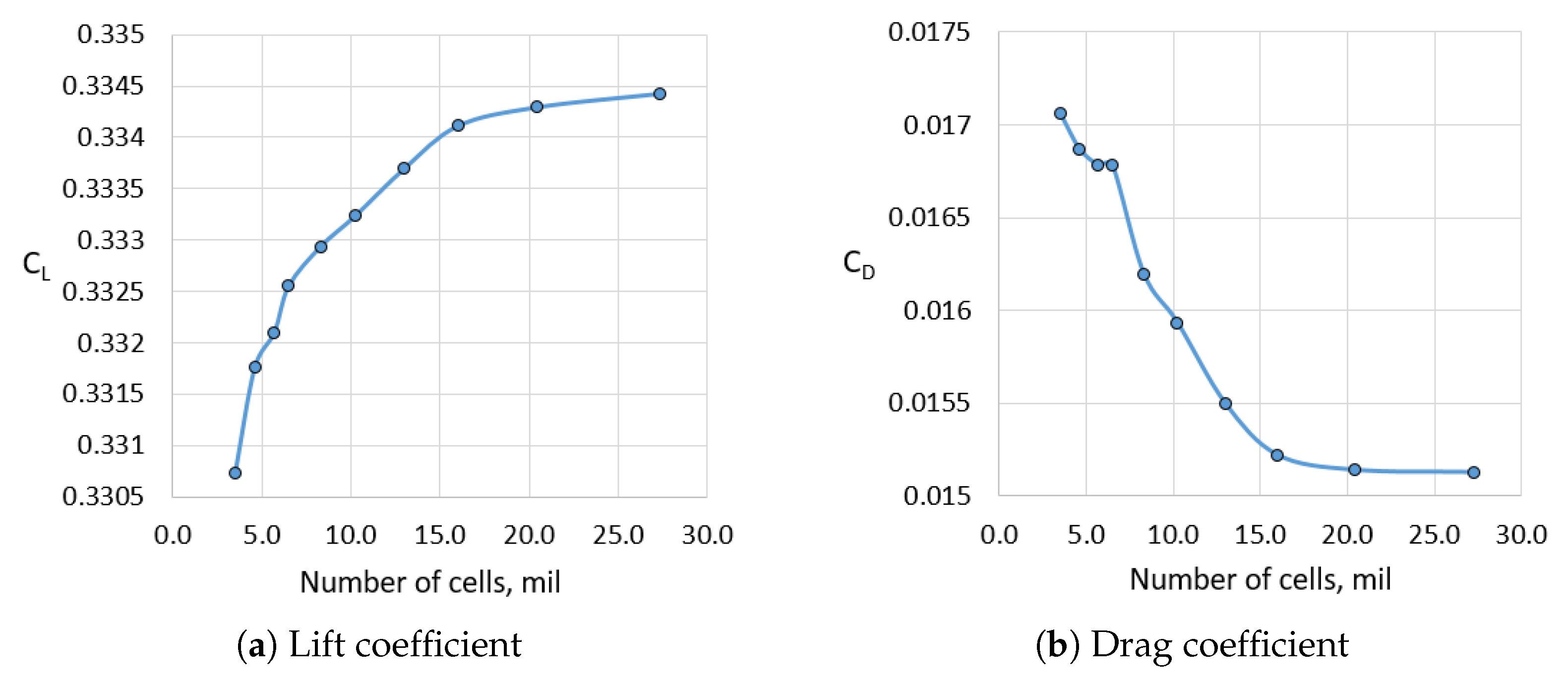
4.3. Verification of Aerodynamics Using High-Fidelity CFD
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- European Commission, Directorate-General for Research and Innovation. Fly the Green Deal—Europe’s Vision for Sustainable Aviation; Advisory Council for Aviation Research and Innovation in Europe (ACARE): Brussels, Belgium, 2022; Available online: https://perma.cc/8JJN-LEKV (accessed on 29 May 2023).
- Liebeck, R. Design of the Blended Wing Body Subsonic Transport. J. Aircr. 2004, 41, 1. [Google Scholar] [CrossRef]
- Chen, Z.; Zhang, M.; Chen, Y.; Sang, W.; Tan, Z.; Li, D.; Zhang, B. Assessment on Critical Technologies for Conceptual Design of Blended-wing-body Civil Aircraft. Chin. J. Aeronaut. 2019, 32, 8. [Google Scholar] [CrossRef]
- Okonkwo, P.; Smith, H. Review of Evolving Trends in Blended Wing Body Aircraft Design. Prog. Aerosp. Sci. 2016, 82. [Google Scholar] [CrossRef]
- Brown, M.; Vos, R. Conceptual Design and Evaluation of Blended-Wing Body Aircraft. In Proceedings of the 2018 AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 8 January 2018. [Google Scholar] [CrossRef]
- van Dommelen, J.; Vos, R. Conceptual Design and Analysis of Blended-wing-body Aircraft. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2014, 228, 13. [Google Scholar] [CrossRef]
- Dorsey, A.; Uranga, A. Design Space Exploration of Blended Wing Bodies. In Proceedings of the AIAA Aviation 2021 Forum, Virtual event, 2–6 August 2021. [Google Scholar] [CrossRef]
- Scholz, D. A Student Project of a Blended Wing Body Aircraft—From Conceptual Design to Flight Testing. In Proceedings of the European Workshop on Aircraft Design Education 2007, Samara, Russia, 29 May–2 June 2007; Available online: http://hdl.handle.net/20.500.12738/12617 (accessed on 14 June 2023).
- Bradley, K. A Sizing Methodology for the Conceptual Design of Blended-Wing-Body Transports; NASA/CR-2004-213016; NASA Langley: Hampton, VA, USA, 2004. Available online: https://ntrs.nasa.gov/citations/20040110949 (accessed on 29 May 2023).
- Hansen, L.; Heinze, W.; Horst, P. Blended wing body structures in multidisciplinary pre-design. Struct. Multidiscip. Optim. 2008, 36, 93–106. [Google Scholar] [CrossRef]
- Sgueglia, A. Methodology for Sizing and Optimising a Blended Wing-Body with Distributed Electric Ducted Fans. Ph.D. Dissertation, ISAE-SUPAERO, Toulouse, France, 2019. Available online: https://oatao.univ-toulouse.fr/25515/ (accessed on 29 May 2023).
- Gray, J.; Hwang, J.; Martins, J.; Moore, K.; Naylor, B. OpenMDAO: An Open-Source Framework for Multidisciplinary Design, Analysis, and Optimization. Struct. Multidiscip. Optim. 2019, 59, 4. [Google Scholar] [CrossRef]
- Seeckt, K.; Heinze, W.; Scholz, D. Hydrogen Powered Freighter Aircraft – The Final Results of the Green Freighter Project. In Proceedings of the 27th International Congress of the Aeronautical Sciences, Nice, France, 19–24 September 2010; Available online: https://perma.cc/XD44-M9KY (accessed on 29 May 2023).
- Guynn, M.; Freeh, J.; Olson, E. Evaluation of a Hydrogen Fuel Cell Powered Blended-Wing-Body Aircraft Concept for Reduced Noise and Emissions; NASA/TM-2004-212989; NASA Langley: Hampton, VA, USA, 2004. Available online: https://ntrs.nasa.gov/citations/20040033924 (accessed on 29 May 2023).
- Westenberger, A. H2 Technology for Commercial Aircraft. In Proceedings of the Advances on Propulsion Technology for High-Speed Aircraft, RTO, Rhode-Saint-Geneese, Belgium, 12–15 March 2007; pp. 216–258. Available online: https://perma.cc/M2V6-62ZJ (accessed on 3 June 2023).
- Bramsiepe, K.; Klimmek, T.; Krüger, W.; Tichy, L. Aeroelastic Method to Investigate Nonlinear Elastic Wing Structures. CEAS Aeronaut. J. 2022, 13, 939–949. [Google Scholar] [CrossRef]
- Dähne, S.; Hühne, C. Gradient Based Structural Optimization of a Stringer Stiffened Composite Wing Box with Variable Stringer Orientation. In World Congress of Structural and Multidisciplinary Optimisation; WCSMO 2017; Springer: Cham, Switzerland, 2017. [Google Scholar] [CrossRef]
- Wunderlich, F.; Dähne, S.; Reimer, L.; Schuster, A. Global Aero-Structural Design Optimization of More Flexible Wings for Commercial Aircraft. In Proceedings of the AIAA Aviation 2020 Forum, Virtual event, 15–19 June 2020. [Google Scholar] [CrossRef]
- Hahn, D.; Haupt, M. Exploration of the effect of wing component post-buckling on bending-twist coupling for nonlinear wing twist. CEAS Aeronaut. J. 2022, 13, 663–676. [Google Scholar] [CrossRef]
- Horst, P.; Elham, A.; Radespiel, R. Reduction of Aircraft Drag, Loads and Mass for Energy Transition in Aeronautics; Deutsche Gesellschaft für Luft-und Raumfahrt: Bonn, Germany, 2021. [Google Scholar] [CrossRef]
- Liu, X.; Sun, Q. Gust Load Alleviation with Robust Control for a Flexible Wing. Shock Vib. 2016, 2016, 1060574. [Google Scholar] [CrossRef]
- Ying, B.; Changchum, X. Gust Load Alleviation Wind Tunnel Tests of a Large-aspect-ratio Flexible Wing with Piezoelectric Control. J. Aeronaut. 2017, 30, 292–309. [Google Scholar] [CrossRef]
- Beyer, Y.; Kuzolap, A.; Steen, M.; Diekmann, H.; Fezans, N. Adaptive Nonlinear Flight Control of STOL-Aircraft Based on Incremental Nonlinear Dynamic Inversion. In Proceedings of the 2018 AIAA Aviation Forum, Atlanta, GA, USA, 25–29 June 2018. [Google Scholar] [CrossRef]
- Ehlers, J.; Fezans, N. Airborne Doppler LiDAR Sensor Parameter Analysis for Wake Vortex Impact Alleviation Purposes. In Proceedings of the 2015 CEAS Conference on Guidance, Navigation, and Control, Toulouse, France, 13–15 April 2015. [Google Scholar] [CrossRef]
- Khalil, K.; Asaro, S.; Bauknecht, A. Active Flow Control Devices for Wing Load Alleviation. J. Aircr. 2022, 59, 2. [Google Scholar] [CrossRef]
- Yu, M.; Lourie, O.; Dyer, M.; Moloni, K.; Kelly, T.; Ruoff, R. Strength and Breaking Mechanism of Multiwalled Carbon Nanotubes Under Tensile Load. Science 2000, 287, 5453. [Google Scholar] [CrossRef]
- Peng, B.; Locascio, M.; Zapol, P.; Li, S.; Mielke, S.; Schatz, G.; Espinosa, H. Measurements of Near-ultimate Strength for Multiwalled Carbon Nanotubes and Irradiation-induced Crosslinking Improvements. Nat. Nanotechnol. 2008, 3, 626–631. [Google Scholar] [CrossRef] [PubMed]
- Brooks, T.; Martins, J. On Manufacturing Constraints for Tow-steered Composite Design Optimization. Compos. Struct. 2018, 204, 548–559. [Google Scholar] [CrossRef]
- Brooks, T.; Kennedy, G.; Martins, J. High-fidelity Multipoint Aerostructural Optimization of a High Aspect Ratio Tow-steered Composite Wing. In Proceedings of the 58th AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Grapevine, TX, USA, 9–13 January 2017. [Google Scholar] [CrossRef]
- Stanford, B.; Jutte, C. Aeroelastic Tailoring via Tow Steered Composites; NASA/TM–2014-218517; NASA: Langley, VA, USA, 2010. Available online: https://core.ac.uk/works/24799315 (accessed on 4 April 2023).
- Wang, X.; Tang, F.; Cao, Q.; Qi, X.; Pearson, M.; Li, M.; Pan, H.; Zhang, Z.; Lin, Z. Comparative Study of Three Carbon Additives: Carbon Nanotubes, Graphene, and Fullerene-C60, for Synthesizing Enhanced Polymer Nanocomposites. Nanomaterials 2020, 10, 838. [Google Scholar] [CrossRef]
- Carvalho, J.; Sohouli, A.; Suleman, A. Fundamental Frequency Optimization of Variable Angle Tow Laminates with Embedded Gap Defects. J. Compos. Sci. 2022, 6, 64. [Google Scholar] [CrossRef]
- Greitzer, E.; Bonnefoy, P.; delaRosaBlanco, E.; Dorbian, C.; Drela, M.; Hall, D.; Hansman, R.; Hileman, J.; Liebeck, R.; Lovegren, J.; et al. N+3 Aircraft Concept Designs and Trade Studies, Volume 1; NASA/CR—2010-216794/VOL1; NASA: Cleveland, OH, USA, 2010. Available online: https://core.ac.uk/display/10557299 (accessed on 4 April 2023).
- Dagget, D.; Brown, S.; Kawai, R. Ultra-Efficient Engine Diameter Study; NASA/CR-2003-212309; NASA: Seattle, WA, USA, 2003. Available online: https://ntrs.nasa.gov/citations/20030061085 (accessed on 29 May 2023).
- Bijewitz, J.; Seitz, A.; Hornung, M. Architectural Comparison of Advanced Ultra-High Bypass Ratio Turbofans for Medium to Long Range Application. In Proceedings of the Deutscher Luft-und Raumfahrtkongress 2014, Augsburg, Germany, 16–18 September 2014; Available online: https://perma.cc/YDF6-A66D (accessed on 29 May 2023).
- Giescke, D.; Lehmler, M.; Friedrichs, J.; Blinstrub, J.; Bertsch, L.; Heinze, W. Evaluation of Ultra-high Bypass Ratio Engines for an Over-wing Aircraft Configuration. J. Glob. Power Propuls. Soc. 2018, 2, 493–515. [Google Scholar] [CrossRef]
- Gray, J.; Mader, C.; Kenway, G.; Martins, J. Modeling Boundary Layer Ingestion Using a Coupled Aeropropulsive Analysis. J. Aircr. 2018, 55, 3. [Google Scholar] [CrossRef]
- Seitz, A.; Habermann, A.L.; Peter, F.; Troeltsch, F.; Castillo Pardo, A.; Della Corte, B.; van Sluis, M.; Goraj, Z.; Kowalski, M.; Zhao, X.; et al. Proof of Concept Study for Fuselage Boundary Layer Ingesting Propulsion. Aerospace 2021, 8, 16. [Google Scholar] [CrossRef]
- Voigt, J.; Friedrichs, J. Development of a Multi-Segment Parallel Compressor Model for a Boundary Layer Ingesting Fuselage Fan Stage. Energies 2021, 14, 5746. [Google Scholar] [CrossRef]
- Budziszewski, N.; Friedrichs, J. Modelling of A Boundary Layer Ingesting Propulsor. Energies 2018, 11, 708. [Google Scholar] [CrossRef]
- Uranga, A.; Drela, M.; Greitzer, E.; Hall, D.; Tichener, N.; Lieu, M.; Siu, N.; Casses, C.; Huang, A. Boundary Layer Ingestion Benefit of the D8 Transport Aircraft. AIAA J. 2017, 55, 11. [Google Scholar] [CrossRef]
- Blumenthal, A.; Elmiligui, A.; Geiselhart, K.; Campbell, K.; Maughmer, M.; Schmitz, S. Computational Investigation of a Boundary Layer Ingestion Propulsion System for the Common Research Model. In Proceedings of the 6th AIAA Fluid Dynamics Conference, Washington, DC, USA, 13–17 June 2016. [Google Scholar] [CrossRef]
- Kawai, R.; Friedman, D.; Serrano, L. Blended Wing Body (BWB) Boundary Layer Ingestion (BLI) Inlet Configuration and System Studies; NASA/CR-2006-214534; NASA: Huntington Beach, CA, USA, 2006. Available online: https://core.ac.uk/works/15254411 (accessed on 4 April 2023).
- Karpuk, S.; Elham, A. Influence of Novel Airframe Technologies on the Feasibility of Fully-Electric Regional Aviation. Aerospace 2021, 8, 163. [Google Scholar] [CrossRef]
- Karpuk, S.; Radespiel, R.; Elham, A. Assessment of Future Airframe and Propulsion Technologies on Sustainability of Next-Generation Mid-Range Aircraft. Aerospace 2022, 9, 279. [Google Scholar] [CrossRef]
- Lukaczyk, T.; Wendroff, A.; Colonno, M.; Economon, T.; Alonso, J.; Orra, T.; Ilario, C. SUAVE: An Open-Source Environment for Multi-Fidelity Conceptual Vehicle Design. In Proceedings of the 16th AIAA ISSMO Multidisciplinary Analysis and Optimization Conference, Dallas, TX, USA, 22–26 June 2015. [Google Scholar] [CrossRef]
- Gudmundsson, S. General Aviation Aircraft Design: Applied Methods and Procedures, 1st ed.; Butterworth-Heinemann: Oxford, UK, 2013. [Google Scholar] [CrossRef]
- Loftin, L. Subsonic Aircraft: Evolution and the Matching of Size to Performance; NASA RP 1060; NASA Langley: Hampton, VA, USA, 1980. Available online: https://ntrs.nasa.gov/citations/19800020744 (accessed on 4 April 2023).
- Torenbeek, E. Synthesis of Subsonic Airplane Design; Springer: Delft, The Netherlands, 1982. [Google Scholar] [CrossRef]
- Howe, D. Aircraft Conceptual Design Synthesis; Professional Engineering Publishing Limited: London, UK, 2000. [Google Scholar]
- Drela, M.; Youngren, H. Athena Vortex-Lattice Method. Available online: https://perma.cc/RWM5-NFK9 (accessed on 4 April 2023).
- Nita, M.; Scholz, D. Estimating the Oswald Factor from Basic Aircraft Geometrical Parameters. In Proceedings of the Deutscher Luft-und Raumfahrtkongress 2012, Berlin, Germany, 10–12 September 2012; Available online: https://nbn-resolving.org/urn:nbn:de:101:1-201212176728 (accessed on 4 April 2023).
- Shevell, R.; Bayan, F. Development of a Method for Predicting the Drag Divergence Mach Number and the Drag Due to Compressibility for Conventional and Supercritical Wings; Research Report SUDAAR 522; Stanford University: Stanford, CA, USA, 1980; Available online: https://perma.cc/XN5X-EUZN (accessed on 28 April 2022).
- Wells, D.; Horvath, B.; McCullers, L. The Flight Optimization System Weights Estimation Method; NASA TM 20170005851; NASA: Hampton, VA, USA, 2017. Available online: https://core.ac.uk/display/84913944 (accessed on 28 April 2022).
- Brewer, D. Hydrogen Aircraft Technology; CRC Press: Boca Raton, FL, USA, 1991. [Google Scholar]
- Verstraete, D. The Potential of Liquid Hydrogen for Long Range Aircraft Propulsion. Ph.D. Dissertation, Cranfield University, Cranfield, UK, 2009. Available online: https://dspace.lib.cranfield.ac.uk/handle/1826/4089 (accessed on 3 April 2023).
- Winnefeld, C.; Kadyk, T.; Bensmann, B.; Krewer, U.; Hanke-Rauschenbach, R. Modelling and Designing Cryogenic Hydrogen Tanks for Future Aircraft Applications. Energies 2018, 11, 105. [Google Scholar] [CrossRef]
- Lin, C.; Van Dresar, N.; Hasan, M. A Pressure Control Analysis of Cryogenic Storage Systems. In Proceedings of the 27th Joint Propulsion Conference, Sacramento, CA, USA, 24–26 June 1991. [Google Scholar] [CrossRef]
- Cantwell, B. Aircraft and Rocket Propulsion; Stanford University: Stanford, CA, USA, 2022. [Google Scholar]
- Bardenhagen, A.; Gobbin, A. Flugzeugentwurf II; TU Berlin: Berlin, Germany, 2017. [Google Scholar]
- Roskam, J. Airplane Design, 2nd ed.; Darcorporation: Lawrence, KS, USA, 2003; Volume 1–8. [Google Scholar]
- Hoelzen, J.; Silberhorn, D.; Zill, T.; Bensmann, B.; Hanke-Rauschenbach, R. Hydrogen-powered Aviation and its Reliance on Green Hydrogen Infrastructure – Review and Research Gaps. Int. J. Hydrogen Energy 2022, 47, 5. [Google Scholar] [CrossRef]
- Hydrogen Council. A Perspective on Hydrogen Investment, Market Development, and Cost Competitiveness; McKinsey & Co.: New York, NY, USA, 2021; Available online: https://perma.cc/4APJ-7ZMB (accessed on 29 May 2023).
- Scholz, D. Limits to Principles of Electric Flight; Deutscher Luft-und Raumfahrtkongress: Darmstadt, Germany, 2019. [Google Scholar] [CrossRef]
- Baughcum, S.; Tritz, T.; Henderson, S.; Pickett, D. Scheduled Civil Aircraft Emission Inventories for 1992: Database Development and Analysis; NASA Contractor Report 4700; Langley Research Center: Hampton, VA, USA, 1996. Available online: https://ntrs.nasa.gov/citations/19960038445 (accessed on 28 April 2022).
- Dallara, E. Aircraft Design for Reduced Climate Impact. Ph.D. Dissertation, Stanford University, Stanford, CA, USA, 2011. Available online: https://perma.cc/3TDH-TB2S (accessed on 28 April 2022).
- Scholz, D. Calculation of the Emission Characteristics of Aircraft Kerosene and Hydrogen Propulsion; Harvard Data-verse; HAW Hamburg: Hamburg, Germany, 2020. [Google Scholar] [CrossRef]
- Fuel Cells and Hydrogen 2 Joint Undertaking. In Hydrogen-Powered Aviation: A Fact-based Study of Hydrogen Technology, Economics, and Climate Impact by 2050; Publications Office of EU: Luxembourg, 2020. [CrossRef]
- Svensson, F.; Hasselrot, A.; Moldanova, J. Reduced environmental impact by lowered cruise altitude for liquid hydrogen-fuelled aircraft. Aerosp. Sci. Technol. 2004, 8, 4. [Google Scholar] [CrossRef]
- Marquart, S.; Suasen, R.; Ponater, M.; Grewe, V. Estimate of the Climate Impact of Cryoplanes. Aerosp. Sci. Technol. 2001, 5, 1. [Google Scholar] [CrossRef]
- Howarth, R.; Jacobson, M. How green is blue hydrogen? Energy Sci. Eng. 2021, 9, 10. [Google Scholar] [CrossRef]
- Pavlenko, N.; Searle, S. Assessing the Sustainability Implications of Alternative Aviation Fuels. In Proceedings of the International Council on Clean Transportation 2021; Available online: https://perma.cc/XPG7-UK79 (accessed on 28 April 2022).
- Andersson, J.; Krus, P. A Multi-Objective Optimization Approach to Aircraft Preliminary Design. In Proceedings of the SAE World Aviation Congress, Montreal, QC, Canada, 8–11 September 2003. [Google Scholar] [CrossRef]
- Karpuk, S.; Liu, Y.; Elham, A. Multi-Fidelity Design Optimization of a Long-Range Blended Wing Body Aircraft with New Airframe Technologies. Aerospace 2020, 7, 87. [Google Scholar] [CrossRef]
- Mader, C.; Kenway, G.; Yildirim, A.; Martins, J. ADflow—An open-source computational fluid dynamics solver for aerodynamic and multidisciplinary optimization. J. Aerosp. Inf. Syst. 2020, 17, 508–527. [Google Scholar] [CrossRef]
- Kenway, G.; Kennedy, G.; Martins, J. A CAD-free approach to high-fidelity aerostructural optimization. In Proceedings of the 13th AIAA/ISSMO Multidisciplinary Analysis Optimization Conference, AIAA 2010-9231, Fort Worth, TX, USA, 13–15 September 2010. [Google Scholar] [CrossRef]
- Secco, N.R.; Kenway, G.K.; He, P.; Mader, C.; Martins, J.R. Efficient Mesh Generation and Deformation for Aerodynamic Shape Optimization. AIAA J. 2021, 4, 1151–1168. [Google Scholar] [CrossRef]
- Gill, P.; Murray, W.; Saunders, M. SNOPT: An SQP algorithm for large-scale constrained optimization. SIAM J. Optim. 2002, 12, 4. [Google Scholar] [CrossRef]
- Silberhorn, D.; Atanasov, G.; Walther, J.; Zill, T. Assessment of Hydrogen Fuel Tank Integration at Aircraft Level. In Proceedings of the Deutscher Luft- und Raumfahrtkongress 2019, Darmstadt, Germany, 30 September–2 October 2019; Available online: https://core.ac.uk/download/pdf/237080603.pdf (accessed on 29 May 2023).
- Cengel, Y.; Cimbala, J. Fluid Mechanics Fundamentals and Applications, 3rd ed.; McGraw-Hill Education: Columbus, OH, USA, 2013. [Google Scholar]
- Merkl, E. Final Report Summary-ENOVAL (Engine Module Validators); European Commission: Amsterdam, The Netherlands, 2018; Available online: https://perma.cc/59JE-877J (accessed on 29 May 2023).
- Fichter, C.; Marquart, S.; Sausen, R.; Lee, D. The Impact of Cruise Altitude on Contrails and Related Radiative Forcing. Meteorol. Z. 2005, 14, 4. [Google Scholar] [CrossRef]
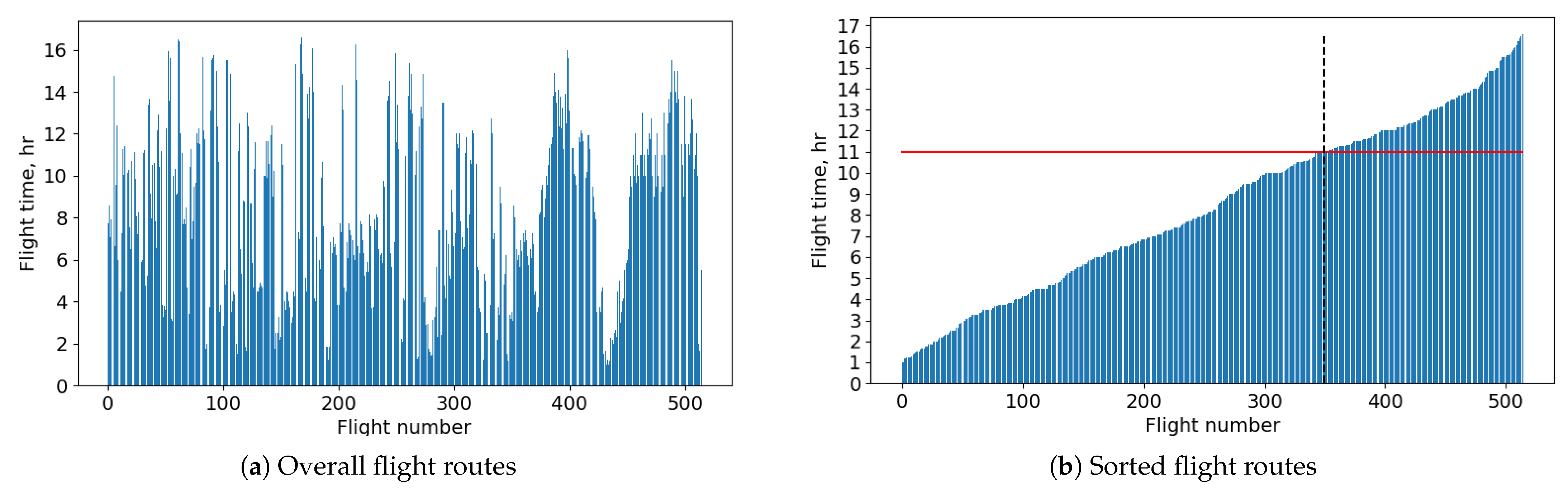
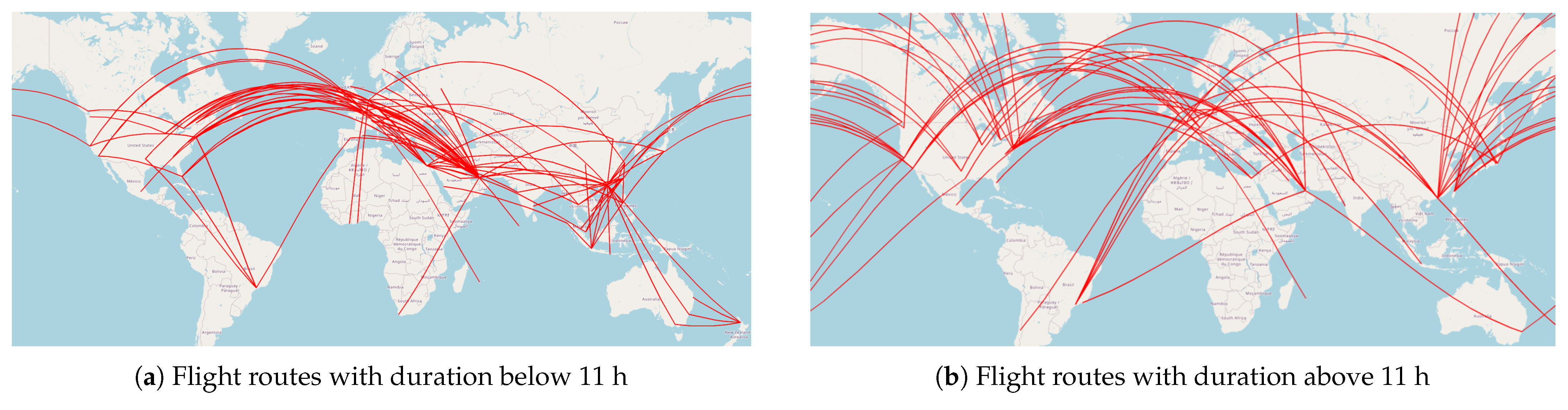
- Infinite Flight. Online Flight Routes Database. 2021. Available online: https://perma.cc/VGB8-6PV7 (accessed on 29 May 2023).
- Cook, A.; Tanner, G. European Airline Delay Cost Reference Values; University of Westminster: London, UK, 2015; p. 60. Available online: https://perma.cc/GFX2-8JSE (accessed on 29 May 2023).
- Dahlmann, K.; Koch, A.; Linke, F.; Lührs, B.; Grewe, V.; Otten, T.; Seider, D.; Gollnick, V.; Schumann, U. Climate-Compatible Air Transport System—Climate Impact Mitigation Potential for Actual and Future Aircraft. Aerospace 2016, 3, 38. [Google Scholar] [CrossRef]
- Certification Specifications and Acceptable Means of Compliance for Large Aeroplanes CS-25. June 2020. Available online: https://perma.cc/9K76-KJPW (accessed on 4 April 2023).
- Annex 14 to the Convention on International Civil Aviation, Aerodromes, Volume 1, 8th ed. July 2018. Available online: https://perma.cc/H853-AMWW (accessed on 4 April 2023).
- Scholz, D. Design of Hydrogen Passenger Aircraft—How much “Zero-Emission” is Possible? In Hamburg Aerospace Lecture Series (DGLR, RAeS, VDI, ZAL, HAW Hamburg); Zenodo: Hamburg, Germany, 2020. [Google Scholar] [CrossRef]
- Valdes, R.; Comendador, V.; Campos, L. How Much Can Carbon Taxes Contribute to Aviation Decarbonization by 2050. Sustainability 2021, 13, 1086. [Google Scholar] [CrossRef]

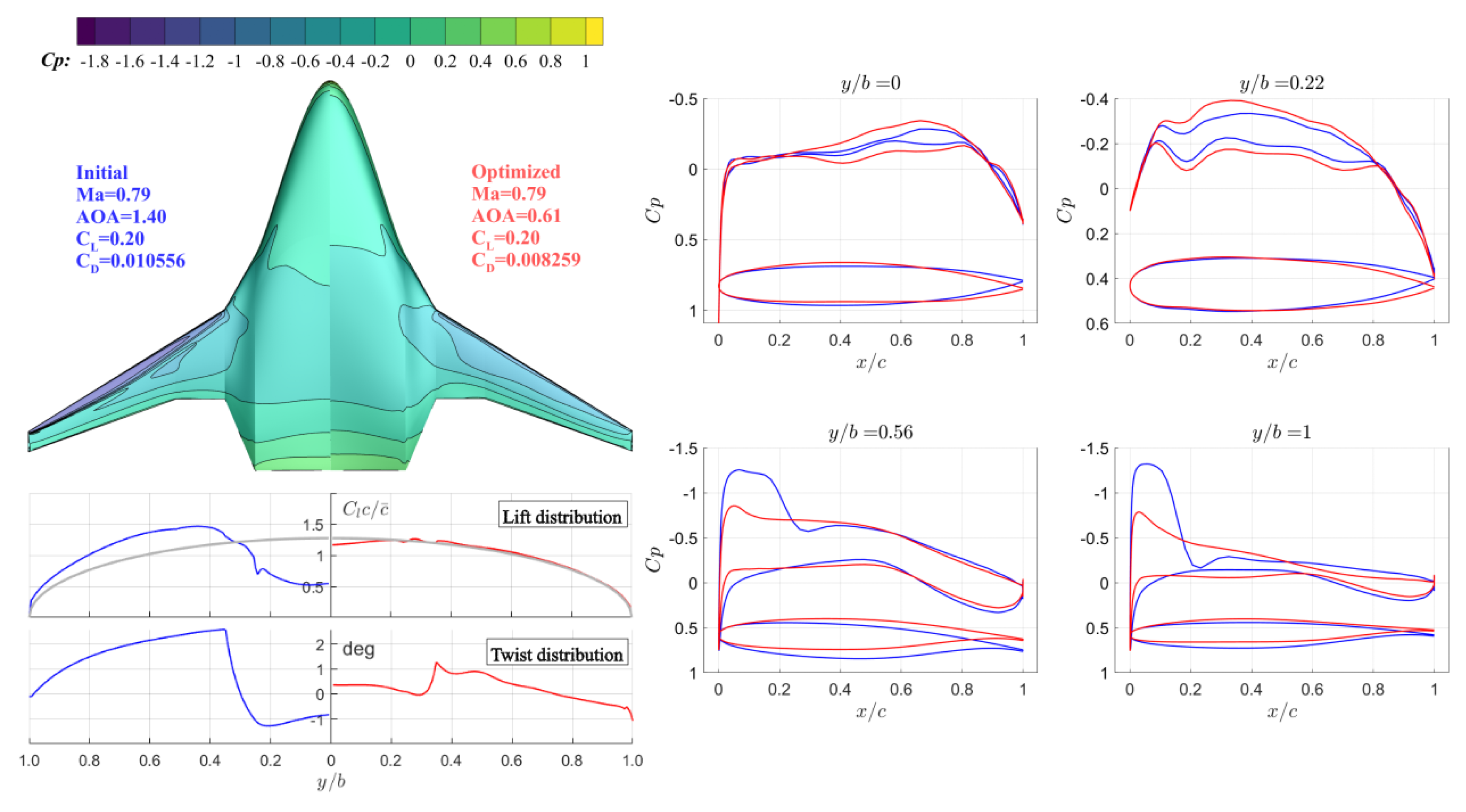
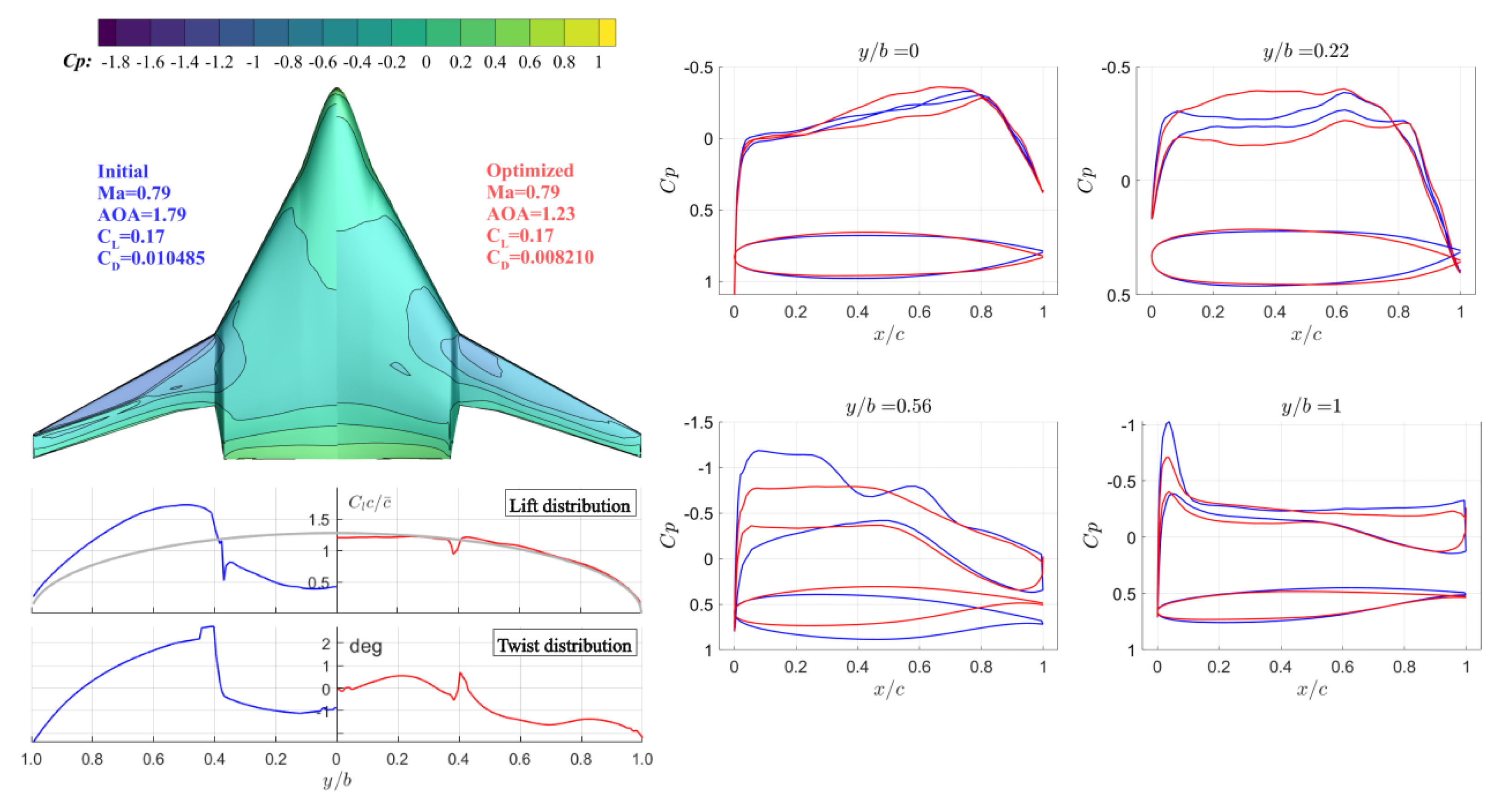

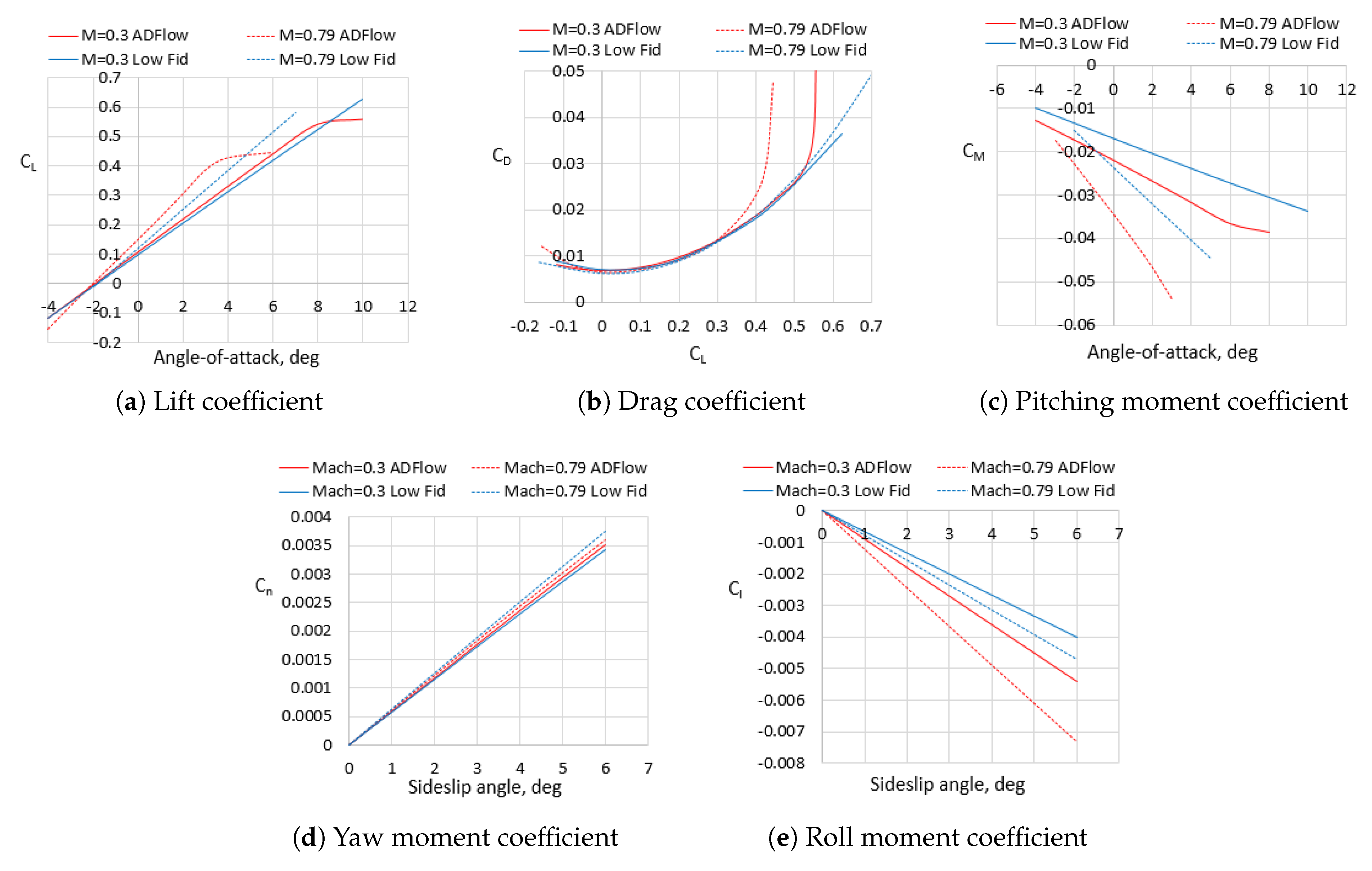

| Production Type | Scenario | Minimum | Maximum |
|---|---|---|---|
| Blue | Base | 1.0 | 2.0 |
| Green | Optimistic | 0.8 | 2.5 |
| Base | 1.8 | 3.8 | |
| Pessimistic | 2.6 | 6.5 |
| Scenario | Minimum | Maximum |
|---|---|---|
| Optimistic | 0.2 | 1.0 |
| Base | 0.8 | 2.0 |
| Pessimistic | 1.7 | 3.0 |
| Constant | Kerosene | Hydrogen | Correction Description |
|---|---|---|---|
| 1.0 | 0.0 | Accounts for the fuel type | |
| 1.0 | 2.79 | Accounts for higher hydrogen combustion temperature | |
| 1.0 | 0.1–0.75 | Lean combustion | |
| 1.0 | 2.58 | Higher H2O emission | |
| 1.0 | 0.27–0.36 | Thinner ice crystals and less visibility of contrails |
| Technology | Assumption | Reference |
|---|---|---|
| Load alleviation | Limit load factor 2.0 | Cluster goal |
| Advanced structures | 19% airframe weight reduction | [29,33] Cluster goal |
| Aircraft |
Rotations Per Day |
Flight Duration (min) |
Taxi Hours Per Day |
Ground Time at-Gate (min) |
Block Hours Per Day |
|---|---|---|---|---|---|
| B747-400 | 1.5 | 510 | 0.7 | 180 | 13.9 |
| Mean | Standard Deviation | Median | Absolute Minimum | Absolute Maximum | 1 Standard Deviation |
|---|---|---|---|---|---|
| 8.37 | 4.03 | 8.17 | 1.0 | 16.58 | 4.33–12.40 |
| Requirement | Value | Units |
|---|---|---|
| Harmonic range | 10,580 | km |
| Maximum payload | 70,000 | kg |
| Passengers (2-class seating) | 378 | |
| Cruise Mach number | 0.79 | |
| Maximum wingspan | 80.0 | |
| Maximum operating Mach number | ≥0.84 | |
| Design cruise altitude | 7650 | m |
| Service ceiling | ≥10,500 | m |
| Take-off field length | 3050 | m |
| Mission reserve | CS-25 [86] |
| Parameter | Lower | Upper | Units | |
|---|---|---|---|---|
| minimize | ||||
| wrt | 4.00 | 6.50 | ||
| 700.0 | 1550.0 | m2 | ||
| 0.25 | 0.4 | |||
| 45.0 | 65.0 | deg | ||
| 20.0 | 40.0 | deg | ||
| 0.0 | 2.0 | deg | ||
| 0.2 | 0.35 | |||
| 0.03 | 0.2 | |||
| 0.65 | 0.85 | |||
| 0.09 | 0.11 | |||
| 0.09 | 0.11 | |||
| 0.0 | 3.0 | deg | ||
| −3.0 | 0.0 | deg | ||
| 1.0 | 2.0 | |||
| 40.0 | 60.0 | deg | ||
| 0.0 | 50.0 | deg | ||
| 0.3 | 0.6 | |||
| 5.5 | 7.5 | m | ||
| 0.03 | 0.15 | |||
| 0.225 | 0.35 | N/N | ||
| subject to | Take-off field length | 3050 | m | |
| b | 80.0 | m | ||
| 2.0 | m | |||
| 0.025 | ||||
| −0.057 | 0.0 | |||
| 0.02 |
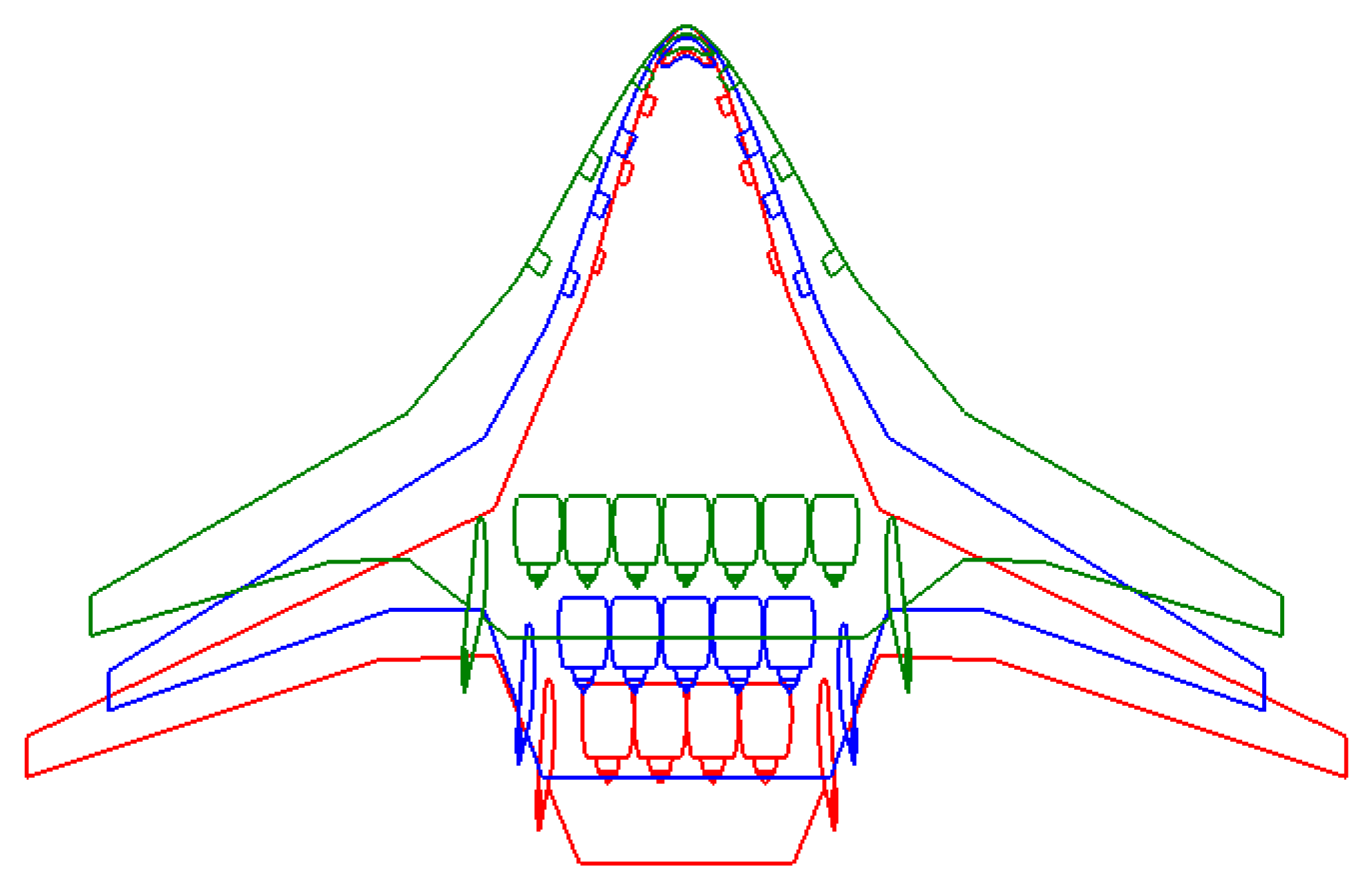
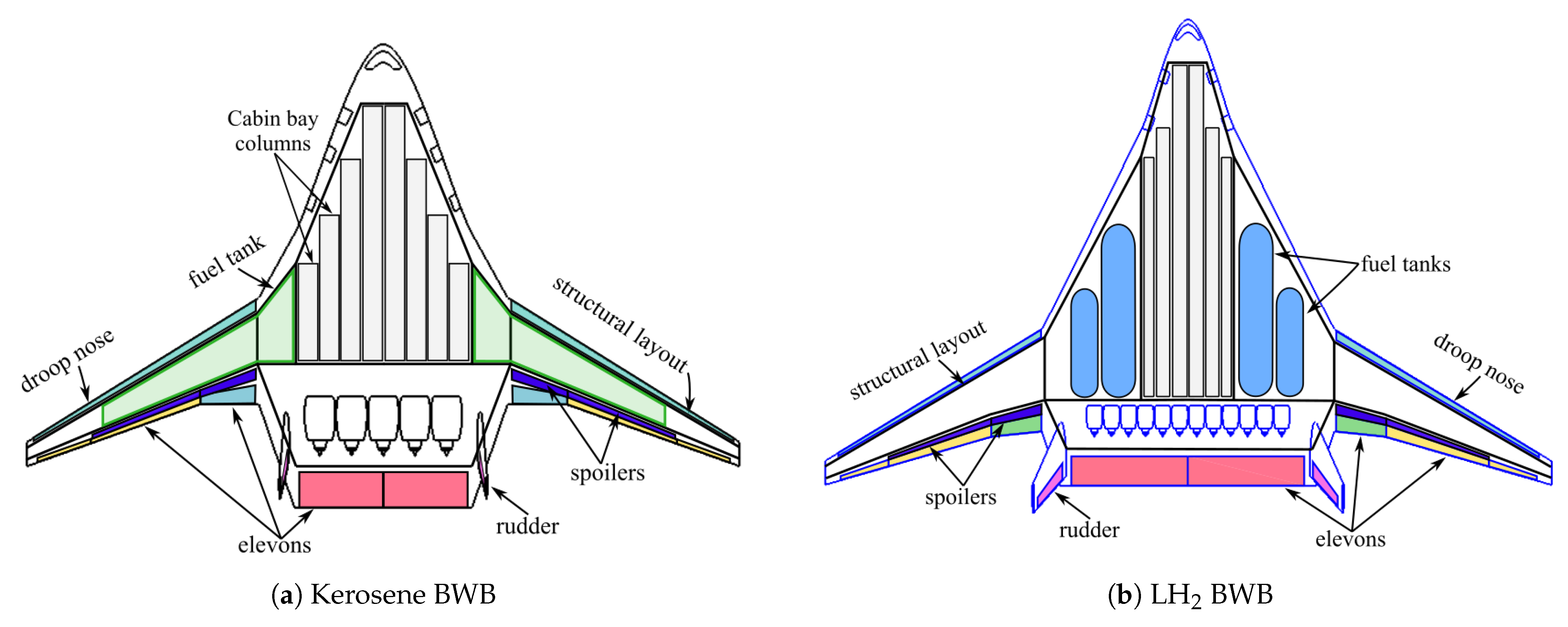
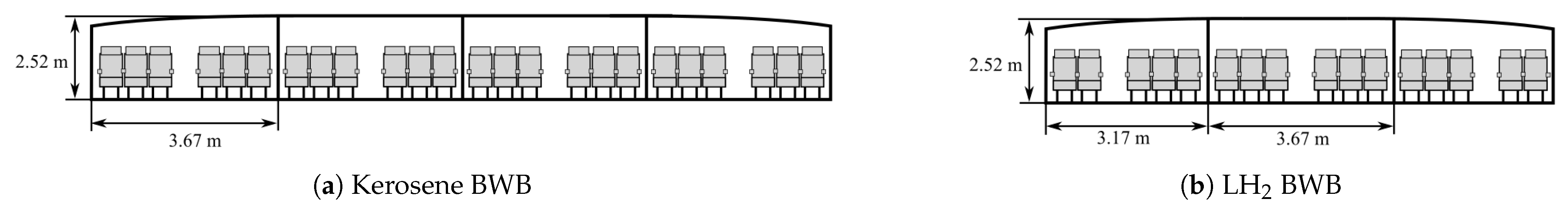
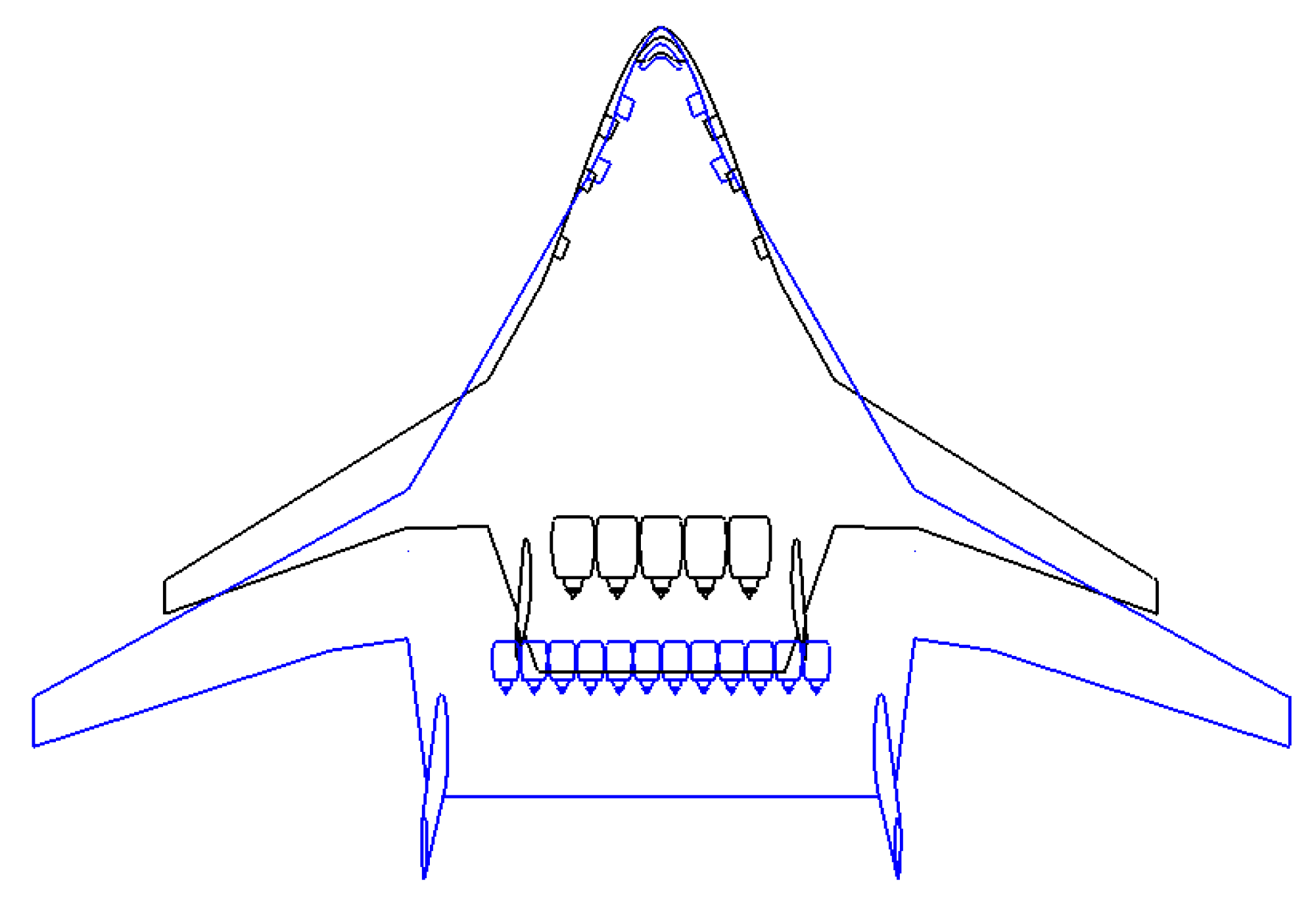
| Parameter | Kerosene BWB | LH2 BWB | Units | ||
|---|---|---|---|---|---|
| Wing | |||||
| Number of bays | 3 | 4 | 5 | 3 | |
| 6.2 | 4.88 | 5.37 | 4.67 | ||
| 745 | 710 | 702 | 1203 | m2 | |
| b | 68.0 | 58.9 | 51.8 | 74.9 | m |
| 42.25 | 36.45 | 29.6 | 46.1 | m | |
| 28.77 | 22.35 | 17.9 | 20.2 | m | |
| 2.1 | 2.02 | 2.07 | 2.38 | m | |
| 67.8 | 62.5 | 51.7 | 60.3 | deg | |
| 26.0 | 31.8 | 30.0 | 28.8 | deg | |
| 1.0 | 1.0 | 1.6 | 1.7 | deg | |
| 0.23 | |||||
| 0.13 | 0.10 | 0.17 | 0.04 | deg | |
| 0.76 | 0.67 | 0.66 | 0.83 | deg | |
| 0.092 | 0.097 | 0.094 | 0.096 | ||
| 0.092 | 0.094 | 0.090 | 0.098 | ||
| 3.0 | 2.7 | 2.0 | 2.6 | deg | |
| 0.0 | −0.1 | −0.1 | −2.5 | deg | |
| Vertical tail | |||||
| 1.32 | 1.27 | 1.40 | 1.3 | ||
| b | 5.9 | 5.0 | 5.75 | 7.3 | m |
| 0.38 | 0.35 | 0.40 | 0.5 | ||
| 54.5 | 56.5 | 56.0 | 51.2 | deg | |
| 9.5 | 12.0 | 2.0 | 10.0 | deg | |
| 0.09 | 0.09 | 0.09 | 0.09 | ||
| Propulsion | |||||
| Number of engines | 3 | 5 | 7 | 12 | |
| Engine diameter | 3.20 | 2.70 | 2.42 | 1.66 | m |
| 0.31 | 0.31 | 0.32 | 0.25 | N/N | |
| Hydrogen tanks | |||||
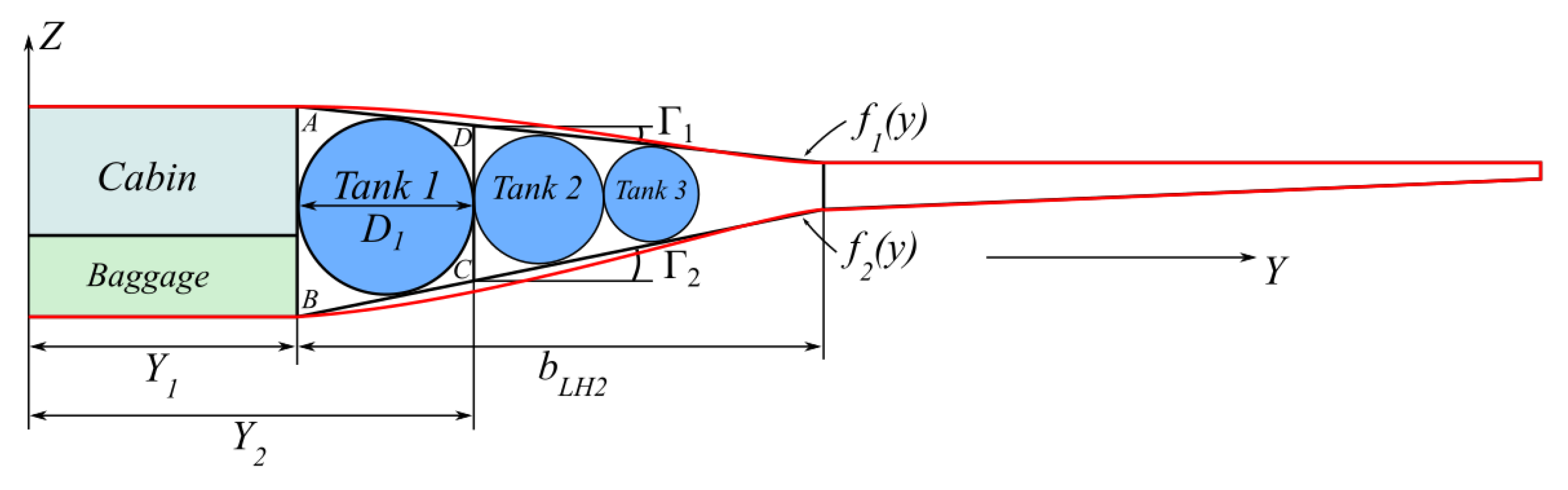
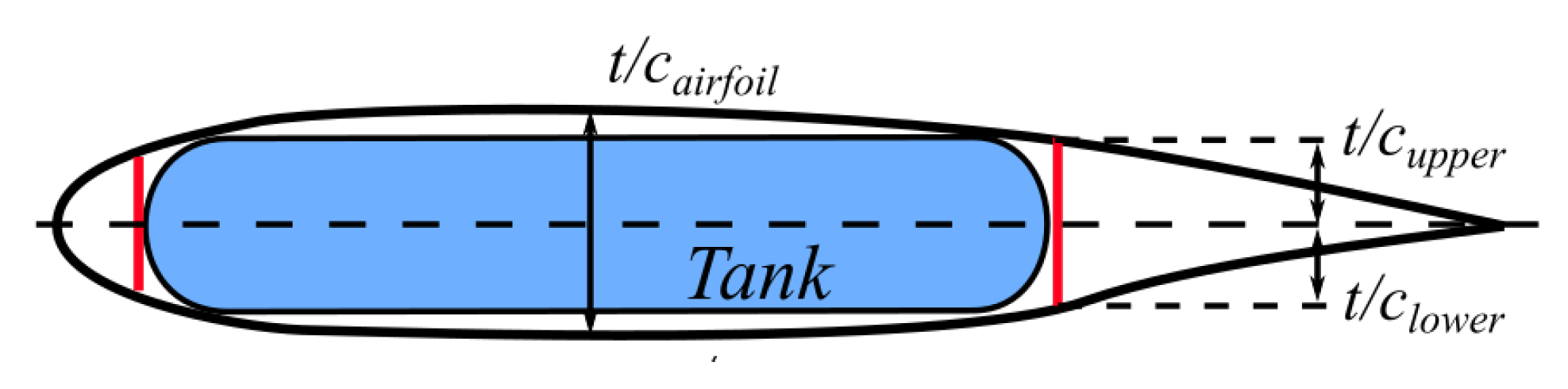
| Number of tanks | 4 | ||||
| 0.13 | |||||
| Configuration | Kerosene BWB | LH2 BWB | Units | ||
|---|---|---|---|---|---|
| Number of bays | 3 bays | 4 bays | 5 bays | ||
| 254,892 | 262,358 | 265,911 | 260,846 | kg | |
| 59,800 | 66,800 | 72,100 | 27,900 | kg | |
| 125,115 | 125,577 | 123,824 | 162,900 | kg | |
| 18,000 | 17,952 | 17,066 | 9941 | kg | |
| 3123 | 2914 | 2936 | 1923 | kg | |
| 9988 | 10,258 | 10,387 | 10,203 | kg | |
| 21,333 | 23,893 | 23,124 | 42,652 | kg | |
| 1737 | 1472 | 1836 | 3266 | kg | |
| 28,712 | 26,376 | 25,546 | 23,148 | kg | |
| 41,3406 | 41,846 | 42,151 | 44,943 | kg | |
| 25,503 | kg | ||||
| 815 | 865 | 777 | 1320 | kg | |
| Parameter | Kerosene BWB | LH2 BWB | B777-300ER | Units |
|---|---|---|---|---|
| 371,770 | 303,070 | 567,803 | kg/flight | |
| (69,148) | ||||
| Energy demand | 2631 | 3348 | 5210 | GJ/flight |
| Parameter | No Technologies | Airframe Technologies | UHBPR | BLI | All Technologies | Units |
|---|---|---|---|---|---|---|
| Wing | ||||||
| 4.50 | 4.56 | 4.76 | 5.48 | 4.67 | ||
| 1423 | 1348 | 1236 | 1101 | 1203 | m2 | |
| b | 80.0 | 78.4 | 76.6 | 77.8 | 74.9 | m |
| 50.8 | 51.0 | 43.5 | 42.4 | 46.1 | m | |
| 30.4 | 27.6 | 20.2 | 20.0 | 20.2 | m | |
| 2.8 | 2.7 | 2.2 | 1.63 | 2.38 | m | |
| 56.7 | 60.0 | 55.3 | 57.0 | 60.3 | deg | |
| 35.0 | 36.7 | 34.7 | 32.1 | 28.8 | deg | |
| 1.3 | 0.3 | 0.7 | 0.4 | 1.7 | deg | |
| 0.24 | 0.25 | 0.3 | 0.25 | 0.23 | ||
| 0.84 | 0.85 | 0.82 | 0.85 | 0.83 | deg | |
| 0.09 | 0.1 | 0.096 | 0.092 | 0.096 | ||
| 0.096 | 0.095 | 0.103 | 0.096 | 0.098 | ||
| 3.0 | 2.9 | 2.7 | 2.9 | 2.6 | deg | |
| −0.19 | 0.0 | −0.58 | −0.4 | −2.5 | deg | |
| Vertical tail | ||||||
| 1.68 | 1.5 | 1.35 | 1.33 | 1.3 | ||
| b | 7.4 | 7.2 | 6.9 | 6.9 | 7.3 | m |
| 0.37 | 0.4 | 0.36 | 0.32 | 0.45 | ||
| 50.2 | 49.6 | 51.5 | 52.3 | 51.2 | deg | |
| 28.6 | 40.0 | 34.6 | 30.0 | 10.0 | deg | |
| Propulsion | ||||||
| Number of engines | 2 | 2 | 3 | 13 | 12 | |
| Engine diameter | 2.66 | 2.57 | 3.22 | 1.32 | 1.75 | m |
| 0.25 | 0.29 | 0.26 | 0.29 | 0.25 | N/N | |
| Hydrogen tanks | ||||||
| Number of tanks | 4 | 4 | 6 | 6 | 4 | |
| 0.096 | 0.1 | 0.08 | 0.08 | 0.13 |
| Kerosene BWB | LH2 BWB | Description | Quantity | |
|---|---|---|---|---|
| Minimize | Drag coefficient | |||
| with respect to | Angle of attack [deg] | 1 | ||
| Wing sections twist [deg] | 9 | |||
| Vertical displacement of FFD control points [m] | 100 | |||
| Total design variables | 110 | |||
| subject to | CL = 0.20 | CL = 0.17 | Lift coefficient | 1 |
| ini,inwing ≥ 1.0 | Inner wing segment thickness constraint | 50 | ||
| ini,outwing ≥ 0.9 | Outer wing segment thickness constraint | 100 | ||
| LE,upper = LE,lower | Wing fixed trailing edge constraint | 10 | ||
| TE,upper = TE,lower | Wing fixed trailing edge constraint | 10 | ||
| ≥ 1.0 | inner wing internal volume constraint | 10 | ||
| Total constraints | 181 | |||
| Parameter | Kerosene BWB before Refinement | Kerosene BWB after Refinement | LH2 BWB before Refinement | LH2 BWB after Refinement | Units |
|---|---|---|---|---|---|
| 262,358 | 267,962 | 260,846 | 264,968 | kg | |
| 125,577 | 128,707 | 162,900 | 165,800 | kg | |
| 63,602 | 65,958 | 25,316 | 26,541 | kg | |
| 25,500 | 27,816 | kg | |||
| 371,770 | 384,023 | 303,070 | 315,010 | kg/flight | |
| (69,769) | (69,148) | (69,769) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karpuk, S.; Ma, Y.; Elham, A. Design Investigation of Potential Long-Range Hydrogen Combustion Blended Wing Body Aircraft with Future Technologies. Aerospace 2023, 10, 566. https://doi.org/10.3390/aerospace10060566
Karpuk S, Ma Y, Elham A. Design Investigation of Potential Long-Range Hydrogen Combustion Blended Wing Body Aircraft with Future Technologies. Aerospace. 2023; 10(6):566. https://doi.org/10.3390/aerospace10060566
Chicago/Turabian StyleKarpuk, Stanislav, Yiyuan Ma, and Ali Elham. 2023. "Design Investigation of Potential Long-Range Hydrogen Combustion Blended Wing Body Aircraft with Future Technologies" Aerospace 10, no. 6: 566. https://doi.org/10.3390/aerospace10060566
APA StyleKarpuk, S., Ma, Y., & Elham, A. (2023). Design Investigation of Potential Long-Range Hydrogen Combustion Blended Wing Body Aircraft with Future Technologies. Aerospace, 10(6), 566. https://doi.org/10.3390/aerospace10060566








