Effect of Structural Materials on Monopropellant Thruster Propulsion Performance in Micro Scale
Abstract
1. Introduction
2. Materials for Microthruster Fabrication
3. Numerical Comparative Performance Analysis
3.1. Governing Equations
3.2. Geometry and Computational Domain
3.3. Gas and Materials Properties
3.4. Computational Scheme and Boundary Conditions
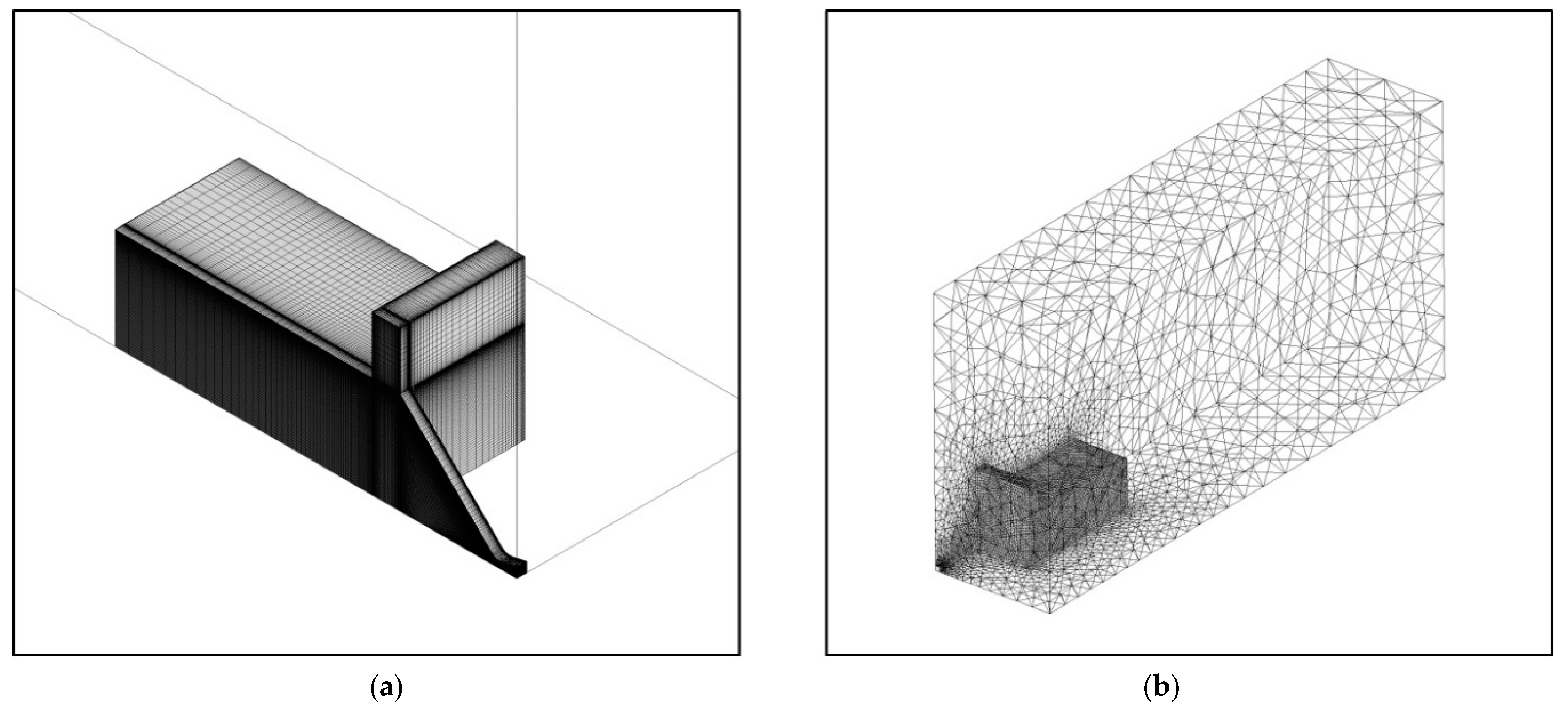
3.5. Grid Convergence Study
4. Results and Discussion
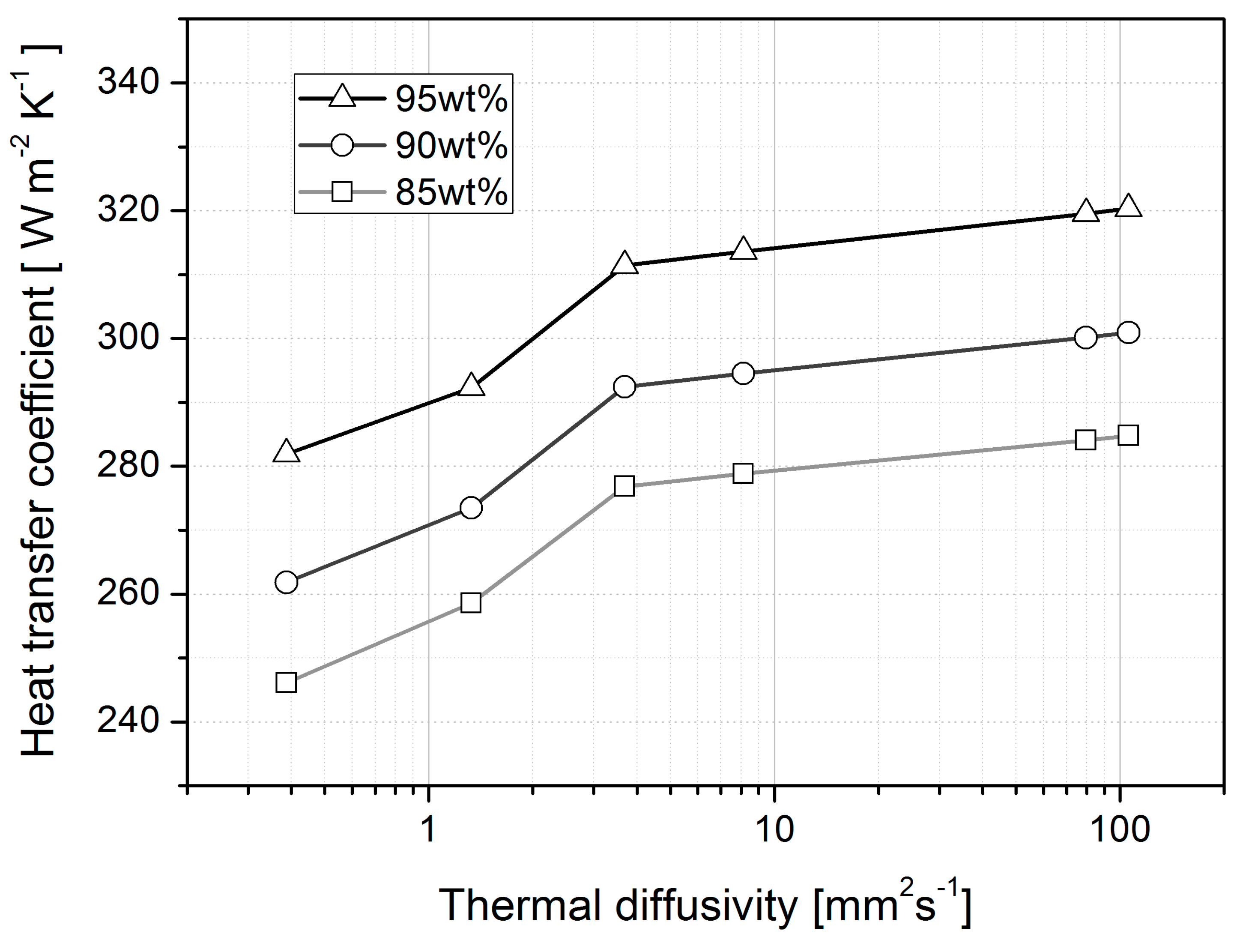
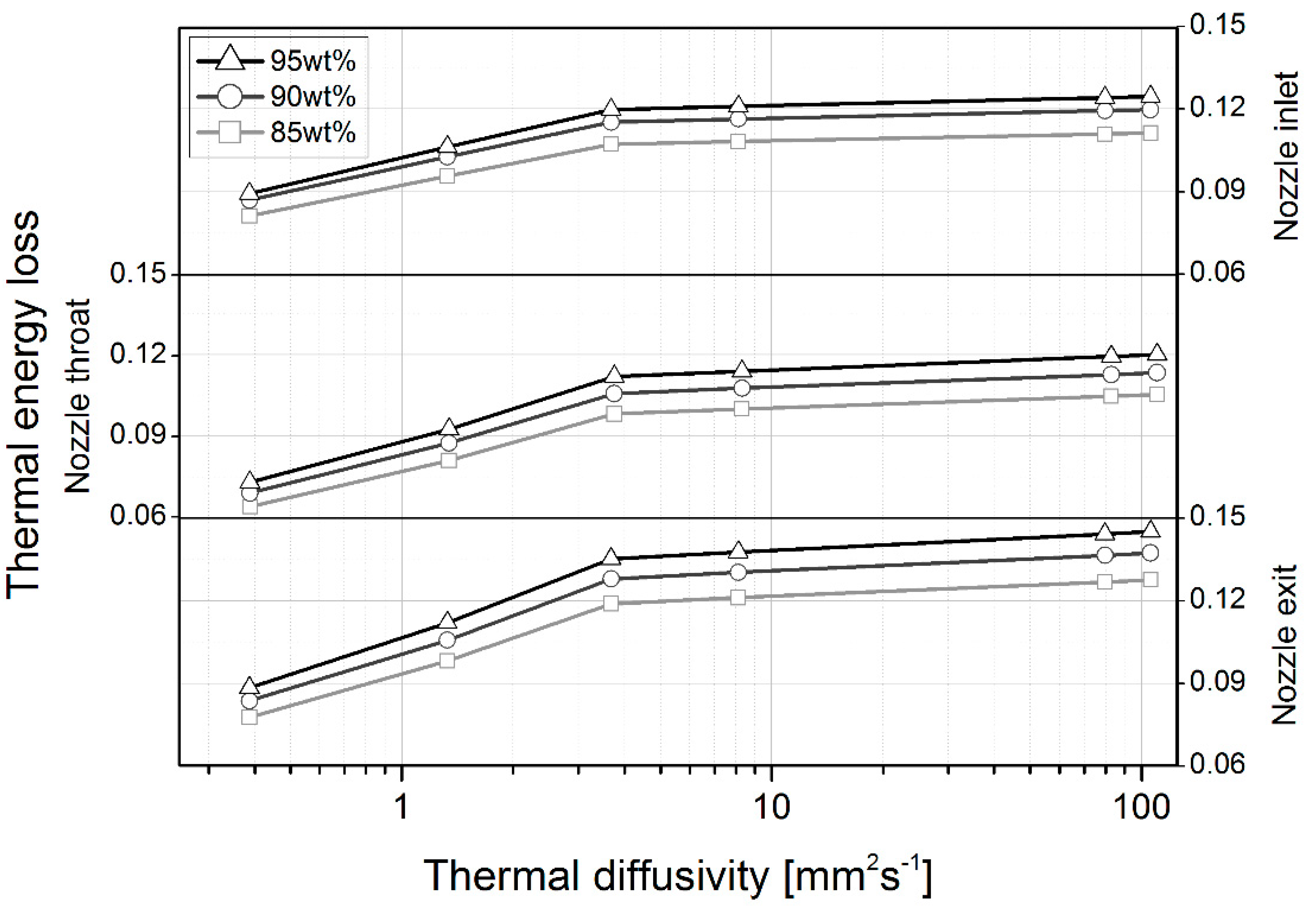
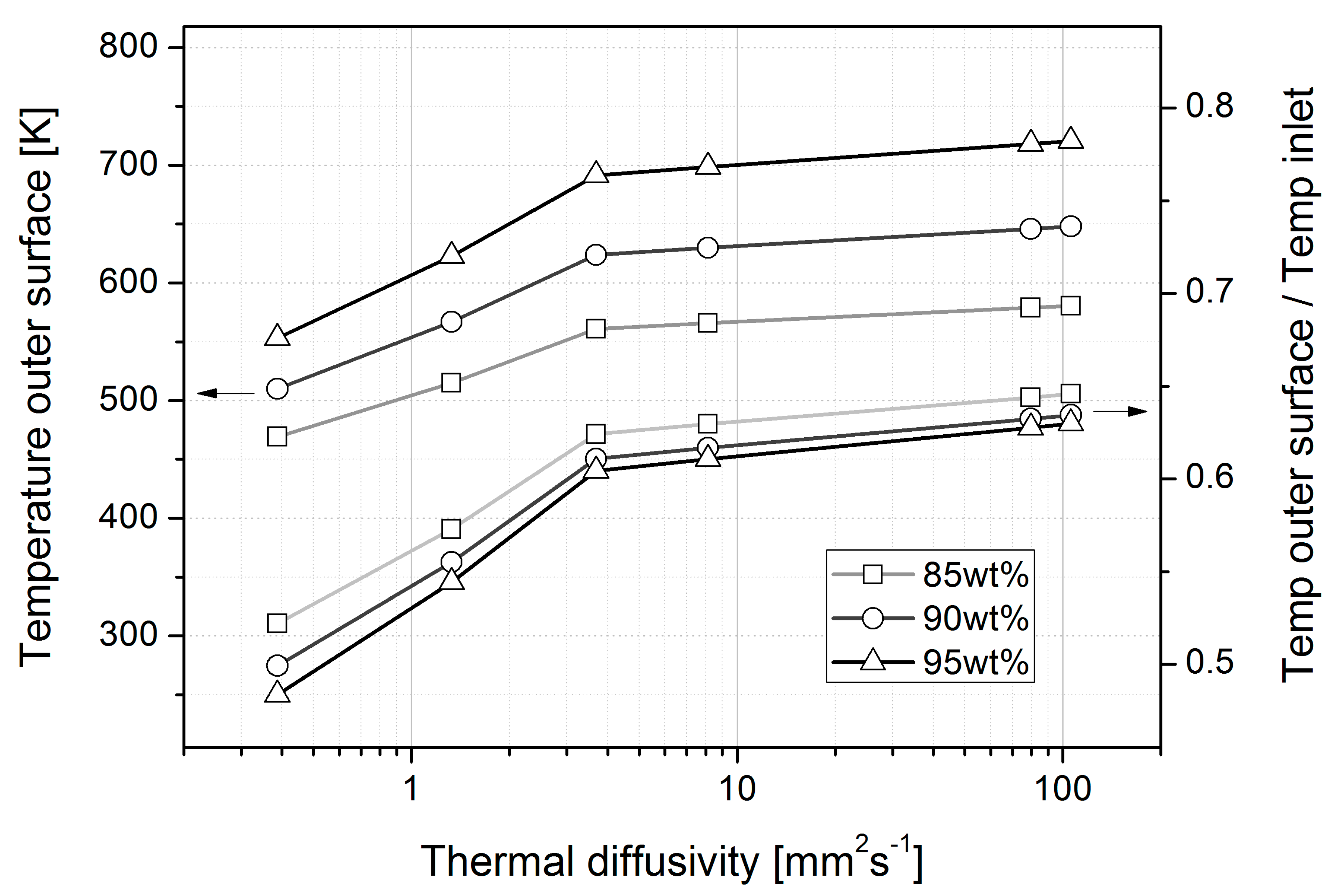
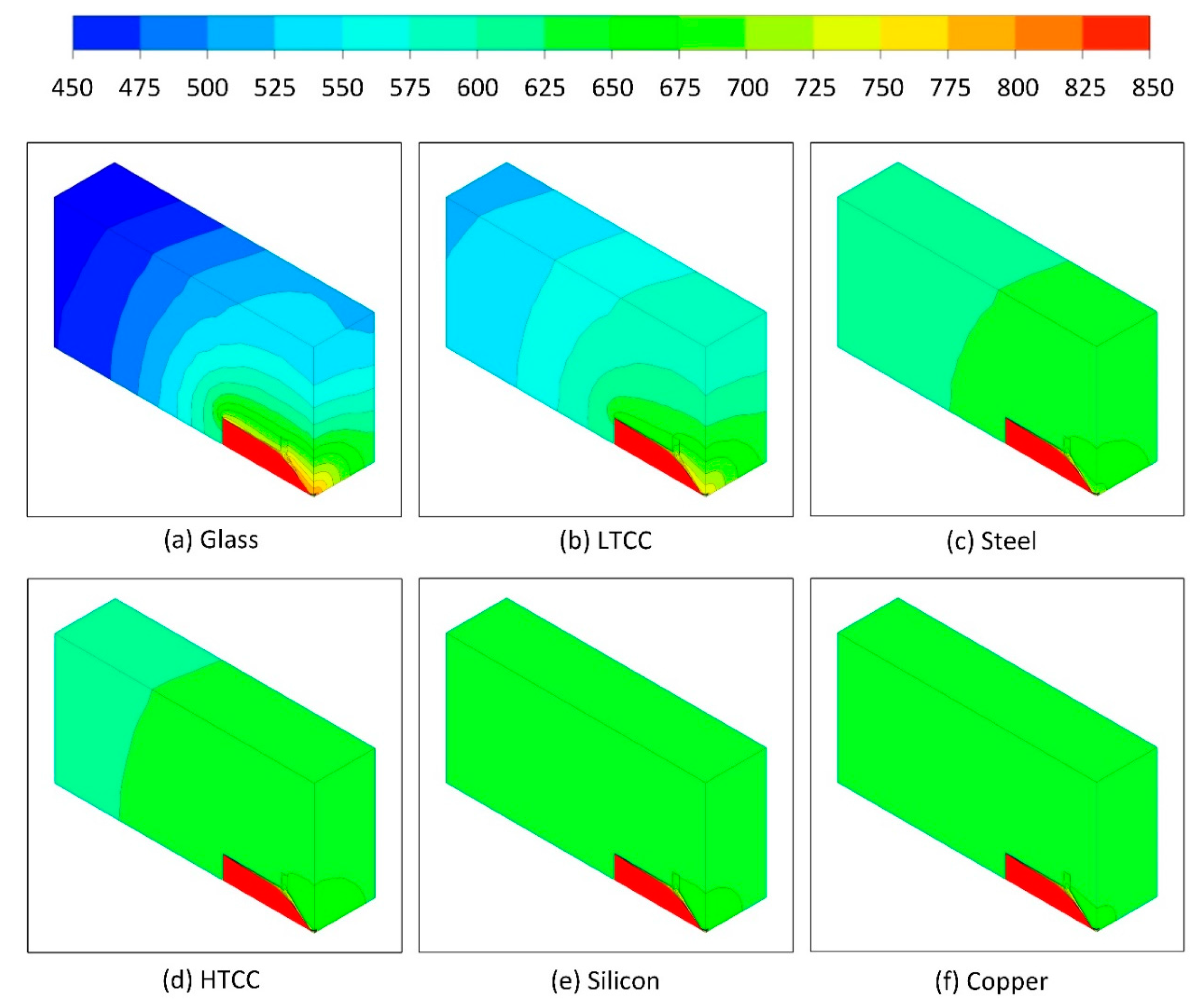
4.1. Heat Loss
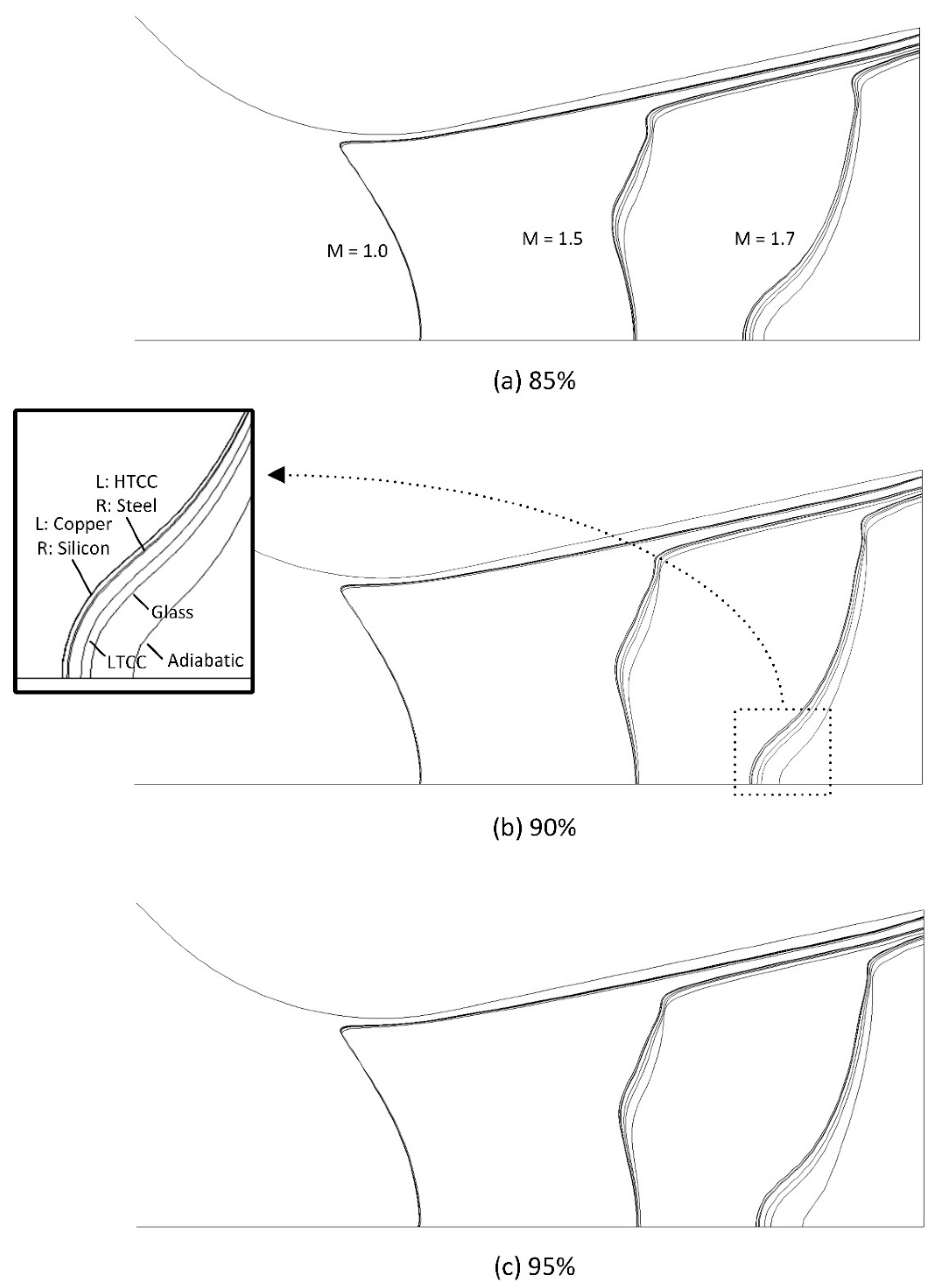
4.2. Internal Flow
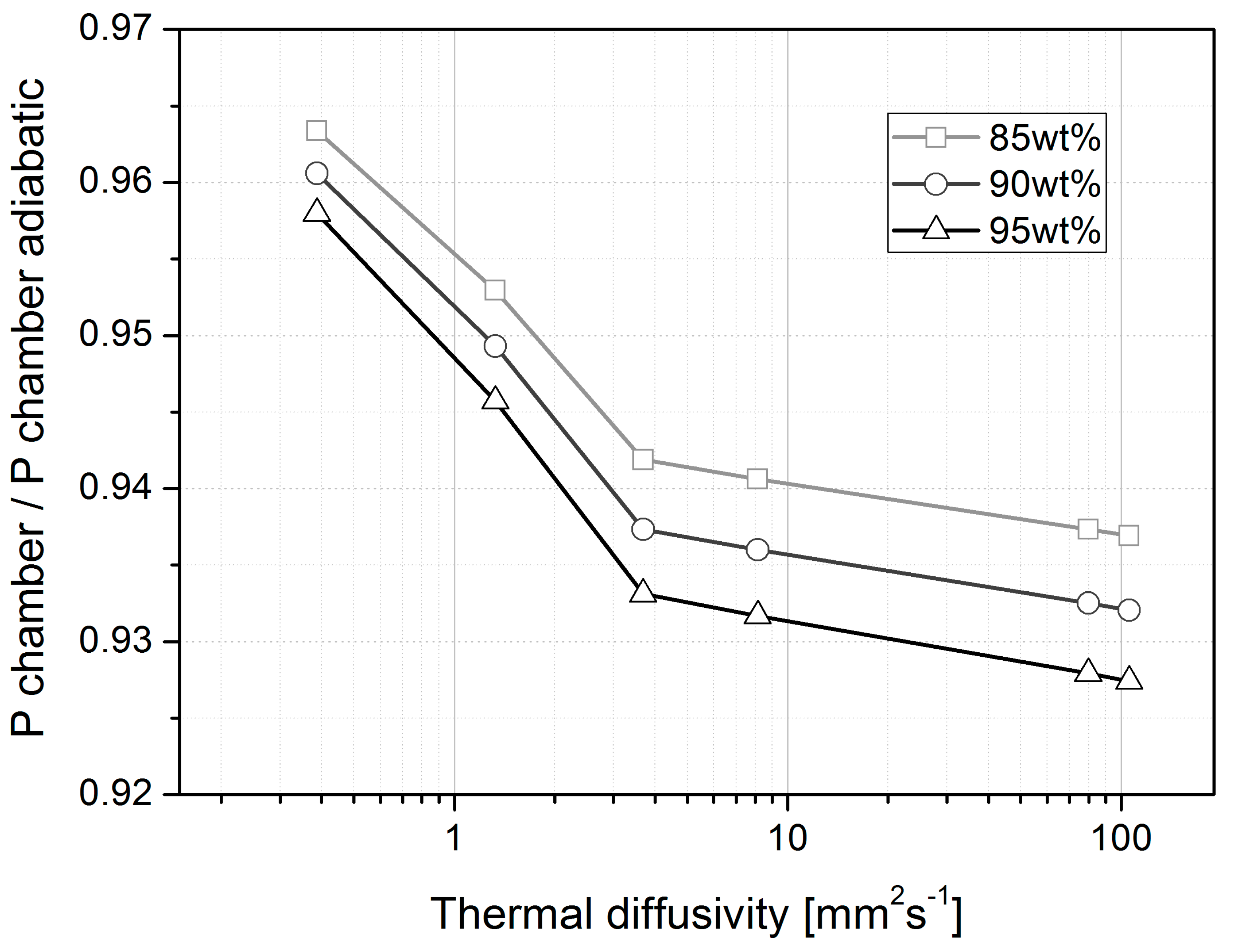
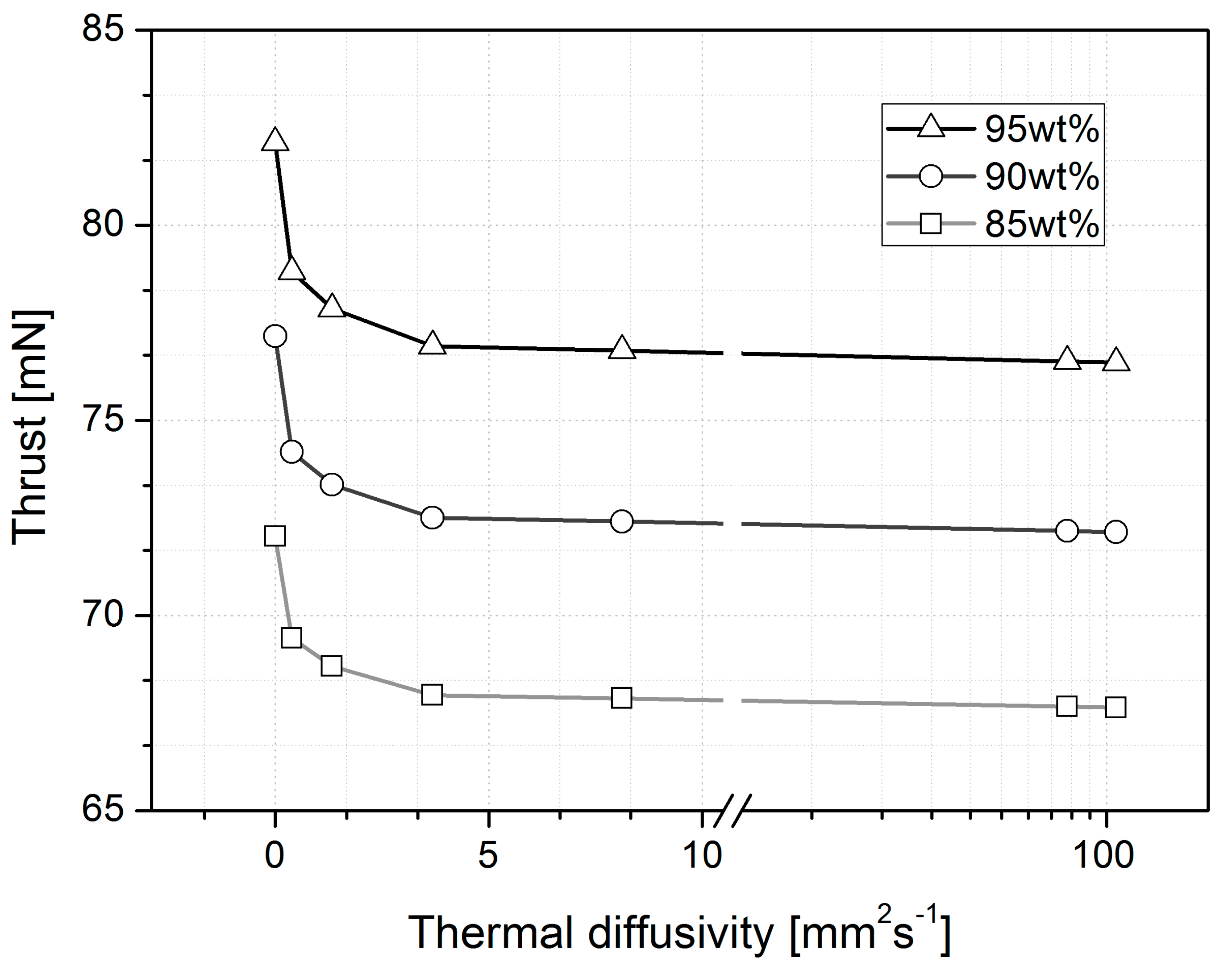
4.3. Chamber Pressure and Thrust
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Massaro Tieze, S.; Liddell, L.C.; Santa Maria, S.R.; Bhattacharya, S. BioSentinel: A Biological CubeSat for Deep Space Exploration. Astrobiology 2020, 20. [Google Scholar] [CrossRef]
- Klesh, A.; Clement, B.; Colley, C.; Essmiller, J.; Forgette, D.; Krajewski, J.; Marinan, A.; Martin-Mur, T.; Steinkraus, J.; Sternberg, D.; et al. MarCO: Early Operations of the First CubeSats to Mars. In Proceedings of the 32nd Annual AIAA/USU Conference on Small Satellites, Logan, UT, USA, 4–9 August 2018; AIAA: Logan, UT, USA, 2018. [Google Scholar]
- Ricco, A.J.; Maria, S.R.S.; Hanel, R.P.; Bhattacharya, S. BioSentinel: A 6U Nanosatellite for Deep-Space Biological Science. IEEE Aerosp. Electron. Syst. Mag. 2020, 35, 6–18. [Google Scholar] [CrossRef]
- Cho, D.-H.; Choi, W.-S.; Kim, M.-K.; Kim, J.-H.; Sim, E.; Kim, H.-D. High-Resolution Image and Video CubeSat (HiREV): Development of Space Technology Test Platform Using a Low-Cost CubeSat Platform. Int. J. Aerosp. Eng. 2019, 2019, 8916416. [Google Scholar] [CrossRef]
- Cheng, A.F.; Atchison, J.; Kantsiper, B.; Rivkin, A.S.; Stickle, A.; Reed, C.; Galvez, A.; Carnelli, I.; Michel, P.; Ulamec, S. Asteroid Impact and Deflection Assessment mission. Acta Astronaut. 2015, 115, 262–269. [Google Scholar] [CrossRef]
- Michel, P.; Cheng, A.; Küppers, M.; Pravec, P.; Blum, J.; Delbo, M.; Green, S.F.; Rosenblatt, P.; Tsiganis, K.; Vincent, J.B.; et al. Science case for the Asteroid Impact Mission (AIM): A component of the Asteroid Impact & Deflection Assessment (AIDA) mission. Adv. Space Res. 2016, 57, 2529–2547. [Google Scholar]
- Mao, Z.; Yoshida, K.; Kim, J.-W. A micro vertically-allocated SU-8 check valve and its characteristics. Microsyst. Technol. 2018, 25, 245–255. [Google Scholar] [CrossRef]
- Mao, Z.; Yoshida, K.; Kim, J.-W. A droplet-generator-on-a-chip actuated by ECF (electro-conjugate fluid) micropumps. Microfluid. Nanofluidics 2019, 23, 12. [Google Scholar] [CrossRef]
- Hitt, D.L.; Zakrzwski, C.M.; Thomas, M.A. MEMS-based satellite micropropulsion via catalyzed hydrogen peroxide decomposition. Smart Mater. Struct. 2001, 10, 1163–1175. [Google Scholar] [CrossRef]
- Kundu, P.; Sinha, A.K.; Bhattacharyya, T.K.; Das, S. MnO2 nanowire embedded hydrogen peroxide monopropellant MEMS thruster. J. Microelectromech. Syst. 2013, 22, 406–417. [Google Scholar] [CrossRef]
- Khaji, Z.; Klintberg, L.; Barbade, D.; Palmer, K.; Thornell, G. Endurance and failure of an alumina-based monopropellant microthruster with integrated heater, catalytic bed and temperature sensors. J. Micromech. Microeng. 2017, 27, 055011. [Google Scholar] [CrossRef]
- Kim, J.W.; Bhosale, V.K.; Kim, K.-S.; Lee, S.; Kwon, S. Room-temperature catalytically reactive ammonium dinitramide–H2O2 monopropellant for microsatellites. Adv. Space Res. 2022, 69, 1631–1644. [Google Scholar] [CrossRef]
- Huh, J.; Seo, D.; Kwon, S. Fabrication of a liquid monopropellant microthruster with built-in regenerative micro-cooling channels. Sens. Actuators A Phys. 2017, 263, 332–340. [Google Scholar] [CrossRef]
- Lee, J.; Kim, T. MEMS solid propellant thruster array with micro membrane igniter. Sens. Actuators A Phys. 2013, 190, 52–60. [Google Scholar] [CrossRef]
- Lee, D.; Kim, J.; Kwon, S. High performance microthruster with ammonium-dinitramide-based monopropellant. Sens. Actuators A Phys. 2018, 283, 211–219. [Google Scholar] [CrossRef]
- Huh, J.; Kwon, S. Microcooling Channel Effect on a Monopropellant Microelectromechanical System Thruster Performance. J. Propuls. Power 2017, 33, 1591–1595. [Google Scholar] [CrossRef]
- Lee, J.; Kim, S.; Kwon, S.; Yu, M. Fabrication of catalyst-insertion-type microelectromechanical systems monopropellant thruster. J. Propuls. Power 2013, 28, 396–404. [Google Scholar] [CrossRef]
- Seo, D.; Lee, J.; Kwon, S. The development of the micro-solid propellant thruster array with improved repeatability. J. Micromech. Microeng. 2012, 22, 094004. [Google Scholar] [CrossRef]
- Markandan, K.; Zhang, Z.; Chin, J.; Cheah, K.H.; Tang, H.-B. Fabrication and preliminary testing of hydroxylammonium nitrate (HAN)-based ceramic microthruster for potential application of nanosatellites in constellation formation flying. Microsyst. Technol. 2019, 25, 4209–4217. [Google Scholar] [CrossRef]
- Huh, J.; Kwon, S. Design, fabrication and thrust measurement of a micro liquid monopropellant thruster. J. Micromech. Microeng. 2014, 24, 9. [Google Scholar] [CrossRef]
- Miyakawa, N.; Legner, W.; Ziemann, T.; Telitschkin, D.; Fecht, H.-J.; Friedberger, A. MEMS-based microthruster with integrated platinum thin film resistance temperature detector (RTD), heater meander and thermal insulation for operation up to 1000 °C. Microsyst. Technol. 2012, 18, 1077–1087. [Google Scholar] [CrossRef]
- Takahashi, K.; Ikuta, T.; Dan, Y.; Nagayama, K.; Kishida, M. Catalytic porous microchannel for hydrogen peroxide MEMS thruster. In Proceedings of the 23rd Sensor Symposium., Takamatsu, Japan, 5–6 October 2006; IEEJ: Takamatsu, Japan, 2006; pp. 513–516. [Google Scholar]
- Yuan, T.; Li, A.; Huang, B.; Chen, Y.-T.; Chen, C. Design, Fabrication, and Test of a Microelectromechanical-System-Based Millinewton-Level Hydrazine Thruster. J. Propuls. Power 2011, 27, 509–512. [Google Scholar] [CrossRef]
- Wu, M.H.; Yetter, R.A. Development and analysis of a LTCC micro stagnation-point flow combustor. J. Micromech. Microeng. 2008, 18, 9. [Google Scholar] [CrossRef]
- Cheah, K.H.; Khiew, P.S.; Chin, J.K. Fabrication of a zirconia MEMS-based microthruster by gel casting on PDMS soft molds. J. Micromech. Microeng. 2012, 22, 095013. [Google Scholar] [CrossRef]
- Huh, J.; Park, K.S.; Lee, J.; Kwon, S. Performance of MEMS-Based Monopropellant Microthruster With Insulating Effect. J. Microelectromech. Syst. 2022, 31, 612–624. [Google Scholar] [CrossRef]
- Chan, Y.A.; Tseng, K.C.; Kuo, T.C. Preliminary Development of a Hydrogen Peroxide Thruster. Int. J. Mech. Aerosp. Ind. Mechatron. Manuf. Eng. 2013, 7, 7. [Google Scholar]
- Scharlemann, C.; Schiebl, M.; Marhold, K.; Tajmar, M.; Miotti, P.; Kappenstein, C.; Batonneau, Y.; Brahmi, R.; Hunter, C. Development and Test of a Miniature Hydrogen Peroxide Monopropellant Thruster. In Proceedings of the 42nd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Sacramento, CA, USA, 9 July 2006; AIAA: Sacramento, CA, USA, 2006. [Google Scholar]
- Kuan, C.K.; Chen, G.B.; Chao, Y.C. Development and ground tests of a 100-millinewton hydrogen peroxide monopropellant microthruster. J. Propuls. Power 2007, 23, 1313–1320. [Google Scholar] [CrossRef]
- Chen, X.; Li, Y.; Zhou, Z.; Fan, R. A homogeneously catalyzed micro-chemical thruster. Sens. Actuators A Phys. 2003, 108, 149–154. [Google Scholar] [CrossRef]
- Xu, X.; Li, X.; Zhou, J.; Zhang, B.; Xiao, D.; Huang, Y.; Wu, X. Numerical and experimental analysis of cold gas microthruster geometric parameters by univariate and orthogonal method. Microsyst. Technol. 2017, 23, 5003–5016. [Google Scholar] [CrossRef]
- Wu, M.H.; Yetter, R.A. A novel electrolytic ignition monopropellant microthruster based on low temperature co-fired ceramic tape technology. Lab Chip 2009, 9, 910–916. [Google Scholar] [CrossRef]
- Bartsch, M.S.; McCrink, M.H.; Crocker, R.W.; Mosier, B.P.; Peterson, K.A.; Wally, K.; Patel, K.D. Electrokinetically Pumped Liquid Propellant Microthrusters for Orbital Station Keeping. In Proceedings of the TRANSDUCERS 2007—International Solid-State Sensors, Actuators and Microsystems Conference, Lyon, France, 10–14 June 2007; IEEE: Lyon, France, 2007; pp. 2437–2440. [Google Scholar]
- Wu, M.H.; Lin, P.S. Design, fabrication and characterization of a low-temperature co-fired ceramic gaseous bi-propellant microthruster. J. Micromechanics Microengineering 2010, 20, 085026. [Google Scholar] [CrossRef]
- Tropea, C.; Yarin, A.L.; Foss, J.F. Springer Handbook of Experimental Fluid Mechanics, 1st ed.; Springer: Berlin, Heidelberg, 2007. [Google Scholar]
- Linstrom, P.J.; Mallard, W.G. NIST Chemistry WebBook; National Institute of Standards and Technology: Gaithersburg, MD, USA, 1996.
- Sebastian, M.T.; Jantunen, H. Low loss dielectric materials for LTCC applications: A review. Int. Mater. Rev. 2013, 53, 57–90. [Google Scholar] [CrossRef]
- Golonka, L.J. Technology and applications of Low Temperature Cofired Ceramic (LTCC) based sensors and microsystems. Bull. Pol. Acad. Sci. Tech. Sci. 2006, 54, 221–231. [Google Scholar]
- Bechtold, F. A comprehensive overview on today’s ceramic substrate technologies. In Proceedings of the European Microelectronics and Packaging Conference, Rimini, Italy, 15–18 June 2009; IEEE: Rimini, Italy, 2009; pp. 1–12. [Google Scholar]
- Sebastian, M.T.; Jantunen, H. High Temperature Cofired Ceramic (HTCC), Low Temperature Cofired Ceramic (LTCC), and Ultralow Temperature Cofired Ceramic (ULTCC) Materials; Wiley: Hoboken, NJ, USA, 2017; pp. 355–425. [Google Scholar]
- Huh, J.; Kwon, S. Effect of micro cooling channels on a hydrogen peroxide monopropellant microthruster performance. J. Phys. Conf. Ser. 2015, 660, 012020. [Google Scholar] [CrossRef]





| Fabrication Material | Target Thrust † (mN) | Reference | Type | Propellant | Catalyst |
|---|---|---|---|---|---|
| Stainless steel (Machining) | 850 | [27] | Monopropellant | H2O2 90% | Ag |
| 500 | [28] | Monopropellant | H2O2 80–87% | MnO2/Al2O3 | |
| 100 | [29] | Monopropellant | H2O2 92% | Ag/flake | |
| Silicon | 13.5 | [30] | Monopropellant | H2O2 87% | FeCl2 liquid |
| 1 | [23] | Monopropellant | Hydrazine | Ir/Ti, SiOx, Si | |
| 1 | [10] | Monopropellant | H2O2 50% | MnO2 nanowire | |
| 0.5 | [9] | Monopropellant | H2O2 90% | Ag | |
| N/A | [21] | Monopropellant | Hydrazine | Metallic substrate | |
| Silicon (w/Glass) | 3.78 | [31] | Cold gas | Nitrogen gas | N/A |
| ~1 | [22] | Monopropellant | H2O2 60, 90% | Pt | |
| HTCC | N/A | [25] | Monopropellant | HAN | N/A |
| 0.96 | [11] | Monopropellant | H2O2 31% | Pt | |
| 360 | [19] | Monopropellant | HAN based | Electrolytic ignition | |
| LTCC | 150 | [32] | Monopropellant | HAN based | Electrolytic ignition |
| 3 | [33] | Monopropellant | H2O2 | Ag | |
| 1 | [34] | Bipropellant | Ethylene/argon–oxygen | Spark ignition | |
| Glass | 100 | [17] | Monopropellant | H2O2 90% | Pt |
| 50 | [20] | Monopropellant | H2O2 90% | Pt | |
| 35 | [12] | Monopropellant | ADN/H2O2 | Pt/La/Al2O3 |
| f(T) = C6T6 + C5T5 + C4T4 + C3T3 + C2T2 + C1T + C0 | ||||||||
|---|---|---|---|---|---|---|---|---|
| Concentrations | Coefficients | |||||||
| C6 | C5 | C4 | C3 | C2 | C1 | C0 | ||
| Cp: ×10−13 | Cp: ×10−10 | Cp: ×10−7 | Cp: ×10−4 | Cp: ×10−1 | Cp: ×102 | Cp: ×104 | ||
| k: ×10−19 | k: ×10−15 | k: ×10−12 | k: ×10−9 | k: ×10−6 | k: ×10−4 | k: ×10−2 | ||
| μ: ×10−24 | μ: ×10−20 | μ: ×10−17 | μ: ×10−14 | μ: ×10−11 | μ: ×10−8 | μ: ×10−6 | ||
| 95% | Cp | 1.10128 | −4.94483 | 9.16607 | −8.98223 | 4.91171 | −1.41838 | 1.83952 |
| k | 2.89022 | −1.36077 | 2.67550 | −2.83258 | 1.73078 | −4.83433 | 7.24316 | |
| μ | 5.01273 | −2.70294 | 6.26318 | −7.89123 | 4.97555 | 2.80154 | 2.48434 | |
| 90% | Cp | 1.14765 | −5.15273 | 9.55068 | −9.35823 | 5.11684 | −1.47763 | 1.91276 |
| k | 2.99845 | −1.41087 | 2.77169 | −2.93117 | 1.78915 | −5.02033 | 7.47042 | |
| μ | 5.32318 | −2.87547 | 6.68007 | −8.46258 | 5.45069 | 2.58741 | 2.57703 | |
| 85% | Cp | 1.19401 | −5.36055 | 9.93516 | −9.73410 | 5.32189 | −1.53686 | 1.98596 |
| k | 3.10478 | −1.46009 | 2.86620 | −3.02803 | 1.84651 | −5.20307 | 7.69370 | |
| μ | 5.62945 | −3.04568 | 7.09135 | −9.02626 | 5.91946 | 2.37615 | 2.66848 | |
| Materials | Thermal Conductivity (W m−1 K−1) | Specific Heat (J kg−1 K−1) | Density (kg m−3) | Thermal Diffusivity (mm2 s−1) |
|---|---|---|---|---|
| Copper | 385 | 406 | 8960 | 106.8 |
| Silicon | 130 | 700 | 2330 | 79.7 |
| HTCC | 20 | 750 | 3280 | 8.1 |
| Stainless Steel | 15 | 502 | 7920 | 3.7 |
| LTCC | 3 | 729 | 3100 | 1.3 |
| Glass | 1 | 1200 | 2365 | 0.4 |
| Grid | No. of Elements in Fluid Domain | No. of Elements in Solid Domain | Thrust (mN) | Average Temperature * (K) |
|---|---|---|---|---|
| Coarse | 394,000 | 81,503 | 74.13 | 509.84 |
| Intermediate | 936,400 | 116,419 | 74.19 | 510.00 |
| Dense | 1,736,000 | 399,672 | 74.24 | 510.10 |
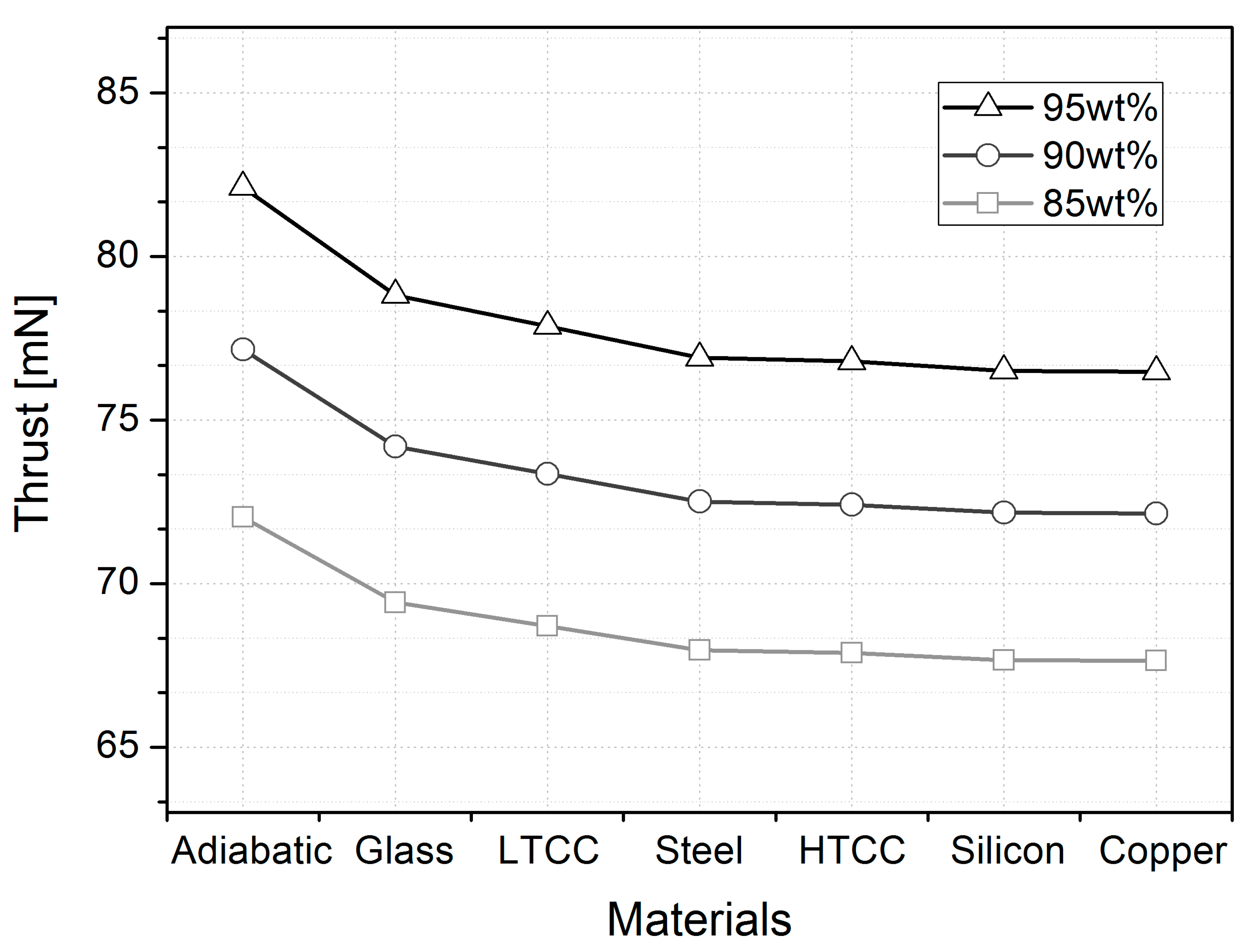
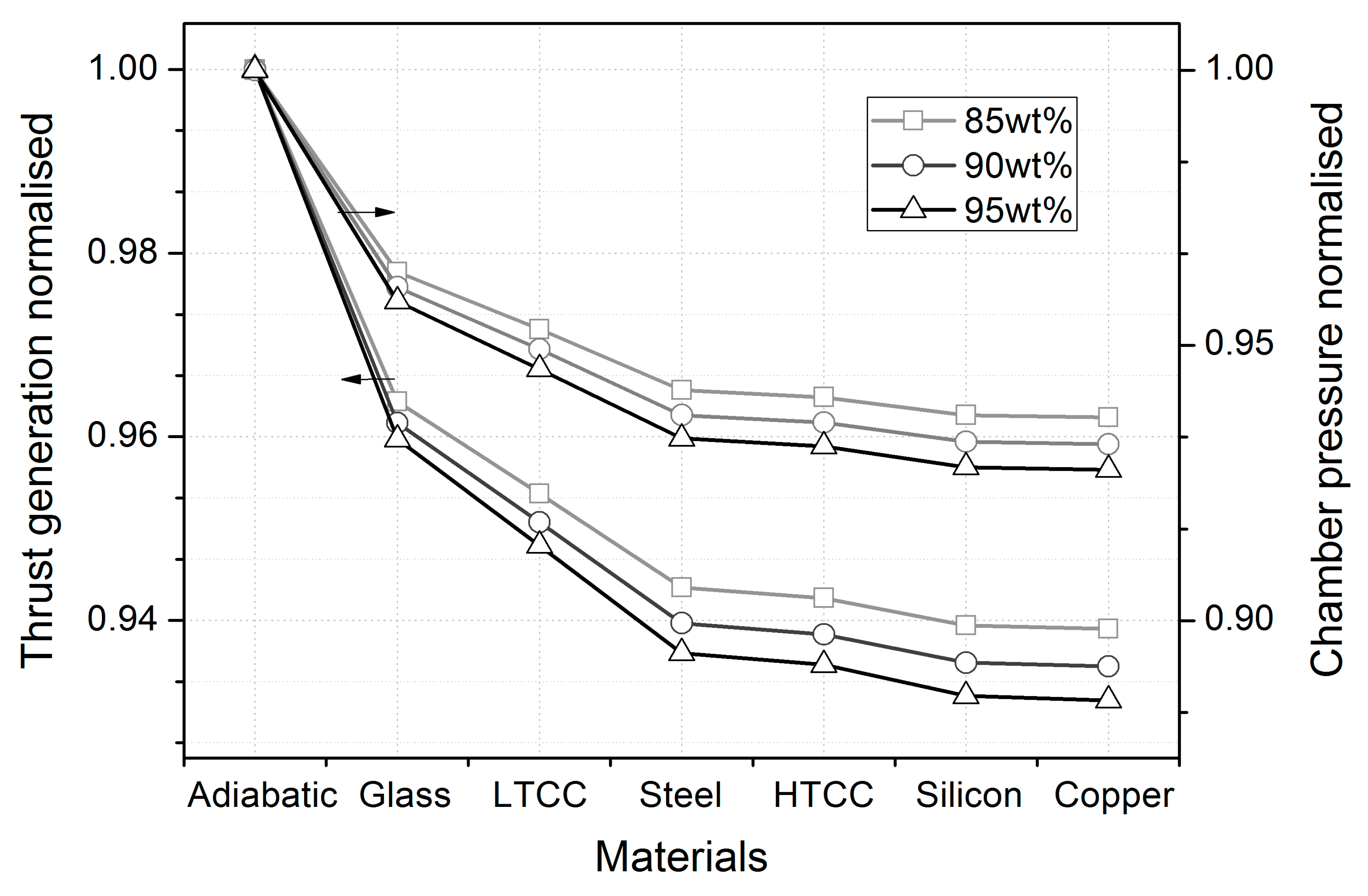
| Parameters | wt% | Adiabatic | Glass | LTCC | Stainless Steel | HTCC | Silicon | Copper |
|---|---|---|---|---|---|---|---|---|
| Thrust (mN) | 95% | 82.12 | 78.81 | 77.86 | 76.90 | 76.79 | 76.51 | 76.48 |
| 90% | 77.16 | 74.19 | 73.35 | 72.51 | 72.41 | 72.17 | 72.14 | |
| 85% | 72.03 | 69.42 | 68.70 | 67.96 | 67.88 | 67.66 | 67.64 | |
| Heat transfer coefficient # (W m−2 K−1) | 95% | N/A | 281.91 | 292.31 | 311.41 | 313.63 | 319.52 | 320.31 |
| 90% | N/A | 261.80 | 273.48 | 292.40 | 294.52 | 300.14 | 300.89 | |
| 85% | N/A | 246.13 | 258.56 | 276.86 | 278.86 | 284.10 | 284.80 | |
| Temperature outside surface * (K) | 95% | N/A | 553.27 | 622.81 | 691.45 | 698.73 | 718.04 | 720.64 |
| 90% | N/A | 510.00 | 567.04 | 623.92 | 629.96 | 645.98 | 648.13 | |
| 85% | N/A | 469.23 | 514.94 | 560.95 | 565.84 | 578.82 | 580.56 | |
| Temperature nozzle inlet * (K) | 95% | 1144.00 | 1042.00 | 1022.7 | 1007.2 | 1005.8 | 1002.1 | 1001.6 |
| 90% | 1021.40 | 932.83 | 916.71 | 903.84 | 902.65 | 899.63 | 899.23 | |
| 85% | 898.69 | 825.96 | 812.96 | 802.58 | 801.62 | 799.19 | 798.87 | |
| Temperature nozzle throat * (K) | 95% | 1026.6 | 951.48 | 931.41 | 911.73 | 909.66 | 904.2 | 903.47 |
| 90% | 908.55 | 845.64 | 829.01 | 812.52 | 810.78 | 806.19 | 805.57 | |
| 85% | 795.75 | 744.52 | 731.06 | 717.56 | 716.14 | 712.4 | 711.9 | |
| Temperature nozzle exit * (K) | 95% | 831.55 | 757.99 | 738.37 | 719.09 | 717.01 | 711.60 | 710.89 |
| 90% | 730.29 | 669.17 | 653.08 | 636.82 | 635.12 | 630.61 | 629.98 | |
| 85% | 634.83 | 585.48 | 572.50 | 559.32 | 557.93 | 554.33 | 553.83 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huh, J.; Park, K.S. Effect of Structural Materials on Monopropellant Thruster Propulsion Performance in Micro Scale. Aerospace 2023, 10, 362. https://doi.org/10.3390/aerospace10040362
Huh J, Park KS. Effect of Structural Materials on Monopropellant Thruster Propulsion Performance in Micro Scale. Aerospace. 2023; 10(4):362. https://doi.org/10.3390/aerospace10040362
Chicago/Turabian StyleHuh, Jeongmoo, and Ki Sun Park. 2023. "Effect of Structural Materials on Monopropellant Thruster Propulsion Performance in Micro Scale" Aerospace 10, no. 4: 362. https://doi.org/10.3390/aerospace10040362
APA StyleHuh, J., & Park, K. S. (2023). Effect of Structural Materials on Monopropellant Thruster Propulsion Performance in Micro Scale. Aerospace, 10(4), 362. https://doi.org/10.3390/aerospace10040362







