Gust Response of Spanwise Morphing Wing by Simulation and Wind Tunnel Testing
Abstract
1. Introduction
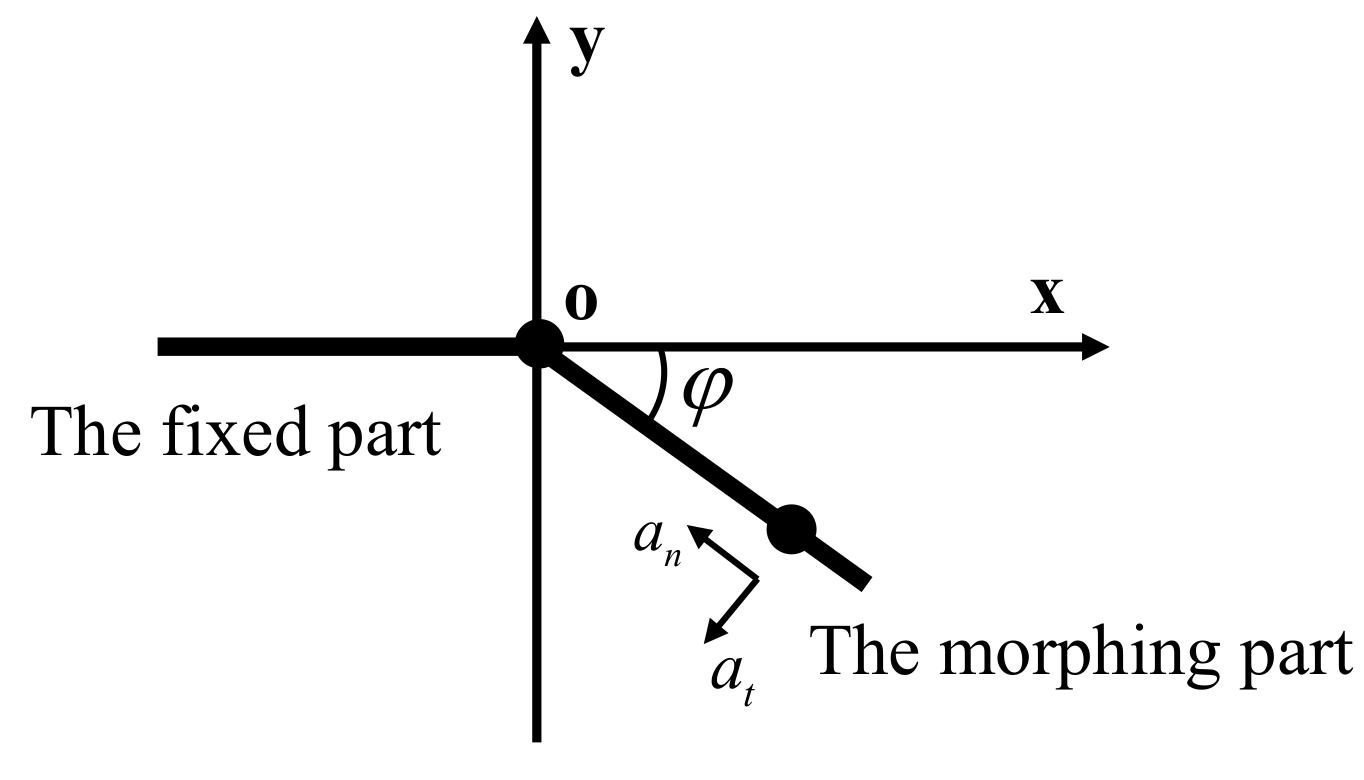
2. Test Model and Method
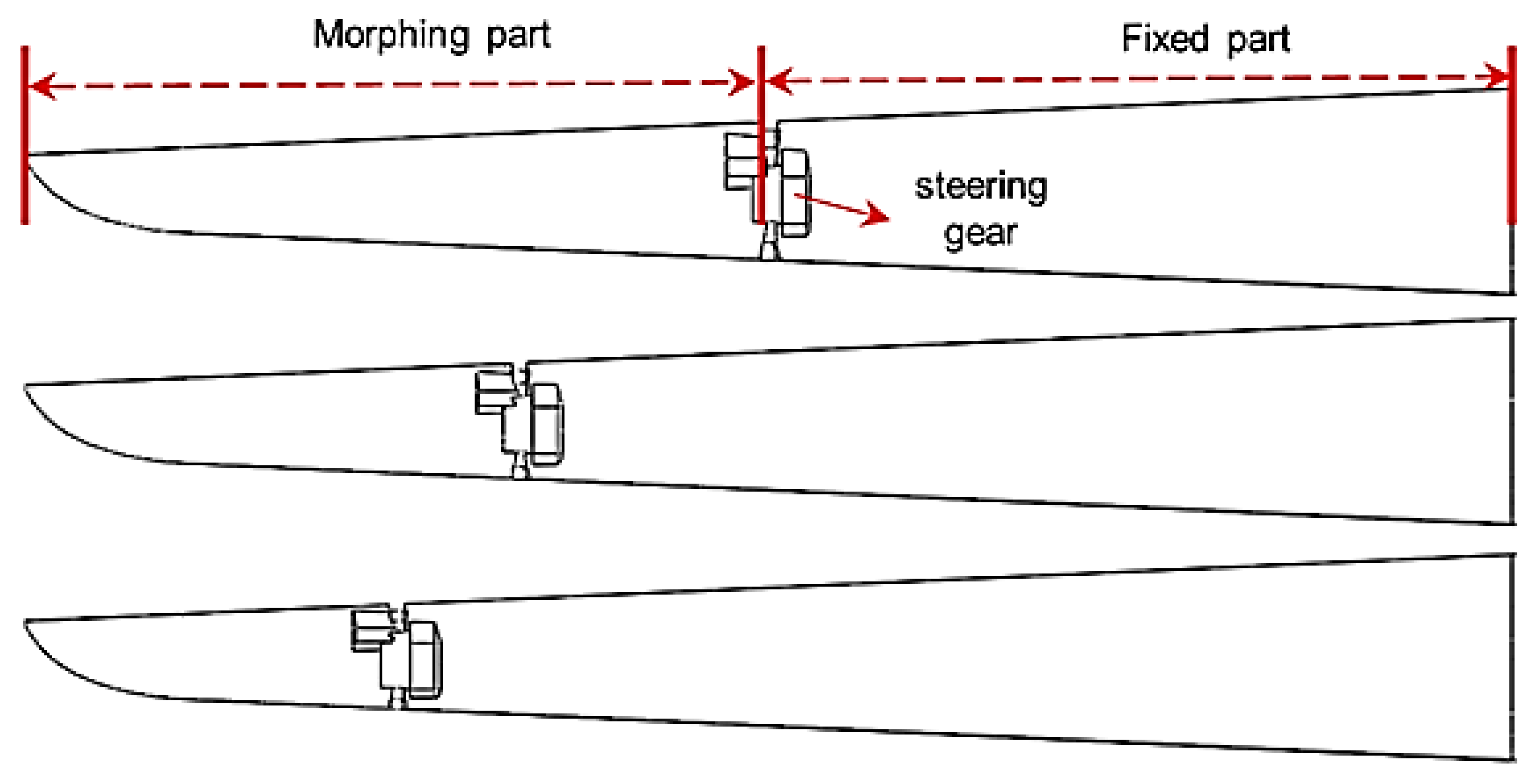
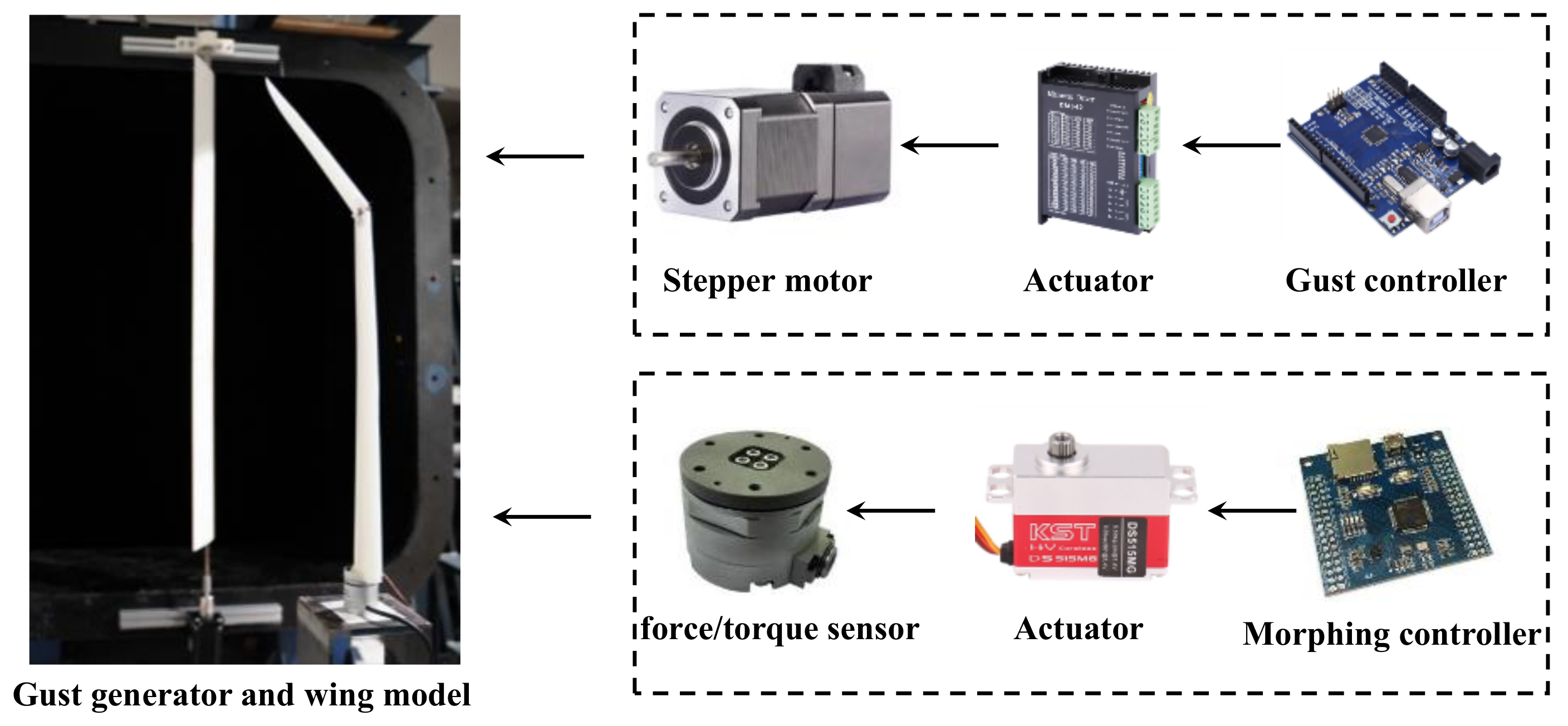
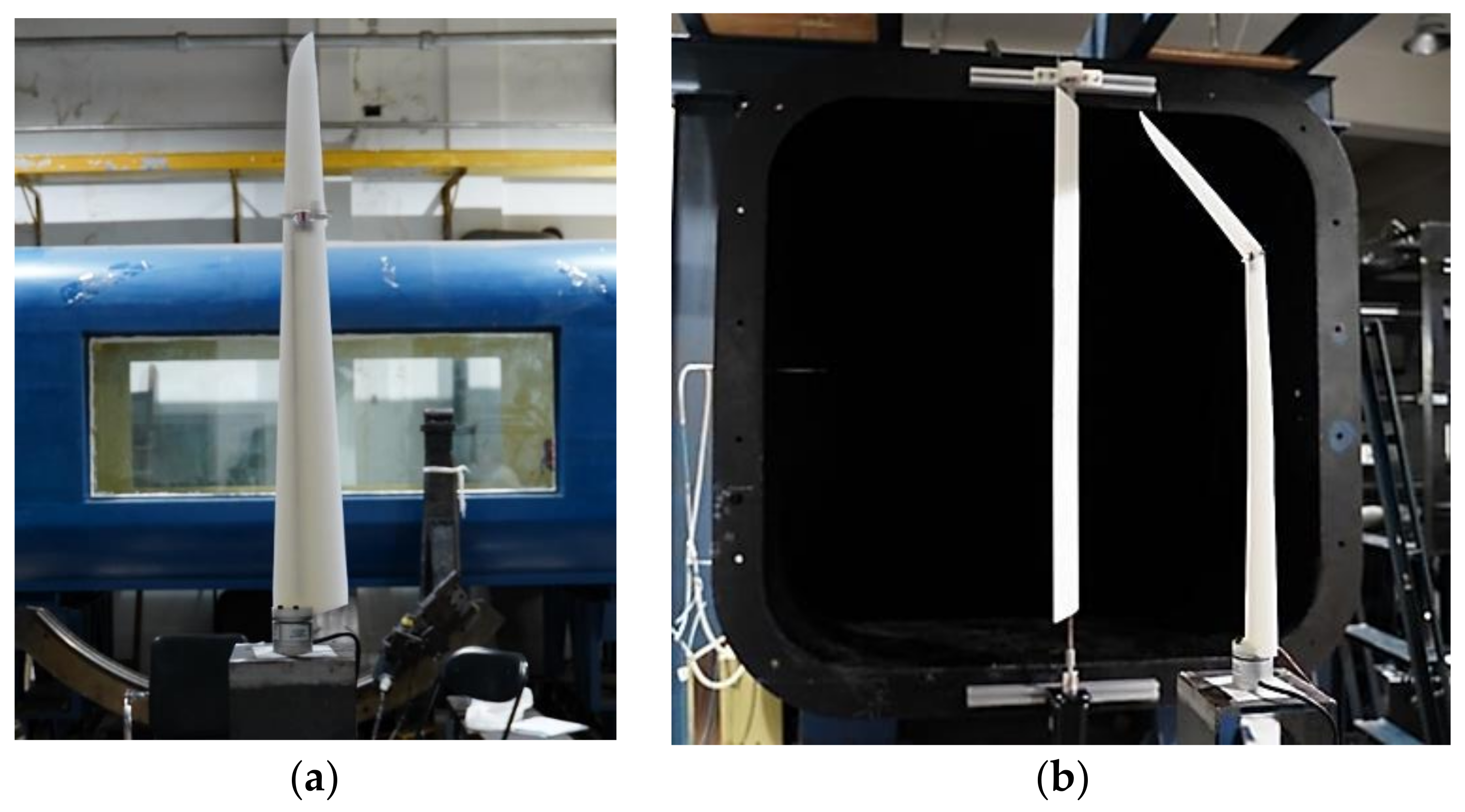
2.1. Wind Tunnel Test Model
2.2. Test Process and Data Processing Method
2.2.1. Test Process
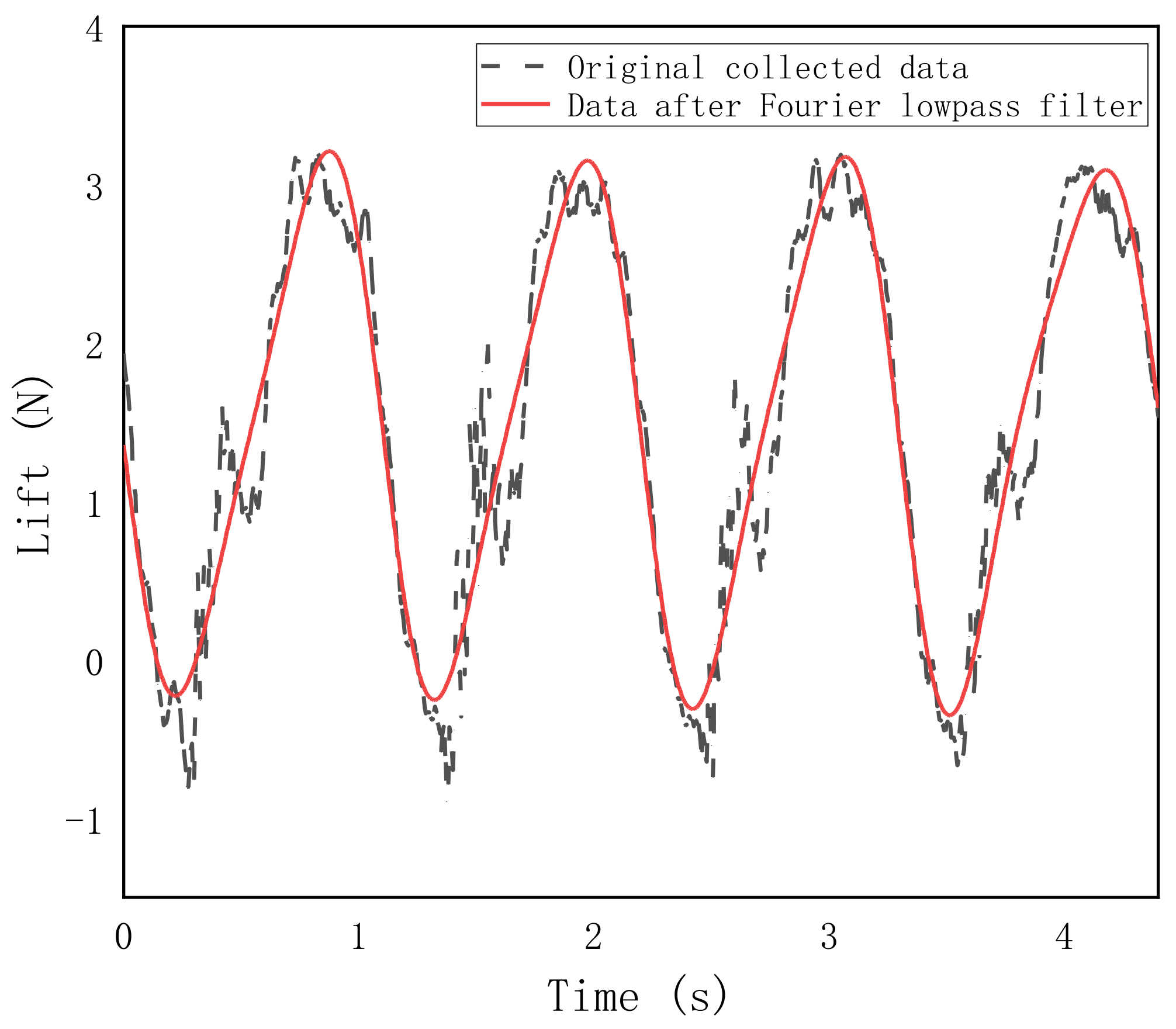
2.2.2. Data Processing
2.3. Selection Reasons of the Testing Parameters
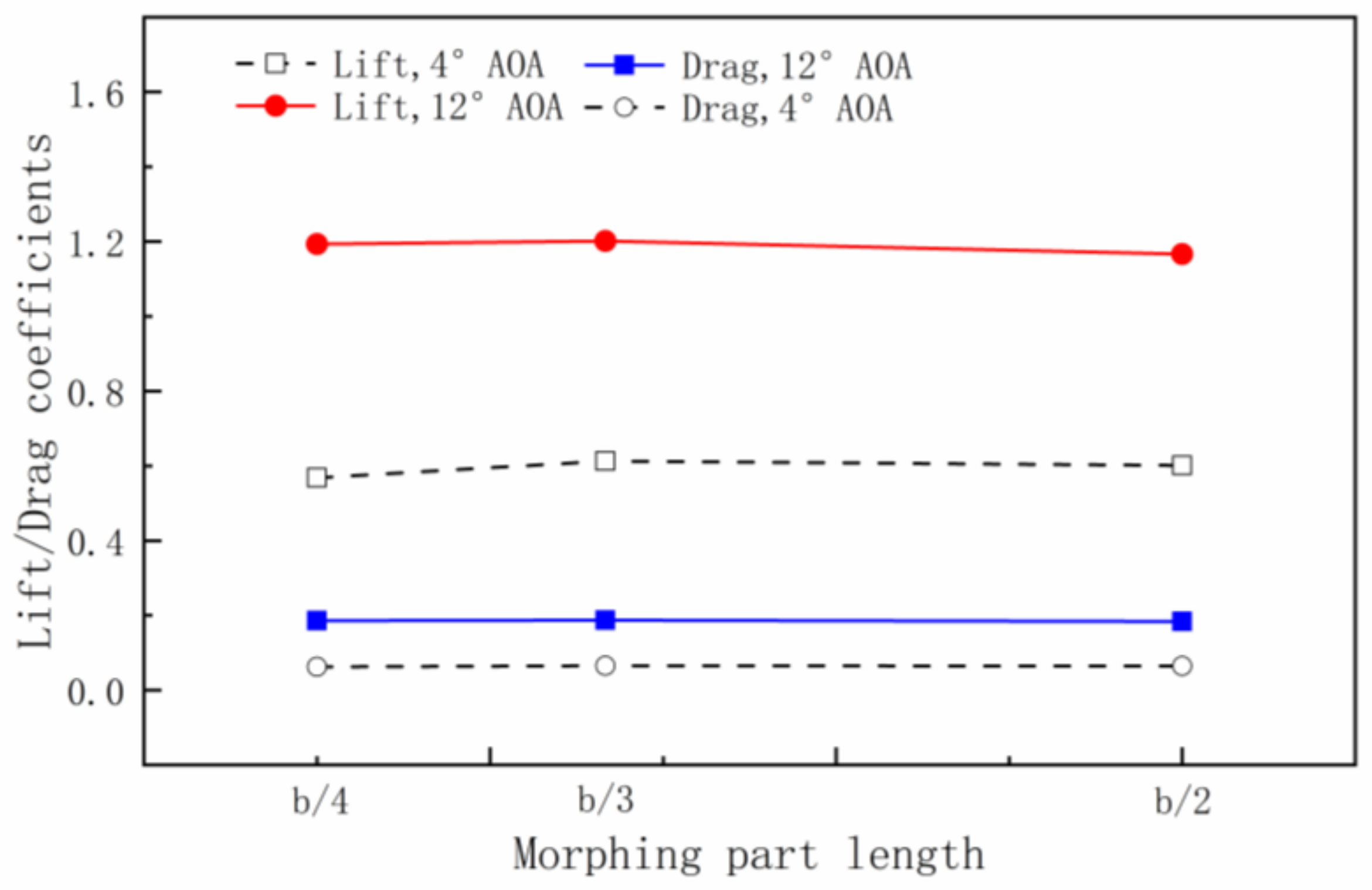
2.3.1. Morphing Part Length
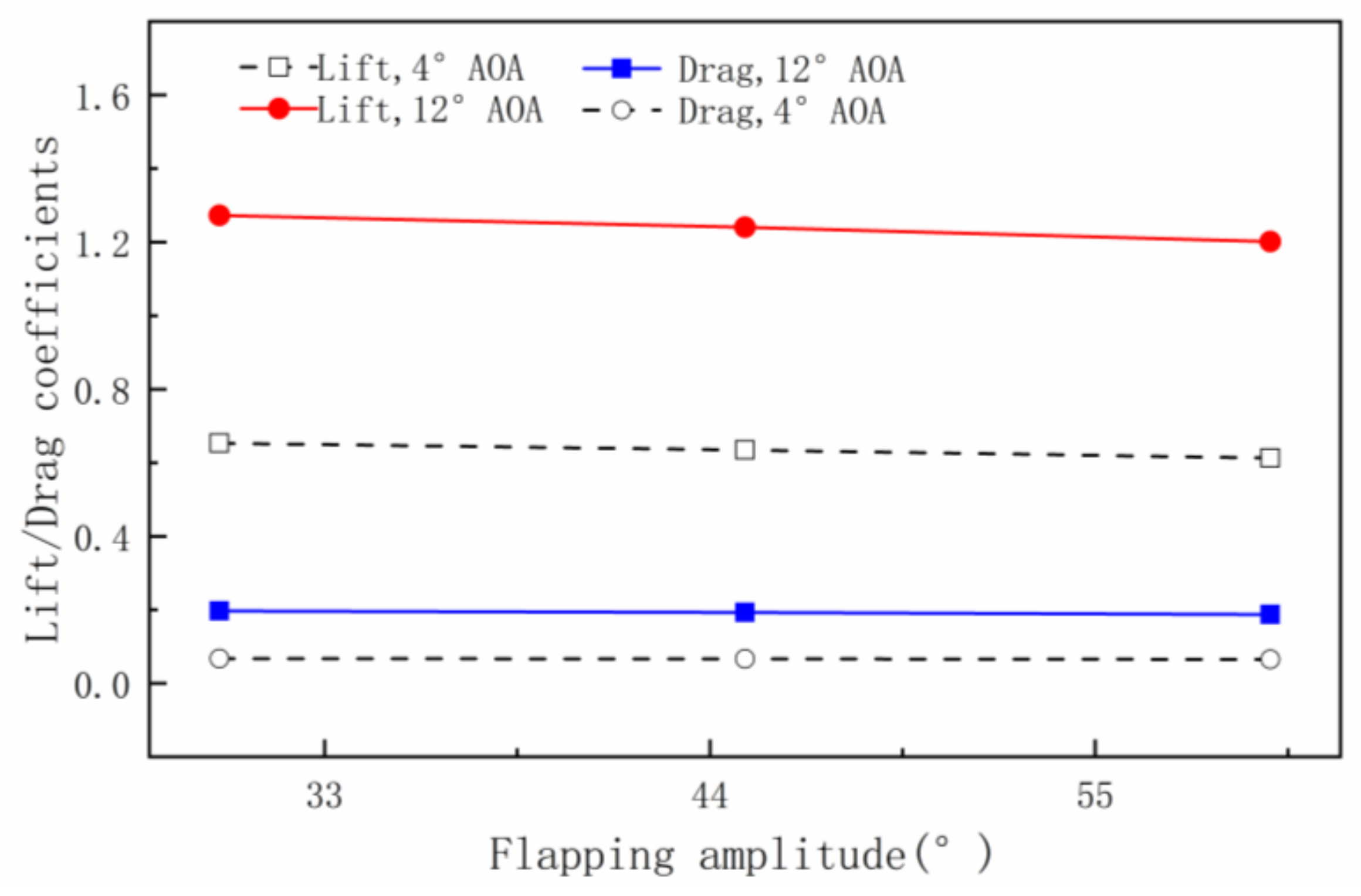
2.3.2. Flapping Amplitude
2.3.3. Morphing Frequency
3. Results and Discussions
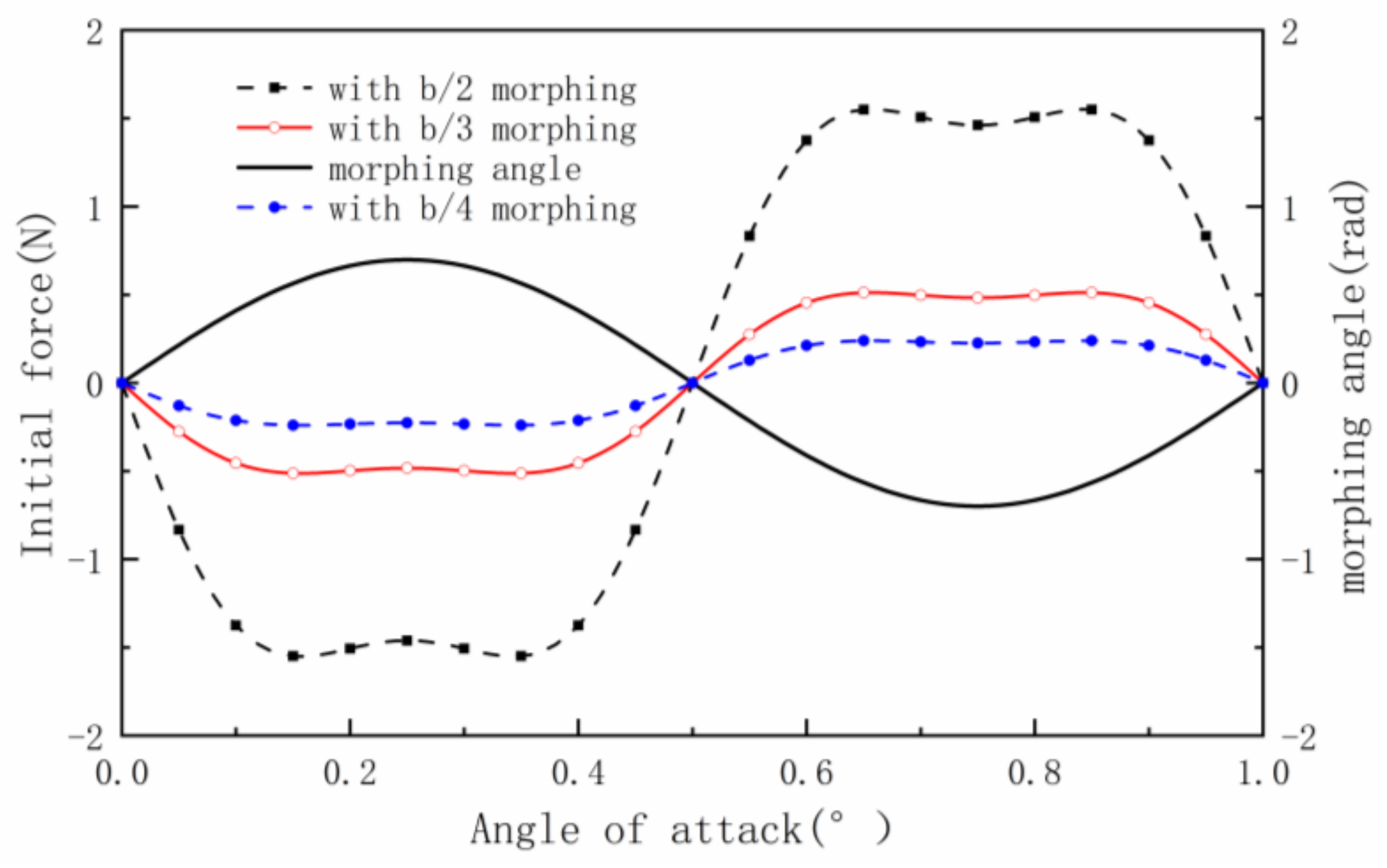
3.1. Influence of Morphing Parameters
3.1.1. The Gust Frequency Is Equal to the Morphing Frequency
3.1.2. The Gust Frequency Is Different from the Morphing Frequency
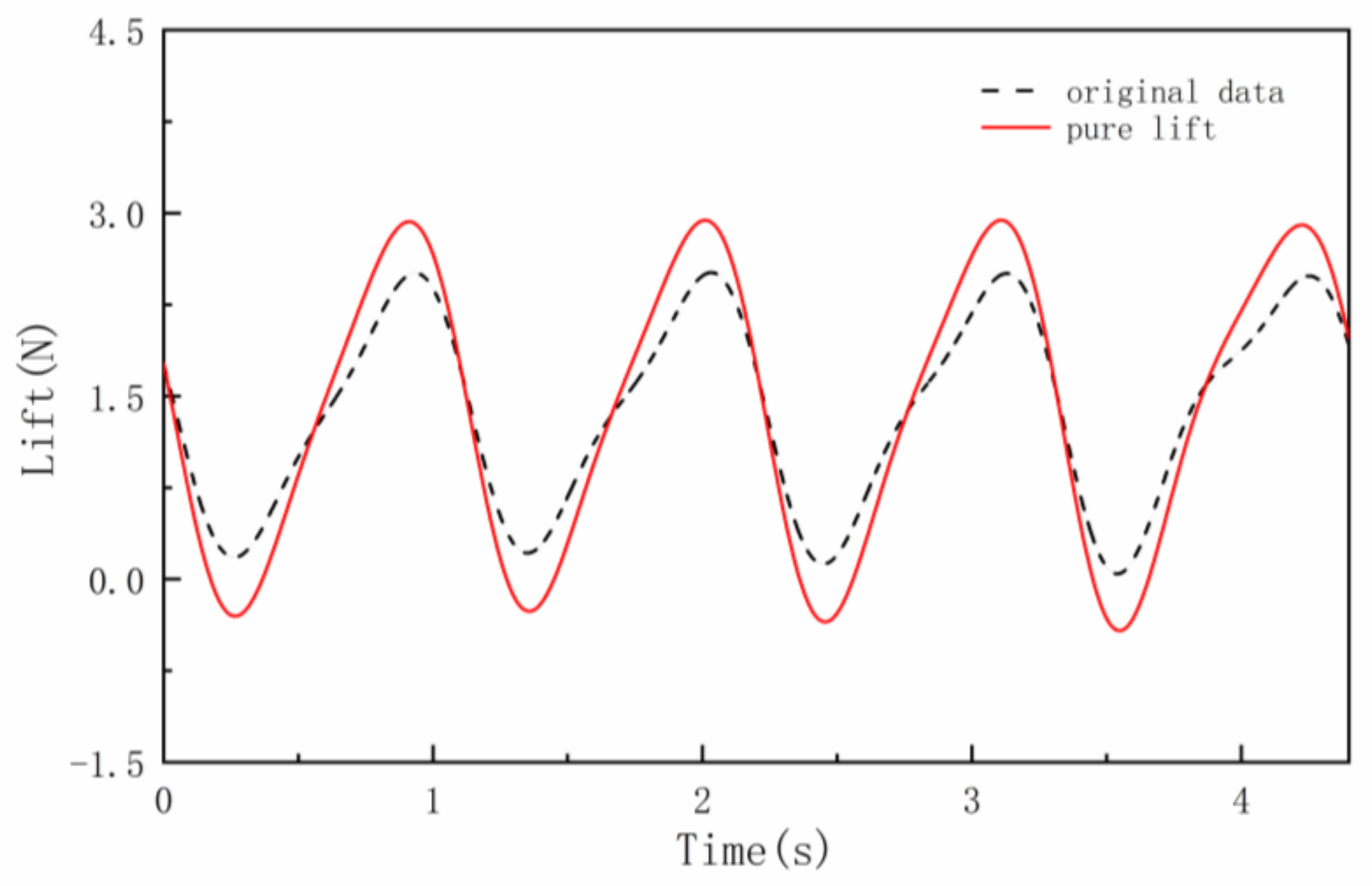
3.2. Gust Alleviation Effect of Instantaneous Lift Force
3.3. Numerical Results and Analysis
3.3.1. Numerical Simulation Model
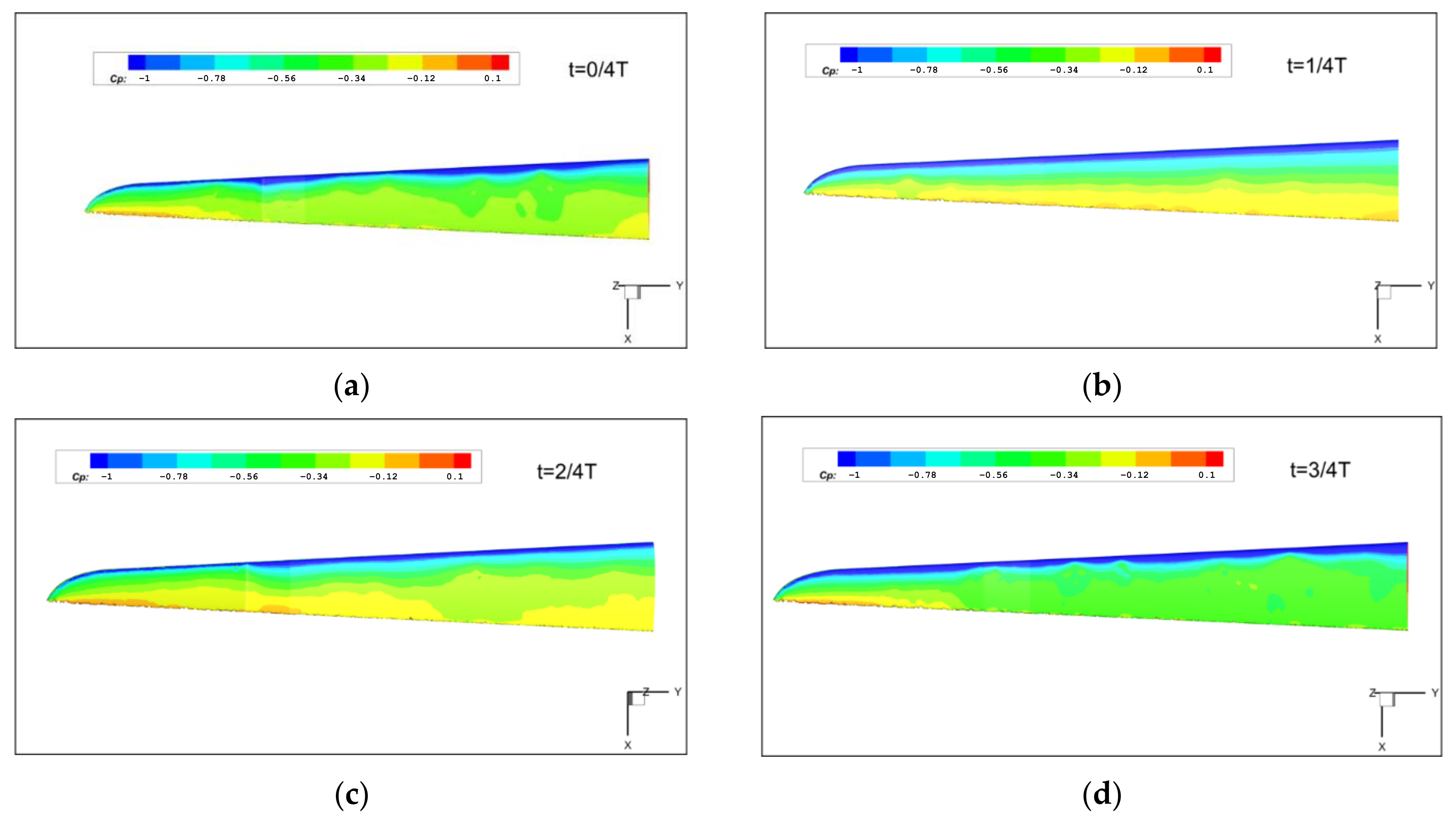
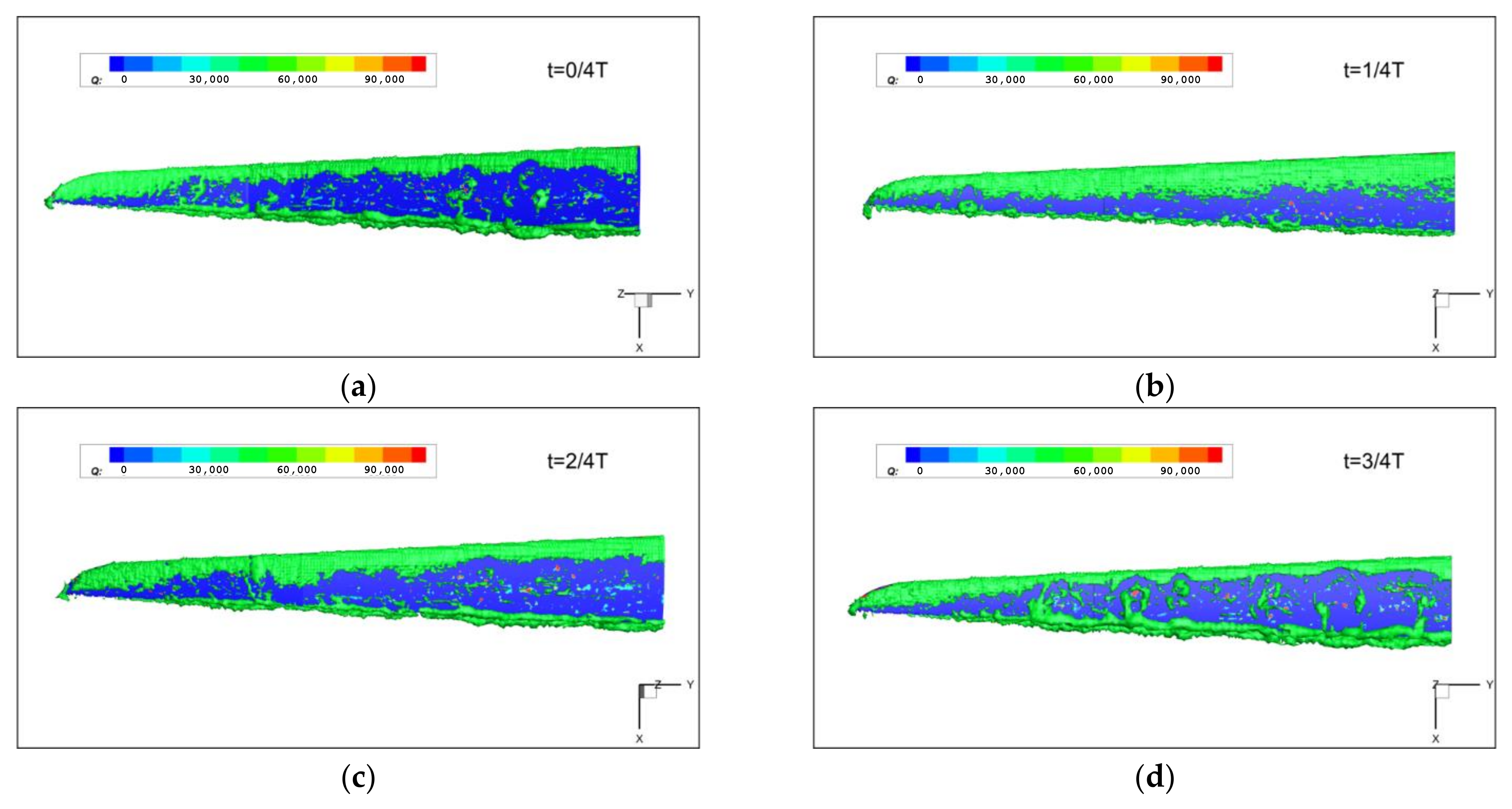
3.3.2. Pressure and Flow Field Analysis
4. Conclusions
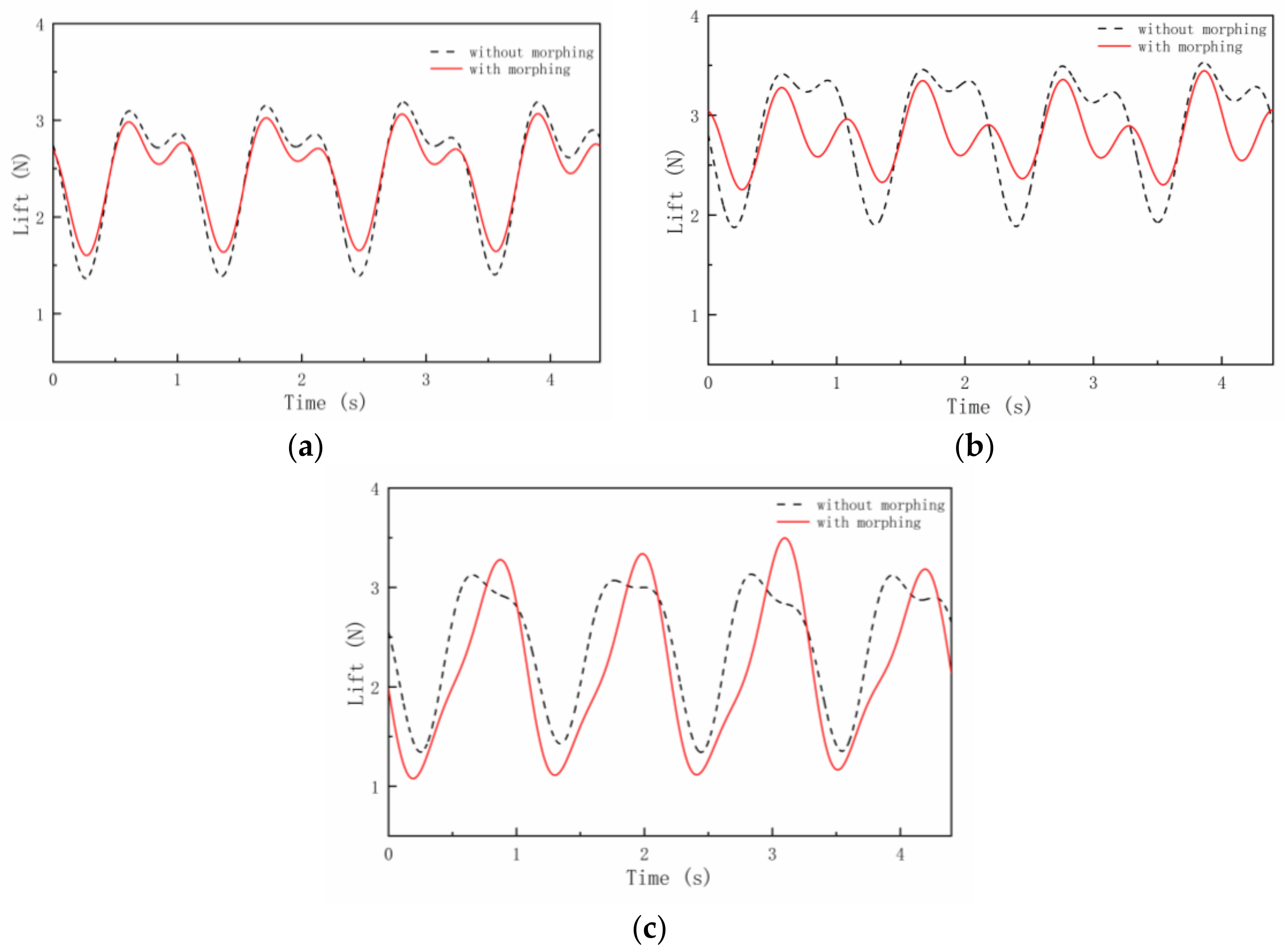
- The wind tunnel results of the spanwise morphing wing show that when the gust frequency and morphing frequency are equal, the wing with a b/3 morphing part length has the greatest lift but also produces greater drag. When the gust frequency is greater than the morphing frequency, the lift force of the gust alleviation is very close to the lift force of the gust response, and the lift curves with different morphing part lengths cross with each other, but the drag forces all increase. When the gust frequency is less than the morphing frequency, the spanwise morphing wing not only faces difficulty in achieving the effect of gust alleviation but also has the possibility of deterioration.
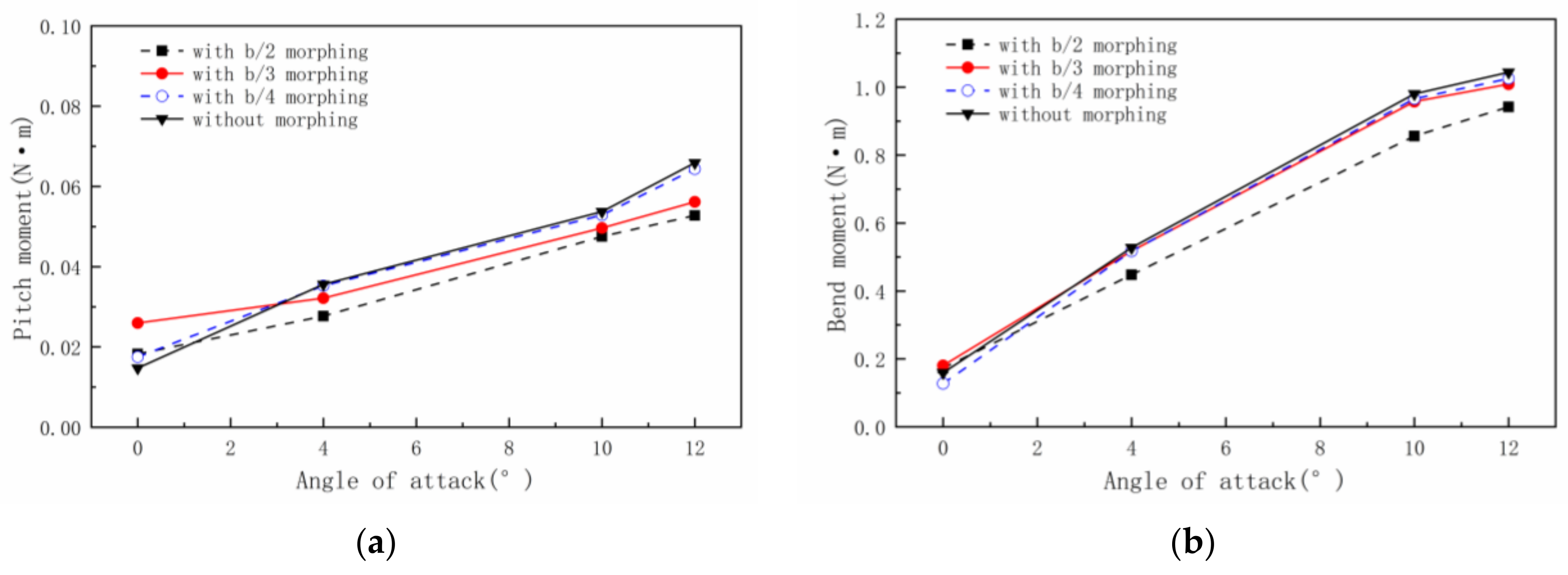
- The results of the instantaneous lift force show that the lift fluctuation is more stable when the morphing part length is larger and the AOA is small. When the AOA is large, the appropriate morphing part length will also make the lift fluctuation stable. Therefore, the appropriate morphing part length can improve flight performance.
- The numerical results show that from the distribution of the pressure coefficient and the change in the vortex structure, it can be seen that the lift force decreases to the minimum in the downward flapping process and increases to the maximum in the upward flapping process. The lift force variation of the spanwise morphing wing is mainly affected by the distribution of the vortex structure at the trailing edge of the wing. In the process of morphing, the morphing part flaps down/up, making the lift increase/decrease. The upward flapping and downward flapping of the wing will simultaneously reduce the effective area of the wing and reduce the lift force. The change in lift force comes from the combined effect of the two.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, D.; Zhao, S.; Da Ronch, A.; Xiang, J.; Drofelnik, J.; Li, Y.; Zhang, L.; Wu, Y.; Kintscher, M.; Monner, H.P.; et al. A Review of Modelling and Analysis of Morphing Wings. Prog. Aerosp. Sci. 2018, 100, 46–62. [Google Scholar] [CrossRef]
- Li, Y.; Ge, W.; Zhou, J.; Zhang, Y.; Zhao, D.; Wang, Z.; Dong, D. Design and Experiment of Concentrated Flexibility-based Variable Camber Morphing Wing. Chin. J. Aeronaut. 2021, 5, 455–469. [Google Scholar] [CrossRef]
- Huang, R.; Zhou, X. Parameterized Fictitious Mode of Morphing Wing with Bilinear Hinge Stiffness. AIAA J. 2021, 59, 2641–2656. [Google Scholar] [CrossRef]
- Chen, Z.; Zhang, W.; Mou, J.; Zheng, K. Horizontal Take-off of an Insect-like FMAV based on Stroke Plane Modulation. Aircr. Eng. Aerosp. Technol. 2022, 94, 1068–1077. [Google Scholar] [CrossRef]
- Lee, S.-G.; Yang, H.-H.; Addo-Akoto, R.; Han, J.-H. Transition Flight Trajectory Optimization for a Flapping-Wing Micro Air Vehicle with Unsteady Vortex-Lattice Method. Aerospace 2022, 9, 660. [Google Scholar] [CrossRef]
- Carruthers, A.C.; Thomas, A.L.; Taylor, G.K. Automatic Aeroelastic Devices in the Wings of a Steppe Eagle Aquila Nipalensis. J. Exp. Biol. 2007, 210, 4136–4149. [Google Scholar] [CrossRef]
- Bie, D.; Li, D.; Xiang, J.; Li, H.; Kan, Z.; Sun, Y. Design, Aerodynamic Analysis and Test Flight of a Bat-Inspired Tailless Flapping Wing Unmanned Aerial Vehicle. Aerosp. Sci. Technol. 2021, 112, 106557. [Google Scholar] [CrossRef]
- Bie, D.; Li, D.; Li, H.; Kan, Z.; Tu, Z. Analytical Study on Lift Performance of a Bat-Inspired Foldable Flapping Wing: Effect of Wing Arrangement. Aerospace 2022, 9, 653. [Google Scholar] [CrossRef]
- Cheney, J.A.; Stevenson, J.P.; Durston, N.E.; Song, J.; Usherwood, J.R.; Bomphrey, R.J.; Windsor, S.P. Bird Wings Act as a Suspension System that Rejects Gusts. Proc. R. Soc. B Biol. Sci. 2020, 287, 20201748. [Google Scholar] [CrossRef]
- Richardson, P.L. How Do Albatrosses Fly Around the World without Flapping their Wings? Prog. Oceanogr. 2011, 88, 46–58. [Google Scholar] [CrossRef]
- Kan, Z.; Li, D.; Shen, T.; Xiang, J.; Zhang, L. Aerodynamic Characteristics of Morphing Wing with Flexible Leading-edge. Chin. J. Aeronaut. 2020, 33, 2610–2619. [Google Scholar] [CrossRef]
- Weisshaar, T.A. Morphing Aircraft Systems: Historical Perspectives and Future Challenges. J. Aircr. 2013, 50, 337–353. [Google Scholar] [CrossRef]
- Bashir, M.; Rajendran, P. Static Structural Analysis of a Variable Span Morphing Wing for Unmanned Aerial Vehicle. IOP Conf. Ser. Mater. Sci. Eng. 2018, 370, 012040. [Google Scholar] [CrossRef]
- Ajaj, R.M.; Saavedra Flores, E.I.; Friswell, M.I.; De la, O.F. Span Morphing Using the Compliant Spar. J. Aerosp. Eng. 2015, 28, 04014108. [Google Scholar] [CrossRef]
- Kan, Z.; Li, D.; Xiang, J.; Cheng, C. Delaying Stall of Morphing Wing by Periodic Trailing-Edge Deflection. Chin. J. Aeronaut. 2020, 33, 493–500. [Google Scholar] [CrossRef]
- Munday, P.M.; Taira, K.; Suwa, T.; Numata, D.; Asai, K. Nonlinear Lift on a Triangular Airfoil in Low-Reynolds Number Compressible Flow. J. Aircr. 2015, 52, 924–931. [Google Scholar] [CrossRef]
- Parancheerivilakkathil, M.S.; Haider, Z.; Ajaj, R.M.; Amoozgar, M. A Polymorphing Wing Capable of Span Extension and Variable Pitch. Aerospace 2022, 9, 205. [Google Scholar] [CrossRef]
- Boston, D.M.; Phillips, F.R.; Henry, T.C.; Arrieta, A.F. Spanwise Wing Morphing Using Multistable Cellular Metastructures. Extrem. Mech. Lett. 2022, 53, 101706. [Google Scholar] [CrossRef]
- Ajaj, R.M.; Friswell, M.I.; Bourchak, M.; Harasani, W. Span Morphing Using the GNATSpar Wing. Aerosp. Sci. Technol. 2016, 53, 38–46. [Google Scholar] [CrossRef]
- Ajaj, R.M.; Jankee, G.K. The Transformer Aircraft: A Multimission Unmanned Aerial Vehicle Capable of Symmetric and Asymmetric Span Morphing. Aerosp. Sci. Technol. 2018, 76, 512–522. [Google Scholar] [CrossRef]
- Yang, C.; Qiu, Q.; Zhou, Y.; Wu, Z. Review of Aircraft Gust Alleviation Technology. Act Aeronaut. Astronaut. Sin. 2022, 43, 527350. [Google Scholar]
- Cheung, R.C.; Rezgui, D.; Cooper, J.E.; Wilson, T. Testing of Folding Wingtip for Gust Load Alleviation of Flexible High-Aspect-Ratio Wing. J. Aircr. 2020, 57, 876–888. [Google Scholar] [CrossRef]
- Szczyglowski, C.P.; Neild, S.A.; Titurus, B.; Jiang, J.Z.; Coetzee, E. Passive Gust Loads Alleviation in a Truss-Braced Wing Using an Inerter-Based Device. J. Aircr. 2019, 56, 2260–2271. [Google Scholar] [CrossRef]
- Welstead, J.; Crouse, G.L. Segmented-Freewing Concept for Gust Alleviation. J. Aircr. 2015, 47, 1047–1059. [Google Scholar] [CrossRef]
- He, S.; Guo, S.; Liu, Y.; Luo, W. Passive Gust Alleviation of a Flying-wing Aircraft by Analysis and Wind-tunnel Test of a Scaled Model in Dynamic Similarity. Aerosp. Sci. Technol. 2021, 113, 106689. [Google Scholar] [CrossRef]
- Chowdhury, J.; Ringuette, M.J. Effect of a Rotating and Swept Wingtip on Streamwise Gust Alleviation. AIAA J. 2021, 59, 1–12. [Google Scholar] [CrossRef]
- Cheung, R.C.; Rezgui, D.; Cooper, J.E.; Wilson, T. Testing of a Hinged Wingtip Device for Gust Loads Alleviation. J. Aircr. 2018, 55, 2050–2067. [Google Scholar] [CrossRef]
- Castrichini, A.; Hodigere Siddaramaiah, V.; Calderon, D.E.; Cooper, J.E.; Wilson, T.; Lemmens, Y. Nonlinear Folding Wing Tips for Gust Loads Alleviation. J. Aircr. 2016, 53, 1391–1399. [Google Scholar] [CrossRef]
- Guo, S.; Jing, Z.W.; Li, H.; Lei, W.T.; He, Y.Y. Gust Response and Body Freedom Flutter of a Flying-wing Aircraft with a Passive Gust Alleviation Device. Aerosp. Sci. Technol. 2017, 70, 277–285. [Google Scholar] [CrossRef]
- Guo, S.; De Los Monteros, J.E.; Liu, Y. Gust Alleviation of a Large Aircraft with a Passive Twist Wingtip. Aerospace 2015, 2, 135–154. [Google Scholar] [CrossRef]









| Morphing Part Length | Angle of Attack | Gust Model | Morphing Parameters |
|---|---|---|---|
| b/2 | 0° | G1(deflection frequency 1 Hz) | Frequency: 1 Hz Amplitude: 40° |
| b/3 | 4° | G2(deflection frequency 0.5 Hz) | |
| 10° | |||
| b/4 | 12° | G3(deflection frequency 2 Hz) |
| Morphing Part Length | Weight m (g) | Distance to the Rotation Axis r (mm) |
|---|---|---|
| b/2 | 55.38 | 127.5 |
| b/3 | 27.31 | 85.4 |
| b/4 | 17.08 | 63.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, Z.; Kan, Z.; Li, D. Gust Response of Spanwise Morphing Wing by Simulation and Wind Tunnel Testing. Aerospace 2023, 10, 328. https://doi.org/10.3390/aerospace10040328
Yao Z, Kan Z, Li D. Gust Response of Spanwise Morphing Wing by Simulation and Wind Tunnel Testing. Aerospace. 2023; 10(4):328. https://doi.org/10.3390/aerospace10040328
Chicago/Turabian StyleYao, Zhuoer, Zi Kan, and Daochun Li. 2023. "Gust Response of Spanwise Morphing Wing by Simulation and Wind Tunnel Testing" Aerospace 10, no. 4: 328. https://doi.org/10.3390/aerospace10040328
APA StyleYao, Z., Kan, Z., & Li, D. (2023). Gust Response of Spanwise Morphing Wing by Simulation and Wind Tunnel Testing. Aerospace, 10(4), 328. https://doi.org/10.3390/aerospace10040328







