Analyzing the Effect of Dynamic Pressure Drop on Corona Discharges for Aircraft Applications
Abstract
:1. Introduction
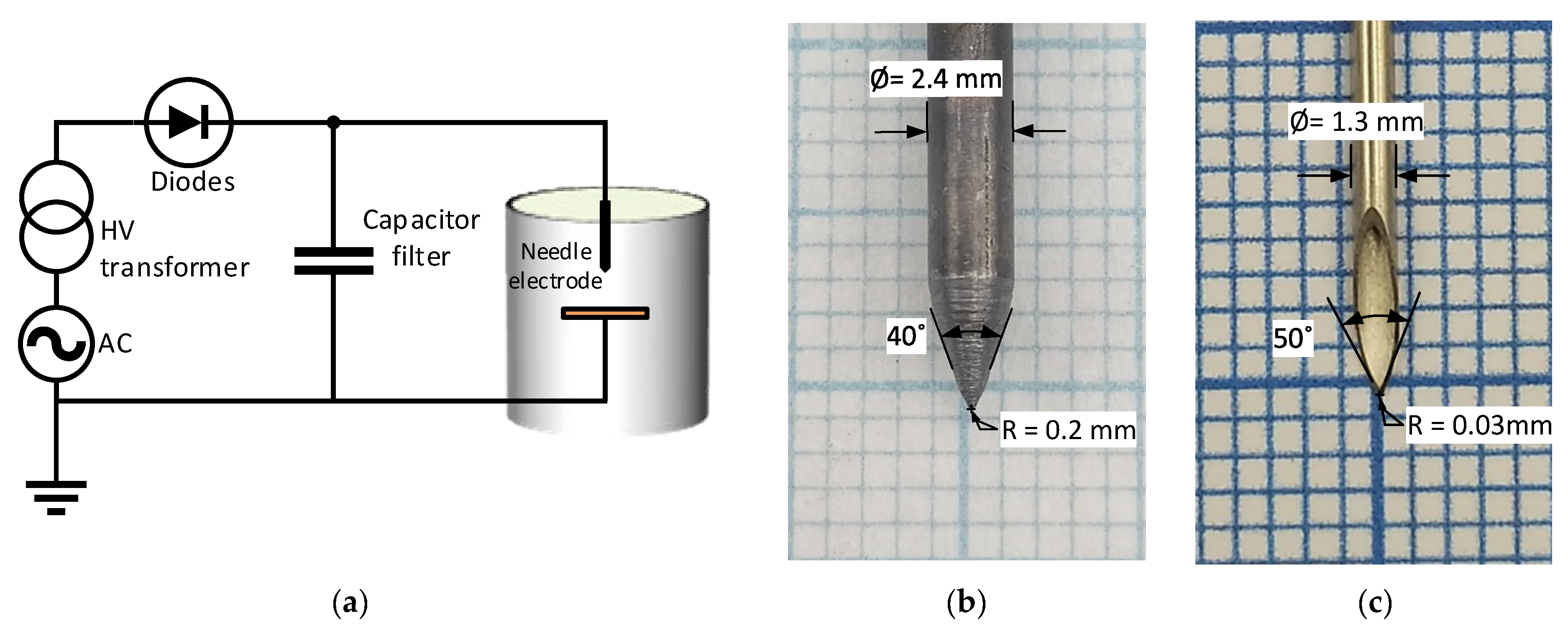
2. Experimental Setup
3. Experimental Method
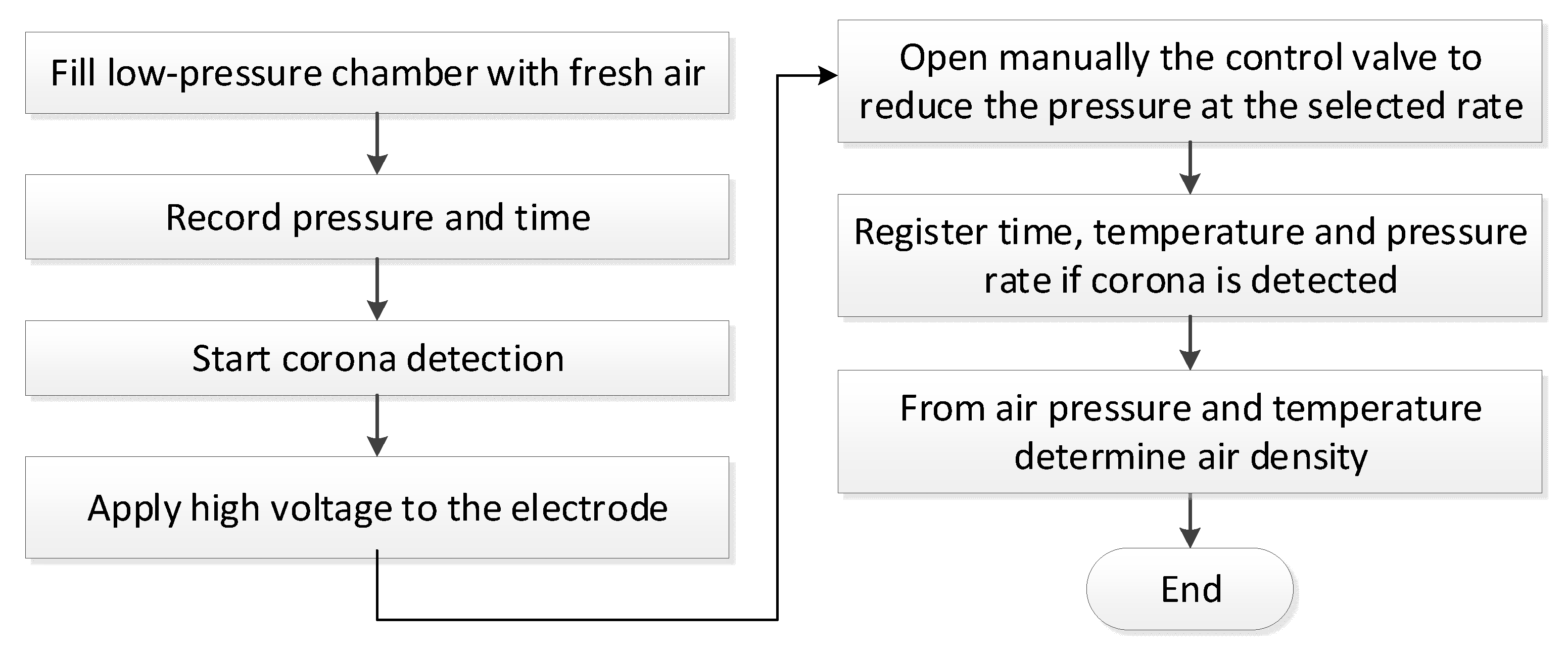
3.1. Experimental Process
3.2. Relationship between Pressure and Air Density
4. Experimental Results
4.1. Uncertainty Calculation
4.1.1. Dynamic Pressure Tests
4.1.2. Static Pressure Tests
4.2. Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- EPA. Inventory of U.S. Greenhouse Gas Emissions and Sinks: 1990–2020; EPA: Washington, DC, USA, 2022.
- European Commission. Reducing Emissions from Aviation. 2023. Available online: https://climate.ec.europa.eu/eu-action/transport-emissions/reducing-emissions-aviation_en (accessed on 25 January 2023).
- Borghei, M.; Ghassemi, M. Insulation Materials and Systems for More- and All-Electric Aircraft: A Review Identifying Challenges and Future Research Needs. IEEE Trans. Transp. Electrif. 2021, 7, 1930–1953. [Google Scholar] [CrossRef]
- Riba, J.-R.; Gómez-Pau, Á.; Moreno-Eguilaz, M. Experimental Study of Visual Corona under Aeronautic Pressure Conditions Using Low-Cost Imaging Sensors. Sensors 2020, 20, 411. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sumsurooah, S.; He, Y.; Torchio, M.; Kouramas, K.; Guida, B.; Cuomo, F.; Atkin, J.; Bozhko, S.; Renzetti, A.; Russo, A.; et al. ENIGMA—A Centralised Supervisory Controller for Enhanced Onboard Electrical Energy Management with Model in the Loop Demonstration. Energies 2021, 14, 5518. [Google Scholar] [CrossRef]
- Cavallo, A.; Canciello, G.; Russo, A. Buck-Boost Converter Control for Constant Power Loads in Aeronautical Applications. In Proceedings of the 2018 IEEE Conference on Decision and Control (CDC), Miami, FL, USA, 17–19 December 2018; pp. 6741–6747. [Google Scholar] [CrossRef]
- Li, C.; Shahsavarian, T.; Baferani, M.; Ronzello, J.; Cao, Y. Surface discharge behaviors of high temperature insulation subjected to gas pressure variations in hybrid propulsion systems. In Proceedings of the 2020 IEEE Conference on Electrical Insulation and Dielectric Phenomena (CEIDP), East Rutherford, NJ, USA, 18–30 October 2020; pp. 288–291. [Google Scholar] [CrossRef]
- Shahsavarian, T.; Li, C.; Baferani, M.; Cao, Y. Surface discharge studies of insulation materials in aviation power system under DC voltage. In Proceedings of the 2020 IEEE Conference on Electrical Insulation and Dielectric Phenomena (CEIDP), East Rutherford, NJ, USA, 18–30 October 2020; pp. 271–274. [Google Scholar] [CrossRef]
- Riba, J.-R.; Gómez-Pau, Á.; Moreno-Eguilaz, M.; Bogarra, S. Arc Tracking Control in Insulation Systems for Aeronautic Applications: Challenges, Opportunities, and Research Needs. Sensors 2020, 20, 1654. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- FAA. Aircraft Electrical Wiring Interconnect System (EWIS) Best Practices; FAA: Washington, DC, USA, 2020.
- Shafiq, M.; Robles, G.; Kauhaniemi, K.; Stewart, B.; Lehtonen, M. Propagation Characteristics of Partial discharge Signals in Medium Voltage Branched Cable Joints using HFCT Sensor. In CIRED 2019 Conference; CIRED: Madrid, Spain, 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Kuffel, J.; Zaengl, W.; Kuffel, P. High Voltage Engineering Fundamentals, 2nd ed.; Newnes: Oxford, UK, 2000; Available online: http://www.amazon.com/Voltage-Engineering-Fundamentals-Second-Edition/dp/0750636343 (accessed on 20 December 2015).
- Riba, J.-R.; Gomez-Pau, A.; Moreno-Eguilaz, M. Sensor Comparison for Corona Discharge Detection Under Low Pressure Conditions. IEEE Sens. J. 2020, 20, 11698–11706. [Google Scholar] [CrossRef]
- Huang, S.; Ha, L.; Liu, Y. The altitude correction term of the corona loss on alternating-current transmission lines. Energy Rep. 2023, 9, 2149–2152. [Google Scholar] [CrossRef]
- Riba, J.-R.; Bas-Calopa, P.; Moreno-Eguilaz, M. Analysing the influence of geometry and pressure on corona discharges. Eur. J. Phys. 2022, 43, 055201. [Google Scholar] [CrossRef]
- Lusuardi, L.; Rumi, A.; Neretti, G.; Seri, P.; Cavallini, A. Assessing the severity of partial discharges in aerospace applications. In Proceedings of the 2019 IEEE Conference on Electrical Insulation and Dielectric Phenomena (CEIDP), Richland, WA, USA, 20–23 October 2019; pp. 267–270. [Google Scholar] [CrossRef] [Green Version]
- Muhr, M.; Schwarz, R. Experience with optical partial discharge detection. Mater. Sci. Pol. 2009, 27, 1139–1146. Available online: https://yadda.icm.edu.pl/yadda/element/bwmeta1.element.baztech-article-BPW7-0012-0020 (accessed on 11 June 2022).
- Zhao, Y.; Li, Y.; Li, K.; Han, D.; Qiu, Z.; Zhang, G. Emission Spectrum Analysis of Two Typical Partial Discharge Forms Under High Frequency Square Wave Voltages. IEEE Access 2020, 8, 219946–219954. [Google Scholar] [CrossRef]
- Czech, T.; Sobczyk, A.T.; Jaworek, A. Optical emission spectroscopy of point-plane corona and back-corona discharges in air. Eur. Phys. J. D 2011, 65, 459–474. [Google Scholar] [CrossRef]
- Janda, M.; Martišovitš, V.; Hensel, K.; Machala, Z. Generation of Antimicrobial NOx by Atmospheric Air Transient Spark Discharge. Plasma Chem. Plasma Process. 2016, 36, 767–781. [Google Scholar] [CrossRef]
- Riba, J.-R. Spectrum of Corona Discharges and Electric Arcs in Air under Aeronautical Pressure Conditions. Aerospace 2022, 9, 524. [Google Scholar] [CrossRef]
- Aircraft Performance Database. 2023. Available online: https://contentzone.eurocontrol.int/aircraftperformance/default.aspx? (accessed on 23 January 2023).
- The Engineering ToolBox. Available online: https://www.engineeringtoolbox.com/ (accessed on 23 January 2023).
- ISO 2533:1975; Standard Atmosphere. ISO: Geneva, Switzerland, 1975; pp. 1–11. Available online: http://www.iso.org/iso/catalogue_detail?csnumber=7472 (accessed on 3 January 2016).
- Wan, J.; Zhang, H.; Liu, F.; Lv, W.; Zhao, Y. Optimization of Aircraft Climb Trajectory considering Environmental Impact under RTA Constraints. J. Adv. Transp. 2020, 2020, 1–17. [Google Scholar] [CrossRef]
- Driessen, A.B.J.M.; Van Duivenbode, J.; Wouters, P.A.A.F. Operational conditions influencing the partial discharge performance of cables under low and medium vacuum. IEEE Trans. Dielectr. Electr. Insul. 2019, 26, 81–89. [Google Scholar] [CrossRef]
- 4-2013; IEEE Standard for High-Voltage Testing Techniques. IEEE: Piscataway, NJ, USA, 2013; pp. 1–213. [CrossRef]
- Bas-Calopa, P.; Riba, J.-R.; Moreno-Eguilaz, M. Measurement of Corona Discharges under Variable Geometry, Frequency and Pressure Environment. Sensors 2022, 22, 1856. [Google Scholar] [CrossRef] [PubMed]
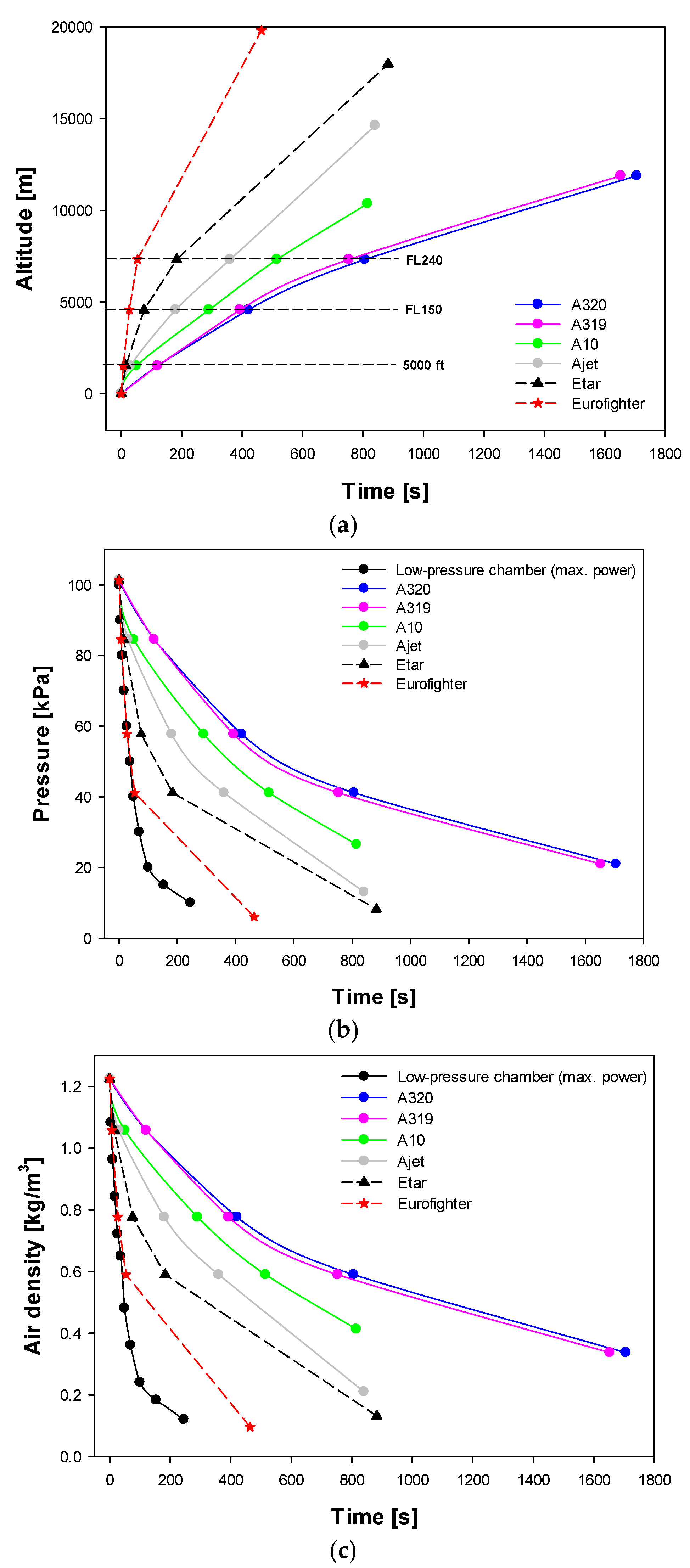



| Aircraft Model | Pressure Drop Rate at 4000 m [kPa·s−1] | Air Density Drop Rate at 4000 m [kg·m−3·s−1] | Ceiling Altitude [ft] | Pressure * [kPa] | Temperature * [°C] |
|---|---|---|---|---|---|
| B747-100 | −0.061 | −0.00066 | 45,000 | 14.8 | −56.5 |
| A320 | −0.081 | −0.00087 | 39,000 | 19.9 | −56.5 |
| B787-8 DL | −0.081 | −0.00087 | 43,000 | 16.3 | −56.5 |
| A319 | −0.089 | −0.00096 | 39,000 | 19.9 | −56.5 |
| B777-200 | −0.101 | −0.00109 | 43,000 | 16.3 | −56.5 |
| CRJ1 (RJ-100) | −0.101 | −0.00109 | 41,000 | 18.1 | −56.5 |
| A330-200 | −0.101 | −0.00109 | 41,000 | 18.1 | −56.5 |
| A380-800 | −0.101 | −0.00109 | 43,000 | 16.3 | −56.5 |
| B737-700 | −0.101 | −0.00109 | 41,000 | 18.1 | −56.5 |
| A10 Thunderbolt 2 | −0.101 | −0.00109 | 34,000 | 25.0 | −52.0 |
| Ajet (Alpha Jet) | −0.162 | −0.00175 | 48,000 | 12.8 | −56.5 |
| Etar (Étendard IV) | −0.406 | −0.00437 | 50,000 | 11.6 | −56.5 |
| Eurofighter | −1.218 | −0.01312 | 65,000 | 5.6 | −56.5 |
| Applied Voltage | Pressure Drop Rate [kPa/s] | Mean Air Density ρmean [kg/m3] | Standard Deviation σρ [kg/m3] | Instrument Uncertainty Δρa,instruments [%] | Delay Uncertainty Δρa,delay [%] | Standard Deviation Uncertainty Δρa,2σ [%] | Total Uncertainty Δρa [%] | |
|---|---|---|---|---|---|---|---|---|
| Electrode #1 placed 80 mm above ground | 3.07 kVRMS 400 Hz sinusoidal AC | −0.10 ± 0.09 | 0.856 | 0.006 | 0.8 | 0.1 | 1.4 | 1.6 |
| −0.30 ± 0.07 | 0.855 | 0.003 | 0.8 | 0.1 | 0.7 | 1.1 | ||
| −0.60 ± 0.14 | 0.850 | 0.008 | 0.8 | 0.2 | 1.9 | 2.1 | ||
| −1.00 ± 0.21 | 0.843 | 0.016 | 0.8 | 0.2 | 3.8 | 3.9 | ||
| 4.85 kV negative DC | −0.10 ± 0.09 | 0.825 | 0.010 | 0.8 | 0.2 | 2.4 | 2.6 | |
| −0.30 ± 0.13 | 0.825 | 0.014 | 0.8 | 0.2 | 3.4 | 3.5 | ||
| −0.60 ± 0.14 | 0.822 | 0.016 | 0.8 | 0.2 | 3.9 | 4.0 | ||
| −1.00 ± 0.36 | 0.810 | 0.014 | 0.8 | 0.3 | 3.5 | 3.6 | ||
| 5.74 kV positive DC | −0.10 ± 0.04 | 0.833 | 0.020 | 0.8 | 0.1 | 4.8 | 4.9 | |
| −0.30 ± 0.08 | 0.835 | 0.019 | 0.8 | 0.2 | 4.6 | 4.6 | ||
| −0.60 ± 0.15 | 0.833 | 0.021 | 0.8 | 0.2 | 5.0 | 5.1 | ||
| −1.00 ± 0.30 | 0.843 | 0.023 | 0.8 | 0.2 | 5.5 | 5.5 | ||
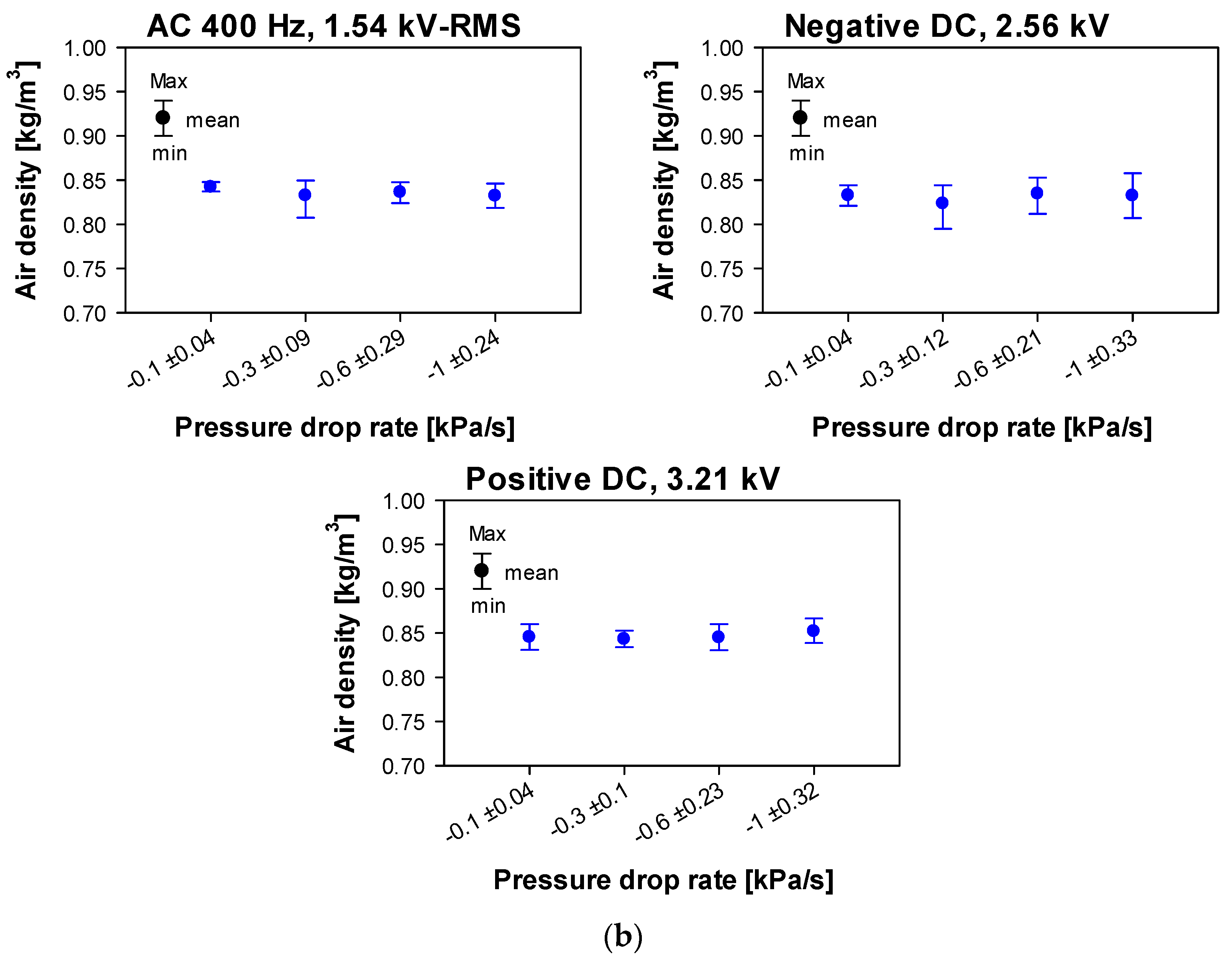
| Electrode #2 placed 40 mm above ground | 1.54 kVRMS 400 Hz sinusoidal AC | −0.10 ± 0.04 | 0.841 | 0.003 | 0.8 | 0.2 | 0.7 | 1.1 |
| −0.30 ± 0.09 | 0.840 | 0.012 | 0.8 | 0.2 | 2.9 | 3.0 | ||
| −0.60 ± 0.29 | 0.837 | 0.009 | 0.8 | 0.2 | 2.2 | 2.3 | ||
| −1.00 ± 0.24 | 0.832 | 0.010 | 0.8 | 0.3 | 2.4 | 2.5 | ||
| 2.56 kV negative DC | −0.10 ± 0.04 | 0.833 | 0.007 | 0.8 | 0.2 | 1.7 | 1.9 | |
| −0.30 ± 0.12 | 0.831 | 0.016 | 0.8 | 0.2 | 3.9 | 3.9 | ||
| −0.60 ± 0.21 | 0.839 | 0.011 | 0.8 | 0.2 | 2.6 | 2.8 | ||
| −1.00 ± 0.33 | 0.832 | 0.017 | 0.8 | 0.3 | 4.1 | 4.2 | ||
| 3.21 kV positive DC | −0.10 ± 0.04 | 0.845 | 0.010 | 0.8 | 0.2 | 2.4 | 2.5 | |
| −0.30 ± 0.10 | 0.842 | 0.006 | 0.8 | 0.2 | 1.4 | 1.6 | ||
| −0.60 ± 0.23 | 0.844 | 0.011 | 0.8 | 0.2 | 2.6 | 2.7 | ||
| −1.00 ± 0.32 | 0.850 | 0.010 | 0.8 | 0.3 | 2.4 | 2.5 |
| Applied Voltage | Mean Voltage Vmean [kV] | Standard Deviation σV [V] | Instruments Uncertainty ΔVa,instruments [%] | Step Uncertainty ΔVa,step [%] | Standard Deviation Uncertainty ΔVa,2σ [%] | Total Uncertainty ΔVa [%] | |
|---|---|---|---|---|---|---|---|
| Electrode #1 placed 80 mm above ground | 400 Hz AC | 3.09 (RMS) | 0.027 | 3.0 | 0.3 | 1.7 | 3.5 |
| Negative DC | 4.73 | 0.093 | 3.0 | 0.2 | 3.9 | 5.0 | |
| Positive DC | 5.63 | 0.055 | 3.0 | 0.2 | 2.0 | 3.6 | |
| Electrode #2 placed 40 mm above ground | 400 Hz AC | 1.58 (RMS) | 0.024 | 3.0 | 0.6 | 3.0 | 4.3 |
| Negative DC | 2.55 | 0.027 | 3.0 | 0.4 | 2.1 | 3.7 | |
| Positive DC | 3.11 | 0.025 | 3.0 | 0.3 | 1.6 | 3.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bas-Calopa, P.; Riba, J.-R.; Moreno-Eguilaz, M. Analyzing the Effect of Dynamic Pressure Drop on Corona Discharges for Aircraft Applications. Aerospace 2023, 10, 320. https://doi.org/10.3390/aerospace10030320
Bas-Calopa P, Riba J-R, Moreno-Eguilaz M. Analyzing the Effect of Dynamic Pressure Drop on Corona Discharges for Aircraft Applications. Aerospace. 2023; 10(3):320. https://doi.org/10.3390/aerospace10030320
Chicago/Turabian StyleBas-Calopa, Pau, Jordi-Roger Riba, and Manuel Moreno-Eguilaz. 2023. "Analyzing the Effect of Dynamic Pressure Drop on Corona Discharges for Aircraft Applications" Aerospace 10, no. 3: 320. https://doi.org/10.3390/aerospace10030320
APA StyleBas-Calopa, P., Riba, J.-R., & Moreno-Eguilaz, M. (2023). Analyzing the Effect of Dynamic Pressure Drop on Corona Discharges for Aircraft Applications. Aerospace, 10(3), 320. https://doi.org/10.3390/aerospace10030320








