A Multivariable Method for Calculating Failure Probability of Aeroengine Rotor Disk
Abstract
1. Introduction
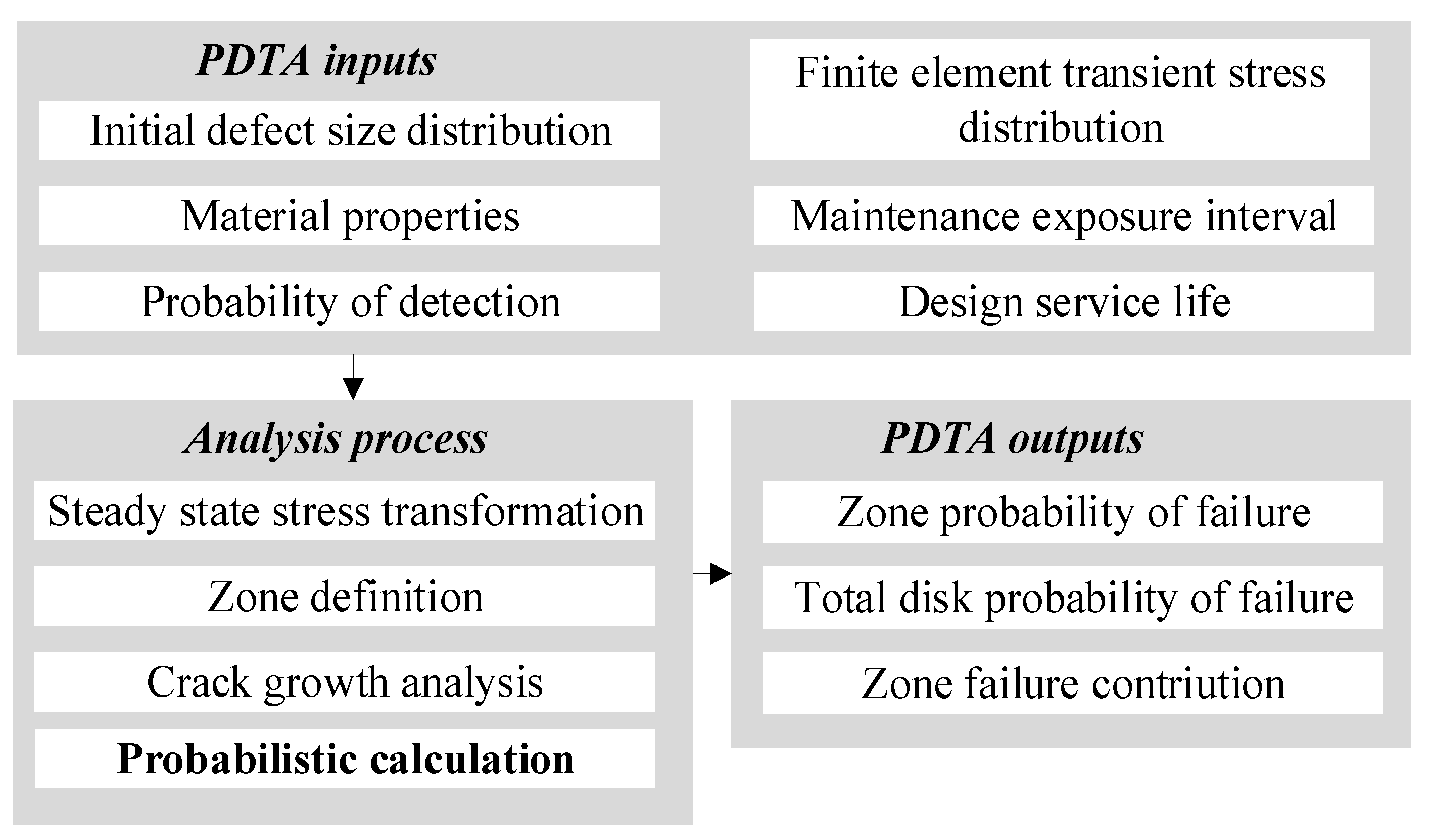
2. Multivariable Numerical Integration Method of Probabilistic Damage Tolerance Analysis
2.1. Multiple Random Variables Considered in the PDTA
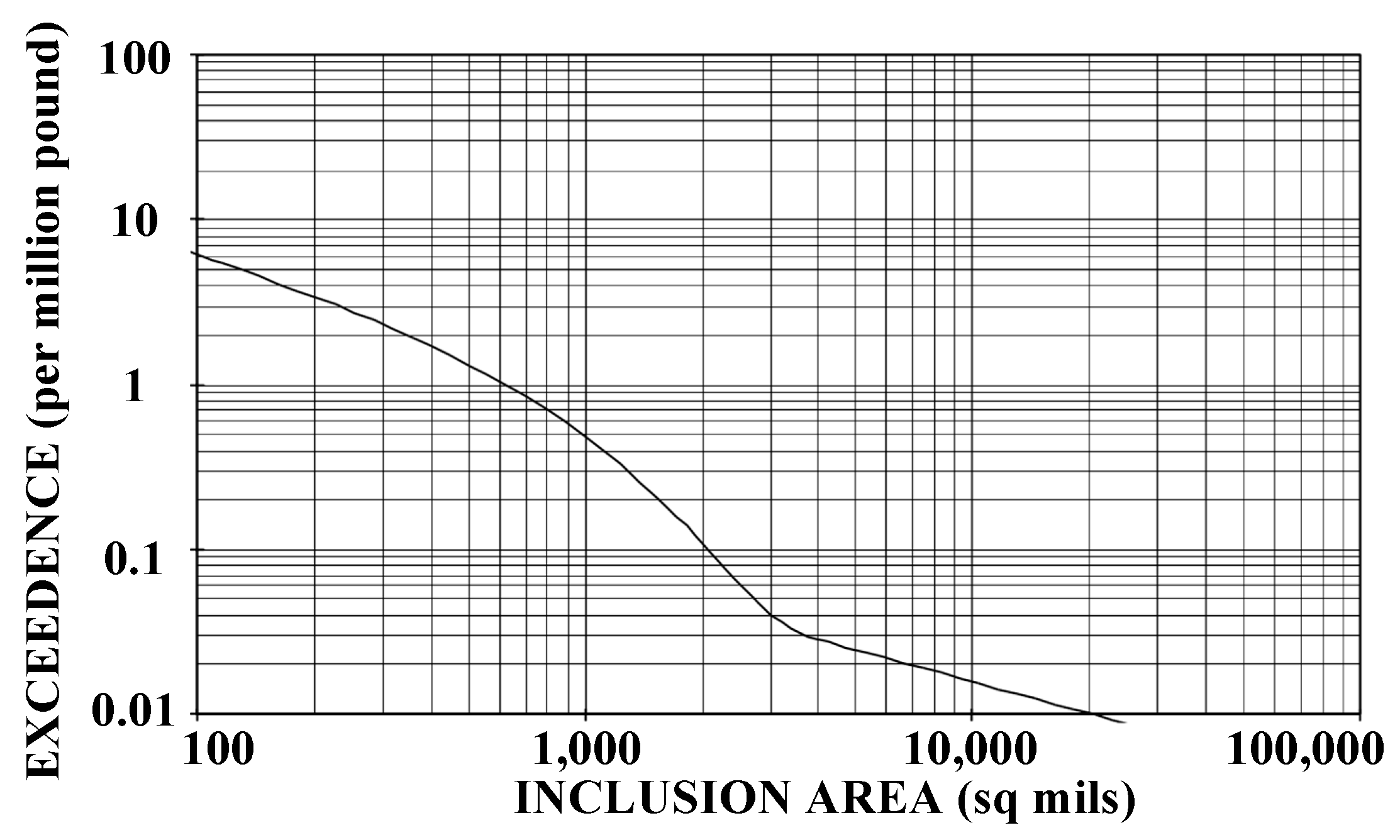
2.1.1. Initial Defect Size of the Material
2.1.2. Life Scatter Factor
2.1.3. Stress Scatter Factor
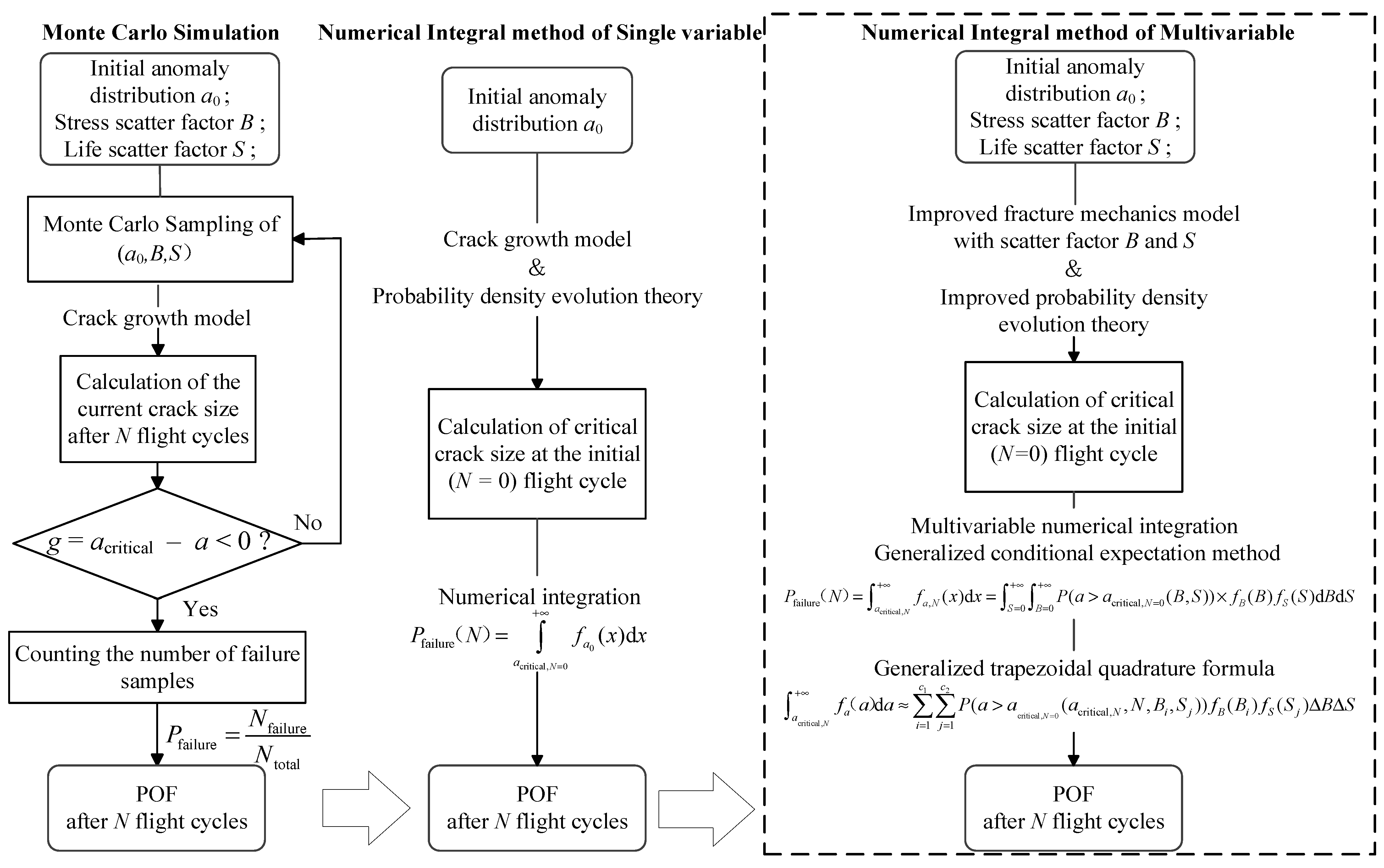
2.2. Mechanism of the Establishment of Multiple Integration in Multivariable PDTA
2.2.1. Probability Conservation and Spatial Transformation in the Theory of Probability Density Evolution
2.2.2. The Establishment of Multiple Integration in Multivariable PDTA
2.3. The Multivariable NI Method of PDTA
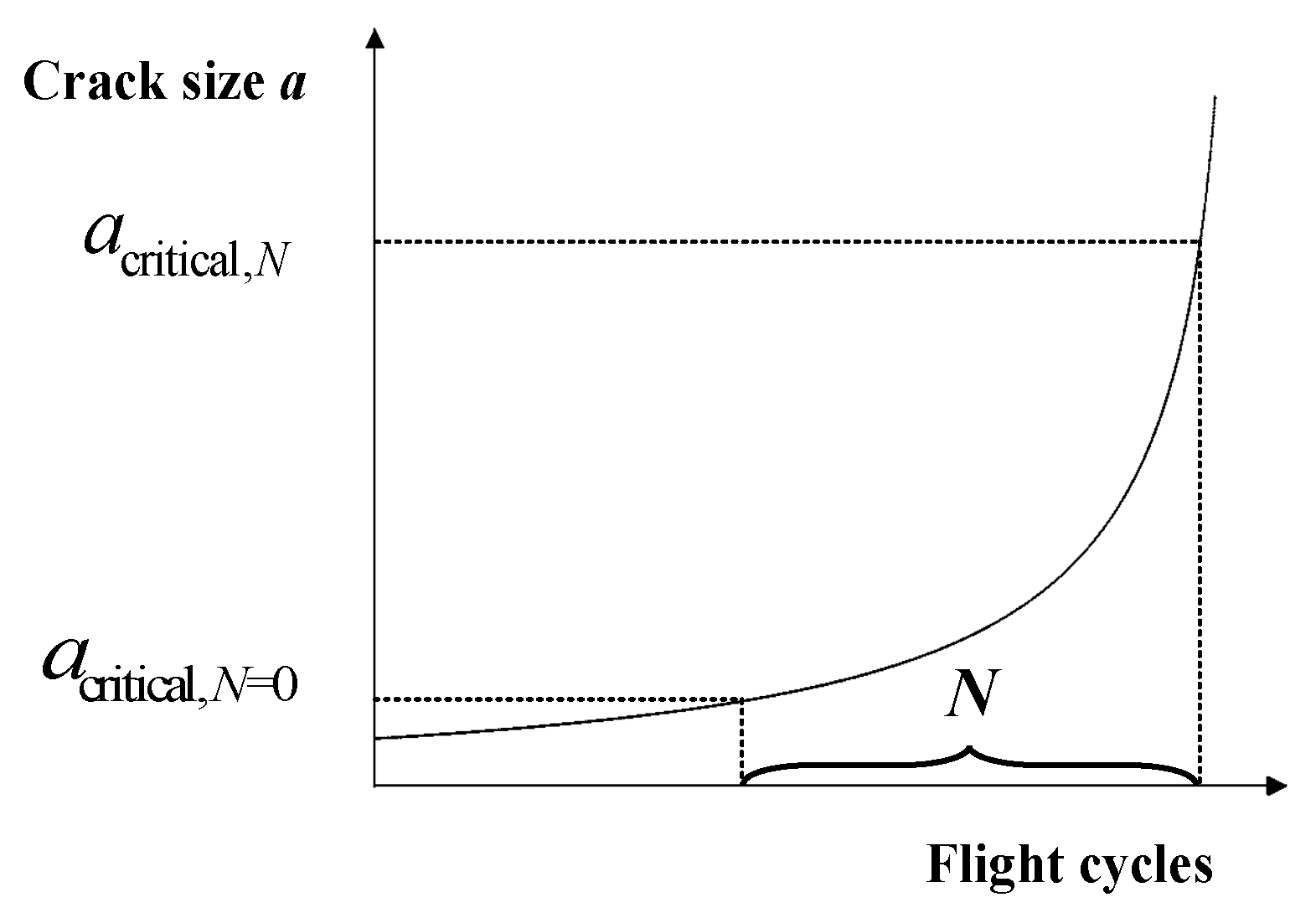
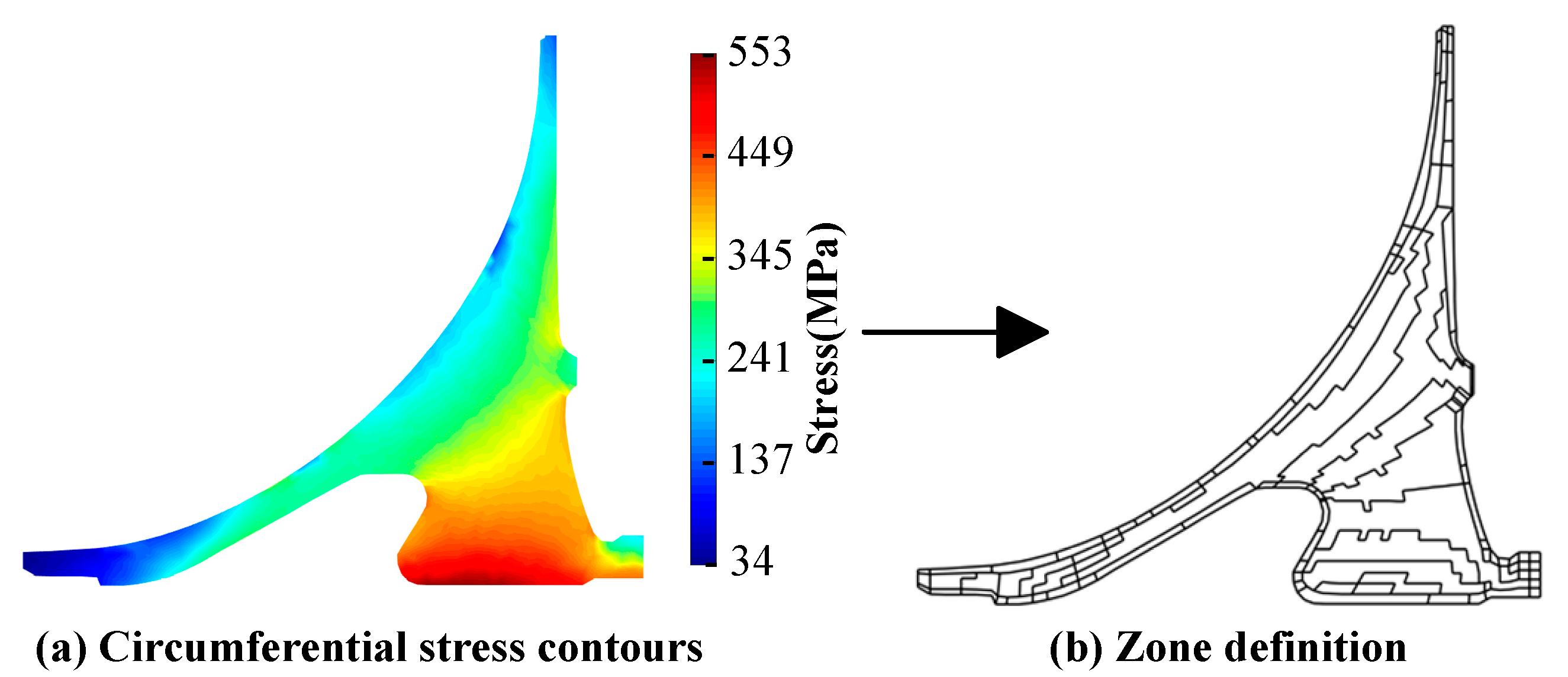
2.3.1. Zone and Disk POF Calculation Model
2.3.2. The Implementation Algorithm of the Multivariable NI Method
3. Computational Model and Inputs
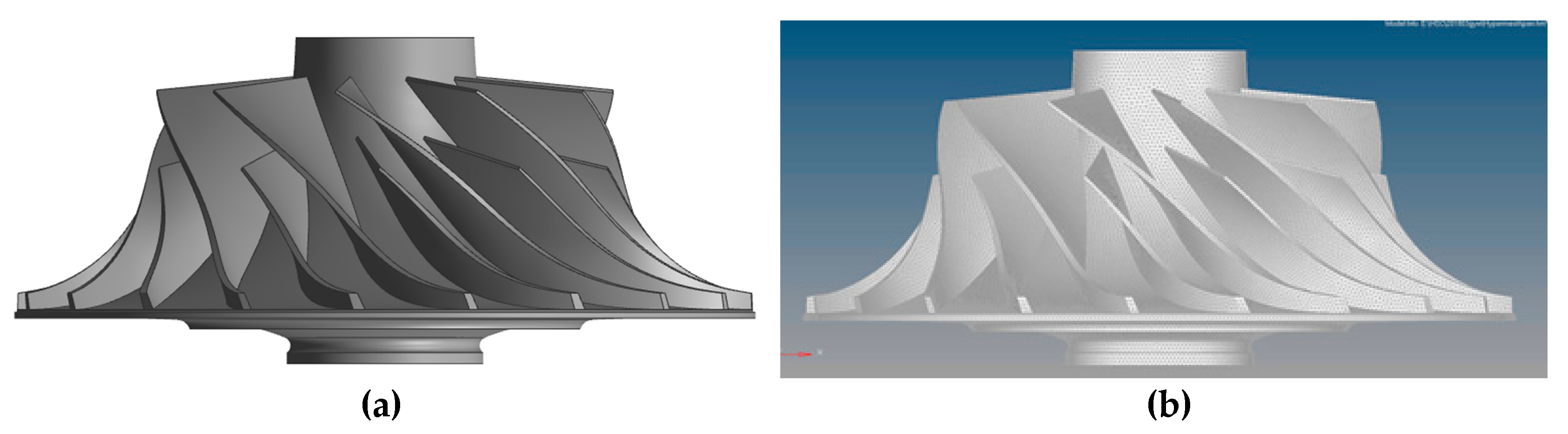
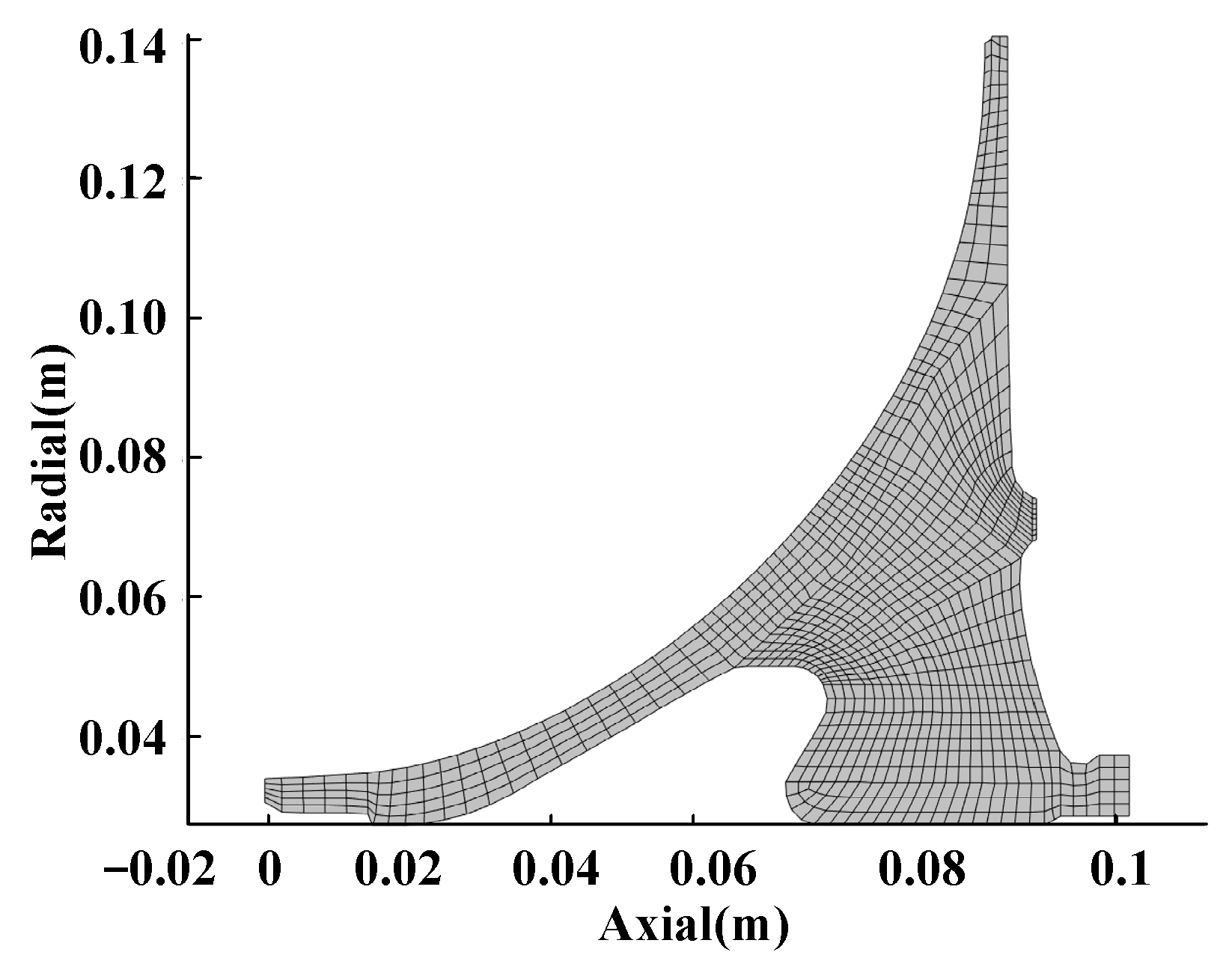
3.1. Computational Model
3.2. Inputs for the PDTA
3.3. Considered Cases for Converge Result
4. Results and Discussion
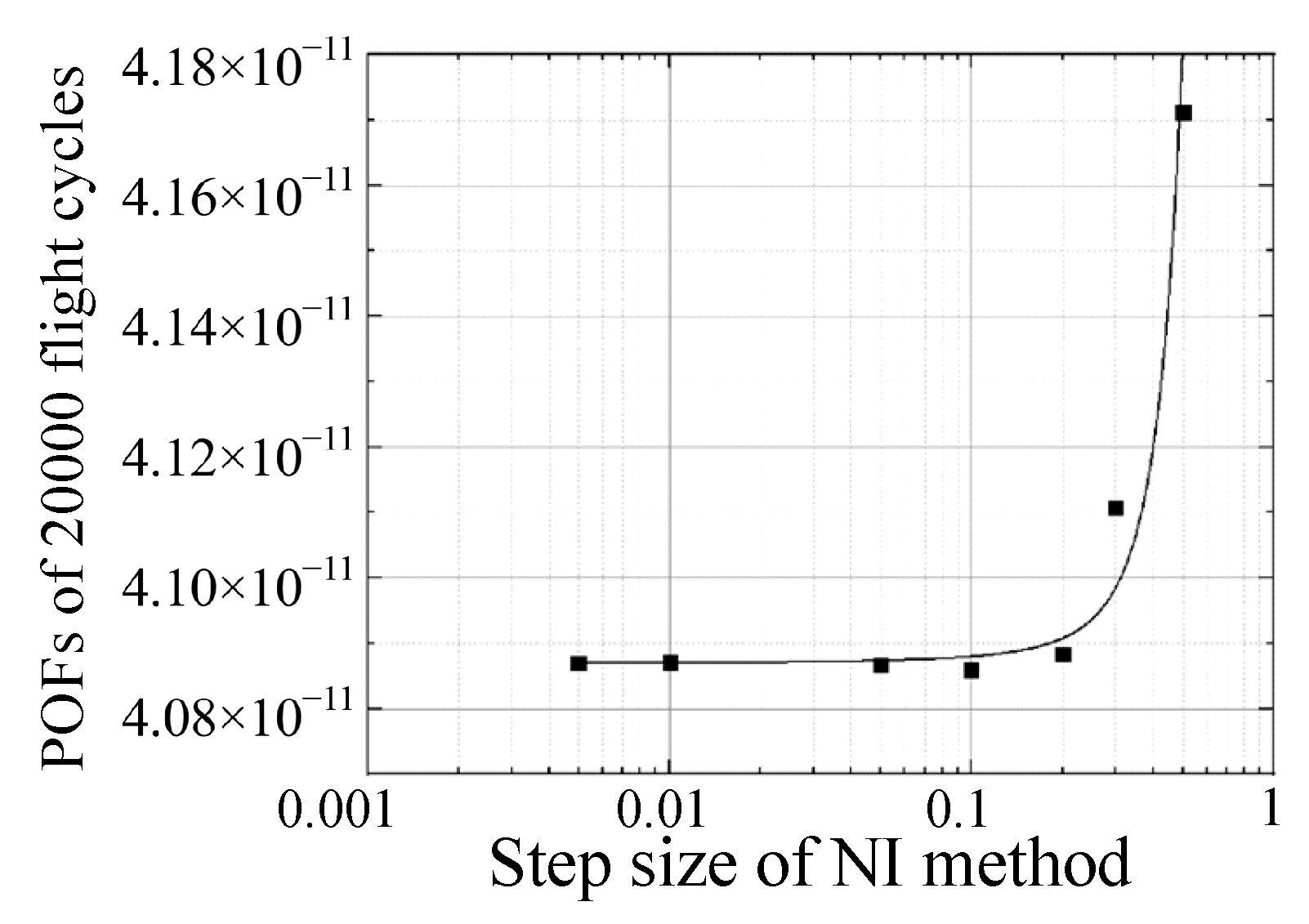
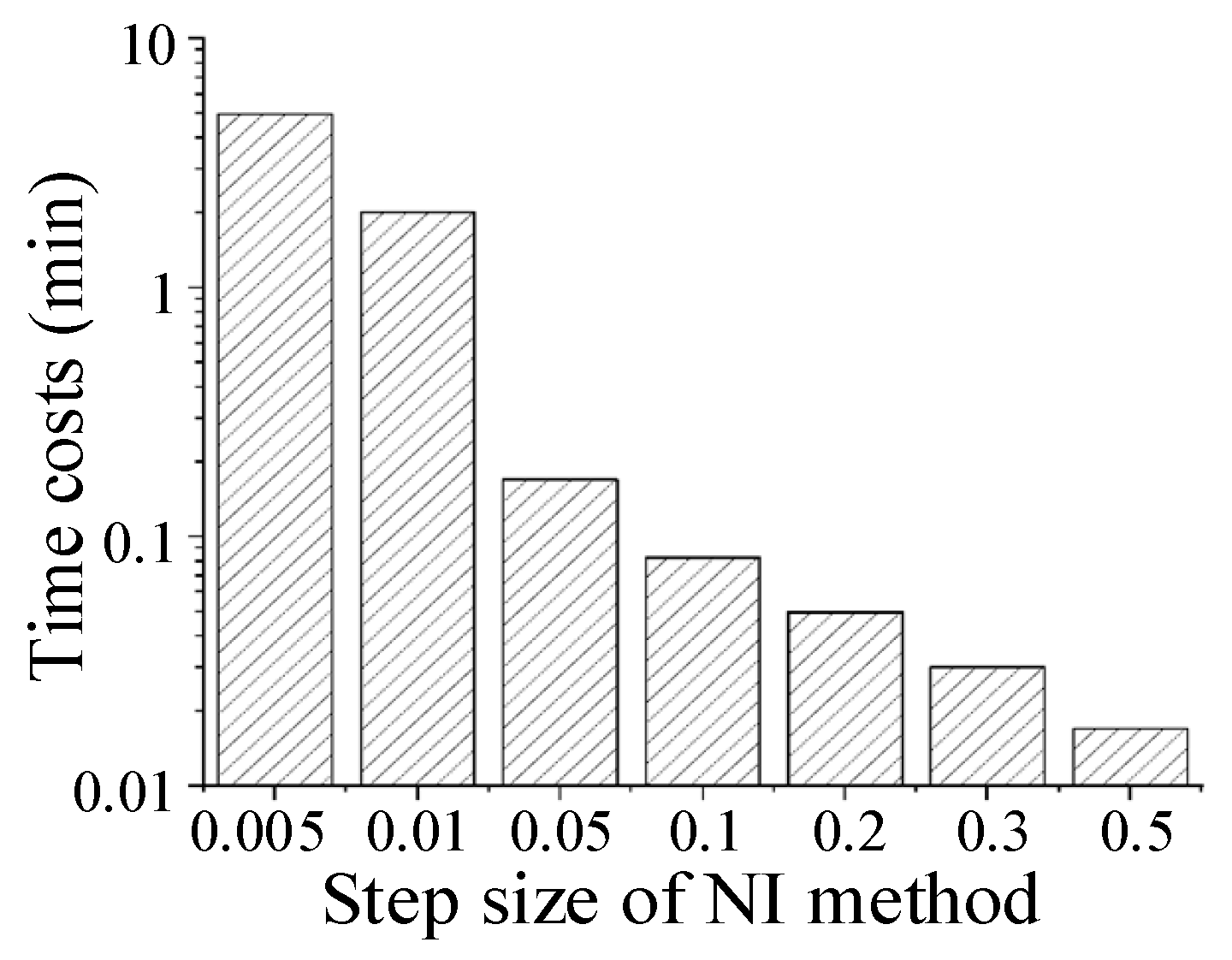
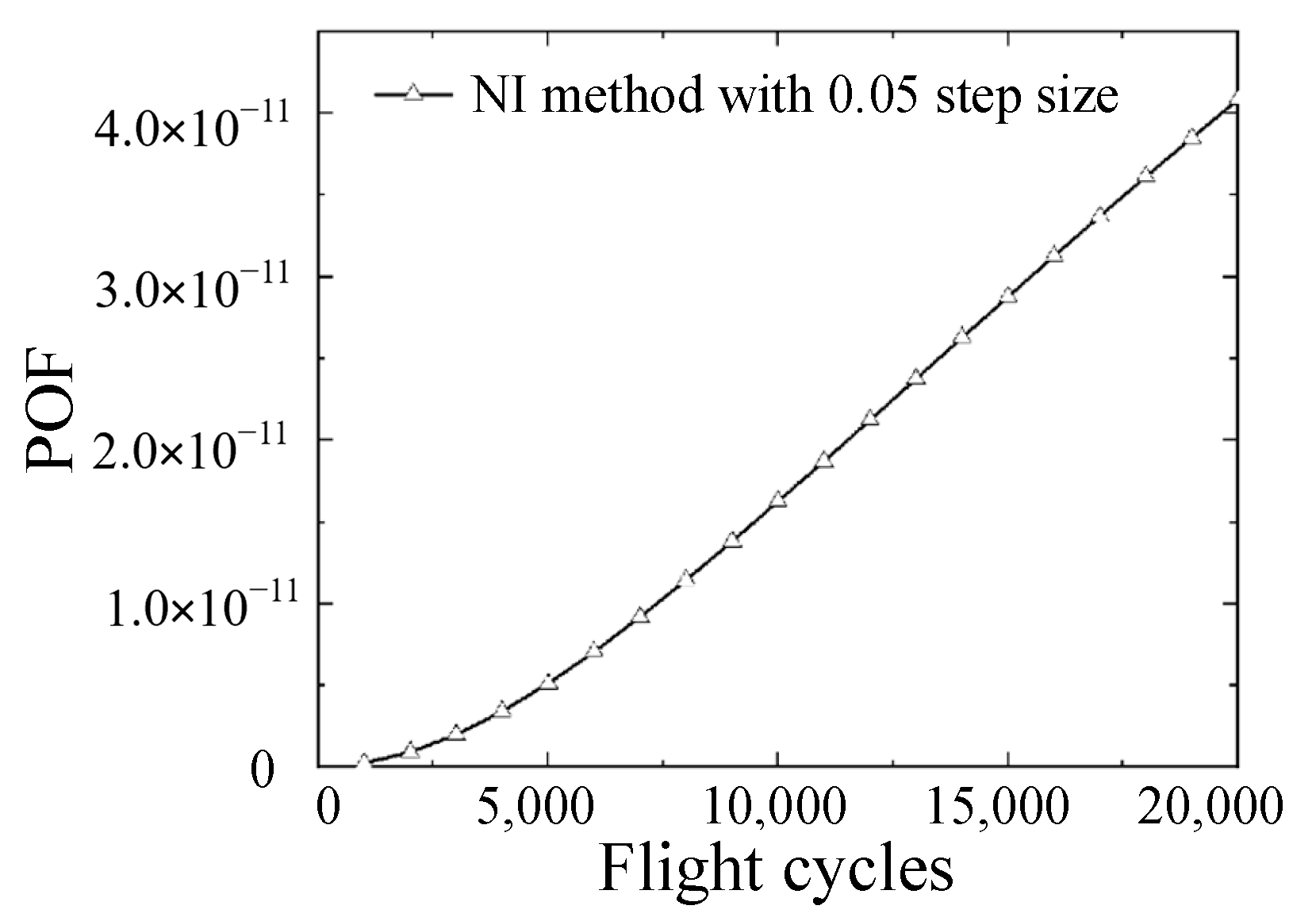
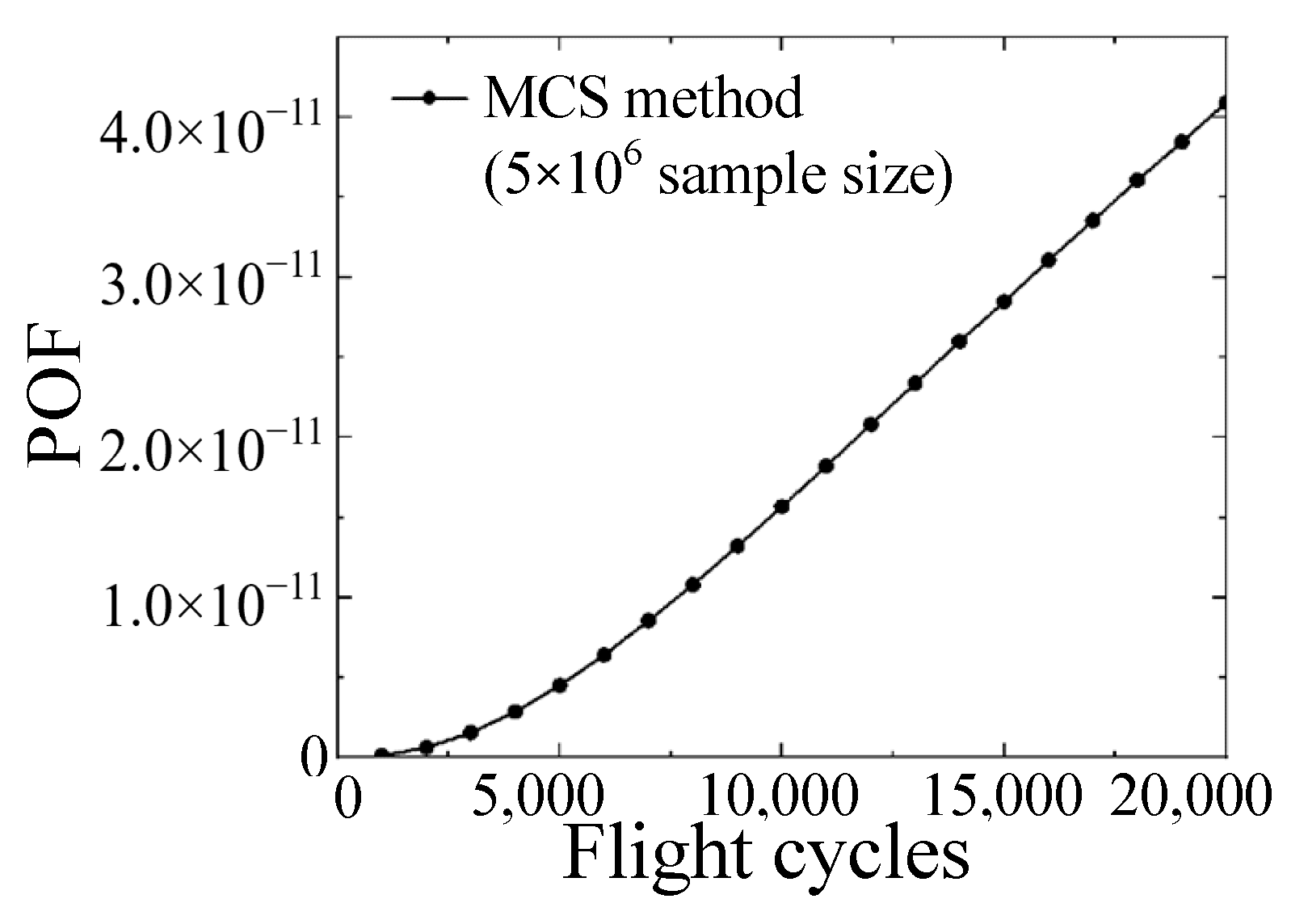
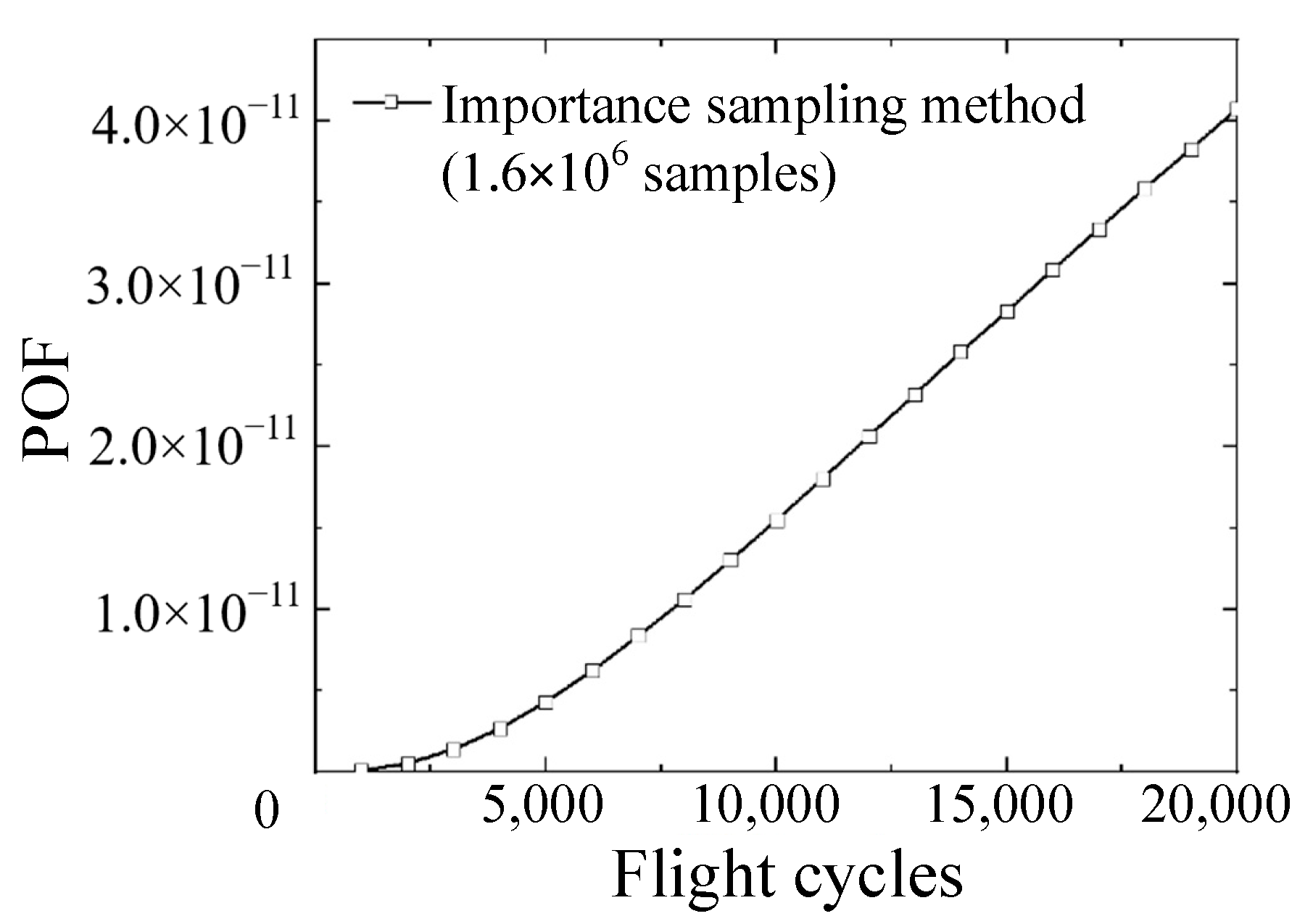
4.1. Convergence Results with Different Methods
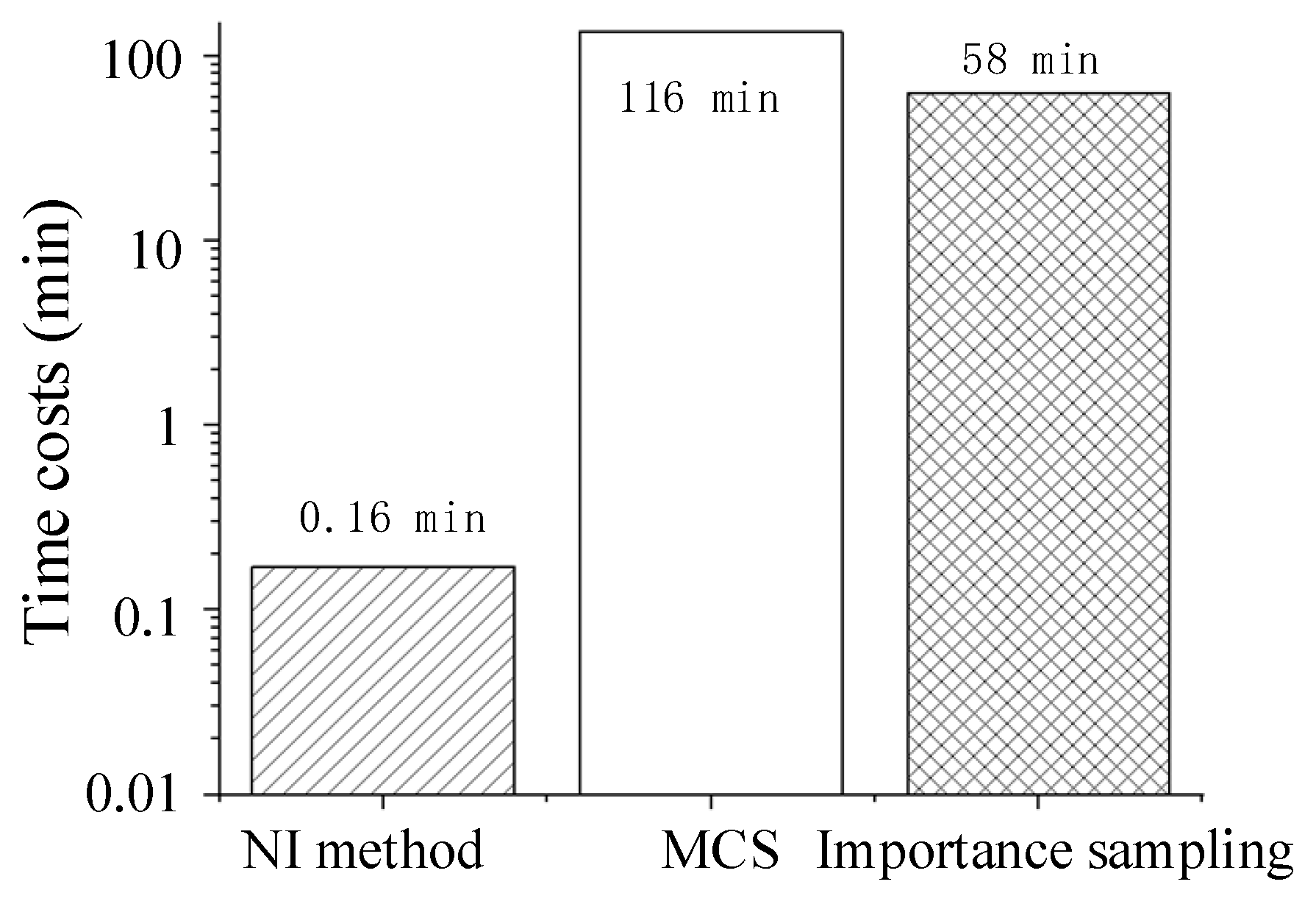
4.2. Comparison of Computational Costs and Precision with Different Methods
4.3. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| PDTA | Probabilistic damage tolerance analysis |
| POF | Probability of failure |
| MCS | Monte Carlo simulation |
| NI | Numerical integration |
| DTR | Designed targeted risk |
| NDI | Non-destructive inspection |
| COV | Coefficient of variation |
References
- Vittal, S.; Hajela, P.; Joshi, A. Review of Approaches to Gas Turbine Life Management. In Proceedings of the 10th AIAA/ISSMO Multidisciplinary Analysis and Optimization Conference, Albany, NY, USA, 30 August–1 September 2004. [Google Scholar]
- Kadau, K.; Enright, M.; Amann, C. Probabilistic Lifing. In Handbook of Nondestructive Evaluation 4.0; Meyendorf, N., Ida, N., Singh, R., Vrana, J., Eds.; Springer International Publishing: Cham, Switzerland, 2021; pp. 1–38. ISBN 978-3-030-48200-8. [Google Scholar]
- Enright, M.P.; Moody, J.P.; Zaman, Y.; Sobotka, J.C.; McClung, R.C. A Probabilistic Framework for Minimum Low Cycle Fatigue Life Prediction. In Proceedings of the ASME Turbo Expo 2022: Turbomachinery Technical Conference and Exposition, Rotterdam, The Netherlands, 13–17 June 2022; p. V08BT25A001. [Google Scholar]
- Federal Aviation Administration. Advisory Circular—Damage Tolerance of Hole Features in High-Energy Turbine Engine Rotors; Federal Aviation Administration, U.S. Department of Transportation: Washington, DC, USA, 2009; AC 33.70-2.
- European Aviation Safety Agency, Certification Specifications and Acceptable Means of Compliance for Large Aeroplanes. Amendment, 14. 2012. Available online: https://www.easa.europa.eu/en/document-library/easy-access-rules/easy-access-rules-engines-cs-e (accessed on 7 February 2020).
- Li, J.X. CCAR-33-R2 Provisions on Airworthiness of Aircraft Engines; Civil Aviation Administration of China, Bulletin of the State Council of the People’s Republic of China: Beijing, China, 2016. Available online: http://www.caac.gov.cn/XXGK/XXGK/index_172.html?fl=12 (accessed on 17 March 2016).
- Enright, M.P.; Moody, J.P.; Chandra, R.; Pentz, A.C. Influence of Mission Variability on Fracture Risk of Gas Turbine Engine Components. In Proceedings of the ASME Turbo Expo 2012: Turbine Technical Conference and Exposition, Copenhagen, Denmark, 11–15 June 2012; pp. 439–446. [Google Scholar]
- Enright, M.P.; Huyse, L. Methodology for Probabilistic Life Prediction of Multiple-Anomaly Materials. AIAA J. 2006, 44, 787–793. [Google Scholar] [CrossRef]
- Enright, M.P.; McClung, R.C.; Liang, W.; Lee, Y.-D.; Moody, J.P.; Fitch, S. A Tool for Probabilistic Damage Tolerance of Hole Features in Turbine Engine Rotors. In Proceedings of the ASME Turbo Expo 2012: Turbine Technical Conference and Exposition, Copenhagen, Denmark, 11–15 June 2012; pp. 447–458. [Google Scholar]
- Chan, K.S.; Enright, M.P.; Moody, J.; Thomas, C.; Goodrum, W. HOTPITS: The DARWIN Approach to Assessing Risk of Hot Corrosion-Induced Fracture in Gas Turbine Components. Eng. Fract. Mech. 2020, 228, 106889. [Google Scholar] [CrossRef]
- McClung, R.C.; Enright, M.P.; Moody, J.P.; Lee, Y.D.; McFarland, J. Integrating Fatigue Crack Growth into Reliability Analysis and Computational Materials Design. Adv. Mater. Res. 2014, 891–892, 1009–1014. [Google Scholar] [CrossRef]
- Enright, M.P.; McClung, R.C.; Chan, K.S.; McFarland, J.; Moody, J.P.; Sobotka, J.C. Micromechanics-Based Fracture Risk Assessment Using Integrated Probabilistic Damage Tolerance Analysis and Manufacturing Process Models. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, South Korea, 13–17; June 2016; p. V07BT29A004. [Google Scholar]
- Enright, M.P.; McClung, R.C. A Probabilistic Framework for Gas Turbine Engine Materials with Multiple Types of Anomalies. J. Eng. Gas Turbines Power 2011, 133, 082502. [Google Scholar] [CrossRef]
- Federal Aviation Administration. Advisory Circular—Guidance Material for Aircraft Engine Life-Limited Parts Requirements; Federal Aviation Administration, U.S. Department of Transportation: Washington, DC, USA, 2009; AC 33.70-1.
- Federal Aviation Administration. Advisory Circular—Damage Tolerance for High Energy Turbine Engine Rotors; Federal Aviation Administration, U.S. Department of Transportation: Washington, DC, USA, 2001; AC 33.14-1.
- Millwater, H.; Ocampo, J.D.; Castaldo, A. Probabilistic Damage Tolerance Analysis for General Aviation. Adv. Mater. Res. 2014, 891–892, 1191–1196. [Google Scholar] [CrossRef]
- Wu, Y.-T. Computational Methods for Efficient Structural Reliability and Reliability Sensitivity Analysis. AIAA J. 1994, 32, 1717–1723. [Google Scholar] [CrossRef]
- Su, B.; Zhou, Q. A Semi-Analytical and Monte Carlo-Based Phase Dynamic Evolution Approach for LEO Mega-Constellations. Aerospace 2022, 9, 128. [Google Scholar] [CrossRef]
- Jing, H.; Jian, C.; Liu, L. An Efficient Method of Calculating Stress Intensity Factor for Surface Cracks in Holes Under Uni-Variant Stressing. In Methods and Applications for Modeling and Simulation of Complex Systems; Fan, W., Zhang, L., Li, N., Song, X., Eds.; Communications in Computer and Information Science; Springer Nature Singapore: Singapore, 2022; Volume 1713, pp. 14–25. ISBN 978-981-19919-4-3. [Google Scholar]
- Amann, C.; Kadau, K. Numerically Efficient Modified Runge–Kutta Solver for Fatigue Crack Growth Analysis. Eng. Fract. Mech. 2016, 161, 55–62. [Google Scholar] [CrossRef]
- L’Ecuyer, P.; Simard, R.; Chen, E.J.; Kelton, W.D. An Object-Oriented Random-Number Package with Many Long Streams and Substreams. Oper. Res. 2002, 50, 1073–1075. [Google Scholar] [CrossRef]
- Kadau, K.; Gravett, P.W.; Amann, C. Probabilistic Fracture Mechanics for Heavy-Duty Gas Turbine Rotor Forgings. J. Eng. Gas Turbines Power 2018, 140, 062503. [Google Scholar] [CrossRef]
- Huyse, L.; Enright, M. Efficient Statistical Analysis of Failure Risk in Engine Rotor Disks Using Importance Sampling Techniques. In Proceedings of the 44th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Norfolk, VA, USA, 7–10 April 2003. [Google Scholar]
- Enright, M.P.; Millwater, H.R.; Huyse, L. Adaptive Optimal Sampling Methodology for Reliability Prediction of Series Systems. AIAA J. 2006, 44, 523–528. [Google Scholar] [CrossRef]
- Millwater, H.; Enright, M.; Fitch, S. A Convergent Probabilistic Technique for Risk Assessment of Gas Turbine Disks Subject to Metallurgical Defects. In Proceedings of the 43rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Denver, CO, USA, 22–25 April 2002. [Google Scholar]
- Enright, M.; Millwater, H.; Moody, J. Efficient Integration of Sampling-Based Spatial Conditional Failure Joint Probability Densities. In Proceedings of the 48th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Honolulu, HI, USA, 23–26 April 2007. [Google Scholar]
- Enright, M.; Millwater, H. Optimal Sampling Techniques for Zone-Based Probabilistic Fatigue Life Prediction. In Proceedings of the 43rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Denver, CO, USA, 22–25 April 2002. [Google Scholar]
- Orisamolu, I.; Luo, X.; Orisamolu, I.; Luo, X. Probabilistic Assessment of Corrosion Effects on the Damage Tolerance of Aircraft Structures. In Proceedings of the 38th Structures, Structural Dynamics, and Materials Conference, Kissimmee, FL, USA, 7–10 April 1997. [Google Scholar]
- Yang, L.; Ding, S.; Wang, Z.; Li, G. Efficient Probabilistic Risk Assessment for Aeroengine Turbine Disks Using Probability Density Evolution. AIAA J. 2017, 55, 2755–2761. [Google Scholar] [CrossRef]
- Leverant, G.R.; Littlefield, D.L.; McClung, R.C.; Millwater, H.R.; Wu, J.Y. A Probabilistic Approach to Aircraft Turbine Rotor Material Design. In Proceedings of the ASME 1997 International Gas Turbine and Aeroengine Congress and Exhibition, Orlando, FL, USA, 2–5 June 1997; p. V004T14A010. [Google Scholar]
- Wu, Y.-T.; Enright, M.P.; Millwater, H.R. Probabilistic Methods for Design Assessment of Reliability with Inspection. AIAA J. 2002, 40, 937–946. [Google Scholar] [CrossRef]
- Millwater, H.R.; Fitch, S.H.K.; Wu, Y.-T.; Riha, D.S.; Enright, M.P.; Leverant, G.R.; McClung, R.C.; Kuhlman, C.J.; Chell, G.G.; Lee, Y.-D. A Probabilistically-Based Damage Tolerance Analysis Computer Program for Hard Alpha Anomalies in Titanium Rotors. In Proceedings of the ASME Turbo Expo 2000: Power for Land, Sea, and Air, Munich, Germany, 8–11 May 2000; p. V004T03A062. [Google Scholar]
- Aerospace Industries Association Rotor Integrity Sub-Committee. The development of anomaly distributions for aircraft engine titanium disk alloys. In Proceedings of the 38th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Kissimmee, FL, USA, 7–10 April 1997. [Google Scholar]
- Raju, I.S.; Newman, J.C. A Reinvestigation of Stress-Intensity Factors for Surface and Corner Cracks in Three-Dimensional Solids. In Contemporary Research in Engineering Science; Batra, R.C., Ed.; Springer: Berlin/Heidelberg, Germany, 1995; pp. 418–441. ISBN 978-3-642-80003-0. [Google Scholar]
- Glinka, G.; Shen, G. Universal Features of Weight Functions for Cracks in Mode I. Eng. Fract. Mech. 1991, 40, 1135–1146. [Google Scholar] [CrossRef]
- Huang, X.; Chen, C.; Xuan, H.; Guo, X.; Shan, X.; Hong, W. Fatigue Crack Propagation Analysis in an Aero-Engine Turbine Disc Using Computational Methods and Spin Test. Theor. Appl. Fract. Mech. 2023, 124, 103745. [Google Scholar] [CrossRef]
- Paris, P.; Erdogan, F. A Critical Analysis of Crack Propagation Laws. J. Basic Eng. 1963, 85, 528–533. [Google Scholar] [CrossRef]
- Li, J.; Chen, J. Advances in The Research on Probability Density Evolution Equations of Stochastic Dynamical Systems. Adv. Mech. 2010, 40, 170–188. [Google Scholar] [CrossRef]
- Lasota, A.; Mackey, M.C.; Chaos, F. Noise: Stochastic Aspects of Dynamics. Appl. Math. Sci. 1994, 97, 37–49. [Google Scholar]
- Bontempi, F.; Casciati, F. Chaotic Motion and Stochastic Excitation. Nonlinear Dyn. 1994, 6, 179–191. [Google Scholar] [CrossRef]
- Kozin, F. On the Probability Densities of the Output of Some Random Systems. J. Appl. Mech. 1961, 28, 161–164. [Google Scholar] [CrossRef]
- Li, J.; Chen, J. The Principle of Preservation of Probability and the Generalized Density Evolution Equation. Struct. Saf. 2008, 30, 65–77. [Google Scholar] [CrossRef]
- Ayyub, B.M.; Chia, C.-Y. Generalized Conditional Expectation for Structural Reliability Assessment. Struct. Saf. 1992, 11, 131–146. [Google Scholar] [CrossRef]
- Atkinson, K.E.; Wiley. An Introduction to Numerical Analysis. Math. Comput. Simul. 1990, 32, 319. [Google Scholar] [CrossRef]
- McClung, R.; Enright, M.; Liang, W.; Chan, K.; Moody, J.; Wu, W.-T.; Shankar, R.; Luo, W.; Oh, J.; Fitch, S. Integration of Manufacturing Process Simulation with Probabilistic Damage Tolerance Analysis of Aircraft Engine Components. In Proceedings of the 53rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Honolulu, HI, USA, 23–26 April 2012. [Google Scholar]
- Enright, M.P.; McFarland, J.; McClung, R.; Wu, W.-T.; Shankar, R. Probabilistic Integration of Material Process Modeling and Fracture Risk Assessment Using Gaussian Process Models. In Proceedings of the 54th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Boston, MA, USA, 8–11 April 2013. [Google Scholar]
- Ding, S.; Wang, Z.; Qiu, T.; Zhang, G.; Li, G.; Zhou, Y. Probabilistic Failure Risk Assessment for Aeroengine Disks Considering a Transient Process. Aerosp. Sci. Technol. 2018, 78, 696–707. [Google Scholar] [CrossRef]
- Junbo, L.; Shuiting, D.; Guo, L. Influence of Random Variable Dimension on the Fast Numerical Integration Method of Aero Engine Rotor Disk Failure Risk Analysis. In Proceedings of the ASME 2020 International Mechanical Engineering Congress and Exposition, Virtual, 16–19 November 2020; p. V014T14A039. [Google Scholar]






| Boundary Conditions | Value |
|---|---|
| Disk rotation speed | 35,000 rev/min |
| Mass Flow | 6.825 × 10−5 kg/s |
| Inlet temperature | 288.15 K |
| Outlet temperature | 445.83 K |
| Outlet pressure | 383 KPa |
| Parameters | Value |
|---|---|
| Density | 4450 kg/m3 |
| Young’s modulus | 120,000 MPa |
| Poisson’s ratio | 0.361 |
| da/dN | 9.25 × 10−13(ΔK) 3.87 m/cycle |
| K threshold | 0.0 MPa√m |
| Fracture toughness | 64.5 MPa√m |
| Yield strength | 834 MPa |
| Calculation Method | POF at 20,000 Flight Cycles |
|---|---|
| NI method (step size of 0.05) | 4.0867 × 10−11 |
| MCS (sample size of 5 × 106) | 4.0936 × 10−11 |
| Importance sampling method (sample size of 1.5 × 106) | 4.0777 × 10−11 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Liu, J.; Yang, L.; Zhou, H.; Ding, S. A Multivariable Method for Calculating Failure Probability of Aeroengine Rotor Disk. Aerospace 2023, 10, 296. https://doi.org/10.3390/aerospace10030296
Li G, Liu J, Yang L, Zhou H, Ding S. A Multivariable Method for Calculating Failure Probability of Aeroengine Rotor Disk. Aerospace. 2023; 10(3):296. https://doi.org/10.3390/aerospace10030296
Chicago/Turabian StyleLi, Guo, Junbo Liu, Liu Yang, Huimin Zhou, and Shuiting Ding. 2023. "A Multivariable Method for Calculating Failure Probability of Aeroengine Rotor Disk" Aerospace 10, no. 3: 296. https://doi.org/10.3390/aerospace10030296
APA StyleLi, G., Liu, J., Yang, L., Zhou, H., & Ding, S. (2023). A Multivariable Method for Calculating Failure Probability of Aeroengine Rotor Disk. Aerospace, 10(3), 296. https://doi.org/10.3390/aerospace10030296





