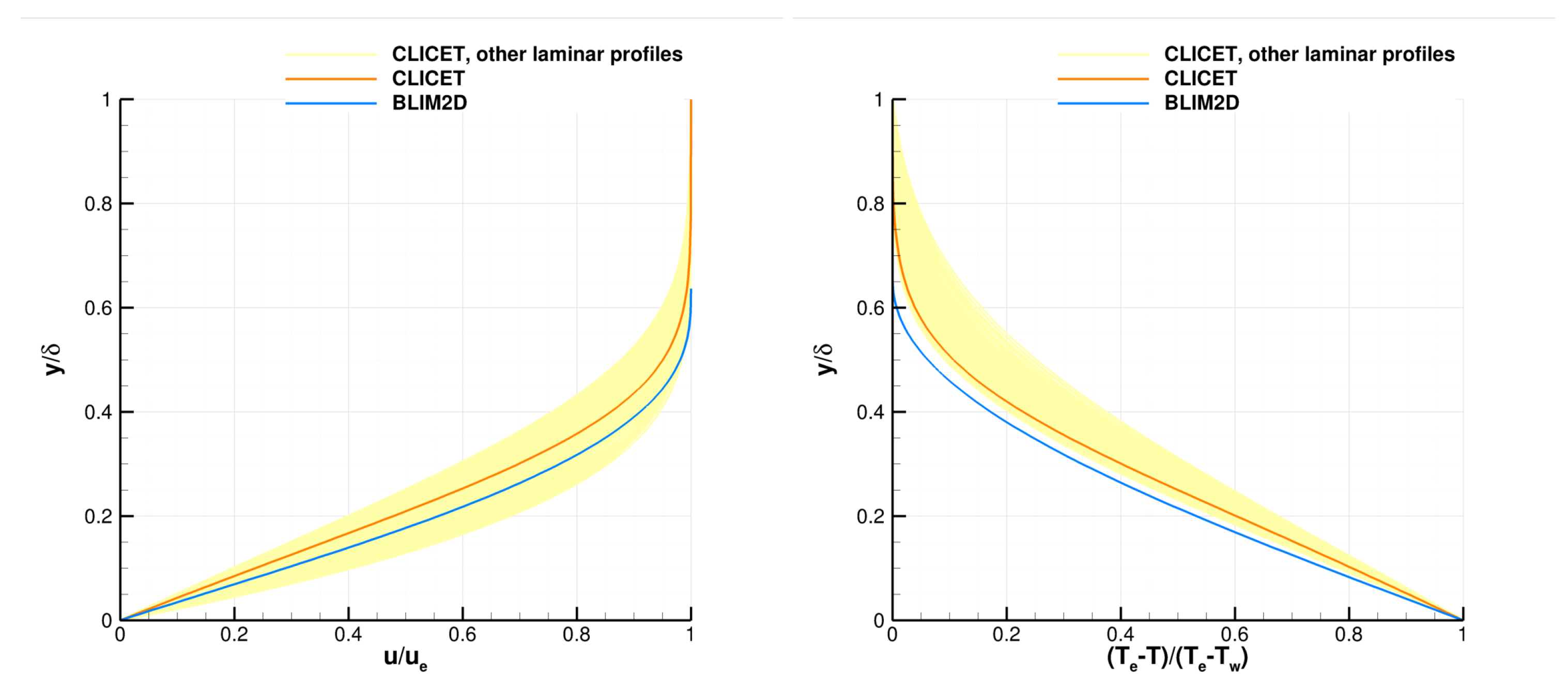
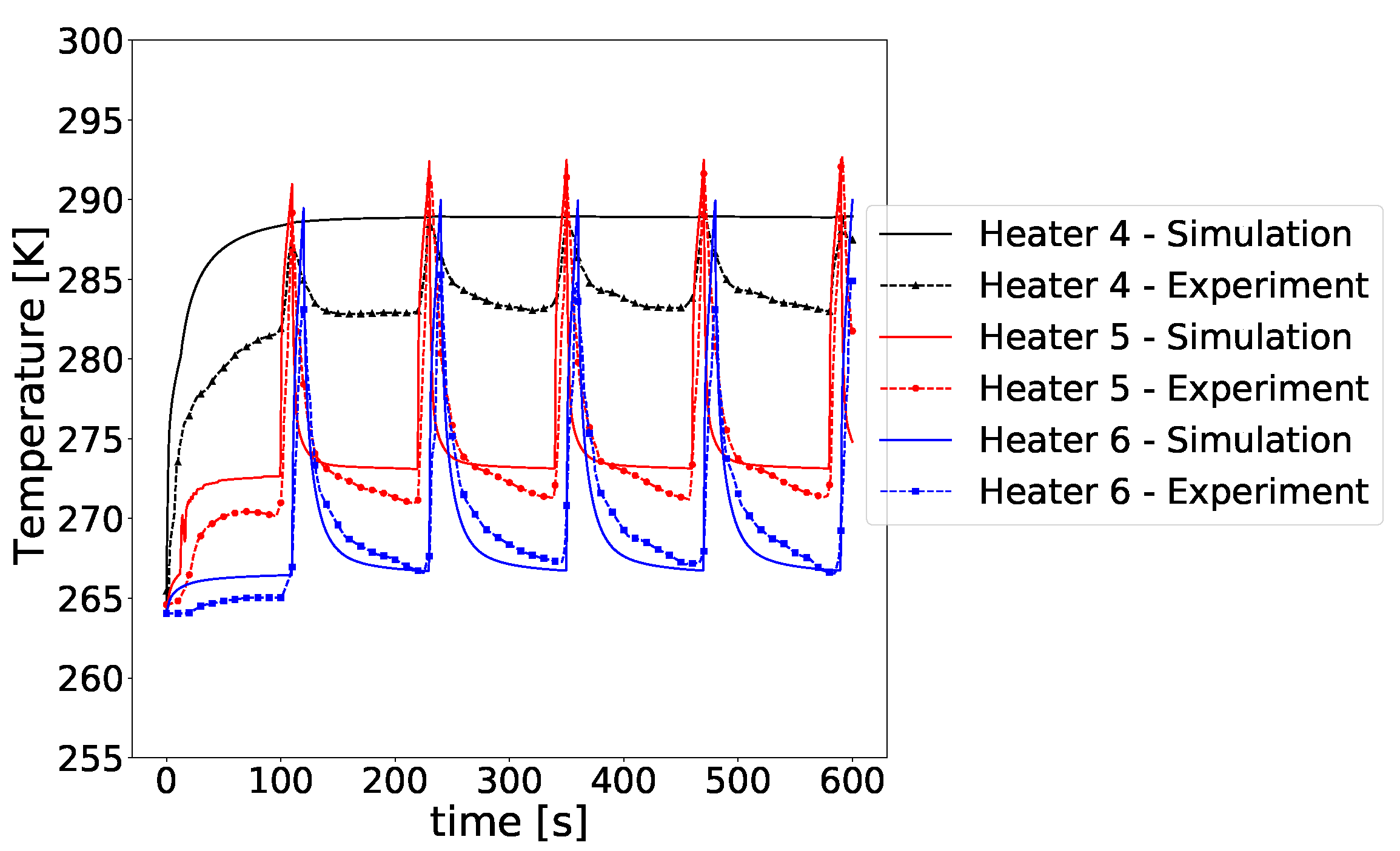
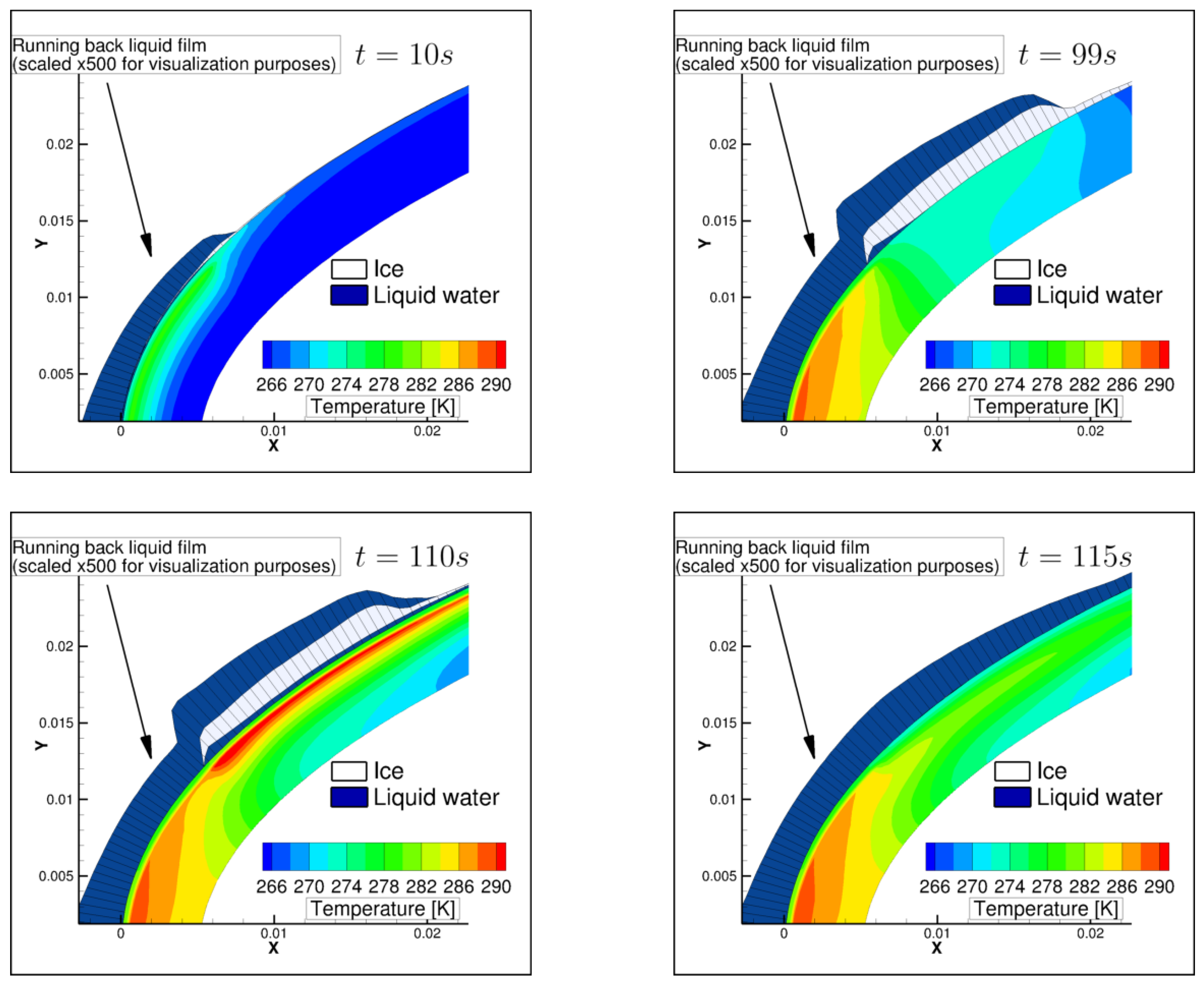
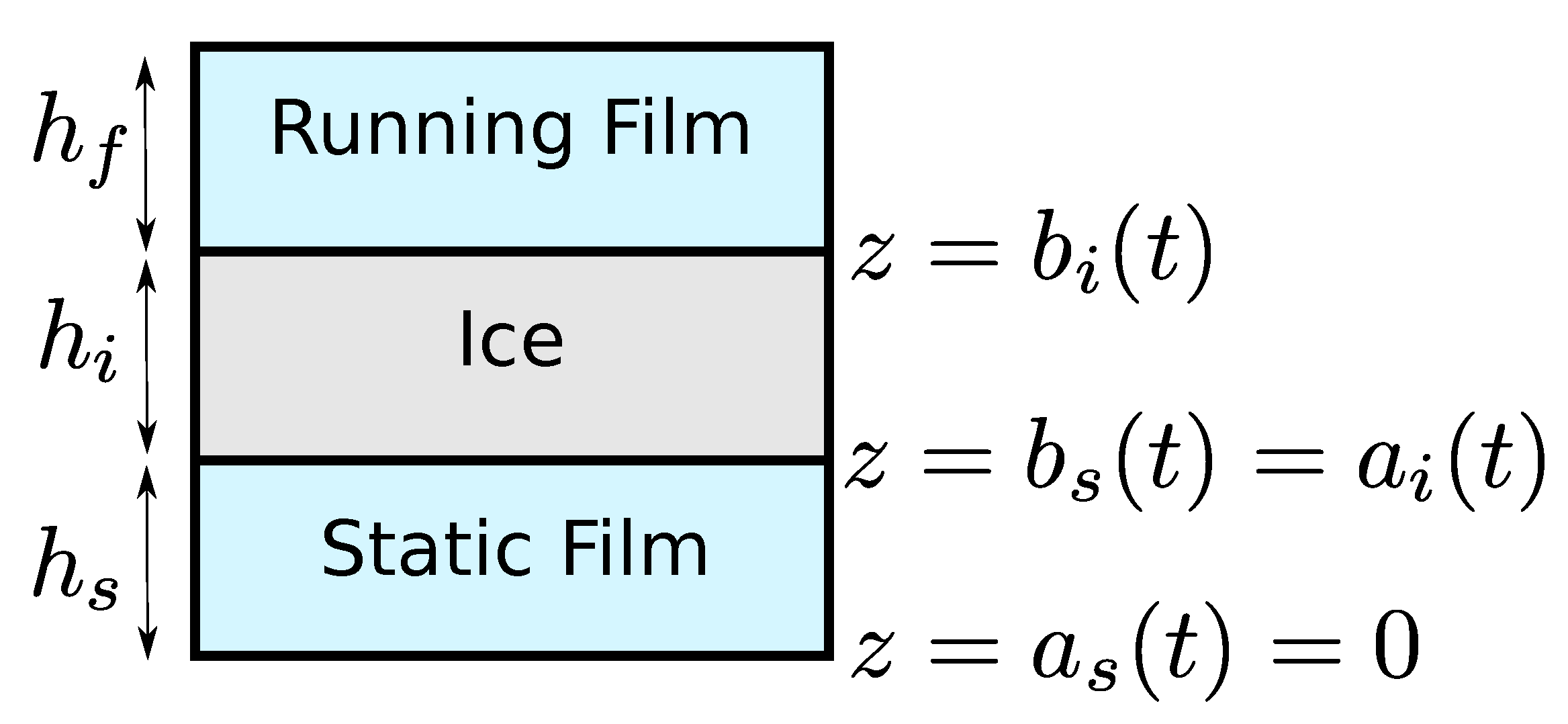Appendix A.1. Validation in Laminar Regime
Some simulations of a database generated by Han and Palacios [
57,
58] were performed with CLICET, BLIM2D, and SIM2D to validate these tools. In the experimental database of Han and Palacios, some measurements of heat transfer coefficient were performed, for which
is given by:
where
is the measured wall heat flux, whereas
is the measured wall temperature, and
is the airflow temperature set in the wind-tunnel inlet. This database is mainly dedicated to the investigation of the influence of wall roughness on
. The effects of roughness being out of the scope of this paper, we are only interested in the laminar area where roughness is not expected to affect
.
The experimental conditions are as follows: a NACA0012 airfoil, whose chord length is m, was put in a wind tunnel. The aerodynamic flow has an upstream velocity of about m/s, the outside air temperature is about K, while the wall temperature is about K. The experimental data are unfortunately known approximately. The measurements of have been digitized, and more precisely, they have been reconstructed from the graphs of Frossling number , where the air conductivity was taken equal to W/m/K and the Reynolds number is ( J/K/kg for air, is given by the Sutherland law, and the pressure was set to 93,000 Pa here).
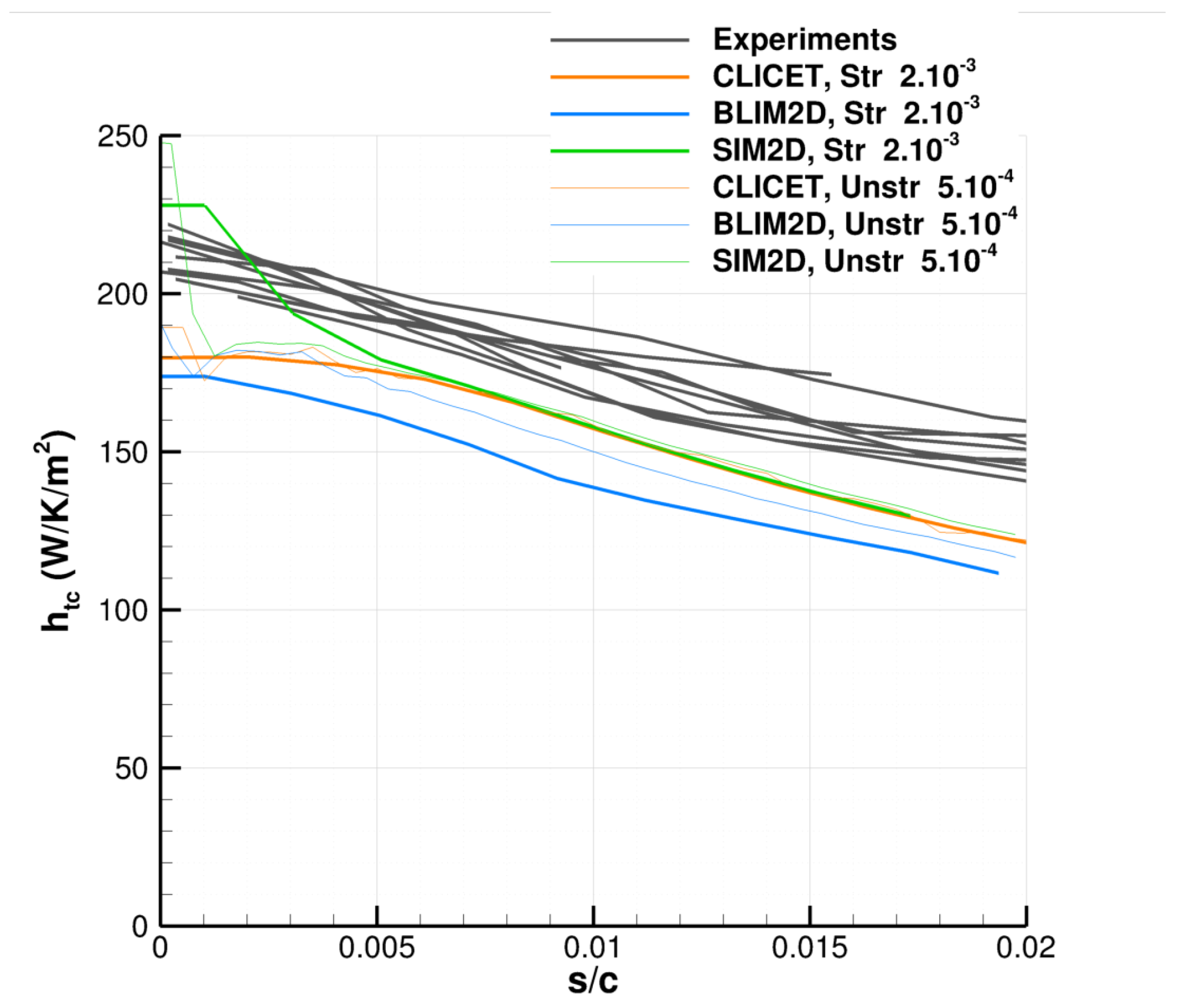
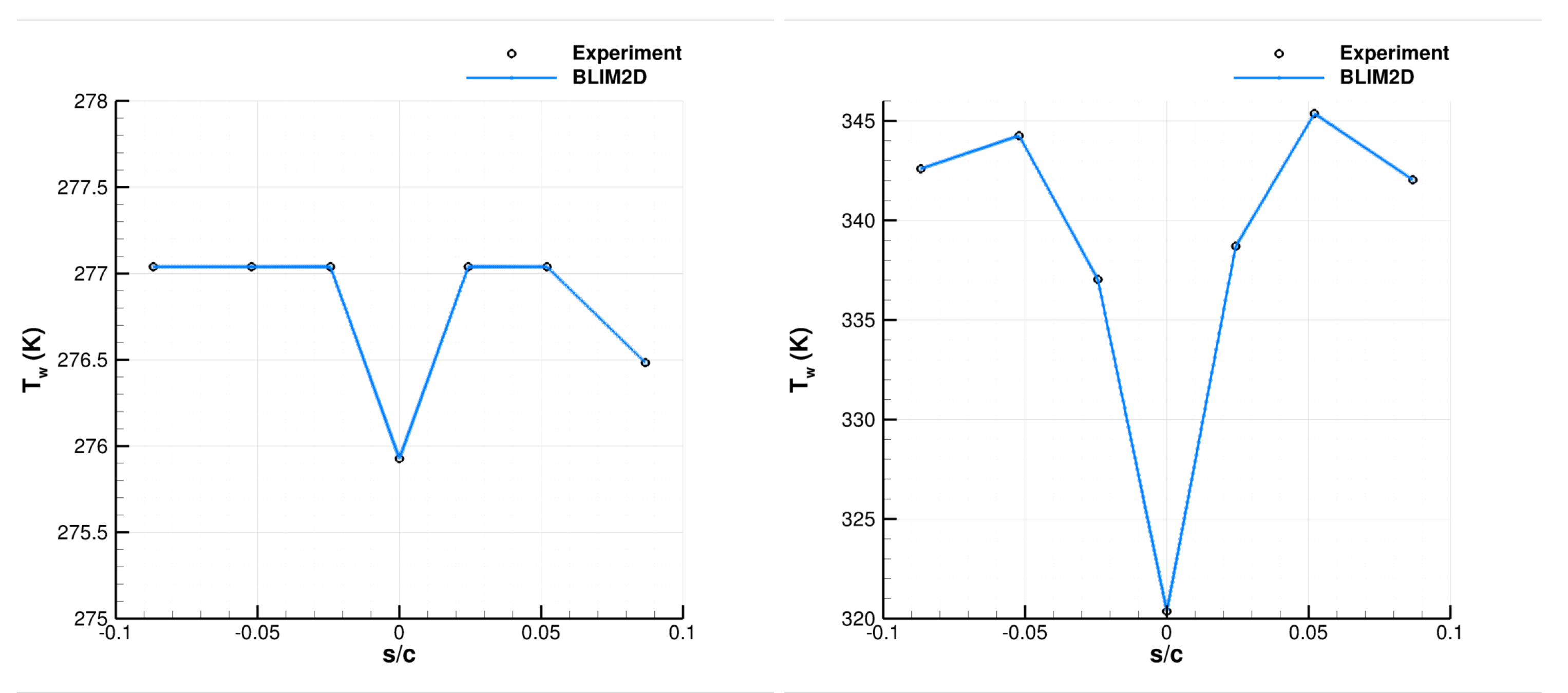
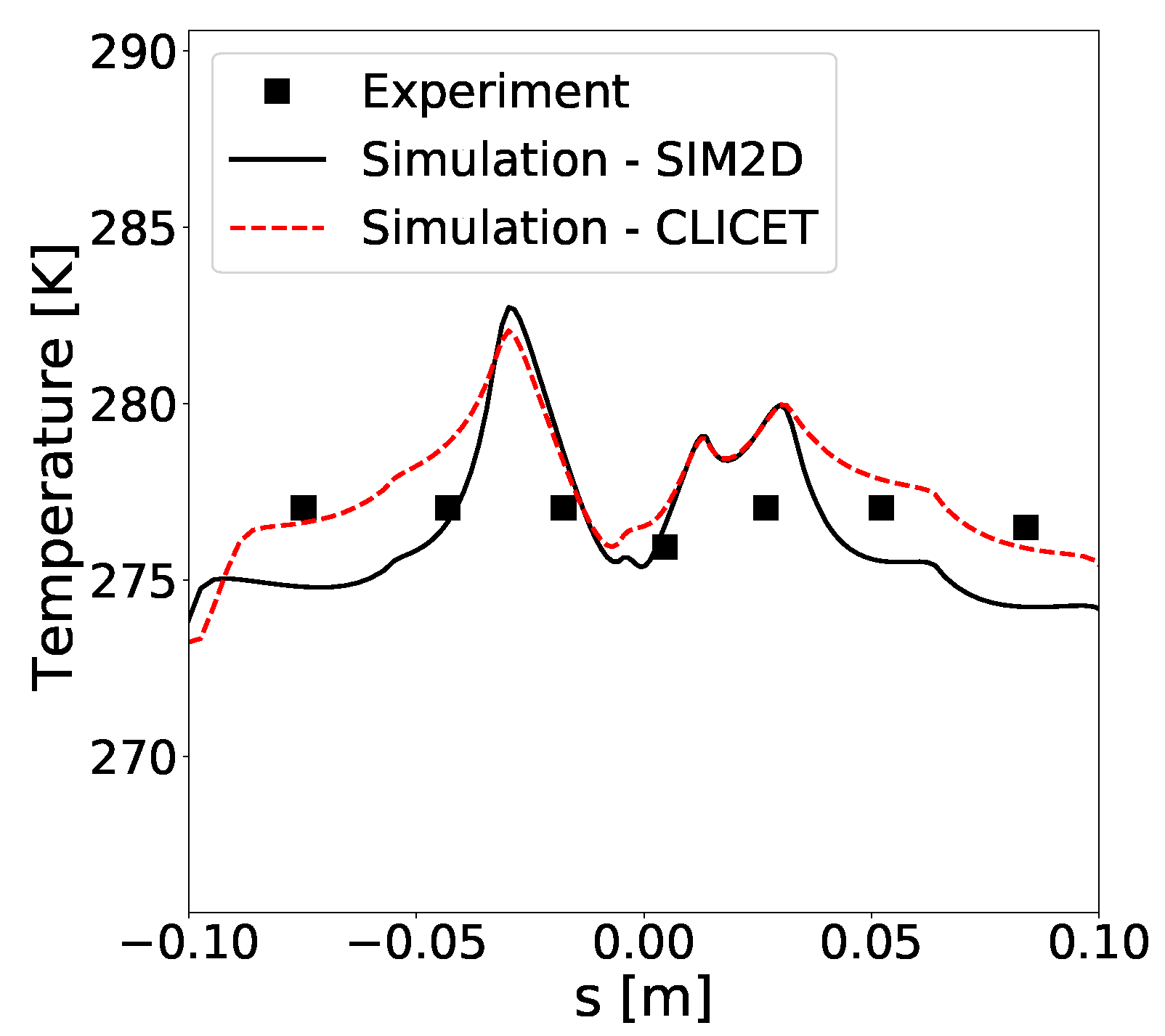
Despite the low precision of the input data, the agreement on
between the codes and the experimental data is rather good. The experimental data set has been plotted in
Figure A1 for different roughness sizes. As expected, the roughness size affects only the extent of the laminar area, and the values of
coincide rather well for all sets of measurements.
Figure A1.
Solution produced by CLICET, SIM2D, and BLIM2D for the heat transfer coefficient with respect to the curvilinear abscissa s in the laminar area of Han and Palacios’s experiment for several meshes. Comparison against the experimental measurements.
Figure A1.
Solution produced by CLICET, SIM2D, and BLIM2D for the heat transfer coefficient with respect to the curvilinear abscissa s in the laminar area of Han and Palacios’s experiment for several meshes. Comparison against the experimental measurements.
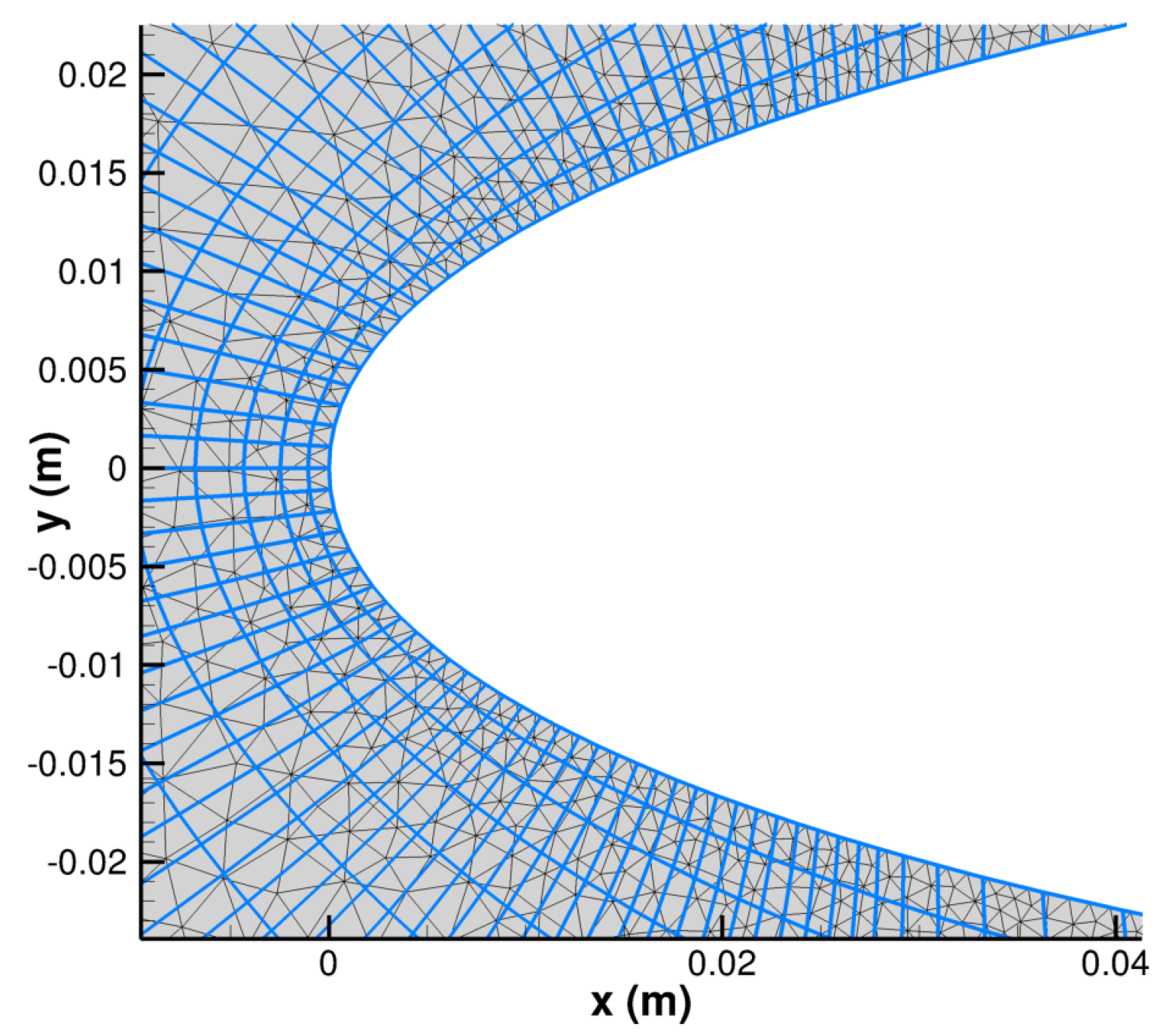
For the simulations, several meshes were used. The first mesh is the default structured mesh of IGLOO2D [
11]. The others are unstructured meshes of different refinement levels. The structured mesh is rather refined around the leading edge of the airfoil (
Figure A2), where the mesh size is around
. Further downstream, the mesh is coarsened, especially downstream of the first five percent of the chord length, the mesh size reaching around
in the downstream half of the airfoil.
Figure A2 shows that the mesh size is more uniform for the unstructured meshes, here on the example of the coarsest mesh for which the same level of refinement is used at the leading edge as for the structured mesh. Two additional levels of refinement were employed for these unstructured meshes, two times (
) and four times (
) finer than the coarse mesh, respectively.
Figure A2.
Meshes used for the simulation of the Han and Palacios test case. Default structured mesh in blue lines, coarsest unstructured mesh in black lines.
Figure A2.
Meshes used for the simulation of the Han and Palacios test case. Default structured mesh in blue lines, coarsest unstructured mesh in black lines.
As in
Section 4.4, inviscid simulations were performed with the solver EULER2D. The boundary-layer solution was then computed with the three solvers presented earlier, CLICET, BLIM2D, and SIM2D, fed with the inviscid solution. For these simulations, the laminar area was maintained up to the non-dimensional abscissa
. The simulations with the intermediate unstructured mesh (
) are not shown in the following figures because the results are very close to those of the finest mesh (
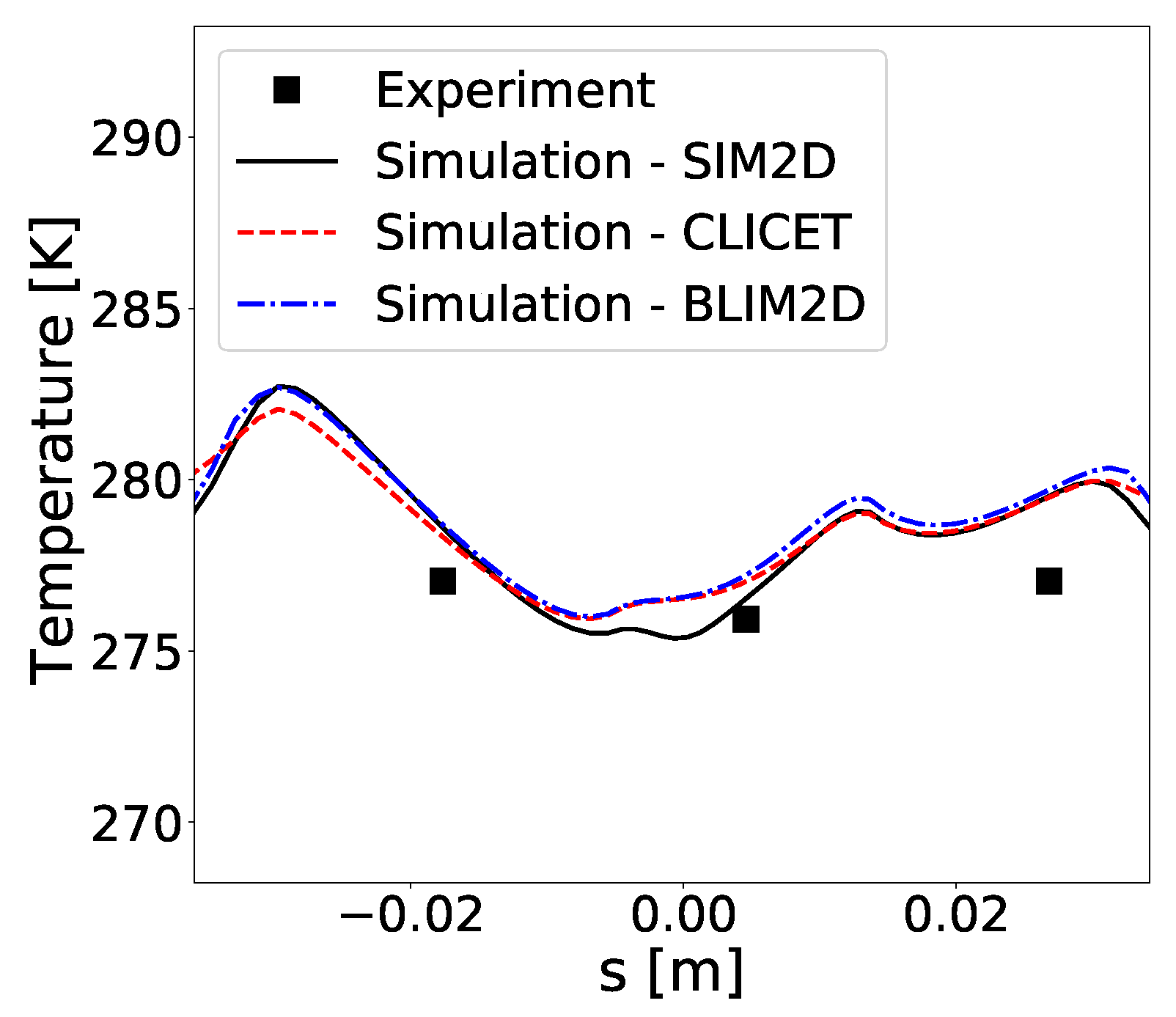
), except for the region near the stagnation point (
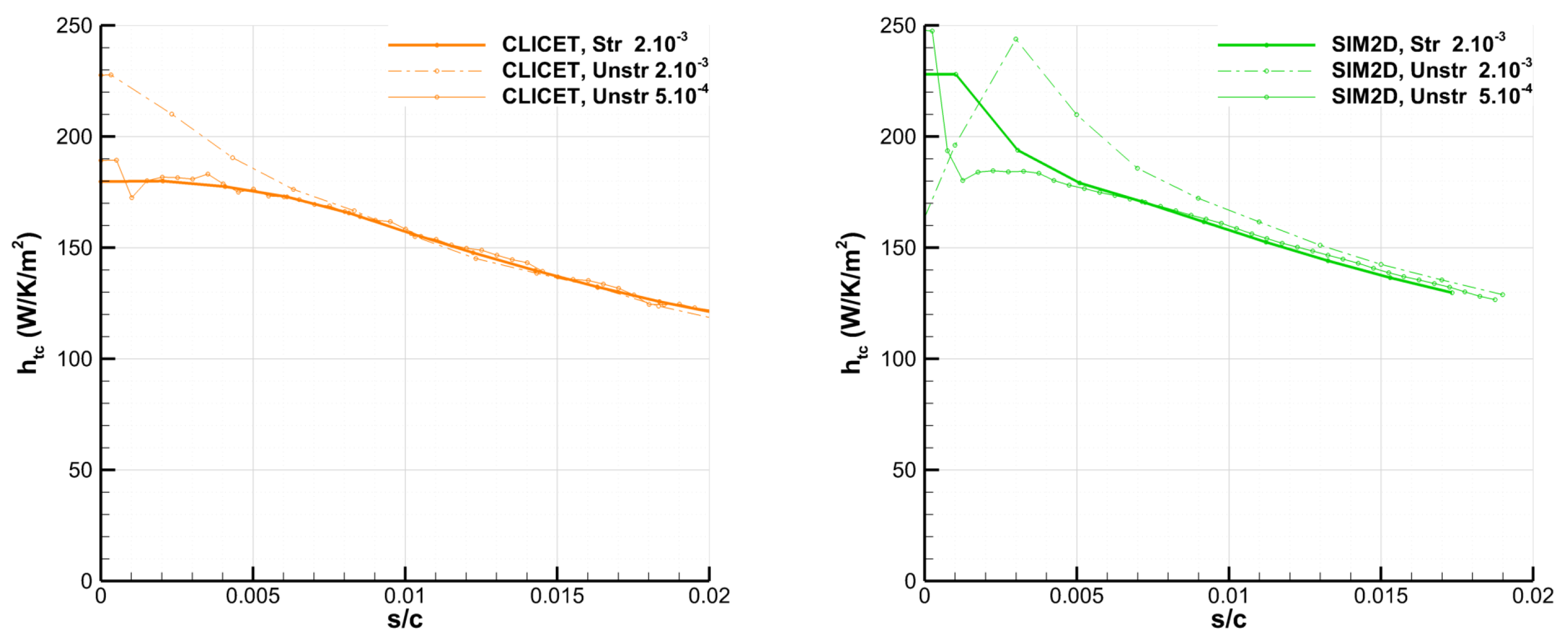
) showing that mesh convergence is achieved. The simulation with CLICET captures quite well the experimental data, taking into account the uncertainties of the experimental inputs (
Figure A1). In addition,
Figure A3 (left) shows that all the meshes produce very similar solutions, except in the vicinity of the stagnation point, which is more difficult to capture and requires sufficient mesh refinement and quality. The SIM2D solution, which is fed with the same inviscid data from EULER2D, is only slightly more sensitive to mesh convergence than CLICET (
Figure A3, right), and the solution is very good compared to the experiments too (
Figure A1), which justifies even more the use of this type of solver for unheated applications. For this, however, it was necessary to correct
in the following way:
because the heat transfer coefficient
of SIM2D is used as
, where
is the recovery temperature. Moreover, it is worth noting that SIM2D only slightly overstimates
compared to CLICET in the immediate vicinity of
.
Figure A3.
Solution produced by CLICET and SIM2D for the heat transfer coefficient in the laminar area of Han and Palacios’s experiment for several meshes. (Left): CLICET solution. (Right): SIM2D solution.
Figure A3.
Solution produced by CLICET and SIM2D for the heat transfer coefficient in the laminar area of Han and Palacios’s experiment for several meshes. (Left): CLICET solution. (Right): SIM2D solution.
The simulation with BLIM2D, also fed with the same EULER2D data, slightly underestimates
, but the error is rather small (
Figure A1). However, the sensitivity to the mesh is larger than for the other solvers, as shown in
Figure A4. The standard structured mesh is indeed not fine enough and slightly underestimates
all along the profile. To sum up, for sufficiently fine meshes, the difference between the results of the three codes is very small (
Figure A1). The main difference is finally that SIM2D overestimates
near
, which is a particularly tricky region to capture with this code.
Figure A4.
Solution produced by BLIM2D for the heat transfer coefficient in the laminar area of Han and Palacios’s experiment for several meshes.
Figure A4.
Solution produced by BLIM2D for the heat transfer coefficient in the laminar area of Han and Palacios’s experiment for several meshes.
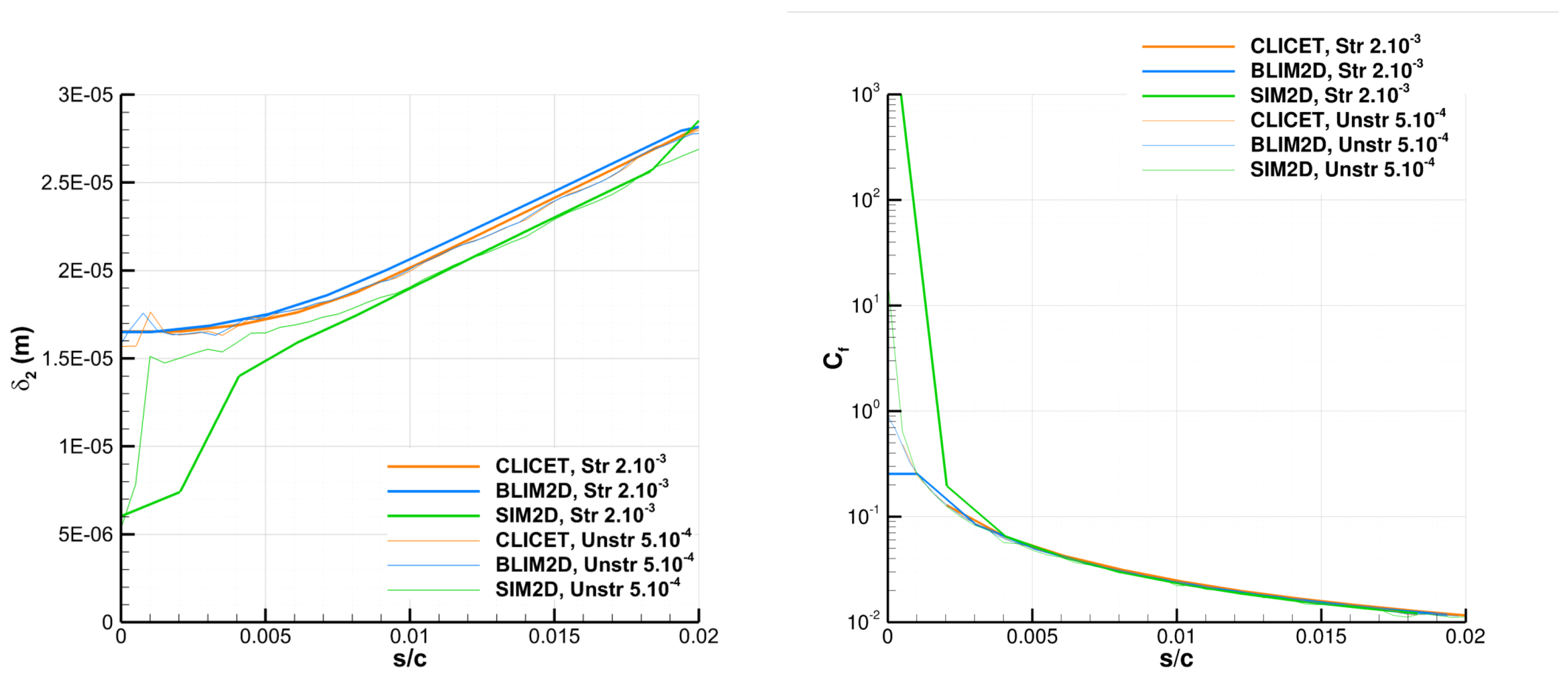
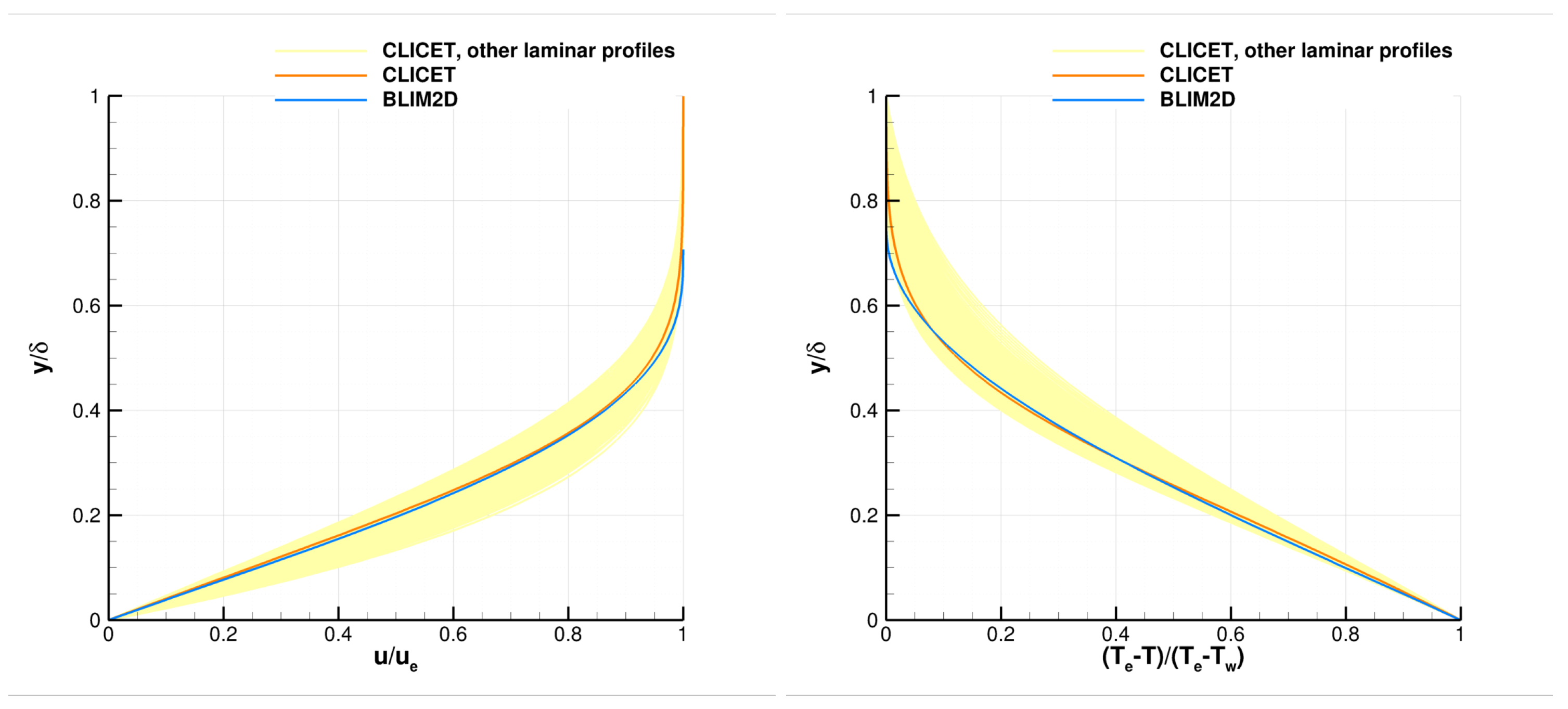
In addition, regarding other classical data of boundary-layer approaches, the agreement is very good between BLIM2D and CLICET on the momentum thickness
and the skin friction coefficient
, as shown in
Figure A5. In addition, BLIM2D’s sensitivity to the mesh is weaker than for
for these dynamic boundary-layer parameters. SIM2D is less accurate for these two variables compared to CLICET (although still providing a rather good approximation), which highlights the lack of generality of the approach.
Figure A5.
Solution produced by CLICET, BLIM2D, and SIM2D for the momentum thickness and the skin friction coefficient in the laminar area of Han and Palacios’s experiment for several meshes. (Left): . (Right): .
Figure A5.
Solution produced by CLICET, BLIM2D, and SIM2D for the momentum thickness and the skin friction coefficient in the laminar area of Han and Palacios’s experiment for several meshes. (Left): . (Right): .
Appendix A.2. Simulations on an Airfoil with Uniform Wall Temperature
For further analysis, comparisons between CLICET, BLIM2D, and SIM2D are performed on the cases of Bayeux’s article [
34] (
Table A1), for which the dynamic boundary layer has already been studied in detail by Bayeux et al. All simulations are again performed by feeding the different boundary-layer codes with the velocity fields computed by the inviscid code EULER2D. In this section, a brief reminder is given on the accuracy of the results obtained for the dynamic boundary layer in Case 1 of
Table A1, with CLICET as the reference. Concerning the thermal boundary layer, the convective heat transfer coefficient
is the key result. There is no ambiguity about the calculation method for the simplified SIM2D method (see
Section 4.3, Equation (
18)). However, both CLICET and BLIM2D perform a heat flux calculation for an imposed wall temperature. In practice, for these two codes, the heat transfer coefficient is thus derived from the linearization:
where
and
are, respectively, the two wall temperatures imposed for two different simulations,
and
are the wall heat fluxes produced for each of these simulations. For CLICET,
K and
K [
35]. For BLIM2D, as the method does not allow simulating cooled walls,
K and
K [
35]. It has been verified in Bayeux’s Ph.D. thesis [
35] that the impact of the choice of
and
is small (in particular by using
K and
K for CLICET on some cases).
Table A1.
Test cases for the analysis of the boundary-layer solvers.
Table A1.
Test cases for the analysis of the boundary-layer solvers.
| Case | Profile | c (m) | AOA () | | (K) | (Pa) |
|---|
| Case 1 | NACA0012 | 0.500 | 4 | 0.30 | 263 | 80,000 |
| Case 2 | NACA0012 | 0.500 | 0 | 0.15 | 263 | 80,000 |
| Case 3 | MS317 | 0.914 | 0 | 0.2420 | 263 | 101,325 |
| Case 4 | MS317 | 0.914 | 8 | 0.2420 | 263 | 101,325 |
| Case 5 | GLC305 | 0.9144 | 4.5 | 0.2730 | 268.30 | 101,325 |
| Case 6 | GLC305 | 0.9144 | 1.5 | 0.3940 | 263.60 | 101,325 |
Cases 1 and 2 of
Table A1 are NACA0012 airfoils for which the Vassberg and Jameson meshes were used [
51]. In Case 2 of
Table A1 in particular, it was shown that the mesh composed of 1024 points on the surface of the airfoil ensures mesh convergence [
34]. Therefore, this mesh was used here for Case 1 as well. To first address the results of the dynamic boundary layer,
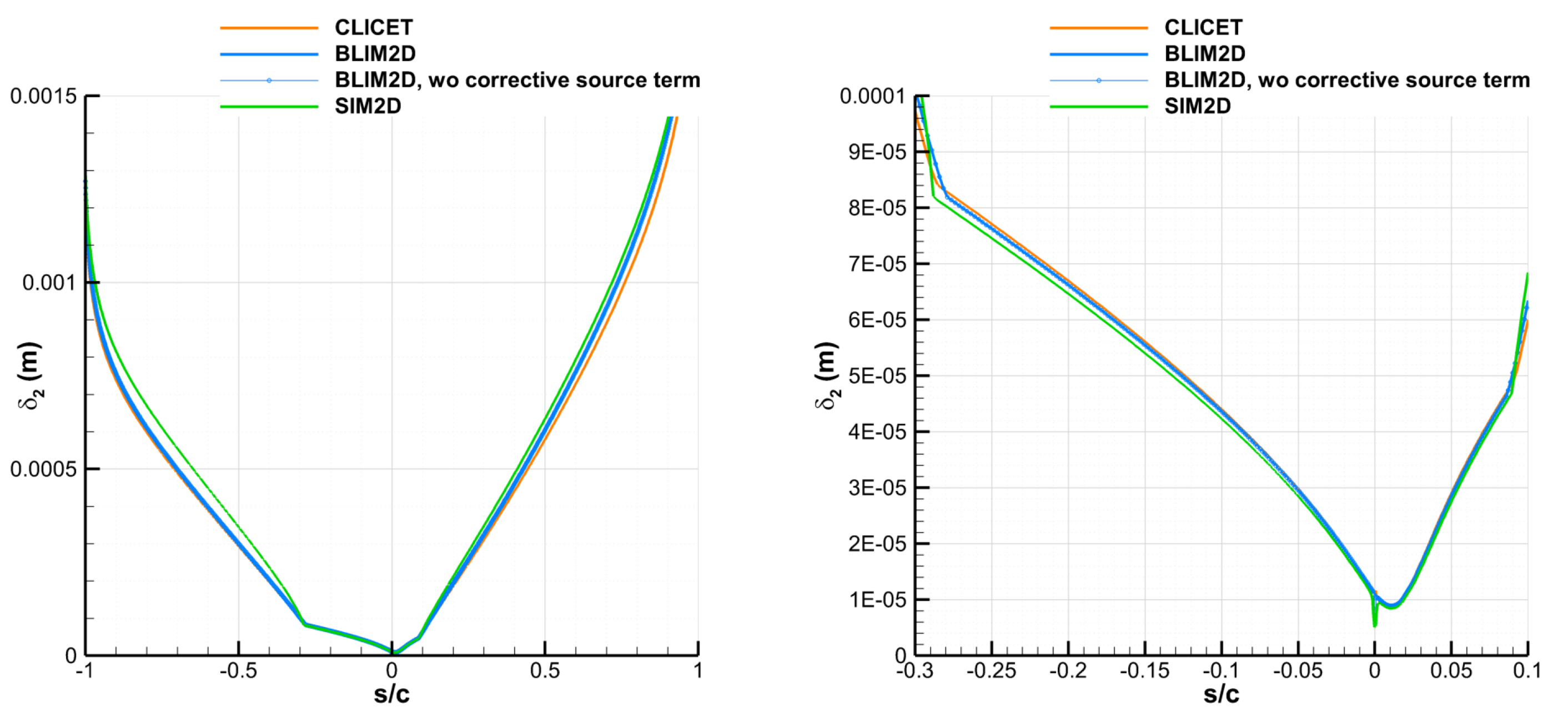
Figure A6 (left) shows that the shape factor
is well reproduced by BLIM2D, compared to CLICET.
Figure A6 (right) shows the interest in introducing a corrective source term for the numerical issue faced at the stagnation point (
), which was shown in Case 2 in Bayeux’s article [
34]. This source term corrects a significant error in
H, and it affects only the immediate vicinity of
. It also affects the dynamic integral thicknesses, such as the momentum thickness
(
Figure A7, right). Regarding
,
Figure A7 (left) shows that BLIM2D captures very well the evolution predicted by CLICET in the laminar area (
in the range between −0.3 and 0.1), especially on pressure side (
). The results are still very good outside this area but a little less accurate. The simplified SIM2D method produces slightly worse results.
Figure A6.
Solution produced by CLICET and BLIM2D for the shape factor
H in Case 1 of
Table A1. (
Left): whole airfoil. (
Right): focus in the vicinity of the stagnation point.
Figure A6.
Solution produced by CLICET and BLIM2D for the shape factor
H in Case 1 of
Table A1. (
Left): whole airfoil. (
Right): focus in the vicinity of the stagnation point.
Figure A7.
Solution produced by CLICET, SIM2D, and BLIM2D for the momentum thickness
in Case 1 of
Table A1. (
Left): whole airfoil. (
Right): laminar area.
Figure A7.
Solution produced by CLICET, SIM2D, and BLIM2D for the momentum thickness
in Case 1 of
Table A1. (
Left): whole airfoil. (
Right): laminar area.
The resolution of the thermal boundary layer does not suffer from the issue identified at the stagnation point for the dynamic boundary layer (which is related to the fact that the numerical flux term evolves linearly with respect to
and that the issue comes from discretization errors of derivatives of
terms present in the flux terms, with exponents
n larger than one, as shown in Bayeux’s article [
34]).
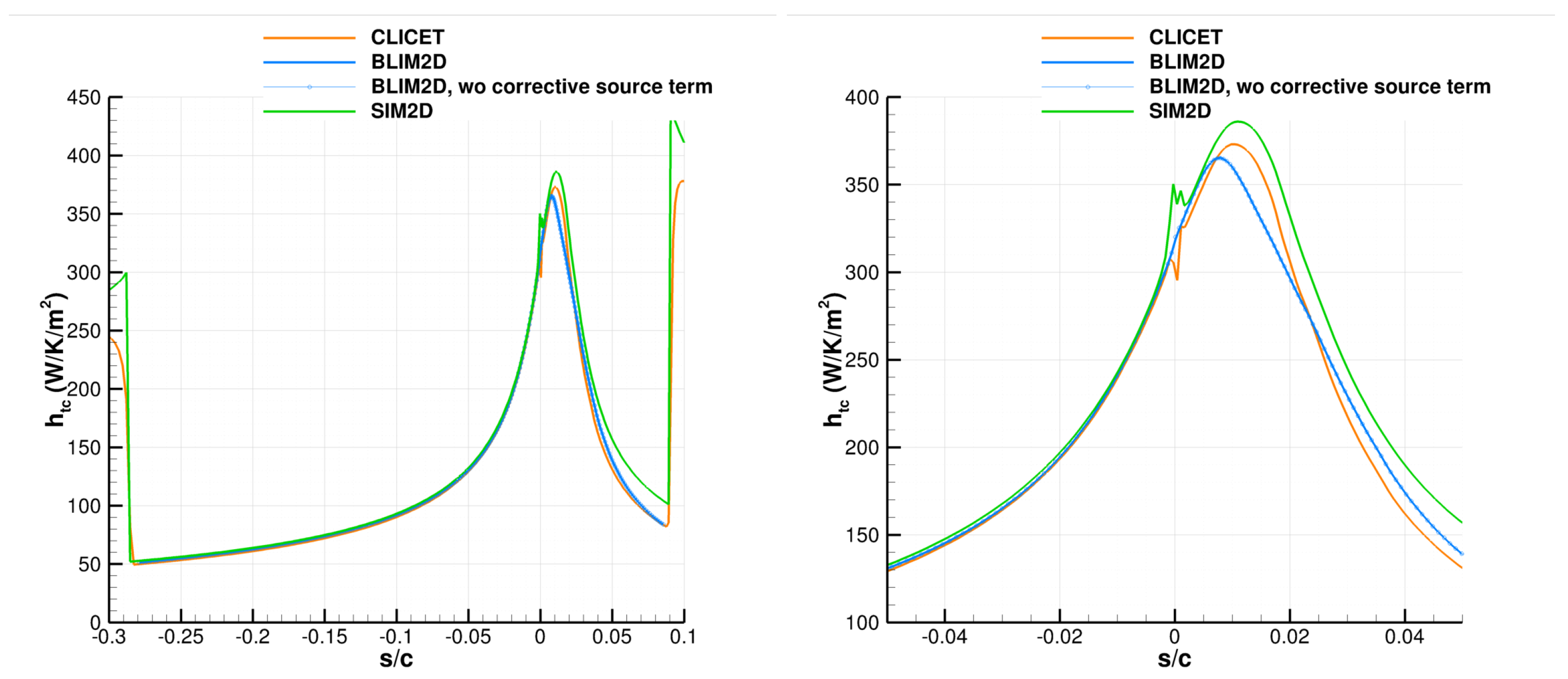
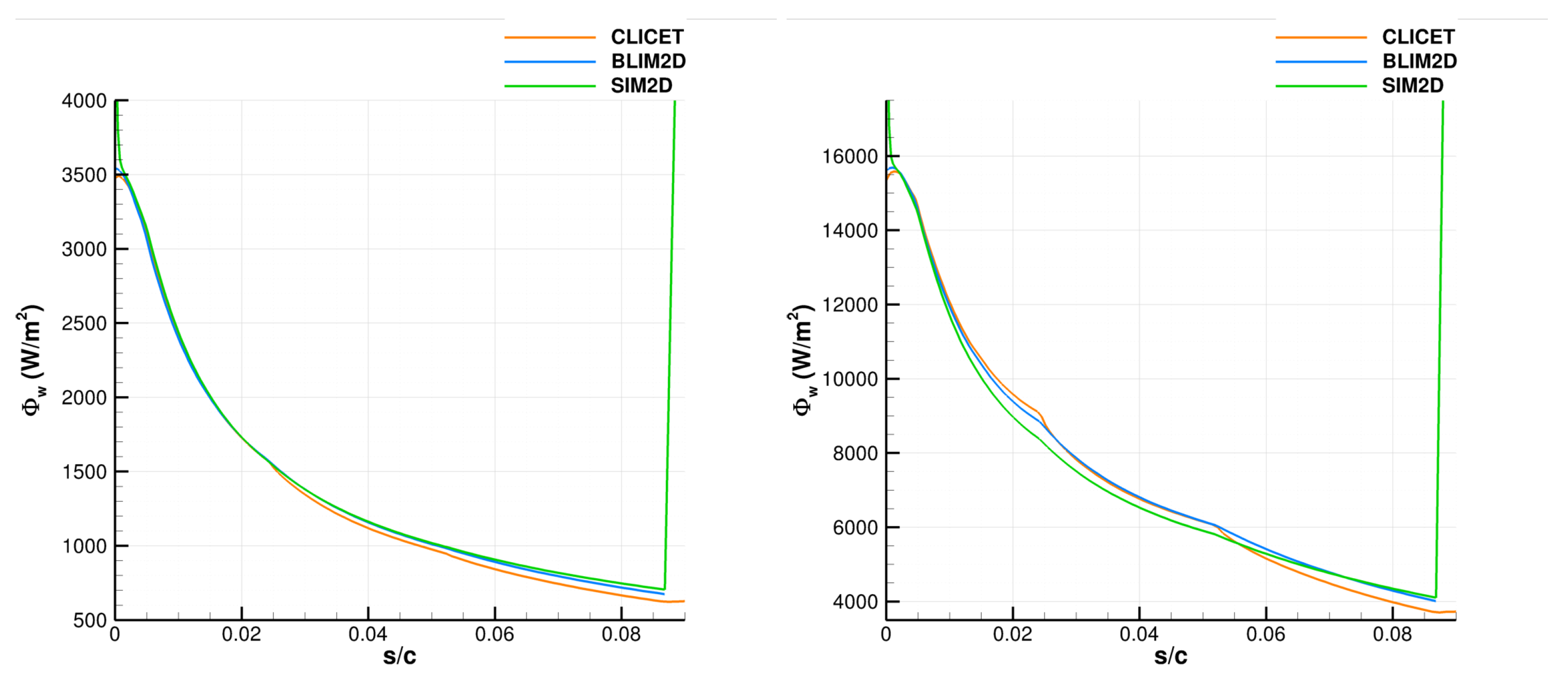
Figure A8 (right) indeed shows that
is the same whether the corrective source term is activated or not. This area is even simulated more smoothly than for CLICET. Moreover,
Figure A8 (left) shows the very good agreement between BLIM2D and CLICET on
in the whole laminar area. The BLIM2D method for the thermal boundary layer was developed for the laminar regime only, so the comparison is limited to this region. The SIM2D solution is quite correct, which justifies the widespread use of such simplified approaches for ice-accretion calculations. However, the BLIM2D solution is rather better at the stagnation point for the maximum value of
(in the accelerated area of the suction side of the airfoil) and on the suction side in general.
Figure A8.
Solution produced by CLICET, SIM2D, and BLIM2D for the convective heat transfer coefficient
in Case 1 of
Table A1. (
Left): laminar area. (
Right): focus in the vicinity of the stagnation point.
Figure A8.
Solution produced by CLICET, SIM2D, and BLIM2D for the convective heat transfer coefficient
in Case 1 of
Table A1. (
Left): laminar area. (
Right): focus in the vicinity of the stagnation point.
Regarding the other cases of
Table A1, the same observations are made concerning
.
Table A2 indeed shows that the average error (relative error L
, compared to CLICET) in the laminar region is indeed systematically higher for SIM2D, reaching almost
for Case 6, while it is limited to around
for BLIM2D (Cases 4, 5, and 6). The error at the stagnation point is even higher. With the exception of Case 4 for which the CLICET solution is oscillating near
. the error made by SIM2D is indeed again systematically larger (in general significantly) than for BLIM2D.
Table A2.
Relative errors in
in the laminar area for the six cases of
Table A1. L2-norm relative error
. Relative error at the stagnation point
.
Table A2.
Relative errors in
in the laminar area for the six cases of
Table A1. L2-norm relative error
. Relative error at the stagnation point
.
| Case | | | | |
|---|
| Case 1 | 0.0400 | 0.0729 | 0.0302 | 0.1445 |
| Case 2 | 0.0157 | 0.0568 | 0.0227 | 0.2813 |
| Case 3 | 0.0132 | 0.0450 | 0.0486 | 0.1461 |
| Case 4 | 0.0528 | 0.0668 | 0.2047 | 0.0384 |
| Case 5 | 0.0463 | 0.0662 | 0.0549 | 0.1476 |
| Case 6 | 0.0540 | 0.0965 | 0.0742 | 0.1476 |
Even if SIM2D can capture these cases well, BLIM2D reduces the error on , even for these very simple cases where the wall has a nearly constant temperature.