Deployment Modes and Aerodynamic Analysis of UAV Orthogonal Biaxial Folding Wing
Abstract
1. Introduction
2. Materials and Methods
2.1. Design of Deployment Modes of the Orthogonal Biaxial Folding Wing
2.1.1. Analysis of the Folding Wing Development Condition
2.1.2. Orthogonal Biaxial Folding Wing Multiple Deployment Modes’ Design
2.2. Aerodynamic Analysis of the Orthogonal Biaxial Folding Wing during Deployment
2.2.1. Aerodynamic Analysis of Synchronous Deployment Mode of Folding Wing
2.2.2. Aerodynamic Analysis of Stepwise Deployment Mode of Folding Wing
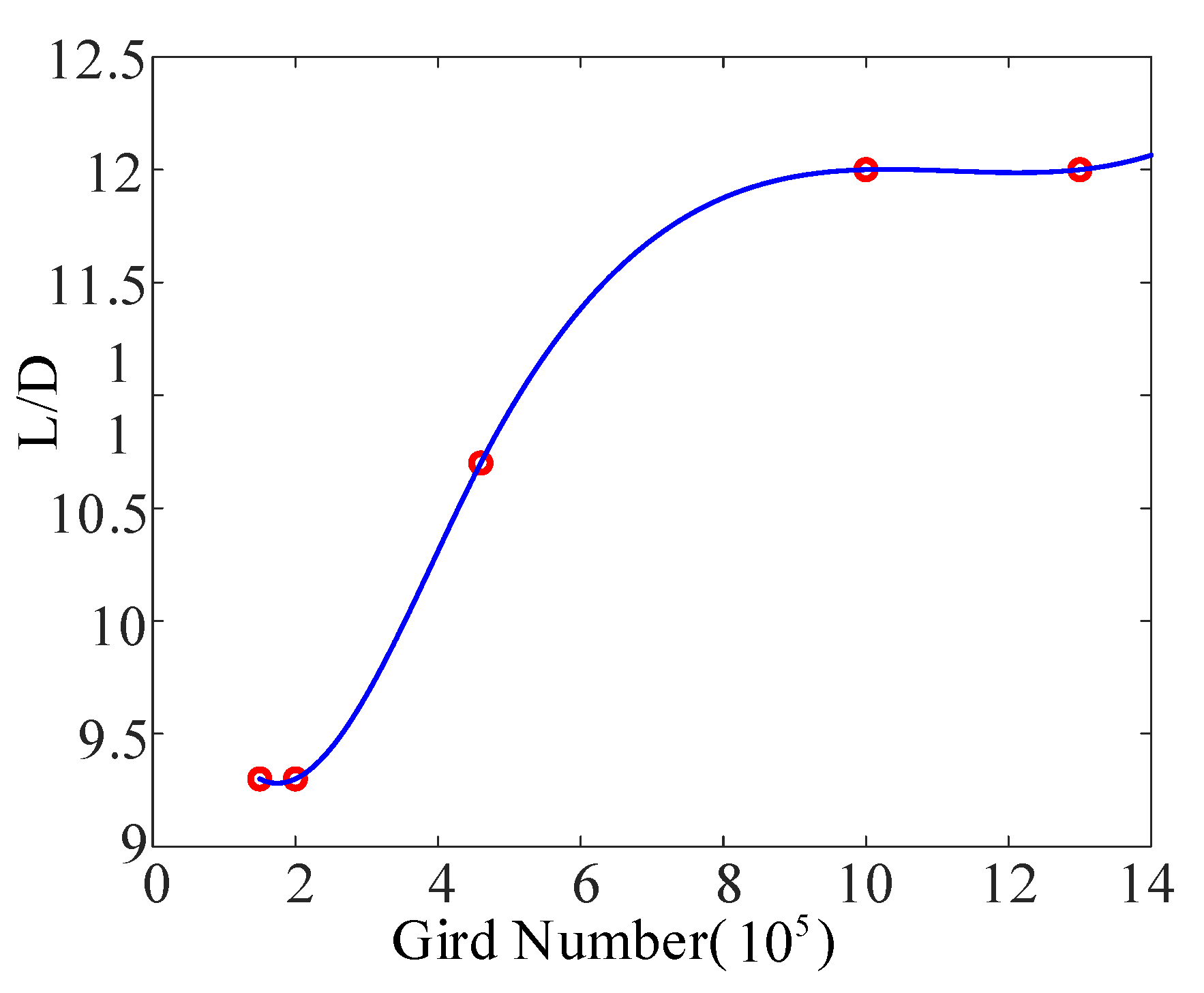
2.3. Aerodynamic Example and Modeling of the Orthogonal Biaxial Folding Wing during Deployment
3. Results and Discussions
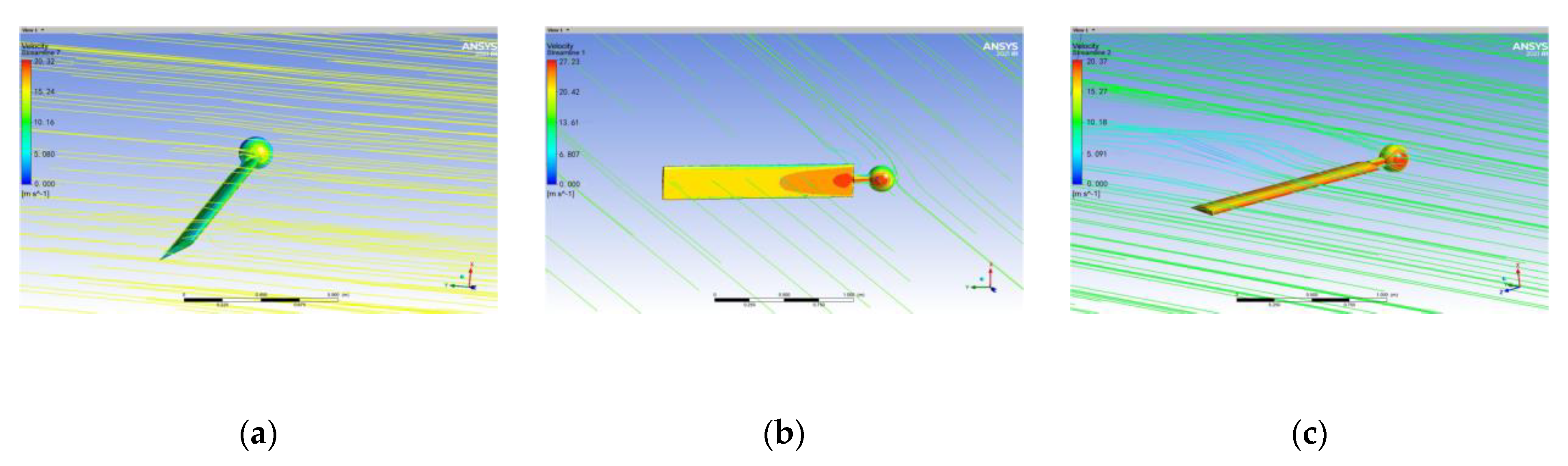
3.1. Aerodynamic Simulation of the Folding Wing under Different Airspeed and Deployment Modes


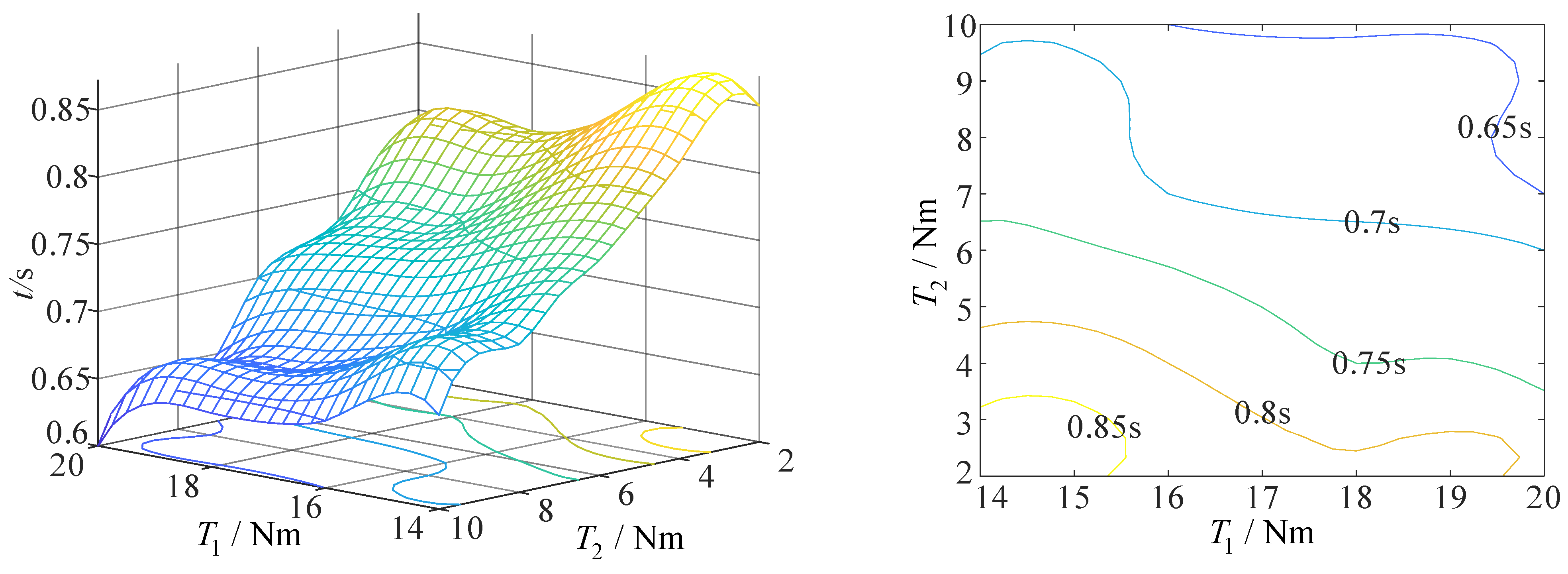
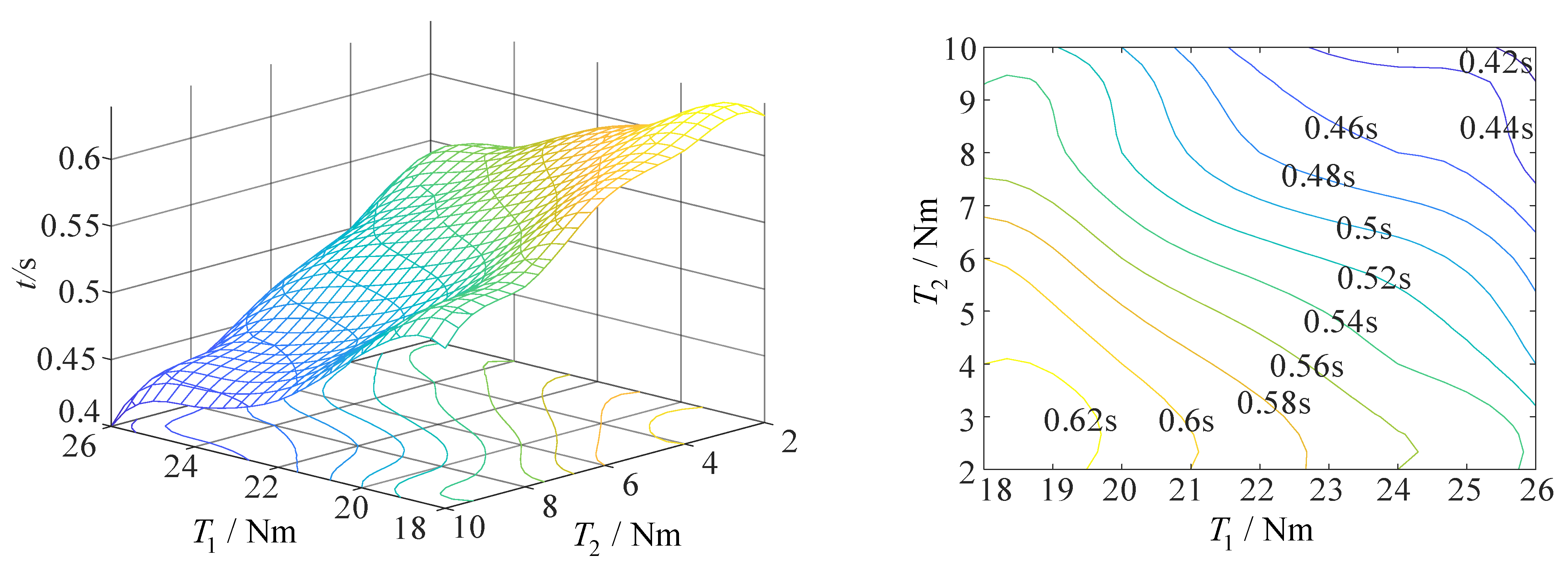


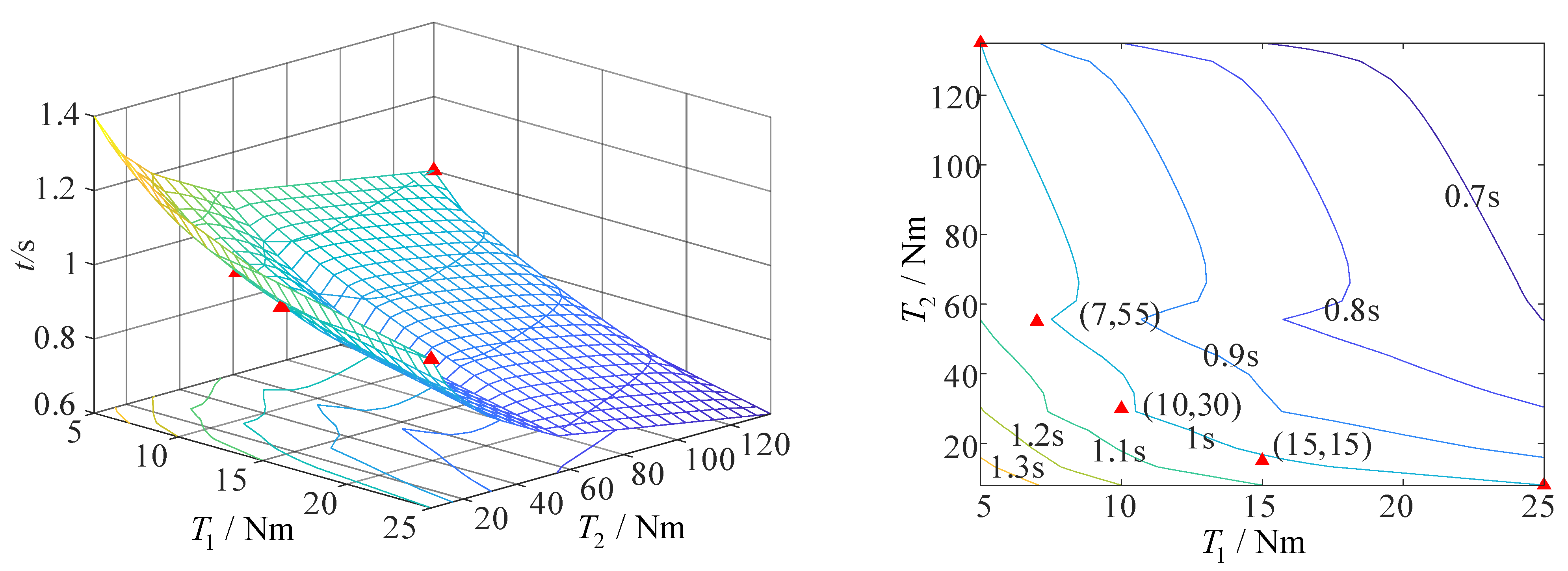

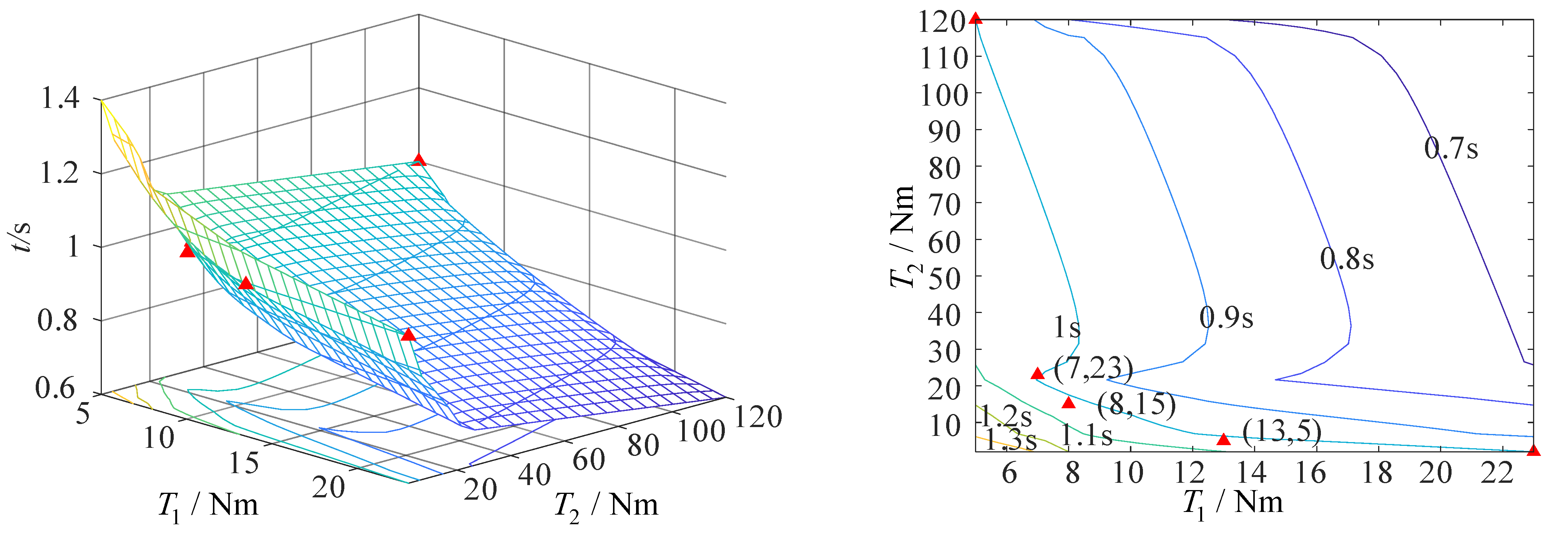
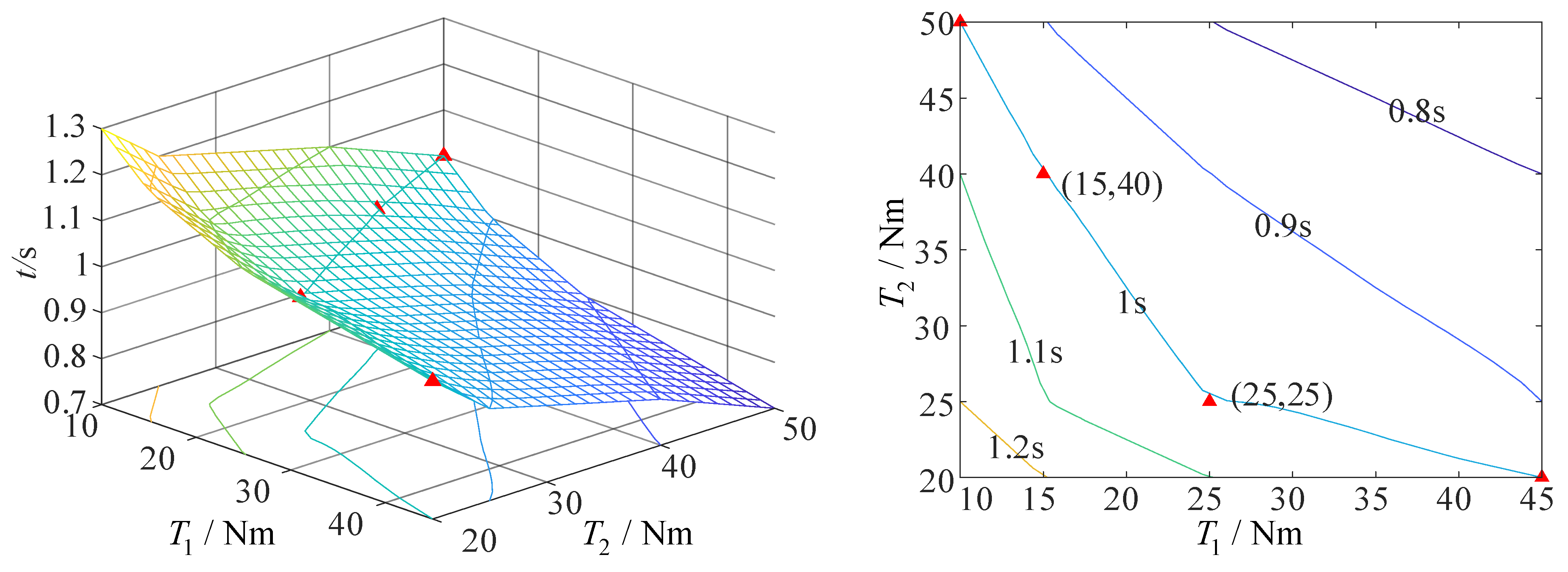
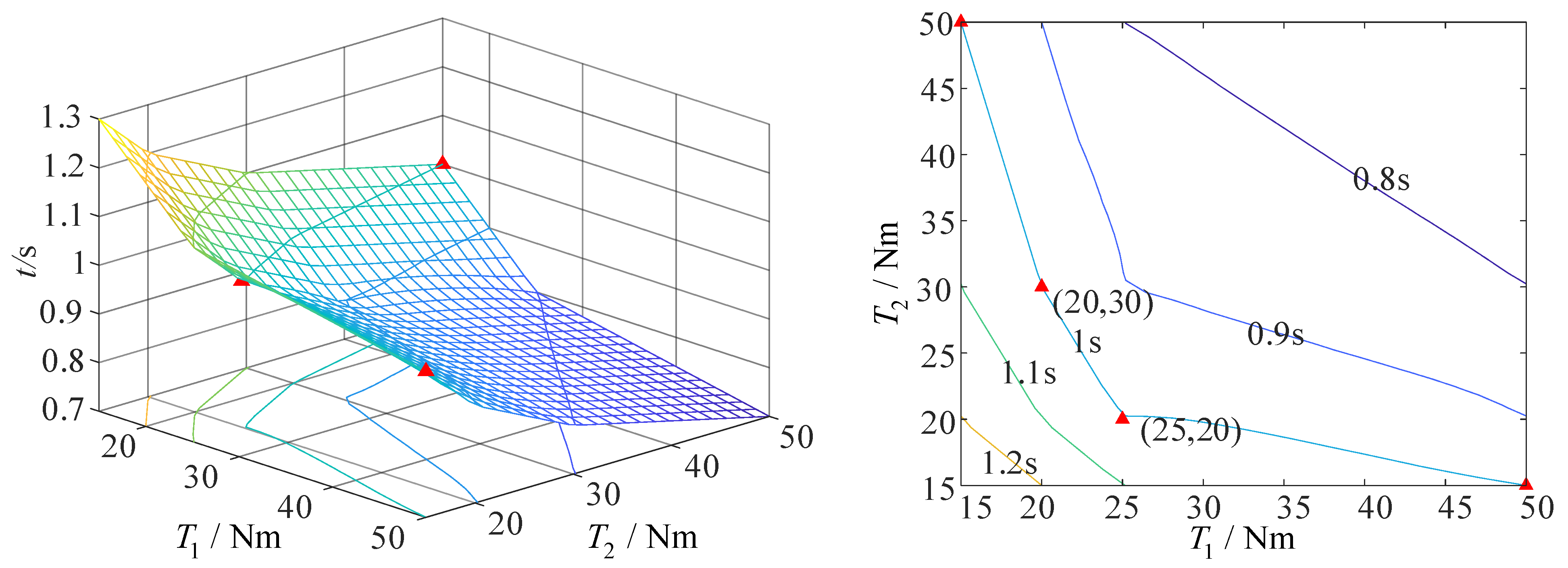
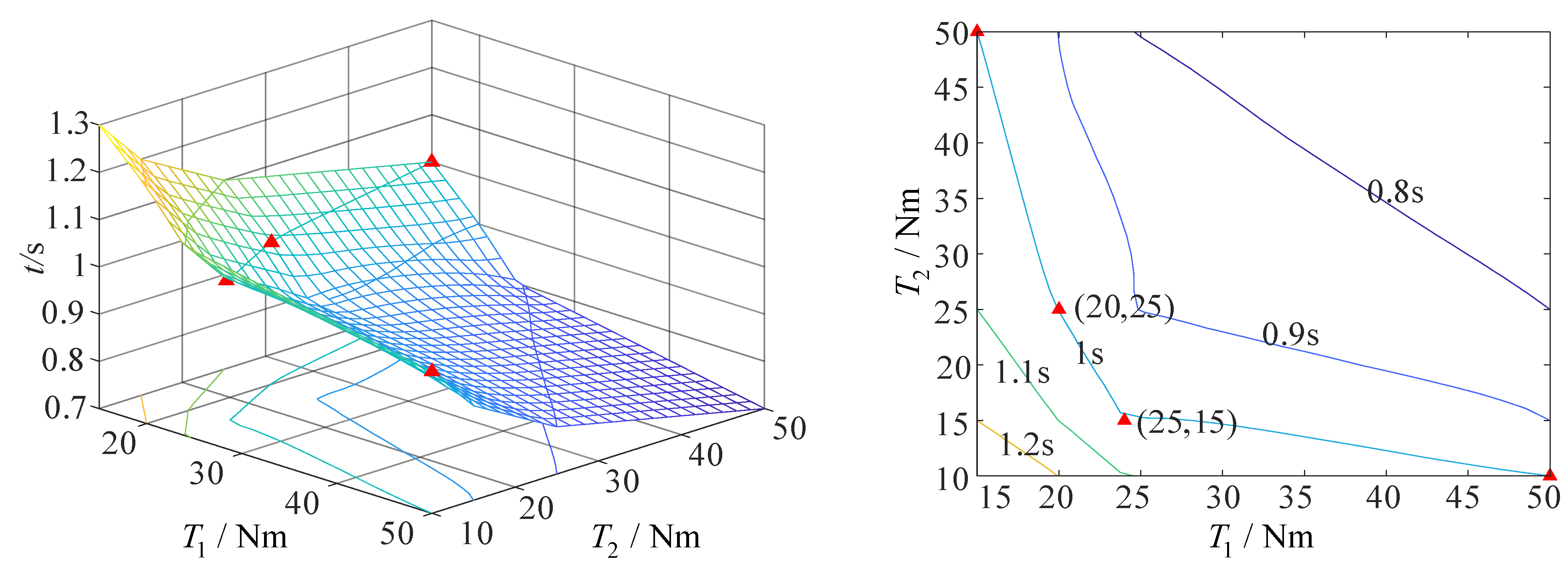
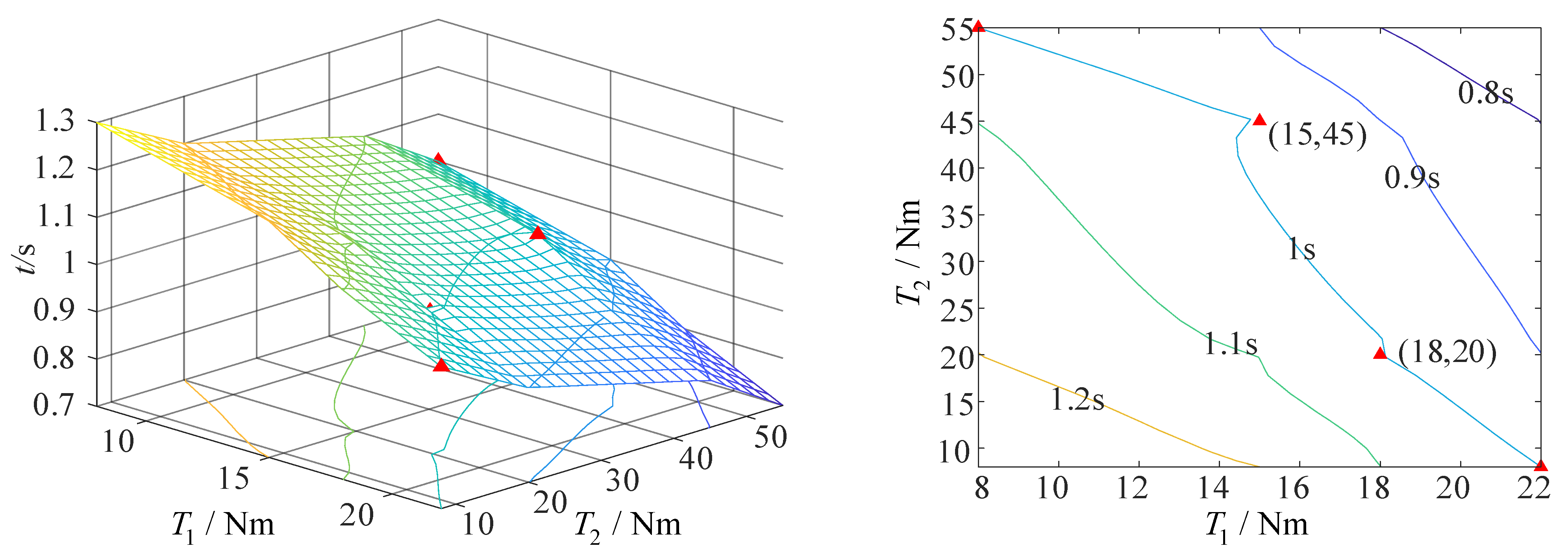
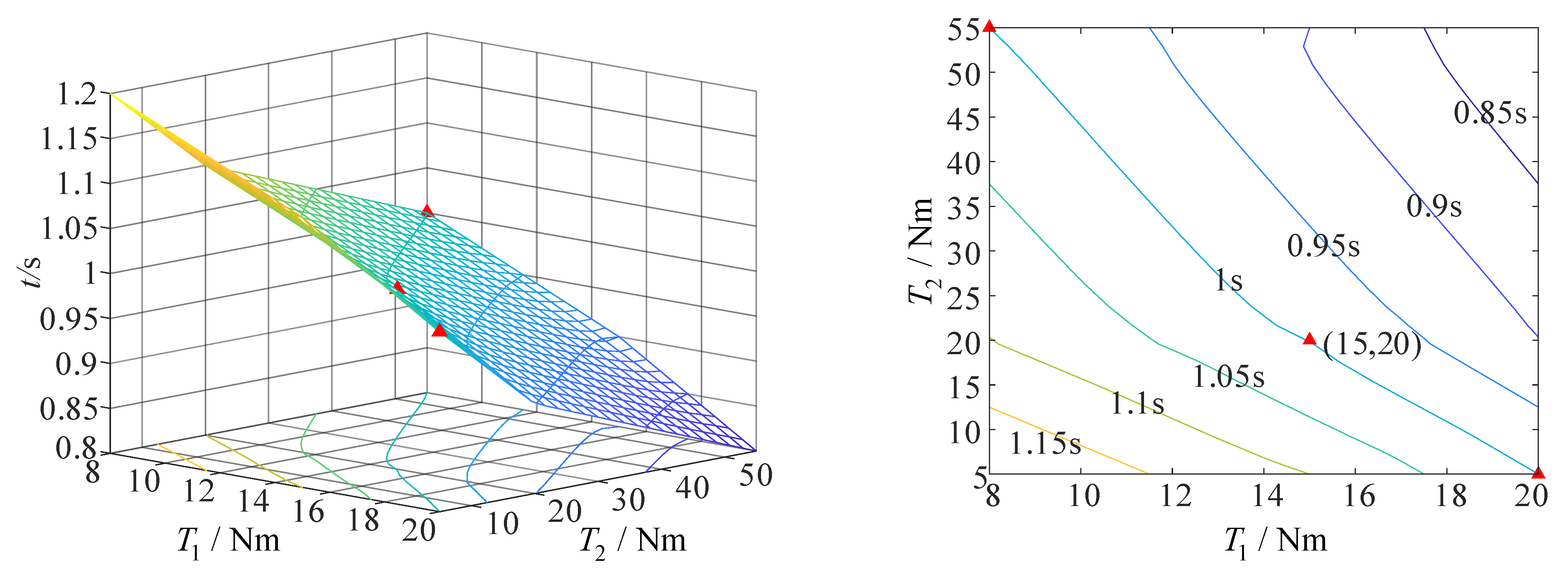
3.2. Comparative Analysis of Simulation Results and Theoretical Calculation Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ozel, C.; Özbek, E.; Ekici, S. A Review on applications and effects of morphing wing technology on UAVs. Int. J. Aviat. Sci. Technol. 2021, 1, 30–40. [Google Scholar]
- Lee, C.; Kim, S.; Chu, B. A survey: Flight mechanism and mechanical structure of the UAV. Int. J. Precis. Eng. Manuf. 2021, 22, 719–743. [Google Scholar] [CrossRef]
- Li, D.; Zhao, S.; Da Ronch, A.; Xiang, J.; Drofelnik, J.; Li, Y.; Zhang, L.; Wu, Y.; Kintscher, M.; Monner, H.P. A review of modelling and analysis of morphing wings. Prog. Aerosp. Sci. 2018, 100, 46–62. [Google Scholar] [CrossRef]
- Burdette, D.A.; Martins, J.R.R.A. Design of a transonic wing with an adaptive morphing trailing edge via aerostructural optimization. Aerosp. Sci. Technol. 2018, 81, 192–203. [Google Scholar] [CrossRef]
- Arena, M.; Concilio, A.; Pecora, R. Aero-servo-elastic design of a morphing wing trailing edge system for enhanced cruise performance. Aerosp. Sci. Technol. 2019, 86, 215–235. [Google Scholar] [CrossRef]
- Weaver-Rosen, J.M.; Leal, P.B.; Hartl, D.J.; Malak, R.J. Parametric optimization for morphing structures design: Application to morphing wings adapting to changing flight conditions. Struct. Multidiscip. Optim. 2020, 62, 2995–3007. [Google Scholar] [CrossRef]
- Gao, L.; Zhu, Y.; Liu, Y.; Zhang, J.; Liu, B.; Zhao, J. Analysis and Control for the Mode Transition of Tandem-Wing Aircraft with Variable Sweep. Aerospace 2022, 9, 463. [Google Scholar] [CrossRef]
- Geva, A.; Abramovich, H.; Arieli, R. Investigation of a Morphing Wing Capable of Airfoil and Span Adjustment Using a Retractable Folding Mechanism. Aerospace 2019, 6, 85. [Google Scholar] [CrossRef]
- Guo, T.; Feng, L.; Zhu, C.; Zhou, X.; Chen, H. Conceptual Research on a Mono-Biplane Aerodynamics-Driven Morphing Aircraft. Aerospace 2022, 9, 380. [Google Scholar] [CrossRef]
- Hui, Z.; Zhang, Y.; Chen, G. Aerodynamic performance investigation on a morphing unmanned aerial vehicle with bio-inspired discrete wing structures. Aerosp. Sci. Technol. 2019, 95, 105419. [Google Scholar] [CrossRef]
- Zhu, Z.; Guo, H.; Ma, J. Aerodynamic layout optimization design of a barrel-launched UAV wing considering control capability of multiple control surfaces. Aerosp. Sci. Technol. 2019, 93, 105297. [Google Scholar] [CrossRef]
- Mills, J.; Ajaj, R. Flight dynamics and control using folding wingtips: An experimental study. Aerospace 2017, 4, 19. [Google Scholar] [CrossRef]
- Xu, H.; Han, J.; Yun, H.; Chen, X. Calculation of the hinge moments of a folding wing aircraft during the flight-folding process. Int. J. Aerosp. Eng. 2019, 2019, 9362629. [Google Scholar] [CrossRef]
- Dussart, G.; Yusuf, S.; Lone, M. Identification of in-flight wingtip folding effects on the roll characteristics of a flexible aircraft. Aerospace 2019, 6, 63. [Google Scholar] [CrossRef]
- Fujita, K.; Nagai, H. Robustness analysis on aerial deployment motion of a Mars aircraft using multibody dynamics simulation: Effects of wing-unfolding torque and timing. Aeronaut. J. 2017, 121, 449–468. [Google Scholar] [CrossRef]
- Guo, X.; Wang, S.; Qu, Y.; Cao, D. Nonlinear dynamics of Z-shaped morphing wings in subsonic flow. Aerosp. Sci. Technol. 2021, 119, 107145. [Google Scholar] [CrossRef]
- Kan, Z.; Li, D.; Shen, T.; Xiang, J.; Zhang, L. Aerodynamic characteristics of morphing wing with flexible leading-edge. Chin. J. Aeronaut. 2020, 33, 2610–2619. [Google Scholar] [CrossRef]
- Otsuka, K.; Makihara, K. Aeroelastic deployable wing simulation considering rotation hinge joint based on flexible multibody dynamics. J. Sound Vib. 2016, 369, 147–167. [Google Scholar] [CrossRef]
- Otsuka, K.; Wang, Y.; Makihara, K. Deployable wing model considering structural flexibility and aerodynamic unsteadiness for deployment system design. J. Sound Vib. 2017, 408, 105–122. [Google Scholar] [CrossRef]
- Rosid, N.H.; Lukman, E.I.; Fadlillah, M.A.; Moelyadi, M.A. Aerodynamic Characteristics of Tube-Launched Tandem Wing Unmanned Aerial Vehicle. In Proceedings of the Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2018; p. 012015. [Google Scholar]
- Tiegang, L.; Guoguang, C.; Shuai, L. Kinematic characteristics of longitudinal double folding wings. Aeronaut. J. 2021, 125, 1977–2001. [Google Scholar] [CrossRef]
- Zhang, J.; Shaw, A.D.; Wang, C.; Gu, H.; Amoozgar, M.; Friswell, M.I.; Woods, B.K. Aeroelastic model and analysis of an active camber morphing wing. Aerosp. Sci. Technol. 2021, 111, 106534. [Google Scholar] [CrossRef]
- Yan, B.; Li, Y.; Dai, P.; Liu, S. Aerodynamic analysis, dynamic modeling, and control of a morphing aircraft. J. Aerosp. Eng. 2019, 32, 04019058. [Google Scholar] [CrossRef]
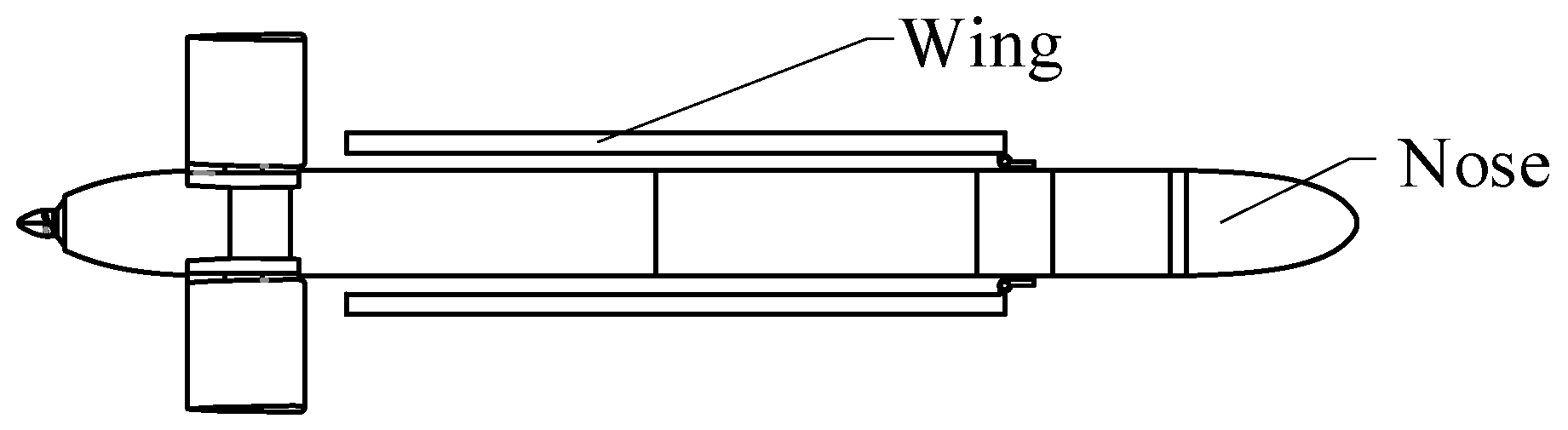
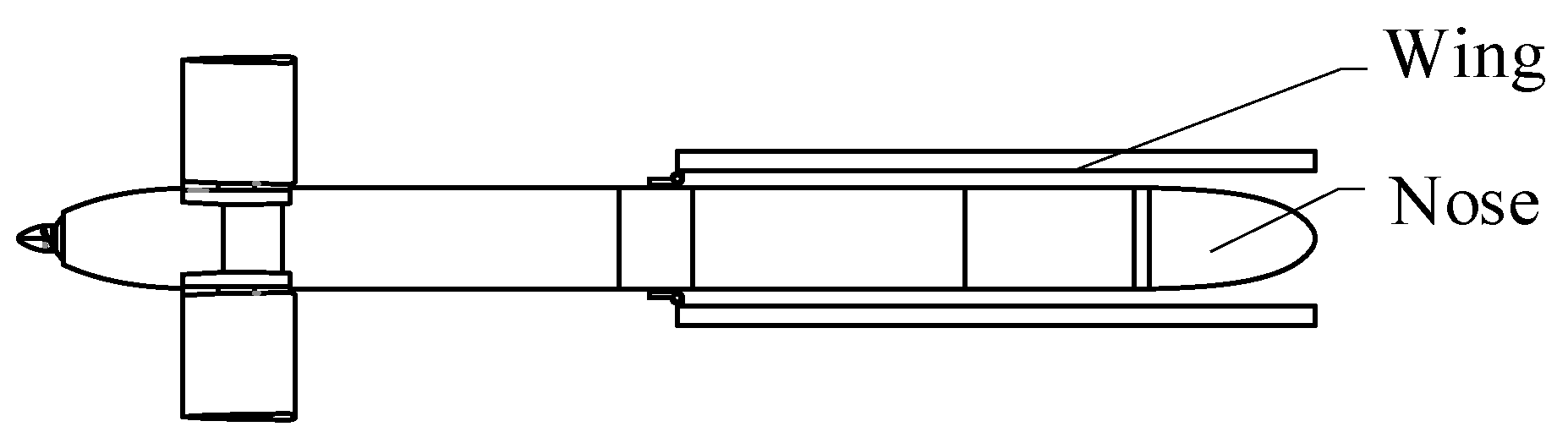
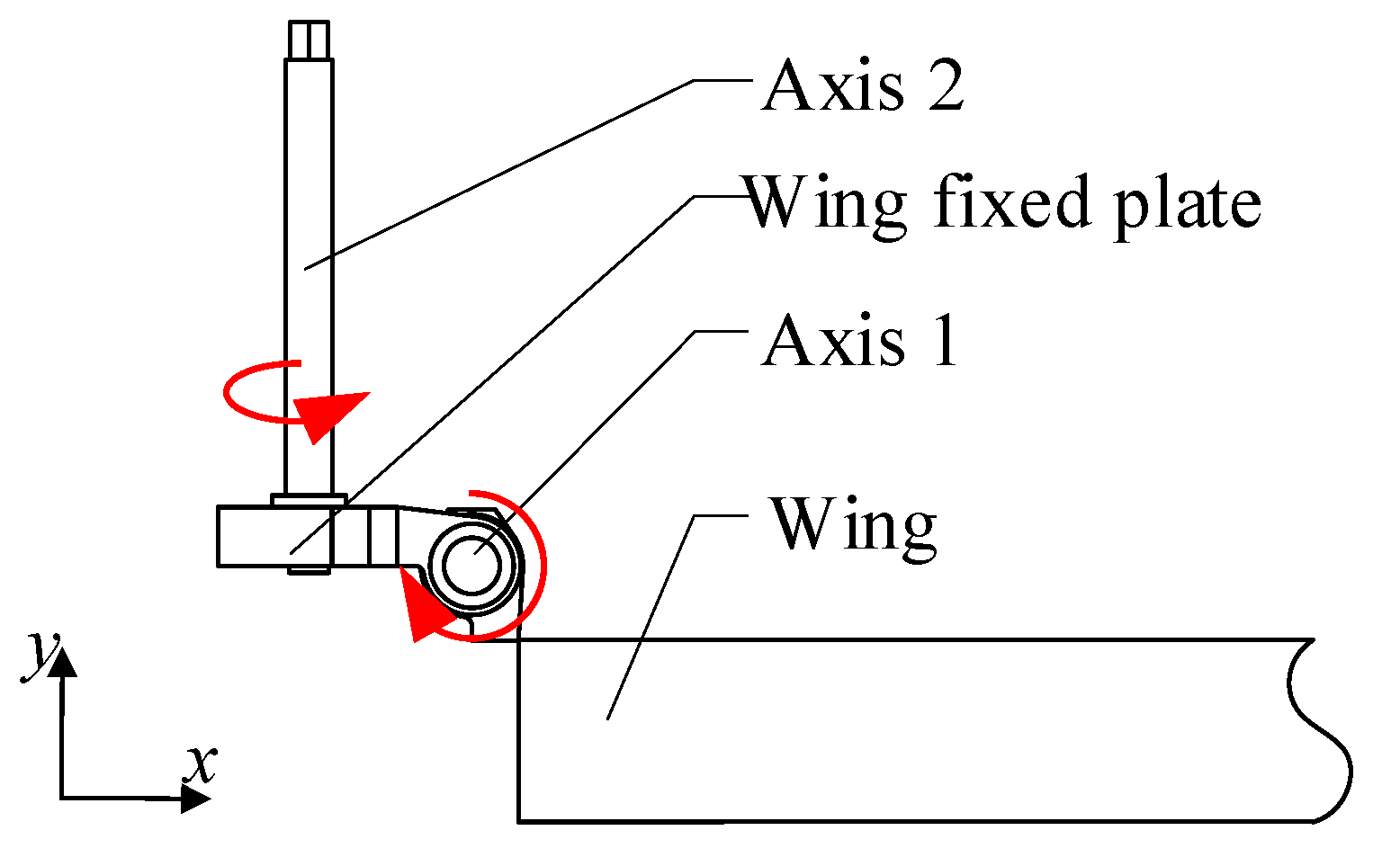
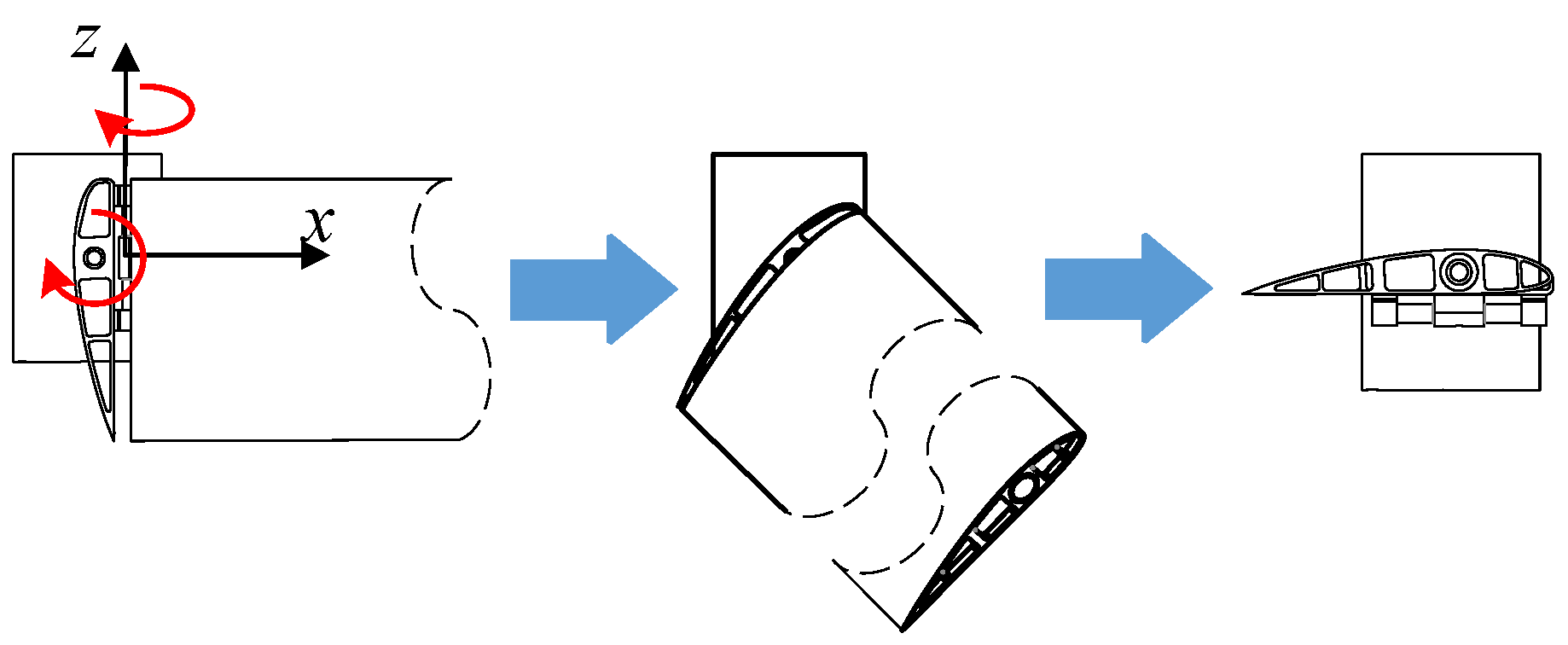
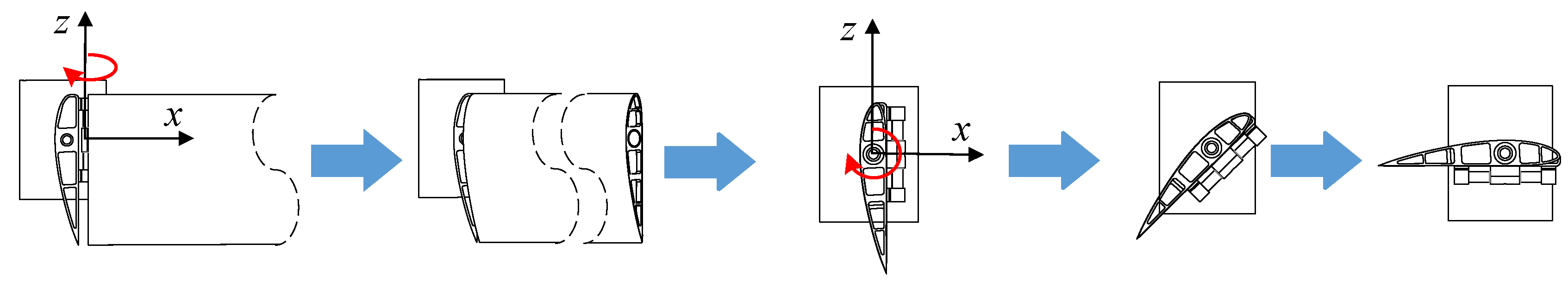
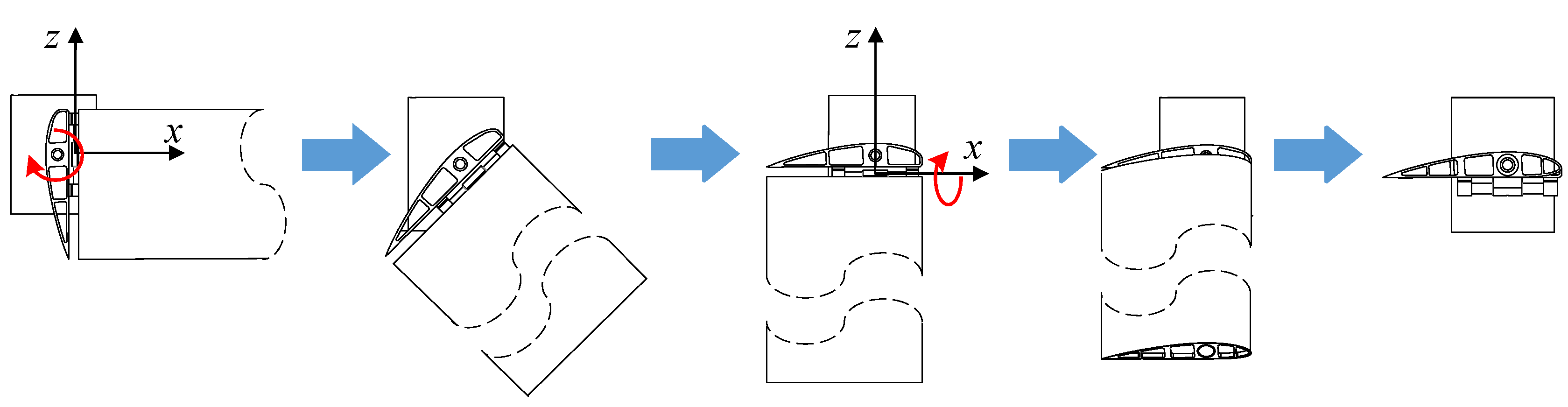
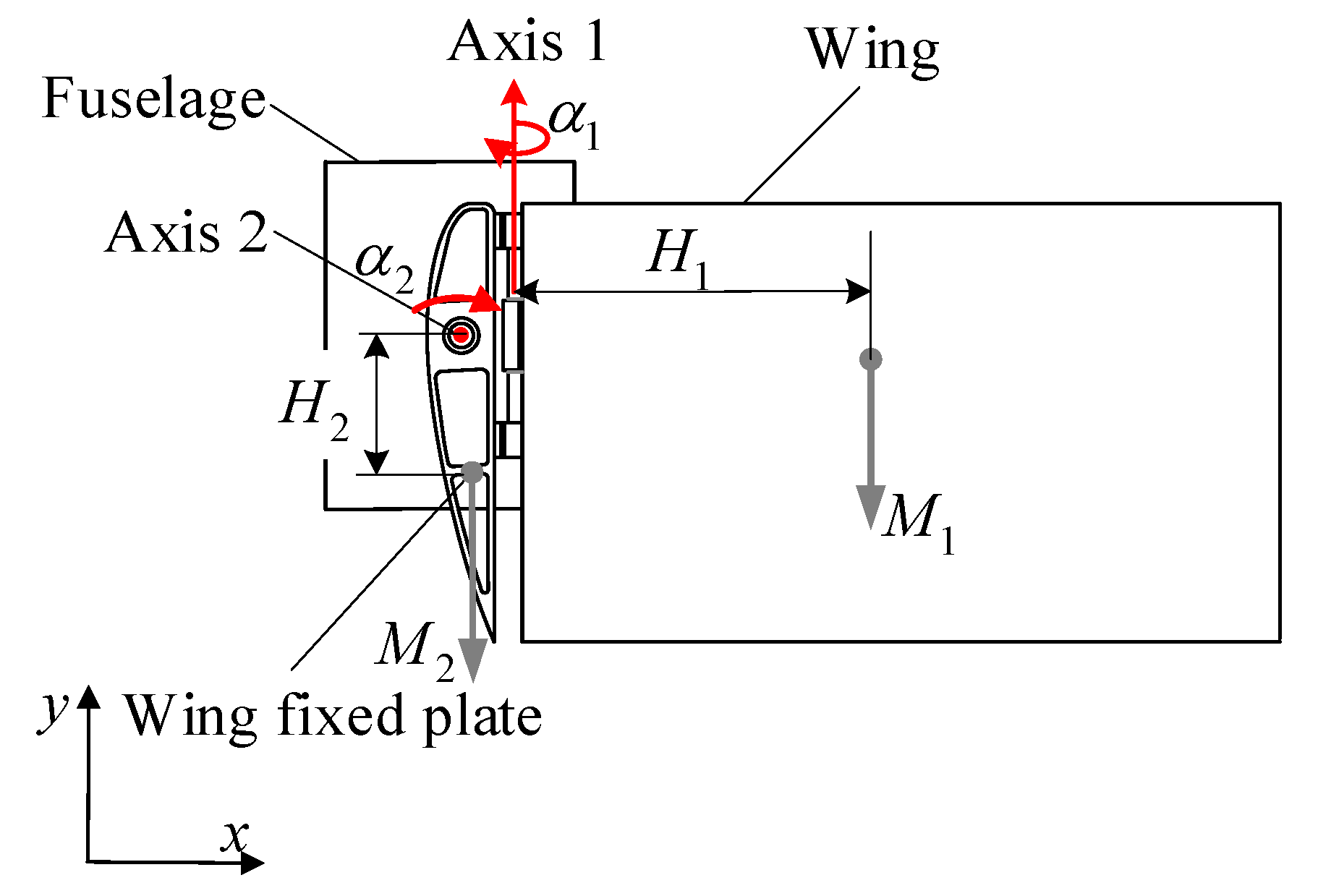
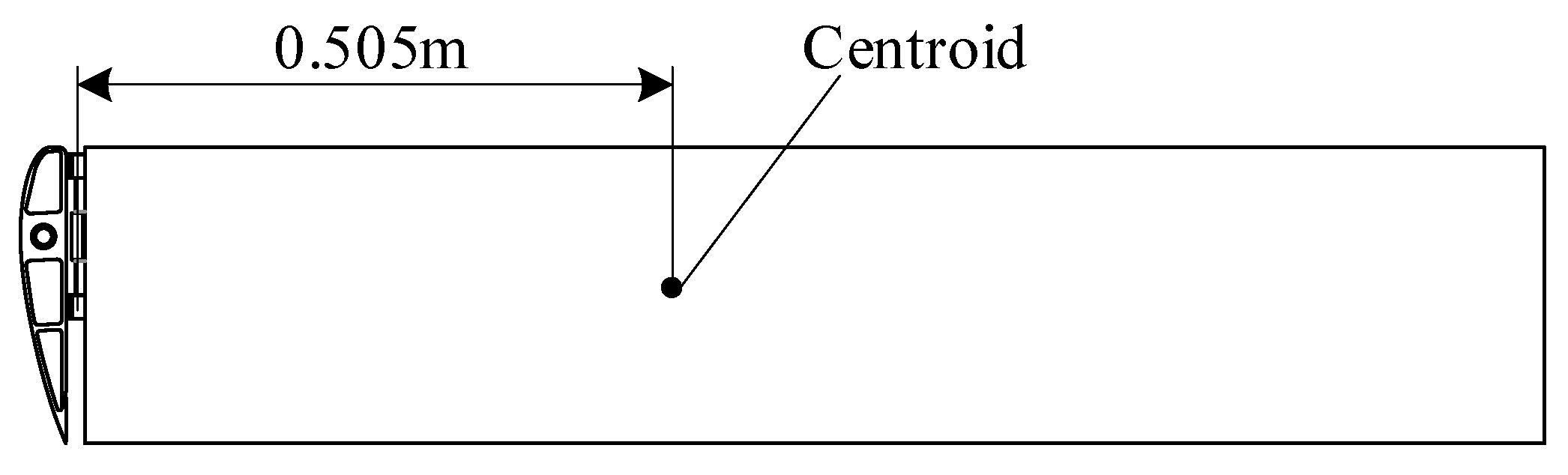


| Mass of Wing | The Moment of Inertia of the Centroid along the Three Coordinate Axes |
|---|---|
| 1.56 kg | Ixx = 2.6362 × 105 kg·mm2 |
| Iyy = 2.6824 × 105 kg·mm2 | |
| Izz = 5.051 × 103 kg·mm2 |
| Airspeed (m/s) | Air Density (kg/m3) | Air Viscosity (pa·s) |
|---|---|---|
| 10/20/30/40/50 | 1.205 | 2.593 × 10−5 |
| Airspeed (m/s) | Synchronous Deployment Mode | Non-Fixed-Axis–Fixed-Axis Stepwise Deployment Mode | Fixed-Axis–Non-Fixed-Axis Stepwise Deployment Mode | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Deployment Time (s) | Driving Torque (Nm) | Deployment Time (s) | Driving Torque (Nm) | Deployment Time (s) | Driving Torque (Nm) | ||||
| Axis 1 | Axis 2 | Axis 1 | Axis 2 | Axis 1 | Axis 2 | ||||
| 10 | 1 | 14 | 14 | 1 | 26 | 20 | 1 | 25 | 25 |
| 20 | 1 | 10 | 8 | 1 | 16 | 22 | 1 | 25 | 20 |
| 30 | 0.85 | 12 | 2 | 1 | 15 | 15 | 1 | 24 | 15 |
| 40 | 0.63 | 18 | 2 | 1 | 15 | 8 | 1 | 15 | 8 |
| 50 | - | - | - | 1 | 13 | 5 | 1 | 20 | 5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Xu, G.; Wang, Y.; Yao, Y.; Wang, K.; Hu, X.; Sun, H.; Liu, J. Deployment Modes and Aerodynamic Analysis of UAV Orthogonal Biaxial Folding Wing. Aerospace 2023, 10, 26. https://doi.org/10.3390/aerospace10010026
Wang G, Xu G, Wang Y, Yao Y, Wang K, Hu X, Sun H, Liu J. Deployment Modes and Aerodynamic Analysis of UAV Orthogonal Biaxial Folding Wing. Aerospace. 2023; 10(1):26. https://doi.org/10.3390/aerospace10010026
Chicago/Turabian StyleWang, Gang, Guosheng Xu, Yukun Wang, Yimeng Yao, Kun Wang, Xi Hu, Heyao Sun, and Jingwang Liu. 2023. "Deployment Modes and Aerodynamic Analysis of UAV Orthogonal Biaxial Folding Wing" Aerospace 10, no. 1: 26. https://doi.org/10.3390/aerospace10010026
APA StyleWang, G., Xu, G., Wang, Y., Yao, Y., Wang, K., Hu, X., Sun, H., & Liu, J. (2023). Deployment Modes and Aerodynamic Analysis of UAV Orthogonal Biaxial Folding Wing. Aerospace, 10(1), 26. https://doi.org/10.3390/aerospace10010026






