Figure 1.
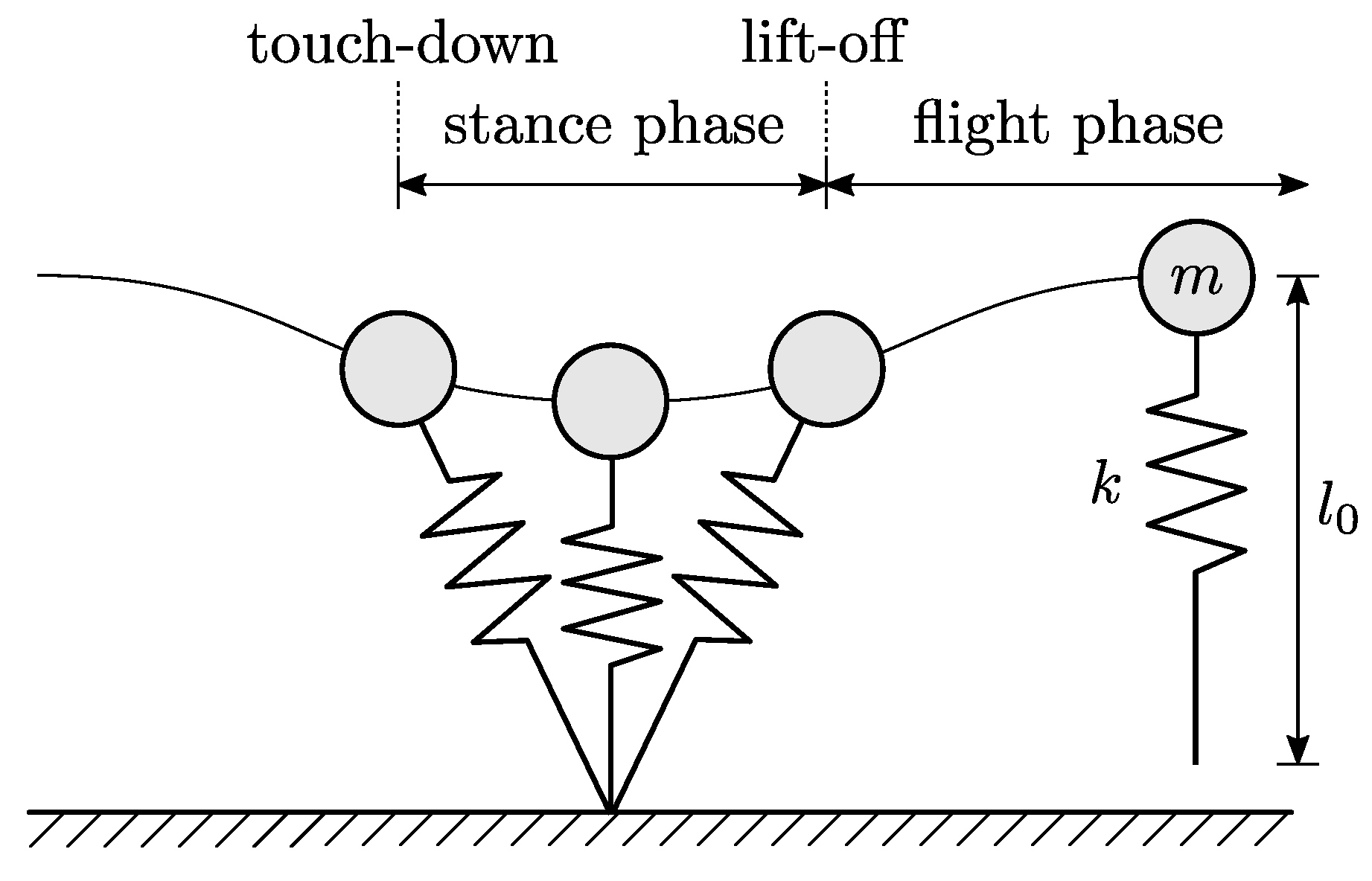
SLIP model for dynamically balanced running with body point mass m, spring stiffness k, and uncompressed leg length .
Figure 1.
SLIP model for dynamically balanced running with body point mass m, spring stiffness k, and uncompressed leg length .
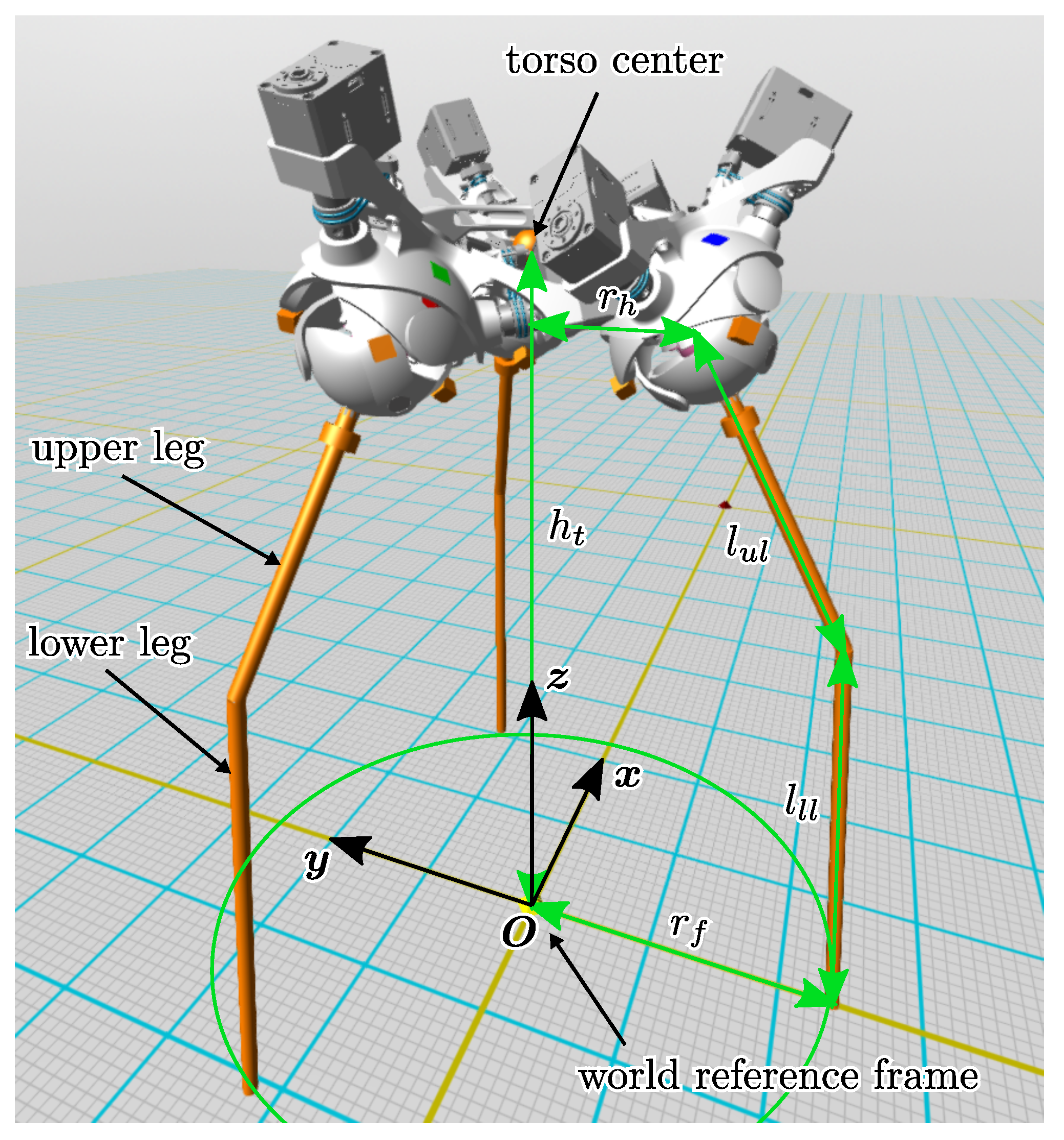
Figure 2.
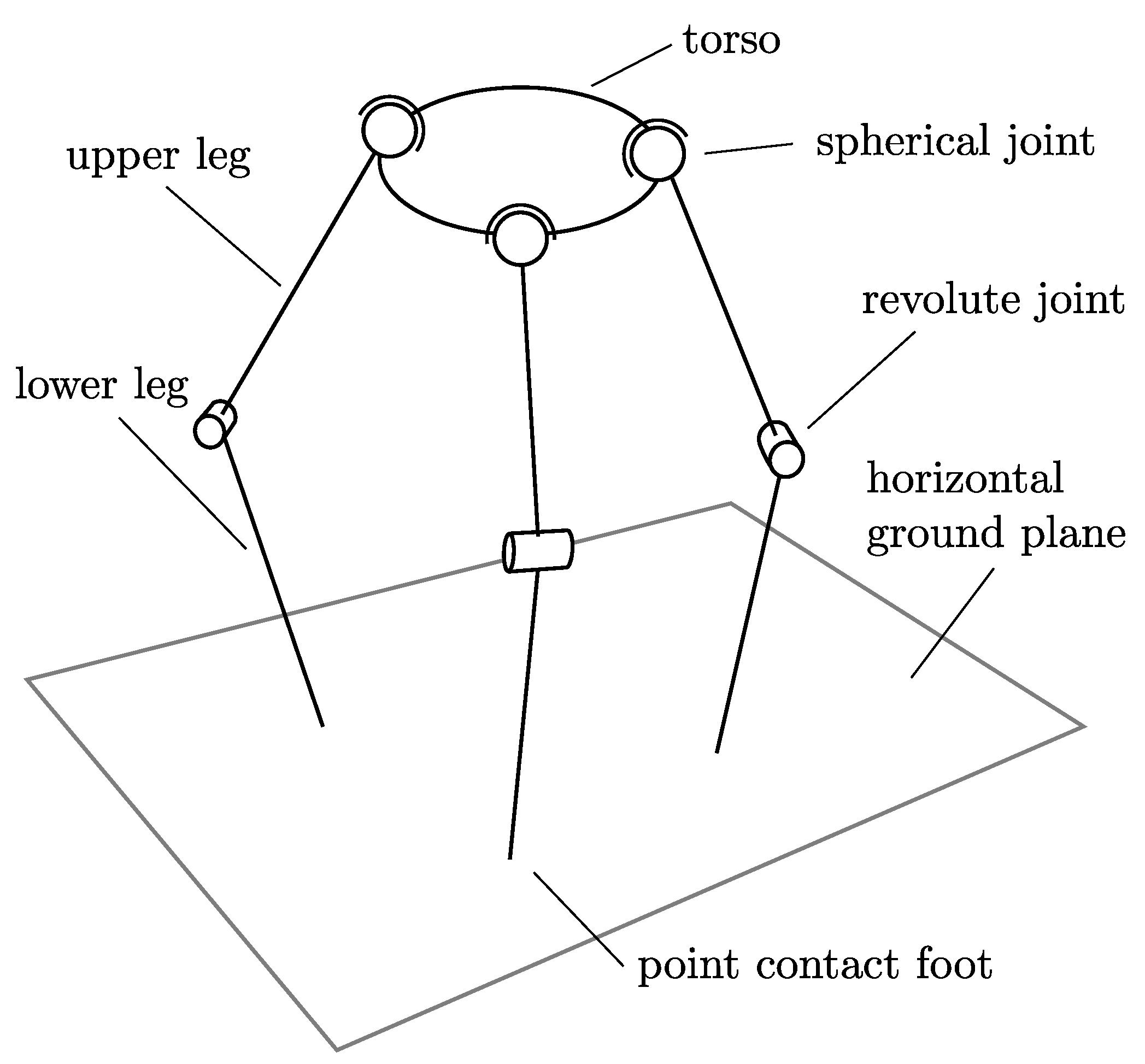
General conceptual robot design overview. The feet are considered as points, colliding with an even ground plane.
Figure 2.
General conceptual robot design overview. The feet are considered as points, colliding with an even ground plane.
Figure 3.
Actuator placement inside the leg. Dashed lines mark influence of the individual actuators on the actual topological joints of the robot model.
Figure 3.
Actuator placement inside the leg. Dashed lines mark influence of the individual actuators on the actual topological joints of the robot model.
Figure 4.
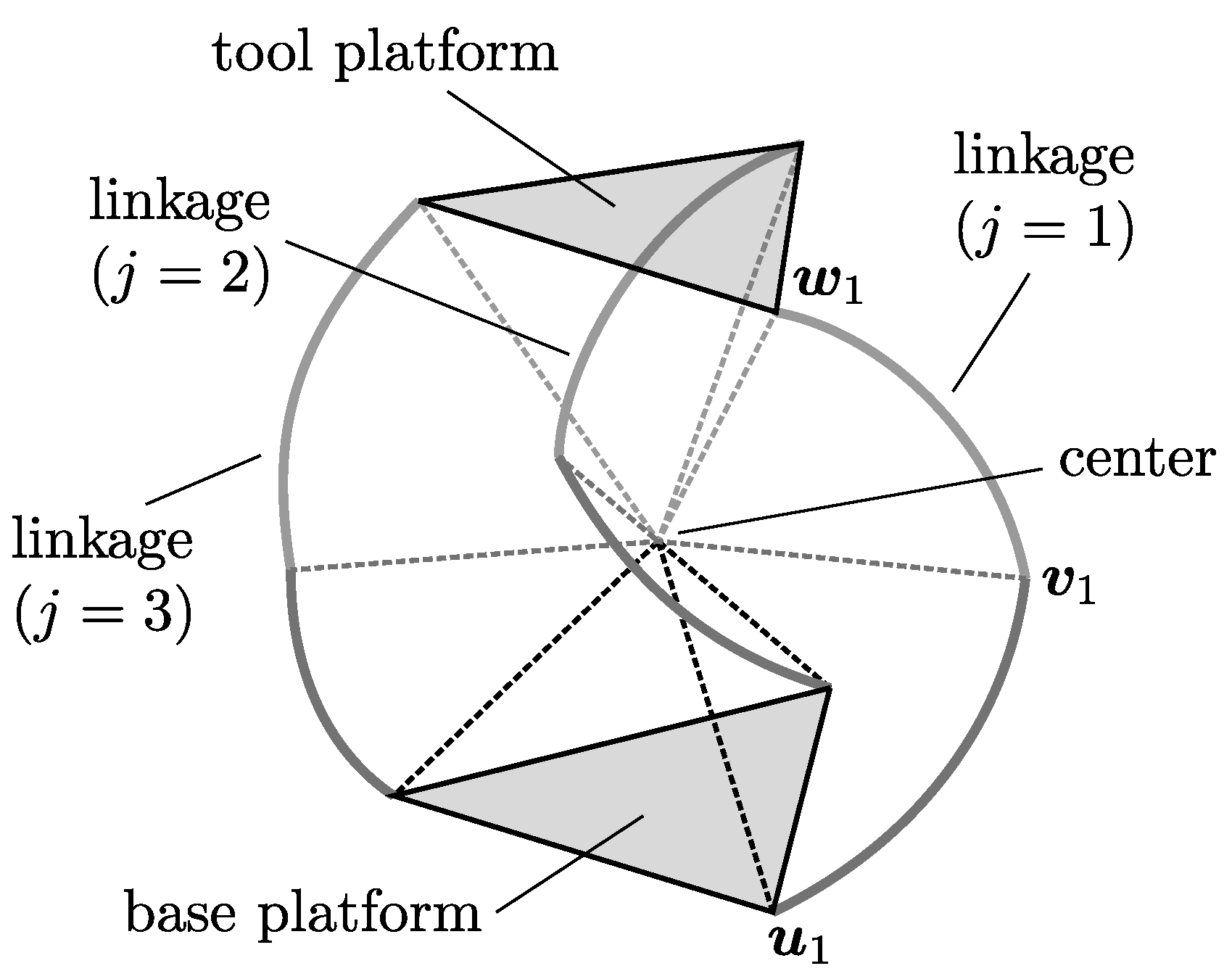
General layout of a spherical parallel manipulator. Dotted lines indicate actuated (black) and passive (gray) rotatory axes , , .
Figure 4.
General layout of a spherical parallel manipulator. Dotted lines indicate actuated (black) and passive (gray) rotatory axes , , .
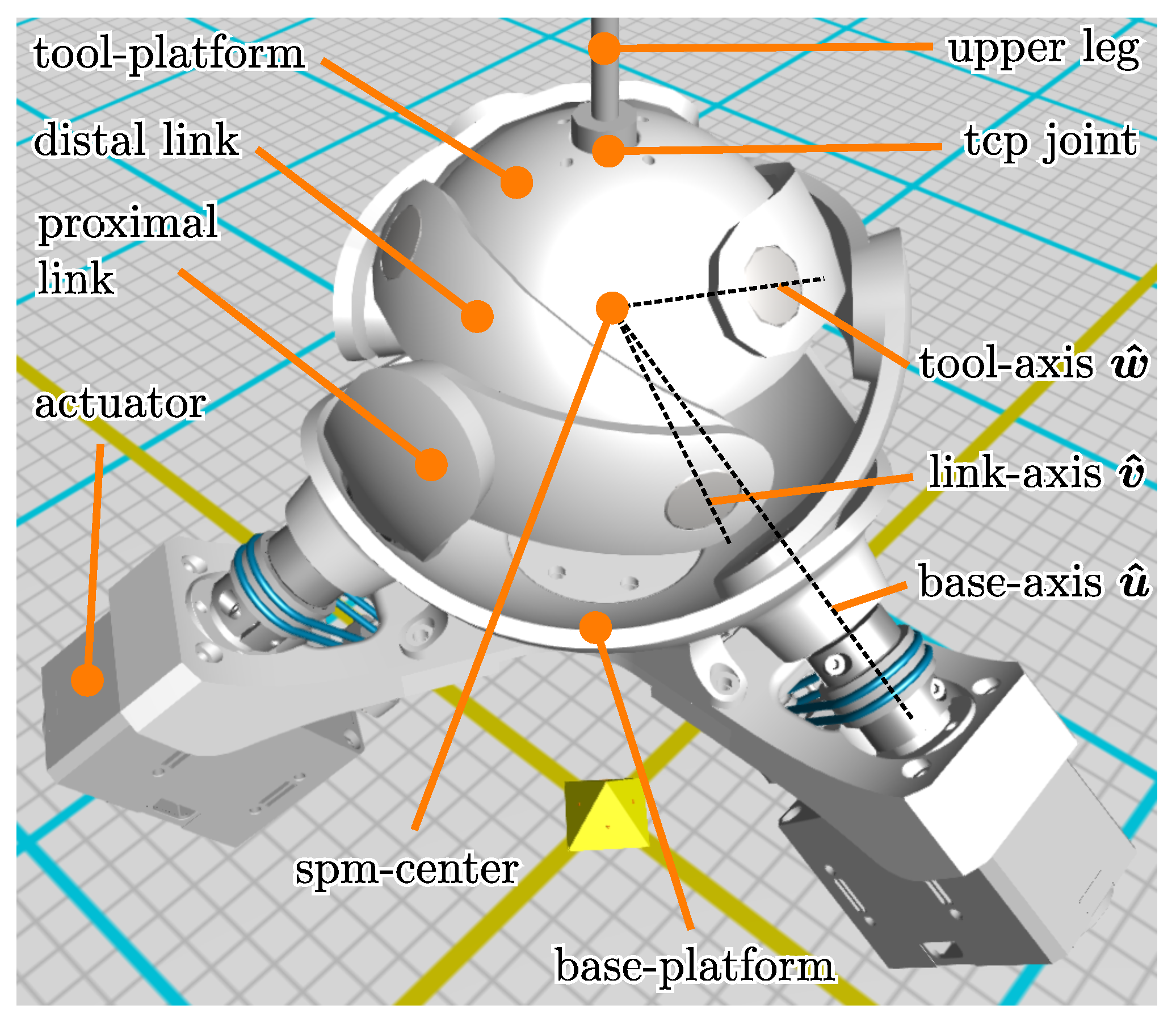
Figure 5.
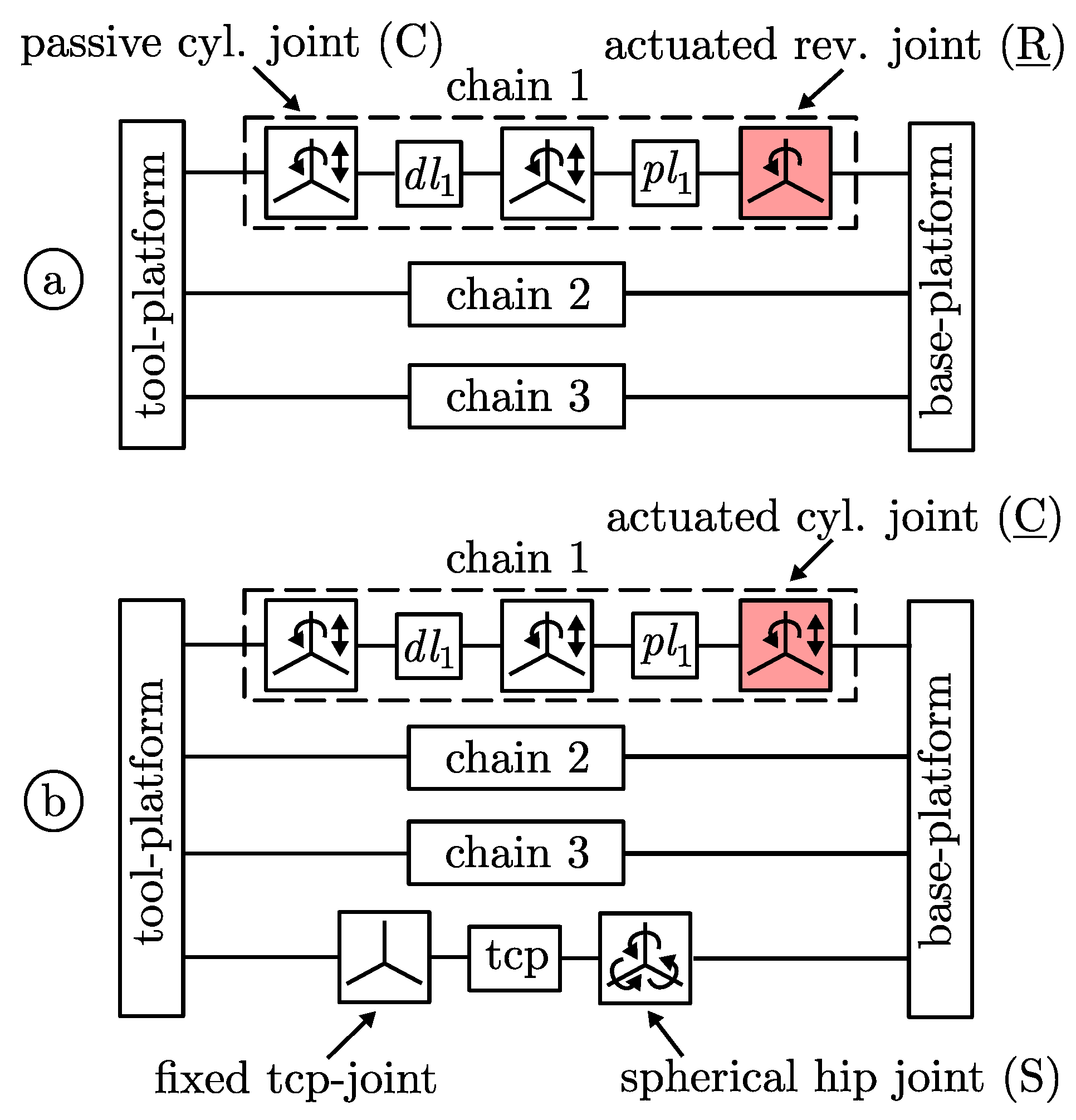
Typical 3RCC (a) and altered (b) topological non-redundant 3CCC+S layout of the SPM. The topology of the single linkage structure with proximal link and distal link can be observed for both layouts. Red marks actuated joints. In the case (b), an additional joint with no DOF connects the tool-platform and an additional tcp body, which was included for load measurement purposes. Note that the tcp body in this model is the actual interface to external forces/torques or tools.
Figure 5.
Typical 3RCC (a) and altered (b) topological non-redundant 3CCC+S layout of the SPM. The topology of the single linkage structure with proximal link and distal link can be observed for both layouts. Red marks actuated joints. In the case (b), an additional joint with no DOF connects the tool-platform and an additional tcp body, which was included for load measurement purposes. Note that the tcp body in this model is the actual interface to external forces/torques or tools.
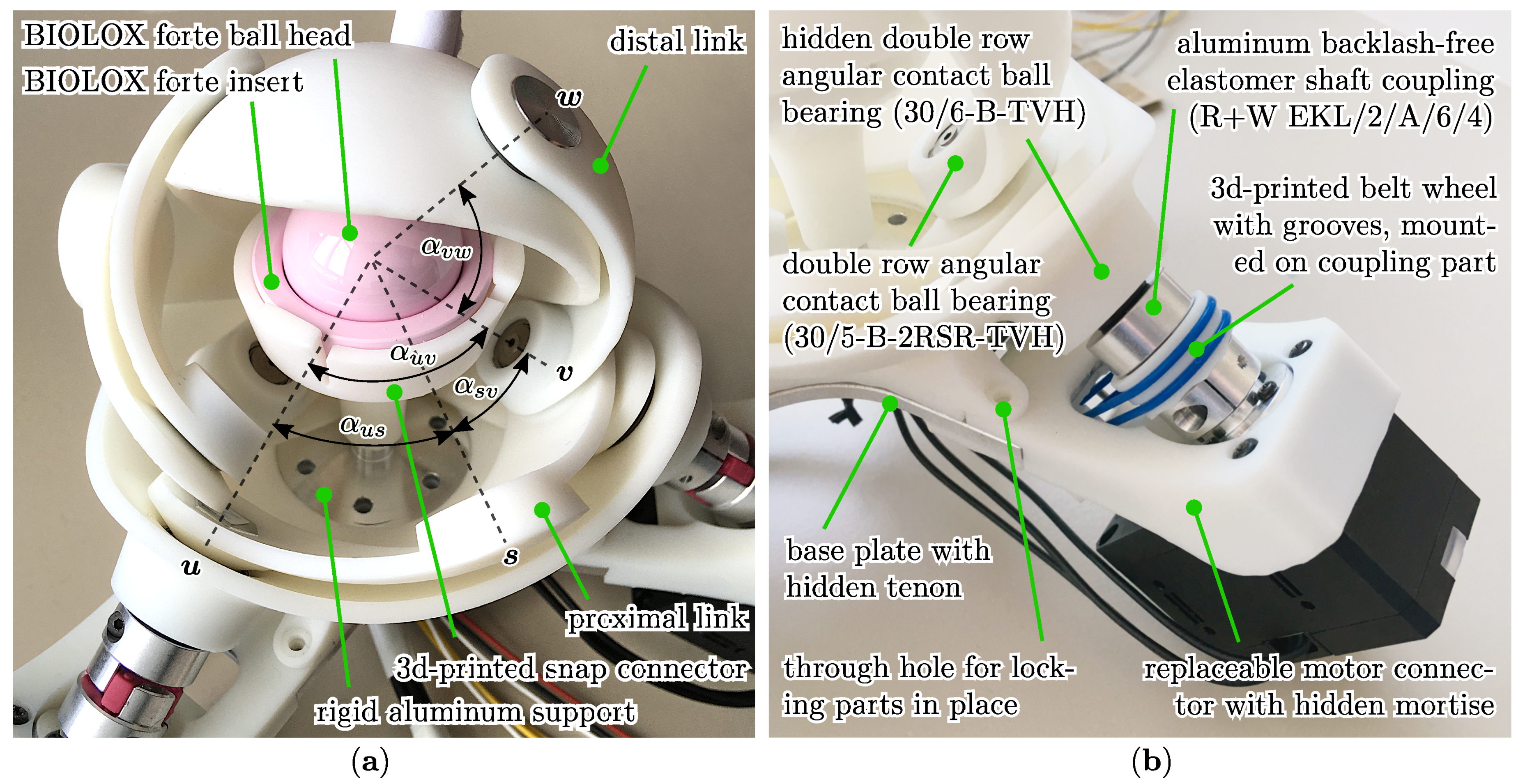
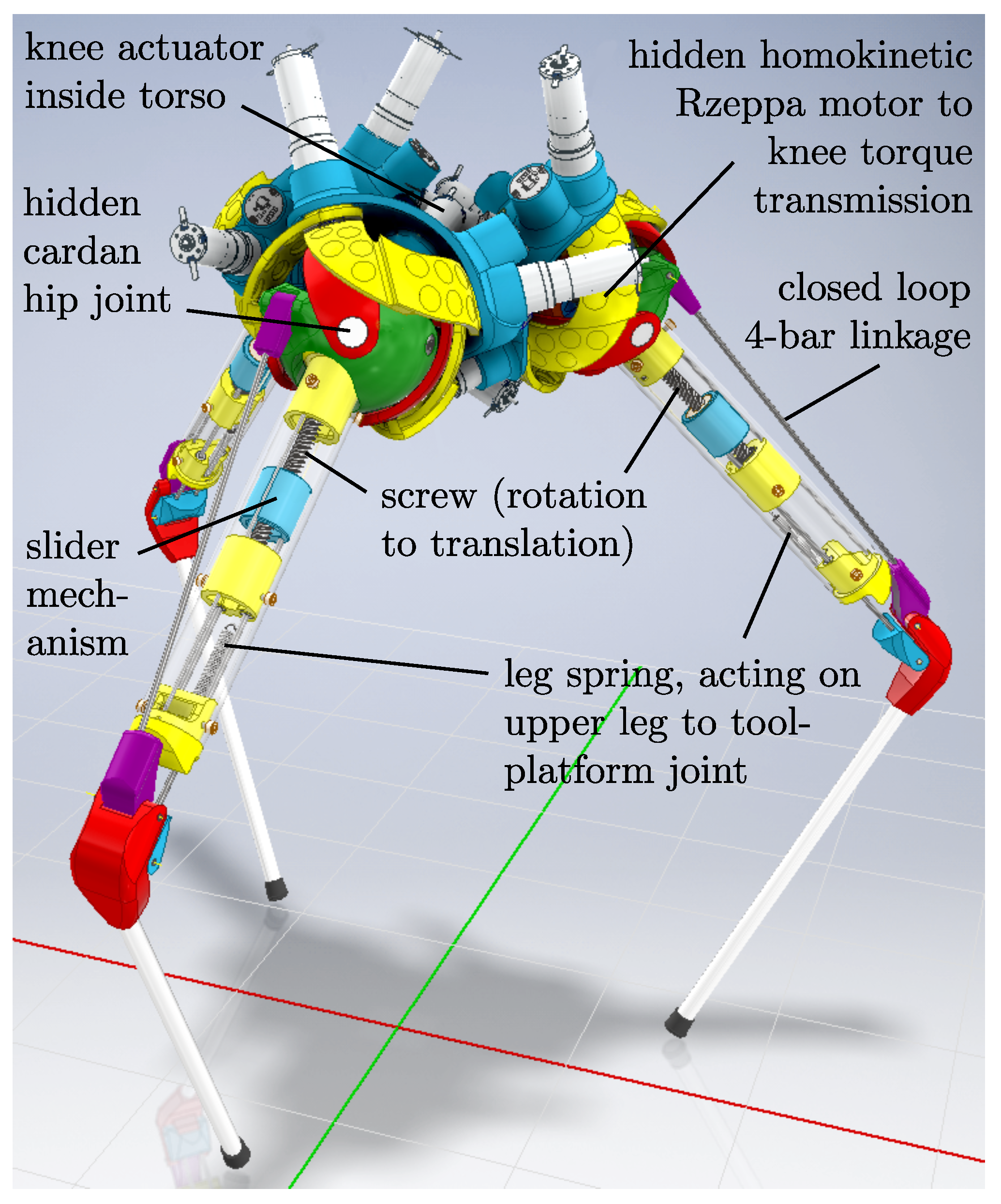
Figure 6.
Real-world prototype (a) of the hip joint mechanism based on stereolithography 3d printing and thin supporting aluminium structures. Encoder integration (b) and visible screw holes for the torso connector structure on the bottom side of the manipulator unit.
Figure 6.
Real-world prototype (a) of the hip joint mechanism based on stereolithography 3d printing and thin supporting aluminium structures. Encoder integration (b) and visible screw holes for the torso connector structure on the bottom side of the manipulator unit.
Figure 7.
Spherical ball and socket artificial hip joint (
a) made of ceramic by CeramTec [
29]. Image (
b) shows mechanical details of the motor connection.
Figure 7.
Spherical ball and socket artificial hip joint (
a) made of ceramic by CeramTec [
29]. Image (
b) shows mechanical details of the motor connection.
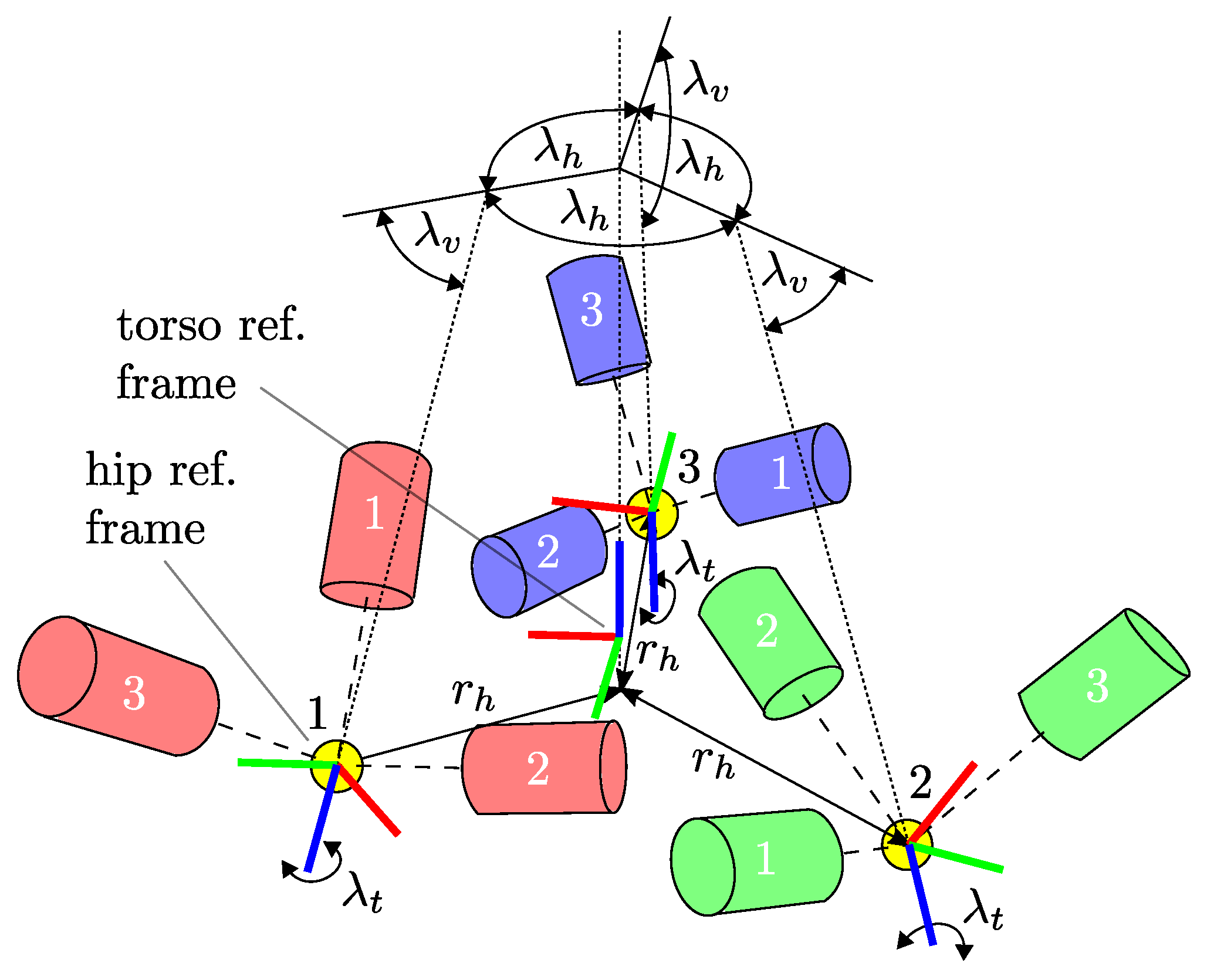
Figure 8.
Interlocking radial symmetrical arrangement of the hip units around the vertical centre axis. Yellow circles mark the centre of the SPM units, and origins of the SPM centre reference frames. Red, green, and blue colours indicate x-, y-, and z-coordinates and individual hip units, respectively. White numbers relate to the individual branches, while black numbers identify the unit itself. The radius to the spherical centre of each hip joint is perpendicular to the vertical centre axis, which is the z-axis of the torso reference frame. SPM (3) is placed inside the y-z-plane of the torso reference frame.
Figure 8.
Interlocking radial symmetrical arrangement of the hip units around the vertical centre axis. Yellow circles mark the centre of the SPM units, and origins of the SPM centre reference frames. Red, green, and blue colours indicate x-, y-, and z-coordinates and individual hip units, respectively. White numbers relate to the individual branches, while black numbers identify the unit itself. The radius to the spherical centre of each hip joint is perpendicular to the vertical centre axis, which is the z-axis of the torso reference frame. SPM (3) is placed inside the y-z-plane of the torso reference frame.
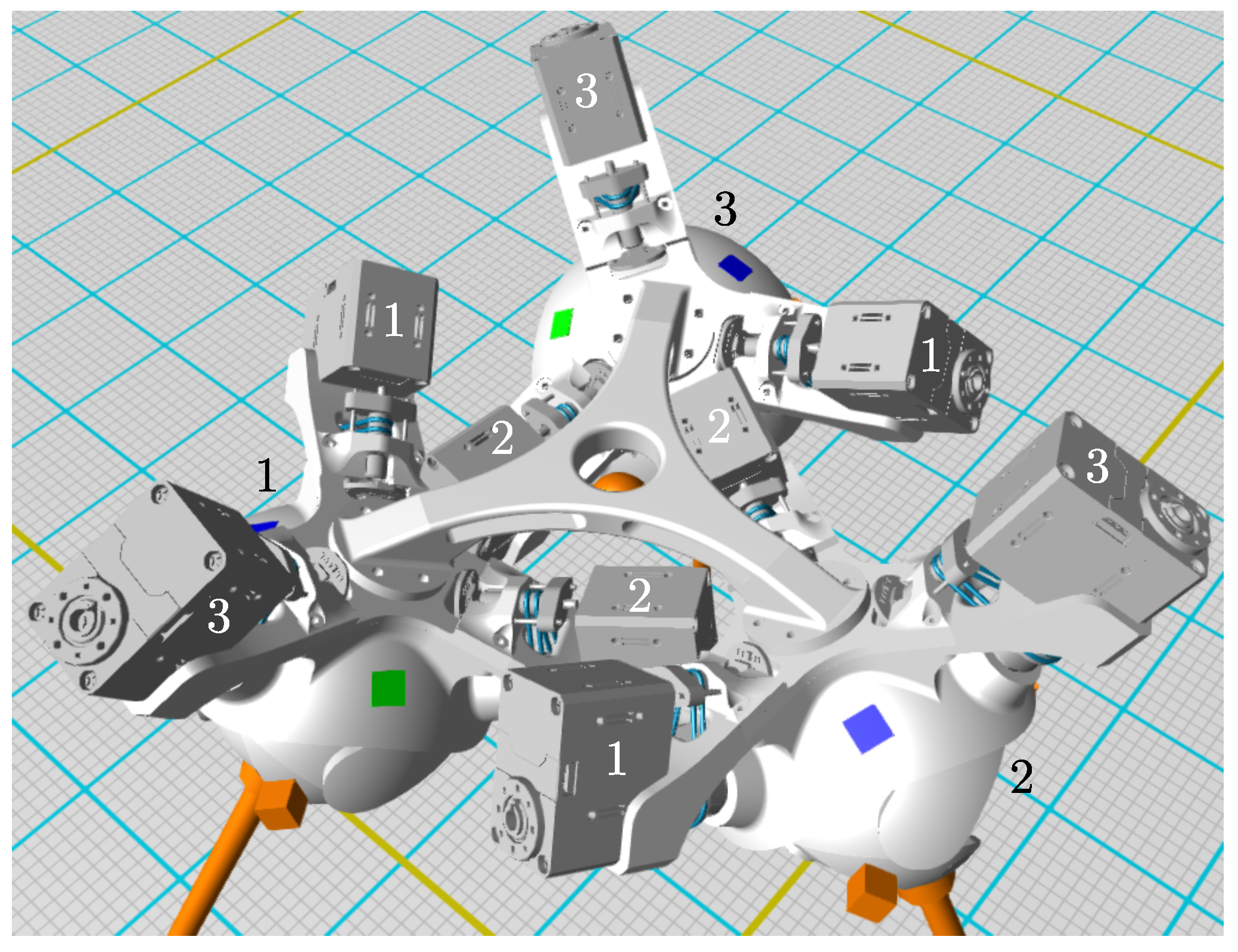
Figure 9.
Robot assembly with actual CAD models, showing the interlocking arrangement of the individual hip units.
Figure 9.
Robot assembly with actual CAD models, showing the interlocking arrangement of the individual hip units.
Figure 10.
CAD model of the robot in the simulation framework. Grid distances and colour mapping: 1 m (yellow), 1 dm (cyan), 1 cm (grey).
Figure 10.
CAD model of the robot in the simulation framework. Grid distances and colour mapping: 1 m (yellow), 1 dm (cyan), 1 cm (grey).
Figure 11.
Simplified component view of the simulation framework.
Figure 11.
Simplified component view of the simulation framework.
Figure 12.
Overview of the plant model. Dotted parts were not active for the current robot model.
Figure 12.
Overview of the plant model. Dotted parts were not active for the current robot model.
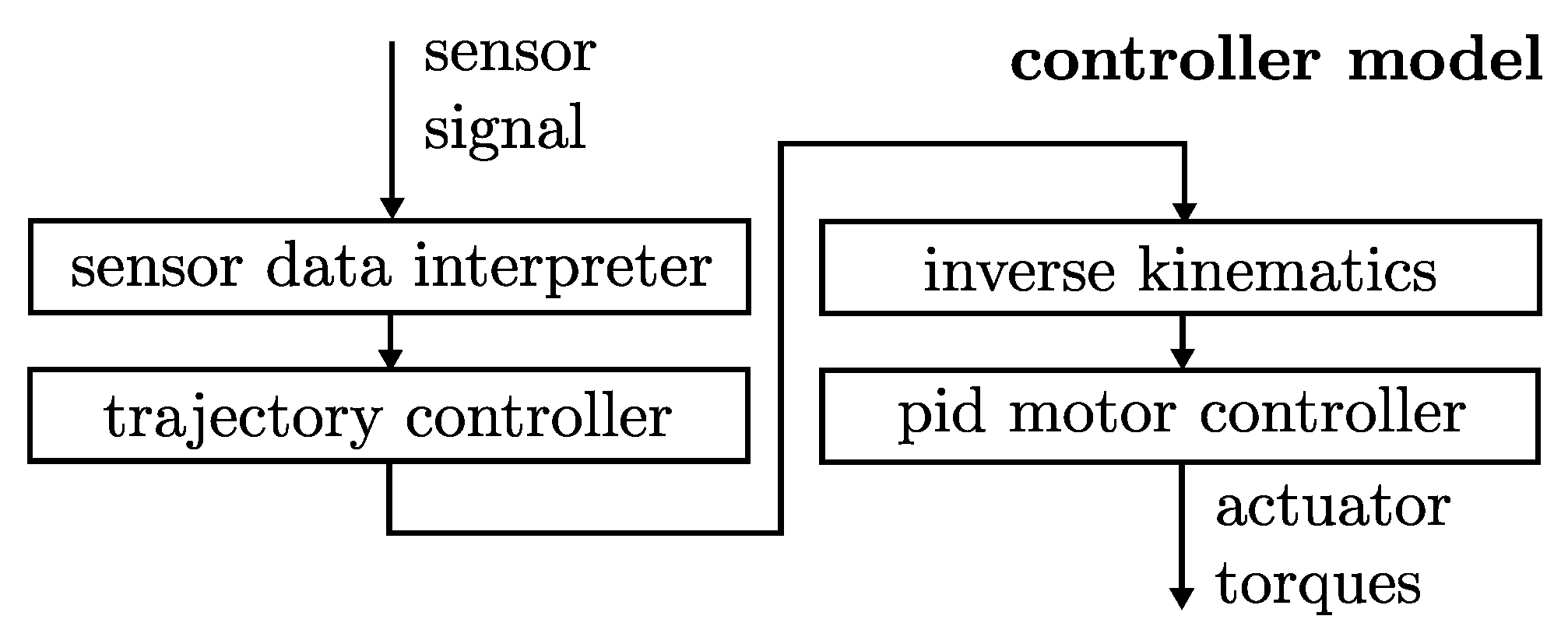
Figure 13.
Overview of the controller model.
Figure 13.
Overview of the controller model.
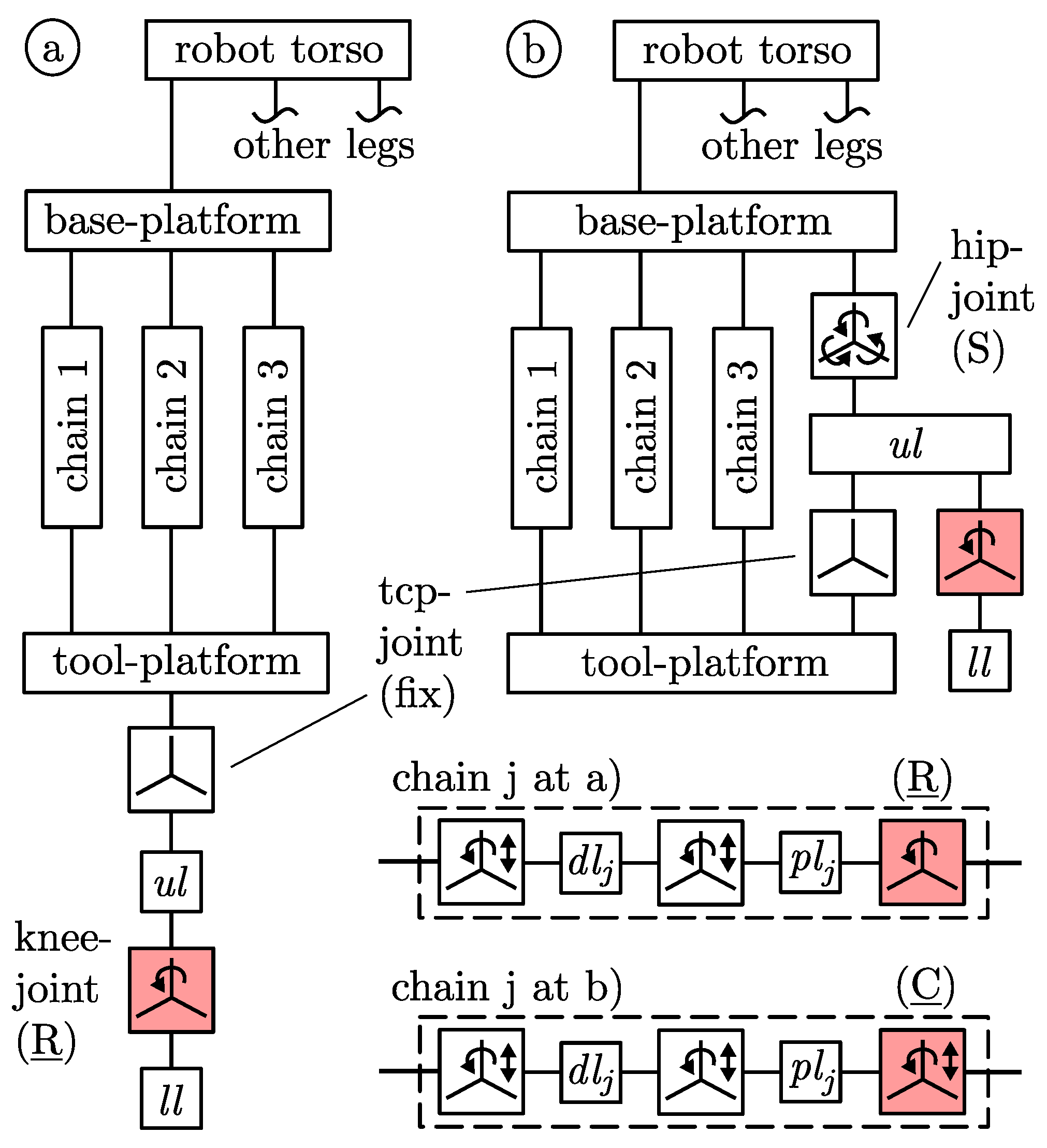
Figure 14.
Full robot topology without (a) and topology with supporting load joint (b). Red-coloured connections are actuated (active) joints. Other joints are passive, non-actuated joints. Abbreviations are pl for proximal link, dl for distal link, ul for upper leg, and ll for lower leg.
Figure 14.
Full robot topology without (a) and topology with supporting load joint (b). Red-coloured connections are actuated (active) joints. Other joints are passive, non-actuated joints. Abbreviations are pl for proximal link, dl for distal link, ul for upper leg, and ll for lower leg.
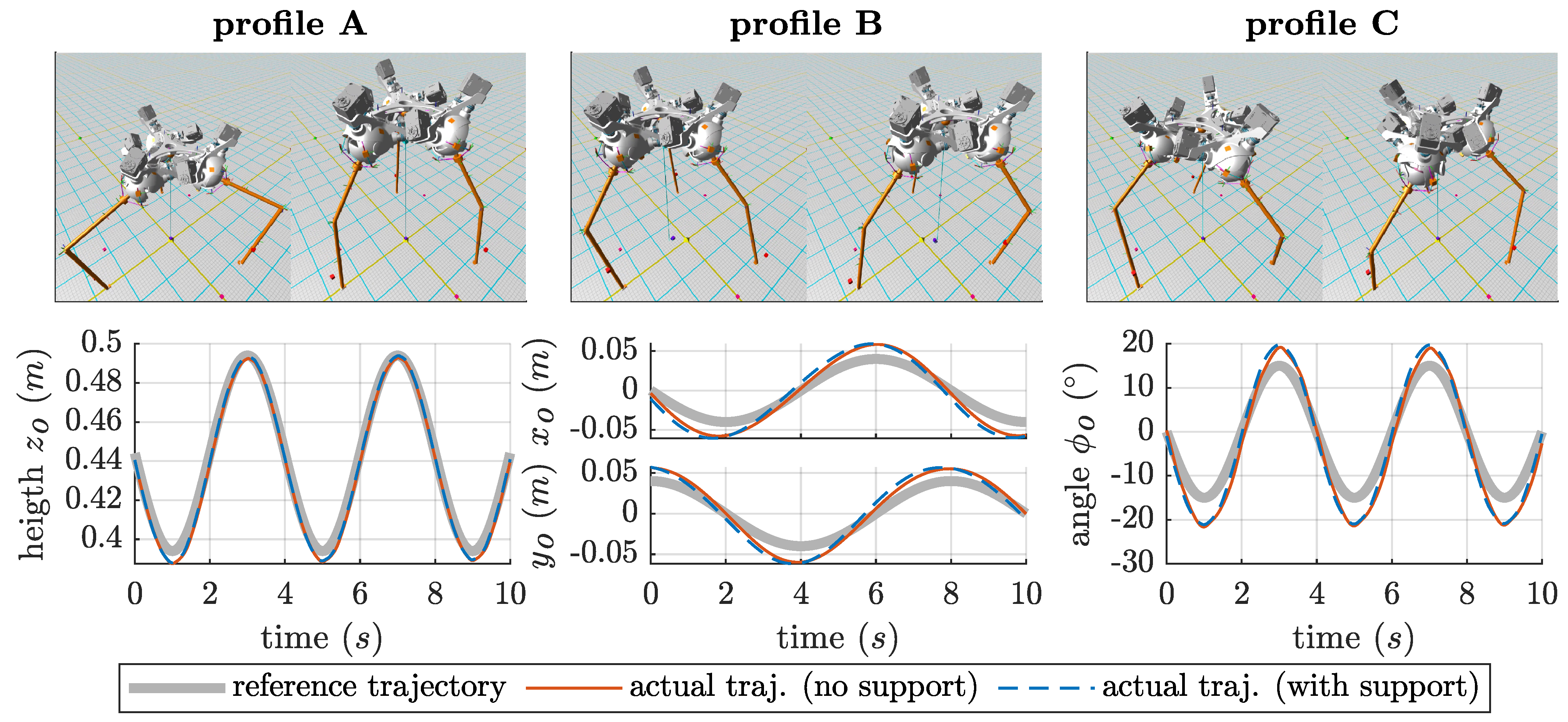
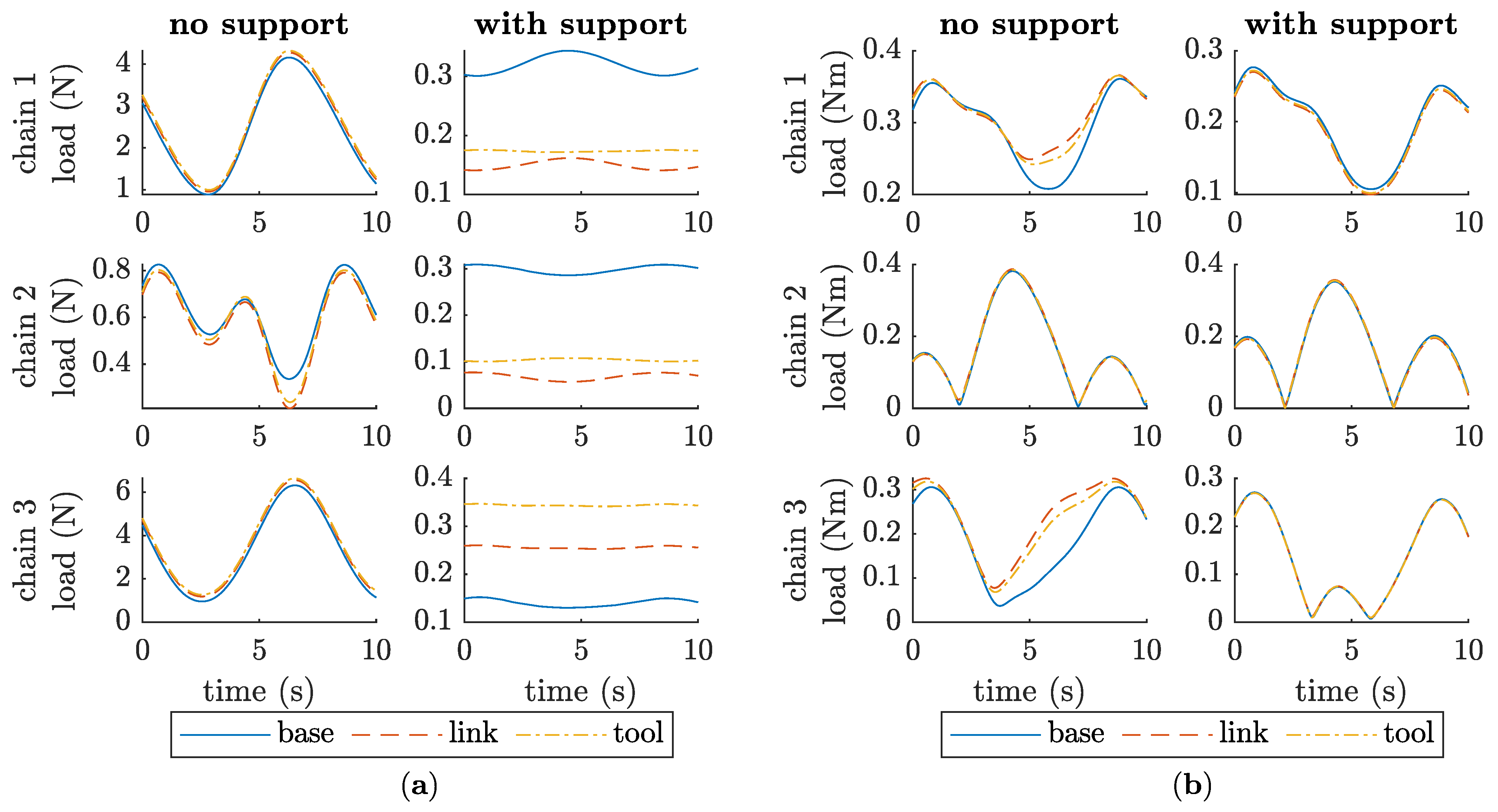
Figure 15.
Motion profiles and screenshots of the robot model in simulation, following the reference trajectory. The term with support relates to the configuration with load support joint and no support to the conf. without bespoke joint. The screenshots display extrema of the corresponding motion plots.
Figure 15.
Motion profiles and screenshots of the robot model in simulation, following the reference trajectory. The term with support relates to the configuration with load support joint and no support to the conf. without bespoke joint. The screenshots display extrema of the corresponding motion plots.
Figure 16.
CAD model of the hip mechanism inside the specially purposed simulation framework for this project.
Figure 16.
CAD model of the hip mechanism inside the specially purposed simulation framework for this project.
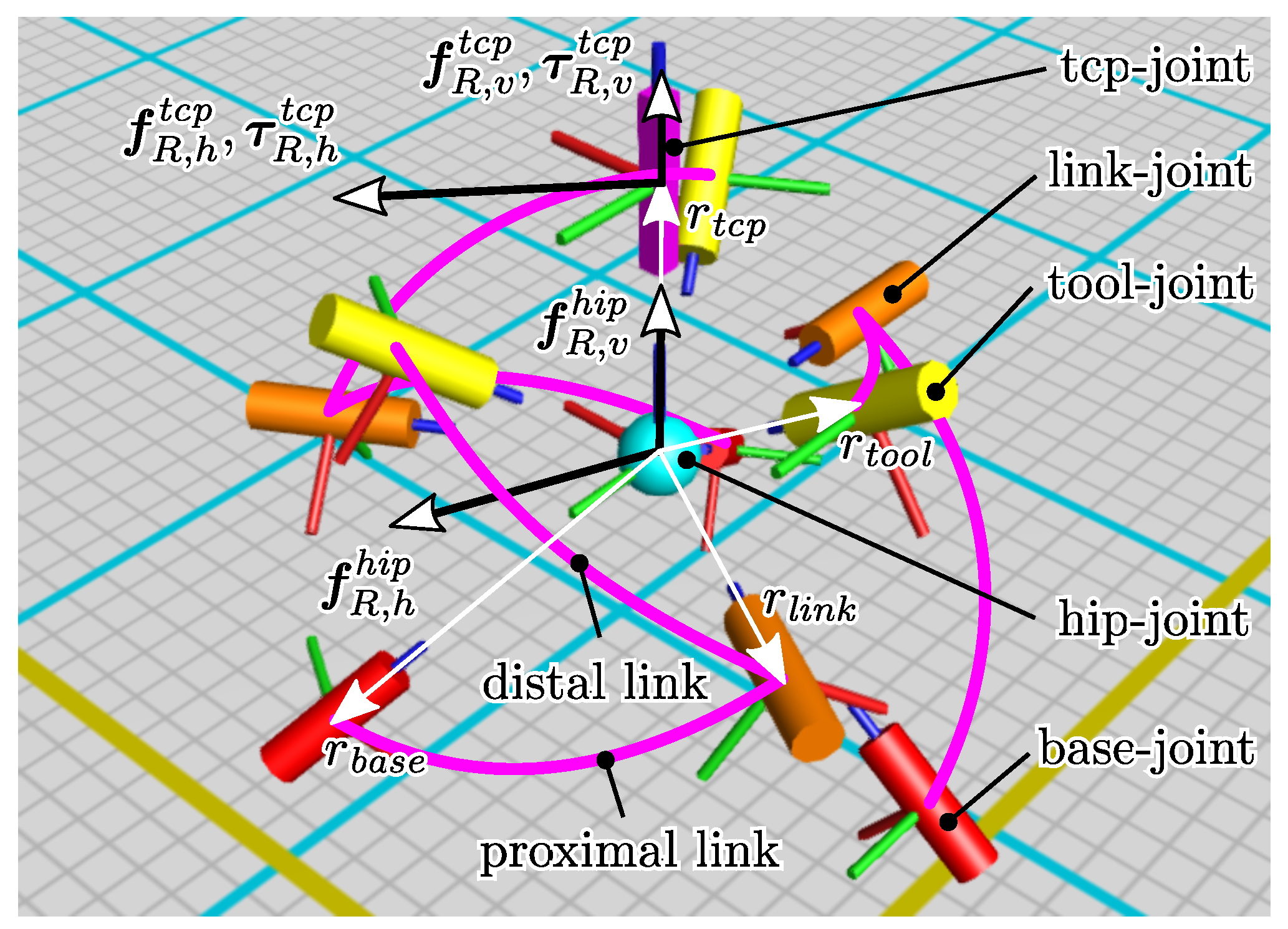
Figure 17.
Reference frame view of the hip unit, showing base (red), link (orange), tool (yellow), hip (cyan), and tcp (magenta) joints. Cylinders visualize the joint axis direction. Magenta lines show connections between joints. Red, green, and blue axes represent the orthogonal x-, y-, and z-axes of the respective reference frames, respectively.
Figure 17.
Reference frame view of the hip unit, showing base (red), link (orange), tool (yellow), hip (cyan), and tcp (magenta) joints. Cylinders visualize the joint axis direction. Magenta lines show connections between joints. Red, green, and blue axes represent the orthogonal x-, y-, and z-axes of the respective reference frames, respectively.
Figure 18.
Trajectory controller design for legged locomotion.
Figure 18.
Trajectory controller design for legged locomotion.
Figure 19.
Load forces and torques in horizontal and vertical directions, measured at the hip and the tcp joint for both configurations and .
Figure 19.
Load forces and torques in horizontal and vertical directions, measured at the hip and the tcp joint for both configurations and .
Figure 20.
Required actuator torques (a) and velocities (b) for both robot configurations and each motion profile A, B and C.
Figure 20.
Required actuator torques (a) and velocities (b) for both robot configurations and each motion profile A, B and C.
Figure 21.
Simulated reaction forces (a) and torques (b) of each joint per linkage chain over time for motion profile B.
Figure 21.
Simulated reaction forces (a) and torques (b) of each joint per linkage chain over time for motion profile B.
Figure 22.
Angular joint distribution for different motion profiles, depicting the actuator joint angles and the resulting hip orientation in Euler angles. For the purpose of compact visualization, data for , , and are shifted by .
Figure 22.
Angular joint distribution for different motion profiles, depicting the actuator joint angles and the resulting hip orientation in Euler angles. For the purpose of compact visualization, data for , , and are shifted by .
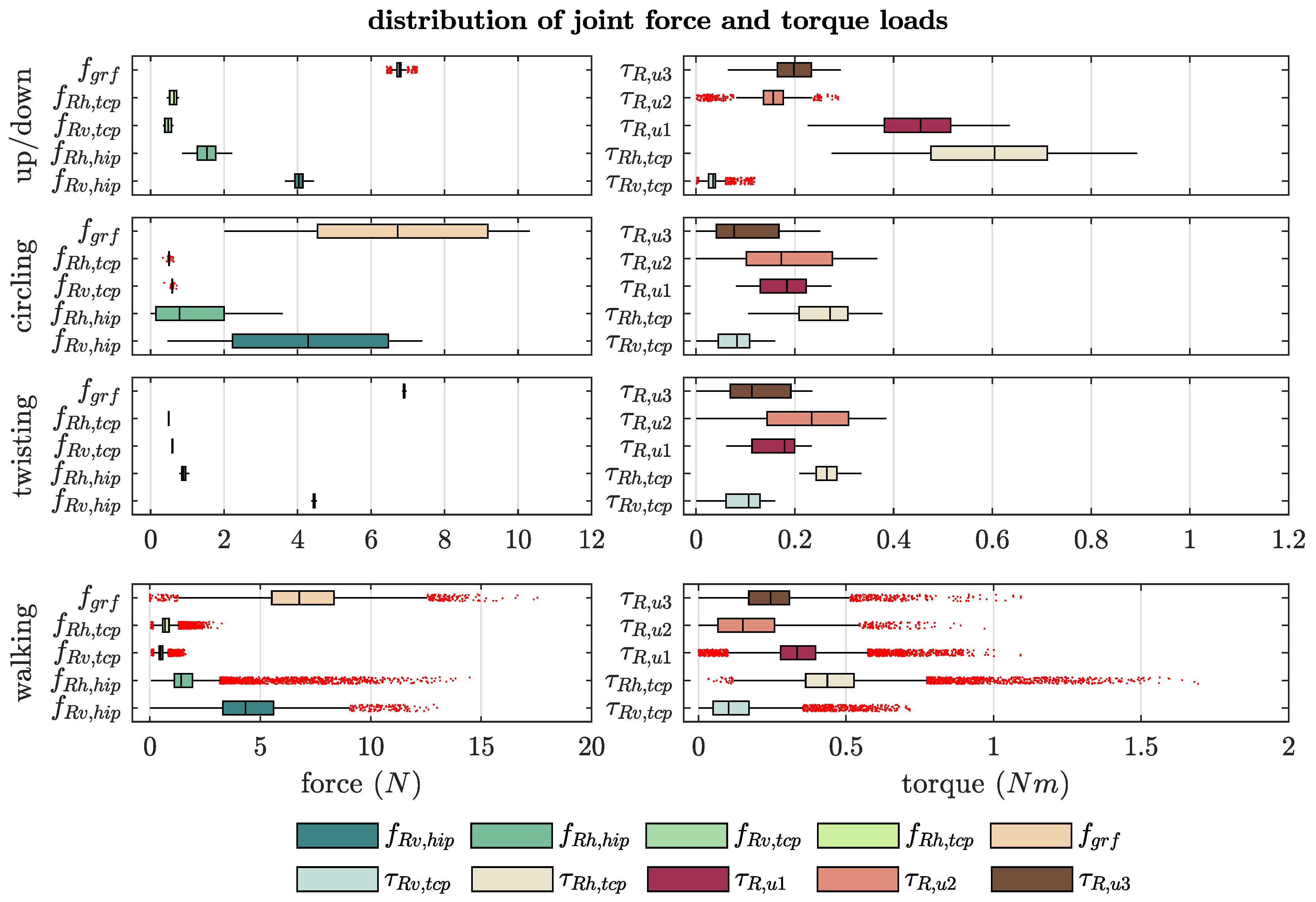
Figure 23.
Reaction force and torque of the hip and the tcp joint in the horizontal and vertical component view. shows ground reaction forces, and displays the joint reaction torques after regarding actuator torques and joint friction. All values are absolute resp. norms of vector values. A different axis range is used for the walking profile.
Figure 23.
Reaction force and torque of the hip and the tcp joint in the horizontal and vertical component view. shows ground reaction forces, and displays the joint reaction torques after regarding actuator torques and joint friction. All values are absolute resp. norms of vector values. A different axis range is used for the walking profile.
Figure 24.
Layout of a single linkage structure.
Figure 24.
Layout of a single linkage structure.
Figure 25.
Segment of the walking gait. Black bars indicate swing phases of a particular leg.
Figure 25.
Segment of the walking gait. Black bars indicate swing phases of a particular leg.
Figure 26.
Internal forces along the proximal and distal link for the EEF-torque . Link arc angles are set to for visualization purposes.
Figure 26.
Internal forces along the proximal and distal link for the EEF-torque . Link arc angles are set to for visualization purposes.
Figure 27.
Refined current robot model, including leg elasticities.
Figure 27.
Refined current robot model, including leg elasticities.
Table 1.
Configuration parameters for different topologies. Abbreviations for joint DOFs are base (b), link (l), tool (t), hip (h), knee (k), foot–ground contact (f), and passive (p).
Table 1.
Configuration parameters for different topologies. Abbreviations for joint DOFs are base (b), link (l), tool (t), hip (h), knee (k), foot–ground contact (f), and passive (p).
| Config. | l | n | | | | | | | | F |
| 3SRS | 8 | 9 | - | - | - | 3 | 1 | 3 | 3 | 6 |
| 3RRR | 8 | 9 | 1 | 1 | 1 | - | - | - | 0 | −3 |
| 3RCC | 8 | 9 | 1 | 2 | 2 | - | - | - | 0 | 3 |
| 3CCC + S | 8 | 10 | 2 | 2 | 2 | 3 | - | - | 0 | 3 |
| 3CCC | 8 | 9 | 2 | 2 | 2 | - | - | - | 0 | 6 |
Table 2.
Angles between intersecting axes of the SPM.
Table 2.
Angles between intersecting axes of the SPM.
| Parameter | | | | | | |
| Angle (deg) | 90 | 90 | | | 90 | 90 |
Table 3.
Z-X-Z-Euler angles in degree of the individual hip centre frames in relation to the torso body reference frame.
Table 3.
Z-X-Z-Euler angles in degree of the individual hip centre frames in relation to the torso body reference frame.
| Hip j | | | |
|---|
| 1 | 120 | 150 | 5 |
| 2 | −120 | 150 | 5 |
| 3 | 0 | 150 | 5 |
Table 4.
Properties of the physical robot model. N refers to the number of repeated assemblies in the full robot with part mass m.
Table 4.
Properties of the physical robot model. N refers to the number of repeated assemblies in the full robot with part mass m.
| Assembly | N | Mass (g) | (%) |
| Torso-connector-structure | 1 | 47.504 | 2.32 |
| SPM base-platform | 3 | 354.199 | 51.84 |
| SPM prox. link | 9 | 27.810 | 12.21 |
| SPM dist. link | 9 | 10.009 | 4.39 |
| SPM tool-platform | 3 | 28.701 | 4.20 |
| Upper leg | 3 | 155.034 | 22.69 |
| Lower leg | 3 | 15.984 | 2.34 |
| Robot | 1 | 2049.629 | 100.00 |
Table 5.
Geometrical properties of the robot model.
Table 5.
Geometrical properties of the robot model.
| Parameter | Value | Unit |
|---|
| Hip radius | | m |
| Foot radius | | m |
| Torso height | | m |
| Upper leg length | | m |
| Lower leg length | | m |
Table 6.
Simulation parameters of the plant model.
Table 6.
Simulation parameters of the plant model.
| Parameter | Value | Unit |
|---|
| Floor stiffn. | 5000 | N/m |
| Floor damp. | 20 | Ns/m |
| Static fric. | 0.8 | - |
| Dyn. fric. | 0.7 | - |
| Velocity threshold | | m/s |
| Plant step time | | s |
| Contr. step time | | s |
| Simulink solver | ode2 (Heun) | - |
| Joint fric. coeff. | 0.01 | - |
| Joint fric. damp. | | Nm·s/rad |
Table 7.
Motion profile parameters, expressing the simulated torso trajectory.
Table 7.
Motion profile parameters, expressing the simulated torso trajectory.
| Var. | Unit | Profile A | Profile B | Profile C |
|---|
| m | | | |
| m | | | |
| | | | |
| m | 0 | | 0 |
| m | 0 | | 0 |
| m | 0 | | 0 |
| m | | | |
| deg | 0 | 0 | |
Table 8.
Geometry of SPM joint reference frame placement for load measurements.
Table 8.
Geometry of SPM joint reference frame placement for load measurements.
| Variable | | | | |
|---|
| Radius (m) | | | | |
Table 9.
Profile-dependent controller parameters.
Table 9.
Profile-dependent controller parameters.
| Profile | | | | | | |
|---|
| A/B/C | 8.50 | 0.70 | 0.03 | 8.50 | 1.00 | 0.03 |
| Walking | 11.00 | 0.00 | 0.07 | 14.00 | 0.00 | 0.06 |
Table 10.
Load comparison for and with fixed actuator axes, depicting static tripodal standing.
Table 10.
Load comparison for and with fixed actuator axes, depicting static tripodal standing.
| Var. | | | Unit |
|---|
| 5.025 | 0.769 | N |
| 0.801 | 0.876 | Nm |
| −0.785 | Nm |
| 0.371 | Nm |
| 0.402 | Nm |
| 0.259 | Nm |
| 0.513 | m |
| 125.7 | |
| 125.7 | |
| 125.7 | |
| −22.5 | |
Table 11.
Load reduction ratios over motion profiles, covering a sequence of 30 s.
Table 11.
Load reduction ratios over motion profiles, covering a sequence of 30 s.
| Load | A | B | C |
|---|
| 0.84 | 0.84 | 0.86 |
| 0.05 | 0.17 | −0.43 |
Table 12.
Construction parameters for and axes for the SPM home configuration and conditioning index .
Table 12.
Construction parameters for and axes for the SPM home configuration and conditioning index .
| Parameter | Used | Ideal |
|---|
| |
| |
| , , |
| | |
| 0.978 | 1.000 |
Table 13.
Amount of stance status per leg over the motion sequence.
Table 13.
Amount of stance status per leg over the motion sequence.
| Leg | 1 | 2 | 3 |
|---|
| 96.7 | 96.5 | 96.7 |
Table 14.
Average execution time on
Intel i5-8365U CPU, based on
samples, measured for calculating the solution to Equation (
39).
Table 14.
Average execution time on
Intel i5-8365U CPU, based on
samples, measured for calculating the solution to Equation (
39).
| Method | Time (s) |
|---|
| (A) Equation (28) w. Matlab inv() operator | 16.772 |
| (B) Equation (28) w. Matlab∖operator | 12.189 |
| (C) Equation (28) in max. compact notation | 10.118 |
| (D) Equation (40) with reused vector | 9.937 |