Total Cross Sections for Electron and Positron Scattering on Molecules: In Search of the Dispersion Relation
Abstract
:1. The Need for Cross Sections
2. Semi-Empirical Models
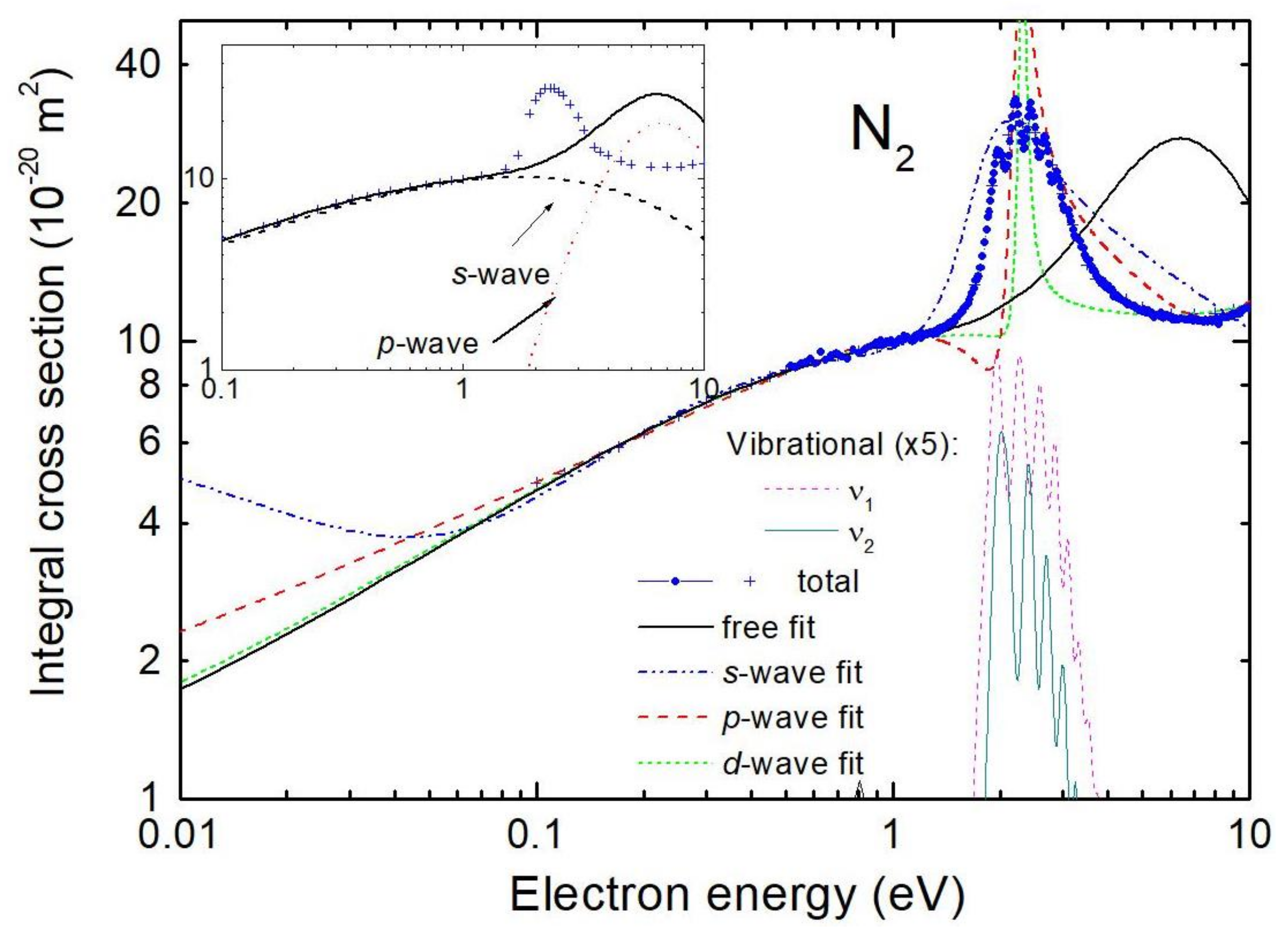
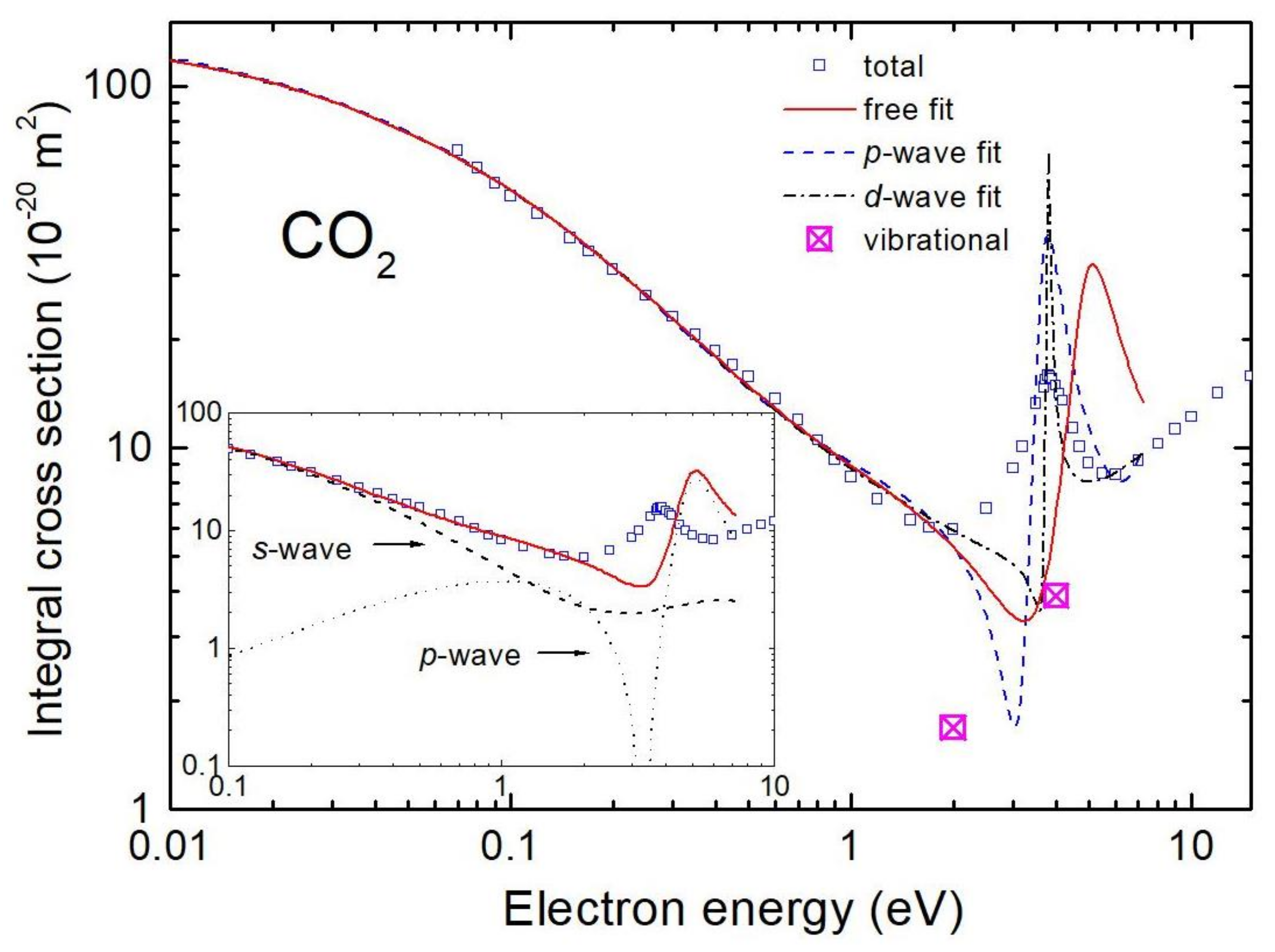
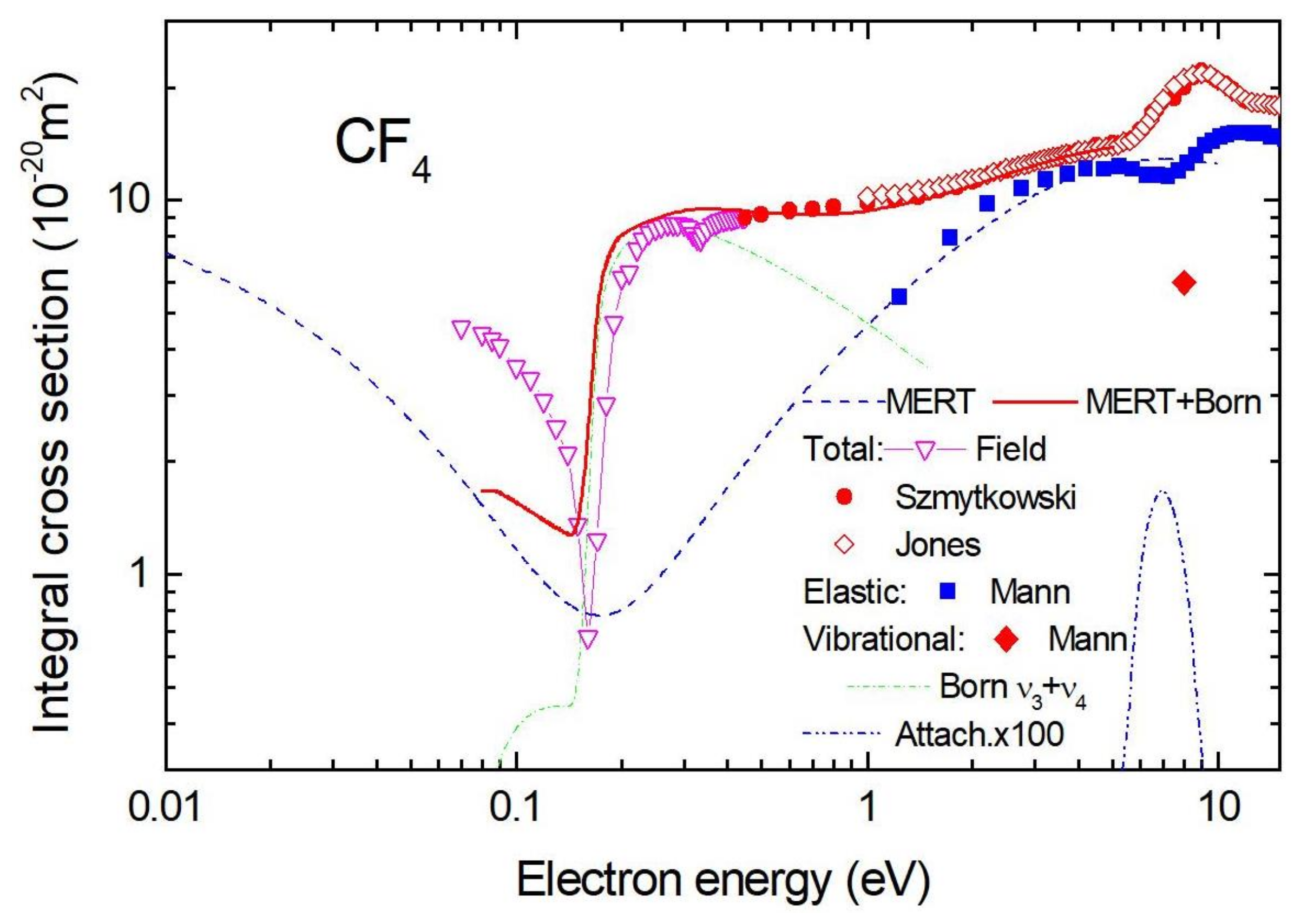
3. Is Total Cross Section Merely a Sum of Partials?
4. Dispersion Relation
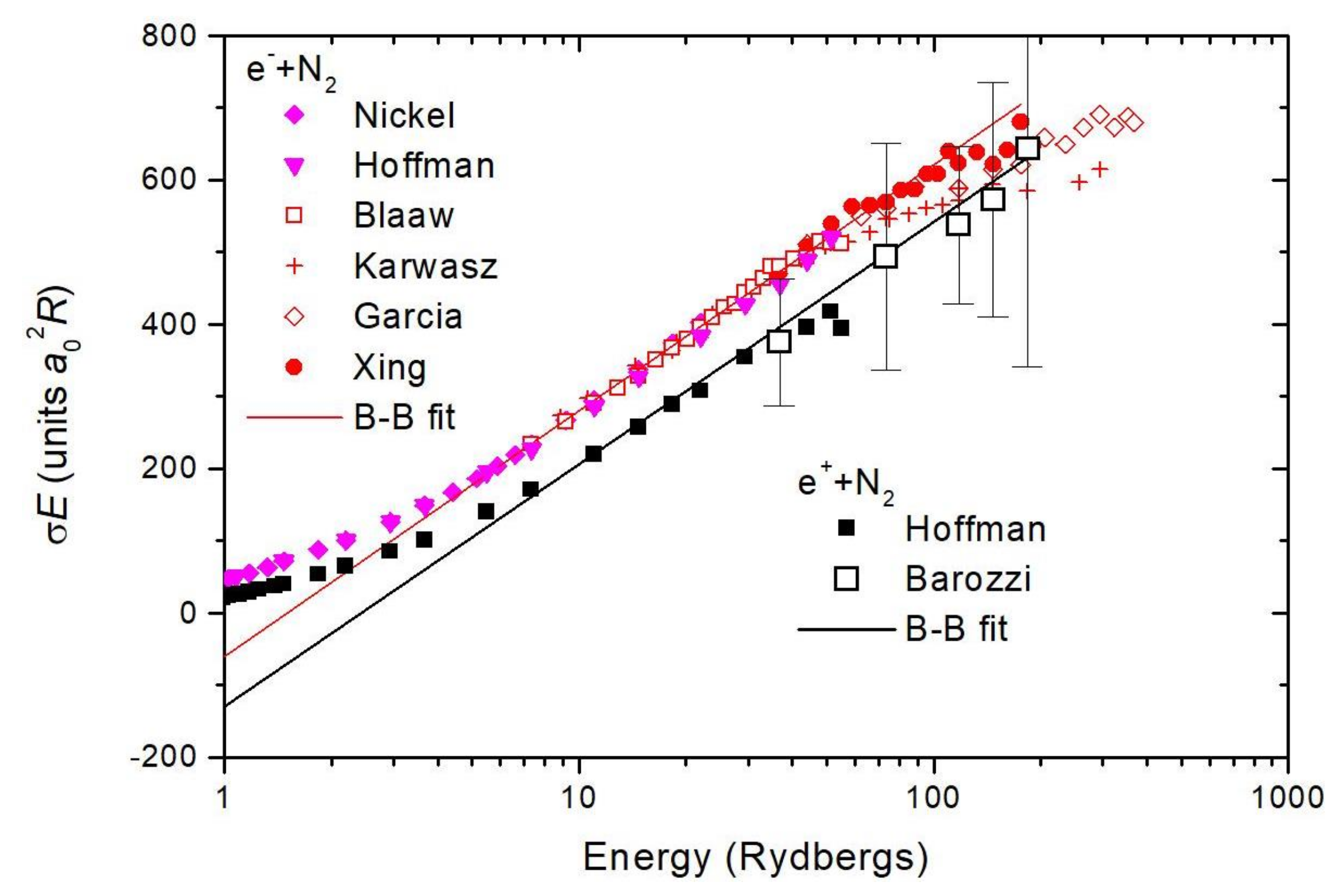

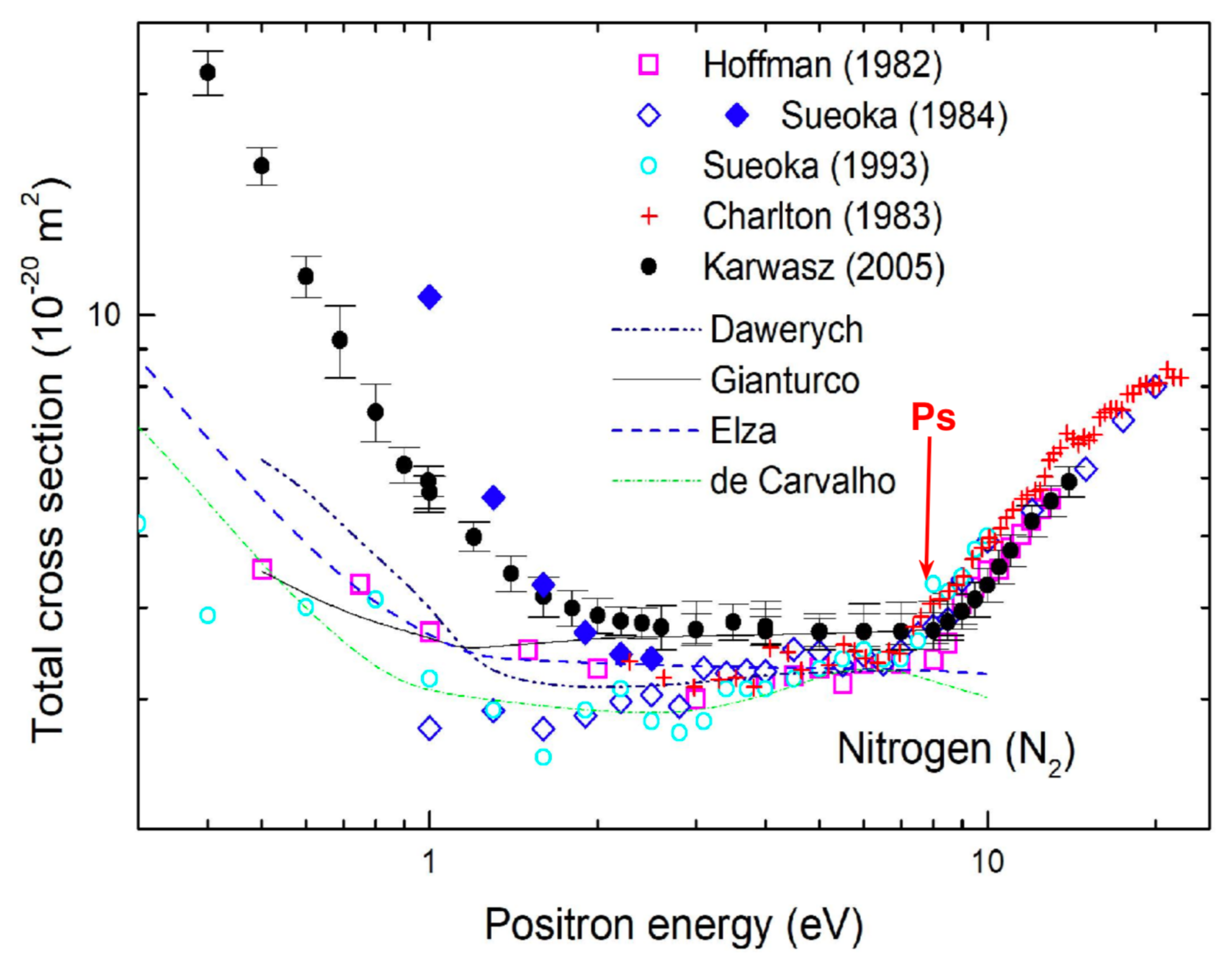
5. Experimental Input
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dodt, D.; Dinklage, A.; Bartschat, K.; Zatsarinny, O. Validation of atomic data using a plasma discharge. New J. Phys. 2010, 12, 073018. [Google Scholar] [CrossRef]
- Nakano, T.; Higashijima, S.; Kubo, H.; Asakura, N.; Fukumoto, M. The emission rates of CH, CD and C2 spectral bands and a re-evaluation of the chemical sputtering yield of the JT-60U carbon divertor plates. Nucl. Fusion 2014, 54, 043004. [Google Scholar] [CrossRef]
- Sahoo, A.K.; Sharma, L. Electron Impact Excitation of Extreme Ultra-Violet Transitions in Xe7 – Xe10 Ions. Atoms 2021, 9, 76. [Google Scholar] [CrossRef]
- Munoz, A.; Blanco, F.; García, G.; Thorne, P.A.; Brunger, M.J.; Sullivan, J.P.; Buckman, S.J. Single electron tracks in water vapour for energies below 100 eV. Int. J. Mass Spectrom. 2008, 277, 175. [Google Scholar] [CrossRef]
- Sinha, N.; Antony, B. Mean Free Paths and Cross Sections for Electron Scattering from Liquid Water. J. Phys. Chem. B 2021, 125, 5479. [Google Scholar] [CrossRef] [PubMed]
- Modak, P.; Antony, B. Electron scattering from HNCO. Eur. Phys. J. D 2021, 75, 54. [Google Scholar] [CrossRef]
- Song, M.Y.; Yoon, J.S.; Cho, H.; Itikawa, Y.; Karwasz, G.P.; Kokoouline, V.; Nakamura, Y.; Tennyson, J. Cross Sections for Electron Collisions with Methane. J. Phys. Chem. Ref. Data 2015, 44, 023101. [Google Scholar] [CrossRef] [Green Version]
- Pietanza, L.D.; Guaitella, O.; Aquilanti, V.; Armenise, I.; Bogaerts, A.; Capitelli, M.; Colonna, G.; Guerra, V.; Engeln, R.; Kustova, E.; et al. Advances in non-equilibrium CO2 plasma kinetics: A theoretical and experimental review. Eur. Phys. J. D 2021, 75, 237. [Google Scholar] [CrossRef]
- Yoon, J.S.; Song, M.Y.; Han, J.M.; Hwang, S.H.; Chang, W.S.; Lee, B.; Itikawa, Y. Cross Sections for Electron Collisions with Hydrogen Molecules. J. Phys. Chem. Ref. Data 2008, 37, 913. [Google Scholar] [CrossRef]
- Szmytkowski, C.; Możejko, P. Recent total cross section measurements in electron scattering from molecules. Eur. Phys. J. D 2020, 74, 90. [Google Scholar] [CrossRef]
- Kawaguchi, S.; Takahashi, K.; Satoh, K. Electron collision cross section set for N2 and electron transport in N2, N2/He, and N2/Ar. Plasma Sources Sci. Technol. 2021, 30, 035010. [Google Scholar] [CrossRef]
- Song, M.Y.; Yoon, J.S.; Cho, H.; Karwasz, G.P.; Kokoouline, V.; Nakamura, Y.; Tennyson, J. “Recommended” cross sections for electron collisions with molecules. Eur. Phys. J. D 2020, 74, 60. [Google Scholar] [CrossRef]
- Lindsay, B.G.; Mangan, M.A. 5.1 Ionization: Datasheet from Landolt-Börnstein—Group I Elementary Particles, Nuclei and Atoms. In Interactions of Photons and Electrons with Molecules; Springer Materials; Springer: Berlin/Heidelberg, Germany, 2003; Volume 17C. [Google Scholar] [CrossRef]
- Kłosowski, Ł.; Piwiński, M. Magnetic Angle Changer for Studies of Electronically Excited Long-Living Atomic States. Atoms 2021, 9, 71. [Google Scholar] [CrossRef]
- Ptasińska, S. A Missing Puzzle in Dissociative Electron Attachment to Biomolecules: The Detection of Radicals. Atoms 2021, 9, 77. [Google Scholar] [CrossRef]
- Karwasz, G.P. Positrons—An alternative probe to electron scattering. Eur. Phys. J. D 2005, 35, 267. [Google Scholar] [CrossRef]
- Campeanu, R.I.; Whelan, C.T. Few Body Effects in the Electron and Positron Impact Ionization of Atoms. Atoms 2021, 9, 33. [Google Scholar] [CrossRef]
- Carelli, F.; Gianturco, F.A.; Franz, J.; Satta, M. A dipole-driven path for electron and positron attachments to gas-phase uracil and pyrimidine molecules: A quantum scattering analysis. Eur. Phys. J. D 2015, 69, 143. [Google Scholar] [CrossRef]
- Karwasz, G.P.; Zecca, A.; Brusa, R.S.; Pliszka, D. Application of positron annihilation techniques for semiconductor studies. Alloy. Compd. 2004, 382, 244. [Google Scholar] [CrossRef]
- García-Abenza, A.; Lozano, A.I.; Oller, J.C.; Blanco, F.; Gorfinkiel, J.D.; Limao-Vieira, P.; García, G. Evaluation of Recommended Cross Sections for the simulation of Electron Tracks in Water. Atoms 2021, 9, 98. [Google Scholar]
- Franz, J.; Fedus, K.; Karwasz, G.P. Do positrons measure atomic and molecular diameters? Eur. Phys. J. D 2016, 70, 155. [Google Scholar] [CrossRef] [Green Version]
- O’Malley, T.F.; Spruch, L.; Rosenberg, L. Modification of Effective-Range Theory in the Presence of a Long—Range r-4 Potential. J. Math. Phys. 1961, 2, 491. [Google Scholar] [CrossRef]
- Buckman, S.J.; Mitroy, J. Analysis of low-energy electron scattering cross sections via effective-range theory. J. Phys. B. 1989, 22, 1365. [Google Scholar] [CrossRef]
- Fedus, K.; Karwasz, G.P. Ramsauer-Townsend minimum in methane—Modified effective range analysis. Eur. Phys. J. D 2014, 68, 93. [Google Scholar] [CrossRef] [Green Version]
- Idziaszek, Z.; Karwasz, G. Applicability of modified effective-range theory to positron-atom and positron-molecule scattering. Phys. Rev. A 2006, 73. [Google Scholar] [CrossRef] [Green Version]
- Harland, P.W.; Vallance, C. Ionization cross-sections and ionization efficiency curves from polarizability volumes and ionization potentials. Int. J. Mass Spectr. Ion Proc. 1997, 171, 173–181. [Google Scholar] [CrossRef]
- Karwasz, G.P.; Możejko, P.; Song, M.Y. Electron-impact ionization of fluoromethanes—Review of experiments and binary-encounter models. Int. J. Mass Spectrom. 2014, 365–366, 232–237. [Google Scholar] [CrossRef]
- Szmytkowski, C. On trends in total cross sections for electron (positron) scattering on atoms and molecules at intermediate energies. Z. Phys. D 1989, 13, 69–73. [Google Scholar] [CrossRef]
- Karwasz, G.P.; Brusa, R.S.; Piazza, A.; Zecca, A. Total cross sections for electron scattering on chloromethanes: Formulation of the additivity rule. Phys. Rev. A 1999, 59, 1341. [Google Scholar] [CrossRef] [Green Version]
- Kim, Y.K.; Rudd, M.E. Binary-encounter-dipole model for electron-impact ionization. Phys. Rev. A 1994, 50, 3954. [Google Scholar] [CrossRef] [Green Version]
- Song, M.; Yoon, J.; Cho, H.; Karwasz, G.; Kokoouline, V.; Nakamura, Y.; Hamilton, J.R.; Tennyson, J. Cross Sections for Electron Collisions with NF3. J. Phys. Chem. Ref. Data 2017, 46, 043104. [Google Scholar] [CrossRef] [Green Version]
- Kawahara, H.; Suzuki, D.; Kato, H.; Hoshino, M.; Tanaka, H.; Ingolfsson, O.; Campbell, L.; Brunger, M.J. Cross sections for electron impact excitation of the C1Π and D1Σ+ electronic states in N2O. J. Chem. Phys. 2009, 131, 114307. [Google Scholar] [CrossRef] [PubMed]
- Xu, W.Q.; Ma, Z.R.; Peng, Y.G.; Du, X.J.; Xu, Y.C.; Wang, L.H.; Li, B.; Zhang, H.R.; Zhang, B.Y.; Zhu, J.H.; et al. Cross sections for the electron-impact excitations A˜1B1 and B˜1A1 of H2O determined by high-energy electron scattering. Phys. Rev. A 2021, 103, 032808. [Google Scholar] [CrossRef]
- Kang, X.; Xu, L.Q.; Liu, Y.W.; Wang, S.X.; Yang, K.; Peng, Y.G.; Ni, D.D.; Hiraoka, N.; Tsuei, K.D.; Zhu, L.F. A study on the validity of the first Born approximation for high-energy electron scattering with nitrogen molecules. J. Phys. B At. Mol. Opt. Phys. 2019, 52, 245202. [Google Scholar] [CrossRef]
- Marler, J.P.; Surko, C.M. Systematic comparison of positron—And electron-impact excitation of the ν3 vibrational mode of CF4. Phys. Rev. A 2005, 72, 062702. [Google Scholar] [CrossRef] [Green Version]
- Ayouz, M.; Faure, A.; Tennyson, J.; Kokoouline, V.; Tudorovskaya, M. Cross Sections and Rate Coefficients for Vibrational Excitation of H2O by Electron Impact. Atoms 2021, 9, 62. [Google Scholar] [CrossRef]
- Poveda, L.A.; Varella, M.T.d.N.; Mohallem, J.R. Vibrational Excitation Cross-Section by Positron Impact: A Wave-Packet Dynamics Study. Atoms 2021, 9, 64. [Google Scholar] [CrossRef]
- Kitajima, M.; Kishino, T.; Okumura, T.; Kobayashi, N.; Sayama, A.; Mori, Y.; Kosaka, K.; Odigiri, F.; Hoshino, M.; Tanaka, H. Low-energy and very-low energy total cross sections for electron collisions with N2. Eur. Phys. J. D 2017, 71, 139. [Google Scholar] [CrossRef]
- Idziaszek, Z.; Karwasz, G.P. Modified effective-range theory for low energy e -N2 scattering. Eur. Phys. J. D 2009, 51, 347–355. [Google Scholar] [CrossRef]
- Idziaszek, Z.; Karwasz, G.P.; Brusa, R.S. Modified effective range analysis of low energy electron and positron scattering on CO2. J. Phys. Conf. Ser. 2008, 115, 012002. [Google Scholar] [CrossRef]
- Fedus, K.; Karwasz, G. Ramsauer–Townsend minimum in electron scattering from CF4: Modified effective range analysis. Eur. Phys. J. D 2021, 75, 76. [Google Scholar] [CrossRef]
- Allan, M. Excitation of vibrational levels up to ν = 17 in N2 by electron impact in 0-5 eV region. J. Phys. B 1986, 18, 4511. [Google Scholar] [CrossRef]
- Zecca, A.; Karwasz, G.P.; Brusa, R.S. One century of experiments on electron-atom and molecule scattering: A critical review of integral cross-sections. La Riv. del Nuovo C. 1996, 19, 1–146. [Google Scholar] [CrossRef]
- Antoni, T.; Jung, K.; Ehrhardt, H.; Chang, E.S. Rotational branch analysis of the excitation of the fundamental vibrational modes of CO2 by slow electron collisions. J. Phys. B 1986, 19, 1377. [Google Scholar] [CrossRef]
- Karwasz, G.P.; Brusa, R.S.; Zecca, A. 6.1 Total scattering cross sections: Datasheet from Landolt-Börnstein—Group I Elementary Particles, Nuclei and Atoms. In Interactions of Photons and Electrons with Molecules; Springer Materials; Springer: Berlin/Heidelberg, Germany, 2003; Volume 17C. [Google Scholar] [CrossRef]
- Isaacs, W.A.; McCurdy, C.W.; Rescigno, T.N. Theoretical support for a Ramsauer-Townsend minimum in electron-CF4 scattering. Phys. Rev. A 1998, 58, 309. [Google Scholar] [CrossRef]
- Winstead, C.; McKoy, V.; Sun, Q. Low-energy elastic electron scattering by tetrafluoromethane (CF4). J. Chem. Phys. 1993, 98, 1105–1109. [Google Scholar] [CrossRef] [Green Version]
- Costa, R.F.d.; Varella, M.T.D.N.; Bettega, M.H.F.; Lima, M.A.P. Recent advances in the application of the Schwinger multichannel method with pseudopotentials to electron-molecule collisions. Eur. Phys. J. D 2015, 69, 159. [Google Scholar] [CrossRef] [Green Version]
- Gianturco, F.A.; Lucchese, R.R. The elastic scattering of electrons from molecules: II. Molecular features and spatial symmetries of some resonant states. J. Phys. B. 1996, 29, 3955. [Google Scholar] [CrossRef]
- Hamilton, J.R.; Tennyson, J.; Huang, S.; Kushner, M.J. Calculated cross sections for electron collisions with NF3, NF2 and NF with applications to remote plasma sources. Plasma Sources Sci. Technol. 2017, 26, 065010. [Google Scholar] [CrossRef] [Green Version]
- Jain, A. Total (elastic+absorption) cross sections for e-CH4 collisions in a spherical model at 0.10–500 eV. Phys. Rev. A 1986, 34, 3707. [Google Scholar] [CrossRef] [PubMed]
- Thirumalai, D.; Staszewska, G.; Truhlar, D.G. Dispersion Relation Techniques for Approximating the Optical Model Potential for Electron Scattering. Comments At. Mol. Phys. 1987, 20, 217–243. [Google Scholar]
- Goswami, B.; Naghma, R.; Antony, B. Calculation of electron impact total ionization cross sections for tungsten, uranium and their oxide radicals. Int. J. Mass Spectr. 2014, 372, 8. [Google Scholar] [CrossRef]
- Gorfinkiel, J.D.; Morgan, L.A.; Tennyson, J. Electron impact dissociative excitation of water within the adiabatic nuclei approximation. J. Phys. B. 2002, 35, 543. [Google Scholar] [CrossRef]
- Bishop, D.M.; Cheung, L.M. Vibrational Contributions to Molecular Dipole Polarizabilities. J. Phys. Chem. Ref. Data 1982, 11, 119. [Google Scholar] [CrossRef] [Green Version]
- Laporta, V.; Celiberto, R.; Vadehra, J.M. Theoretical vibrational-excitation cross sections and rate coefficients for electron-impact resonant collisions involving rovibrationally excited N2 and NO molecules. Plasma Sources Sci. Technol. 2012, 21, 055018. [Google Scholar] [CrossRef] [Green Version]
- Trevisan, C.S.; Houfek, K.; Shang, Z.; Orel, A.E.; McCurdy, C.W.; Rescigno, T.N. Nonlocal model of dissociative electron attachment and vibrational excitation of NO. Phys. Rev. A 2005, 71, 052714. [Google Scholar] [CrossRef] [Green Version]
- Bartschat, K.; McEachran, R.P.; Stauffer, A.D. Optical potential approach to electron and positron scattering from noble gases. I. Argon. J. Phys. B. 1988, 21, 2789. [Google Scholar] [CrossRef]
- Joachain, C.J.; Potvliege, R.M. Importance of absorption effects on fast positron-argon differential cross sections. Phys. Rev. A 1987, 35, 4873. [Google Scholar] [CrossRef]
- Karwasz, G.P.; Pliszka, D.; Brusa, R.S. Total cross sections for positron scattering in argon, nitrogen and hydrogen below 20 eV. Nucl. Instr. Meth. B 2006, 247, 68. [Google Scholar] [CrossRef]
- Fedus, K. A rigid sphere approach to positron elastic scattering by noble gases, molecular hydrogen, nitrogen and methane. Eur. Phys. J. D 2016, 70, 261. [Google Scholar] [CrossRef] [Green Version]
- Borghesani, F.A. Accurate Electron Drift Mobility Measurements in Moderately Dense Helium Gas at Several Temperatures. Atoms 2021, 9, 52. [Google Scholar] [CrossRef]
- Gerjuoy, E.; Krall, N.A. Dispersion Relations in Atomic Scattering Problems. Phys. Rev. 1960, 119, 705. [Google Scholar] [CrossRef]
- Temkin, A.; Bhatia, A.K.; Kim, Y.S. A new dispersion relation for electron-atom scattering. J. Phys. B. 1986, 19, L701. [Google Scholar] [CrossRef]
- Kauppila, W.E.; Stein, T.S.; Smart, J.H.; Dababneh, M.S.; Ho, Y.K.; Downing, J.P.; Pol, V. Measurements of total scattering cross sections for intermediate-energy positrons and electrons colliding with helium, neon, and argon. Phys. Rev. A 1982, 24, 725. [Google Scholar] [CrossRef]
- Salvat, F. Optical-model potential for electron and positron elastic scattering by atoms. Phys. Rev. A 2003, 68, 012708. [Google Scholar] [CrossRef] [Green Version]
- Heer, F.J.d.; McDowell, M.R.C.; Wagenaar, R.W. Numerical study of the dispersion relation for e–H scattering. J. Phys. B. 1977, 10, 1945. [Google Scholar] [CrossRef]
- Jansen, R.H.J.; Heer, F.J.d.; Luyken, H.J.; Wingerden, B.v.; Blaauw, H.J. Absolute differential cross sections for elastic scattering of electrons by helium, neon, argon and molecular nitrogen. J. Phys. B. 1975, 9, 185. [Google Scholar] [CrossRef]
- Bromberg, J.P. Absolute differential cross sections of elastically scattered electrons. V. O2 and CO2 at 500, 400, and 300 eV. J. Chem. Phys. 1974, 60, 1717. [Google Scholar] [CrossRef]
- Zhang, Y.; Ross, A.W.; Fink, M. Electron correlation and charge density study of N2 and O2 by high energy electron scattering. Z. Phys. D 1991, 18, 163–169. [Google Scholar] [CrossRef]
- Hyder, M.A.; Dababneb, M.S.; Hsieh, Y.F.; Kauppila, W.E.; Kwan, C.K.; Mahdavi-Hezaveh, M.; Stein, T.S. Positron Differential Elastic-Scattering Cross-Section Measurements for Argon. Phys. Rev. Lett. 1986, 57, 2252. [Google Scholar] [CrossRef]
- Joachain, C.J.; Vanderpoorten, R.; Winters, K.H.; Byron, F.W., Jr. Optical model theory of elastic electron- and positron-argon scattering at intermediate energies. J. Phys. B 1977, 10, 227. [Google Scholar] [CrossRef]
- Fedus, K. Elastic Scattering of Slow Electrons by Noble Gases—The Effective Range Theory and the Rigid Sphere Model. Atoms 2021, 9, 91. [Google Scholar] [CrossRef]
- Hoffman, K.R.; Dababneh, M.S.; Hsieh, Y.F.; Kauppila, W.E.; Pol, V.; Smart, J.H.; Stein, T.S. Total-cross-section measurements for positrons and electrons colliding with H2, N2, and CO2. Phys. Rev. A 1982, 25, 1393. [Google Scholar] [CrossRef]
- Barozzi, M. Misure di Sezione d’Urto Positrone-Molecola: Realizzazione dell’Apparato Sperimentale e Prime Misure. Master’s Thesis, Universita’ degli Studi di Trento, Trento, Italy, 1997. [Google Scholar]
- Garcia, G.; Manero, F. Total cross sections for electron scattering by CO2 molecules in the energy range 400–5000 eV. Phys. Rev. A 1996, 53, 250. [Google Scholar] [CrossRef]
- Szmytkowski, C.; Zecca, A.; Karwasz, G.; Oss, S.; Maciąg, K.; Marinković, B.; Brusa, R.S.; Grisenti, R. Absolute total cross sections for electron-CO2 scattering at energies form 0.5 to 3000 eV. J. Phys. B. 1987, 20, 5817. [Google Scholar] [CrossRef]
- Zecca, A.; Karwasz, G.P.; Brusa, R.S. Total-cross-section measurements for electron scattering by NH3, SiH4, and H2S in the intermediate-energy range. Phys. Rev. A 1992, 45, 2777. [Google Scholar] [CrossRef]
- Kwan, C.K.; Hsieh, Y.F.; Kauppila, W.E.; Smith, S.J.; Stein, T.S.; Uddin, M.N.; Dababneh, M.S. e±—CO and e±—CO2 total cross-section measurements. Phys. Rev. A 1983, 27, 1328. [Google Scholar] [CrossRef]
- Szmytkowski, C.; Krzysztofowicz, A.; Janicki, P.; Rosenthal, L. Electron scattering from CF4 and CCl4. Total cross section measurements. Chem. Phys. Lett. 1992, 199, 191. [Google Scholar] [CrossRef]
- Iga, I.; Homem, M.G.P.; Mazon, K.T.; Lee, M.T. Elastic and total cross sections for electron-carbon dioxide collisions in the intermediate energy range. J. Phys. B. 1999, 32. [Google Scholar] [CrossRef]
- Karwasz, G.P. Intermediate-energy total cross sections for electron scattering on GeH4. J. Phys. B. 1995, 28, 1301. [Google Scholar] [CrossRef] [Green Version]
- Zecca, A.; Szmytkowski, C.; Karwasz, G.; Brusa, R.S. Absolute total cross sections for electron scattering on CH4 molecules in the 1-4000 eV energy range. J. Phys. B. 1991, 24, 2547–2554. [Google Scholar] [CrossRef]
- Karwasz, G.; Brusa, R.S.; Gasparoli, A.; Zecca, A. Total cross-section measurements for e-—CO scattering: 80–4000 eV. Chem. Phys. Lett. 1993, 211, 529–533. [Google Scholar] [CrossRef]
- García, G.; Manero, F. Electron scattering by CH4 molecules at intermediate energies (400–5000 eV). Phys. Rev. A 1998, 57, 1069. [Google Scholar] [CrossRef]
- Bransden, B.H.; Joachain, C.J. Physics of Atoms and Molecules, 2nd ed.; Prentice-Hall: Hoboken, NJ, USA, 2003. [Google Scholar]
- Dubois, R.D.; Rudd, M.E. Differential cross sections for elastic scattering of electrons from argon, neon, nitrogen and carbon monoxide. J. Phys. B 1976, 9, 2657. [Google Scholar] [CrossRef]
- Sakae, T.; Sumiyoshi, S.; Murakami, E.; Matsumoto, Y.; Ishibashi, K.; Katase, A. Scattering of electrons by CH4, CF4 and SF6 in the 75–700 eV range. J. Phys. B. 1989, 22, 1385. [Google Scholar] [CrossRef]
- Szabo, A.; Ostlund, N.S. Calculation of high energy elastic electron-molecule scattering cross sections with CNDO wavefunctions. J. Chem. Phys. 1974, 60, 946–950. [Google Scholar] [CrossRef]
- Garcia, G.; Manero, F. Correlation of the total cross section for electron scattering by molecules with 10–22 electrons, and some molecular parameters at intermediate energies. Chem. Phys. Lett. 1997, 280, 4373. [Google Scholar] [CrossRef]
- Nickel, J.C.; Kanik, I.; Trajmar, S.; Imre, K. Total cross section measurements for electron scattering on H2 and N2 from 4 to 300 eV. J. Phys. B 2017, 25, 2427–2431. [Google Scholar] [CrossRef]
- Kanik, I.; Trajmar, S.; Nickel, J.C. Total cross section measurements for electron scattering on CH4 from 4 to 300 eV. Chem. Phys. Lett. 1992, 193, 281–286. [Google Scholar] [CrossRef]
- Experimental values of Polarizability. Available online: https://cccbdb.nist.gov/xp1x.asp?prop=9 (accessed on 1 September 2021).
- Fedus, K.; Karwasz, G.P.; Idziaszek, Z. Analytic approach to modified effective-range theory for electron and positron elastic scattering. Phys. Rev. A 2013, 88, 012704. [Google Scholar] [CrossRef]
- Khandker, M.H.; Haque, A.K.F.; Haque, M.M.; Billah, M.M.; Watabe, H.; Uddin, M.A. Relativistic Study on the Scattering of e± from Atoms and Ions of the Rn Isonuclear Series. Atoms 2021, 9, 59. [Google Scholar] [CrossRef]
- Dababneh, M.S.; Hsieh, Y.F.; Kauppila, W.E.; Kwan, C.K.; Smith, S.J.; Stein, T.S.; Uddin, M.N. Total-cross-section measurements for positron and electron scattering by O2, CH4, and SF6. Phys. Rev. A 1988, 38, 1207. [Google Scholar] [CrossRef] [PubMed]
- Sueoka, O.; Mori, S. Total cross sections for low and intermediate energy positrons and electrons colliding with CH4, C2H4 and C2H6 molecules. J. Phys. B 1986, 19, 4035. [Google Scholar] [CrossRef]
- Vrinceanu, D.; Msezane, A.Z.; Bessis, D.; Temkin, A. Exchange Forces in Dispersion Relations Investigated Using Circuit Relations. Phys. Rev. Lett. 2001, 86, 3256. [Google Scholar] [CrossRef] [PubMed]
- Coat, Y.L.; Ziesel, J.P.; Guillotin, J.P. Negative ion resonances in CF4 probed by dissociative electron attachment. J. Phys. B. 1994, 27, 965. [Google Scholar] [CrossRef]
- Sullivan, J.P.; Gilbert, S.J.; Buckman, S.; Surko, C. Search for resonances in the scattering of low-energy positrons from atoms and molecules. J. Phys. B 2001, 34, L467. [Google Scholar] [CrossRef]

| Molecule | † | ∫ TCS ‡ | § | TCS@30 eV ¶ | B‖ | ||
|---|---|---|---|---|---|---|---|
| N | [39] | 7.89 [70] | 16.5 | 11.5 | 12.8 [91] | 310 | |
| * [38] | |||||||
| CO | [40] | 15.4 [69] | 23.9 | 16.9 | 16.2 [77] | 510 | |
| CH | [24] | 6.5 * [88] | 16.1 | 16.5 | 16.5 [92] | 232 | |
| CF | [41] | 19 * [88] | 35.7 | 19.1 | 20.4 [80] | 923 |
| Molecule | † | ∫ TCS | TCS@30 eV ‡ | |||
|---|---|---|---|---|---|---|
| N | [25] | [70] | 17.16 | 13.8 | 11.5 | 8.2 [74] |
| CO | [40] | [69] | 20.01 | 18.7 | 16.9 | 10.2 [74] |
| CH | to | [88] | 12.10 | 13.8 | 16.5 | 10.6 [96] |
| * [24] | 15.00 | 11.1 [97] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carelli, F.; Fedus, K.; Karwasz, G. Total Cross Sections for Electron and Positron Scattering on Molecules: In Search of the Dispersion Relation. Atoms 2021, 9, 97. https://doi.org/10.3390/atoms9040097
Carelli F, Fedus K, Karwasz G. Total Cross Sections for Electron and Positron Scattering on Molecules: In Search of the Dispersion Relation. Atoms. 2021; 9(4):97. https://doi.org/10.3390/atoms9040097
Chicago/Turabian StyleCarelli, Fabio, Kamil Fedus, and Grzegorz Karwasz. 2021. "Total Cross Sections for Electron and Positron Scattering on Molecules: In Search of the Dispersion Relation" Atoms 9, no. 4: 97. https://doi.org/10.3390/atoms9040097
APA StyleCarelli, F., Fedus, K., & Karwasz, G. (2021). Total Cross Sections for Electron and Positron Scattering on Molecules: In Search of the Dispersion Relation. Atoms, 9(4), 97. https://doi.org/10.3390/atoms9040097








