On the Accuracy of Random Phase Approximation for Dynamical Structure Factors in Cold Atomic Gases
Abstract
:1. Introduction
2. Models and Methods
2.1. The Fermi–Hubbard Model and Correlation Functions
2.2. BCS, GRPA, and Linear Response
3. Results and Discussion
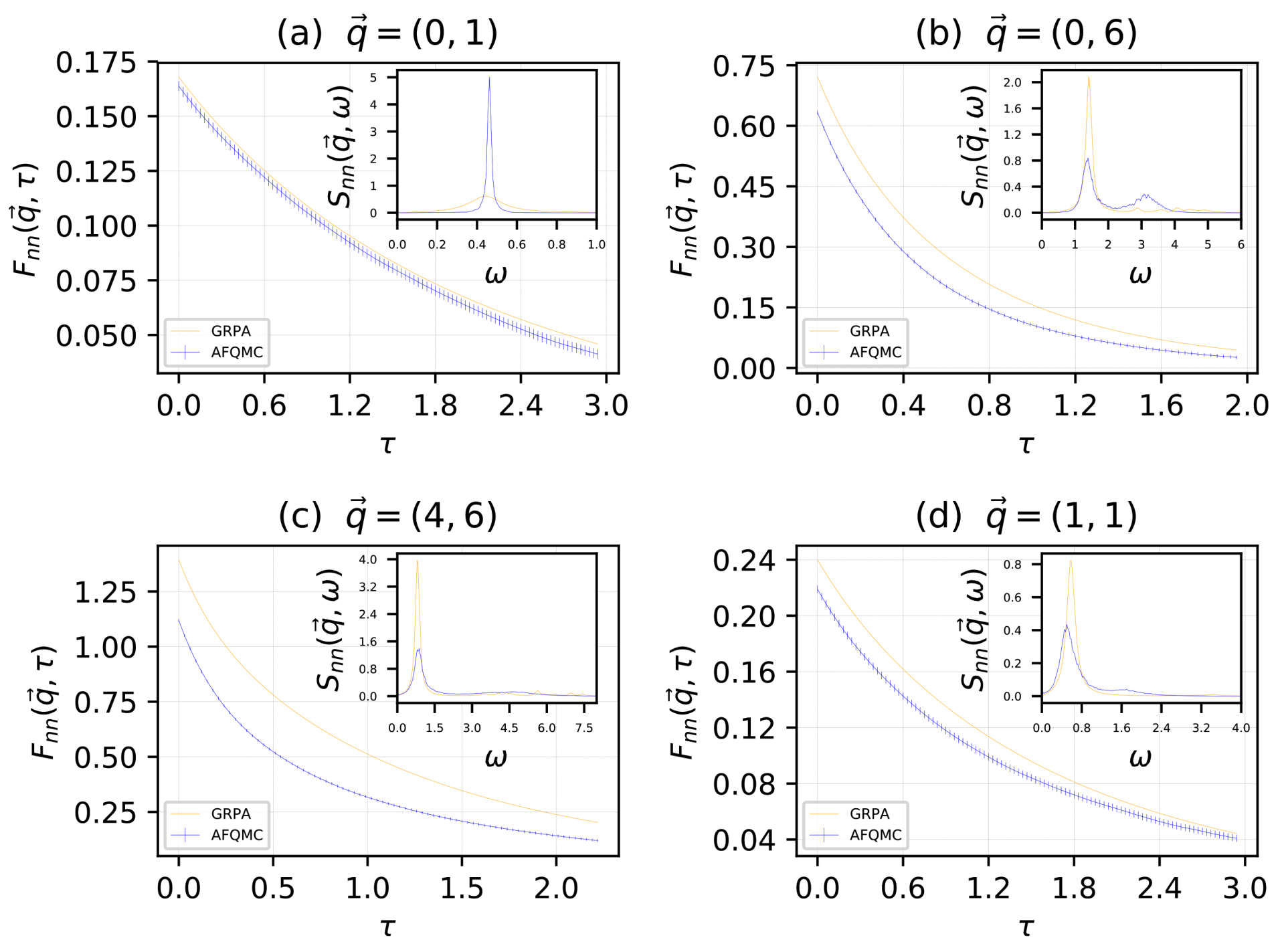
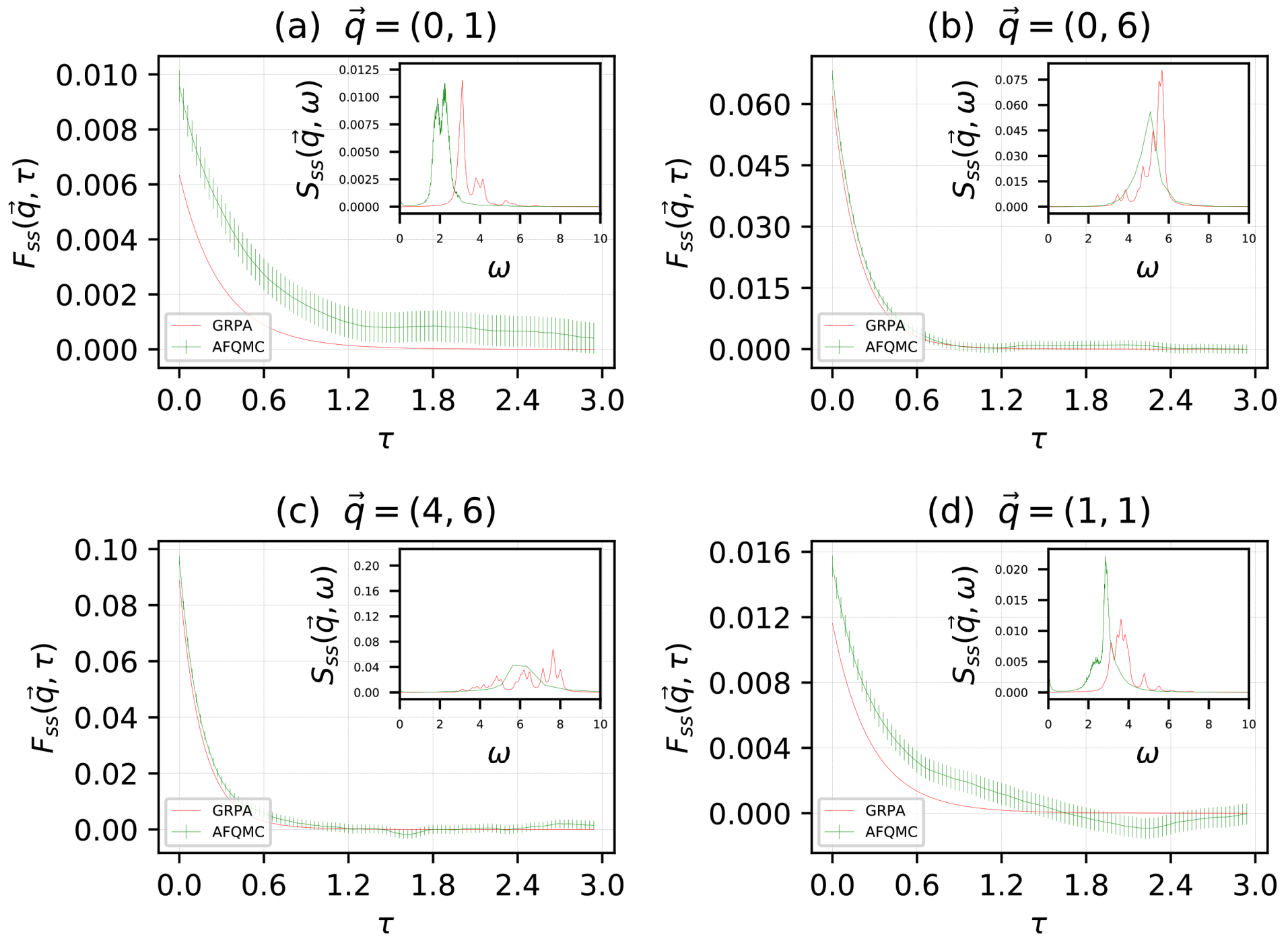
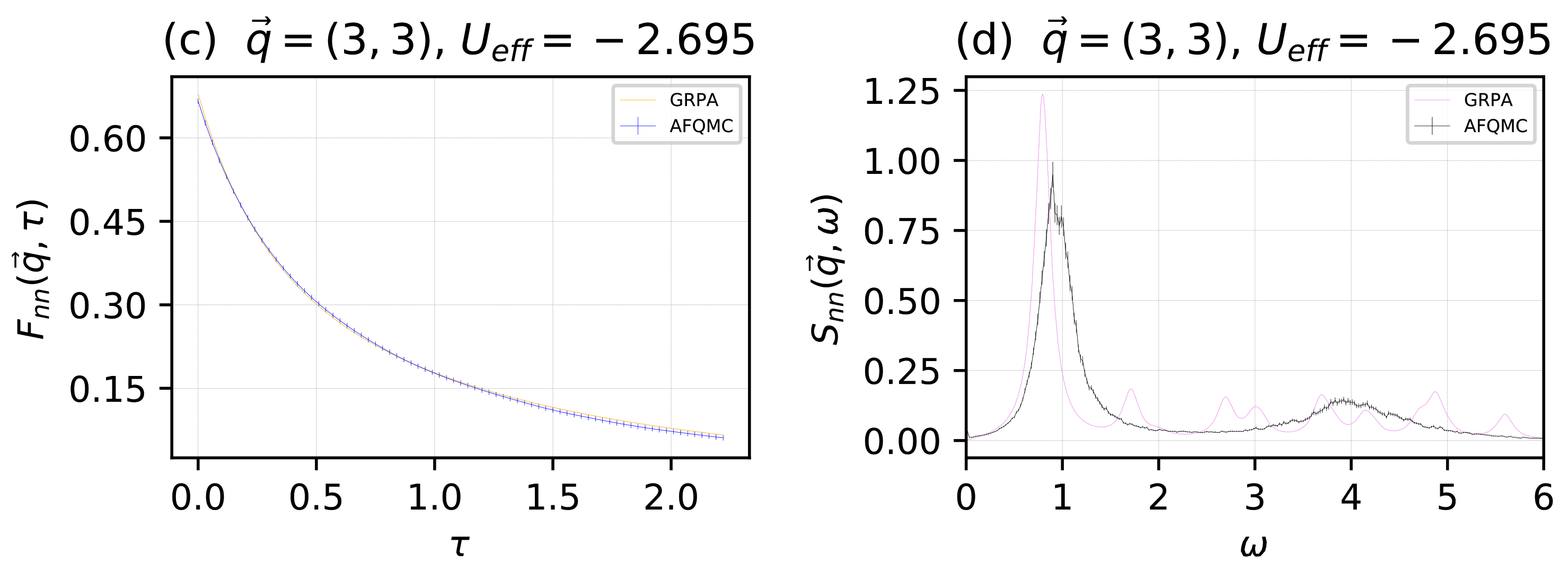
3.1. Comparison of GRPA and AFQMC
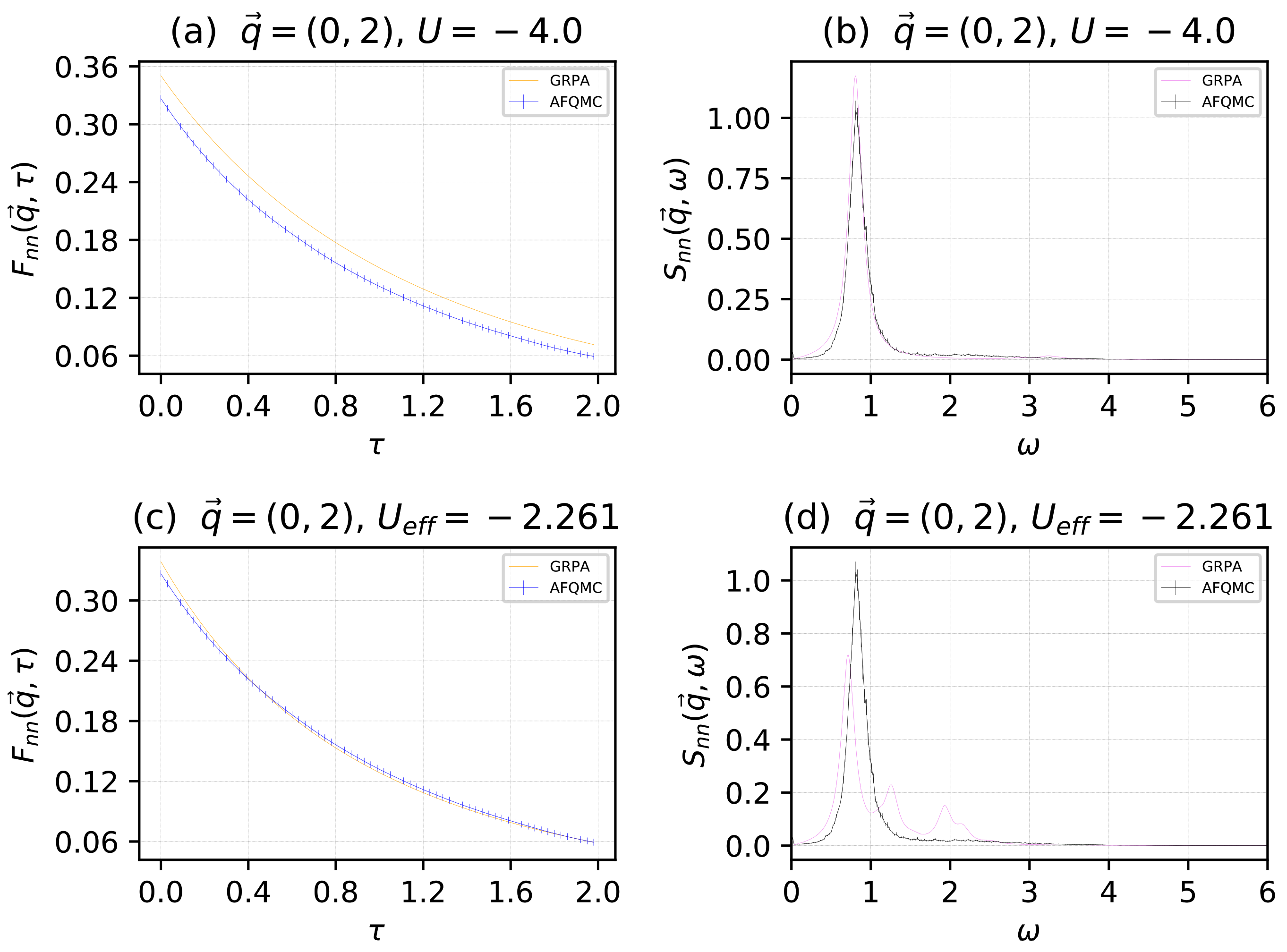
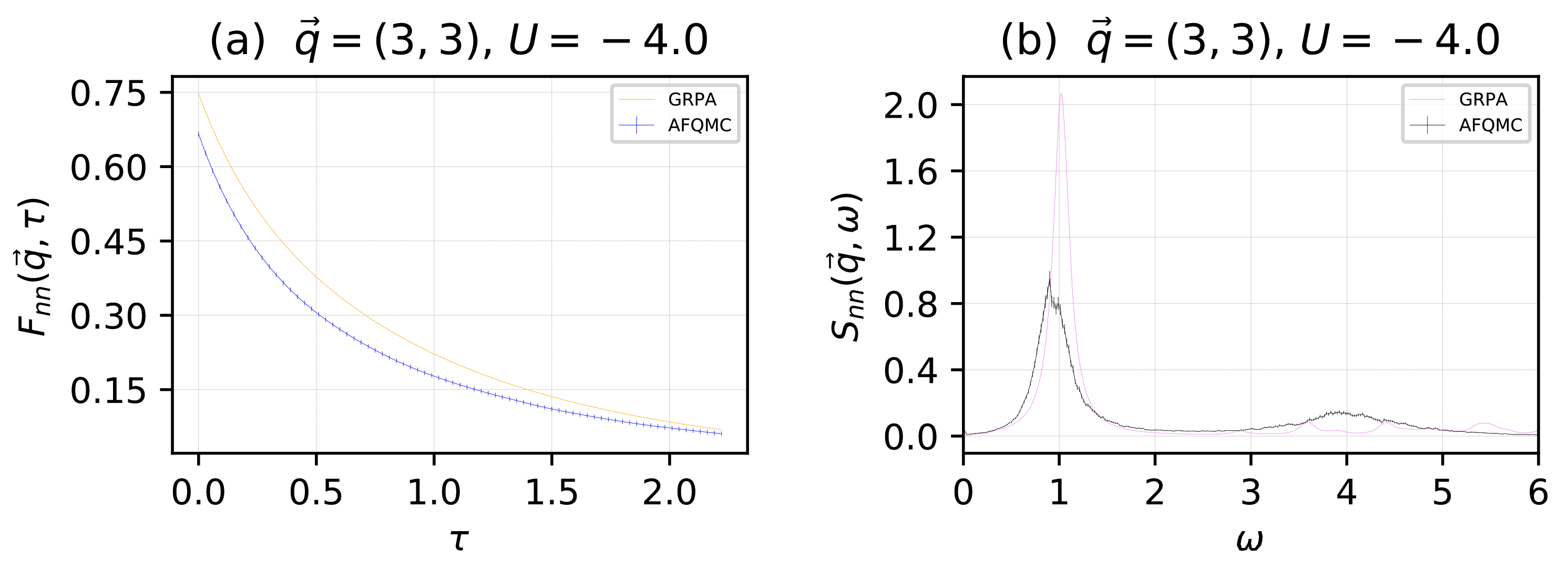
3.2. Optimizing GRPA Using a Renormalized Effect Potential
3.3. Accuracy of GRPA for Static and Dynamic Properties
4. Conclusions and Future Directions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AFQMC | Auxiliary Field Quantum Monte Carlo |
| BCS | Bardeen–Cooper–Schrieffer |
| DDFT | Dynamical Density Functional Theory |
| DMRG | Density Matrix Renormalization Group |
| GRPA | Generalized Random Phase Approximation |
| HFB | Hartree–Fock–Bogoliubov |
| QMC | Quantum Monte Carlo |
References
- Giorgini, S.; Pitaevskii, L.P.; Stringari, S. Theory of ultracold atomic Fermi gases. Rev. Mod. Phys. 2008, 80, 1215–1274. [Google Scholar] [CrossRef] [Green Version]
- Bloch, I.; Dalibard, J.; Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 2008, 80, 885–964. [Google Scholar] [CrossRef] [Green Version]
- Gross, C.; Bloch, I. Quantum simulations with ultracold atoms in optical lattices. Science 2017, 357, 995–1001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rosenberg, P.; Shi, H.; Zhang, S. Ultracold Atoms in a Square Lattice with Spin-Orbit Coupling: Charge Order, Superfluidity, and Topological Signatures. Phys. Rev. Lett. 2017, 119, 265301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lin, Y.J.; Compton, R.L.; Jiménez-García, K.; Porto, J.V.; Spielman, I.B. Synthetic magnetic fields for ultracold neutral atoms. Nature 2009, 462, 628–632. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Carlson, J.; Gandolfi, S.; Gezerlis, A. Quantum Monte Carlo approaches to nuclear and atomic physics. Prog. Theor. Exp. Phys. 2012, 2012, 01A209. [Google Scholar] [CrossRef] [Green Version]
- van Wyk, P.; Tajima, H.; Inotani, D.; Ohnishi, A.; Ohashi, Y. Superfluid Fermi atomic gas as a quantum simulator for the study of the neutron-star equation of state in the low-density region. Phys. Rev. A 2018, 97, 013601. [Google Scholar] [CrossRef] [Green Version]
- Brown, P.T.; Mitra, D.; Guardado-Sanchez, E.; Nourafkan, R.; Reymbaut, A.; Hébert, C.D.; Bergeron, S.; Tremblay, A.M.S.; Kokalj, J.; Huse, D.A.; et al. Bad metallic transport in a cold atom Fermi-Hubbard system. Science 2019, 363, 379–382. [Google Scholar] [CrossRef] [Green Version]
- Zhang, D.W.; Zhu, Y.Q.; Zhao, Y.X.; Yan, H.; Zhu, S.L. Topological quantum matter with cold atoms. Adv. Phys. 2018, 67, 253–402. [Google Scholar] [CrossRef]
- Mitra, D.; Brown, P.T.; Guardado-Sanchez, E.; Kondov, S.S.; Devakul, T.; Huse, D.A.; Schauß, P.; Bakr, W.S. Quantum Gas Microscopy of an Attractive Fermi–Hubbard System. Nat. Phys. 2018, 14, 173–177. [Google Scholar] [CrossRef] [Green Version]
- Bulgac, A. Time-Dependent Density Functional Theory and the Real-Time Dynamics of Fermi Superfluids. Annu. Rev. Nucl. Part. Sci. 2013, 63, 97. [Google Scholar] [CrossRef] [Green Version]
- Ganesh, R.; Paramekanti, A.; Burkov, A.A. Collective modes and superflow instabilities of strongly correlated Fermi superfluids. Phys. Rev. A 2009, 80, 043612. [Google Scholar] [CrossRef] [Green Version]
- Yunomae, Y.; Yamamoto, D.; Danshita, I.; Yokoshi, N.; Tsuchiya, S. Instability of superfluid Fermi gases induced by a rotonlike density mode in optical lattices. Phys. Rev. A 2009, 80, 063627. [Google Scholar] [CrossRef] [Green Version]
- Zhao, H.; Gao, X.; Liang, W.; Zou, P.; Yuan, F. Dynamical structure factors of a two-dimensional Fermi superfluid within random phase approximation. New J. Phys. 2020, 22, 093012. [Google Scholar] [CrossRef]
- Vitali, E.; Shi, H.; Qin, M.; Zhang, S. Visualizing the BEC-BCS crossover in a two-dimensional Fermi gas: Pairing gaps and dynamical response functions from ab initio computations. Phys. Rev. A 2017, 96, 061601. [Google Scholar] [CrossRef] [Green Version]
- Vitali, E.; Kelly, P.; Lopez, A.; Bertaina, G.; Galli, D.E. Dynamical structure factor of a fermionic supersolid on an optical lattice. Phys. Rev. A 2020, 102, 053324. [Google Scholar] [CrossRef]
- Shi, H.; Chiesa, S.; Zhang, S. Ground-state properties of strongly interacting Fermi gases in two dimensions. Phys. Rev. A 2015, 92, 033603. [Google Scholar] [CrossRef] [Green Version]
- Bertaina, G.; Giorgini, S. BCS-BEC Crossover in a Two-Dimensional Fermi Gas. Phys. Rev. Lett. 2011, 106, 110403. [Google Scholar] [CrossRef] [Green Version]
- Vitali, E.; Shi, H.; Qin, M.; Zhang, S. Computation of dynamical correlation functions for many-fermion systems with auxiliary-field quantum Monte Carlo. Phys. Rev. B 2016, 94, 085140. [Google Scholar] [CrossRef] [Green Version]
- Hoinka, S.; Lingham, M.; Delehaye, M.; Vale, C.J. Dynamic Spin Response of a Strongly Interacting Fermi Gas. Phys. Rev. Lett. 2012, 109, 050403. [Google Scholar] [CrossRef]
- Zhang, S. Auxiliary-Field Quantum Monte Carlo for Correlated Electron Systems. In Emergent Phenomena in Correlated Matter: Modeling and Simulation; Pavarini, E., Koch, E., Schollwöck, U., Eds.; Verlag des Forschungszentrum: Jülich, Germany, 2013; Volume 3. [Google Scholar]
- Bertaina, G.; Galli, D.E.; Vitali, E. Statistical and computational intelligence approach to analytic continuation in Quantum Monte Carlo. Adv. Phys. X 2017, 2, 302–323. [Google Scholar] [CrossRef] [Green Version]
- Vitali, E.; Rossi, M.; Reatto, L.; Galli, D.E. Ab initio low-energy dynamics of superfluid and solid 4He. Phys. Rev. B 2010, 82, 174510. [Google Scholar] [CrossRef] [Green Version]
- Moskowitz, J.W.; Schmidt, K.E.; Lee, M.A.; Kalos, M.H. A new look at correlation energy in atomic and molecular systems. II. The application of the Green’s function Monte Carlo method to LiH. J. Chem. Phys. 1982, 77, 349–355. [Google Scholar] [CrossRef]
- Reynolds, P.J.; Ceperley, D.M.; Alder, B.J.; Lester, W.A. Fixed-node quantum Monte Carlo for molecules. J. Chem. Phys. 1982, 77, 5593–5603. [Google Scholar] [CrossRef]
- Varma, C.M. Higgs Boson in Superconductors. J. Low Temp. Phys. 2002, 126, 901–909. [Google Scholar] [CrossRef]
- Cea, T.; Castellani, C.; Seibold, G.; Benfatto, L. Nonrelativistic Dynamics of the Amplitude (Higgs) Mode in Superconductors. Phys. Rev. Lett. 2015, 115, 157002. [Google Scholar] [CrossRef]
- Podolsky, D.; Auerbach, A.; Arovas, D.P. Visibility of the amplitude (Higgs) mode in condensed matter. Phys. Rev. B 2011, 84, 174522. [Google Scholar] [CrossRef] [Green Version]
- Shimano, R.; Tsuji, N. Higgs Mode in Superconductors. Annu. Rev. Condens. Matter Phys. 2020, 11, 103–124. [Google Scholar] [CrossRef] [Green Version]
- Pekker, D.; Varma, C. Amplitude/Higgs Modes in Condensed Matter Physics. Annu. Rev. Condens. Matter Phys. 2015, 6, 269–297. [Google Scholar] [CrossRef] [Green Version]
- Saccani, S.; Moroni, S.; Boninsegni, M. Excitation Spectrum of a Supersolid. Phys. Rev. Lett. 2012, 108, 175301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Saccani, S.; Moroni, S.; Vitali, E.; Boninsegni, M. Bose Soft Discs: A Minimal Model for Supersolidity. Mol. Phys. 2011, 109, 2807–2812. [Google Scholar] [CrossRef]
- Chomaz, L.; Petter, D.; Ilzhöfer, P.; Natale, G.; Trautmann, A.; Politi, C.; Durastante, G.; van Bijnen, R.M.W.; Patscheider, A.; Sohmen, M.; et al. Long-Lived and Transient Supersolid Behaviors in Dipolar Quantum Gases. Phys. Rev. X 2019, 9, 021012. [Google Scholar] [CrossRef] [Green Version]
- Natale, G.; van Bijnen, R.M.W.; Patscheider, A.; Petter, D.; Mark, M.J.; Chomaz, L.; Ferlaino, F. Excitation Spectrum of a Trapped Dipolar Supersolid and Its Experimental Evidence. Phys. Rev. Lett. 2019, 123, 050402. [Google Scholar] [CrossRef] [Green Version]
- Guo, M.; Böttcher, F.; Hertkorn, J.; Schmidt, J.N.; Wenzel, M.; Büchler, H.P.; Langen, T.; Pfau, T. The Low-Energy Goldstone Mode in a Trapped Dipolar Supersolid. Nature 2019, 574, 386–389. [Google Scholar] [CrossRef] [Green Version]
- Tanzi, L.; Roccuzzo, S.M.; Lucioni, E.; Famà, F.; Fioretti, A.; Gabbanini, C.; Modugno, G.; Recati, A.; Stringari, S. Supersolid Symmetry Breaking from Compressional Oscillations in a Dipolar Quantum Gas. Nature 2019, 574, 382–385. [Google Scholar] [CrossRef] [Green Version]
- Hansen, J.P.; McDonald, I.R. (Eds.) Chapter 7—Time-dependent Correlation and Response Functions. In Theory of Simple Liquids, 4th ed.; Academic Press: Oxford, UK, 2013; pp. 265–310. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kelly, P.; Vitali, E. On the Accuracy of Random Phase Approximation for Dynamical Structure Factors in Cold Atomic Gases. Atoms 2021, 9, 88. https://doi.org/10.3390/atoms9040088
Kelly P, Vitali E. On the Accuracy of Random Phase Approximation for Dynamical Structure Factors in Cold Atomic Gases. Atoms. 2021; 9(4):88. https://doi.org/10.3390/atoms9040088
Chicago/Turabian StyleKelly, Patrick, and Ettore Vitali. 2021. "On the Accuracy of Random Phase Approximation for Dynamical Structure Factors in Cold Atomic Gases" Atoms 9, no. 4: 88. https://doi.org/10.3390/atoms9040088
APA StyleKelly, P., & Vitali, E. (2021). On the Accuracy of Random Phase Approximation for Dynamical Structure Factors in Cold Atomic Gases. Atoms, 9(4), 88. https://doi.org/10.3390/atoms9040088




