Approximate Solution of the Thomas–Fermi Equation for Free Positive Ions
Abstract
1. Introduction
2. Calculations of k(q) Coefficients
3. Approximations of Functions , and Their Derivatives
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Calculation of Functions ϕ0(x), η0(x) and Their Derivatives
| x | x | ||||
|---|---|---|---|---|---|
| 0.00 | 1.0000000000 | 1.5880710226 | 10.00 | 0.0243142930 | 0.0046028819 |
| 0.01 | 0.9854466129 | 1.3895561166 | 11.00 | 0.0202503650 | 0.0035798152 |
| 0.02 | 0.9719766389 | 1.3093049632 | 12.00 | 0.0170639223 | 0.0028305364 |
| 0.05 | 0.9351919580 | 1.1559954352 | 13.00 | 0.0145265176 | 0.0022705246 |
| 0.10 | 0.8816970767 | 0.9953546461 | 14.00 | 0.0124784060 | 0.0018445014 |
| 0.20 | 0.7930594320 | 0.7942270092 | 15.00 | 0.0108053588 | 0.0015153231 |
| 0.30 | 0.7206394761 | 0.6617997801 | 16.00 | 0.0094240789 | 0.0012574353 |
| 0.40 | 0.6595411608 | 0.5646424441 | 17.00 | 0.0082727639 | 0.0010528868 |
| 0.50 | 0.6069863834 | 0.4894116126 | 18.00 | 0.0073048459 | 0.0008888311 |
| 0.60 | 0.5611620236 | 0.4291718717 | 19.00 | 0.0064847464 | 0.0007559214 |
| 0.70 | 0.5207914565 | 0.3797947453 | 20.00 | 0.0057849412 | 0.0006472543 |
| 0.80 | 0.4849309880 | 0.3386071561 | 21.00 | 0.0051838934 | 0.0005576616 |
| 0.90 | 0.4528587154 | 0.3037757561 | 22.00 | 0.0046645758 | 0.0004832257 |
| 1.00 | 0.4240080521 | 0.2739890516 | 23.00 | 0.0042133981 | 0.0004209437 |
| 1.10 | 0.3979253017 | 0.2482781190 | 24.00 | 0.0038194181 | 0.0003684892 |
| 1.20 | 0.3742412296 | 0.2259085936 | 25.00 | 0.0034737544 | 0.0003240430 |
| 1.30 | 0.3526512782 | 0.2063121826 | 26.00 | 0.0031691444 | 0.0002861695 |
| 1.40 | 0.3329013700 | 0.1890414262 | 27.00 | 0.0028996077 | 0.0002537267 |
| 1.50 | 0.3147774637 | 0.1737387990 | 28.00 | 0.0026601879 | 0.0002257990 |
| 1.60 | 0.2980977070 | 0.1601150078 | 29.00 | 0.0024467526 | 0.0002016471 |
| 1.70 | 0.2827064352 | 0.1479333856 | 30.00 | 0.0022558366 | 0.0001806700 |
| 1.80 | 0.2684695100 | 0.1369984380 | 31.00 | 0.0020845191 | 0.0001623762 |
| 1.90 | 0.2552706498 | 0.1271472890 | 32.00 | 0.0019303255 | 0.0001463611 |
| 2.00 | 0.2430085072 | 0.1182431916 | 33.00 | 0.0017911496 | 0.0001322900 |
| 2.20 | 0.2209499788 | 0.1028309760 | 34.00 | 0.0016651908 | 0.0001198846 |
| 2.40 | 0.2017027012 | 0.0900262759 | 35.00 | 0.0015509032 | 0.0001089121 |
| 2.60 | 0.1848021494 | 0.0792857632 | 36.00 | 0.0014469544 | 0.0000991772 |
| 2.80 | 0.1698782637 | 0.0702003884 | 37.00 | 0.0013521916 | 0.0000905149 |
| 3.00 | 0.1566326732 | 0.0624571309 | 38.00 | 0.0012656139 | 0.0000827855 |
| 3.40 | 0.1342470024 | 0.0500771162 | 39.00 | 0.0011863493 | 0.0000758704 |
| 3.80 | 0.1161656951 | 0.0407527383 | 40.00 | 0.0011136356 | 0.0000696680 |
| 4.20 | 0.1013578688 | 0.0335900970 | 41.00 | 0.0010468047 | 0.0000640915 |
| 4.60 | 0.0890854399 | 0.0279948614 | 42.00 | 0.0009852690 | 0.0000590661 |
| 5.00 | 0.0788077793 | 0.0235600750 | 43.00 | 0.0009285103 | 0.0000545274 |
| 5.50 | 0.0681603623 | 0.0192213484 | 44.00 | 0.0008760706 | 0.0000504195 |
| 6.00 | 0.0594229493 | 0.0158675495 | 45.00 | 0.0008275439 | 0.0000466941 |
| 6.50 | 0.0521729373 | 0.0132356072 | 46.00 | 0.0007825691 | 0.0000433088 |
| 7.00 | 0.0460978186 | 0.0111425318 | 47.00 | 0.0007408251 | 0.0000402270 |
| 7.50 | 0.0409624662 | 0.0094582646 | 48.00 | 0.0007020247 | 0.0000374164 |
| 8.00 | 0.0365872553 | 0.0080886030 | 49.00 | 0.0006659114 | 0.0000348487 |
| 9.00 | 0.0295909353 | 0.0060330747 | 50.00 | 0.0006322548 | 0.0000324989 |
| x | x | ||||
|---|---|---|---|---|---|
| 0.00 | 0.0000 | 1.0000 | 10.00 | 202.6583 | 63.1040 |
| 0.01 | 0.0100 | 1.0010 | 11.00 | 273.6843 | 79.3834 |
| 0.02 | 0.0200 | 1.0028 | 12.00 | 362.3564 | 98.4141 |
| 0.05 | 0.0502 | 1.0110 | 13.00 | 471.5289 | 120.4630 |
| 0.10 | 0.1012 | 1.0306 | 14.00 | 604.3719 | 145.7945 |
| 0.20 | 0.2069 | 1.0846 | 15.00 | 764.3028 | 174.6881 |
| 0.30 | 0.3186 | 1.1528 | 16.00 | 955.0359 | 207.4327 |
| 0.40 | 0.4378 | 1.2321 | 17.00 | 1180.5679 | 244.3242 |
| 0.50 | 0.5654 | 1.3210 | 18.00 | 1445.1810 | 285.6650 |
| 0.60 | 0.7023 | 1.4187 | 19.00 | 1753.4709 | 331.7663 |
| 0.70 | 0.8494 | 1.5246 | 20.00 | 2110.3680 | 382.9501 |
| 0.80 | 1.0075 | 1.6384 | 21.00 | 2521.1430 | 439.5482 |
| 0.90 | 1.1773 | 1.7599 | 22.00 | 2991.3916 | 501.8995 |
| 1.00 | 1.3597 | 1.8889 | 23.00 | 3527.0042 | 570.3437 |
| 1.10 | 1.5554 | 2.0255 | 24.00 | 4134.2403 | 645.2326 |
| 1.20 | 1.7651 | 2.1696 | 25.00 | 4819.7341 | 726.9275 |
| 1.30 | 1.9895 | 2.3212 | 26.00 | 5590.4890 | 815.7977 |
| 1.40 | 2.2295 | 2.4805 | 27.00 | 6453.8716 | 912.2193 |
| 1.50 | 2.4859 | 2.6474 | 28.00 | 7417.6127 | 1016.5747 |
| 1.60 | 2.7593 | 2.8222 | 29.00 | 8489.8225 | 1129.2531 |
| 1.70 | 3.0506 | 3.0048 | 30.00 | 9679.0108 | 1250.6516 |
| 1.80 | 3.3605 | 3.1954 | 31.00 | 10,994.1034 | 1381.1762 |
| 1.90 | 3.6899 | 3.3942 | 32.00 | 12,444.4521 | 1521.2416 |
| 2.00 | 4.0396 | 3.6012 | 33.00 | 14,039.8362 | 1671.2709 |
| 2.20 | 4.8032 | 4.0406 | 34.00 | 15,790.4536 | 1831.6937 |
| 2.40 | 5.6582 | 4.5149 | 35.00 | 17,706.9028 | 2002.9441 |
| 2.60 | 6.6116 | 5.0253 | 36.00 | 19,800.1671 | 2185.4578 |
| 2.80 | 7.6708 | 5.5730 | 37.00 | 22,081.7477 | 2379.6860 |
| 3.00 | 8.8434 | 6.1594 | 38.00 | 24,563.5873 | 2586.0847 |
| 3.40 | 11.5604 | 7.4537 | 39.00 | 27,258.1046 | 2805.1179 |
| 3.80 | 14.8292 | 8.9197 | 40.00 | 30,178.1922 | 3037.2577 |
| 4.20 | 18.7207 | 10.5690 | 41.00 | 33,337.2044 | 3282.9824 |
| 4.60 | 23.3105 | 12.4137 | 42.00 | 36,748.9585 | 3542.7757 |
| 5.00 | 28.6793 | 14.4660 | 43.00 | 40,427.7448 | 3817.1274 |
| 5.50 | 36.6166 | 17.3427 | 44.00 | 44,388.3414 | 4106.5335 |
| 6.00 | 46.0833 | 20.5888 | 45.00 | 48,646.0302 | 4411.4968 |
| 6.50 | 57.2708 | 24.2302 | 46.00 | 53,216.6120 | 4732.5279 |
| 7.00 | 70.3838 | 28.2939 | 47.00 | 58,116.4192 | 5070.1454 |
| 7.50 | 85.6401 | 32.8070 | 48.00 | 63,362.3244 | 5424.8761 |
| 8.00 | 103.2702 | 37.7972 | 49.00 | 68,971.7455 | 5797.2546 |
| 9.00 | 146.6512 | 49.3222 | 50.00 | 74,962.6446 | 6187.8231 |
References
- Thomas, L.H. The calculation of atomic fields. Proc. Camb. Philos. Soc. 1927, 23, 542–598. [Google Scholar] [CrossRef]
- Fermi, E. Un metodo statistico per la determinazione di alcune priorietà dell’atome. Rend. Acad. Naz. Lincei 1927, 6, 602–607. [Google Scholar]
- Fermi, E. Eine statistische Methode zur Bestimmung einiger Eigenschaften des Atoms und ihre Anwendung auf die Theorie des periodischen Systems der Elemente. Z. Physik. 1928, 48, 73–79. [Google Scholar] [CrossRef]
- Fermi, E. Sul calcolo degli spettri degli ioni. Nuovo C. 1931, 8, 7–14. [Google Scholar] [CrossRef]
- Miranda, C. Teoremi e metodi per l’integrazione numerica della equazione differenziale di Fermi. Mem. Accad. Ital. 1934, 5, 285–322. [Google Scholar]
- Fermi, E.; Amaldi, E. Le orbite ∞ s degli elementi. Mem. Accad. Ital. 1934, 6, 119–149. [Google Scholar]
- Sommerfeld, A. Asymptotische Integration der Differentialgleichung des Thomas–Fermischen Atoms. Z. Physik. 1932, 78, 283–308. [Google Scholar] [CrossRef]
- Gombás, P. Die Statistische Theorie des Atoms und ihre Anwendungen; Springer: Vienna, Austria, 1949. [Google Scholar]
- Umeda, K.; Kobayashi, S. Systematization of the approximate solutions of the Thomas–Fermi equation. J. Phys. Soc. Jpn. 1955, 10, 749–752. [Google Scholar] [CrossRef]
- Kobayashi, S.; Matsukuma, T.; Nagai, S.; Umeda, K. Accurate value of the initial slope of the ordinary TF function. J. Phys. Soc. Jpn. 1955, 10, 759–762. [Google Scholar] [CrossRef]
- Kobayashi, S. Fermi part η0 of the TF function for free positive ion. J. Phys. Soc. Jpn. 1956, 11, 609–610. [Google Scholar] [CrossRef]
- Kobayashi, S. Thomas–Fermi model of positive ion. J. Phys. Soc. Jpn. 1959, 14, 1039–1054. [Google Scholar] [CrossRef]
- Mason, J.C. Rational approximations to the ordinary Thomas–Fermi function and its derivative. Proc. Phys. Soc. 1964, 84, 357–359. [Google Scholar] [CrossRef]
- Dmitrieva, I.K.; Plindov, G.I. Non–relativistic binding energy of heavy ion. Phys. Lett. 1975, 55A, 3–4. [Google Scholar] [CrossRef]
- Plindov, G.I.; Dmitrieva, I.K. On the nonrelativistic binding energy for positive ions. J. Phys. 1977, 38, 1061–1064. [Google Scholar] [CrossRef][Green Version]
- Grout, P.J.; March, N.H.; Tal, Y. Total energy of heavy positive ions especially near the weak ionization limit. J. Chem. Phys. 1983, 79, 331–333. [Google Scholar] [CrossRef]
- Dmitrieva, I.K.; Plindov, G.I. The improved Thomas‒Fermi model: Chemical and ionization potentials in atoms. J. Phys. 1984, 45, 85–95. [Google Scholar] [CrossRef][Green Version]
- Jablonski, A. Statistical model of an atom in electron scattering calculations. Phys. A 1985, 129, 591–600. [Google Scholar] [CrossRef]
- Englert, B.-G. Semiclassical Theory of Atoms; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]
- Shevelko, V.P.; Ulantsev, A.D. Static multipole polarizability of atoms and ions in the Thomas–Fermi model. J. Russ. Laser Res. 1994, 15, 529–545. [Google Scholar] [CrossRef]
- Esposito, P. Majorana solution of the Thomas–Fermi equation. Am. J. Phys. 2002, 70, 852–856. [Google Scholar] [CrossRef]
- Oulne, M. Variation and series approach to the Thomas–Fermi equation. Appl. Math. Comput. 2011, 218, 303–307. [Google Scholar] [CrossRef][Green Version]
- Boyd, J.P. Rational Chebyshev series for the Thomas–Fermi function: Endpoint singularities and spectral methods. J. Comput. Appl. Math. 2013, 244, 90–101. [Google Scholar] [CrossRef]
- Parand, K.; Ghaderi, A.; Yousefi, H.; Delkhosh, M. A new approach for solving nonlinear Thomas–Fermi equation based on fractional order of rational Bessel functions. Electron. J. Diff. Equ. 2016, 2016, 331. [Google Scholar]
- Parand, K.; Rabiei, K.; Delkhosh, M. An efficient numerical method for solving nonlinear Thomas–Fermi equation. Acta Univ. Sapientiae Math. 2018, 10, 134–151. [Google Scholar] [CrossRef]
- Zhang, X.; Boyd, J.P. Revisiting the Thomas–Fermi equation: Accelerating rational Chebyshev series through coordinate transformations. Appl. Num. Math. 2019, 135, 186–205. [Google Scholar] [CrossRef]
- Pikulin, S.V. The Thomas–Fermi problem and solutions of the Emden–Fowler equation. Comput. Math. Math. Phys. 2019, 59, 1292–1313. [Google Scholar] [CrossRef]
- Pikulin, S.V. Analytical–numerical method for calculating the Thomas–Fermi potential. Russ. J. Math. Phys. 2019, 26, 544–552. [Google Scholar] [CrossRef]
- Hairer, E.; Nørsett, S.P.; Wanner, G. Solving Ordinary Differential Equations I. Nonstiff Problems; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
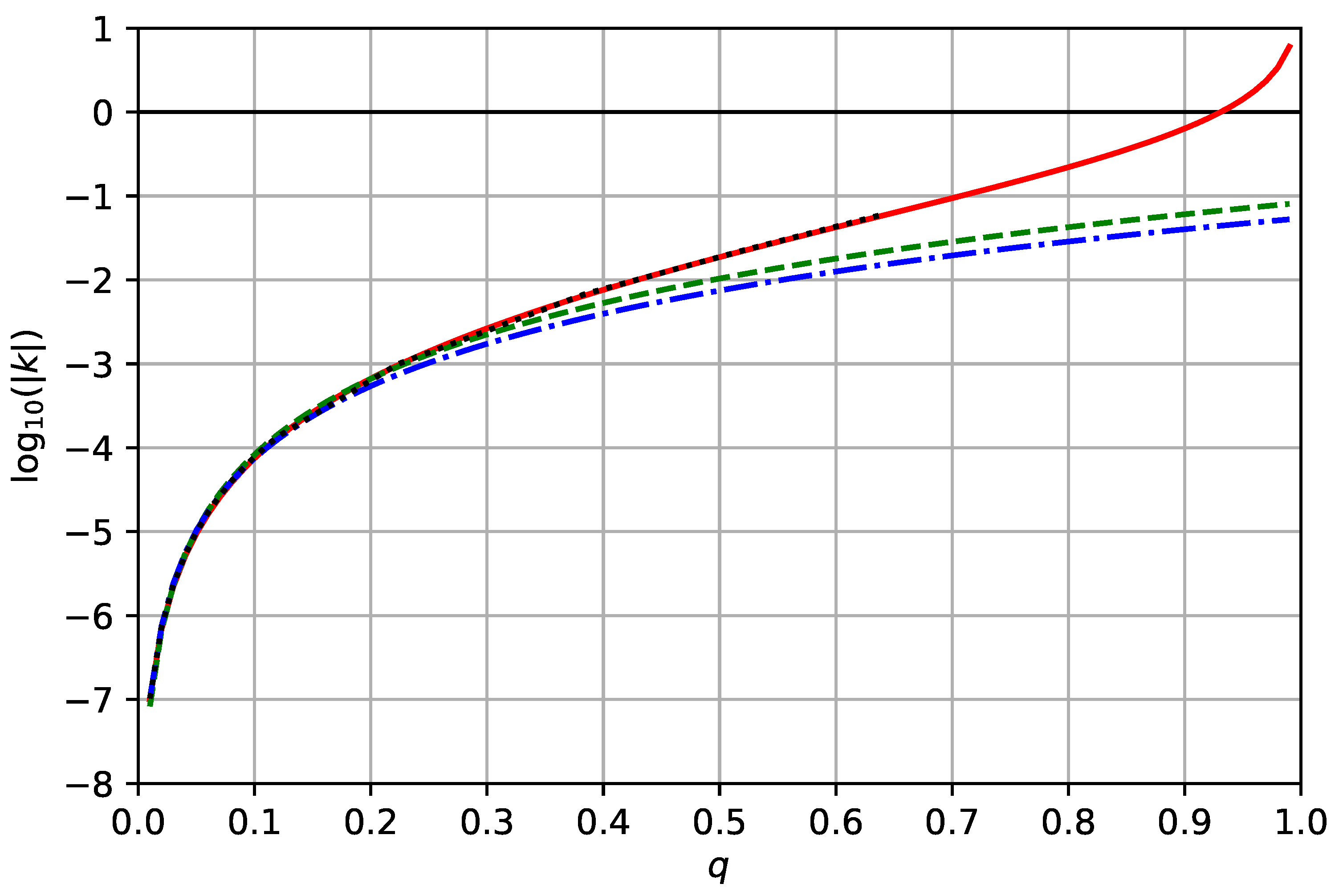

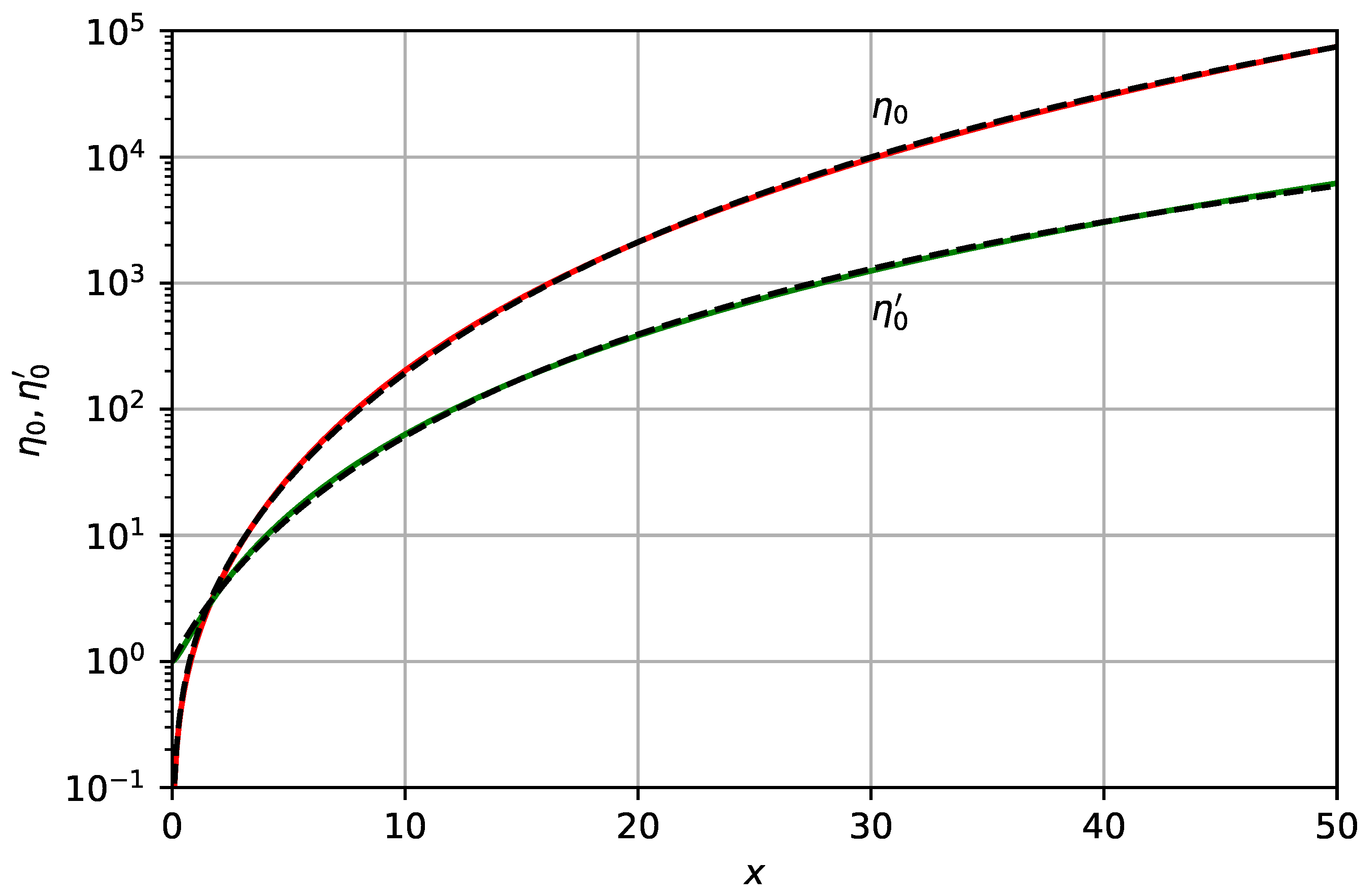
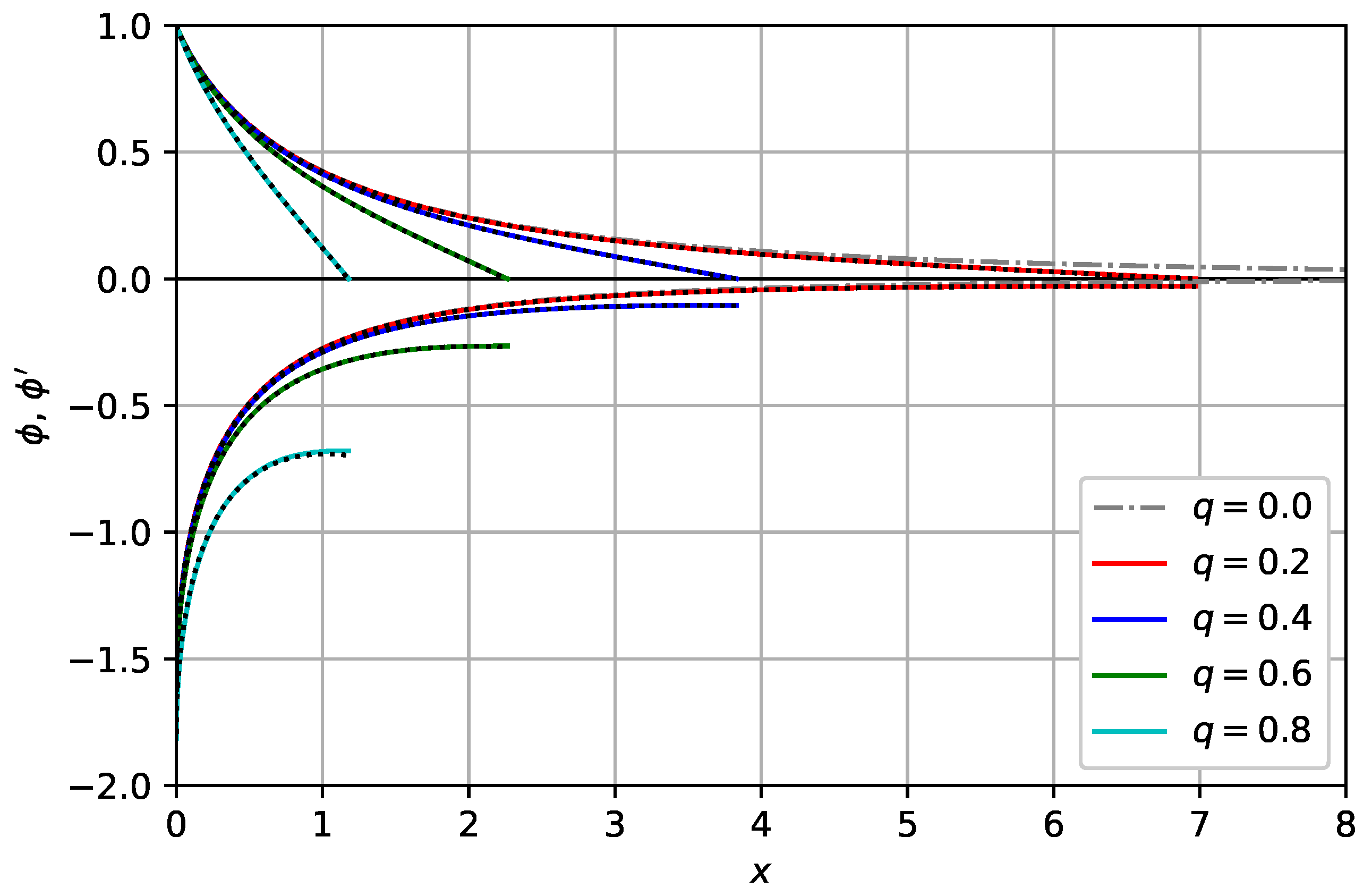
| q | q | q | ||||||
|---|---|---|---|---|---|---|---|---|
| 0.01 | 34.2658 | −6.999 | 0.34 | 4.5050 | −2.383 | 0.67 | 1.8529 | −1.131 |
| 0.02 | 25.1693 | −6.160 | 0.35 | 4.3823 | −2.336 | 0.68 | 1.7975 | −1.096 |
| 0.03 | 20.7772 | −5.660 | 0.36 | 4.2642 | −2.291 | 0.69 | 1.7429 | −1.061 |
| 0.04 | 18.0472 | −5.303 | 0.37 | 4.1502 | −2.247 | 0.70 | 1.6892 | −1.026 |
| 0.05 | 16.1026 | −5.021 | 0.38 | 4.0397 | −2.203 | 0.71 | 1.6361 | −0.991 |
| 0.06 | 14.6268 | −4.789 | 0.39 | 3.9335 | −2.161 | 0.72 | 1.5833 | −0.955 |
| 0.07 | 13.4520 | −4.591 | 0.40 | 3.8302 | −2.119 | 0.73 | 1.5314 | −0.919 |
| 0.08 | 12.4863 | −4.418 | 0.41 | 3.7307 | −2.077 | 0.74 | 1.4799 | −0.883 |
| 0.09 | 11.6718 | −4.264 | 0.42 | 3.6340 | −2.036 | 0.75 | 1.4287 | −0.847 |
| 0.10 | 10.9720 | −4.125 | 0.43 | 3.5400 | −1.996 | 0.76 | 1.3783 | −0.810 |
| 0.11 | 10.3613 | −3.998 | 0.44 | 3.4488 | −1.957 | 0.77 | 1.3280 | −0.773 |
| 0.12 | 9.8220 | −3.882 | 0.45 | 3.3606 | −1.918 | 0.78 | 1.2780 | −0.735 |
| 0.13 | 9.3410 | −3.774 | 0.46 | 3.2743 | −1.879 | 0.79 | 1.2286 | −0.696 |
| 0.14 | 8.9072 | −3.673 | 0.47 | 3.1905 | −1.841 | 0.80 | 1.1792 | −0.657 |
| 0.15 | 8.5136 | −3.579 | 0.48 | 3.1089 | −1.803 | 0.81 | 1.1300 | −0.617 |
| 0.16 | 8.1539 | −3.490 | 0.49 | 3.0293 | −1.766 | 0.82 | 1.0810 | −0.576 |
| 0.17 | 7.8243 | −3.405 | 0.50 | 2.9519 | −1.729 | 0.83 | 1.0318 | −0.534 |
| 0.18 | 7.5191 | −3.325 | 0.51 | 2.8761 | −1.692 | 0.84 | 0.9833 | −0.491 |
| 0.19 | 7.2366 | −3.249 | 0.52 | 2.8019 | −1.656 | 0.85 | 0.9343 | −0.447 |
| 0.20 | 6.9733 | −3.176 | 0.53 | 2.7298 | −1.620 | 0.86 | 0.8851 | −0.401 |
| 0.21 | 6.7273 | −3.107 | 0.54 | 2.6589 | −1.584 | 0.87 | 0.8359 | −0.353 |
| 0.22 | 6.4964 | −3.039 | 0.55 | 2.5895 | −1.548 | 0.88 | 0.7864 | −0.304 |
| 0.23 | 6.2795 | −2.975 | 0.56 | 2.5217 | −1.513 | 0.89 | 0.7363 | −0.252 |
| 0.24 | 6.0747 | −2.913 | 0.57 | 2.4555 | −1.478 | 0.90 | 0.6857 | −0.197 |
| 0.25 | 5.8811 | −2.852 | 0.58 | 2.3901 | −1.443 | 0.91 | 0.6344 | −0.138 |
| 0.26 | 5.6978 | −2.794 | 0.59 | 2.3262 | −1.408 | 0.92 | 0.5822 | −0.076 |
| 0.27 | 5.5234 | −2.738 | 0.60 | 2.2635 | −1.373 | 0.93 | 0.5285 | −0.007 |
| 0.28 | 5.3578 | −2.683 | 0.61 | 2.2020 | −1.338 | 0.94 | 0.4735 | 0.068 |
| 0.29 | 5.1998 | −2.630 | 0.62 | 2.1415 | −1.304 | 0.95 | 0.4163 | 0.152 |
| 0.30 | 5.0486 | −2.578 | 0.63 | 2.0820 | −1.269 | 0.96 | 0.3561 | 0.251 |
| 0.31 | 4.9040 | −2.527 | 0.64 | 2.0232 | −1.235 | 0.97 | 0.2918 | 0.371 |
| 0.32 | 4.7655 | −2.478 | 0.65 | 1.9656 | −1.200 | 0.98 | 0.2212 | 0.528 |
| 0.33 | 4.6328 | −2.430 | 0.66 | 1.9088 | −1.165 | 0.99 | 0.1383 | 0.778 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mavrin, A.A.; Demura, A.V. Approximate Solution of the Thomas–Fermi Equation for Free Positive Ions. Atoms 2021, 9, 87. https://doi.org/10.3390/atoms9040087
Mavrin AA, Demura AV. Approximate Solution of the Thomas–Fermi Equation for Free Positive Ions. Atoms. 2021; 9(4):87. https://doi.org/10.3390/atoms9040087
Chicago/Turabian StyleMavrin, Aleksey A., and Alexander V. Demura. 2021. "Approximate Solution of the Thomas–Fermi Equation for Free Positive Ions" Atoms 9, no. 4: 87. https://doi.org/10.3390/atoms9040087
APA StyleMavrin, A. A., & Demura, A. V. (2021). Approximate Solution of the Thomas–Fermi Equation for Free Positive Ions. Atoms, 9(4), 87. https://doi.org/10.3390/atoms9040087







