Photoionization and Electron-Ion Recombination of n = 1 to Very High n-Values of Hydrogenic Ions
Abstract
1. Introduction
1.1. Existing Values for Photoionization Cross Sections
1.2. Existing Values for Electron-Ion Recombination of Hydrogenic Ions
2. Theory of Photoionization and Electron-Ion Recombination of Hydrogenic Ions
2.1. Photoionization of Hydrogenic Ions
2.1.1. Recurrence Relations for the g Bound-Free Transition Integral
2.1.2. Ground State Photoionization Cross Sections of Hydrogen
2.2. Electron-Ion Recombination of Hydrogenic Ions
2.2.1. Top-Up Contribution from Very High-n Recombination
2.2.2. Recombination with Respect to Photoelectron Energy
2.3. Relation between Hydrogen and Hydrogenic Ions
2.4. Relativistic Fine Structure Splitting of and from LS Coupling
3. Programs for Photoionization and Electron-Ion Recombination, and the Data Files
4. Results and Discussions
4.1. Photoionization Cross Sections
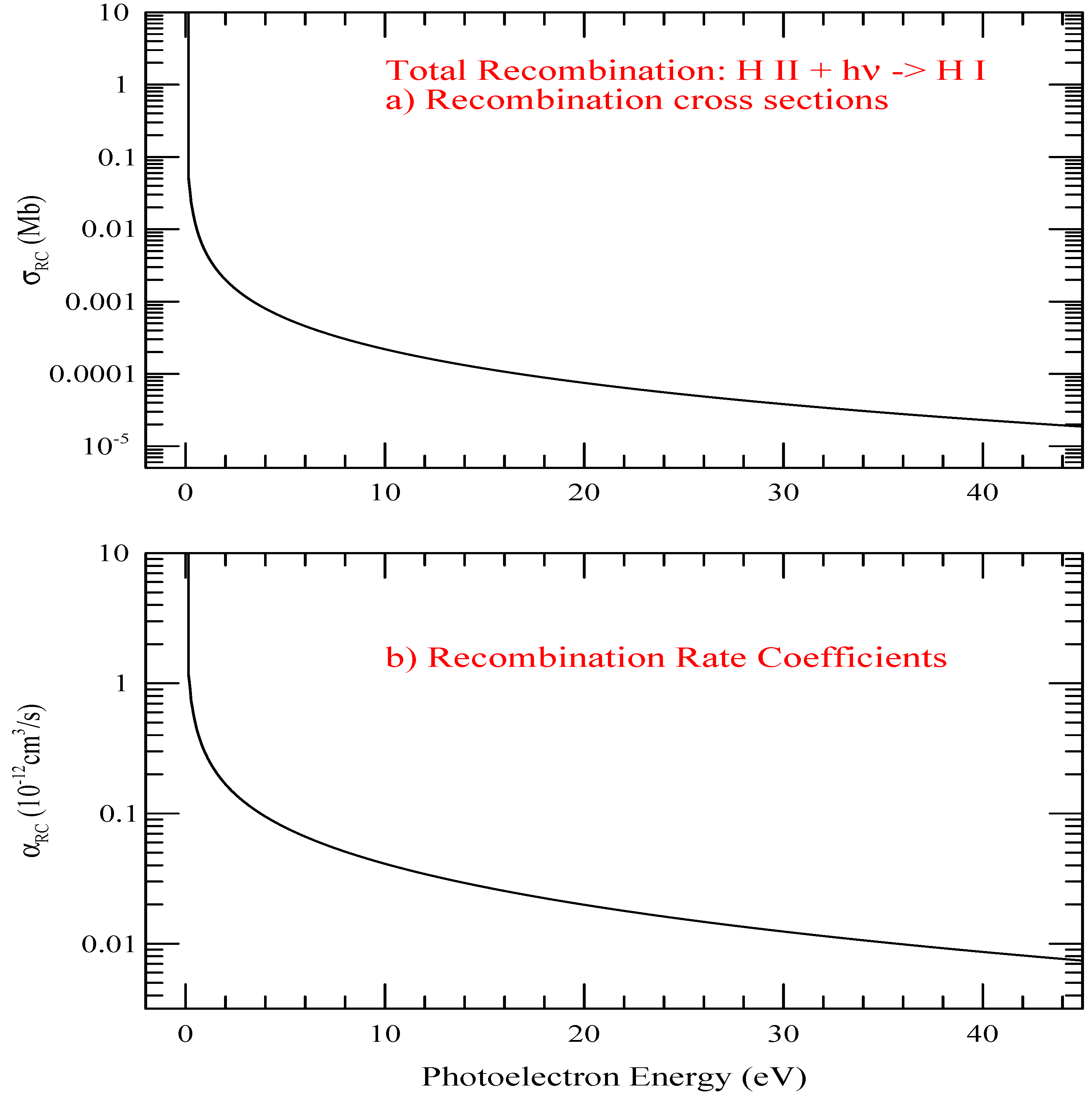
4.2. Electron-Ion Recombination
5. Conclusions
- Study of the two inverse processes of photoionization and electron-ion recombination are presented with a brief review of theory that treat them precisely and more accurately compared to all existing atomic structure codes that use other approximation, mainly distorted wave approximation.
- Detailed features of both the processes are illustrated. Although hydrogen and hydrogenic ions do not have any resonant features, accurate characteristic variation with energy and temperature are crucial for precise astrophysical spectroscopy and modeling.
- The present work provides atomic data files containing for all l-levels of n from 1 to 800, and of all values of n from 1 to 800. It also provides total with temperature, and total , and total with photoelectron energy. This is the first time that all these data with very high n have been made available for applications.
- Use of precise theory, numerical methods, high precision computers, as explained at the end of the sections of photoionization, and electron-ion recombination, can predict the accuracy of the present results is within 5% for most energy and temperature ranges.
- The work provides the FORTRAN program “hpxrrc.f” which can generate all these values. It also computes l-level specific . The program “hpxrrc.f” can also compute for any hydrogenic ion of charge Z using the data of hydrogen.
- Importance of relativistic effects and how to obtain fine structure components for photoionization and electron-ion from their values in the present LS coupling approximation have been discussed.
- All atomic data and the program will be available online at database, NORAD-Atomic-Data ([24], http://norad.astronomy.ohio-state.edu (accessed on 1 September 2007)).
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kramers, H.A. XCIII. On the theory of X-ray absorption and of the continuum X-ray spectrum. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1923, 46, 836–871. [Google Scholar] [CrossRef]
- Kotelnikov, I.A.; Milstein, A.I. Electron radiative recombination with ahydrogen-like ion. Phys. Scr. 2019, 94, 055403. [Google Scholar] [CrossRef]
- Rosmej, F.B.; Vainshtein, L.A.; Astapenko, V.A.; Lisitsa, V.S. Statistical and quantum photoionization cross sections in plasmas: Analytical approaches for any configurations including inner shells. Matter Radiat. Extremes 2020, 5, 064202. [Google Scholar] [CrossRef]
- Sugiura, Y.J. Sur le nombre des électrons de dispersion pour les spectres continus et pour les spectres de séries de l’hydrogène. Phys. Radium 1927, 8, 113. [Google Scholar] [CrossRef]
- Gordon, V.W. Zur Berechnung der Matrizen beun Wosserstoff atom. Ann. Phys. 1929, 2, 1031. [Google Scholar] [CrossRef]
- Gaunt, A. Continuous absorption. Philos. Trans. R. Soc. Lond. A 1930, 229, 163. [Google Scholar]
- Menzel, D.H.; Pekeris, C.L. Absorption Coefficients and Hydrogen Line Intensities. Mon. Not. R. Astron. Soc. 1935, 96, 77. [Google Scholar] [CrossRef]
- Bethe, J.A.; Salpeter, E.E. Quantum Mechanics of One- and Two-Electron Atoms; Academics: New York, NY, USA, 1957. [Google Scholar]
- Burgess, A. The Hydrogen Recombination Spectrum. Mon. Not. R. Astron. Soc. 1958, 118, 477. [Google Scholar] [CrossRef]
- Seaton, M.J. Radiative recombination of hydrogenic ions. Mon. Not. R. Astron. Soc. 1959, 119, 81. [Google Scholar] [CrossRef]
- Burgess, A.; Seaton, M.J. A general formula for the calculation of atomic photo-ionization cross sections. Mon. Not. R. Astron. Soc. 1960, 120, 121. [Google Scholar] [CrossRef]
- Burgess, A. Tables of hydrogenic photoionization cross sections and recombination coefficients. Mem. R. Astron. Soc. 1965, 69, 1. [Google Scholar]
- Omidvar, K.; Guimaraes, P.T. New tabulation of the bound-continuum optical oscillator strength in hydrogenic atoms. Astrophys. J. Suppl. 1990, 73, 555–602. [Google Scholar] [CrossRef]
- Green, L.C.; Rush, P.P.; Chandler, C.D. Atomic Transition Prota lities. Astrophys. J. Suppl. 1957, 3, 37. [Google Scholar] [CrossRef]
- Karzas, W.J.; Latter, R. Electron Radiative Transitions in a Coulomb Field. Astrophys. J. Suppl. 1961, 6, 167. [Google Scholar] [CrossRef]
- Ditchburn, R.W.; Öpik, U. Photoionization processes. In Atomic and Molecular Processes; Bates, D.R., Ed.; Academic: New York, NY, USA, 1962; p. 79. [Google Scholar]
- Palenius, H.P.; Kohl, J.L.; Parkinson, W.H. Absolute measurement of the photoionition cross section of atomic hydrogen with a shock tube for the extreme ultraviolet. Phys. Rev. A 1976, 13, 1805. [Google Scholar] [CrossRef]
- Norwood, K.; Ng, C.Y. Photoionization of hydrogen atoms near the ionization threshold. J. Chem. Phys. 1990, 93, 1480. [Google Scholar] [CrossRef]
- Nahar, S.N.; Manson, S.T. Photoionization of the 7d excited state of cesium. Phys. Rev. A 1989, 40, 6300. [Google Scholar] [CrossRef]
- Gu, M.F. The Flexible Atomic Code. AIP Conf. Proc. 2004, 730, 127. [Google Scholar]
- The Opacity Project Team. The Opacity Project; Institute of Physics Publishing: London, UK, 1995; Volume 1, 1996; Volume 2. [Google Scholar]
- TOPbase Website Address for Atomic Database. Available online: http://cdsweb.u-strasbg.fr/topbase/topbase.html (accessed on 1 June 1993).
- Norad. Available online: http://norad.astronomy.ohio-state.edu (accessed on 1 September 2007).
- Nahar, S.N. Database NORAD-Atomic-Data for atomic processes in plasma. Atoms 2020, 8, 68. [Google Scholar] [CrossRef]
- Flower, D.R.; Seaton, M.J. A program to calculate radiative recombination coefficients of hydrogenic ions. Comp. Phys. Commun. 1969, 1, 31. [Google Scholar] [CrossRef]
- Brocklehurst, M. Level populations of hydrogen in gaseous nebulae. Mon. Not. R. Astron. Soc. 1970, 148, 417–434. [Google Scholar] [CrossRef]
- Hummer, D.B.; Storey, P.J. Recombination-line intensities for hydrogenic ions—I. Case B calculations for H I and He II. Mon. Not. R. Astron. Soc. 1987, 224, 801–820. [Google Scholar] [CrossRef]
- Hummer, D.G. Total recombination and energy-loss coefficients for hydrogenic ions at low density for 10 ≤ Te/Z2 ≤ 107 K. Mon. Not. R. Astron. Soc. 1994, 268, 109–112. [Google Scholar] [CrossRef][Green Version]
- Ferland, G.J.; Peterson, B.M.; Horne, K.; Welsh, W.F.; Nahar, S. Anisotropic line emission and the geometry of the broad-line region in active galactic nuclei. Astrophys. J. 1992, 387, 95. [Google Scholar] [CrossRef]
- Nahar, S.N.; Pradhan, A.K. Electron-ion recombination in the close coupling approximation. Phys. Rev. Lett. 1992, 68, 1488. [Google Scholar] [CrossRef]
- Nahar, S.N.; Pradhan, A.K. Unified Treatment of Electron-Ion Recombination in the Close Coupling Approximation. Phys. Rev. A 1994, 49, 1816. [Google Scholar] [CrossRef]
- Nahar, S.N. Total electron-ion recombination for Fe III. Phys. Rev. A 1996, 53, 2417. [Google Scholar] [CrossRef]
- Nahar, S.N. Electron-ion recombination rate coefficients for Si I, Si II, S II, S III, C II, and C-like ions C I, N II, O III, F IV, Ne V, Na VI, Mg VII, Al VIII, Si IX, and S XI. Astrophys. J. Suppl. 1995, 101, 423, Erratum in 1996, 106, 213. [Google Scholar] [CrossRef]
- Burke, P.G. Photoionization of atomic systems. In Atomic Processes and Applications: In Honour of David R. Bates’ 60th Birthday; Burke, P.G., Moiseiwitsch, B.L., Eds.; North-Holland Publishing: Amsterdam, The Netherlands, 1976; pp. 199–248. [Google Scholar]
- Kogan, V.I.; Kukushkin, A.B.; Lisitsa, V.S. Kramers Electrodynamics and electron—Atomic radiative—Collisional processes. Phys. Rep. 1992, 213, 1–116. [Google Scholar] [CrossRef]
- Pradhan, A.K.; Nahar, S.N. Atomic Astrophysics and Spectroscopy; Cambridge University Press: New York, NY, USA, 2011. [Google Scholar]
- Biedenharn, L.C.; McHale, J.L.; Thaler, R.M. Quantum calculation of Coulomb excitation. I. Phys. Rev. 1955, 100, 376. [Google Scholar] [CrossRef]
- Alder, K.; Winther, A.K. On the Exact Evaluation of the Coulomb Excitation. Det Kongelge Dankske Videnskabernes Selskab. Mat.-Fys. Medd. 1955, 29, 19. [Google Scholar]
- Sommefeld, A. Atombau und Spektrallinien: Band I und II; Harri Deutsch: Frankfurt, Germany, 1978. [Google Scholar]
- Krainov, V.; Reiss, H.R.; Smirnov, B.M. Radiative Processes in Atomic Physics; John Wiley and Sons Inc.: New York, NY, USA, 1997. [Google Scholar]
- Samson, A.R. The measurement of the photoionization cross sections of the atomic gases. Adv. At. Mol. Phys. 1966, 2, 237. [Google Scholar]
- Zhang, H.L.; Nahar, S.N.; Pradhan, A.K. Close coupling R-matrix calculations for electron-ion recombination cross sections. J. Phys. B 1999, 32, 1459. [Google Scholar] [CrossRef]
- Pradhan, A.K.; Nahar, S.N.; Zhang, H.L. Unified electronic recombination of ne-like fe xvii: Implications for modeling X-ray plasmas. Astrophys. J. Lett. 2001, 549, L265. [Google Scholar] [CrossRef]
- Nahar, S.N. Atomic Data from the IRON Project VII. Radiative Dipole Transition Probabilities for Fe II. Astron. Astrophys. 1995, 293, 967. [Google Scholar]
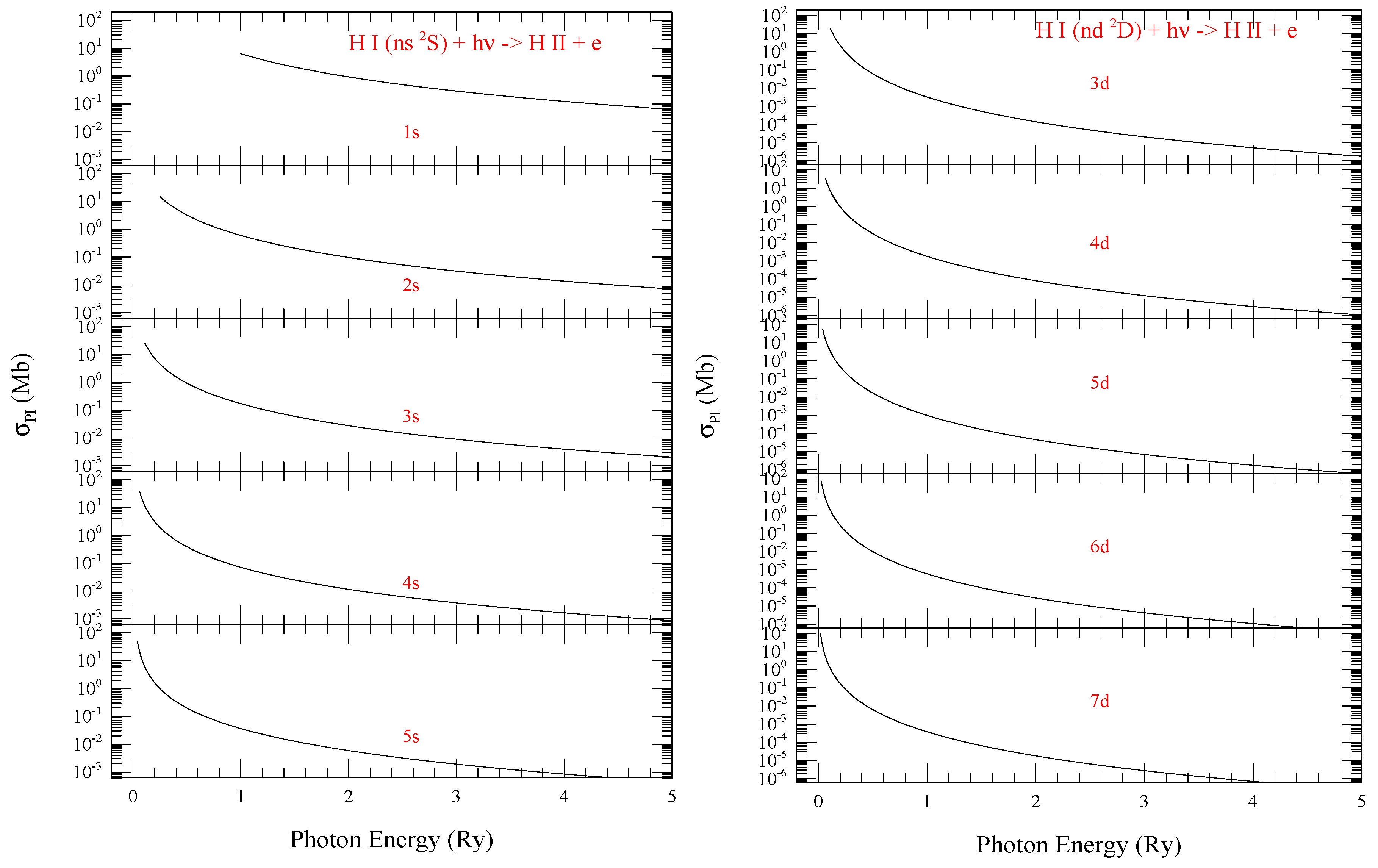
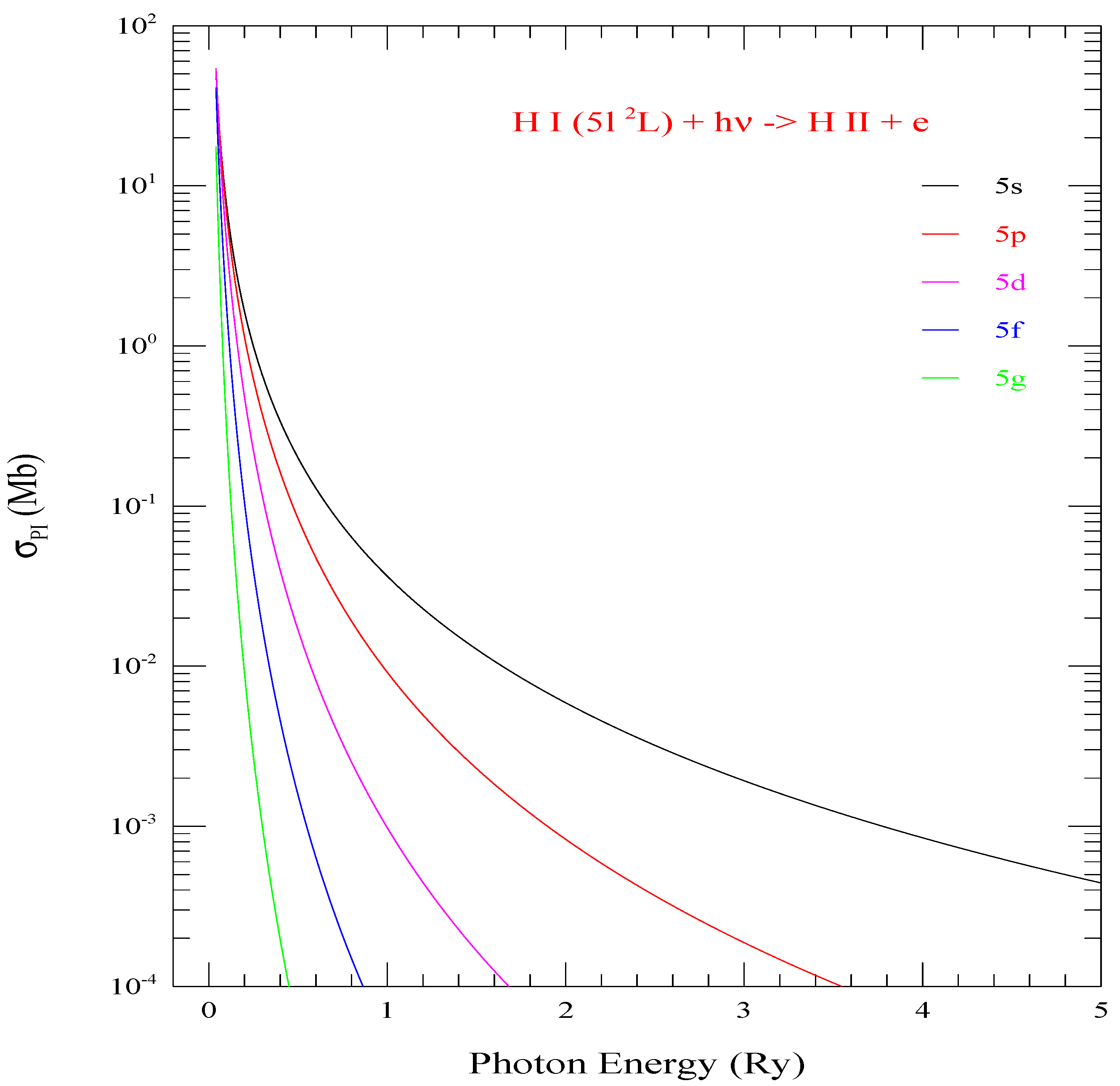
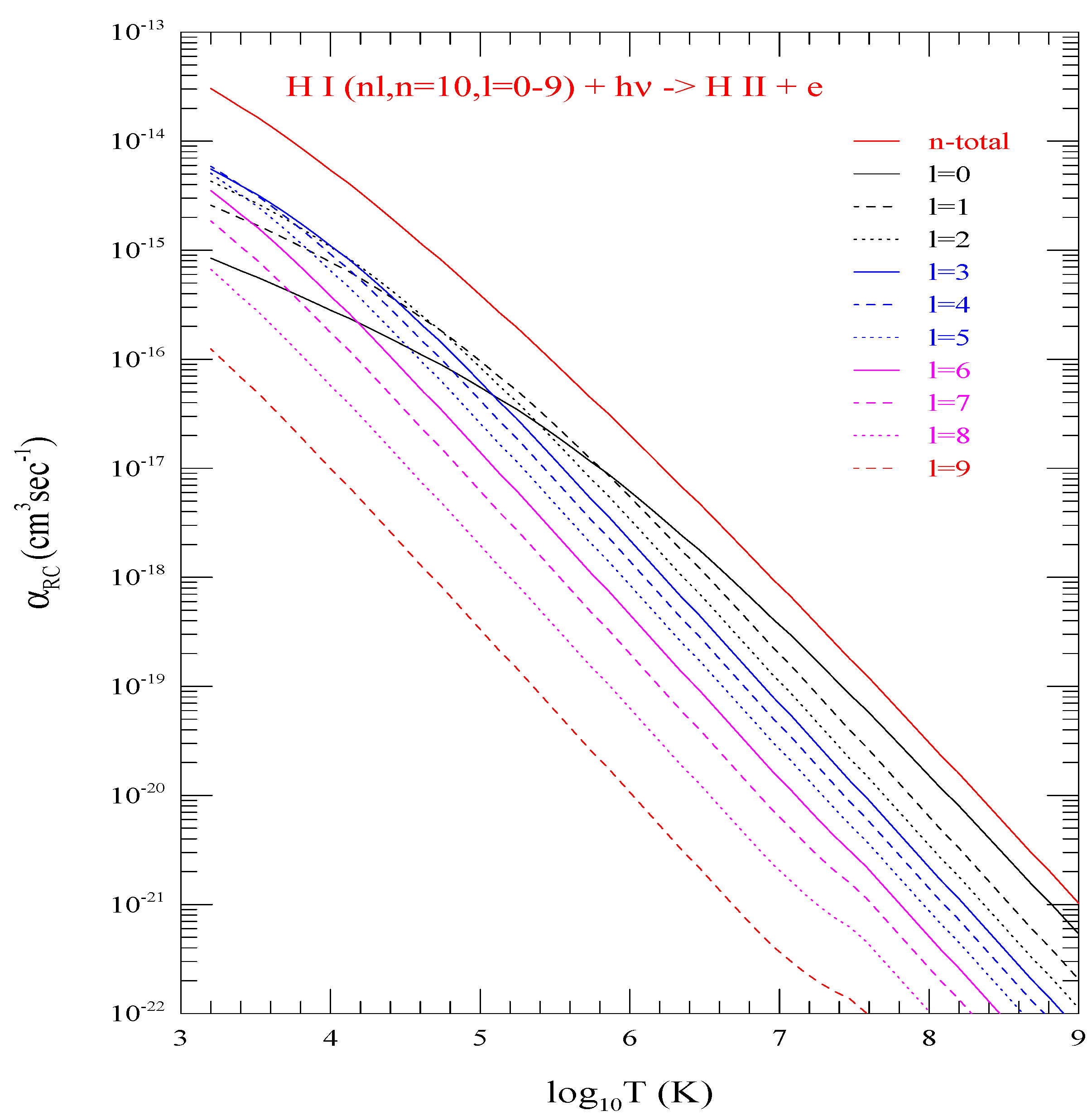

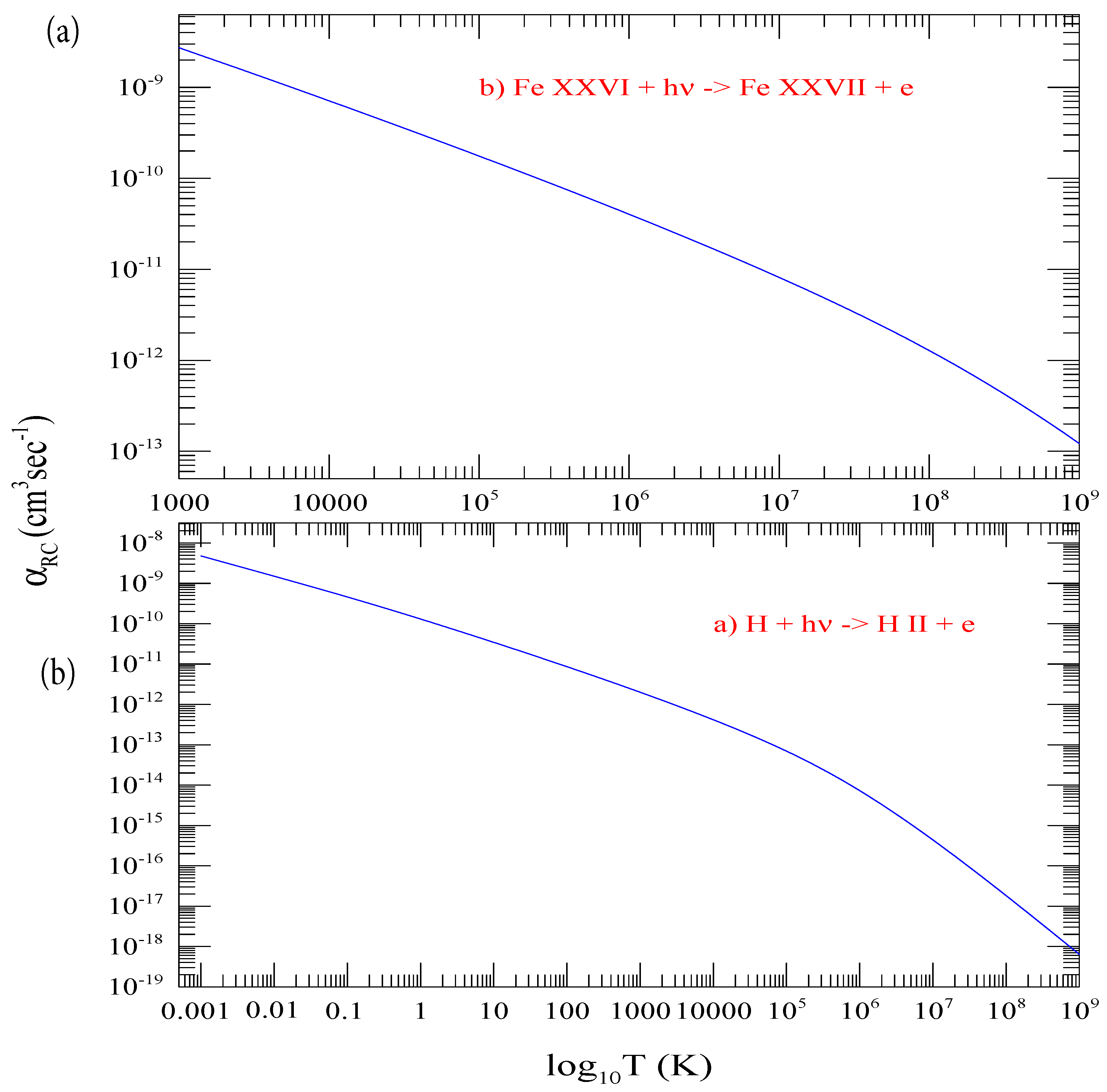
| logT | logT | logT | |||
|---|---|---|---|---|---|
| −3.00 | 4.779 | 0.73 | 4.974 | 4.60 | 1.479 |
| −2.67 | 3.254 | 0.75 | 4.842 | 4.70 | 1.232 |
| −2.33 | 2.214 | 0.77 | 4.714 | 4.80 | 1.022 |
| −2.00 | 1.504 | 0.79 | 4.588 | 4.90 | 8.447 |
| −1.67 | 1.020 | 0.81 | 4.466 | 5.00 | 6.949 |
| −1.33 | 6.877 | 0.83 | 4.347 | 5.10 | 5.691 |
| −1.00 | 4.606 | 0.85 | 4.231 | 5.20 | 4.637 |
| −0.67 | 3.057 | 0.87 | 4.118 | 5.30 | 3.755 |
| −0.33 | 2.009 | 0.89 | 4.008 | 5.40 | 3.027 |
| 0.00 | 1.308 | 0.91 | 3.901 | 5.50 | 2.424 |
| 0.01 | 1.291 | 1.00 | 3.452 | 5.60 | 1.930 |
| 0.03 | 1.258 | 1.10 | 3.012 | 5.70 | 1.528 |
| 0.05 | 1.226 | 1.20 | 2.627 | 5.80 | 1.202 |
| 0.07 | 1.194 | 1.30 | 2.290 | 5.90 | 9.396 |
| 0.09 | 1.163 | 1.40 | 1.995 | 6.00 | 7.303 |
| 0.11 | 1.133 | 1.50 | 1.737 | 6.10 | 5.643 |
| 0.13 | 1.104 | 1.60 | 1.511 | 6.20 | 4.333 |
| 0.15 | 1.075 | 1.70 | 1.314 | 6.30 | 3.311 |
| 0.17 | 1.048 | 1.80 | 1.142 | 6.40 | 2.516 |
| 0.19 | 1.020 | 1.90 | 9.922 | 6.50 | 1.902 |
| 0.21 | 9.939 | 2.00 | 8.613 | 6.60 | 1.431 |
| 0.23 | 9.680 | 2.10 | 7.471 | 6.70 | 1.071 |
| 0.25 | 9.429 | 2.20 | 6.476 | 6.80 | 7.989 |
| 0.27 | 9.183 | 2.30 | 5.609 | 6.90 | 5.932 |
| 0.29 | 8.944 | 2.40 | 4.855 | 7.00 | 4.389 |
| 0.31 | 8.711 | 2.50 | 4.199 | 7.10 | 3.232 |
| 0.33 | 8.484 | 2.60 | 3.628 | 7.20 | 2.374 |
| 0.35 | 8.262 | 2.70 | 3.132 | 7.30 | 1.739 |
| 0.37 | 8.046 | 2.80 | 2.702 | 7.40 | 1.270 |
| 0.39 | 7.835 | 2.90 | 2.328 | 7.50 | 9.251 |
| 0.41 | 7.630 | 3.00 | 2.004 | 7.60 | 6.718 |
| 0.43 | 7.430 | 3.10 | 1.723 | 7.70 | 4.866 |
| 0.45 | 7.235 | 3.20 | 1.480 | 7.80 | 3.518 |
| 0.47 | 7.045 | 3.30 | 1.269 | 7.90 | 2.538 |
| 0.49 | 6.860 | 3.40 | 1.087 | 8.00 | 1.828 |
| 0.51 | 6.680 | 3.50 | 9.304 | 8.10 | 1.315 |
| 0.53 | 6.504 | 3.60 | 7.949 | 8.20 | 9.440 |
| 0.55 | 6.332 | 3.70 | 6.781 | 8.30 | 6.769 |
| 0.57 | 6.165 | 3.80 | 5.774 | 8.40 | 4.848 |
| 0.59 | 6.003 | 3.90 | 4.909 | 8.50 | 3.468 |
| 0.61 | 5.844 | 4.00 | 4.165 | 8.60 | 2.478 |
| 0.61 | 5.844 | 4.00 | 4.165 | 8.60 | 2.478 |
| 0.63 | 5.690 | 4.10 | 3.527 | 8.70 | 1.769 |
| 0.65 | 5.539 | 4.20 | 2.980 | 8.80 | 1.262 |
| 0.67 | 5.392 | 4.30 | 2.511 | 8.90 | 8.997 |
| 0.69 | 5.249 | 4.40 | 2.111 | 9.00 | 6.409 |
| 0.71 | 5.110 | 4.50 | 1.770 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nahar, S.N. Photoionization and Electron-Ion Recombination of n = 1 to Very High n-Values of Hydrogenic Ions. Atoms 2021, 9, 73. https://doi.org/10.3390/atoms9040073
Nahar SN. Photoionization and Electron-Ion Recombination of n = 1 to Very High n-Values of Hydrogenic Ions. Atoms. 2021; 9(4):73. https://doi.org/10.3390/atoms9040073
Chicago/Turabian StyleNahar, Sultana N. 2021. "Photoionization and Electron-Ion Recombination of n = 1 to Very High n-Values of Hydrogenic Ions" Atoms 9, no. 4: 73. https://doi.org/10.3390/atoms9040073
APA StyleNahar, S. N. (2021). Photoionization and Electron-Ion Recombination of n = 1 to Very High n-Values of Hydrogenic Ions. Atoms, 9(4), 73. https://doi.org/10.3390/atoms9040073






