Positron Processes in the Sun
Abstract
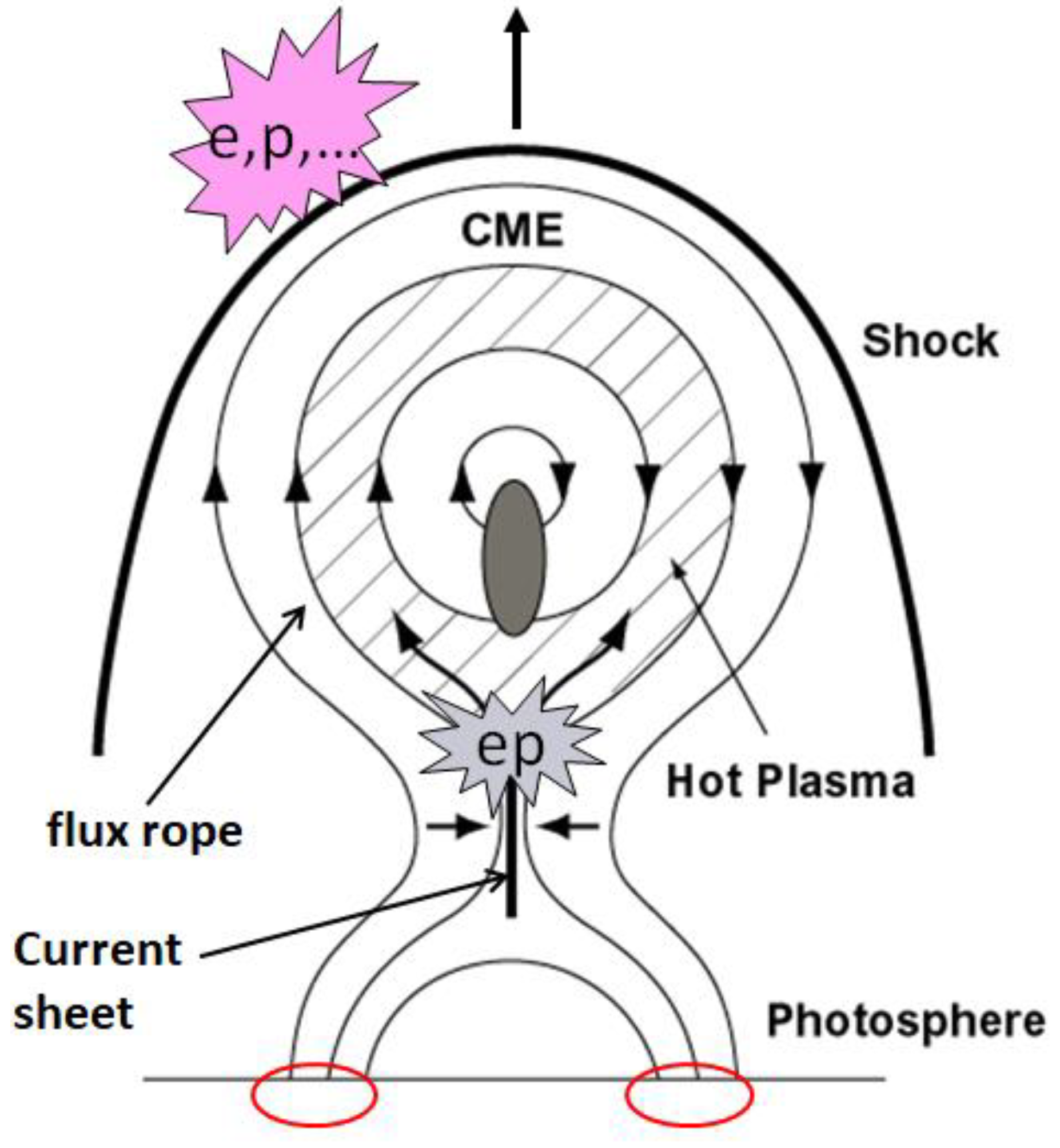
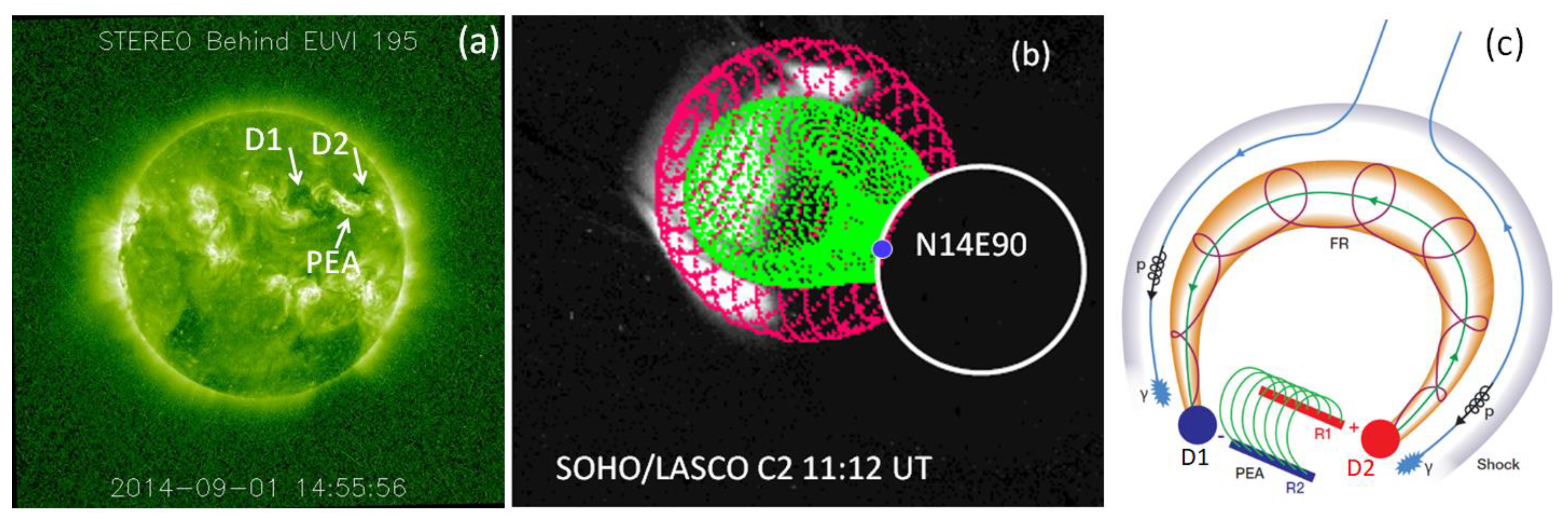
1. Introduction
2. Mechanisms of Positron Production
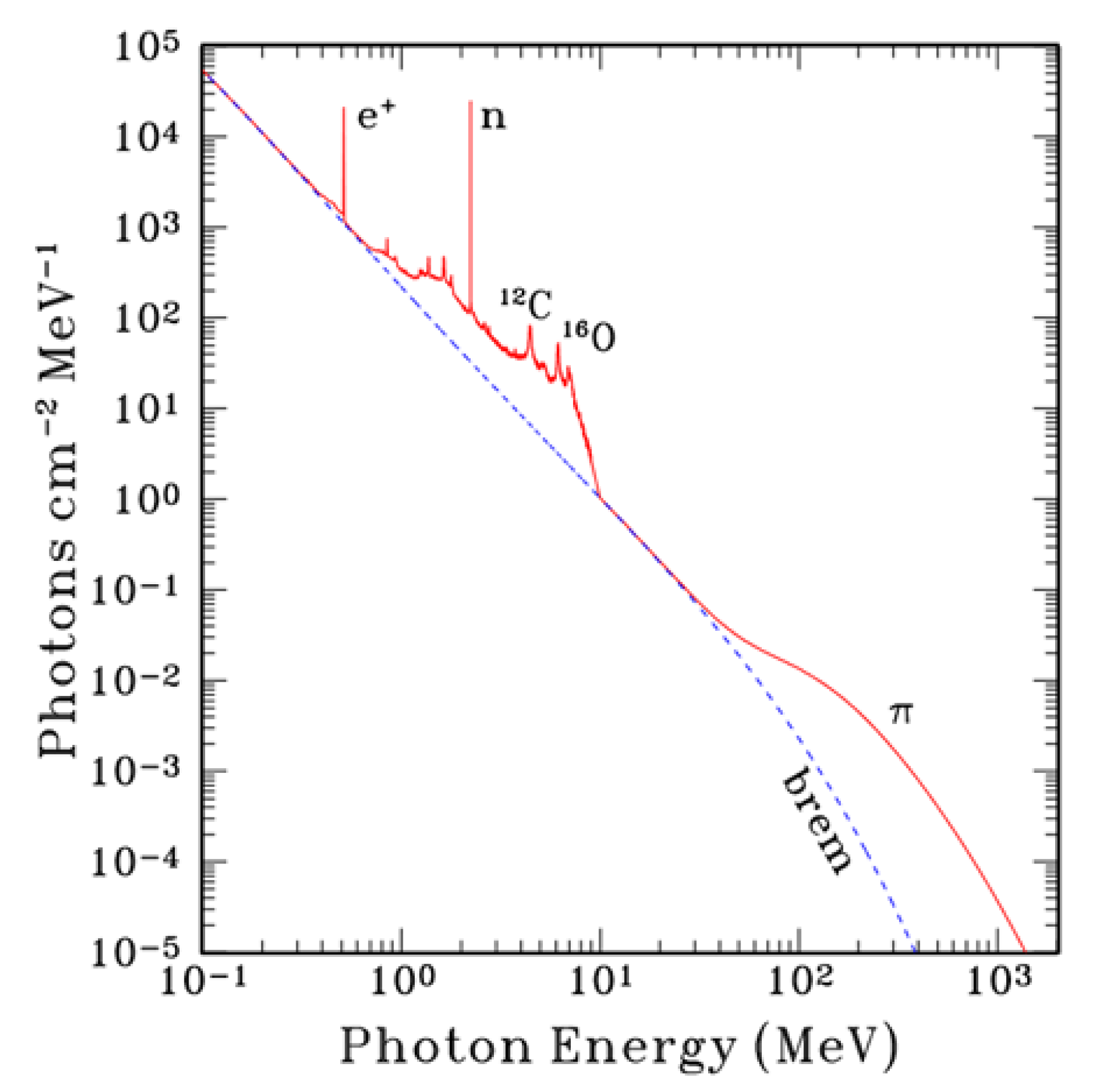
3. Gamma-Rays Due to Positrons
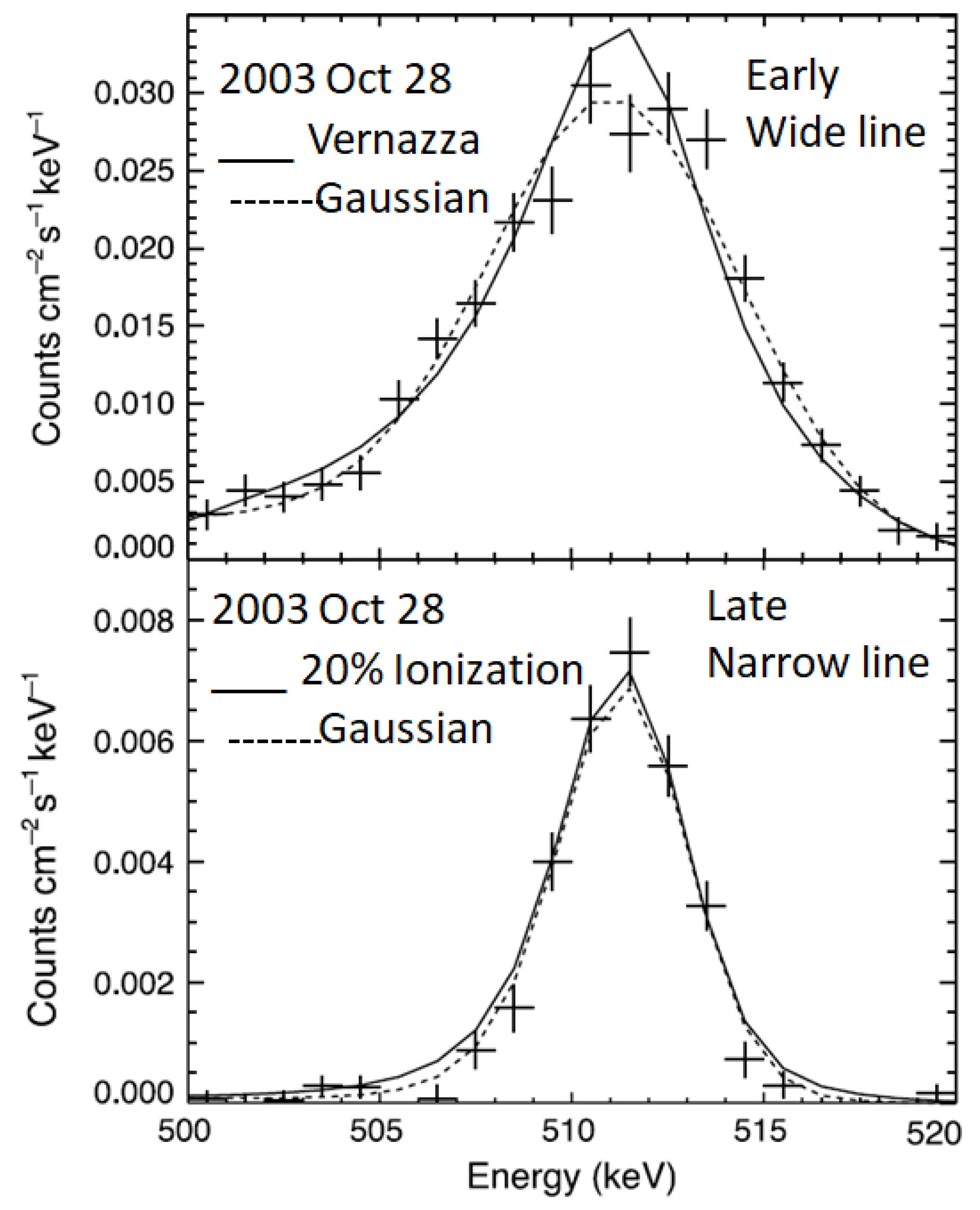
3.1. The 0.511 MeV Gamma-Ray Line
3.2. Pion Continuum
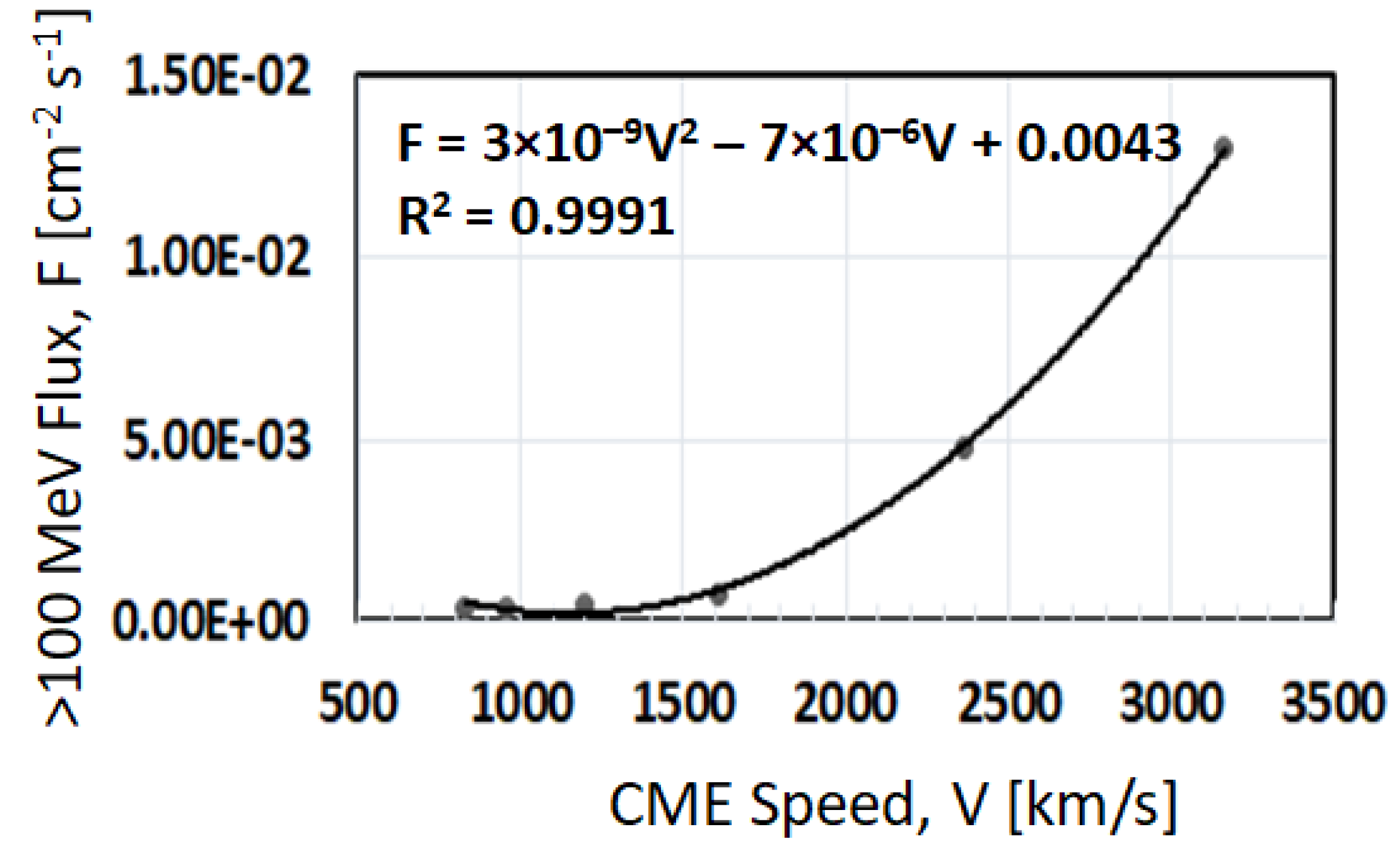
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Anderson, C.D. The Positive Electron. Phys. Rev. 1933, 43, 491–494. [Google Scholar] [CrossRef]
- Mills, A.P. 2-Positron and Positronium Sources. In Methods in Experimental Physics; Dunning, F.B., Hulet, R.G., Eds.; Atomic, Molecular, and Optical Physics: Charged Particles; Academic Press: Cambridge, MA, USA, 1995; Volume 29, pp. 39–68. [Google Scholar] [CrossRef]
- Guessoum, N.; Jean, P.; Gillard, W. The Lives and Deaths of Positrons in the Interstellar Medium. Astron. Astrophys. 2005, 436, 171–185. [Google Scholar] [CrossRef]
- Knödlseder, J.; Jean, P.; Lonjou, V.; Weidenspointner, G.; Guessoum, N.; Gillard, W.; Skinner, G.; von Ballmoos, P.; Vedrenne, G.; Roques, J.-P.; et al. The All-Sky Distribution of 511 KeV Electron-Positron Annihilation Emission. Astron. Astrophys. 2005, 441, 513–532. [Google Scholar] [CrossRef]
- Murphy, R.J.; Share, G.H.; Skibo, J.G.; Kozlovsky, B. The Physics of Positron Annihilation in the Solar Atmosphere. Astrophys. J. Suppl. Ser. 2005, 161, 495–519. [Google Scholar] [CrossRef]
- Gopalswamy, N. Properties of Interplanetary Coronal Mass Ejections. Space Sci. Rev. 2006, 124, 145–168. [Google Scholar] [CrossRef]
- Aschwanden, M.J. Particle Acceleration and Kinematics in Solar Flares—A Synthesis of Recent Observations and Theoretical Concepts (Invited Review). Space Sci. Rev. 2002, 101, 1–227. [Google Scholar] [CrossRef]
- Wild, J.P.; Smerd, S.F.; Weiss, A.A. Solar Bursts. Annu. Rev. Astron. Astrophys. 1963, 1, 291. [Google Scholar] [CrossRef]
- Forbush, S.E. Three Unusual Cosmic-Ray Increases Possibly Due to Charged Particles from the Sun. Phys. Rev. 1946, 70, 771–772. [Google Scholar] [CrossRef]
- Morrison, P. Solar Origin of Cosmic Ray Time Variations. Il Nuovo Cimento 1958, 7, 858–865. [Google Scholar] [CrossRef]
- Elliot, H. The Nature of Solar Flares. Planet. Space Sci. 1964, 12, 657–660. [Google Scholar] [CrossRef]
- Lingenfelter, R.E.; Ramaty, R. High Energy Nuclear Reactions in Solar Flares; Univrsity of California, Los Angeles: Los Angeles, CA, USA, 1967; pp. 99–124. [Google Scholar]
- Chupp, E.L.; Forrest, D.J.; Higbie, P.R.; Suri, A.N.; Tsai, C.; Dunphy, P.P. Solar Gamma Ray Lines Observed during the Solar Activity of 2 August to 11 August 1972. Nature 1973, 241, 333–335. [Google Scholar] [CrossRef]
- Kozlovsky, B.; Lingenfelter, R.E.; Ramaty, R. Positrons from Accelerated Particle Interactions. Astrophys. J. 1987, 316, 801–818. [Google Scholar] [CrossRef]
- Kozlovsky, B.; Murphy, R.J.; Share, G.H. Positron-Emitter Production in Solar Flares from 3He Reactions. Astrophys. J. 2004, 604, 892–899. [Google Scholar] [CrossRef]
- Ramaty, R.; Murphy, R.J. Nuclear Processes and Accelerated Particles in Solar Flares. Space Sci. Rev. 1987, 45, 213–268. [Google Scholar] [CrossRef]
- Murphy, R.J.; Dermer, C.D.; Ramaty, R. High-Energy Processes in Solar Flares. Astrophys. J. Suppl. Ser. 1987, 63, 28. [Google Scholar] [CrossRef]
- Benz, A.O. Flare Observations. Living Rev. Sol. Phys. 2016, 14, 2. [Google Scholar] [CrossRef]
- Ramaty, R.; Mandzhavidze, N. Solar Flares: Gamma Rays. In Encyclopedia of Astronomy and Astrophysics; Murdin, P., Ed.; Article id. 2292; Institute of Physics Publishing: Bristol, UK, 2001; pp. 1–4. Available online: http://eaa.crcpress.com/default.asp (accessed on 22 April 2020).
- Share, G.H.; Murphy, R.J.; Smith, D.M.; Schwartz, R.A.; Lin, R.P. RHESSI e+-e- Annihilation Radiation Observations: Implications for Conditions in the Flaring Solar Chromosphere. Astrophys. J. Lett. 2004, 615, L169–L172. [Google Scholar] [CrossRef]
- Ley, R. Atomic Physics of Positronium with Intense Slow Positron Beams. Appl. Surf. Sci. 2002, 194, 301–306. [Google Scholar] [CrossRef]
- Vernazza, J.E.; Avrett, E.H.; Loeser, R. Structure of the Solar Chromosphere. III - Models of the EUV Brightness Components of the Quiet-Sun. Astrophys. J. Suppl. Ser. 1981, 45, 635–725. [Google Scholar] [CrossRef]
- Song, P. A Model of the Solar Chromosphere: Structure and Internal Circulation. Astrophys. J. 2017, 846, 92. [Google Scholar] [CrossRef]
- Avrett, E.H.; Loeser, R. Models of the Solar Chromosphere and Transition Region from SUMER and HRTS Observations: Formation of the Extreme-Ultraviolet Spectrum of Hydrogen, Carbon, and Oxygen. Astrophys. J. Suppl. Ser. 2008, 175, 229–276. [Google Scholar] [CrossRef]
- Forrest, D.J.; Vestrand, W.T.; Chupp, E.L.; Rieger, E.; Cooper, J.F.; Share, G.H. Neutral Pion Production in Solar Flares. ICRC 1985, 4, 146–149. [Google Scholar]
- Ellison, D.C.; Ramaty, R. Shock Acceleration of Electrons and Ions in Solar Flares. Astrophys. J. 1985, 298, 400–408. [Google Scholar] [CrossRef]
- Chupp, E.L.; Ryan, J.M. High Energy Neutron and Pion-Decay Gamma-Ray Emissions from Solar Flares. Res. Astron. Astrophys. 2009, 9, 11–40. [Google Scholar] [CrossRef]
- Kuznetsov, S.N.; Kurt, V.G.; Yushkov, B.Y.; Myagkova, I.N.; Galkin, V.I.; Kudela, K. Protons Acceleration in Solar Flares: The Results of the Analysis of Gamma-Emission and Neutrons Recorded by the SONG Instrument Onboard the CORONAS-F Satellite. Astrophys. Space Sci. Lib. 2014, 400, 301. [Google Scholar] [CrossRef]
- Akimov, V.V.; Afanassyey, V.G.; Belaousov, A.S.; Blokhintsev, I.D.; Kalinkin, L.F.; Leikov, N.G.; Nesterov, V.E.; Volsenskaya, V.A.; Galper, A.M.; Chesnokov, V.J.; et al. Observation of High Energy Gamma-Rays from the Sun with the GAMMA-1 Telescope (E > 30 MeV). ICRC 1991, 3, 73. [Google Scholar]
- Kanbach, G.; Bertsch, D.L.; Fichtel, C.E.; Hartman, R.C.; Hunter, S.D.; Kniffen, D.A.; Kwok, P.W.; Lin, Y.C.; Mattox, J.R.; Mayer-Hasselwander, H.A. Detection of a Long-Duration Solar Gamma-Ray Flare on 11 June 1991 with EGRET on COMPTON-GRO. Astron. Astrophys. Suppl. Ser. 1993, 97, 349–353. [Google Scholar]
- Atwood, W.B.; Abdo, A.A.; Ackermann, M.; Althouse, W.; Anderson, B.; Axelsson, M.; Baldini, L.; Ballet, J.; Band, D.L.; Barbiellini, G.; et al. The Large Area Telescope on the Fermi Gamma-Ray Space Telescope Mission. Astrophys. J. 2009, 697, 1071–1102. [Google Scholar] [CrossRef]
- Share, G.H.; Murphy, R.J.; White, S.M.; Tolbert, A.K.; Dennis, B.R.; Schwartz, R.A.; Smart, D.F.; Shea, M.A. Characteristics of Late-Phase $\greater$100 MeV Gamma-Ray Emission in Solar Eruptive Events. Astrophys. J. 2018, 869, 182. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Mäkelä, P.; Yashiro, S.; Lara, A.; Akiyama, S.; Xie, H. On the Shock Source of Sustained Gamma-Ray Emission from the Sun. J. Phys. Conf. Ser. 2019, 1332, 012004. [Google Scholar] [CrossRef]
- Ryan, J.M. Long-Duration Solar Gamma-Ray Flares. Space Sci. Rev. 2000, 93, 581–610. [Google Scholar] [CrossRef]
- De Nolfo, G.A.; Bruno, A.; Ryan, J.M.; Dalla, S.; Giacalone, J.; Richardson, I.G.; Christian, E.R.; Stochaj, S.J.; Bazilevskaya, G.A.; Boezio, M.; et al. Comparing Long-Duration Gamma-Ray Flares and High-Energy Solar Energetic Particles. Astrophys. J. 2019, 879, 90. [Google Scholar] [CrossRef]
- Plotnikov, I.; Rouillard, A.P.; Share, G.H. The Magnetic Connectivity of Coronal Shocks from Behind-the-Limb Flares to the Visible Solar Surface during γ-Ray Events. Astron. Astrophys. 2017, 608, A43. [Google Scholar] [CrossRef]
- Ryan, J.M.; Lee, M.A. On the Transport and Acceleration of Solar Flare Particles in a Coronal Loop. Astrophys. J. 1991, 368, 316. [Google Scholar] [CrossRef]
- Cliver, E.W.; Kahler, S.W.; Vestrand, W.T. On the Origin of Gamma-Ray Emission from the Behind-the-Limb Flare on 29 September 1989. ICRC 1993, 3, 91. [Google Scholar]
- Pesce-Rollins, M.; Omodei, N.; Petrosian, V.; Liu, W.; Rubio da Costa, F.; Allafort, A.; Fermi-LAT Collaboration. Fermi Large Area Telescope Observations of High-Energy Gamma-Ray Emission from behind-the-Limb Solar Flares. ICRC 2015, 34, 128. [Google Scholar]
- Gopalswamy, N.; Mäkelä, P.; Yashiro, S.; Akiyama, S.; Xie, H.; Thakur, N. Source of Energetic Protons in the 2014 September 1 Sustained Gamma-Ray Emission Event. Sol. Phys. 2020, 295, 18. [Google Scholar] [CrossRef]
- Jin, M.; Petrosian, V.; Liu, W.; Nitta, N.V.; Omodei, N.; Rubio da Costa, F.; Effenberger, F.; Li, G.; Pesce-Rollins, M.; Allafort, A.; et al. Probing the Puzzle of Behind-the-Limb γ-Ray Flares: Data-Driven Simulations of Magnetic Connectivity and CME-Driven Shock Evolution. Astrophys. J. 2018, 867, 122. [Google Scholar] [CrossRef]
- Minasyants, G.; Minasyants, T.; Tomozov, V. Features of Development of Sustained Fluxes of High-Energy Gamma-Ray Emission at Different Stages of Solar Flares. Sol.-Terr. Phys. 2019, 5, 10–17. [Google Scholar] [CrossRef]
- Reames, D.V. Solar Energetic-Particle Release Times in Historic Ground-Level Events. Astrophys. J. 2009, 706, 844–850. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Xie, H.; Yashiro, S.; Akiyama, S.; Mäkelä, P.; Usoskin, I.G. Properties of Ground Level Enhancement Events and the Associated Solar Eruptions during Solar Cycle 23. Space Sci. Rev. 2012, 171, 23–60. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Xie, H.; Akiyama, S.; Yashiro, S.; Usoskin, I.G.; Davila, J.M. The First Ground Level Enhancement Event of Solar Cycle 24: Direct Observation of Shock Formation and Particle Release Heights. Astrophys. J. Lett. 2013, 765, L30. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Xie, H.; Mäkelä, P.; Yashiro, S.; Akiyama, S.; Uddin, W.; Srivastava, A.K.; Joshi, N.C.; Chandra, R.; Manoharan, P.K.; et al. Height of Shock Formation in the Solar Corona Inferred from Observations of Type II Radio Bursts and Coronal Mass Ejections. Adv. Space Res. 2013, 51, 1981–1989. [Google Scholar] [CrossRef]
- Omodei, N.; Pesce-Rollins, M.; Longo, F.; Allafort, A.; Krucker, S. Fermi-LAT Observations of the 2017 September 10 Solar Flare. Astrophys. J. Lett. 2018, 865, L7. [Google Scholar] [CrossRef]

| Interaction | Target Nuclei | Radioactive Nuclei |
|---|---|---|
| p - carbon | 12C | 11C, 12N, 10C, 13N |
| p - carbon | 13C | 13N |
| α - carbon | 12C | 11C, 15O |
| p - nitrogen | 14N | 11C, 13N, 14O |
| p - nitrogen | 15N | 15O |
| α - nitrogen | 14N | 17F, 13N, 11C |
| p - oxygen | 16O | 11C, 13N, 15O, 16O* |
| p - oxygen | 18O | 18F |
| α - oxygen | 16O | 19Ne, 18F, 15O, 13N, 11C, 16O* |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gopalswamy, N. Positron Processes in the Sun. Atoms 2020, 8, 14. https://doi.org/10.3390/atoms8020014
Gopalswamy N. Positron Processes in the Sun. Atoms. 2020; 8(2):14. https://doi.org/10.3390/atoms8020014
Chicago/Turabian StyleGopalswamy, Nat. 2020. "Positron Processes in the Sun" Atoms 8, no. 2: 14. https://doi.org/10.3390/atoms8020014
APA StyleGopalswamy, N. (2020). Positron Processes in the Sun. Atoms, 8(2), 14. https://doi.org/10.3390/atoms8020014





