The Screening Characteristics of the Dense Astrophysical Plasmas: The Three-Component Systems
Abstract
:1. Introduction
- The presence of an immobile probe particle, which represents one kind of charged particle in the real system (plasma, or electrolyte).
- The treatment of the considered components, which contain free charged particles of different kinds as ideal gases in states of thermodynamical equilibrium, without the assumption that all temperatures are equal.
- The treatment of the existing total electrostatic field in the considered system as an external field with respect to the considered ideal gas.
- Finally, among the properties of this model is usage, as its relevant mathematical apparatus, of equations, which describe the mean local electrostatic field and the conditions of conservation of thermodynamical equilibrium for the considered components. As in the previous papers, this model is treated here as the basic model.
2. Theory Assumptions
2.1. The Initial System and Basic Characteristic
2.2. The System Properties and Conditions
2.3. The System Equations
2.4. The Additional Conditions
3. Ion Cases: Complete Expressions
3.1. The Ion Densities
3.2. The Electron Densities
4. Complete Expressions for the Electron and Ion Densities: The Case (e)
5. Determination of the Parameters
6. Results and Discussions
6.1. The Properties of the Obtained Solutions
6.2. Interpretation of the Obtained Results
6.3. The Possible Ion–Ion Probe Systems
7. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Mihajlov, A.; Vitel, Y.; Ignjatović, L.M. The new screening characteristics of strongly non-ideal and dusty plasmas. Part 1: Single-component systems. High Temp. 2008, 46, 737–745. [Google Scholar] [CrossRef]
- Mihajlov, A.; Vitel, Y.; Ignjatović, L.M. The new screening characteristics of strongly non-ideal and dusty plasmas. Part 2: Two-component systems. High Temp. 2009, 47, 1–12. [Google Scholar] [CrossRef]
- Mihajlov, A.; Vitel, Y.; Ignjatović, L.M. The new screening characteristics of strongly non-ideal and dusty plasmas. Part 3: Properties and applications. High Temp. 2009, 47, 147–157. [Google Scholar] [CrossRef]
- Demura, A. Physical models of plasma microfield. Int. J. Spectrosc. 2009, 2010, 42. [Google Scholar] [CrossRef]
- Calisti, A.; Ferri, S.; Mossé, C.; Talin, B.; Lisitsa, V.; Bureyeva, L.; Gigosos, M.A.; González, M.A.; del Río Gaztelurrutia, T.; Dufty, J.W. Slow and fast micro-field components in warm and dense hydrogen plasmas. arXiv, 2007; arXiv:0710.2091. [Google Scholar]
- Mihajlov, A.A.; Sakan, N.M.; Srećković, V.A.; Vitel, Y. Modeling of continuous absorption of electromagnetic radiation in dense partially ionized plasmas. J. Phys. A Math. Gen. 2011, 44, 095502. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Sakan, N.M.; Srećković, V.A.; Vitel, Y. Modeling of the Continuous Absorption of Electromagnetic Radiation in Dense Hydrogen Plasma. Balt. Astron. 2011, 20, 604–608. [Google Scholar] [CrossRef]
- Mihajlov, A.; Srećković, V.; Sakan, N.; Ignjatović, L.M.; Simić, Z.; Dimitrijević, M.S. The inverse bremsstrahlung absorption coefficients and Gaunt factors in astrophysical plasmas. J. Phys. Conf. Ser. 2017, 810. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Srećković, V.A.; Sakan, N.M. Inverse Bremsstrahlung in Astrophysical Plasmas: The Absorption Coefficients and Gaunt Factors. J. Astrophys. Astron. 2015, 36, 635–642. [Google Scholar] [CrossRef]
- Mao, D.; Mussack, K.; Dappen, W. Dynamic Screening in Solar Plasma. Astrophys. J. 2009, 701, 1204. [Google Scholar] [CrossRef]
- Das, M.; Sahoo, B.K.; Pal, S. Plasma screening effects on the electronic structure of multiply charged Al ions using Debye and ion-sphere models. Phys. Rev. A 2016, 93, 052513. [Google Scholar] [CrossRef]
- Leng, Y.; Goldhar, J.; Griem, H.; Lee, R.W. C VI Lyman line profiles from 10-ps KrF-laser-produced plasmas. Phys. Rev. E 1995, 52, 4328–4337. [Google Scholar] [CrossRef]
- Debye, P.; Huckel, E. The theory of electrolytes. I. Lowering of freezing point and related phenomena. Phys. Z. 1923, 24, 185–206. [Google Scholar]
- Ichimaru, S. Basic Principles of Plasma Physics; Benjamin: Reading, MA, USA, 1973. [Google Scholar]
- Kittel, C. Introduction to Solid State Physics, 4th ed.; Wiley: New York, NY, USA, 1977. [Google Scholar]
- Drawin, H.; Felenbok, P. Data for Plasmas in Local Thermodynamic Equilibrium; Gauthier-Villars: Paris, France, 1965. [Google Scholar]
- Kraeft, W.; Kremp, D.; Ebeling, W.; Ropke, G. Quantum Statistics of Charged Particle System; Academie-Verlag: Berlin, Germany, 1986. [Google Scholar]
- Dimitrijević, M.S.; Mihajlov, A.A.; Djurić, Z.; Grabowski, B. On the influence of Debye shielding on the Stark broadening of ion lines within the classical model. J. Phys. B 1989, 22, 3845–3850. [Google Scholar] [CrossRef]
- Mussack, K.; Däppen, W. Dynamic screening in solar and stellar nuclear reactions. Astrophys. Space Sci. 2010, 328, 153–156. [Google Scholar] [CrossRef]
- Nina, A.; Čadež, V.; Srećković, V.; Šulić, D. Altitude distribution of electron concentration in ionospheric D-region in presence of time-varying solar radiation flux. Nucl. Instrum. Methods Phys. Res. B 2012, 279, 110–113. [Google Scholar] [CrossRef]
- Nina, A.; Čadež, V.; Šulić, D.; Srećković, V.; Žigman, V. Effective electron recombination coefficient in ionospheric D-region during the relaxation regime after solar flare from 18 February 2011. Nucl. Instrum. Methods Phys. Res. B 2012, 279, 106–109. [Google Scholar] [CrossRef]
- Potekhin, A.Y.; Chabrier, G. Thermonuclear fusion in dense stars—Electron screening, conductive cooling, and magnetic field effects. Astron. Astrophys. 2012, 538, A115. [Google Scholar] [CrossRef]
- Nina, A.; Čadež, V.M.; Popović, L.Č.; Srećković, V.A. Diagnostics of plasma in the ionospheric D-region: Detection and study of different ionospheric disturbance types. Eur. Phys. J. D 2017, 71, 189. [Google Scholar] [CrossRef]
- Gajo, T.; Ivković, M.; Konjević, N.; Savić, I.; Djurović, S.; Mijatović, Z.; Kobilarov, R. Stark shift of neutral helium lines in low temperature dense plasma and the influence of Debye shielding. Mon. Not. R. Astron. Soc. 2016, 455, 2969–2979. [Google Scholar] [CrossRef]
- Kobzev, G.; Jakubov, I.; Popovich, M. (Eds.) Transport and Optical Properties of Non-Ideal Plasmas; Plenum Press: New York, NY, USA; London, UK, 1995. [Google Scholar]
- Shukla, P.K.; Eliasson, B. Screening and wake potentials of a test charge in quantum plasmas. Phys. Lett. A 2008, 372, 2897–2899. [Google Scholar] [CrossRef]
- Zhao, L.B.; Ho, Y.K. Influence of plasma environments on photoionization of atoms. Phys. Plasmas 2004, 11, 1695–1700. [Google Scholar] [CrossRef]
- Lin, C.Y.; Ho, Y.K. Effects of screened Coulomb (Yukawa) and exponential-cosine-screened Coulomb potentials on photoionization of H and He+. Eur. Phys. J. D 2010, 57, 21–26. [Google Scholar] [CrossRef]
- Lin, C.Y.; Ho, Y.K. The photoionization of excited hydrogen atom in plasmas. Comput. Phys. Commun. 2011, 182, 125–129. [Google Scholar] [CrossRef]
- Gunther, K.; Radtke, R. Electric Properties of Weakly Nonideal Plasmas; Akademie: Berlin, Germany, 1984. [Google Scholar]
- Vitel, Y.; El Bezzari, M.; Mihajlov, A.; Djurić, Z. Experimental verification of semiclassical and RPA calculations of the static conductivity in moderately nonideal plasmas. Phys. Rev. E 2001, 63, 026408. [Google Scholar] [CrossRef] [PubMed]
- Günther, K.; Radtke, R. Elektrische Leitfähigkeit von Xenon-Impulsplasmen. Contrib. Plasma Phys. 1972, 12, 63–72. [Google Scholar] [CrossRef]
- Gunther, K.; Popović, M.; Popović, S.; Radtke, R. Electrical conductivity of highly ionized dense hydrogen plasma. II. Comparison of experiment and theory. J. Phys. D 1976, 9, 1139–1147. [Google Scholar] [CrossRef]
- Goldbach, C.; Nollez, G.; Popović, S.; Popović, M. Electrical conductivity of high pressure ionized argon. Z. Naturforsch. A 1978, 33, 11–17. [Google Scholar] [CrossRef]
- Gunther, K.; Lang, S.; Radtke, R. Electrical conductivity and charge carrier screening in weakly non-ideal argon plasmas. J. Phys. D 1983, 16, 1235–1243. [Google Scholar] [CrossRef]
- Mihajlov, A.A.; Ignjatović, L.M.; Srećković, V.A. The new model method of the electrostatic screening describing: three-component system of the ’closed’ type. arXiv, 2017; arXiv:1703.07613. [Google Scholar]
- Thomas, L.H. The calculation of atomic fields. In Mathematical Proceedings of the Cambridge Philosophical Society; Cambridge University Press: Cambridge, UK, 1927; Volume 23, pp. 542–548. [Google Scholar]
- Fermi, E. Eine statistische Methode zur Bestimmung einiger Eigenschaften des Atoms und ihre Anwendung auf die Theorie des periodischen Systems der Elemente. Z. Phys. 1928, 48, 73–79. [Google Scholar] [CrossRef]
- Gombas, P. Theorie und Losungsmethoden des Mehrteilchenproblems der Wellenmechanik; Springer: Basel, Switzerland, 1950. [Google Scholar]
- Mendonça, J.; Tsintsadze, N.; Guerreiro, A. Thomas-Fermi model for a dust particle in a plasma. Europhys. Lett. 2002, 57, 362–367. [Google Scholar] [CrossRef]
- Reinholz, H.; Morozov, I.; Röpke, G.; Millat, T. Internal versus external conductivity of a dense plasma: Many-particle theory and simulations. Phys. Rev. E 2004, 69, 066412. [Google Scholar] [CrossRef] [PubMed]

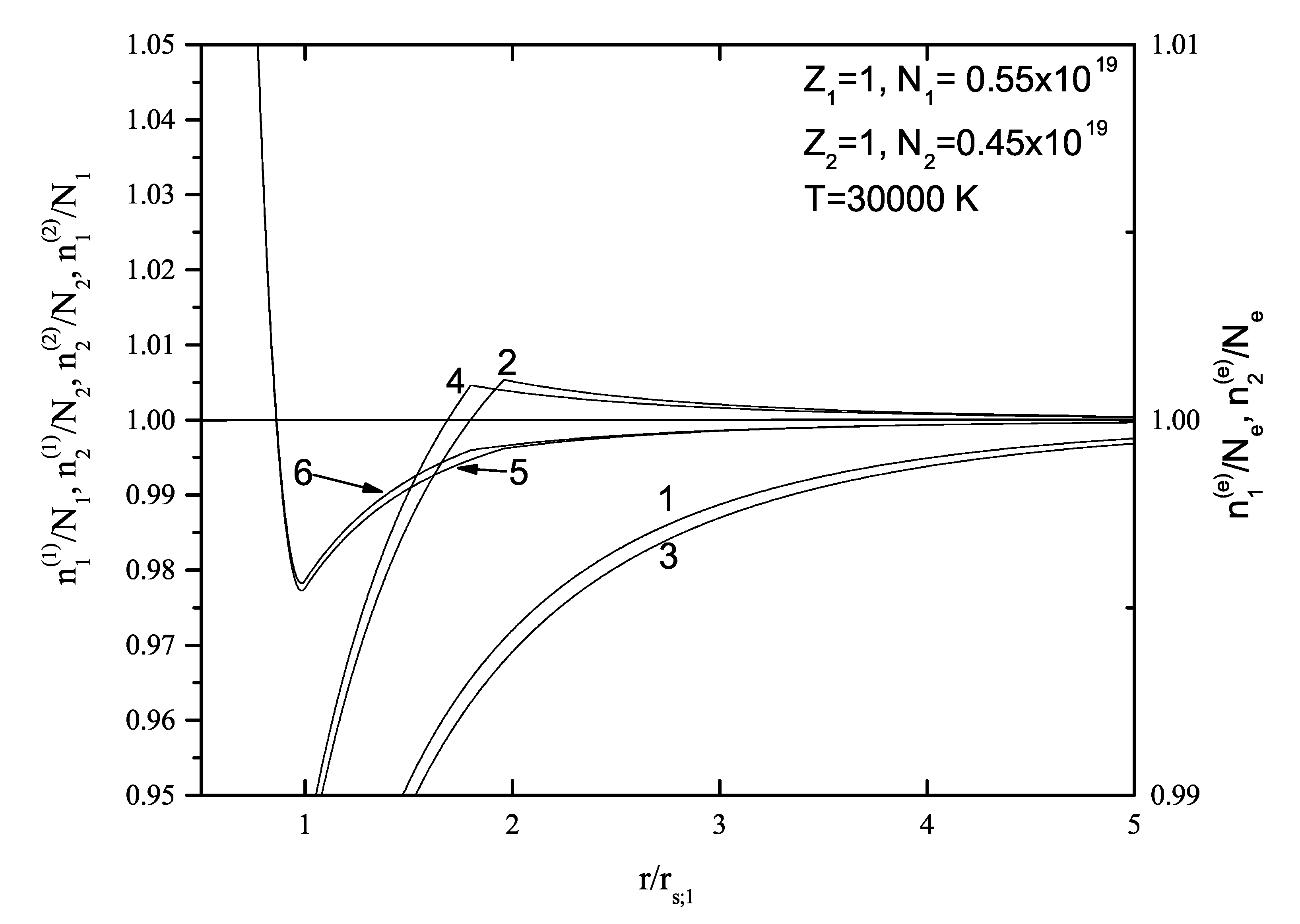
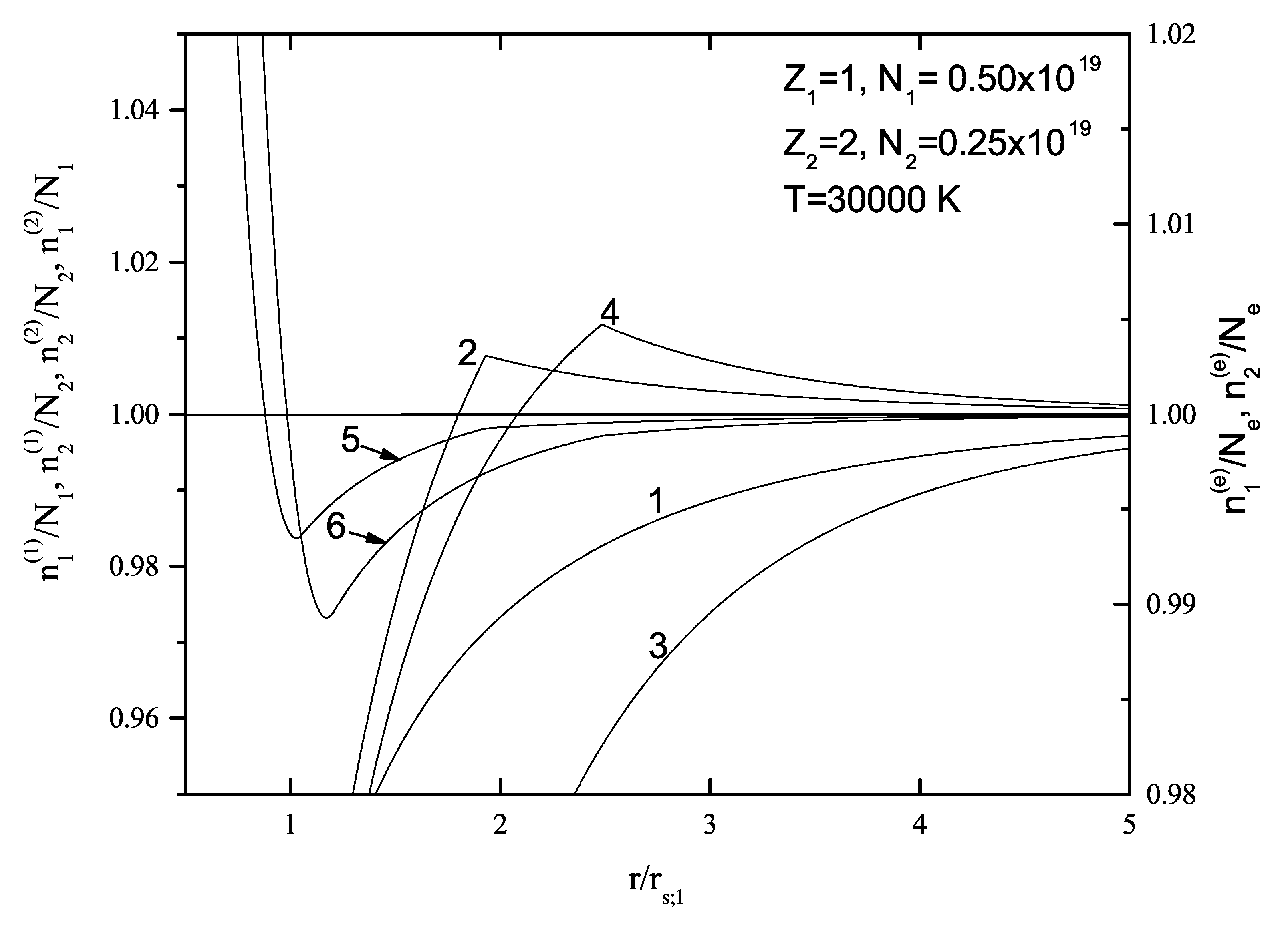

| 0.1 | 0.45 | 14.53 | 16.71 | 0.01 | 0.02 | 22.96 | 73.37 | 0.10 | −1.09 | −1.19 |
| 0.2 | 0.4 | 14.42 | 16.58 | 0.01 | 0.02 | 23.07 | 55.56 | 0.09 | −2.79 | −2.84 |
| 0.3 | 0.35 | 14.30 | 16.45 | 0.01 | 0.02 | 24.60 | 47.87 | 0.09 | −2.39 | −2.43 |
| 0.4 | 0.3 | 14.19 | 16.31 | 0.01 | 0.02 | 26.53 | 42.90 | 0.09 | −2.14 | −2.17 |
| 0.5 | 0.25 | 14.06 | 16.17 | 0.01 | 0.02 | 30.51 | 41.40 | 0.10 | −2.07 | −2.09 |
| 0.6 | 0.2 | 13.93 | 16.02 | 0.01 | 0.02 | 30.23 | 34.29 | 0.08 | −1.71 | −1.72 |
| 0.7 | 0.15 | 13.80 | 15.87 | 0.01 | 0.02 | 32.15 | 29.99 | 0.07 | −1.60 | −1.61 |
| 0.8 | 0.1 | 13.66 | 15.71 | 0.01 | 0.02 | 41.26 | 30.32 | 0.08 | −2.07 | −2.07 |
| 0.9 | 0.05 | 13.52 | 15.54 | 0.01 | 0.02 | 55.42 | 28.75 | 0.08 | −2.83 | −2.78 |
| 1 | 4.5 | 6.74 | 7.76 | 0.03 | 0.05 | 10.24 | 29.40 | 0.18 | −1.35 | −4.77 |
| 2 | 4 | 6.69 | 7.70 | 0.03 | 0.05 | 10.10 | 22.79 | 0.16 | −3.45 | −3.62 |
| 3 | 3.5 | 6.63 | 7.64 | 0.03 | 0.05 | 10.41 | 19.40 | 0.15 | −2.89 | −3.00 |
| 4 | 3 | 6.58 | 7.58 | 0.03 | 0.05 | 11.18 | 17.57 | 0.15 | −2.64 | −2.71 |
| 5 | 2.5 | 6.52 | 7.51 | 0.03 | 0.05 | 12.59 | 16.75 | 0.16 | −2.53 | −2.58 |
| 6 | 2 | 6.46 | 7.44 | 0.03 | 0.05 | 14.41 | 16.22 | 0.17 | −2.46 | −2.49 |
| 7 | 1.5 | 6.40 | 7.37 | 0.03 | 0.05 | 10.31 | 9.66 | 0.07 | −3.32 | −3.25 |
| 8 | 1 | 6.34 | 7.30 | 0.03 | 0.04 | 16.16 | 12.04 | 0.12 | −2.47 | −2.44 |
| 9 | 0.5 | 6.27 | 7.22 | 0.03 | 0.04 | 22.20 | 11.92 | 0.13 | −3.48 | −3.36 |
| 10 | 45 | 3.12 | 3.60 | 0.06 | 0.09 | 4.93 | 12.25 | 0.34 | −5.52 | −5.56 |
| 20 | 40 | 3.10 | 3.58 | 0.06 | 0.09 | 4.71 | 9.62 | 0.29 | −4.04 | −4.25 |
| 30 | 35 | 3.07 | 3.55 | 0.06 | 0.09 | 4.88 | 8.48 | 0.28 | −3.49 | −3.75 |
| 40 | 30 | 3.05 | 3.52 | 0.06 | 0.09 | 5.12 | 7.67 | 0.27 | −3.25 | −3.42 |
| 50 | 25 | 3.02 | 3.49 | 0.06 | 0.09 | 5.56 | 7.15 | 0.27 | −3.07 | −3.20 |
| 60 | 20 | 3.00 | 3.46 | 0.06 | 0.09 | 8.12 | 8.92 | 0.43 | −3.99 | −4.00 |
| 70 | 15 | 2.97 | 3.43 | 0.06 | 0.09 | 3.86 | 3.60 | 0.09 | −3.44 | −3.38 |
| 80 | 10 | 2.94 | 3.39 | 0.06 | 0.09 | 5.88 | 4.45 | 0.15 | −2.55 | −6.03 |
| 90 | 5 | 2.91 | 3.36 | 0.06 | 0.09 | 8.35 | 4.67 | 0.18 | −3.77 | −3.37 |
| 100 | 450 | 1.44 | 1.67 | 0.12 | 0.18 | 3.01 | 5.85 | 0.70 | −6.89 | −6.16 |
| 200 | 400 | 1.43 | 1.66 | 0.12 | 0.18 | 3.79 | 5.93 | 0.81 | −7.36 | −6.44 |
| 300 | 350 | 1.42 | 1.65 | 0.12 | 0.18 | 4.49 | 6.13 | 0.87 | −2.11 | −7.01 |
| 400 | 300 | 1.41 | 1.64 | 0.12 | 0.18 | 4.18 | 5.37 | 0.81 | −6.46 | −5.75 |
| 500 | 250 | 1.40 | 1.62 | 0.12 | 0.18 | 3.79 | 4.51 | 0.71 | −5.00 | −4.66 |
| 600 | 200 | 1.39 | 1.61 | 0.12 | 0.18 | 4.08 | 4.38 | 0.71 | −4.88 | −4.61 |
| 700 | 150 | 1.37 | 1.60 | 0.12 | 0.18 | 1.83 | 1.72 | 0.17 | −3.91 | −3.10 |
| 800 | 100 | 1.36 | 1.58 | 0.12 | 0.18 | 2.06 | 1.60 | 0.16 | −4.86 | −3.86 |
| 900 | 50 | 1.35 | 1.57 | 0.12 | 0.18 | 3.00 | 1.75 | 0.21 | −3.20 | −3.06 |
| 0.1 | 0.9 | 13.37 | 13.37 | 0.01 | 0.01 | 24.73 | 64.82 | 0.06 | −0.48 | −0.50 |
| 0.2 | 0.8 | 13.37 | 13.37 | 0.01 | 0.01 | 26.20 | 48.92 | 0.06 | −1.23 | −1.24 |
| 0.3 | 0.7 | 13.37 | 13.37 | 0.01 | 0.01 | 25.39 | 37.56 | 0.05 | −0.94 | −0.94 |
| 0.4 | 0.6 | 13.37 | 13.37 | 0.01 | 0.01 | 29.94 | 36.09 | 0.06 | −0.90 | −0.90 |
| 0.5 | 0.5 | 13.37 | 13.37 | 0.01 | 0.01 | 18.71 | 18.71 | 0.02 | −0.98 | −0.98 |
| 0.6 | 0.4 | 13.37 | 13.37 | 0.01 | 0.01 | 36.09 | 29.94 | 0.06 | −0.90 | −0.90 |
| 0.7 | 0.3 | 13.37 | 13.37 | 0.01 | 0.01 | 37.56 | 25.39 | 0.05 | −0.94 | −0.94 |
| 0.8 | 0.2 | 13.37 | 13.37 | 0.01 | 0.01 | 48.92 | 26.20 | 0.06 | −1.24 | −1.23 |
| 0.9 | 0.1 | 13.37 | 13.37 | 0.01 | 0.01 | 64.82 | 24.73 | 0.06 | −0.50 | −0.48 |
| 1 | 9 | 6.20 | 6.20 | 0.03 | 0.03 | 10.30 | 25.74 | 0.10 | −2.02 | −2.06 |
| 2 | 8 | 6.20 | 6.20 | 0.02 | 0.02 | 10.92 | 19.79 | 0.10 | −1.50 | −1.54 |
| 3 | 7 | 6.20 | 6.20 | 0.02 | 0.02 | 11.66 | 16.81 | 0.10 | −1.28 | −1.29 |
| 4 | 6 | 6.20 | 6.20 | 0.02 | 0.02 | 12.47 | 14.89 | 0.10 | −1.13 | −1.13 |
| 5 | 5 | 6.20 | 6.20 | 0.02 | 0.02 | 7.32 | 7.32 | 0.03 | −1.14 | −1.14 |
| 6 | 4 | 6.20 | 6.20 | 0.02 | 0.02 | 14.89 | 12.47 | 0.10 | −1.13 | −1.13 |
| 7 | 3 | 6.20 | 6.20 | 0.02 | 0.02 | 16.81 | 11.66 | 0.10 | −1.29 | −1.28 |
| 8 | 2 | 6.20 | 6.20 | 0.02 | 0.02 | 19.79 | 10.92 | 0.10 | −1.54 | −1.50 |
| 9 | 1 | 6.20 | 6.20 | 0.02 | 0.02 | 25.74 | 10.30 | 0.10 | −2.06 | −2.02 |
| 10 | 90 | 2.88 | 2.88 | 0.06 | 0.06 | 4.32 | 10.11 | 0.16 | −2.28 | −2.39 |
| 20 | 80 | 2.88 | 2.88 | 0.06 | 0.06 | 4.41 | 7.72 | 0.15 | −1.63 | −1.73 |
| 30 | 70 | 2.88 | 2.88 | 0.06 | 0.06 | 4.66 | 6.62 | 0.15 | −1.43 | −1.46 |
| 40 | 60 | 2.88 | 2.88 | 0.06 | 0.06 | 5.01 | 5.93 | 0.15 | −1.28 | −1.29 |
| 50 | 50 | 2.88 | 2.88 | 0.06 | 0.06 | 3.08 | 3.08 | 0.05 | −1.34 | −1.34 |
| 60 | 40 | 2.88 | 2.88 | 0.06 | 0.06 | 5.93 | 5.01 | 0.15 | −1.29 | −1.28 |
| 70 | 30 | 2.88 | 2.88 | 0.06 | 0.06 | 6.62 | 4.66 | 0.15 | −1.46 | −1.43 |
| 80 | 20 | 2.88 | 2.88 | 0.06 | 0.06 | 7.72 | 4.41 | 0.15 | −1.73 | −1.63 |
| 90 | 10 | 2.88 | 2.88 | 0.06 | 0.06 | 10.11 | 4.32 | 0.16 | −2.39 | −2.28 |
| 100 | 900 | 1.34 | 1.34 | 0.12 | 0.12 | 1.82 | 3.96 | 0.24 | −2.02 | −2.29 |
| 200 | 800 | 1.34 | 1.34 | 0.12 | 0.12 | 1.78 | 2.98 | 0.21 | −1.43 | −1.61 |
| 300 | 700 | 1.34 | 1.34 | 0.12 | 0.12 | 1.92 | 2.66 | 0.22 | −1.34 | −1.41 |
| 400 | 600 | 1.34 | 1.34 | 0.12 | 0.12 | 1.95 | 2.29 | 0.20 | −2.97 | −3.00 |
| 500 | 500 | 1.34 | 1.34 | 0.12 | 0.12 | 1.39 | 1.39 | 0.09 | −1.36 | −1.36 |
| 600 | 400 | 1.34 | 1.34 | 0.12 | 0.12 | 2.29 | 1.95 | 0.20 | −3.00 | −2.97 |
| 700 | 300 | 1.34 | 1.34 | 0.12 | 0.12 | 2.66 | 1.92 | 0.22 | −1.41 | −1.34 |
| 800 | 200 | 1.34 | 1.34 | 0.12 | 0.12 | 2.98 | 1.78 | 0.21 | −1.61 | −1.43 |
| 900 | 100 | 1.34 | 1.34 | 0.12 | 0.12 | 3.96 | 1.82 | 0.24 | −2.29 | −2.02 |
| 10,000 K | 15,000 K | 20,000 K | 25,000 K | ||||||
| 0.5 | 4.75 | −1.98 | −1.53 | −1.00 | −3.90 | −1.53 | −1.71 | −1.93 | −2.16 |
| 1.0 | 4.50 | −1.69 | −1.33 | −0.97 | −3.51 | −3.94 | −3.86 | −4.70 | −4.71 |
| 1.5 | 4.25 | −1.37 | −1.15 | −3.17 | −2.93 | −3.32 | −3.36 | −3.88 | −4.01 |
| 2.0 | 4.00 | −1.33 | −1.11 | −1.05 | −3.59 | −1.97 | −1.95 | −3.42 | −3.62 |
| 2.5 | 3.75 | −1.15 | −1.01 | −1.11 | −3.97 | −2.49 | −2.72 | −3.13 | −3.37 |
| 3.0 | 3.50 | −1.20 | −1.04 | −1.13 | −3.99 | −2.44 | −2.61 | −3.02 | −3.22 |
| 3.5 | 3.25 | −1.35 | −1.12 | −1.91 | −1.96 | −2.34 | −2.50 | −2.94 | −3.11 |
| 4.0 | 3.00 | −1.19 | −1.04 | −1.16 | −4.16 | −2.37 | −2.50 | −2.91 | −3.06 |
| 4.5 | 2.75 | −1.14 | −1.29 | −2.34 | −2.24 | −2.36 | −2.47 | −2.78 | −2.92 |
| 5.0 | 2.50 | −1.37 | −1.17 | −2.52 | −2.38 | −2.57 | −2.62 | −2.83 | −2.94 |
| 5.5 | 2.25 | −1.05 | −1.21 | −2.44 | −2.32 | −4.02 | −3.83 | −2.77 | −2.87 |
| 6.0 | 2.00 | −1.08 | −1.27 | −2.32 | −2.24 | −4.32 | −4.09 | −3.78 | −3.74 |
| 6.5 | 1.75 | −1.64 | −1.39 | −2.39 | −2.30 | −3.48 | −3.38 | −1.89 | -6.48 |
| 7.0 | 1.50 | −1.01 | −0.99 | −1.86 | −1.51 | −2.43 | −2.18 | −2.92 | −2.84 |
| 7.5 | 1.25 | −1.20 | −1.28 | −2.09 | −1.71 | −2.73 | −2.44 | −3.62 | −3.51 |
| 8.0 | 1.00 | −1.54 | −1.85 | −2.55 | −2.12 | −3.61 | −3.42 | −2.14 | −4.98 |
| 8.5 | 0.75 | −2.03 | −1.32 | −1.32 | −3.01 | −1.93 | −2.10 | −2.56 | −2.89 |
| 9.0 | 0.50 | −0.83 | −1.94 | −1.62 | −1.59 | −2.41 | −2.55 | −3.11 | −2.70 |
| 9.5 | 0.25 | −1.20 | −1.00 | −2.36 | −2.74 | −3.37 | −2.83 | −4.38 | −3.85 |
| 10,000 K | 15,000 K | 20,000 K | 25,000 K | ||||||
| 0.5 | 9.50 | −0.82 | −0.94 | −1.50 | −1.62 | −2.13 | −2.26 | −0.76 | −0.87 |
| 1.0 | 9.00 | −0.52 | −0.65 | −1.04 | −1.16 | −1.49 | −1.61 | −1.91 | −2.03 |
| 1.5 | 8.50 | −0.39 | −0.52 | −0.84 | −0.96 | −1.21 | −1.33 | −1.56 | −1.67 |
| 2.0 | 8.00 | −0.39 | −0.47 | −0.76 | −0.84 | −1.07 | −1.15 | −1.38 | −1.49 |
| 2.5 | 7.50 | −0.37 | −0.42 | −0.74 | −0.79 | −1.00 | −1.05 | −1.30 | −1.35 |
| 3.0 | 7.00 | −0.35 | −0.38 | −0.66 | −0.70 | −0.97 | −1.01 | −1.18 | −1.22 |
| 3.5 | 6.50 | −1.03 | −1.05 | −0.65 | −0.67 | −0.92 | −0.95 | −1.16 | −1.18 |
| 4.0 | 6.00 | −0.94 | −0.95 | −0.64 | −0.65 | −0.85 | −0.87 | −1.06 | −1.08 |
| 4.5 | 5.50 | −0.95 | −0.95 | −1.50 | −1.51 | −0.82 | −0.83 | −1.05 | −1.06 |
| 5.0 | 5.00 | −0.34 | −0.34 | −0.66 | −0.66 | −0.95 | −0.95 | −1.18 | −1.18 |
| 5.5 | 4.50 | −0.95 | −0.95 | −1.51 | −1.50 | −0.83 | −0.82 | −1.06 | −1.05 |
| 6.0 | 4.00 | −0.95 | −0.94 | −0.65 | −0.64 | −0.87 | −0.85 | −1.08 | −1.06 |
| 6.5 | 3.50 | −1.05 | −1.03 | −0.67 | −0.65 | −0.95 | −0.92 | −1.18 | −1.16 |
| 7.0 | 3.00 | −0.38 | −0.35 | −0.70 | −0.66 | −1.01 | −0.97 | −1.22 | −1.18 |
| 7.5 | 2.50 | −0.42 | −0.37 | −0.79 | −0.74 | −1.05 | −1.00 | −1.35 | −1.30 |
| 8.0 | 2.00 | −0.47 | −0.39 | −0.84 | −0.76 | −1.15 | −1.07 | −1.49 | −1.38 |
| 8.5 | 1.50 | −0.52 | −0.39 | −0.96 | −0.84 | −1.33 | −1.21 | −1.67 | −1.56 |
| 9.0 | 1.00 | −0.65 | −0.52 | −1.16 | −1.04 | −1.61 | −1.49 | −2.03 | −1.91 |
| 9.5 | 0.50 | −0.94 | −0.82 | −1.62 | −1.50 | −2.26 | −2.13 | −0.87 | −0.76 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ignjatović, L.M.; Srećković, V.A.; Dimitrijević, M.S. The Screening Characteristics of the Dense Astrophysical Plasmas: The Three-Component Systems. Atoms 2017, 5, 42. https://doi.org/10.3390/atoms5040042
Ignjatović LM, Srećković VA, Dimitrijević MS. The Screening Characteristics of the Dense Astrophysical Plasmas: The Three-Component Systems. Atoms. 2017; 5(4):42. https://doi.org/10.3390/atoms5040042
Chicago/Turabian StyleIgnjatović, Ljubinko M., Vladimir A. Srećković, and Milan S. Dimitrijević. 2017. "The Screening Characteristics of the Dense Astrophysical Plasmas: The Three-Component Systems" Atoms 5, no. 4: 42. https://doi.org/10.3390/atoms5040042
APA StyleIgnjatović, L. M., Srećković, V. A., & Dimitrijević, M. S. (2017). The Screening Characteristics of the Dense Astrophysical Plasmas: The Three-Component Systems. Atoms, 5(4), 42. https://doi.org/10.3390/atoms5040042








