Continuous Acceleration Sensing Using Optomechanical Droplets
Abstract
1. Introduction
2. Model
3. Existence of Optomechanical Droplets
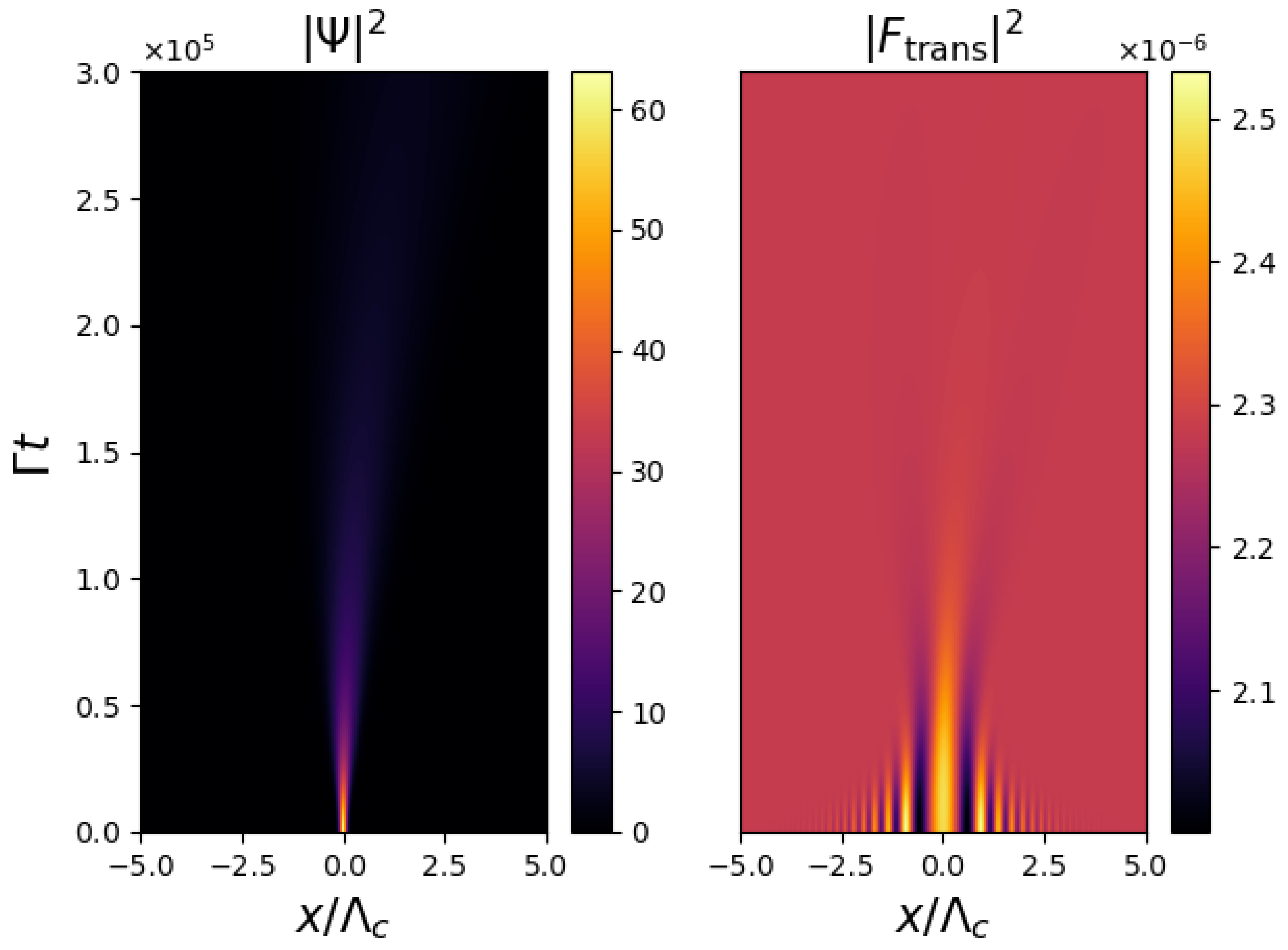
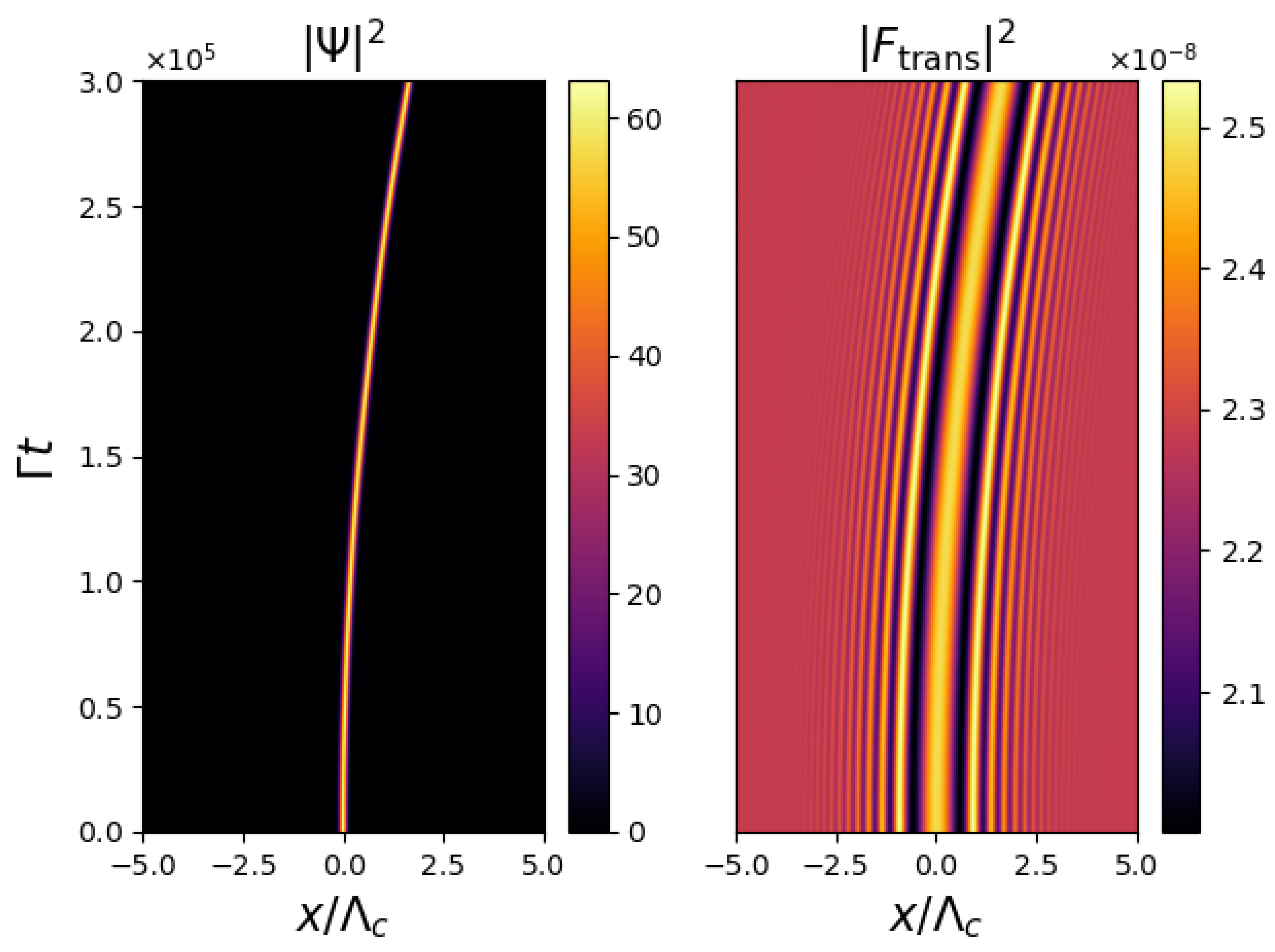
4. Continuous Acceleration Sensing Using Optomechanical Droplets
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Barratt, B.; Gominet, P.A.; Cantin, E.; Antoni-Micollier, L.; Bertoldi, A.; Battelier, B.; Bouyer, P.; Lautier, J.; Landragin, L. Mobile and remote inertial sensing with atom interferometers. In Proceedings of the Proceedings International School Physics ‘Enrico Fermi’, Varenna, Italy, 30 June–5 July 2014; Volume 188, p. 493. [Google Scholar]
- Kasevich, M.; Chu, S. Measurement of the gravitational acceleration of an atom with a light-pulse atom interferometer. Appl. Phys. B 1992, 54, 321. [Google Scholar] [CrossRef]
- Adams, C.S.; Sigel, M.; Mlynek, J. Atom Optics. Phys. Rep. 1994, 240, 143. [Google Scholar] [CrossRef]
- Peters, A.; Chung, K.Y.; Chu, S. Measurement of gravitational acceleration by dropping atoms. Nature 1999, 400, 849. [Google Scholar] [CrossRef]
- Bloom, A.L. Principles of Operation of the Rubidium Vapor Magnetometer. Appl. Opt. 1962, 1, 61–68. [Google Scholar] [CrossRef]
- Budker, D.; Romalis, M. Optical magnetometry. Nat. Phys. 2007, 3, 227–234. [Google Scholar] [CrossRef]
- Kitching, J.; Knappe, S.; Donley, E.A. Atomic Sensors—A Review. IEEE Sens. 2011, 11, 1749. [Google Scholar] [CrossRef]
- Andrews, M.; Mewes, M.O.; Van Druten, N.; Durfee, D.; Kurn, D.; Ketterle, W. Direct, nondestructive observation of a Bose condensate. Science 1996, 273, 84–87. [Google Scholar] [CrossRef] [PubMed]
- Andrews, M.R.; Kurn, D.; Miesner, H.J.; Durfee, D.S.; Townsend, C.G.; Inouye, S.; Ketterle, W. Propagation of sound in a Bose–Einstein condensate. Phys. Rev. Lett. 1997, 79, 553–556. [Google Scholar] [CrossRef]
- Bradley, C.; Sackett, C.; Hulet, R. Bose–Einstein condensation of lithium: Observation of limited condensate number. Phys. Rev. Lett. 1997, 78, 985–989. [Google Scholar] [CrossRef]
- Stenger, J.; Inouye, S.; Chikkatur, A.P.; Stamper-Kurn, D.M.; Pritchard, D.E.; Ketterle, W. Bragg Spectroscopy of a Bose–Einstein Condensate. Phys. Rev. Lett. 1999, 82, 4569–4573. [Google Scholar] [CrossRef]
- Saba, M.; Pasquini, T.A.; Sanner, C.; Shin, Y.; Ketterle, W.; Pritchard, D.E. Light Scattering to Determine the Relative Phase of Two Bose–Einstein Condensates. Science 2005, 307, 1945–1948. [Google Scholar] [CrossRef]
- Peden, B.M.; Meiser, D.; Chiofalo, M.L.; Holland, M.J. Nondestructive cavity QED probe of Bloch oscillations in a gas of ultracold atoms. Phys. Rev. A 2009, 80, 043803. [Google Scholar] [CrossRef]
- Venkatesh, B.P.; Trupke, M.; Hinds, E.A.; O’Dell, D.H.J. Atomic Bloch-Zener oscillations for sensitive force measurements in a cavity. Phys. Rev. A 2009, 80, 063834. [Google Scholar] [CrossRef]
- Goldwin, J.; Venkatesh, B.P.; O’Dell, D. Backaction-Driven Transport of Bloch Oscillating Atoms in Ring Cavities. Phys. Rev. Lett. 2014, 113, 073003. [Google Scholar] [CrossRef] [PubMed]
- Keßler, H.; Klinder, J.; Venkatesh, B.P.; Georges, C.; Hemmerich, A. In situ observation of optomechanical Bloch oscillations in an optical cavity. New J. Phys. 2016, 18, 102001. [Google Scholar] [CrossRef]
- Samoylova, M.; Piovella, N.; Hunter, D.; Robb, G.R.M.; Bachelard, R.; Courteille, P.W. Mode-locked Bloch oscillations in a ring cavity. Laser Phys. Lett. 2015, 11, 126005. [Google Scholar] [CrossRef]
- Samoylova, M.; Piovella, N.; Robb, G.R.M.; Bachelard, R.; Courteille, P.W. Synchronization of Bloch oscillations by a ring cavity. Opt. Express 2015, 23, 14823. [Google Scholar] [CrossRef] [PubMed]
- Dahan, M.B.; Peik, E.; Reichel, J.; Castin, Y.; Salomon, C. Bloch Oscillations of Atoms in an Optical Potential. Phys. Rev. Lett. 1996, 76, 4508. [Google Scholar] [CrossRef]
- Kruse, D.; von Cube, C.; Zimmermann, C.; Courteille, P.W. Observation of lasing mediated by collective atomic recoil. Phys. Rev. Lett. 2003, 91, 183601. [Google Scholar] [CrossRef]
- Ritsch, H.; Domokos, P.; Brennecke, F.; Esslinger, T. Cold atoms in cavity-generated dynamical optical potentials. Rev. Mod. Phys. 2013, 85, 553–601. [Google Scholar] [CrossRef]
- Mivehvar, F.; Piazza, F.; Donner, T.; Ritsch, H. Cavity QED with quantum gases: New paradigms in many-body physics. Adv. Phys. 2021, 70, 1–153. [Google Scholar] [CrossRef]
- Kollar, A.J.; Papageorge, A.T.; Vaidya, V.D.; Guo, Y.; Keeling, J.; Lev, B.L. Supermode-density-wave-polariton condensation with a Bose–Einstein condensate in a multimode cavity. Nat. Commun. 2017, 8, 14386. [Google Scholar] [CrossRef]
- Kroeze, R.M.; Guo, Y.; Vaidya, V.D.; Keeling, J.; Lev, B.L. Spinor self-ordering of a quantum gas in a cavity. Phys. Rev. Lett. 2018, 121, 163601. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Kroeze, R.M.; Marsh, B.P.; Gopalakrishnan, S.; Keeling, J.; Lev, B.L. An optical lattice with sound. Nature 2021, 599, 211. [Google Scholar] [CrossRef] [PubMed]
- Cross, M.C.; Hohenberg, P.C. Pattern formation outside of equilibrium. Rev. Mod. Phys. 1993, 65, 851. [Google Scholar] [CrossRef]
- Grynberg, G.; Le Bihan, E.; Verkerk, P.; Simoneau, P.; Leite, J.R.; Bloch, D.; Le Boiteux, S.; Ducloy, M. Observation of instabilities due to mirrorless four-wave mixing oscillation in sodium. Opt. Commun. 1988, 67, 363–366. [Google Scholar] [CrossRef]
- Lippi, G.; Ackemann, T.; Hoffer, L.; Lange, W. Transverse structures in a sodium-filled Fabry-Pérot resonator—I. Experimental results: Symmetries and the role of the incoupling conditions. Chaos Solitons Fractals 1994, 4, 1409–1431. [Google Scholar] [CrossRef]
- Lippi, G.; Ackemann, T.; Hoffer, L.; Lange, W. Transverse structures in a sodium-filled Fabry-Pérot resonator—II. Interpretation of experimental results. Chaos Solitons Fractals 1994, 4, 1433–1449. [Google Scholar] [CrossRef]
- Giusfredi, G.; Valley, J.; Pon, R.; Khitrova, G.; Gibbs, H. Optical instabilities in sodium vapor. JOSA B 1988, 5, 1181–1192. [Google Scholar] [CrossRef]
- Firth, W. Spatial instabilities in a Kerr medium with single feedback mirror. J. Mod. Opt. 1990, 37, 151–153. [Google Scholar] [CrossRef]
- Arecchi, F.T.; Boccaletti, S.; Ramazza, P.L. Pattern formation and competition in nonlinear optics. Phys. Rep. 1999, 318, 1–83. [Google Scholar] [CrossRef]
- Lugiato, L.A. Transverse nonlinear optics: Introduction and review (Editorial to special issue: Nonlinear optical structures, patterns, chaos). Chaos Solitons Fractals 1994, 4, 1251–1258. [Google Scholar] [CrossRef]
- Rosanov, N.N. Transverse patterns in wide-aperture nonlinear optical systems. Prog. Opt. 1996, 35, 1–60. [Google Scholar]
- Barbay, S.; Kuszelewicz, R.; Tredicce, J.R. Cavity Solitons in VCSEL Devices. Adv. Opt. Tech. 2011, 2011, 628761. [Google Scholar] [CrossRef]
- Grynberg, G.; Maître, A.; Petrossian, A. Flowerlike patterns generated by a laser beam transmitted through a rubidium cell with a single feedback mirror. Phys. Rev. Lett. 1994, 72, 2379–2382. [Google Scholar] [CrossRef] [PubMed]
- Ackemann, T.; Lange, W. Non- and nearly hexagonal patterns in sodium vapor generated by single-mirror feedback. Phys. Rev. A 1994, 50, R4468–R4471. [Google Scholar] [CrossRef] [PubMed]
- Ackemann, T.; Lange, W. Optical pattern formation in alkali metal vapors: Mechanisms, phenomena and use. Appl. Phys. B 2001, 72, 21–34. [Google Scholar] [CrossRef]
- Greenberg, J.A.; Schmittberger, B.L.; Gauthier, D. Bunching-induced optical nonlinearity and instability in cold atoms. Opt. Exp. 2011, 19, 22535. [Google Scholar] [CrossRef] [PubMed]
- Schmittberger, B.L.; Gauthier, D.J. Spontaneous emergence of free-space optical and atomic patterns. New J. Phys. 2016, 18, 10302. [Google Scholar] [CrossRef]
- Labeyrie, G.; Tesio, E.; Gomes, P.M.; Oppo, G.L.; Firth, W.J.; Robb, G.R.; Arnold, A.S.; Kaiser, R.; Ackemann, T. Optomechanical self-structuring in a cold atomic gas. Nat. Photonics 2014, 8, 321–325. [Google Scholar] [CrossRef]
- Tesio, E. Theory of Self-Organisation in Cold Atoms. Ph.D. Thesis, University of Strathclyde, Glasgow, UK, 2014. [Google Scholar]
- Firth, W.J.; Krešić, I.; Labeyrie, G.; Camara, A.; Ackemann, T. Thick-medium model of transverse pattern formation in optically excited cold two-level atoms with a feedback mirror. Phys. Rev. A 2017, 96, 053806. [Google Scholar] [CrossRef]
- Robb, G.; Tesio, E.; Oppo, G.L.; Firth, W.; Ackemann, T.; Bonifacio, R. Quantum threshold for optomechanical self-structuring in a Bose–Einstein condensate. Phys. Rev. Lett. 2015, 114, 173903. [Google Scholar] [CrossRef]
- Zhang, Y.C.; Walther, V.; Pohl, T. Long-range interactions and symmetry breaking in quantum gases through optical feedback. Phys. Rev. Lett. 2018, 121, 073604. [Google Scholar] [CrossRef]
- Zhang, Y.C.; Walther, V.; Pohl, T. Self-bound droplet clusters in laser-driven Bose–Einstein condensates. Phys. Rev. A 2021, 103, 023308. [Google Scholar] [CrossRef]
- Walker, J.G.M.; Robb, G.R.M.; Oppo, G.L.; Ackemann, T. Dynamics of optomechanical droplets in a Bose–Einstein condensate. Phys. Rev. A 2022, 105, 063305. [Google Scholar] [CrossRef]
- Edmonds, M.; Bland, T.; Parker, N. Quantum droplets of quasi-one-dimensional dipolar Bose–Einstein condensates. J. Phys. Commun. 2020, 4, 125008. [Google Scholar] [CrossRef]
- Cabrera, C.R.; Tanzi, L.; Sanz, J.; Naylor, B.; Thomas, P.; Cheiney, P.; Tarruell, L. Quantum liquid droplets in a mixture of Bose–Einstein condensates. Science 2018, 359, 301–304. [Google Scholar] [CrossRef]
- Ackemann, T.; Firth, W.; Oppo, G.L. Fundamentals and applications of spatial dissipative solitons in photonic devices. Adv. At. Mol. Opt. Phys. 2009, 57, 323–421. [Google Scholar]
- von Cube, C.; Slama, S.; Kruse, D.; Zimmermann, C.; Courteille, P.W.; Robb, G.R.M.; Piovella, N.; Bonifacio, R. Self-Synchronization and Dissipation-Induced Threshold in Collective Atomic Recoil Lasing. Phys. Rev. Lett. 2004, 93, 083601. [Google Scholar] [CrossRef] [PubMed]
- Baumann, K.; Guerlin, C.; Brennecke, F.; Esslinger, T. Dicke quantum phase transition with a superfluid gas in an optical cavity. Nature 2010, 464, 1301–1306. [Google Scholar] [CrossRef]
- Ferrier-Barbut, I.; Kadau, H.; Schmitt, M.; Wenzel, M.; Pfau, T. Observation of Quantum Droplets in a Strongly Dipolar Bose Gas. Phys. Rev. Lett. 2016, 116, 215301. [Google Scholar] [CrossRef] [PubMed]
- Chomaz, L.; Baier, S.; Petter, D.; Mark, M.J.; Wachtler, F.; Santos, L.; Ferlaino, F. Quantum-Fluctuation-Driven Crossover from a Dilute Bose–Einstein Condensate to a Macrodroplet in a Dipolar Quantum Fluid. Phys. Rev. X 2016, 6, 041039. [Google Scholar] [CrossRef]
- Goldberg, A.; Schwartz, J.L. Integration of the Schrödinger equation in imaginary time. J. Comput. Phys. 1967, 1, 433–447. [Google Scholar] [CrossRef]
- Robb, G.; Walker, J.; Oppo, G.L.; Ackemann, T. Long-range interactions in a quantum gas mediated by diffracted light. Phys. Rev. Res. 2023, 5, L032004. [Google Scholar] [CrossRef]
- Plestid, R.; O’Dell, D. Balancing long-range interactions and quantum pressure. Phys. Rev. E 2019, 100, 022216. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Robb, G.R.M.; Walker, J.G.; Oppo, G.-L.; Ackemann, T. Continuous Acceleration Sensing Using Optomechanical Droplets. Atoms 2024, 12, 15. https://doi.org/10.3390/atoms12030015
Robb GRM, Walker JG, Oppo G-L, Ackemann T. Continuous Acceleration Sensing Using Optomechanical Droplets. Atoms. 2024; 12(3):15. https://doi.org/10.3390/atoms12030015
Chicago/Turabian StyleRobb, Gordon R. M., Josh G. Walker, Gian-Luca Oppo, and Thorsten Ackemann. 2024. "Continuous Acceleration Sensing Using Optomechanical Droplets" Atoms 12, no. 3: 15. https://doi.org/10.3390/atoms12030015
APA StyleRobb, G. R. M., Walker, J. G., Oppo, G.-L., & Ackemann, T. (2024). Continuous Acceleration Sensing Using Optomechanical Droplets. Atoms, 12(3), 15. https://doi.org/10.3390/atoms12030015