New Data on Autoionizing States of Ne Induced by Low-Energy Electrons from 45 to 64 eV
Abstract
1. Introduction
2. Experiment
3. Results and Discussion
3.1. The 2s2p63s(3,1S) and 2s2p63p(3,1P) States
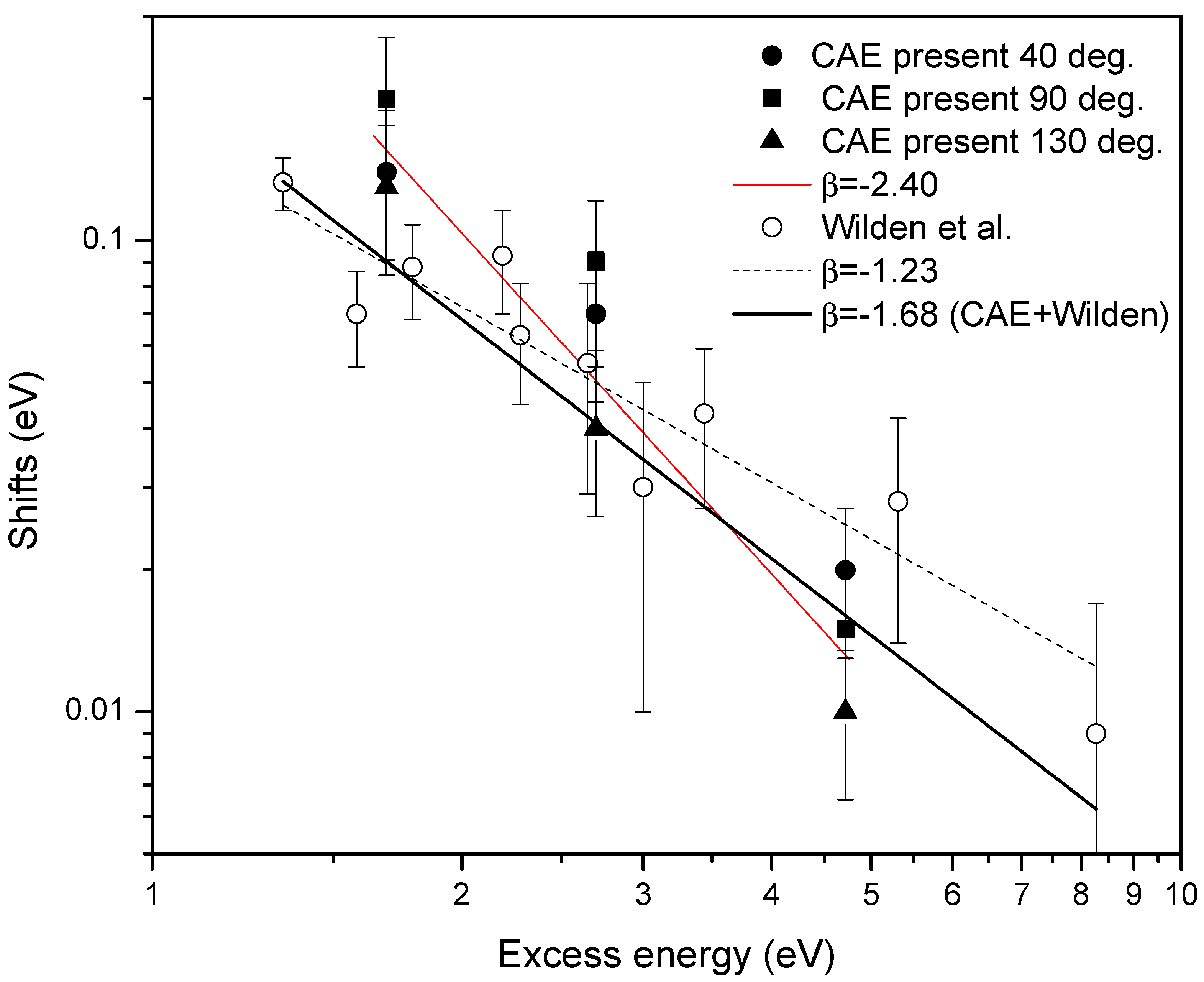
3.2. The Post-Collision Interaction (PCI)
3.3. The First Doubly Excited 2s22p43s2(3P) State
3.4. The Doubly Excited 3s3p Region
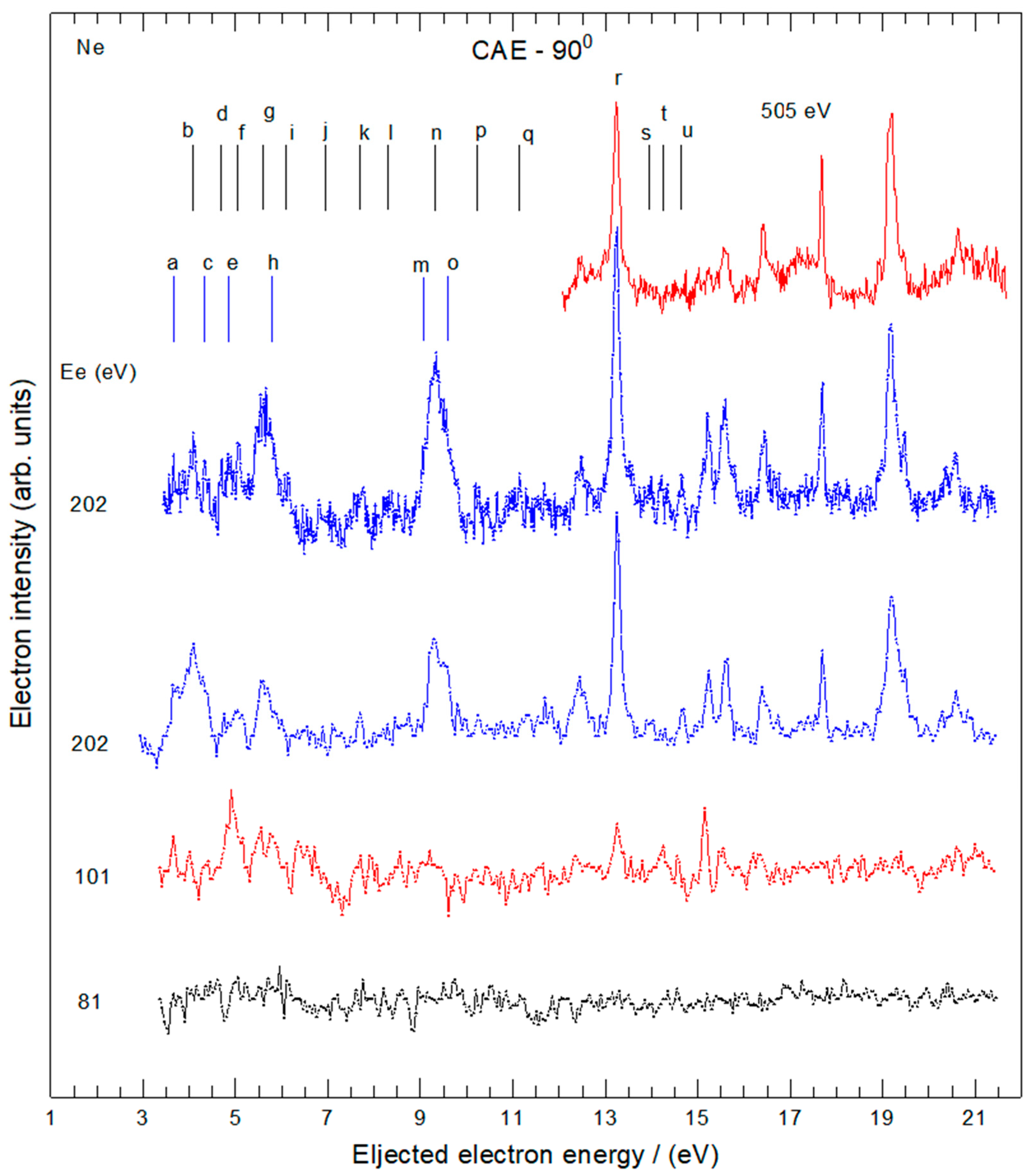
3.5. The Ejected Electron Energy Region from 3 to 21 eV
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Prantzos, N.; Ekström, S. Stellar Nucleosynthesis. In Encyclopedia of Astrobiology; Gargaud, M., Amils, R., Quintanilla, J.C., Cleaves, H.J., Irvine, W.M., Pinti, D.L., Viso, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 1584–1592. [Google Scholar] [CrossRef]
- Nishimura, T.; Aikawa, M.; Suda, T.; Fujimoto, M.Y. Oxygen and Light-Element Synthesis by Neutron-Capture Reactions in Metal-Free and Extremely Metal-Poor AGB Stars. Publ. Astron. Soc. Jpn. 2009, 61, 909–929. [Google Scholar] [CrossRef]
- Zha, S.; Leung, S.-C.; Suzuki, T.; Nomoto, K. Evolution of ONeMg Core in Super-AGB Stars toward Electron-capture Supernovae: Effects of Updated Electron-capture Rate. Astrophys. J. 2019, 886, 22. [Google Scholar] [CrossRef]
- Asplund, M.; Grevesse, N.; Sauval, A.J.; Pat, S. The Chemical Composition of the Sun. Annu. Rev. A&A 2009, 47, 481–522. [Google Scholar]
- Young, P.R. Element Abundance Ratios in the Quiet Sun Transition Region. Astrophys. J. 2018, 855, 15. [Google Scholar] [CrossRef]
- Christensen-Dalsgaard, J. Solar structure and evolution. Living Rev. Sol. Phys. 2021, 18, 2. [Google Scholar] [CrossRef]
- Chang, E.S.; Schoenfeld, W.G.; Bikmont, E.; Quinet, P.; Palmeri, P. Improved Experimental and Theoretical Energy Levels of Neon I. Phys. Scr. 1994, 49, 26–33. [Google Scholar] [CrossRef]
- Saloman, E.B.; Sansonetti, C.J. Wavelengths, Energy Level Classifications, and Energy Levels for the Spectrum of Neutral Neon. J. Phys. Chem. Ref. Data 2004, 33, 1113–1158. [Google Scholar] [CrossRef]
- Persson, W. The Spectrum of Singly Ionized Neon, Ne II. Phys. Scr. 1971, 3, 133–155. [Google Scholar] [CrossRef]
- Kramida, A.E.; Nave, G. The Ne II spectrum. Eur. Phys. J. D 2006, 39, 331–350. [Google Scholar] [CrossRef]
- Kramida, A.E.; Nave, G. New FTS measurements, optimized energy levels and refined VUV standards in the Ne III spectrum. Eur. Phys. J. D 2006, 37, 1–21. [Google Scholar] [CrossRef]
- Zatsarinny, O.; Bartschat, K. Large-scale pseudostate calculations for electron scattering from neon atoms. Phys. Rev. A 2012, 85, 062710. [Google Scholar] [CrossRef]
- Wang, S.-X.; Du, X.-J.; Sun, Q.; Liu, Y.-W.; Qi, D.-G.; Zhu, L.-F. Revisiting the oscillator strengths and cross sections of atomic neon by fast electron scattering. J. Quant. Spectr. Rad. Transf. 2022, 277, 107988. [Google Scholar] [CrossRef]
- Jureta, J.J.; Marinković, B.P.; Milosavljević, A.R.; Avaldi, L. Singly and doubly excited states in ejected electron spectra of neon at high incident electron energies. Eur. Phys. J. D 2015, 69, 74. [Google Scholar] [CrossRef]
- Comer, J.; Read, F.H. Electron impact studies of autoionizing states in neon and helium. J. Electron. Spectrosc. 1972, 1, 3–11. [Google Scholar] [CrossRef]
- Tahira, S.; Nishimura, F.; Oda, N. Energy and angular distributions of electrons ejected from autoionization states in neon by electron impact. J. Phys. B Atom. Mol. Phys. 1973, 6, 2306–2309. [Google Scholar] [CrossRef]
- Sharp, J.M.; Comer, J.; Hicks, P.J. Autoionizing transitions in neon studied by low-energy electron impact. J. Phys. B At. Mol. Phys. 1975, 8, 2512–2519. [Google Scholar] [CrossRef]
- Wilden, D.G.; Hicks, P.J.; Comer, J. Electron impact studies of resonances and autoionizing states of neon. J. Phys. B At. Mol. Phys. 1977, 10, 1477–1486. [Google Scholar] [CrossRef]
- Mitchell, P.; Baxter, J.A.; Comer, J. Electron energy-loss study of the 2s2p63s and 2s2p63p configurations of neon. J. Phys. B At. Mol. Phys. 1980, 13, 2817–2827. [Google Scholar] [CrossRef]
- van den Brink, J.P.; den Outer, P.N.; van Eck, J.; Heideman, H.G.M. Coherence and correlation in electron impact autoionisation of neon and argon. J. Phys. B At. Mol. Opt. Phys. 1990, 23, 2349S–2362S. [Google Scholar] [CrossRef]
- Paripás, B.; Jureta, J.J.; Palásthy, B.; Marinković, B.P.; Pszota, G. High resolution study of the autoionizing states of He in their exchange interference energy region. J. Electron. Spectrosc. Relat. Phenom. 2018, 225, 10–15. [Google Scholar] [CrossRef]
- Avaldi, L.; Jureta, J.J.; Marinković, B.P. Energy analysis of ejected electrons in the region of the Ar L1–L2,3M Coster-Kronig transitions (25–56 eV) induced by electron impact. J. Electron. Spectrosc. Relat. Phenom. 2019, 237, 146898. [Google Scholar] [CrossRef]
- Jureta, J.J.; Marinković, B.P.; Avaldi, L. The M4,5-NN Auger and M2,3-M4,5N Coster-Kronig spectra of krypton induced by electron impact. J. Quant. Spectrosc. Radiat. Transf. 2021, 268, 107638. [Google Scholar] [CrossRef]
- Jureta, J.J.; Marinković, B.P.; Avaldi, L. The N4,5-OO Auger and “N3”-M4,5O2,3 Coster-Kronig spectra of xenon induced by electron impact. Adv. Space Res. 2023, 71, 1338–1351. [Google Scholar] [CrossRef]
- Codling, K.; Madden, R.P.; Ederer, D.L. Resonances in the Photo-Ionization Continuum of Ne I (20–150 eV). Phys. Rev. 1967, 155, 26–38. [Google Scholar] [CrossRef]
- Edwards, A.K.; Rudd, M.E. Excitation of Auto-Ionizing Levels in Neon by Ion Impact. Phys. Rev. 1968, 170, 140–144. [Google Scholar] [CrossRef]
- Smith, A.J.; Hicks, P.J.; Read, F.H.; Cvejanović, S.; King, G.C.; Comer, J.; Sharp, J.M. Phenomena associated with near-threshold excitation of autoionizing levels of helium by electron impact. J. Phys. B At. Mol. Phys. 1974, 7, L496–L502. [Google Scholar] [CrossRef]
- Barker, R.B.; Berry, H.W. Electron Energy Distributions from Ionizing Collisions of Helium and Neon Ions with Helium. Phys. Rev. 1966, 151, 14–19. [Google Scholar] [CrossRef]
- Hicks, P.J.; Cvejanović, S.; Comer, J.; Read, F.H.; Sharp, J.M. Displacements of electron ejection energies in near-threshold excitation of autoionizing levels of helium by electron impact. Vacuum 1974, 24, 573–580. [Google Scholar] [CrossRef]
- Grissom, J.T.; Garrett, W.R.; Compton, R.N. Autoionizing and negative-ion states in neon. Phys. Rev. Lett. 1969, 23, 1011–1014. [Google Scholar] [CrossRef]
- Bolduc, E.; Quemener, J.J.; Marmet, P. Autoionizing 2s22p43s3l States of Ne and Related Ne− Resonances. J. Chem. Phys. 1972, 57, 1957–1966. [Google Scholar] [CrossRef]
- Buckman, S.J.; Clark, C.W. Atomic negative-ion resonances. Rev. Mod. Phys. 1994, 66, 539–655. [Google Scholar] [CrossRef]
- Wills, A.A.; Cafolla, A.A.; Svensson, A.; Comer, J. Resonance structure in the neon photoelectron satellites. J. Phys. B At. Mol. Opt. Phys. 1990, 23, 2013–2028. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenko, Y.; Reader, J.; NIST ASD Team. NIST Atomic Spectra Database (Ver. 5.11); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2023. Available online: https://physics.nist.gov/asd (accessed on 12 April 2023). [CrossRef]
- Avaldi, L.; Dawber, G.; Gilley, N.; Rojas, H.; King, G.C.; Hall, R.; Stuhec, M.; Zitnik, M. A study of Ne2+ and Ar2+ satellite states observed by `threshold photoelectrons coincidence’ spectroscopy. J. Phys. B At. Mol. Opt. Phys. 1997, 30, 5197–5212. [Google Scholar] [CrossRef]
- Kikas, A.; Osborne, S.J.; Ausmees, A.; Svensson, S.; Sairanen, O.-P.; Aksela, S. High-resolution study of the correlation satellites in photoelectron spectra of the rare gases. J. Electron Spectrosc. Relat. Phenom. 1996, 77, 241–266. [Google Scholar] [CrossRef]
- Svensson, S.; Eriksson, B.; Mårtensson, N.; Wendin, G. Electron shake-up and correlation satellites and continuum shake-off distributions in X-Ray photoelectron spectra of the rare gas atoms. J. Electron. Spectrosc. Relat. Phenom. 1988, 47, 327–384. [Google Scholar] [CrossRef]
- Roy, D.; Delage, A.; Carette, J.-D. Resonances in the differential excitation functions of five electronic states of Ne in the autoionization region. Phys. Rev. A 1975, 12, 45–51. [Google Scholar] [CrossRef]
- Spence, D. A technique to enhance and separate negative ions from neutral autoionising features in scattered electron spectra in the ionisation continuum: Application to negative ions in neon. J. Phys. B At. Mol. Phys. 1980, 13, 1611–1624. [Google Scholar] [CrossRef]
- Xiao-Bin, D.; Chen-Zhong, D.; Koike, F.; Kato, T.; Fritzsche, S. Excitation and decay dynamics of 1s2s inner-shell double-vacancy states of neon atoms. Chin. Phys. B 2008, 17, 592–598. [Google Scholar] [CrossRef]
- Moretto-Capelle, P.; Benhenni, M.; Bordenave-Montesquieu, D.; Bordenave-Montesquieu, A. Distortion of He (2l2l’) Fano lineshapes by strong post-collision interaction in HC–He collisions. J. Phys. B At. Mol. Opt. Phys. 1996, 29, 2007–2020. [Google Scholar] [CrossRef]

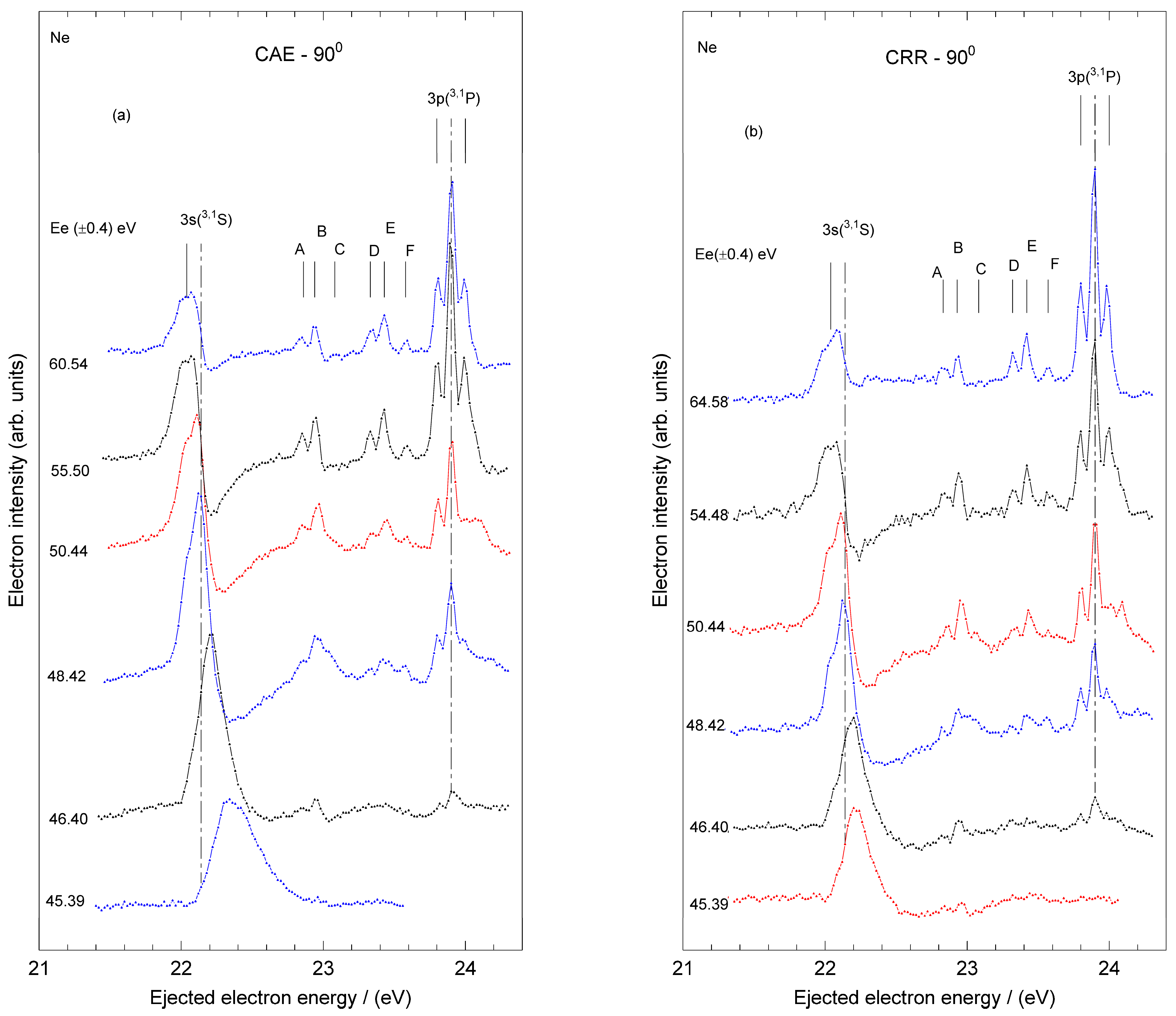
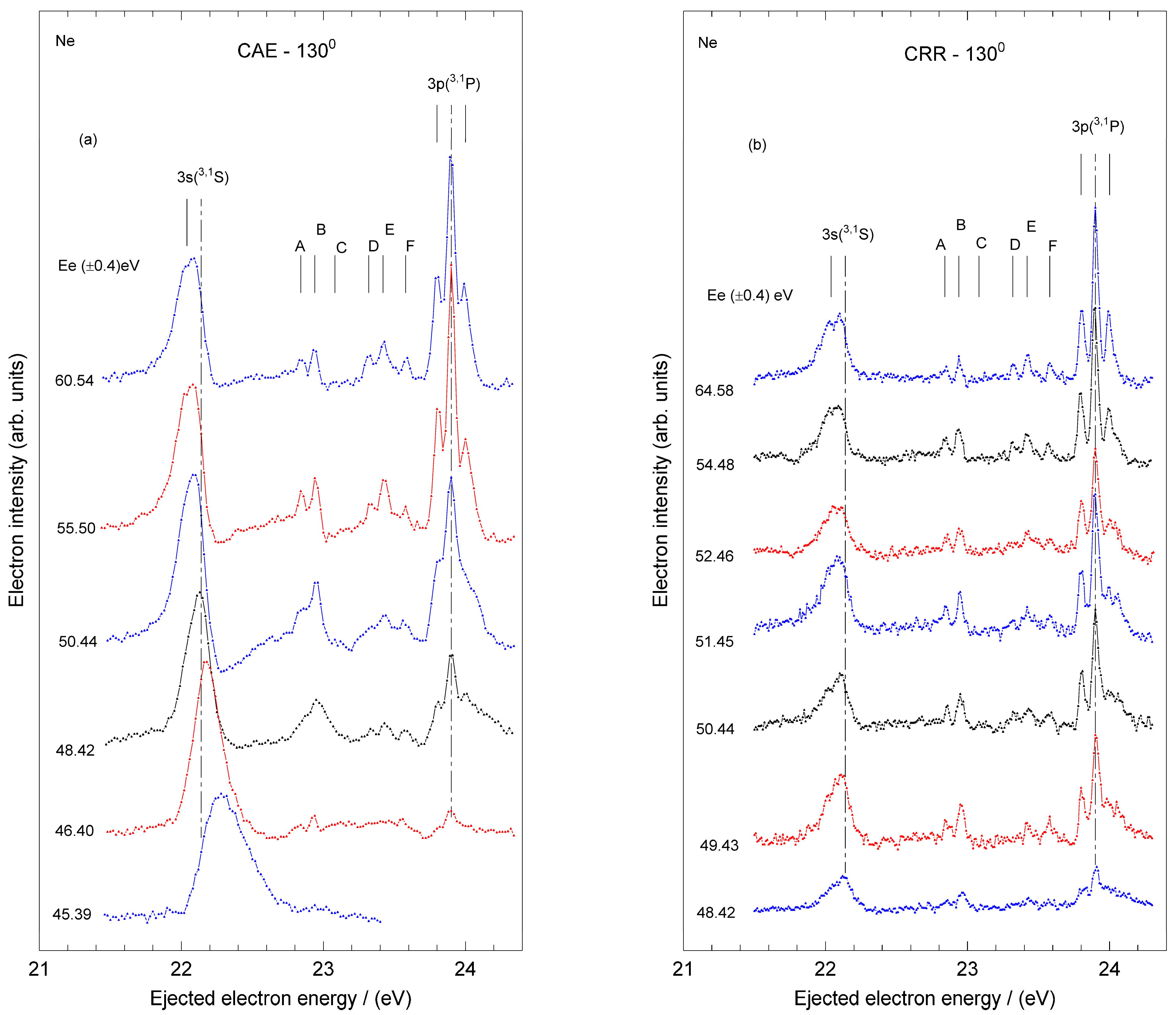

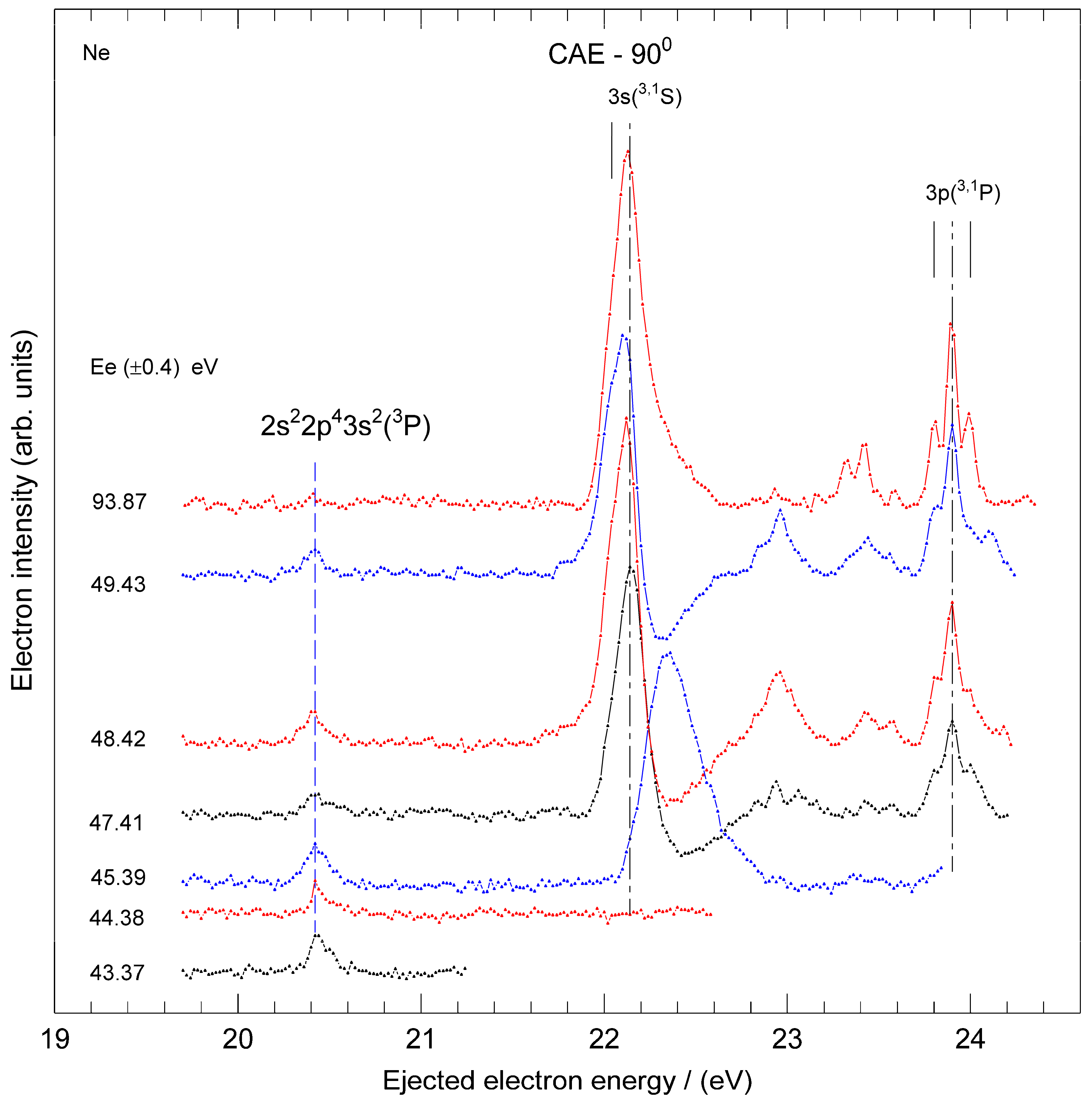
| This Work | Reference | Exc. En. (eV) * | Assignment |
|---|---|---|---|
| KE (eV) | |||
| 22.04 | This work, Ee = 50 eV | 43.60 * | 2s2p63s(3S) |
| [14,19,20,26] | (43.60 *, 43.59 *, 43.59 *, 43.41 *) | ||
| 22.14 | This work, Ee = 50 eV | 43.70 * | 2s2p63s(1S) |
| [14,15,17,19,20] | (43.70 *, 43.64 *, 43.67 *, 43.67 *, 43.67 *) | ||
| 23.80 | This work, Ee = 50 eV | 45.46 * | 2s2p63p(3P) |
| 23.90 | [14,15,17,19,26] | (45.46 *, 45.45 *, 45.45 *, 45.46 *, 45.44 *) | |
| 23.90 | This work, Ee = 50 eV | 45.56 * | 2s2p63p(1P) |
| 24.00 | [14,15,17,19,25] | (45.56 *, 45.55 *, 45.54 *, 45.55 *, 45.546 *) |
| This Work | Reference | Exc. En. (eV) * | Assignment | |
|---|---|---|---|---|
| Label | KE (eV) | |||
| 20.42 | This work, | 41.98 * | 2s22p4(3P)3s2(3P) | |
| [18,30,31] | (41.87 *, 42.10 *, 42.04 *) | |||
| (A) | 22.84 | This work, | 44.50 * | |
| (B) | 22.94 | [17], (b) | 44.48 * | 2p4(3P)3s(4P)3p(3D0) or (3P0) |
| [31], E | 44.43 * | |||
| (C) | 23.08 | This work | 44.64 * | |
| (D) | 23.32 | This work, | 44.99 * | |
| (E) | 23.44 | [17,25], (c) | (44.97 *, 44.98 *) | 2p4(3P)3s(2P)3p(1P0) |
| [31,33], F | (45.00 *, 44.981 *) | 2p4(3P)[3s3p(3P)](1P) | ||
| (F) | 23.58 | This work, | 45.14 * | 2p4(1D)3s2(1D) or |
| [15,17], (d) | 45.12 * | 2p4(3P)3s(2P)3p(3P0) | ||
| This Work | Reference | Exc. En. (eV) * | Assignment | |
|---|---|---|---|---|
| Label | KE (eV) | |||
| (a) | 3.66 | This work, | 66.19 * | |
| [36], Line 49 | 65.99 * | 2p4(1S)4d(2D) | ||
| (b) | 4.08 | This work, | 66.61 * | |
| [36], Line 50 | 66.64 * | 2p4(1S)5p(2P0) | ||
| (c) | 4.32 | This work, | 66.85 * | |
| [36], Line 51 | 66.78 * | (Tail of previous) | ||
| (d) | 4.68 | This work, | 67.21 * | |
| [36], Line 52 | 67.26 * | 2p4(1S)6s(2S) | ||
| (e) | 4.84 | This work | 67.37 * | |
| (f) | 5.04 | This work, | 67.53 * | |
| [36], Line 53 | 67.61 * | 2p4(1S)6p(2P0) | ||
| (g) | 5.58 | This work, | 68.11 * | |
| [36], Line 55 | 68.16 * | 2p4(1S)7d(2D) | ||
| (h) | 5.78 | This work, | 68.31 * | |
| [36], Line 56 | 68.36 * | 2p4(1S)8s(2S) | ||
| (i) | 6.10 | This work | 68.63 * | |
| (j) | 6.94 | This work | 69.47 * | |
| (k) | 7.70 | This work, | 70.23 * | |
| [36], Line 59 | 70.27 * | |||
| (l) | 8.30 | This work | 70.83 * | |
| (m) | 9.08 | This work | 71.61 * | |
| (n) | 9.32 | This work, | 75.06 * | |
| [36], Line 60 | 74.99 * | 2s12p5(3P)3s(2P) | ||
| [37] | 75.11 * | |||
| (o) | 9.58 | This work | 75.32 * | |
| (p) | 10.22 | This work | 75.96 * | |
| (q) | 11.13 | This work, | 76.87 * | |
| [34] | 76.85 * | 2s2p5(1P1) | ||
| (r) | 13.24 | This work, | 78.98 * | |
| [14], (B) | 78.97 * | 2s12p5(3P)3p(2S) | ||
| [36], Line 62 | 78.97 * | |||
| [37] | 78.90 * | |||
| (s) | 13.94 | This work | 83.37 * | |
| [36], Line 65 | 83.42 * | 2s12p5(3P)4p(2S) | ||
| [37] | 83.34 * | |||
| (t) | 14.25 | This work | 83.68 * | |
| (u) | 14.65 | This work | 84.08 * | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jureta, J.J.; Marinković, B.P.; Avaldi, L. New Data on Autoionizing States of Ne Induced by Low-Energy Electrons from 45 to 64 eV. Atoms 2024, 12, 6. https://doi.org/10.3390/atoms12020006
Jureta JJ, Marinković BP, Avaldi L. New Data on Autoionizing States of Ne Induced by Low-Energy Electrons from 45 to 64 eV. Atoms. 2024; 12(2):6. https://doi.org/10.3390/atoms12020006
Chicago/Turabian StyleJureta, Jozo J., Bratislav P. Marinković, and Lorenzo Avaldi. 2024. "New Data on Autoionizing States of Ne Induced by Low-Energy Electrons from 45 to 64 eV" Atoms 12, no. 2: 6. https://doi.org/10.3390/atoms12020006
APA StyleJureta, J. J., Marinković, B. P., & Avaldi, L. (2024). New Data on Autoionizing States of Ne Induced by Low-Energy Electrons from 45 to 64 eV. Atoms, 12(2), 6. https://doi.org/10.3390/atoms12020006







