Electron-Impact Excitation and Dissociation of Heavy Rare Gas Heteronuclear Ions via Transitions to Charge Transfer States
Abstract
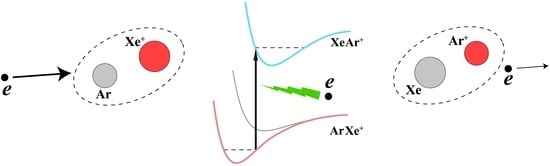
1. Introduction
2. Formulation of the Problem
3. Brief Description of the Theoretical Approach
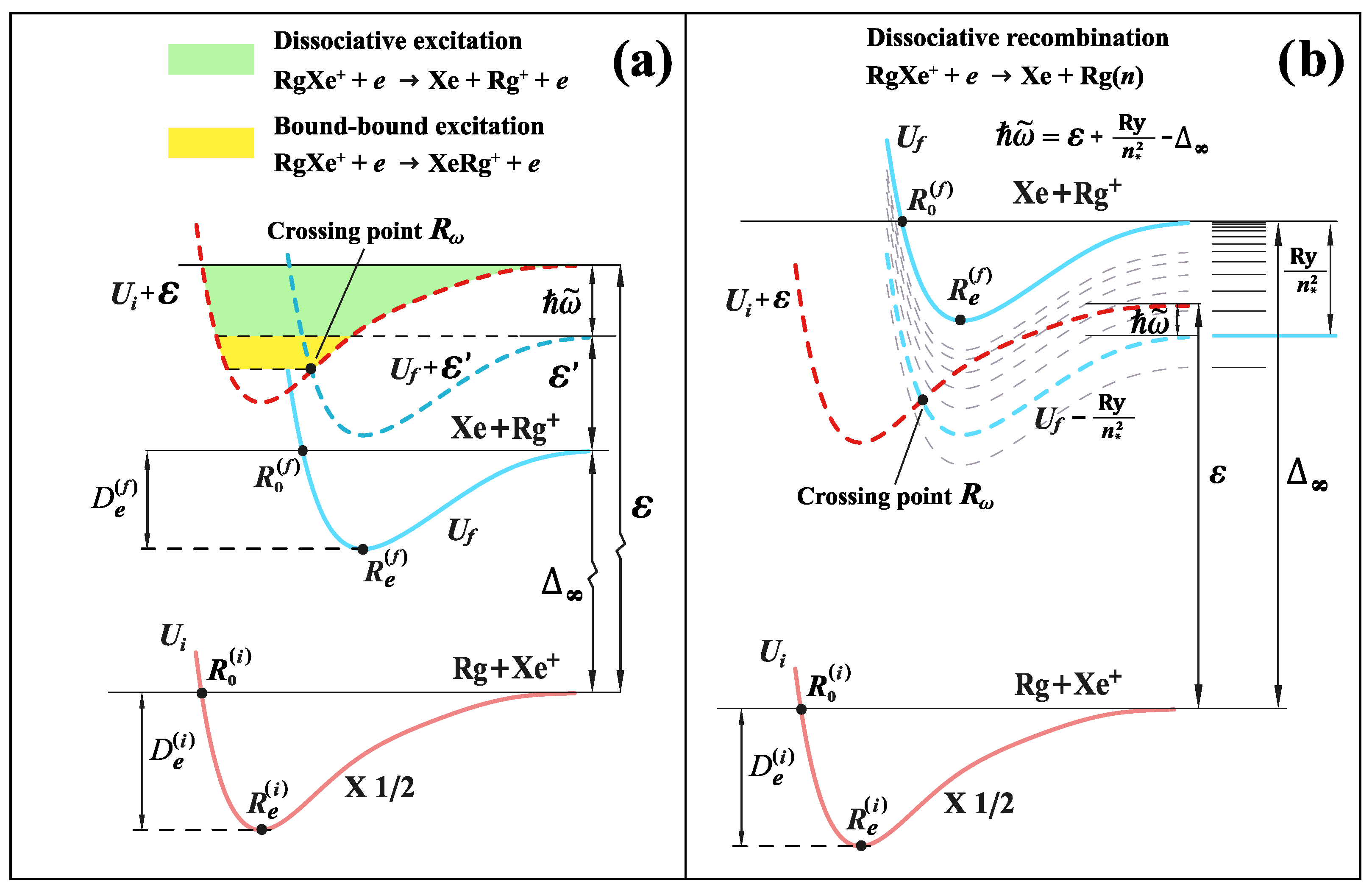
3.1. Cross Sections of the Dissociative Excitation and Electron-Impact Bound–Bound Excitation Involving Transitions to Charge Transfer Terms
3.2. Cross Sections of Dissociative Recombination of Ions via Transitions to Charge Transfer Terms
3.3. Evaluation of the Coupling Parameter and the Autoionization Width
4. Results
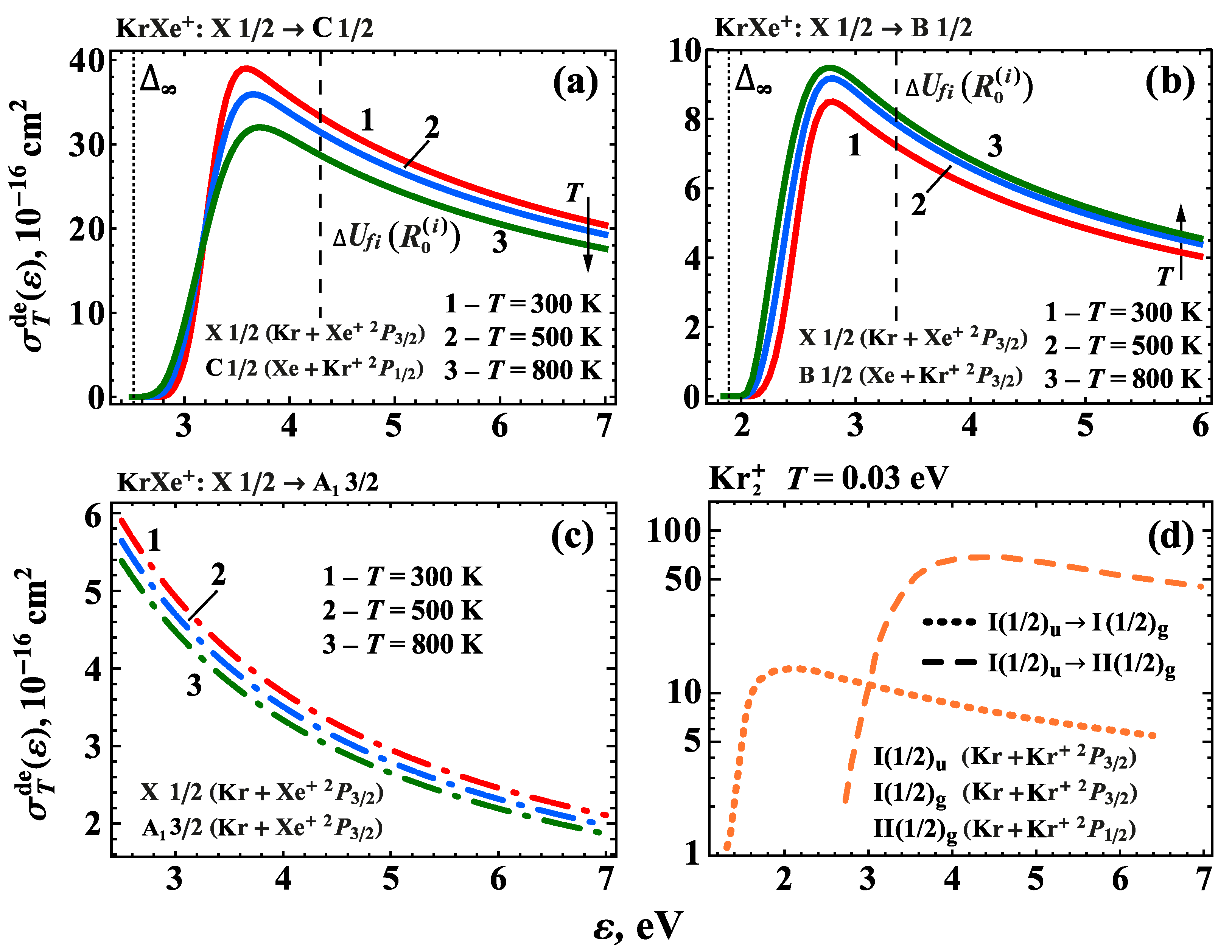
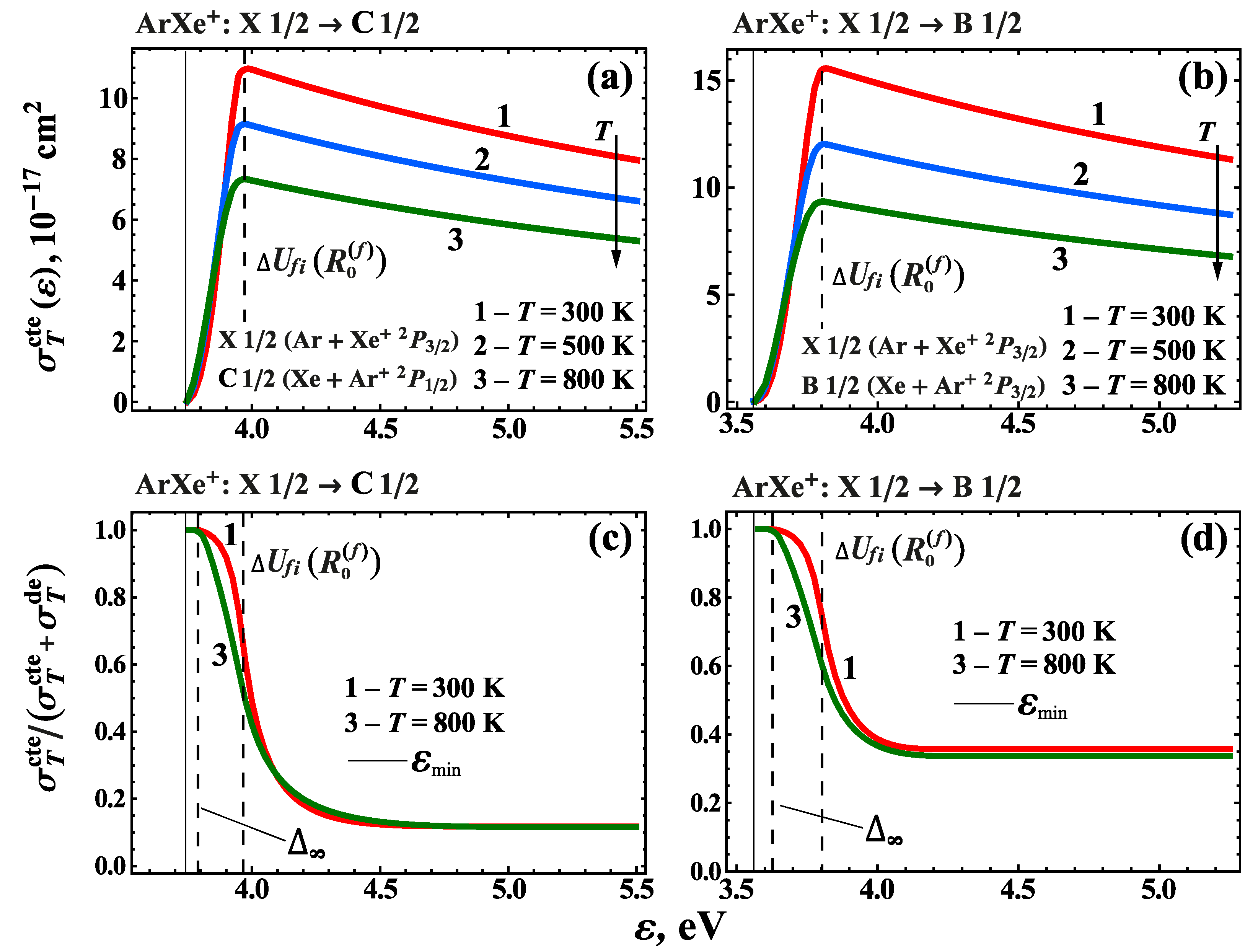
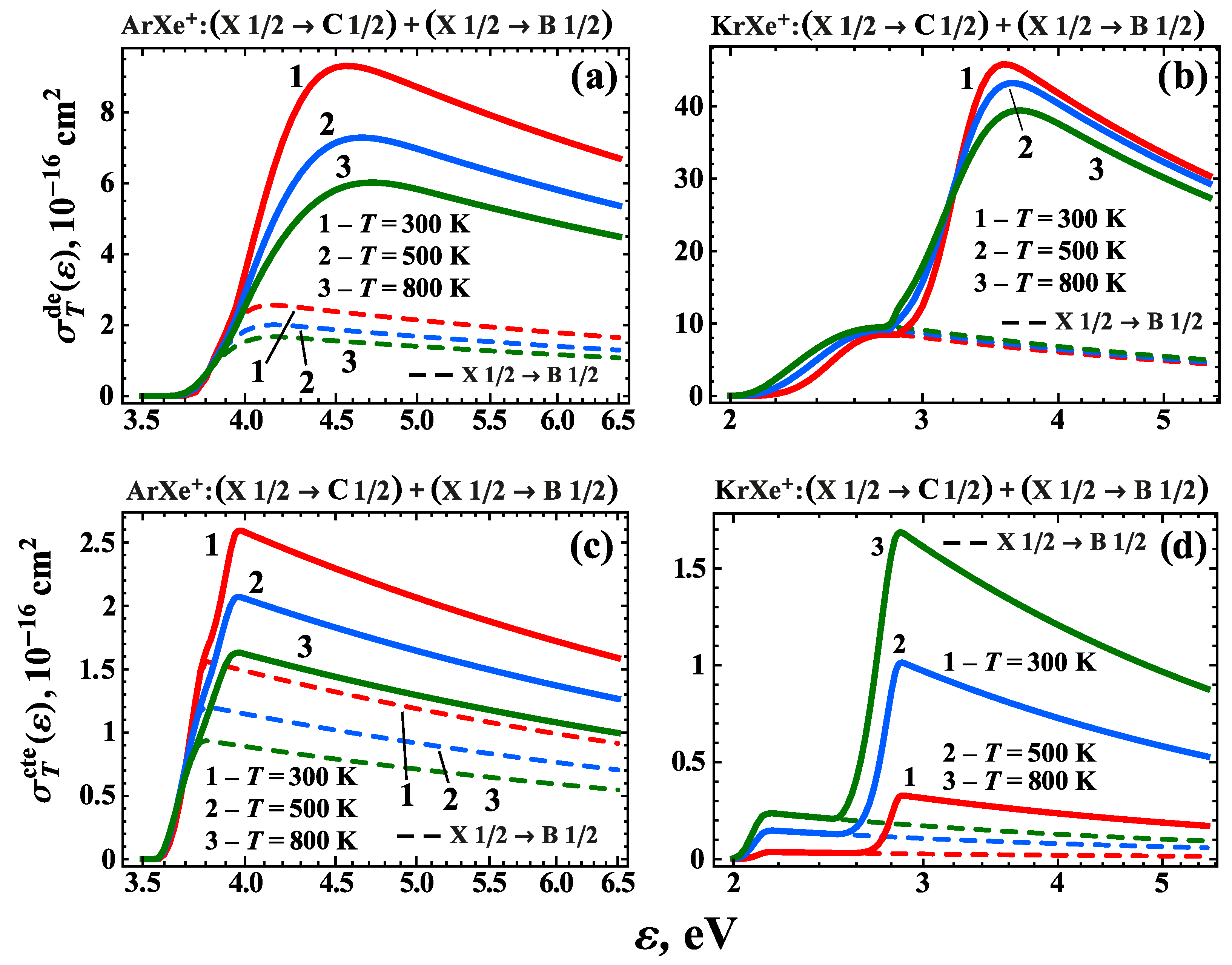
4.1. Electron-Impact Dissociative Excitation of Heteronuclear Rare Gas Ions
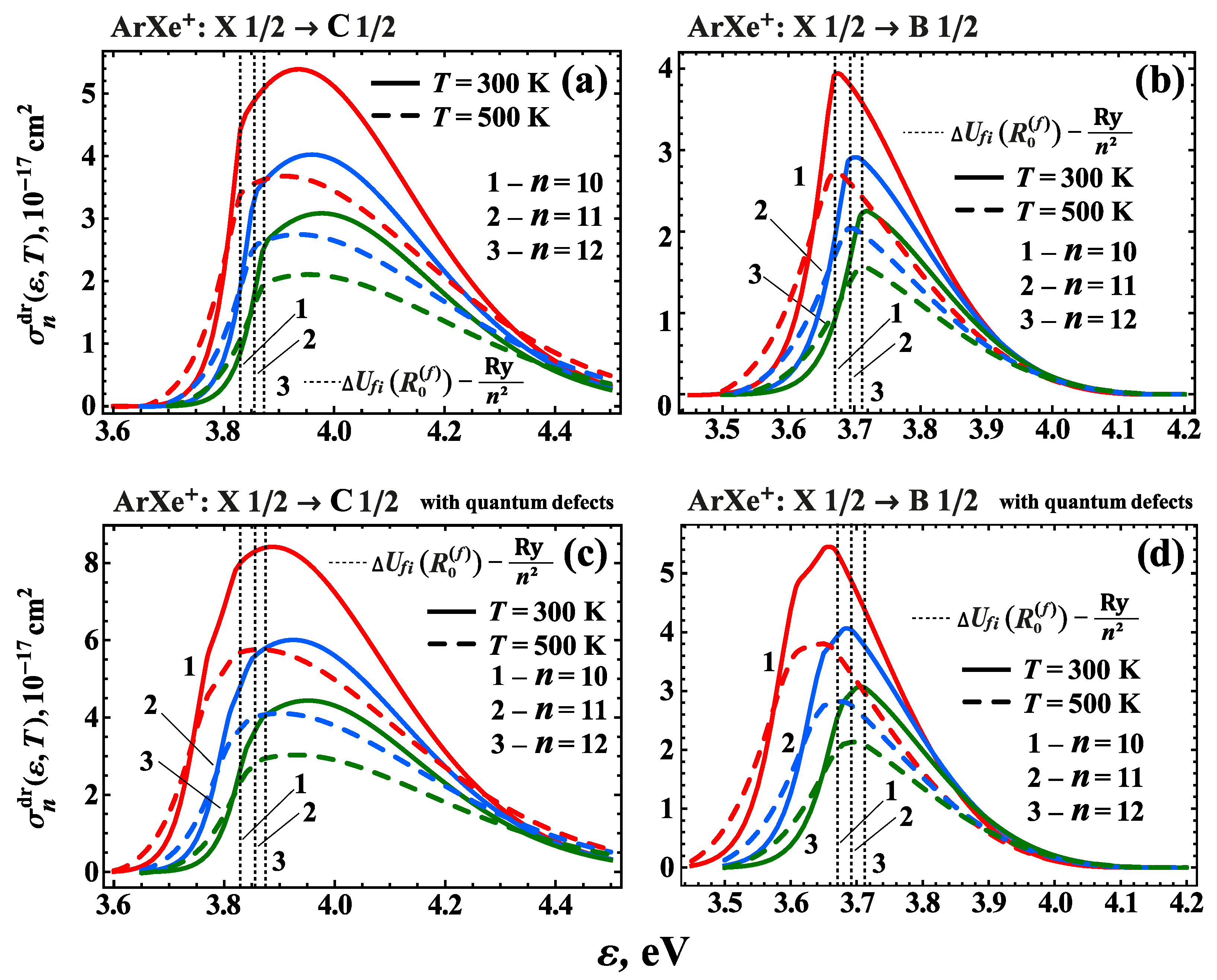
4.2. Electron-Impact Excitation of ArXe and KrXe Ions to Bound Rovibrational States in Charge Transfer Electronic Terms
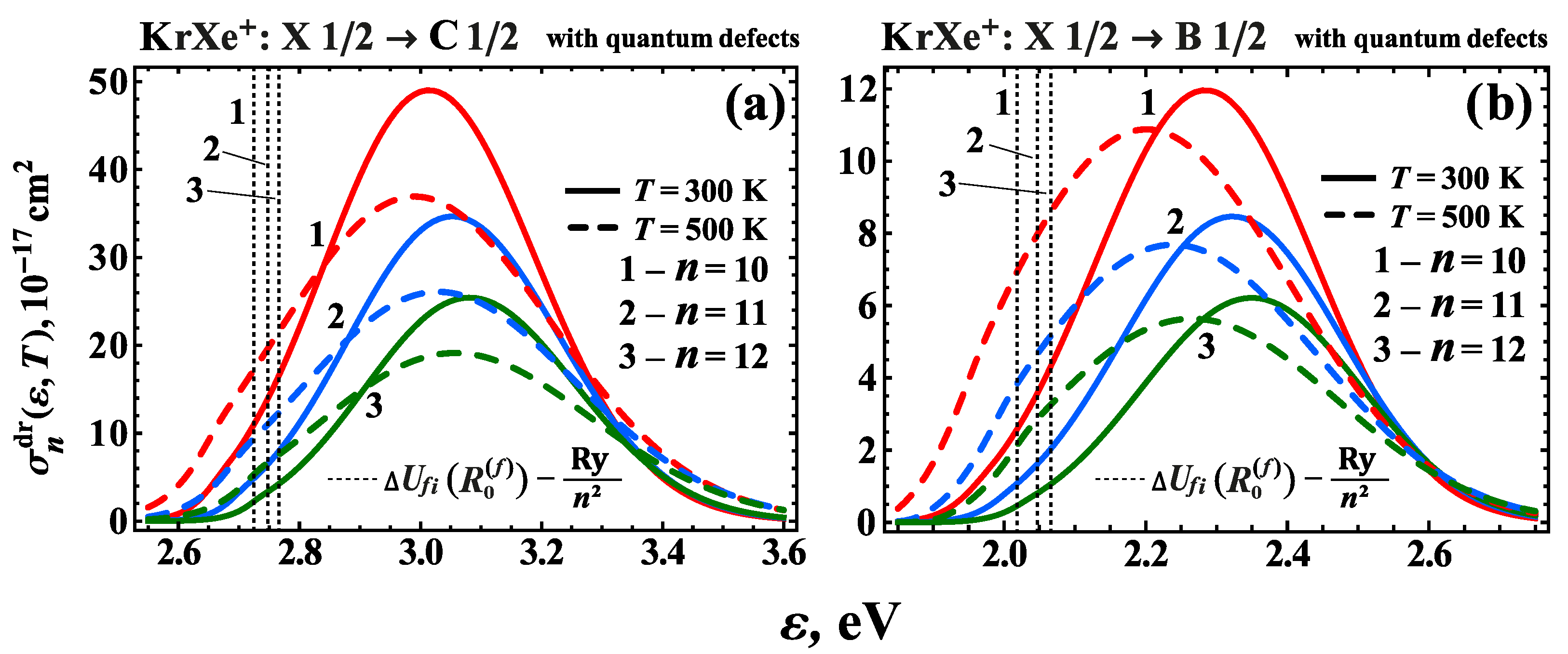
4.3. Dissociative Recombination of RgXe Ions via Transitions to Charge Transfer States
5. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| VUV | Vacuum ultraviolet |
| IR | infrared |
| OPRGL | Optically pumped rare gas lasers |
| CT | Charge transfer |
| MQDT | Multi-Channel Quantum Defect Theory |
| DR | Dissociative recombination |
| DE | Dissociative excitation |
References
- Cooley, J.E.; Urdahl, R.; Xue, J.; Denning, M.; Tian, P.; Kushner, M.J. Properties of microplasmas excited by microwaves for VUV photon sources. Plasma Sources Sci. Technol. 2015, 24, 065009. [Google Scholar] [CrossRef]
- Park, S.-J.; Herring, C.M.; Mironov, A.E.; Cho, J.H.; Eden, J.G. 25 W of average power at 172 nm in the vacuum ultraviolet from flat, efficient lamps driven by interlaced arrays of microcavity plasmas. APL Photonics 2017, 2, 041302. [Google Scholar] [CrossRef]
- Saidi, S.; Loukil, H.; Khodja, K.; Belasri, A.; Caillier, B.; Guillot, P. Experimental and Theoretical Investigations of Dielectric Barrier Discharge (DBD) Lamp in Ne/Xe Mixture. IEEE Trans. Plasma. Sci. 2022, 50, 2147–2155. [Google Scholar] [CrossRef]
- Kim, H.; Hopwood, J. Scalable microplasma array for argon metastable lasing medium. J. Appl. Phys. 2019, 126, 163301. [Google Scholar] [CrossRef]
- Qu, C.; Tian, P.; Semnani, A.; Kushner, M.J. Properties of arrays of microplasmas: Application to control of electromagnetic waves. Plasma Sources Sci. Technol. 2017, 26, 105006. [Google Scholar] [CrossRef]
- Emmons, D.J.; Weeks, D.E.; Eshel, B.; Perram, G.P. Metastable Ar(1s5) density dependence on pressure and argon-helium mixture in a high pressure radio frequency dielectric barrier discharge. J. Appl. Phys. 2018, 123, 043304. [Google Scholar] [CrossRef]
- Sun, P.; Zuo, D.; Mikheyev, P.A.; Han, J.; Heaven, M.C. Time-dependent simulations of a CW pumped, pulsed DC discharge Ar metastable laser system. Opt. Express 2019, 27, 22289. [Google Scholar] [CrossRef]
- Kholin, I.V. High-power, high-pressure IR Ar-Xe lasers. Quantum Electron. 2003, 33, 129. [Google Scholar] [CrossRef]
- Han, J.; Heaven, M.C. Gain and lasing of optically pumped metastable rare gas atoms. Opt. Lett. 2012, 37, 2157–2159. [Google Scholar] [CrossRef]
- Gerasimov, G.N. Optical spectra of binary rare-gas mixtures. Phys.–Usp. 2004, 47, 149–168. [Google Scholar] [CrossRef]
- Bhoj, A.N.; Kushner, M.J. Avalanche process in an idealized lamp: II. Modelling of breakdown in Ar/Xe electric discharges. J. Phys. D Appl. Phys. 2004, 37, 2510–2526. [Google Scholar] [CrossRef]
- Emmons, D.J.; Weeks, D.E. Kinetics of high pressure argon-helium pulsed gas discharge. J. Appl. Phys. 2017, 121, 203301. [Google Scholar] [CrossRef]
- Viehl, L.A.; Gray, B.R.; Wright, T.G. Interactions of rare gas cations with lighter rare gas atoms. Mol. Phys. 2010, 108, 547–555. [Google Scholar]
- Zehnder, O.; Merkt, F. The low-lying electronic states of ArXe+ and their potential energy functions. J. Chem. Phys. 2008, 128, 014306. [Google Scholar] [CrossRef]
- Bardsley, J.N.; Biondi, M.A. Dissociative Recombination. In Advances in Atomic and Molecular Physics; Bates, D.R., Esterman, I., Eds.; Academic Press: New York, NY, USA; London, UK, 1970; Volume 6, pp. 1–57. [Google Scholar]
- Millet, P.; Barrie, A.M.; Birot, A.; Brunet, H.; Dijols, H.; Galy, J.; Salamero, Y. Kinetic study of (ArKr)+ and (ArXe)+ heteronuclear ion emissions. J. Phys. B At. Mol. Phys. 1981, 14, 459. [Google Scholar] [CrossRef]
- Tsuji, M.; Tanaka, M.; Nishimura, Y. New emission spectra of KrXe+ produced from Kr afterglow reactions of Xe. Chem. Phys. Lett. 1996, 262, 349–354. [Google Scholar] [CrossRef]
- Khasenov, M. Emission and level population in noble gases and their binary mixtures under ion beam excitation. Nucl. Instrum. Methods Phys. Res. B 2020, 482, 45–52. [Google Scholar] [CrossRef]
- Samarkhanov, K.; Khasenov, M.; Batyrbekov, E.; Kenzhina, I.; Sapatayev, Y.; Bochkov, V. Emission of Noble Gases Binary Mixtures under Excitation by the Products of the 6Li (n,α)3H Nuclear Reaction. Sci. Technol. Nucl. Install. 2020, 2020, 8891891. [Google Scholar]
- Lebedev, V.S.; Kislov, K.S.; Narits, A.A. Rydberg states population via three-body and dissociative recombination in low-temperature plasmas of rare gas mixtures. Plasma Sources Sci. Technol. 2020, 29, 025002. [Google Scholar] [CrossRef]
- Lebedev, V.S.; Kislov, K.S.; Narits, A.A. Resonant electron capture by ions into Rydberg states of atoms. J. Exp. Theor. Phys. 2020, 130, 483–498. [Google Scholar] [CrossRef]
- Narits, A.A.; Kislov, K.S.; Lebedev, V.S. Semiclassical theory of resonant dissociative excitation of molecular ions by electron impact. J. Phys. B At. Mol. Opt. Phys. 2020, 53, 195201. [Google Scholar] [CrossRef]
- Hausamann, D.; Morgner, H. The heteronuclear rare gas ions: A simple model for the determination of the potential curves. Mol. Phys. 1985, 54, 1085–1099. [Google Scholar] [CrossRef]
- Narits, A.A.; Kislov, K.S.; Lebedev, V.S. Continuous absorption and emission of light by the heteronuclear rare gas (ArXe)+ and (KrXe)+ ions. J. Chem. Phys. 2022, 157, 204307. [Google Scholar] [CrossRef]
- Stroömholmm, C.; Semaniak, J.; Rosén, S.; Danared, H.; Datz, S.; van der Zande, W.; Larsson, M. Dissociative recombination and dissociative excitation of 4HeH+: Absolute cross sections and mechanisms. Phys. Rev. A 1996, 54, 3086–3094. [Google Scholar] [CrossRef] [PubMed]
- Lecointre, J.; Jureta, J.J.; Urbain, X.; Defrance, P. Electron-impact dissociation of HeH+: Absolute cross sections for the production of Heq+ (q = 1–2) fragments. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 015203. [Google Scholar] [CrossRef]
- Scarlett, L.H.; Zammit, M.C.; Bray, I.; Schneider, B.I.; Fursa, D.V. Convergent close-coupling calculations of electron scattering on HeH+. Phys. Rev A 2022, 106, 042818. [Google Scholar] [CrossRef]
- Demyanov, A.V.; Kochetov, I.V.; Mikheyev, P.A.; Azyazov, V.N.; Heaven, M.C. Kinetic analysis of rare gas metastable production and optically pumped Xe lasers. J. Phys. D Appl. Phys. 2018, 51, 045201. [Google Scholar] [CrossRef]
- Ohwa, M.; Moratz, T.J.; Kushner, M.J. Excitation mechanisms of the electron-beam-pumped atomic xenon (5d→6p) laser in Ar/Xe mixtures. J. Appl. Phys. 1989, 66, 5131. [Google Scholar] [CrossRef]
- Lebedev, V.S.; Presnyakov, L.P. Photodissociation from a manifold of rovibrational states and free–free absorption by a diatomic molecule. J. Phys. B At. Mol. Opt. Phys. 2002, 35, 4347. [Google Scholar] [CrossRef]
- Jungen, C. Molecular Applications of Quantum Defect Theory, 1st ed.; CRC Press: New York, NY, USA, 1996. [Google Scholar]
- Tennyson, J. Electron–molecule collision calculations using the R-matrix method. Phys. Rep. 2010, 491, 29–76. [Google Scholar] [CrossRef]
- Khamesian, M.; Ayouz, M.; Singh, J.; Kokoouline, V. Cross Sections and Rate Coefficients for Rotational Excitation of HeH+ Molecule by Electron Impact. Atoms 2018, 6, 49. [Google Scholar] [CrossRef]
- Mezei, Z.J.; Epée Epée, M.D.; Motapon, O.; Schneider, I.F. Dissociative Recombination of CH+ Molecular Ion Induced by Very Low Energy Electrons. Atoms 2019, 7, 82. [Google Scholar] [CrossRef]
- Ayouz, M.; Kokoouline, V. Cross Sections and Rate Coefficients for Rovibrational Excitation of HeH+ Isotopologues by Electron Impact. Atoms 2019, 7, 67. [Google Scholar] [CrossRef]
- Mezei, Z.J.; Chakrabarti, K.; Epée Epée, M.D.; Motapon, O.; Yuen, C.H.; Ayouz, M.A.; Douguet, N.; dos Santos, S.F.; Kokoouline, V.; Schneider, I.F. Electron-Induced Excitation, Recombination, and Dissociation of Molecular Ions Initiating the Formation of Complex Organic Molecules. ACS Earth Space Chem. 2019, 3, 2376–2389. [Google Scholar] [CrossRef]
- Zammit, M.C.; Fursa, D.V.; Savage, J.S.; Bray, I. Electron– and positron–molecule scattering: Development of the molecular convergent close-coupling method. J. Phys. B At. Mol. Opt. Phys. 2017, 50, 123001. [Google Scholar] [CrossRef]
- Rice, O.K. Predissociation and the crossing of molecular potential energy curves. J. Chem. Phys. 1933, 1, 375. [Google Scholar] [CrossRef]
- Lebedev, V.S. Ionization of Rydberg atoms by neutral particles. II. Mechanisms of the perturber-core scattering. J. Phys. B At. Mol. Opt. Phys. 1991, 24, 1993. [Google Scholar] [CrossRef]
- Kislov, K.S.; Narits, A.A.; Lebedev, V.S. Semiclassical Description of Radiative Processes Involving Heteronuclear Molecular and Quasimolecular Rare Gas Ions. J. Russ. Laser Res. 2022, 43, 556–578. [Google Scholar] [CrossRef]
- Larsson, M.; Orel, A.E. Dissociative Recombination of Molecular Ions; Cambridge University Press: New York, NY, USA, 2008. [Google Scholar]
- Bates, D.R. Super dissociative recombination. J. Phys. B At. Mol. Opt. Phys. 1991, 24, 703–709. [Google Scholar] [CrossRef]
- Pratt, S.T.; Jungen, C. The isotope dependence of dissociative recombination via the indirect mechanism. J. Chem. Phys. 2012, 137, 174306. [Google Scholar] [CrossRef]
- Du, N.Y.; Greene, C.H. Multichannel Rydberg spectra of the rare gas dimers. J. Chem. Phys. 1989, 90, 6347–6360. [Google Scholar] [CrossRef]
- Dehmer, P.M.; Pratt, S.T. Photoionization of ArKr, ArXe, and KrXe and bond dissociation energies of the rare gas dimer ions. J. Chem. Phys. 1982, 77, 4804–4817. [Google Scholar] [CrossRef]
- Sobelman, I.I. Atomic Spectra and Radiative Transitions; Springer: Berlin/Heidelberg, Germany, 1979. [Google Scholar]
- Lebedev, V.S.; Beigman, I.L. Physics of Highly Excited Atoms and Ions; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Sukhorukov, V.L.; Petrov, I.D.; Schäfer, M.; Merkt, F.; Ruf, M.-W.; Hotop, H. Photoionization dynamics of excited Ne, Ar, Kr and Xe atoms near threshold. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 092001. [Google Scholar] [CrossRef]
- Narits, A.A.; Kislov, K.S. Electronic Terms and Oscillator Strengths of ArXe+ and KrXe+ Molecular Cations. Bull. Lebedev Phys. Inst. 2022, 49, 366–372. [Google Scholar] [CrossRef]
- Neese, F. Software update: The ORCA program system—Version 5.0. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2022, 12, e1606. [Google Scholar] [CrossRef]
- Angeli, C.; Cimiraglia, R.; Evangelisti, S.; Leininger, T.; Malrieu, J.-P. Introduction of n-electron valence states for multireference perturbation theory. J. Chem. Phys. 2001, 114, 10252. [Google Scholar] [CrossRef]
- Marchenko, V.S. Dissociation of homonuclear ions by electron impact. Sov. Phys. JETP 1983, 58, 292–298. [Google Scholar]
- Zehnder, O.; Merkt, F. The low-lying electronic states of KrXe+ and their potential energy functions. Mol. Phys. 2008, 106, 1215–1226. [Google Scholar] [CrossRef]
- Berry, R.S.; Nielsen, S.E. Dynamic Coupling Phenomena in Molecular Excited States. II. Autoionization and Predissociation in H2, HD, and D2. Phys. Rev. A 1970, 1, 395–411. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Narits, A.; Kislov, K.; Lebedev, V. Electron-Impact Excitation and Dissociation of Heavy Rare Gas Heteronuclear Ions via Transitions to Charge Transfer States. Atoms 2023, 11, 60. https://doi.org/10.3390/atoms11030060
Narits A, Kislov K, Lebedev V. Electron-Impact Excitation and Dissociation of Heavy Rare Gas Heteronuclear Ions via Transitions to Charge Transfer States. Atoms. 2023; 11(3):60. https://doi.org/10.3390/atoms11030060
Chicago/Turabian StyleNarits, Alexander, Konstantin Kislov, and Vladimir Lebedev. 2023. "Electron-Impact Excitation and Dissociation of Heavy Rare Gas Heteronuclear Ions via Transitions to Charge Transfer States" Atoms 11, no. 3: 60. https://doi.org/10.3390/atoms11030060
APA StyleNarits, A., Kislov, K., & Lebedev, V. (2023). Electron-Impact Excitation and Dissociation of Heavy Rare Gas Heteronuclear Ions via Transitions to Charge Transfer States. Atoms, 11(3), 60. https://doi.org/10.3390/atoms11030060