Excitation of the 2P State of Atomic Hydrogen by Electron Impact
Abstract
1. Introduction
2. Calculations
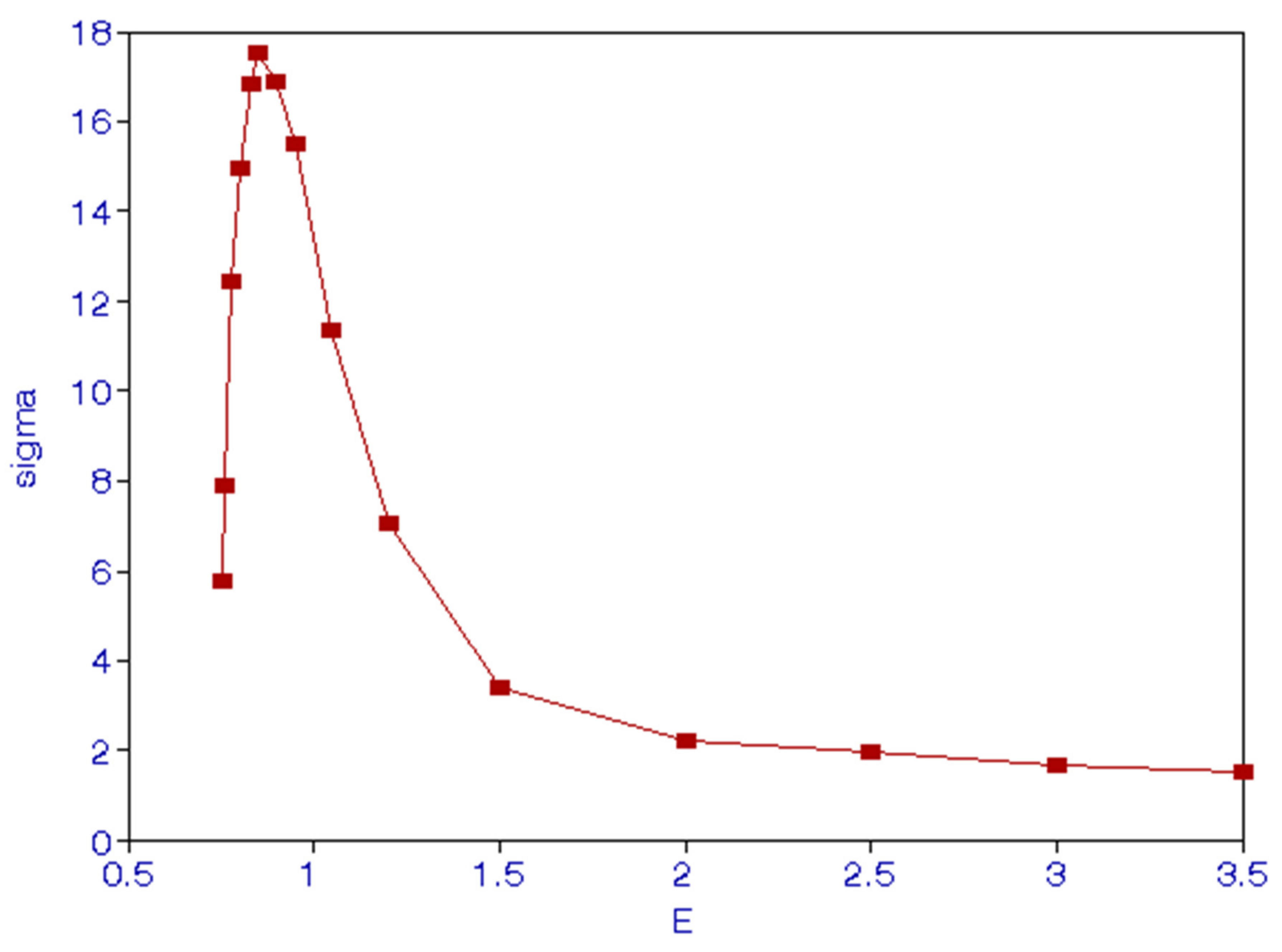
3. Results
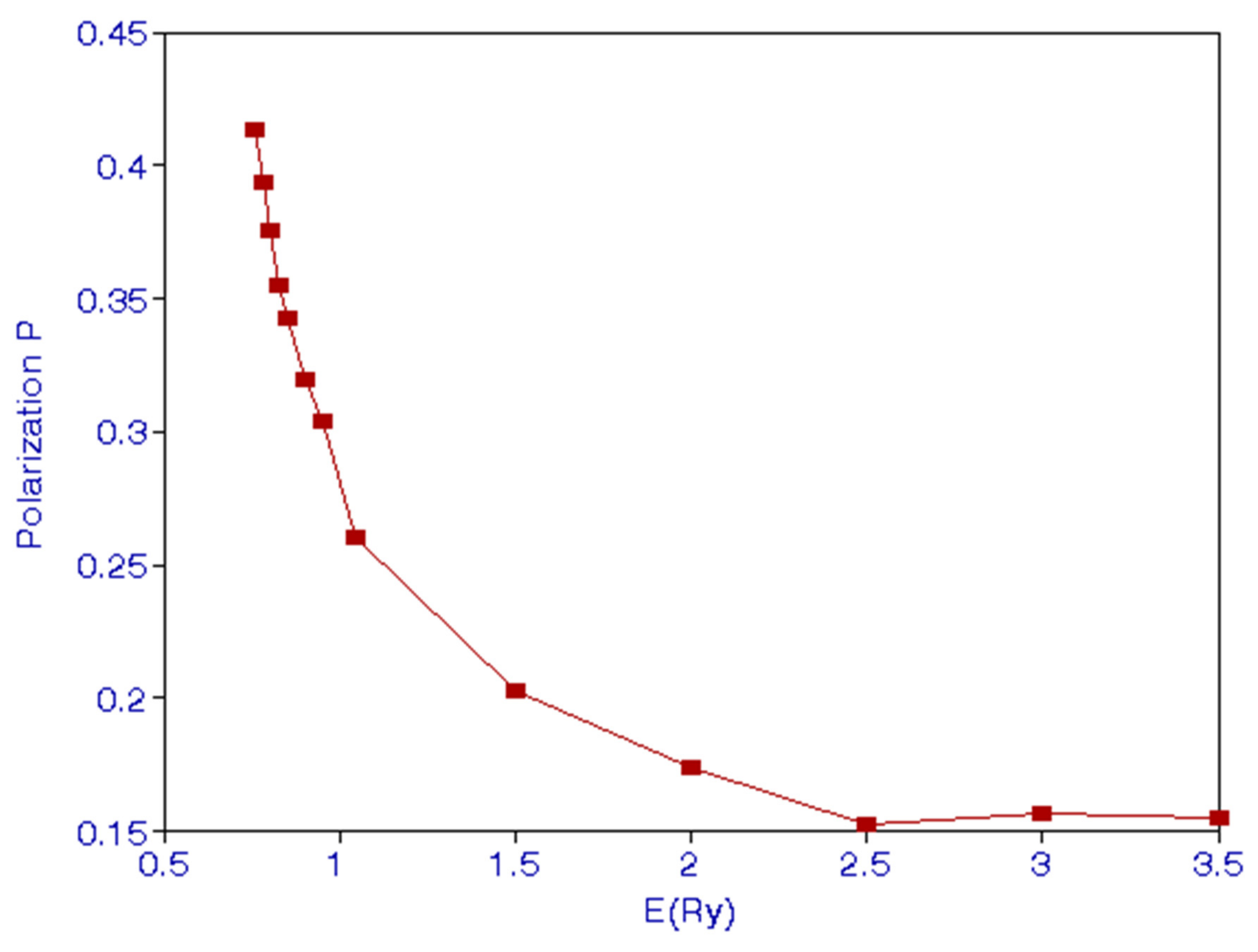
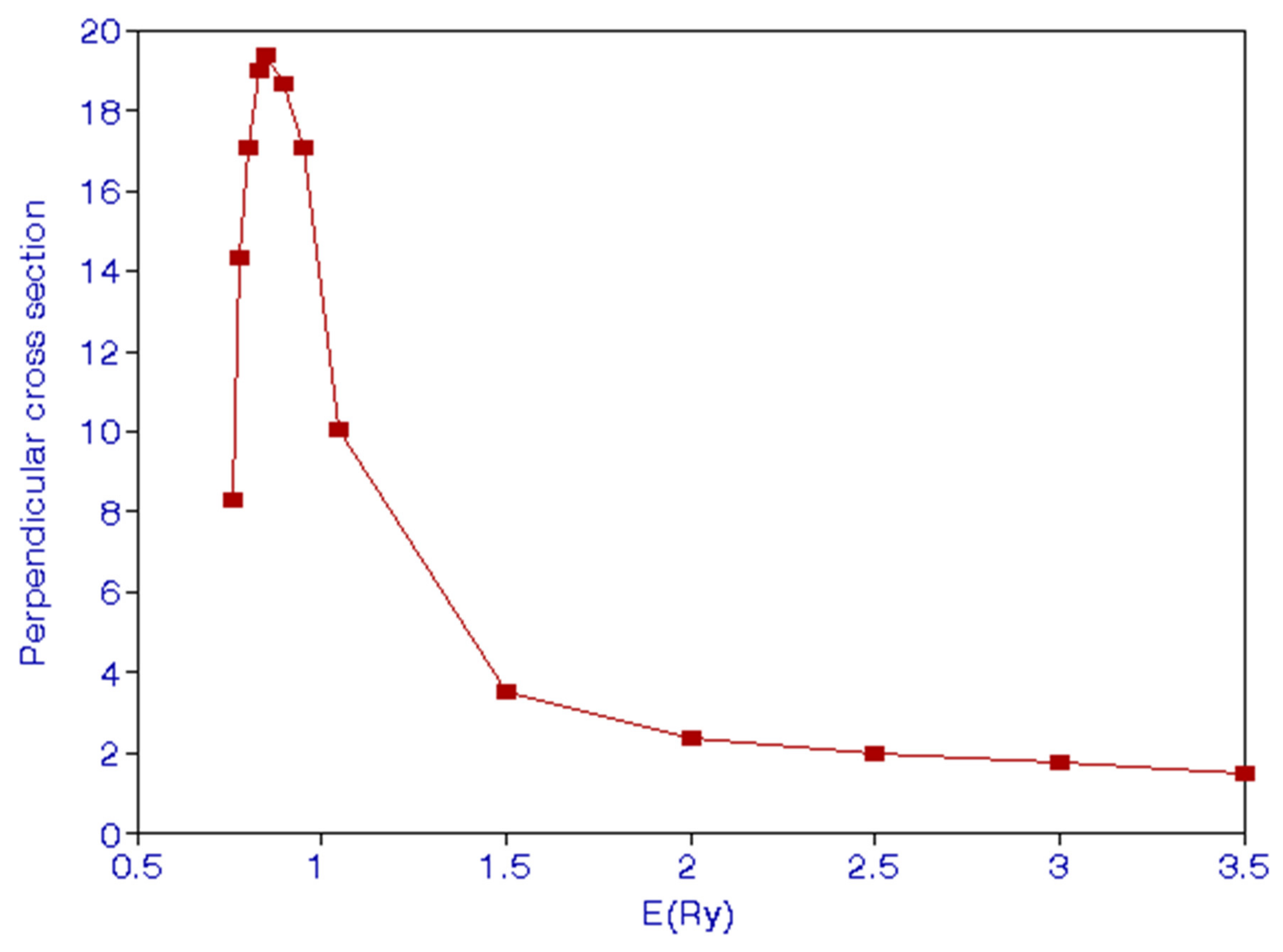
4. Polarization and Perpendicular Cross Section
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bhatia, A.K. Excitation of the 2S State of Atomic Hydrogen by Electron Impact. Atoms 2018, 6, 7. [Google Scholar] [CrossRef]
- Bhatia, A.K. Hybrid Theory of electron-hydrogen elastic scattering. Phys. Rev. A 2007, 75, 032713. [Google Scholar] [CrossRef]
- Bhatia, A.K. Positron-hydrogen scattering, annihilation, and positronium formation. Atoms 2016, 4, 27. [Google Scholar] [CrossRef]
- Bhatia, A.K. Application of P-wave hybrid theory to the scattering of electrons from He+ and resonances in He and H−. Phys. Rev. A 2012, 86, 032709. [Google Scholar] [CrossRef]
- Bhatia, A.K. Hybrid theory of P-wave electron-Li+ elastic scattering and photoabsorption in two-electron systems. Phys. Rev. A 2013, 87, 042705. [Google Scholar] [CrossRef]
- Burke, P.G.; Taylor, A.J.; Ormonde, A. Low energy electron scattering by atomic hydrogen III, comparison of theory and experiment for electron-induced excitation of hydrogen to the n =2 level. Proc. Phys. Soc. 1967, 92, 345. [Google Scholar] [CrossRef]
- Calhoun, R.V.; Madison, D.H.; Shelton, W.N. Excitation of the n =2 state of atomic hydrogen by electron impact in the distorted-wave approximation. Phys. Rev. A 1976, 14, 1380. [Google Scholar] [CrossRef]
- William, J.F. Electron-photon angular correlations from the electro-impact excitation of the 2s and 2p electronic configurations of atomic hydrogen. J. Phys. B 1981, 14, 1197. [Google Scholar] [CrossRef]
- Temkin, A. A note on the scattering of electrons from atomic hydrogen. Phys. Rev. 1959, 116, 358. [Google Scholar] [CrossRef]
- Shertzer, J.; Temkin, A. Direct calculation of the scattering effects. Phys. Rev. A 2006, 74, 052701. [Google Scholar] [CrossRef]
- Bhatia, A.K.; Temkin, A. A distorted-wave methodology of electron-ion impact excitation. J. Phys. B 1977, 10, 2893. [Google Scholar] [CrossRef]
- Wigner, E.P. On the behavior of cross section near threshold. Phys. Rev. 1948, 73, 1002. [Google Scholar] [CrossRef]
- Sadeghpour, H.R.; Cavagnero, M.J.; Esry, B.D.; Fabrikant, I.T.; Macek, J.H.; Rau, A.R.P. Collisions near threshold in atomic and molecular physics. J. Phys. B 2000, 33, R93. [Google Scholar] [CrossRef]
- Perceval, I.C.; Seaton, M.J. The polarization of atomic line radiation excited by electron impact. Phil. Trans. R. Soc. A 1958, 251, 113–138. [Google Scholar]
- James, G.K.; Slevin, J.A.; Dziczek, D.; McConkey, J.W.; Bray, I. Polarization of Lyman radiation from atomic hydrogen excited by electron impact from near threshold to 188 eV. Phys. Rev. A 1998, 57, 1787. [Google Scholar] [CrossRef]
- Bhatia, A.K. Excitation of the nS states of atomic hydrogen by electron impact, excitation rate coefficients, and phase shifts: Comparison with positron impact excitation. Atoms 2022, 10, 5. [Google Scholar] [CrossRef]

| E | E | ||
|---|---|---|---|
| 0.755 | 5.808 | 1.05 | 11.366 |
| 0.76 | 7.623 | 1.2 | 7.062 |
| 0.78 | 12.457 | 1.5 | 3.418 |
| 0.80 | 14.947 | 2.0 | 2.230 |
| 0.83 | 16.830 | 2.5 | 1.959 |
| 0.85 | 17.522 | 3.0 | 1.700 |
| 0.90 | 16.878 | 3.5 | 1.543 |
| 0.95 | 15.474 |
| Lm/E | 0.755 | 0.76 | 0.78 | 0.80 | 0.83 | 0.85 | 0.90 | 0.95 | 1.05 | 1.2 |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 5.646 | 7.187 | 10.564 | 11.604 | 11.602 | 11.133 | 9.943 | 7.708 | 5.017 | 2.658 |
| 2 | 5.807 | 7.622 | 12.433 | 14.936 | 16.718 | 17.102 | 16.515 | 14.850 | 10.934 | 6.325 |
| 3 | 5.808 | 7.623 | 12.437 | 14.946 | 16.829 | 17.515 | 16.768 | 15.420 | 11.230 | 6.745 |
| 4 | 12.457 | 14.947 | 16.830 | 17.522 | 16.878 | 15.465 | 11.335 | 6.949 | ||
| 5 | 15.474 | 11.366 | 7.031 | |||||||
| 6 | 7.062 |
| Lm/E | 1.50 | 2.00 | 2.50 | 3.0 | 3.5 |
|---|---|---|---|---|---|
| 1 | 0.947 | 0.289 | 0.127 | 0.069 | 0.046 |
| 2 | 2.218 | 0.611 | 0.270 | 0.155 | 0.104 |
| 3 | 2.704 | 0.998 | 0.628 | 0.339 | 0.231 |
| 4 | 3.044 | 1.376 | 0.950 | 0.593 | 0.427 |
| 5 | 3.235 | 1.661 | 1.240 | 0.853 | 0.648 |
| 6 | 3.334 | 1.851 | 1.467 | 1.078 | 0.856 |
| 7 | 3.383 | 1.972 | 1.631 | 1.258 | 1.034 |
| 8 | 3.407 | 2.077 | 1.747 | 1.395 | 1.178 |
| 9 | 3.418 | 2.148 | 1.826 | 1.498 | 1.292 |
| 10 | 2.196 | 1.881 | 1.573 | 1.381 | |
| 11 | 2.229 | 1.918 | 1.628 | 1.449 | |
| 12 | 2.239 | 1.941 | 1.670 | 1.502 | |
| 13 | 1.959 | 1.700 | 1.543 |
| E | P | E | P |
|---|---|---|---|
| 0.755 | 0.4218 | 0.95 | 0.3040 |
| 0.76 | 0.4136 | 1.05 | 0.2647 |
| 0.78 | 0.3935 | 1.50 | 0.2031 |
| 0.80 | 0.3757 | 2.00 | 0.1741 |
| 0.83 | 0.3544 | 2.50 | 0.1521 |
| 0.85 | 0.3428 | 3.00 | 0.1566 |
| 0.90 | 0.3200 | 3.50 | 0.1547 |
| E | E | ||
|---|---|---|---|
| 0.755 | 6.339 | 0.95 | 17.020 |
| 0.76 | 8.286 | 1.05 | 10.033 |
| 0.78 | 14.285 | 1.50 | 3.524 |
| 0.80 | 17.055 | 2.00 | 2.348 |
| 0.83 | 18.949 | 2.50 | 1.993 |
| 0.85 | 19.342 | 3.00 | 1.793 |
| 0.90 | 18.660 | 3.50 | 1.576 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhatia, A.K. Excitation of the 2P State of Atomic Hydrogen by Electron Impact. Atoms 2022, 10, 113. https://doi.org/10.3390/atoms10040113
Bhatia AK. Excitation of the 2P State of Atomic Hydrogen by Electron Impact. Atoms. 2022; 10(4):113. https://doi.org/10.3390/atoms10040113
Chicago/Turabian StyleBhatia, Anand K. 2022. "Excitation of the 2P State of Atomic Hydrogen by Electron Impact" Atoms 10, no. 4: 113. https://doi.org/10.3390/atoms10040113
APA StyleBhatia, A. K. (2022). Excitation of the 2P State of Atomic Hydrogen by Electron Impact. Atoms, 10(4), 113. https://doi.org/10.3390/atoms10040113






