Mission Target: Tetraquark Mesons of Flavour-Cryptoexotic Type

Abstract

Currently, flavour-cryptoexotic tetraquarks form the most common sort of all experimentally established exotic multiquark hadrons. This note points out a few promising concepts that should help improve theoretical (but, for several reasons, not quite straightforward) analyses of this kind of states; among others, their scope of application encompasses the strong interactions in the limit of (arbitrarily) large numbers of colours, and equally analytical and nonperturbative approaches to multiquark states.

1. Species of Bound States within Quantum Chromodynamics: The Crucial Diverseness

Within the framework of theoretical elementary particle physics, strong interactions are described, at the presently most fundamental level, by quantum chromodynamics (QCD), a renormalizable relativistic quantum field theory invariant under local gauge transformations constituting representations of SU(3), the non-Abelian, compact, special unitary Lie group of degree three. Presumably out of sheer desperation, the degree of freedom introduced by this gauge symmetry has been called colour; that naming is reflected by the related quantum field theory, QCD. The two sorts of dynamical degrees of freedom of QCD transform according to irreducible representations of SU(3): the massless (spin-1) vector gauge bosons called gluons necessarily according to the eight-dimensional adjoint representation of SU(3), and any of its spin-$\frac{1}{2}$ fermions subsumed under the notion of “quarks” according to the three-dimensional fundamental representation of SU(3). The different members of the class of quarks—below labelled $q_a$—are discriminated by their flavour quantum number, $a\in \{u,d,s,c,b,t(,\dots ?\left)\right\}$. Consequently, the strong coupling $g_{\mathrm{s}}$ governs both the interactions of three or four gluons among each other and the couplings of gluons to quarks, and, because of its usually pairwise occurrence, frequently enters analyses under the disguise of a strong fine-structure coupling:

$${\alpha }_{\mathrm{s}}\equiv \frac{g_{\mathrm{s}}^2}{4\pi }.$$

(1)

This coupling and the quark masses $m_a$ constitute the totality of basic parameters of QCD.

The mathematical structure of QCD as a gauge theory is, comparatively, simple: an even unbroken gauge symmetry based on a simple albeit non-Abelian group. Nevertheless, QCD produces various peculiar phenomena. Most prominent among these is the confinement of all colour degrees of freedom: the dynamical degrees of freedom of QCD, its quarks and gluons, each carrying some definitely nonzero amount of colour, are not experimentally accessible in an isolated form. In fact, exclusively those of their bound states that transform under the gauge group as singlets, collectively subsumed under the name of hadrons, are directly observable.1 On conceptual grounds, these hadron states are to be separated into two disjoint subdivisions:

• The ordinary quark–antiquark mesons and three-quark baryons are called conventional.
• All other (hence, non-conventional) types of hadrons—multiquark states, quark–gluon hybrid mesons, totally gluonic glueballs—are captured by the notion of exotic hadrons.

Needless to stress, it would be desirable if the suspected (exotic) multiquark nature of an experimentally detected state could be straightforwardly decided already from its apparently non-conventional number of different quark flavours. This fortunate coincidence, however, may only happen if, for strictly none of the quarks and antiquarks constituting the candidate multiquark state under investigation, its specific flavour is counterbalanced by the respective antiflavour. Otherwise, the multiquark will not be able to exhibit this particular flavour. The precise identification of all the states where such compensations do take place is provided by

Definition 1.

A multiquark hadron is termed flavour-cryptoexotic if it does not exhibit more open quark flavours than the corresponding category of conventional hadrons, that is, trivially, at most two open quark flavours in the case of meson states or three open quark flavours in the case of baryon states.

Now, for every multiquark exotic hadron of the flavour-cryptoexotic type, its net overall content of quark flavour not counterbalanced by an antiflavour is, by Definition 1, necessarily identical to that of its certainly existing counterpart(s) among the set of conventional hadrons. As a consequence thereof, in an investigation of flavour-cryptoexotic hadron states, there arise (additional) complications that are not encountered in any analysis of multiquark states built from quarks and antiquarks carrying totally disparate quark flavours. Namely, for matching spin and parity quantum numbers, the flavour-cryptoexotic hadron can and, quite generally, will undergo mixing with its appropriate conventional counterpart; this circumstance should be taken into account in comprehensive descriptions of the former hadron (cf., e.g., Section 3).

The category of multiquark states with the lowest number of constituents is formed by the totality of tetraquark mesons, generically identified by T: bound states of two quarks $q_b,q_d$ and two antiquarks ${\overline{q}}_a,{\overline{q}}_c$ with flavour quantum numbers $a,b,c,d\in \{u,d,s,c,b\}$ and masses $m_a,m_b,m_c,m_d$. Utilising Definition 1, let us put the focus on their flavour-cryptoexotic variant:

$$T=\left[{\overline{q}}_a{q}_b{\overline{q}}_b{q}_c\right],a,b,c\in \{u,d,s,c,b\}.$$

(2)

The (presumably not surprising) motivation for this choice is predicated on the circumstance that the subset of flavour-cryptoexotic tetraquarks (2) constitutes that sort of multiquarks for which (at present) the largest count of candidate hadrons has been observed by experiment [1]. In order to pave the way towards (eventually optimised) approaches to flavour-cryptoexotic tetraquarks, it appears to be worthwhile to collect, in a systematic manner, various concepts, notions, or pieces of information, both proposed and “lurking around” [2,3,4,5,6,7,8] (Section 2, Section 3 and Section 4).

Table 1. Flavour-cryptoexotic tetraquarks (2) distinguished by different and open quark-flavour content $a\ne b\ne c$: open flavour means any flavour not compensated by its antiflavour; distilled from Refs. [7,9].

Number of Different	Quark Composition	Number of Open
Quark Flavours Involved	${\overline{\mathit{q}}}_{\square }{\mathit{q}}_{\square }{\overline{\mathit{q}}}_{\square }{\mathit{q}}_{\square }$	Quark Flavours Involved
3	${\overline{q}}_a{q}_b{\overline{q}}_b{q}_c$	2
	${\overline{q}}_a{q}_b{\overline{q}}_c{q}_c$	2
2	${\overline{q}}_a{q}_a{\overline{q}}_a{q}_b$	2
	${\overline{q}}_a{q}_a{\overline{q}}_b{q}_a$	2
	${\overline{q}}_a{q}_b{\overline{q}}_b{q}_a$	0
	${\overline{q}}_a{q}_a{\overline{q}}_b{q}_b$	0
1	${\overline{q}}_a{q}_a{\overline{q}}_a{q}_a$	0

categorises the conceivable configurations of all flavour-cryptoexotic tetraquarks. Simplicity suggests to adopt, for illustration, states that involve three mutually different quark flavours, i.e., two open flavours and a compensation of a different flavour and its antiflavour:

$$T=\left[{\overline{q}}_a{q}_b{\overline{q}}_b{q}_c\right],a,b,c\in \{u,d,s,c,b\},a\ne b\ne c.$$

(3)

2. Multiquark-Phile Four-Point Correlation Functions of Hadron Interpolating Operators

Within the framework of QCD, productive contact between all coloured (thus, confined and not directly accessible experimentally) dynamical degrees of freedom of QCD, on the one hand, and the colourless (hence, experimentally observable) hadrons, on the other hand, can be established by means of the tool labelled hadron interpolating operators. By construction, (a candidate for) any such hadron interpolating operator is an (inevitably gauge-invariant) local operator, defined in terms of the quark and gluon fields, carrying the same flavour, spin, and parity quantum numbers as the hadron under investigation, and exhibiting overlap with the hadron, proven by its nonzero matrix element between the hadron state and the QCD vacuum.

For the subsequent discussions, neither spin nor parity degrees of freedom appear to be of utmost importance. Thus, upon suppressing Dirac matrices and Lorentz structures, in the following all reference to spin and parity in a hadron interpolating operator may be dropped. For the interpolating operator of a conventional meson composed of an antiquark of flavour a and a quark of flavour b, the (generic) quark–antiquark bilinear-current form may be chosen:

$$j_{\overline{a}b}\left(x\right)\equiv {\overline{q}}_a\left(x\right)q_b\left(x\right).$$

(4)

Within quantum field theories, information about bound states of some basic degrees of freedom may be deduced from the contributions of the bound states, in form of intermediate states, to scattering processes of the potential bound-state constituents. The related scattering amplitudes encoding this information can be derived from appropriate correlation functions of the particles involved in these scattering reactions. An (n-point) correlation function is, by definition, the vacuum expectation value of the (time-ordered) product of the field operators of the ($n=2,3,\dots$) particles under consideration. (Below, the time-ordering prescription is indicated by the symbol T, whereas the respective vacuum states are notationally reduced to their mere state brackets.) Upon truncation of the propagators of all external particles, any scattering amplitudes sought result as the on-mass-shell limits of the correlation functions.

For its application to the tetraquark mesons, the (standard) procedure for the extraction of bound-state properties sketched above necessitates an inspection of scattering amplitudes formalising scattering reactions of two conventional mesons into two conventional mesons. Scattering amplitudes for reactions of this kind may receive intermediate-state contributions of some tetraquark mesons. These bound states would reveal their existence by contributing in the form of intermediate-state poles. Now, following the above procedures, the scattering amplitudes can be derived from the associated four-point correlation functions. In these, the conventional mesons undergoing scattering may enter (from the aspect of QCD) by means of the interpolating currents (4). Accordingly, leaving aside, for a moment, all details related to the involved quark flavours $a,b,c,d$, approaching the features of tetraquark mesons requires coping with generic four-point correlation functions of quark–antiquark bilinear currents (4):

$$〈\mathrm{T}\left(j\left(y\right)j\left(y^{\prime }\right)j^{†}\left(x\right)j^{†}\left(x^{\prime }\right)\right)〉.$$

(5)

Evidently, the first and primary goal of all of the analyses envisaged above has to be the both unique and unambiguous identification of those contributions to four-point correlation functions (5) that might support the development of an intermediate-state pole interpretable as being related to an exotic tetraquark state. This task may be accomplished by, for instance, identifying and, subsequently, discarding all those contributions of QCD origin that certainly exhibit no relationship to any tetraquark state. To this end, by use of the Mandelstam variable

$$s\equiv {(p_1+p_2)}^2={(q_1+q_2)}^2$$

(6)

(fixed by either the external momenta of the initial state $p_1,p_2$ or the external momenta of the final state $q_1,q_2$), a simple but rigorous, maybe also useful, criterion [2,4] has been formulated:

Proposition 1.

A QCD-level contribution to a four-point correlation function (5) is considered to be potentially capable of supporting the formation of intermediate-state tetraquark poles and is labelled as tetraquark-phile [3,10] if, as a function of the Mandelstam variable s, it exhibits a nonpolynomial dependence on s and develops a four-quark intermediate-state branch cut starting at the branch point

$$\widehat{s}\equiv {(m_a+m_b+m_c+m_d)}^2.$$

(7)

This simple selection criterion, inherited from or inspired by the situation encountered in the class of conventional mesons, has to (and may only) be applied order by order of perturbation theory: Proposition 1 constitutes a purely perturbative tool. Nonperturbatively, a tetraquark is expected to betray its actual presence by the development of an (in general, complex) pole.

For a specific (perturbative) QCD contribution to a correlation function (5), the question of the presence or absence of a four-quark s-channel branch cut can be decided systematically: its existence may unambiguously be verified or excluded by consulting, i.e., more frankly, by solving the relevant set of Landau equations [11]. Examples of the application of the Landau equations have been given, also for the flavour-cryptoexotic states (3), by References [4,8,12].

When reinstalling, in the four-point correlation function (5), the quark-flavour indices of its conventional-meson interpolating currents (4), one notices that, with respect to the flavour arrangements in its initial and final states, for the flavour content of any flavour-cryptoexotic tetraquark of the sort (3), there exist two different conceivable configurations, highlighted by

Definition 2.

A (by Definition 1) flavour-cryptoexotic correlation function (5) is labelled as follows:

• flavour-preserving [5] if the incoming and outgoing quark-flavour distributions are identical;
• flavour-rearranging [5] if the incoming and outgoing quark-flavour distributions do not agree.

In principle, in theoretical tetraquark analyses (such as those touched on in Section 3 and Section 4), the necessity of these subdivisions of the correlation functions (5) into two disjoint categories has to be taken into account wherever applicable.2 Consequently, for the flavour-cryptoexotic objects of present desire, a split discussion of two types of correlation functions is in order:

$$\begin{array}{ccc}& 〈\mathrm{T}\left(j_{\overline{a}b}\left(y\right)j_{\overline{b}c}\left(y^{\prime }\right)j_{\overline{a}b}^{†}\left(x\right)j_{\overline{b}c}^{†}\left(x^{\prime }\right)\right)〉,〈\mathrm{T}\left(j_{\overline{a}c}\left(y\right)j_{\overline{b}b}\left(y^{\prime }\right)j_{\overline{a}c}^{†}\left(x\right)j_{\overline{b}b}^{†}\left(x^{\prime }\right)\right)〉,& \hfill \end{array}$$

(8)

$$\begin{array}{ccc}& 〈\mathrm{T}\left(j_{\overline{a}b}\left(y\right)j_{\overline{b}c}\left(y^{\prime }\right)j_{\overline{a}c}^{†}\left(x\right)j_{\overline{b}b}^{†}\left(x^{\prime }\right)\right)〉.& \hfill \end{array}$$

(9)

The decisive move towards a discussion of the flavour-cryptoexotic tetraquarks (2) that complies with their exotic nature is the identification of those contributions to the four-point correlation functions (5) that belong to the tetraquark-phile type requested by Proposition 1. For the case of the three-flavour tetraquarks (3) adopted for illustration, the crucial four-point correlation functions are precisely those of the form (8) and (9). The systematic and thorough scrutiny for their tetraquark-phile contributions may be accomplished, order by order, in the (perturbative) expansion of the four-point correlation functions (5) with respect to the strong coupling (1), by application of the (analytic) tool provided by the Landau equations [11]. For lower-order flavour-preserving cases, this can be achieved by a mere visual inspection: their separability either is obvious or arises from the vanishing trace of all the generators of SU(3).

For the flavour-cryptoexotic tetraquarks (3), the overall outcome [2,4] of these studies is that, for both the flavour-rearranging quark distribution (9) and the flavour-preserving quark distributions (8), the tetraquark-phile contributions to the four-point correlation function (5) cannot show up at a lower than the second order of the strong coupling (1). In other words, all the tetraquark-phile contributions are, necessarily, at least of the order $O\left({\alpha }_{\mathrm{s}}^2\right)$, corresponding to two internal gluon exchanges of the appropriate topology, of course, or even higher ones.3 Insights of this kind prove to be of utmost importance for the exploitation of such correlation functions in Section 3 and Section 4. 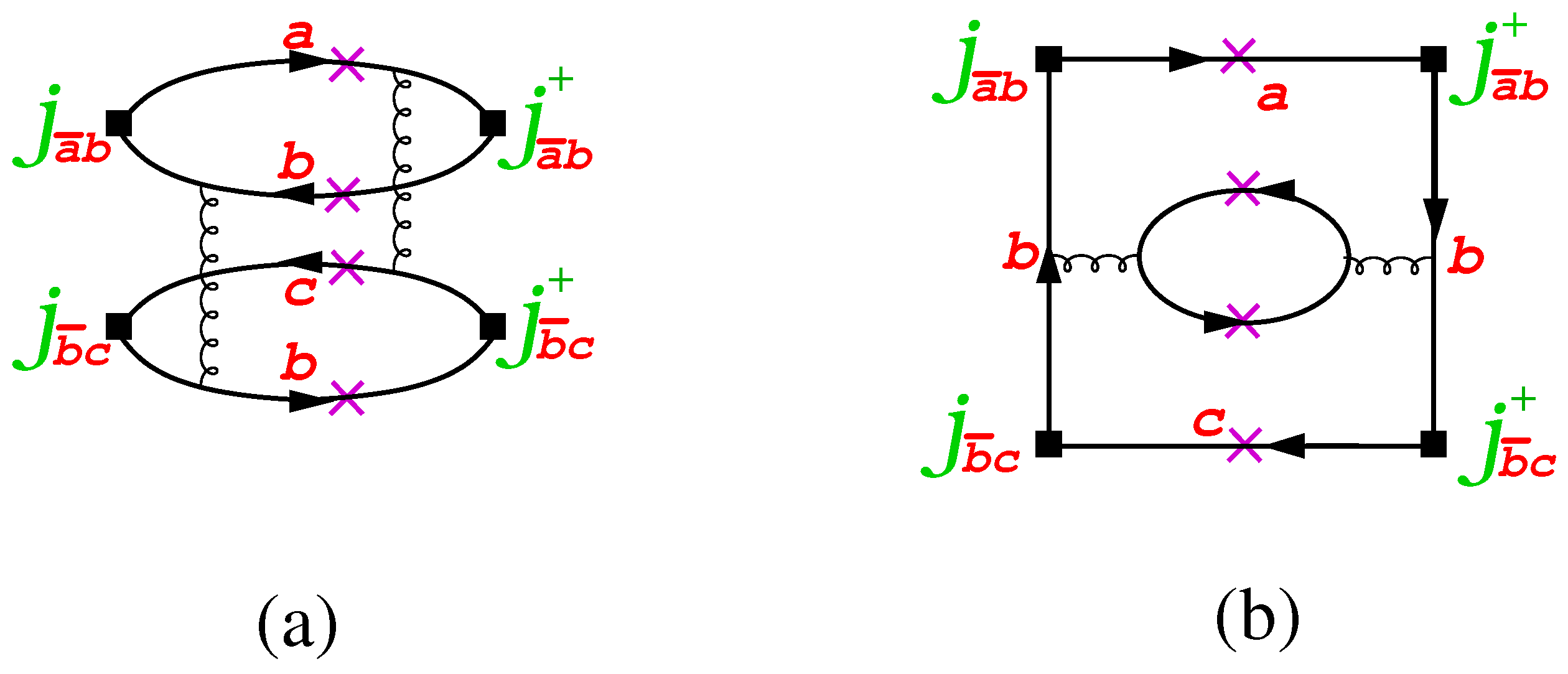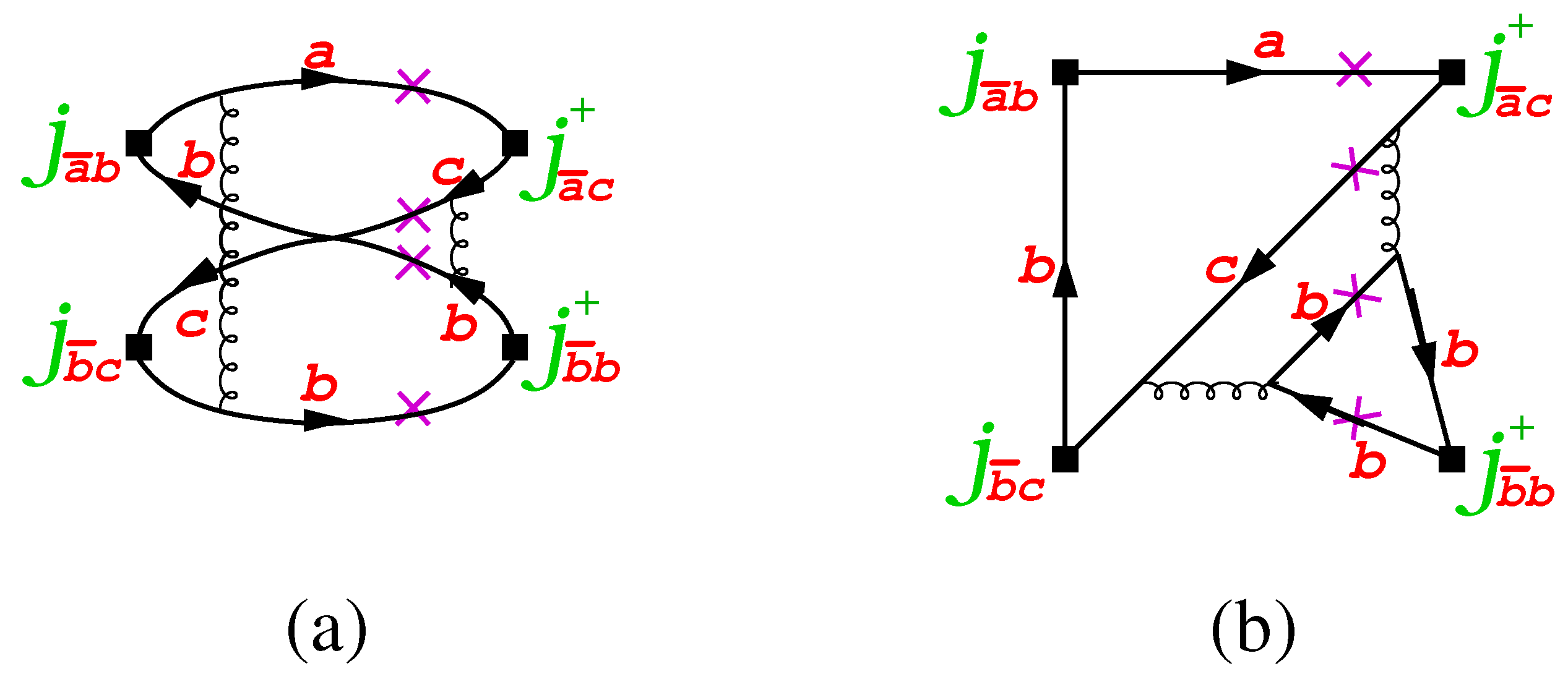For the two types (8) and (9) of flavour-cryptoexotic four-point correlation functions, some tetraquark-phile contributions of lowest order $O\left({\alpha }_{\mathrm{s}}^2\right)$ illustrating their conceivable topology are exemplified, for both varieties of flavour-preserving correlation functions (8), by Figure 1 and, for all flavour-rearranging correlation functions (9), by Figure 2.

3. Increase without Bound of the Number of Colours Entails Useful Qualitative Insights

In order to diminish the obstacles posed by the complexity of QCD to all the attempts of working out its implications exactly, already almost 50 years ago, ’t Hooft [13,14] suggested to generalise the gauge group of QCD, SU(3), to SU($N_{\mathrm{c}}$), the non-Abelian, special, unitary Lie group of degree $N_{\mathrm{c}}$. Within the class of gauge theories thus defined, the simplification may then be achieved by considering the limit of the number of colour degrees of freedom, $N_{\mathrm{c}}$, increasing to infinity, $N_{\mathrm{c}}\to \infty$, and the relevant expansion thereabout, in powers of $1/N_{\mathrm{c}}$. Keeping the large-$N_{\mathrm{c}}$ limit under control demands the product$N_{\mathrm{c}}{\alpha }_{\mathrm{s}}$ of the number of colours and the strong fine-structure coupling (1) to remain finite for $N_{\mathrm{c}}\to \infty$; this can be assured by postulating for the strong-interaction strength, that is, for either the strong coupling $g_{\mathrm{s}}$ or the strong fine-structure coupling ${\alpha }_{\mathrm{s}}$, its adequate (viz., more frankly, sufficiently rapid) decrease:

$$\begin{array}{cc}\hfill g_{\mathrm{s}}\propto \frac{1}{\sqrt{N_{\mathrm{c}}}}& =O\left(N_{\mathrm{c}}^{-1/2}\right)\underset{N_{\mathrm{c}}\to \infty }{\to }0\hfill \\ & \Updownarrow \hfill \end{array}$$

(10)

$$\begin{array}{cc}\hfill {\alpha }_{\mathrm{s}}\propto \frac{1}{N_{\mathrm{c}}}& =O\left(N_{\mathrm{c}}^{-1}\right)\underset{N_{\mathrm{c}}\to \infty }{\to }0.\hfill \end{array}$$

(11)

The concomitant generalisation of the two types of dynamical degrees of freedom of QCD to their counterparts in any of the quantum field theories that arise for $N_{\mathrm{c}}\ne 3$ is, unfortunately, just partially unique: Beyond doubt, the gluonic gauge vector bosons have to be placed in the $(N_{\mathrm{c}}^2-1)$-dimensional adjoint representation of SU($N_{\mathrm{c}}$). For any of the quarkonic fermions (of given quark flavour), however, the admittedly most common, but by no means compulsory, option is their assignment still to the $N_{\mathrm{c}}$-dimensional fundamental representation of SU($N_{\mathrm{c}}$).4 Hence, the dependence of perturbative large-$N_{\mathrm{c}}$ QCD contributions to a correlation function such as the one of the kind (5) on the number of colours $N_{\mathrm{c}}$ can be immediately inferred from the number of involved closed colour loops and the number of involved strong couplings (1).

Within large-$N_{\mathrm{c}}$ QCD, any colour-singlet conventional mesons $M_{\overline{a}b}$ (with masses $M_{M_{\overline{a}b}}$) continue5 to be given by bound states of one quark of flavour b and one antiquark of flavour a:

$$M_{\overline{a}b}=\left[{\overline{q}}_a{q}_b\right],a,b\in \{u,d,s,c,b,t(,\dots ?)\}.$$

(12)

Still skipping notationally all explicit reference to any spin or parity degrees of freedom by dropping all Dirac matrices, polarisation vectors, momenta, etc., the nonvanishing matrix element of any conventional-meson interpolating operator (4) between the state of the related conventional meson (12) and the vacuum defines the decay constant $f_{M_{\overline{a}b}}$ of that meson $M_{\overline{a}b}$:

$$f_{M_{\overline{a}b}}\equiv \langle 0|j_{\overline{a}b}|M_{\overline{a}b}\rangle \ne 0.$$

(13)

Because of the single quark loop involved, the large-$N_{\mathrm{c}}$ behaviour of $f_{M_{\overline{a}b}}$ is easy to guess [16]:

$$f_{M_{\overline{a}b}}\propto \sqrt{N_{\mathrm{c}}}=O\left(N_{\mathrm{c}}^{1/2}\right).$$

(14)

Large-$N_{\mathrm{c}}$ considerations allow us to shed light on crucial aspects of any flavour-cryptoexotic tetraquarks (3): their total decay width and their potential mixing with conventional mesons. In these analyses, those contributions to any flavour-cryptoexotic correlation functions (8) or (9) that (by Proposition 1) may be expected to be tetraquark-phile and thus might take part in an eventual formation of some tetraquark poles are identified by below the subscript label “tp”.

3.1. Total Decay Width of Flavour-Cryptoexotic Tetraquarks: Upper Bounds on Large-$N_{\mathrm{c}}$ Behaviour

The experimental observability in nature of some tetraquark meson requires a sufficient narrowness of that hadron: compared to its mass, its decay width $\mathsf{\Gamma }$ should not be too broad. For the large-$N_{\mathrm{c}}$ generalisation of QCD, supposing the tetraquark mass to be independent of the number $N_{\mathrm{c}}$ of colours, the decay width of such a tetraquark should not grow with $N_{\mathrm{c}}$ [17]. Therefore, the exploration of the large-$N_{\mathrm{c}}$ behaviour of the tetraquark decay widths seems to be in order. Tetraquarks need not show up already at the largest tetraquark-phile orders of $N_{\mathrm{c}}$: generally, merely upper bounds on any extracted large-$N_{\mathrm{c}}$ dependences should be expected.

Now, the decay of some tetraquark meson state, T, may be assumed to be dominated by the decay into a pair of conventional mesons of the generic kind (12). Hence, the total decay width of T is governed by the corresponding amplitudes $A(T⟷M_{\overline{a}b}{M}_{\overline{c}d})$ that encode all transitions between the tetraquark T and a conventional-meson pair $M_{\overline{a}b}$ and $M_{\overline{c}d}$. These tetraquark–two-conventional-meson transition amplitudes A, in turn, can be extracted from the intermediate-state tetraquark-pole contributions to the scattering amplitudes for the appropriate scattering processes of two conventional mesons into two conventional mesons.

Specifically, zooming in to the flavour-cryptoexotic states (3), the leading-$N_{\mathrm{c}}$ behaviour of all tetraquark-phile contributions to the correlation functions (8) and (9) is easily pinned down:

• In the flavour-preserving case (8), both sorts of tetraquark-phile QCD-level contributions of lowest perturbative order, illustrated in Figure 1a,b, exhibit rather similar behaviour: both types are built from two closed quark loops and two internal gluon exchanges. This then translates into two closed colour loops and two powers of the strong coupling (11). Accordingly, the order of all $N_{\mathrm{c}}$-leading contributions is $O\left(N_{\mathrm{c}}^2{\alpha }_{\mathrm{s}}^2\right)=O\left(N_{\mathrm{c}}^0\right)$ [2,4].
• In the flavour-rearranging case (9), the two examples of tetraquark-phile contributions of lowest perturbative-QCD order, depicted in Figure 2a,b, are of undoubtedly unlike structures: The contributions exemplified in Figure 2a involve merely one closed quark loop and two internal gluon exchanges. This corresponds to only a single closed colour loop and two powers of the strong coupling (11). On the other hand, any contribution of the sort shown in Figure 2b is formed by two closed quark loops and two internal gluon exchanges, which is tantamount to two closed colour loops and two powers of the strong coupling (11). This entails a large-$N_{\mathrm{c}}$ dependence of the order $O\left(N_{\mathrm{c}}^2{\alpha }_{\mathrm{s}}^2\right)=O\left(N_{\mathrm{c}}^0\right)$ [2,4].

In total, the upper bounds on the large-$N_{\mathrm{c}}$ behaviour of the tetraquark-phile contributions to both types of the flavour-cryptoexotic correlation functions (8) and (9) prove to be identical:6

$$\begin{array}{cc}& {〈\mathrm{T}\left(j_{\overline{a}b}\left(y\right)j_{\overline{b}c}\left(y^{\prime }\right)j_{\overline{a}b}^{†}\left(x\right)j_{\overline{b}c}^{†}\left(x^{\prime }\right)\right)〉}_{\mathrm{tp}}=O\left(N_{\mathrm{c}}^2{\alpha }_{\mathrm{s}}^2\right)=O\left(N_{\mathrm{c}}^0\right),\hfill \end{array}$$

(15)

$$\begin{array}{cc}& {〈\mathrm{T}\left(j_{\overline{a}c}\left(y\right)j_{\overline{b}b}\left(y^{\prime }\right)j_{\overline{a}c}^{†}\left(x\right)j_{\overline{b}b}^{†}\left(x^{\prime }\right)\right)〉}_{\mathrm{tp}}=O\left(N_{\mathrm{c}}^2{\alpha }_{\mathrm{s}}^2\right)=O\left(N_{\mathrm{c}}^0\right),\hfill \end{array}$$

(16)

$$\begin{array}{cc}& {〈\mathrm{T}\left(j_{\overline{a}b}\left(y\right)j_{\overline{b}c}\left(y^{\prime }\right)j_{\overline{a}c}^{†}\left(x\right)j_{\overline{b}b}^{†}\left(x^{\prime }\right)\right)〉}_{\mathrm{tp}}=O\left(N_{\mathrm{c}}^2{\alpha }_{\mathrm{s}}^2\right)=O\left(N_{\mathrm{c}}^0\right).\hfill \end{array}$$

(17)

Recalling that the finding (14) for the conventional-meson decay constants (13) enters in any four-point correlation functions (5) with fourth power, the large-$N_{\mathrm{c}}$ dependences of both encountered tetraquark–two-conventional-meson transition amplitudes $A(T⟷M_{\overline{a}b}{M}_{\overline{b}c})$ and $A(T⟷M_{\overline{a}c}{M}_{\overline{b}b})$, as deduced from the findings (15), (16), and (17), are parametrically identical: both are of the order $O\left(N_{\mathrm{c}}^{-1}\right)$. More precisely, these transition amplitudes exhibit a parametrically identical decrease proportional to $1/N_{\mathrm{c}}$ with increasing number $N_{\mathrm{c}}$ of colours. The associated tetraquark decay width $\mathsf{\Gamma }$ involves the squares of these transition amplitudes. Hence, the decay width $\mathsf{\Gamma }\left(T\right)$ of all flavour-cryptoexotic tetraquarks (3) is of the order $O\left(N_{\mathrm{c}}^{-2}\right)$. Schematically, the relations among these quantities are perhaps best summarised in the form

$$\underset{⟹\mathsf{\Gamma }\left(T\right)=O\left(N_{\mathrm{c}}^{-2}\right)}{\underbrace{A(T⟷M_{\overline{a}b}{M}_{\overline{b}c})=O\left(N_{\mathrm{c}}^{-1}\right)\stackrel{\begin{array}{|c|}\hline N_{\mathrm{c}}\mathrm{order}\\ \hline\end{array}}{\equiv }A(T⟷M_{\overline{a}c}{M}_{\overline{b}b})=O\left(N_{\mathrm{c}}^{-1}\right)}}.$$

(18)

In Table 2, the large-$N_{\mathrm{c}}$ upper bound (18) found for the decay widths $\mathsf{\Gamma }$ is confronted with the outcomes of comparable considerations from (marginally) different perspectives [18,19].

Table 2. Theoretically predicted upper bounds on the large-$N_{\mathrm{c}}$ behaviour of the total decay width $\mathsf{\Gamma }$ of the flavour-cryptoexotic tetraquarks (2); distilled by condensing Table 2 of Ref. [7] or Table 3 of Ref. [9].

Author Collective	Large-${\mathit{N}}_{\mathbf{c}}$ Total Decay Width	References
Knecht and Peris	$O(1/N_{\mathrm{c}})$	[18]
Maiani, Polosa, and Riquer	$O(1/N_{\mathrm{c}}^3)$	[19]
Lucha, Melikhov, and Sazdjian	$O(1/N_{\mathrm{c}}^2)$	[2,4]

Table 2. Theoretically predicted upper bounds on the large-$N_{\mathrm{c}}$ behaviour of the total decay width $\mathsf{\Gamma }$ of the flavour-cryptoexotic tetraquarks (2); distilled by condensing Table 2 of Ref. [7] or Table 3 of Ref. [9].

Author Collective	Large-${\mathit{N}}_{\mathbf{c}}$ Total Decay Width	References
Knecht and Peris	$O(1/N_{\mathrm{c}})$	[18]
Maiani, Polosa, and Riquer	$O(1/N_{\mathrm{c}}^3)$	[19]
Lucha, Melikhov, and Sazdjian	$O(1/N_{\mathrm{c}}^2)$	[2,4]

The undoubtedly conspicuous incongruences of the large-$N_{\mathrm{c}}$ expectations compiled in Table 2 originate very likely either from the—from the point of view of Proposition 1 unjustified and, thus, perhaps misleading—trust [18] in contributions that do not qualify as tetraquark-phile or from the neglect [19] of all $N_{\mathrm{c}}$-leading contributions of the kind put forward in Figure 2b.7

The lessons to be learned from the bulk of all large-$N_{\mathrm{c}}$ considerations of this kind can be subsumed as follows: the total decay widths, $\mathsf{\Gamma }\left(T\right)$, of the flavour-cryptoexotic tetraquarks (3) neither grow with $N_{\mathrm{c}}$ nor are independent of $N_{\mathrm{c}}$. Rather, they decrease with $N_{\mathrm{c}}$. This implies that, from the large-$N_{\mathrm{c}}$ aspect, the flavour-cryptoexotic tetraquarks are narrow hadrons. The comparison with the large-$N_{\mathrm{c}}$ behaviour of generic conventional mesons (12) reveals, maybe surprisingly, differences. In the large-$N_{\mathrm{c}}$ limit, the total decay widths of flavour-cryptoexotic tetraquarks (3) in Equation (18), found to be (at most) of the order $O\left(N_{\mathrm{c}}^{-2}\right)$, decrease (at least) proportionally to $1/N_{\mathrm{c}}^2$ and, thus, definitely faster than the conventional-meson decay widths, which—having been found [16] to be of an order $O\left(N_{\mathrm{c}}^{-1}\right)$—decrease proportionally to $1/N_{\mathrm{c}}$. Flavour-cryptoexotic tetraquarks (3) are parametrically narrower than the conventional mesons.

3.2. Mixing of Flavour-Cryptoexotic Tetraquark Mesons and Conventional Mesons: Large-$N_{\mathrm{c}}$ Limit

As already pointed out in Section 1, any flavour-cryptoexotic multiquark hadron state, in Definition 1 characterised by presenting a set of discernible quark-flavour degrees of freedom that is identical to the flavour content of a conventional hadron state, may undergo mixing with such a conventional hadron state if not forbidden by any conserved degrees of freedom. In particular, any flavour-cryptoexotic tetraquark (2), evidently involving the two open quark flavours ${\overline{q}}_a$ and $q_c$, has to be supposed to mix with any conventional meson (12) composed of the matching quark flavours, $M_{\overline{a}c}$. In view of this, within large-$N_{\mathrm{c}}$ considerations, an urgent question immediately emerges: what is the impact of these tetraquark–conventional-meson mixings on the large-$N_{\mathrm{c}}$ behaviour of the flavour-cryptoexotic tetraquarks (2)? Does this sort of mixing qualitatively modify any large-$N_{\mathrm{c}}$ predictions for flavour-cryptoexotic tetraquarks?

The strength of any coupling of a flavour-cryptoexotic tetraquark T of quark content (2) and an adequate conventional meson $M_{\overline{a}c}$ may be encoded by their mixing parameter $g_{T{M}_{\overline{a}c}}$. The behaviour of all mixing parameters $g_{T{M}_{\overline{a}c}}$ in the large-$N_{\mathrm{c}}$ limit can, without problem, be inferred from the knowledge of the generic large-$N_{\mathrm{c}}$ dependences of the conventional-meson decay constants $f_{M_{\overline{a}b}}$, recalled by Equation (14), of the two amplitudes describing transitions between a tetraquark state and a pair of conventional mesons, found in Equation (18), and of the amplitudes $A(M_{\overline{a}c}⟷M_{\overline{a}b}{M}_{\overline{b}c})$ of all couplings among three conventional mesons [16]:

$$A(M_{\overline{a}c}⟷M_{\overline{a}b}{M}_{\overline{b}c})\propto \frac{1}{\sqrt{N_{\mathrm{c}}}}=O\left(N_{\mathrm{c}}^{-1/2}\right)\underset{N_{\mathrm{c}}\to \infty }{\to }0.$$

(19)

For instance, upon truncation or neglect of all quantities regarded as independent of $N_{\mathrm{c}}$ (such as the propagators of internal and external mesons), the portion of the tetraquark-phile contribution (15) to one of the flavour-cryptoexotic correlation functions (8) that receives just a single tetraquark–conventional-meson mixing is boiled down to the large-$N_{\mathrm{c}}$ constraint [2]

$$f_M^{4}A(T⟷M_{\overline{a}b}{M}_{\overline{b}c})g_{T{M}_{\overline{a}c}}A(M_{\overline{a}c}⟷M_{\overline{a}b}{M}_{\overline{b}c})=O\left(N_{\mathrm{c}}^0\right).$$

(20)

Similar studies can be performed for tetraquark-phile contributions exhibiting more than one such mixing [4]. The large-$N_{\mathrm{c}}$ upper bounds resulting thereof “fortunately” agree. The mixing parameters $g_{T{M}_{\overline{a}c}}$ are of the order $O\left(N_{\mathrm{c}}^{-1/2}\right)$ and decrease not more slowly than $1/\sqrt{N_{\mathrm{c}}}$ [2,4]:

$$g_{T{M}_{\overline{a}c}}\propto \frac{1}{\sqrt{N_{\mathrm{c}}}}=O\left(N_{\mathrm{c}}^{-1/2}\right)\underset{N_{\mathrm{c}}\to \infty }{\to }0.$$

(21)

All products of one three-meson amplitude (19) and one mixing parameter (21) reproduce the large-$N_{\mathrm{c}}$ behaviour of the transition amplitudes (18); thus, the mixing of a tetraquark with its conventional-meson quark-flavour counterparts does not alter the findings of Section 3.1:

$$A(T⟷M_{\overline{a}b}{M}_{\overline{b}c})=O\left(N_{\mathrm{c}}^{-1}\right)\widehat{=}{g}_{T{M}_{\overline{a}c}}A(M_{\overline{a}c}⟷M_{\overline{a}b}{M}_{\overline{b}c})=O\left(N_{\mathrm{c}}^{-1}\right).$$

(22)

4. Application of the QCD Sum-Rule Formalism to Multiquarks: Immediate Implication

Ideally (or, perhaps, from a somewhat puristic point of view), the theoretical description of bound states in terms of some underlying quantum field theory should be carried out in a manner that is both analytical and nonperturbative. For the strong interactions, an approach that comes pretty close to these two requirements is the framework of QCD sum rules [20,21,22]. The basic idea behind the latter tool is the construction of relations between the fundamental parameters of QCD, on the one hand, and (experimentally observable) properties of hadrons emerging as bound states of the set of dynamical QCD degrees of freedom, on the other hand.

This derivation can be enacted or achieved by, first, the definition of suitable correlation functions that involve hadron interpolating operators, related (among others) to the hadrons of interest, followed by the simultaneous evaluation of one and the same correlation function, at both the phenomenological level of the hadronic states and the fundamental level of QCD:

• At the hadron level, the insertion of a complete set of hadron states brings into the game all hadrons potentially contributing in the form of intermediate states (specifically, their observable characteristics, such as masses, decay constants, and transition amplitudes); among the latter hadrons, there should show up the particular multiquark under study.
• At the QCD level, the conversion [23] of the nonlocal product of interpolating operators in any such correlation function into a series of local operators enables the separation of the perturbative from the nonperturbative contributions: the perturbative contributions might be obtained, for lower orders of the strong coupling, order by order (as discussed in Section 2). The nonperturbative contributions, however, cannot be derived (at present) from the underlying quantum field theory. They can be parametrised by quantities that may be inferred from experiment and can be interpreted as effective parameters of QCD.

Equating the eventual outcomes of the two procedures generates the desired QCD sum rules.

When applying the generally valid QCD sum-rule technique, specifically, to any type of multiquark state, particular attention must be paid to two “aggravating inconveniences”: the construction of (suitable) multiquark interpolating operators and, in view of the discussion in Section 2, the multiquark adequacy [12,24] of the predictions emerging from this technique:8

• If narrowing down the envisaged quest for multiquark-adequate QCD sum rules to the subcategory of multiquark exotics that is formed by all tetraquark mesons, the problem of identifying, for particular states, the most appropriate set of tetraquark interpolating operators is considerably mitigated by the observation that, upon application of proper Fierz transformations [27], every colour-singlet operator constructed of two quark fields and two antiquark fields can easily be rearranged to a linear combination of products of two conventional-meson interpolating operators (4). As far as the quark flavour quantum numbers $a,b,c,d$ are concerned, not more than two products of such kind are available:

  $$j_{\overline{a}b}\left(x\right)j_{\overline{c}d}\left(x\right),j_{\overline{a}d}\left(x\right)j_{\overline{c}b}\left(x\right).$$

  (23)
  The set (23) of products of colour-singlet quark–antiquark bilinear operators (4) may be regarded to provide a sort of basis of the space of all tetraquark interpolating operators.
• The product nature of an element of the tetraquark interpolating operator basis (23) may be imagined to arise from the identification or “contraction” of the configuration-space coordinates of proper pairs of quark-bilinear currents (4) similar to those showing up in each of the four-point correlation functions (5). This fact, in turn, offers the opportunity to construct correlation functions that involve either a sole, or even a pair of, tetraquark interpolating operators by subjecting appropriately selected correlation functions (5) of four quark-bilinear operators (4) to one or two of these spatial-coordinate contractions.
• In the course of invoking the standard QCD sum-rule technique for the investigation of multiquarks, this tool’s intended improvement, dubbed its multiquark adequacy, may be accomplished by diminishing, to the utmost reasonable extent, all its “contaminations” by contributions evidentially irrelevant to any exotic state momentarily under scrutiny. For the tetraquark mesons, this demands to retain exclusively QCD-level contributions to correlation functions that are tetraquark-phile, in full compliance with Proposition 1, and to carefully match any of these contributions with the corresponding mirror images in the set of hadron-level contributions to the very same kind of correlation functions.9
• Focusing one very last time on the subset of all flavour-cryptoexotic tetraquarks (3) that involve three disparate quark flavours, the analysis carried out in Section 2 implies that tetraquark-phile contributions to any correlation function that is on the verge of being calculationally converted to a QCD sum rule cannot arise before the second order of the perturbative expansion in powers of the strong coupling strength ${\alpha }_{\mathrm{s}}$. All concomitant contributions at the hadronic level, however, ought to be thoroughly disentangled with respect to their actual relevance for each tetraquark state considered. This task proves to be (comparatively) straightforward for all flavour-preserving correlation functions (8). For every flavour-rearranging correlation function (9), a case-by-case judgement might turn out to be in order. The actual feasibility of any such analysis has been claimed and a conceivable route briefly indicated, for the flavour-preserving quark distributions (8), in Reference [24], and, for the flavour-rearranging quark distribution (9), in Reference [12].

The tetraquark-adequate QCD sum rules gained from this optimisation effort may be expected to provide, for various basic properties of any flavour-cryptoexotic tetraquark (3) considered, predictions of inevitably higher credibility. Among these characteristics of any such state are its mass, its decay constants, given by the vacuum–tetraquark matrix elements of the hadron interpolating operators (23), and all strengths of its couplings to two quark-bilinear currents, given by the vacuum–tetraquark matrix elements of not contracted pairs of the operators (4).

5. Conclusions: Promising Prospects of Approaches to Flavour-Cryptoexotic Tetraquarks

The possible existence of multiquark exotic hadrons in the form of tightly bound states, as an inevitable implication of the strong interactions described by QCD, has been predicted long ago. Substantial experimental evidence for the actual existence, and observability, of multiquark hadrons has been accumulated only comparatively recently. The (by far) largest number of experimental candidates for multiquarks belongs to the class of tetraquarks of the flavour-cryptoexotic kind: tetraquarks that, by including a quark and an antiquark of the same quark flavour, exhibit, at most, only two openly discernible quark flavour quantum numbers.

For their theoretical interpretations, these experimental findings still form considerable challenges: they constitute, at present, one of the greatest riddles of hadron phenomenology. In order to promote, to some extent, the progress in the systematic theoretical investigation of all tetraquark mesons, a rigorous constraint on any meaningful (thus, acceptable) theoretical input to analyses of this kind has been proposed [2,4]. (It goes without saying that each such type of constraint may merely serve as a necessary but not sufficient condition for the factual participation of a given tetraquark state in any physical process under study.) Among others, this tool can then be used to confirm that the decay widths of any tetraquark states should be expected to be parametrically narrower than those of ordinary (quark–antiquark) mesons, or to point out directions towards a more adequate description of tetraquark mesons, by means of suitably adapted QCD sum rules; this has yet to be utilised in multiquark studies [28,29,30,31,32,33,34,35].