Excited States of Maximal Warm Holes
Abstract
1. Introduction
2. The Model
3. Numerical Results
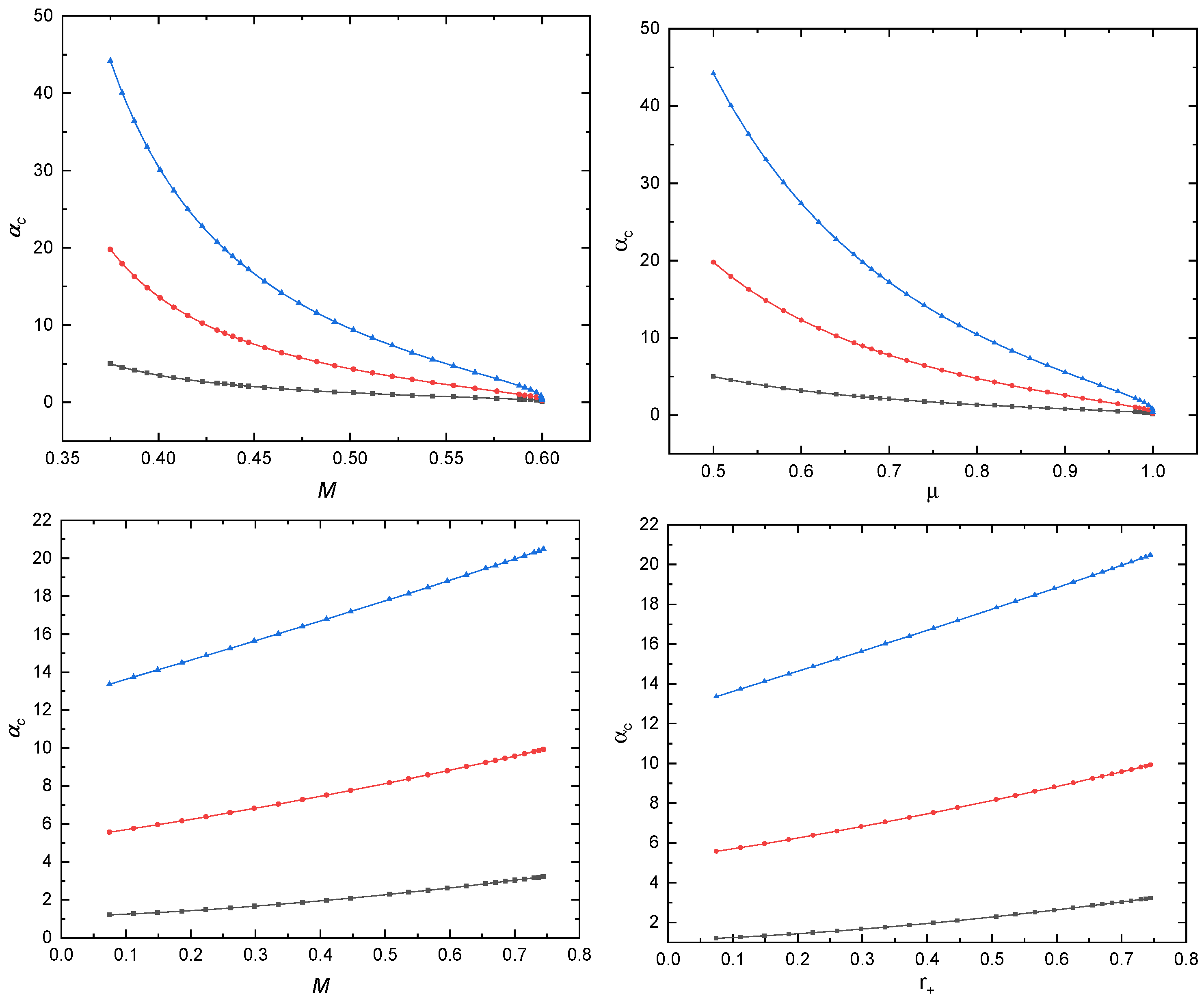
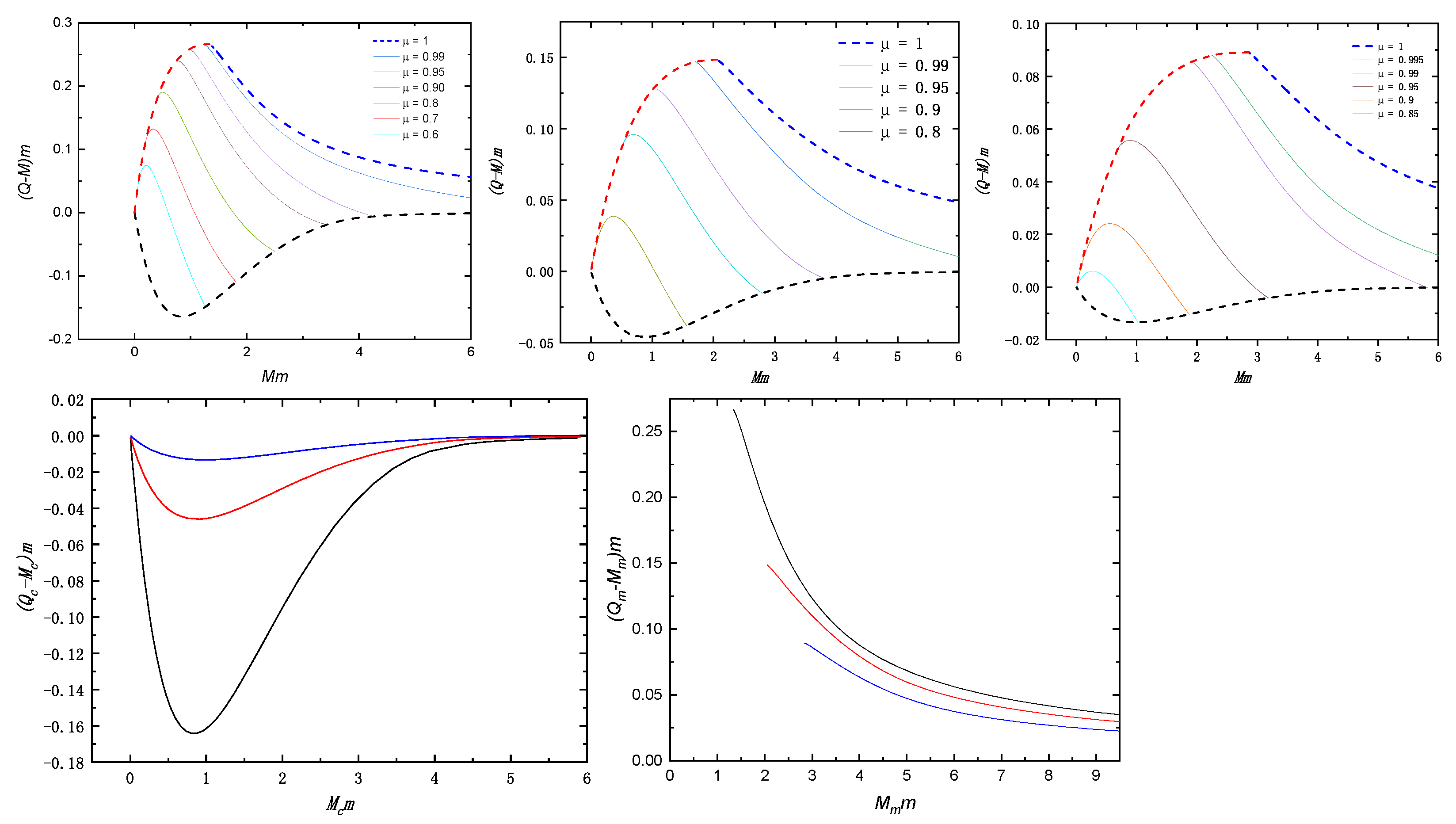
3.1. Critical Analysis of Scalar Field Stability
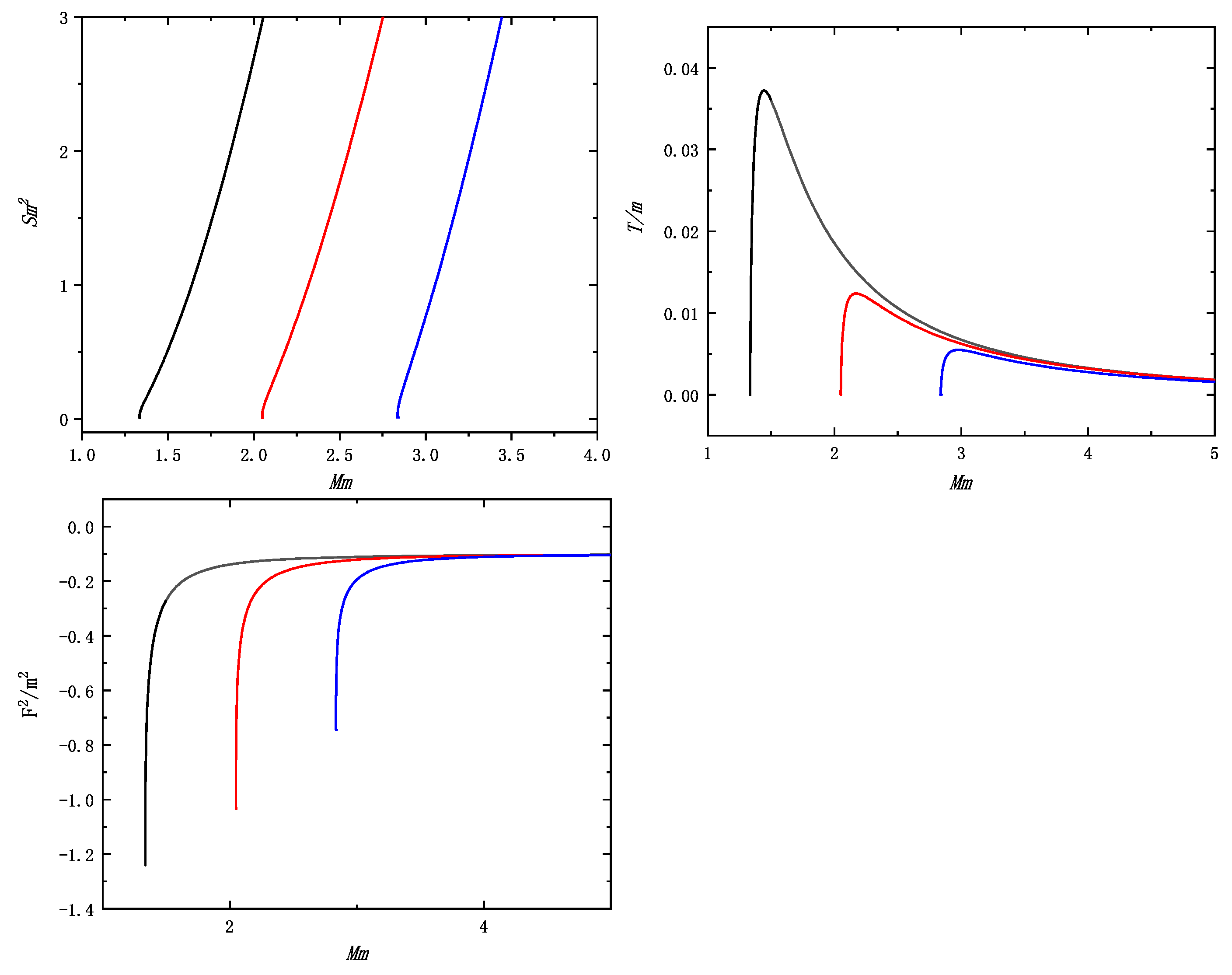
3.2. Numerical Hairy Black Hole Solution
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abbott, B.P. et al. [LIGO Scientific and Virgo] Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef] [PubMed]
- Akiyama, K. et al. [Event Horizon Telescope] First M87 Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole. Astrophys. J. Lett. 2019, 875, L1. [Google Scholar]
- Akiyama, K. et al. [Event Horizon Telescope] First M87 Event Horizon Telescope Results. II. Array and Instrumentation. Astrophys. J. Lett. 2019, 875, L2. [Google Scholar]
- Akiyama, K. et al. [Event Horizon Telescope] First M87 Event Horizon Telescope Results. III. Data Processing and Calibration. Astrophys. J. Lett. 2019, 875, L3. [Google Scholar]
- Akiyama, K. et al. [Event Horizon Telescope] First M87 Event Horizon Telescope Results. IV. Imaging the Central Supermassive Black Hole. Astrophys. J. Lett. 2019, 875, L4. [Google Scholar]
- Akiyama, K. et al. [Event Horizon Telescope] First M87 Event Horizon Telescope Results. V. Physical Origin of the Asymmetric Ring. Astrophys. J. Lett. 2019, 875, L5. [Google Scholar]
- Akiyama, K. et al. [Event Horizon Telescope] First M87 Event Horizon Telescope Results. VI. The Shadow and Mass of the Central Black Hole. Astrophys. J. Lett. 2019, 875, L6. [Google Scholar]
- Misner, C.W.; Thorne, K.S.; Wheeler, J.A. Gravitation; WH Freeman and Company Limited: New York, NY, USA, 1973; ISBN 978-0-7167-0344-0, 978-0-691-17779-3. [Google Scholar]
- Hawking, S.W.; Horowitz, G.T.; Ross, S.F. Entropy, Area, and black hole pairs. Phys. Rev. D 1995, 51, 4302–4314. [Google Scholar] [CrossRef]
- Garfinkle, D.; Horowitz, G.T.; Strominger, A. Charged black holes in string theory. Phys. Rev. D 1991, 43, 3140, Erratum in Phys. Rev. D 1992, 45, 3888. [Google Scholar] [CrossRef]
- Strominger, A.; Vafa, C. Microscopic origin of the Bekenstein-Hawking entropy. Phys. Lett. B 1996, 379, 99–104. [Google Scholar] [CrossRef]
- Callan, C.G.; Maldacena, J.M.; Peet, A.W. Extremal black holes as fundamental strings. Nucl. Phys. B 1996, 475, 645–678. [Google Scholar] [CrossRef]
- Horowitz, G.T.; Strominger, A. Counting states of near extremal black holes. Phys. Rev. Lett. 1996, 77, 2368–2371. [Google Scholar] [CrossRef] [PubMed]
- Teitelboim, C. Action and entropy of extreme and nonextreme black holes. Phys. Rev. D 1995, 51, 4315, Erratum in Phys. Rev. D 1995, 52, 6201. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, A.; Mitra, P. Understanding the area proposal for extremal black hole entropy. Phys. Rev. Lett. 1997, 78, 1858–1860. [Google Scholar] [CrossRef]
- Hod, S. Evidence for a null entropy of extremal black holes. Phys. Rev. D 2000, 61, 084018. [Google Scholar] [CrossRef]
- Dias, O.J.C.; Horowitz, G.T.; Santos, J.E. Extremal black holes that are not extremal: Maximal warm holes. JHEP 2022, 1, 64. [Google Scholar] [CrossRef]
- Hartnoll, S.A.; Herzog, C.P.; Horowitz, G.T. Building a Holographic Superconductor. Phys. Rev. Lett. 2008, 101, 031601. [Google Scholar] [CrossRef]
- Dias, O.J.C.; Horowitz, G.T.; Santos, J.E. Inside an asymptotically flat hairy black hole. JHEP 2021, 12, 179. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Hu, T.T.; Liu, Y.X.; Yang, J.; Zhao, L. Excited states of holographic superconductors. JHEP 2020, 6, 13. [Google Scholar] [CrossRef]
- Qiao, X.; Wang, D.; OuYang, L.; Wang, M.; Pan, Q.; Jing, J. An analytic study on the excited states of holographic superconductors. Phys. Lett. B 2020, 811, 135864. [Google Scholar] [CrossRef]
- Wang, D.; Du, X.; Pan, Q.; Jing, J. Holographic p-Wave Superconductor with Excited States in 4D Einstein–Gauss–Bonnet Gravity. Universe 2023, 9, 104. [Google Scholar] [CrossRef]
- Bernal, A.; Barranco, J.; Alic, D.; Palenzuela, C. Multi-state Boson Stars. Phys. Rev. D 2010, 81, 044031. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Liu, Y.X.; Wei, S.W. Excited Kerr black holes with scalar hair. Phys. Rev. D 2019, 99, 064036. [Google Scholar] [CrossRef]
- Trefethen, L.N. Spectral Methods in MATLAB; SIAM: Philadelphia, PA, USA, 2000. [Google Scholar]
- Horowitz, G.T.; Santos, J.E. General Relativity and the Cuprates. JHEP 2013, 6, 87. [Google Scholar] [CrossRef]
- Brito, R.; Cardoso, V.; Herdeiro, C.A.R.; Radu, E. Proca stars: Gravitating Bose–Einstein condensates of massive spin 1 particles. Phys. Lett. B 2016, 752, 291–295. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yue, Y.; Wang, Y.-Q. Excited States of Maximal Warm Holes. Universe 2023, 9, 338. https://doi.org/10.3390/universe9070338
Yue Y, Wang Y-Q. Excited States of Maximal Warm Holes. Universe. 2023; 9(7):338. https://doi.org/10.3390/universe9070338
Chicago/Turabian StyleYue, Yuan, and Yong-Qiang Wang. 2023. "Excited States of Maximal Warm Holes" Universe 9, no. 7: 338. https://doi.org/10.3390/universe9070338
APA StyleYue, Y., & Wang, Y.-Q. (2023). Excited States of Maximal Warm Holes. Universe, 9(7), 338. https://doi.org/10.3390/universe9070338




