The Central Engine of GRB170817A and the Energy Budget Issue: Kerr Black Hole versus Neutron Star in a Multi-Messenger Analysis
Abstract
1. Introduction
2. Gravitational Collapse of an HMNS to a Kerr Black Hole
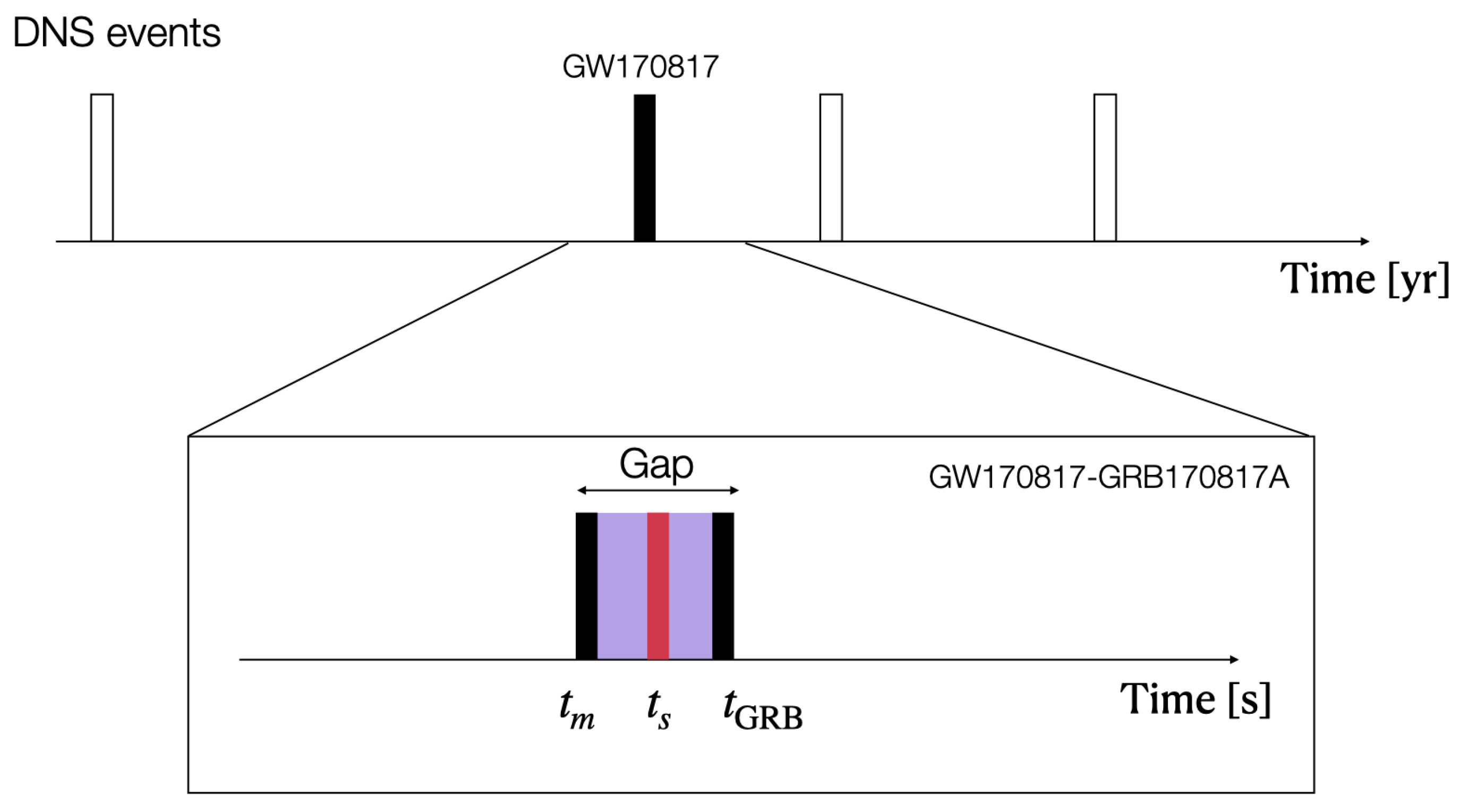
3. Event Timing in Multi-Messenger Observations
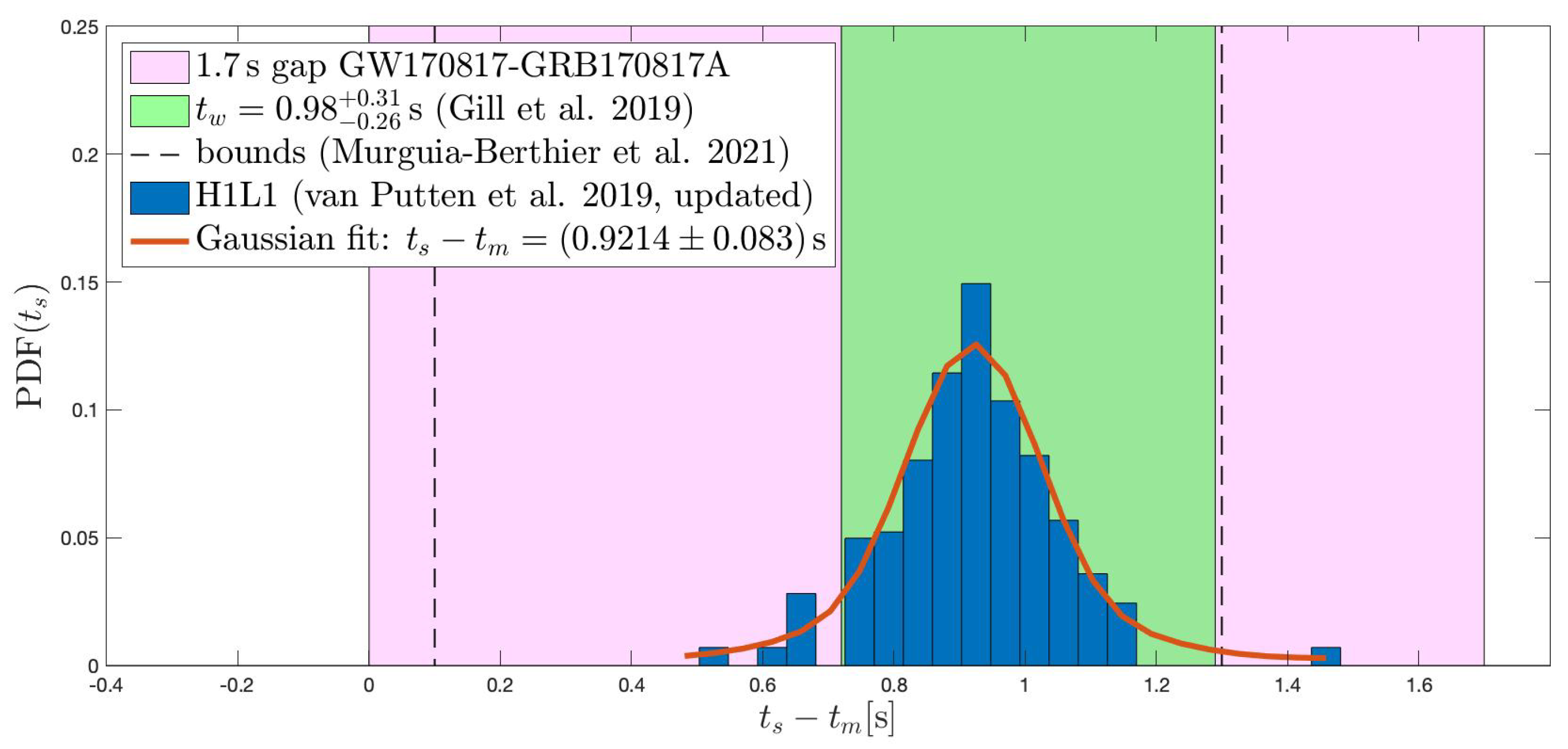
3.1. The Gap Time between GRB170817A and Its Progenitor GW170817
3.2. Event Timing by the Trigger Time of GRB170817A
- GW170817 initially produced an HMNS, evidenced by the kilonova AT2017gfo, followed by GRB170817A across a duration gap of s, containing the time of the gravitational collapse of the HMNS to a BH, .
- The black hole is initially rapidly spinning, as evidenced by an output in Hz, exceeding the spin energy of the HMNS.
4. Observations of GW Transient Emission in Time-Symmetric Spectrograms
4.1. Butterfly Matched Filtering
4.1.1. Sensitivity Gain over the Time-Sliced Fourier Analysis
4.1.2. Application to Gravitational-Wave Data
- GW transients may be ascending or descending chirps in gravitational radiation of mergers and, respectively, the spin-down of a compact object.
- GW transients can be searched for in high-resolution spectrograms covering the bandwidth of sensitivity of LVK over a time scale of time-slicing.
- A gain in sensitivity (23) obtains in Butterfly matched filtering over a bank of time-symmetric chirp-like templates densely covering a region of spaces.
5. Ascending–Descending GW Transient Emission during GW170817-GRB170817A
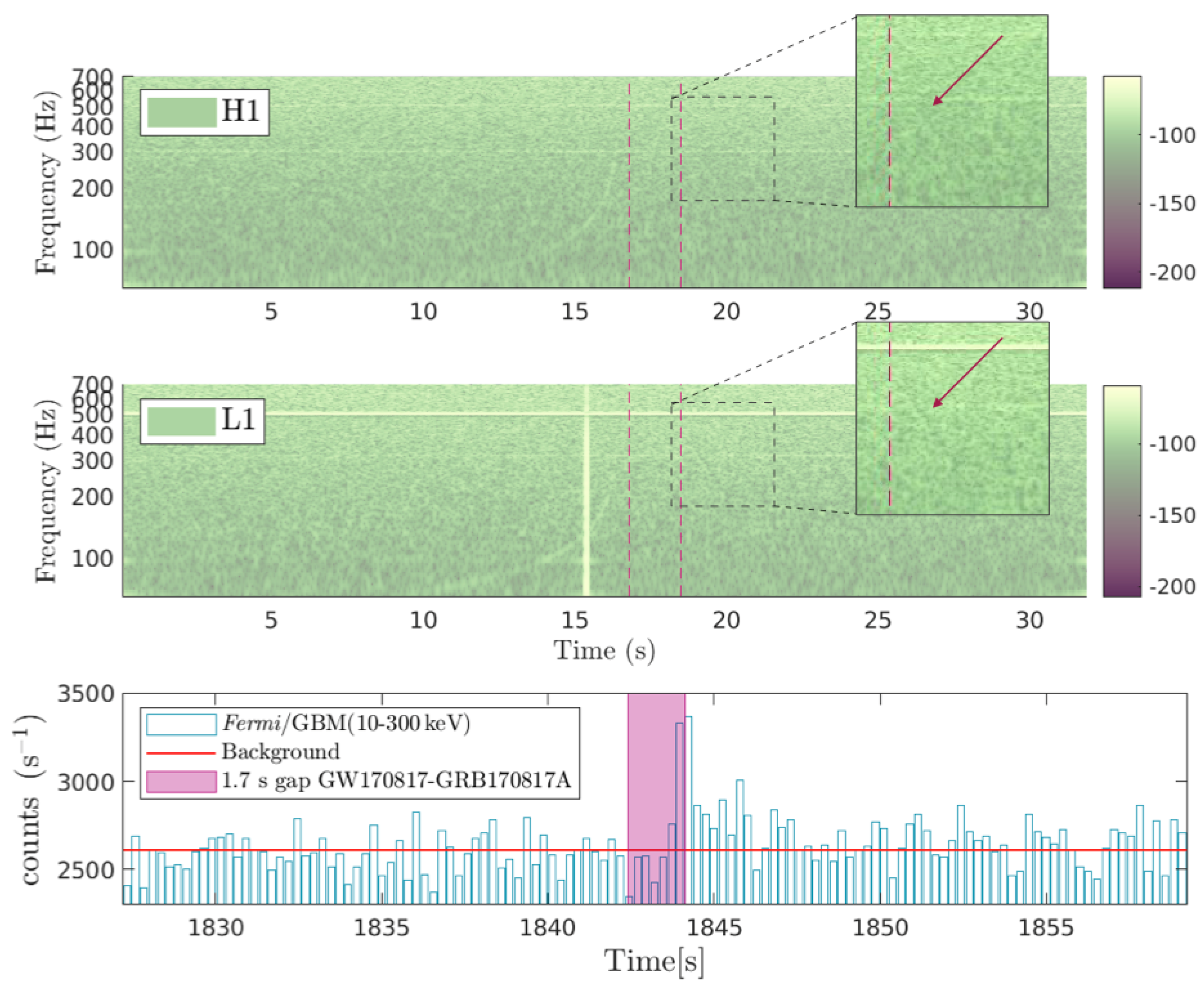
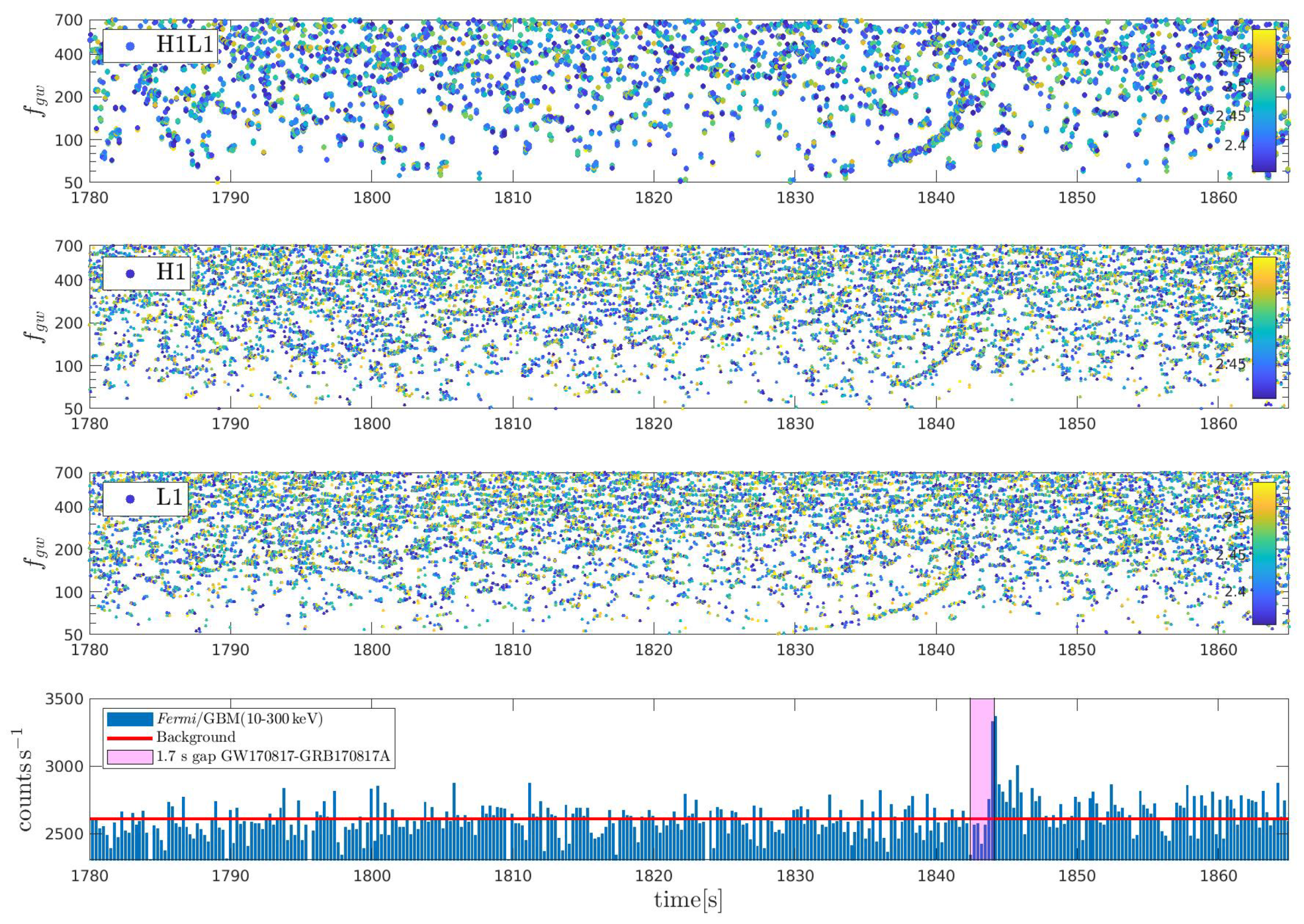
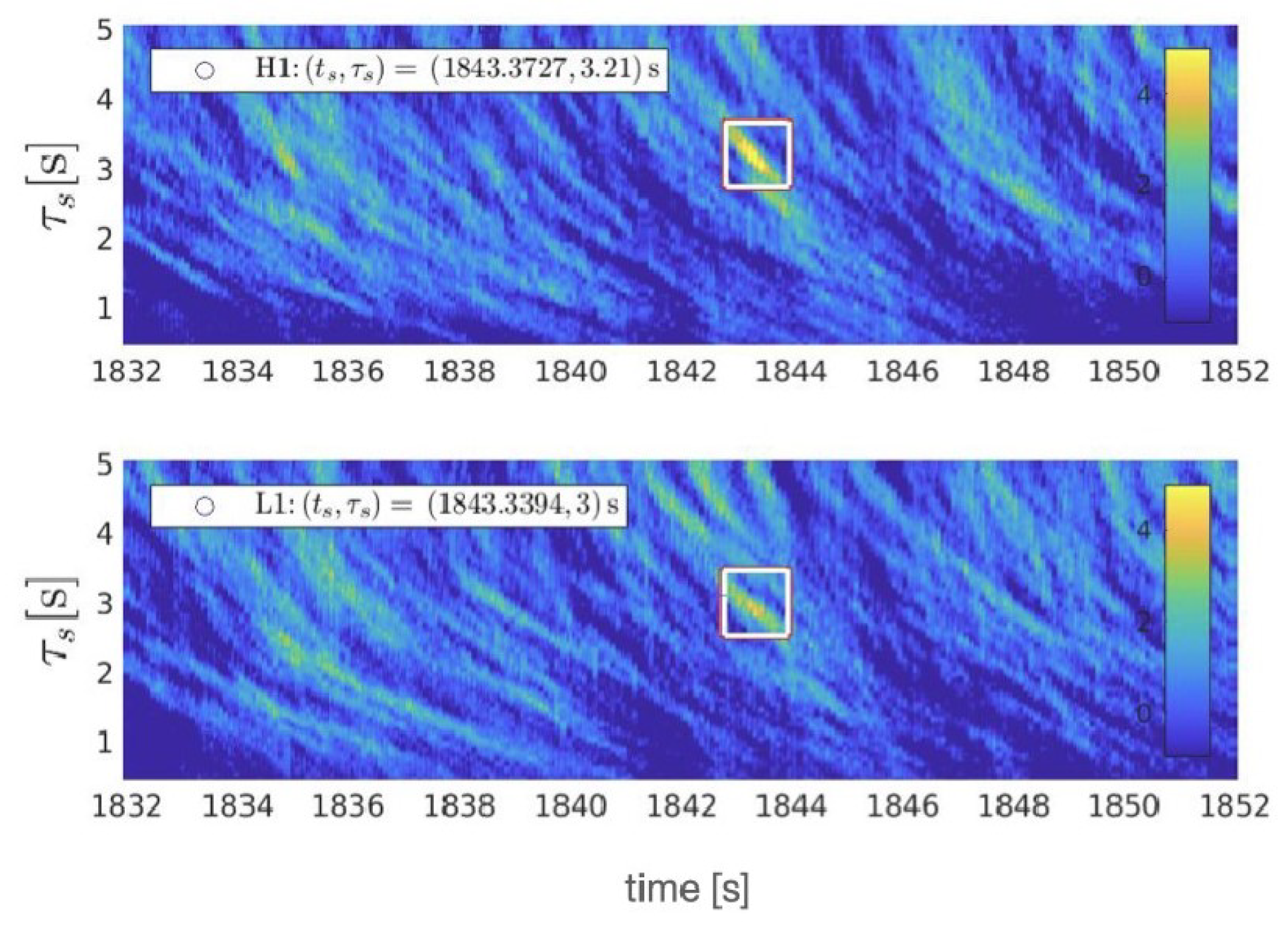
5.1. Observation in H1L1 Data
5.2. Calibrated Response Curves
- The ascending–descending chirp in GW170817 represents a merger followed by delayed spin-down of a Kerr black hole during GRB170817A.
- This observation is seen in spectrograms generated by Butterfly matched filtering calibrated by signal injection experiments.
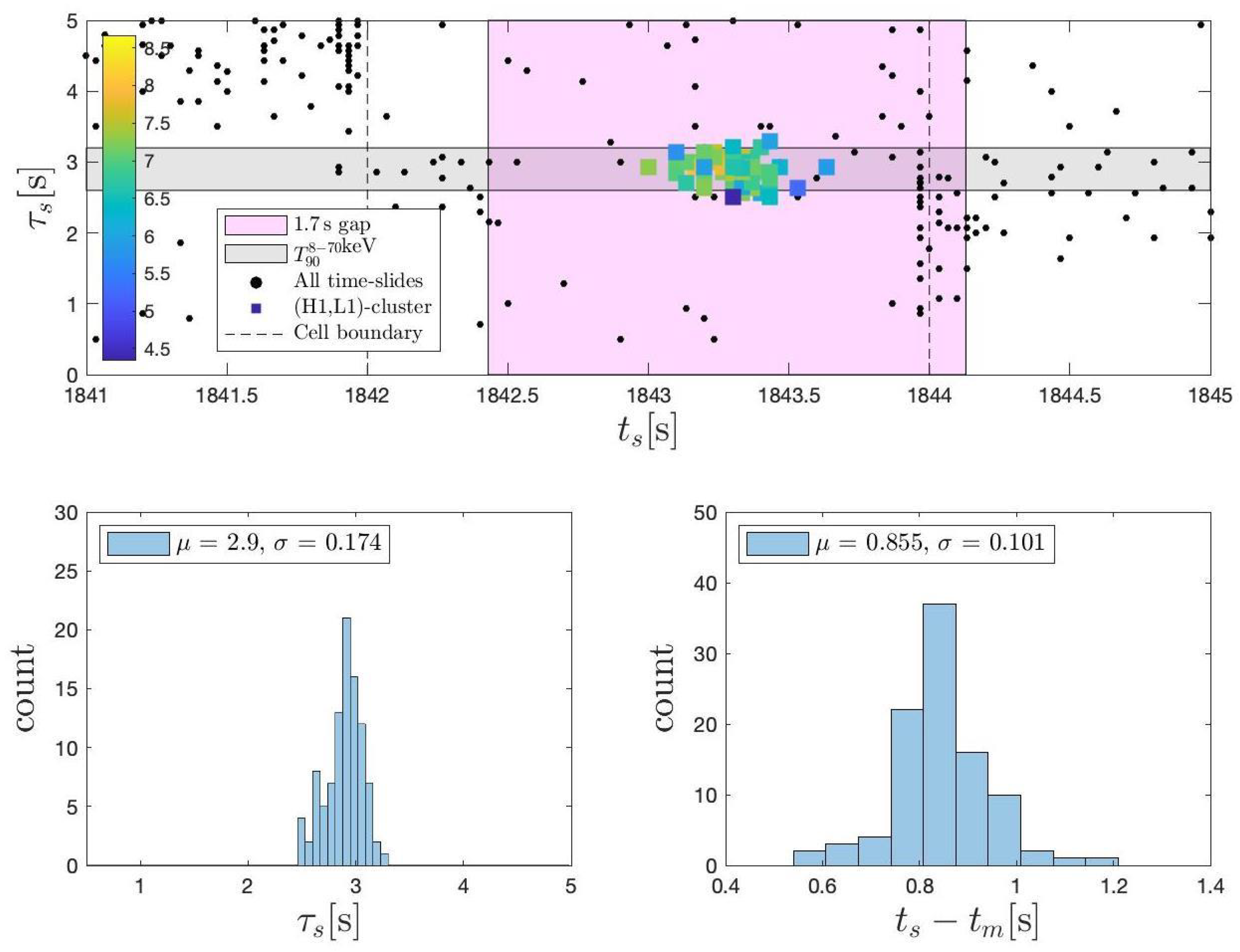
- EM-GW event timing shows consistency between s of GRB170817A [90], s and time scale of descent s.
6. Exascale Computing by Synaptic Parallel Processing
6.1. Acceleration by High-Performance Computing
- Efficient evaluation in the Fourier domain using the fast Fourier transform (FFT);
- Heterogeneous computing by offloading inverse FFTs to graphics processor units (GPUs);
- Distributed computing on a platform, load-balanced by synaptic parallel processing.
6.2. Dynamical Load Balancing by Synaptic Parallel Processing
7. Parameter Estimation in an Extended Foreground of (H1,L1)-Spectrograms
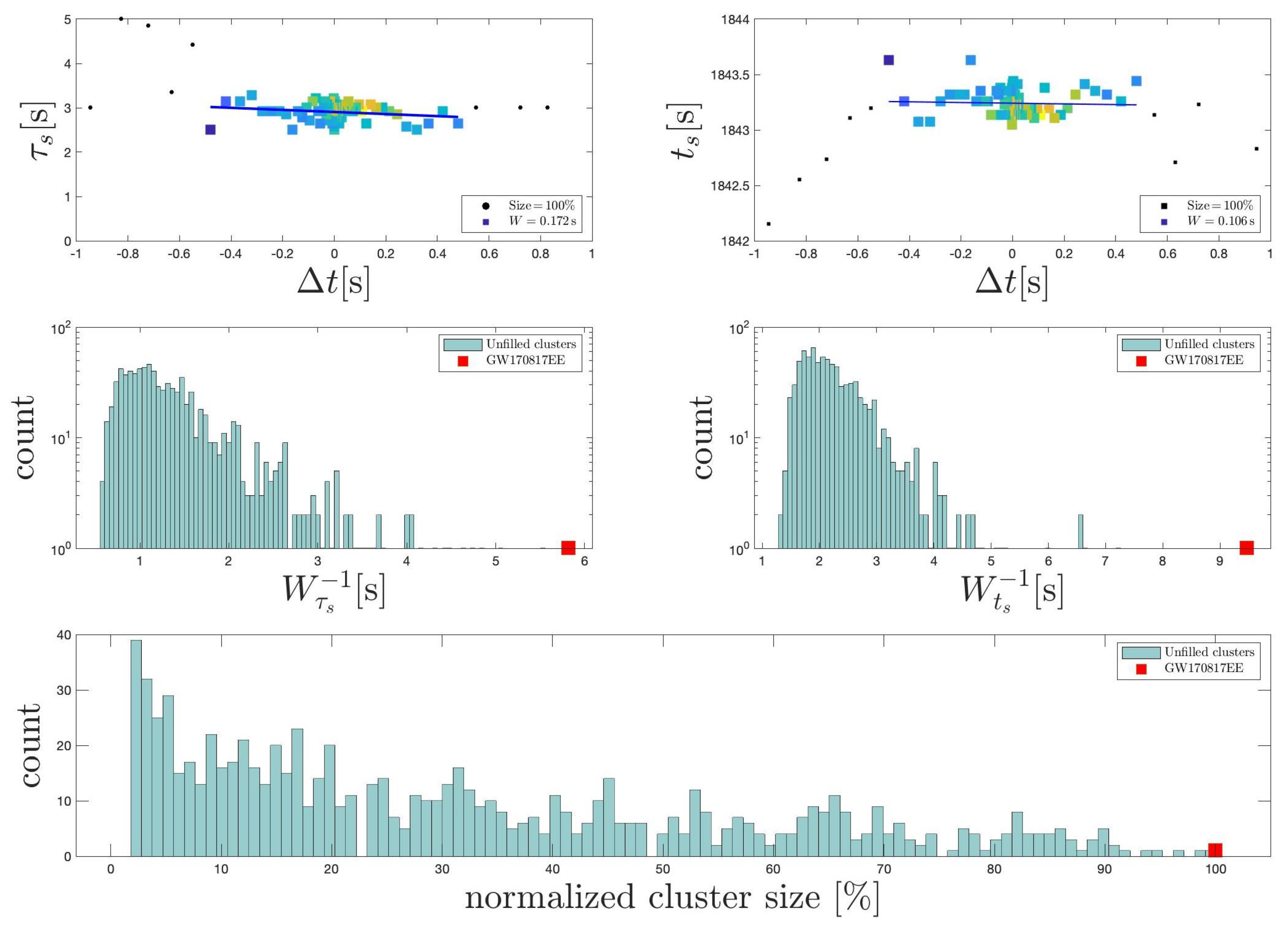
7.1. PDFs from the Extended Foreground Analysis over Small Time Slides
7.2. Clustering in Parameter Space
- Exascale HPC is achieved by synaptic parallel processing on a heterogeneous compute platform [162] in the mixed F90/C++/C99 using OpenCL and bash.
8. Consistent Event Timing in Independent H1 and L1 Analyses
- Independent event timings derive from the mean and difference in , equivalent to a unitary transformation of H1 and L1 data, here obtained from merged and individual H1 and L1 spectrograms.
9. Conclusions and Outlook
9.1. Principal Results
9.2. Outlook on Upcoming LVK Observations
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| OpenCL | open compute language |
| GPU | graphics processor unit |
| PCIe | peripheral computer interface express |
References
- Abbott, R. et al. [The LIGO Scientific Collaboration and the Virgo Collaboration] GWTC-2: Compact Binary Coalescences Observed by LIGO and Virgo during the First Half of the Third Observing Run. Phys. Rev. X 2021, 11, 021053. [Google Scholar]
- Abbott, R. et al. [The LIGO Scientific Collaboration and the Virgo Collaboration] Population Properties of Compact Objects from the Second LIGO—Virgo Gravitational-Wave Transient Catalog. Astrophys. J. Lett. 2021, 913, L7. [Google Scholar] [CrossRef]
- Abbott, R. et al. [The LIGO Scientific Collaboration and the Virgo Collaboration] GWTC-3: Compact Binary Coalescences Observed by LIGO and Virgo During the Second Part of the Third Observing Run. arXiv 2021, arXiv:2111.03606. [Google Scholar]
- Salpeter, E.E. The Luminosity Function and Stellar Evolution. Astrophys. J. 1955, 121, 161. [Google Scholar] [CrossRef]
- Park, H.J.; Kim, S.J.; Kim, S.; van Putten, M.H.P.M. On the Mass Function of GWTC-2 Binary Black Hole Systems and Their Progenitors. Astrophys. J. 2022, 938, 69. [Google Scholar] [CrossRef]
- Abbott, B.P. et al. [The LIGO Scientific Collaboration and the Virgo Collaboration] Multi-messenger Observations of a Binary Neutron Star Merger. Astrophys. J. 2017, 848, L12. [Google Scholar] [CrossRef]
- Dai, Z.G. Gravitational waves from post-merger radially oscillating millisecond pulsars. Astron. Astrophys. 2019, 662, 194. [Google Scholar] [CrossRef]
- De Pietri, R.; Feo, A.; Font, J.A.; Löffler, F.; Pasquali, M.; Stergioulas, N. Numerical-relativity simulations of long-lived remnants of binary neutron star mergers. Phys. Rev. D 2020, 101, 064052. [Google Scholar] [CrossRef]
- Murguia-Berthier, A.; Ramirez-Ruiz, E.; De Colle, F.; Janiuk, A.; Rosswog, S.; Lee, W.H. The Fate of the Merger Remnant in GW170817 and Its Imprint on the Jet Structure. Astrophys. J. 2021, 908, 152. [Google Scholar] [CrossRef]
- Klebesadel, R.W.; Strong, I.B.; Olson, R.A. Observations of Gamma-Ray Bursts of Cosmic Origin. Astrophys. J. 1973, 182, L85. [Google Scholar] [CrossRef]
- Band, D.; Matteson, J.; Ford, L.; Schaefer, B.; Palmer, D.; Teegarden, B.; Cline, T.; Briggs, M.; Paciesas, W.; Pendleton, G.; et al. BATSE Observations of Gamma-Ray Burst Spectra. I. Spectral Diversity. Astrophys. J. 1993, 413, 281. [Google Scholar] [CrossRef]
- Paciesas, W.S.; Meegan, C.A.; Pendleton, G.N.; Briggs, M.S.; Kouveliotou, C.; Koshut, T.M.; Lestrade, J.P.; McCollough, M.L.; Brainerd, J.J.; Hakkila, J.; et al. The Fourth BATSE Gamma-Ray Burst Catalog (Revised). Astrophys. J. Suppl. Ser. 1999, 122, 465. [Google Scholar] [CrossRef]
- Nakar, E. Short-Hard Gamma-Ray Bursts. Phys. Rep. 2007, 442, 166. [Google Scholar] [CrossRef]
- Berger, E. Short-Duration Gamma-Ray Bursts. Annu. Rev. Astron. Astrophys. 2015, 52, 43–105. [Google Scholar] [CrossRef]
- Amati, L.; Frontera, F.; Tavani, M.; in ’t Zand, J.J.M.; Antonelli, A.; Costa, E.; Feroci, M.; Guidorzi, C.; Heise, J.; Masetti, N.; et al. Intrinsic spectra and energetics of BeppoSAX Gamma-Ray Bursts with known redshifts. Astron. Astrophys. 2002, 390, 81. [Google Scholar] [CrossRef]
- Ghirlanda, G.; Ghisillini, G.; Lazzati, D. The Collimation-corrected Gamma-Ray Burst Energies Correlate with the Peak Energy of Their νFν Spectrum. Astrophys. J. 2004, 616, 331. [Google Scholar] [CrossRef]
- Nava, L.; Ghisellini, G.; Ghirlanda, G.; Tavecchio, F.; Firmani, C. On the interpretation of spectral-energy correlations in long gamma-ray bursts. Astron. Astrophys. 2006, 450, 471. [Google Scholar] [CrossRef]
- Campana, S.; Guidorzi, C.; Tagliaferri, G.; Chincarini, G.; Moretti, A.; Rizzuto, D.; Romano, P. Are Swift gamma-ray bursts consistent with the Ghirlanda relation? Astron. Astrophys. 2007, 472, 395–401. [Google Scholar] [CrossRef]
- Ghisellini, G.; Celotti, A.; Ghirlanda, G.; Firmani, C.; Nava, L. Re-born fireballs in gamma-ray bursts. Mon. Not. R. Astron. Soc. 2007, 382, L72. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M. Nonthermal High-Energy Emissions from Black Holes by a Relativistic Capillary Effect. Astrophys. J. 2008, 685, L63. [Google Scholar] [CrossRef]
- Shahmoradi, A.; Nemiroff, R.J. Short versus long gamma-ray bursts: A comprehensive study of energetics and prompt gamma-ray correlations. Mon. Not. R. Astron. Soc. 2015, 451, 126. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M.; Levinson, A. Relativistic Astrophysics of the Transient Universe; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Zhang, B. The delay time of gravitational wave—Gamma-ray burst associations. Front. Phys. 2019, 14, 64402. [Google Scholar] [CrossRef]
- Lazzati, D.; Ciolfi, R.; Perna, R. Intrinsic Properties of the Engine and Jet that Powered the Short Gamma-Ray Burst Associated with GW170817. Astrophys. J. 2020, 898, 59. [Google Scholar] [CrossRef]
- Sari, R.; Piran, T. Variability in Gamma-Ray Bursts: A Clue. Astrophys. J. 1997, 485, 270. [Google Scholar] [CrossRef]
- Piran, T.; Sari, R. Implications of Temporal Structure in GRBs. In Proceedings of the 18th Texas Symposium on Relativistic Astrophysics and Cosmology, Chicago, IL, USA, 15–20 December 1996; Olinto, A.V., Frieman, J.A., Schramm, D.N., Eds.; p. 494. [Google Scholar]
- Kobayashi, S.; Piran, T.; Sari, R. Can Internal Shocks Produce the Variability in Gamma-Ray Bursts? Astrophys. J. 1997, 490, 92. [Google Scholar] [CrossRef]
- Nakar, E.; Piran, T. Temporal properties of short gamma-ray bursts. Mon. Not. R. Astron. Soc. 2002, 330, 920. [Google Scholar] [CrossRef]
- Eichler, D.; Livio, M.; Piran, T.; Schramm, D.N. Nucleosynthesis, neutrino bursts and γ-rays from coalescing neutron stars. Nature 1989, 340, 126. [Google Scholar] [CrossRef]
- Woosley, S.E. Gamma-ray bursts from stellar mass accretion disks around black holes. Astrophys. J. 1993, 405, 273. [Google Scholar] [CrossRef]
- Paczynski, B. Are Gamma-Ray Bursts in Star-Forming Regions? Astrophys. J. 1998, 494, L45. [Google Scholar] [CrossRef]
- Paczynski, B. Cosmological gamma-ray bursts. Acta Astron. 1991, 41, 257. [Google Scholar]
- Levinson, A.; Eichler, D. Baryon Purity in Cosmological Gamma-Ray Bursts as a Manifestation of Event Horizons. Astrophys. J. 1993, 418, 386. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M.; Levinson, A. Theory and Astrophysical Consequences of a Magnetized Torus around a Rapidly Rotating Black Hole. Astrophys. J. 2003, 584, 937. [Google Scholar] [CrossRef]
- Paczynski, B. Gamma-ray bursters at cosmological distances. Astrophys. J. 1986, 308, L43. [Google Scholar] [CrossRef]
- Gal-Yam, A.; Bruch, R.; Schulze, S.; Yang, Y.; Perley, D.A.; Irani, I.; Sollerman, J.; Kool, E.C.; Soumagnac, M.T.; Yaron, O.; et al. A WC/WO star exploding within an expanding carbon-oxygen-neon nebula. Nature 2022, 601, 201. [Google Scholar] [CrossRef]
- Costa, E.; Frontera, F.; Heise, J.; Feroci, M.; in’t Zand, J.; Fiore, F.; Cinti, M.N.; Dal Fiume, D.; Nicastro, L.; Orlandini, M.; et al. Discovery of an X-ray afterglow associated with the γ-ray burst of 28 February 1997. Nature 1997, 387, 783. [Google Scholar] [CrossRef]
- van Paradijs, J.; Groot, P.J.; Galama, T.; Kouveliotou, C.; Strom, R.G.; Telting, J.; Rutten, R.G.M.; Fishman, G.J.; Meegan, C.A.; Pettini, M.; et al. Transient optical emission from the error box of the γ-ray burst of 28 February 1997. Nature 1997, 386, 686–689. [Google Scholar] [CrossRef]
- Metzger, M.R.; Djorgovski, S.G.; Kulkarni, S.R.; Steidel, C.C.; Adelberger, K.L.; Frail, D.A.; Costa, E.; Frontera, F. Spectral constraints on the redshift of the optical counterpart to the γ-ray burst of 8 May 1997. Nature 1997, 387, 879. [Google Scholar] [CrossRef]
- Amati, L.; Piro, L.; Antonelli, A.; Butler, R.C.; Costa, E.; Cusumano, G.; Feroci, M.; Frontera, F.; Heise, J.; in’t Zand, J.; et al. BeppoSAX observations of GRB970508: First evidence of bursting activity continuing on very long time scale. Nucl. Phys.-Proc. Suppl. 1998, 69, 656–659. [Google Scholar] [CrossRef]
- Bloom, J.S.; Djorgovski, S.G.; Kulkarni, S.R. The redshift and the ordinary host galaxy of GRB 970228. Astrophys. J. 2001, 554, 678. [Google Scholar] [CrossRef]
- Galama, T.J.; Vreeswijk, P.M.; van Paradijs, J.; Kouveliotou, C.; Augusteijn, T.; Patat, F.; Heise, J.; in’t Zand, J.; Groot, P.J.; Wijers, R.A.M.J.; et al. On the possible association of SN 1998bw and GRB 980425. Nature 1998, 395, 670. [Google Scholar] [CrossRef]
- Hjorth, J.; Sollerman, J.; Møller, P.; Fynbo, J.P.U.; Woosley, S.E.; Kouveliotou, C.; Tanvir, N.R.; Greiner, J.; Andersen, M.I.; Castro-Tirado, A.J.; et al. A very energetic supernova associated with the γ-ray burst of 29 March 2003. Nature 2003, 423, 847. [Google Scholar] [CrossRef] [PubMed]
- Stanek, K.Z.; Matheson, T.; Garnavich, P.M.; Martini, P.; Berlind, P.; Caldwell, N.; Challis, P.; Brown, W.R.; Schild, R.; Krisciunas, K.; et al. Spectroscopic Discovery of the Supernova 2003dh Associated with GRB 030329. Astrophys. J. 2003, 591, L17. [Google Scholar] [CrossRef]
- Matheson, T.; Garnavich, P.M.; Stanek, K.Z.; Bersier, D.; Holland, S.T.; Krisciunas, K.; Caldwell, N.; Berlind, P.; Bloom, J.S.; Bolte, M.; et al. Photometry and Spectroscopy of GRB 030329 and Its Associated Supernova 2003dh: The First Two Months. Astrophys. J. 2003, 599, 394. [Google Scholar] [CrossRef]
- Modjaz, M.; Stanek, K.Z.; Garnavich, P.M.; Berlind, P.; Blondin, S.; Brown, W.; Calkins, M.; Challis, P.; Diamond-Stanic, A.M.; Hao, H.; et al. Early-Time Photometry and Spectroscopy of the Fast Evolving SN 2006aj Associated with GRB 060218. Astrophys. J. 2006, 645, L21. [Google Scholar] [CrossRef]
- Guetta, D.; Della Valle, M. On the Rates of Gamma-Ray Bursts and Type Ib/c Supernovae. Astrophys. J. 2007, 657, L73. [Google Scholar] [CrossRef]
- Kelly, P.L.; Kirshner, R.P.; Kahre, M. Long γ-Ray Bursts and Type Ic Core-Collapse Supernovae Have Similar Locations in Hosts. Astrophys. J. 2008, 687, 1201. [Google Scholar] [CrossRef]
- Amati, L.; Della Valle, M.; Frontera, F.; Malesani, D.; Guidorzi, C.; Montanari, E.; Pian, E. On the consistency of peculiar GRBs 060218 and 060614. with the Ep,i − Eiso correlation. Astron. Astrophys. 2007, 463, 913. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M.; Lee, G.M.; Della Valle, M.; Amati, L.; Levinson, A. On the origin of short GRBs with extended emission and long GRBs without associated SN. Mon. Not. R. Astron. Soc. 2014, 444, L58. [Google Scholar] [CrossRef]
- Della Valle, M.; Chincarini, G.; Panagia, N.; Tagliaferri, G.; Malesani, D.; Testa, V.; Fugazza, D.; Campana, S.; Covino, S.; Mangano, V.; et al. An enigmatic long-lasting gamma-ray burst not accompanied by a bright supernova. Nature 2006, 444, 1050. [Google Scholar] [CrossRef]
- Fynbo, J.P.U.; Watson, D.; Thoene, C.C.; Sollerman, J.; Bloom, J.S.; Davis, T.M.; Hjorth, J.; Jakobsson, P.; Joergensen, U.G.; Graham, J.F.; et al. No supernovae associated with two long-duration γ-ray bursts. Nature 2006, 444, 1047. [Google Scholar] [CrossRef]
- Gehrels, N.; Norris, J.P.; Barthelmy, S.D.; Granot, J.; Kaneko, Y.; Kouveliotou, C.; Markwardt, C.B.; Mészáros, P.; Nakar, E.; Nousek, J.A.; et al. A new γ-ray burst classification scheme from GRB060614. Nature 2006, 444, 1044. [Google Scholar] [CrossRef]
- Gal-Yam, A.; Ofek, E.O.; Poznanski, D.; Levinson, A.; Waxman, E.; Frail, D.A.; Soderberg, A.M.; Nakar, E.; Li, W.D.; Filippenko, A.V. Radio and Optical Follow-up Observations of a Uniform Radio Transient Search: Implications for Gamma-Ray Bursts and Supernovae. Astrophys. J. 2006, 639, 331. [Google Scholar] [CrossRef]
- Gal-Yam, A.; Fox, D.B.; Price, P.A.; Ofek, E.O.; Davis, M.R.; Leonard, D.C.; Soderberg, A.M.; Schmidt, B.P.; Lewis, K.M.; Peterson, B.A.; et al. A novel explosive process is required for the γ-ray burst GRB 060614. Nature 2006, 444, 1053. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M. Superradiance in a torus magnetosphere around a black hole. Science 1999, 284, 115. [Google Scholar] [CrossRef]
- Frail, D.A.; Kulkarni, S.R.; Sari, R.; Djorgovski, S.G.; Bloom, J.S.; Galama, T.J.; Reichart, D.E.; Berger, E.; Harrison, F.A.; Price, P.A.; et al. Beaming in Gamma-Ray Bursts: Evidence for a Standard Energy Reservoir. Astrophys. J. 2001, 567, L41. [Google Scholar] [CrossRef]
- Ghirlanda, G.; Ghisillini, G.; Firmani, C. Gamma-ray bursts as standard candles to constrain the cosmological parameters. New J. Phys. 2006, 8, 123. [Google Scholar] [CrossRef]
- Ghirlanda, G.; Ghisellini, G.; Salvaterra, R.; Nava, L.; Burlon, D.; Tagliaferri, G.; Campana, S.; D’Avanzo, P.; Melandri, A. The faster the narrower: Characteristic bulk velocities and jet opening angles of gamma-ray bursts. Mon. Not. R. Astron. Soc. 2013, 428, 123. [Google Scholar] [CrossRef]
- Reichart, D.E.; Lamb, D.Q.; Fenimore, E.E.; Ramirez-Ruiz, E.; Cline, T.L.; Hurley, K. A Possible Cepheid-like Luminosity Estimator for the Long Gamma-Ray Bursts. Astrophys. J. 2001, 552, 57. [Google Scholar] [CrossRef]
- Levinson, A.; Boldt, E. UHECR production by a compact black hole dynamo: Application to Sgr A*. Astrop. Phys. 2002, 16, 265. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M.; Gupta, A.C. Non-thermal transient sources from rotating black holes. Mon. Not. R. Astron. Soc. 2009, 394, 2238. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M. Extreme luminosities in ejecta produced by intermittent outflows around rotating black holes. Mon. Not. R. Astron. Soc. 2015, 447, L11. [Google Scholar] [CrossRef]
- Moncada, R.J.; Colon, R.A.; Guerra, J.J.; O’Dowd, M.J.; Anchordoqui, L.A. Ultrahigh energy cosmic ray nuclei from remnants of dead quasars. J. High Energy Astrophys. 2017, 13, 32. [Google Scholar] [CrossRef]
- Gottlieb, O.; Bromberg, O.; Levinson, A. Intermittent mildly magnetized jets as the source of GRBs. Mon. Not. R. Astron. Soc. 2021, 504, 3947. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M. Electron-Positron Outflow from Black Holes. Phys. Rev. Lett. 2000, 84, 3752. [Google Scholar] [CrossRef] [PubMed]
- van Putten, M.H.P.M.; Levinson, A.; Frontera, F.; Guidorzi, C.; Amati, L.; Della Valle, M. Prospects for multi-messenger extended emission from core-collapse supernovae in the Local Universe. EPJ Plus 2019, 134, 547. [Google Scholar] [CrossRef]
- Mirabel, I.F.; Rodriguez, L.F. A superluminal source in the Galaxy. Nature 1994, 371, 46. [Google Scholar] [CrossRef]
- Piran, T. The physics of gamma-ray bursts. Rev. Mod. Phys. 2004, 76, 1143. [Google Scholar] [CrossRef]
- Thompson, C. A model of gamma-ray bursts. Mon. Not. R. Astron. Soc. 1994, 270, 480. [Google Scholar] [CrossRef]
- Metzger, B.D.; Giannios, D.; Thompson, T.A.; Bucciantini, N.; Quataert, E. The protomagnetar model for gamma-ray bursts. Mon. Not. R. Astron. Soc. 2011, 413, 2031. [Google Scholar] [CrossRef]
- Piran, T.; Nakar, E.; Mazzali, P.; Pian, E. Relativistic Jets in Core-collapse Supernovae. Astrophys. J. 2019, 871, L25. [Google Scholar] [CrossRef]
- Nakar, E. The electromagnetic counterparts of compact binary mergers. Phys. Rep. 2020, 886, 1–84. [Google Scholar] [CrossRef]
- Hewish, A. Pulsars. Annu. Rev. Astron. Astrophys. 1970, 8, 265. [Google Scholar] [CrossRef]
- Hulse, R.A.; Taylor, J.H. Discovery of a pulsar in a binary system. Astrophys. J. 1975, 195, L51. [Google Scholar] [CrossRef]
- Burgay, M.; D’Amico, N.; Possenti, A.; Manchester, R.N.; Lyne, A.G.; Joshi, B.C.; McLaughlin, M.A.; Kramer, M.; Sarkissian, J.M.; Camilo, F.; et al. An increased estimate of the merger rate of double neutron stars from observations of a highly relativistic system. Nature 2003, 426, 531–533. [Google Scholar] [CrossRef]
- Carter, B. Global Structure of the Kerr Family of Gravitational Fields. Phys. Rev. 1968, 174, 1559. [Google Scholar] [CrossRef]
- Wald, R.M. Black hole in a uniform magnetic field. Phys. Rev. D 1974, 10, 1680. [Google Scholar] [CrossRef]
- Kerr, R.P. Gravitational Field of a Spinning Mass as an Example of Algebraically Special Metrics. Phys. Rev. Lett. 1963, 11, 237. [Google Scholar] [CrossRef]
- LSC. LSC-Virgo White Paper on GW Data Analysis and Astrophysics, LIGO T1800058-v2. 2018. Available online: https://dcc.ligo.org/LIGO-T1800058/public (accessed on 25 May 2023).
- van Putten, M.H.P.M.; Della Valle, M.; Levinson, A. Multi-messenger Extended Emission from the Compact Remnant in GW170817. Astrophys. J. 2019, 876, L2. [Google Scholar] [CrossRef]
- Coulter, D.A.; Foley, R.J.; Kilpatrick, C.D.; Drout, M.R.; Piro, A.L.; Shappee, B.J.; Siebert, M.R.; Simon, J.D.; Ulloa, N.; Kasen, D.; et al. Swope Supernova Survey 2017a (SSS17a), the optical counterpart to a gravitational wave source. Science 2017, 358, 1556. [Google Scholar] [CrossRef]
- Cantiello, M.; Jensen, J.B.; Blakeslee, J.P.; Berger, E.; Levan, A.J.; Tanvir, N.R.; Raimondo, G.; Brocato, E.; Alexander, K.D.; Blanchard, P.K.; et al. A Precise Distance to the Host Galaxy of the Binary Neutron Star Merger GW170817 Using Surface Brightness Fluctuations. Astrophys. J. 2018, 854, L31. [Google Scholar] [CrossRef]
- Abbott, B.P. et al. [The LIGO Scientific Collaboration and the Virgo Collaboration] GW170817: Measurements of Neutron Star Radii and Equation of State. Phys. Rev. Lett. 2018, 121, 161101. [Google Scholar] [CrossRef] [PubMed]
- Drago, A.; Pagliara, G. Merger of Two Neutron Stars: Predictions from the Two-families Scenario. Astrophys. J. 2018, 852, L32. [Google Scholar] [CrossRef]
- De Pietri, R.; Drago, A.; Feo, A.; Pagliara, G.; Pasquali, M.; Traversi, S.; Wiktorowicz, G. Merger of compact stars in the two-families scenario. Astrophys. J. 2019, 881, 122. [Google Scholar] [CrossRef]
- Bauswein, A. Equation of state constraints from multi-messenger observations of neutron star mergers. Ann. Phys. 2019, 411, 167958. [Google Scholar] [CrossRef]
- Acernese, F.; Amico, P.; Alshourbagy, M.; Antonucci, F.; Aoudia, S.; Astone, P.; Avino, S.; Babusci, B.; Ballardin, G.; Barone, F.; et al. Gravitational waves by gamma-ray bursts and the Virgo detector: The case of GRB 050915a. Class. Quantum Grav. 2007, 24, S671. [Google Scholar] [CrossRef]
- Cutler, C.; Thorne, K.S. An Overview of Gravitational-Wave Sources. In Proceedings of the 16th International Conference, Durban, South Africa, 15–21 July 2001; Bishop, N.T., Maharaj, S.D., Eds.; [Google Scholar]
- Pozanenko, A.S.; Barkov, M.V.; Minaev, P.Y.; Volnova, A.A.; Mazaeva, E.D.; Moskvitin, A.S.; Krugov, M.A.; Samodurov, V.A.; Loznikov, V.M.; Lyutikov, M. GRB 170817A Associated with GW170817: Multi-frequency Observations and Modeling of Prompt Gamma-Ray Emission. Astrophys. J. 2018, 852, L30. [Google Scholar] [CrossRef]
- Akutsu, M. et al. [KAGRA Collaboration] Overview of KAGRA: KAGRA science. Prog. Theor. Exp. Phys. 2021, 05A103. [Google Scholar] [CrossRef]
- Fernández, R.; Metzger, B.D. Delayed outflows from black hole accretion tori following neutron star binary coalescence. Mon. Not. R. Astron. Soc. 2013, 435, 502. [Google Scholar] [CrossRef]
- Just, O.; Bauswein, A.; Ardevol Pulpillo, R.; Goriely, S.; Thomas Janka, H. Comprehensive nucleosynthesis analysis for ejecta of compact binary mergers. Mon. Not. R. Astron. Soc. 2015, 448, 541. [Google Scholar] [CrossRef]
- Siegel, D.M.; Metzger, B.D. Three-Dimensional General-Relativistic Magnetohydrodynamic Simulations of Remnant Accretion Disks from Neutron Star Mergers: Outflows and r-Process Nucleosynthesis. Phys. Rev. Lett. 2017, 119, 231102. [Google Scholar] [CrossRef]
- Zhu, J.-P.; Yang, Y.-P.; Liu, L.-D.; Huang, Y.; Zhang, B.; Li, Z.; Yu, Y.-W.; Gao, H. Kilonova Emission from Black Hole–Neutron Star Mergers. I. Viewing-angle-dependent Lightcurves. Astrophys. J. 2020, 897, 20. [Google Scholar] [CrossRef]
- Fujibayashi, S.; Shibata, M.; Wanajo, S.; Kiuchi, K.; Kyutoku, K.; Sekiguchi, Y. Mass ejection from disks surrounding a low-mass black hole: Viscous neutrino-radiation hydrodynamics simulation in full general relativity. Phys. Rev. D 2020, 101, 083029. [Google Scholar] [CrossRef]
- Abbott, B.P. et al. [The LIGO Scientific Collaboration and the Virgo Collaboration] A guide to LIGO-Virgo detector noise and extraction of transient gravitational-wave signals. Class. Quantum Grav. 2020, 37, 055002. [Google Scholar] [CrossRef]
- Baiotti, L.; Giacomazzo, B.; Rezolla, L. Accurate evolutions of inspiralling neutron-star binaries: Prompt and delayed gravitational collapse to a black hole. Phys. Rev. D 2008, 78, 084033. [Google Scholar] [CrossRef]
- Dall’Osso, S.; Giacomazzo, B.; Perna, R.; Stella, L. Gravitational waves from massive magnetars formed in binary neutron star mergers. Astrophys. J. 2015, 798, 25. [Google Scholar] [CrossRef]
- Baiotti, L.; Rezzolla, L. Binary neutron-star mergers: A review of Einstein’s richest laboratory. Rep. Prog. Phys. 2017, 80, 096901. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M.; Frontera, F.; Guidorzi, C. Broadband Turbulent Spectra in Gamma-Ray Burst Light Curves. Astrophys. J. 2014, 286, 146. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M. Deep searches for broadband extended gravitational-wave emission bursts by heterogeneous computing. Prog. Theor. Exp. Phys. 2017, 093F01. [Google Scholar] [CrossRef]
- Blocker, C.; Conway, J.; Demortier, L.; Heinrich, J.; Junk, T.; Lyons, L.; Punzi, G. (CDF Statistics Committee). 2006. Available online: http://physics.rockefeller.edu/~luc/technical_reports/cdf8023_facts_about_p_values.pdf (accessed on 25 May 2023).
- Fisher, R.A. Statistical Methods for Research Workers; Oliver and Boyd: Edinburgh, UK, 1932. [Google Scholar]
- Fisher, R.A. Questions and answers #14. Am. Stat. 1948, 2, 30. [Google Scholar]
- Simonson, K.M.; West, R.D.; Hansen, R.L.; LaBruyere, T.E., III; Van Benthem, M.H. A statistical approach to combining multisource information in one-class classifiers. Stat. Anal. Data Min. 2017, 10, 199. [Google Scholar] [CrossRef]
- Rosswog, S.; Liebendörfer, M.; Thielemann, F.-K.; Davies, M.B.; Benz, W.; Piran, T. Mass ejection in neutron star mergers. Astron. Astrophys. 1999, 341, 499–526. [Google Scholar]
- Ciolfi, R. The key role of magnetic fields in binary neutron star mergers. Gen. Rel. Grav. 2020, 52, 59. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M.; Della Valle, M. Central engine of GRB170817A: Neutron star versus Kerr black hole based on multimessenger calorimetry and event timing. Astron. Astrophys. 2023, 669, A36. [Google Scholar] [CrossRef]
- West, R.M.; Lauberts, A.; Jorgensen, H.E.; Schuster, H.E. Astrometry of SN 1987A and Sanduleak-69 202. Astron. Astrophys. 1987, 177, L1. [Google Scholar]
- Pietrzynski, G.; Graczyk, D.; Gallenne, A.; Gieren, W.; Thompson, I.B.; Pilecki, B.; Karczmarek, P.; Gorski, M.; Suchomska, K.; Taormina, M.; et al. A distance to the Large Magellanic Cloud that is precise to one per cent. Nature 2019, 567, 200. [Google Scholar] [CrossRef]
- Hirata, K.; Kajita, T.; Koshiba, M.; Nakahata, M.; Oyama, Y.; Sato, N.; Suzuki, A.; Takita, M.; Totsuka, Y.; Kifune, T.; et al. Observation of a neutrino burst from the supernova SN1987A. Phys. Rev. Lett. 1987, 58, 1490. [Google Scholar] [CrossRef]
- Arnett, W.D.; Bahcall, J.N.; Kirshner, R.P.; Woosley, S.E. Supernova 1987A. Annu. Rev. Astron. Astrophys. 1989, 27, 629. [Google Scholar] [CrossRef]
- Haensel, P.; Zdunik, J.L.; Bejger, M.; Lattimer, J.M. Keplerian frequency of uniformly rotating neutron stars and strange stars. Astron. Astrophys. 2009, 502, 605. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M. Near-extremal black holes as initial conditions of long GRB supernovae and probes of their gravitational-wave emission. Astrophys. J. 2015, 810, 7. [Google Scholar] [CrossRef]
- Bardeen, J.M. Kerr Metric Black Holes. Nature 1970, 226, 64–65. [Google Scholar] [CrossRef]
- Levinson, A.; Globus, N. Ultra-relativistic, neutrino driven flows in GRBs: A double transonic flow solution in Schwarzschild spacetime. Astrophys. J. 2013, 770, 159. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M.; Della Valle, M. Observational evidence for extended emission to GW170817. Mon. Not. R. Astron. Soc. 2019, 482, L46. [Google Scholar] [CrossRef]
- Beniamini, P.; Lu, B. Survival Times of Supramassive Neutron Stars Resulting from Binary Neutron Star Mergers. Astrophys. J. 2021, 920, 109. [Google Scholar] [CrossRef]
- Connaughton, V. >LIGO/Virgo G298048: Fermi GBM Trigger 170817.529 and LIGO Single IFO Trigge. GRB Coordinates Network: 2017; No. 21506. Available online: https://gcn.gsfc.nasa.gov/gcn3/21506.gcn3 (accessed on 25 May 2023).
- Savchenko, V.; Ferrigno, C.; Kuulkers, E.; Bazzano, A.; Bozzo, E.; Brandt, S.; Chenevez, J.; Courvoisier, T.J.-L.; Diehl, R.; Domingo, A.; et al. INTEGRAL Detection of the First Prompt Gamma-Ray Signal Coincident with the Gravitational-wave Event GW170817. Astrophys. J. 2017, 848, L15. [Google Scholar] [CrossRef]
- Mooley, K.P.; Deller, A.T.; Gottlieb, O.; Nakar, E.; Hallinan, G.; Bourke, S.; Frail, D.A.; Horesh, A.; Corsi, A.; Hotokezaka, K. A mildly relativistic wide-angle outflow in the neutron-star merger event GW170817. Nature 2018, 554, 207. [Google Scholar] [CrossRef]
- Mooley, K.P.; Deller, A.T.; Gottlieb, O.; Nakar, E.; Hallinan, G.; Bourke, S.; Frail, D.A.; Horesh, A.; Corsi, A.; Hotokezaka, K. Superluminal motion of a relativistic jet in the neutron-star merger GW170817. Nature 2018, 561, 355. [Google Scholar] [CrossRef]
- Ascenzi, S.; Oganesyan, G.; Branchesi, M.; Ciolfi, R. Electromagnetic Counterparts of Compact Binary Mergers. J. Plasma Phys. 2021, 71, 845870102. [Google Scholar] [CrossRef]
- Bionta, R.M. Observation of a neutrino burst in coincidence with supernova 1987A in the Large Magellanic Cloud. Phys. Rev. Lett. 1987, 58, 1494. [Google Scholar] [CrossRef]
- Imshennik, V.S.; Ryazkshaya, O.G. A Rotating Collapsar and Possible Interpretation of the LSD Neutrino Signal from SN 1987 A. Astron. Lett. 2004, 30, 14. [Google Scholar] [CrossRef]
- Ryazhskaya, O.G. Problems of Neutrino Radiation from SN 1987A: 30 years later. Phys. Atomic Nucl. 2018, 81, 113. [Google Scholar] [CrossRef]
- Galeotti, P.; Pizella, G. Supernova 1987A, 30 Years Later. Phys. At. Nucl. 2018, 81, 105. [Google Scholar]
- Gill, R.; Nathanail, A.; Rezzolla, L. When Did the Remnant of GW170817 Collapse to a Black Hole? Astrophys. J. 2019, 876, 139. [Google Scholar] [CrossRef]
- Goldstein, A.; Veres, P.; Burns, E.; Briggs, M.S.; Hamburg, R.; Kocevski, D.; Wilson-Hodge, C.A.; Preece, R.D.; Poolakkil, S.; Roberts, O.J.; et al. An ordinary short gamma-ray burst with extraordinary implications: Fermi-GBM detection of GRB170817A. Astrophys. J. 2017, 848, L14. [Google Scholar] [CrossRef]
- Ioka, K.; Nakamura, T. Can an off-axis gamma-ray burst jet in GW170817 explain all the electromagnetic counterparts? Prog. Theor. Exp. Phys. 2018, 043E02. [Google Scholar] [CrossRef]
- Lazzati, D. Short Duration Gamma-Ray Bursts and Their Outflows in Light of GW170817. Front. Astron. Space Sci. 2020, 7, 78. [Google Scholar] [CrossRef]
- Mooley, K.P.; Anderson, J.; Lu, W.B. Optical measurement of superluminal motion in the neutron-star merger GW170817. Nature 2022, 610, 273. [Google Scholar] [CrossRef]
- Smartt, S.J.; Chen, T.-W.; Jerkstrand, A.; Coughlin, M.; Kankare, E.; Sim, S.A.; Fraser, M.; Inserra, C.; Maguire, K.; Chambers, K.C.; et al. A kilonova as the electromagnetic counterpart to a gravitational-wave source. Nature 2017, 551, 75. [Google Scholar] [CrossRef]
- Pian, E.; D’Avanzo, P.; Benetti, S.; Branchesi, M.; Brocato, E.; Campana, S.; Cappellaro, E.; Covino, S.; D’Elia, V.; Fynbo, J.P.U.; et al. Spectroscopic identification of r-process nucleosynthesis in a double neutron-star merger. Nature 2017, 551, 67. [Google Scholar] [CrossRef]
- Pooley, D.; Kumar, P.; Wheeler, J.C.; Grossan, B. GW170817 Most Likely Made a Black Hole. Astrophys. J. 2018, 859, L23. [Google Scholar] [CrossRef]
- Radice, D.; Perego, A.; Hotokezaka, K.; Fromm, S.A.; Bernuzzi, S.; Roberts, L.F. Binary Neutron Star Mergers: Mass Ejection, Electromagnetic Counterparts and Nucleosynthesis. Astrophys. J. 2018, 869, 130. [Google Scholar] [CrossRef]
- Lucca, M.; Sagunski, L. The lifetime of binary neutron star merger remnants. J. High Energy Astrophys. 2020, 27, 33–37. [Google Scholar] [CrossRef]
- Ren, J.; Lin, D.B.; Zhang, L.L.; Li, X.Y.; Liu, T.; Lu, R.J.; Wang, X.G.; Liang, E.W. A Pulsar Wind Nebula Embedded in the Kilonova AT 2017gfo Associated with GW170817/GRB 170817A. Astrophys. J. 2019, 885, 60. [Google Scholar] [CrossRef]
- Ravi, V.; Lasky, P.D. The birth of black holes: Neutron star collapse times, gamma-ray bursts and fast radio bursts. Mon. Not. R. Astron. Soc. 2014, 441, 2433. [Google Scholar]
- Lü, H.-J.; Shen, J.; Lan, L.; Rice, J.; Lei, W.-H.; Liang, E.-W. Diagnosing the remnants of binary neutron star merger from GW170817/GRB170817A event. Mon. Not. R. Astron. Soc. 2019, 486, 4479–4484. [Google Scholar] [CrossRef]
- Piro, L.; Troja, E.; Zhang, B.; Ryan, G.; van Eerten, H.; Ricci, R.; Wieringa, M.H.; Tiengo, A.; Butler, N.R.; Cenko, S.B.; et al. A long-lived neutron star merger remnant in GW170817: Constraints and clues from X-ray observations. Mon. Not. R. Astron. Soc. 2019, 483, 1912. [Google Scholar] [CrossRef]
- Granot, J.; Guetta, D.; Gill, R. Lessons from the Short GRB 170817A: The First Gravitational-wave Detection of a Binary Neutron Star Merger. Astrophys. J. Lett. 2017, 850, L24. [Google Scholar] [CrossRef]
- Gottlieb, O.; Nakar, E.; Piran, T.; Hotokezaka, K. A cocoon shock breakout as the origin of the γ-ray emission in GW170817. Mon. Not. R. Astron. Soc. 2018, 479, 588. [Google Scholar] [CrossRef]
- Nakar, E.; Gottlieb, O.; Piran, T.; Kasliwal, M.M.; Hallinan, G. From γ to Radio: The Electromagnetic Counterpart of GW170817. Astrophys. J. 2018, 867, 18. [Google Scholar] [CrossRef]
- Metzger, B.D.; Thompson, T.A.; Quataert, E.A. A Magnetar Origin for the Kilonova Ejecta in GW170817. Astrophys. J. 2018, 856, 101. [Google Scholar] [CrossRef]
- Xie, X.; Zrake, J.; MacFadyen, A. Numerical Simulations of the Jet Dynamics and Synchrotron Radiation of Binary Neutron Star Merger Event GW170817/GRB 170817A. Astrophys. J. 2018, 863, 58. [Google Scholar] [CrossRef]
- Hamidani, H.; Kiuchi, K.; Ioka, K. Jet propagation in neutron star mergers and GW170817. Mon. Not. R. Astron. Soc. 2020, 491, 3192. [Google Scholar] [CrossRef]
- Abchouyey, M.A.; van Putten, M.H.P.M.; Amati, L. Observational prospects of double neutrons star mergers and their multi-messenger afterglows: LIGO discovery power, event rates and diversity. Astrophys. J. 2023; to appear. [Google Scholar]
- van Putten, M.H.P.M.; van Putten, P.F.A.M. Roulette Registration System. US Patent 5785321, 27 July 1998. Available online: https://portal.unifiedpatents.com/patents/patent/US-5785321-A (accessed on 25 May 2023).
- Theiler, J. Combining Statistical Tests by Multiplying p-Values. Astrophysics and Radiation Measurements Group: NIS-2, 2004; Available online: https://citeseerx.ist.psu.edu (accessed on 25 May 2023).
- Heard, N.; Rubin-Delancy, P. Choosing between Methods of Combining p-values. Biometrika 2018, 105, 239. [Google Scholar] [CrossRef]
- Whitlock, M.C. Combining probability from independent tests: The weighted Z-method is superior to Fisher’s approach. J. Evol. Biol. 2005, 18, 1368. [Google Scholar]
- Accadia, T.; Acernese, F.; Antonucci, F.; Astone, P.; Ballardin, G.; Barone, F.; Barsuglia, M.; Bauer, T.S.; Beker, M.G.; Belletoile, A.; et al. Noise from scattered light in Virgo’s second science run data. Class. Quantum Grav. 2010, 27, 194011. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M. Directed searches for broadband extended emission in gravitational-wave emission from nearby energetic core-collapse supsernovae. Astrophys. J. 2016, 810, 7. [Google Scholar] [CrossRef]
- Abbott, B.P. et al. [The LIGO Scientific Collaboration and the Virgo Collaboration] Search for Gravitational Waves from a Long-lived Remnant of the Binary Neutron Star Merger GW170817. Astrophys. J. 2019, 875, 160. [Google Scholar] [CrossRef]
- Abbott, B.P. et al. [The LIGO Scientific Collaboration and the Virgo Collaboration] [The LIGO Scientific Collaboration and the Virgo Collaboration]. Model comparison from LIGO-Virgo data on GW170817’s binary components and consequences for the merger remnant. Class. Quantum Grav. 2020, 37, 045006. [Google Scholar] [CrossRef]
- Sun, L.; Melatos, A. Application of hidden Markov model tracking to the search for long-duration transient gravitational waves from the remnant of the binary neutron star merger GW170817. Phys. Rev. D 2019, 99, 123003. [Google Scholar] [CrossRef]
- AMD Inc. 2022. Available online: https://sep5.readthedocs.io/en/latest/Programming_Guides/Opencl-programming-guide.html (accessed on 25 May 2023).
- Khronos Group. 2022. Available online: https://www.khronos.org/opencl (accessed on 25 May 2023).
- Rocki, K.; Van Essendelft, D.; Sharapov, I.; Schreiber, R.; Morrison, M.; Kibardin, V.; Portnoy, A.; Dietiker, J.F.; Syamlal, M.; James, M. Fast Stencil-Code Computation on a Wafer-Scale Processor. In Proceedings of the SC20: International Conference for High Performance Computing, Networking, Storage and Analysis, Atlanta, GA, USA, 9–19 November 2020. [Google Scholar]
- van Putten, M.H.P.M. Method for High-Throughput Data-Processing by Distributed Heterogeneous Computing. US Patent Appl. 18/182,338, 12 March 2023. [Google Scholar]
- Huntington, A.S.; Williams, G.M.; Lee, A.O. Modeling false alarm rate and related characteristics of laser ranging and LIDAR avalanche photodiode photoreceivers. Opt. Eng. 2018, 57, 073106. [Google Scholar] [CrossRef]
- Alp, D.; Larsson, J.; Fransson, C.; Indebetouw, R.; Jerkstrand, A.; Ahola, A.; Burrows, D.; Challis, P.; Cigan, P.; Cikota, A. The 30 Year Search for the Compact Object in SN 1987A. Astrophys. J. 2018, 864, 174. [Google Scholar] [CrossRef]
- Coughlin, M.W.; Dietrich, T. Can a black hole–neutron star merger explain GW170817, AT2017gfo, and GRB170817A? Phys. Rev. D 2019, 100, 043011. [Google Scholar] [CrossRef]
- Dong, S.; Shappee, B.J.; Prieto, J.L.; Jha, S.W.; Stanek, K.Z.; Holoien, T.W.-S.; Kochanek, C.S.; Thompson, T.A.; Morrell, N.; Thompson, I.B.; et al. ASASSN-15lh: A highly super-luminous supernova. Science 2015, 351, 6270. [Google Scholar] [CrossRef]
- van Putten, M.H.P.M.; Della Valle, M. On extreme transient events from rotating black holes and their gravitational wave emission. Mon. Not. R. Astron. Soc. 2017, 464, 3219. [Google Scholar] [CrossRef]





| H1 | L1 | H1 | L1 | H1,L1 | H1,L1 | Merged (H1,L1) | Merged (H1,L1) |
|---|---|---|---|---|---|---|---|
| [s] | [s] | [s] | [s] | [s] | [s] | [s] | [s] |
| T [s] | FAR | FAR | FAR | ||||
| 2048 | 1 month | 1.4 yr | 1.6 kyr | ||||
| 204,800 | 1.7/204,800 | 782 yr | 1.4 yr | > kyr |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
van Putten, M.H.P.M. The Central Engine of GRB170817A and the Energy Budget Issue: Kerr Black Hole versus Neutron Star in a Multi-Messenger Analysis. Universe 2023, 9, 279. https://doi.org/10.3390/universe9060279
van Putten MHPM. The Central Engine of GRB170817A and the Energy Budget Issue: Kerr Black Hole versus Neutron Star in a Multi-Messenger Analysis. Universe. 2023; 9(6):279. https://doi.org/10.3390/universe9060279
Chicago/Turabian Stylevan Putten, Maurice H. P. M. 2023. "The Central Engine of GRB170817A and the Energy Budget Issue: Kerr Black Hole versus Neutron Star in a Multi-Messenger Analysis" Universe 9, no. 6: 279. https://doi.org/10.3390/universe9060279
APA Stylevan Putten, M. H. P. M. (2023). The Central Engine of GRB170817A and the Energy Budget Issue: Kerr Black Hole versus Neutron Star in a Multi-Messenger Analysis. Universe, 9(6), 279. https://doi.org/10.3390/universe9060279






