Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism
Abstract
1. Introduction
2. Relativistic Stars in General Relativity
3. Vanishing Complexity Factor Formalism
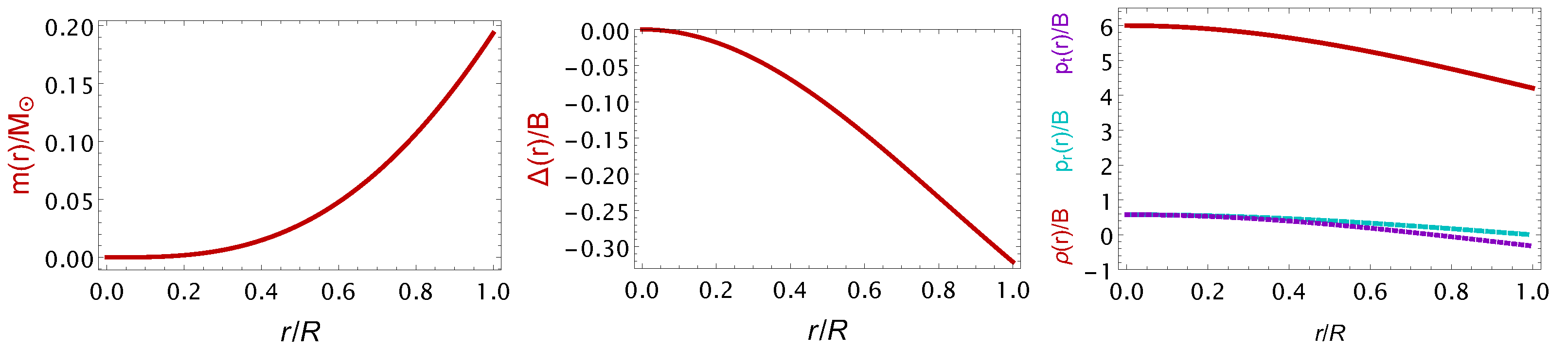
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Shapiro, S.L.; Teukolsky, S.A. Black Holes, White Dwarfs, and Neutron Stars: The Physics of Compact Objects; John Wiley & Sons: Hoboken, NJ, USA, 1983. [Google Scholar]
- Psaltis, D. Probes and Tests of Strong-Field Gravity with Observations in the Electromagnetic Spectrum. Living Rev. Rel. 2008, 11, 9. [Google Scholar] [CrossRef]
- Lorimer, D.R. Binary and Millisecond Pulsars. Living Rev. Rel. 2008, 11, 8. [Google Scholar] [CrossRef] [PubMed]
- Zorotovic, M.; Schreiber, M.R. Cataclysmic variable evolution and the white dwarf mass problem: A Review. Adv. Space Res. 2020, 66, 1080–1089. [Google Scholar] [CrossRef]
- Ruiter, A.J. Type Ia supernova sub-classes and progenitor origin. IAU Symp. 2020, 357, 1–15. [Google Scholar] [CrossRef]
- Burrows, A.; Vartanyan, D. Core-collapse supernova explosion theory. Nature 2021, 589, 29–39. [Google Scholar] [CrossRef]
- Einstein, A. The Field Equations of Gravitation. Sitzungsber. Preuss. Akad. Wiss. Berl. (Math. Phys.) 1915, 1915, 844–847. [Google Scholar]
- Mösta, P.; Ott, C.D.; Radice, D.; Roberts, L.F.; Schnetter, E.; Haas, R. A large-scale dynamo and magnetoturbulence in rapidly rotating core-collapse supernovae. Nature 2015, 528, 376–379. [Google Scholar] [CrossRef]
- Guilet, J.; Müller, E. Numerical simulations of the magnetorotational instability in protoneutron stars—I. Influence of buoyancy. Mon. Not. R. Astron. Soc. 2015, 450, 2153–2171. [Google Scholar] [CrossRef]
- Kondratyev, V.N. R-Process with Magnetized Nuclei at Dynamo-Explosive Supernovae and Neutron Star Mergers. Universe 2021, 7, 487. [Google Scholar] [CrossRef]
- Alcock, C.; Farhi, E.; Olinto, A. Strange stars. Astrophys. J. 1986, 310, 261–272. [Google Scholar] [CrossRef]
- Alcock, C.; Olinto, A. Exotic Phases of Hadronic Matter and their Astrophysical Application. Ann. Rev. Nucl. Part. Sci. 1988, 38, 161–184. [Google Scholar] [CrossRef]
- Madsen, J. Physics and astrophysics of strange quark matter. Lect. Notes Phys. 1999, 516, 162–203. [Google Scholar] [CrossRef]
- Weber, F. Strange quark matter and compact stars. Prog. Part. Nucl. Phys. 2005, 54, 193–288. [Google Scholar] [CrossRef]
- Yue, Y.L.; Cui, X.H.; Xu, R.X. Is psr b0943+10 a low-mass quark star? Astrophys. J. Lett. 2006, 649, L95–L98. [Google Scholar] [CrossRef]
- Leahy, D.; Ouyed, R. Supernova SN2006gy as a first ever Quark Nova? Mon. Not. R. Astron. Soc. 2008, 387, 1193. [Google Scholar] [CrossRef]
- Witten, E. Cosmic Separation of Phases. Phys. Rev. D 1984, 30, 272–285. [Google Scholar] [CrossRef]
- Farhi, E.; Jaffe, R.L. Strange Matter. Phys. Rev. D 1984, 30, 2379. [Google Scholar] [CrossRef]
- Henderson, J.A.; Page, D. RX J1856.5-3754 as a possible Strange Star candidate. Astrophys. Space Sci. 2007, 308, 513–517. [Google Scholar] [CrossRef]
- Li, A.; Peng, G.X.; Lu, J.F. Strange star candidates revised within a quark model with chiral mass scaling. Res. Astron. Astrophys. 2011, 11, 482–490. [Google Scholar] [CrossRef]
- Aziz, A.; Ray, S.; Rahaman, F.; Khlopov, M.; Guha, B.K. Constraining values of bag constant for strange star candidates. Int. J. Mod. Phys. D 2019, 28, 1941006. [Google Scholar] [CrossRef]
- Benic, S.; Blaschke, D.; Alvarez-Castillo, D.E.; Fischer, T.; Typel, S. A new quark-hadron hybrid equation of state for astrophysics—I. High-mass twin compact stars. Astron. Astrophys. 2015, 577, A40. [Google Scholar] [CrossRef]
- Yazdizadeh, T.; Bordbar, G.; Panah, B.E. The structure of hybrid neutron star in Einstein-λ gravity. Phys. Dark Universe 2022, 35, 100982. [Google Scholar] [CrossRef]
- Eslam Panah, B.; Yazdizadeh, T.; Bordbar, G.H. Contraction of cold neutron star due to in the presence a quark core. Eur. Phys. J. C 2019, 79, 815. [Google Scholar] [CrossRef]
- Jaikumar, P.; Reddy, S.; Steiner, A.W. The Strange star surface: A Crust with nuggets. Phys. Rev. Lett. 2006, 96, 041101. [Google Scholar] [CrossRef] [PubMed]
- Ofek, E.O.; Cameron, P.; Kasliwal, M.; Gal-Yam, A.; Rau, A.; Kulkarni, S.; Frail, D.; Chandra, P.; Cenko, S.; Soderberg, A.; et al. SN 2006gy: An extremely luminous supernova in the early-type galaxy NGC 1260. Astrophys. J. Lett. 2007, 659, L13–L16. [Google Scholar] [CrossRef]
- Ouyed, R.; Leahy, D.; Jaikumar, P. Predictions for signatures of the quark-nova in superluminous supernovae. arXiv 2009, arXiv:0911.5424. [Google Scholar]
- Mukhopadhyay, P.; Schaffner-Bielich, J. Quark stars admixed with dark matter. Phys. Rev. D 2016, 93, 083009. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Lopes, I. Radial oscillations of strange quark stars admixed with fermionic dark matter. Phys. Rev. D 2018, 98, 083001. [Google Scholar] [CrossRef]
- Ruderman, M. Pulsars: Structure and dynamics. Ann. Rev. Astron. Astrophys. 1972, 10, 427–476. [Google Scholar] [CrossRef]
- Bowers, R.L.; Liang, E.P.T. Anisotropic Spheres in General Relativity. Astrophys. J. 1974, 188, 657–665. [Google Scholar] [CrossRef]
- Weber, F. Pulsars as Astrophysical Laboratories for Nuclear and Particle Physics; Routledge: New York, NY, USA, 2017. [Google Scholar]
- Bordbar, G.H.; Karami, M. Anisotropic magnetized neutron star. Eur. Phys. J. C 2022, 82, 74. [Google Scholar] [CrossRef]
- Sokolov, A.I. Universal effective coupling constants for the generalized Heisenberg model. Fiz. Tverd. Tela 1998, 40, 1284. [Google Scholar] [CrossRef]
- Sawyer, R.F. Condensed pi- phase in neutron star matter. Phys. Rev. Lett. 1972, 29, 382–385. [Google Scholar] [CrossRef]
- Herrera, L.; Santos, N.O. Local anisotropy in self-gravitating systems. Phys. Rep. 1997, 286, 53–130. [Google Scholar] [CrossRef]
- Barreto, W.; Rojas, S. An Equation of State for Radiating Dissipative Spheres in General Relativity. Astrophys. Space Sci. 1992, 193, 201–215. [Google Scholar] [CrossRef]
- Letelier, P.S. Anisotropic fluids with two-perfect-fluid components. Phys. Rev. D 1980, 22, 807–813. [Google Scholar] [CrossRef]
- Kippenhahn, R.; Weigert, A.; Weiss, A. Stellar Structure and Evolution; Springer: Berlin/Heidelberg, Germany, 2012; ISBN 9783642303043. [Google Scholar] [CrossRef]
- Mak, M.K.; Harko, T. An exact anisotropic quark star model. Chin. J. Astron. Astrophys. 2002, 2, 248–259. [Google Scholar] [CrossRef]
- Deb, D.; Chowdhury, S.R.; Ray, S.; Rahaman, F.; Guha, B.K. Relativistic model for anisotropic strange stars. Ann. Phys. 2017, 387, 239–252. [Google Scholar] [CrossRef]
- Deb, D.; Roy Chowdhury, S.; Ray, S.; Rahaman, F. A New Model for Strange Stars. Gen. Rel. Grav. 2018, 50, 112. [Google Scholar] [CrossRef]
- Gabbanelli, L.; Rincón, A.; Rubio, C. Gravitational decoupled anisotropies in compact stars. Eur. Phys. J. C 2018, 78, 370. [Google Scholar] [CrossRef]
- Ovalle, J. Decoupling gravitational sources in general relativity: From perfect to anisotropic fluids. Phys. Rev. D 2017, 95, 104019. [Google Scholar] [CrossRef]
- Ovalle, J.; Casadio, R.; da Rocha, R.; Sotomayor, A. Anisotropic solutions by gravitational decoupling. Eur. Phys. J. C 2018, 78, 122. [Google Scholar] [CrossRef]
- Sharif, M.; Butt, I.I. Complexity Factor for Charged Spherical System. Eur. Phys. J. C 2018, 78, 688. [Google Scholar] [CrossRef]
- Sharif, M.; Butt, I.I. Complexity factor for static cylindrical system. Eur. Phys. J. C 2018, 78, 850. [Google Scholar] [CrossRef]
- Abbas, G.; Nazar, H. Complexity Factor For Anisotropic Source in Non-minimal Coupling Metric f(R) Gravity. Eur. Phys. J. C 2018, 78, 957. [Google Scholar] [CrossRef]
- Herrera, L.; Di Prisco, A.; Ospino, J. Complexity factors for axially symmetric static sources. Phys. Rev. D 2019, 99, 044049. [Google Scholar] [CrossRef]
- Prasad, A.K.; Kumar, J. Anisotropic relativistic fluid spheres with a linear equation of state. New Astron. 2022, 95, 101815. [Google Scholar] [CrossRef]
- Herrera, L. New definition of complexity for self-gravitating fluid distributions: The spherically symmetric, static case. Phys. Rev. D 2018, 97, 044010. [Google Scholar] [CrossRef]
- Sanudo, J.; Pacheco, A.F. Complexity and white-dwarf structure. Phys. Lett. A 2009, 373, 807–810. [Google Scholar] [CrossRef]
- Arias, C.; Contreras, E.; Fuenmayor, E.; Ramos, A. Anisotropic star models in the context of vanishing complexity. Ann. Phys. 2022, 436, 168671. [Google Scholar] [CrossRef]
- Andrade, J.; Contreras, E. Stellar models with like-Tolman IV complexity factor. Eur. Phys. J. C 2021, 81, 889. [Google Scholar] [CrossRef]
- Gomez-Lobo, A.G.P. Dynamical laws of superenergy in General Relativity. Class. Quant. Grav. 2008, 25, 015006. [Google Scholar] [CrossRef]
- Herrera, L.; Ospino, J.; Di Prisco, A.; Fuenmayor, E.; Troconis, O. Structure and evolution of self-gravitating objects and the orthogonal splitting of the Riemann tensor. Phys. Rev. D 2009, 79, 064025. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Lopes, I. Millisecond pulsars modeled as strange quark stars admixed with condensed dark matter. Int. J. Mod. Phys. D 2018, 27, 1850093. [Google Scholar] [CrossRef]
- Moraes, P.H.R.S.; Panotopoulos, G.; Lopes, I. Anisotropic Dark Matter Stars. Phys. Rev. D 2021, 103, 084023. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A. Electrically charged strange quark stars with a non-linear equation-of-state. Eur. Phys. J. C 2019, 79, 524. [Google Scholar] [CrossRef]
- Lopes, I.; Panotopoulos, G.; Rincón, A. Anisotropic strange quark stars with a non-linear equation-of-state. Eur. Phys. J. Plus 2019, 134, 454. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A. Relativistic strange quark stars in Lovelock gravity. Eur. Phys. J. Plus 2019, 134, 472. [Google Scholar] [CrossRef]
- Abellán, G.; Rincon, A.; Fuenmayor, E.; Contreras, E. Beyond classical anisotropy and a new look to relativistic stars: A gravitational decoupling approach. arXiv 2020, arXiv:2001.07961. [Google Scholar]
- Panotopoulos, G.; Rincón, A.; Lopes, I. Interior solutions of relativistic stars in the scale-dependent scenario. Eur. Phys. J. C 2020, 80, 318. [Google Scholar] [CrossRef]
- Bhar, P.; Tello-Ortiz, F.; Rincón, A.; Gomez-Leyton, Y. Study on anisotropic stars in the framework of Rastall gravity. Astrophys. Space Sci. 2020, 365, 145. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A.; Lopes, I. Radial oscillations and tidal Love numbers of dark energy stars. Eur. Phys. J. Plus 2020, 135, 856. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A.; Lopes, I. Interior solutions of relativistic stars with anisotropic matter in scale-dependent gravity. Eur. Phys. J. C 2021, 81, 63. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincón, A.; Lopes, I. Slowly rotating dark energy stars. Phys. Dark Univ. 2021, 34, 100885. [Google Scholar] [CrossRef]
- Becerra-Vergara, E.A.; Mojica, S.; Lora-Clavijo, F.D.; Cruz-Osorio, A. Anisotropic Quark Stars with an Interacting Quark Equation of State. Phys. Rev. D 2019, 100, 103006. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Tangphati, T.; Banerjee, A. Electrically charged compact stars with an interacting quark equation of state. Chin. J. Phys. 2022, 77, 1682–1690. [Google Scholar] [CrossRef]
- Beringer, J.; Arguin, J.F.; Barnett, R.M.; Copic, K.; Dahl, O.; Groom, D.E.; Lin, C.J.; Lys, J.; Murayama, H.; Wohl, C.G.; et al. Review of Particle Physics (RPP). Phys. Rev. D 2012, 86, 010001. [Google Scholar] [CrossRef]
- Fiorella Burgio, G.; Fantina, A.F. Nuclear Equation of state for Compact Stars and Supernovae. Astrophys. Space Sci. Libr. 2018, 457, 255–335. [Google Scholar] [CrossRef]
- Blaschke, D.; Chamel, N. Phases of dense matter in compact stars. Astrophys. Space Sci. Libr. 2018, 457, 337–400. [Google Scholar] [CrossRef]
- Silva, H.O.; Macedo, C.F.B.; Berti, E.; Crispino, L.C.B. Slowly rotating anisotropic neutron stars in general relativity and scalar–tensor theory. Class. Quant. Grav. 2015, 32, 145008. [Google Scholar] [CrossRef]
- Folomeev, V.; Dzhunushaliev, V. Magnetic fields in anisotropic relativistic stars. Phys. Rev. D 2015, 91, 044040. [Google Scholar] [CrossRef]
- Cattoen, C.; Faber, T.; Visser, M. Gravastars must have anisotropic pressures. Class. Quant. Grav. 2005, 22, 4189–4202. [Google Scholar] [CrossRef]
- Horvat, D.; Ilijic, S.; Marunovic, A. Radial pulsations and stability of anisotropic stars with quasi-local equation of state. Class. Quant. Grav. 2011, 28, 025009. [Google Scholar] [CrossRef]
- Arbañil, J.D.V.; Panotopoulos, G. Tidal deformability and radial oscillations of anisotropic polytropic spheres. Phys. Rev. D 2022, 105, 024008. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rincón, Á.; Panotopoulos, G.; Lopes, I. Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism. Universe 2023, 9, 72. https://doi.org/10.3390/universe9020072
Rincón Á, Panotopoulos G, Lopes I. Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism. Universe. 2023; 9(2):72. https://doi.org/10.3390/universe9020072
Chicago/Turabian StyleRincón, Ángel, Grigoris Panotopoulos, and Ilídio Lopes. 2023. "Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism" Universe 9, no. 2: 72. https://doi.org/10.3390/universe9020072
APA StyleRincón, Á., Panotopoulos, G., & Lopes, I. (2023). Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism. Universe, 9(2), 72. https://doi.org/10.3390/universe9020072







