Stark Broadening of N VI Spectral Lines
Abstract
1. Introduction
2. The Semiclassical Perturbation Method
3. Stark Broadening Parameter Calculations
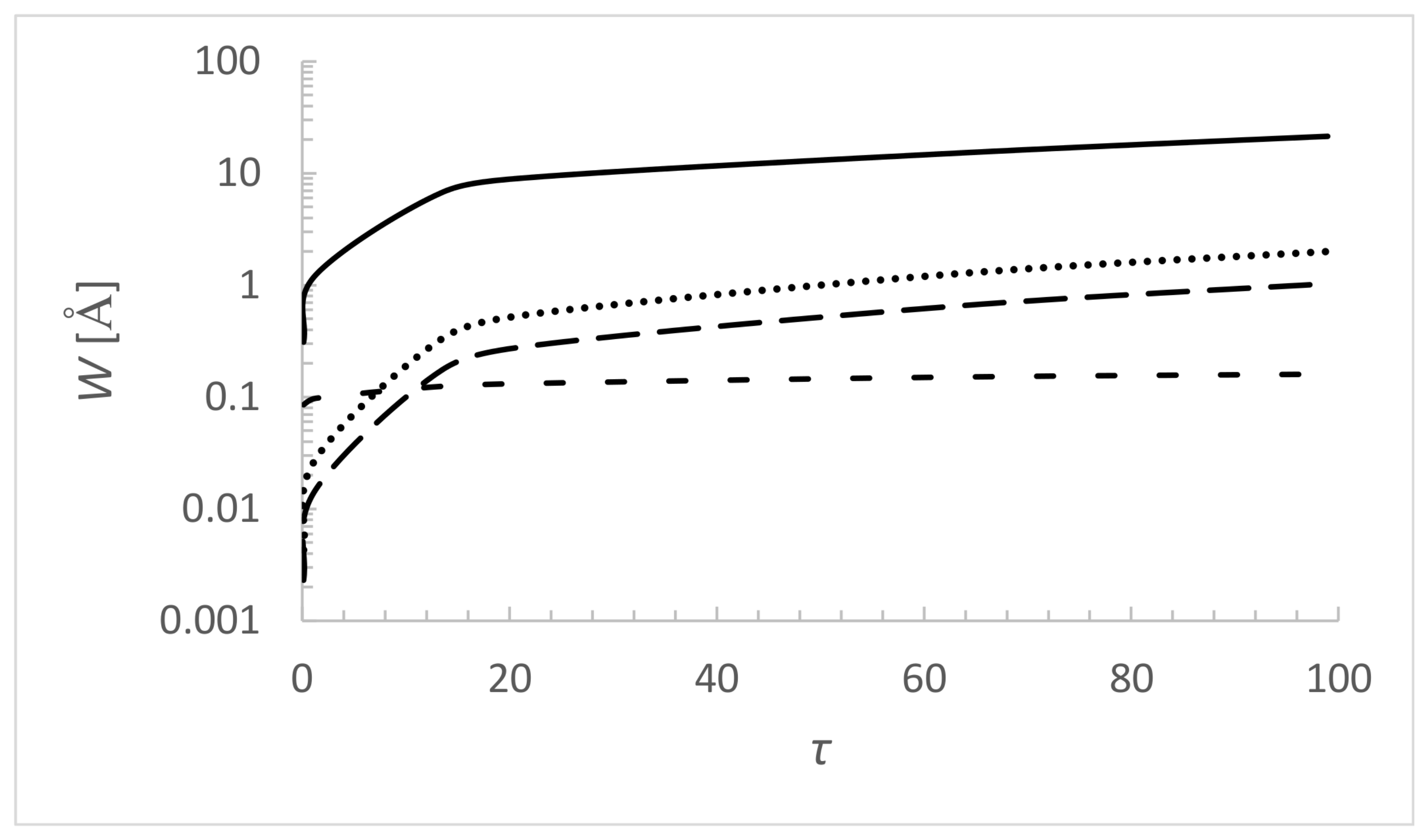
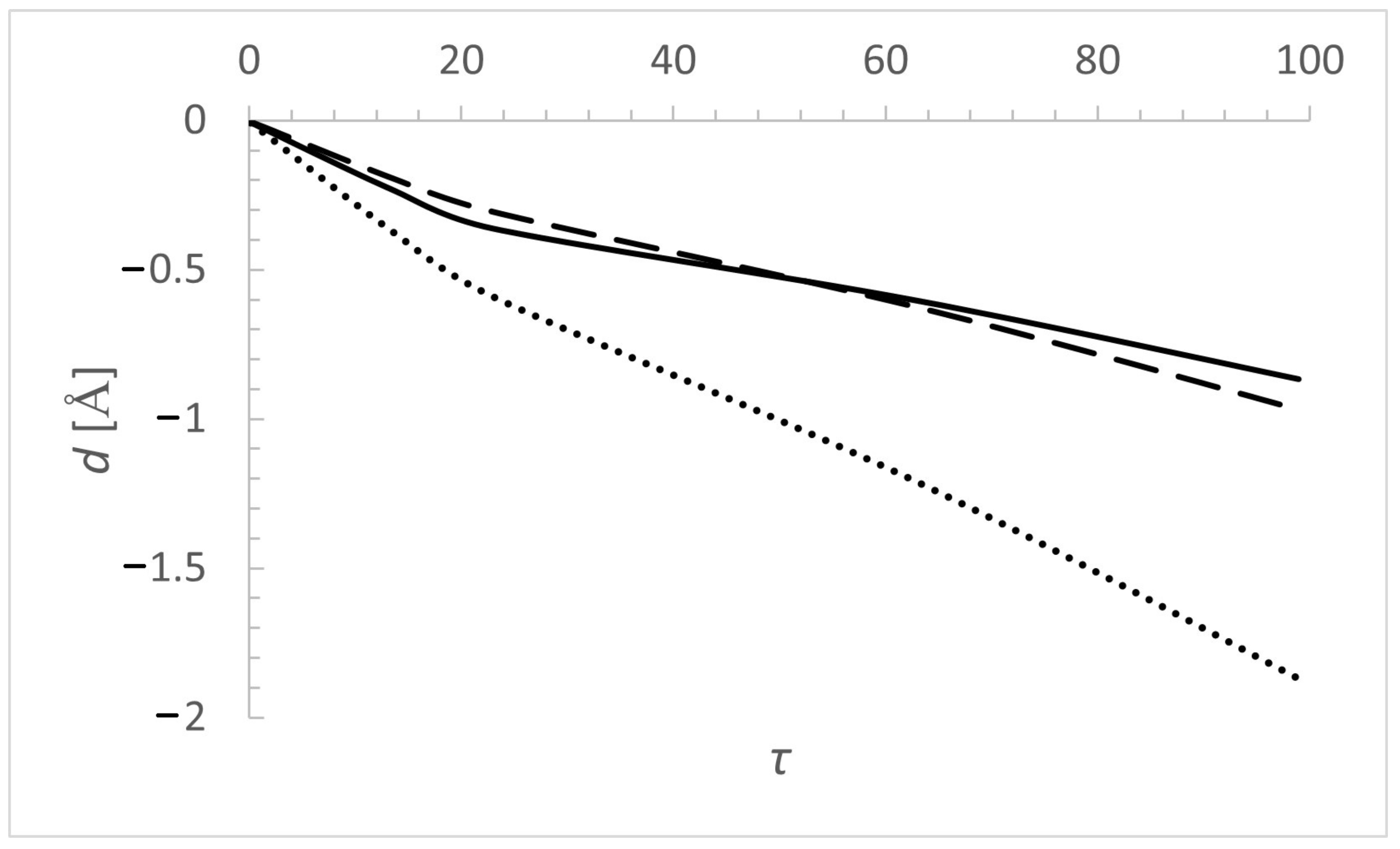
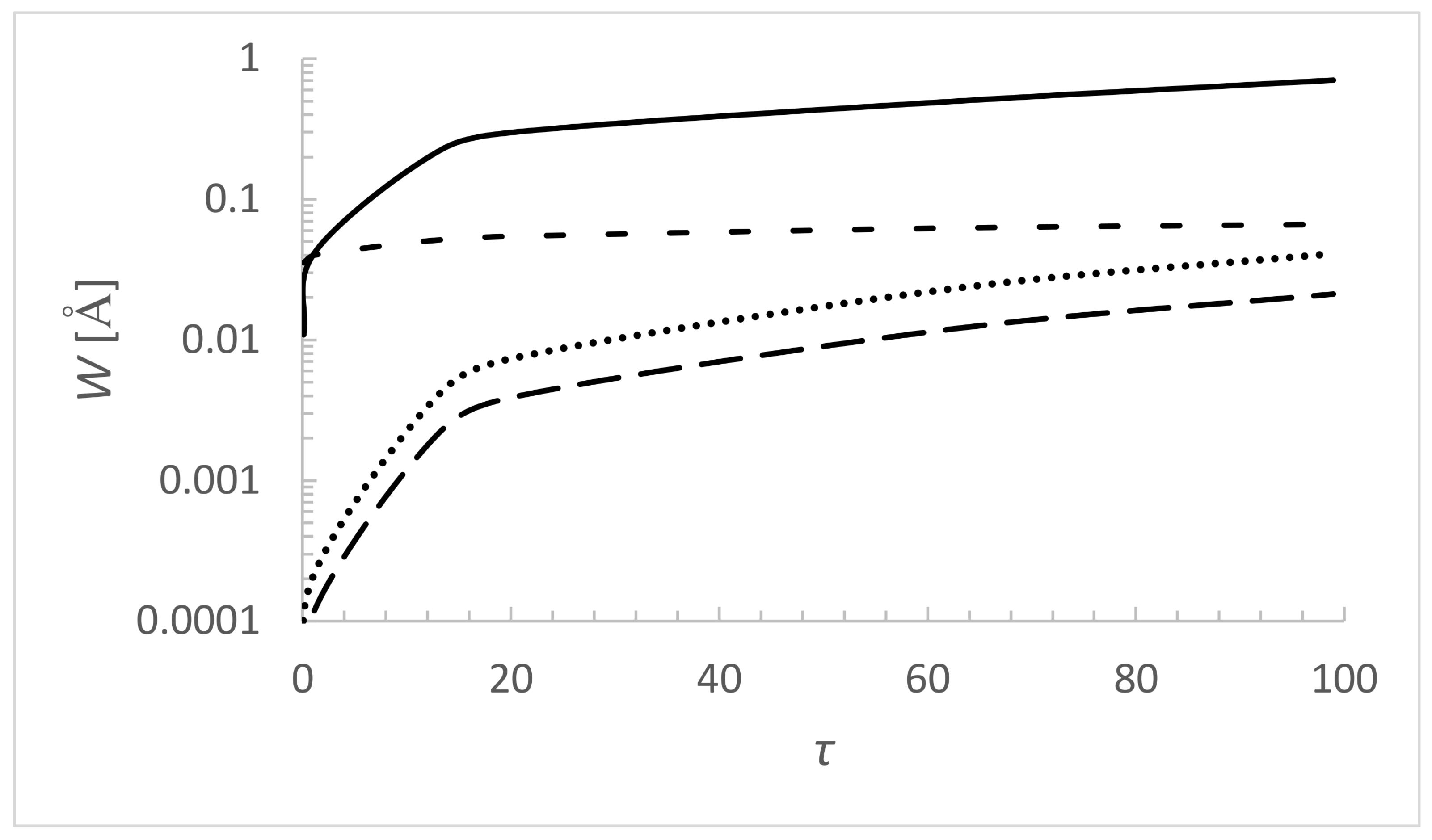
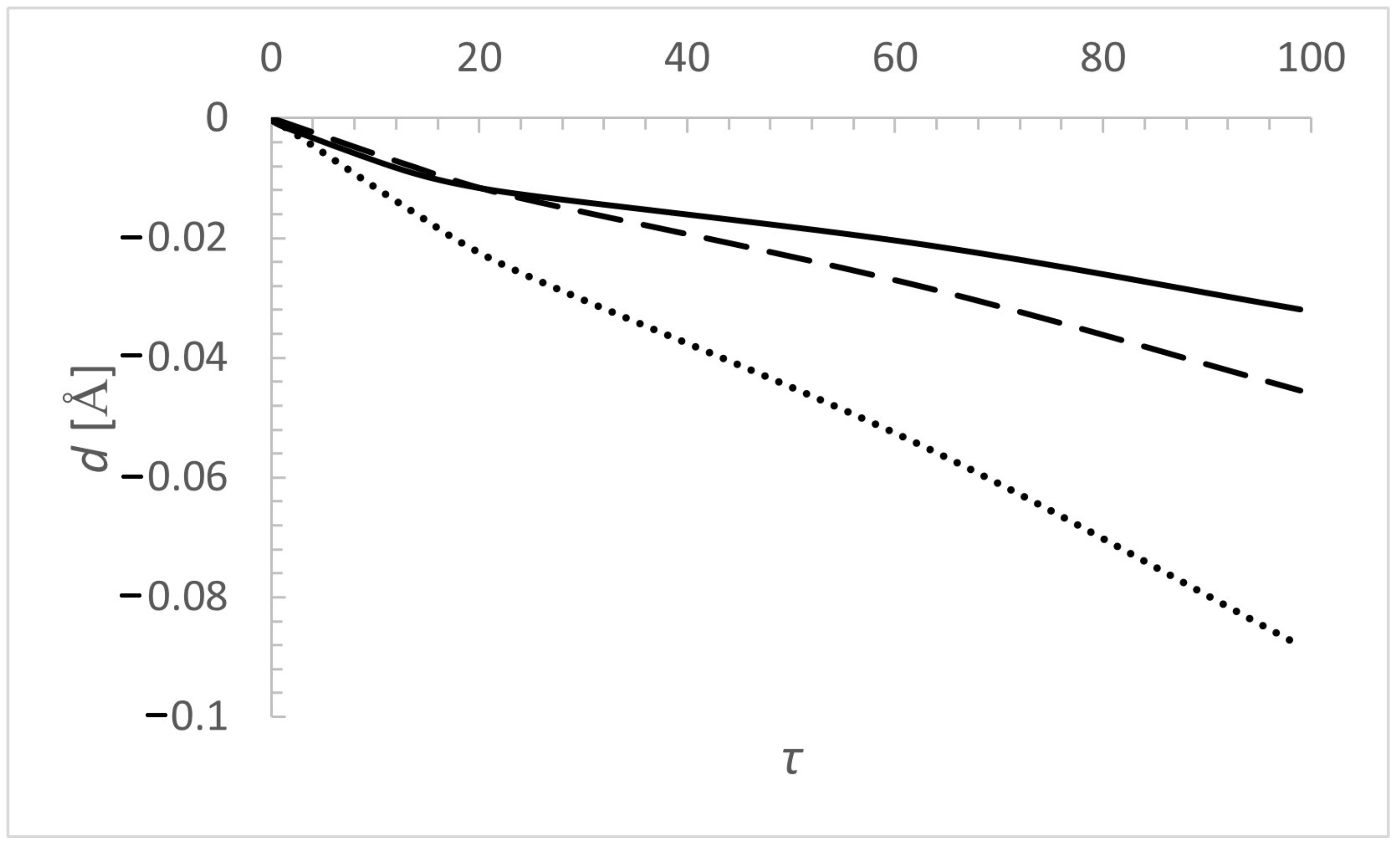
4. Application to DO White Dwarf Atmospheres
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Beauchamp, A.; Wesemael, F.; Bergeron, P. Spectroscopic Studies of DB White Dwarfs: Improved Stark Profiles for Optical Transitions of Neutral Helium. Astrophys. J. Suppl. Ser. 1997, 108, 559–573. [Google Scholar] [CrossRef]
- Konjević, N. Plasma broadening and shifting of non-hydrogenic spectral lines: Present status and applications. Phys. Rep. 1999, 316, 339–401. [Google Scholar] [CrossRef]
- Griem, H.R. Plasma spectroscopy in inertial confinement fusion and soft X-ray laser research. Phys. Fluids 1992, 4, 2346–2361. [Google Scholar] [CrossRef]
- Iglesias, E.; Griem, H.R.; Welch, B.; Weaver, J. UV Line Profiles of B V from a 10-Ps KrF-Laser-Produced Plasma. Astrophys. Space Sci. 1997, 256, 327–331. [Google Scholar] [CrossRef]
- Wang, J.S.; Griem, H.R.; Huang, Y.W.; Böttcher, F. Measurements of line broadening of B V Hα and Lδ in a laser-produced plasma. Phys. Rev. A 1992, 45, 4010–4014. [Google Scholar] [CrossRef] [PubMed]
- Gornushkin, I.B.; King, L.A.; Smith, B.W.; Omenetto, N.; Winefordner, J.D. Line broadening mechanisms in the low pressure laser-induced plasma. Spectrochim. Acta 1999, 54, 1207–1217. [Google Scholar] [CrossRef]
- Sorge, S.; Wierling, A.; Röpke, G.; Theobald, W.; Suerbrey, R.; Wilhein, T. Diagnostics of a laser-induced dense plasma by hydrogen-like carbon spectra. J. Phys. B 2000, 33, 2983–3000. [Google Scholar] [CrossRef]
- Yilbas, B.S.; Patel, F.; Karatas, C. Laser controlled melting of H12 hot-work tool steel with B4C particles at the surface. Opt. Laser Technol. 2015, 74, 36–42. [Google Scholar] [CrossRef]
- Rauch, T.; Ziegler, M.; Werner, K.; Kruk, J.W.; Oliveira, C.M.; Vande Putte, D.; Mignani, R.P.; Kerber, F. High-resolution FUSE and HST ultraviolet spectroscopy of the white dwarf central star of Sh 2-216. Astron. Astrophys. 2007, 470, 317–329. [Google Scholar] [CrossRef]
- Rauch, T.; Suleimanov, V.; Werner, K. Absorption features in the spectra of X-ray bursting neutron stars. Astron. Astrophys. 2008, 490, 1127–1134. [Google Scholar] [CrossRef][Green Version]
- Fleig, J.; Rauch, T.; Werner, K.; Kruk, J.W. FUSE spectroscopy of the sdOB primary of the post common-envelope binary LB3459 (AADoradus). Astron. Astrophys. 2008, 492, 565–573. [Google Scholar] [CrossRef]
- Nagel, T.; Rauch, T.; Werner, K. A new grid of NLTE accretion-disc models for AMCVn systems: Application to CE 315. Astron. Astrophys. 2009, 499, 773–781. [Google Scholar] [CrossRef][Green Version]
- Rauch, T.; Orio, M.; Gonzales-Riestra, R.; Nelson, T.; Still, M.; Werner, K.; Wilms, J. Non-local thermal equilibrium model atmospheres for the hottest white dwarfs: Spectral analysis of the compact component in nova V4743 Sgr. Astrophys. J. 2010, 717, 363–371. [Google Scholar] [CrossRef]
- Newman, J.; Tsuruta, S.; Liebmann, A.C.; Kunieda, H.; Haba, Y. Combined Analysis of X-ray Spectra of NGC 3227. Astrophys. J. 2021, 907, 45. [Google Scholar] [CrossRef]
- Ness, J.-U.; Beardmore, A.P.; Bode, M.F.; Darnley, M.J.; Dobrotka, A.; Drake, J.J.; Magdolen, J.; Munari, U.; Osborne, J.P.; Orio, M.; et al. High-resolution X-ray spectra of RS Ophiuchi (2006 and 2021): Revealing the cause of SSS variability. Astron. Astrophys. 2023, 670, A131. [Google Scholar] [CrossRef]
- Rauch, T. White Dwarf Model Atmospheres: Synthetic Spectra for Supersoft Sources. In Proceedings of the IAU Symposium No. 281, Padova, Italy, 4–8 July 2011; Di Stefano, R., Orio, M., Moe, M., Eds.; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Sokolovsky, K.V.; Li, K.L.; Lopes de Oliveira, R.; Ness, J.U.; Mukai, K.; Chomiuk, L.; Aydi, E.; Steinberg, E.; Vurm, I.; Metzger, B.D.; et al. The first nova eruption in a novalike variable: YZ Ret as seen in X-rays and γ-rays. Mon. Not. R. Astron. Soc. 2022, 514, 2239–2258. [Google Scholar] [CrossRef]
- Jansen, F.; Lumb, D.; Altieri, B.; Clavel, J.; Ehle, M.; Erd, C.; Gabriel, C.; Guainazzi, M.; Gondoin, P.; Much, R.; et al. XMM-Newton observatory. I. The spacecraft and operations. Astron. Astrophys. 2001, 365, L1–L6. [Google Scholar] [CrossRef]
- Batani, K. Perspectives on research on laser driven proton-boron fusion and applications. J. Instrum. 2023, 18, C09012. [Google Scholar] [CrossRef]
- Schollmeier, M.S. Investigation of Proton Beam-Driven Fusion Reactions Generated by an Ultra-Short Petawatt-Scale Laser Pulse. Laser Part. Beams 2022, 2404263. [Google Scholar] [CrossRef]
- Hegelich, B.M.; Labun, L.O.; Labun, Z.; Mehlhorn, T.A. Photon and Neutron Production as In Situ Diagnostics of Proton-Boron Fusion. Laser Part. Beams 2023, 6924841. [Google Scholar] [CrossRef]
- Kong, D.; Xu, S.; Shou, Y.; Gao, Y.; Mei, Z.; Pan, Z.; Liu, Z.; Cao, Z.; Liang, Y.; Peng, Z.; et al. Alpha-Particle Generation from H-11B Fusion Initiated by Laser-Accelerated Boron Ions. Laser Part. Beams 2022, 2022, 5733475. [Google Scholar] [CrossRef]
- Sahal-Bréchot, S.; Dimitrijević, M.S.; Ben Nessib, N. Widths and Shifts of Isolated Lines of Neutral and Ionized Atoms Perturbed by Collisions with Electrons and Ions: An Outline of the Semiclassical Perturbation (SCP) Method and of the Approximations Used for the Calculations. Atoms 2014, 2, 225–252. [Google Scholar] [CrossRef]
- Sahal–Bréchot, S. Impact Theory of the Broadening and Shift of Spectral Lines due to Electrons and Ions in a Plasma. Astron. Astrophys. 1969, 1, 91–123. [Google Scholar]
- Sahal–Bréchot, S. Impact Theory of the Broadening and Shift of Spectral Lines due to Electrons and Ions in a Plasma (Continued). Astron. Astrophys. 1969, 2, 322–354. [Google Scholar]
- Sahal–Bréchot, S. The Semiclassical Limit of the Gailitis Formula Applied to Electron Impact Broadening of Spectral Lines of Ionized Atoms. Atoms 2021, 9, 29. [Google Scholar] [CrossRef]
- Kelly, R.L. Atomic and Ionic Spectrum Lines Below 2000 Angstroms: Hydrogen through Krypton. J. Phys. Chem. Ref. Data 1987, 16 (Suppl. S1), 1–1698, Erratum: J. Phys. Chem. Ref. Data 1998, 17, 953. [Google Scholar]
- Kramida, A.; Ralchenko, Y.; Reader, J.; NIST ASD Team. NIST Atomic Spectra Database (Ver. 5.10); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2022. Available online: https://physics.nist.gov/asd (accessed on 30 October 2023).
- Bates, D.R.; Damgaard, A. The Calculation of the Absolute Strengths of Spectral Lines. Philos. Trans. R. Soc. Lond. Ser. A 1949, 242, 101–122. [Google Scholar]
- Oertel, G.K.; Shomo, L.P. Tables for the Calculation of Radial Multipole Matrix Elements by the Coulomb Approximation. Astrophys. J. Suppl. Ser. 1968, 16, 175–218. [Google Scholar] [CrossRef]
- Van Regemorter, H.; Dy Hoang, B.; Prud’homme, M. Radial transition integrals involving low or high effective quantum numbers in the Coulomb approximation. J. Phys. B 1979, 12, 1053–1061. [Google Scholar] [CrossRef]
- Dimitrijević, M.S.; Sahal-Bréchot, S. Stark broadening of neutral helium lines. J. Quant. Spectrosc. Radiat. Transf. 1984, 31, 301–313. [Google Scholar] [CrossRef]
- Dimitrijević, M.S. Electron-impact widths of four- and five-times charged ion lines of astrophysical importance. Astron. Astrophys. Suppl. Ser. 1993, 100, 237–241. [Google Scholar]
- Dimitrijević, M.S.; Konjević, N. Stark widths of doubly- and triply-ionized atom lines. J. Quant. Spectrosc. Radiat. Transf. 1980, 24, 451–459. [Google Scholar] [CrossRef]
- Dimitrijević, M.S. Stark widths of fourtly and fifthly charged ion lines. Astrophys. Lett. Commun. 1993, 28, 385–388. [Google Scholar]
- Griem, H.R. Spectral line Broadening by Plasmas; McGraw-Hill: New York, NY, USA, 1974. [Google Scholar]
- Wesemael, F. Atmospheres for hot, high-gravity stars. II. Pure helium models. Astrophys. J. Suppl. Ser. 1981, 45, 177–257. [Google Scholar] [CrossRef]
- Sahal-Bréchot, S.; Dimitrijević, M.S.; Moreau, N.; Ben Nessib, N. The STARK-B database VAMDC node: A repository for spectral line broadening and shifts due to collisions with charged particles. Phys. Scr. 2015, 90, 054008. [Google Scholar] [CrossRef]
- Sahal-Bréchot, S.; Dimitrijević, M.S.; Moreau, N. STARK-B Database. Available online: http://stark-B.obspm.fr (accessed on 30 October 2023).
- Dubernet, M.L.; Boudon, V.; Culhane, J.L.; Dimitrijevic, M.S.; Fazliev, A.Z.; Joblin, C.; Kupka, F.; Leto, G.; Le Sidaner, P.; Loboda, P.A.; et al. Virtual atomic and molecular data centre. J. Quant. Spectrosc. Radiat. Transf. 2010, 111, 2151–2159. [Google Scholar] [CrossRef]
- Dubernet, M.L.; Antony, B.K.; Ba, Y.A.; Babikov, Y.L.; Bartschat, K.; Boudon, V.; Braams, B.J.; Chung, H.K.; Daniel, F.; Delahaye, F.; et al. The virtual atomic and molecular data centre (VAMDC) consortium. J. Phys. B 2016, 49, 074003. [Google Scholar] [CrossRef]
- Albert, D.; Antony, B.K.; Ba, Y.A.; Babikov, Y.L.; Bollard, P.; Boudon, V.; Delahaye, F.; Del Zanna, G.; Dimitrijević, M.S.; Drouin, B.J.; et al. A Decade with VAMDC: Results and Ambitions. Atoms 2020, 8, 76. [Google Scholar] [CrossRef]
| Electrons | Protons | ||||
|---|---|---|---|---|---|
| Transition | T [10 K] | Width [Å] | Shift [Å] | Width [Å] | Shift [Å] |
| Singlets | |||||
| N VI 1s-2p | 0.5 | 0.130 × 10 | −0.132 × 10 | 0.188 × 10 | −0.501 × 10 |
| 28.8 Å | 1.0 | 0.925 × 10 | −0.176 × 10 | 0.549 × 10 | −0.100 × 10 |
| C = 0.29 × 10 | 3.0 | 0.543 × 10 | −0.649 × 10 | 0.243 × 10 | −0.265 × 10 |
| 5.0 | 0.431 × 10 | −0.477 × 10 | 0.401 × 10 | −0.371 × 10 | |
| 10.0 | 0.322 × 10 | −0.339 × 10 | 0.650 × 10 | −0.514 × 10 | |
| 20.0 | 0.247 × 10 | −0.116 × 10 | 0.914 × 10 | −0.631 × 10 | |
| N VI 1s-3p | 0.5 | 0.498 × 10 | −0.324 × 10 | 0.179 × 10 | −0.414 × 10 |
| 24.9 Å | 1.0 | 0.363 × 10 | −0.365 × 10 | 0.414 × 10 | −0.605 × 10 |
| C = 0.16 × 10 | 3.0 | 0.240 × 10 | −0.209 × 10 | 0.966 × 10 | −0.895 × 10 |
| 5.0 | 0.200 × 10 | −0.181 × 10 | 0.122 × 10 | −0.101 × 10 | |
| 10.0 | 0.158 × 10 | −0.141 × 10 | 0.170 × 10 | −0.120 × 10 | |
| 20.0 | 0.127 × 10 | −0.105 × 10 | 0.223 × 10 | −0.139 × 10 | |
| N VI 2s-2p | 0.5 | 0.183 × 10 | −0.690 × 10 | 0.266 × 10 | −0.226 × 10 |
| 2897.2 Å | 1.0 | 0.136 × 10 | −0.761 × 10 | 0.113 × 10 | −0.439 × 10 |
| C = 0.29 × 10 | 3.0 | 0.838 × 10 | −0.503 × 10 | 0.636 × 10 | −0.961 × 10 |
| 5.0 | 0.681 × 10 | −0.483 × 10 | 0.954 × 10 | −0.122 × 10 | |
| 10.0 | 0.525 × 10 | −0.453 × 10 | 0.156 × 10 | −0.153 × 10 | |
| 20.0 | 0.414 × 10 | −0.382 × 10 | 0.215 × 10 | −0.183 × 10 | |
| N VI 2s-3p | 0.5 | 0.254 × 10 | −0.134 × 10 | 0.887 × 10 | −0.205 × 10 |
| 173.3 Å | 1.0 | 0.191 × 10 | −0.141 × 10 | 0.204 × 10 | −0.300 × 10 |
| C = 0.79 × 10 | 3.0 | 0.126 × 10 | −0.117 × 10 | 0.477 × 10 | −0.441 × 10 |
| 5.0 | 0.106 × 10 | −0.103 × 10 | 0.600 × 10 | −0.499 × 10 | |
| 10.0 | 0.839 × 10 | −0.831 × 10 | 0.840 × 10 | −0.591 × 10 | |
| 20.0 | 0.673 × 10 | −0.640 × 10 | 0.110 × 10 | −0.678 × 10 | |
| N VI 3s-3p | 0.5 | 0.112 | −0.717 × 10 | 0.418 × 10 | −0.895 × 10 |
| 9624.6 Å | 1.0 | 0.851 × 10 | −0.799 × 10 | 0.894 × 10 | −0.127 × 10 |
| C = 0.24 × 10 | 3.0 | 0.573 × 10 | −0.713 × 10 | 0.189 × 10 | −0.179 × 10 |
| 5.0 | 0.484 × 10 | −0.660 × 10 | 0.237 × 10 | −0.202 × 10 | |
| 10.0 | 0.388 × 10 | −0.544 × 10 | 0.333 × 10 | −0.240 × 10 | |
| 20.0 | 0.311 × 10 | −0.424 × 10 | 0.419 × 10 | −0.259 × 10 | |
| N VI 2p-3s | 0.5 | 0.206 × 10 | 0.144 × 10 | 0.386 × 10 | 0.133 × 10 |
| 187.9 Å | 1.0 | 0.157 × 10 | 0.171 × 10 | 0.118 × 10 | 0.209 × 10 |
| C = 0.37 × 10 | 3.0 | 0.105 × 10 | 0.155 × 10 | 0.291 × 10 | 0.320 × 10 |
| 5.0 | 0.890 × 10 | 0.151 × 10 | 0.365 × 10 | 0.367 × 10 | |
| 10.0 | 0.712 × 10 | 0.129 × 10 | 0.495 × 10 | 0.440 × 10 | |
| 20.0 | 0.569 × 10 | 0.102 × 10 | 0.652 × 10 | 0.499 × 10 | |
| Triplets | |||||
| N VI 2s-2p | 0.5 | 0.714 × 10 | −0.969 × 10 | 0.807 × 10 | −0.582 × 10 |
| 1901.5 Å | 1.0 | 0.529 × 10 | −0.982 × 10 | 0.298 × 10 | −0.115 × 10 |
| C = 0.19 × 10 | 3.0 | 0.321 × 10 | −0.176 × 10 | 0.171 × 10 | −0.273 × 10 |
| 5.0 | 0.259 × 10 | −0.165 × 10 | 0.268 × 10 | −0.350 × 10 | |
| 10.0 | 0.198 × 10 | −0.155 × 10 | 0.461 × 10 | −0.455 × 10 | |
| 20.0 | 0.155 × 10 | −0.140 × 10 | 0.634 × 10 | −0.544 × 10 | |
| N VI 2s-3p | 0.5 | 0.198 × 10 | 0.172 × 10 | 0.209 × 10 | 0.357 × 10 |
| 161.2 Å | 1.0 | 0.147 × 10 | 0.118 × 10 | 0.502 × 10 | 0.618 × 10 |
| C = 0.19 × 10 | 3.0 | 0.959 × 10 | 0.152 × 10 | 0.124 × 10 | 0.109 × 10 |
| 5.0 | 0.800 × 10 | 0.157 × 10 | 0.160 × 10 | 0.126 × 10 | |
| 10.0 | 0.634 × 10 | 0.114 × 10 | 0.218 × 10 | 0.151 × 10 | |
| 20.0 | 0.511 × 10 | 0.892 × 10 | 0.303 × 10 | 0.179 × 10 | |
| N VI 2s-4p | 0.5 | 0.337 × 10 | 0.102 × 10 | 0.139 × 10 | 0.183 × 10 |
| 122.4 Å | 1.0 | 0.258 × 10 | 0.882 × 10 | 0.236 × 10 | 0.254 × 10 |
| C = 0.48 × 10 | 3.0 | 0.177 × 10 | 0.805 × 10 | 0.415 × 10 | 0.352 × 10 |
| 5.0 | 0.150 × 10 | 0.685 × 10 | 0.505 × 10 | 0.397 × 10 | |
| 10.0 | 0.121 × 10 | 0.542 × 10 | 0.661 × 10 | 0.464 × 10 | |
| 20.0 | 0.979 × 10 | 0.443 × 10 | 0.822 × 10 | 0.531 × 10 | |
| N VI 3s-3p | 0.5 | 0.531 × 10 | −0.103 × 10 | 0.383 × 10 | −0.617 × 10 |
| 6993.0 Å | 1.0 | 0.399 × 10 | −0.133 × 10 | 0.911 × 10 | −0.107 × 10 |
| C = 0.36 × 10 | 3.0 | 0.265 × 10 | −0.133 × 10 | 0.227 × 10 | −0.192 × 10 |
| 5.0 | 0.223 × 10 | −0.127 × 10 | 0.300 × 10 | −0.223 × 10 | |
| 10.0 | 0.179 × 10 | −0.118 × 10 | 0.429 × 10 | −0.268 × 10 | |
| 20.0 | 0.144 × 10 | −0.937 × 10 | 0.614 × 10 | −0.315 × 10 | |
| N VI 3s-4p | 0.5 | 0.578 × 10 | 0.900 × 10 | 0.187 × 10 | 0.235 × 10 |
| 474.5 Å | 1.0 | 0.443 × 10 | 0.611 × 10 | 0.320 × 10 | 0.330 × 10 |
| C = 0.72 × 10 | 3.0 | 0.304 × 10 | 0.463 × 10 | 0.568 × 10 | 0.465 × 10 |
| 5.0 | 0.258 × 10 | 0.306 × 10 | 0.699 × 10 | 0.532 × 10 | |
| 10.0 | 0.208 × 10 | 0.172 × 10 | 0.956 × 10 | 0.604 × 10 | |
| 20.0 | 0.169 × 10 | 0.157 × 10 | 0.120 × 10 | 0.692 × 10 | |
| N VI 4s-4p | 0.5 | 1.05 | −0.282 × 10 | 0.190 × 10 | −0.184 × 10 |
| 17,182.1 Å | 1.0 | 0.812 | −0.311 × 10 | 0.325 × 10 | −0.270 × 10 |
| C = 0.94 × 10 | 3.0 | 0.564 | −0.306 × 10 | 0.592 × 10 | −0.403 × 10 |
| 5.0 | 0.481 | −0.292 × 10 | 0.756 × 10 | −0.459 × 10 | |
| 10.0 | 0.388 | −0.237 × 10 | 0.106 | −0.539 × 10 | |
| 20.0 | 0.313 | −0.188 × 10 | 0.150 | −0.617 × 10 | |
| N VI 2p-3s | 0.5 | 0.172 × 10 | 0.999 × 10 | 0.203 × 10 | 0.877 × 10 |
| 180.7 Å | 1.0 | 0.129 × 10 | 0.113 × 10 | 0.704 × 10 | 0.143 × 10 |
| C = 0.47 × 10 | 3.0 | 0.857 × 10 | 0.124 × 10 | 0.199 × 10 | 0.230 × 10 |
| 5.0 | 0.721 × 10 | 0.120 × 10 | 0.254 × 10 | 0.262 × 10 | |
| 10.0 | 0.576 × 10 | 0.107 × 10 | 0.333 × 10 | 0.310 × 10 | |
| 20.0 | 0.462 × 10 | 0.865 × 10 | 0.434 × 10 | 0.360 × 10 | |
| N VI 2p-4s | 0.5 | 0.267 × 10 | 0.291 × 10 | 0.162 × 10 | 0.289 × 10 |
| 131.9 Å | 1.0 | 0.207 × 10 | 0.292 × 10 | 0.295 × 10 | 0.397 × 10 |
| C = 0.10 × 10 | 3.0 | 0.144 × 10 | 0.283 × 10 | 0.542 × 10 | 0.538 × 10 |
| 5.0 | 0.123 × 10 | 0.260 × 10 | 0.658 × 10 | 0.610 × 10 | |
| 10.0 | 0.986 × 10 | 0.210 × 10 | 0.842 × 10 | 0.696 × 10 | |
| 20.0 | 0.786 × 10 | 0.169 × 10 | 0.110 × 10 | 0.780 × 10 | |
| N VI 3p-4s | 0.5 | 0.574 × 10 | 0.432 × 10 | 0.243 × 10 | 0.433 × 10 |
| 524.5 Å | 1.0 | 0.441 × 10 | 0.440 × 10 | 0.448 × 10 | 0.597 × 10 |
| C = 0.16 × 10 | 3.0 | 0.304 × 10 | 0.417 × 10 | 0.803 × 10 | 0.812 × 10 |
| 5.0 | 0.258 × 10 | 0.381 × 10 | 0.101 × 10 | 0.924 × 10 | |
| 10.0 | 0.208 × 10 | 0.308 × 10 | 0.131 × 10 | 0.105 × 10 | |
| 20.0 | 0.167 × 10 | 0.247 × 10 | 0.170 × 10 | 0.122 × 10 |
| He III | B III | ||||
|---|---|---|---|---|---|
| Transition | T [10 K] | Width [Å] | Shift [Å] | Width [Å] | Shift [Å] |
| Singlets | |||||
| N VI 1s-2p | 0.5 | 0.344 × 10 | −0.994 × 10 | 0.481 × 10 | −0.994 × 10 |
| 28.8 Å | 1.0 | 0.101 × 10 | −0.200 × 10 | 0.138 × 10 | −0.200 × 10 |
| C = 0.29 × 10 | 3.0 | 0.463 × 10 | −0.536 × 10 | 0.544 × 10 | −0.514 × 10 |
| 5.0 | 0.769 × 10 | −0.754 × 10 | 0.822 × 10 | −0.704 × 10 | |
| 10.0 | 0.121 × 10 | −0.105 × 10 | 0.125 × 10 | −0.968 × 10 | |
| 20.0 | 0.161 × 10 | −0.130 × 10 | 0.154 × 10 | −0.116 × 10 | |
| N VI 1s-3p | 0.5 | 0.349 × 10 | −0.829 × 10 | 0.383 × 10 | −0.798 × 10 |
| 24.9 Å | 1.0 | 0.813 × 10 | −0.123 × 10 | 0.812 × 10 | −0.116 × 10 |
| C = 0.16 × 10 | 3.0 | 0.177 × 10 | −0.183 × 10 | 0.164 × 10 | −0.167 × 10 |
| 5.0 | 0.215 × 10 | −0.209 × 10 | 0.195 × 10 | −0.189 × 10 | |
| 10.0 | 0.274 × 10 | −0.246 × 10 | 0.235 × 10 | −0.220 × 10 | |
| 20.0 | 0.332 × 10 | −0.282 × 10 | 0.312 × 10 | −0.256 × 10 | |
| N VI 2s-2p | 0.5 | 0.488 × 10 | −0.449 × 10 | 0.674 × 10 | −0.448 × 10 |
| 2897.2 Å | 1.0 | 0.212 × 10 | −0.880 × 10 | 0.268 × 10 | −0.866 × 10 |
| C = 0.29 × 10 | 3.0 | 0.125 × 10 | −0.196 × 10 | 0.128 × 10 | −0.181 × 10 |
| 5.0 | 0.188 × 10 | −0.249 × 10 | 0.190 × 10 | −0.232 × 10 | |
| 10.0 | 0.297 × 10 | −0.312 × 10 | 0.284 × 10 | −0.285 × 10 | |
| 20.0 | 0.388 × 10 | −0.372 × 10 | 0.362 × 10 | −0.339 × 10 | |
| N VI 2s-3p | 0.5 | 0.173 × 10 | −0.411 × 10 | 0.190 × 10 | −0.396 × 10 |
| 173.3 Å | 1.0 | 0.402 × 10 | −0.609 × 10 | 0.403 × 10 | −0.574 × 10 |
| C = 0.79 × 10 | 3.0 | 0.872 × 10 | −0.907 × 10 | 0.808 × 10 | −0.820 × 10 |
| 5.0 | 0.106 × 10 | −0.103 × 10 | 0.961 × 10 | −0.934 × 10 | |
| 10.0 | 0.135 × 10 | −0.121 × 10 | 0.115 × 10 | −0.110 × 10 | |
| 20.0 | 0.161 × 10 | −0.139 × 10 | 0.156 × 10 | −0.125 × 10 | |
| N VI 3s-3p | 0.5 | 0.805 × 10 | −0.179 × 10 | 0.905 × 10 | −0.170 × 10 |
| 9624.6 Å | 1.0 | 0.175 × 10 | −0.259 × 10 | 0.173 × 10 | −0.239 × 10 |
| C = 0.24 × 10 | 3.0 | 0.357 × 10 | −0.367 × 10 | 0.327 × 10 | −0.333 × 10 |
| 5.0 | 0.432 × 10 | −0.419 × 10 | 0.393 × 10 | −0.375 × 10 | |
| 10.0 | 0.540 × 10 | −0.500 × 10 | 0.473 × 10 | −0.436 × 10 | |
| 20.0 | 0.698 × 10 | −0.538 × 10 | 0.546 × 10 | −0.502 × 10 | |
| N VI 2p-3s | 0.5 | 0.738 × 10 | 0.267 × 10 | 0.843 × 10 | 0.257 × 10 |
| 187.9 Å | 1.0 | 0.232 × 10 | 0.426 × 10 | 0.236 × 10 | 0.393 × 10 |
| C = 0.37 × 10 | 3.0 | 0.584 × 10 | 0.658 × 10 | 0.537 × 10 | 0.599 × 10 |
| 5.0 | 0.727 × 10 | 0.759 × 10 | 0.660 × 10 | 0.684 × 10 | |
| 10.0 | 0.921 × 10 | 0.910 × 10 | 0.822 × 10 | 0.822 × 10 | |
| 20.0 | 0.115 × 10 | 0.103 × 10 | 0.105 × 10 | 0.942 × 10 | |
| Triplets | |||||
| N VI 2s-2p | 0.5 | 0.148 × 10 | −0.116 × 10 | 0.205 × 10 | −0.115 × 10 |
| 1901.5 Å | 1.0 | 0.552 × 10 | −0.230 × 10 | 0.729 × 10 | −0.228 × 10 |
| C = 0.19 × 10 | 3.0 | 0.331 × 10 | −0.554 × 10 | 0.365 × 10 | −0.522 × 10 |
| 5.0 | 0.527 × 10 | −0.711 × 10 | 0.549 × 10 | −0.668 × 10 | |
| 10.0 | 0.902 × 10 | −0.937 × 10 | 0.852 × 10 | −0.844 × 10 | |
| 20.0 | 0.117 × 10 | −0.113 × 10 | 0.111 × 10 | −0.102 × 10 | |
| N VI 2s-3p | 0.5 | 0.390 × 10 | 0.711 × 10 | 0.501 × 10 | 0.701 × 10 |
| 161.2 Å | 1.0 | 0.960 × 10 | 0.125 × 10 | 0.113 × 10 | 0.120 × 10 |
| C = 0.19 × 10 | 3.0 | 0.236 × 10 | 0.224 × 10 | 0.241 × 10 | 0.206 × 10 |
| 5.0 | 0.295 × 10 | 0.259 × 10 | 0.289 × 10 | 0.237 × 10 | |
| 10.0 | 0.375 × 10 | 0.311 × 10 | 0.350 × 10 | 0.281 × 10 | |
| 20.0 | 0.462 × 10 | 0.367 × 10 | 0.405 × 10 | 0.329 × 10 | |
| N VI 2s-4p | 0.5 | 0.270 × 10 | 0.368 × 10 | 0.295 × 10 | 0.345 × 10 |
| 122.4 Å | 1.0 | 0.464 × 10 | 0.518 × 10 | 0.480 × 10 | 0.482 × 10 |
| C = 0.48 × 10 | 3.0 | 0.789 × 10 | 0.719 × 10 | 0.743 × 10 | 0.652 × 10 |
| 5.0 | 0.938 × 10 | 0.822 × 10 | 0.854 × 10 | 0.740 × 10 | |
| 10.0 | 0.110 × 10 | 0.943 × 10 | 0.105 × 10 | 0.853 × 10 | |
| 20.0 | 0.127 × 10 | 0.107 × 10 | 0.124 × 10 | 0.991 × 10 | |
| N VI 3s-3p | 0.5 | 0.714 × 10 | −0.123 × 10 | 0.920 × 10 | −0.121 × 10 |
| 6993.0 Å | 1.0 | 0.174 × 10 | −0.217 × 10 | 0.205 × 10 | −0.209 × 10 |
| C = 0.36 × 10 | 3.0 | 0.425 × 10 | −0.393 × 10 | 0.437 × 10 | −0.363 × 10 |
| 5.0 | 0.531 × 10 | −0.456 × 10 | 0.520 × 10 | −0.416 × 10 | |
| 10.0 | 0.677 × 10 | −0.550 × 10 | 0.643 × 10 | −0.501 × 10 | |
| 20.0 | 0.832 × 10 | −0.647 × 10 | 0.762 × 10 | −0.581 × 10 | |
| N VI 3s-4p | 0.5 | 0.361 × 10 | 0.472 × 10 | 0.402 × 10 | 0.445 × 10 |
| 474.5 Å | 1.0 | 0.629 × 10 | 0.674 × 10 | 0.652 × 10 | 0.626 × 10 |
| C = 0.72 × 10 | 3.0 | 0.107 × 10 | 0.954 × 10 | 0.102 × 10 | 0.866 × 10 |
| 5.0 | 0.123 × 10 | 0.107 × 10 | 0.116 × 10 | 0.982 × 10 | |
| 10.0 | 0.156 × 10 | 0.128 × 10 | 0.136 × 10 | 0.113 × 10 | |
| 20.0 | 0.174 × 10 | 0.138 × 10 | 0.155 × 10 | 0.133 × 10 | |
| N VI 4s-4p | 0.5 | 0.363 × 10 | −0.369 × 10 | 0.428 × 10 | −0.354 × 10 |
| 17,182.1 Å | 1.0 | 0.625 × 10 | −0.550 × 10 | 0.679 × 10 | −0.518 × 10 |
| C = 0.94 × 10 | 3.0 | 0.106 | −0.831 × 10 | 0.103 | −0.748 × 10 |
| 5.0 | 0.123 | −0.941 × 10 | 0.119 | −0.857 × 10 | |
| 10.0 | 0.149 | −0.111 | 0.138 | −0.995 × 10 | |
| 20.0 | 0.175 | −0.128 | 0.162 | −0.117 | |
| N VI 2p-3s | 0.5 | 0.387 × 10 | 0.175 × 10 | 0.466 × 10 | 0.171 × 10 |
| 180.7 Å | 1.0 | 0.138 × 10 | 0.291 × 10 | 0.147 × 10 | 0.273 × 10 |
| C = 0.47 × 10 | 3.0 | 0.397 × 10 | 0.472 × 10 | 0.377 × 10 | 0.424 × 10 |
| 5.0 | 0.503 × 10 | 0.538 × 10 | 0.472 × 10 | 0.489 × 10 | |
| 10.0 | 0.658 × 10 | 0.642 × 10 | 0.583 × 10 | 0.583 × 10 | |
| 20.0 | 0.791 × 10 | 0.738 × 10 | 0.737 × 10 | 0.663 × 10 | |
| N VI 2p-4s | 0.5 | 0.319 × 10 | 0.583 × 10 | 0.324 × 10 | 0.539 × 10 |
| 131.9 Å | 1.0 | 0.595 × 10 | 0.813 × 10 | 0.587 × 10 | 0.747 × 10 |
| C = 0.10 × 10 | 3.0 | 0.105 × 10 | 0.110 × 10 | 0.962 × 10 | 0.994 × 10 |
| 5.0 | 0.127 × 10 | 0.126 × 10 | 0.113 × 10 | 0.114 × 10 | |
| 10.0 | 0.158 × 10 | 0.143 × 10 | 0.144 × 10 | 0.131 × 10 | |
| 20.0 | 0.193 × 10 | 0.166 × 10 | 0.157 × 10 | 0.146 × 10 | |
| N VI 3p-4s | 0.5 | 0.477 × 10 | 0.873 × 10 | 0.494 × 10 | 0.808 × 10 |
| 524.5 Å | 1.0 | 0.894 × 10 | 0.122 × 10 | 0.887 × 10 | 0.112 × 10 |
| C = 0.16 × 10 | 3.0 | 0.163 × 10 | 0.166 × 10 | 0.150 × 10 | 0.149 × 10 |
| 5.0 | 0.192 × 10 | 0.188 × 10 | 0.174 × 10 | 0.168 × 10 | |
| 10.0 | 0.246 × 10 | 0.218 × 10 | 0.211 × 10 | 0.197 × 10 | |
| 20.0 | 0.293 × 10 | 0.254 × 10 | 0.250 × 10 | 0.211 × 10 |
| B IV | B V | ||||
|---|---|---|---|---|---|
| Transition | T [10K] | Width [Å] | Shift [Å] | Width [Å] | Shift [Å] |
| Singlets | |||||
| N VI 1s-2p | 0.5 | 0.482 × 10 | −0.149 × 10 | 0.483 × 10 | −0.195 × 10 |
| 28.8 Å | 1.0 | 0.142 × 10 | −0.300 × 10 | 0.143 × 10 | −0.398 × 10 |
| C = 0.29 × 10 | 3.0 | 0.671 × 10 | −0.810 × 10 | 0.767 × 10 | −0.111 × 10 |
| 5.0 | 0.113 × 10 | −0.115 × 10 | 0.137 × 10 | −0.160 × 10 | |
| 10.0 | 0.179 × 10 | −0.159 × 10 | 0.230 × 10 | −0.227 × 10 | |
| 20.0 | 0.240 × 10 | −0.198 × 10 | 0.324 × 10 | −0.285 × 10 | |
| N VI 1s-3p | 0.5 | 0.515 × 10 | −0.125 × 10 | 0.631 × 10 | −0.169 × 10 |
| 24.9 Å | 1.0 | 0.123 × 10 | −0.187 × 10 | 0.168 × 10 | −0.264 × 10 |
| C = 0.16 × 10 | 3.0 | 0.268 × 10 | −0.282 × 10 | 0.373 × 10 | −0.406 × 10 |
| 5.0 | 0.325 × 10 | −0.320 × 10 | 0.465 × 10 | −0.464 × 10 | |
| 10.0 | 0.408 × 10 | −0.378 × 10 | 0.559 × 10 | −0.548 × 10 | |
| 20.0 | 0.483 × 10 | −0.430 × 10 | 0.703 × 10 | −0.644 × 10 | |
| N VI 2s-2p | 0.5 | 0.684 × 10 | −0.671 × 10 | 0.689 × 10 | −0.881 × 10 |
| 2897.2 Å | 1.0 | 0.302 × 10 | −0.132 × 10 | 0.322 × 10 | −0.177 × 10 |
| C = 0.29 × 10 | 3.0 | 0.184 × 10 | −0.299 × 10 | 0.231 × 10 | −0.420 × 10 |
| 5.0 | 0.283 × 10 | −0.380 × 10 | 0.370 × 10 | −0.538 × 10 | |
| 10.0 | 0.451 × 10 | −0.478 × 10 | 0.640 × 10 | −0.695 × 10 | |
| 20.0 | 0.593 × 10 | −0.574 × 10 | 0.842 × 10 | −0.835 × 10 | |
| N VI 2s-3p | 0.5 | 0.256 × 10 | −0.619 × 10 | 0.314 × 10 | −0.839 × 10 |
| 173.3 Å | 1.0 | 0.606 × 10 | −0.925 × 10 | 0.830 × 10 | −0.131 × 10 |
| C = 0.79 × 10 | 3.0 | 0.133 × 10 | −0.139 × 10 | 0.184 × 10 | −0.200 × 10 |
| 5.0 | 0.160 × 10 | −0.158 × 10 | 0.227 × 10 | −0.228 × 10 | |
| 10.0 | 0.201 × 10 | −0.186 × 10 | 0.276 × 10 | −0.274 × 10 | |
| 20.0 | 0.235 × 10 | −0.214 × 10 | 0.352 × 10 | −0.316 × 10 | |
| N VI 3s-3p | 0.5 | 0.118 × 10 | −0.270 × 10 | 0.147 × 10 | −0.364 × 10 |
| 9624.6 Å | 1.0 | 0.261 × 10 | −0.393 × 10 | 0.350 × 10 | −0.547 × 10 |
| C = 0.24 × 10 | 3.0 | 0.542 × 10 | −0.563 × 10 | 0.770 × 10 | −0.817 × 10 |
| 5.0 | 0.645 × 10 | −0.639 × 10 | 0.933 × 10 | −0.928 × 10 | |
| 10.0 | 0.777 × 10 | −0.750 × 10 | 0.117 | −0.110 | |
| 20.0 | 0.103 | −0.861 × 10 | 0.137 | −0.126 | |
| N VI 2p-3s | 0.5 | 0.107 × 10 | 0.401 × 10 | 0.122 × 10 | 0.537 × 10 |
| 187.9 Å | 1.0 | 0.343 × 10 | 0.647 × 10 | 0.433 × 10 | 0.904 × 10 |
| C = 0.37 × 10 | 3.0 | 0.891 × 10 | 0.101 × 10 | 0.124 × 10 | 0.147 × 10 |
| 5.0 | 0.110 × 10 | 0.116 × 10 | 0.157 × 10 | 0.168 × 10 | |
| 10.0 | 0.139 × 10 | 0.137 × 10 | 0.205 × 10 | 0.200 × 10 | |
| 20.0 | 0.178 × 10 | 0.159 × 10 | 0.246 × 10 | 0.231 × 10 | |
| Triplets | |||||
| N VI 2s-2p | 0.5 | 0.207 × 10 | −0.173 × 10 | 0.207 × 10 | −0.227 × 10 |
| 1901.5 Å | 1.0 | 0.780 × 10 | −0.345 × 10 | 0.808 × 10 | −0.460 × 10 |
| C = 0.19 × 10 | 3.0 | 0.483 × 10 | −0.840 × 10 | 0.574 × 10 | −0.116 × 10 |
| 5.0 | 0.790 × 10 | −0.108 × 10 | 0.107 × 10 | −0.153 × 10 | |
| 10.0 | 0.136 × 10 | −0.144 × 10 | 0.185 × 10 | −0.209 × 10 | |
| 20.0 | 0.178 × 10 | −0.172 × 10 | 0.251 × 10 | −0.250 × 10 | |
| Triplets | |||||
| N VI 2s-3p | 0.5 | 0.555 × 10 | 0.107 × 10 | 0.584 × 10 | 0.141 × 10 |
| 161.2 Å | 1.0 | 0.140 × 10 | 0.189 × 10 | 0.165 × 10 | 0.259 × 10 |
| C = 0.19 × 10 | 3.0 | 0.352 × 10 | 0.341 × 10 | 0.451 × 10 | 0.483 × 10 |
| 5.0 | 0.442 × 10 | 0.395 × 10 | 0.616 × 10 | 0.577 × 10 | |
| 10.0 | 0.560 × 10 | 0.476 × 10 | 0.781 × 10 | 0.691 × 10 | |
| 20.0 | 0.679 × 10 | 0.561 × 10 | 0.923 × 10 | 0.819 × 10 | |
| N VI 2s-4p | 0.5 | 0.396 × 10 | 0.555 × 10 | 0.468 × 10 | 0.754 × 10 |
| 122.4 Å | 1.0 | 0.694 × 10 | 0.788 × 10 | 0.904 × 10 | 0.111 × 10 |
| C = 0.48 × 10 | 3.0 | 0.120 × 10 | 0.111 × 10 | 0.169 × 10 | 0.161 × 10 |
| 5.0 | 0.141 × 10 | 0.127 × 10 | 0.198 × 10 | 0.183 × 10 | |
| 10.0 | 0.174 × 10 | 0.143 × 10 | 0.234 × 10 | 0.213 × 10 | |
| 20.0 | 0.193 × 10 | 0.166 × 10 | 0.304 × 10 | 0.247 × 10 | |
| N VI 3s-3p | 0.5 | 0.101 × 10 | −0.184 × 10 | 0.106 × 10 | −0.243 × 10 |
| 6993.0 Å | 1.0 | 0.254 × 10 | −0.328 × 10 | 0.297 × 10 | −0.450 × 10 |
| C = 0.36 × 10 | 3.0 | 0.630 × 10 | −0.598 × 10 | 0.810 × 10 | −0.848 × 10 |
| 5.0 | 0.801 × 10 | −0.700 × 10 | 0.111 × 10 | −0.102 × 10 | |
| 10.0 | 0.101 × 10 | −0.852 × 10 | 0.140 × 10 | −0.123 × 10 | |
| 20.0 | 0.126 × 10 | −0.999 × 10 | 0.171 × 10 | −0.144 × 10 | |
| N VI 3s-4p | 0.5 | 0.525 × 10 | 0.710 × 10 | 0.616 × 10 | 0.959 × 10 |
| 474.5 Å | 1.0 | 0.937 × 10 | 0.103 × 10 | 0.119 × 10 | 0.143 × 10 |
| C = 0.72 × 10 | 3.0 | 0.161 × 10 | 0.145 × 10 | 0.226 × 10 | 0.211 × 10 |
| 5.0 | 0.190 × 10 | 0.166 × 10 | 0.262 × 10 | 0.241 × 10 | |
| 10.0 | 0.233 × 10 | 0.198 × 10 | 0.333 × 10 | 0.281 × 10 | |
| 20.0 | 0.273 × 10 | 0.209 × 10 | 0.379 × 10 | 0.327 × 10 | |
| N VI 4s-4p | 0.5 | 0.527 × 10 | −0.555 × 10 | 0.613 × 10 | −0.753 × 10 |
| 17,182.1 Å | 1.0 | 0.922 × 10 | −0.837 × 10 | 0.118 | −0.119 |
| C = 0.94 × 10 | 3.0 | 0.159 | −0.127 | 0.215 | −0.183 |
| 5.0 | 0.185 | −0.146 | 0.254 | −0.211 | |
| 10.0 | 0.225 | −0.170 | 0.307 | −0.247 | |
| 20.0 | 0.251 | −0.194 | 0.350 | −0.285 | |
| N VI 2p-3s | 0.5 | 0.560 × 10 | 0.262 × 10 | 0.638 × 10 | 0.349 × 10 |
| 180.7 Å | 1.0 | 0.203 × 10 | 0.441 × 10 | 0.245 × 10 | 0.608 × 10 |
| C = 0.47 × 10 | 3.0 | 0.601 × 10 | 0.723 × 10 | 0.814 × 10 | 0.104 × 10 |
| 5.0 | 0.775 × 10 | 0.821 × 10 | 0.109 × 10 | 0.120 × 10 | |
| 10.0 | 0.100 × 10 | 0.989 × 10 | 0.141 × 10 | 0.143 × 10 | |
| 20.0 | 0.124 × 10 | 0.111 × 10 | 0.169 × 10 | 0.168 × 10 | |
| N VI 2p-4s | 0.5 | 0.472 × 10 | 0.883 × 10 | 0.597 × 10 | 0.122 × 10 |
| 131.9 Å | 1.0 | 0.892 × 10 | 0.124 × 10 | 0.120 × 10 | 0.174 × 10 |
| C = 0.10 × 10 | 3.0 | 0.164 × 10 | 0.168 × 10 | 0.233 × 10 | 0.244 × 10 |
| 5.0 | 0.194 × 10 | 0.191 × 10 | 0.284 × 10 | 0.278 × 10 | |
| 10.0 | 0.247 × 10 | 0.222 × 10 | 0.339 × 10 | 0.321 × 10 | |
| 20.0 | 0.293 × 10 | 0.258 × 10 | 0.385 × 10 | 0.365 × 10 | |
| N VI 3p-4s | 0.5 | 0.707 × 10 | 0.132 × 10 | 0.886 × 10 | 0.182 × 10 |
| 524.5 Å | 1.0 | 0.134 × 10 | 0.186 × 10 | 0.181 × 10 | 0.261 × 10 |
| C = 0.16 × 10 | 3.0 | 0.247 × 10 | 0.252 × 10 | 0.358 × 10 | 0.368 × 10 |
| 5.0 | 0.296 × 10 | 0.289 × 10 | 0.427 × 10 | 0.422 × 10 | |
| 10.0 | 0.367 × 10 | 0.340 × 10 | 0.524 × 10 | 0.472 × 10 | |
| 20.0 | 0.438 × 10 | 0.392 × 10 | 0.596 × 10 | 0.548 × 10 |
| B VI | B VI | |||||
|---|---|---|---|---|---|---|
| Transition | T [10 K] | Width [Å] | Shift [Å] | Transition | Width [Å] | Shift [Å] |
| Singlets | Triplets | |||||
| N VI1s-2p | 0.5 | 0.483 × 10 | −0.242 × 10 | N VI 2s-4p | 0.533 × 10 | 0.957 × 10 |
| 28.8 Å | 1.0 | 0.144 × 10 | −0.498 × 10 | 122.4 Å | 0.110 × 10 | 0.144 × 10 |
| C = 0.29 × 10 | 3.0 | 0.831 × 10 | −0.141 × 10 | C = 0.48 × 10 | 0.220 × 10 | 0.215 × 10 |
| 5.0 | 0.155 × 10 | −0.206 × 10 | 0.257 × 10 | 0.243 × 10 | ||
| 10.0 | 0.278 × 10 | −0.297 × 10 | 0.326 × 10 | 0.288 × 10 | ||
| 20.0 | 0.419 × 10 | −0.382 × 10 | 0.383 × 10 | 0.331 × 10 | ||
| N VI1s-3p | 0.5 | 0.716 × 10 | −0.215 × 10 | N VI 3s-3p | 0.109 × 10 | −0.302 × 10 |
| 24.9 Å | 1.0 | 0.208 × 10 | −0.348 × 10 | 6993.0 Å | 0.331 × 10 | −0.576 × 10 |
| C = 0.16 × 10 | 3.0 | 0.494 × 10 | −0.541 × 10 | C = 0.36 × 10 | 0.990 × 10 | −0.111 × 10 |
| 5.0 | 0.608 × 10 | −0.618 × 10 | 0.141 × 10 | −0.137 × 10 | ||
| 10.0 | 0.775 × 10 | −0.745 × 10 | 0.181 × 10 | −0.163 × 10 | ||
| 20.0 | 0.948 × 10 | −0.849 × 10 | 0.223 × 10 | −0.196 × 10 | ||
| N VI 2s-2p | 0.5 | 0.690 × 10 | −0.109 × 10 | N VI 3s-4p | 0.707 × 10 | 0.122 × 10 |
| 2897.2 Å | 1.0 | 0.334 × 10 | −0.222 × 10 | 474.5 Å | 0.147 × 10 | 0.186 × 10 |
| C = 0.29 × 10 | 3.0 | 0.267 × 10 | −0.544 × 10 | C = 0.72 × 10 | 0.295 × 10 | 0.284 × 10 |
| 5.0 | 0.463 × 10 | −0.699 × 10 | 0.349 × 10 | 0.322 × 10 | ||
| 10.0 | 0.828 × 10 | −0.931 × 10 | 0.430 × 10 | 0.380 × 10 | ||
| 20.0 | 0.110 × 10 | −0.112 × 10 | 0.501 × 10 | 0.434 × 10 | ||
| N VI 2s-3p | 0.5 | 0.358 × 10 | −0.107 × 10 | N VI 4s-4p | 0.682 × 10 | −0.956 × 10 |
| 173.3 Å | 1.0 | 0.103 × 10 | −0.172 × 10 | 17,182.1 Å | 0.143 | −0.156 |
| C = 0.79 × 10 | 3.0 | 0.244 × 10 | −0.266 × 10 | C = 0.94 × 10 | 0.274 | −0.243 |
| 5.0 | 0.298 × 10 | −0.306 × 10 | 0.327 | −0.280 | ||
| 10.0 | 0.386 × 10 | −0.366 × 10 | 0.400 | −0.336 | ||
| 20.0 | 0.475 × 10 | −0.416 × 10 | 0.475 | −0.381 | ||
| N VI 3s-3p | 0.5 | 0.174 × 10 | −0.464 × 10 | N VI 2p-3s | 0.694 × 10 | 0.438 × 10 |
| 9624.6 Å | 1.0 | 0.452 × 10 | −0.714 × 10 | 180.7 Å | 0.291 × 10 | 0.777 × 10 |
| C = 0.24 × 10 | 3.0 | 0.995 × 10 | −0.109 | C = 0.47 × 10 | 0.102 × 10 | 0.138 × 10 |
| 5.0 | 0.121 | −0.126 | 0.142 × 10 | 0.160 × 10 | ||
| 10.0 | 0.160 | −0.149 | 0.185 × 10 | 0.190 × 10 | ||
| 20.0 | 0.184 | −0.166 | 0.241 × 10 | 0.224 × 10 | ||
| N VI 2p-3s | 0.5 | 0.134 × 10 | 0.674 × 10 | N VI 2p-4s | 0.687 × 10 | 0.156 × 10 |
| 187.9 Å | 1.0 | 0.503 × 10 | 0.117 × 10 | 131.9 Å | 0.153 × 10 | 0.229 × 10 |
| C = 0.37 × 10 | 3.0 | 0.158 × 10 | 0.197 × 10 | C = 0.10 × 10 | 0.309 × 10 | 0.327 × 10 |
| 5.0 | 0.209 × 10 | 0.225 × 10 | 0.369 × 10 | 0.369 × 10 | ||
| 10.0 | 0.264 × 10 | 0.266 × 10 | 0.471 × 10 | 0.439 × 10 | ||
| 20.0 | 0.343 × 10 | 0.317 × 10 | 0.533 × 10 | 0.473 × 10 | ||
| Triplets | ||||||
| N VI 2s-2p | 0.5 | 0.207 × 10 | −0.281 × 10 | N VI 3p-4s | 0.101 × 10 | 0.233 × 10 |
| 1901.5 Å | 1.0 | 0.825 × 10 | −0.576 × 10 | 524.5 Å | 0.228 × 10 | 0.343 × 10 |
| C = 0.19 × 10 | 3.0 | 0.673 × 10 | −0.148 × 10 | C = 0.16 × 10 | 0.468 × 10 | 0.490 × 10 |
| 5.0 | 0.134 × 10 | −0.201 × 10 | 0.564 × 10 | 0.560 × 10 | ||
| 10.0 | 0.232 × 10 | −0.277 × 10 | 0.706 × 10 | 0.669 × 10 | ||
| 20.0 | 0.328 × 10 | −0.333 × 10 | 0.875 × 10 | 0.709 × 10 | ||
| N VI 2s-3p | 0.5 | 0.602 × 10 | 0.176 × 10 | |||
| 161.2 Å | 1.0 | 0.185 × 10 | 0.332 × 10 | |||
| C = 0.19 × 10 | 3.0 | 0.557 × 10 | 0.636 × 10 | |||
| 5.0 | 0.788 × 10 | 0.773 × 10 | ||||
| 10.0 | 0.101 × 10 | 0.921 × 10 | ||||
| 20.0 | 0.127 × 10 | 0.111 × 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dimitrijević, M.S.; Christova, M.D.; Sahal-Bréchot, S. Stark Broadening of N VI Spectral Lines. Universe 2023, 9, 511. https://doi.org/10.3390/universe9120511
Dimitrijević MS, Christova MD, Sahal-Bréchot S. Stark Broadening of N VI Spectral Lines. Universe. 2023; 9(12):511. https://doi.org/10.3390/universe9120511
Chicago/Turabian StyleDimitrijević, Milan S., Magdalena D. Christova, and Sylvie Sahal-Bréchot. 2023. "Stark Broadening of N VI Spectral Lines" Universe 9, no. 12: 511. https://doi.org/10.3390/universe9120511
APA StyleDimitrijević, M. S., Christova, M. D., & Sahal-Bréchot, S. (2023). Stark Broadening of N VI Spectral Lines. Universe, 9(12), 511. https://doi.org/10.3390/universe9120511








