GEMS Embeddings of Hayward Regular Black Holes in Massless and Massive Gravities
Abstract
1. Introduction
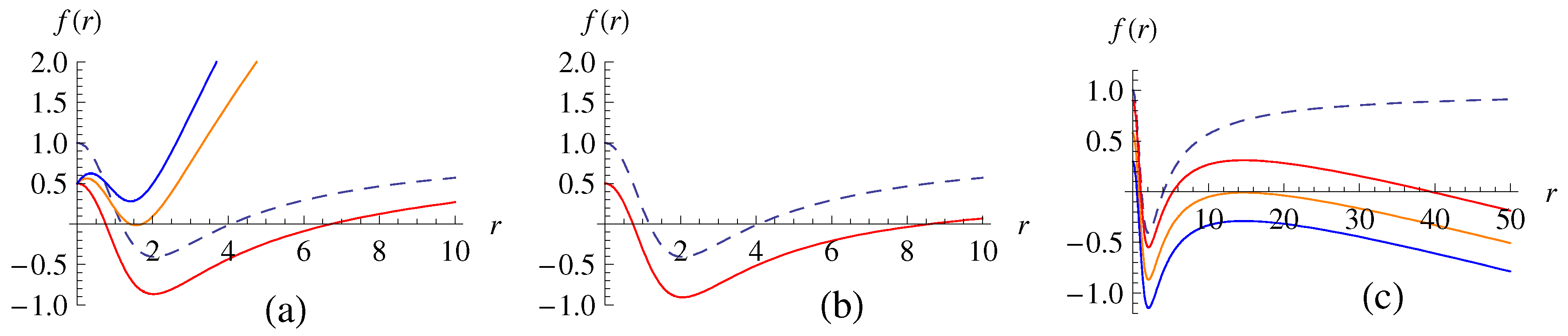
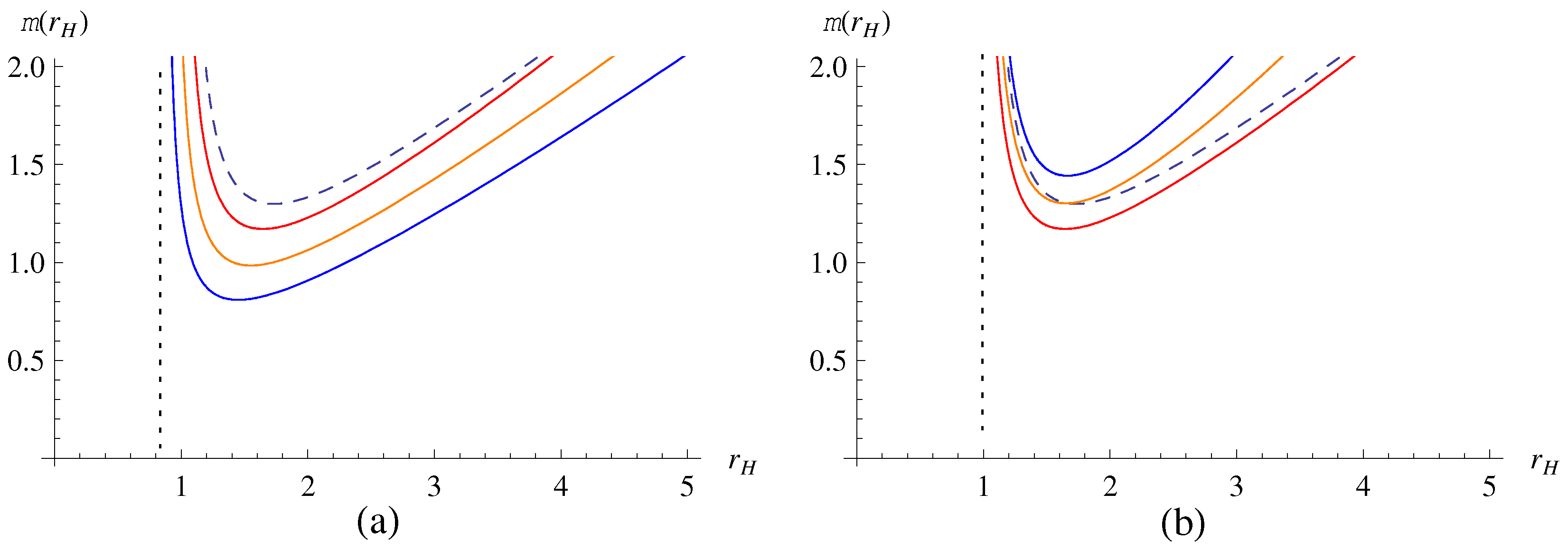
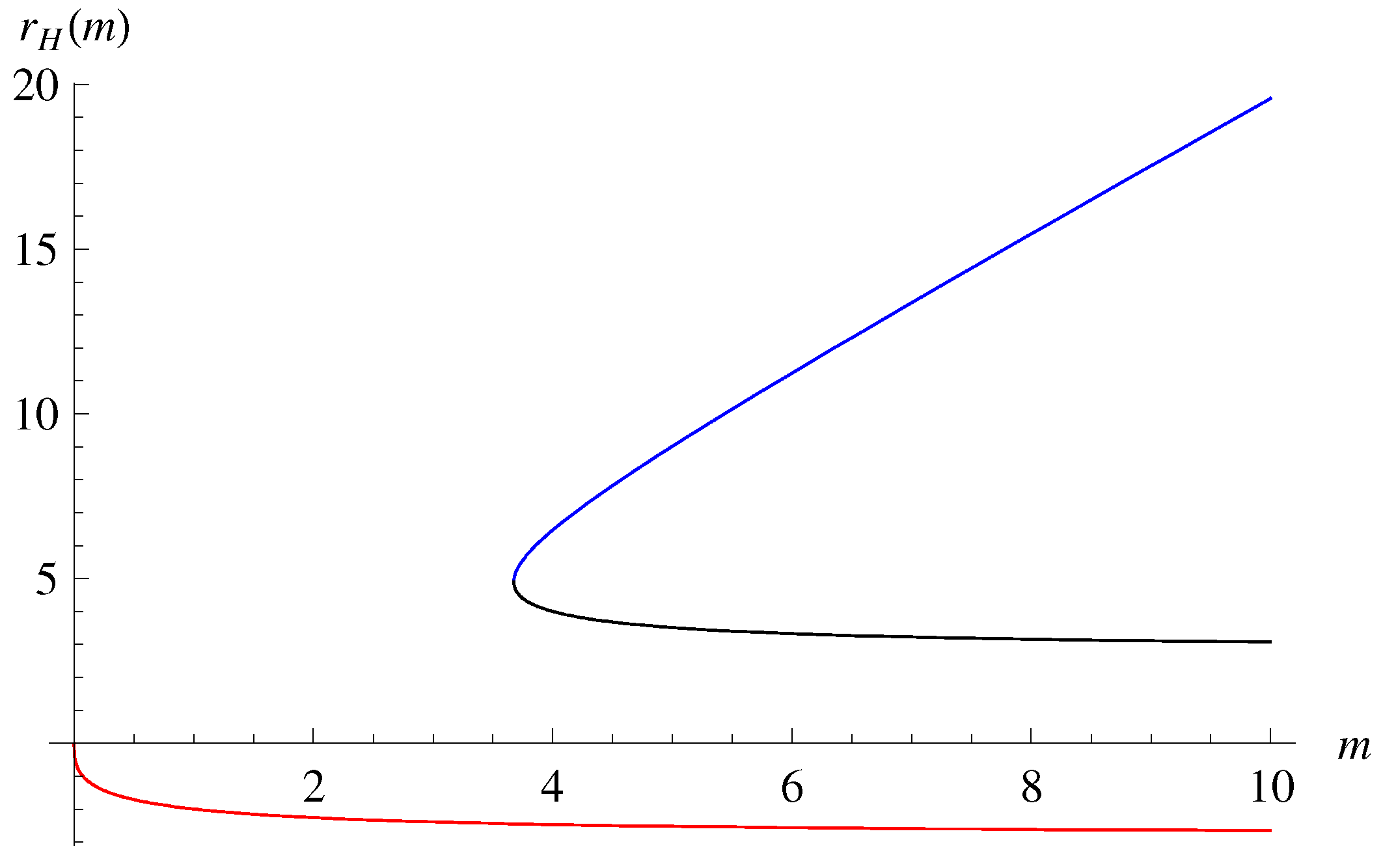
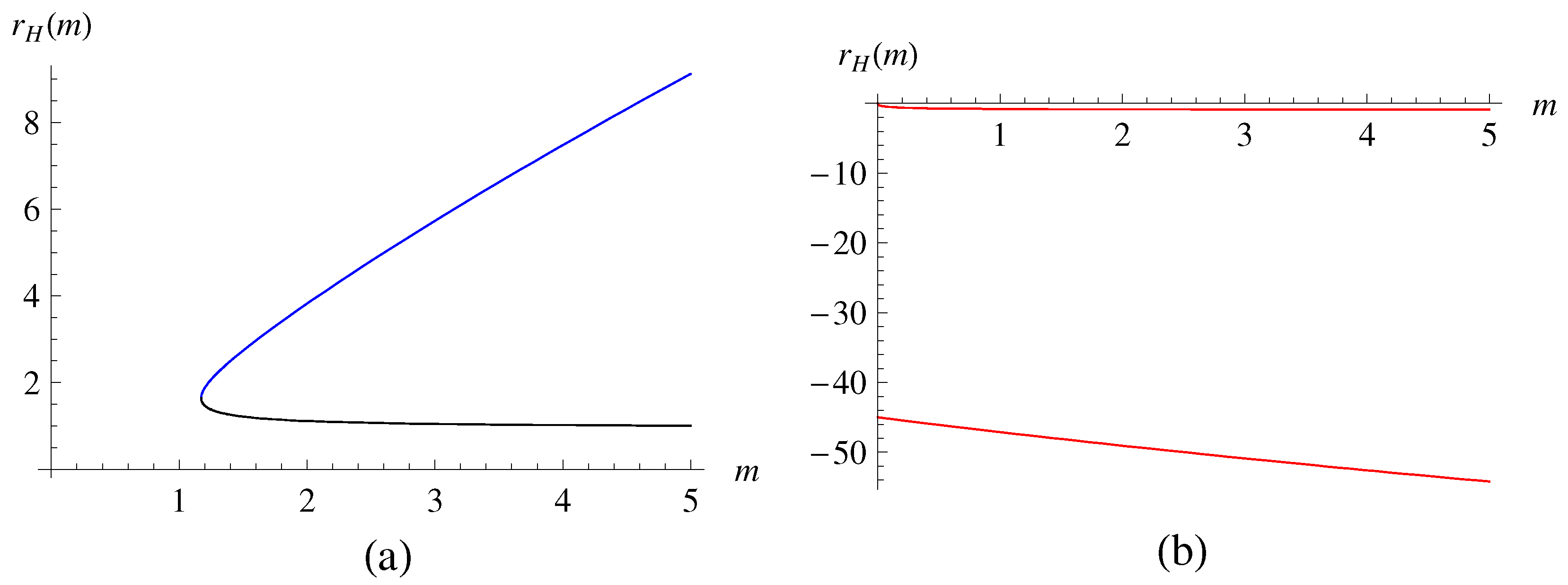
2. HRBH in Massive Gravity
2.1. Solutions of the HRBH in Massless Gravity
2.2. Solution of the HRBH in Massive Gravity
3. GEMS Embedding of HRBH
3.1. HRBH in Massless Gravity
3.2. HRBH in Massive Gravity
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Coefficients of Embedding Coordinates of zi (i = 5,6,7,8)
References
- Abbott, B.P. et al. [LIGO Scientific and Virgo] Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Akiyama, K. et al. [Event Horizon Telescope] First M87 Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole. Astrophys. J. Lett. 2019, 875, L1. [Google Scholar] [CrossRef]
- Cornish, N.; Blas, D.; Nardini, G. Bounding the speed of gravity with gravitational wave observations. Phys. Rev. Lett. 2017, 119, 161102. [Google Scholar] [CrossRef]
- Zhang, J.; Zhou, S.Y. Can the graviton have a large mass near black holes? Phys. Rev. D 2018, 97, 081501. [Google Scholar] [CrossRef]
- de Rham, C. The gravitational rainbow beyond Einstein gravity. Int. J. Mod. Phys. D 2019, 28, 1942003. [Google Scholar] [CrossRef]
- Vagnozzi, S.; Roy, R.; Tsai, Y.D.; Visinelli, L.; Afrin, M.; Allahyari, A.; Bambhaniya, P.; Dey, D.; Ghosh, S.G.; Joshi, P.S.; et al. Horizon-scale tests of gravity theories and fundamental physics from the Event Horizon Telescope image of Sagittarius A. Class. Quant. Grav. 2023, 40, 165007. [Google Scholar] [CrossRef]
- Reissner, H. Uber die eigengravitation des elektrischen feldes nach der einsteinschen theorie. Ann. Physik. 1916, 50, 106. [Google Scholar] [CrossRef]
- Nordström, G. Een en ander over de energie van het zwaarte krachtsveld volgens de theorie van einstein. Koninkl. Ned. Akad. Wetenschap. Proc. 1918, 20, 9. [Google Scholar]
- Kerr, R.P. Gravitational field of a spinning mass as an example of algebraically special metrics. Phys. Rev. Lett. 1963, 11, 237. [Google Scholar] [CrossRef]
- Newman, E.T.; Couch, E.; Chinnapared, K.; Exton, A.; Prakash, A.; Torrence, R. Metric of a rotating, charged Mass. J. Math. Phys. 1965, 6, 918. [Google Scholar] [CrossRef]
- Hawking, S.W.; Ellis, G.F.R. The Large Scale Structure of Space-Time; Cambridge University: Cambridge, UK, 1973. [Google Scholar]
- Gliner, E. Algebraic properties of the energy-momentum tensor and vacuumlike states of matter. Sov. Phys. JETP 1966, 22, 378. [Google Scholar]
- Sakharov, A.D. The Initial Stage of an Expanding Universe and the Appearance of a Nonuniform Distribution of Matter. Sov. Phys. JETP 1966, 22, 241. [Google Scholar]
- Bardeen, J. Non-singular general relativistic gravitational collapse. In Proceedings of the International Conference on Gravitation and the Theory of Relativity, Tbilisi, Georgia, 16–19 September 1968; p. 174. [Google Scholar]
- Ayon-Beato, E.; Garcia, A. The Bardeen model as a nonlinear magnetic monopole. Phys. Lett. B 2000, 493, 149. [Google Scholar] [CrossRef]
- Dymnikova, I. Vacuum nonsingular black hole. Gen. Rel. Grav. 1992, 24, 235. [Google Scholar] [CrossRef]
- Dymnikova, I.G. The algebraic structure of a cosmological term in spherically symmetric solutions. Phys. Lett. B 2000, 472, 33. [Google Scholar] [CrossRef]
- Bronnikov, K.A. Regular magnetic black holes and monopoles from nonlinear electrodynamics. Phys. Rev. D 2001, 63, 044005. [Google Scholar] [CrossRef]
- Hayward, S.A. Formation and evaporation of regular black holes. Phys. Rev. Lett. 2006, 96, 031103. [Google Scholar] [CrossRef]
- Ayon-Beato, E.; Garcia, A. Regular black hole in general relativity coupled to nonlinear electrodynamics. Phys. Rev. Lett. 1998, 80, 5056. [Google Scholar] [CrossRef]
- Mars, M.; Martín-Prats, M.M.; Senovilla, J.M.M. Models of regular Schwarzschild black holes satisfying weak energy conditions. Class. Quant. Grav. 1996, 13, L51. [Google Scholar] [CrossRef]
- Borde, A. Regular black holes and topology change. Phys. Rev. D 1997, 55, 7615. [Google Scholar] [CrossRef]
- Burinskii, A.; Hildebrandt, S.R. New type of regular black holes and particle-like solutions from NED. Phys. Rev. D 2002, 65, 104017. [Google Scholar] [CrossRef]
- Mbonye, M.R.; Kazanas, D. A Non-singular black hole model as a possible end-product of gravitational collapse. Phys. Rev. D 2005, 72, 024016. [Google Scholar] [CrossRef]
- Bronnikov, K.A.; Fabris, J.C. Regular phantom black holes. Phys. Rev. Lett. 2006, 96, 251101. [Google Scholar] [CrossRef]
- Berej, W.; Matyjasek, J.; Tryniecki, D.; Woronowicz, M. Regular black holes in quadratic gravity. Gen. Rel. Grav. 2006, 38, 885. [Google Scholar] [CrossRef]
- Bambi, C.; Modesto, L. Rotating regular black holes. Phys. Lett. B 2013, 721, 329. [Google Scholar] [CrossRef]
- Balart, L.; Vagenas, E.C. Regular black holes with a nonlinear electrodynamics source. Phys. Rev. D 2014, 90, 124045. [Google Scholar] [CrossRef]
- Balart, L.; Peña, F. Regular Charged Black Holes, Quasilocal Energy and Energy Conditions. Int. J. Mod. Phys. D 2016, 25, 1650072. [Google Scholar] [CrossRef]
- Fan, Z.Y.; Wang, X. Construction of Regular Black Holes in General Relativity. Phys. Rev. D 2016, 94, 124027. [Google Scholar] [CrossRef]
- Abbas, G.; Sabiullah, U. Geodesic Study of Regular Hayward Black Hole. Astrophys. Space Sci. 2014, 352, 769. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Schee, J. Circular geodesic of Bardeen and Ayon–Beato–Garcia regular black-hole and no-horizon spacetimes. Int. J. Mod. Phys. D 2014, 24, 1550020. [Google Scholar] [CrossRef]
- Zhao, S.S.; Xie, Y. Strong deflection gravitational lensing by a modified Hayward black hole. Eur. Phys. J. C 2017, 77, 272. [Google Scholar] [CrossRef]
- Chiba, T.; Kimura, M. A note on geodesics in the Hayward metric. PTEP 2017, 2017, 043E01. [Google Scholar] [CrossRef]
- Pradhan, P. Circular Geodesics, Paczyński-Witta Potential and QNMs in the Eikonal Limit for Ayón-Beato-García Black Hole. Universe 2018, 4, 55. [Google Scholar] [CrossRef]
- Dymnikova, I.; Poszwa, A. Classification and basic properties of circular orbits around regular black holes and solitons with the de Sitter center. Class. Quant. Grav. 2019, 36, 105002. [Google Scholar] [CrossRef]
- Zhang, H.; Zhou, N.; Liu, W.; Wu, X. Charged Particle Motions near Non-Schwarzschild Black Holes with External Magnetic Fields in Modified Theories of Gravity. Universe 2021, 7, 488. [Google Scholar] [CrossRef]
- Khan, S.U.; Ren, J.; Rayimbaev, J. Circular motion around a regular rotating Hayward black hole. Mod. Phys. Lett. A 2022, 37, 2250064. [Google Scholar] [CrossRef]
- Rayimbaev, J.; Bardiev, D.; Abdulxamidov, F.; Abdujabbarov, A.; Ahmedov, B. Magnetized and Magnetically Charged Particles Motion around Regular Bardeen Black Hole in 4D Einstein Gauss–Bonnet Gravity. Universe 2022, 8, 549. [Google Scholar] [CrossRef]
- Lamy, F.; Gourgoulhon, E.; Paumard, T.; Vincent, F.H. Imaging a non-singular rotating black hole at the center of the Galaxy. Class. Quant. Grav. 2018, 35, 115009. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Schee, J. Shadow of the regular Bardeen black holes and comparison of the motion of photons and neutrinos. Eur. Phys. J. C 2019, 79, 44. [Google Scholar] [CrossRef]
- Becerril, R.; Valdez-Alvarado, S.; Nucamendi, U.; Sheoran, P.; Dávila, J.M. Mass parameter and the bounds on redshifts and blueshifts of photons emitted from geodesic particle orbiting in the vicinity of regular black holes. Phys. Rev. D 2021, 103, 084054. [Google Scholar] [CrossRef]
- Ling, Y.; Wu, M.H. The Shadows of Regular Black Holes with Asymptotic Minkowski Cores. Symmetry 2022, 14, 2415. [Google Scholar] [CrossRef]
- Fernando, S.; Correa, J. Quasinormal Modes of Bardeen Black Hole: Scalar Perturbations. Phys. Rev. D 2012, 86, 064039. [Google Scholar] [CrossRef]
- Flachi, A.; Lemos, J.P.S. Quasinormal modes of regular black holes. Phys. Rev. D 2013, 87, 024034. [Google Scholar] [CrossRef]
- Lin, K.; Li, J.; Yang, S. Quasinormal Modes of Hayward Regular Black Hole. Int. J. Theor. Phys. 2013, 52, 3771. [Google Scholar] [CrossRef]
- Saleh, M.; Thomas, B.B.; Kofane, T.C. Quasinormal modes of gravitational perturbation around regular Bardeen black hole surrounded by quintessence. Eur. Phys. J. C 2018, 78, 325. [Google Scholar] [CrossRef]
- Cai, X.C.; Miao, Y.G. Quasinormal modes and shadows of a new family of Ayón-Beato-García black holes. Phys. Rev. D 2021, 103, 124050. [Google Scholar] [CrossRef]
- Myung, Y.S.; Kim, Y.W.; Park, Y.J. Thermodynamics of regular black hole. Gen. Rel. Grav. 2009, 41, 1051. [Google Scholar] [CrossRef]
- Sharif, M.; Javed, W. Thermodynamics of a Bardeen black hole in noncommutative space. Can. J. Phys. 2011, 89, 1027. [Google Scholar] [CrossRef]
- Saadat, H. Thermodynamical Stability of a New Regular Black Hole. Int. J. Theor. Phys. 2013, 52, 3255. [Google Scholar] [CrossRef]
- Tharanath, R.; Suresh, J.; Kuriakose, V.C. Phase transitions and Geometrothermodynamics of Regular black holes. Gen. Rel. Grav. 2015, 47, 46. [Google Scholar] [CrossRef]
- Sebastian, S.; Kuriakose, V.C. Spectroscopy and Thermodynamics of a Regular Black Hole. Int. J. Theor. Phys. 2015, 54, 3162. [Google Scholar] [CrossRef]
- Gan, Q.S.; Chen, J.H.; Wang, Y.J. Thermodynamics and geometrothermodynamics of regular black hole with nonlinear electrodynamics. Chin. Phys. B 2016, 25, 120401. [Google Scholar] [CrossRef]
- Ghosh, S.G.; Singh, D.V.; Maharaj, S.D. Regular black holes in Einstein-Gauss-Bonnet gravity. Phys. Rev. D 2018, 97, 104050. [Google Scholar] [CrossRef]
- Ali, M.S.; Ghosh, S.G. Exact d-dimensional Bardeen-de Sitter black holes and thermodynamics. Phys. Rev. D 2018, 98, 084025. [Google Scholar] [CrossRef]
- Aros, R.; Estrada, M. Regular black holes and its thermodynamics in Lovelock gravity. Eur. Phys. J. C 2019, 79, 259. [Google Scholar]
- Nam, C.H. Extended phase space thermodynamics of regular charged AdS black hole in Gauss–Bonnet gravity. Gen. Rel. Grav. 2019, 51, 100. [Google Scholar] [CrossRef]
- Kruglov, S.I. Non-Singular Model of Magnetized Black Hole Based on Nonlinear Electrodynamics. Universe 2019, 5, 225. [Google Scholar] [CrossRef]
- Kumar, A.; Walia, R.K.; Ghosh, S.G. Bardeen Black Holes in the Regularized 4D Einstein–Gauss–Bonnet Gravity. Universe 2022, 8, 232. [Google Scholar] [CrossRef]
- Kumar, A.; Baboolal, D.; Ghosh, S.G. Nonsingular Black Holes in 4D Einstein–Gauss–Bonnet Gravity. Universe 2022, 8, 244. [Google Scholar] [CrossRef]
- Merriam, A.; Sarwar, M.Z. Thermodynamics of Bardeen regular black hole with generalized uncertainty principle. Int. J. Mod. Phys. D 2022, 31, 2150128. [Google Scholar] [CrossRef]
- Sharif, M.; Khan, A. Thermodynamics of regular black hole with de Sitter core. Mod. Phys. Lett. A 2022, 37, 2250049. [Google Scholar] [CrossRef]
- Amelino-Camelia, G. Quantum-Spacetime Phenomenology. Living Rev. Rel. 2013, 16, 5. [Google Scholar] [CrossRef]
- Fierz, M.; Pauli, W. On relativistic wave equations for particles of arbitrary spin in an electromagnetic field. Proc. Roy. Soc. Lond. A 1939, 173, 211. [Google Scholar]
- Boulware, D.G.; Deser, S. Can gravitation have a finite range? Phys. Rev. D 1972, 6, 3368. [Google Scholar] [CrossRef]
- van Dam, H.; Veltman, M.J.G. Massive and massless Yang-Mills and gravitational fields. Nucl. Phys. B 1970, 22, 397. [Google Scholar] [CrossRef]
- Zakharov, V.I. Linearized gravitation theory and the graviton mass. JETP Lett. 1970, 12, 312. [Google Scholar]
- Vainshtein, A.I. To the problem of nonvanishing gravitation mass. Phys. Lett. B 1972, 39, 393. [Google Scholar] [CrossRef]
- de Rham, C.; Gabadadze, G. Generalization of the Fierz-Pauli Action. Phys. Rev. D 2010, 82, 044020. [Google Scholar] [CrossRef]
- de Rham, C.; Gabadadze, G.; Tolley, A.J. Resummation of Massive Gravity. Phys. Rev. Lett. 2011, 106, 231101. [Google Scholar] [CrossRef]
- Hassan, S.F.; Rosen, R.A. Resolving the Ghost Problem in non-Linear Massive Gravity. Phys. Rev. Lett. 2012, 108, 041101. [Google Scholar] [CrossRef]
- Hassan, S.F.; Rosen, R.A.; Schmidt-May, A. Ghost-free Massive Gravity with a General Reference Metric. JHEP 2012, 1202, 026. [Google Scholar] [CrossRef]
- Kluson, J. Note About Hamiltonian Structure of Non-Linear Massive Gravity. JHEP 2012, 1201, 013. [Google Scholar] [CrossRef]
- Kluson, J. Comments About Hamiltonian Formulation of Non-Linear Massive Gravity with Stuckelberg Fields. JHEP 2012, 1206, 170. [Google Scholar] [CrossRef]
- Kluson, J. Remark about Hamiltonian Formulation of Non-Linear Massive Gravity in Stuckelberg Formalism. Phys. Rev. D 2012, 86, 124005. [Google Scholar] [CrossRef]
- Comelli, D.; Crisostomi, M.; Nesti, F.; Pilo, L. Degrees of Freedom in Massive Gravity. Phys. Rev. D 2012, 86, 101502(R). [Google Scholar] [CrossRef]
- Golovnev, A. On the Hamiltonian analysis of non-linear massive gravity. Phys. Lett. B 2012, 707, 404. [Google Scholar] [CrossRef]
- Deffayet, C.; Mourad, J.; Zahariade, G. Covariant constraints in ghost free massive gravity. JCAP 2013, 1, 032. [Google Scholar] [CrossRef]
- Ghosh, S.G.; Tannukij, L.; Wongjun, P. A class of black holes in dRGT massive gravity and their thermodynamical properties. Eur. Phys. J. C 2016, 76, 119. [Google Scholar] [CrossRef]
- Arraut, I. The Black Hole Radiation in Massive Gravity. Universe 2018, 4, 27. [Google Scholar] [CrossRef]
- Panpanich, S.; Burikham, P. Fitting rotation curves of galaxies by de Rham-Gabadadze-Tolley massive gravity. Phys. Rev. D 2018, 98, 064008. [Google Scholar] [CrossRef]
- Hou, M.S.; Xu, H.; Ong, Y.C. Hawking Evaporation of Black Holes in Massive Gravity. Eur. Phys. J. C 2020, 80, 1090. [Google Scholar] [CrossRef]
- Akbarieh, A.R.; Kazempour, S.; Shao, L. Cosmological perturbations in Gauss-Bonnet quasi-dilaton massive gravity. Phys. Rev. D 2021, 103, 123518. [Google Scholar] [CrossRef]
- Aslmarand, S.M.; Akbarieh, A.R.; Izadi, Y.; Kazempour, S.; Shao, L. Cosmological aspects of cubic Galileon massive gravity. Phys. Rev. D 2021, 104, 083543. [Google Scholar] [CrossRef]
- Akbarieh, A.R.; Kazempour, S.; Shao, L. Cosmology and perturbations in tachyonic massive gravity. Phys. Rev. D 2022, 105, 023501. [Google Scholar] [CrossRef]
- Kazempour, S.; Zou, Y.C.; Akbarieh, A.R. Analysis of accretion disk around a black hole in dRGT massive gravity. Eur. Phys. J. C 2022, 82, 190. [Google Scholar] [CrossRef]
- Kazempour, S.; Akbarieh, A.R. Cosmology in Brans–Dicke–de Rham–Gabadadze–Tolley massive gravity. Phys. Rev. D 2022, 105, 123515. [Google Scholar] [CrossRef]
- Kazempour, S.; Akbarieh, A.R.; Motavalli, H.; Shao, L. Cosmology of Dirac-Born-Infeld-de Rham-Gabadadze-Tolley massive gravity. Phys. Rev. D 2022, 106, 023508. [Google Scholar] [CrossRef]
- Panpanich, S.; Ponglertsakul, S.; Tannukij, L. Particle motions and Gravitational Lensing in de Rham-Gabadadze-Tolley Massive Gravity Theory. Phys. Rev. D 2019, 100, 044031. [Google Scholar] [CrossRef]
- Upadhyay, S.; Mandal, S.; Myrzakulov, Y.; Myrzakulov, K. Weak deflection angle, greybody bound and shadow for charged massive BTZ black hole. Ann. Phys. 2023, 450, 169242. [Google Scholar] [CrossRef]
- Hendi, S.H.; Jafarzade, K.; Eslam Panah, B. Black holes in dRGT massive gravity with the signature of EHT observations of M87. JCAP 2023, 2, 022. [Google Scholar] [CrossRef]
- Vegh, D. Holography without translational symmetry. arXiv 2013, arXiv:1301.0537. [Google Scholar]
- Davison, R.A. Momentum relaxation in holographic massive gravity. Phys. Rev. D 2013, 88, 086003. [Google Scholar] [CrossRef]
- Blake, M.; Tong, D. Universal Resistivity from Holographic Massive Gravity. Phys. Rev. D 2013, 88, 106004. [Google Scholar] [CrossRef]
- Blake, M.; Tong, D.; Vegh, D. Holographic Lattices Give the Graviton an Effective Mass. Phys. Rev. Lett. 2014, 112, 071602. [Google Scholar] [CrossRef] [PubMed]
- Cai, R.G.; Hu, Y.P.; Pan, Q.Y.; Zhang, Y.L. Thermodynamics of Black Holes in Massive Gravity. Phys. Rev. D 2015, 91, 024032. [Google Scholar] [CrossRef]
- Adams, A.; Roberts, D.A.; Saremi, O. Hawking-Page transition in holographic massive gravity. Phys. Rev. D 2015, 91, 046003. [Google Scholar] [CrossRef]
- Hendi, S.H.; Panahiyan, S.; Eslam Panah, B. Charged Black Hole Solutions in Gauss-Bonnet-Massive Gravity. JHEP 2016, 1601, 129. [Google Scholar] [CrossRef]
- Hu, Y.P.; Wu, X.M.; Zhang, H. Generalized Vaidya Solutions and Misner-Sharp mass for n-dimensional massive gravity. Phys. Rev. D 2017, 95, 084002. [Google Scholar] [CrossRef]
- Zou, D.C.; Yue, R.; Zhang, M. Reentrant phase transitions of higher-dimensional AdS black holes in dRGT massive gravity. Eur. Phys. J. C 2017, 77, 256. [Google Scholar] [CrossRef]
- Hendi, S.H.; Mann, R.B.; Panahiyan, S.; Eslam Panah, B. Van der Waals like behavior of topological AdS black holes in massive gravity. Phys. Rev. D 2017, 95, 021501(R). [Google Scholar] [CrossRef]
- Tannukij, L.; Wongjun, P.; Ghosh, S.G. Black String in dRGT Massive Gravity. Eur. Phys. J. C 2017, 77, 846. [Google Scholar] [CrossRef]
- Hendi, S.H.; Bordbar, G.H.; Eslam Panah, B.; Panahiyan, S. Neutron stars structure in the context of massive gravity. JCAP 2017, 7, 004. [Google Scholar] [CrossRef]
- Hendi, S.H.; Eslam Panah, B.; Panahiyan, S.; Liu, H.; Meng, X.-H. Black holes in massive gravity as heat engines. Phys. Lett. B 2018, 781, 40. [Google Scholar] [CrossRef]
- Panah, B.E.; Liu, H.L. White dwarfs in de Rham-Gabadadze-Tolley like massive gravity. Phys. Rev. D 2019, 99, 104074. [Google Scholar] [CrossRef]
- Hendi, S.H.; Dehghani, A. Criticality and extended phase space thermodynamics of AdS black holes in higher curvature massive gravity. Eur. Phys. J. C 2019, 79, 227. [Google Scholar] [CrossRef]
- Chabab, M.; El Moumni, H.; Iraoui, S.; Masmar, K. Phase transitions and geothermodynamics of black holes in dRGT massive gravity. Eur. Phys. J. C 2019, 79, 342. [Google Scholar] [CrossRef]
- Panah, B.E.; Hendi, S.H. Black hole solutions correspondence between conformal and massive theories of gravity. EPL 2019, 125, 60006. [Google Scholar] [CrossRef]
- Hong, S.T.; Kim, Y.W.; Park, Y.J. Tidal effects in Schwarzschild black hole in holographic massive gravity. Phys. Lett. B 2020, 811, 135967. [Google Scholar] [CrossRef]
- Hong, S.T.; Kim, Y.W.; Park, Y.J. GUP corrected entropy of the Schwarzschild black hole in massive gravity. Mod. Phys. Lett. A 2022, 37, 2250186. [Google Scholar] [CrossRef]
- Hong, S.T.; Kim, Y.W.; Park, Y.J. Local free-fall temperatures of charged BTZ black holes in massive gravity. Phys. Rev. D 2019, 99, 024047. [Google Scholar] [CrossRef]
- Hong, S.T.; Kim, Y.W.; Park, Y.J. GEMS embeddings and freely falling temperatures of Schwarzschild(-AdS) black holes in massive gravity. Phys. Lett. B 2020, 800, 135116. [Google Scholar] [CrossRef]
- Fronsdal, C. Completion and Embedding of the Schwarzschild Solution. Phys. Rev. 1959, 116, 778. [Google Scholar] [CrossRef]
- Rosen, J. Embedding of Various Relativistic Riemannian Spaces in Pseudo-Euclidean Spaces. Rev. Mod. Phys. 1965, 37, 204. [Google Scholar] [CrossRef]
- Goenner, H.F. Local isometric embedding of Riemannian manifolds and Einstein’s theory of gravitation. In General Relativity and Gravitation: One Hundred Years after the Birth of Albert Einstein; Held, A., Ed.; Plenum: New York, NY, USA, 1980; Volume 1, pp. 441–468. [Google Scholar]
- Hawking, S.W. Particle Creation by Black Holes. Commun. Math. Phys. 1975, 43, 199, Erratum in: Commun. Math. Phys. 1976, 46, 206. [Google Scholar] [CrossRef]
- Unruh, W.G. Notes on black hole evaporation. Phys. Rev. D 1976, 14, 870. [Google Scholar] [CrossRef]
- Deser, S.; Levin, O. Accelerated detectors and temperature in (anti)-de Sitter spaces. Class. Quant. Grav. 1997, 14, L163. [Google Scholar] [CrossRef]
- Deser, S.; Levin, O. Equivalence of Hawking and Unruh temperatures through flat space embeddings. Class. Quant. Grav. 1998, 15, L85. [Google Scholar] [CrossRef]
- Deser, S.; Levin, O. Mapping Hawking into Unruh thermal properties. Phys. Rev. D 1999, 59, 064004. [Google Scholar] [CrossRef]
- Hong, S.T.; Kim, Y.W.; Park, Y.J. Higher dimensional flat embeddings of (2+1)-dimensional black holes. Phys. Rev. D 2000, 62, 024024. [Google Scholar] [CrossRef]
- Kim, Y.W.; Park, Y.J.; Soh, K.S. Reissner-Nordstrom AdS black hole in the GEMS approach. Phys. Rev. D 2000, 62, 104020. [Google Scholar] [CrossRef]
- Hong, S.T. Complete higher dimensional global embedding structures of various black holes. Gen. Rel. Grav. 2004, 36, 1919. [Google Scholar] [CrossRef]
- Chen, H.Z.; Tian, Y.; Gao, Y.H.; Song, X.C. The GEMS approach to stationary motions in the spherically symmetric spacetimes. JHEP 2004, 0410, 011. [Google Scholar] [CrossRef]
- Santos, N.L.; Dias, O.J.C.; Lemos, J.P.S. Global embedding of D-dimensional black holes with a cosmological constant in Minkowskian spacetimes: Matching between Hawking temperature and Unruh temperature. Phys. Rev. D 2004, 70, 124033. [Google Scholar] [CrossRef]
- Banerjee, R.; Majhi, B.R. A New Global Embedding Approach to Study Hawking and Unruh Effects. Phys. Lett. B 2010, 690, 83. [Google Scholar] [CrossRef]
- Cai, R.G.; Myung, Y.S. Hawking temperature for constant curvature black bole and its analogue in de Sitter space. Phys. Rev. D 2011, 83, 107502. [Google Scholar] [CrossRef]
- Hu, B.; Li, H.F. Mapping Hawking temperature in the spinning constant curvature black hole spaces into Unruh temperature. Mod. Phys. Lett. A 2012, 27, 1250002. [Google Scholar]
- Hong, S.T.; Kim, W.T.; Kim, Y.W.; Park, Y.J. Global embeddings of scalar-tensor theories in (2+1)-dimensions. Phys. Rev. D 2000, 62, 064021. [Google Scholar] [CrossRef]
- Hong, S.T. Thermodynamics of (1+1) dilatonic black holes in global flat embedding scheme. Phys. Lett. B 2005, 623, 135. [Google Scholar] [CrossRef]
- Hong, S.T.; Kim, S.W. Can wormholes have negative temperatures? Mod. Phys. Lett. A 2006, 21, 789. [Google Scholar] [CrossRef]
- Paston, S.A. Hawking into Unruh mapping for embeddings of hyperbolic type. Class. Quant. Grav. 2015, 32, 145009. [Google Scholar] [CrossRef][Green Version]
- Sheykin, A.A.; Solovyev, D.P.; Paston, S.A. Global embeddings of BTZ and Schwarzschild-AdS type black holes in a flat space. Symmetry 2019, 11, 841. [Google Scholar] [CrossRef]
- Paston, S.A.; Sheykin, A.A. From the Embedding Theory to General Relativity in a result of inflation. Int. J. Mod. Phys. D 2012, 21, 1250043. [Google Scholar] [CrossRef]
- Paston, S.A.; Sheykin, A.A. Embedding theory as new geometrical mimetic gravity. Eur. Phys. J. C 2018, 78, 989. [Google Scholar] [CrossRef]
- Paston, S.A. Dark matter from non-relativistic embedding gravity. Mod. Phys. Lett. A 2021, 36, 2150101. [Google Scholar] [CrossRef]
- Paston, S.A. Non-Relativistic Limit of Embedding Gravity as General Relativity with Dark Matter. Universe 2020, 6, 163. [Google Scholar] [CrossRef]
- Brynjolfsson, E.J.; Thorlacius, L. Taking the Temperature of a Black Hole. JHEP 2008, 2008, 066. [Google Scholar] [CrossRef]
- Kim, Y.W.; Choi, J.; Park, Y.J. Local free-fall temperature of Gibbons-Maeda-Garfinkle-Horowitz-Strominger black holes. Phys. Rev. D 2014, 89, 044004. [Google Scholar] [CrossRef]
- Hong, S.T.; Kim, Y.W.; Park, Y.J. GEMS Embeddings of Schwarzschild and RN Black Holes in Painlevé-Gullstrand Spacetimes. Universe 2021, 8, 15. [Google Scholar] [CrossRef]
- Zhou, T.; Modesto, L. Geodesic incompleteness of some popular regular black holes. Phys. Rev. D 2023, 107, 044016. [Google Scholar] [CrossRef]
- Barriola, M.; Vilenkin, A. Gravitational Field of a Global Monopole. Phys. Rev. Lett. 1989, 63, 341. [Google Scholar] [CrossRef]
- Riegert, R.J. Birkhoff’s Theorem in Conformal Gravity. Phys. Rev. Lett. 1984, 53, 315. [Google Scholar] [CrossRef]
- Saffari, R.; Rahvar, S. f(R) Gravity: From the Pioneer Anomaly to the Cosmic Acceleration. Phys. Rev. D 2008, 77, 104028. [Google Scholar] [CrossRef]
- Milgrom, M. A Modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. Astrophys. J. 1983, 270, 365. [Google Scholar] [CrossRef]
- Gregoris, D.; Ong, Y.C.; Wang, B. A critical assessment of black hole solutions with a linear term in their redshift function. Eur. Phys. J. C 2021, 81, 684. [Google Scholar] [CrossRef]
- Hong, S.T.; Kim, Y. Warp products and (2+1) dimensional spacetimes. Gen. Rel. Grav. 2014, 46, 1781. [Google Scholar] [CrossRef][Green Version]
- Weisstein, E.W. CRC Concise Encyclopedia of Mathematics; Chapman & Hall: New York, NY, USA; CRC: New York, NY, USA, 2003. [Google Scholar]

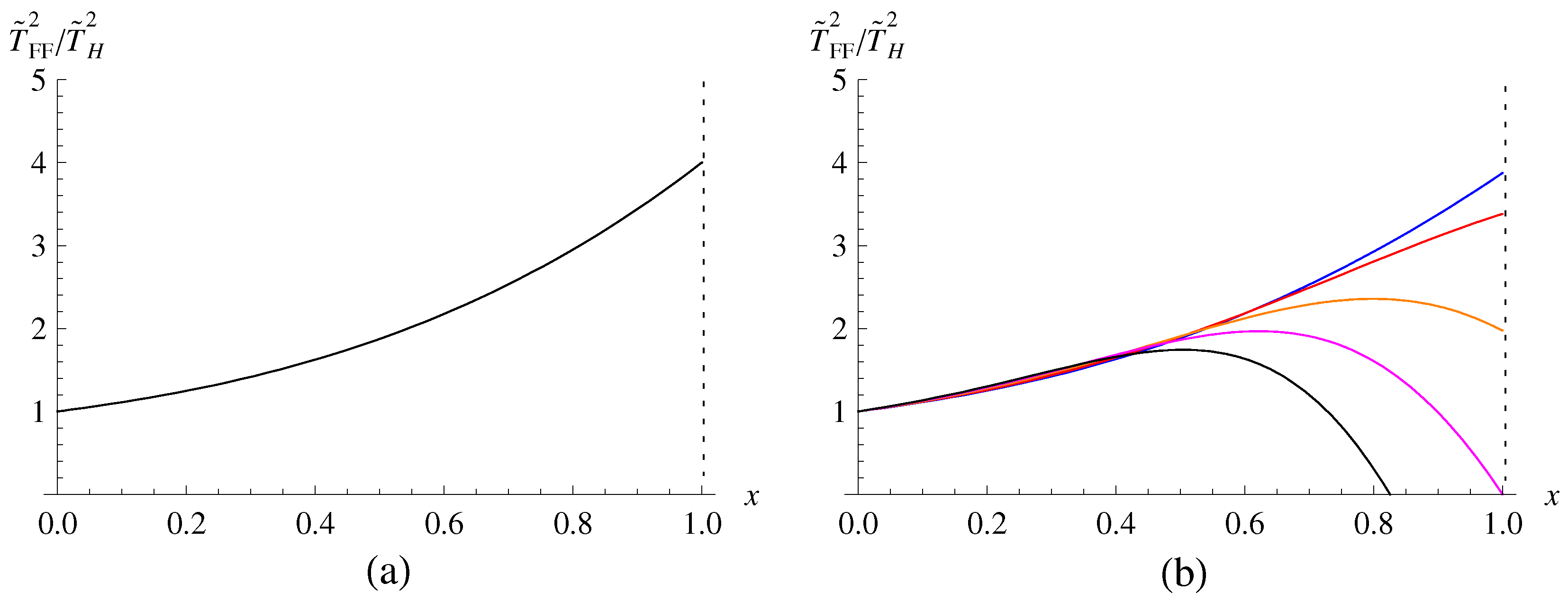
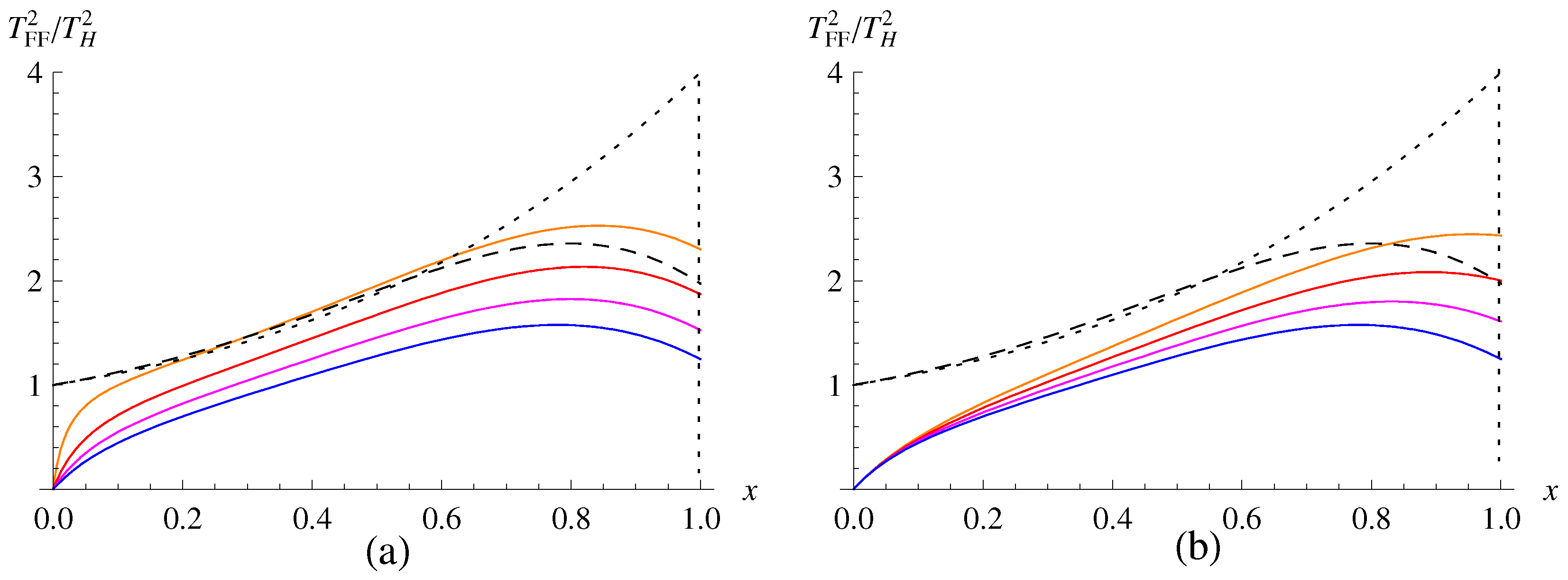
| max(3) | max(2) | max(2) | max(2) | max(2) | |
| 0 | 0 | 2 | 2 | max(2) | |
| 0 | 0 | max(3) | max(3) | max(3) |
| 1 | 1 | max(2) | 0 | 0 | |
| 0 | 0 | 2 | 1 | 0 | |
| 0 | 0 | max(3) | max(3) | max(3) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hong, S.-T.; Kim, Y.-W.; Park, Y.-J. GEMS Embeddings of Hayward Regular Black Holes in Massless and Massive Gravities. Universe 2023, 9, 486. https://doi.org/10.3390/universe9110486
Hong S-T, Kim Y-W, Park Y-J. GEMS Embeddings of Hayward Regular Black Holes in Massless and Massive Gravities. Universe. 2023; 9(11):486. https://doi.org/10.3390/universe9110486
Chicago/Turabian StyleHong, Soon-Tae, Yong-Wan Kim, and Young-Jai Park. 2023. "GEMS Embeddings of Hayward Regular Black Holes in Massless and Massive Gravities" Universe 9, no. 11: 486. https://doi.org/10.3390/universe9110486
APA StyleHong, S.-T., Kim, Y.-W., & Park, Y.-J. (2023). GEMS Embeddings of Hayward Regular Black Holes in Massless and Massive Gravities. Universe, 9(11), 486. https://doi.org/10.3390/universe9110486






