Anisotropic Universes Sourced by Modified Chaplygin Gas
Abstract
1. Introduction
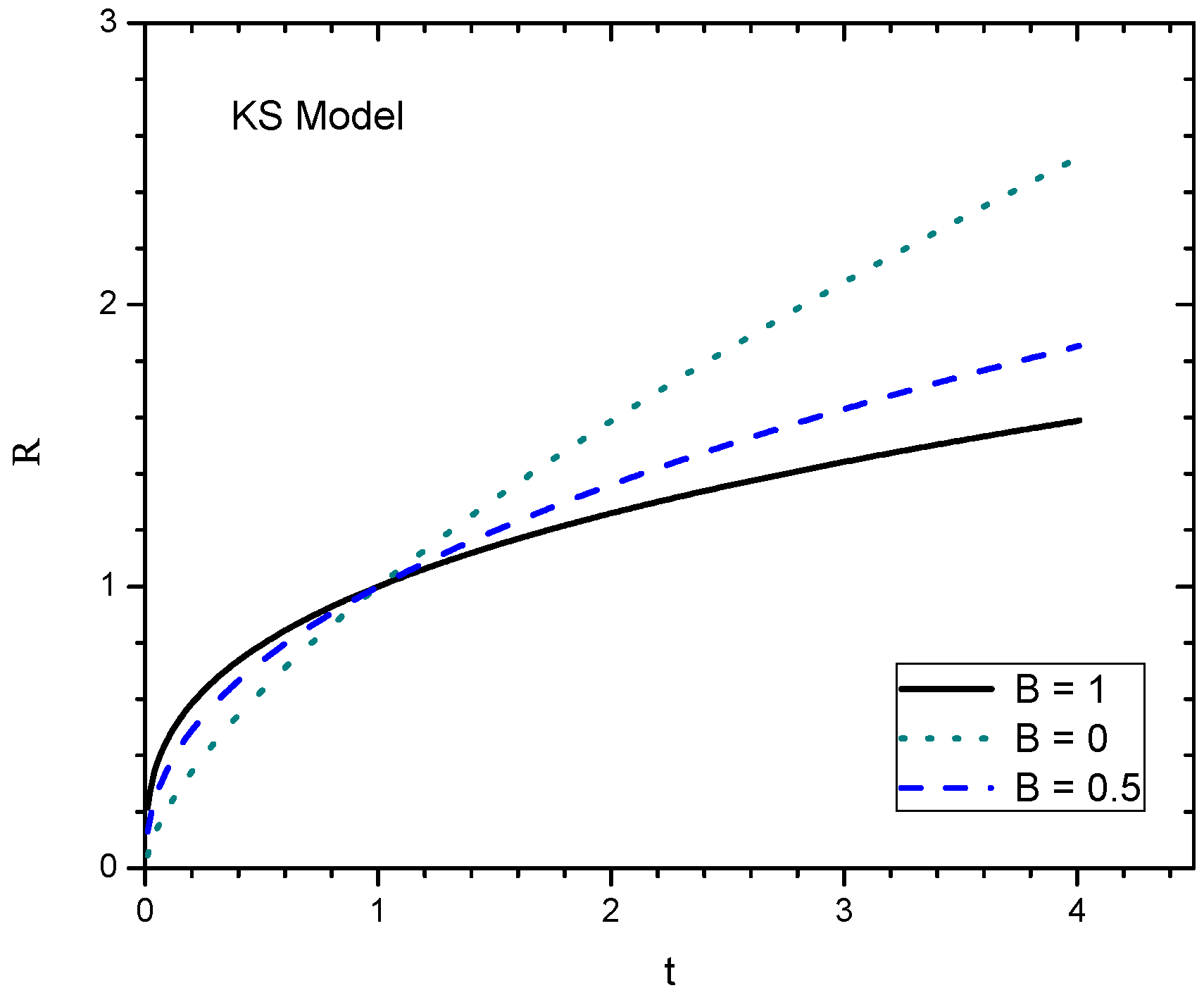
2. KS Model
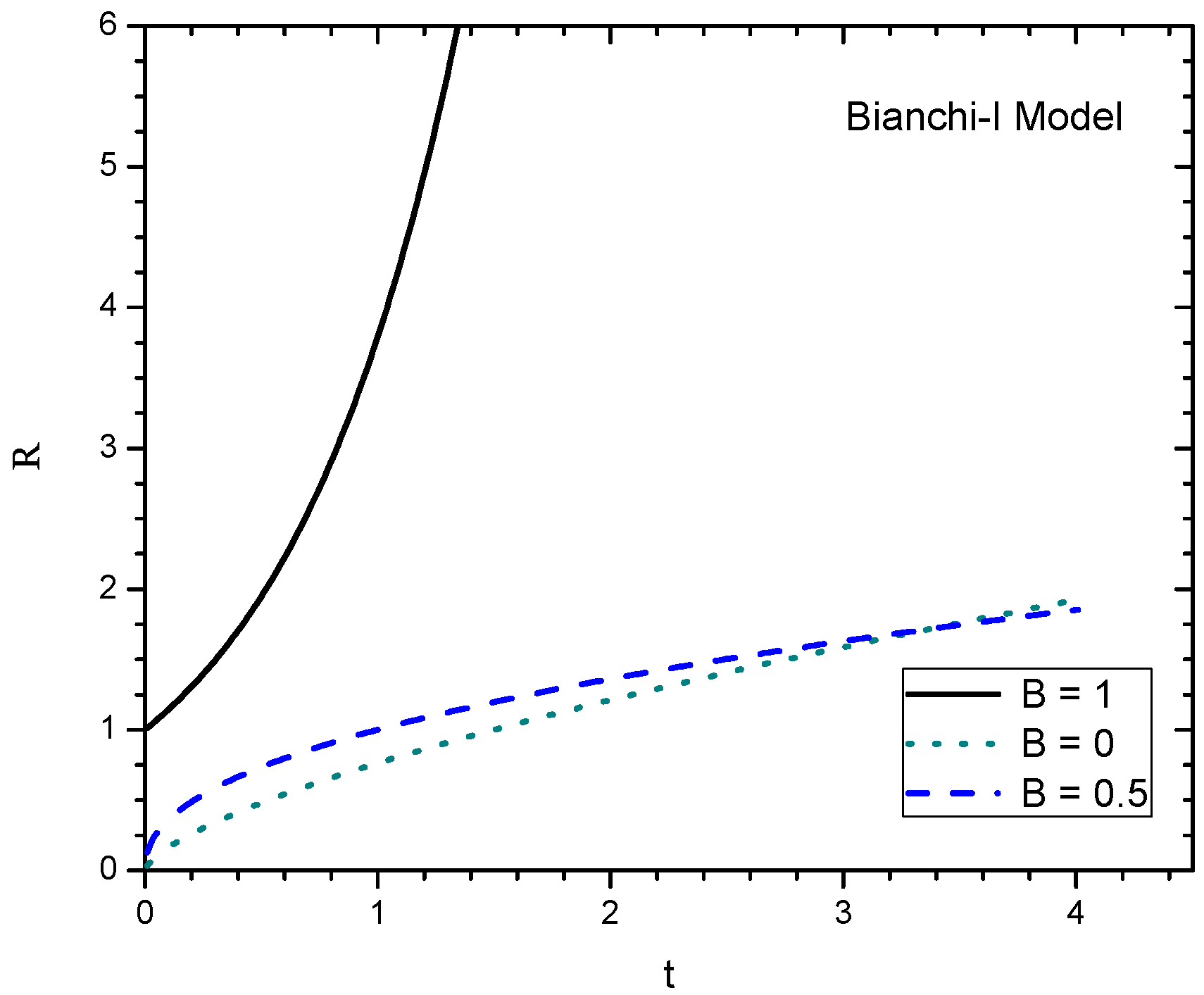
3. Bianchi-I Model
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Antoniou, I.; Perivolaropoulos, L. Searching for a Cosmological Preferred Axis: Union2 data analysis and comparison with other probes. J. Cosmol. Astropart. Phys. 2010, 12, 012. [Google Scholar] [CrossRef]
- Colin, J.; Mohayaee, R.; Rameez, M.; Sarkar, S. Evidence for anisotropy of cosmic acceleration. Astron. Astrophys. 2019, 631, L13. [Google Scholar] [CrossRef]
- Fosalba, P.; Gaztanaga, E. Explaining cosmological anisotropy: Evidence for causal horizons from CMB data. Mon. Not. R. Astron. Soc. 2021, 504, 5840–5862. [Google Scholar] [CrossRef]
- Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; Benabed, K.; et al. Planck 2018 results: VII. Isotropy and statistics of the CMB. Astron. Astrophys. 2020, 641, A7. [Google Scholar]
- Tripathy, S.K. Late time acceleration and role of skewness in anisotropic models. Astrophys. Space Sci. 2014, 350, 367–374. [Google Scholar] [CrossRef]
- Schwarz, D.J.; Copi, C.J.; Huterer, D.; Starkman, G.D. CMB anomalies after Planck. Class. Quant. Grav. 2016, 33, 184001. [Google Scholar] [CrossRef]
- Misner, C.W. The Isotropy of the Universe. Astrophys. J. 1968, 151, 431–457. [Google Scholar] [CrossRef]
- Kanotowski, R.; Sachs, R.K. Some Spatially Homogeneous Anisotropic Relativistic Cosmological Models. J. Math. Phys. 1966, 7, 443–446. [Google Scholar] [CrossRef]
- Bianchi, L. On three dimensional spaces which admit a group of motions. Gen. Relativ. Gravit. 2001, 33, 2157–2253. [Google Scholar] [CrossRef]
- Taub, A.H. Empty space-times admitting a three parameter group of motions. Ann. Math. 1951, 53, 472–490, Reprinted in Gen. Relativ. Gravit. 2004, 36, 2699–2719. [Google Scholar] [CrossRef]
- Heckman, O.; Schücking, E. Relativistic Cosmology in Gravitation: An Introduction to Current Research; Witten, L., Ed.; Wiley: New York, NY, USA; London, UK, 1962. [Google Scholar]
- Ellis, G.F.R.; MacCallum, M.A.H. A class of homogeneous cosmological models. Comm. Math. Phys. 1969, 12, 108–141. [Google Scholar] [CrossRef]
- Huang, W. Anisotropic Cosmological Models with Energy Density Dependent Bulk Viscosity. J. Math. Phys. 1990, 31, 1456–1462. [Google Scholar] [CrossRef]
- Chimento1, L.P.; Jakubi1, A.S.; Méndez, V.; Maartens, R. Cosmological solutions with nonlinear bulk viscosity. Class. Quantum Gravit. 1997, 14, 3363–3375. [Google Scholar] [CrossRef]
- Saha, B. Anisotropic cosmological models with perfect fluid and dark energy reexamined. Int. J. Theor. Phys. 2006, 45, 983–995. [Google Scholar] [CrossRef]
- Saha, B. Anisotropic Cosmological Models with a Perfect Fluid and a Term. Astrophys. Space Sci. 2006, 302, 83–91. [Google Scholar] [CrossRef]
- Sengupta, R.; Paul, P.; Paul, B.C.; Ray, S. Inflation in anisotropic brane universe using tachyon field. Int. J. Mod. Phys. D 2019, 28, 1941010. [Google Scholar] [CrossRef]
- Collins, C.B. Global structure of the “Kantowski–Sachs’’ cosmological models. J. Math. Phys. 1977, 18, 2116–2124. [Google Scholar] [CrossRef]
- Weber, E. Kantowski–Sachs cosmological models approaching isotropy. J. Math. Phys. 1984, 25, 3279–3285. [Google Scholar] [CrossRef]
- Linde, A.D. Initial conditions for inflation. Phys. Lett. B 1985, 162, 281–286. [Google Scholar] [CrossRef]
- Bradley, M.; Dunsby, P.K.S.; Forsberg, M.; Keresztes, Z. Perturbations of Kantowski-Sachs models. Class. Quantum Gravit. 2012, 29, 095023. [Google Scholar] [CrossRef]
- Kamenshchik, A.; Moschella, U.; Pasquier, V. An alternative to quintessence. Phys. Lett. B 2001, 511, 265–268. [Google Scholar] [CrossRef]
- Bento, M.C.; Bertolami, O.; Sen, A.A. Generalized Chaplygin gas, accelerated expansion, and dark-energy-matter unification. Phys. Rev. D 2002, 66, 043507. [Google Scholar] [CrossRef]
- Pourhassan, B.; Kahya, E.O. Extended Chaplygin gas model. Res. Phys. 2014, 4, 101. [Google Scholar] [CrossRef]
- Hulke, N.; Singh, G.P.; Bishi, B.K.; Singh, A. Variable Chaplygin gas cosmologies in f(R,T) gravity with particle creation. New Astron. 2020, 77, 101357. [Google Scholar] [CrossRef]
- Bilic, N.; Tupper, G.B.; Viollier, R. Dark Matter, Dark Energy and the Chaplygin Gas. arXiv 2002, arXiv: Astro-ph/0207423. [Google Scholar]
- Benaoum, H.B. Modified Chaplygin Gas Cosmology. Adv. High Energy Phys. 2012, 2012, 357802. [Google Scholar] [CrossRef]
- Benaoum, H.B. Modified Chaplygin Gas Cosmology with Bulk Viscosity in D Dimensions. Int. J. Mod. Phys. D 2014, 23, 1450082. [Google Scholar] [CrossRef]
- Bandyopadhyay, T. Thermodynamics of Gauss-Bonnet brane with modified Chaplygin gas. Astrophys. Space Sci. 2012, 341, 689–693. [Google Scholar] [CrossRef]
- Panigrahi, D.; Chatterjee, S. Viability of Variable Generalised Chaplygin gas—A thermodynamical approach. Int. J. Mod. Phys. D 2012, 21, 1250079. [Google Scholar] [CrossRef]
- Wu, Y.-B.; Li, S.; Lu, J.-B.; Yang, X.-Y. The modified Chaplygin gas as a unified dark sector model. Mod. Phys. Lett. A 2007, 22, 783–790. [Google Scholar] [CrossRef]
- Bilic, N.; Tupper, G.B.; Violler, R. Unification of dark matter and dark energy: The inhomogeneous Chaplygin gas. Phys. Lett. B 2002, 535, 17–21. [Google Scholar] [CrossRef]
- Liu, D.-J.; Li, X.-Z. Cosmic Microwave Background Radiation constraints on a modified Chaplygin gas model. Chin. Phys. Lett. 2005, 22, 1600. [Google Scholar]
- Lixin, X.; Yuting, W.; Hyerim, N. Modified Chaplygin gas as a unified dark matter and dark energy model and cosmic constraints. Eur. Phys. J. C 2012, 72, 1931. [Google Scholar]
- Dark Matter and Dark Energy: A Challenge for Modern Cosmology; Matarrese, S., Colpi, M., Gorini, V., Moschella, U., Eds.; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Jawad, A.; Ilyas, A.; Rani, S. Dynamics of modified Chaplygin gas inflation on the Brane with bulk viscous pressure. Int. J. Mod. Phys. D 2017, 26, 1750031. [Google Scholar] [CrossRef]
- Safsafi, A.; Khay, I.; Salamate, F.; Chakir, H.; Bennai, M. On Chaplygin Gas Braneworld Inflation with Monomial Potential. Adv. High Energy Phys. 2018, 73, 1. [Google Scholar] [CrossRef]
- Silva, P.T.; Bertolami, O. Expected constraints on the generalized Chaplygin equation of state from future supernova experiments and gravitational lensing statistics. Astron. Astrophys. 2003, 599, 829–838. [Google Scholar] [CrossRef]
- Dev, A.; Jain, D.; Alcaniz, J.S. Constraints on Chaplygin quartessence from the CLASS gravitational lens statistics and supernova data. Astron. Astrophys. 2004, 417, 847–852. [Google Scholar] [CrossRef]
- Bertolami, O.; Silva, P.T. Gamma-ray bursts as dark energy–matter probes in the context of the generalized Chaplygin gas model. Mon. Not. R. Astron. Soc. 2006, 365, 1149–1159. [Google Scholar] [CrossRef][Green Version]
- Thakur, P.; Ghosh, S.; Paul, B.C. Modified Chaplygin gas and constraints on its B parameter from cold dark matter and unified dark matter energy cosmological models. Mon. Not. R. Astron. Soc. 2009, 397, 1935–1939. [Google Scholar] [CrossRef]
- Chakraborty, S.; Debnath, U.; Ranjit, C. Observational constraints of modified Chaplygin gas in loop quantum cosmology. Eur. Phys. J. C 2012, 72, 2101. [Google Scholar] [CrossRef]
- Ray, S.; Paul, P.; Sengupta, R.; Pant, N.; Nag, R. Modified Chaplygin gas in anisotropic universes on the brane. Int. J. Mod. Phys. D 2021, 30, 2150093. [Google Scholar] [CrossRef]
- Shukla, A.K.; Raushan, R.; Mandal, S.; Chaubey, R.; Nag, R. Observational constraints in modified Chaplygin gas cosmological model. Astron. Comput. 2023, 44, 100736. [Google Scholar] [CrossRef]
- Sengupta, R.; Paul, P.; Paul, B.C.; Kalam, M. Can extended Chaplygin gas source a Hubble tension resolved emergent universe? arXiv 2023, arXiv:2307.02602. [Google Scholar]
- Zheng, J.; Cao, S.; Lian, Y.; Liu, T.; Liu, Y.; Zhu, Z.H. Revisiting Chaplygin gas cosmologies with the recent observations of high-redshift quasars. Eur. Phys. J. C 2022, 82, 582. [Google Scholar] [CrossRef]
- Benaoum, H.B.; Luongo, O.; Quevedo, H.; Chaubey, R.; Nag, R. Extensions of modified Chaplygin gas from Geometrothermodynamics. Eur. Phys. J. C 2019, 79, 577. [Google Scholar] [CrossRef]
- Benaoum, H.B. Accelerated Universe from Modified Chaplygin Gas and Tachyonic Fluid. Universe 2022, 8, 340. [Google Scholar] [CrossRef]
- Guo, Z.K.; Zhang, Y.Z. Cosmology with a Variable Chaplygin Gas. Phys. Lett. B 2007, 645, 326. [Google Scholar] [CrossRef]
- Lu, J.; Geng, D.; Xu, L.; Wu, Y.; Liu, M. Reduced modified Chaplygin gas cosmology. JHEP 2015, 02, 071. [Google Scholar] [CrossRef]
- Panotopoulos, G.; Rincon, Á.; Lopes, I. Slowly rotating dark energy stars. arXiv 2021, arXiv:2109.05619. [Google Scholar] [CrossRef]
- Abellán, G.; Rincón, Á.; Sanchez, E. A Generalized Double Chaplygin Model for Anisotropic Matter: The Newtonian Case. Universe 2023, 9, 352. [Google Scholar] [CrossRef]
- Chaubey, R.; Shukla, A.K.; Singh, A.; Singh, T. General class of Bianchi cosmological models with Λ in creation-field cosmology. Astrophys. Space Sci. 2014, 352, 839–857. [Google Scholar] [CrossRef]
- Chaubey, R.; Singh, A.; Raushan, R. Chameleon scalar field in LRS Bianchi type I cosmological model. Gravit. Cosmol 2016, 22, 54–63. [Google Scholar] [CrossRef]
- Sengupta, R.; Paul, B.C.; Paul, P. Skyrme Fluid in Anisotropic Universe. Pramana Phys. 2022, 96, 114. [Google Scholar] [CrossRef]
- Alvarado, R. The Hubble constant and the deceleration parameter in anisotropic cosmological spaces of Petrov type D. Adv. Stud. Theor. Phys. 2016, 10, 421. [Google Scholar] [CrossRef]
- Tripathy, S.K.; Behera, D.; Mishra, B. Unified dark fluid in Brans–Dicke theory. Eur. Phys. J. C 2015, 75, 149. [Google Scholar] [CrossRef]
- Mishra, B.; Esmaeili, F.M.D.; Ray, S. Cosmological models with variable anisotropic parameter in f(R,T) gravity. Ind. J. Phys. 2021, 95, 2245–2254. [Google Scholar] [CrossRef]
- Weinberg, S. Cosmology; Oxford University Press: Oxford, UK, 2008. [Google Scholar]
- Tripathy, S.K.; Mishra, B. Phantom cosmology in an extended theory of gravity. Chin. J. Phys. 2020, 63, 448–458. [Google Scholar] [CrossRef]
- Mukhopadhyay, U.; Ray, S.; Usmani, A.A.; Ghosh, P.P. Time variable Λ and the accelerating universe. Int. J. Theor. Phys. 2011, 50, 752–759. [Google Scholar] [CrossRef]
- Rapetti, D.; Allen, S.W.; Amin, M.A.; Blandford, R.D. A kinematical approach to dark energy studies. Mon. Not. R. Astron. Soc. 2007, 375, 1510–1520. [Google Scholar] [CrossRef]
- Kumar, S. Observational constraints on Hubble constant and deceleration parameter in power-law cosmology. Mon. Not. R. Astron. Soc. 2012, 422, 2532–2538. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ray, S.; Tripathy, S.K.; Sengupta, R.; Bal, B.; Rout, S.M. Anisotropic Universes Sourced by Modified Chaplygin Gas. Universe 2023, 9, 453. https://doi.org/10.3390/universe9100453
Ray S, Tripathy SK, Sengupta R, Bal B, Rout SM. Anisotropic Universes Sourced by Modified Chaplygin Gas. Universe. 2023; 9(10):453. https://doi.org/10.3390/universe9100453
Chicago/Turabian StyleRay, Saibal, Sunil Kumar Tripathy, Rikpratik Sengupta, Bibhudutta Bal, and Sonali Monalisa Rout. 2023. "Anisotropic Universes Sourced by Modified Chaplygin Gas" Universe 9, no. 10: 453. https://doi.org/10.3390/universe9100453
APA StyleRay, S., Tripathy, S. K., Sengupta, R., Bal, B., & Rout, S. M. (2023). Anisotropic Universes Sourced by Modified Chaplygin Gas. Universe, 9(10), 453. https://doi.org/10.3390/universe9100453






