Investigation of Alpha-Proton Drift Speeds in the Solar Wind: WIND and HELIOS Observations
Abstract
1. Introduction
2. Data and Methodology
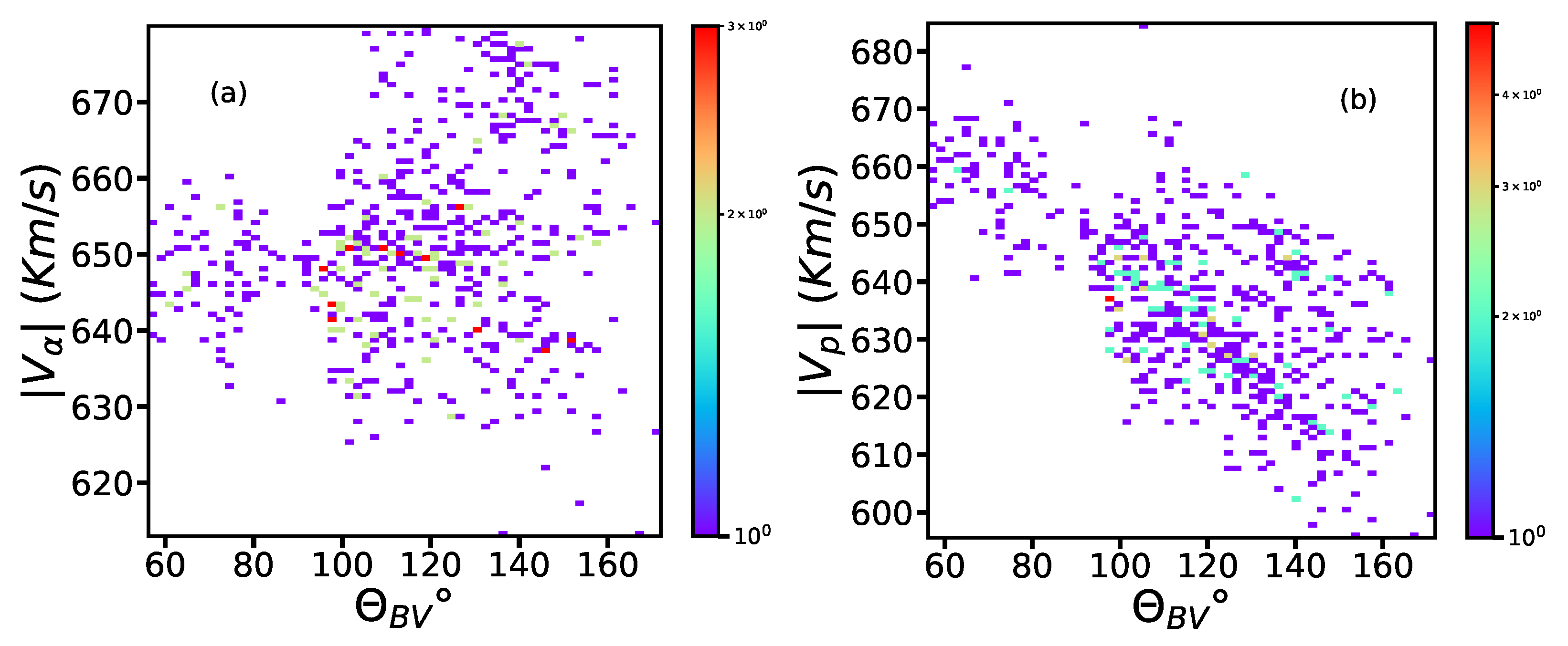
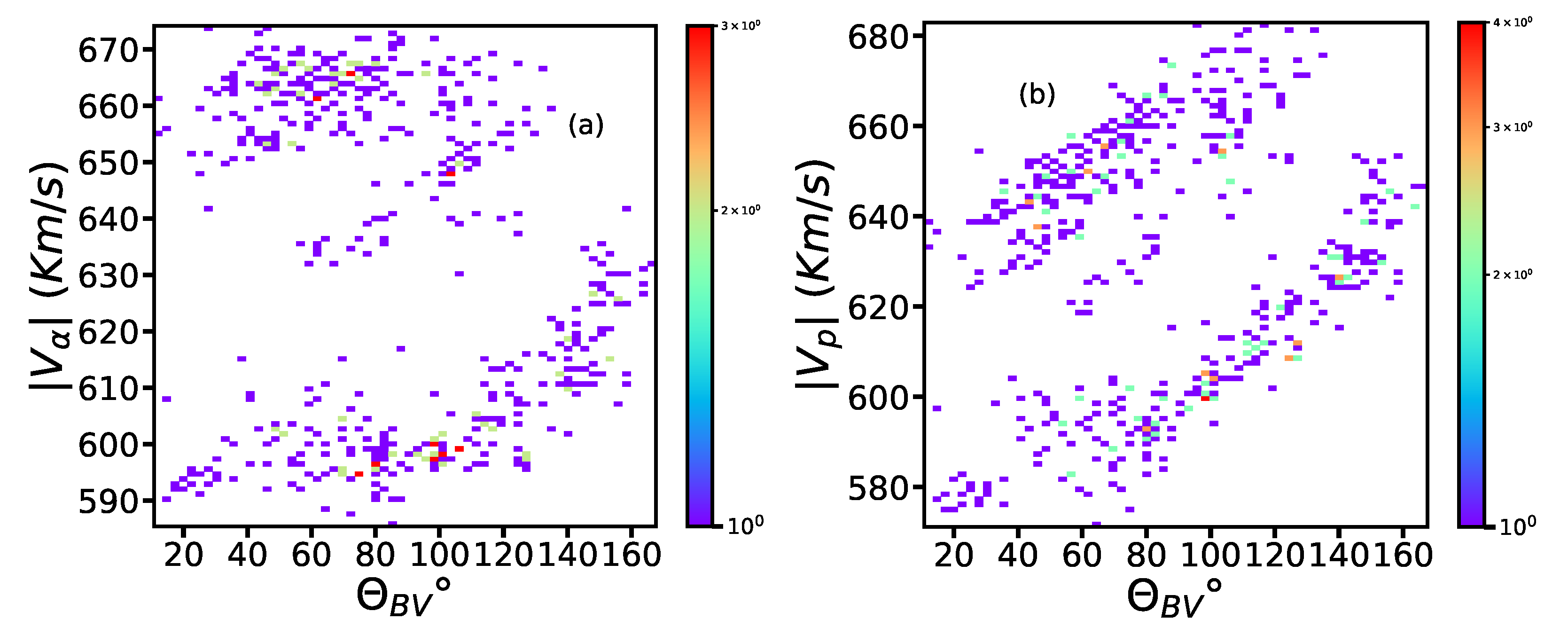
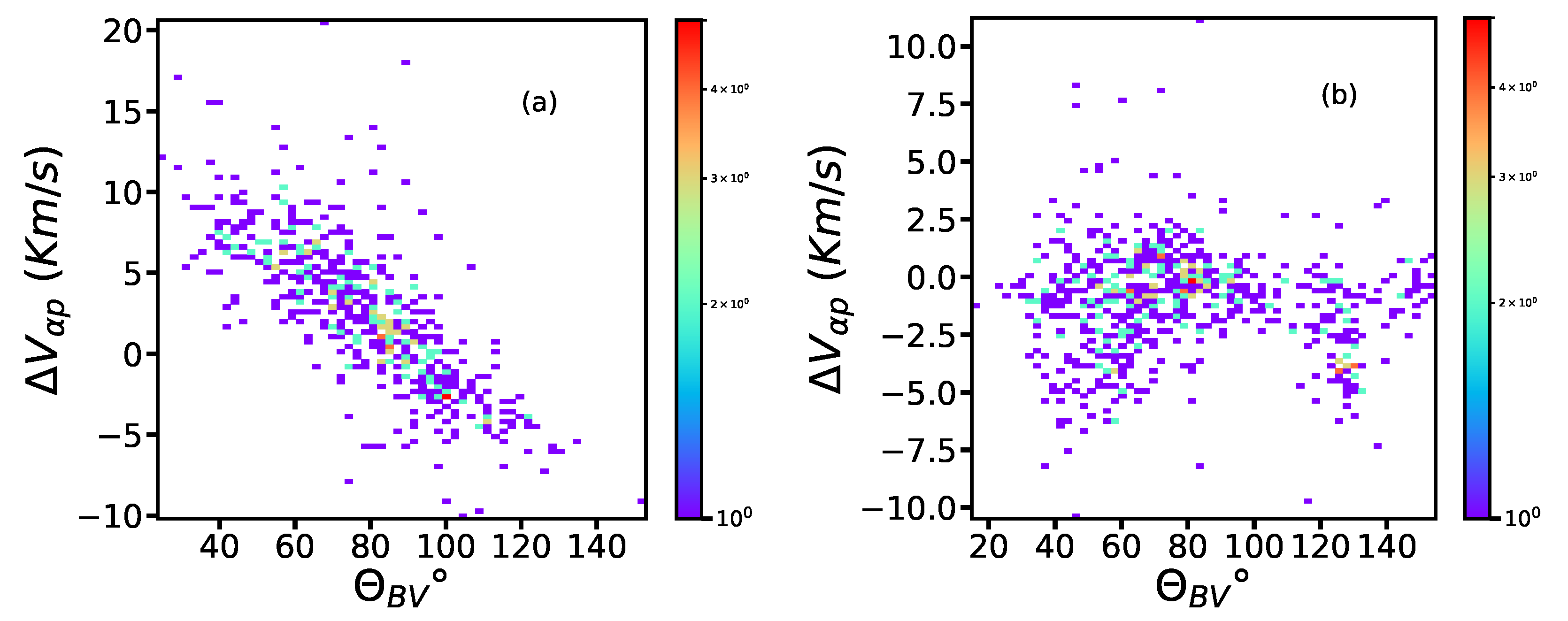
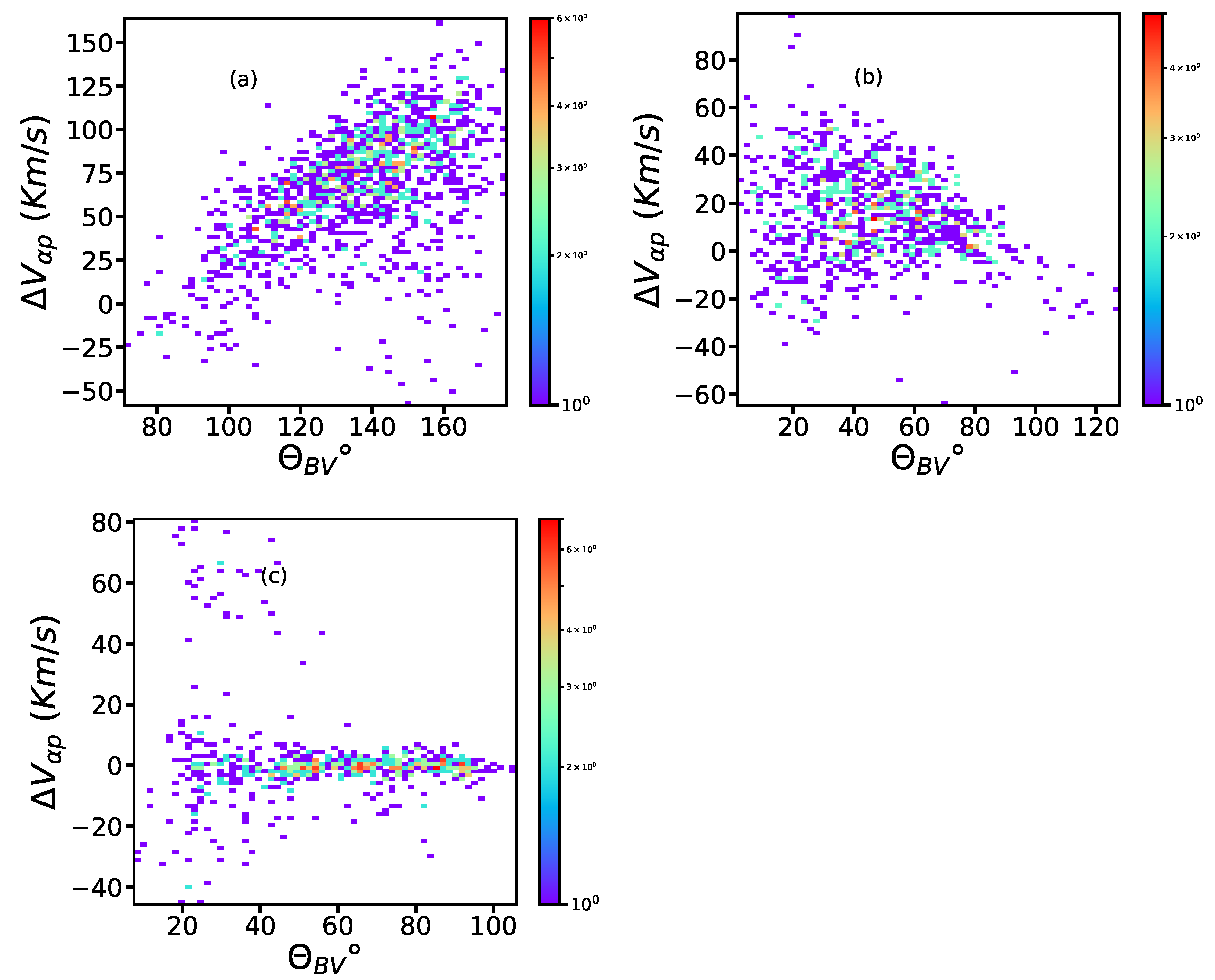
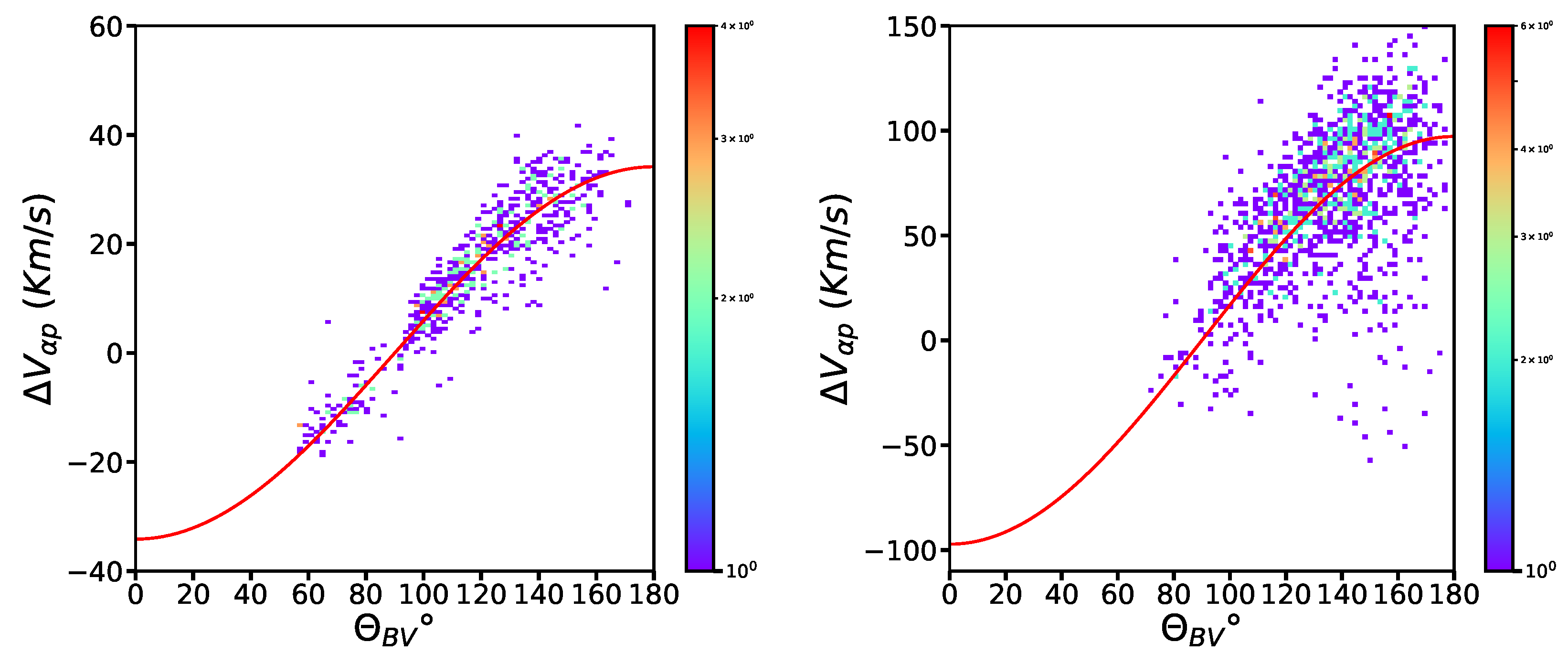
3. Results
Model Fitting
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Robbins, D.E.; Hundhausen, A.J.; Bame, S.J. Helium in the solar wind. J. Geophys. Res. 1970, 75, 1178. [Google Scholar] [CrossRef]
- Bame, S.J.; Asbridge, J.R.; Feldman, W.C.; Felthauser, H.E.; Gosling, J.T. A search for a general gradient in the solar wind speed at low solar latitudes. J. Geophys. Res. 1977, 82, 173. [Google Scholar] [CrossRef]
- Kasper, J.C.; Stevens, M.L.; Lazarus, A.J.; Steinberg, J.T.; Ogilvie, K.W. Solar Wind Helium Abundance as a Function of Speed and Heliographic Latitude: Variation through a Solar Cycle. Astrophys. J. 2007, 660, 901–910. [Google Scholar] [CrossRef]
- Ebert, R.W.; McComas, D.J.; Elliott, H.A.; Forsyth, R.J.; Gosling, J.T. Bulk properties of the slow and fast solar wind and interplanetary coronal mass ejections measured by Ulysses: Three polar orbits of observations. J. Geophys. Res. Space Phys. 2009, 114, A01109. [Google Scholar] [CrossRef]
- Kasper, J.C.; Abiad, R.; Austin, G.; Balat-Pichelin, M.; Bale, S.D.; Belcher, J.W.; Berg, P.; Bergner, H.; Berthomier, M.; Bookbinder, J.; et al. Solar Wind Electrons Alphas and Protons (SWEAP) Investigation: Design of the Solar Wind and Coronal Plasma Instrument Suite for Solar Probe Plus. Space Sci. Rev. 2016, 204, 131–186. [Google Scholar] [CrossRef]
- Ogilvie, K.W. Differences between the bulk speeds of hydrogen and helium in the solar wind. J. Geophys. Res. 1975, 80, 1335. [Google Scholar] [CrossRef]
- Asbridge, J.R.; Bame, S.J.; Feldman, W.C.; Montgomery, M.D. Helium and hydrogen velocity differences in the solar wind. J. Geophys. Res. 1976, 81, 2719. [Google Scholar] [CrossRef]
- Grünwaldt, H.; Rosenbauer, H. Study of Helium and Hydrogen Velocity Differences as derived from Heos-2 S-210 Solar Wind Measurements. In Pleins Feux sur la Physique Solaire; Dumont, S., Roesch, J., Eds.; Centre National de la Recherche Scientifique: Paris, France, 1978; p. 377. [Google Scholar]
- Marsch, E.; Rosenbauer, H.; Schwenn, R.; Muehlhaeuser, K.H.; Neubauer, F.M. Solar wind helium ions: Obsevations of the Helios solar probes between 0.3 and 1 AU. J. Geophys. Res. 1982, 87, 35–51. [Google Scholar] [CrossRef]
- Durovcová, T.; Šafránková, J.; Němeček, Z. Evolution of Relative Drifts in the Expanding Solar Wind: Helios Observations. Sol. Phys. 2019, 294, 97. [Google Scholar] [CrossRef]
- Berger, L.; Wimmer-Schweingruber, R.F.; Gloeckler, G. Systematic Measurements of Ion-Proton Differential Streaming in the Solar Wind. Phys. Rev. Lett. 2011, 106, 151103. [Google Scholar] [CrossRef]
- Ogilvie, K.W.; Chornay, D.J.; Fritzenreiter, R.J.; Hunsaker, F.; Keller, J.; Lobell, J.; Miller, G.; Scudder, J.D.; Sittler, E.C.; Torbert, R.B.; et al. SWE, A Comprehensive Plasma Instrument for the Wind Spacecraft. Space Sci. Rev. 1995, 71, 55–77. [Google Scholar] [CrossRef]
- Lepping, R.P.; Acũna, M.H.; Burlaga, L.F.; Farrell, W.M.; Slavin, J.A.; Schatten, K.H.; Mariani, F.; Ness, N.F.; Neubauer, F.M.; Whang, Y.C.; et al. The Wind Magnetic Field Investigation. Space Sci. Rev. 1995, 71, 207–229. [Google Scholar] [CrossRef]
- Schwenn, R.; Rosenbauer, H.; Miggenrieder, H. Das Plasmaexperiment auf Helios (E1). Raumfahrtforschung 1975, 19, 226–232. [Google Scholar]
- Musmann, G.; Neubauer, F.M.; Maier, A.; Lammers, E. The Foerstersonden magnetic field experiment /E 2/. Raumfahrtforschung 1975, 19, 232–237. [Google Scholar]
- Scearce, C.; Cantarano, S.; Ness, N.; Mariani, F.; Terenzi, R.; Burlaga, L. The Rome-GSFC magnetic field experiment for Helios A and B (E3). Raumfahrtforschung 1975, 19, 237–240. [Google Scholar]
- Stansby, D.; Salem, C.; Matteini, L.; Horbury, T. A New Inner Heliosphere Proton Parameter Dataset from the Helios Mission. Sol. Phys. 2018, 293, 155. [Google Scholar] [CrossRef]
- Stansby, D.; Perrone, D.; Matteini, L.; Horbury, T.S.; Salem, C.S. Alpha particle thermodynamics in the inner heliosphere fast solar wind. Astron. Astrophys. 2019, 623, L2. [Google Scholar] [CrossRef]
- Matteini, L.; Horbury, T.S.; Neugebauer, M.; Goldstein, B.E. Dependence of solar wind speed on the local magnetic field orientation: Role of Alfvénic fluctuations. Geophys. Res. Lett. 2014, 41, 259–265. [Google Scholar] [CrossRef]
- Matteini, L.; Horbury, T.S.; Pantellini, F.; Velli, M.; Schwartz, S.J. Ion Kinetic Energy Conservation and Magnetic Field Strength Constancy in Multi-fluid Solar Wind Alfvénic Turbulence. Astrophys. J. 2015, 802, 11. [Google Scholar] [CrossRef]
- Goldstein, B.E.; Neugebauer, M.; Smith, E.J. Alfvén waves, alpha particles, and pickup ions in the solar wind. Geophys. Res. Lett. 1995, 22, 3389–3392. [Google Scholar] [CrossRef]
- Kohl, J.L.; Noci, G.; Antonucci, E.; Tondello, G.; Huber, M.C.E.; Cranmer, S.R.; Strachan, L.; Panasyuk, A.V.; Gardner, L.D.; Romoli, M.; et al. UVCS/SOHO Empirical Determinations of Anisotropic Velocity Distributions in the Solar Corona. Astrophys. J. 1998, 501, L127–L131. [Google Scholar] [CrossRef]
- Li, X.; Habbal, S.R.; Kohl, J.L.; Noci, G. The Effect of Temperature Anisotropy on Observations of Doppler Dimming and Pumping in the Inner Corona. Astrophys. J. 1998, 501, L133–L137. [Google Scholar] [CrossRef]
- Cranmer, S.R.; Kohl, J.L.; Noci, G.; Antonucci, E.; Tondello, G.; Huber, M.C.E.; Strachan, L.; Panasyuk, A.V.; Gardner, L.D.; Romoli, M.; et al. An Empirical Model of a Polar Coronal Hole at Solar Minimum. Astrophys. J. 1999, 511, 481–501. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jagarlamudi, V.K.; Bruno, R.; De Marco, R.; D’Amicis, R.; Perrone, D.; Telloni, D.; Raouafi, N.E. Investigation of Alpha-Proton Drift Speeds in the Solar Wind: WIND and HELIOS Observations. Universe 2023, 9, 21. https://doi.org/10.3390/universe9010021
Jagarlamudi VK, Bruno R, De Marco R, D’Amicis R, Perrone D, Telloni D, Raouafi NE. Investigation of Alpha-Proton Drift Speeds in the Solar Wind: WIND and HELIOS Observations. Universe. 2023; 9(1):21. https://doi.org/10.3390/universe9010021
Chicago/Turabian StyleJagarlamudi, Vamsee Krishna, Roberto Bruno, Rossana De Marco, Raffaella D’Amicis, Denise Perrone, Daniele Telloni, and Nour E. Raouafi. 2023. "Investigation of Alpha-Proton Drift Speeds in the Solar Wind: WIND and HELIOS Observations" Universe 9, no. 1: 21. https://doi.org/10.3390/universe9010021
APA StyleJagarlamudi, V. K., Bruno, R., De Marco, R., D’Amicis, R., Perrone, D., Telloni, D., & Raouafi, N. E. (2023). Investigation of Alpha-Proton Drift Speeds in the Solar Wind: WIND and HELIOS Observations. Universe, 9(1), 21. https://doi.org/10.3390/universe9010021





