Quantum Non-Locality and the CMB: What Experiments Say
Abstract
1. Introduction
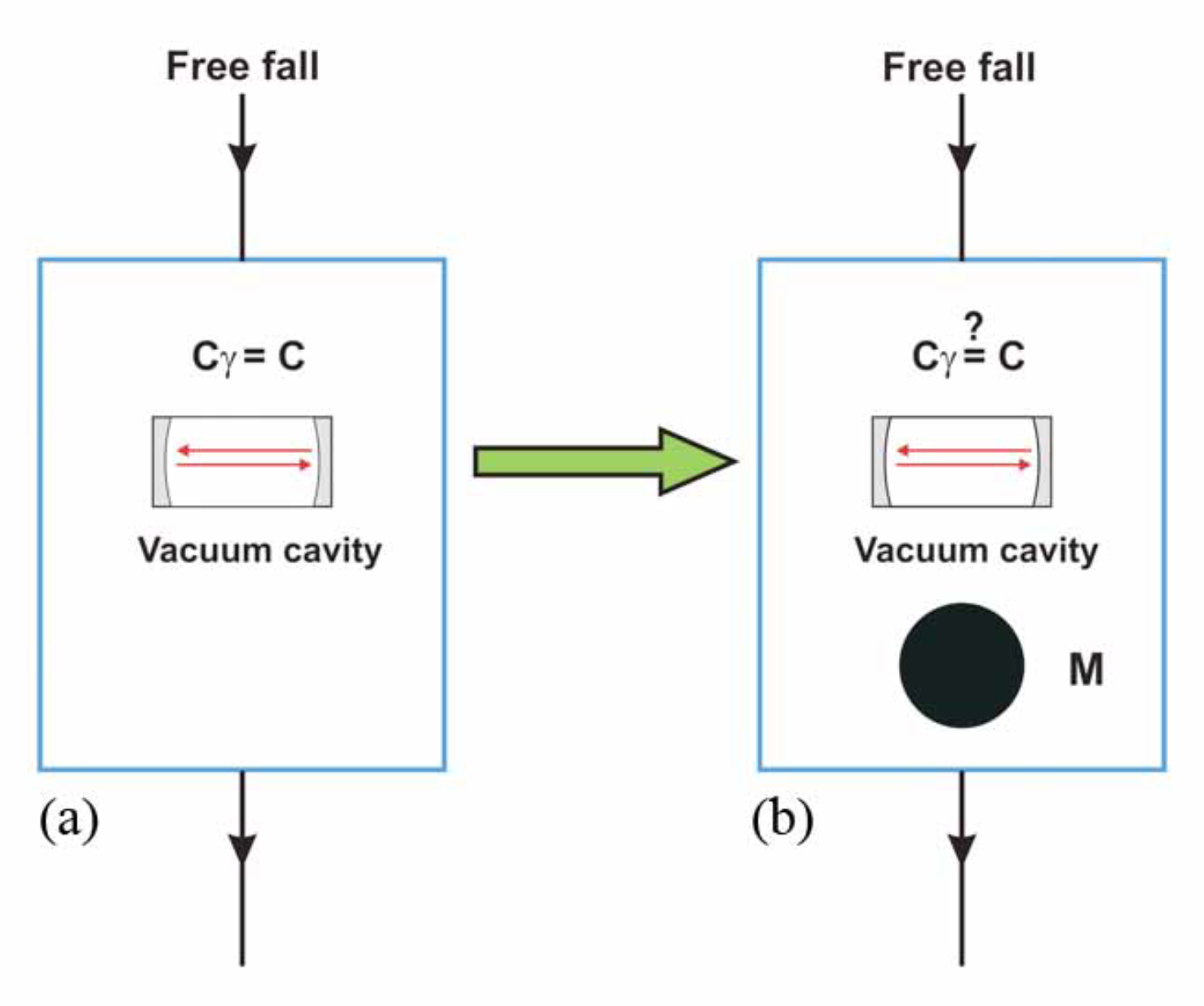
2. Vacuum State and Its Lorentz Invariance
3. The Basics of the Ether Drift Experiments
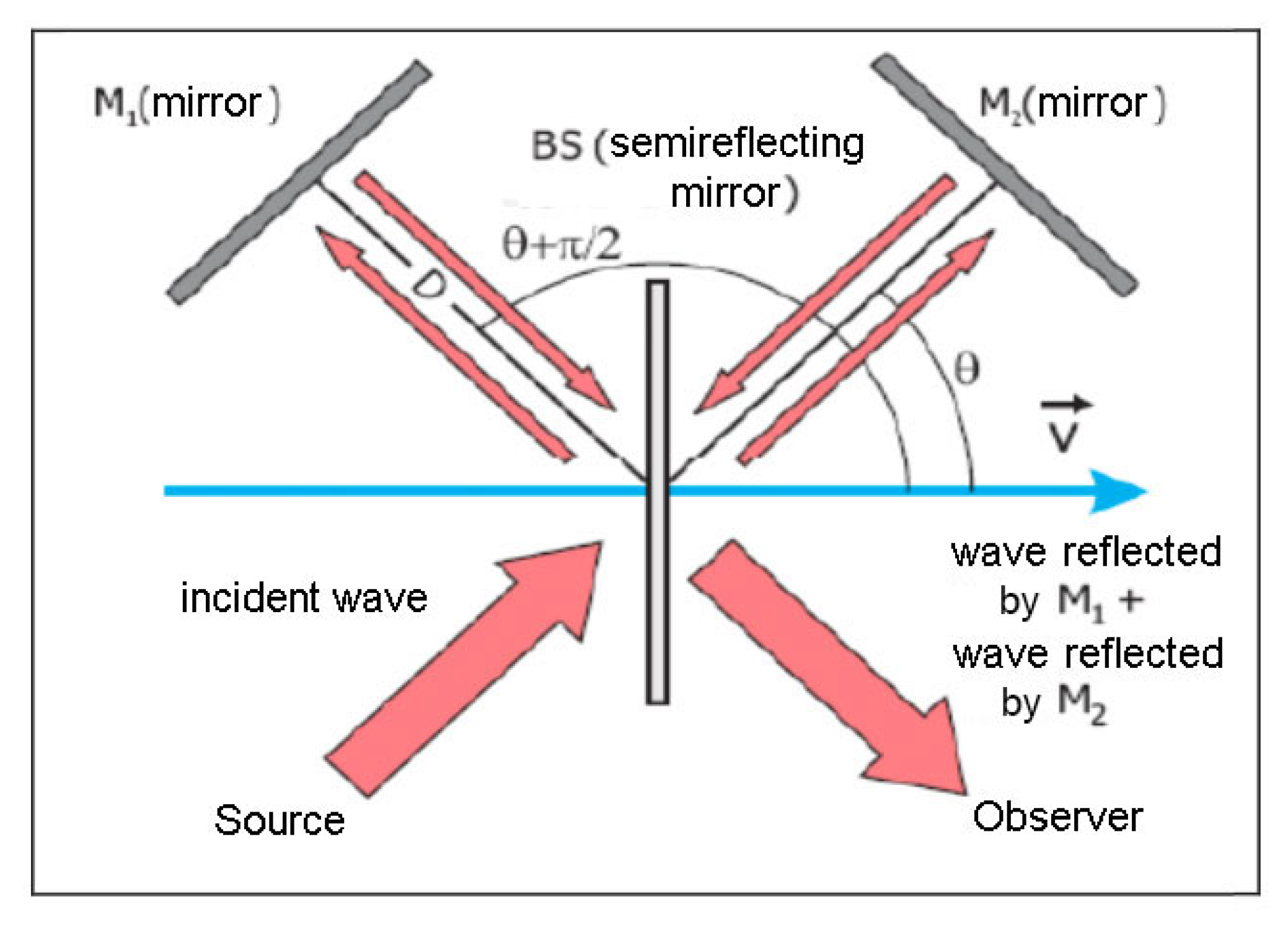
3.1. Which Preferred Frame?
3.2. The Old Experiments in Gaseous Media
3.3. The Modern Experiments in Vacuum and Solid Dielectrics
4. Summary and Outlook
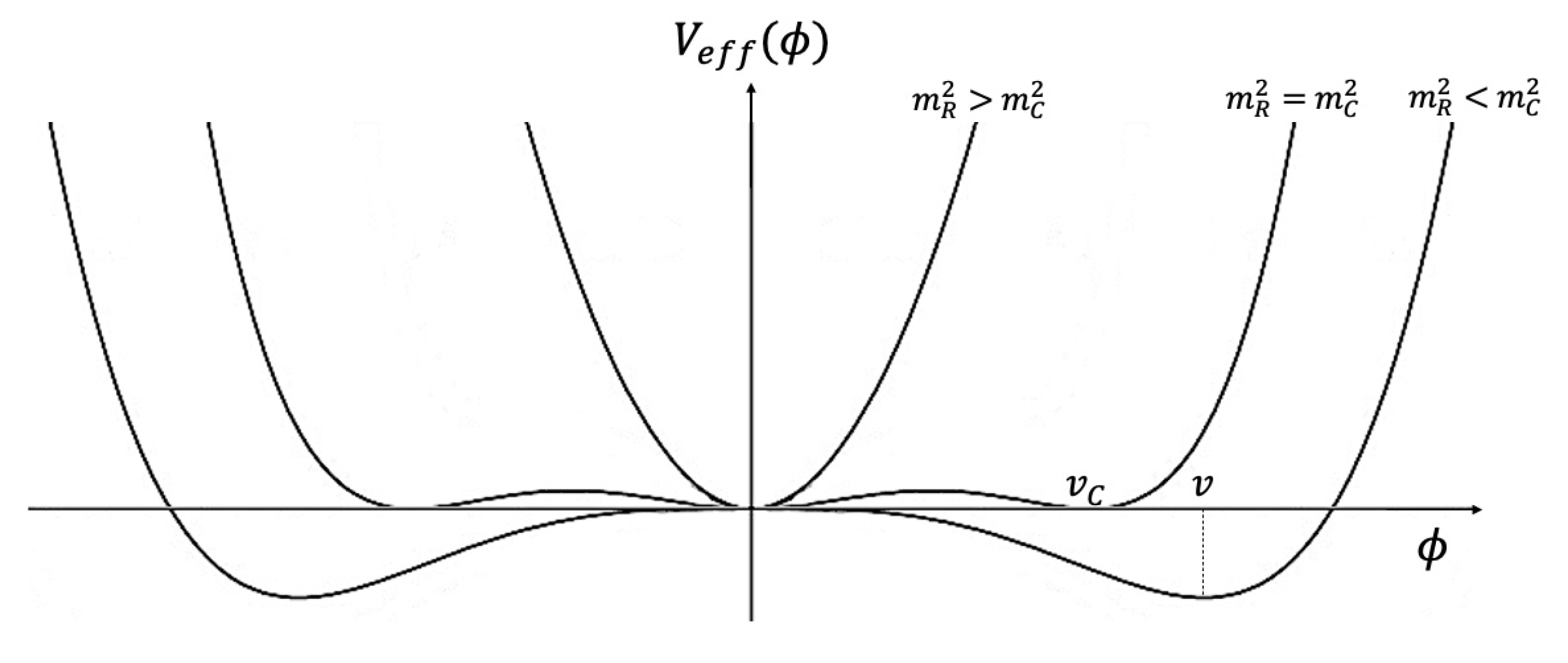
Author Contributions
Funding
Conflicts of Interest
Appendix A
| 1 | According to Weinberg, “It is a bad sign that those physicists today who are most comfortable with quantum mechanics do not agree with one another about what it all means” [1]; or, according to Blanchard, Frohlich and Schubnel, “Given that quantum mechanics was discovered ninety years ago, the present rather low level of understanding of its deeper meaning may be seen to represent some kind of intellectual scandal” [2]. |
| 2 | “The impossibility of sending messages is sometimes taken to mean that there is nothing non-local going on. But non-locality refers here to causal interactions as described (in principle) by physical theories. Messages are far more anthropocentric than that, and require that humans be able to control these interactions in order to communicate. As remarked by Maudlin [8], the Big Bang and earthquakes cannot be used to send messages, but they have causal effects nevertheless” [7]. |
| 3 | The system where the CMB Kinematic Dipole [18] vanishes describes a motion of the solar system with average velocity km/s, right ascension and declination , approximately pointing toward the constellation Leo. |
| 4 | This reductive interpretation of Bell’s work is contested by Bricmont [25]. Spelling out precisely the meaning of Bell’s theorem, he is very explicit on this point: “Bell’s result, combined with the EPR argument, is rather that there are non-local physical effects (and not just correlations between distant events) in Nature”. |
| 5 | |
| 6 | In connection with the idea of ether, it should be better underlined that Einstein’s original point of view had been later reconsidered with the transition from Special Relativity to General Relativity [28]. Most probably, he realized that Riemannian geometry is also the natural framework to describe the dynamics of elastic media; see, e.g., [29]. |
| 7 | After these arguments, Chiao immediately adds the usual remark about the impossibility of information propagating at superluminal speed: “Relativistic causality forbids only the front velocity, i.e., the velocity of discontinuities, which connects causes to their effects, from exceeding the speed of light, but does not forbid a wave packet group velocity from being superluminal” [30]. |
| 8 | The presence of the cubic interaction should not be overlooked. In fact, in the infrared region, it induces a strong coupling between bare and components in the Fock space of the broken-symmetry phase [50]. The net result is that, in the limit, the effective 1-(quasi)particle spectrum deviates sizeably from the spectrum of the bare states. |
| 9 | One may object that this conflict is merely a consequence of describing SSB as a (weak) first-order phase transition. Apparently, in the standard second-order picture, where no meaningful quantization in the symmetric phase is possible, there would be no such problem. However, this is illusory. In fact, as previously recalled, the same (weak) first-order scenario is found, within the conventional loop expansion [37], when studying SSB in the more realistic case of complex scalar fields interacting with gauge bosons. This first-order scenario is at the base of ’t Hooft’s description [26] of the physical vacuum as a Bose condensate of real, physical Higgs quanta (not of tachions with imaginary mass). Thus, the problem goes beyond the simplest model considered here and reflects the general constraint imposed by SSB on the renormalized mass parameter of the scalar fields in the symmetric phase. |
| 10 | being a space–time-independent constant. In fact, from
If is an eigenstate of the Hamiltonian, then an eigenvalue is needed to obtain . Instead, Equation (40) amounts to . Thus, it is no surprise that one can run into contradictory statements. |
| 11 | By extending the Poincaré algebra, a remarkable case, which fulfills the zero-energy condition exactly, is that of an unbroken supersymmetric theory. This is because the Hamiltonian is bilinear in the supersymmetry generators . Therefore, an exact supersymmetric state, for which , has automatically zero energy. At present, however, an unbroken supersymmetry is not phenomenologically acceptable. |
| 12 | |
| 13 | A conceptual detail concerns the relation of the gas refractive index , as introduced in Equation (1), to the experimental quantity , which is extracted from measurements of the two-way velocity in the Earth laboratory. By assuming a dependent refractive index as in Equation (48), one should thus define by an angular average, i.e., . One can then determine the unknown value (as if the container of the gas were at rest in ), in terms of the experimentally known quantity and of v. As discussed in refs. [32,33,34,35], for km/s, the difference in the two quantities is well below the experimental accuracy and, for all practical purposes, can be neglected. |
| 14 | The idea of the physical vacuum as an underlying stochastic medium, similar to a turbulent fluid, is deeply rooted in basic foundational aspects of both quantum physics and relativity. For instance, at the end of the XIX century, the last model of the ether was a fluid full of very small whirlpools (a “vortex-sponge”) [72]. The hydrodynamics of this medium was accounting for Maxwell’s equations and thus providing a model of Lorentz symmetry as emerging from a system whose elementary constituents are governed by Newtonian dynamics. More recently, the turbulent ether model has been re-formulated by Troshkin [73] (see also [74,75]) within the Navier–Stokes equation, by Saul [76] by starting from Boltzmann’s transport equation and in [77] within Landau’s hydrodynamics. The same picture of the vacuum (or ether) as a turbulent fluid was Nelson’s [78] starting point. In particular, the zero-viscosity limit gave him the motivation to expect that “the Brownian motion in the ether will not be smooth” and, therefore, to conceive the particular form of kinematics at the base of his stochastic derivation of the Schrodinger equation. A qualitatively similar picture is also obtained by representing relativistic particle propagation from the superposition, at short time scales, of non-relativistic particle paths with different Newtonian mass [79]. In this formulation, particles randomly propagate (as in a Brownian motion) in an underlying granular medium, which replaces the trivial empty vacuum [80]. |
| 15 | In Ref. [34], a numerical simulation of the Piccard–Stahel experiment [63] is reported, for both the individual sets of 10 rotations of the interferometer and the experimental sessions (12 sets, each set consisting of 10 rotations). Our analysis confirms their idea that the optical path was much shorter than the instruments in the United States but their measurements were more precise because spurious disturbances were less important. |
| 16 | Joos’ optical system was enclosed in a hermetic housing and, as reported by Miller [59,88], measurements were performed in a partial vacuum. In his article, however, Joos is not clear on this particular aspect. Only when describing his device for electromagnetic fine movements of the mirrors, he refers to the condition of an evacuated apparatus [67]. Instead, Swenson [89,90] declares that Joos’ fringe shifts were finally recorded with optical paths placed in a helium bath. Therefore, we have followed Swenson’s explicit statements and assumed the presence of gaseous helium at atmospheric pressure. |
| 17 | |
| 18 | The RAV gives the variation of a function sampled over steps of time . By defining
The integration time is given in seconds and the factor of 2 is introduced to obtain the same standard variance for uncorrelated data as for a white-noise signal with uniform spectral amplitude at all frequencies. |
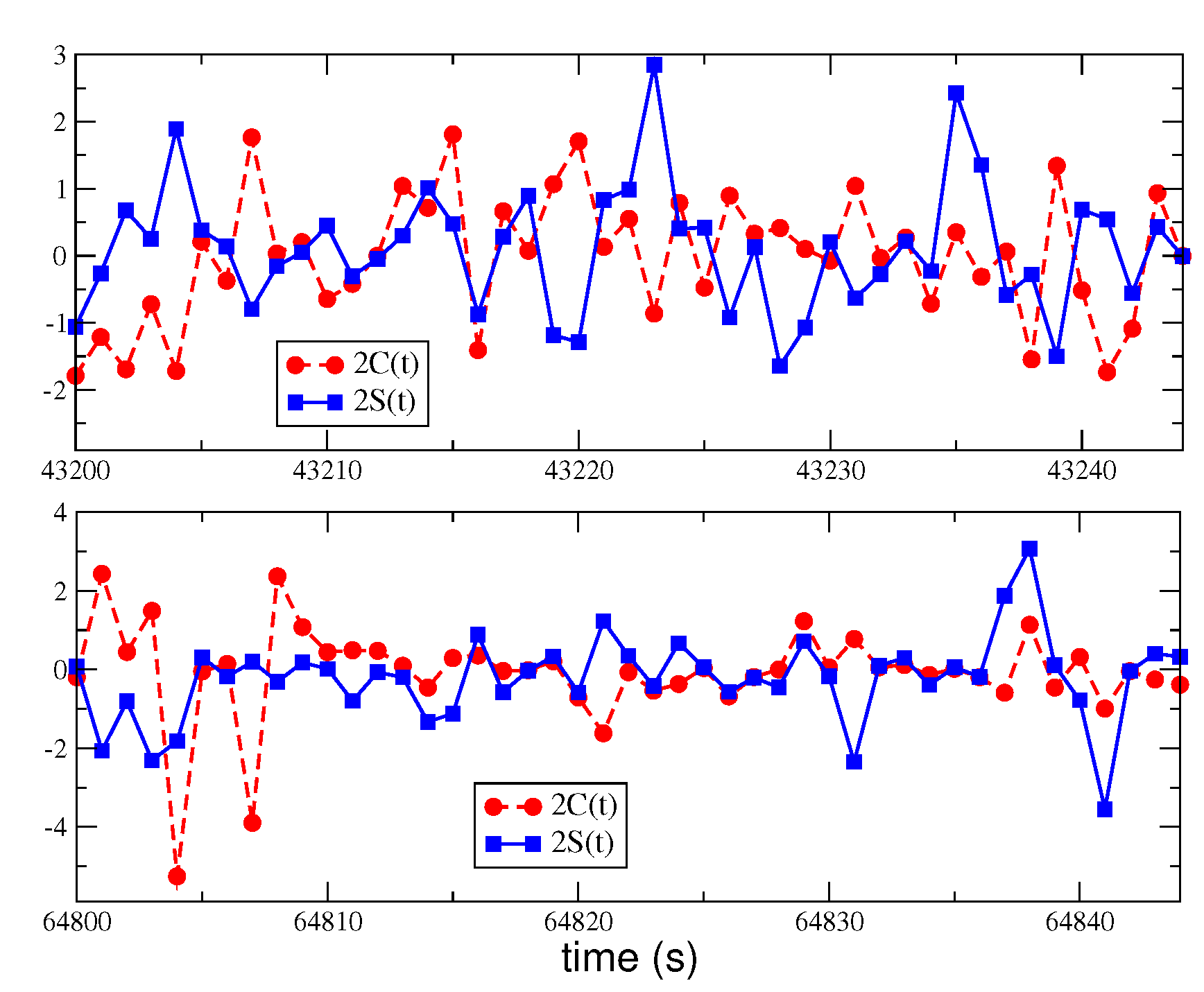
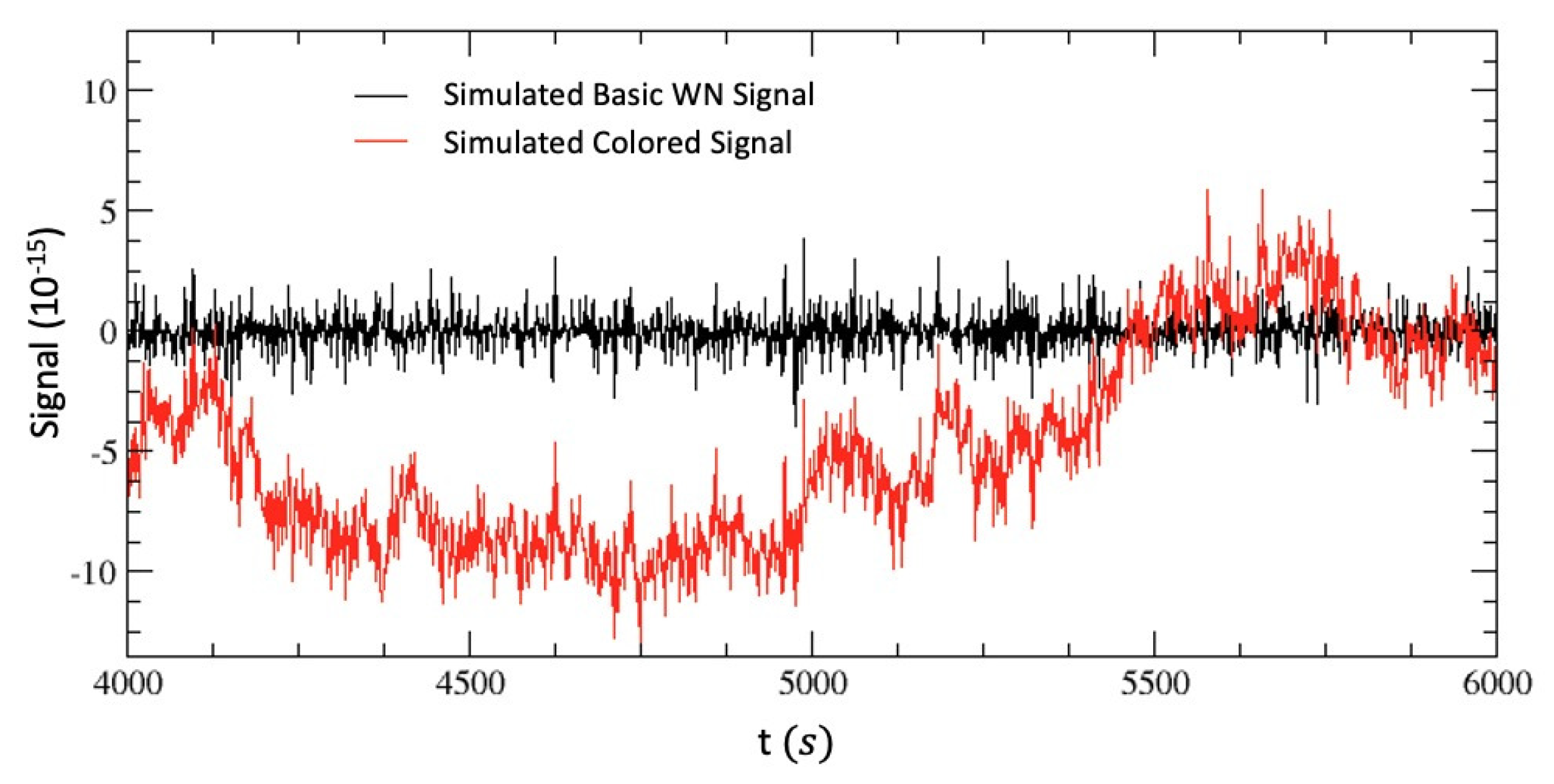
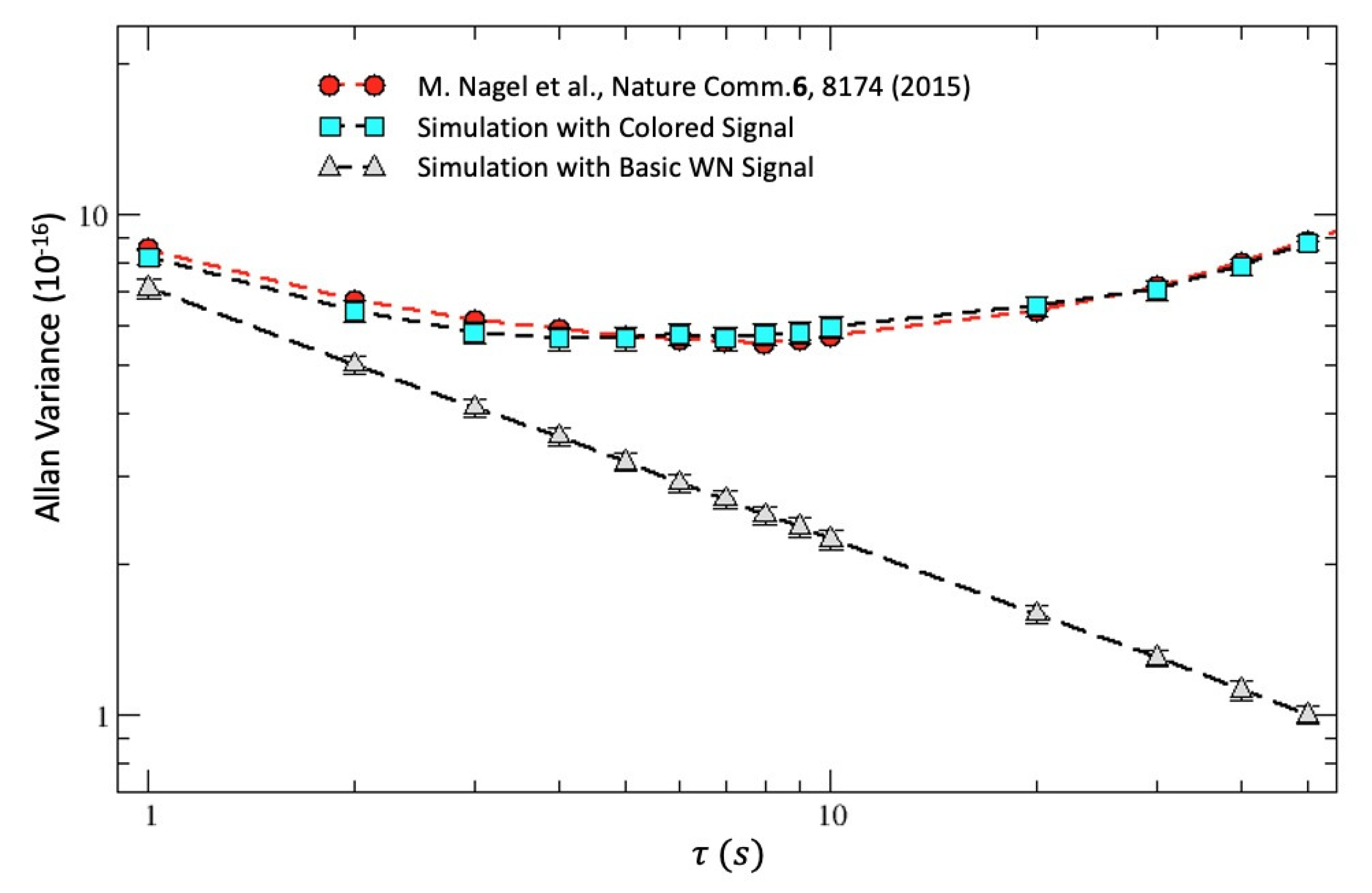
| 19 | Numerical simulations indicate that our vacuum signal has the same characteristics of universal white noise. Thus, strictly speaking, it should be compared with the frequency shift of two optical resonators at the largest integration time , where the pure white-noise branch is as small as possible but other types of noise are not yet important. In the experiments, we are presently considering this as typically 1 s. However, in principle, could also be considerably larger than 1 s, as, for instance, in the cryogenic experiment of Ref. [97]. There, the RAV at 1 s was around 10 times larger than the range of Equation (63) but, in the quiet phases between two refills of the refrigerator, was monotonically following the white-noise trend up to s, where it reached its minimum value . Remarkably, for , this is still consistent with the theoretical range of Equation (63). |
References
- Weinberg, S. The Trouble with Quantum Mechanics. The New York Review of Books, 19 January 2017. [Google Scholar]
- Blanchard, P.; Frohlich, J.; Schubnel, B. A “garden of forking paths”—The quantum mechanics of histories of events. Nucl. Phys. B 2016, 912, 463. [Google Scholar] [CrossRef]
- Einstein, A.; Podolski, B.; Rosen, N. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 1935, 47, 777. [Google Scholar] [CrossRef]
- Full reference to all papers by J. S. Bell, can be found in the Volume Collection; Speakable and Unspeakable in Quantum Mechanics, 2nd ed.; Cambridge University Press: Cambridge, UK, 2004.
- Stapp, H.P. A Bell-type theorem without hidden variables. Am. J. Phys. 2004, 72, 30. [Google Scholar] [CrossRef]
- Shimony, A. An Analysis of Stapp’s “A Bell-type theorem without hidden variables”. Found. Phys. 2006, 36, 61–72. [Google Scholar] [CrossRef]
- Bricmont, J. What Did Bell Really Prove? In Quantum NonLocality and Reality, 50 Years of Bell’s Theorem; Bell, M., Gao, S., Eds.; Cambridge University Press: Cambridge, UK, 2016; p. 49. [Google Scholar]
- Maudlin, T. Quantum Non-Locality and Relativity; Blackwell: Cambridge, UK, 2011. [Google Scholar]
- Dirac, P.A.M. Development of the Physicist’s Conception of Nature. In The Physicist’s Conception of Nature; Mehra, J., Ed.; Reidel: Boston, MA, USA, 1973. [Google Scholar]
- Weinberg, S. Gravitation and Cosmology; John Wiley and Sons, Inc.: Hoboken, NJ, USA, 1972; p. 52. [Google Scholar]
- Bohm, D.; Hiley, B. The Undivided Universe; Routledge: London, UK, 1993. [Google Scholar]
- Hardy, L. Quantum mechanics, local realistic theories, and Lorentz-invariant realistic theories. Phys. Rev. Lett. 1992, 68, 2981. [Google Scholar] [CrossRef]
- Caban, P.; Rembielinski, J. Lorentz-covariant quantum mechanics and preferred frame. Phys. Rev. A 1999, 59, 4187. [Google Scholar] [CrossRef]
- Liberati, S.; Sonego, S.; Visser, M. Faster-than-c signals, special relativity, and causality. Ann. Phys. 2002, 298, 167. [Google Scholar] [CrossRef]
- Eberhard, P.H. Bell’s Theorem and the Different Concept of Locality. Nuovo C. B 1978, 46, 392. [Google Scholar] [CrossRef]
- Eberhard, P.H. A realistic model for Quantum Theory with a locality property. In Quantum Theories and Pictures of Reality; Schommers, W., Ed.; Springer: Berlin/Heidelberg, Germany, 1989; p. 169. [Google Scholar]
- Garisto, R. What is the speed of quantum information? arXiv 2002, arXiv:quant-ph/0212078. [Google Scholar]
- Yoon, M.; Huterer, D. Kinematic Dipole Detection With Galaxy Surveys: Forecasts And Requirements. Astrophys. J. Lett. 2015, 813, L18. [Google Scholar] [CrossRef]
- Scarani, V.; Tittel, W.; Zbinden, H.; Gisin, N. The speed of quantum information and the preferred frame: Analysis of experimental data. Phys. Lett. A 2000, 276, 1. [Google Scholar] [CrossRef]
- Cocciaro, B.; Faetti, S.; Fronzoni, L. A lower bound for the velocity of quantum communication in the preferred frame. Phys. Lett. A 2011, 375, 379. [Google Scholar] [CrossRef]
- Salart, D.; Baas, A.; Branciard, C.; Gisin, N.; Zbinden, H. Testing spooky action at a distance. Nature 2008, 454, 861. [Google Scholar] [CrossRef] [PubMed]
- Bancal, J.-D.; Pironio, S.; Acin, A.; Liang, Y.-C.; Scarani, V.; Gisin, N. Quantum nonlocality based on finite-speed causal influences leads to superluminal signaling. Nat. Phys. 2012, 8, 867. [Google Scholar] [CrossRef]
- Cocciaro, B.; Faetti, S.; Fronzoni, L. Fast measurements of entanglement over a kilometric distance to test superluminal models of Quantum Mechanics: Final results. J. Phys. Conf. Ser. 2019, 1275, 012035. [Google Scholar] [CrossRef]
- Maiani, L.; Testa, M. Causality in quantum field theory. Phys. Lett. B 1995, 356, 319. [Google Scholar] [CrossRef]
- Bricmont, J. Making Sense of Quantum Mechanics; Springer International Publ.: Cham, Switzerland, 2016. [Google Scholar]
- Hooft, G. Search of the Ultimate Building Blocks; Cambridge University Press: Cambridge, UK, 1997; p. 70. [Google Scholar]
- Consoli, M.; Stevenson, P.M. Physical mechanisms generating spontaneous symmetry breaking and a hierarchy of scales. Int. J. Mod. Phys. A 2000, 15, 133. [Google Scholar] [CrossRef]
- Kostro, L. Einstein and the Ether; Italian translation, Ed.; Dedalo: Bari, Italy, 2001. [Google Scholar]
- Sommerfeld, A. Mechanics of Deformable Bodies; Academic Press: New York, NY, USA, 1950. [Google Scholar]
- Chiao, R.Y. Conceptual tensions between quantum mechanics and general relativity: Are there experimental consequences. In “Science and Ultimate Reality: From Quantum to Cosmos”, Honoring John Wheeler’s 90th Birthday; Barrow, J.D., Davies, P.C.W., Harper, C.L., Eds.; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Jauch, J.M.; Watson, K.M. Phenomenological Quantum-Electrodynamics. Phys. Rev. 1948, 74, 950. [Google Scholar] [CrossRef]
- Consoli, M.; Matheson, C.; Pluchino, A. The classical ether-drift experiments: A modern re-interpretation. Eur. Phys. J. Plus 2013, 128, 71. [Google Scholar] [CrossRef][Green Version]
- Consoli, M.; Pluchino, A. Cosmic Microwave Background and the issue of a fundamental preferred frame. Eur. Phys. J. Plus 2018, 133, 295. [Google Scholar] [CrossRef]
- Consoli, M.; Pluchino, A. Michelson-Morley Experiments: An Enigma for Physics and the History of Science; World Scientific Publishing Co., Pte Ltd.: Singapore, 2019; ISBN 978-981-3278-18-9. [Google Scholar]
- Consoli, M.; Pluchino, A. CMB, preferred reference system and dragging of light in the earth’s frame. Universe 2021, 7, 311. [Google Scholar] [CrossRef]
- Consoli, M.; Pluchino, A.; Rapisarda, A. Cosmic Background Radiation and ‘ether-drift’ experiments. Europhys. Lett. 2016, 113, 19001. [Google Scholar] [CrossRef][Green Version]
- Coleman, S.R.; Weinberg, E.J. Radiative Corrections as the Origin of Spontaneous Symmetry Breaking. Phys. Rev. D 1973, 7, 1888. [Google Scholar] [CrossRef]
- Lundow, P.H.; Markstrom, K. Critical behavior of the Ising model on the four-dimensional cubic lattice. Phys. Rev. E 2009, 80, 031104. [Google Scholar] [CrossRef]
- Lundow, P.H.; Markstrom, K. Non-vanishing boundary effects and quasi-first order phase transitions in high dimensional Ising models. Nucl. Phys. B 2011, 845, 120. [Google Scholar] [CrossRef]
- Akiyama, S.; Kuramashi, Y.; Yamashita, T.; Yoshimura, Y. Phase transition of four-dimensional Ising model with higher-order tensor renormalization group. Phys. Rev. D 2019, 100, 054510. [Google Scholar] [CrossRef]
- Streater, R.F.; Wightman, A.S. PCT, Spin and Statistics, and All That; W. A. Benjamin: New York, NY, USA, 1964. [Google Scholar]
- Segal, I.E. Is the Physical Vacuum Really Lorentz-Invariant. In Differential Geometry, Group Representations, and Quantization; Lecture Notes in Physics; Hennig, J.-D., Lücke, W., Tolar, J., Eds.; Springer: Berlin/Heidelberg, Germany, 1991; Volume 379. [Google Scholar]
- Stefanovich, E.V. Is Minkowski Space-Time Compatible with Quantum Mechanics? Found. Phys. 2002, 32, 673. [Google Scholar] [CrossRef]
- Glazek, S.D.; Maslowski, T. Renormalized Poincaré algebra for effective particles in quantum field theory. Phys. Rev. D 2002, 65, 065011. [Google Scholar] [CrossRef]
- Consoli, M.; Ciancitto, A. Indications of the occurrence of spontaneous symmetry breaking in massless λΦ4 theory. Nucl. Phys. B 1985, 254, 653. [Google Scholar] [CrossRef][Green Version]
- Weinberg, S. The Quantum Theory of Fields; Cambridge University Press: Cambridge, UK, 1995; Volume II, pp. 163–167. [Google Scholar]
- Consoli, M.; Costanzo, E. Is the physical vacuum a preferred frame? Eur. Phys. J. C 2008, 54, 285. [Google Scholar] [CrossRef]
- Consoli, M.; Costanzo, E. Precision tests with a new class of dedicated ether-drift experiments. Eur. Phys. J. C 2008, 55, 469. [Google Scholar] [CrossRef][Green Version]
- Consoli, M. Probing the vacuum of particle physics with precise laser interferometry. Found. Phys. 2015, 45, 22. [Google Scholar] [CrossRef]
- Consoli, M. On the low-energy spectrum of spontaneously broken Φ4 theories. Mod. Phys. Lett. A 2011, 26, 531. [Google Scholar] [CrossRef]
- Zeldovich, Y.B. The Cosmological constant and the theory of elementary particles. Sov. Phys. Uspekhi 1968, 11, 381. [Google Scholar] [CrossRef]
- Weinberg, S. The cosmological constant problem. Rev. Mod. Phys. 1989, 61, 1. [Google Scholar] [CrossRef]
- Smoot, G.F. Cosmic microwave background radiation anisotropies: Their discovery and utilization. Nobel Lect. Rev. Mod. Phys. 2007, 79, 1349. [Google Scholar] [CrossRef]
- Ungar, A. The relativistic composite-velocity reciprocity principle. Found. Phys. 2000, 30, 331. [Google Scholar] [CrossRef]
- Costella, J.P.; McKellar, B.H.; Rawlinson, A.A.; Stephenson, G.J., Jr. The Thomas rotation. Am. J. Phys. 2001, 69, 837. [Google Scholar] [CrossRef]
- O’Donnell, K.; Visser, M. Elementary analysis of the special relativistic combination of velocities, Wigner rotation, and Thomas precession. Eur. J. Phys. 2011, 32, 1033. [Google Scholar] [CrossRef]
- Nagel, M.; Parker, S.R.; Kovalchuk, E.V.; Stanwix, P.L.; Hartnett, J.G.; Ivanov, E.N.; Peters, A.; Tobar, M.E. Direct terrestrial test of Lorentz symmetry in electrodynamics to 10−18. Nat. Comm. 2015, 6, 8174. [Google Scholar] [CrossRef]
- Michelson, A.A.; Morley, E.W. On the Relative Motion of the Earth and the Luminiferous Ether. Am. J. Sci. 1887, 34, 333. [Google Scholar] [CrossRef]
- Miller, D.C. The Ether-Drift Experiment and the Determination of the Absolute Motion of the Earth. Rev. Mod. Phys. 1933, 5, 203. [Google Scholar] [CrossRef]
- Michelson, A.A.; Lorentz, H.A.; Miller, D.C.; Kennedy, R.J.; Hedrick, E.R.; Epstein, P.S. Conference on the Ether-Drift Experiments. Astrophys. J. 1928, 68, 341–402. [Google Scholar] [CrossRef]
- Illingworth, K.K. A Repetition of the Michelson-Morley Experiment Using Kennedy’s Refinement. Phys. Rev. 1927, 30, 692. [Google Scholar] [CrossRef]
- Tomaschek, R. About the Michelson experiment with fixed star light. Astron. Nachrichten 1923, 219, 301, English translation. [Google Scholar] [CrossRef]
- Piccard, A.; Stahel, E. Realization of the experiment of michelson in balloon and on dry land. J. Phys. Le Radium 1928, IX, 2. [Google Scholar]
- Michelson, A.A.; Pease, F.G.; Pearson, F. Repetition of the Michelson-Morley Experiment. Nature 1929, 123, 88. [Google Scholar] [CrossRef]
- Michelson, A.A.; Pease, F.G.; Pearson, F. Repetition of the Michelson-Morley experiment. J. Opt. Soc. Am. 1929, 18, 181. [Google Scholar] [CrossRef]
- Pease, F.G. Ether-Drift Data. Publ. Astr. Soc. Pac. 1930, XLII, 197. [Google Scholar] [CrossRef]
- Joos, G. Die Jenaer Wiederholung des Michelsonversuchs. Ann. Phys. 1930, 7, 385. [Google Scholar] [CrossRef]
- Kennedy, R.J. Simplified theory of the Michelson-Morley experiment. Phys. Rev. 1935, 47, 965. [Google Scholar]
- Maxwell, J.C. Ether. In Encyclopaedia Britannica, 9th ed.; University of Chicago Press: Chicago, IL, USA, 1878. [Google Scholar]
- Consoli, M.; Pluchino, A.; Rapisarda, A. Basic randomness of nature and ether-drift experiments. Chaos, Solitons Fractals 2011, 44, 1089. [Google Scholar] [CrossRef][Green Version]
- Consoli, M.; Pluchino, A.; Rapisarda, A.; Tudisco, S. The vacuum as a form of turbulent fluid: Motivations, experiments, implications. Phys. A 2014, 394, 61. [Google Scholar] [CrossRef]
- Whittaker, E.T. A History of the Theories of Aether and Electricity; Dover Publ.: New York, NY, USA, 1989. [Google Scholar]
- Troshkin, O.V. On wave properties of an incompressible turbulent fluid. Physica A 1990, 168, 881. [Google Scholar] [CrossRef]
- Puthoff, H.E. Linearized turbulent flow as an analog model for linearized General Relativity. arXiv 2008, arXiv:0808.3401. [Google Scholar]
- Tsankov, T.D. Classical Electrodynamics and the Turbulent Aether Hypothesis. Preprint February 2009, unpublished.
- Saul, L.A. Spin Waves as Metric in a Kinetic Space-Time. Phys. Lett. A 2003, 314, 472. [Google Scholar] [CrossRef]
- Consoli, M. A kinetic basis for space-time symmetries. Phys. Lett. A 2012, 376, 3377. [Google Scholar] [CrossRef]
- Nelson, E. A derivation of the Schrodinger Equation from Newtonian Mechanics. Phys. Rev. 1966, 150, 1079. [Google Scholar] [CrossRef]
- Jizba, P.; Kleinert, H. Superstatistics approach to path integral for a relativistic particle. Phys. Rev. D 2010, 82, 085016. [Google Scholar] [CrossRef]
- Jizba, P.; Scardigli, F. Special Relativity induced by Granular Space. Eur. Phys. J. C 2013, 73, 2491. [Google Scholar] [CrossRef]
- Feynman, R.P.; Leighton, R.B.; Sands, M. The Feynman Lectures on Physics; Addison Wesley Publ. Co.: Boston, MA, USA, 1963. [Google Scholar]
- Onsager, L. Statistical hydrodynamics. Nuovo C. 1949, (Suppl. 6), 279. [Google Scholar] [CrossRef]
- Eyink, G.L.; Sreenivasan, K.R. Onsager and the theory of hydrodynamic turbulence. Rev. Mod. Phys. 2006, 78, 87. [Google Scholar] [CrossRef]
- Nassau, J.J.; Morse, P.M. A Study of Solar Motion by Harmonic Analysis. Astrophys. J. 1927, 65, 73. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Fluid Mechanics; Pergamon Press: Oxford, UK, 1959; Chaptrt III. [Google Scholar]
- Fung, J.C.H.; Hunt, J.C.R.; Malik, N.A.; Perkins, R.J. Kinematic simulation of homogeneous turbulence by unsteady random Fourier modes. J. Fluid Mech. 1992, 236, 281. [Google Scholar] [CrossRef]
- Shankland, R.S.; McCuskey, S.W.; Leone, F.C.; Kuerti, G. New Analysis of the Interferometer Observations of Dayton C. Miller. Rev. Mod. Phys. 1955, 27, 167. [Google Scholar] [CrossRef]
- Miller, D.C. Comments on Dr. Georg Joos’s Criticism of the Ether-Drift Experiment. Phys. Rev. 1934, 45, 114. [Google Scholar] [CrossRef]
- Swenson, L.S., Jr. The Ethereal Aether, A History of the Michelson-Morley-Miller Aether-Drift Experiments, 1880–1930; University of Texas Press: Austin, TX, USA, 1972. [Google Scholar]
- Swenson, L.S., Jr. The Michelson-Morley-Miller Experiments before and after 1905. J. Hist. Astron. 1970, 1, 56. [Google Scholar] [CrossRef]
- Shamir, J.; Fox, R. A New Experimental Test of Special Relativity. Nuovo C B 1969, 62, 258. [Google Scholar] [CrossRef]
- Joos, G. Note on the Repetition of the Michelson-Morley Experiment. Phys. Rev. 1934, 45, 114. [Google Scholar] [CrossRef]
- Farkas, E.R.; Webb, W.W. Precise and millidegree stable control for fluorescense imaging. Rev. Sci. Instrum. 2010, 81, 093704. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Trumper, D.L.; Heilmann, R.K.; Schattenburg, M.L. Optimizatiom and temperature mapping of an ultra-high thermal stability enviromental enclosure. Precis. Eng. 2010, 34, 164. [Google Scholar] [CrossRef]
- Prikhodko, I.P.; Trusov, A.A.; Shkel, A.M. Compensation of drifts in high-Q MEMS gyroscopes using temperature self-sensing. Sens. Actuators A 2013, 201, 517. [Google Scholar] [CrossRef]
- Muller, H.; Herrmann, S.; Braxmaier, C.; Schiller, S.; Peters, A. Precision test of the isotropy of light propagation. Appl. Phys. B 2003, 77, 719. [Google Scholar] [CrossRef]
- Muller, H.; Herrmann, S.; Braxmaier, C.; Schiller, S.; Peters, A. Modern Michelson-Morley Experiment using Cryogenic Optical Resonators. Phys. Rev. Lett. 2003, 91, 020401. [Google Scholar] [CrossRef] [PubMed]
- Eisele, C.; Okhapkin, M.; Nevsky, A.; Schiller, S. A crossed optical cavities apparatus for a precision test of the isotropy of light propagation. Opt. Commun. 2008, 281, 1189. [Google Scholar] [CrossRef]
- Herrmann, S.; Senger, A.; Mohle, K.; Nagel, M.; Kovalchuk, E.V.; Peters, A. Rotating optical cavity experiment testing Lorentz invariance at the 10−17 level. Phys. Rev. D 2009, 80, 10511. [Google Scholar] [CrossRef]
- Eisele, C.; Newsky, A.; Schiller, S. Laboratory Test of the Isotropy of Light Propagation at the 10−17 Level. Phys. Rev. Lett. 2009, 103, 090401. [Google Scholar] [CrossRef]
- Nagel, M.; Mohle, K.; Doringshoff, K.; Schikora, S.; Kovalchuk, E.V.; Peters, A. Ultra-stable Cryogenic Optical Resonators For Tests Of Fundamental Physics. arXiv 2013, arXiv:1308.5582. [Google Scholar]
- Chen, Q.; Magoulakis, E.; Schiller, S. High-sensitivity crossed-resonator laser apparatus for improved tests of Lorentz invariance and of space-time fluctuations. Phys. Rev. D 2016, 93, 022003. [Google Scholar] [CrossRef]
- Numata, K.; Kemery, A.; Camp, J. Thermal-Noise Limit in the Frequency Stabilization of Lasers with Rigid Cavities. Phys. Rev. Lett. 2004, 93, 250602. [Google Scholar] [CrossRef] [PubMed]
- Consoli, M.; Pappalardo, L. Emergent gravity and ether-drift experiments. Gen. Relativ. Gravit. 2010, 42, 2585. [Google Scholar] [CrossRef][Green Version]
- Broekaert, J. A Spatially-VSL Gravity Model with 1-PN limit of GRT. Found. Phys. 2008, 38, 409. [Google Scholar] [CrossRef][Green Version]
- Eddington, A.S. Space, Time and Gravitation; Cambridge University Press: Cambridge, UK, 1920. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. The Classical Theory of Fields; Pergamon Press: Oxford, UK, 1971; p. 257. [Google Scholar]
- Lammerzahl, C.; Dittus, H.; Peters, A.; Schiller, S. OPTIS: A satellite-based test of special and general relativity. Class. Quantum Gravity 2001, 18, 2499. [Google Scholar] [CrossRef]
- Kirzhnits, D.A.; Polyachenko, V.L. On the Possibility of Macroscopic Manifestations of Violation of Microscopic Causality. Sov. Phys. JETP 1964, 19, 514. [Google Scholar]
- Bludman, S.A.; Ruderman, M.A. Possibility of the Speed of Sound Exceeding the Speed of Light in Ultradense Matter. Phys. Rev. 1968, 170, 1176. [Google Scholar] [CrossRef]
- Ruderman, M. Causes of Sound Faster than Light in Classical Models of Ultradense Matter. Phys. Rev. 1968, 172, 1286. [Google Scholar] [CrossRef]
- Bludman, S.A.; Ruderman, M.A. Noncausality and Instability in Ultradense Matter. Phys. Rev. D 1970, 1, 3243. [Google Scholar] [CrossRef]
- Keister, B.D.; Polyzou, W.N. Causality in dense matter. Phys. Rev. C 1996, 54, 2023. [Google Scholar] [CrossRef]
- Stevenson, P.M. How do sound waves in a Bose-Einstein condensate move so fast? Phys. Rev. A 2003, 68, 055601. [Google Scholar] [CrossRef]
- Stevenson, P.M. Hydrodynamics of the vacuum. Int. J. Mod. Phys. A 2006, 21, 2877. [Google Scholar] [CrossRef]
- Stevenson, P.M. Are There Pressure Waves in the Vacuum? In Proceedings of the Second Meeting on CPT and Lorentz Symmetry, Online, 17–26 May 2022; Kostelecky, V.A., Ed.; World Scientific: Singapore, 2002. [Google Scholar]
- Zizzi, P. Quantum Holography from Fermion Fields. Quantum Rep. 2021, 3, 576–591. [Google Scholar] [CrossRef]
- Zizzi, P. Consciousness and logic in a quantum computing universe. In The Emerging Physics of Consciousness; Tuszynski, J., Ed.; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]


| Experiment | Gas | |||
|---|---|---|---|---|
| Michelson (1881) | air | |||
| Michelson–Morley (1887) | air | |||
| Morley–Miller (1902–1905) | air | |||
| Miller (1921–1926) | air | |||
| Tomaschek (1924) | air | |||
| Kennedy (1926) | helium | |||
| Illingworth (1927) | helium | |||
| Piccard–Stahel (1928) | air | |||
| Mich.–Pease–Pearson (1929) | air | |||
| Joos (1930) | helium |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Consoli, M.; Pluchino, A.; Zizzi, P. Quantum Non-Locality and the CMB: What Experiments Say. Universe 2022, 8, 481. https://doi.org/10.3390/universe8090481
Consoli M, Pluchino A, Zizzi P. Quantum Non-Locality and the CMB: What Experiments Say. Universe. 2022; 8(9):481. https://doi.org/10.3390/universe8090481
Chicago/Turabian StyleConsoli, Maurizio, Alessandro Pluchino, and Paola Zizzi. 2022. "Quantum Non-Locality and the CMB: What Experiments Say" Universe 8, no. 9: 481. https://doi.org/10.3390/universe8090481
APA StyleConsoli, M., Pluchino, A., & Zizzi, P. (2022). Quantum Non-Locality and the CMB: What Experiments Say. Universe, 8(9), 481. https://doi.org/10.3390/universe8090481









