Constraints on the Duration of Inflation from Entanglement Entropy Bounds
Abstract
:Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | |
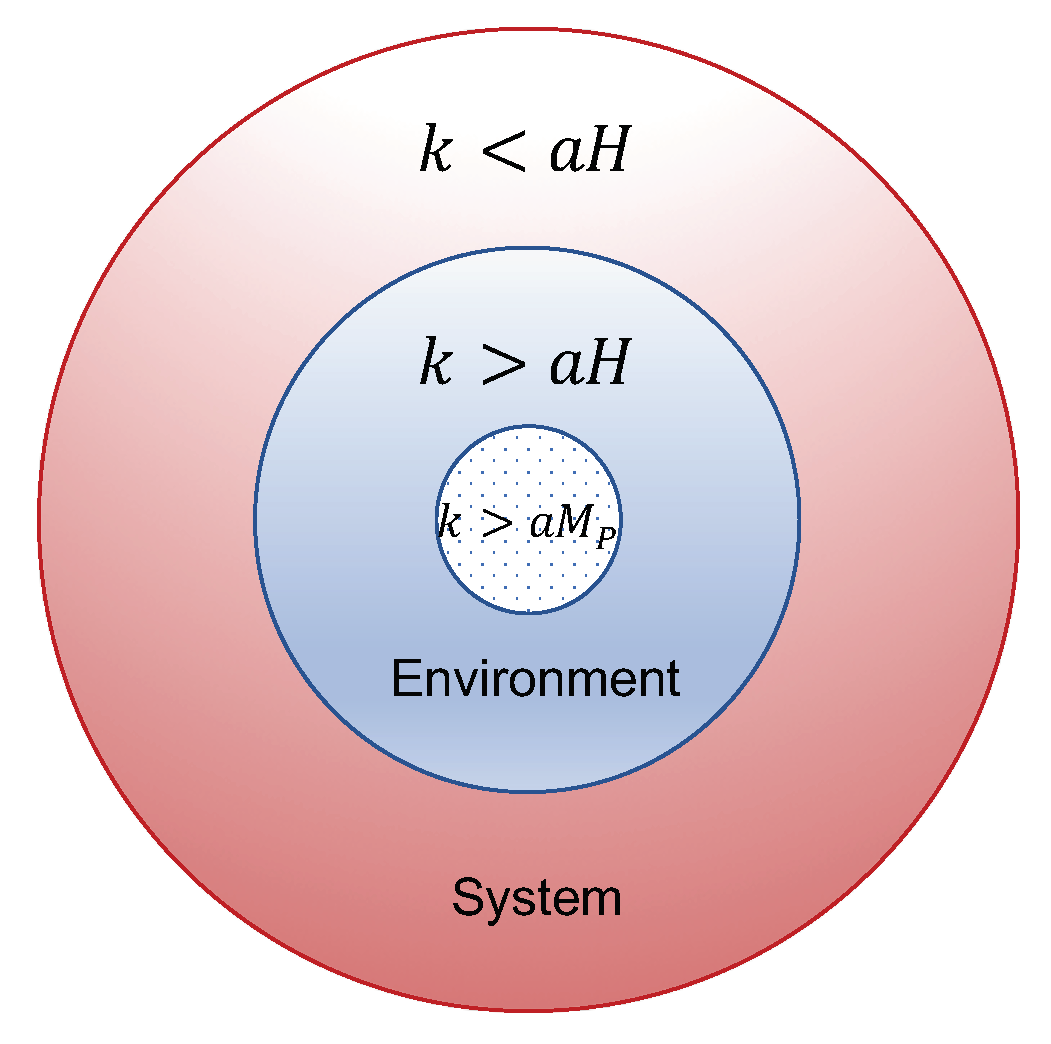
| 2 | A direct evaluation of EE in momentum space for a scalar field on pure dS, as well as a more sophisticated calculation of the inflationary system allowing for a slowly varying H and , shall be carried out in the future. |
References
- Palti, E. The swampland: Introduction and review. Fortschritte der Physik 2019, 67, 1900037. [Google Scholar] [CrossRef] [Green Version]
- Ooguri, H.; Palti, E.; Shiu, G.; Vafa, C. Distance and de Sitter Conjectures on the Swampland. Phys. Lett. B 2019, 788, 180–184. [Google Scholar] [CrossRef]
- Garg, S.K.; Krishnan, C. Bounds on slow roll and the de Sitter swampland. J. High Energy Phys. 2019, 2019, 75. [Google Scholar] [CrossRef] [Green Version]
- Bedroya, A.; Vafa, C. Trans-Planckian censorship and the swampland. J. High Energy Phys. 2020, 2020, 123. [Google Scholar] [CrossRef]
- Burgess, C.P.; de Alwis, S.P.; Quevedo, F. Cosmological trans-Planckian conjectures are not effective. J. Cosmol. Astropart. Phys. 2021, 2021, 37. [Google Scholar] [CrossRef]
- Dvali, G.; Kehagias, A.; Riotto, A. Inflation and decoupling. arXiv 2020, arXiv:2005.05146. [Google Scholar]
- Bedroya, A. de Sitter Complementarity, TCC, and the Swampland. arXiv 2020, arXiv:2010.09760. [Google Scholar] [CrossRef]
- Rudelius, T. Dimensional reduction and (Anti) de Sitter bounds. J. High Energy Phys. 2021, 2021, 41. [Google Scholar] [CrossRef]
- Andriot, D. Tachyonic de Sitter solutions of 10d type II supergravities. Fortschritte der Physik 2021, 69, 2100063. [Google Scholar] [CrossRef]
- Andriot, D.; Cribiori, N.; Erkinger, D. The web of swampland conjectures and the TCC bound. J. High Energy Phys. 2020, 2020, 162. [Google Scholar] [CrossRef]
- Berera, A.; Brahma, S.; Calderón, J.R. Role of trans-Planckian modes in cosmology. J. High Energy Phys. 2020, 2020, 71. [Google Scholar] [CrossRef]
- Brahma, S. Trans-Planckian censorship conjecture from the swampland distance conjecture. Phys. Rev. D 2020, 101, 046013. [Google Scholar] [CrossRef] [Green Version]
- Aalsma, L.; Shiu, G. Chaos and complementarity in de Sitter space. J. High Energy Phys. 2020, 2020, 152. [Google Scholar] [CrossRef]
- Goheer, N.; Kleban, M.; Susskind, L. The trouble with de Sitter space. J. High Energy Phys. 2003, 2003, 056. [Google Scholar] [CrossRef]
- Arkani-Hamed, N.; Dubovsky, S.; Nicolis, A.; Trincherini, E.; Villadoro, G. A measure of de Sitter entropy and eternal inflation. J. High Energy Phys. 2007, 2007, 55. [Google Scholar] [CrossRef] [Green Version]
- Kachru, S.; Kallosh, R.; Linde, A.; Trivedi, S.P. De Sitter vacua in string theory. Phys. Rev. D 2003, 68, 046005. [Google Scholar] [CrossRef] [Green Version]
- Dubovsky, S.; Senatore, L.; Villadoro, G. The volume of the universe after inflation and de Sitter entropy. J. High Energy Phys. 2009, 2009, 118. [Google Scholar] [CrossRef] [Green Version]
- Dvali, G.; Gómez, C.; Zell, S. Quantum break-time of de Sitter. J. Cosmol. Astropart. Phys. 2017, 2017, 028. [Google Scholar] [CrossRef] [Green Version]
- Brahma, S.; Dasgupta, K.; Tatar, R. de Sitter space as a Glauber-Sudarshan state. J. High Energy Phys. 2021, 2021, 104. [Google Scholar] [CrossRef]
- Brahma, S.; Dasgupta, K.; Tatar, R. Four-dimensional de Sitter space is a Glauber-Sudarshan state in string theory. J. High Energy Phys. 2021, 2021, 114. [Google Scholar] [CrossRef]
- Susskind, L. Addendum to fast scramblers. arXiv 2011, arXiv:1101.6048. [Google Scholar]
- Gibbons, G.W.; Hawking, S.W. Cosmological event horizons, thermodynamics, and particle creation. In Euclidean Quantum Gravity; World Scientific: Singapore, 1993; pp. 281–294. [Google Scholar]
- Bedroya, A.; Brandenberger, R.; Loverde, M.; Vafa, C. Trans-Planckian censorship and inflationary cosmology. Phys. Rev. D 2020, 101, 103502. [Google Scholar] [CrossRef]
- Solodukhin, S.N. Entanglement entropy of black holes. Living Rev. Relativ. 2011, 14, 8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Casini, H.; Huerta, M. Entanglement entropy in free quantum field theory. J. Phys. A Math. Theor. 2009, 42, 504007. [Google Scholar] [CrossRef]
- Maldacena, J.; Pimentel, G.L. Entanglement entropy in de Sitter space. J. High Energy Phys. 2013, 2013, 38. [Google Scholar] [CrossRef] [Green Version]
- Giataganas, D.; Tetradis, N. Entanglement entropy in FRW backgrounds. Phys. Lett. B 2021, 820, 136493. [Google Scholar] [CrossRef]
- Giantsos, V.; Tetradis, N. Entanglement entropy in a four-dimensional cosmological background. arXiv 2022, arXiv:2203.06699. [Google Scholar] [CrossRef]
- Balasubramanian, V.; McDermott, M.B.; Van Raamsdonk, M. Momentum-space entanglement and renormalization in quantum field theory. Phys. Rev. D 2012, 86, 045014. [Google Scholar] [CrossRef] [Green Version]
- Brahma, S.; Alaryani, O.; Brandenberger, R. Entanglement entropy of cosmological perturbations. Phys. Rev. D 2020, 102, 043529. [Google Scholar] [CrossRef]
- Albrecht, A.; Ferreira, P.; Joyce, M.; Prokopec, T. Inflation and squeezed quantum states. Phys. Rev. D 1994, 50, 4807. [Google Scholar] [CrossRef] [Green Version]
- Brahma, S.; Berera, A.; Calderón-Figueroa, J. Universal signature of quantum entanglement across cosmological distances. arXiv 2021, arXiv:2107.06910. [Google Scholar]
- Maldacena, J. Non-Gaussian features of primordial fluctuations in single field inflationary models. J. High Energy Phys. 2003, 2003, 13. [Google Scholar] [CrossRef]
- Bousso, R. A covariant entropy conjecture. J. High Energy Phys. 1999, 1999, 4. [Google Scholar] [CrossRef] [Green Version]
- Bhattacharyya, A.; Das, S.; Haque, S.S.; Underwood, B. Rise of cosmological complexity: Saturation of growth and chaos. Phys. Rev. Res. 2020, 2, 033273. [Google Scholar] [CrossRef]
- Brandenberger, R. Initial conditions for inflation—A short review. Int. J. Mod. Phys. D 2017, 26, 1740002. [Google Scholar] [CrossRef] [Green Version]
- Banks, T. Cosmological breaking of supersymmetry? Int. J. Mod. Phys. A 2001, 16, 910–921. [Google Scholar] [CrossRef]
- Banks, T.; Fischler, W. An upper bound on the number of e-foldings. arXiv 2003, arXiv:astro-ph/0307459. [Google Scholar]
- Brahma, S.; Brandenberger, R.; Wang, Z. Entanglement entropy of cosmological perturbations for S-brane Ekpyrosis. J. Cosmol. Astropart. Phys. 2021, 2021, 94. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brahma, S. Constraints on the Duration of Inflation from Entanglement Entropy Bounds. Universe 2022, 8, 438. https://doi.org/10.3390/universe8090438
Brahma S. Constraints on the Duration of Inflation from Entanglement Entropy Bounds. Universe. 2022; 8(9):438. https://doi.org/10.3390/universe8090438
Chicago/Turabian StyleBrahma, Suddhasattwa. 2022. "Constraints on the Duration of Inflation from Entanglement Entropy Bounds" Universe 8, no. 9: 438. https://doi.org/10.3390/universe8090438
APA StyleBrahma, S. (2022). Constraints on the Duration of Inflation from Entanglement Entropy Bounds. Universe, 8(9), 438. https://doi.org/10.3390/universe8090438






