Maximal Kinematical Invariance Group of Fluid Dynamics and Applications

Abstract

The maximal kinematical invariance group of the Euler equations of fluid dynamics for the standard polytropic exponent is larger than the Galilei group. Specifically, the inversion transformation $(\Sigma :t\to -1/t,\overrightarrow{x}\to \overrightarrow{x}/t)$ leaves the Euler equation’s invariant. This duality has been used to explain the striking similarities observed in simulations of the supernova explosions and laboratory implosions induced in plasma by intense lasers. The inversion symmetry extends to discontinuous fluid flows as well. In this contribution, we provide a concise review of these ideas and discuss some applications. We also explicitly work out the implosion dual of the Sedov’s explosion solution.

1. Introduction

Surprises lurk in unexpected corners of physics. This review summarises a body of results that ensue from one such surprise, viz. the striking similarity between the earlier simulations of supernova explosions and the experimental evolution of an imploding plasma contained in a fusion capsule bombarded by high-intensity lasers [1]. It was hoped [1] that, over time, laser experiments would become more in line with actual supernovae behaviour. Hence, considerable efforts were devoted to simulating astrophysical systems in the laboratory. Modern supernova simulations have become much more complex; several new physical effects and numerical techniques are incorporated [2]. At the present stages of development, it is not clear how much one can learn about the astrophysical systems in the laboratory setting. However, the observations mentioned above have led to some intriguing theoretical developments. In this paper we concentrate on discussing the theoretical explanation [3,4,5] for the observed similarity [1], and some ramifications of the resulting analysis [6]. We limit our considerations mostly to references [3,4,5,6].

Earlier computational studies of the evolution of a supernova remnant (as cited in [1]) usually used initial conditions of dense pressure-free ejecta expanding ballistically outwards from the site of the explosion, taken for convenience to be the origin, and interacting with a stationary ambient medium of much lower density and negligible pressure. At early times, the bulk of the ejecta expands ballistically, except for a thin interaction region on the outside consisting of a forward shock running into the ambient medium, a zone of hot-shocked ambient medium, a contact discontinuity, a zone of shocked ejecta, and a reverse shock propagating into the ejecta. At later times, when the mass of the swept-up ambient medium becomes comparable with the ejecta mass, the reverse shock detaches itself from the contact discontinuity and implodes on the origin.

In the laboratory plasma, we have, initially, a stationary sphere of high density material surrounded by a low density converging flow. The inflowing gas has to decelerate at the shock front, building up pressure and driving a reverse shock which leads to an implosion. From an experimental point of view, a perfectly spherically symmetric explosion is not realistic. The ejecta emerging from a supernova explosion is also highly nonuniform on a wide range of scales making it computationally challenging to calculate its evolution.

From a theoretical point of view, it is interesting to study the underlying symmetry that enables one to map an explosion to an implosion. One can straightaway rule out time-reversal as an answer because the supernova explosions occur over astronomical time-scales, while the plasma implosions happen in a few nano-seconds.

Both an exploding star and an imploding plasma can be modelled by the equations of a perfect fluid, as we are taught in standard textbooks [7,8]. The explanation offered in Refs. [3,4] for the observed explosion–implosion duality stems from a hitherto unnoticed nonlinear symmetry of these equations, which we expand upon in the next section.

This analysis of ref. [4] highlights that the maximal kinematical invariance group of the Euler equations of fluid dynamics for the standard polytropic exponent is larger than the Galilei group. The techniques required to establish this find applications in other situations, viz. fluid flows in non-inertial frames. The Earth’s oceans and atmosphere are important examples of fluid flows in non-inertial reference frames, where the Earth’s rotation provides the underlying non-inertial frame. In order to describe oceanic and atmospheric fluid flows, it is natural to analyse fluid phenomena in the Earth’s frame. This requires adding Coriolis forces to the right hand side of the fluid equations. The Coriolis force terms lead to surprising phenomenon: weather storms and ocean’s currents. Going to the non-inertial reference frame allows us to separate out the rotational component of the fluid flows. We can then concentrate on the parts of flow patterns that matter the most.

Related situations arise when fluid flows are characterised by a large degree of expansion or contraction. Poludnenko and Khokhlov [6] considered the formulation of Euler equations of fluid dynamics in an expanding or contracting or possibly rotating reference frame. The motivation being that by going to an appropriately chosen frame we can discard the expanding or contracting or rotating nature of the fluid flows. The frame motion is adjusted to minimise the local fluid velocities. This method allows to accommodate efficiently large degrees of change in the flow extent, such as those encountered in astrophysical flows: supernovae, contracting stars, etc. Their work investigated numerical computations in such non-inertial reference frames.

As in the case of rigidly rotating reference frames, going to an expanding or contracting reference frame requires adjustments of the fluid flow equations. Ref. [6] argued that these adjustments do not come at any additional numerical cost: the new equations can be as easily implemented numerically using any of the standard numerical schemes. However, by separating out the global component of the fluid flow, it leads to significant improvement in the physical understanding of the fluid flows, which would be difficult to extract in inertial reference frame simulations. (More precisely, in numerical work it is important to work with smaller local fluid velocities. If a fluid flow is dominated by the global component associated with expansion or contraction or rotation, then it is inefficient to model such flows in inertial frames.) They carried out extensive numerical testing of the method for a variety of reference frames representative of realistic applications.

The rest of the article is organised as follows. In Section 2, we review the maximal kinematical invariance group of fluid dynamics, based on the original work of O’ Raifeartaigh and one of the authors [4]. In Section 3, we discuss the symmetries of discontinuous flows, based on our original work with Oliver Jahn [5]. In Section 4, we review the work of Poludnenko and Khokhlov [6], who considered the formulation of Euler equations of fluid dynamics in non-inertial reference frames. In Section 5, we present the conclusions. Appendix A explicitly works out the implosion dual of Sedov’s explosion solution. Throughout the review we will be concise, referring the reader to original references for further details.

2. The Fluid Equations

This section is based on the original work of O’Raifeartaigh and one of the authors [4]. The general fluid dynamic equations in n-dimensional space are (see, e.g., textbooks [7,8])

$$\begin{array}{c}D\rho =-\rho \nabla ·\mathbf{u},\end{array}$$

(1)

$$\begin{array}{c}\rho D\mathbf{u}=-\nabla p+\mathbf{V},\end{array}$$

(2)

$$\begin{array}{c}Dϵ=-(ϵ+p)\nabla ·\mathbf{u},\end{array}$$

(3)

where the convective derivative D and the viscosity terms $\mathbf{V}$ are defined by

$$\begin{array}{c}D=\frac{\partial }{\partial t}+\mathbf{u}·\nabla ,\end{array}$$

(4)

$$\begin{array}{c}{V}_i={\nabla }_j\left(\eta ({\nabla }_j{u}_i+{\nabla }_i{u}_j-\frac{2}{n}{\delta }_{ij}{\nabla }_k{u}_k)\right)+{\nabla }_i\left(\zeta {\nabla }_k{u}_k\right),\end{array}$$

(5)

respectively. In the above equations $\rho ,\mathbf{u},p,ϵ$ stand for the density, the velocity vector field, the pressure, and the energy density respectively, and $\eta$ and $\zeta$ are the bulk and shear viscosity fields. These partial differential equations are augmented by an algebraic condition called the equation of state that relates the pressure and energy density. According to the polytropic equation of state

$$p=({\gamma }_0-1)ϵ\Rightarrow p+ϵ={\gamma }_0ϵ,$$

(6)

where the constant ${\gamma }_0$ is called the polytropic exponent. This equation can be used to eliminate p from the fluid equations. Further, by making the substitution

$$ϵ=\chi {\rho }^{{\gamma }_0}$$

(7)

the equations can be rewritten in the form

$$\begin{array}{c}D\rho =-\rho \nabla ·\mathbf{u},\end{array}$$

(8)

$$\begin{array}{c}\rho D\mathbf{u}=-({\gamma }_0-1)\nabla \left(\chi {\rho }^{{\gamma }_0}\right)+\mathbf{V},\end{array}$$

(9)

$$\begin{array}{c}D\chi =0.\end{array}$$

(10)

2.1. Action Formulation

The fluid equations may be given an action formulation by switching off the viscosity fields, i.e., $\eta =\zeta =0$. Such fluids are called inviscid, or perfect fluids, and the equations are called Euler’s equations. We next set $\chi =1$ without loss of consistency, representing the isentropicity condition. Further, the Clebsch parametrisation [9,10,11]

$$\mathbf{u}=\nabla \varphi -\nu \nabla \theta ,$$

(11)

allows us to isolate the irrotational parts by setting $\nu =\theta =0$. The resulting action for inviscid, isentropic, irrotational flows in three dimensions is given by,

$$S=\int d^{3}xdt\left[\rho \left(\dot{\varphi }-\frac{1}{2}{(\nabla \varphi )}^2\right)-{\rho }^{{\gamma }_0}\right].$$

(12)

It may be mentioned in passing that the terms contained in the parentheses represent the Hamilton–Jacobi function for a free particle.

The symmetries of the aforementioned special flows, represented by the action, follow from the requirement of its form-invariance. The transformation properties of the fields in the general fluid equations may be extracted from these transformation properties once again by requiring the equations to transform covariantly.

2.2. Symmetries

We begin a priori with the most general transformations involving the coordinates and fields. The transformed coordinates $\xi$ and $\tau$ are defined by

$$\overrightarrow{\xi }=\overrightarrow{\xi }(\overrightarrow{x},t),\tau =\tau (\overrightarrow{x},t),\tilde{\varphi }=\tilde{\varphi }(\xi ,\tau ,\varphi ),\tilde{\rho }=\tilde{\rho }(\xi ,\tau ,\rho ).$$

(13)

Substituting the transformations and demanding the form-invariance of the action produces a set of equations which can be solved exactly to yield [4],

$$\begin{array}{c}\overrightarrow{\xi }=f\left(t\right)(\mathbf{R}\overrightarrow{x}+\overrightarrow{a}+\overrightarrow{v}t),\end{array}$$

(14)

$$\begin{array}{c}\tilde{\rho }=f^{-3}\left(t\right)\rho ,\end{array}$$

(15)

$$\begin{array}{c}\tilde{\varphi }=\varphi +\lambda (\xi ,\tau ),\end{array}$$

(16)

where

$$\begin{array}{c}\tau =\frac{\alpha t+\beta }{\gamma t+\delta },f\left(t\right)=\frac{1}{\gamma t+\delta },\alpha \delta -\beta \gamma =1,\end{array}$$

(17)

$$\begin{array}{c}\frac{\partial \lambda }{\partial \tau }-\frac{1}{2}{\left(\frac{\partial \lambda }{\partial \xi }\right)}^2=0.\end{array}$$

(18)

In the above, $\mathbf{R}$ represents the usual rotation matrix, $\overrightarrow{a}$ the translations, $\overrightarrow{v}$ the boosts, and $f\left(t\right)$, a time-dependent scale parameter. The $\alpha ,\beta ,\gamma ,\delta$ represent parameters of the $SL(2,R)$ group, a non-relativistic remnant of the special conformal group, to be discussed in Section 2.4. For details on $\lambda (\xi ,\tau )$, we refer the reader to the original reference [4]. We note that the following discrete symmetries are permitted:

$$(\alpha ,\beta ,\gamma ,\delta )\sim (\alpha ,-\beta ,-\gamma ,\delta )\sim (-\alpha ,\beta ,\gamma ,-\delta )\sim (-\alpha ,-\beta ,-\gamma ,-\delta ).$$

(19)

2.3. Transformation Functions for General Flows

The transformation functions for general flows may be obtained by requiring the general fluid equations to transform covariantly. It is straightforward to see that for general non-isentropic flows, the equations transform covariantly if $\chi$ is a scalar under the coordinate transformations.

The velocity vector transforms inhomogeneously as,

$$\tilde{\overrightarrow{u}}=(\gamma t+\delta )\overrightarrow{u}-\gamma \overrightarrow{x}$$

(20)

We note that these transformations do not preserve the condition $\nabla ·\mathbf{u}=0$, implying that unlike Galilean symmetry, the above symmetry is valid only when the fluid is compressible.

The viscosity fields transform similar to scalar densities [4]. This implies that the symmetry we are discussing is broken in the case of Navier–Stokes equations which approximate the viscosity to be constant.

2.4. The Maximal Invariance Group

Let g be a general element of the symmetry group G obtained by setting $\beta =\gamma =0,\alpha =1$. It follows

$$\overrightarrow{\xi }=\mathbf{R}\overrightarrow{x}+\overrightarrow{v}t+\overrightarrow{a},\tau =t$$

(21)

In this case,

$$\tilde{\rho }=\rho \mathrm{and}\tilde{\overrightarrow{u}}=\overrightarrow{u}+\overrightarrow{v}$$

(22)

We notice that these correspond to the static Galilei transformations.

Let $\sigma$ denote an element of the $SL(2,R)$ group obtained by setting $\overrightarrow{a}=\overrightarrow{v}=0$. In this case,

$$\begin{array}{c}\overrightarrow{\xi }=f\left(t\right)\overrightarrow{x},\tau =\frac{\alpha t+\beta }{\gamma t+\delta },\end{array}$$

(23)

$$\begin{array}{c}\tilde{\rho }={(\gamma t+\delta )}^3\rho \mathrm{and}\tilde{\overrightarrow{u}}=(\gamma t+\delta )\overrightarrow{u}-\gamma \overrightarrow{x}.\end{array}$$

(24)

This represents a combination of dilatations and inversions, which are a nonrelativistic limit of the special conformal group.

It is a straightforward exercise to check that G is an invariant subgroup:

$${\sigma }^{-1}·g(\mathbf{R},\overrightarrow{a},\overrightarrow{v})·\sigma =g(\mathbf{R},{\overrightarrow{a}}_{\sigma },{\overrightarrow{v}}_{\sigma }),$$

(25)

where

$$\left(\begin{array}{c}{\overrightarrow{a}}_{\sigma }\\ {\overrightarrow{v}}_{\sigma }\end{array}\right)=\left(\begin{array}{cc}\delta & \beta \\ \gamma & \alpha \end{array}\right)\left(\begin{array}{c}\overrightarrow{a}\\ \overrightarrow{v}\end{array}\right).$$

(26)

It therefore follows that the full group under which the fluid equations are invariant under the specified transformation properties for the coordinates and the fields is a semi-direct product

$$\mathcal{G}=SL(2,R)\wedge G.$$

A special element of the group viz. $\Sigma :(\alpha ,\beta ,\gamma ,\delta )=(0,-1,1,0)$ corresponds to a composition of an inversion and reversal of time, and plays an important role in the explosion–implosion duality discovered by Drury and Mendonça in [3]. A curious result follows immediately: ${\Sigma }^2=P$, where P is the parity operator.

Cosets defined using these elements, $g_{\Sigma }(\mathbf{R},\overrightarrow{a},\overrightarrow{v})=\Sigma ·g(\mathbf{R},\overrightarrow{a},\overrightarrow{v})$ have the interesting property that they are fourth roots of Galilean transformations,

$$g_{\Sigma }^4(\mathbf{R},\overrightarrow{a},\overrightarrow{v})=g\left({\mathbf{R}}^{\mathbf{4}},\left({\mathbf{R}}^{\mathbf{2}}-\mathbf{1}\right)(\mathbf{R}\overrightarrow{a}-\overrightarrow{v}),\left({\mathbf{R}}^{\mathbf{2}}-\mathbf{1}\right)(\mathbf{R}\overrightarrow{v}+\overrightarrow{a})\right)$$

(27)

Since $(\mathbf{R}\overrightarrow{a}-\overrightarrow{v})$ and $(\mathbf{R}\overrightarrow{v}-\overrightarrow{a})$ are linearly independent, it follows that every Galilean transformation is a fourth power of a coset transformation [4].

2.5. Conservation Laws

Euler’s equations for a perfect fluid can be expressed in the form of conservation laws for mass, momentum, and energy, as follows:

$$\begin{array}{c}\frac{\partial \rho }{\partial t}=-\frac{\partial }{\partial x_j}\left(\rho u_j\right),\end{array}$$

(28)

$$\begin{array}{c}\frac{\partial }{\partial t}\left(\rho u_i\right)=-\frac{\partial }{\partial x_j}(\rho u_i{u}_j+{\delta }_{ij}p),\end{array}$$

(29)

$$\begin{array}{c}\frac{\partial }{\partial t}\left(\frac{1}{2}\rho {\overrightarrow{u}}^2+ϵ\right)=-\frac{\partial }{\partial x_j}\left[\left(\frac{1}{2}\rho {\overrightarrow{u}}^2+ϵ+p\right)u_j\right].\end{array}$$

(30)

These equations can be expressed succinctly as follows:

$${\partial }_{\mu }{J}_{\rho }^{\mu }={\partial }_{\mu }{J}_{\overrightarrow{P}}^{\mu }={\partial }_{\mu }{J}_H^{\mu }=0.$$

(31)

The zeroth components of the above currents namely, $\rho ,\rho \overrightarrow{u},\frac{1}{2}\rho {\overrightarrow{u}}^2+ϵ$, give the charge densities which, when integrated over all space, give the conserved charges. The corresponding current densities are

$$\begin{array}{c}{J}_{\rho }^j=\rho u_j,\end{array}$$

(32)

$$\begin{array}{c}{J}_{P_i}^j=(\rho u_i{u}_j+{\delta }_{ij}p),\end{array}$$

(33)

$$\begin{array}{c}{J}_H^j=\left(\frac{1}{2}\rho {\overrightarrow{u}}^2+ϵ+p\right)u_j.\end{array}$$

(34)

The conservation laws corresponding to rotations ($\delta x^i={\omega }^{ij}{x}^j$), boosts ($\delta x^i=v^{i}t$), dilatations ($\delta x^i=\lambda x^i,\delta t=2\lambda t$), and expansions ($\delta x^i=-\mu t{x}^i,\delta t=-\mu t^2$) can be stated similarly,

$${\partial }_{\mu }{J}_{\overrightarrow{L}}^{\mu }={\partial }_{\mu }{J}_{\overrightarrow{K}}^{\mu }={\partial }_{\mu }{J}_D^{\mu }={\partial }_{\mu }{J}_A^{\mu }=0,$$

(35)

where the appropriate charge densities are [5],

$$\overrightarrow{L}=\overrightarrow{P}\times \overrightarrow{x},\overrightarrow{K}=\overrightarrow{P}t-\rho \overrightarrow{x},D=-2tH+\overrightarrow{x}·\overrightarrow{P},A=t^{2}H-t\overrightarrow{x}·\overrightarrow{P}+\frac{{\rho }^2}{2}{\overrightarrow{x}}^2.$$

(36)

The corresponding current densities can also be written down in a straightforward manner

$$\begin{array}{c}{\overrightarrow{J}}_{L_i}={ϵ}_{ikl}{x}_k{\overrightarrow{J}}_{P_l},\end{array}$$

(37)

$$\begin{array}{c}{\overrightarrow{J}}_{K_i}=t{J}_{P_i}-x_i{\overrightarrow{J}}_{\rho },\end{array}$$

(38)

$$\begin{array}{c}{\overrightarrow{J}}_D=x_i{\overrightarrow{J}}_{P_i}-2t{\overrightarrow{J}}_H,\end{array}$$

(39)

$$\begin{array}{c}{\overrightarrow{J}}_A=\frac{1}{2}{\overrightarrow{x}}^2{\overrightarrow{J}}_{\rho }-t{x}_i{\overrightarrow{J}}_{P_i}+t^2{\overrightarrow{J}}_H.\end{array}$$

(40)

These laws follow as a direct consequence of Noether’s theorem. They will be useful in studying flows with discontinuities, a topic to which we now pass.

3. Discontinuous Flows

This section is based on our original work with Oliver Jahn [5]. As long as the flows are smooth, i.e., the functions $\rho ,\overrightarrow{u},p,ϵ$ are smooth functions of $\overrightarrow{x},t$, Equations (1)–(3) and (31) are equivalent. Real flows, however, may develop discontinuities as they evolve. Such flows are described by weak solutions of differential Equations [12]. A weak solution is generally piecewise smooth. The smooth parts satisfy the differential equations in the usual strong form. The entire solution required to specify the course of motion of the initial conditions is obtained by supplementing the strong solutions by jump conditions. The jump conditions are derived from the conservation laws. We briefly review these concepts in the next two subsections. For pedagogical discussions on these topics we refer the reader to [7,8,13].

3.1. Weak Solutions and Jump Conditions

By definition, any, possibly non-smooth, function $J^{\mu }(\overrightarrow{x},t)$ that satisfies

$$\int {\partial }_{\mu }\omega (\overrightarrow{x},t)J^{\mu }(\overrightarrow{x},t)d^{3}xdt=0,$$

(41)

for all test functions $\omega (\overrightarrow{x},t)$ is called a weak solution of the differential equation ${\partial }_{\mu }{J}^{\mu }=0$.

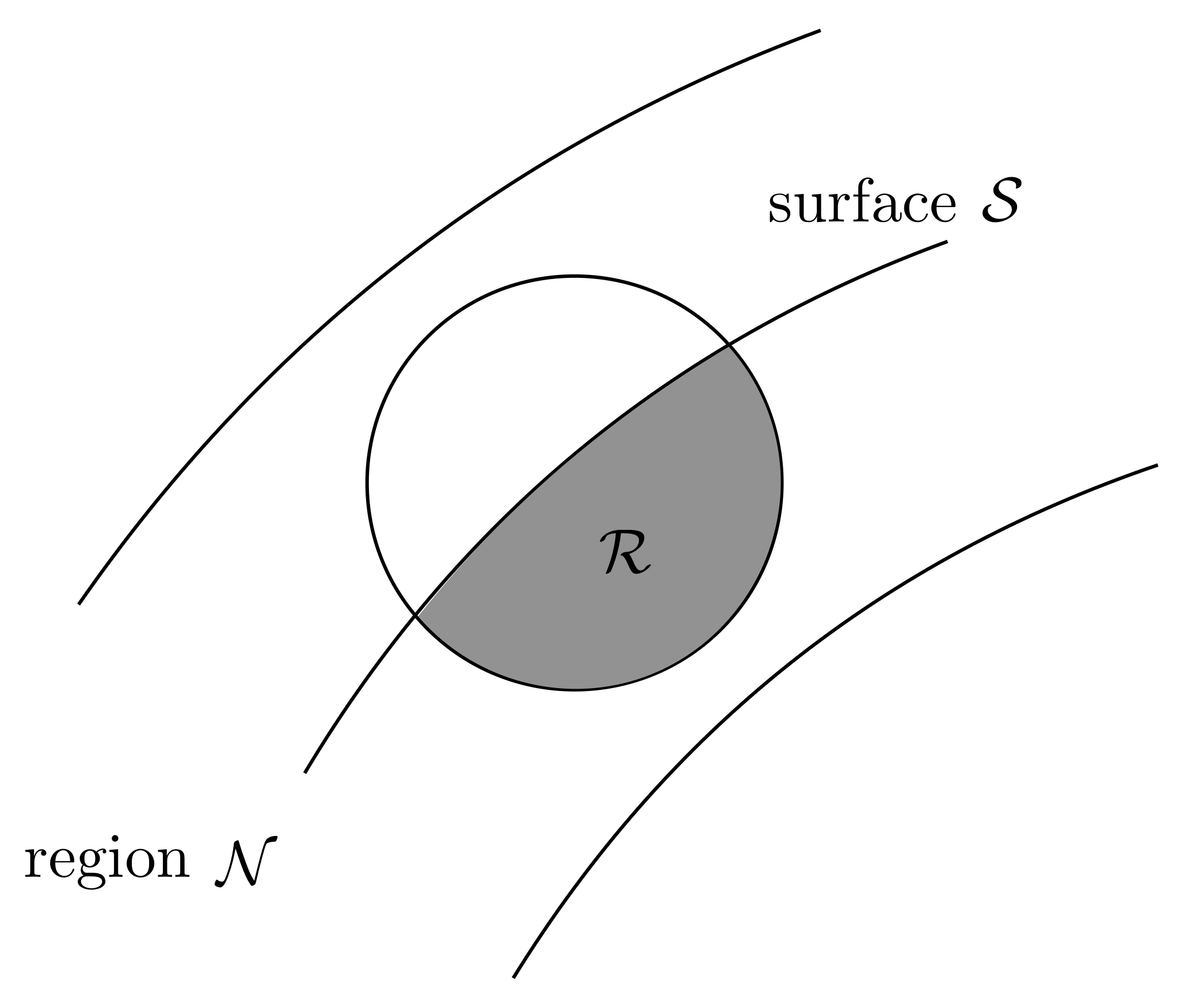
Suppose $J^{\mu }(\overrightarrow{x},t)$ has a jump discontinuity across a hypersurface $\mathcal{S}$ in $\overrightarrow{x},t$ space while otherwise being continuously differentiable in some neighbourhood $\mathcal{N}$ of $\mathcal{S}$; see Figure 1. Let $\omega (\overrightarrow{x},t)$ be a test function with support in region $\mathcal{N}$. Let $\mathcal{R}$ be the part of the region $\mathcal{N}$ that lies on one side of $\mathcal{S}$, say to the right. We take $\omega (\overrightarrow{x},t)=0$ on the boundary of $\mathcal{R}$, except on $\mathcal{S}$. Then by Gauss’s theorem,

$${\int }_{\mathcal{R}}{\partial }_{\mu }\omega J^{\mu }{d}^{3}xdt+{\int }_{\mathcal{R}}\omega {\partial }_{\mu }{J}^{\mu }{d}^{3}xdt={\int }_{\mathcal{R}}{\partial }_{\mu }\left(\omega J^{\mu }\right)d^{3}xdt={\int }_{\mathcal{S}}\omega n_{\mu }{J}^{\mu }d\mathcal{S},$$

(42)

since $\omega (\overrightarrow{x},t)=0$ on the boundary of $\mathcal{R}$, except on $\mathcal{S}$. Here $n^{\mu }$ is the outward normal to the hypersurface $\mathcal{S}$. The second integral on the left hand side is zero because the conservation law holds in the strong sense in the interior of $\mathcal{R}$. If we integrate similarly over the left part of the support $\omega (\overrightarrow{x},t)$, and add the two results we obtain for a weak solution

$$0={\int }_{\mathcal{S}}\omega n_{\mu }\Delta J^{\mu }d\mathcal{S},$$

(43)

where $\Delta f$ denotes the difference of the two limiting values of the the function f on the two sides of the hypersurface $\mathcal{S}$, i.e., the jump of the function. This result follows as the normal which points outwards by convention, flips its sign when we move from the right to the left side of the hypersurface. Since $\omega (\overrightarrow{x},t)$ is an arbitrary function, it follows that

$$n_{\mu }\Delta J^{\mu }=0\mathrm{on}\mathcal{S}.$$

(44)

This equation is the jump condition we mentioned earlier.

3.2. Rankine–Hugoniot Conditions

The general expression for the jump condition derived above can be applied to the conservation laws derived earlier. The conservation laws associated with mass, momentum, and energy yield

$$\begin{array}{c}{n}_{\mu }\Delta J_{\left(\rho \right)}^{\mu }=0,\end{array}$$

(45)

$$\begin{array}{c}{n}_{\mu }\Delta J_{\left(\overrightarrow{P}\right)}^{\mu }=0,\end{array}$$

(46)

$$\begin{array}{c}{n}_{\mu }\Delta J_{\left(H\right)}^{\mu }=0,\end{array}$$

(47)

and are called the Rankine–Hugoniot conditions in the fluid dynamics literature [7,8,13,14].

Similar equations can be derived for the other conservation laws, viz.

$$\begin{array}{c}{n}_{\mu }\Delta J_{\left(\overrightarrow{L}\right)}^{\mu }=0,\end{array}$$

(48)

$$\begin{array}{c}{n}_{\mu }\Delta J_{\left(\overrightarrow{K}\right)}^{\mu }=0,\end{array}$$

(49)

$$\begin{array}{c}{n}_{\mu }\Delta J_{\left(D\right)}^{\mu }=0,\end{array}$$

(50)

$$\begin{array}{c}{n}_{\mu }\Delta J_{\left(A\right)}^{\mu }=0.\end{array}$$

(51)

These new jump conditions are associated with angular momentum, boosts, dilatations, and expansions.

3.3. Dual Rankine–Hugoniot Conditions

The new set of jump conditions are identically true because the current densities associated with angular momentum, boosts, dilatations, and expansions are linear combinations of the current densities associated with mass, momentum and energy conservation as shown in Equation (40). This suggests that the Rankine–Hugoniot conditions are invariant under the full kinematical invariance group of smooth flows including the $SL(2,R)$ part. We examine this point in what follows.

If an (abstract) symmetry generator $T_r$ transforms under the $SL(2,R)$ transformation $\sigma$ as

$$T_r^{{}^{\prime }}={\sigma }^{-1}{T}_r\sigma =\sum _s{M}_{rs}\left(\sigma \right)T_s,$$

(52)

then the corresponding currents transform as [5]

$$J_r^{\mu {}^{\prime }}\left(x^{{}^{\prime }}\right)=\det \left(\frac{\partial x}{\partial x^{{}^{\prime }}}\right)\frac{\partial x^{\mu {}^{\prime }}}{\partial x^{\nu }}\sum _s{M}_{rs}\left(\sigma \right)J_s^{\nu }\left(x\right).$$

(53)

The fact that the currents transform similar to vector densities can be appreciated by looking at the temporal components, which pick up the multiplicative factor ${(\gamma t+\delta )}^3$.

Arranging the currents in a column $J^{\mu }={\left(J_{\left(\rho \right)}^{\mu },J_{(\overrightarrow{K})}^{\mu },J_{(\overrightarrow{P})}^{\mu },J_{\left(A\right)}^{\mu },J_{\left(D\right)}^{\mu },J_{\left(H\right)}^{\mu }\right)}^T$, one has the following transformation matrix

$$M=\left(\begin{array}{cccccc}1& 0& 0& 0& 0& 0\\ 0& \alpha & \beta & 0& 0& 0\\ 0& \gamma & \delta & 0& 0& 0\\ 0& 0& 0& \alpha & -\alpha \beta & {\beta }^2\\ 0& 0& 0& -2\alpha \gamma & (\beta \gamma +\alpha \delta )& -2\beta \gamma \\ 0& 0& 0& {\gamma }^2& -\gamma \delta & {\delta }^2\end{array}\right).$$

(54)

Using $\alpha \delta -\beta \gamma =1$, it is easy to check that the matrix M has unit determinant.

The temporal components transform according to the transformations,

$$\begin{array}{c}\rho {}^{\prime }={(\gamma t+\delta )}^3\rho ,\end{array}$$

(55)

$$\begin{array}{c}\overrightarrow{P}{}^{\prime }={(\gamma t+\delta )}^3(\delta \overrightarrow{P}+\gamma \overrightarrow{K}),\end{array}$$

(56)

$$\begin{array}{c}H{}^{\prime }={(\gamma t+\delta )}^3({\gamma }^{2}A-\delta \gamma D+{\delta }^{2}H).\end{array}$$

(57)

Thus, $\rho$ transforms under the singlet representation of $SL(2,R)$ as a scalar density. The translations and boosts constitute the doublet representation and transform similar to vector densities. Likewise the Hamiltonian, and the generators of dilatations and expansions transform similar to densities under the triplet (adjoint) representation of $SL(2,R)$. The transformation properties of the spatial components of the current can similarly be read off from the above matrix.

The dual Rankine-Hugoniot conditions are now easily obtained. The normal vector $n_{\mu }$ appearing in the jump condition (44) transforms like a covector

$$n_{\mu }^{{}^{\prime }}=\frac{\partial x^{\nu }}{\partial x^{\mu {}^{\prime }}}{n}_{\nu }.$$

(58)

Thus, the transformed jump conditions for the currents are

$$n_{\mu }^{{}^{\prime }}\Delta J_r^{\mu {}^{\prime }}\propto \det \left(\frac{\partial x}{\partial x^{{}^{\prime }}}\right)\sum _s{M}_{rs}\left(\sigma \right)n_{\mu }\Delta J_s^{\mu }\left(x\right)=0\mathrm{on}\mathcal{S}.$$

(59)

Since the determinant is smooth across the hypersurface $\mathcal{S}$, the factor in front can be omitted, and the transformed jump condition is a linear combination of the original jump conditions.

In particular, the transformed conditions for $J_{\left(\rho \right)}^{\mu },J_{(\overrightarrow{P})}^{\mu },J_{\left(H\right)}^{\mu }$ (the Rankine–Hugoniot conditions), become linear combinations of the jump conditions of $J_{\left(\rho \right)}^{\mu },J_{(\overrightarrow{P})}^{\mu },J_{\left(H\right)}^{\mu },J_{(\overrightarrow{K})}^{\mu },J_{\left(D\right)}^{\mu }$ and $J_{\left(A\right)}^{\mu }$. Specifically,

$$\begin{array}{c}{n}_{\mu }^{{}^{\prime }}\Delta J_{\left(\rho \right)}^{\mu {}^{\prime }}\propto n_{\mu }\Delta J_{\left(\rho \right)}^{\mu }\end{array}$$

(60)

$$\begin{array}{c}{n}_{\mu }^{{}^{\prime }}\Delta J_{(\overrightarrow{P})}^{\mu {}^{\prime }}\propto n_{\mu }(\gamma \Delta J_{(\overrightarrow{K})}^{\mu }+\delta \Delta J_{(\overrightarrow{P})}^{\mu })\end{array}$$

(61)

$$\begin{array}{c}{n}_{\mu }^{{}^{\prime }}\Delta J_{\left(H\right)}^{\mu {}^{\prime }}\propto n_{\mu }({\delta }^2\Delta J_{\left(H\right)}^{\mu }-\gamma \delta \Delta J_{\left(D\right)}^{\mu }+{\gamma }^2\Delta J_{\left(A\right)}^{\mu })\end{array}$$

(62)

The original Rankine-Hugoniot conditions, in conjunction with the new conditions (48)–(51), imply that the right hand sides of the above equations are identically zero, i.e., the Rankine-Hugoniot conditions are form-invariant [5].

In particular, this holds for the Drury–Mendonça transformation [3] $t\to -1/t,\overrightarrow{x}\to \overrightarrow{x}/t$ used to relate the explosion and implosion problems. This corresponds to the choice $(\alpha ,\beta ,\gamma ,\delta )=(0,-1,1,0)$. We conclude that, if an explosion is described by the standard Rankine–Hugoniot conditions, the corresponding implosion is described by the dual Rankine–Hugoniot conditions.

3.4. Physical Conditions

As already mentioned, for a polytropic gas, $ϵ=\chi {\rho }^{{\gamma }_0}$, which enables us to write the third of Euler’s Equation (3) as $D\chi =0$. $\chi$ transforms similar to a scalar. For a polytropic gas, it is well known [14] that $\chi$ is related to the specific entropy (entropy per unit mass) as follows:

$$S-S_0=C_V\log \left[\chi {(\rho ,V)}^{{\gamma }_0}\right],$$

(63)

where $C_V=R/({\gamma }_0-1)$, R being the universal gas constant divided by the molecular weight, V, the volume, and $S_0$, an appropriate constant. Since $\chi$ transforms similar to a scalar, it follows that the specific entropy of a moving particle remains constant under an $SL(2,R)$ transformation. Hence a physical shock is mapped to a physical shock under the transformation.

The requirement that the transformation preserves the physicality of a shock puts a condition on the viscosity viz. that its positivity is preserved. As already pointed out, the viscosity fields transform as scalar densities, similar to $\rho$. It follows that the total viscosity, such as mass, is an invariant under the transformation.

4. Fluid Equations in Non-Inertial Frames

In this section, we review the work of Poludnenko and Khokhlov [6], who considered the formulation of Euler equations of fluid dynamics in non-inertial reference frames. We start with the inertial reference frame Euler Equations (1)–(3)

$$\begin{array}{c}{\partial }_t\rho +{\partial }_i\left(\rho u_i\right)=0,\end{array}$$

(64)

$$\begin{array}{c}{\partial }_t\left(\rho u_i\right)+{\partial }_j\left(\rho u_i{u}_j\right)+{\partial }_{i}p=0,\end{array}$$

(65)

$$\begin{array}{c}{\partial }_tϵ+{\partial }_i\left((ϵ+p)u_i\right)=0,\end{array}$$

(66)

where $\rho$ is the density, $u_i$ the fluid velocity, p the pressure, and $ϵ$ the total energy density. The total energy $ϵ$ is related to the internal energy per unit mass e as,

$$ϵ=e\rho +\frac{1}{2}\rho u^2.$$

(67)

Consider a non-inertial reference frame $\{{\tilde{x}}_i,\tau \}$ that expands or contracts with respect to the inertial frame $\{t,x_i\}$:

$$\begin{array}{cccc}\hfill {\tilde{x}}_i& =\frac{x_i}{a\left(t\right)},\hfill & \hfill \tau & ={\int }_0^t\frac{dt}{a{\left(t\right)}^{\beta +1}},\hfill \end{array}$$

(68)

where $\beta$ is a constant and the scale factor $a\left(t\right)$ is a smooth non-vanishing function of time. (Poludnenko and Khokhlov [6] also considered additional rotational terms in transformation (68).) For simplicity we do not consider such terms; essential ideas are all captured by the simplified transformation). We use quantities with tilde signs to refer to quantities in the non-inertial reference frame $\{{\tilde{x}}_i,\tau \}$. Time t is the physical time, and $\tau$ is the computational time.

For the density, pressure, and energy density fields we introduce the scaling,

$$\begin{array}{c}\tilde{\rho }(\tilde{x},\tau )=a^{\alpha }\rho (x,t),\end{array}$$

(69)

$$\begin{array}{c}\tilde{p}(\tilde{x},\tau )=a^{\alpha }+2\beta p(x,t),\end{array}$$

(70)

$$\begin{array}{c}\tilde{e}(\tilde{x},\tau )=a^2\beta e(x,t),\end{array}$$

(71)

where $\alpha$ and $\beta$ are constant scaling exponents. A short calculation shows that

$$u_i=\frac{d}{dt}{x}_i\left(t\right)=a^{-\beta }\frac{dlna}{d\tau }{\tilde{x}}_i+a^{-\beta }{\tilde{u}}_i,$$

(72)

and

$$\ddot{a}=\frac{1}{a^{2\beta +1}}\left[\frac{d^{2}lna}{d{\tau }^2}-\beta {\left(\frac{dlna}{d\tau }\right)}^2\right].$$

(73)

As a result, the mass conservation Equation (64) in the non-intertial frame (68) become,

$${\partial }_{\tau }\tilde{\rho }+{\tilde{\partial }}_i\left(\tilde{\rho }{\tilde{u}}_i\right)=(\alpha -n)\frac{dlna}{d\tau }\tilde{\rho },$$

(74)

where ${\tilde{\partial }}_i$ are partial derivatives with respect to ${\tilde{x}}_i$ and n is the dimension of space. The momentum conservation Equation (65) becomes

$${\partial }_{\tau }\left(\tilde{\rho }{\tilde{u}}_i\right)+{\tilde{\partial }}_j\left(\tilde{\rho }{\tilde{u}}_i{\tilde{u}}_j\right)+{\tilde{\partial }}_i\tilde{p}=(\alpha -n+\beta -1)\frac{dlna}{d\tau }\tilde{\rho }{\tilde{u}}_i-a^{2\beta +1}\ddot{a}\tilde{\rho }{\tilde{x}}_i.$$

(75)

The transformation of the energy Equation (66) to the non-intertial frame (68) is quite tedious. When the dust settles one finds,

$${\partial }_{\tau }\widetilde{ϵ}+{\tilde{\partial }}_i\left((\widetilde{ϵ}+\tilde{p}){\tilde{u}}_i\right)=\frac{dlna}{d\tau }\left[(\alpha -n+2\beta )\widetilde{ϵ}-n\tilde{p}-\tilde{\rho }{\tilde{u}}_i{\tilde{u}}_i\right]-a^{2\beta +1}\ddot{a}\tilde{\rho }{\tilde{u}}_i{\tilde{x}}_i.$$

(76)

4.1. Conditions for Invariance of the Fluid Equations

There is subclass of transformations (68) that preserve the form of the Euler’s equation. Let us look at this subclass in relation to the discussion of the previous sections. For the form invariance of mass conservation Equation (64) we require $\alpha =n$ from Equation (74). For the invariance of momentum and energy conservation (65) and (66), we require from Equations (75) and (76)$,\ddot{a}=0,$ together with

$$\begin{array}{cccc}\hfill & \alpha =n,\hfill & \hfill \beta =1,& \hfill \end{array}$$

(77)

and

$$ϵ=\frac{n}{2}p+\frac{1}{2}\rho u^2.$$

(78)

Recalling that the total energy $ϵ$ is related to the internal energy e via (67), we have

$$e=\frac{np}{2\rho }$$

(79)

This is a restriction on the equation of state. For a polytropic gas $p=\chi {\rho }^{{\gamma }_0}$ we have the general relation,

$$e=\frac{p}{({\gamma }_0-1)\rho }.$$

(80)

Thus, we conclude that the form invariance of Equations (74)–(76) singles out a special value of the polytropic index,

$${\gamma }_0=1+\frac{2}{n}.$$

(81)

For $n=3$, ${\gamma }_0=5/3$. Low mass white-dwarf stars are well approximated by this polytropic index. These results are perfectly consistent with [4] reviewed in the previous sections. $\ddot{a}=0$ implies,

$$a\left(t\right)=ct+d.$$

(82)

Thus, transformation (68) becomes

$$\begin{array}{cccc}\hfill x_i& \to \frac{x_i}{ct+d},\hfill & \hfill t& \to -\frac{1}{c(\gamma t+d)}.\hfill \end{array}$$

(83)

Comparing this with general $SL(2,R)$ transformations [4]

$$\begin{array}{cccccc}\hfill x_i& \to \frac{x_i}{ct+d},\hfill & \hfill t& \to \frac{at+b}{ct+d},\hfill & \hfill ad-bc& =1,\hfill \end{array}$$

(84)

we have $a=0,b=-1/c$. The scaling of density, pressure, and energy density (69)–(71) are also compatible with the scalings in [4], and so is the value of the polytropic index.

4.2. Non-Invariant Terms as Sources

Poludnenko and Khokhlov argue that the above formulation based on general scaling of the fluid variables provides a degree of flexibility, provided we treat the non-invariant terms as sources. They consider values of exponents other than in Equation (77) that do not leave the form of the equations invariant. For example, a set of exponents can be obtained by demanding the invariance of the first law of thermodynamics. For isentropic flows, the first law of thermodynamics in inertial frames take the form

$$ds=0\Rightarrow de=-pd\left(\frac{1}{\rho }\right),$$

(85)

which for fluid flows implies,

$${\partial }_{t}e+u_i{\partial }_{i}e=\frac{p}{{\rho }^2}({\partial }_t\rho +u_i{\partial }_i\rho ).$$

(86)

In non-inertial frames (68), Equation (86) becomes

$${\partial }_{\tau }\tilde{e}+{\tilde{u}}_i{\tilde{\partial }}_i\tilde{e}=\frac{\tilde{p}}{{\tilde{\rho }}^2}({\tilde{\partial }}_{\tau }\tilde{\rho }+{\tilde{u}}_i{\tilde{\partial }}_i\tilde{\rho })-(\alpha \tilde{p}-2\beta \tilde{e}\tilde{\rho })\frac{1}{\tilde{\rho }}\frac{dlna}{d\tau }.$$

(87)

Using the polytropic equation of state (80), it simplifies to

$${\partial }_{\tau }\tilde{e}+{\tilde{u}}_i{\tilde{\partial }}_i\tilde{e}=\frac{\tilde{p}}{{\tilde{\rho }}^2}({\tilde{\partial }}_{\tau }\tilde{\rho }+{\tilde{u}}_i{\tilde{\partial }}_i\tilde{\rho })+(2\beta -\alpha ({\gamma }_0-1))\frac{dlna}{d\tau }\tilde{e}.$$

(88)

The choice of exponents

$$\begin{array}{cccc}\hfill & \alpha =n,\hfill & \hfill \beta =\frac{n({\gamma }_0-1)}{2}& ,\hfill \end{array}$$

(89)

ensures the invariance of the first law of thermodynamics together with the mass conservation for all values of the polytropic index. The momentum conservation equation no longer takes the conservative form, however. With this choice of exponents (89), the first of the source terms of the momentum conservation Equation (76),

$$(\alpha -n+2\beta )\widetilde{ϵ}-n\tilde{p}-\tilde{\rho }{\tilde{u}}_i{\tilde{u}}_i$$

(90)

simplifies to

$$(\beta -1)\tilde{\rho }{\tilde{u}}_i{\tilde{u}}_i.$$

(91)

This source term is proportional to the kinetic energy. This new set of exponents may be a preferred choice in numerical simulations if the thermal energy dominates the local kinetic energy in the non-inertial frame.

4.3. Primitive Fields as Simulation Variables

The key drawback in working with exponents (77) or (89) is the fact that they modify the primitive fields (69)–(71). From the general transformed equations (74)–(76), we immediately note that the homogeneous part of the equations is form-invariant. This allows for straightforward numerical implementations of the transformed equations for any value of the scaling exponents $\alpha$ and $\beta$ treating the right hand side terms in Equations (74)–(76) as sources. The equations no longer take the form of conservation laws, but this is not a problem. In most practical situations this is a necessity. For example, if systems are governed by a non-polytropic equation of state, then we must work with source terms irrespective of the choice of the scaling exponents. We may as well work with the primitive fields as simulation variables, that is, we choose

$$\begin{array}{cccc}\hfill & \alpha =0,\hfill & \hfill \beta =0& .\hfill \end{array}$$

(92)

The use of primitive fields as simulation variables has the advantage of direct interpretation.

4.4. Numerical Results

In numerical work, source terms are frequently treated. Depending on the problem under consideration, source terms representing gravitational forces, electromagnetic forces, energy release due to radiation, etc are routinely added. Therefore, numerical computation in a moving frame can be performed at virtually no extra technical complication and at no extra computational cost. Poludnenko and Khokhlov mostly focus on tests of moving frame formulation of the fluid flow Equations (74)–(76) with zero exponents (92). They only briefly discuss other choices of scaling parameters. They perform their numerical simulations in a variety of frames for diverse physical problems. The details can be found in their paper. The key points are summarised as follows:

• They consider several types of non-inertial reference frames: accelerating, expanding, contracting, oscillating (sinusoidal) reference frames, etc.
• They treat in detail simulations of blast solutions (e.g., Sedov solution), converging shock solutions (e.g., Guderley blast wave solution), problems involving expansion of a gas sphere in vacuum, etc. They work in different reference frames best suited for the problem at hand.
• They note that the computation in moving frames does not introduce systematic errors. Numerical solutions properly converge to the exact ones when they are known, e.g., the Sedov solution.
• The method accuracy is valid even when solving the fluid equations for non-zero values of the exponents $\alpha$ and $\beta$.

There are some problems for which numerical simulations in non-inertial frames are not ideally suited. Such problems typically involve stationary regions of fluids in an inertial frame. The canonical example being the strong explosion in an otherwise stationary environment. (Expanding or collapsing environments where the ambient conditions are vacuous or dynamically unimportant can be optimally treated in non-inertial frames. In such problems, the ambient fluid can be set to be stationary in the computational–non-inertial–frame.) However, the demonstration in [6] that the numerical solution converges to the correct analytic Sedov solution is a crucial test of the method. The success of the simulation clearly shows that the non-conservative nature of the method does not introduce systematic errors and the Rankine–Hugoniot conditions are valid in the transformed reference frame too. The Rankine–Hugoniot conditions were shown to be valid in a subclass of transformed reference frames with scaling exponents Equation (77) in [5], as reviewed above. Further generalisation for different scaling exponents has not yet been carried out; however, given the numerical results it is likely that a useful formulation exists in more general situations.

5. Conclusions

In this article, we reviewed that the maximal kinematical invariance group of the Euler equations of fluid dynamics is larger than the Galilei group. Specifically, the inversion transformation $(\Sigma :t\to -1/t,\overrightarrow{x}\to \overrightarrow{x}/t)$ leaves the Euler equations invariant. This duality has been used to explain the striking similarities observed in simulations of the supernova explosions and laboratory implosions induced in plasma by intense lasers. It is quite remarkable that the inversion symmetry extends to discontinuous fluid flows as well. We also reviewed how this comes about.

We summarised the work of Poludnenko and Khokhlov [6]. They presented methods for computation of fluid flows characterised by large degree of expansion or contraction. The key idea is the transformation to a non-inertial reference frame. The scaling transformation of the primitive fluid variables provides a degree of flexibility. The use of non-inertial frames often leads to non-conservative formulation of the fluid equations, however, this does not affect the accuracy of the numerical work. For many problems of astrophysical and geophysical interests, going to an appropriate non-inertial frame allows for a cleaner extraction of relevant physics. We focused on [6] because of its close connection to the invariance properties of fluid equations.

There are several other papers addressing these issues, see, for example [15,16] and references therein. Similar ideas are frequently used in simulations of galaxies and the large-scale structure in an expanding universe and in atmospheric simulations.