Lattice Computations for Beyond Standard Model Physics
Abstract
1. Introduction
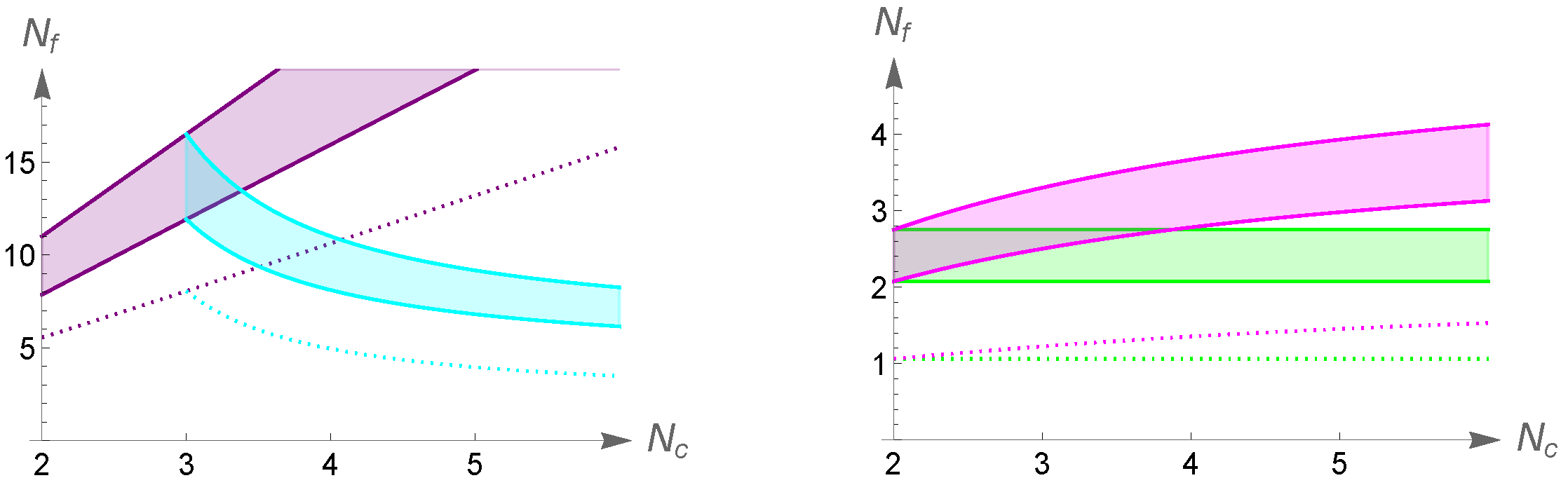
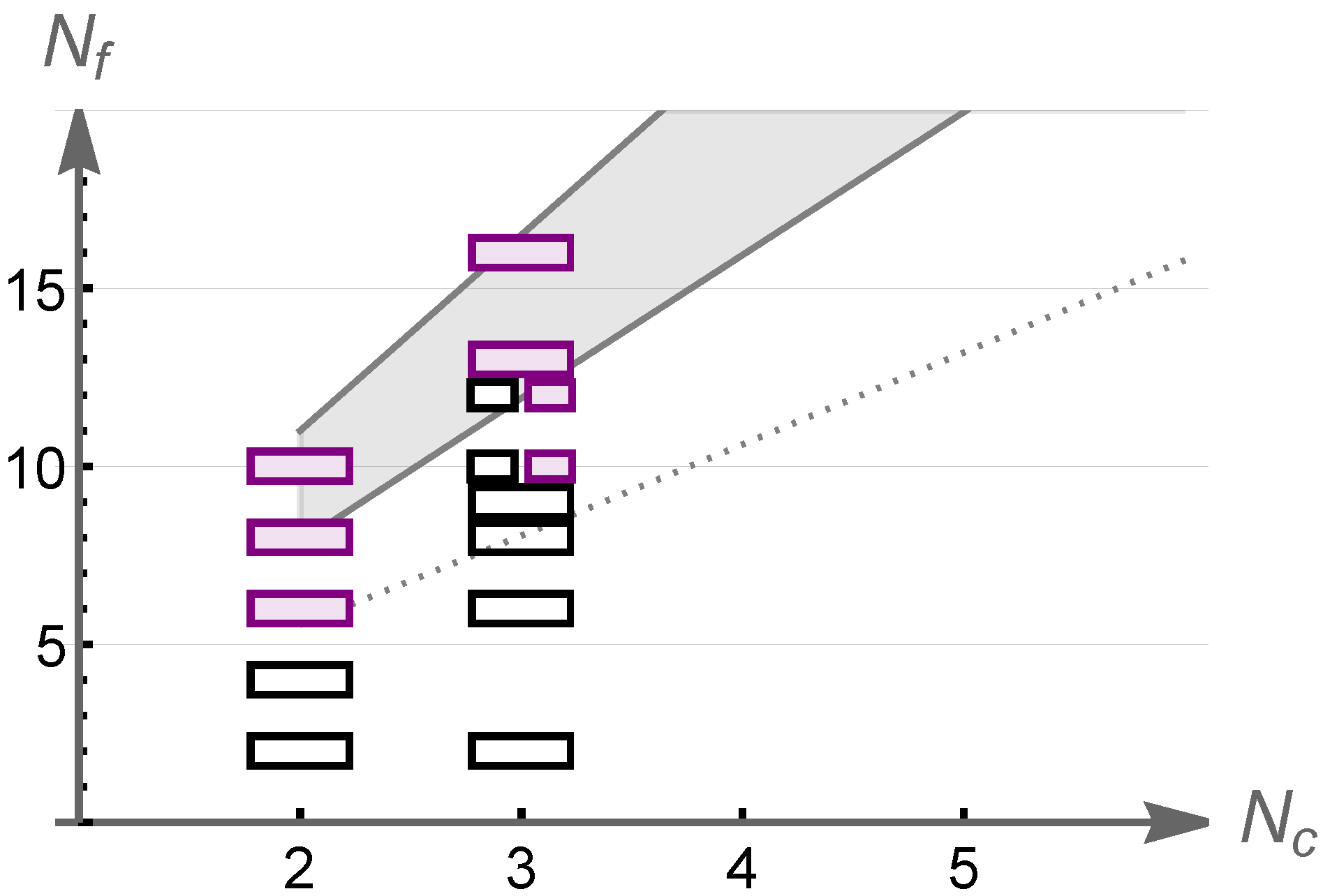
2. Estimates of Gauge Theory Phase Diagrams
3. The Lattice Formulation
3.1. The Lattice Action
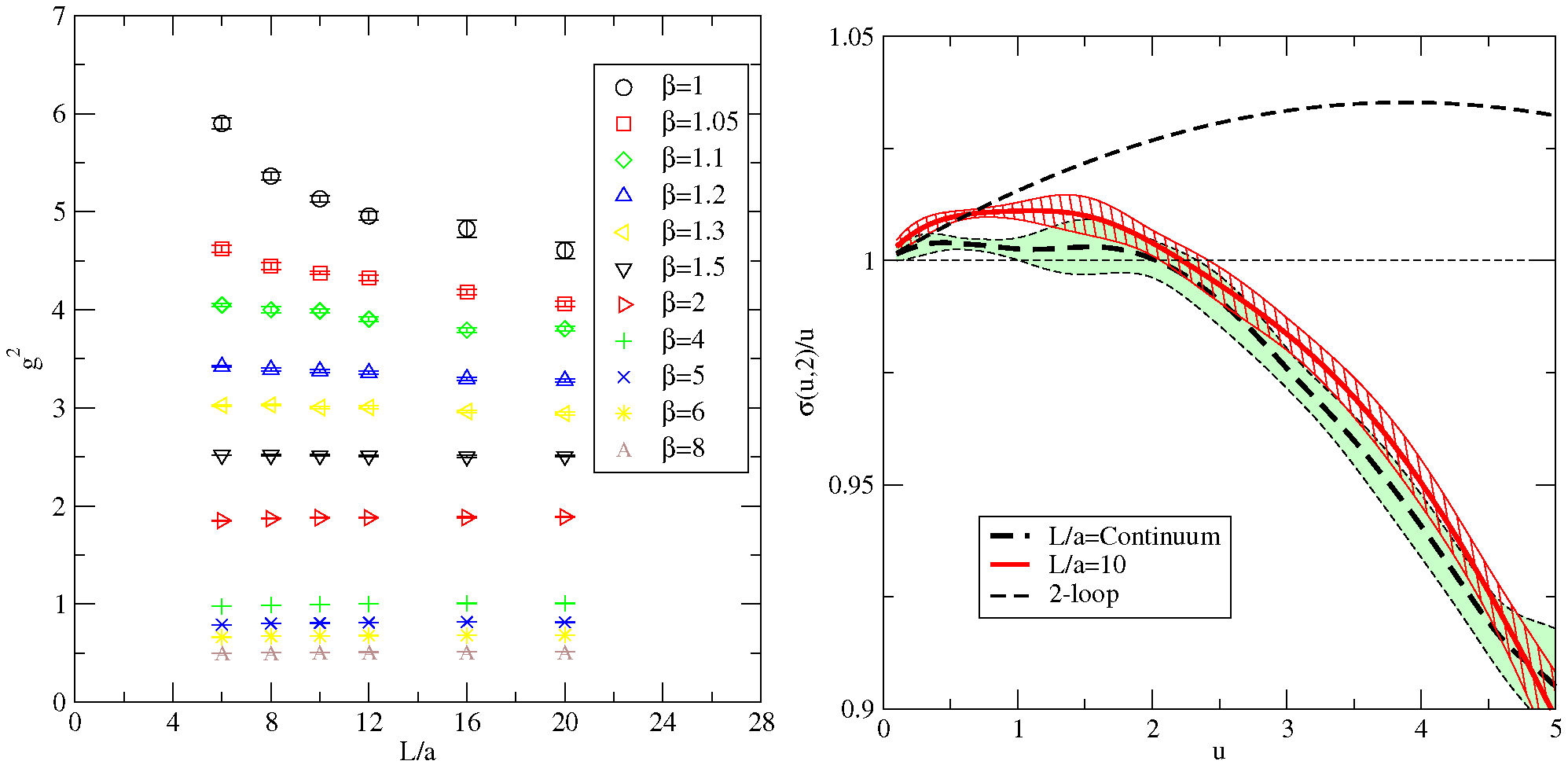
3.2. Measurement of the Coupling
3.2.1. Schrödinger Functional Method
3.2.2. Gradient Flow Method
3.3. Step Scaling Analysis
3.4. Determination of Anomalous Dimensions
3.4.1. The Fermion Mass Anomalous Dimension
3.4.2. The Leading Irrelevant Exponent
4. Case Study: SU(2) Gauge Theory with Fermions on the Lattice
4.1. Fermions in the Adjoint Representation
4.2. Fermions in the Fundamental Representation
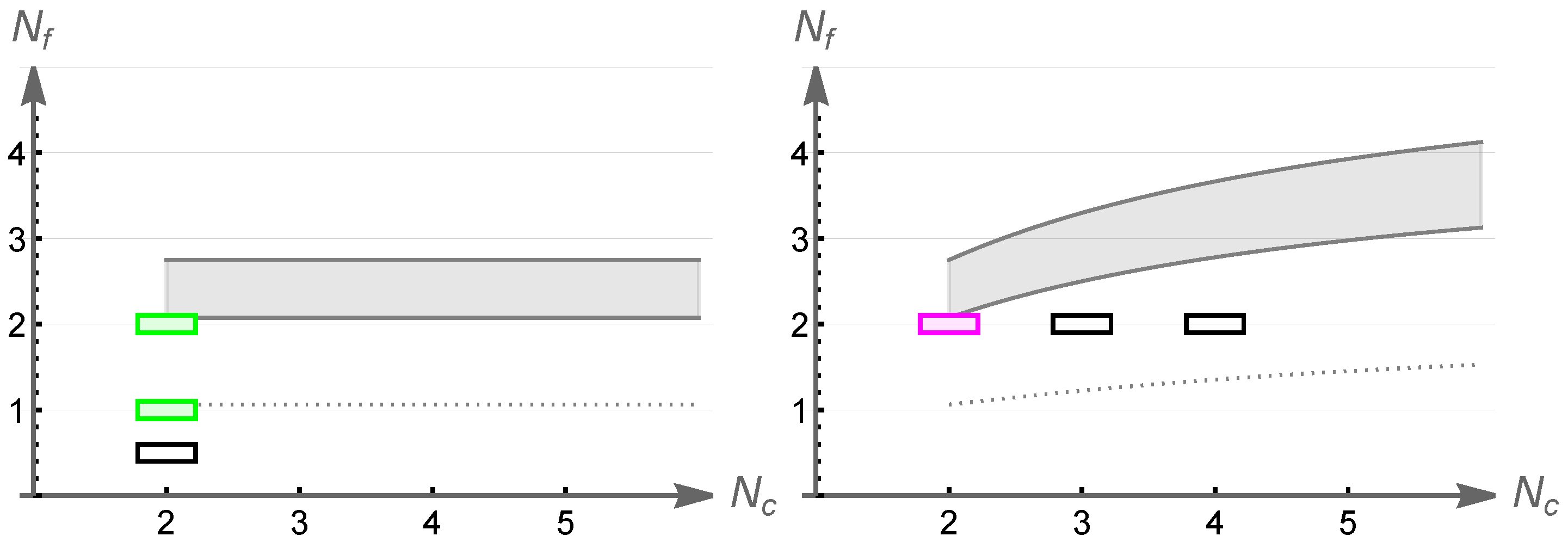
5. Overview of Results for Different Gauge Groups
6. Conclusions and Outlook
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, C.N.; Mills, R.L. Conservation of Isotopic Spin and Isotopic Gauge Invariance. Phys. Rev. 1954, 96, 191–195. [Google Scholar] [CrossRef]
- Gross, D.J.; Wilczek, F. Ultraviolet Behavior of Nonabelian Gauge Theories. Phys. Rev. Lett. 1973, 30, 1343–1346. [Google Scholar] [CrossRef]
- Politzer, H.D. Reliable Perturbative Results for Strong Interactions? Phys. Rev. Lett. 1973, 30, 1346–1349. [Google Scholar] [CrossRef]
- Smith, C.H.L. Inelastic lepton scattering in gluon models. Phys. Rev. D 1971, 4, 2392. [Google Scholar] [CrossRef]
- Gross, D.J. How to Test Scaling in Asymptotically Free Theories. Phys. Rev. Lett. 1974, 32, 1071. [Google Scholar] [CrossRef]
- Feynman, R.P. The behavior of hadron collisions at extreme energies. Conf. Proc. C 1969, 690905, 237–258. [Google Scholar]
- Brock, R.L.; Collins, J.; Huston, J.; Kuhlmann, S.E.; Mishra, S.; Morfín, J.G.; Olness, F.I.; Owens, J.; Pumplin, J.; Iu, J.W.; et al. Handbook of Perturbative QCD, Version 1.1. Rev. Mod. Phys. 1995, 67, 157–248. [Google Scholar]
- Particle Data Group; Zyla, P.A.; Barnett, R.M.; Beringer, J.; Dahl, O.; Dwyer, D.A.; Groom, D.E.; Lin, C.-J.; Lugovsky, K.S.; Pianori, E.; et al. Review of Particle Physics. Prog. Theor. Exp. Phys. 2020, 2020, 083C01. [Google Scholar] [CrossRef]
- Wilson, K.G. Confinement of Quarks. Phys. Rev. D 1974, 10, 2445–2459. [Google Scholar] [CrossRef]
- DeGrand, T.; Detar, C.E. Lattice Methods for Quantum Chromodynamics; World Scientific: Singapore, 2006. [Google Scholar]
- Weinberg, S. Implications of Dynamical Symmetry Breaking. Phys. Rev. D 1976, 13, 974–996. [Google Scholar] [CrossRef]
- Susskind, L. Dynamics of Spontaneous Symmetry Breaking in the Weinberg-Salam Theory. Phys. Rev. D 1979, 20, 2619–2625. [Google Scholar] [CrossRef]
- Holdom, B. Raising Condensates Beyond the Ladder. Phys. Lett. B 1988, 213, 365–369. [Google Scholar] [CrossRef]
- Hill, C.T.; Simmons, E.H. Strong Dynamics and Electroweak Symmetry Breaking. Phys. Rept. 2003, 381, 235–402, Erratum in Phys. Rept. 2004, 390, 553–554. [Google Scholar] [CrossRef]
- Sannino, F. Dynamical Stabilization of the Fermi Scale: Phase Diagram of Strongly Coupled Theories for (Minimal) Walking Technicolor and Unparticles. arXiv 2008, arXiv:0804.0182. [Google Scholar]
- Kribs, G.D.; Neil, E.T. Review of strongly-coupled composite dark matter models and lattice simulations. Int. J. Mod. Phys. A 2016, 31, 1643004. [Google Scholar] [CrossRef]
- Intriligator, K.A.; Seiberg, N. Lectures on supersymmetric gauge theories and electric-magnetic duality. Nucl. Phys. B Proc. Suppl. 1996, 45BC, 1–28. [Google Scholar] [CrossRef]
- Peskin, M.E. Duality in supersymmetric Yang-Mills theory. arXiv 1997, arXiv:hep-th/9702094. [Google Scholar]
- Appelquist, T.; Lane, K.D.; Mahanta, U. On the Ladder Approximation for Spontaneous Chiral Symmetry Breaking. Phys. Rev. Lett. 1988, 61, 1553. [Google Scholar] [CrossRef] [PubMed]
- Cohen, A.G.; Georgi, H. Walking Beyond the Rainbow. Nucl. Phys. B 1989, 314, 7–24. [Google Scholar] [CrossRef]
- Banks, T.; Zaks, A. On the Phase Structure of Vector-Like Gauge Theories with Massless Fermions. Nucl. Phys. B 1982, 196, 189–204. [Google Scholar] [CrossRef]
- Sannino, F.; Tuominen, K. Orientifold theory dynamics and symmetry breaking. Phys. Rev. D 2005, 71, 051901. [Google Scholar] [CrossRef]
- Dietrich, D.D.; Sannino, F. Conformal window of SU(N) gauge theories with fermions in higher dimensional representations. Phys. Rev. D 2007, 75, 085018. [Google Scholar] [CrossRef]
- DeGrand, T.; Shamir, Y.; Svetitsky, B. Infrared fixed point in SU(2) gauge theory with adjoint fermions. Phys. Rev. D 2011, 83, 074507. [Google Scholar] [CrossRef]
- Capitani, S.; Durr, S.; Hoelbling, C. Rationale for UV-filtered clover fermions. J. High Energy Phys. 2006, 11, 028. [Google Scholar] [CrossRef]
- Shamir, Y.; Svetitsky, B.; Yurkovsky, E. Improvement via hypercubic smearing in triplet and sextet QCD. Phys. Rev. D 2011, 83, 097502. [Google Scholar] [CrossRef]
- Luscher, M.; Sommer, R.; Weisz, P.; Wolff, U. A Precise determination of the running coupling in the SU(3) Yang-Mills theory. Nucl. Phys. B 1994, 413, 481–502. [Google Scholar] [CrossRef]
- Luscher, M.; Weisz, P. O(a) improvement of the axial current in lattice QCD to one loop order of perturbation theory. Nucl. Phys. B 1996, 479, 429–458. [Google Scholar] [CrossRef]
- Luscher, M.; Narayanan, R.; Weisz, P.; Wolff, U. The Schrödinger functional: A Renormalizable probe for nonAbelian gauge theories. Nucl. Phys. B 1992, 384, 168–228. [Google Scholar] [CrossRef]
- Jansen, K.; Sommer, R. O(a) improvement of lattice QCD with two flavors of Wilson quarks. Nucl. Phys. B 1998, 530, 185–203, Erratum in Nucl. Phys. B 2002, 643, 517–518. [Google Scholar] [CrossRef]
- Della Morte, M.; Frezzotti, R.; Heitger, J.; Rolf, J.; Sommer, R.; Wolff, U.; Alpha Collaboration. Computation of the strong coupling in QCD with two dynamical flavors. Nucl. Phys. B 2005, 713, 378–406. [Google Scholar] [CrossRef][Green Version]
- Lüscher, M. Properties and uses of the Wilson flow in lattice QCD. J. High Energy Phys. 2010, 8, 071, Erratum in J. High Energy Phys. 2014, 3, 092. [Google Scholar] [CrossRef]
- Ramos, A. The Yang-Mills gradient flow and renormalization. arXiv 2015, arXiv:1506.00118. [Google Scholar]
- Narayanan, R.; Neuberger, H. Infinite N phase transitions in continuum Wilson loop operators. J. High Energy Phys. 2006, 3, 064. [Google Scholar] [CrossRef]
- Luscher, M. Trivializing maps, the Wilson flow and the HMC algorithm. Commun. Math. Phys. 2010, 293, 899–919. [Google Scholar] [CrossRef]
- Luscher, M.; Weisz, P. Perturbative analysis of the gradient flow in non-abelian gauge theories. J. High Energy Phys. 2011, 2, 051. [Google Scholar] [CrossRef]
- Luscher, M.; Weisz, P. On-Shell Improved Lattice Gauge Theories. Commun. Math. Phys. 1985, 97, 59, Erratum in Commun. Math. Phys. 1985, 98, 433. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Nogradi, D.; Wong, C.H. The Yang-Mills gradient flow in finite volume. J. High Energy Phys. 2012, 11, 007. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Nogradi, D.; Wong, C.H. The gradient flow running coupling scheme. arXiv 2012, arXiv:1211.3247. [Google Scholar]
- Fritzsch, P.; Ramos, A. The gradient flow coupling in the Schrödinger Functional. J. High Energy Phys. 2013, 10, 008. [Google Scholar] [CrossRef]
- Lüscher, M. Step scaling and the Yang-Mills gradient flow. J. High Energy Phys. 2014, 6, 105. [Google Scholar] [CrossRef]
- Capitani, S.; Lüscher, M.; Sommer, R.; Wittig, H. Non-perturbative quark mass renormalization in quenched lattice QCD. Nucl. Phys. B 1999, 544, 669–698, Erratum in Nucl. Phys. B 2000, 582, 762–762. [Google Scholar] [CrossRef][Green Version]
- Della Morte, M.; Hoffmann, R.; Knechtli, F.; Rolf, J.; Sommer, R.; Wetzorke, I.; Wolffa, U.; Alpha Collaboration. Non-perturbative quark mass renormalization in two-flavor QCD. Nucl. Phys. B 2005, 729, 117–134. [Google Scholar] [CrossRef][Green Version]
- Del Debbio, L.; Zwicky, R. Hyperscaling relations in mass-deformed conformal gauge theories. Phys. Rev. D 2010, 82, 014502. [Google Scholar] [CrossRef]
- Giusti, L.; Luscher, M. Chiral symmetry breaking and the Banks-Casher relation in lattice QCD with Wilson quarks. J. High Energy Phys. 2009, 3, 013. [Google Scholar] [CrossRef]
- Patella, A. GMOR-like relation in IR-conformal gauge theories. Phys. Rev. D 2011, 84, 125033. [Google Scholar] [CrossRef]
- Hietanen, A.J.; Rantaharju, J.; Rummukainen, K.; Tuominen, K. Spectrum of SU(2) lattice gauge theory with two adjoint Dirac flavours. J. High Energy Phys. 2009, 5, 025. [Google Scholar] [CrossRef]
- Hietanen, A.J.; Rummukainen, K.; Tuominen, K. Evolution of the coupling constant in SU(2) lattice gauge theory with two adjoint fermions. Phys. Rev. D 2009, 80, 094504. [Google Scholar] [CrossRef]
- Rantaharju, J.; Rantalaiho, T.; Rummukainen, K.; Tuominen, K. Running coupling in SU(2) gauge theory with two adjoint fermions. Phys. Rev. D 2016, 93, 094509. [Google Scholar] [CrossRef]
- Rantaharju, J. Gradient Flow Coupling in the SU(2) gauge theory with two adjoint fermions. Phys. Rev. D 2016, 93, 094516. [Google Scholar] [CrossRef]
- Karavirta, T.; Rantaharju, J.; Rummukainen, K.; Tuominen, K. Determining the conformal window: SU(2) gauge theory with Nf = 4, 6 and 10 fermion flavours. J. High Energy Phys. 2012, 5, 003. [Google Scholar] [CrossRef]
- Leino, V.; Rantaharju, J.; Rantalaiho, T.; Rummukainen, K.; Suorsa, J.M.; Tuominen, K. The gradient flow running coupling in SU(2) gauge theory with Nf = 8 fundamental flavors. Phys. Rev. D 2017, 95, 114516. [Google Scholar] [CrossRef]
- Leino, V.; Rummukainen, K.; Suorsa, J.M.; Tuominen, K.; Tähtinen, S. Infrared fixed point of SU(2) gauge theory with six flavors. Phys. Rev. D 2018, 97, 114501. [Google Scholar] [CrossRef]
- Leino, V.; Rummukainen, K.; Tuominen, K. Slope of the beta function at the fixed point of SU(2) gauge theory with six or eight flavors. Phys. Rev. D 2018, 98, 054503. [Google Scholar] [CrossRef]
- Omelyan, I.P.; Mryglod, I.M.; Folk, R. Symplectic analytically integrable decomposition algorithms: Classification, derivation, and application to molecular dynamics, quantum and celestial mechanics simulations. Comput. Phys. Commun. 2003, 151, 272–314. [Google Scholar] [CrossRef]
- Takaishi, T.; de Forcrand, P. Testing and tuning new symplectic integrators for hybrid Monte Carlo algorithm in lattice QCD. Phys. Rev. E 2006, 73, 036706. [Google Scholar] [CrossRef]
- Brower, R.C.; Ivanenko, T.; Levi, A.R.; Orginos, K.N. Chronological inversion method for the Dirac matrix in hybrid Monte Carlo. Nucl. Phys. B 1997, 484, 353–374. [Google Scholar] [CrossRef]
- Hasenbusch, M.; Jansen, K. Speeding up the Hybrid Monte Carlo algorithm for dynamical fermions. Nucl. Phys. B Proc. Suppl. 2002, 106, 1076–1078. [Google Scholar] [CrossRef]
- Hasenbusch, M.; Jansen, K. Speeding up lattice QCD simulations with clover improved Wilson fermions. Nucl. Phys. B 2003, 659, 299–320. [Google Scholar] [CrossRef][Green Version]
- Catterall, S.; Sannino, F. Minimal walking on the lattice. Phys. Rev. D 2007, 76, 034504. [Google Scholar] [CrossRef]
- Del Debbio, L.; Patella, A.; Pica, C. Higher representations on the lattice: Numerical simulations. SU(2) with adjoint fermions. Phys. Rev. D 2010, 81, 094503. [Google Scholar] [CrossRef]
- del Debbio, L.; Lucini, B.; Patella, A.; Pica, C.; Rago, A. Conformal versus confining scenario in SU(2) with adjoint fermions. Phys. Rev. D 2009, 80, 074507. [Google Scholar] [CrossRef]
- Bursa, F.; del Debbio, L.; Keegan, L.; Pica, C.; Pickup, T. Mass anomalous dimension in SU(2) with two adjoint fermions. Phys. Rev. D 2010, 81, 014505. [Google Scholar] [CrossRef]
- Patella, A.; del Debbio, L.; Lucini, B.; Pica, C.; Rago, A. Confining vs. conformal scenario for SU(2) with adjoint fermions. Gluonic observables. arXiv 2010, arXiv:1011.0864. [Google Scholar]
- Bursa, F.; del Debbio, L.; Henty, D.; Kerrane, E.; Lucini, B.; Patella, A.; Pica, C.; Pickup, T.; Rago, A. Improved Lattice Spectroscopy of Minimal Walking Technicolor. Phys. Rev. D 2011, 84, 034506. [Google Scholar] [CrossRef]
- Patella, A. A precise determination of the psibar-psi anomalous dimension in conformal gauge theories. Phys. Rev. D 2012, 86, 025006. [Google Scholar] [CrossRef]
- Ramos, A.; Sint, S. Symanzik improvement of the gradient flow in lattice gauge theories. Eur. Phys. J. C 2016, 76, 15. [Google Scholar] [CrossRef]
- Cheng, A.; Hasenfratz, A.; Liu, Y.; Petropoulos, G.; Schaich, D. Improving the continuum limit of gradient flow step scaling. J. High Energy Phys. 2014, 5, 137. [Google Scholar] [CrossRef]
- Arthur, R.; Drach, V.; Hansen, M.; Hietanen, A.; Pica, C.; Sannino, F. SU(2) gauge theory with two fundamental flavors: A minimal template for model building. Phys. Rev. D 2016, 94, 094507. [Google Scholar] [CrossRef]
- Arthur, R.; Drach, V.; Hietanen, A.; Pica, C.; Sannino, F. SU(2) Gauge Theory with Two Fundamental Flavours: Scalar and Pseudoscalar Spectrum. arXiv 2016, arXiv:1607.06654. [Google Scholar]
- Amato, A.; Leino, V.; Rummukainen, K.; Tuominen, K.; Tähtinen, S. From chiral symmetry breaking to conformality in SU(2) gauge theory. arXiv 2018, arXiv:1806.07154. [Google Scholar]
- Appelquist, T.; Avakian, A.; Babich, R.; Brower, R.C.; Cheng, M.; Clark, M.A.; Cohen, G.T.; Fleming, J.; Kiskis, E.T.; Neil, J.C.; et al. Toward TeV Conformality. Phys. Rev. Lett. 2010, 104, 071601. [Google Scholar] [CrossRef] [PubMed]
- Appelquist, T.; Fleming, G.T.; Neil, E.T. Lattice Study of Conformal Behavior in SU(3) Yang-Mills Theories. Phys. Rev. D 2009, 79, 076010. [Google Scholar] [CrossRef]
- Appelquist, T.; Brower, R.C.; Fleming, G.T.; Kiskis, J.; Lin, M.F.; Neil, E.T.; Osborn, J.; Rebbi, C.; Rinaldi, E.; Schaich, D.; et al. Lattice simulations with eight flavors of domain wall fermions in SU(3) gauge theory. Phys. Rev. D 2014, 90, 114502. [Google Scholar] [CrossRef]
- Hasenfratz, A.; Schaich, D.; Veernala, A. Nonperturbative β function of eight-flavor SU(3) gauge theory. J. High Energy Phys. 2015, 6, 143. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Mondal, S.; Nogradi, D.; Wong, C.H. The running coupling of 8 flavors and 3 colors. J. High Energy Phys. 2015, 6, 019. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Nogradi, D.; Schroeder, C. Chiral symmetry breaking in nearly conformal gauge theories. arXiv 2009, arXiv:0911.2463. [Google Scholar]
- Hayakawa, M.; Ishikawa, K.I.; Osaki, Y.; Takeda, S.; Uno, S.; Yamada, N. Running coupling constant of ten-flavor QCD with the Schrödinger functional method. Phys. Rev. D 2011, 83, 074509. [Google Scholar] [CrossRef]
- Appelquist, T.; Brower, R.C.; Buchoff, M.I.; Cheng, M.; Cohen, S.D.; Fleming, G.T.; Kiskis, J.; Lin, M.; Na, H.; Neil, E.T.; et al. Approaching Conformality with Ten Flavors. arXiv 2012, arXiv:1204.6000. [Google Scholar]
- Hasenfratz, A.; Rebbi, C.; Witzel, O. Gradient flow step-scaling function for SU(3) with ten fundamental flavors. Phys. Rev. D 2020, 101, 114508. [Google Scholar] [CrossRef]
- Appelquist, T.; Brower, R.C.; Cushman, K.K.; Fleming, G.T.; Gasbarro, A.D.; Hasenfratz, A.; Jin, X.-Y.; Neil, E.T.; Osborn, J.C.; Rebbi, C.; et al. Near-conformal dynamics in a chirally broken system. Phys. Rev. D 2021, 103, 014504. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Nogradi, D.; Wong, C.H. Fate of a recent conformal fixed point and β-function in the SU(3) BSM gauge theory with ten massless flavors. arXiv 2018, arXiv:1812.03972. [Google Scholar]
- Hasenfratz, A.; Schaich, D. Nonperturbative β function of twelve-flavor SU(3) gauge theory. J. High Energy Phys. 2018, 2, 132. [Google Scholar] [CrossRef]
- Hasenfratz, A.; Rebbi, C.; Witzel, O. Gradient flow step-scaling function for SU(3) with twelve flavors. Phys. Rev. D 2019, 100, 114508. [Google Scholar] [CrossRef]
- Lin, C.J.D.; Ogawa, K.; Ohki, H.; Shintani, E. Lattice study of infrared behaviour in SU(3) gauge theory with twelve massless flavours. J. High Energy Phys. 2012, 8, 096. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Nogradi, D.; Schroeder, C.; Holland, K.; Kuti, J.; Nogradi, D.; Schroeder, C. Twelve massless flavors and three colors below the conformal window. Phys. Lett. B 2011, 703, 348–358. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Mondal, S.; Nogradi, D.; Wong, C.H. Fate of the conformal fixed point with twelve massless fermions and SU(3) gauge group. Phys. Rev. D 2016, 94, 091501. [Google Scholar] [CrossRef]
- Appelquist, T.; Fleming, G.T.; Lin, M.F.; Neil, E.T.; Schaich, D.A. Lattice Simulations and Infrared Conformality. Phys. Rev. D 2011, 84, 054501. [Google Scholar] [CrossRef]
- Aoki, Y.; Aoyama, T.; Kurachi, M.; Maskawa, T.; Nagai, K.I.; Ohki, H.; Shibata, A.; Yamawaki, K.; Yamazaki, T. Lattice study of conformality in twelve-flavor QCD. Phys. Rev. D 2012, 86, 054506. [Google Scholar] [CrossRef]
- DeGrand, T. Finite-size scaling tests for spectra in SU(3) lattice gauge theory coupled to 12 fundamental flavor fermions. Phys. Rev. D 2011, 84, 116901. [Google Scholar] [CrossRef]
- Cheng, A.; Hasenfratz, A.; Petropoulos, G.; Schaich, D. Scale-dependent mass anomalous dimension from Dirac eigenmodes. J. High Energy Phys. 2013, 7, 061. [Google Scholar] [CrossRef][Green Version]
- Cheng, A.; Hasenfratz, A.; Liu, Y.; Petropoulos, G.; Schaich, D. Finite size scaling of conformal theories in the presence of a near-marginal operator. Phys. Rev. D 2014, 90, 014509. [Google Scholar] [CrossRef]
- Lombardo, M.P.; Miura, K.; da Silva, T.J.N.; Pallante, E. On the particle spectrum and the conformal window. J. High Energy Phys. 2014, 12, 183. [Google Scholar] [CrossRef]
- Athenodorou, A.; Bennett, E.; Bergner, G.; Lucini, B. Investigating the conformal behavior of SU(2) with one adjoint Dirac flavor. Phys. Rev. D 2021, 104, 074519. [Google Scholar] [CrossRef]
- Del Debbio, L.; Lucini, B.; Patella, A.; Pica, C.; Rago, A. The infrared dynamics of Minimal Walking Technicolor. Phys. Rev. D 2010, 82, 014510. [Google Scholar] [CrossRef]
- Lopez, C.; Bergner, G.; Montvay, I.; Piemonte, S. Measurement of the mass anomalous dimension of near-conformal adjoint QCD with the gradient flow. arXiv 2020, arXiv:2011.02815. [Google Scholar]
- DeGrand, T.; Shamir, Y.; Svetitsky, B. Near the Sill of the Conformal Window: Gauge Theories with Fermions in Two-Index Representations. Phys. Rev. D 2013, 88, 054505. [Google Scholar] [CrossRef]
- DeGrand, T.; Shamir, Y.; Svetitsky, B. Running coupling and mass anomalous dimension of SU(3) gauge theory with two flavors of symmetric-representation fermions. Phys. Rev. D 2010, 82, 054503. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Mondal, S.; Nogradi, D.; Wong, C.H. The running coupling of the minimal sextet composite Higgs model. J. High Energy Phys. 2015, 9, 039. [Google Scholar] [CrossRef]
- Fodor, Z.; Holland, K.; Kuti, J.; Wong, C.H. Tantalizing dilaton tests from a near-conformal EFT. arXiv 2019, arXiv:1901.06324. [Google Scholar]
- DeGrand, T.; Shamir, Y.; Svetitsky, B. SU(4) lattice gauge theory with decuplet fermions: Schrödinger functional analysis. Phys. Rev. D 2012, 85, 074506. [Google Scholar] [CrossRef]
- Cacciapaglia, G.; Pica, C.; Sannino, F. Fundamental Composite Dynamics: A Review. Phys. Rept. 2020, 877, 1–70. [Google Scholar] [CrossRef]
- Dietrich, D.D.; Sannino, F.; Tuominen, K. Light composite Higgs from higher representations versus electroweak precision measurements: Predictions for CERN LHC. Phys. Rev. D 2005, 72, 055001. [Google Scholar] [CrossRef]
- Ayyar, V.; DeGrand, T.; Golterman, M.; Hackett, D.C.; Jay, W.I.; Neil, E.T.; Shamir, Y.; Svetitsky, B. Spectroscopy of SU(4) composite Higgs theory with two distinct fermion representations. Phys. Rev. D 2018, 97, 074505. [Google Scholar] [CrossRef]
- Ayyar, V.; DeGrand, T.; Hackett, D.C.; Jay, W.I.; Neil, E.T.; Shamir, Y.; Svetitsky, B. Finite-temperature phase structure of SU(4) gauge theory with multiple fermion representations. Phys. Rev. D 2018, 97, 114502. [Google Scholar] [CrossRef]
- Cossu, G.; del Debbio, L.; Panero, M.; Preti, D. Strong dynamics with matter in multiple representations: SU(4) gauge theory with fundamental and sextet fermions. Eur. Phys. J. C 2019, 79, 638. [Google Scholar] [CrossRef]
- Leino, V.; Rindlisbacher, T.; Rummukainen, K.; Sannino, F.; Tuominen, K. Safety versus triviality on the lattice. Phys. Rev. D 2020, 101, 074508. [Google Scholar] [CrossRef]
- Rantaharju, J.; Rindlisbacher, T.; Rummukainen, K.; Salami, A.; Tuominen, K. Spectrum of SU(2) gauge theory at large number of flavors. Phys. Rev. D 2021, 104, 114504. [Google Scholar] [CrossRef]

| R | |||
|---|---|---|---|
| G | |||
| F | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rummukainen, K.; Tuominen, K. Lattice Computations for Beyond Standard Model Physics. Universe 2022, 8, 188. https://doi.org/10.3390/universe8030188
Rummukainen K, Tuominen K. Lattice Computations for Beyond Standard Model Physics. Universe. 2022; 8(3):188. https://doi.org/10.3390/universe8030188
Chicago/Turabian StyleRummukainen, Kari, and Kimmo Tuominen. 2022. "Lattice Computations for Beyond Standard Model Physics" Universe 8, no. 3: 188. https://doi.org/10.3390/universe8030188
APA StyleRummukainen, K., & Tuominen, K. (2022). Lattice Computations for Beyond Standard Model Physics. Universe, 8(3), 188. https://doi.org/10.3390/universe8030188






