One-Minute Quasi-Periodic Pulsations during an M-Class Solar Flare
Abstract
1. Introduction
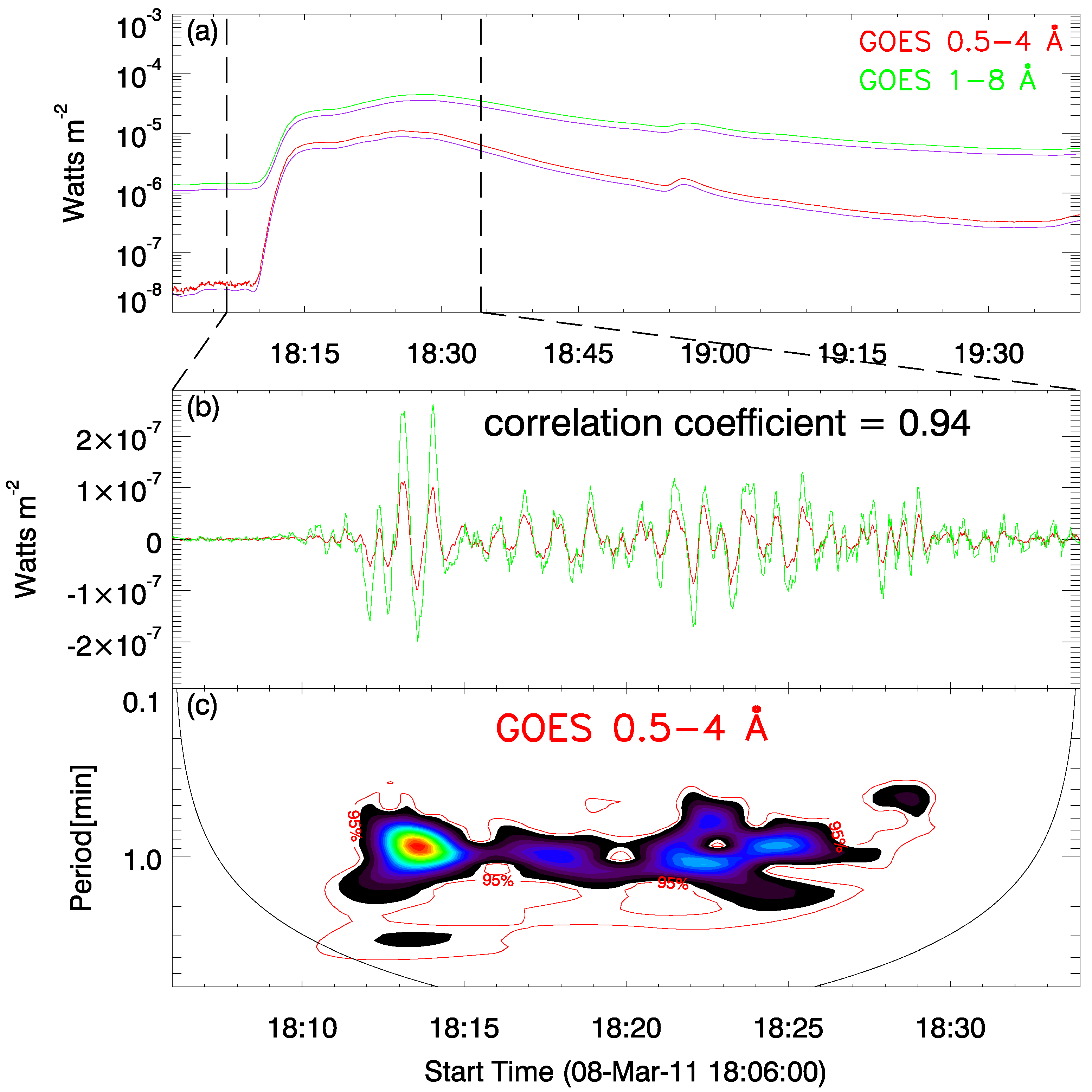
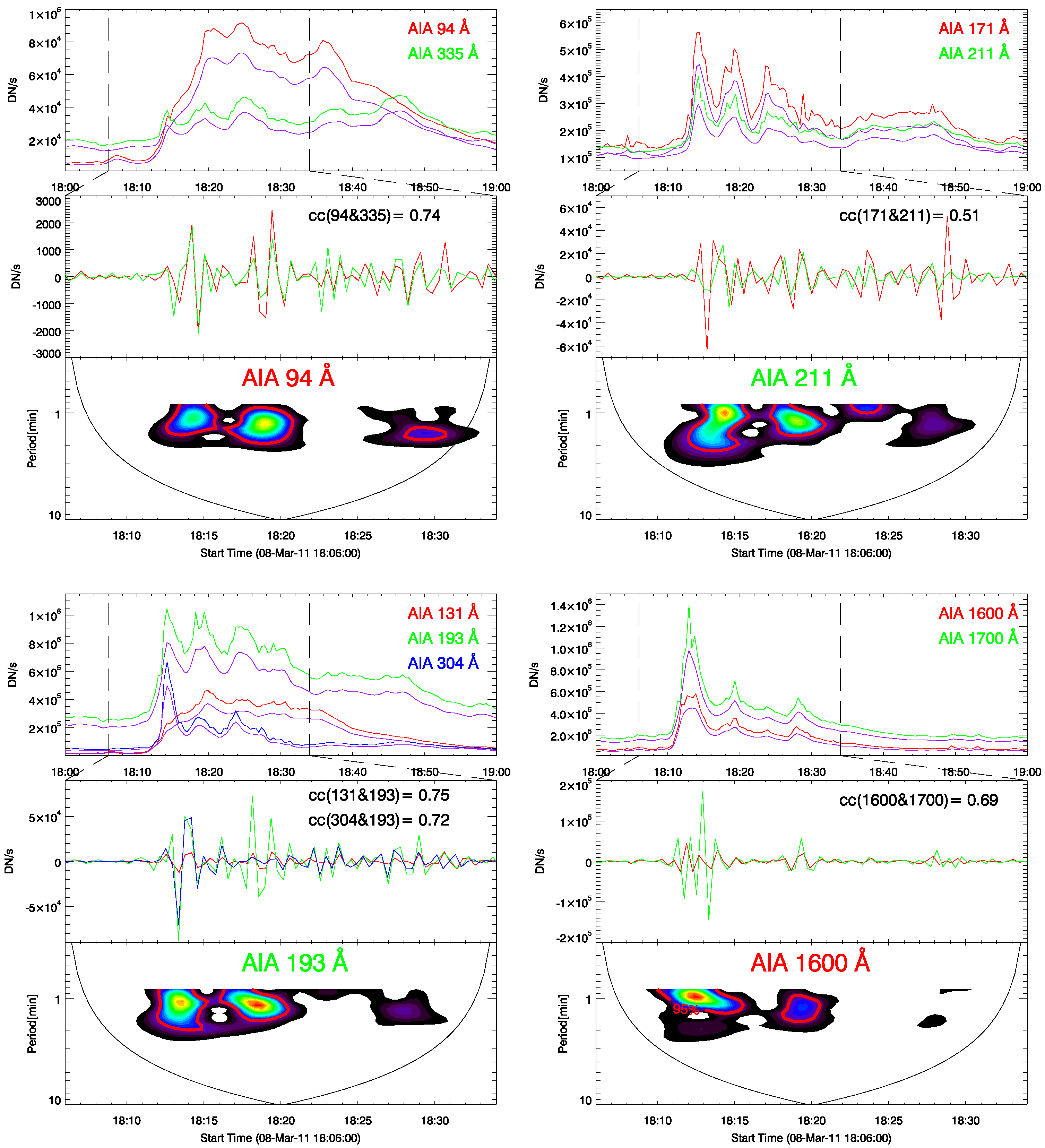
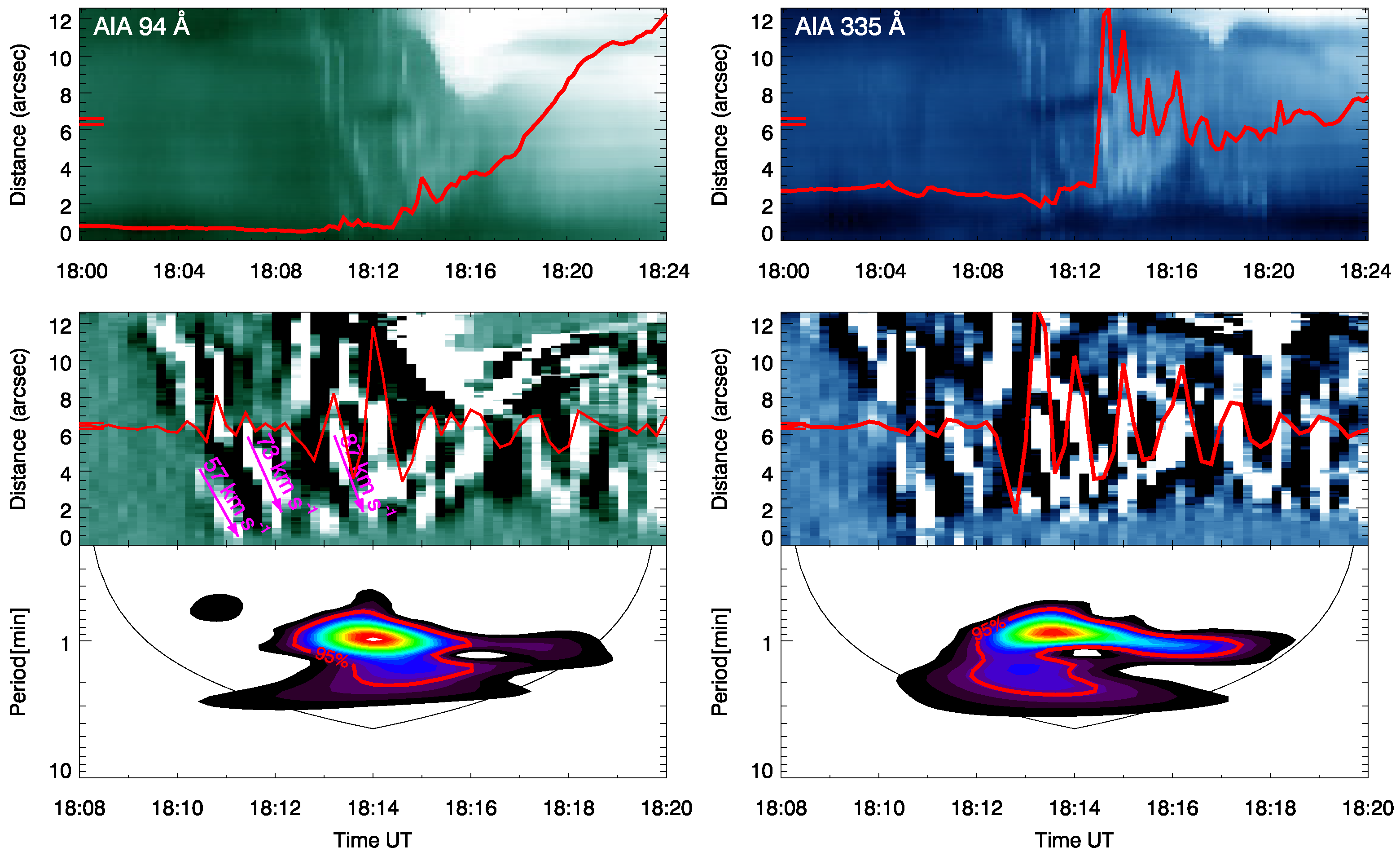
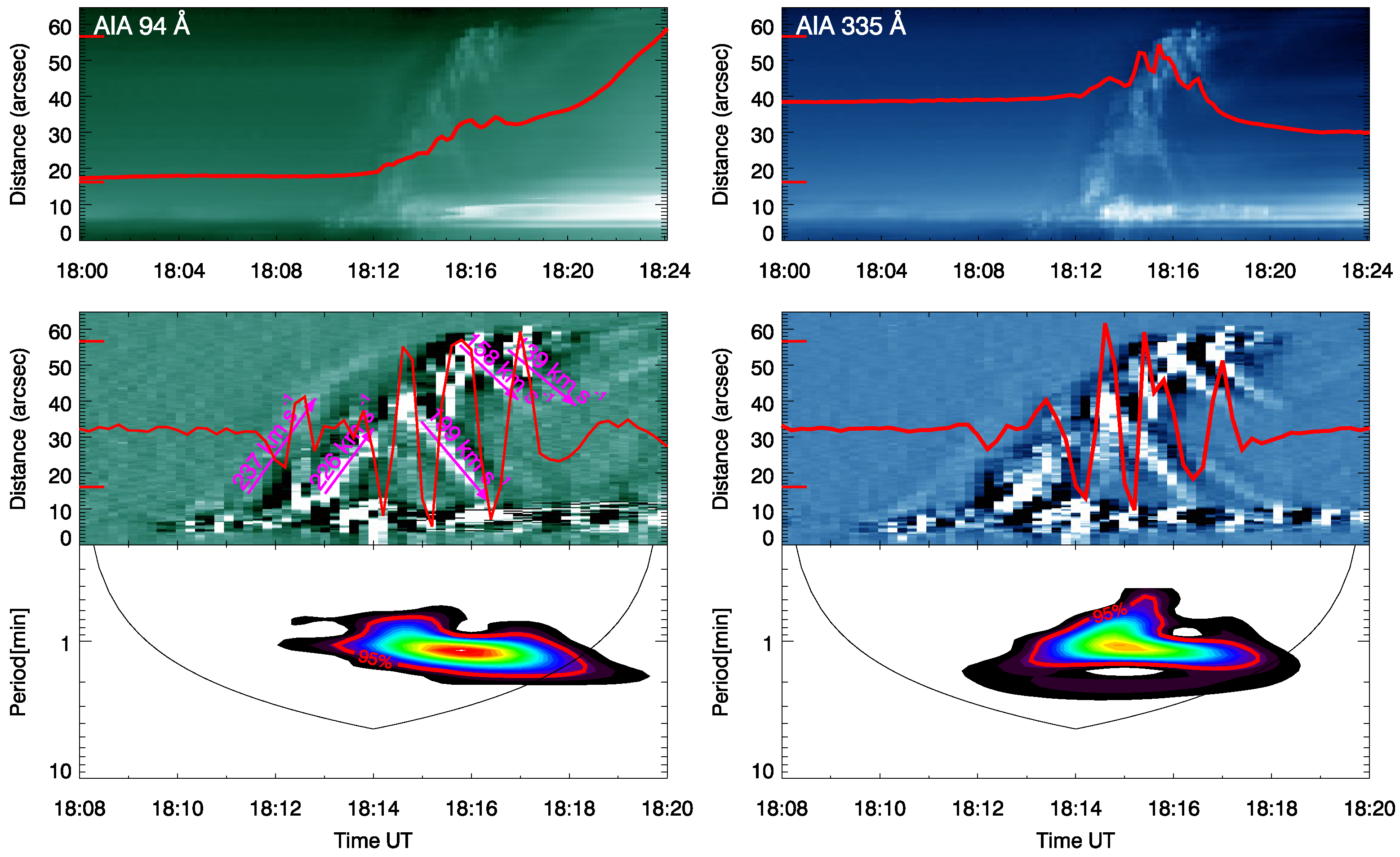
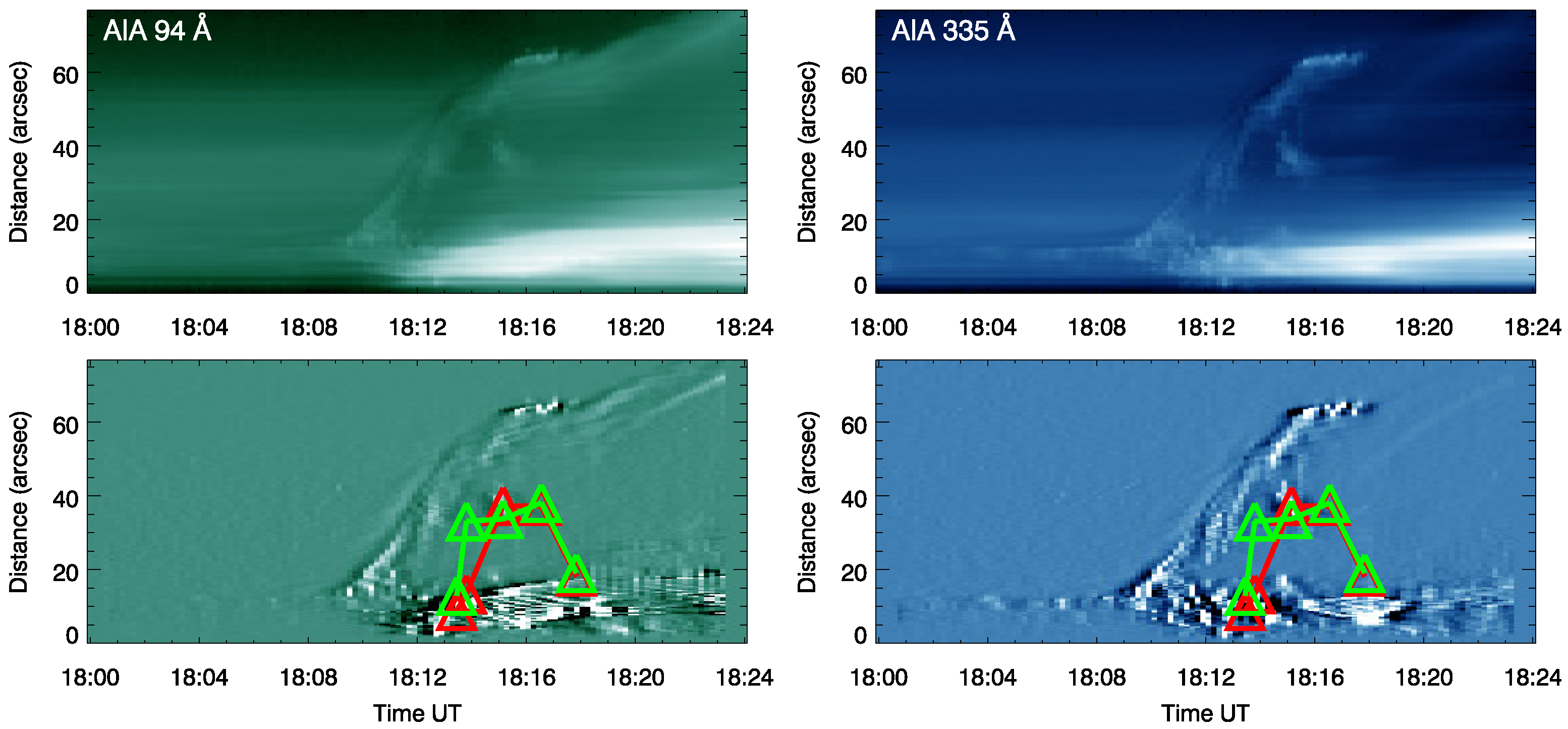
2. Observation and Measurement
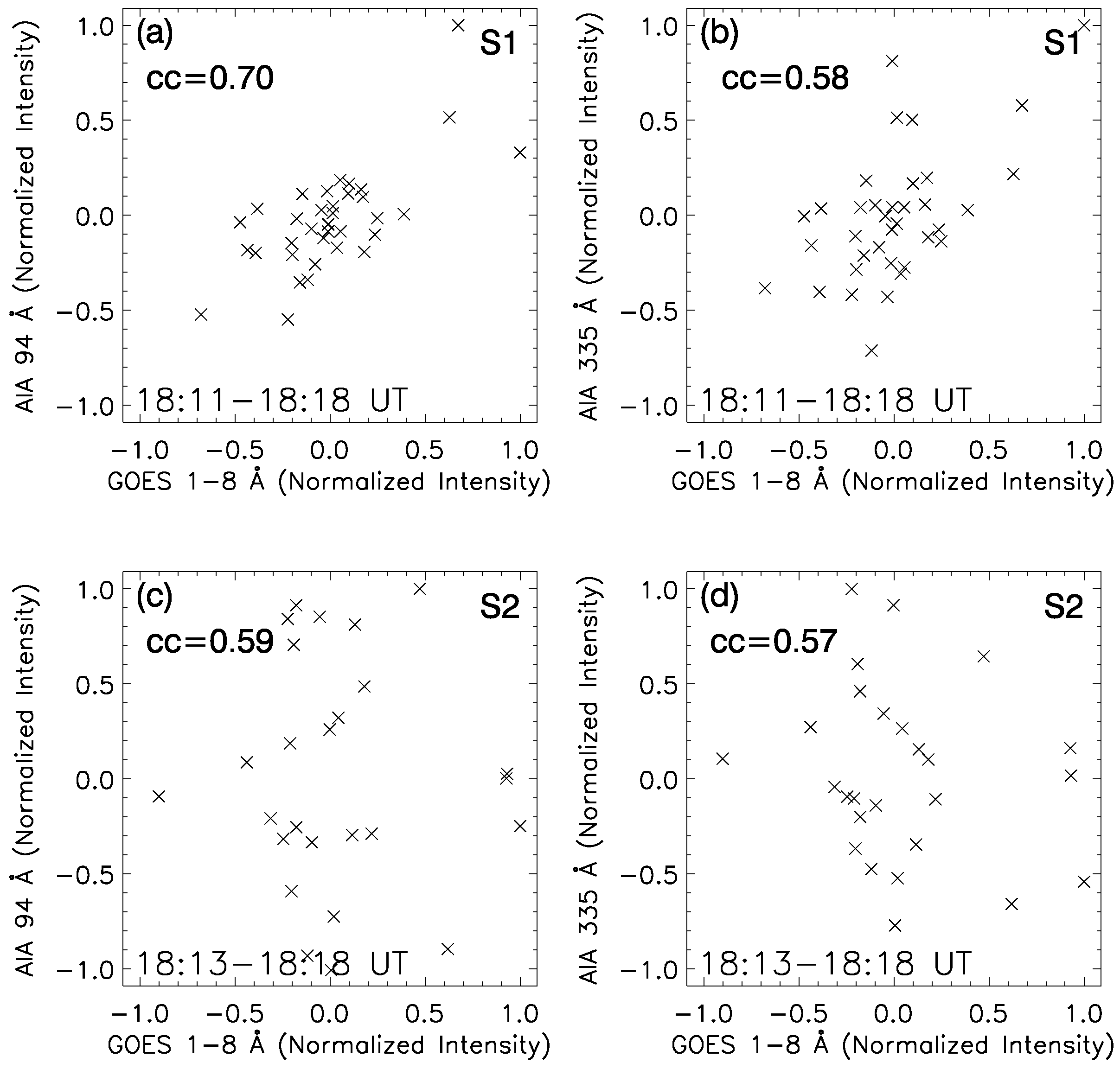
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | https://cdaw.gsfc.nasa.gov/CME_list/UNIVERSAL/2011_03/univ2011_03.html (accessed on 20 January 2022) |
| 2 | https://hesperia.gsfc.nasa.gov/rhessi_extras/flare_images/2011/03/08/20110308_1809_1823/hsi_20110308_1809_1823.html (accessed on 17 November 2021) |
References
- Zimovets, I.V.; McLaughlin, J.A.; Srivastava, A.K.; Kolotkov, D.Y.; Kuznetsov, A.A.; Kupriyanova, E.G.; Cho, I.H.; Inglis, A.R.; Reale, F.; Pascoe, D.J.; et al. Quasi-Periodic Pulsations in Solar and Stellar Flares: A Review of Underpinning Physical Mechanisms and Their Predicted Observational Signatures. Space Sci. Rev. 2021, 217, 66. [Google Scholar] [CrossRef]
- Tan, B.; Zhang, Y.; Tan, C.; Liu, Y. Microwave Quasi-Periodic Pulsations in Multi-timescales Associated with a Solar Flare/CME Event. Astrophys. J. 2010, 723, 25–39. [Google Scholar] [CrossRef][Green Version]
- Inglis, A.R.; Nakariakov, V.M. A multi-periodic oscillatory event in a solar flare. Astron. Astrophys. 2009, 493, 259–266. [Google Scholar] [CrossRef]
- Van Doorsselaere, T.; De Groof, A.; Zender, J.; Berghmans, D.; Goossens, M. LYRA Observations of Two Oscillation Modes in a Single Flare. Astrophys. J. 2011, 740, 90. [Google Scholar] [CrossRef]
- Zimovets, I.V. Harmonic oscillations of the X-ray emission of a solar flare. Astron. Rep. 2010, 54, 657–674. [Google Scholar] [CrossRef]
- Sych, R.; Nakariakov, V.M.; Karlicky, M.; Anfinogentov, S. Relationship between wave processes in sunspots and quasi-periodic pulsations in active region flares. Astron. Astrophys. 2009, 505, 791–799. [Google Scholar] [CrossRef]
- Kashapova, L.K.; Kupriyanova, E.G.; Xu, Z.; Reid, H.A.S.; Kolotkov, D.Y. The origin of quasi-periodicities during circular ribbon flares. Astron. Astrophys. 2020, 642, A195. [Google Scholar] [CrossRef]
- Li, D.; Zhang, Q.M.; Huang, Y.; Ning, Z.J.; Su, Y.N. Quasi-periodic pulsations with periods that change depending on whether the pulsations have thermal or nonthermal components. Astron. Astrophys. 2017, 597, L4. [Google Scholar] [CrossRef][Green Version]
- Li, D.; Lu, L.; Ning, Z.; Feng, L.; Gan, W.; Li, H. Quasi-periodic Pulsation Detected in Lyα Emission During Solar Flares. Astrophys. J. 2020, 893, 7. [Google Scholar] [CrossRef]
- Li, D.; Ge, M.; Dominique, M.; Zhao, H.; Li, G.; Li, X.; Zhang, S.; Lu, F.; Gan, W.; Ning, Z. Detection of Flare Multiperiodic Pulsations in Mid-ultraviolet Balmer Continuum, Lyα, Hard X-Ray, and Radio Emissions Simultaneously. Astrophys. J. 2021, 921, 179. [Google Scholar] [CrossRef]
- Nakariakov, V.M.; Anfinogentov, S.; Storozhenko, A.A.; Kurochkin, E.A.; Bogod, V.M.; Sharykin, I.N.; Kaltman, T.I. Quasi-periodic Pulsations in a Solar Microflare. Astrophys. J. 2018, 859, 154. [Google Scholar] [CrossRef]
- Chen, P.F.; Priest, E.R. Transition-Region Explosive Events: Reconnection Modulated by p-Mode Waves. Sol. Phys. 2006, 238, 313–327. [Google Scholar] [CrossRef]
- Aschwanden, M.J. Theory of Radio Pulsations in Coronal Loops. Sol. Phys. 1987, 111, 113–136. [Google Scholar] [CrossRef]
- Van Doorsselaere, T.; Kupriyanova, E.G.; Yuan, D. Quasi-periodic Pulsations in Solar and Stellar Flares: An Overview of Recent Results (Invited Review). Sol. Phys. 2016, 291, 3143–3164. [Google Scholar] [CrossRef]
- Tian, H.; Young, P.R.; Reeves, K.K.; Wang, T.; Antolin, P.; Chen, B.; He, J. Global Sausage Oscillation of Solar Flare Loops Detected by the Interface Region Imaging Spectrograph. Astrophys. J. Lett. 2016, 823, L16. [Google Scholar] [CrossRef]
- Kolotkov, D.Y.; Nakariakov, V.M.; Kupriyanova, E.G.; Ratcliffe, H.; Shibasaki, K. Multi-mode quasi-periodic pulsations in a solar flare. Astron. Astrophys. 2015, 574, A53. [Google Scholar] [CrossRef]
- Yuan, D.; Van Doorsselaere, T. Forward Modeling of Standing Kink Modes in Coronal Loops. II. Applications. Astrophys. J. Suppl. Ser. 2016, 223, 24. [Google Scholar] [CrossRef]
- Nakariakov, V.M.; Zimovets, I.V. Slow Magnetoacoustic Waves in Two-ribbon Flares. Astrophys. J. Lett. 2011, 730, L27. [Google Scholar] [CrossRef]
- McLaughlin, J.A.; Thurgood, J.O.; MacTaggart, D. On the periodicity of oscillatory reconnection. Astron. Astrophys. 2012, 548, A98. [Google Scholar] [CrossRef]
- Nakariakov, V.M. MHD oscillations in solar and stellar coronae: Current results and perspectives. Adv. Space Res. 2007, 39, 1804–1813. [Google Scholar] [CrossRef]
- Lin, R.P.; Dennis, B.R.; Hurford, G.J.; Smith, D.M.; Zehnder, A.; Harvey, P.R.; Curtis, D.W.; Pankow, D.; Turin, P.; Bester, M.; et al. The Reuven Ramaty High-Energy Solar Spectroscopic Imager (RHESSI). Sol. Phys. 2002, 210, 3–32. [Google Scholar] [CrossRef]
- Lemen, J.R.; Title, A.M.; Akin, D.J.; Boerner, P.F.; Chou, C.; Drake, J.F.; Duncan, D.W.; Edwards, C.G.; Friedlaender, F.M.; Heyman, G.F.; et al. The Atmospheric Imaging Assembly (AIA) on the Solar Dynamics Observatory (SDO). Sol. Phys. 2012, 275, 17–40. [Google Scholar] [CrossRef]
- Woods, T.N.; Eparvier, F.G.; Hock, R.; Jones, A.R.; Woodraska, D.; Judge, D.; Didkovsky, L.; Lean, J.; Mariska, J.; Warren, H.; et al. Extreme Ultraviolet Variability Experiment (EVE) on the Solar Dynamics Observatory (SDO): Overview of Science Objectives, Instrument Design, Data Products, and Model Developments. Sol. Phys. 2012, 275, 115–143. [Google Scholar] [CrossRef]
- Pesnell, W.D.; Thompson, B.J.; Chamberlin, P.C. The Solar Dynamics Observatory (SDO). Sol. Phys. 2012, 275, 3–15. [Google Scholar] [CrossRef]
- Ning, Z. Imaging Observations of X-Ray Quasi-periodic Oscillations at 3 - 6 keV in the 26 December 2002 Solar Flare. Sol. Phys. 2014, 289, 1239–1256. [Google Scholar] [CrossRef]
- Ning, Z. One-Minute Quasi-Periodic Pulsations Seen in a Solar Flare. Sol. Phys. 2017, 292, 11. [Google Scholar] [CrossRef]
- Ning, Z.; Li, D.; Zhang, Q.M. Emission Measure and Temperature Analysis of the Upper Coronal Source of a Solar Flare. Sol. Phys. 2016, 291, 1783–1798. [Google Scholar] [CrossRef]
- Sui, L.; Holman, G.D. Evidence for the Formation of a Large-Scale Current Sheet in a Solar Flare. Astrophys. J. 2003, 596, L251–L254. [Google Scholar] [CrossRef]
- Shen, C.; Lin, J.; Murphy, N.A. Numerical Experiments on Fine Structure within Reconnecting Current Sheets in Solar Flares. Astrophys. J. 2011, 737, 14. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, F.; Li, D.; Ning, Z. One-Minute Quasi-Periodic Pulsations during an M-Class Solar Flare. Universe 2022, 8, 104. https://doi.org/10.3390/universe8020104
Shi F, Li D, Ning Z. One-Minute Quasi-Periodic Pulsations during an M-Class Solar Flare. Universe. 2022; 8(2):104. https://doi.org/10.3390/universe8020104
Chicago/Turabian StyleShi, Fanpeng, Dong Li, and Zongjun Ning. 2022. "One-Minute Quasi-Periodic Pulsations during an M-Class Solar Flare" Universe 8, no. 2: 104. https://doi.org/10.3390/universe8020104
APA StyleShi, F., Li, D., & Ning, Z. (2022). One-Minute Quasi-Periodic Pulsations during an M-Class Solar Flare. Universe, 8(2), 104. https://doi.org/10.3390/universe8020104





