Gravitating Electron Based on Overrotating Kerr-Newman Solution

Abstract

We consider a consistent with gravity electron based on the overrotating Kerr-Newman (KH) solution and show that the earlier KH electron models proposed by Carter, Israel and López in 1970–1990 should be modified by the Landau-Ginzburg theory, leading to a superconducting electron model consistent with gravity and quantum theory. Truncated by Israel and López, the second sheet of the KN solution is rearranged and represented in a mirror form as a sheet of the positron, so that the modified KN system forms a quantum electron-positron vacuum interacting with gravity. Regularization of the KN black hole solution creates two new important effects leading to a strong gravitational interaction that acts on the Compton scale contrary to the usual Planck scale of Schwarzschild gravity: (A)—gravitational frame-dragging creates two Wilson loops acting at two boundaries of the modified KN solution, and (B)—formation of the flat superconducting core of the regularized KN solution creates a superconducting electron-positron vacuum state. The Landau-Ginzburg model shows that Wilson loops determine phases of two Higgs fields forming superconducting vacuum state of the modified KN solution, quantum vacuum of the electron-positron pairs. The phases of these Higgs fields correspond to two light-like modes of a classical relativistic ring string. We come to the conclusion that the electron models considered by Israel and López are not complete and must be supplemented by a mirror structure that forms a quantum system consistent with QED.

1. Introduction

Two approaches to the problem of interaction between gravity and quantum theory are well known and usually widely discussed: theory of superstrings and loop quantum gravity. Meanwhile, there is a third area of research that has already been discussed over fifty years, and was recently again reactivated. This is the treatment of the elementary quantum particle as an overrotating the Kerr-Newman (KN) solution [1,2,3,4,5,6,7,8,9,10,11,12,13]. Formation of black holes is related with classical gravitational effect of frame-dragging [14], which has not previously been considered in particle physics and, when applied to the problem of interaction between quantum theory and gravity, it leads to two important new consequences:

(A)—the emergence of the topologically non-trivial space-time structure that changes effective scale of the gravitational interaction;

(B)—formation of the Wilson loops of vector potential through gravitational effect of frame-dragging, which creates strong influence of gravity on the particle quantization.

The effects (A) works in the famous Kerr-Newman (KN) solution, which is the well-known solution for a charged and rotating BH, and the effect (B) appears in the regularized KN solution considered as a non-perturbative model of the electron, and possibly other leptons as well.

In 1968, Carter noticed that KN solution has gyromagnetic ratio $g=2,$ just the same as that of the Dirac electron [1], which gave rise to two lines in the study of the electron model based on the KN solution: the disk-like and bubble models [3,4,8,15], and the string-like models [5,6,11], based on the analogue of the Kerr singular ring with a string, similar to the Nielsen-Olesen string model in superconductor [16]. These lines were united in the subsequent series of the works [9,10], where the source of the KN electron model was considered as a superconducting bag model with a string formed on the sharp border of the bag-like core.

The KN model of electron is consistent with gravity by nature [2], and these works show, how the known insuperable contradictions between gravity and quantum physics can actually be resolved. 1

As opposed to gravitational radius of the Schwarzschild solution $l_s=\frac{Gm}{c^2},$ effective zone of gravitational interaction in the KN solution is determined by radius of the Kerr singular ring

$$a=\frac{J}{mc},$$

(1)

which is inverse proportional to mass m and proportional to angular momentum of the Kerr solution $J.$ One sees that for electron parameters $J=\hslash /2$ and $m,$ parameter a is the reduced Compton wave length $a=\frac{\hslash }{2mc},$ and the usual arguments on the exclusive role of the Planck length (see for example [17]) turn out to be incorrect when applied to Kerr’s spinning gravity.

Kerr singular ring is the branch line of the KN space into two sheets, and the KN solution with parameters of elementary particles is not really black hole, because (in the dimensionless units) the typical spinning particles have $a^2\gg e^2+m^2$ which is condition for disappearance of the black hole horizons, and therefore, the naked singular ring (not covered by horizon) created in 1970s the problem of the physical interpretation of Kerr space.

The energy momentum tensor of the naked Kerr singular ring diverges, and the over-rotating KN solution carries infinite energy. Contrarily to the case of the spherically symmetric gravity, the overrotating KN gravity brings infinite energy on the Compton scale and needs a regularization and even the renormalization similar to QED.

The electromagnetic KN field [2,18] is also unlimited, and being depended on cut-off $r_e$, it forms a closed Wilson loop of the KN vector potential, which plays important role in formation of the quantum system of electron-positron vacuum.

The cut-off $r_e$, determines increment of the KN vector potential along the closed Wilson loop [19] that sets balance between gravitational and electromagnetic interaction, and determines the regularized mass of the particle as a result of a nonlinear gravito-electromagnetic interaction.

The overrotating KN solution represents a topologically nontrivial two-folded manifold composed of the “positive” sheet of space, coupled with a mirror “negative” sheet, similarly to the Einstein-Rosen bridge or the wormhole solution.

W.Israel in [3] (1970) formed the KN electron model, truncating “negative” sheet of KN solution, and completing the resulting manifold by a specially selected energy-momentum tensor consistent with the Einstein equations.

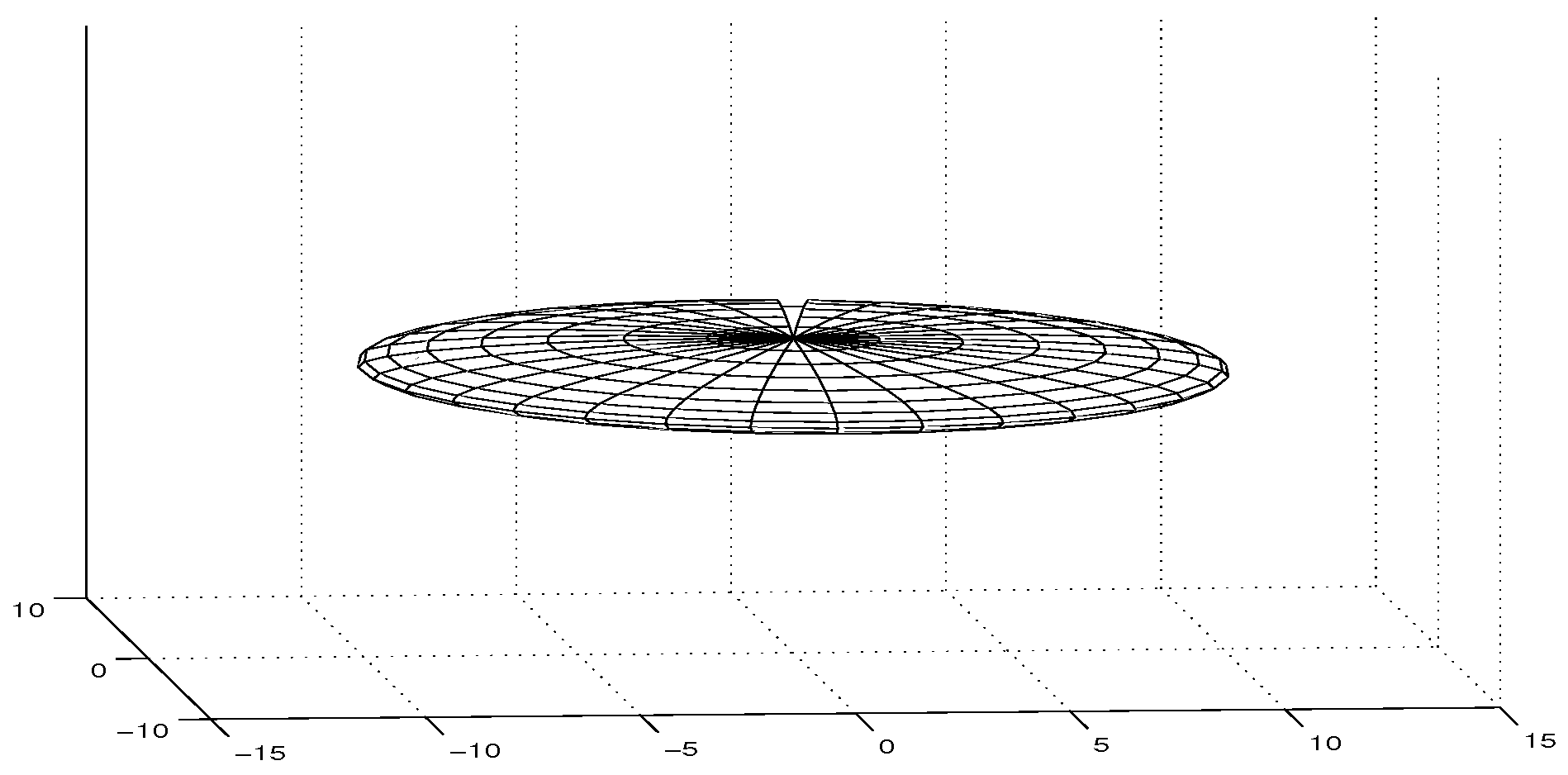
López [4] regularized KN solution truncating singular ring (together with “negative” sheet) at the “classical” radius $r_e=e^2/2m,$ corresponding to the boundary where the energy of electromagnetic field starts compensate the energy of gravitational field. This regularization formed a flat core of the electron as a model of vacuum bubble, which is free from gravitational and electromagnetic field. López’s electron formed a disk with the thickness $r_e$ and the radius $a,$ equal to the half of the Compton wave length (1), see Figure 1. As a result, core of the regularized electron acquires the flat internal (Minkowskian) metric, retaining the exact external gravitational and electromagnetic field of the KN solution.

One of the main point of the our consideration of the KN solution is related with the observation that the truncated by W. Israel and C. López “negative” sheet of the KN solution are not unnecessary, and after a small modification (the space reflection with change of the sign of charge), the “negative” sheet starts describe positron, as a counter-part of electron model, and they form, together with electron, the electron-positron vacuum energy, providing strong interaction with the KN gravitational field.

As a result, we arrive to the conclusion that the electron models considered by Israel, as well as by López, are not complete and must be supplemented by the mirror “negative” structure forming a quantum electron-positron system.

This point of view is supported by the Landau-Ginzburg model of the superconducting phase transition, which indicates that the corresponding quantum vacuum core of the KN electron is to be superconducting and core of the KN electron is formed by a strong magnetic coupling of the electron and positron counterparts.

As a result, the KN solution has undergone a transformation that replaces the “negative” sheet with a “mirror” sheet, carrying the opposite charge and current coupled with mirror boundary. Under regularization, the mirror boundary acquires the mirror Wilson line.

Since Wilson lines are integral curves of the strong gravitational and electromagnetic field in a fixed world time t, the evolution of these fields in time is best described in the Kerr-Schild coordinate system. Meanwhile, the relativistic system can also be described by the “proper time s”, where the size of the system is reduced by the Lorentz contraction. We show that the regularized boundary of KN solution represents a classical relativistic ring string taking intermediate position between the string (word time t) and the light-like particle (proper time s) that is created from this string by relativistic contraction. This string-particle correspondence is a copy of the Heisenberg-Schrödinger correspondence in quantum theory and plays very important role in quantum interpretation of the KN electron model.

2. Kerr-Newman Solution in the Kerr-Schild Form

In the Kerr-Schild coordinates, metric of the KN solutions is [2]

$$g_{\mu \nu }={\eta }_{\mu \nu }+2H{k}_{\mu }{k}_{\nu },$$

(2)

where ${\eta }_{\mu \nu }$ is metric of an auxiliary Minkowski space $M^4,$ (signature $(-+++)$), and H is the scalar function which for the KN solution takes the form

$$H_{KN}=\frac{mr-e^2/2}{r^2+a^2{cos}^2\theta },$$

(3)

and the KN vector potential is

$$A_{\mu }=\frac{-er}{(r^2+a^2{cos}^2\theta )}{k}_{\mu },$$

(4)

where

$$k_{\mu }d{x}^{\mu }=dr-dt-a{sin}^2\theta d{\varphi }_K,$$

(5)

and the Kerr angular coordinates are related to Cartesian coordinates as follows

$$\begin{array}{ccc}\hfill x+iy& =& (r+ia)exp\left\{i{\varphi }_K\right\}sin\theta ,\hfill \\ \hfill z& =& rcos\theta ,\rho =r-t,\hfill \end{array}$$

(6)

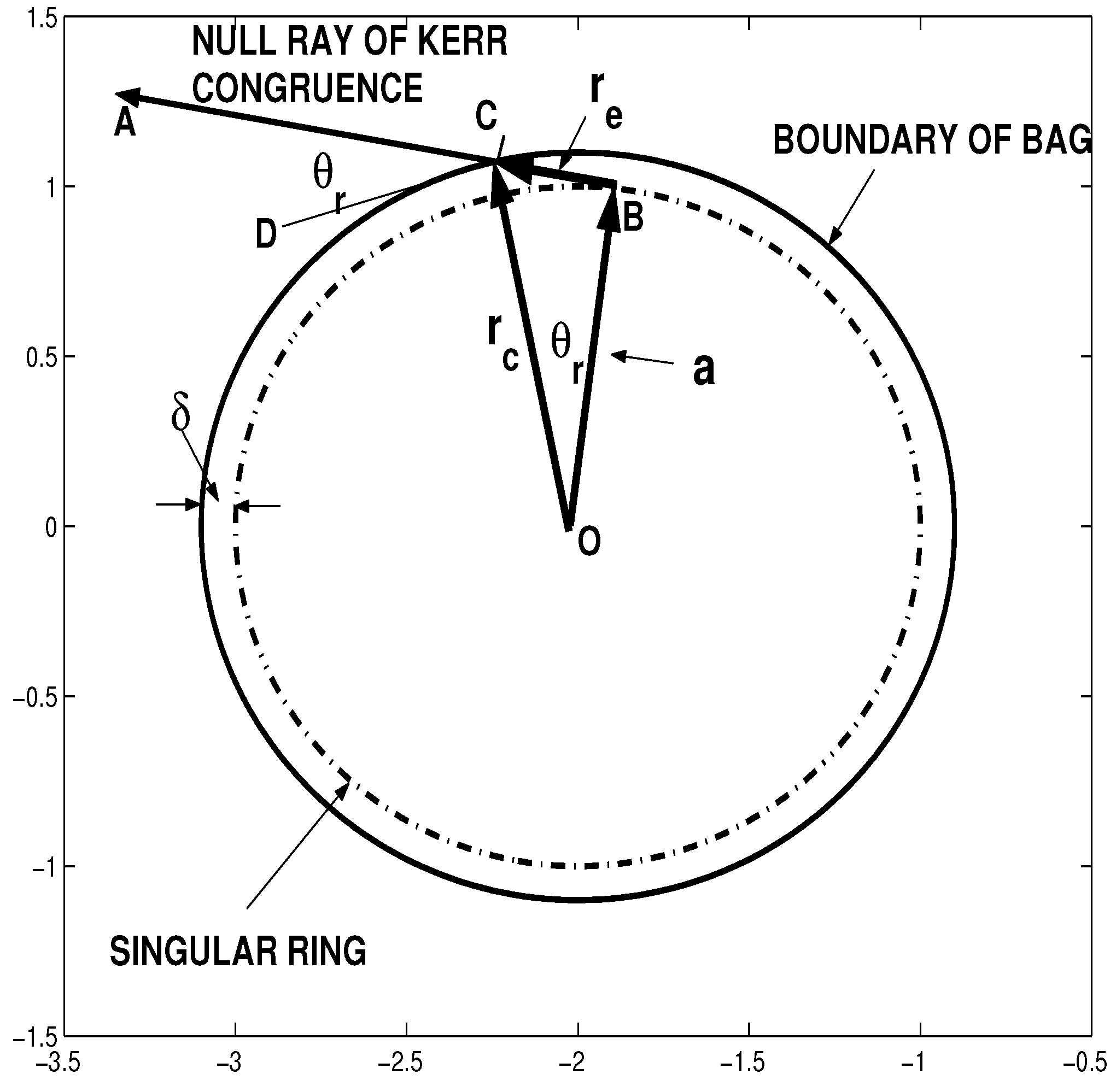
Vector field $k_{\mu },$ ($k_{\mu }{k}^{\mu }=0$), forms Principal Null Congruence of the Kerr solution [2]. Congruence $k^{\mu }\left(x\right)$ forms a vortex of the null field which propagates analytically from negative sheet of Kerr metric, $r<0,$ to positive one, $r>0,$ where the ingoing congruence turns into the outgoing field. In equatorial plane, $cos\theta =0,$ null lines of the Kerr congruence focus on singular ring $k_{\mu }$=0, see Figure 2.

The “mirror” modification of the KN metric corresponds to a change in the direction of the Kerr congruence $k_{\mu }$ on the mirror sheet $r<0$, when passing through the boundary $r=0$. The change $r\to -r,$ coupled with a mirror change of the direction of rotation $a\to -a$, gives $k_{\mu }\to k_{\mu }^{\pm }=\pm dr-dt\mp a{sin}^2\theta d{\varphi }_K,$ and we have

$$k_d^{\pm }x=\pm dr-dt+a{sin}^2\theta d{\varphi }_K.$$

(7)

The metrics on the ingoing and outgoing $(\pm )$-sheets become different $g_{\mu \nu }^{\pm }={\eta }_{\mu \nu }+2H{k}_{\mu }^{\pm }{k}_{\nu }^{\pm }.$ There is a freedom in choice of the $k_{\mu }^{+}$ or $k_{\mu }^{-}$ for the “basic” sheet of space [14]. We take as the “basic” the outgoing field $k_{\mu }^{+},$ that corresponds to the classical physical picture with the retarded electromagnetic field, and we chose as positive rotation the anticlockwise direction of the Kerr congruence near the Kerr singular ring.

Taking the “mirror sheet” as a sheet of positron, we must also change the sign of the charge, $e\to -e$ that gives as two vector potentials

$$A_{\mu }^{\pm }=\frac{-er}{(r^2+a^2{cos}^2\theta )}{k}_{\mu }^{\pm },$$

(8)

acting on the ingoing and outgoing sheets of the KN solution.

3. The Kerr Theorem

Kerr theorem defines two fields of PNC, $k^{+}\left(x\right)$ and $k^{-}\left(x\right),$ in terms of Penrose’s twistor theory [20,21]. Kerr theorem presents two complex analytic solutions $Y^{\pm }$ of the equation

$$F\left(T^A\right)=0,$$

(9)

where F is quadratic holomorphic function of the projective twistor coordinates $T^A=\{Y,\zeta -Yv,u+Y\overline{\zeta }\},A=1,2,3,$ and

$$\begin{array}{ccc}\hfill 2^{\frac{1}{2}}\zeta & =& x+iy,2^{\frac{1}{2}}\overline{\zeta }=x-iy,\hfill \\ \hfill 2^{\frac{1}{2}}u& =& z+t,2^{\frac{1}{2}}v=z-t,\hfill \end{array}$$

(10)

are the null Cartesian coordinates of the auxiliary Minkowski space $x^{\mu }\in {\mathbb{M}}^4.$

In the class of quadratic in Y functions $F\left(T^A\right),$ the Kerr theorem gives two analytic solutions $Y^{\pm }\left(x^{\mu }\right),$ of the Equation (9), which correspond to two projective spinor coordinates

$$Y^{+}={\xi }^{\dot{1}}/{\xi }^{\dot{0}},Y^{-}={\eta }_1/{\eta }_0,$$

(11)

which are antipodally conjugate

$$Y^{+}=-1/{\overline{Y}}^{-},$$

(12)

and the corresponding Weyl spinors ${\xi }^{\dot{\alpha }}$ and ${\eta }_{\alpha }$ define two antipodal fields of the principal null directions

$$k^{\mu +}={\overline{\xi }}^{\alpha }{\sigma }_{\alpha \dot{\alpha }}^{\mu }{\xi }^{\dot{\alpha }},k^{\mu -}={\overline{\eta }}_{\dot{\alpha }}{\overline{\sigma }}^{\mu \dot{\alpha }\alpha }{\eta }_{\alpha }.$$

(13)

4. López Electron as a Regularized KN Solution

In regularized by López KN solution, singular region is replaced by a flat core forming interior of the bubble model. The boundary which separates flat core from the external KN gravity is defined unambiguously as a boundary surface $B\left(r\right),$ where the nonlinear term in (2) is cancelled, $H\left(r\right)=H_{KN}\left(r\right)=0,$ and the KN metric becomes flat.

According (3) it corresponds to

$$r=r_e^{+}=e^2/2m,$$

(14)

(López, 1984 [4]).

The metric at $r\le r_e^{+}$ is set flat, $g_{\mu \nu }={\eta }_{\mu \nu },$ and the external KN space is joined continuously with flat interior of the electron core, which corresponds to the Gürsey and Gürses class of the deformed KN metrics [15]. Since r is Kerr’s radial coordinate, (6), the core takes form of a thin ellipsoidal disk (see Figure 1 and Figure 3),which has the thickness $2r_e=e^2/m$ and radius $r_c=\sqrt{r_e^2+a^2}\approx a,$ determined by the Compton wave length $a=\hslash /2mc,$ so that the ratio

$$r_e/a=\alpha \approx 1/137,$$

(15)

is the fine structure constant. The small Cartesian distance $\delta =r_e-a$ on Figure 3 works as a cutoff for the regularization of the KN electromagnetic and gravitational fields.

Since the initial KN manifold is two-sheeted, the López regularization creates also the mirror boundary surface $B^{-}\left(r^{-}\right),$ where the nonlinear term in (2) is also cancelled, $H\left(r^{-}\right)=H_{KN}\left(r^{-}\right)=0,$ and metric (2) on the mirror side of the core $B^{-}$ is also flat, $g_{\mu \nu }^{-}={\eta }_{\mu \nu }.$

As shows Figure 3, the original Kerr congruence of the KN solution propagates analytically from the basic sheet of the KN space $r>0$ on the mirror negative sheet, where $r<0.$

Taking into account the above-mentioned reinterpretation of the mirror sheet as a sheet for a positron solution, we perform a space reflection $P:r\to -r$ reversing the direction of Kerr congruence $k^{+}\left(x\right)\to k^{-}\left(x\right),$ and changing the sign of the direction of rotation $a\to -a.$ Then, the transformation relations to Kerr’s coordinates (6) have to be changed, and on the mirror sheet of the KN solution for $r<r^{-}=-e^2/2m,$ they take the form

$$\begin{array}{ccc}\hfill x-iy& =& (r-ia)exp\{-i{\varphi }_K\}sin\theta ,\hfill \\ \hfill z& =& rcos\theta ,\rho =r-t,\hfill \end{array}$$

(16)

5. Wilson Loops, Quantization and Emergence of the Monopole-Antimonopole Pair

In the regularized KN solution, potential (4) increases strongly near the sharp boundaries of the disk-like core $B^{\pm }$ and forms two string-like loops $C^{\pm }$ on the borders $r=r^{\pm }$, which are placed in the equatorial plane $cos\theta =0$ at fixed $t=t_0=const.$. On the boundary $C^{+}$ potential is dragged by the lightlike direction $k_{\mu }^{+},$ and angular component of the potential (4) increases, forming the closed loop

$$C^{+}:\{{\varphi }_K\in [0,2\pi ]\},$$

(17)

like a smoothed $\delta$-function with maximal value at the ring string $r=r^{+},$

$$A_{\mu }^{max}d{x}^{\mu }=\frac{2m}{e}(dt+d{\varphi }_K).$$

(18)

When integrating over the contour $C^{+}$, the Wilson loop $W\left(C^{+}\right)=exp\left\{e{\oint }_{C^{+}}{A}_{\mu }^{+max}d{x}^{\mu }\right\},$ gives the phase increment

$$\delta \varphi =e{\oint }_{C^{+}}{A}_{{\varphi }_K}^{+max}d{\varphi }_K=4\pi ma,$$

(19)

which, in accordance with the Kerr principal relation

$$J=ma=\frac{\hslash }{2},$$

(20)

becomes proportional to $2\pi \hslash .$

We see that phase increment of Wilson line is quantized, similar to phase of the wave function, giving quantum contribution to classical action through “minimal” coupling $p_{\mu }\to p_{\mu }-e{A}_{\mu }.$

To unambiguously determine the $W\left(C^{+}\right)$ we need to put $\delta \varphi =2\pi ,$ and we obtain ([19]) that the Wilson line gives the electron a quantization condition of angular momentum $J=\frac{1}{2},$ and also a series of additional quantum solutions, $\delta \varphi =n2\pi ,$ with $n=2,3\dots$

The Wilson loop carries the tension energy of the ring string and forms a particle with a spin in the form of a regularized Kerr disk, Figure 3, whose radius is equal to half the Compton wavelength [1,3,4].

In the works [9,19], the boundary of KN disk was formed as a domain wall phase transition in the Ginzburg-Landau field model, where the space inside the disk forms a superconducting vacuum core of the KN bag model, created by a phase transition from the external gravitational solution KN to the vacuum state inside the Kerr disk.

According to Stokes’ theorem, such Wilson loop $C^{+}$ should generate a magnetic flux

$$\mathrm{\Phi }={\oint }_{C^{+}}{A}_{{\varphi }_K}^{+max}d{\varphi }_K=4\pi ma=4\pi \hslash /2=h/e,$$

(21)

However, we know that a monopole cannot be born alone, and the second Wilson loop should exist with the contour $C^{-}$, generating an anti-monopole equal to half of the quantum of the magnetic field ${\mathrm{\Phi }}_0=\hslash /2e$, and the Dirac monopole mast be born simultaneously.

Indeed, the similar potential $A_{\mu }^{-max}d{x}^{\mu },$ related with the ingoing Kerr congruence, is concentrated on the mirror side of the Kerr space. It is connected with the ingoing Kerr congruence, and at the moment of $t=t_0=const.$ potential $A_{\mu }^{-max}d{x}^{\mu },$ is positioned on the boundary $r^{-}=-e^2/2m$ placed in the equatorial plane $cos\theta =0$, forming the ring string along the loop

$$C^{-}:\{{\varphi }_K\in [0,2\pi ]\}.$$

(22)

Integration the Wilson loop $W\left(C^{-}\right)=exp-e{\oint }_C{A}_{\mu }^{-max}d{x}^{\mu },$ with opposite orientation of the contour $C^{-},$ gives the opposite phase increment

$$-\delta \varphi =-e{\oint }_{C^{-}}{A}_{{\varphi }_K}^{-max}d{\varphi }_K=-4\pi ma=-4\pi J.$$

(23)

Therefore, contribution of the Wilson loop at the $C^{-}$ boundary almost completely reduces contribution of the loop at $C^{+}$, except for an important asymmetry: integration over the $C^{+}$ boundary is associated with a retarded vector potential, i.e., with the outgoing “basic” congruence, which generates the gravitational KN field, while the $C^{-}$ boundary is related with ingoing vector potential $A_{\mu }^{-max}d{x}^{\mu },$ and does not contain the electrostatic component

$$A_{dt}=\frac{-er}{(r^2+a^2{cos}^2\theta )}dt,$$

(24)

which is usually associated with the mass of electron [22] appearing as the mass term “m” in the Dirac equations $(i{\gamma }_{\mu }{p}^{\mu }+m)\psi =0$ and in the expression (3) for KN metric. Therefore, the strong total interaction between the KN gravitational field and two Wilson loops $C^{\pm }$ does not manifest itself explicitly in the mass term “m”, but acts non-linearly, increasing the mass by reducing the radius a in the Kerr principal relation (1).

Thus, two Wilson loops generate on two boundaries of the Kerr disk $C^{+}$ and $C^{-}$ a magnetically coupled pair consisting of a Dirac monopole and an anti-monopole, that is, a Cooper pair that gives rise to superconducting state in the vacuum core of the Kerr disk.

We should make some reference here to several previous applications of similar ideas in other physical models of elementary particles, not connected with gravitational frame-dragging and KN gravity. First of all we note that the phase increment of this type, similar to the model of Wilson loop, was discussed long ago as a Berry phase, or Pancharatnam-Berry phase, in many models related with classical Bohm-Aharonov magnetic solenoid effect. Second, the close ideas related with classical string models of this type where considered by Frank Wilczek [23], and then, as a 3D non-critical string model of Mezincescu and Townsend [24] and others. Also, the electron vacuum core, and the hidden vacuum magnetic energy of electron-positron vacuum pairs, was considered in the Shulman model [25].

6. Superconducting Core of the KN Solution

Separation of the classical gravity from superconducting core of the KN bag model is performed by the supersymmetric Landau-Ginzburg (LG) domain wall model, see [9,10,26]. The use of supersymmetry is really necessary, because the simpler non-supersymmetric LG models cannot provide the concentration of the Higgs field inside of the bag model and leads to the opposite configuration of the bag, forming the bag as a cavity in a superconductor.

Meanwhile, the simplest LG model, which was used as a phenomenological model of superconductivity in the Nielsen-Olesen string model [16], and in the MIT and SLAC bag models [27,28], can be used to a simplified description of the phase transition between the external KN field and the superconducting state inside the core of the KN electron model.

Lagrangian of the corresponding Nielsen-Olesen field model is

$${\mathcal{L}}_{NO}=-\frac{1}{4}{F}_{\mu \nu }{F}^{\mu \nu }-\frac{1}{2}\left({\mathcal{D}}_{\mu }\mathrm{\Phi }\right){\left({\mathcal{D}}^{\mu }\mathrm{\Phi }\right)}^{*}-V\left(\right|\mathrm{\Phi }\left|\right),$$

(25)

where ${\mathcal{D}}_{\mu }={\nabla }_{\mu }+ie{A}_{\mu }$ is $U\left(1\right)$ covariant derivative, $F_{\mu \nu }=A_{\mu ,\nu }-A_{\nu ,\mu },$ and $\mathrm{\Phi }$ is the complex Higgs field $H=\left|H\right|expi\chi \equiv \mathrm{\Phi }.$

The real curved Domain Wall surface considered in [10] is replaced here by a flat one, and we consider interaction of the external KN electromagnetic field $A_{\mu }^{max}$ with a Higgs field $H\equiv \mathrm{\Phi }=e^{i\chi }$ placed inside the superconducting bag.

From Lagrangian (25) we obtain the equations for boundary current

$$\square A_{\mu }^{max}=I_{\mu }=e{\left|H\right|}^2(\chi {,}_{\mu }+e{A}_{\mu }^{max}),$$

(26)

which show that by penetration of the potential $A_{\mu }^{max}$ in the Higgs vacuum field $H,$ phase of the Higgs field $\chi (t,\varphi ),$ interacts with potential creating on the bag boundary a superficial current $I_{\mu }$, which penetrates inside the bag on a penetration depth, after that phase of the Higgs field field $\chi (t,\varphi )$ eats the potential, leading to $I_{\mu }=0,$ and r.h.s. of (26) takes the form $\chi {,}_{\mu }+e{A}_{\mu }^{max}=0.$ The resulting equations

$$\frac{\partial \chi }{\partial {\varphi }_K}=2ma,\frac{\partial \chi }{\partial t}=2m$$

(27)

can be integrated and give explicit solution for phase of the Higgs field

$$\chi (t,{\varphi }_K)=2m(t+a{\varphi }_K).$$

(28)

These important results follow from the famous old paper by Ginzburg and Landau [29], in which for the first time, a connection was established between superconductivity and the quantum wave function of the Schrödinger equation for the simplest case of a flat boundary between superconductivity and an electromagnetic field.

Ginzburg and Landau were the first to use the wave function of the Schrödinger equation to model the superconducting state, and this was the forerunner the fact that superconductivity will play a central role in the structure of elementary particles, and in particular, in the electron structure discussed here. This idea turned out to be extremely fruitful, and was further developed in the close connection between the Higgs mechanism of elementary particle physics and phase transitions in more complex superconducting models.

Finally, the development of the supersymmetric version of the Ginzburg-Landau theory has shown its equivalence to the supersymmetric model of quantum electrodynamics [30], the super-QED model proposed by Wess and Zumino.

Supersymmetric Ginzburg-Landau Model and Two Sides of the KN Disk

In the KN electron model, the discussed above boundary $B\left(r\right)$ between the exact external KN solution EXT and superconducting core INT represents the oblate ellipsoidal surface shown on Figure 1. It is much more complex then the flat surface, and formation of the phase transition requires the full power of the Supersymmetric Ginzburg-Landau model [30].

As it was shown in [9,10] the boundary $B\left(r\right)$ should be described by a domain wall, which interpolates between the states EXT and INT and controlled by the superpotential W which must be a holomorphic function of five chiral fields.

In terms of the superfield formalism, two of the chiral fields are the Higgs superfields ${\mathrm{\Phi }}_{+}$ and ${\mathrm{\Phi }}_{-},$ and bosonic part of these superfields gives two Higgs fields $H_{\pm }.$ The relation

$$H^{-}{H}^{+}={\eta }^2,$$

(29)

shows that the Higgs fields

$$H_{\pm }=\left|H_{\pm }\right|e^{i{\chi }_{\pm }}$$

(30)

can have different amplitudes $|H_{-}|\ne |H_{+}|,$ but their phases must be correlated

$${\chi }_{+}=-{\chi }_{-}=\chi ,$$

(31)

It was obtained in [10] that the KN bag has also a negative boundary $r^{-}=-r_e^{+},$ which contributes into the BPS state of the supersymmetric bag model. This boundary is just the mirror boundary $B^{-}\left(r^{-}\right)$ corresponding to the in-going fields which fall on the superconducting core of the KN solution before the scattering as ingoing fields.

The coordinate transformations for the mirror sheet (16) are obtained with a space reflection $P,$ that changes the sign of the Kerr radial coordinate $r\to -r,$ and the direction of rotation $a\to -a.$ In addition, the charge sign is changed $e\to -e,$ that changes the sign of potential energy $e{A}^{max}\to -e{A}^{max}$ corresponding to consideration of the mirror sheet as a sheet for a positron. Analogues of the Equations (26)–(28) take the form

$$\square A_{\mu }^{max}=I_{\mu }^{+}+I_{\mu }^{-},$$

(32)

where

$$I_{\mu }^{\pm }=e^{\pm }{\left|H^{\pm }\right|}^2({\chi }^{\pm }{,}_{\mu }-e^{\pm }{A}_{\mu }^{\pm }),$$

(33)

where the current $I_{\mu }^{-}$ is created on the boundary $B^{-}\left(r^{-}\right)$ related with the ingoing metric $g_{\mu \nu }^{-}={\eta }_{\mu \nu }+2H{k}_{\mu }^{-}{k}_{\nu }^{-},$ based on the Kerr congruence $k_{\mu }^{-}.$ Superconducting core of the KN solution acquires two string-like currents of opposite orientations and two Higgs fields $H^{\pm }$ with opposite phases

$${\chi }_{\pm }=2m(t\pm a{\varphi }_K)$$

(34)

acting as the left and right light-like modes of the string model.

The Higgs fields ${\mathrm{\Phi }}_{+}=\left|{\mathrm{\Phi }}_{+}\right|e^{-ie{\chi }_{+}}$ and ${\mathrm{\Phi }}_{-}=\left|{\mathrm{\Phi }}_{-}\right|e^{ie{\chi }_{-}},$ are associated with two superfields of the supersymmetric QED model [30].

7. Emergence of the Classical Ring String Structure

As it was shown in Section 6, the vector potential of Wilson lines creates two surface currents (33) in the core of KN electron model, which are parametrized by the Kerr angular coordinate ${\varphi }_K$ and have a radius of $a=\hslash /2m$ equal to half of Compton wavelength. These currents turn out to be light-like and generate the model of a closed relativistic ring string, forming a minimal surface in 4d Minkowski space. String model turns out to be very important for compatibility of the nonperturbative KN solution with quantum theory, since it removes contradiction between the extended classical particle and the point particle of quantum theory. Description of the KN electron in the Kerr-Schild coordinate is realised in the word time “t” at a fixed moment $t=t_0=const.$, corresponding to image of a closed string placed on the boundary of the KN disk. In quantum theory this corresponds to a state vector in Heisenberg picture. The relativistic ring strings are massless, and their mass-energy is created from their relativistic dynamics. Below we show in Section 7.2 that the length of the relativistic KN string is reduced by the Lorentz contraction, and the KN ring string, which is considered in world time “t” as an extended string of Compton size, is compressed to a point in the proper time “s” coordinates corresponding to Schrödinger state vector of quantum theory.

7.1. Orientifold String

By analogue with the Nielsen-Olesen string model [16], in [6] we considered the Kerr singular ring as a string in the Carter-Israel-López-KN electron model and showed in [11] that this string can have an orientifold structure, which satisfies to equations of motions and constraints corresponding to the classical Nambu-Goto string.

In general, the four-dimensional relativistic bosonic string $X^{\mu }(t,\sigma )$ is 2-dimensional world-sheet embedded in 4-d space-time. It satisfies the wave equation

$${\partial }_{-}{\partial }_{+}{X}^{\mu }=0,$$

(35)

where ${\sigma }^{\pm }=t\pm \sigma$ are the light-cone parameters, t is the world-sheet time parameter, and $\sigma =a{\varphi }_K$ is the space-like parameter along the string length. String solution is formed as a sum of the left and right modes $X^{\mu }(t,\sigma )=X_L^{\mu }(t-\sigma )+X_R^{\mu }(t+\sigma ),$ obeying the light-cone constraints

$${\partial }_{+}{\left(X_L\right)}^2={\partial }_{-}{\left(X_R\right)}^2=0.$$

(36)

At the boundaries $r=r^{\pm },$ phases of the Higgs fields (34) coincide with the left and right null direction of the Kerr congruence $k_{\mu }^{\pm }d{x}^{\mu }{|}_{r^{\pm }}=dt\pm ad{\varphi }_K,$ and the wave Equation (35) and constraints (36) are satisfied.

The extra factor 2 in (34) reflects peculiarity of the orientifold string, which, as it was shown in [11], is consistent with two-folded structure of the Kerr-Schild geometry. Orientifold forms a parity operator $\mathrm{\Omega }:[\sigma \to 2\pi -\sigma ],$ covering the string world-sheet twice: first time as the map $\sigma \to \overrightarrow{[0,\pi ]},$ and second time as the map $\sigma \to \overleftarrow{[2\pi -\sigma ]}$ in opposite direction.

7.2. Electron as a Quantum Particle

Let us return to the Israel electron model, in which negative sheet of the Kerr solution was truncated but the electromagnetic field was absent and the model was not regularized. In spite of the absence of these components, the Israel model demonstrates very important features of the electron as a quantum particle.

First of all, by representing electron as a disk of the reduced Compton radius (1), Israel’s model shows that this does not contradict the point electron of quantum theory.

Indeed, V. Hamity showed in [31] (1986) that the boundary of Israel’s disk moves at the speed of light, and therefore, an observer at rest will see this boundary compressed to a point due to the Lorentz transformations. Therefore, this known discrepancy is due to the use of different coordinate systems for a strictly relativistic object. The reduced Compton radius of Israel’s electron corresponds to description of Israel’s electron in the Kerr-Schild coordinate system which a fixed world time $t=t_0=const.$, that corresponds to Heisenberg picture of quantum theory, while the electron compressed to a point corresponds to its image in relativistically rotating coordinate systems (see [32], par.89, and also [33]), that corresponds to Schrödinger picture of quantum theory.

We see that the Lorentz contraction of the relativistic ring string solves the problems of inconsistency of the extended KN solution, explaining it by the inconsistence of different coordinate systems using for the relativistic light-like particle: the Compton size of the KN disk corresponds to a snapshot picture of the Kerr-Schild coordinate system, or the state vector in the Heisenberg picture at $t=t_0=const.$, while the experimental observation of electron as a quantum point corresponds to the Schrödinger picture of the wave function for the light-like relativistic ring string.

Indeed, the Israel model of electron described in the proper time s tells as still more. Truncating the right part of the two-folded KN solution, Israel represents electron as the most elementary string excitation formed only by left mode of the orientifold string. Impact of the right mode is excluded, and Israel’s electron turns into a classical massless relativistic ring string model consisting only from the left half-string excitation. This string has the zero rest mass $m_{str}=0$ and satisfies the classical Namby-Goto string equations [11].

Consider this string as a state vector $|left>$ of a quantum particle with Hamiltonian

$$H=\sqrt{{\mathbf{p}c}^2+m_{str}^2{c}^4}.$$

(37)

Following the known Dirac’s approach to linearization of the nonlinear Hamiltonian of this type, we obtain that, for the relativistic string with $m_{str}=0$, Hamiltonian (37) is linearized automatically (without the use of Dirac matrices),

$$H=\pm p^{\mu }=\pm i\hslash \partial /{\partial }_{\mu },$$

(38)

and the Schrödinger equation takes the form

$$i\hslash \partial {\Psi }_S/\partial t=\mp \mathbf{p}c{\Psi }_S,$$

(39)

where the momentum operator $\mathbf{p}={\mathbf{p}}^{\left(tr\right)}+{\mathbf{p}}^{\left(s\right)}$ is divided into a transactional part ${\mathbf{p}}^{\left(tr\right)},$ related to a slow movement of the particle as a whole, and the part ${\mathbf{p}}^{\left(s\right)}=\partial /\partial {\varphi }_K,$ associated with circular motion of Kerr’s ring string, parametrized by Kerr’s angular coordinate ${\varphi }_K$.

Thus, for a particle at rest, ${\mathbf{p}}^{\left(tr\right)}=0,$ we have $H=(\partial /\partial t,\partial /\partial {\varphi }_K)$, showing that circular motion of the ring string gives the electron an effective rest mass.

Using the Dirac matrices ${\gamma }^{\mu }=(1,{\gamma }^i)$ in Cartesian coordinates $x^{\mu }=(t,x,y,z)$ of the auxiliary Minkowski space of the Kerr-Schild coordinate system, we obtain the Dirac equation in the form

$$i\hslash \partial \psi ({\varphi }_K,t)/\partial t={\gamma }_i\partial \psi ({\varphi }_K,t)/\partial {\varphi }_K.$$

(40)

It is convenient to use the Weyl basis, where the Dirac matrices have the form

$${\gamma }^{\mu }=\left(\begin{array}{cc}0& {\sigma }^{\mu }\\ {\overline{\sigma }}^{\mu }& 0\end{array}\right).$$

(41)

In this case, the Dirac spinor contains two Weil spinors ${\psi }^D({\varphi }_K,t)=\left(\begin{array}{c}{\chi }_{\alpha }\\ {\overline{\psi }}^{\dot{\alpha }}\end{array}\right).$

In the Weyl basis, the Equations (40) are separated, and two spinors ${\chi }_{\alpha }$ and ${\overline{\psi }}^{\dot{\alpha }}$ describe two independent state vectors $|left>$ and $<right|$ which correspond to opposite helicities, i.e., projections of the spin on the direction of momentum $\mathbf{n}=\mathbf{p}/p$.2

As usual, the Heisenberg and Schrödinger state vectors are related by unitary transformation

$$|{\mathrm{\Psi }}_S(x,t)>=e^{-iHt}|{\mathrm{\Psi }}_H(\varphi ,t_0)>,$$

(42)

and we see that factor $U=e^{-iHt}$ corresponds to kinetic energy of the ring string rotation. Negative sign of (39) should be related with negative frequencies of the wave function, and gives rise to conjugate relation for co-vector representations of positron

$$<{\mathrm{\Psi }}_H(\varphi ,t_0)|e^{+iHt}=<{\mathrm{\Psi }}_S(x,t)|.$$

(43)

This transformation is singular, which complicates interpretation of $|{\mathrm{\Psi }}_S(x,t)>$ and indicates the need of regularization.

8. Wilson Loops of KN Gravity and QED Formalism

8.1. Excess Energy Produces by Wilson Loops, QED and Mass Renormalization

Temporary component of the potential $A_t^{max}=\frac{2m}{e}$, which concentrated on the Wilson loops, gives the electrostatic part of the electron energy which does not contribute to Wilson loops, since the loops are formed by the condition $t=t_0=const.$ including only tangent components of $A_{\mu }^{max},\mu =x,y,z.$ Energy of the electrostatic part

$$U=\frac{e}{2}{\int }_{r_e}^{\infty }{A}_0\left(r\right)dr=m,$$

(44)

when regularizing on the “classical” radius $r_e=e^2/2m,$ (14) corresponds to observable mass of the electron m, as it was shown for example in [22]. The Wilson loops boundaries $C^{+}$ and $C^{-}$ give an additional magnetic contribution to mass-energy of electron—the energy of the interacting with KN gravity electron-positron vacuum pairs.

This shows that López’s regularization requires an additional procedure, known from the QED, as a renormalization of the mass. The rest mass particles m should be formed as the sum

$$m=m_G+\delta m,$$

(45)

consisting of the excessive mass-energy of the interacting with gravity Wilson lines $m_G,$ and the additional hidden contribution $\delta m$, which is reduced during the renormalization process.

8.2. Consistency of Modified KN Structure with QED

The KN solution expressed in Kerr-Schild coordinates is compatible with Heisenberg representation of quantum theory, in which the energy-moment tensor of the KN solution can be obtained in correspondence with the QED (see [35], par. 3.1 ), and takes the form

$$\begin{array}{c}\hfill T_{\mu \nu }=\frac{1}{2}(A_{\nu ,\sigma }{A}_{\mu }^{\sigma }+A_{\mu ,\sigma }{A}_{\nu }^{\sigma }-g_{\mu \nu }{A}_{\sigma ,\lambda }{A}_{\sigma ,\lambda })\\ \hfill +\frac{1}{4}(\overline{\psi }{\gamma }^{\nu }{\partial }_{\mu }\psi -{\partial }_{\mu }\overline{\psi }{\gamma }^{\nu }\psi )\\ \hfill +\frac{1}{4}({\psi }^c{\gamma }^{\nu }{\partial }_{\mu }{\psi }^c-{\partial }_{\mu }{\overline{\psi }}^c{\gamma }^{\nu }{\psi }_c).\end{array}$$

(46)

An analysis of the components in this expression (see also par.89 in [36]) makes it possible to combine quantum theory with gravity through the system of Einstein-Maxwell equations, which in the Kerr-Schild formalism [2] can be presented in the tetrad form as follows

$$R_{ab}-\frac{1}{2}{g}_{ab}R=-8\pi T_{ab},$$

(47)

where $T_{ab}$ is the energy-moment tensor (46), presented in the Kerr-Schild tetrad form

$$T_{ab}=\frac{1}{4\pi }(F_b{F}^c-\frac{1}{4}{g}_{ab}{F}_{cd}{F}^{cd}).$$

(48)

The Contributions to $T_{\mu \nu }$ created by potentials (19) and (23) on the Wilson loops $C^{+}$ and $C^{-}$ are mutually cancelled, for exclusion of the electrostatic (time-) component (24), which plays a special role, being located only on the border $r^{+}=r_e.$ It gives after integration the total mass-energy of the electron the mass term m (44), which is usually taken as the mass in the Dirac equation.

Fermionic components in the expression (46), as shown in [37], correspond to orbital part of the current density fluctuations $\overline{\psi }\overleftarrow{\overrightarrow{{\partial }_{\mu }}}\psi =\frac{2m}{ie}{j}_{\mu }^{\left(orb\right)}$. The vacuum expectation of this sum is zero, since the Dirac electron ring current cancels the oppositely oriented ring current of the positron, forming the vacuum binding energy of the electron-positron pairs.

Alternative, but similar interpretation of these fermionic components in (46) is related to their analogy with Cooper pairs in the Ginzburg-Landau theory of superconductivity (see for example [38]). Comparison with the expression for electron current in QED

$$j_{\mu }\left(x\right)=\frac{ie}{2}(\overline{\psi }{\gamma }_{\mu }\psi -{\overline{\psi }}^c{\gamma }_{\nu }{\psi }^c)$$

(49)

shows that the term $\overline{\psi }{\gamma }^{\nu }{\partial }_{\mu }\psi -{\partial }_{\mu }\overline{\psi }{\gamma }^{\nu }\psi$ in (46) is proportional to the density of the electron current $\frac{ie}{2m}\overline{\psi }{\gamma }^{\mu }\psi ,$ and the term ${\overline{\psi }}^c{\gamma }^{\nu }{\partial }_{\mu }{\psi }^c-{\partial }_{\mu }{\overline{\psi }}^c{\gamma }^{\nu }{\psi }_c$ is proportional to density of the positron current $-\frac{ie}{2m}{\overline{\psi }}^c{\gamma }^{\nu }{\psi }^c$.

9. Conclusions

The KN electron model, considered initially by Carter, Israel and López, and also by Kerr et al. and Newman et al. in works [1,2,3,4,18] demonstrated explicitly that it carries the strong classical gravitational and electromagnetic fields concentrated on the Compton scale in vicinity of the Kerr singular ring. This result is fundamental and shows that the gravity of the KN solution is strong and requires regularization contrary to the widely held that gravity is the weakest interaction.

Principal novelty of the present treatment of the overrotating KN solution is its modification with respect to the original topologically nontrivial two-sheeted structure. The truncation of the negative sheet of the KN solution used by Israel and López is replaced by the action of the supersymmetric Ginzburg-Landau field model, which regularizes the singular gravitational and electromagnetic fields by phase transition to a superconducting vacuum state that forms the core of the nonperturbative electron model.

The strong gravitational frame-dragging interacts with regularized KN electromagnetic field creating two Wilson loops positioned on two boundaries of the superconducting core attached to the fixed world time $t=t_0=const.$. These loops are related with incoming and outgoing Kerr congruences, and in the modified KN solution their action is almost mutually compensated. However, it turns out that these Wilson loops create very the strong indirect gravitational interaction that acts nonlinearly, greatly increasing the mass by reducing the radius a in the basic Kerr relation (1).

Considering the quantum side of the KN electron model, we come to the conclusion that the Kerr-Schild coordinate system allows us to get the most complete nonperturbative description of the electron structure given by the supersymmetric Ginzburg-Landau field model of phase transition, and obtain the superconducting core of the electron in the modified model of KN solution, as well as to give a detailed description of the gravitational interaction through action of the Wilson loops based on the fields at a fixed point of the world time $t=const.$.

The use of world time t is a very important feature of the Kerr-Schild formalism. In the world time t, the surface currents of the Ginzburg-Landau model take the form of an elementary relativistic ring string corresponding to state vector of quantum electron in Heisenberg’s picture of QED. The corresponding Schrödiger state vector is related with unitary transformation, and we obtain that this unitary transformation acts as a relativistic rotation of the elementary ring string, transforming it to a point by the Lorentz contraction. This point electron corresponds to the state vector in the proper time s, which is often used in quantum consideration.

Using the analogy with the KN black hole solution, we can say that, by going from the “t” representation to the “s” representation, the unitary transformation returns the overrotating black hole back to the non-rotating state. This transformation is singular, since the string as well as the core of electron in this case disappear.

We arrive at the main conclusion that the over-rotating Kerr-Newman electron model, initiated by B. Carter in the work [1], and subsequently developed in the work by G.C. Debney, R.P. Kerr and A. Schild [2], and specified in the works by W. Israel [3] and by C. López [4] represents an extremely important progress in the formation of the nonperturbative electron model which answers the questions “What is electron?” and “How to combine gravity with particle physics?”. In the same time we find that these models, at least, are not complete, since they describe a simplified electron model which in many respects is similar to the bare electron described by QED [35].

We obtain that the used by W.Israel and C.López truncation of the negative sheet of the KN solution deprives this model of its mirror side, which is described by the Kerr-Schild metric with ingoing congruence and corresponds to positron. Interaction of the electron with the mirror positron side of KN solution gives the important vacuum contribution in the form of the electron-positron pairs forming the quantum vacuum interacting with gravity through two Wilson loops, carrying the magnetically coupled monopole-antimonopole pairs.

The Ginzburg-Landau field model plays a fundamental role as a base space for elementary excitations of the ring string, generating the wave properties of KN electron, and also as a base space for emergence of Wilson loops creating the superconducting electron-positron vacuum state, leading to a strong interaction of the KN electron with gravity, consistent with QED.