Abstract
Extrasolar circumbinary planets are so called because they orbit two stars instead of just one; to date, an increasing number of such planets have been discovered with a variety of techniques. If the orbital frequency of the hosting stellar pair is much higher than the planetary one, the tight stellar binary can be considered as a matter ring current generating its own post-Newtonian stationary gravitomagnetic field through its orbital angular momentum. It affects the orbital motion of a relatively distant planet with Lense-Thirring-type precessional effects which, under certain circumstances, may amount to a significant fraction of the static, gravitoelectric ones, analogous to the well known Einstein perihelion precession of Mercury, depending only on the masses of the system’s bodies. Instead, when the gravitomagnetic field is due solely to the spin of each of the central star(s), the Lense-Thirring shifts are several orders of magnitude smaller than the gravitoelectric ones. In view of the growing interest in the scientific community about the detection of general relativistic effects in exoplanets, the perspectives of finding new scenarios for testing such a further manifestation of general relativity might be deemed worth of further investigations.
Keywords:
experimental studies of gravity; experimental tests of gravitational theories; extrasolar planetary systems PACS:
04.80.-y; 04.80.Cc; 97.82.-j
1. Introduction
According to the General Theory of Relativity 1 (GTR), the deformed spacetime generated by a localized, non-static distribution of matter-energy such as a rotating star affects the orbital motion of a nearby test particle like, e.g., a planet p in such a way that its trajectory is not closed, as in the case of the unchanging Keplerian ellipse of the Newtonian mechanics. Among other things, there are two types of resulting secular effects to the first post-Newtonian (1pN) order: the static “gravitoelectric” (GE) Einstein precession [] of the pericenter due solely to the total mass M of the system, and the stationary “gravitomagnetic” Lense-Thirring (LT) precessions [] of the longitude of the ascending node , and the pericenter caused by the proper angular momentum of the spinning central object (see, e.g., [,]. In general, the pN gravitomagnetic field is generated by mass-energy currents); for the concept of gravitoelectromagnetism within general relativity, see, e.g., [,,,,,,,,,,,,], and references therein. The gravitoelectric and gravitomagnetic net shifts per orbit of the planet’s pericentre are [,,]
where G is the Newtonian constant of gravitation, c is the speed of light in vacuum, is the planet’s semimajor axis, is the planet’s eccentricity, is the inclination of the planetary orbital plane to the reference plane, customarily identified with the plane of the sky,
is the planet’s Keplerian mean motion,
is a unit vector directed along the planet’s orbital angular momentum, and
is a unit vector in the planetary orbital plane perpendicular to the line of the nodes, which is the intersection of the orbital plane with the reference plane [,]. For the sake of completeness, also the Lense-Thirring node precession is mentioned: its net shift per orbit is [,]
As it turns out from Equations (1) and (2), the gravitoelectric effect is, in general, larger than the gravitomagnetic one; suffice it to say that in the case of Sun and Mercury 2 it is
while for the Earth and, say, the LAGEOS (L) satellite [], it is
This is why the gravitoelectric precessions have been known since the observations of the then anomalous Mercury’s motion by Le Verrier [] in the mid-nineteenth century, while the Lense-Thirring effect is still so difficult to measure with both natural and artificial objects [,].
Nevertheless, for planets orbiting a binary star [,], known as circumbinary planets (CBPs), some of which have already been discovered with different techniques [,,,,,,,,,,,,,,,,,,,,], certain gravitomagnetic effects may be much larger than expected, amounting to about or so of the gravitoelectric ones. The basic idea is as follows. The CBPs discovered so far can be considered as hierarchical triple systems consisting of an inner binary star b and a distant planet p that orbits the centre of mass of b. Thus, the two inner stars A and B can be approximately considered as a mass current sourcing a gravitomagnetic field much stronger than that due to the individual spins of each star through the binary’s orbital angular momentum
where
is the binary’s reduced mass, is the total mass of the binary, is the binary’s semimajor axis and is the binary’s eccentricity. Thus, it is expected that in Equation (2), calculated with 3 Equation (9), yields a much larger pericenter precession.
Testing relativistic frame dragging in as much ways as possible is important since it is believed to play important roles in several high-energy astrophysical phenomena in strong-field systems [,,,,,,]. Extending relativistic gravitomagnetism with confidence to such relatively unknown scenarios, for which no direct access is available, requires that it is corroborated in more than just a single case [].
2. The Gravitomagnetic Precessions Due to a Matter Ring Current
By imposing
with , yields the following condition for the semimajor axis of the planet’s orbit about the inner binary
As an example, for a binary of two Sun-like stars () in a circular orbit () and a Jupiter-type () circumbinary planet in a moderately eccentric orbit (), by imposing
one gets
which fulfils the assumption that the binary is viewed by the planet as a rotating matter ring. By setting
in Equation (12), corresponding to
yields
which implies that the gravitomagnetic precession cannot be as large as the gravitoelectric one. From Figure 1, it turns out that , and , for . Thus, for CBPs, this form of Lense-Thirring effect cannot reach the ≃30% of the gravitoelectric one.
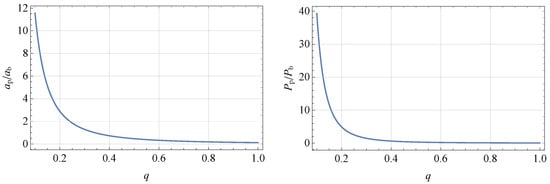
Figure 1.
Left panel: ratio of the planet’s semimajor axis to the inner binary’s one as a function of q according to Equation (12). Right panel: same for the orbital periods of the planet () and of the inner binary ().
According to [], most of the CBPs exhibit a high degree of coplanarity with the inner binary, i.e., and are almost aligned. Thus, the pericentre change of Equation (2) can be approximated by
while the node shift of Equation (6) almost vanishes. The precession of Equation (18) is always negative, i.e., the pericentre moves in the opposite direction of the motion of the iner binary. There are some special cases, reported in the literature, where negative orbital plane precessions were reported, e.g., in the presence of a Kerr naked singularity [] and of a hypothetical gravitomagnetic monopole []. Furthermore, ref. [] remarks that the mass of the primary star varies from to , with a mass ratio between and and eccentricity . As far as the CMPs are concerned, their orbital periods are in the range , with eccentricities varying from to []. In order to be stable around the binary host, the planet’s orbit must be characterized by , a condition that is fulfilled by the CBPs considered in []. However, from the point of view of a possible detection of the sought effect, the issue of the stability of a discovered CBP is not relevant since its lifetime, even if short in astronomical terms, is certainly much longer than any conceivable time span during which observations are collected. Figure 2 displays and , in , as functions of the binary’s orbital period , in d, by imposing the orbital stability condition . For the remaining physical and orbital parameters, the values were adopted. Among other things, also the binary’s semimajor axis and orbital angular momentum are shown; it can be noted that is about times larger than the spin angular momentum of the Sun which is of the order of [].
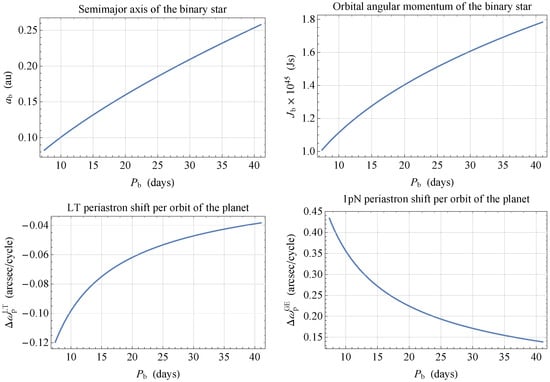
Figure 2.
Upper row: binary’s semimajor axis , in au, and orbital angular momentum , in J s, as functions of the binary’s orbital period ranging from to . Lower row: planet’s gravitomagnetic and gravitoelectric net shifts per orbit, in , as functions of the binary’s orbital period ranging from to . The orbital stability condition was adopted along with .
The size of the Lense-Thirring shift ranges from , while the gravitoelectric one is in the range 0.45–0.15 .
3. Conclusions
So far, a handful of circumbinary planets orbiting different types of stellar pairs, including compact objects as well, have been discovered; although for all of them the matter ring current approximation is substantially valid for their hosting stellar pairs, they are likely too distant from them to allow for a measurement of relativistic effects. Nonetheless, there may be reasons for being somewhat optimistic.
From the one hand, it is not unrealistic to expect that in a not too far future one or more systems with the right characteristics will be at our disposal.
On the other hand, there is a growing interest in the community of extrasolar planetary scientists about the possibility of extracting general relativistic signatures in such scenarios as well [,,,,,,,,,,,,,,,].
Funding
This research received no external funding.
Data Availability Statement
Not applicable.
Acknowledgments
I gratefully thanks B. Mashhoon for constructive remarks.
Conflicts of Interest
The authors declare no conflict of interest.
Notes
| 1 | For a recent overview, see, e.g., [] and references therein. |
| 2 | |
| 3 |
References
- Debono, I.; Smoot, G.F. General Relativity and Cosmology: Unsolved Questions and Future Directions. Universe 2016, 2, 23. [Google Scholar] [CrossRef]
- Einstein, A. Erklärung der Perihelbewegung des Merkur aus der allgemeinen Relativitätstheorie. Sitzungsberichte Preußischen Akad. Wiss. 1915, 47, 831–839. [Google Scholar]
- Lense, J.; Thirring, H. Über den Einfluß der Eigenrotation der Zentralkörper auf die Bewegung der Planeten und Monde nach der Einsteinschen Gravitationstheorie. Phys. Z. 1918, 19, 156–163. [Google Scholar]
- Brumberg, V.A. Essential Relativistic Celestial Mechanics; Adam Hilger: Bristol, UK, 1991. [Google Scholar]
- Soffel, M.H.; Han, W.B. Applied General Relativity; Astronomy and Astrophysics Library; Springer Nature: Cham, Switzerland, 2019. [Google Scholar]
- Cattaneo, C. General relativity: Relative standard mass, momentum, energy and gravitational field in a general system of reference. Il Nuovo Cimento 1958, 10, 318–337. [Google Scholar] [CrossRef]
- Thorne, K.S.; MacDonald, D.A.; Price, R.H. (Eds.) Black Holes: The Membrane Paradigm; Yale University Press: New Haven, CT, USA, 1986. [Google Scholar]
- Thorne, K.S. Black Holes: The Membrane Viewpoint. In Highlights of Modern Astrophysics: Concepts and Controversies; Shapiro, S.L., Teukolsky, S.A., Salpeter, E.E., Eds.; Wiley: New, York, NY, USA, 1986; pp. 103–161. [Google Scholar]
- Thorne, K.S. Gravitomagnetism, jets in quasars, and the Stanford Gyroscope Experiment. In Near Zero: New Frontiers of Physics; Fairbank, J.D., Deaver, B.S.J., Everitt, C.W.F., Michelson, P.F., Eds.; Freeman: New York, NY, USA, 1988; pp. 573–586. [Google Scholar]
- Harris, E.G. Analogy between general relativity and electromagnetism for slowly moving particles in weak gravitational fields. Am. J. Phys. 1991, 59, 421–425. [Google Scholar] [CrossRef]
- Jantzen, R.T.; Carini, P.; Bini, D. The many faces of gravitoelectromagnetism. Ann. Phys. 1992, 215, 1–50. [Google Scholar] [CrossRef]
- Mashhoon, B. Gravitoelectromagnetism. In Reference Frames and Gravitomagnetism, Proceedings of the XXIII Spanish Relativity Meeting, Valladolid, Spain, 6–9 September 2001; Pascual-Sánchez, J.F., Floría, L., San Miguel, A., Vicente, F., Eds.; World Scientific: Singapore, 2001; pp. 121–132. [Google Scholar] [CrossRef]
- Rindler, W. Relativity: Special, General, and Cosmological; Oxford University Press: Oxford, UK, 2001. [Google Scholar]
- Mashhoon, B. Gravitoelectromagnetism: A Brief Review. In The Measurement of Gravitomagnetism: A Challenging Enterprise; Iorio, L., Ed.; Nova Science: New York, NY, USA, 2007; pp. 29–39. [Google Scholar]
- Costa, L.F.O.; Herdeiro, C.A.R. Gravitoelectromagnetic analogy based on tidal tensors. Phys. Rev. D 2008, 78, 024021. [Google Scholar] [CrossRef]
- Costa, L.F.O.; Natário, J. Gravito-electromagnetic analogies. Gen. Relativ. Gravit. 2014, 46, 1792. [Google Scholar] [CrossRef]
- Costa, L.F.O.; Natário, J. Frame-Dragging: Meaning, Myths, and Misconceptions. Universe 2021, 7, 388. [Google Scholar] [CrossRef]
- Ruggiero, M.L. A Note on the Gravitoelectromagnetic Analogy. Universe 2021, 7, 451. [Google Scholar] [CrossRef]
- Barker, B.M.; O’Connell, R.F. Gravitational two-body problem with arbitrary masses, spins, and quadrupole moments. Phys. Rev. D 1975, 12, 329–335. [Google Scholar] [CrossRef]
- Damour, T.; Schäfer, G. Higher-order relativistic periastron advances and binary pulsars. Il Nuovo Cimento B 1988, 101, 127–176. [Google Scholar] [CrossRef]
- Iorio, L. Post-Keplerian perturbations of the orbital time shift in binary pulsars: An analytical formulation with applications to the galactic center. Eur. Phys. J. C 2017, 77, 439. [Google Scholar] [CrossRef]
- Lucchesi, D.M.; Anselmo, L.; Bassan, M.; Magnafico, C.; Pardini, C.; Peron, R.; Pucacco, G.; Visco, M. General Relativity measurements in the field of Earth with laser-ranged satellites: State of the art and perspectives. Universe 2019, 5, 141. [Google Scholar] [CrossRef]
- Le Verrier, U.J. Lettre de M. Le Verrier à M. Faye sur la théorie de Mercure et sur le mouvement du périhélie de cette planète. Comptes Rendus Hebd. Séances l’Académie Sci. 1959, 49, 379–383. [Google Scholar]
- Renzetti, G. History of the attempts to measure orbital frame–dragging with artificial satellites. Cent. Eur. J. Phys. 2013, 11, 531–544. [Google Scholar] [CrossRef]
- Everitt, C.W.F.; Debra, D.B.; Parkinson, B.W.; Turneaure, J.P.; Conklin, J.W.; Heifetz, M.I.; Keiser, G.M.; Silbergleit, A.S.; Holmes, T.; Kolodziejczak, J.; et al. Gravity Probe B: Final Results of a Space Experiment to Test General Relativity. Phys. Rev. Lett. 2011, 106, 221101. [Google Scholar] [CrossRef]
- Haghighipour, N. (Ed.) Planets in Binary Star Systems. In Astrophysics and Space Science Library; Springer: Berlin, Germany, 2010; Volume 366. [Google Scholar] [CrossRef]
- Thebault, P.; Haghighipour, N. Planet Formation in Binaries. In Planetary Exploration and Science: Recent Results and Advances; Jin, S., Haghighipour, N., Ip, W., Eds.; Springer: Heidelberg, Germany, 2015; pp. 309–340. [Google Scholar] [CrossRef]
- Thorsett, S.E.; Arzoumanian, Z.; Taylor, J.H. PSR B1620-26—A binary radio pulsar with a planetary companion? Astrophys. J. Lett. 1993, 412, L33–L36. [Google Scholar] [CrossRef]
- Correia, A.C.M.; Udry, S.; Mayor, M.; Laskar, J.; Naef, D.; Pepe, F.; Queloz, D.; Santos, N.C. The CORALIE survey for southern extra-solar planets. XIII. A pair of planets around HD 202206 or a circumbinary planet? Astron. Astrophys. 2005, 440, 751–758. [Google Scholar] [CrossRef]
- Lee, J.W.; Kim, S.L.; Kim, C.H.; Koch, R.H.; Lee, C.U.; Kim, H.I.; Park, J.H. The sdB+M Eclipsing System HW Virginis and its Circumbinary Planets. Astromomical J. 2009, 137, 3181–3190. [Google Scholar] [CrossRef]
- Qian, S.B.; Liao, W.P.; Zhu, L.Y.; Dai, Z.B. Detection of a Giant Extrasolar Planet Orbiting the Eclipsing Polar DP Leo. Astrophys. J. Lett. 2010, 708, L66–L68. [Google Scholar] [CrossRef]
- Beuermann, K.; Hessman, F.V.; Dreizler, S.; Marsh, T.R.; Parsons, S.G.; Winget, D.E.; Miller, G.F.; Schreiber, M.R.; Kley, W.; Dhillon, V.S.; et al. Two planets orbiting the recently formed post-common envelope binary NN Serpentis. Astron. Astrophys. 2010, 521, L60. [Google Scholar] [CrossRef][Green Version]
- Doyle, L.R.; Carter, J.A.; Fabrycky, D.C.; Slawson, R.W.; Howell, S.B.; Winn, J.N.; Orosz, J.A.; Prša, A.; Welsh, W.F.; Quinn, S.N.; et al. Kepler-16: A Transiting Circumbinary Planet. Science 2011, 333, 1602–1606. [Google Scholar] [CrossRef] [PubMed]
- Orosz, J.A.; Welsh, W.F.; Carter, J.A.; Brugamyer, E.; Buchhave, L.A.; Cochran, W.D.; Endl, M.; Ford, E.B.; MacQueen, P.; Short, D.R.; et al. The Neptune-sized Circumbinary Planet Kepler-38b. Astrophys. J. 2012, 758, 87. [Google Scholar] [CrossRef]
- Orosz, J.A.; Welsh, W.F.; Carter, J.A.; Fabrycky, D.C.; Cochran, W.D.; Endl, M.; Ford, E.B.; Haghighipour, N.; MacQueen, P.J.; Mazeh, T.; et al. Kepler-47: A Transiting Circumbinary Multiplanet System. Science 2012, 337, 1511. [Google Scholar] [CrossRef] [PubMed]
- Qian, S.B.; Zhu, L.Y.; Dai, Z.B.; Fernández-Lajús, E.; Xiang, F.Y.; He, J.J. Circumbinary Planets Orbiting the Rapidly Pulsating Subdwarf B-type Binary NY Vir. Astrophys. J. Lett. 2012, 745, L23. [Google Scholar] [CrossRef]
- Qian, S.B.; Liu, L.; Zhu, L.Y.; Dai, Z.B.; Fernández Lajús, E.; Baume, G.L. A circumbinary planet in orbit around the short-period white dwarf eclipsing binary RR Cae. Mon. Not. R. Astron. Soc. 2012, 422, L24–L27. [Google Scholar] [CrossRef]
- Welsh, W.F.; Orosz, J.A.; Carter, J.A.; Fabrycky, D.C.; Ford, E.B.; Lissauer, J.J.; Prša, A.; Quinn, S.N.; Ragozzine, D.; Short, D.R.; et al. Transiting circumbinary planets Kepler-34 b and Kepler-35 b. Nature 2012, 481, 475–479. [Google Scholar] [CrossRef]
- Schwamb, M.E.; Orosz, J.A.; Carter, J.A.; Welsh, W.F.; Fischer, D.A.; Torres, G.; Howard, A.W.; Crepp, J.R.; Keel, W.C.; Lintott, C.J.; et al. Planet Hunters: A Transiting Circumbinary Planet in a Quadruple Star System. Astrophys. J. 2013, 768, 127. [Google Scholar] [CrossRef]
- Kraus, A.L.; Ireland, M.J.; Cieza, L.A.; Hinkley, S.; Dupuy, T.J.; Bowler, B.P.; Liu, M.C. Three Wide Planetary-mass Companions to FW Tau, ROXs 12, and ROXs 42B. Astrophys. J. 2014, 781, 20. [Google Scholar] [CrossRef]
- Kostov, V.B.; McCullough, P.R.; Carter, J.A.; Deleuil, M.; Díaz, R.F.; Fabrycky, D.C.; Hébrard, G.; Hinse, T.C.; Mazeh, T.; Orosz, J.A.; et al. Kepler-413b: A Slightly Misaligned, Neptune-size Transiting Circumbinary Planet. Astrophys. J. 2014, 784, 14. [Google Scholar] [CrossRef]
- Welsh, W.F.; Orosz, J.A.; Short, D.R.; Cochran, W.D.; Endl, M.; Brugamyer, E.; Haghighipour, N.; Buchhave, L.A.; Doyle, L.R.; Fabrycky, D.C.; et al. Kepler 453 b—The 10th Kepler Transiting Circumbinary Planet. Astrophys. J. 2015, 809, 26. [Google Scholar] [CrossRef]
- Bennett, D.P.; Rhie, S.H.; Udalski, A.; Gould, A.; Tsapras, Y.; Kubas, D.; Bond, I.A.; Greenhill, J.; Cassan, A.; Rattenbury, N.J.; et al. The First Circumbinary Planet Found by Microlensing: OGLE-2007-BLG-349L(AB)c. Astron. J. 2016, 152, 125. [Google Scholar] [CrossRef]
- Kostov, V.B.; Orosz, J.A.; Welsh, W.F.; Doyle, L.R.; Fabrycky, D.C.; Haghighipour, N.; Quarles, B.; Short, D.R.; Cochran, W.D.; Endl, M.; et al. Kepler-1647b: The Largest and Longest-period Kepler Transiting Circumbinary Planet. Astrophys. J. 2016, 827, 86. [Google Scholar] [CrossRef]
- Getley, A.K.; Carter, B.; King, R.; O’Toole, S. Evidence for a planetary mass third body orbiting the binary star KIC 5095269. Mon. Not. R. Astron. Soc. 2017, 468, 2932–2937. [Google Scholar] [CrossRef]
- Jain, C.; Paul, B.; Sharma, R.; Jaleel, A.; Dutta, A. Indication of a massive circumbinary planet orbiting the low-mass X-ray binary MXB 1658-298. Mon. Not. R. Astron. Soc. 2017, 468, L118–L122. [Google Scholar] [CrossRef]
- Asensio-Torres, R.; Janson, M.; Bonavita, M.; Desidera, S.; Thalmann, C.; Kuzuhara, M.; Henning, T.; Marzari, F.; Meyer, M.R.; Calissendorff, P.; et al. SPOTS: The Search for Planets Orbiting Two Stars. III. Complete sample and statistical analysis. Astron. Astrophys. 2018, 619, A43. [Google Scholar] [CrossRef]
- Kostov, V.B.; Powell, B.P.; Orosz, J.A.; Welsh, W.F.; Cochran, W.; Collins, K.A.; Endl, M.; Hellier, C.; Latham, D.W.; MacQueen, P.; et al. TIC 172900988: A Transiting Circumbinary Planet Detected in One Sector of TESS Data. Astron. J. 2021, 162, 234. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Petterson, J.A. The Lense-Thirring Effect and Accretion Disks around Kerr Black Holes. Astrophys. J. 1975, 195, L65–L67. [Google Scholar] [CrossRef]
- Stella, L.; Vietri, M. Lense-Thirring Precession and Quasi-periodic Oscillations in Low-Mass X-ray Binaries. Astrophys. J. Lett. 1998, 492, L59–L62. [Google Scholar] [CrossRef]
- Penrose, R. “Golden Oldie”: Gravitational Collapse: The Role of General Relativity. Gen. Relativ. Gravit. 2002, 34, 1141–1165. [Google Scholar] [CrossRef]
- Schäfer, G. Gravitomagnetism in Physics and Astrophysics. Space Sci. Rev. 2009, 148, 37–52. [Google Scholar] [CrossRef]
- Stella, L.; Possenti, A. Lense-Thirring Precession in the Astrophysical Context. Space Sci. Rev. 2009, 148, 105–121. [Google Scholar] [CrossRef]
- Welsh, W.F.; Orosz, J.A.; Carter, J.A.; Fabrycky, D.C. Recent Kepler Results On Circumbinary Planets. In Formation, Detection, and Characterization of Extrasolar Habitable Planets; Haghighipour, N., Ed.; Cambridge University Press: Cambridge, UK, 2014; Volume 293, pp. 125–132. [Google Scholar] [CrossRef]
- Chakraborty, C.; Kocherlakota, P.; Patil, M.; Bhattacharyya, S.; Joshi, P.S.; Królak, A. Distinguishing Kerr naked singularities and black holes using the spin precession of a test gyro in strong gravitational fields. Phys. Rev. D 2017, 95, 084024. [Google Scholar] [CrossRef]
- Chakraborty, C.; Bhattacharyya, S. Does the gravitomagnetic monopole exist? A clue from a black hole X-ray binary. Phys. Rev. D 2018, 98, 043021. [Google Scholar] [CrossRef]
- Pijpers, F.P. Helioseismic determination of the solar gravitational quadrupole moment. Mon. Not. R. Astron. Soc. 1998, 297, L76–L80. [Google Scholar] [CrossRef]
- Iorio, L. Are we far from testing general relativity with the transitting extrasolar planet HD 209458b “Osiris”? New Astron. 2006, 11, 490–494. [Google Scholar] [CrossRef]
- Jordán, A.; Bakos, G.Á. Observability of the General Relativistic Precession of Periastra in Exoplanets. Astrophys. J. 2008, 685, 543–552. [Google Scholar] [CrossRef]
- Pál, A.; Kocsis, B. Periastron precession measurements in transiting extrasolar planetary systems at the level of general relativity. Mon. Not. R. Astron. Soc. 2008, 389, 191–198. [Google Scholar] [CrossRef]
- Jordán, A.; Bakos, G.Á. Observability of the General Relativistic Precession of Periastra in Exoplanets. In Proceedings of the IAU Symposium, Rio de Janeiro, Brasil, 3–7 August 2009; Volume 253, pp. 492–495. [Google Scholar] [CrossRef]
- Ragozzine, D.; Wolf, A.S. Probing the Interiors of very Hot Jupiters Using Transit Light Curves. Astrophys. J. 2009, 698, 1778–1794. [Google Scholar] [CrossRef]
- Damiani, C.; Lanza, A.F. Prospecting transit duration variations in extrasolar planetary systems. Astron. Astrophys. 2011, 535, A116. [Google Scholar] [CrossRef]
- Fukui, A.; Narita, N.; Tristram, P.J.; Sumi, T.; Abe, F.; Itow, Y.; Sullivan, D.J.; Bond, I.A.; Hirano, T.; Tamura, M.; et al. Measurements of Transit Timing Variations for WASP-5b. Publ. Astron. Soc. Jpn. 2011, 63, 287–300. [Google Scholar] [CrossRef]
- Iorio, L. Classical and relativistic node precessional effects in WASP-33b and perspectives for detecting them. Astrophys. Space Sci. 2011, 331, 485–496. [Google Scholar] [CrossRef]
- Iorio, L. Classical and relativistic long-term time variations of some observables for transiting exoplanets. Mon. Not. R. Astron. Soc. 2011, 411, 167–183. [Google Scholar] [CrossRef]
- Eibe, M.T.; Cuesta, L.; Ullán, A.; Pérez-Verde, A.; Navas, J. Analysis of variations in transit time and transit duration in WASP-3. Evidence of secular perturbations reconsidered. Mon. Not. R. Astron. Soc. 2012, 423, 1381–1389. [Google Scholar] [CrossRef][Green Version]
- Kane, S.R.; Horner, J.; von Braun, K. Cyclic Transit Probabilities of Long-period Eccentric Planets due to Periastron Precession. Astrophys. J. 2012, 757, 105. [Google Scholar] [CrossRef][Green Version]
- Li, L.S. Parameterized post-Newtonian orbital effects in extrasolar planets. Astrophys. Space Sci. 2012, 341, 323–330. [Google Scholar] [CrossRef]
- Zhao, S.S.; Xie, Y. Parametrized post-Newtonian secular transit timing variations for exoplanets. Res. Astron. Astrophys. 2013, 13, 1231–1239. [Google Scholar] [CrossRef]
- Blanchet, L.; Hébrard, G.; Larrouturou, F. Detecting the general relativistic orbital precession of the exoplanet HD 80606b. Astron. Astrophys. 2019, 628, A80. [Google Scholar] [CrossRef]
- Antoniciello, G.; Borsato, L.; Lacedelli, G.; Nascimbeni, V.; Barragán, O.; Claudi, R. Detecting general relativistic orbital precession in transiting hot Jupiters. Mon. Not. R. Astron. Soc. 2021, 505, 1567–1574. [Google Scholar] [CrossRef]
- Gou, X.; Pan, X.; Wang, L. General Relativity Testing in Exoplanetary Systems. IOP Conf. Ser. Earth Environ. Sci. 2021, 658, 012051. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).