Co-Homology of Differential Forms and Feynman Diagrams
Abstract
1. Introduction
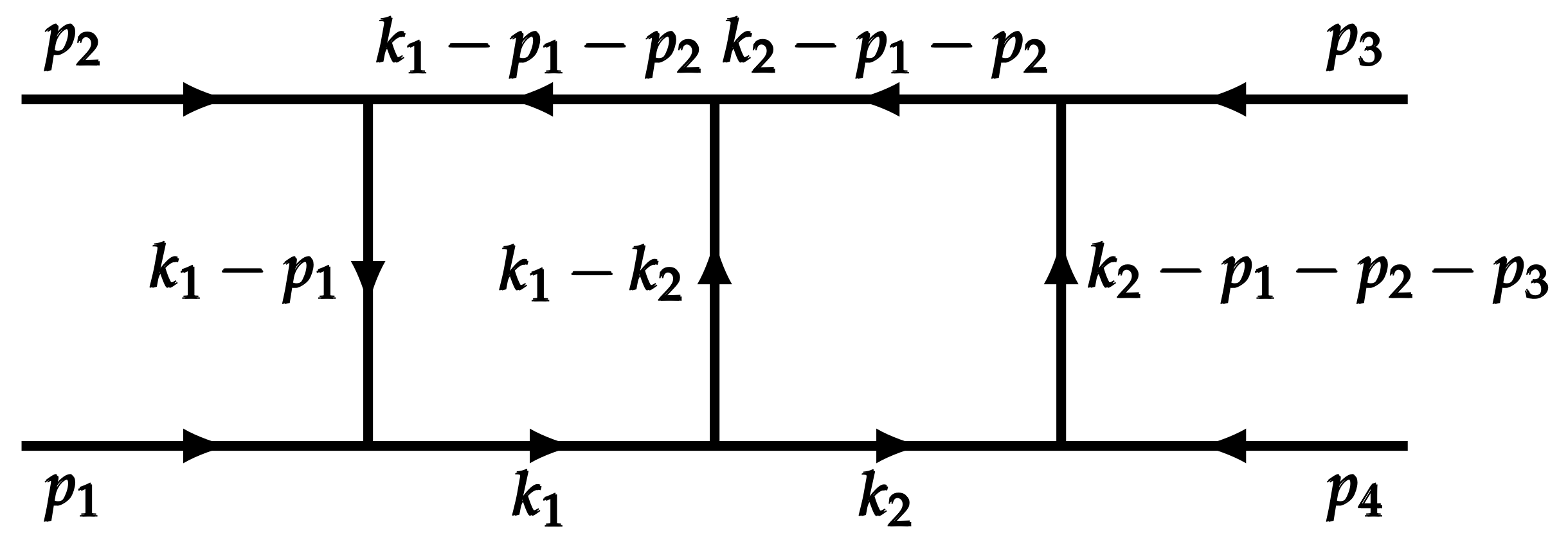
2. Feynman Integral Representation
3. The Twisted Cohomology Vector Space
3.1. Vector-Space Structure
3.2. Dual Cohomology Groups
3.3. Number of Master Integrals
3.4. Linear and Bilinear Identities
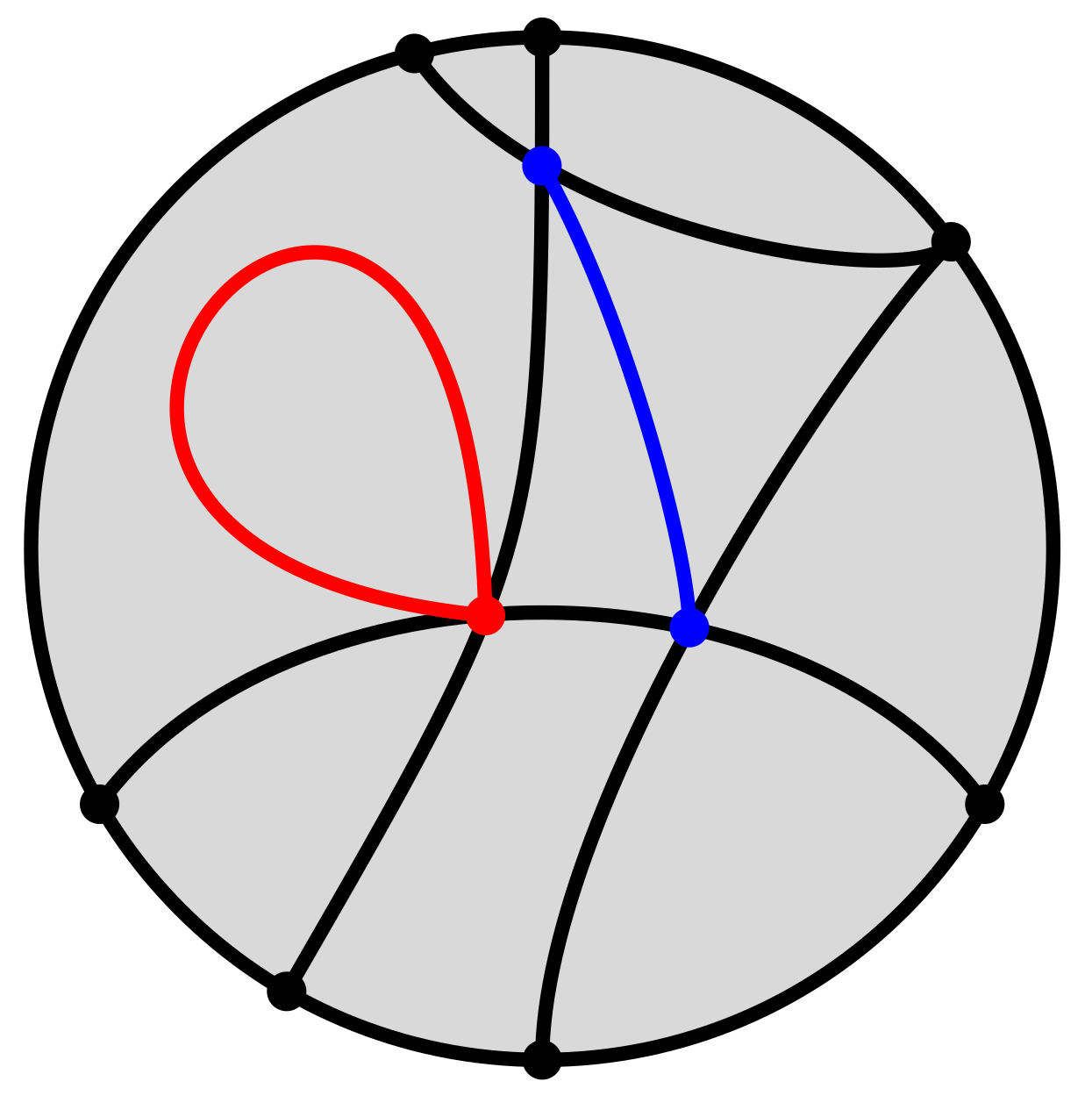
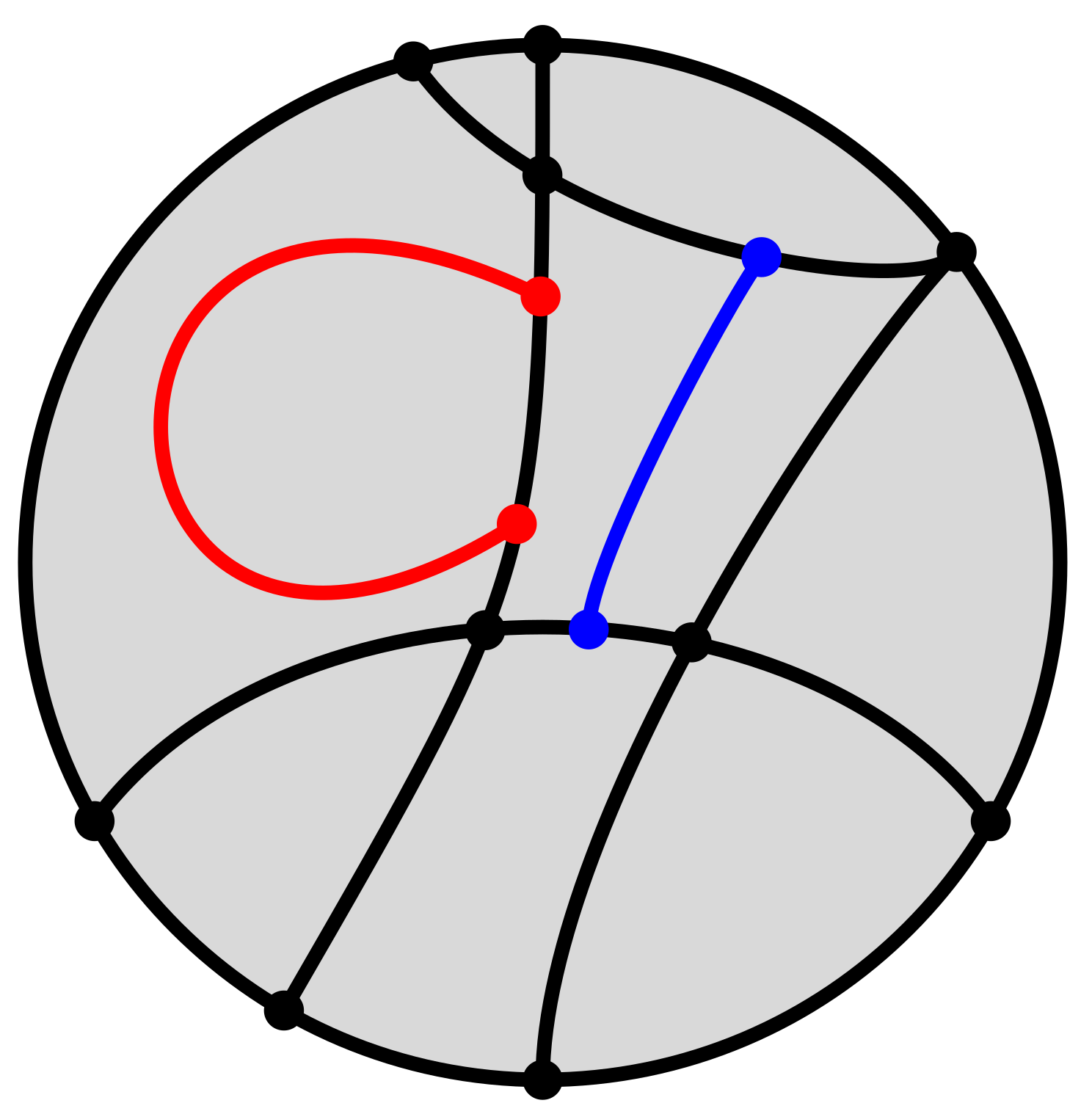
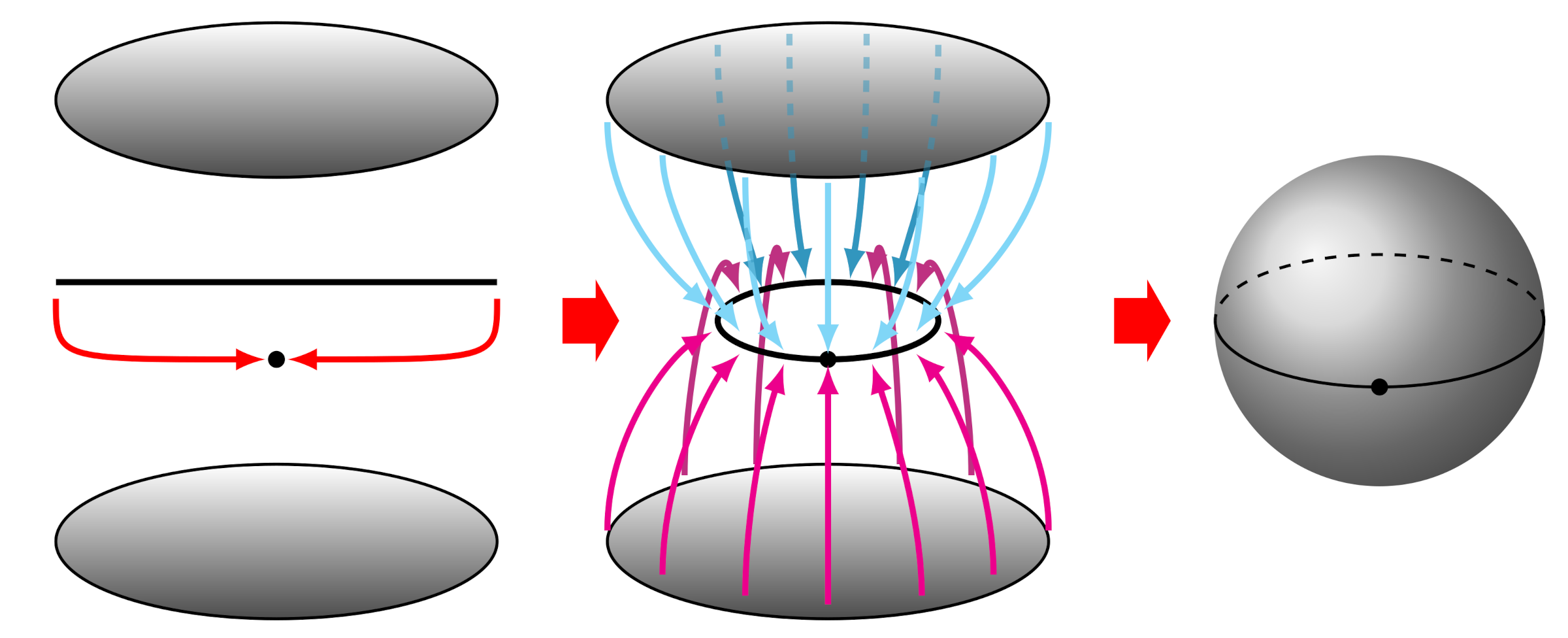
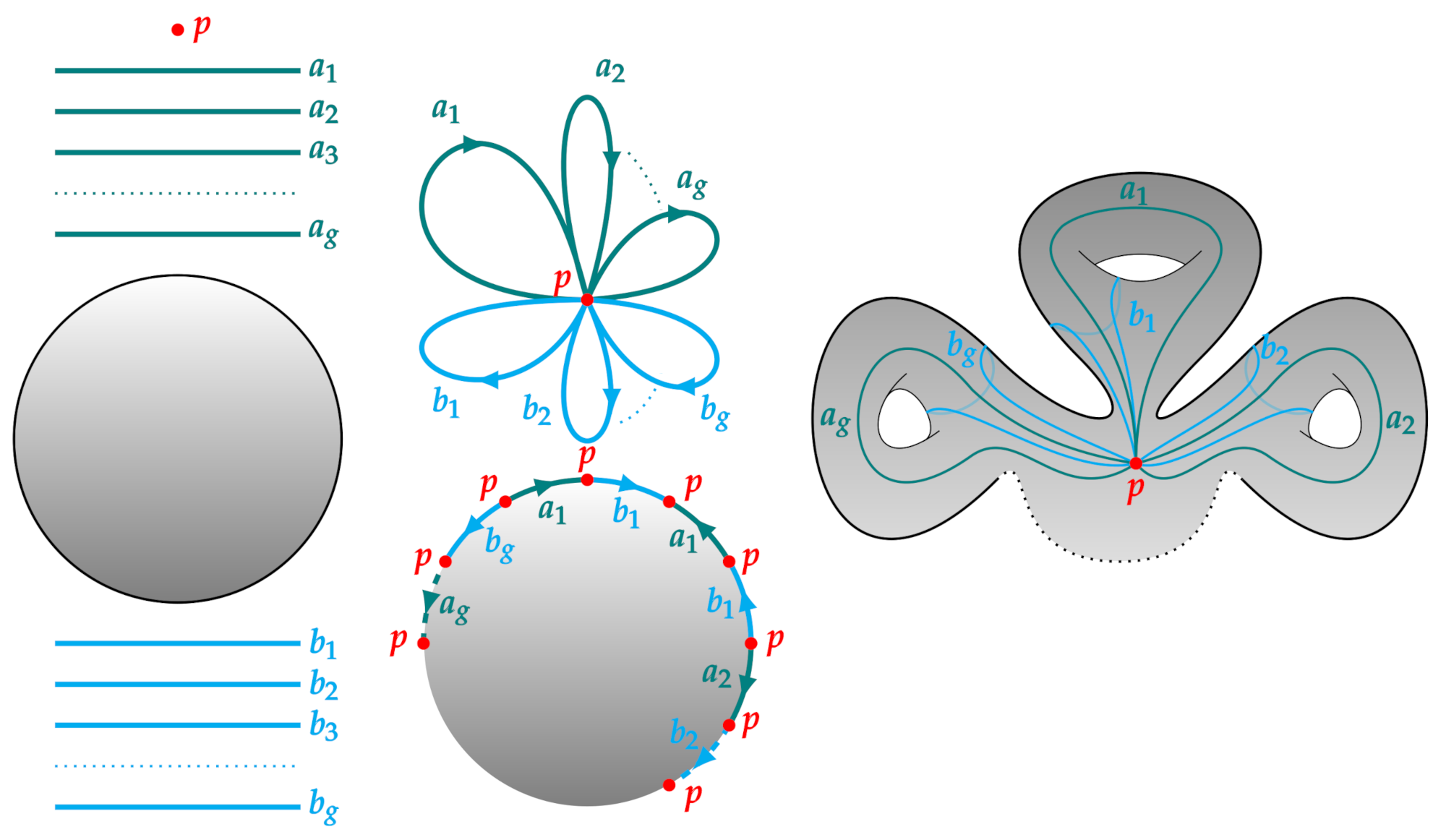
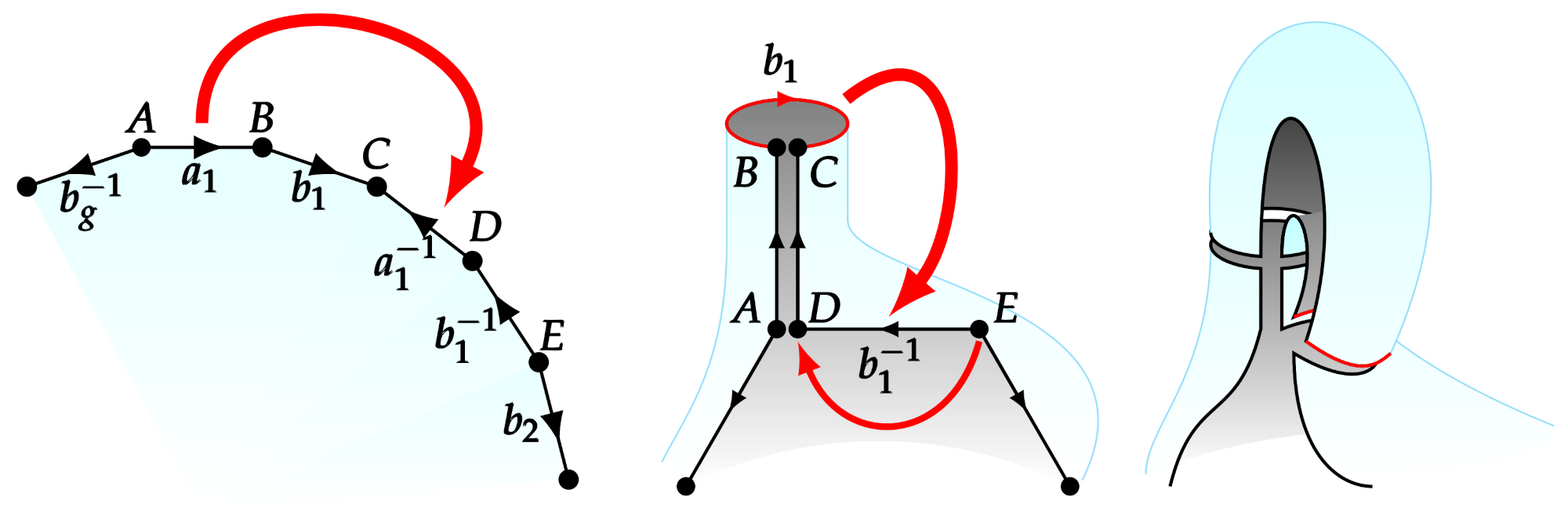
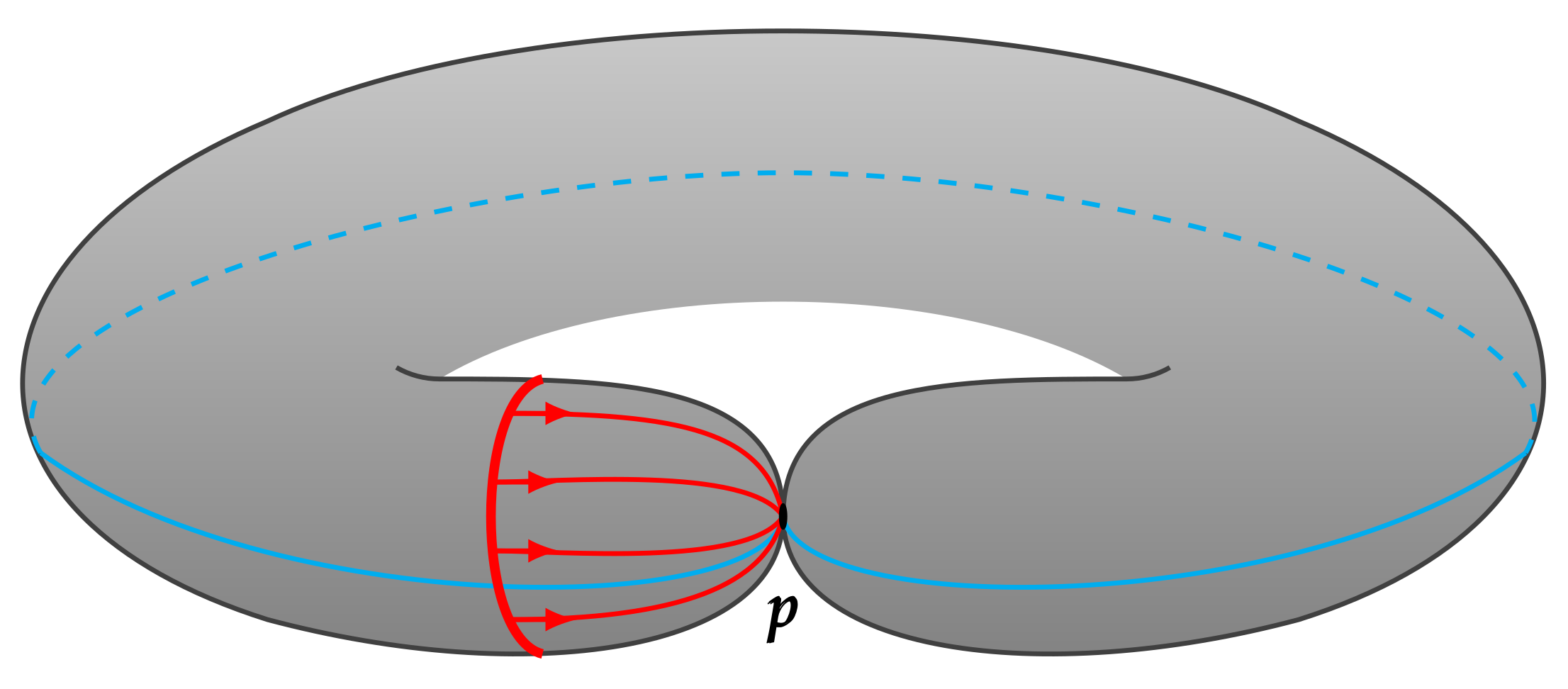
4. Pictorial (Co)Homology
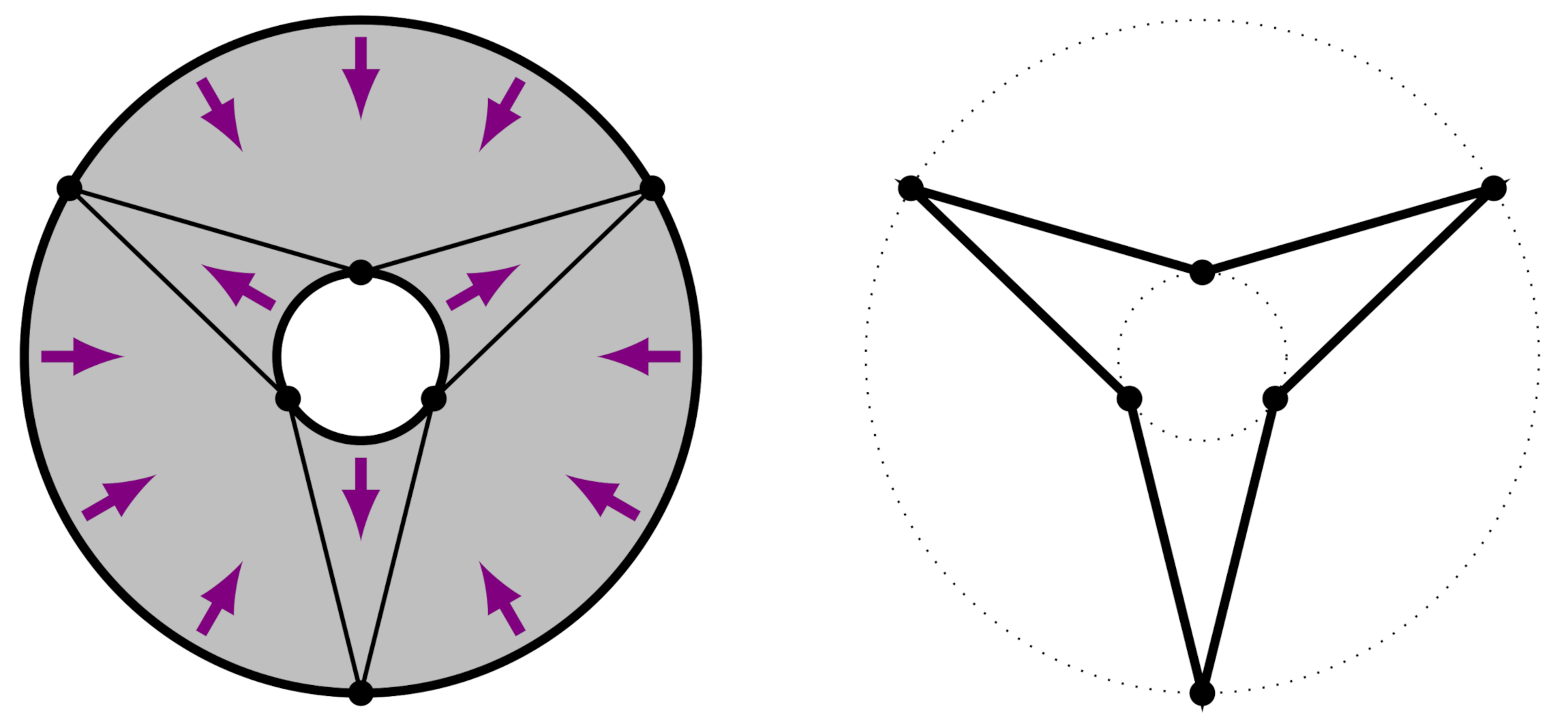
4.1. The Euler Characteristic
- The new edge starts and ends in already existing (and possibly coincident) vertices (Figure 2). In this case, the new edge cuts a face in two, and the new tessellation has , , so that ;
- The new edge extends from an already existing vertex to a new vertex attached to an existing edge (Figure 3). The new edge separates a face into two faces and the new vertex separates the old edge into two edges. Therefore, the new tessellation has , , , so that ;
- The new edge starts from and ends to a unique new vertex, inserted inTO an old edge (Figure 3). The new edge cuts a face into two, and the new vertex cuts the old edge into two. Therefore, the new tessellation has , , , so that ;
- the new edge extends from a new vertex to a different new vertex attached to two different or the same existing edge (Figure 4). The new edge separates a face into two faces. The new vertices separate two edges into two parts each or a unique edge in three. Therefore, the new tessellation has , , , so that .
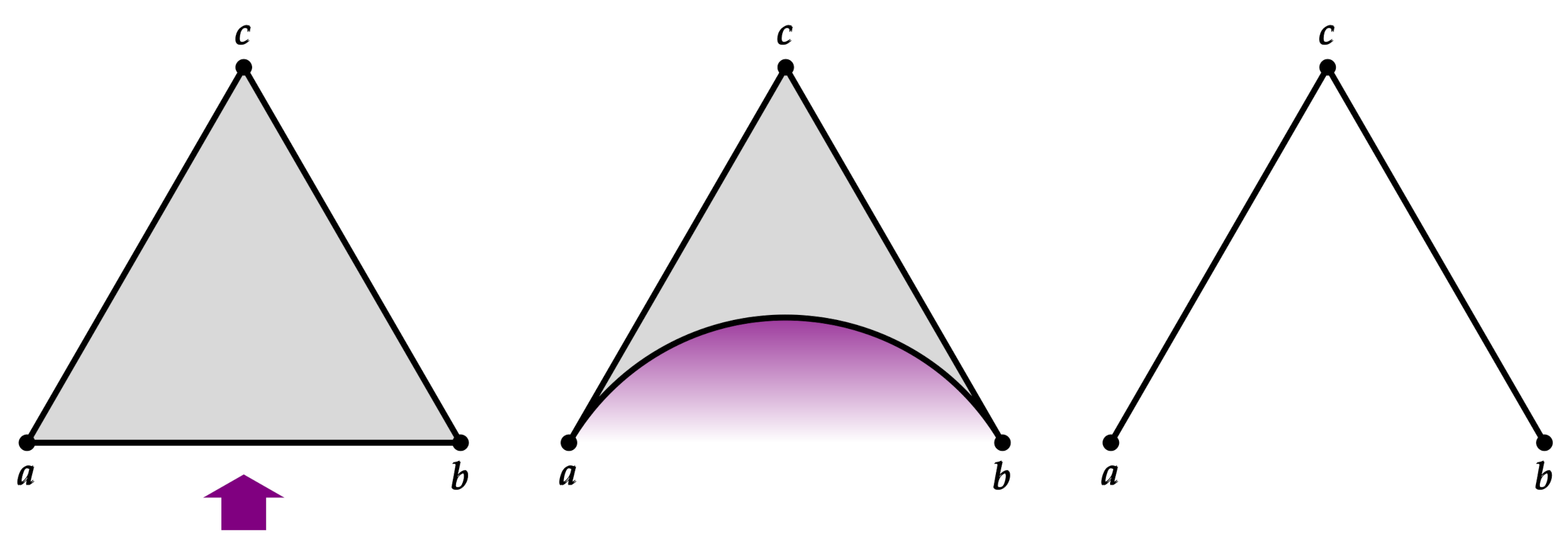
4.2. Simplicial (Co)homology
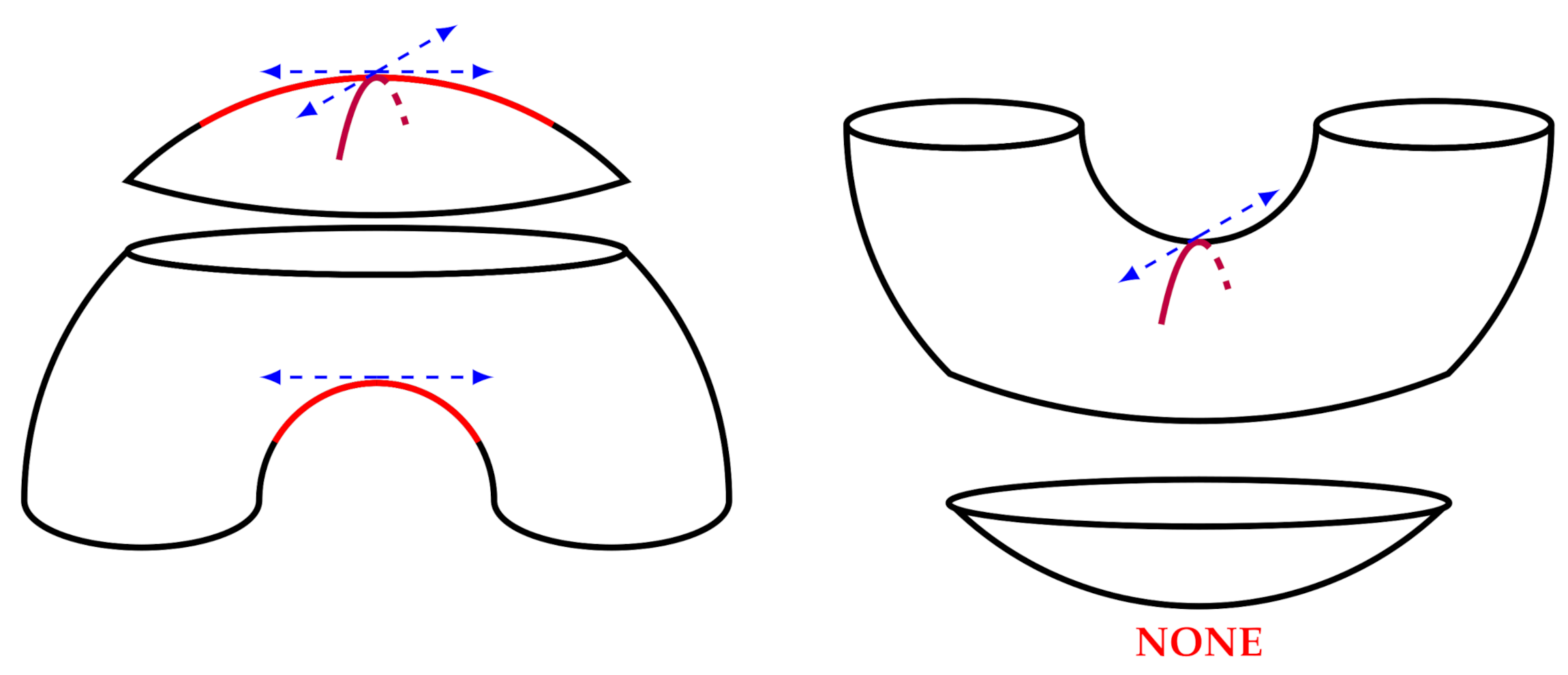
4.3. Morse Theory
- At , the Hessian is negative, so it has two principal directions going down (the eigenvectors). We say that has Morse index 2.
- At and , the Hessian is indefinite, it has only one negative eigenvalue at which it corresponds a descending direction. We say that and have Morse index 1.
- At , the Hessian is positive. There are not descending directions and we say that has Morse index 0.
4.4. Cellular (Co)homology
4.5. de Rham Cohomology
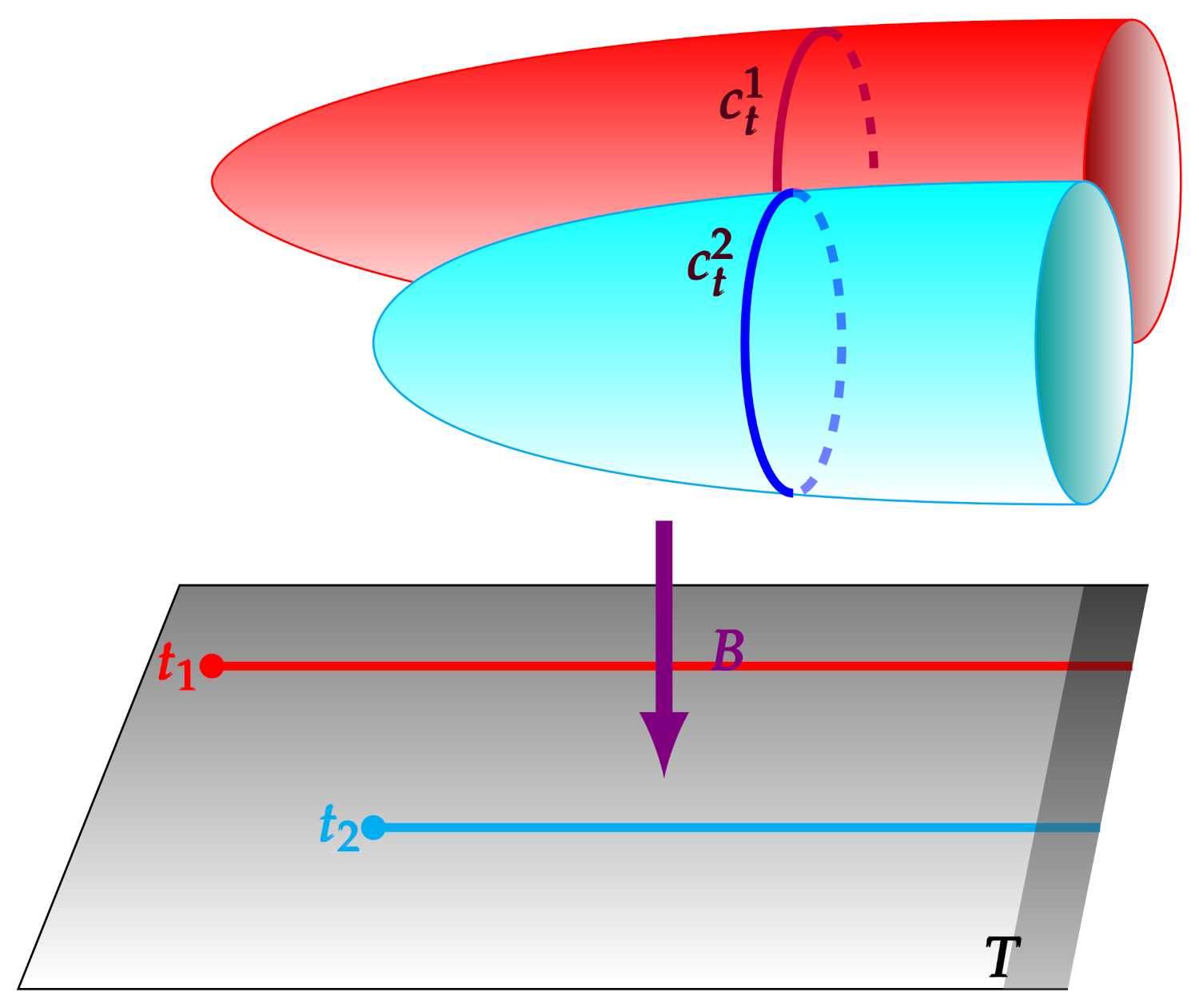
5. Integrals and Cohomologies
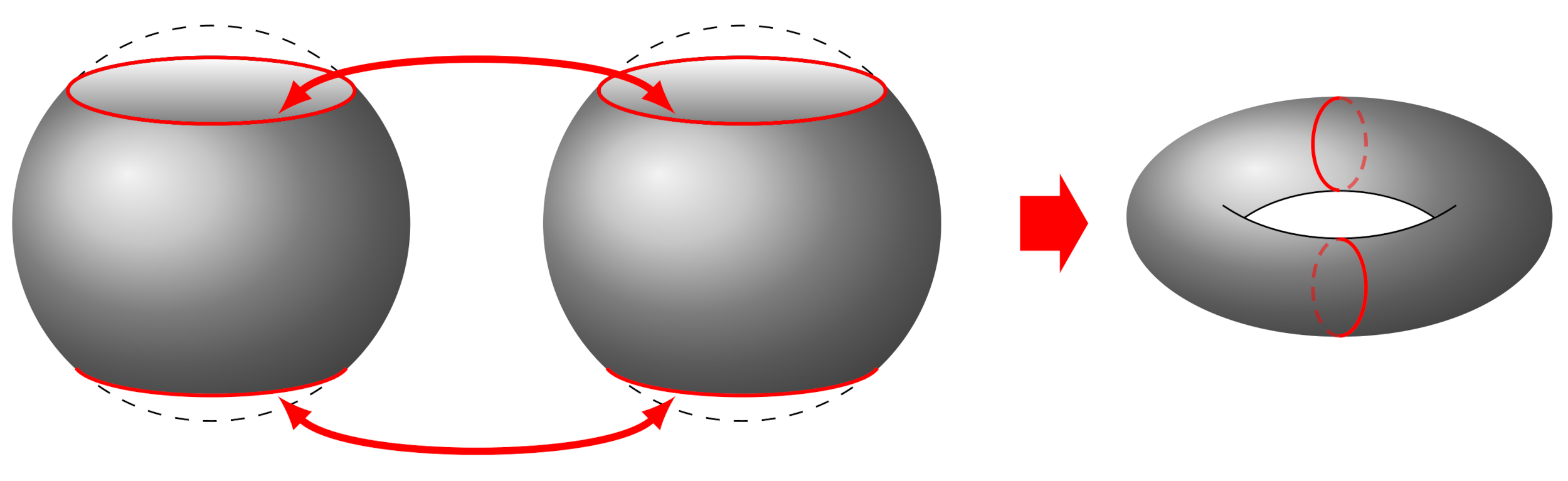
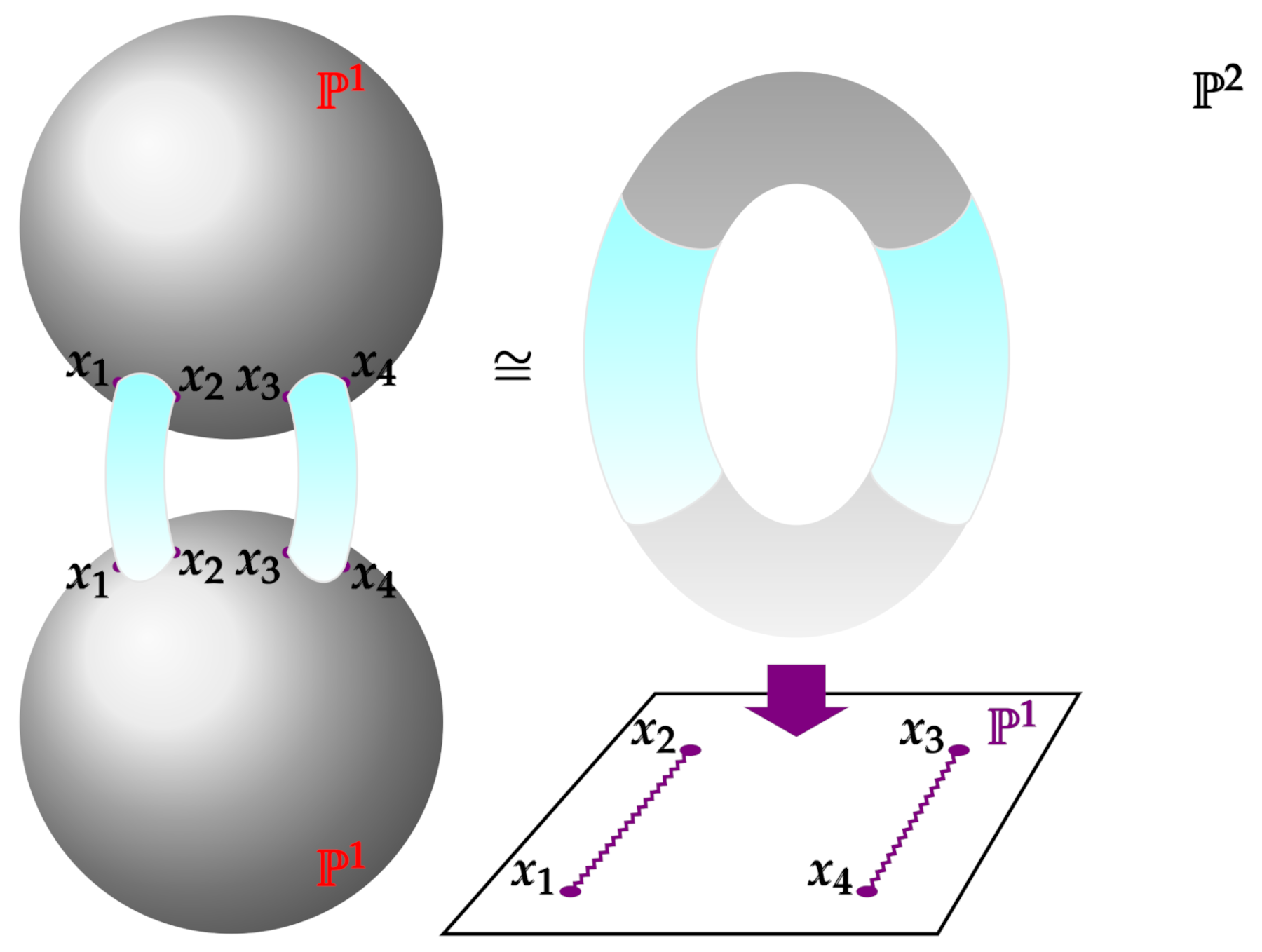
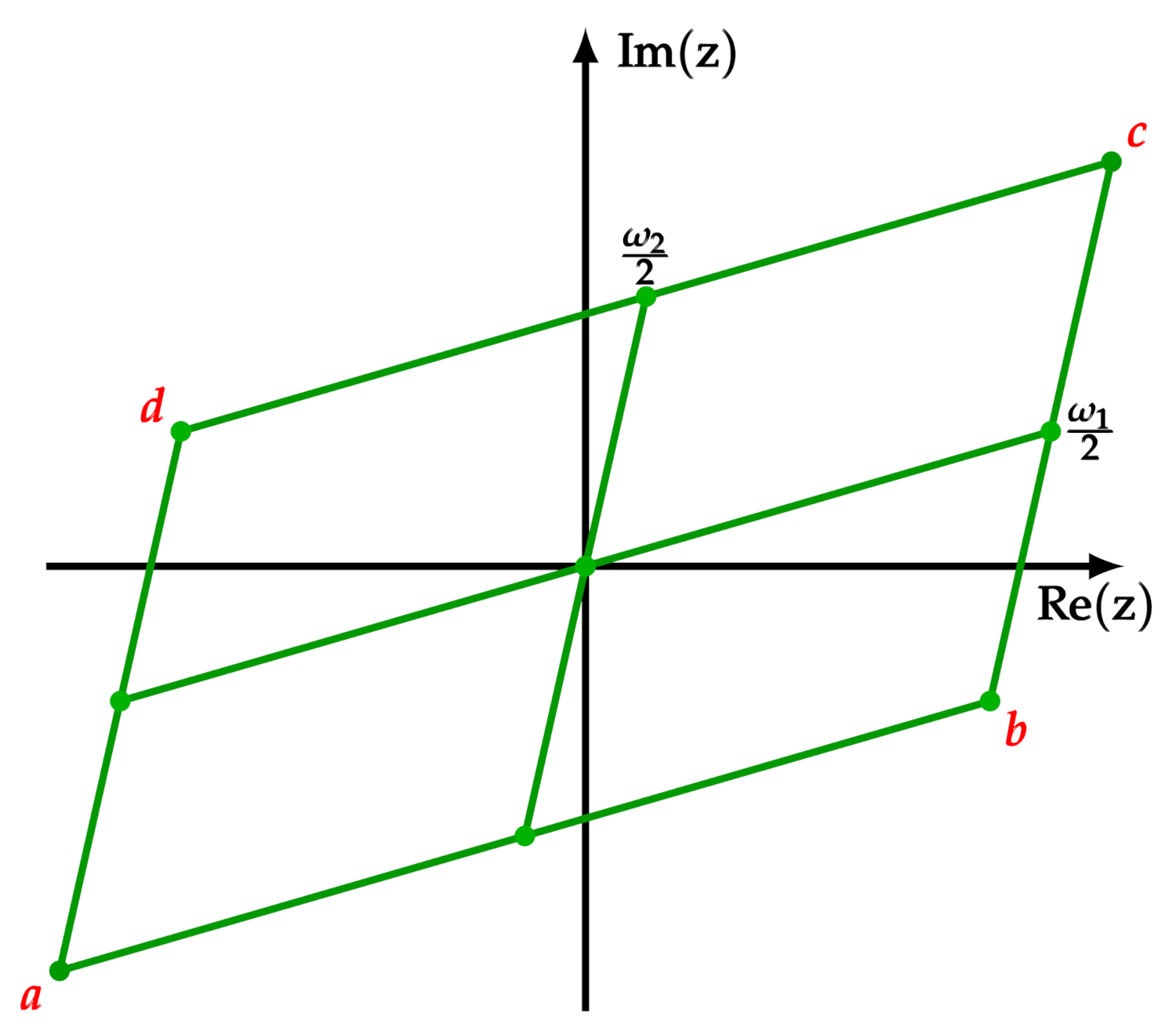
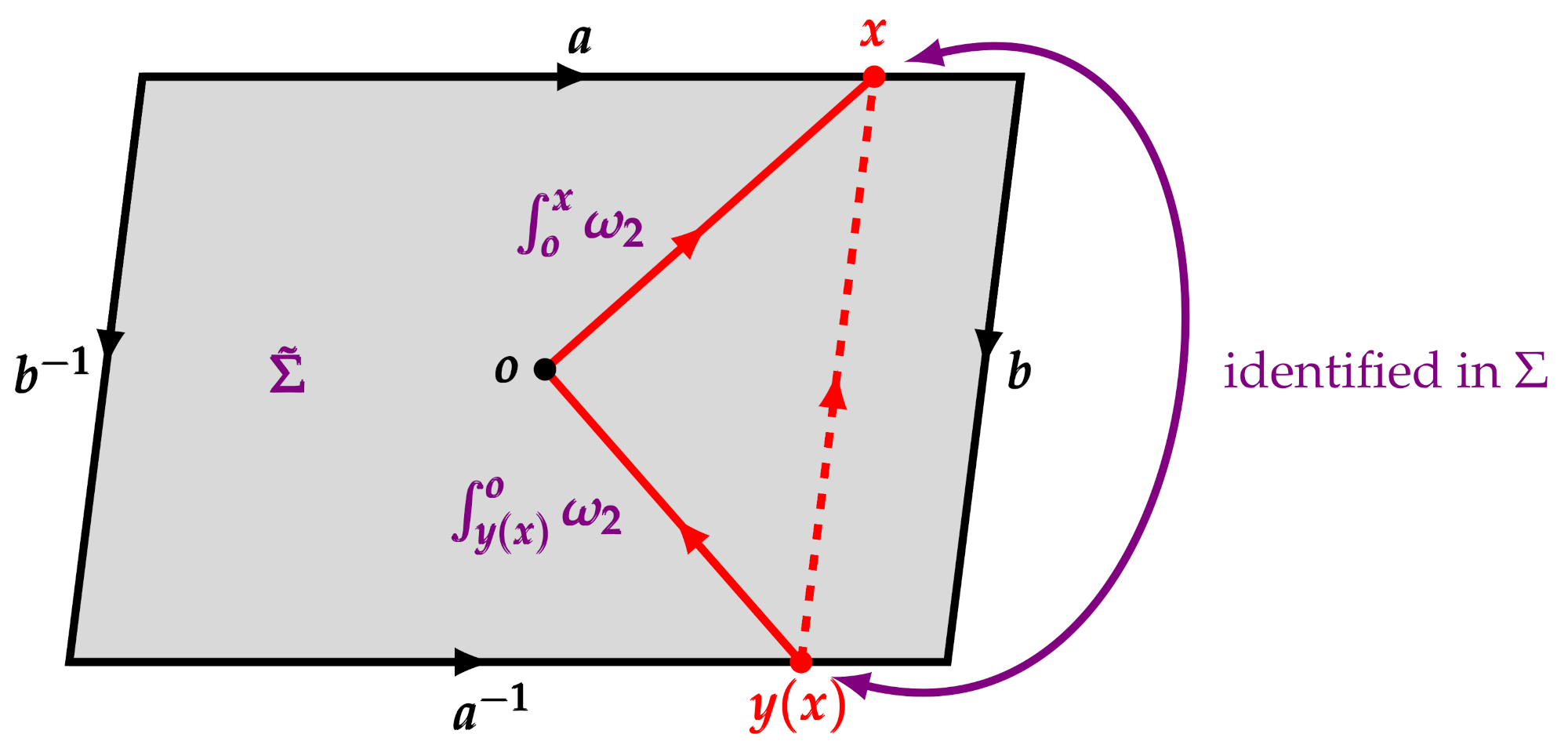
5.1. Elliptic Integrals
5.2. Riemann’s Bilinear Relations and Intersections
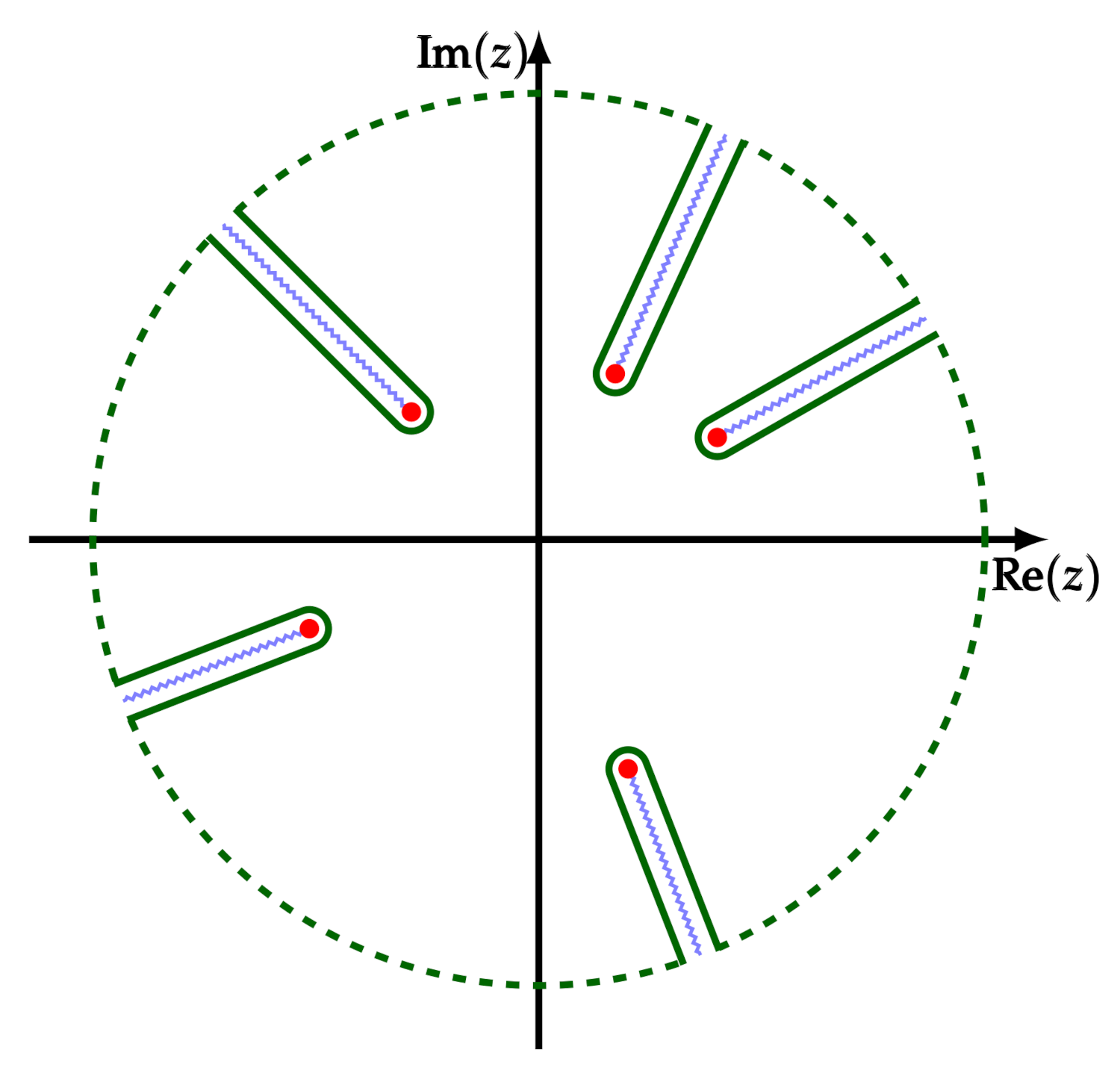
5.3. A Twisted Version
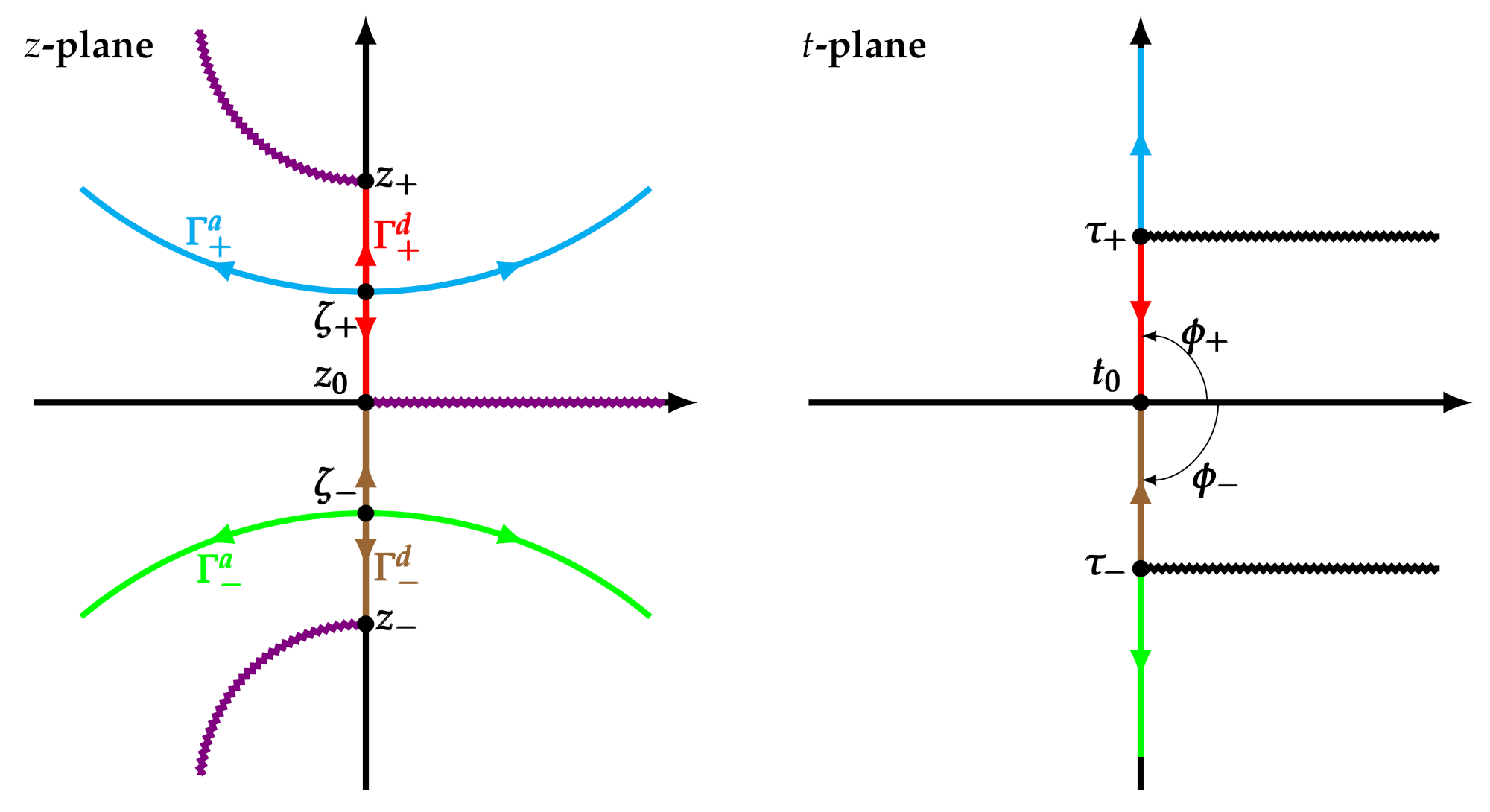
5.4. Perversities and Thimbles
6. Some General Constructions
6.1. On Cohomologies and de Rham Theorem
6.1.1. Singular (Co)homology
6.1.2. Simplicial (co)homology
6.1.3. De Rham Theorem
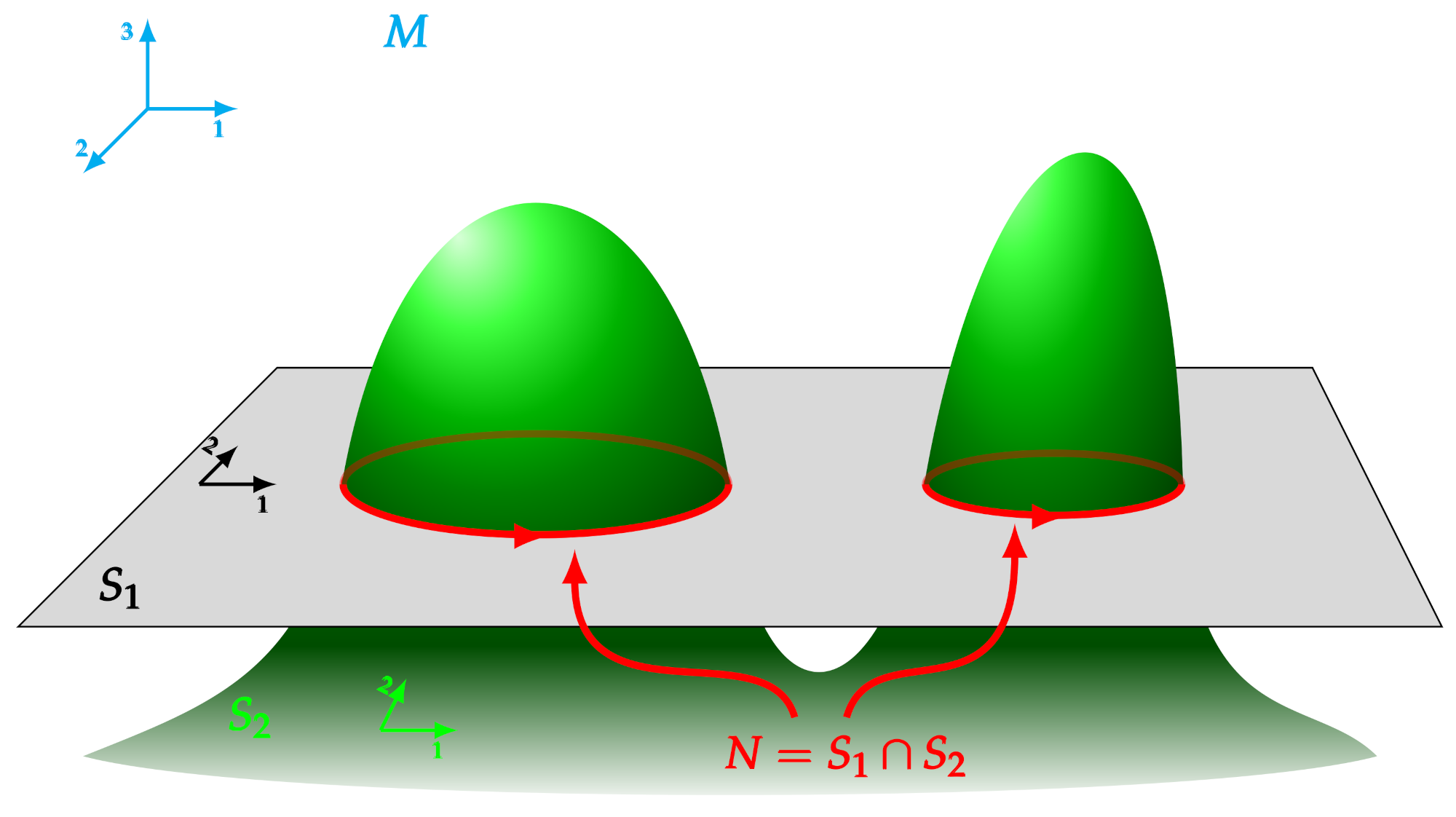
6.2. Cup Products and Intersections on Smooth Manifolds
- We can complete it to an oriented basis according to the orientation of ;
- We can complete it to an oriented basis according to the orientation of ;
- is an oriented basis for according to the orientation of M.
6.3. Lefschetz Theorems, Hodge-Riemann Bilinear Relations and Perversities
- A decreasing filtration , called the Hodge filtration, such that
- An increasing filtration , called the Weight filtration, such that
6.4. From Feynman Integrals to Intersection Theory
7. Computing Intersection Numbers: State of the Art and Open Problems
7.1. Univariate Case
- 1:
- A holomorphic function such that
- 2:
- A function such that
7.2. Logarithmic n-Forms
7.3. Multivariate Case: Recursive Method
7.4. Moving Onwards: An Open Problem
- 1:
- Non-degenerate case.If is non-degenerate (i.e., its Jacobian evaluated in 0 is ), then Equation (273) can be evaluated by the introduction of a change of variables . Using the usual Cauchy formula leads to
- 2:
- ideal generated from the .In this case the residue is 0. If, for example, , then is holomorphic in a bigger set, which we call .Then the contour , which is an element of , has a boundary : by the Stokes theorem, the residue of along is then 0.
8. An Explicit Example of Feynman Integral
8.1. Computation of
8.2. Computation of
8.3. Computation of
9. Final Comments
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Appendix A. Baikov Representation
- 1:
- Parallel component .In the numerator of Equation (A6), we perform the scalar product of along the space generated by the vectors that come next (starting from ): this allows us to find the projections of along such vectors. The denominator is the necessary normalization that allows us to get the correct dimension.
- 2:
- Perpendicular component .Introducing polar coordinates and separating the angular part from the radial part, we getwhere is . Notice that can be seen as the height of a parallelotope with base , so it can be computed as the whole volume divided by the volume of its base: hence, we write
Appendix B. An Introduction on Gröbner Bases
- 1:
- 2:
- G is a Gröbner basis for ;
- If G is a Gröbner basis for I, then are equal modulo .
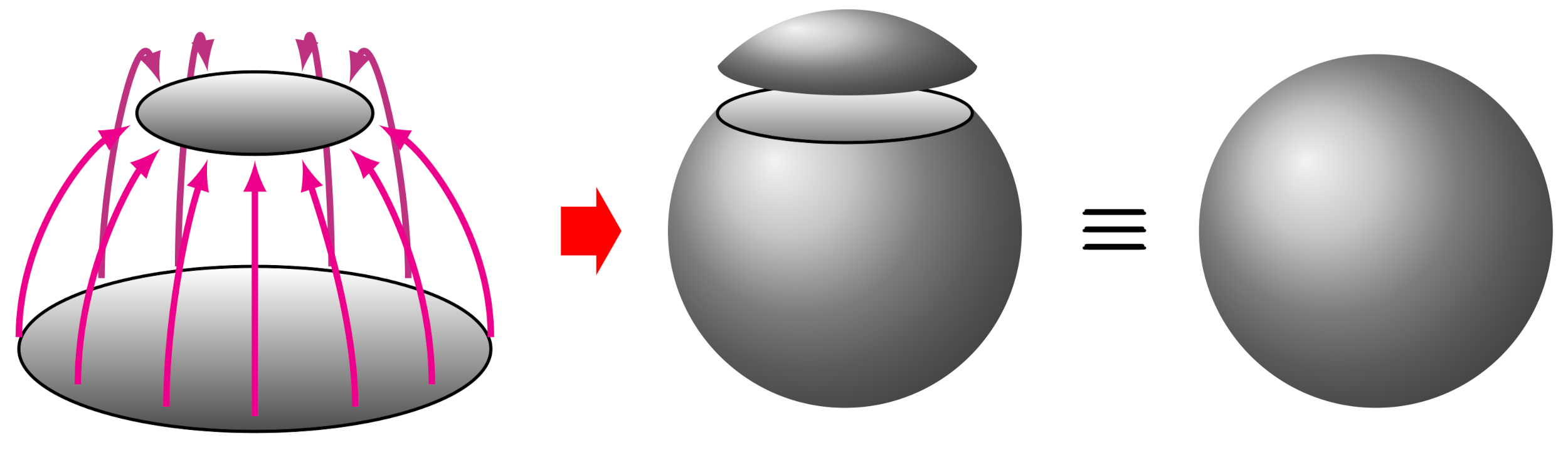
| 1 | Here one has to be careful: if we take a sphere with a hole, its boundary is not a boundary of the hole, but it is for its complement. In the above example this is not so. |
| 2 | If we cut before passing the saddle point, we get two cylinders, which correspond to trivial pieces. |
| 3 | runs along twice, so a further factor appears. |
| 4 | are non homogeneous coordinates on that can be related to homogeneous ones by , on the patch . |
| 5 | For example, assume , and take the transformation
Finally, fixing such that , we get the Weierstrass normal form. |
| 6 | Notice that this cannot be the general case, or at least not assuming b and d remain orthogonal to the equipotential lines. |
| 7 | In addition, they do not change deforming the path and continuously. |
| 8 | If we considered the invariants, the result at the point would be that the points meet with total coefficient . |
| 9 | Therefore, is defined by any given simplicial decomposition of N. |
| 10 | Indeed because of dimensional regularization. |
| 11 | Notice also that the massless condition and momentum conservation imply . |
| 12 | Notice that we are not using an orthonormal frame, so the inverse matrix appears. |
| 13 | Notice that we have changed the connection to . |
| 14 | once again taking into account of the non othonormality through . |
References
- Veltman, M.J.G. Diagrammatica: The Path to Feynman Rules; Cambridge University Press: Cambridge, UK, 2012; Volume 4. [Google Scholar]
- ’t Hooft, G.; Veltman, M.J.G. DIAGRAMMAR. NATO Sci. Ser. B 1974, 4, 177–322. [Google Scholar] [CrossRef]
- Bern, Z.; Dixon, L.J.; Dunbar, D.C.; Kosower, D.A. Fusing gauge theory tree amplitudes into loop amplitudes. Nucl. Phys. 1995, B435, 59–101. [Google Scholar] [CrossRef]
- Bern, Z.; Dixon, L.J.; Dunbar, D.C.; Kosower, D.A. One loop n point gauge theory amplitudes, unitarity and collinear limits. Nucl. Phys. 1994, B425, 217–260. [Google Scholar] [CrossRef]
- Britto, R.; Cachazo, F.; Feng, B. Generalized unitarity and one-loop amplitudes in N=4 super-Yang-Mills. Nucl. Phys. B 2005, 725, 275–305. [Google Scholar] [CrossRef]
- Britto, R.; Buchbinder, E.; Cachazo, F.; Feng, B. One-loop amplitudes of gluons in SQCD. Phys. Rev. D 2005, 72, 065012. [Google Scholar] [CrossRef]
- Cachazo, F.; He, S.; Yuan, E.Y. Scattering equations and Kawai-Lewellen-Tye orthogonality. Phys. Rev. 2014, D90, 065001. [Google Scholar] [CrossRef]
- Bern, Z.; Carrasco, J.J.; Chiodaroli, M.; Johansson, H.; Roiban, R. The Duality Between Color and Kinematics and its Applications. arXiv 2019, arXiv:1909.01358. [Google Scholar]
- Ossola, G.; Papadopoulos, C.G.; Pittau, R. Reducing full one-loop amplitudes to scalar integrals at the integrand level. Nucl. Phys. B 2007, 763, 147–169. [Google Scholar] [CrossRef]
- Ellis, R.; Giele, W.; Kunszt, Z. A Numerical Unitarity Formalism for Evaluating One-Loop Amplitudes. J. High Energy Phys. 2008, 03, 003. [Google Scholar] [CrossRef]
- Ellis, R.; Giele, W.T.; Kunszt, Z.; Melnikov, K. Masses, fermions and generalized D-dimensional unitarity. Nucl. Phys. B 2009, 822, 270–282. [Google Scholar] [CrossRef]
- Mastrolia, P.; Mirabella, E.; Peraro, T. Integrand reduction of one-loop scattering amplitudes through Laurent series expansion. J. High Energy Phys. 2012, 06, 095, Erratum in 2012, 11, 128. [Google Scholar] [CrossRef]
- Zhang, Y. Integrand-Level Reduction of Loop Amplitudes by Computational Algebraic Geometry Methods. J. High Energy Phys. 2012, 09, 042. [Google Scholar] [CrossRef]
- Mastrolia, P.; Mirabella, E.; Ossola, G.; Peraro, T. Scattering Amplitudes from Multivariate Polynomial Division. Phys. Lett. B 2012, 718, 173–177. [Google Scholar] [CrossRef]
- Mastrolia, P.; Ossola, G. On the Integrand-Reduction Method for Two-Loop Scattering Amplitudes. J. High Energy Phys. 2011, 11, 014. [Google Scholar] [CrossRef]
- Badger, S.; Frellesvig, H.; Zhang, Y. A Two-Loop Five-Gluon Helicity Amplitude in QCD. J. High Energy Phys. 2013, 12, 045. [Google Scholar] [CrossRef]
- Chetyrkin, K.G.; Tkachov, F.V. Integration by Parts: The Algorithm to Calculate beta Functions in 4 Loops. Nucl. Phys. 1981, B192, 159–204. [Google Scholar] [CrossRef]
- Laporta, S.; Remiddi, E. The Analytical value of the electron (g-2) at order alpha**3 in QED. Phys. Lett. 1996, B379, 283–291. [Google Scholar] [CrossRef]
- Barucchi, G.; Ponzano, G. Differential equations for one-loop generalized feynman integrals. J. Math. Phys. 1973, 14, 396–401. [Google Scholar] [CrossRef]
- Kotikov, A. Differential equations method. New technique for massive Feynman diagram calculation. Phys. Lett. B 1991, 254, 158–164. [Google Scholar] [CrossRef]
- Bern, Z.; Dixon, L.J.; Kosower, D.A. Dimensionally regulated pentagon integrals. Nucl. Phys. 1994, B412, 751–816. [Google Scholar] [CrossRef]
- Remiddi, E. Differential equations for Feynman graph amplitudes. Nuovo Cim. 1997, A110, 1435–1452. [Google Scholar]
- Gehrmann, T.; Remiddi, E. Differential equations for two loop four point functions. Nucl. Phys. 2000, B580, 485–518. [Google Scholar] [CrossRef]
- Henn, J.M. Multiloop integrals in dimensional regularization made simple. Phys. Rev. Lett. 2013, 110, 251601. [Google Scholar] [CrossRef]
- Argeri, M.; Di Vita, S.; Mastrolia, P.; Mirabella, E.; Schlenk, J.; Schubert, U.; Tancredi, L. Magnus and Dyson Series for Master Integrals. J. High Energy Phys. 2014, 03, 082. [Google Scholar] [CrossRef]
- Adams, L.; Chaubey, E.; Weinzierl, S. Simplifying Differential Equations for Multiscale Feynman Integrals beyond Multiple Polylogarithms. Phys. Rev. Lett. 2017, 118, 141602. [Google Scholar] [CrossRef]
- Laporta, S. High precision calculation of multiloop Feynman integrals by difference equations. Int. J. Mod. Phys. 2000, A15, 5087–5159. [Google Scholar] [CrossRef]
- Laporta, S. Calculation of Feynman integrals by difference equations. Acta Phys. Polon. 2003, B34, 5323–5334. [Google Scholar]
- Tarasov, O.V. Connection between Feynman integrals having different values of the space-time dimension. Phys. Rev. 1996, D54, 6479–6490. [Google Scholar] [CrossRef]
- Lee, R.N. Space-time dimensionality D as complex variable: Calculating loop integrals using dimensional recurrence relation and analytical properties with respect to D. Nucl. Phys. 2010, B830, 474–492. [Google Scholar] [CrossRef]
- Argeri, M.; Mastrolia, P. Feynman Diagrams and Differential Equations. Int. J. Mod. Phys. A 2007, 22, 4375–4436. [Google Scholar] [CrossRef]
- Henn, J.M. Lectures on differential equations for Feynman integrals. J. Phys. 2015, A48, 153001. [Google Scholar] [CrossRef]
- Kalmykov, M.; Bytev, V.; Kniehl, B.A.; Moch, S.O.; Ward, B.F.L.; Yost, S.A. Hypergeometric Functions and Feynman Diagrams. arXiv 2020, arXiv:2012.14492. [Google Scholar]
- Chen, K.T. Iterated path integrals. Bull. Am. Math. Soc. 1977, 83, 831–879. [Google Scholar] [CrossRef]
- Goncharov, A.B. Multiple polylogarithms, cyclotomy and modular complexes. Math. Res. Lett. 1998, 5, 497–516. [Google Scholar] [CrossRef]
- Remiddi, E.; Vermaseren, J.A.M. Harmonic polylogarithms. Int. J. Mod. Phys. 2000, A15, 725–754. [Google Scholar] [CrossRef]
- Broadhurst, D.J.; Kreimer, D. Association of multiple zeta values with positive knots via Feynman diagrams up to 9 loops. Phys. Lett. B 1997, 393, 403–412. [Google Scholar] [CrossRef]
- Broadhurst, D.J.; Kreimer, D. Towards cohomology of renormalization: Bigrading the combinatorial Hopf algebra of rooted trees. Commun. Math. Phys. 2000, 215, 217–236. [Google Scholar] [CrossRef][Green Version]
- Bloch, S.; Esnault, H.; Kreimer, D. On Motives associated to graph polynomials. Commun. Math. Phys. 2006, 267, 181–225. [Google Scholar] [CrossRef]
- Bogner, C.; Weinzierl, S. Periods and Feynman integrals. J. Math. Phys. 2009, 50, 042302. [Google Scholar] [CrossRef]
- Brown, F.C.S. On the periods of some Feynman integrals. arXiv 2009, arXiv:0910.0114. [Google Scholar]
- Marcolli, M. Feynman integrals and motives. arXiv 2009, arXiv:0907.0321. [Google Scholar]
- Arkani-Hamed, N.; Bourjaily, J.L.; Cachazo, F.; Goncharov, A.B.; Postnikov, A.; Trnka, J. Grassmannian Geometry of Scattering Amplitudes; Cambridge University Press: Cambridge, UK, 2016. [Google Scholar] [CrossRef]
- Arkani-Hamed, N.; Bai, Y.; Lam, T. Positive Geometries and Canonical Forms. J. High Energy Phys. 2017, 11, 039. [Google Scholar] [CrossRef]
- Mizera, S. Combinatorics and Topology of Kawai-Lewellen-Tye Relations. J. High Energy Phys. 2017, 08, 097. [Google Scholar] [CrossRef]
- Mizera, S. Scattering Amplitudes from Intersection Theory. Phys. Rev. Lett. 2018, 120, 141602. [Google Scholar] [CrossRef]
- Broedel, J.; Duhr, C.; Dulat, F.; Penante, B.; Tancredi, L. Elliptic Feynman integrals and pure functions. J. High Energy Phys. 2019, 01, 023. [Google Scholar] [CrossRef]
- Henn, J.; Mistlberger, B.; Smirnov, V.A.; Wasser, P. Constructing d-log integrands and computing master integrals for three-loop four-particle scattering. J. High Energy Phys. 2020, 04, 167. [Google Scholar] [CrossRef]
- Sturmfels, B.; Telen, S. Likelihood Equations and Scattering Amplitudes. arXiv 2020, arXiv:2012.05041. [Google Scholar]
- Hwa, R.; Teplitz, V. Homology and Feynman Integrals; Mathematical Physics Monograph Series; W. A. Benjamin: New York, NY, USA, 1966. [Google Scholar]
- Pham, F. Introduction to the topological study of landau singularities 1965. In Singularities of Integrals. Homology, Hyperfunctions and Microlocal Analysis; Report number: PRINT-65-1015; Springer: London, UK, 2011. [Google Scholar]
- Lefschetz, S. Applications of Algebraic Topology. Graphs and Networks. The Picard-Lefschetz Theory and Feynman Integrals; Springer: New York, NY, USA, 1975. [Google Scholar]
- Lee, R.N.; Pomeransky, A.A. Critical points and number of master integrals. J. High Energy Phys. 2013, 11, 165. [Google Scholar] [CrossRef]
- Broadhurst, D.; Mellit, A. Perturbative quantum field theory informs algebraic geometry. PoS 2016, LL2016, 079. [Google Scholar] [CrossRef]
- Broadhurst, D. Feynman integrals, L-series and Kloosterman moments. Commun. Num. Theor. Phys. 2016, 10, 527–569. [Google Scholar] [CrossRef]
- Broadhurst, D.; Roberts, D.P. Quadratic relations between Feynman integrals. PoS 2018, LL2018, 053. [Google Scholar] [CrossRef]
- Abreu, S.; Britto, R.; Duhr, C.; Gardi, E.; Matthew, J. From positive geometries to a coaction on hypergeometric functions. J. High Energy Phys. 2020, 02, 122. [Google Scholar] [CrossRef]
- Abreu, S.; Britto, R.; Duhr, C.; Gardi, E.; Matthew, J. Generalized hypergeometric functions and intersection theory for Feynman integrals. arXiv 2019, arXiv:1912.03205. [Google Scholar]
- Mastrolia, P.; Mizera, S. Feynman Integrals and Intersection Theory. J. High Energy Phys. 2019, 02, 139. [Google Scholar] [CrossRef]
- Mizera, S. Aspects of Scattering Amplitudes and Moduli Space Localization. arXiv 2019, arXiv:1906.02099. [Google Scholar]
- Frellesvig, H.; Gasparotto, F.; Laporta, S.; Mandal, M.K.; Mastrolia, P.; Mattiazzi, L.; Mizera, S. Decomposition of Feynman Integrals on the Maximal Cut by Intersection Numbers. J. High Energy Phys. 2019, 05, 153. [Google Scholar] [CrossRef]
- Mizera, S.; Pokraka, A. From Infinity to Four Dimensions: Higher Residue Pairings and Feynman Integrals. arXiv 2019, arXiv:1910.11852. [Google Scholar] [CrossRef]
- Frellesvig, H.; Gasparotto, F.; Mandal, M.K.; Mastrolia, P.; Mattiazzi, L.; Mizera, S. Vector Space of Feynman Integrals and Multivariate Intersection Numbers. Phys. Rev. Lett. 2019, 123, 201602. [Google Scholar] [CrossRef] [PubMed]
- Mizera, S. Status of Intersection Theory and Feynman Integrals. arXiv 2020, arXiv:2002.10476. [Google Scholar]
- Frellesvig, H.; Gasparotto, F.; Laporta, S.; Mandal, M.K.; Mastrolia, P.; Mattiazzi, L.; Mizera, S. Decomposition of Feynman Integrals by Multivariate Intersection Numbers. arXiv 2020, arXiv:2008.04823. [Google Scholar]
- Weinzierl, S. On the computation of intersection numbers for twisted cocycles. arXiv 2020, arXiv:2002.01930. [Google Scholar]
- Kaderli, A. A note on the Drinfeld associator for genus-zero superstring amplitudes in twisted de Rham theory. arXiv 2019, arXiv:1912.09406. [Google Scholar]
- Kalyanapuram, N.; Jha, R.G. Positive Geometries for all Scalar Theories from Twisted Intersection Theory. Phys. Rev. Res. 2020, 2, 033119. [Google Scholar] [CrossRef]
- Weinzierl, S. Correlation functions on the lattice and twisted cocycles. Phys. Lett. B 2020, 805, 135449. [Google Scholar] [CrossRef]
- Fresán, J.; Sabbah, C.; Yu, J.D. Quadratic relations between periods of connections. arXiv 2020, arXiv:2005.11525. [Google Scholar]
- Fresán, J.; Sabbah, C.; Yu, J.D. Quadratic relations between Bessel moments. arXiv 2020, arXiv:2006.02702. [Google Scholar]
- Chen, J.; Xu, X.; Yang, L.L. Constructing Canonical Feynman Integrals with Intersection Theory. arXiv 2020, arXiv:2008.03045. [Google Scholar]
- Britto, R.; Mizera, S.; Rodriguez, C.; Schlotterer, O. Coaction and double-copy properties of configuration-space integrals at genus zero. arXiv 2021, arXiv:2102.06206. [Google Scholar]
- Cho, K.; Matsumoto, K. Intersection theory for twisted cohomologies and twisted Riemann’s period relations I. Nagoya Math. J. 1995, 139, 67–86. [Google Scholar] [CrossRef]
- Matsumoto, K. Quadratic Identities for Hypergeometric Series of Type (k,l). Kyushu J. Math. 1994, 48, 335–345. [Google Scholar] [CrossRef]
- Matsumoto, K. Intersection numbers for logarithmic k-forms. Osaka J. Math. 1998, 35, 873–893. [Google Scholar]
- Ohara, K.; Sugiki, Y.; Takayama, N. Quadratic Relations for Generalized Hypergeometric Functions pFp−1. Funkc. Ekvacioj 2003, 46, 213–251. [Google Scholar] [CrossRef]
- Goto, Y. Twisted Cycles and Twisted Period Relations for Lauricella’s Hypergeometric Function FC. Int. J. Math. 2013, 24, 1350094. [Google Scholar] [CrossRef]
- Goto, Y.; Matsumoto, K. The monodromy representation and twisted period relations for Appell’s hypergeometric function F4. Nagoya Math. J. 2015, 217, 61–94. [Google Scholar] [CrossRef]
- Goto, Y. Twisted period relations for Lauricella’s hypergeometric functions FA. Osaka J. Math. 2015, 52, 861–879. [Google Scholar]
- Goto, Y. Intersection Numbers and Twisted Period Relations for the Generalized Hypergeometric Function m+1Fm. Kyushu J. Math. 2015, 69, 203–217. [Google Scholar] [CrossRef]
- Matsubara-Heo, S.J.; Takayama, N. An algorithm of computing cohomology intersection number of hypergeometric integrals. arXiv 2019, arXiv:1904.01253. [Google Scholar]
- Smirnov, A.V.; Petukhov, A.V. The Number of Master Integrals is Finite. Lett. Math. Phys. 2011, 97, 37–44. [Google Scholar] [CrossRef]
- Aluffi, P.; Marcolli, M. Feynman motives and deletion-contraction relations. arXiv 2009, arXiv:0907.3225. [Google Scholar]
- Aluffi, P. Generalized euler characteristics, graph hypersurfaces, and feynman periods. In Geometric, Algebraic and Topological Methods for Quantum Field Theory; World Scientific: Singapore, 2014; pp. 95–136. [Google Scholar] [CrossRef]
- Bitoun, T.; Bogner, C.; Klausen, R.P.; Panzer, E. Feynman integral relations from parametric annihilators. Lett. Math. Phys. 2019, 109, 497–564. [Google Scholar] [CrossRef]
- Bitoun, T.; Bogner, C.; Klausen, R.P.; Panzer, E. The number of master integrals as Euler characteristic. PoS 2018, LL2018, 065. [Google Scholar] [CrossRef]
- Zhou, Y. Wick rotations, Eichler integrals, and multi-loop Feynman diagrams. Commun. Num. Theor. Phys. 2018, 12, 127–192. [Google Scholar] [CrossRef]
- Zhou, Y. Wrońskian factorizations and Broadhurst–Mellit determinant formulae. Commun. Num. Theor. Phys. 2018, 12, 355–407. [Google Scholar] [CrossRef]
- Lee, R.N. Symmetric ϵ- and (ϵ+1/2)-forms and quadratic constraints in ”elliptic” sectors. J. High Energy Phys. 2018, 10, 176. [Google Scholar] [CrossRef]
- Baikov, P.A. Explicit solutions of the multiloop integral recurrence relations and its application. Nucl. Instrum. Meth. 1997, A389, 347–349. [Google Scholar] [CrossRef]
- Grozin, A.G. Integration by parts: An Introduction. Int. J. Mod. Phys. 2011, A26, 2807–2854. [Google Scholar] [CrossRef]
- Bogner, C.; Weinzierl, S. Feynman graph polynomials. Int. J. Mod. Phys. A 2010, 25, 2585–2618. [Google Scholar] [CrossRef]
- Mastrolia, P.; Peraro, T.; Primo, A. Adaptive Integrand Decomposition in parallel and orthogonal space. J. High Energy Phys. 2016, 08, 164. [Google Scholar] [CrossRef]
- Lee, R.N.; Smirnov, V.A. The Dimensional Recurrence and Analyticity Method for Multicomponent Master Integrals: Using Unitarity Cuts to Construct Homogeneous Solutions. J. High Energy Phys. 2012, 12, 104. [Google Scholar] [CrossRef]
- Harley, M.; Moriello, F.; Schabinger, R.M. Baikov-Lee Representations Of Cut Feynman Integrals. J. High Energy Phys. 2017, 06, 049. [Google Scholar] [CrossRef]
- Matsubara-Heo, S.J. Euler and Laplace integral representations of GKZ hypergeometric functions. arXiv 2019, arXiv:1904.00565. [Google Scholar]
- Goto, Y.; Matsubara-Heo, S.J. Homology and cohomology intersection numbers of GKZ systems. arXiv 2020, arXiv:2006.07848. [Google Scholar]
- Acres, K.; Broadhurst, D. Empirical determinations of Feynman integrals using integer relation algorithms. arXiv 2021, arXiv:2103.06345. [Google Scholar]
- Hilton, P.; Pedersen, J. Descartes, Euler, Poincaré, Pólya and Polyhedra. Séminaire de Philosophie et Mathématiques 1982, 8, 1–17. [Google Scholar]
- Milnor, J. Morse Theory; AM-51; Number v. 51 in Annals of Mathematics Studies; Princeton University Press: Princeton, NJ, USA, 2016. [Google Scholar]
- Dubrovin, B.A.; Fomenko, A.; Novikov, S. Modern Geometry–methods and Applications: Part 3: Introduction to Homology Theory; Springer: New York, NY, USA, 1984. [Google Scholar]
- Madsen, I.H.; Tornehaven, J. From Calculus to Cohomology: De Rham Cohomology and Characteristic Classes; Cambridge University Press: Cambridge, UK; New York, NY, USA, 1997. [Google Scholar]
- Lefschetz, S. L’analysis Situs et la GéOméTrie Algébrique, par S. Lefschetz.; Collection de Monographies sur la Théorie des Fonctions pub. sous la Direction de m. Émile Borel; Gauthier-Villars et cie: Paris, France, 1924. [Google Scholar]
- Hodge, W.V.D. The Theory and Applications of Harmonic Integrals; Cambridge University Press: Cambridge, UK; New York, NY, USA, 1989. [Google Scholar]
- Hodge, W.; Atiyah, M. Integrals of the Second Kind on an Algebraic Variety. Ann. Math. 1955, 62, 56. [Google Scholar] [CrossRef]
- Grothendieck, A. Standard conjectures on algebraic cycles. In Algebraic Geometry (Internat. Colloq., Tata Inst. Fund. Res., Bombay, 1968); Oxford University Press: London, UK, 1969; pp. 193–199. [Google Scholar]
- Whitney, H. Geometric Integration Theory; Princeton University Press: Princeton, NJ, USA, 1957. [Google Scholar]
- Hancock, H. Elliptic Integrals; Dover Publications Inc.: New York, NY, USA, 1958; p. 104. [Google Scholar]
- Shafarevich, I.R. Basic Algebraic Geometry, 3rd ed.; Springer: Berlin, Germany, 2013. [Google Scholar] [CrossRef]
- Chandrasekharan, K. Elliptic Functions; Springer: Berlin/Heidelberg, Germany, 1985. [Google Scholar]
- Pham, F. Vanishing homologies and the n variable saddlepoint method. In Singularities, Part 2 (Arcata, Calif., 1981); American Mathematical Society: Providence, RI, USA, 1983; Volume 40, pp. 319–333. [Google Scholar]
- Pham, F. La descente des cols par les onglets de Lefschetz, avec vues sur Gauss- Manin. Astérisque 1985, 130, 11–47. [Google Scholar]
- Bredon, G. Topology and Geometry; Springer: New York, NY, USA, 1993. [Google Scholar]
- de Rham, G. Sur l’analysis situs des variétés à n dimensions. J. MathéMatiques Pures AppliquéEs 1931, 10, 115–200. [Google Scholar]
- Weil, A. Sur les théorèmes de de Rham. Comment. Math. Helv. 1952, 26, 119–145. [Google Scholar] [CrossRef]
- Schwartz, J. De Rham’s Theorem for Arbitrary Spaces. Am. J. Math. 1955, 77, 29–44. [Google Scholar] [CrossRef]
- Samelson, H. On the De Rham’s Theorem. Topology 1967, 6, 427–432. [Google Scholar] [CrossRef]
- Christian-Oliver, E. A de Rham isomorphism in singular cohomology and Stokes theorem for stratifolds. Int. J. Geom. Methods Mod. Phys. 2004, 2, 63–81. [Google Scholar] [CrossRef]
- Hutchings, M. Cup Product and Intersections. 2011. Available online: https://math.berkeley.edu/~hutching/teach/215b-2011/cup.pdf (accessed on 26 December 2020).
- Maxim, L.G. Intersection Homology & Perverse Sheaves; Springer: Cham, Switzerland, 2019. [Google Scholar]
- de Cataldo, M.A.; Migliorini, L. The Decomposition Theorem, perverse sheaves and the topology of algebraic maps. Bull. Am. Math. Soc. 2009, 46, 535–633. [Google Scholar] [CrossRef]
- Deligne, P. Théorie de Hodge: I. Actes Congrès Int. Math. 1970, 1, 425–430. [Google Scholar]
- Deligne, P. Théorie de Hodge: II. Publications Mathématiques de l’IHÉS 1971, 40, 5–57. [Google Scholar] [CrossRef]
- Deligne, P. Théorie de Hodge: III. Publications Mathématiques de l’IHÉS 1974, 44, 5–77. [Google Scholar] [CrossRef]
- McCrory, C. Cone Complexes and PL Transversality. Trans. Am. Math. Soc. 1975, 207, 269–291. [Google Scholar] [CrossRef]
- Goresky, M.; MacPherson, R. Intersection Homology Theory. Topology 1980, 19, 135–162. [Google Scholar] [CrossRef]
- Goresky, M.; MacPherson, R. Intersection Homology Theory, II. Invent. Math. 1983, 72, 77–129. [Google Scholar] [CrossRef]
- Goresky, M.; MacPherson, R. La dualité de Poincaré pour les espaces singuliers. C. R. Acad. Sci. Paris 1977, 284, 1549–1551. [Google Scholar]
- Goresky, M.; MacPherson, R. Simplicial Intersection Homology. Inv. Math. 1986, 84, 432–433. [Google Scholar]
- Goresky, M.; MacPherson, R. Lefschetz fixed point theorem for intersection homology. Comment. Math. Helv. 1985, 60, 366–391. [Google Scholar] [CrossRef]
- Goresky, M.; MacPherson, R. On the topology of complex algebraic maps. In Proceedings of the Conference on Algebraic Geometry, La Rabida, Spain, 7–15 January 1981; Springer Lecture Notes in Mathematics. Volume 961, pp. 119–129. [Google Scholar]
- Goresky, M.; MacPherson, R. Stratified Morse Theory; Springer: Berlin, Germany; New York, NY, USA, 1988; Volume 14. [Google Scholar]
- Goresky, M.; MacPherson, R. Stratified Morse Theory. Proc. Symp. Pure Math. 1983, 40, 517–533. [Google Scholar]
- Goresky, M.; MacPherson, R. Morse theory and intersection homology theory, dans Analyse et topologie sur les espaces singuliers (II-III)-6-10 juillet 1981. Astérisque 1983, 58, 101–102. [Google Scholar]
- Williamson, G. An Illustrated Guide to Perverse Sheaves, Course Given in Pisa 19th–30th of January. 2015. Available online: https://www.maths.usyd.edu.au/u/geordie/perverse_course/lectures.pdf (accessed on 20 October 2020).
- Aomoto, K.; Kita, M. Theory of Hypergeometric Functions; Springer Monographs in Mathematics; Springer: Tokyo, Japan, 2011. [Google Scholar] [CrossRef]
- Moser, J. The Order of a Singularity in Fuchs’ Theory. Math. Z. 1959, 72, 379–398. [Google Scholar] [CrossRef]
- Lee, R.N. Reducing differential equations for multiloop master integrals. J. High Energy Phys. 2015, 4, 108. [Google Scholar] [CrossRef]
- Griffiths, P.; Harris, J. Principles of Algebraic Geometry; John Wiley & Sons: New York, NY, USA, 1994. [Google Scholar]
- Søgaard, M.; Zhang, Y. Multivariate Residues and Maximal Unitarity. J. High Energy Phys. 2013, 12, 008. [Google Scholar] [CrossRef][Green Version]
- Adams, W.; Loustaunau, P. An Introduction to Gröbner Bases (Graduate Studies in Mathematics); American Mathematical Society: Providence, RI, USA, 1994; Volume 3. [Google Scholar]
- Grayson, D.R.; Stillman, M.E. Macaulay2, a Software System for Research in Algebraic Geometry. Available online: https://faculty.math.illinois.edu/Macaulay2/ (accessed on 18 May 2021).
- Saito, K. The higher residue pairings K F (k) for a family of hypersurface singular points. In Proceedings of the Symposia in Pure Mathematics; American Mathematical Society: Providence, Rhode Island, 1983; Volume 40. [Google Scholar] [CrossRef]
- Buchberger, B. Ein Algorithmus zum Auffinden der Basiselemente des Restklassenringes nach einem Nulldimensionalen Polynomideal. Master’s Thesis, University of Innsbruck, Innsbruck, Austria, 1965. [Google Scholar]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cacciatori, S.L.; Conti, M.; Trevisan, S. Co-Homology of Differential Forms and Feynman Diagrams. Universe 2021, 7, 328. https://doi.org/10.3390/universe7090328
Cacciatori SL, Conti M, Trevisan S. Co-Homology of Differential Forms and Feynman Diagrams. Universe. 2021; 7(9):328. https://doi.org/10.3390/universe7090328
Chicago/Turabian StyleCacciatori, Sergio Luigi, Maria Conti, and Simone Trevisan. 2021. "Co-Homology of Differential Forms and Feynman Diagrams" Universe 7, no. 9: 328. https://doi.org/10.3390/universe7090328
APA StyleCacciatori, S. L., Conti, M., & Trevisan, S. (2021). Co-Homology of Differential Forms and Feynman Diagrams. Universe, 7(9), 328. https://doi.org/10.3390/universe7090328






