Sterile Neutrinos as Dark Matter: Alternative Production Mechanisms in the Early Universe
Abstract
- Content
- 1
- Introduction 2
- 2
- Cosmological Consequences of Decoupled Particles 2
- 2.1.
- Free Streaming Length…………………………………………………………6
- 2.2.
- Coarse Grained Phase Space Densities………………………………………7
- 3
- Production from Scalar Decay 8
- 3.1.
- Quantum Kinetic Equation for Production…………………………………9
- 3.2.
- The Cutoff in the Power Spectrum: Free Streaming Length………………12
- 4
- Production from Standard Model Processes 13
- 4.1.
- Sterile Neutrinos from Pion Decay…………………………………………15
- 4.2.
- Quantum Kinetic Equation for π → lνs ……………………………………17
- 4.3.
- Cosmological Consequences…………………………………………………22
- 4.3.1.
- Bounds from Dark Matter and Dwarf Spheroidal Galaxies………22
- 4.3.2.
- Equation of State and Free Streaming………………………………24
- 5
- Conclusions 25
- References
- 26
1. Introduction
2. Cosmological Consequences of Decoupled Particles
2.1. Free Streaming Length
2.2. Coarse Grained Phase Space Densities
3. Production from Scalar Decay
3.1. Quantum Kinetic Equation for Production
3.2. The Cutoff in the Power Spectrum: Free Streaming Length
4. Production from Standard Model Processes
4.1. Sterile Neutrinos from Pion Decay
4.2. Quantum Kinetic Equation for
Non-Thermal Sterile Neutrino Distribution Function
- The finite-temperature pion decay constant has been obtained in both non-linear sigma models [108,109,110,111] and Chiral perturbation theory [107,112,114,115,116] with the result given asThis result is required in the quantum kinetic equation since production begins near MeV and continues until the distribution function freezes out. We assume prior to that there are no pions and that hadronization happens instantaneously at MeV.
- The mass of the pion varies with temperature as described in detail in refs. [107,115,116]. The finite temperature corrections to the pion mass is calculated with electromagnetic corrections in chiral perturbation theory and its variation with temperature is shown in Figure 2 of [107]. In these references, it can be seen that between 50 and 150 MeV the pion mass only varies between 140 and 144 MeV. Since this change is so small, we neglect the temperature variation in the pion mass and simply use its average value: MeV (see fig in ref. [107]).
- We assume that the lepton asymmetry in the early universe is very small so that we may neglect the chemical potential in the distribution function of the pions and charged leptons. This asymmetry is required for the Shi–Fuller mechanism, but will not be present in these calculations. We will show a similar enhancement at low moment to SF, but the enhancement found here is with zero lepton asymmetry.
- With the assumption that there is no lepton asymmetry, the contribution to thermodynamic quantities from will be equal to that of . In which case, the degrees of freedom will be set at accounting for both equal particle and antiparticle contributions in the case of Dirac fermions and the two different sources () for Majorana fermions. The different helicities have already been accounted by summing over spins in the evaluation of the matrix elements of the previous section.
- We assume that there had been no production of sterile neutrinos prior to the hadronization period from any other mechanisms (such as scalar decays or DW). This allows us to set the initial distribution of the sterile neutrinos to zero in the kinetic equation, which implies that our results for the distribution function will be a lower bound for the distribution function. Any other prior sources could only enhance the population of sterile neutrinos. By neglecting the initial population, we can neglect the Pauli blocking factor of the ’s in the production term, and we can also neglect the loss term (see discussion below).
4.3. Cosmological Consequences
4.3.1. Bounds from Dark Matter and Dwarf Spheroidal Galaxies
4.3.2. Equation of State and Free Streaming
5. Conclusions
- We began with a description of the dynamical properties of dark matter particles described by frozen distribution functions, such as effective equation of state, phase space density and the free-streaming length, which determines the cutoff in the dark matter power spectrum.
- We considered a simple extension beyond the standard model in which sterile-like neutrinos couple to a Higgs-like scalar via a Yukawa coupling, obtaining the quantum kinetic equations for production, the distribution function at freeze out and from it the clustering properties of this (DM) candidate such as the abundance and free streaming length.
- Within the standard model, we have argued that in the basis of mass eigenstates, standard model processes that produce active neutrinos also produce sterile-like neutrinos; however, these are suppressed by the small mixing angles. We discussed several possible production channels, obtained the general form of the quantum kinetic equations to leading order in the mixing angles and analyzed the possibility of thermalization. This analysis leads us to conclude that the final distribution function of sterile-like neutrinos is a result of the various kinematically allowed production processes available during the cosmological history of the early universe.
- As specific example, we focused on the production of sterile-like mass eigenstates from the decay of pions after the QCD phase transition, including finite temperature effects. Shortly after the QCD transition at , s pions being the lightest degrees of freedom in QCD feature large populations and their main decay channels (for charged pions) produce neutrinos. In the basis of mass eigenstates, these processes produce sterile-like neutrinos. We obtained and solved the quantum kinetic equations for production and freeze-out, and from the solution we obtained the clustering properties of sterile-like neutrinos produced via this mechanism. We find that these could be a suitable warm dark matter candidate with the correct abundance if the mass is of the order of few and their free streaming length of a few consistent with the scale of cores in dwarf spheroidal galaxies.
Funding
Conflicts of Interest
References
- Dodelson, S. Modern Cosmology; Academic Press: New York, NY, USA, 2003. [Google Scholar]
- Lesgourgues, J.; Mangano, G.; Miel, G.; Pastor, S. Neutrino Cosmology; Cambridge University Press: Cambridge, MA, USA, 2013. [Google Scholar]
- Cushman, P. Snowmass Summary. arXiv 2013, arXiv:1310.8327. [Google Scholar]
- Kim, J. A review on axions and the strong CP problem. In AIP Conference Proceedings; American Institute of Physics: New York, NY, USA, 2010; Volume 1200, pp. 83–92. [Google Scholar]
- David, J.; Marsh, E. Axion cosmology. Phys. Rep. 2016, 643, 1–79. [Google Scholar]
- Boyarsky, A.; Iakubovskyi, D.; Ruchayskiy, O. Next decade of sterile neutrino studies. Phys. Dark Univ. 2012, 1, 136–154. [Google Scholar] [CrossRef]
- Boyarsky, A.; Ruchayskiy, O.; Shaposhnikov, M. The role of sterile neutrinos in cosmology and astrophysics. Ann. Rev. Nucl. Part. Sci. 2009, 59, 191–214. [Google Scholar] [CrossRef]
- de Blok, W.J.G. The core-cusp problem. Adv. Astron. 2010, 2010, 789293. [Google Scholar] [CrossRef]
- Dalcanton, J.; Hogan, C. Halo cores and phase-space densities: Observational constraints on dark matter physics and structure formation. Astrophys. J. 2001, 561, 35–45. [Google Scholar] [CrossRef]
- Hogan, C.; Dalcanton, J. New dark matter physics: Clues from halo structure. Phys. Rev. D 2000, 62, 063511. [Google Scholar] [CrossRef]
- Boylan-Kolchin, M.; Bullock, J.; Kaplinghat, M. Too big to fail? The puzzling darkness of massive Milky Way subhaloes. Mon. Not. R. Astron. Soc. 2011, 412, L40. [Google Scholar] [CrossRef]
- Garrison-Kimmel, S.; Boylan-Kolchin, M.; Bullock, J.; Kirby, E. Too big to fail in the local group. arXiv 2014, arXiv:1404.5313. [Google Scholar]
- Papastergis, E.; Giovanelli, R.; Haynes, M.; Shankar, F. Is there a “too big to fail” problem in the field? Astron. Astrophys. 2015, 574, A113. [Google Scholar] [CrossRef]
- Walker, M.G.; Penarrubia, J. A method for measuring (slopes of) the mass profiles of dwarf spheroidal galaxies. Astrophys. J. 2011, 20, 742. [Google Scholar] [CrossRef]
- Tikhonov, A.V.; Klypin, A. The emptiness of voids: Yet another overabundance problem for the cold dark matter model. Mon. Not. R. Astron. Soc. 2009, 395, 1915–1924. [Google Scholar] [CrossRef]
- Tikhonov, A.V.; Gottlöber, S.; Yepes, G.; Hoffman, Y. The sizes of minivoids in the local Universe: An argument in favour of a warm dark matter model? Mon. Not. R. Astron. Soc. 2009, 399, 1611–1621. [Google Scholar] [CrossRef]
- Moore, B.; Quinn, T.; Governato, F.; Stadel, J.; Lake, G. Cold collapse and the core catastrophe. Mon. Not. R. Astron. Soc. 1999, 310, 1147–1152. [Google Scholar] [CrossRef]
- Bode, P.; Ostriker, J.P.; Turok, N. Halo formation in warm dark matter models. Astrophys. J. 2001, 556, 93. [Google Scholar] [CrossRef]
- Avila-Reese, V.; Colín, P.; Valenzuela, O.; D’Onghia, E.; Firmani, C. Formation and structure of halos in a warm dark matter cosmology. Astrophys. J. 2001, 559, 516. [Google Scholar] [CrossRef]
- Lovell, M.R.; Frenk, C.S.; Eke, V.R.; Jenkins, A.; Gao, L.; Theuns, T. The properties of warm dark matter haloes. Mon. Not. R. Astron. Soc. 2014, 439, 300–317. [Google Scholar] [CrossRef]
- Dodelson, S.; Widrow, L.M. Sterile neutrinos as dark matter. Phys. Rev. Lett. 1994, 72, 17–20. [Google Scholar] [CrossRef]
- Simonetti, J.H.; Dennison, B.; Topasna, G.A. The Contribution Of Galactic Free-Free Emission to Anisotropies in the Cosmic Microwave Background Found by the Saskatoon Experiment. Astrophys. J. Lett. 1996, 458, L1. [Google Scholar] [CrossRef]
- Abazajian, K.; Fuller, G.M.; Patel, M. Sterile neutrino hot, warm, and cold dark matter. Phys. Rev. D 2001, 64, 023501. [Google Scholar] [CrossRef]
- Abazajian, K.N.; Acero, M.A.; Agarwalla, S.K.; Aguilar-Arevalo, A.A.; Albright, C.H.; Antusch, S.; Marfatia, D. Light sterile neutrinos: A white paper. arXiv 2012, arXiv:1204.5379. [Google Scholar]
- Chialva, D.; Dev, P.B.; Mazumdar, A. Multiple dark matter scenarios from ubiquitous stringy throats. Phys. Rev. D 2013, 87, 063522. [Google Scholar] [CrossRef]
- Paduroiu, S.; Revaz, Y.; Pfenniger, D. Structure formation in warm dark matter cosmologies: Top-Bottom Upside-Down. arXiv 2015, arXiv:1506.03789. [Google Scholar]
- Maccio, A.V.; Paduroiu, S.; Anderhalden, D.; Schneider, A.; Moore, B. Cores in warm dark matter haloes: A Catch 22 problem. Mon. Not. R. Astron. Soc. 2012, 424, 1105. [Google Scholar] [CrossRef]
- Shao, S.; Gao, L.; Theuns, T.; Frenk, C. The phase-space density of fermionic dark matter haloes. Mon. Not. R. Astron. Soc. 2013, 430, 2346. [Google Scholar] [CrossRef]
- Ade, P.A.; Aghanim, N.; Armitage-Caplan, C.; Arnaud, M.; Ashdown, M.; Atrio-Barandela, F.; Meinhold, P.R. Planck 2013 results. XVI. Cosmological parameters. Astron. Astrophys. 2014, 571, A16. [Google Scholar]
- Barbieri, R.; Dolgov, A.D. Neutrino oscillations in the early universe. Nucl. Phys. B 1991, 349, 743–753. [Google Scholar] [CrossRef]
- Barbieri, R.; Dolgov, A.D. Bounds on sterile neutrinos from nucleosynthesis. Phys. Lett. 1990, B237, 440–445. [Google Scholar] [CrossRef]
- Kainulainen, K. Light singlet neutrinos and the primordial nucleosynthesis. Phys. Lett. B 1990, 244, 191–195. [Google Scholar] [CrossRef]
- Enqvist, K.; Kainulainen, K.; Maalampi, J. Resonant neutrino transitions and nucleosynthesis. Phys. Lett. B 1990, 249, 531–534. [Google Scholar] [CrossRef]
- Enqvist, K.; Kainulainen, K.; Maalampi, J. Refraction and oscillations of neutrinos in the early universe. Nucl. Phys. B 1991, 349, 754–790. [Google Scholar] [CrossRef]
- Enqvist, K.; Kainulainen, K.; Thomson, M. Stringent cosmological bounds on inert neutrino mixing. Nucl. Phys. B 1992, 373, 498–528. [Google Scholar] [CrossRef]
- Dolgov, A.D. Neutrinos in cosmology. Phys. Rep. 2002, 370, 333. [Google Scholar] [CrossRef]
- Dolgov, A.D. Cosmology and neutrino properties. Phys. Atom. Nucl. 2008, 71, 2152. [Google Scholar] [CrossRef][Green Version]
- Dolgov, A.D. Cosmological implications of neutrinos. Surv. High Energy Phys. 2002, 17, 91. [Google Scholar] [CrossRef][Green Version]
- Shi, X.; Fuller, G.M. New dark matter candidate: Nonthermal sterile neutrinos. Phys. Rev. Lett. 1999, 82, 2832. [Google Scholar] [CrossRef]
- Asaka, T.; Laine, M.; Shaposhnikov, M. Lightest sterile neutrino abundance within the νMSM. J. High Energy Phys. 2007, 0701, 091. [Google Scholar] [CrossRef]
- Asaka, T.; Laine, M.; Shaposhnikov, M. On the hadronic contribution to sterile neutrino production. J. High Energy Phys. 2006, 0606, 053. [Google Scholar] [CrossRef]
- Asaka, T.; Shaposhnikov, M.; Kusenko, A. Opening a new window for warm dark matter. Phys. Lett. B 2006, 638, 401. [Google Scholar] [CrossRef]
- Asaka, T.; Shaposhnikov, M. The νMSM, dark matter and baryon asymmetry of the universe. Phys. Lett. B 2005, 620, 17. [Google Scholar] [CrossRef]
- Ghisoiu, I.; Laine, M. Right-handed neutrino production rate at T > 160 GeV. J. Cosmol. Astron. Phys. 2014, 1412, 032. [Google Scholar] [CrossRef]
- Laine, M.; Shaposhnikov, M. Sterile neutrino dark matter as a consequence of νMSM-induced lepton asymmetry. J. Cosmol. Astron. Phys. 2008, 0806, 031. [Google Scholar] [CrossRef]
- Venumadhav, T.; Cyr-Racine, F.Y.; Abazajian, K.N.; Hirata, C.M. Sterile neutrino dark matter: A tale of weak interactions in the strong coupling epoch. arXiv 2015, arXiv:1507.06655. [Google Scholar] [CrossRef]
- Boyanovsky, D. Clustering properties of a sterile neutrino dark matter candidate. Phys. Rev. D 2008, 78, 103505. [Google Scholar] [CrossRef]
- Petraki, K.; Kusenko, A. Dark-matter sterile neutrinos in models with a gauge singlet in the Higgs sector. Phys. Rev. D 2008, 77, 065014. [Google Scholar] [CrossRef]
- Petraki, K. Small-scale structure formation properties of chilled sterile neutrinos as dark matter. Phys. Rev. D 2008, 77, 105004. [Google Scholar] [CrossRef]
- Merle, A. keV neutrino model building. Int. J. Mod. Phys. D 2013, 22, 1330020. [Google Scholar] [CrossRef]
- Merle, A.; Schneider, A. Production of sterile neutrino dark matter and the 3.5 keV line. arXiv 2015, arXiv:1409.6311. [Google Scholar] [CrossRef][Green Version]
- Merle, A.; Totzauer, M. keV sterile neutrino dark matter from singlet scalar decays: Basic concepts and subtle features. J. Cosmol. Astron. Phys. 2015, 6, 011. [Google Scholar] [CrossRef][Green Version]
- Merle, A.; Niro, V.; Schmidt, D. New production mechanism for keV sterile neutrino dark matter by decays of frozen-in scalars. J. Cosmol. Astron. Phys. 2014, 1403, 028. [Google Scholar] [CrossRef]
- Watson, C.R.; Li, Z.; Polley, N.K. Constraining sterile neutrino warm dark matter with Chandra observations of the Andromeda galaxy. J. Cosmol. Astron. Phys. 2012, 3, 018. [Google Scholar] [CrossRef]
- Yuksel, H.; Beacom, J.F.; Watson, C.R. Strong upper limits on sterile neutrino warm dark matter. Phys. Rev. Lett. 2008, 101, 121301. [Google Scholar] [CrossRef]
- Bulbul, E.; Markevitch, M.; Foster, A.; Smith, R.; Loewenstein, M.; Randall, S. Detection of an unidentified emission line in the stacked X-ray spectrum of galaxy clusters. Astrophys. J. 2014, 13, 789. [Google Scholar] [CrossRef]
- Boyarsky, A.; Ruchayskiy, O.; Iakubovskyi, D.; Franse, J. Unidentified line in X-ray spectra of the Andromeda galaxy and Perseus galaxy cluster. arXiv 2014, arXiv:1402.4119. [Google Scholar] [CrossRef]
- Boyarsky, A.; Franse, J.; Iakubovskyi, D.; Rucharyskiy, O. Checking the dark matter origin of a 3.53 keV line with the Milky Way center. Phys. Rev. Lett. 2015, 115, 161301. [Google Scholar] [CrossRef] [PubMed]
- Jeltema, T.; Profumo, S. Discovery of a 3.5 keV line in the Galactic Centre and a critical look at the origin of the line across astronomical targets. Mon. Not. R. Astron. Soc. 2015, 450, 2143. [Google Scholar] [CrossRef]
- Jeltema, T.; Profumo, S. Reply to Two Comments on “Dark matter searches going bananas the contribution of Potassium (and Chlorine) to the 3.5 keV line”. arXiv 2014, arXiv:1411.1759. [Google Scholar]
- Malyshev, D.; Neronov, A.; Eckert, D. Constraints on 3.55 keV line emission from stacked observations of dwarf spheroidal galaxies. Phys. Rev. D 2014, 90, 103506. [Google Scholar] [CrossRef]
- Abazajian, K. Resonantly produced 7 keV sterile neutrino dark matter models and the properties of Milky Way satellites. Phys. Rev. Lett. 2014, 112, 161303. [Google Scholar] [CrossRef] [PubMed]
- Abazajian, K.N.; Fuller, G.M. Bulk QCD thermodynamics and sterile neutrino dark matter. Phys. Rev. D 2002, 66, 023526. [Google Scholar] [CrossRef]
- Horiuchi, S.; Humphrey, P.J.; Onorbe, J.; Abazajian, K.N.; Kaplinghat, M.; Garrison-Kimmel, S. Sterile neutrino dark matter bounds from galaxies of the Local Group. Phys. Rev. D 2014, 89, 025017. [Google Scholar] [CrossRef]
- Kaplinghat, M.; Lopez, R.E.; Dodelson, S.; Scherrer, R.J. Improved treatment of cosmic microwave background fluctuations induced by a late-decaying massive neutrino. Phys. Rev. D 1999, 60, 123508. [Google Scholar] [CrossRef]
- Lello, L.; Boyanovsky, D. Cosmological implications of light sterile neutrinos produced after the QCD phase transition. Phys. Rev. D 2015, 91, 063502. [Google Scholar] [CrossRef]
- Lello, L.; Boyanovsky, D. The case for mixed dark matter from sterile neutrinos. J. Cosmol. Astron. Phys. 2016, 6, 011. [Google Scholar] [CrossRef][Green Version]
- Ng, K.C.; Horiuchi, S.; Gaskins, J.M.; Smith, M.; Preece, R. Improved limits on sterile neutrino dark matter using full-sky Fermi Gamma-ray Burst Monitor data. Phys. Rev. D 2015, 92, 043503. [Google Scholar] [CrossRef]
- De Vega, H.J.; Salucci, P.; Sanchez, N.G. The mass of the dark matter particle: Theory and galaxy observations. New Astron. 2012, 17, 653. [Google Scholar] [CrossRef]
- De Vega, H.J.; Sanchez, N.G. Model-independent analysis of dark matter points to a particle mass at the keV scale. Mon. Not. R. Astron. Soc. 2010, 404, 885. [Google Scholar] [CrossRef]
- Merle, A.; Schneider, A.; Totzauer, M. Dodelson-Widrow production of sterile neutrino dark matter with non-trivial initial abundance. J. Cosmol. Astron. Phys. 2016, 4, 003. [Google Scholar] [CrossRef]
- Bernstein, J. Kinetic Theory in the Expanding Universe; Cambridge University Press: Cambridge, MA, USA, 1988. [Google Scholar]
- Kolb, E.W.; Turner, M.S. The Early Universe; Addison-Wesley: Boston, MA, USA, 1990. [Google Scholar]
- Boyanovsky, D.; De Vega, H.J.; Sanchez, N.G. Constraints on dark matter particles from theory, galaxy observations, and N-body simulations. Phys. Rev. D 2008, 77, 043518. [Google Scholar] [CrossRef]
- Boyanovsky, D.; De Vega, H.J.; Sanchez, N.G. Dark matter transfer function: Free streaming, particle statistics, and memory of gravitational clustering. Phys. Rev. D 2008, 78, 063546. [Google Scholar] [CrossRef]
- Destri, C.; De Vega, H.J.; Sanchez, N.G. Warm dark matter primordial spectra and the onset of structure formation at redshift z. Phys. Rev. D 2013, 88, 083512. [Google Scholar] [CrossRef]
- De Vega, H.J.; Sanchez, N.G. Cosmological evolution of warm dark matter fluctuations. I. Efficient computational framework with Volterra integral equations. Phys. Rev. D 2012, 85, 043516. [Google Scholar] [CrossRef]
- De Vega, H.J.; Sanchez, N.G. Cosmological evolution of warm dark matter fluctuations. II. Solution from small to large scales and keV sterile neutrinos. Phys. Rev. D 2012, 85, 043517. [Google Scholar] [CrossRef]
- Boyanovsky, D.; Wu, J. Small scale aspects of warm dark matter: Power spectra and acoustic oscillations. Phys. Rev. D 2011, 83, 043524. [Google Scholar] [CrossRef]
- Lee, B.W.; Weinberg, S. Cosmological lower bound on heavy-neutrino masses. Phys. Rev. Lett. 1977, 39, 165. [Google Scholar] [CrossRef]
- Hut, P. Limits on masses and number of neutral weakly interacting particles. Phys. Lett. 1977, B69, 85. [Google Scholar] [CrossRef]
- Sato, K.; Kobayashi, H. Cosmological constraints on the mass and the number of heavy lepton neutrinos. Prog. Theor. Phys. 1977, 58, 1775. [Google Scholar] [CrossRef]
- Vysotsky, M.I.; Dolgov, A.D.; Zeldovich, Y.B. Cosmological restriction on neutral lepton masses. JETP Lett. 1977, 26, 188. [Google Scholar]
- Cowsik, R.; McClelland, J. An upper limit on the neutrino rest mass. Phys. Rev. Lett. 1972, 29, 669. [Google Scholar] [CrossRef]
- Tremaine, S.; Gunn, J.E. Dynamical role of light neutral leptons in cosmology. Phys. Rev. Lett. 1979, 42, 407. [Google Scholar] [CrossRef]
- Kusenko, A. Sterile neutrinos, dark matter, and pulsar velocities in models with a Higgs singlet. Phys. Rev. Lett. 2006, 97, 241301. [Google Scholar] [CrossRef] [PubMed]
- Shaposhnikov, M.; Tkachev, I. The vMSM, inflation, and dark matter. Phys. Lett. 2006, B639, 414. [Google Scholar] [CrossRef]
- Notzold, D.; Raffelt, G. Neutrono dispersion at finite temperature and density. Nucl. Phys. B 1988, 307, 924. [Google Scholar] [CrossRef]
- Pal, P.B.; Pham, T.N. Field-theoretic derivation of Wolfenstein’s matter-oscillation formula. Phys. Rev. D 1989, 40, 259. [Google Scholar] [CrossRef]
- Wu, J.; Ho, C.M.; Boyanovsky, D. Sterile neutrinos produced near the electroweak scale: Mixing angles, MSW resonances, and production rates. Phys. Rev. D 2009, 80, 103511. [Google Scholar] [CrossRef]
- Weldon, H.A. Covariant calculations at finite temperature: The relativistic plasma. Phys. Rev. D 1982, 26, 1394. [Google Scholar] [CrossRef]
- Braaten, E.; Pisarski, R. Soft amplitudes in hot gauge theories: A general analysis. Nucl. Phys. B 1990, 337, 569. [Google Scholar] [CrossRef]
- Braaten, E.; Pisarski, R. Deducing hard thermal loops from Ward identities. Nucl. Phys. B 1990, 339, 310. [Google Scholar] [CrossRef]
- Braaten, E.; Pisarski, R. Resummation and gauge invariance of the gluon damping rate in hot QCD. Phys. Rev. Lett. 1990, 64, 1338. [Google Scholar] [CrossRef] [PubMed]
- Braaten, E.; Pisarski, R. Calculation of the gluon damping rate in hot QCD. Phys. Rev. D 1990, 42, 2156. [Google Scholar] [CrossRef]
- Braaten, E.; Pisarski, R. Simple effective Lagrangian for hard thermal loops. Phys. Rev. D 1992, 45, 1827. [Google Scholar] [CrossRef]
- Braaten, E.; Pisarski, R. Calculation of the quark damping rate in hot QCD. Phys. Rev. D 1992, 46, 1829. [Google Scholar] [CrossRef] [PubMed]
- Pisarski, R. Effective Lagrangian at high temperature. Nucl. Phys. A 1992, 554, 527C. [Google Scholar] [CrossRef]
- Pisarski, R. Medley in finite temperature field theory. Can. J. Phys. 1993, 71, 280. [Google Scholar] [CrossRef]
- Pisarski, R. Scattering Amplitudes in Hot Gauge Theories. Phys. Rev. Lett. 1989, 63, 1129. [Google Scholar] [CrossRef] [PubMed]
- Pisarski, R. How to Compute Scattering Amplitudes in Hot Gauge Theories. Physica A 1989, 158, 246. [Google Scholar] [CrossRef]
- Frenkel, J.; Taylor, J.C. High-temperature limit of thermal QCD. Nucl. Phys. B 1990, 334, 199. [Google Scholar] [CrossRef]
- Le Bellac, M. Thermal Field Theory; Cambridge Monographs on Mathematical Physics; Cambridge University Press: Cambridge, MA, USA, 1996. [Google Scholar]
- Lello, L.; Boyanovsky, D.; Pisarski, R.D. Production of heavy sterile neutrinos from vector boson decay at electroweak temperatures. Phys. Rev. D 2017, 95, 043524. [Google Scholar] [CrossRef]
- Boyanovsky, D.; De Vega, H.J.; Wang, S.Y. Nonequilibrium pion dynamics near the critical point in a constituent quark model. Nucl. Phys. A 2004, 741, 323. [Google Scholar] [CrossRef][Green Version]
- Rajagopal, K.; Wilczek, F. Emergence of coherent long wavelength oscillations after a quench: Application to QCD. Nucl. Phys. 1993, B404, 577. [Google Scholar] [CrossRef]
- Nicola, A.G.; Andrés, R.T. Electromagnetic effects in the pion dispersion relation at finite temperature. Phys. Rev. 2014, D89, 116009. [Google Scholar]
- Pisarski, R.D.; Tytgat, M.H.G. pi0–> gamma gamma and the axial anomaly at non zero temperature. arXiv 1997, arXiv:hepph/9705316. [Google Scholar]
- Pisarski, R.D.; Tytgat, M.H.G. Scattering of soft, cool pions. Phys. Rev. Lett. 1997, 78, 3622. [Google Scholar] [CrossRef]
- Pisarski, R.D.; Tytgat, M.H.G. Propagation of cool pions. Phys. Rev. D 1996, 54, 2989–2993. [Google Scholar] [CrossRef]
- Pisarski, R.D.; Trueman, T.L.; Tytgat, M.H.G. How π0→γγ changes with temperature. Phys. Rev. D 1997, 56, 7077. [Google Scholar] [CrossRef]
- Jeon, S.; Kapusta, J. Pion decay constant at finite temperature in the nonlinear model. Phys. Rev. D 1996, 54, 6475–6478. [Google Scholar] [CrossRef] [PubMed]
- Dominguez, C.A.; Fetea, M.S.; Loewe, M. Pions at finite temperature from QCD sum rules. Phys. Lett. B 1996, 387, 151. [Google Scholar] [CrossRef]
- Harada, M.; Shibata, A. Pion decay constant and the meson mass at finite temperature in hidden local symmetry. Phys. Rev. D 1997, 55, 6716. [Google Scholar] [CrossRef]
- Nicola, A.G.; Fernandez-Fraile, D. Chiral symmetry and mesons in hot and dense matter: Recent developments. arXiv 2010, arXiv:1011.3920. [Google Scholar]
- Toublan, D. Pion dynamics at finite temperature. Phys. Rev. D 1997, 56, 5629. [Google Scholar] [CrossRef]
- Bhattacharya, T. The QCD phase transition with physical-mass, chiral quarks. Phys. Rev. Lett. 2014, 113, 082001. [Google Scholar] [CrossRef] [PubMed]
- Bazavov, A. Equation of state in (2+1)-flavor QCD. Phys. Rev. D 2014, 90, 094503. [Google Scholar] [CrossRef]
- Bazavov, A. Chiral and deconfinement aspects of the QCD transition. Phys. Rev. D 2012, 85, 054503. [Google Scholar] [CrossRef]
- Bazavov, A. Equation of state and QCD transition at finite temperature. Phys. Rev. D 2009, 80, 014504. [Google Scholar] [CrossRef]
- Lello, L.; Boyanovsky, D. Searching for sterile neutrinos from and K decays. Phys. Rev. D 2013, 87, 073017. [Google Scholar] [CrossRef]
- Aviles, A.; Banerjee, A. A Lagrangian Perturbation Theory in the presence of massive neutrinos. J. Cosmol. Astron. Phys. 2020, 10, 034. [Google Scholar] [CrossRef]
- Lesgourgues, J.; Pastor, S. Massive neutrinos and cosmology. Phys. Rep. 2006, 429, 307–379. [Google Scholar] [CrossRef]
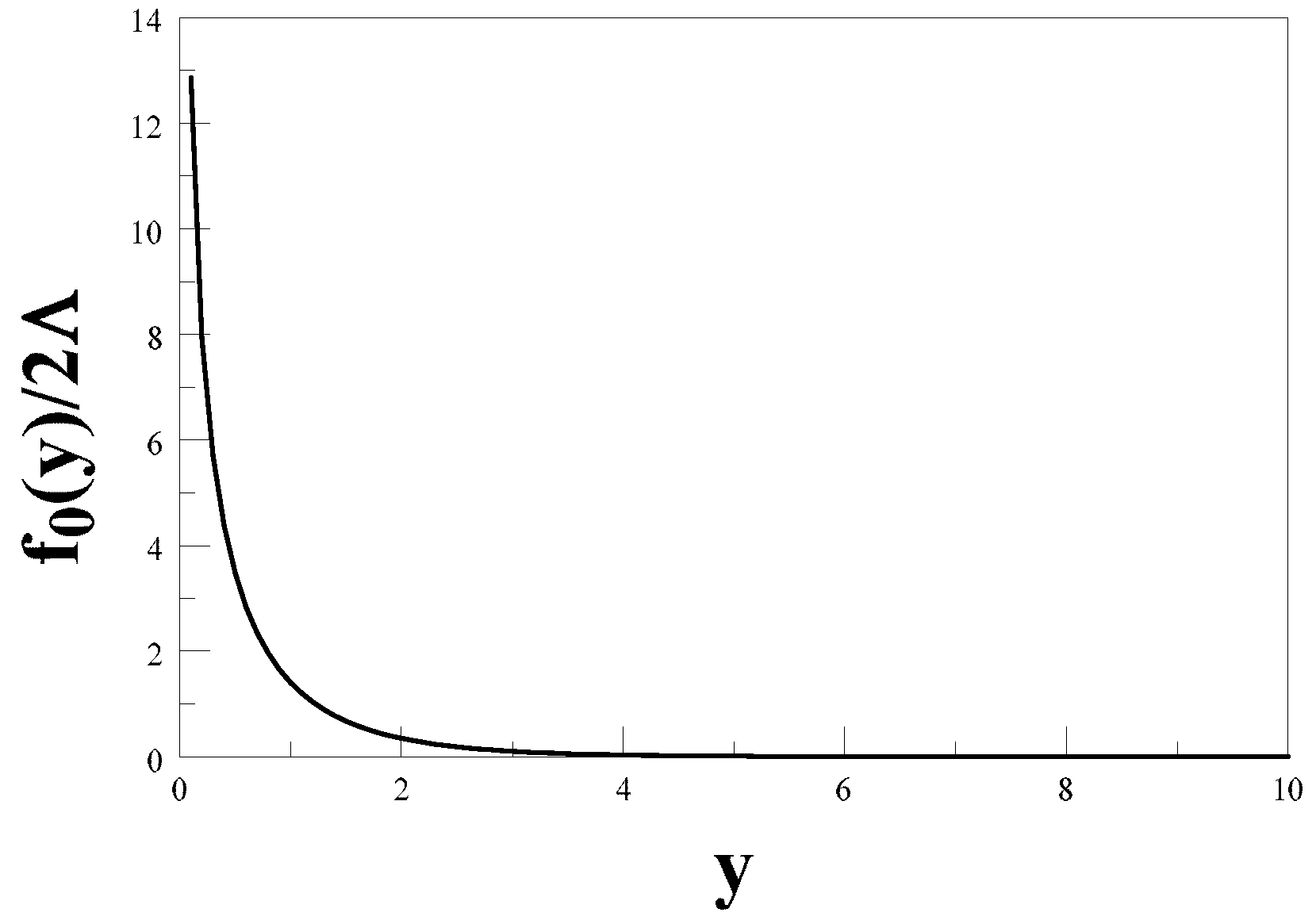
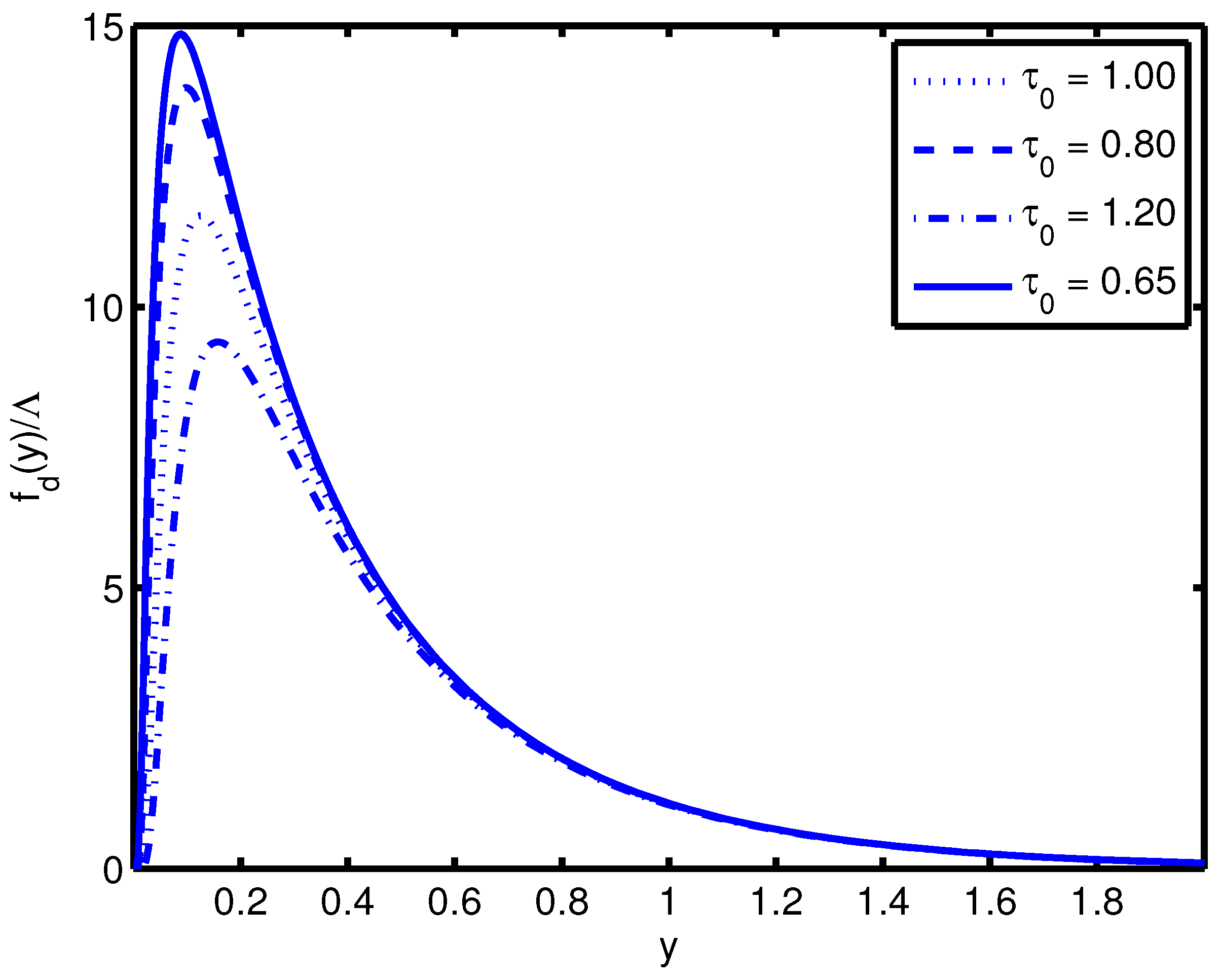

| n | 0 | 1 | 2 |
| 3.756 | 9.675 | 34.300 | |
| 1.830 | 2.140 | 3.426 | |
| Galaxy | |||||
|---|---|---|---|---|---|
| Willman 1 | 19 | 4 | 0.723 | 1.178 | 1.782 |
| Segue 1 | 48 | 4 | 1.69 | 1.456 | 2.204 |
| Coma-Berenices | 123 | 4.6 | 0.04 | 0.571 | 0.864 |
| Leo T | 170 | 7.8 | 0.014 | 0.4392 | 0.665 |
| Canis Venatici II | 245 | 4.6 | 0.04 | 0.571 | 0.864 |
| Draco | 305 | 10.1 | 0.0036 | 0.3128 | 0.473 |
| Fornax | 1730 | 10.7 | 2.56 × | 0.1615 | 0.2445 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boyanovsky, D. Sterile Neutrinos as Dark Matter: Alternative Production Mechanisms in the Early Universe. Universe 2021, 7, 264. https://doi.org/10.3390/universe7080264
Boyanovsky D. Sterile Neutrinos as Dark Matter: Alternative Production Mechanisms in the Early Universe. Universe. 2021; 7(8):264. https://doi.org/10.3390/universe7080264
Chicago/Turabian StyleBoyanovsky, Daniel. 2021. "Sterile Neutrinos as Dark Matter: Alternative Production Mechanisms in the Early Universe" Universe 7, no. 8: 264. https://doi.org/10.3390/universe7080264
APA StyleBoyanovsky, D. (2021). Sterile Neutrinos as Dark Matter: Alternative Production Mechanisms in the Early Universe. Universe, 7(8), 264. https://doi.org/10.3390/universe7080264





