Variable Density Flows in Rotating Astrophysical Plasma. Linear Waves and Resonant Phenomena
Abstract
1. Introduction
1.1. Stratified MHD Flows in Two-Layer Shallow Water Approximation
1.2. Stratified MHD Flows in Boussinesq Approximation
1.3. Compressible MHD Flows with Stable Stratification in Anelastic Approximation
1.4. MHD Flows in Shallow Water Approximation with Large-Scale Compressibility
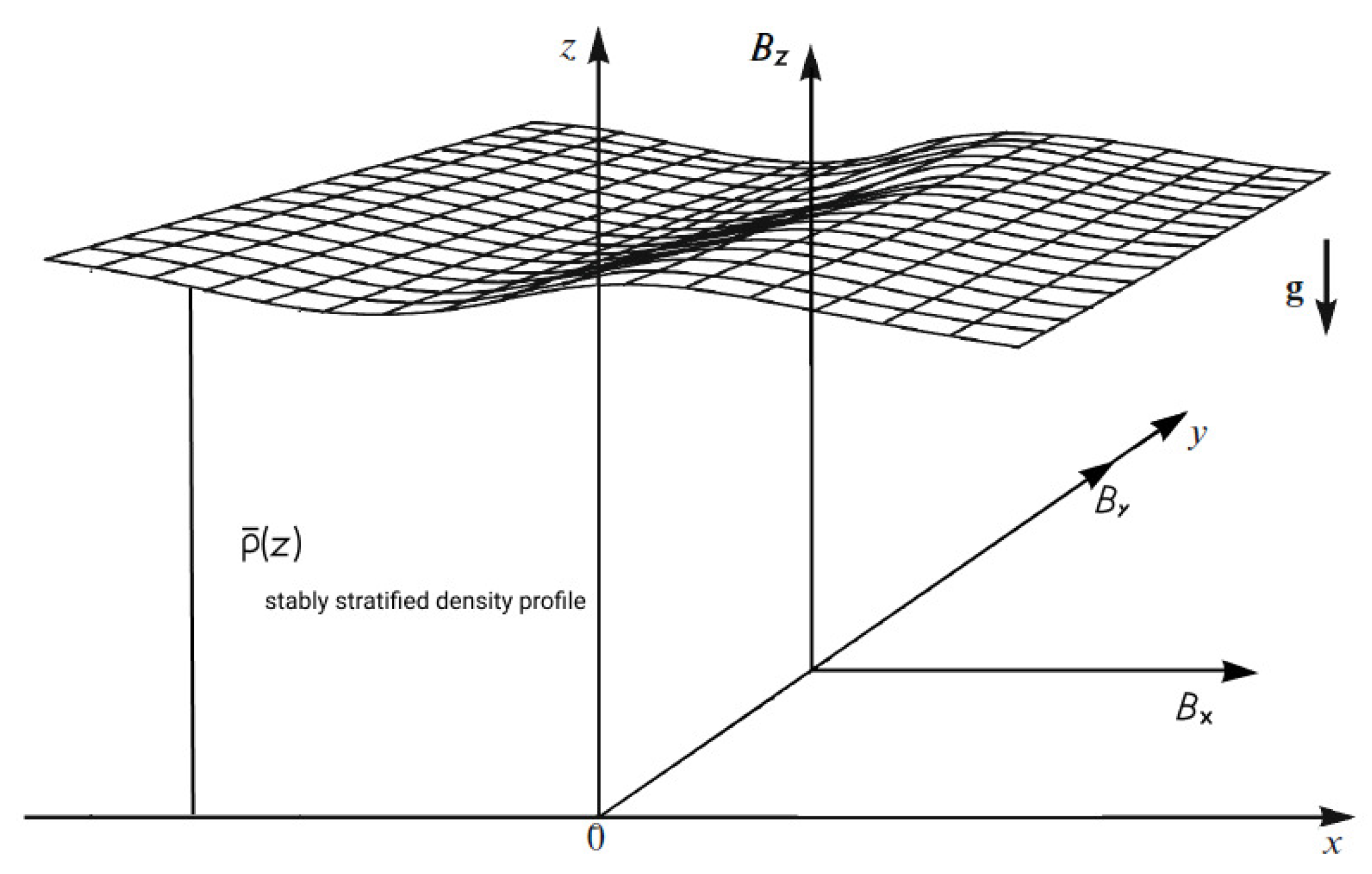
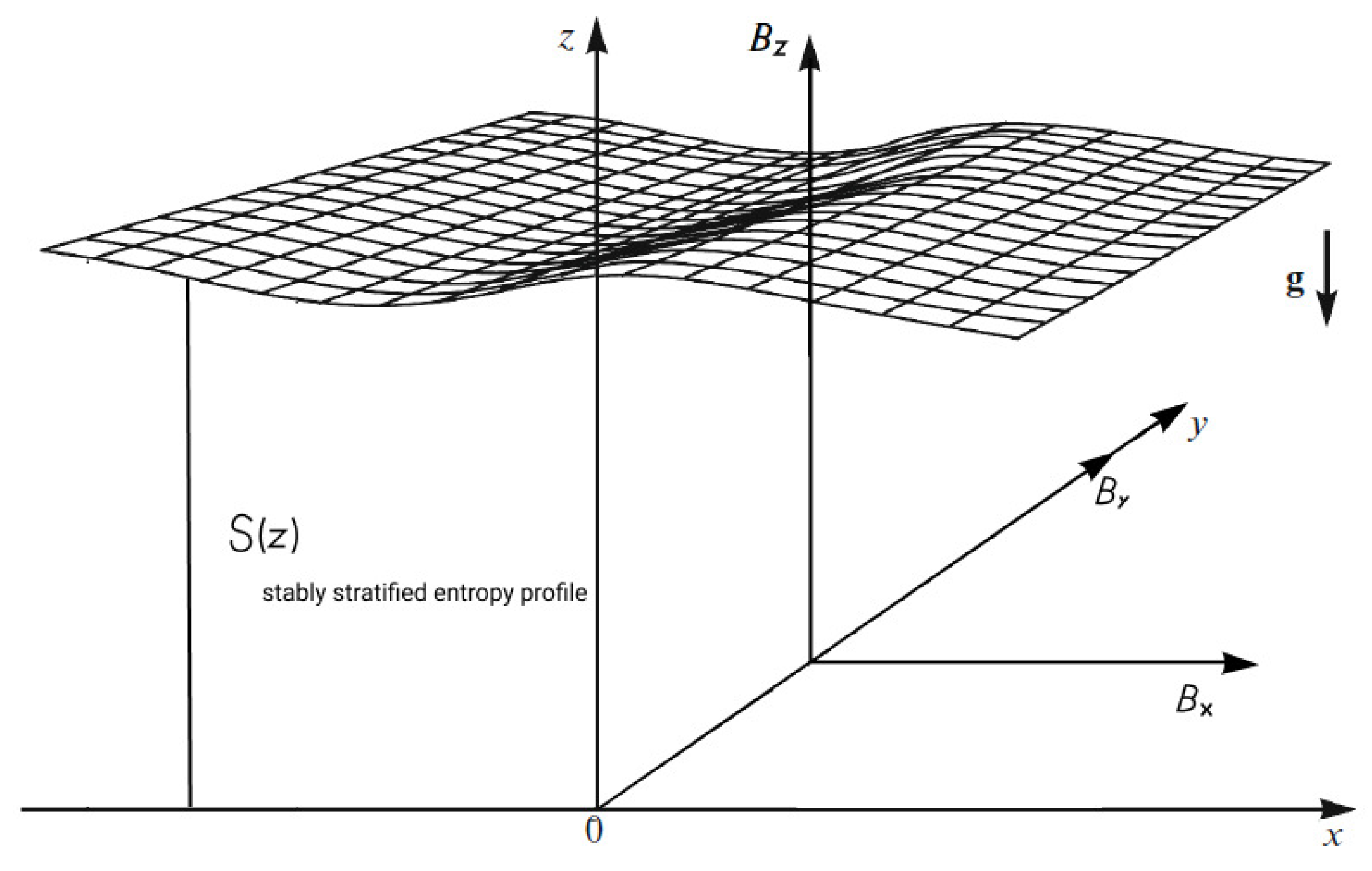
2. Stratified Flows of Rotating Astrophysical Plasma in Two-Layer Magnetohydrodynamic Shallow Water Approximation
2.1. Linear Magneto-Rossby Waves in a Stratified Astrophysical Plasma in Two-Layer Approximation in Presence of an External Vertical Magnetic Field
2.2. Linear Magneto-Rossby Waves in a Stratified Astrophysical Plasma in Two-Layer Approximation in Presence of a Horizontal Magnetic Field
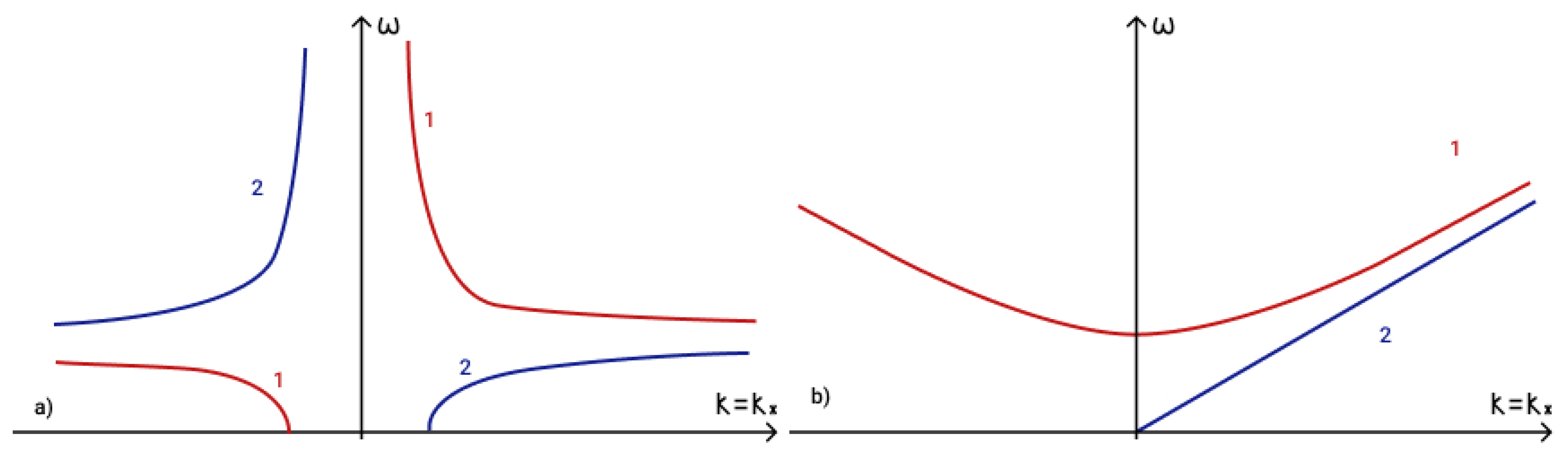
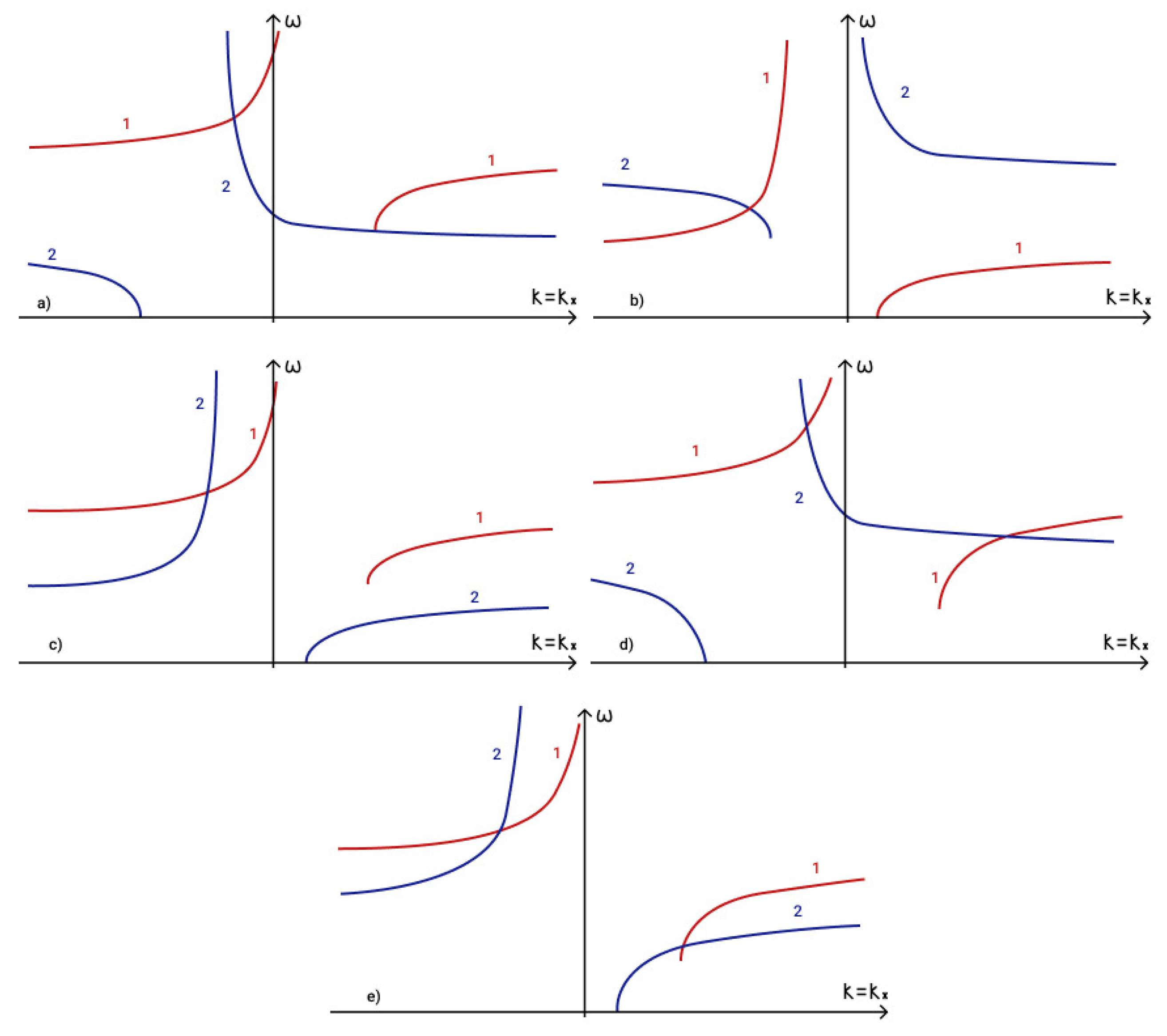
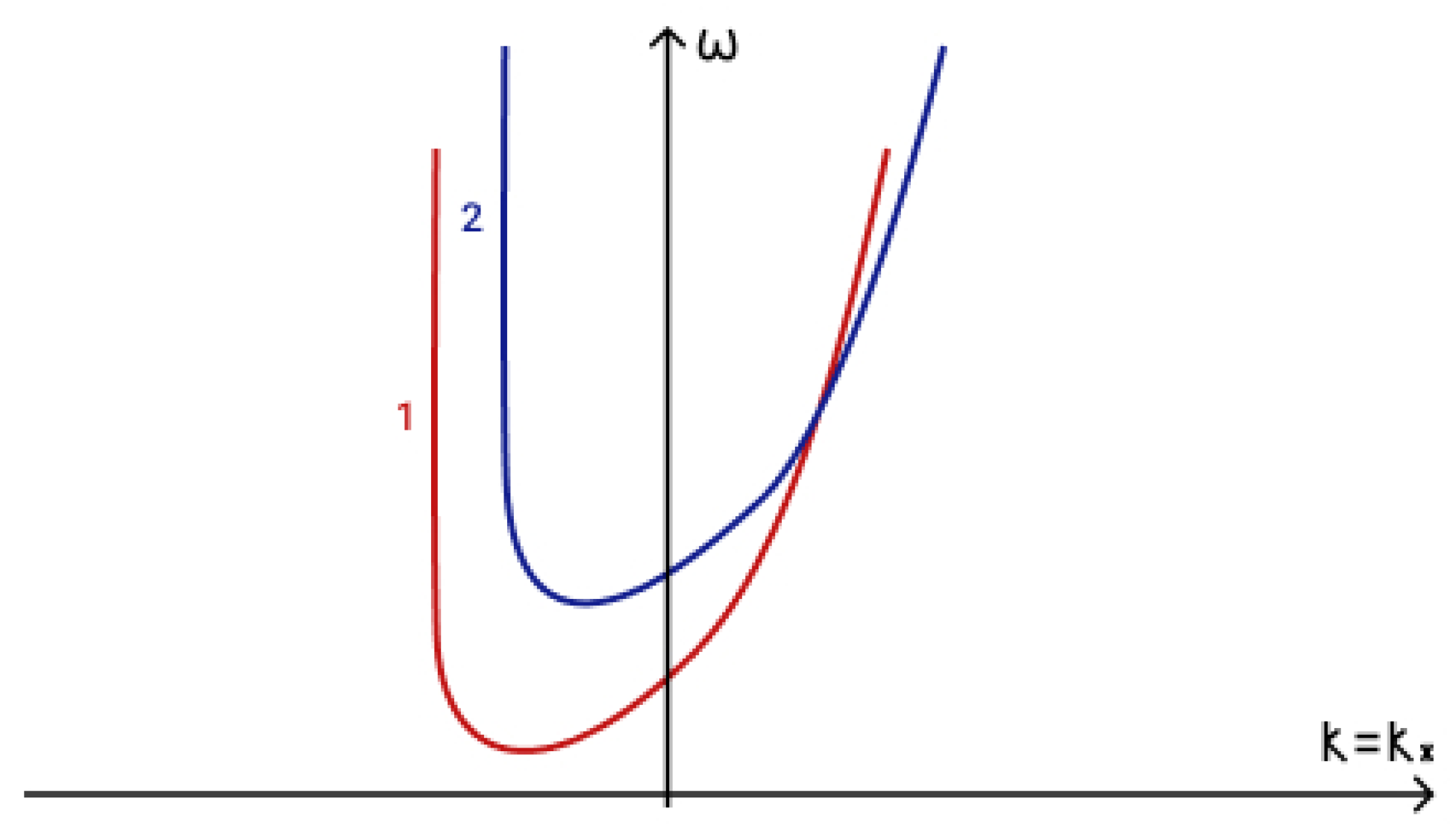
2.3. Three Waves Resonances and Parametric Instabilities in Two-Layer Model for Stratified Astrophysical Plasma
3. Stratified Flows of Rotating Astrophysical Plasma in Boussinesq Approximation
3.1. Linear Waves on f-Plane in a Stratified Astrophysical Plasma in Boussinesq Approximation
3.2. Linear Waves on -Plane in a Stratified Astrophysical Plasma in Boussinesq Approximation
3.3. Three Waves Resonances and Parametric Instabilities in Boussinesq Model for Stratified Astrophysical Plasma
4. Compressible Stratified Flows of Rotating Astrophysical Plasma in Anelastic Approximation
4.1. Linear Waves on f-Plane in a Stratified Compressible Astrophysical Plasma in Anelastic Approximation
4.2. Linear Waves on -Plane in a Stratified Compressible Astrophysical Plasma in Anelastic Approximation
4.3. Three Waves Resonances and Parametric Instabilities in Anelastic Model for Compressible Stratified Astrophysical Plasma
5. Compressible Flows of Rotating Astrophysical Plasma in Magnetohydrodynamic Shallow Water Approximation
5.1. Linear Waves on f-Plane in a Compressible Astrophysical Plasma in Magnetohydrodynamic Shallow Water Approximation
5.2. Linear Waves on -Plane in a Compressible Astrophysical Plasma in Magnetohydrodynamic Shallow Water Approximation
5.3. Three Waves Resonances and Parametric Instabilities in Magnetohydrodynamic Shallow Water Model for Compressible Astrophysical Plasma
6. Conclusions
6.1. Magnetohydrodynamic Two-Layer Shallow-Water Approximation
6.2. Magnetohydrodynamic Boussinesq Approximation
6.3. Magnetohydrodynamic Anelastic Approximation
6.4. Magnetohydrodynamic Shallow Water Approximation with Large-Scale Compressibility
Author Contributions
Funding
Conflicts of Interest
References
- Hughes, D.W.; Rosner, R.; Weiss, N.O. The Solar Tachocline; CUP: Cambridge, UK, 2007. [Google Scholar]
- Gilman, P.A. Magnetohydrodynamic shallow water equations for the solar tachocline. Astrophys. J. Lett. 2000, 544, L79. [Google Scholar] [CrossRef]
- Miesch, M.S.; Gilman, P.A. Thin-shell magnetohydrodynamic equations for the solar tachocline. Sol. Phys. 2004, 220, 287–305. [Google Scholar] [CrossRef]
- Dikpati, M.; Gilman, P.A. Analysis of hydrodynamic stability of solar tachocline latitudinal differential rotation using a shallow-water model. Astrophys. J. 2001, 551, 536. [Google Scholar] [CrossRef]
- Inogamov, N.A.; Sunyaev, R. Spread of matter over a neutron star surface during disk accretion. Astron. Lett. 1999, 25, 269. [Google Scholar]
- Inogamov, N.A.; Sunyaev, R.A. Spread of matter over a neutron-star surface during disk accretion: Deceleration of rapid rotation. Astron. Lett. 2010, 36, 848–894. [Google Scholar] [CrossRef]
- Spitkovsky, A.; Levin, Y.; Ushomirsky, G. Propagation of thermonuclear flames on rapidly rotating neutron stars: Extreme weather during type I X-ray bursts. Astrophys. J. 2002, 566, 1018. [Google Scholar] [CrossRef]
- Cho, J.Y. Atmospheric dynamics of tidally synchronized extrasolar planets. Philos. Trans. R. Soc. A 2008, 366, 4477–4488. [Google Scholar] [CrossRef]
- Petrosyan, A.S.; Klimachkov, D.A.; Fedotova, M.A.; Zinyakov, T.A. Shallow water magnetohydrodynamics in plasma astrophysics. Waves, turbulence, and zonal flows. Atmosphere 2020, 11, 314. [Google Scholar] [CrossRef]
- Zinyakov, T.A.; Petrosyan, A.S. Zonal Flows in Two-Dimensional Decaying Magnetohydrodynamic Turbulence on a β-Plane. JETP Lett. 2018, 108, 85–92. [Google Scholar] [CrossRef]
- Zinyakov, T.A.; Petrosyan, A.S. Spectra of Two-Dimensional Decaying Magnetohydrodynamic Turbulence on a β-Plane. JETP Lett. 2020, 111, 76–84. [Google Scholar] [CrossRef]
- Sirazov, R.A.; Petrosyan, A.S. Nonlinear Transformations of the Kinetic and Magnetic Energies in Rotating Magnetohydrodynamic Turbulent Flows. JETP Lett. 2019, 110, 329–335. [Google Scholar] [CrossRef]
- Dikpati, M.; Charbonneau, P. A Babcock-Leighton flux transport dynamo with solar-like differential rotation. Astrophys. J. 1999, 518, 508. [Google Scholar] [CrossRef]
- Zaqarashvili, T.V.; Oliver, R.; Ballester, J.L.; Shergelashvili, B.M. Rossby waves in shallow water magnetohydrodynamics. Astron. Astrophys. 2007, 470, 815–820. [Google Scholar] [CrossRef]
- Zaqarashvili, T.V.; Oliver, R.; Ballester, J.L.; Carbonell, M.; Khodachenko, M.L.; Lammer, H.; Leitzinger, M.; Odert, P. Rossby waves and polar spots in rapidly rotating stars: Implications for stellar wind evolution. Astron. Astrophys. 2011, 532, A139. [Google Scholar] [CrossRef]
- Braithwaite, J.; Spruit, H.C. Magnetic fields in non-convective regions of stars. R. Soc. Open Sci. 2017, 4, 160271. [Google Scholar] [CrossRef] [PubMed]
- Philidet, J.; Gissinger, C.; Lignières, F.; Petitdemange, L. Magnetohydrodynamics of stably stratified regions in planets and stars. Geophys. Astrophys. Fluid Dyn. 2020, 114, 336–355. [Google Scholar] [CrossRef]
- Stone, J.M.; Hawley, J.F.; Gammie, C.F.; Balbus, S.A. Three-dimensional magnetohydrodynamical simulations of vertically stratified accretion disks. Astrophys. J. 1996, 463, 656. [Google Scholar] [CrossRef]
- Lovelace, R.V.E.; Romanova, M.M. Rossby wave instability in astrophysical discs. Fluid Dyn. Res. 2014, 46, 041401. [Google Scholar] [CrossRef][Green Version]
- Batygin, K.; Stanley, S.; Stevenson, D.J. Magnetically controlled circulation on hot extrasolar planets. Astrophys. J. 2013, 776, 53. [Google Scholar] [CrossRef]
- Böning, V.G.; Hu, H.; Gizon, L. Signature of solar g modes in first-order p-mode frequency shifts. Astron. Astrophys. 2019, 629, A26. [Google Scholar] [CrossRef]
- Loeptien, B.; Gizon, L.; Birch, A.C.; Schou, J.; Proxauf, B.; Duvall, T.L.; Bogart, R.S.; Christensen, U.R. Global-scale equatorial Rossby waves as an essential component of solar internal dynamics. Nat. Astron. 2018, 7, 1. [Google Scholar] [CrossRef]
- Dikpati, M.; Belucz, B.; Gilman, P.A.; McIntosh, S.W. Phase Speed of Magnetized Rossby Waves that Cause Solar Seasons. Astrophys. J. 2018, 862, 159. [Google Scholar] [CrossRef]
- McIntosh, S.W.; Cramer, W.J.; Marcano, M.P.; Leamon, R.J. The detection of Rossby-like waves on the Sun. Nat. Astron. 2017, 1, 0086. [Google Scholar] [CrossRef]
- Zaqarashvili, T.V.; Gurgenashvili, E. Magneto-Rossby waves and seismology of solar interior. Front. Astron. Space Sci. 2018, 5, 7. [Google Scholar] [CrossRef]
- Gizon, L.; Fournier, D.; Albekioni, M. Effect of latitudinal differential rotation on solar Rossby waves: Critical layers, eigenfunctions, and momentum fluxes in the equatorial β plane. Astron. Astrophys. 2020, 642, A178. [Google Scholar] [CrossRef]
- Klimachkov, D.A.; Petrosyan, A.S. Rossby waves in the magnetic fluid dynamics of a rotating plasma in the shallow-water approximation. J. Exp. Theor. Phys. 2017, 125, 597–612. [Google Scholar] [CrossRef]
- Fedotova, M.A.; Klimachkov, D.A.; Petrosyan, A.S. The Shallow-Water Magnetohydrodynamic Theory of Stratified Rotating Astrophysical Plasma Flows: Beta-Plane Approximation and magneto-Rossby Waves. Plasma Phys. Rep. 2020, 46, 50. [Google Scholar] [CrossRef]
- Fedotova, M.A.; Petrosyan, A.S. Wave processes in three-dimensional stratified flows of a rotating plasma in the Boussinesq approximation. J. Exp. Theor. Phys. 2020, 131, 337–355. [Google Scholar] [CrossRef]
- Zaqarashvili, T.V.; Albekioni, M.; Ballester, J.L.; Bekki, Y.; Biancofiore, L.; Birch, A.C.; Dikpati, M.; Gizon, L.; Gurgenashvili, E.; Heifetz, E.; et al. Rossby Waves in Astrophysics. Space Sci. Rev. 2021, 217, 1–93. [Google Scholar] [CrossRef]
- Dikpati, M.; Cally, P.S.; McIntosh, S.W.; Heifetz, E. The Origin of the Seasons in Space Weather. Sci. Rep. 2017, 7, 14750. [Google Scholar] [CrossRef]
- Lou, Y.Q. Rossby-type wave-induced periodicities in flare activities and sunspot areas or groups during solar maxima. Astrophys. J. 2000, 540, 1102. [Google Scholar] [CrossRef]
- Dikpati, M.; McIntosh, S.W. Space weather challenge and forecasting implications of Rossby waves. Space Weather 2020, 18, e2018SW002109. [Google Scholar] [CrossRef]
- Tobias, S.M.; Diamond, P.H.; Hughes, D.W. β-plane magnetohydrodynamic turbulence in the solar tachocline. Astrophys. J. Lett. 2007, 667, L113. [Google Scholar] [CrossRef]
- Hori, K.; Jones, C.A.; Teed, R.J. Slow magneto-Rossby waves in the Earth’s core. Geophys. Res. Lett. 2015, 42, 6622–6629. [Google Scholar] [CrossRef]
- Petviashvili, V.I.; Pokhotelov, O.A. Solitary Waves in Plasmas and in the Atmosphere; Gordon and Breach Science Publishers: Reading, UK; Philadelphia, PA, USA, 1992. [Google Scholar]
- Onishchenko, O.G.E.; Pokhotelov, O.A.; Astafieva, N.M. Generation of large-scale eddies and zonal winds in planetary atmospheres. Phys. Uspekhi 2008, 51, 577. [Google Scholar] [CrossRef]
- Karelsky, K.V.; Petrosyan, A.S.; Tarasevich, S.V. Nonlinear dynamics of magnetohydrodynamic flows of a heavy fluid in the shallow water approximation. J. Exp. Theor. Phys. 2011, 113, 530. [Google Scholar] [CrossRef][Green Version]
- Karelsky, K.V.; Petrosyan, A.S.; Tarasevich, S.V. Nonlinear dynamics of magnetohydrodynamic shallow water flows over an arbitrary surface. Phys. Scr. 2013, 155, 014024. [Google Scholar] [CrossRef]
- Karelsky, K.V.; Petrosyan, A.S.; Tarasevich, S.V. Nonlinear dynamics of magnetohydrodynamic flows of a heavy fluid on slope in the shallow water approximation. J. Exp. Theor. Phys. 2014, 119, 311–325. [Google Scholar] [CrossRef]
- Klimachkov, D.A.; Petrosyan, A.S. Nonlinear wave interactions in shallow water magnetohydrodynamics of astrophysical plasma. J. Exp. Theor. Phys. 2016, 122, 832–848. [Google Scholar] [CrossRef]
- Klimachkov, D.A.; Petrosyan, A.S. Nonlinear theory of magnetohydrodynamic flows of a compressible fluid in the shallow water approximation. J. Exp. Theor. Phys. 2016, 123, 520–539. [Google Scholar] [CrossRef]
- Klimachkov, D.A.; Petrosyan, A.S. Parametric instabilities in shallow water magnetohydrodynamics of astrophysical plasma in external magnetic field. Phys. Lett. A 2017, 381, 106–113. [Google Scholar] [CrossRef]
- Zaqarashvili, T.V.; Oliver, R.; Ballester, J.L. Global shallow water magnetohydrodynamic waves in the solar tachocline. Astrophys. J. Lett. 2009, 691, L41. [Google Scholar] [CrossRef]
- Heng, K.; Spitkovsky, A. Magnetohydrodynamic shallow water waves: Linear analysis. Astrophys. J. 2009, 703, 1819. [Google Scholar] [CrossRef]
- Dikpati, M.; McIntosh, S.W.; Bothun, G.; Cally, P.S.; Ghosh, S.S.; Gilman, P.A.; Umurhan, O.M. Role of Interaction between magneto-Rossby Waves and Tachocline Differential Rotation in Producing Solar Seasons. Astrophys. J. 2018, 853, 144. [Google Scholar] [CrossRef]
- Marquez-Artavia, X.; Jones, C.A.; Tobias, S.M. Rotating magnetic shallow water waves and instabilities in a sphere. Geophys. Astrophys. Fluid 2017, 111, 282–322. [Google Scholar] [CrossRef]
- Zaqarashvili, T. Equatorial magnetohydrodynamic shallow water waves in the solar tachocline. Astrophys. J. 2018, 856, 32. [Google Scholar] [CrossRef]
- Vallis, G.K. Atmospheric and Oceanic Fluid Dynamics: Fundamental and Large-Scale Circulation; CUP: Cambridge, UK, 2006. [Google Scholar]
- Zeitlin, V. Geophysical Fluid Dynamics: Understanding (Almost) Everything with Rotating Shallow Water Models; OUP: Oxford, UK, 2018. [Google Scholar]
- Kaladze, T.D.; Horton, W.; Kahlon, L.Z.; Pokhotelov, O.; Onishchenko, O. Zonal flows and magnetic fields driven by large-amplitude Rossby-Alfvén-Khantadze waves in the E-layer ionosphere. J. Geophys. Res. Space Phys. 2013, 118, 7822–7833. [Google Scholar] [CrossRef]
- Balk, A.M. Large-scale quasi-geostrophic magnetohydrodynamics. Astrophys. J. 2014, 796, 143. [Google Scholar] [CrossRef]
- Dikpati, M.; Gilman, P.A.; Chatterjee, S.; McIntosh, S.W.; Zaqarashvili, T.V. Physics of magnetohydrodynamic Rossby waves in the sun. Astrophys. J. 2020, 896, 141. [Google Scholar] [CrossRef]
- Mandal, K.; Hanasoge, S. Properties of solar Rossby waves from normal mode coupling and characterizing its systematics. Astrophys. J. 2020, 891, 125. [Google Scholar] [CrossRef]
- Raphaldini, B.; Medeiros, E.; Raupp, C.F.; Teruya, A.S. A new mechanism for maunder-like solar minima: Phase synchronization dynamics in a simple nonlinear oscillator of magnetohydrodynamic Rossby waves. Astrophys. J. Lett. 2020, 890, L13. [Google Scholar] [CrossRef]
- Onishchenko, O.G.; Pokhotelov, O.A.; Sagdeev, R.Z.; Shukla, P.K.; Stenflo, L. Generation of zonal flows by Rossby waves in the atmosphere. Nonlinear Process. Geophys. 2004, 11, 241–244. [Google Scholar] [CrossRef]
- Liang, Z.C.; Gizon, L.; Birch, A.C.; Duvall, T.L. Time-distance helioseismology of solar Rossby waves. Astron. Astrophys. 2019, 626, A3. [Google Scholar] [CrossRef]
- Raphaldini, B.; Raupp, C.F. Nonlinear dynamics of magnetohydrodynamic Rossby waves and the cyclic nature of solar magnetic activity. Astrophys. J. 2015, 799, 78. [Google Scholar] [CrossRef]
- Raphaldini, B.; Teruya, A.S.; Raupp, C.F.; Bustamante, M.D. Nonlinear Rossby wave—wave and wave—mean flow theory for long-term solar cycle modulations. Astrophys. J. 2019, 887, 1. [Google Scholar] [CrossRef]
- Lou, Y.Q. Nonlinear magnetohydrodynamic waves in a steady zonal circulation for a shallow fluid shell on the surface of a rotating sphere. Astrophys. J. 1987, 322, 862–869. [Google Scholar] [CrossRef]
- Gilman, P.A. Stability of baroclin flows in a zonal magnetic field: Part I. J. Atmos. Sci. 1967, 24, 101–118. [Google Scholar] [CrossRef][Green Version]
- De Sterck, H. Hyperbolic theory of the shallow water magnetohydrodynamics equations. Phys. Plasmas 2001, 8, 3293–3304. [Google Scholar] [CrossRef]
- Dellar, P.J. Dispersive shallow water magnetohydrodynamics. Phys. Plasmas 2003, 10, 581–590. [Google Scholar] [CrossRef]
- Zeitlin, V. Remarks on rotating shallow-water magnetohydrodynamics. Nonlinear Process. Geophys. 2013, 20, 893–908. [Google Scholar] [CrossRef]
- Brown, B.P.; Vasil, G.M.; Zweibel, E.G. Energy conservation and gravity waves in sound-proof treatments of stellar interiors. Part I. Anelastic approximations. Astrophys. J. 2012, 756, 109. [Google Scholar] [CrossRef]
- Fedotova, M.A.; Petrosyan, A.S. Wave Processes in Rotating Compressible Astrophysical Plasma Flows with Stable Stratification. J. Exp. Theor. Phys. 2020, 131, 1032–1055. [Google Scholar] [CrossRef]
- Klimachkov, D.A.; Petrosyan, A.S. Large-scale compressibility in rotating flows of astrophysical plasma in the shallow water approximation. J. Exp. Theor. Phys. 2018, 127, 1136–1152. [Google Scholar] [CrossRef]
- Kupka, F. Thermal Convection in Stars and in Their Atmosphere. Multi-Dimensional Processes. In Stellar Physics; EDP Sciences: Les Ulis, France, 2021; pp. 69–110. [Google Scholar]
- Miesch, M.; Matthaeus, W.; Brandenburg, A.; Petrosyan, A.; Pouquet, A.; Cambon, C.; Jenko, F.; Uzdensky, D.; Stone, J.; Tobias, S.; et al. Large-Eddy Simulations of Magnetohydrodynamic Turbulence in Heliophysics and Astrophysics. Space Sci. Rev. 2015, 194, 97–137. [Google Scholar] [CrossRef]
- Chernyshov, A.A.; Karelsky, K.V.; Petrosyan, A.S. Subgrid-scale modeling for the study of compressible magnetohydrodynamic turbulence in space plasmas. Phys. Uspekhi 2014, 57, 421. [Google Scholar] [CrossRef]
- Schmidt, W. Large Eddy Simulations in Astrophysics. Living Rev. Comput. Astrophys. 2015, 1, 2. [Google Scholar] [CrossRef]
- Kupka, F.; Muthsam, H.J. Modelling of stellar convection. Living Rev. Comput. Astrophys. 2017, 3, 1. [Google Scholar] [CrossRef]
- Balsara, D.S. Higher-order accurate space-time schemes for computational astrophysics—Part I: Finite volume methods. Living Rev. Comput. Astrophys. 2017, 3, 2. [Google Scholar] [CrossRef]
- Almgren, A.S.; Bell, J.B.; Nonaka, A.; Zingale, M. A New Low Mach Number Approach in Astrophysics. Comput. Sci. Eng. 2009, 11, 24–33. [Google Scholar] [CrossRef]
- Karelsky, K.V.; Petrosyan, A.S.; Chernyak, A.V. Nonlinear dynamics of flows of a heavy compressible gas in the shallow water approximation. J. Exp. Theor. Phys. 2012, 114, 1058–1071. [Google Scholar] [CrossRef]
- Karel’skii, K.V.; Petrosyan, A.S.; Chernyak, A.V. Nonlinear theory of the compressible gas flows over a nonuniform boundary in the gravitational field in the shallow-water approximation. J. Exp. Theor. Phys. 2013, 116, 680–697. [Google Scholar] [CrossRef]
- Batchelor, C.K.; Batchelor, G.K. An Introduction to Fluid Dynamics; CUP: Cambridge, UK, 2000. [Google Scholar]
- Hunter, S. Waves in Shallow Water Magnetohydrodynamics. Ph.D. Thesis, The University of Leeds Department of Applied Mathematics, Leeds, UK, August 2015. Available online: http://etheses.whiterose.ac.uk/11475/ (accessed on 2 March 2021).
- Dolzhanskii, F.V. Fundamentals of Geophysical Hydrodynamics; Fizmatli: Moscow, Russia, 2016. (In Russian) [Google Scholar]
- Nezlin, M.V.; Snezhkin, E.N. Rossby Vortices and Spiral Structures-Astrophysics and Plasma Physics in Shallow Water Experiments; Nauka: Moscow, Russia, 1990. (In Russian) [Google Scholar]
- Saio, H. R-mode oscillations in uniformly rotating stars. Astrophys. J. 1982, 256, 717–735. [Google Scholar] [CrossRef]
- Sturrock, P.A.; Bush, R.; Gough, O.O.; Scargle, J.D. Indications of r-mode oscillations in SOHO/MDI solar radius measurements. Astrophys. J. 2015, 804, 47. [Google Scholar] [CrossRef]
- Wolff, C.L. Linear r-mode oscillations in a differentially rotating star. Astrophys. J. 1998, 502, 961–967. [Google Scholar] [CrossRef]
- Karelsky, K.V.; Petrosyan, A.S. Particular solutions and Riemann problem for modified shallow water equations. Fluid Dyn. Res. 2006, 38, 339. [Google Scholar] [CrossRef]
- Ostrovsky, L. Asymptotic Perturbation Theory of Waves; WS: Singapore, 2014. [Google Scholar]
- Newell, A.C. Rossby wave packet interactions. J. Fluid Mech. 1969, 35, 255–271. [Google Scholar] [CrossRef]
- Rieutord, M.; Valdettaro, L. Inertial waves in a rotating spherical shell. J. Fluid Mech. 1997, 341, 77–99. [Google Scholar] [CrossRef]
- Dintrans, B.; Rieutord, M.; Valdettaro, L. Gravito-inertial waves in a rotating stratified sphere or spherical shell. J. Fluid Mech. 1999, 398, 271–297. [Google Scholar] [CrossRef]
- Berkoff, N.A. The Anelastic Approximation: Magnetic Buoyancy and Magnetoconvection. Ph.D. Thesis, University of Leeds, Leeds, UK, July 2011. Available online: https://etheses.whiterose.ac.uk/15942/1/thesis.pdf (accessed on 2 March 2021).
- Spiegel, E.A.; Veronis, G. On the Boussinesq approximation for a compressible fluid. Astrophys. J. 1960, 131, 442. [Google Scholar] [CrossRef]
- Spiegel, E.A.; Weiss, N.O. Magnetic buoyancy and the Boussinesq approximation. Geophys. Astrophys. Fluid Dyn. 1982, 22, 219–234. [Google Scholar] [CrossRef]
- Billant, P.; Chomaz, J.M. Self-similarity of strongly stratified inviscid flows. Phys. Fluids 2001, 13, 1645–1651. [Google Scholar] [CrossRef]
- Yano, J.I. Inertio-gravity waves under the non-traditional f-plane approximation: Singularity in the large-scale limit. J. Fluid Mech. 2017, 810, 475–488. [Google Scholar] [CrossRef]
- Lee, S.; Takada, R. Dispersive estimates for the stably stratified Boussinesq equations. Indiana Univ. Math. J. 2017, 66, 2037–2070. [Google Scholar]
- Takehiro, S.I. Penetration of Alfvén waves into an upper stably-stratified layer excited by magnetoconvection in rotating spherical shells. Phys. Earth Planet. Inter. 2015, 241, 37–43. [Google Scholar] [CrossRef]
- Takehiro, S.I.; Sasaki, Y. Penetration of steady fluid motions into an outer stable layer excited by MHD thermal convection in rotating spherical shells. Phys. Earth Planet. Inter. 2018, 276, 258–264. [Google Scholar] [CrossRef]
- Nakagawa, T. Effect of a stably stratified layer near the outer boundary in numerical simulations of a magnetohydrodynamic dynamo in a rotating spherical shell and its implications for Earth’s core. Phys. Earth Planet. Inter. 2011, 187, 342–352. [Google Scholar] [CrossRef]
- Raymond, D.J. Sound, Inertia-Gravity Waves, and Lamb Waves. 7 September 2011. Available online: http://kestrel.nmt.edu/~raymond/classes/ph589/notes/ssmodes/ssmodes.pdf (accessed on 2 March 2021).
- Klein, R. Scale Analysis of Compressible Flows from an Application Perspective. In Handbook of Mathematical Analysis in Mechanics of Viscous Fluids; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Batchelor, G.K. The conditions for dynamical similarity of motions of a frictionless perfect-gas atmosphere. Q. J. R. Meteorol. Soc. 1953, 79, 224–235. [Google Scholar] [CrossRef]
- Charney, J.G.; Ogura, Y. A numerical model for thermal convection in the atmosphere. J. Meteorol. Soc. Jpn. 1960, 38, 19a. [Google Scholar] [CrossRef]
- Gough, D.O. The anelastic approximation for thermal convection. J. Atmos. Sci. 1969, 26, 448–456. [Google Scholar] [CrossRef]
- Bannon, P.R. On the anelastic approximation for a compressible atmosphere. J. Atmos. Sci. 1996, 53, 3618–3628. [Google Scholar] [CrossRef]
- Calkins, M.A.; Julien, K.; Marti, P. The breakdown of the anelastic approximation in rotating compressible convection: Implications for astrophysical systems. Proc. R. Soc. A 2015, 471, 20140689. [Google Scholar] [CrossRef] [PubMed]
- Paolucci, S. Filtering of Sound from the Navier-Stokes Equations; Sandia National Laboratories: Livermore, CA, USA, 1982; pp. 3–52.
- Botta, N.; Klein, R.; Almgren, A. Dry Atmosphere Asymptotics; Potsdam Institute for Climate Impact Research: Potsdam, Germany, 1999. [Google Scholar]
- Klein, R.; Botta, N.; Schneider, T.; Munz, C.D.; Roller, S.; Meister, A.; Hoffman, L.; Sonar, T. Asymptotic adaptive methods for multi-scale problems in fluid mechanics. J. Eng. Math. 2001, 39, 261–343. [Google Scholar] [CrossRef]
- Braginsky, S.I.; Roberts, P.H. Equations governing convection in Earth’s core and the geodynamo. Geophys. Astrophys. Fluid Dyn. 1995, 79, 1–97. [Google Scholar] [CrossRef]
- Glatzmaier, G.A.; Roberts, P.H. An anelastic evolutionary geodynamo simulation driven by compositional and thermal convection. Phys. D 1996, 97, 81–94. [Google Scholar] [CrossRef]
- Olson, P.; Christensen, U.R. Dipole moment scaling for convection-driven planetary dynamos. Earth Planet. Sci. Lett. 2006, 250, 561–571. [Google Scholar] [CrossRef]
- Jones, C.A.; Kuzanyan, K.M.; Mitchell, R.H. Linear theory of compressible convection in rapidly rotating spherical shells, using the anelastic approximation. J. Fluid Mech. 2009, 634, 291. [Google Scholar] [CrossRef]
- Gilman, P.A.; Glatzmaier, G.A. Compressible convection in a rotating spherical shell. I-Anelastic equations. II-A linear anelastic model. III-Analytic model for compressible vorticity waves. Astrophys. J. Suppl. Ser. 1981, 45, 335–388. [Google Scholar] [CrossRef]
- Yadav, R.K.; Bloxham, J. Deep rotating convection generates the polar hexagon on Saturn. Proc. Natl. Acad. Sci. USA 2020, 117, 13991–13996. [Google Scholar] [CrossRef]
- Glatzmaier, G.A. Numerical simulations of stellar convective dynamos. I. The model and method. J. Comput. Phys. 1984, 55, 461–484. [Google Scholar] [CrossRef]
- Lantz, S.R.; Fan, Y. Anelastic magnetohydrodynamic equations for modeling solar and stellar convection zones. Astrophys. J. Suppl. Ser. 1999, 121, 247. [Google Scholar] [CrossRef]
- Miesch, M.S.; Elliott, J.R.; Toomre, J.; Clune, T.L.; Glatzmaier, G.A.; Gilman, P.A. Three-dimensional spherical simulations of solar convection. I. Differential rotation and pattern evolution achieved with laminar and turbulent states. Astrophys. J. 2000, 532, 593. [Google Scholar] [CrossRef]
- Brun, A.S.; Miesch, M.S.; Toomre, J. Global-scale turbulent convection and magnetic dynamo action in the solar envelope. Astrophys. J. 2004, 614, 1073. [Google Scholar] [CrossRef]
- Brown, B.P.; Browning, M.K.; Brun, A.S.; Miesch, M.S.; Toomre, J. Rapidly rotating suns and active nests of convection. Astrophys. J. 2008, 689, 1354. [Google Scholar] [CrossRef]
- Brown, B.P.; Miesch, M.S.; Browning, M.K.; Brun, A.S.; Toomre, J. Magnetic cycles in a convective dynamo simulation of a young solar-type star. Astrophys. J. 2011, 731, 69. [Google Scholar] [CrossRef]
- Smolarkiewicz, P.K.; Charbonneau, P. EULAG, a computational model for multiscale flows: An MHD extension. J. Comput. Phys. 2013, 236, 608–623. [Google Scholar] [CrossRef]
- Goldstein, J.; Townsend, R.H.D.; Zweibel, E.G. The Tayler Instability in the Anelastic Approximation. Astrophys. J. 2019, 881, 66. [Google Scholar] [CrossRef]


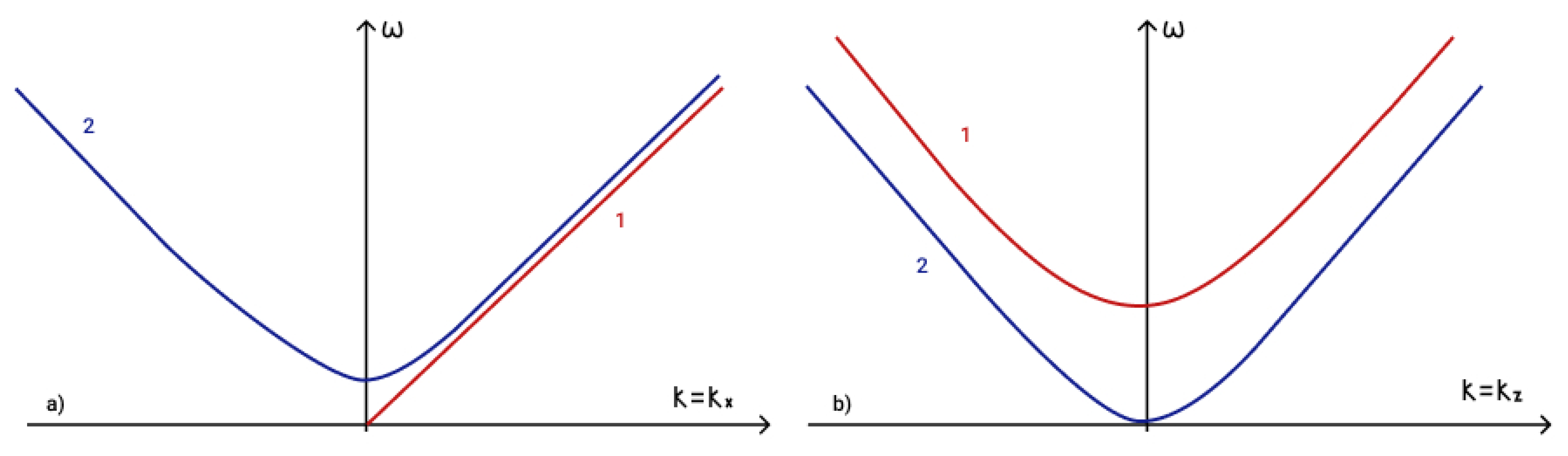
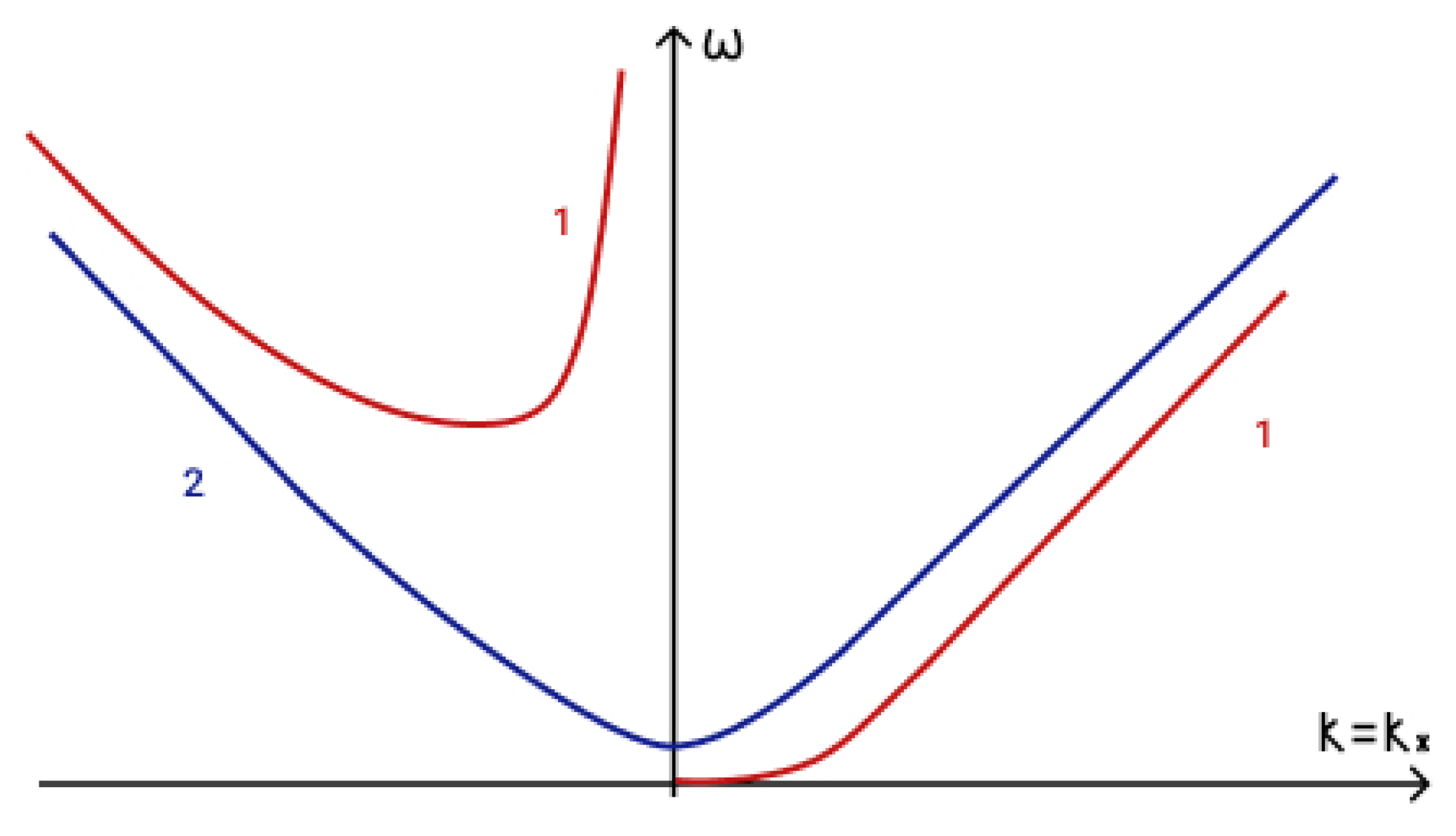



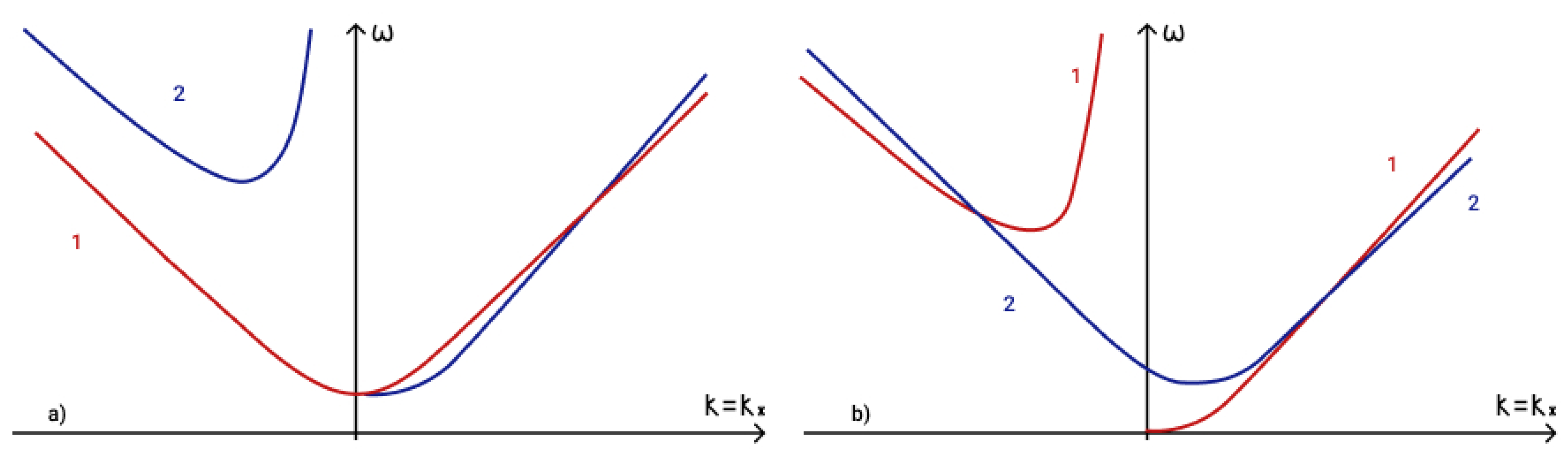
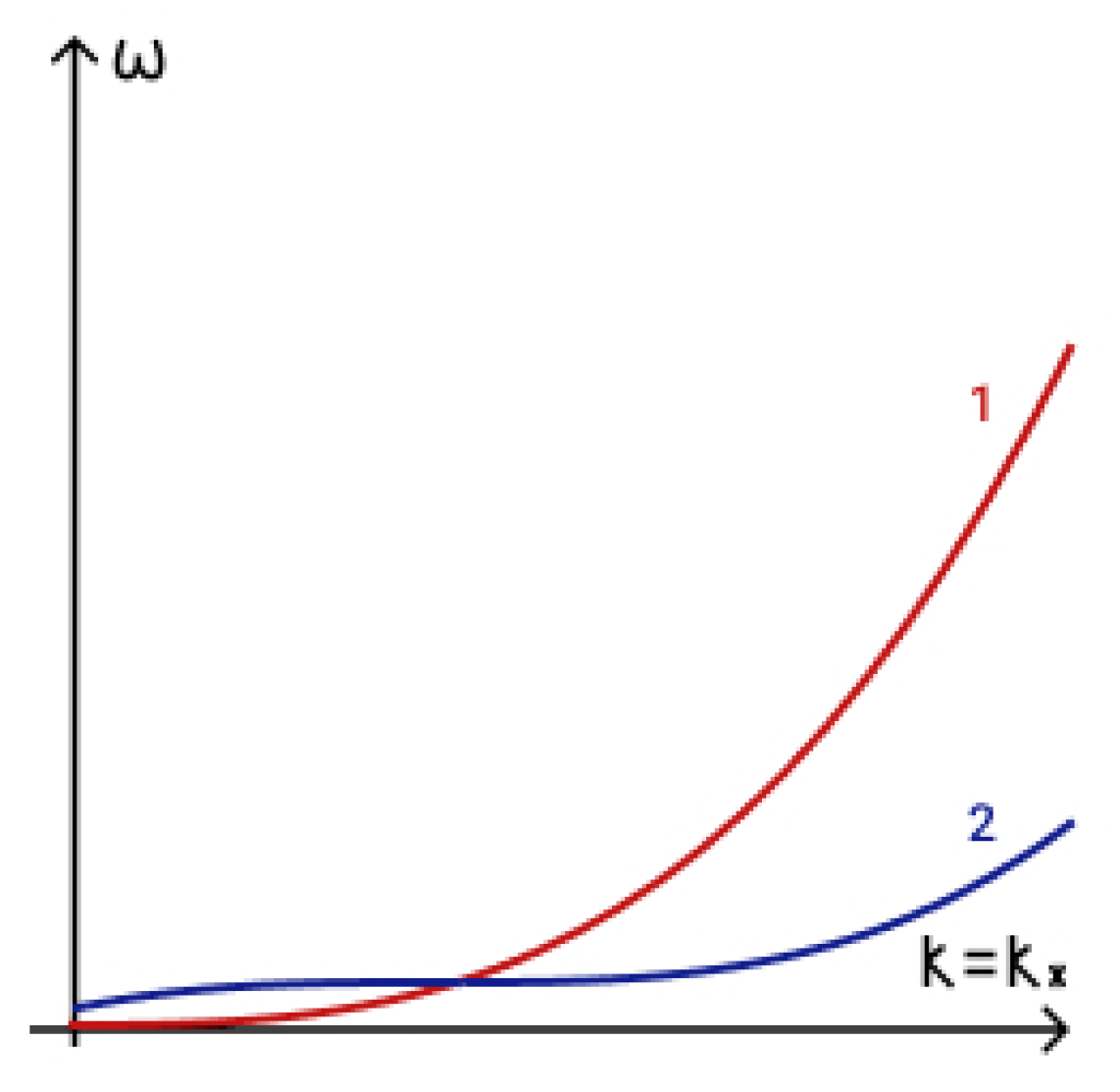



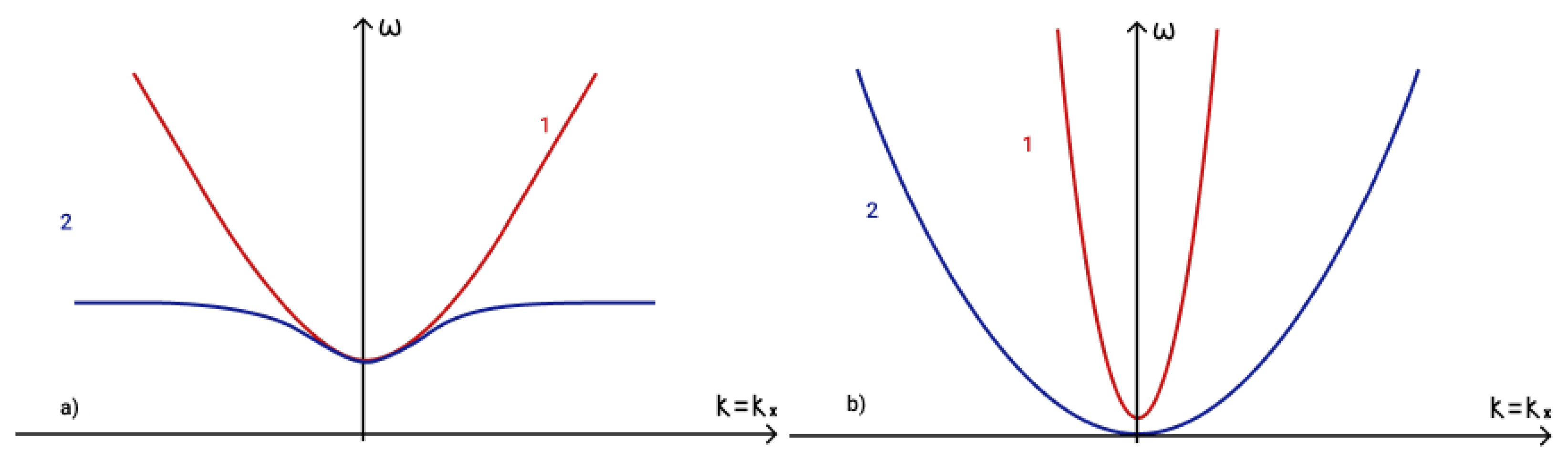
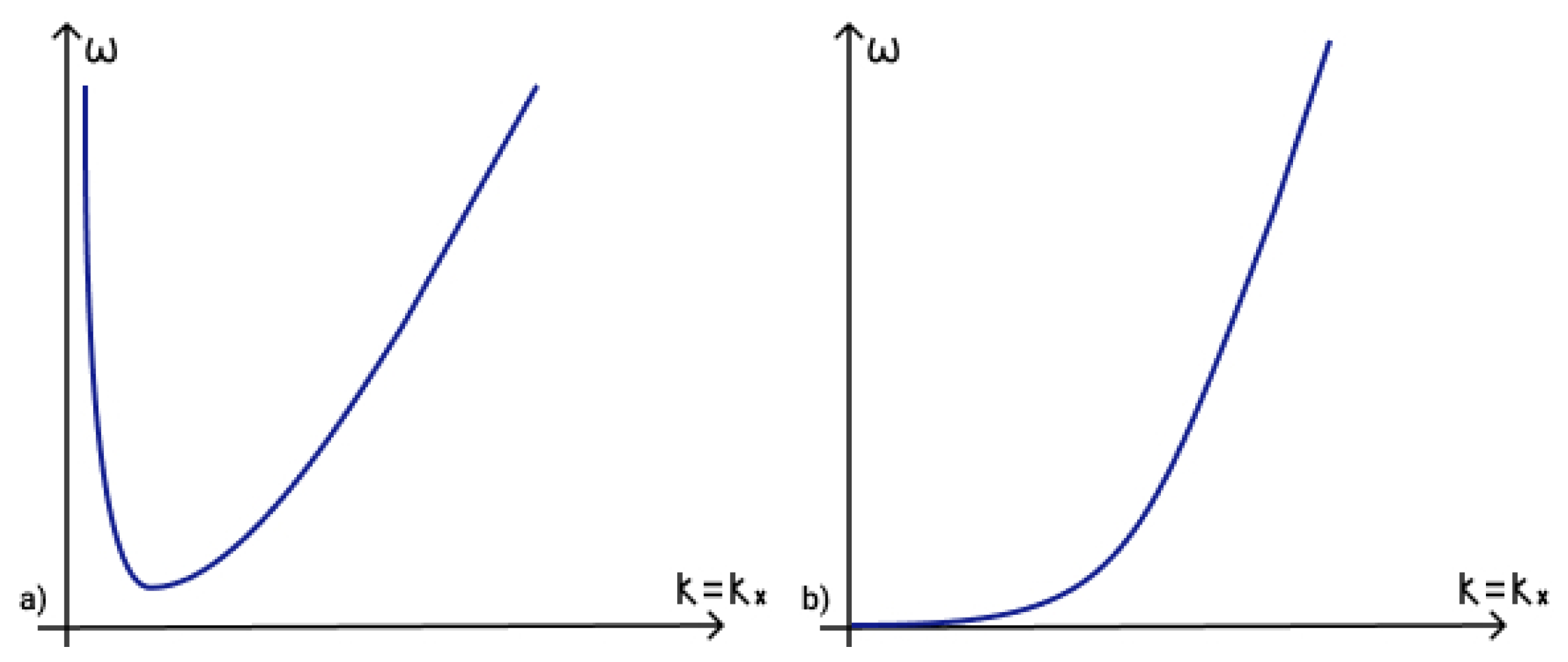

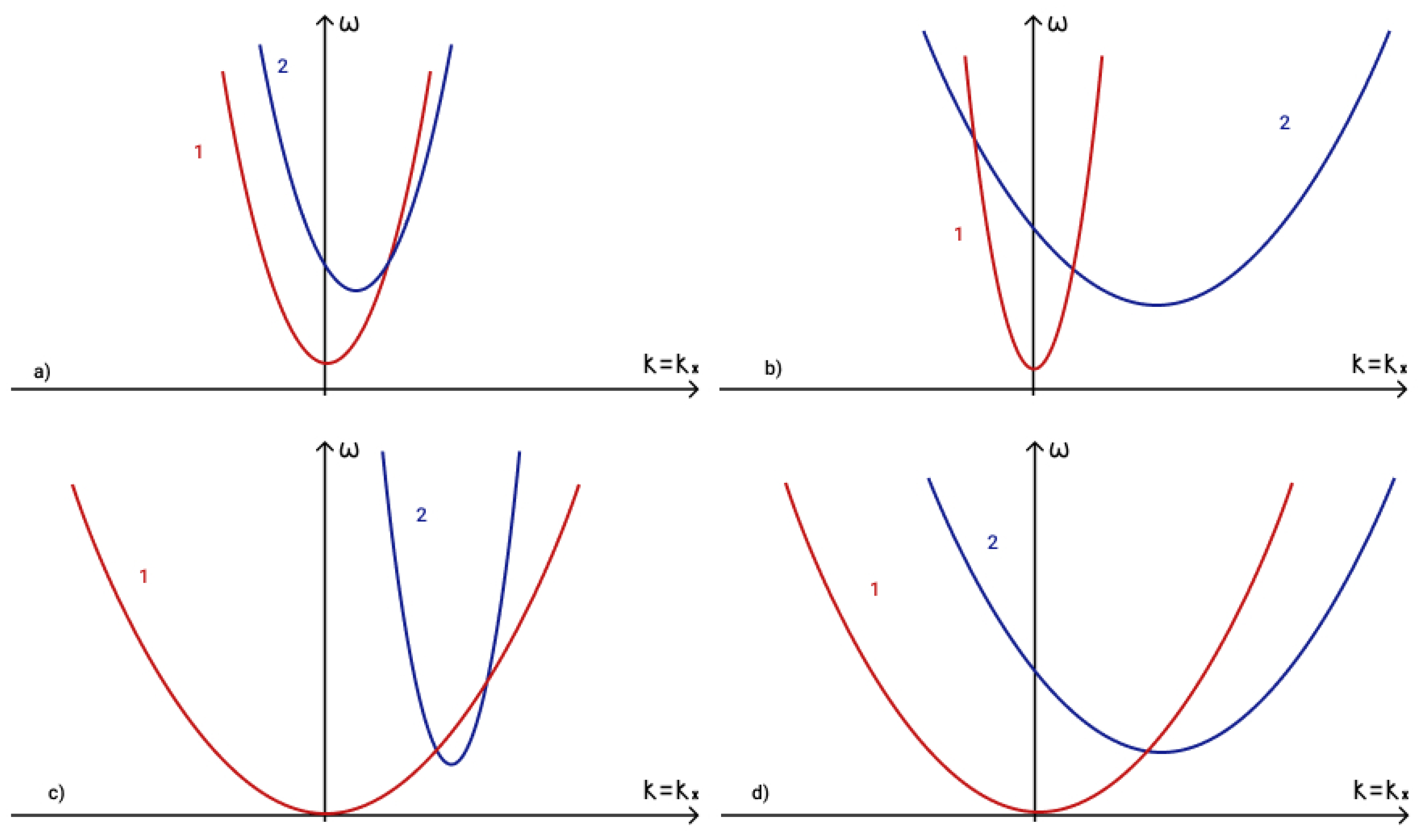

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fedotova, M.; Klimachkov, D.; Petrosyan, A. Variable Density Flows in Rotating Astrophysical Plasma. Linear Waves and Resonant Phenomena. Universe 2021, 7, 87. https://doi.org/10.3390/universe7040087
Fedotova M, Klimachkov D, Petrosyan A. Variable Density Flows in Rotating Astrophysical Plasma. Linear Waves and Resonant Phenomena. Universe. 2021; 7(4):87. https://doi.org/10.3390/universe7040087
Chicago/Turabian StyleFedotova, Maria, Dmitry Klimachkov, and Arakel Petrosyan. 2021. "Variable Density Flows in Rotating Astrophysical Plasma. Linear Waves and Resonant Phenomena" Universe 7, no. 4: 87. https://doi.org/10.3390/universe7040087
APA StyleFedotova, M., Klimachkov, D., & Petrosyan, A. (2021). Variable Density Flows in Rotating Astrophysical Plasma. Linear Waves and Resonant Phenomena. Universe, 7(4), 87. https://doi.org/10.3390/universe7040087





