Revisiting the Planet Mass and Stellar Metallicity Relation for Low-Mass Exoplanets Orbiting GKM Class Stars
Abstract
1. Introduction
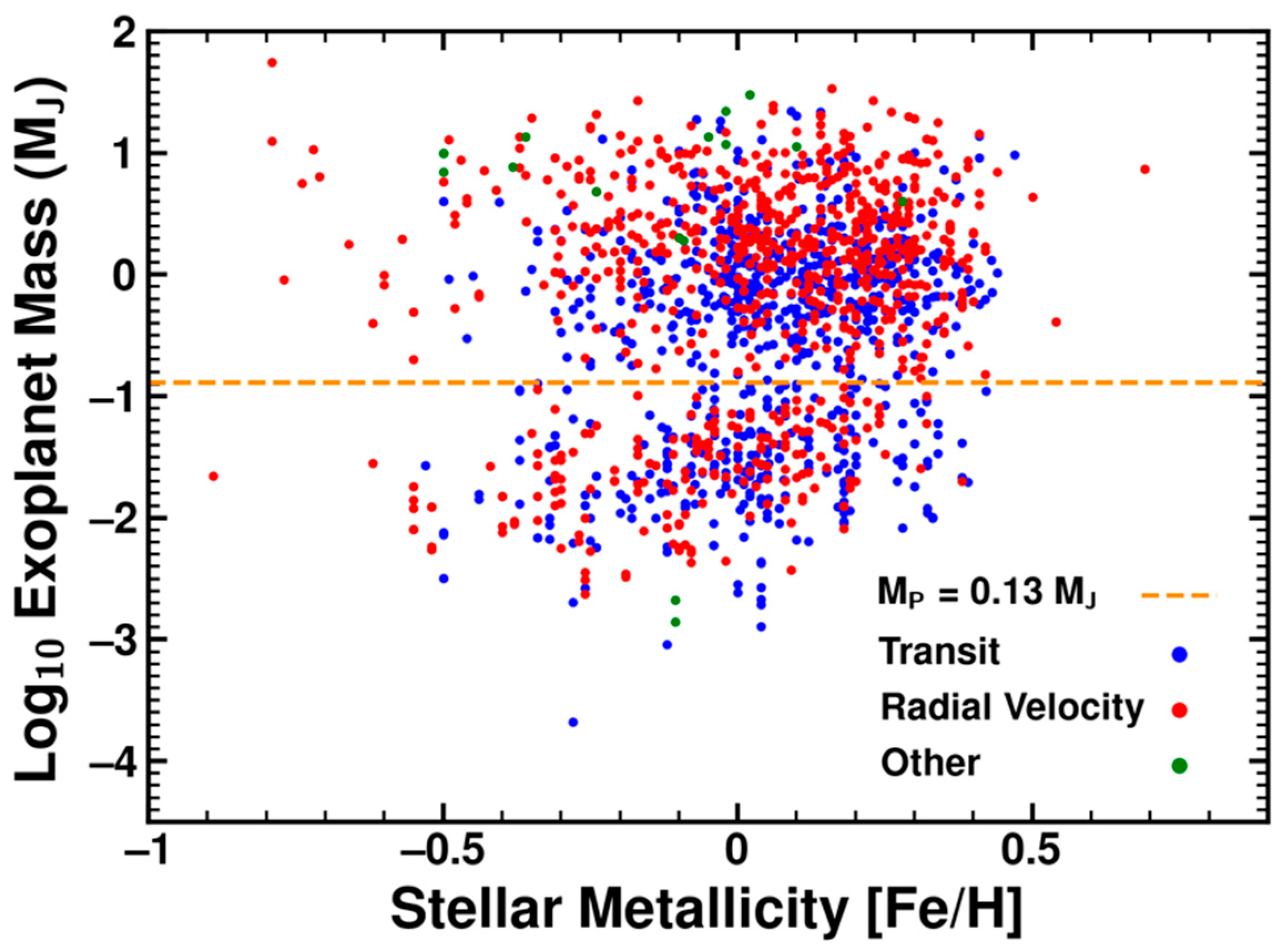
2. Data
3. Results
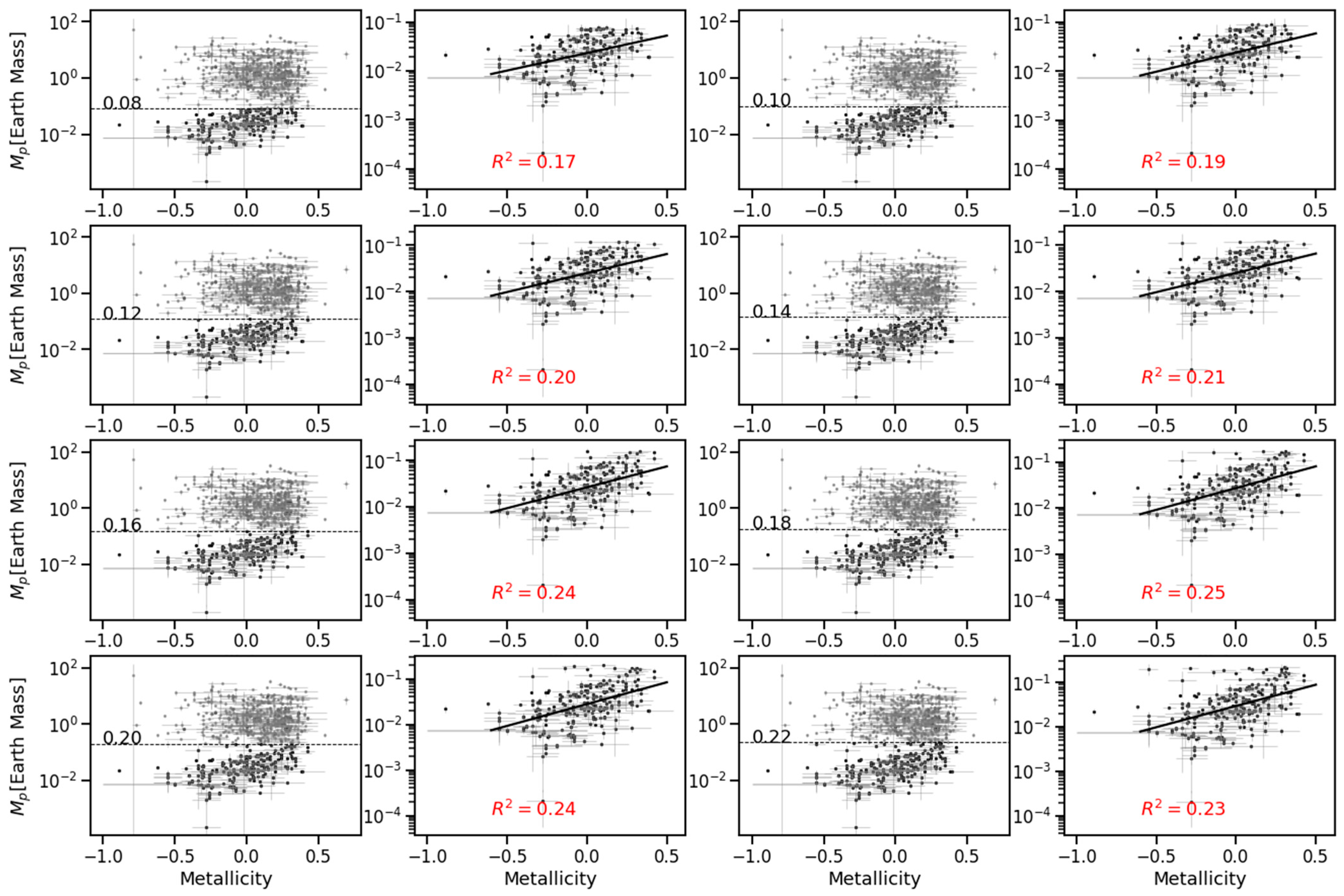
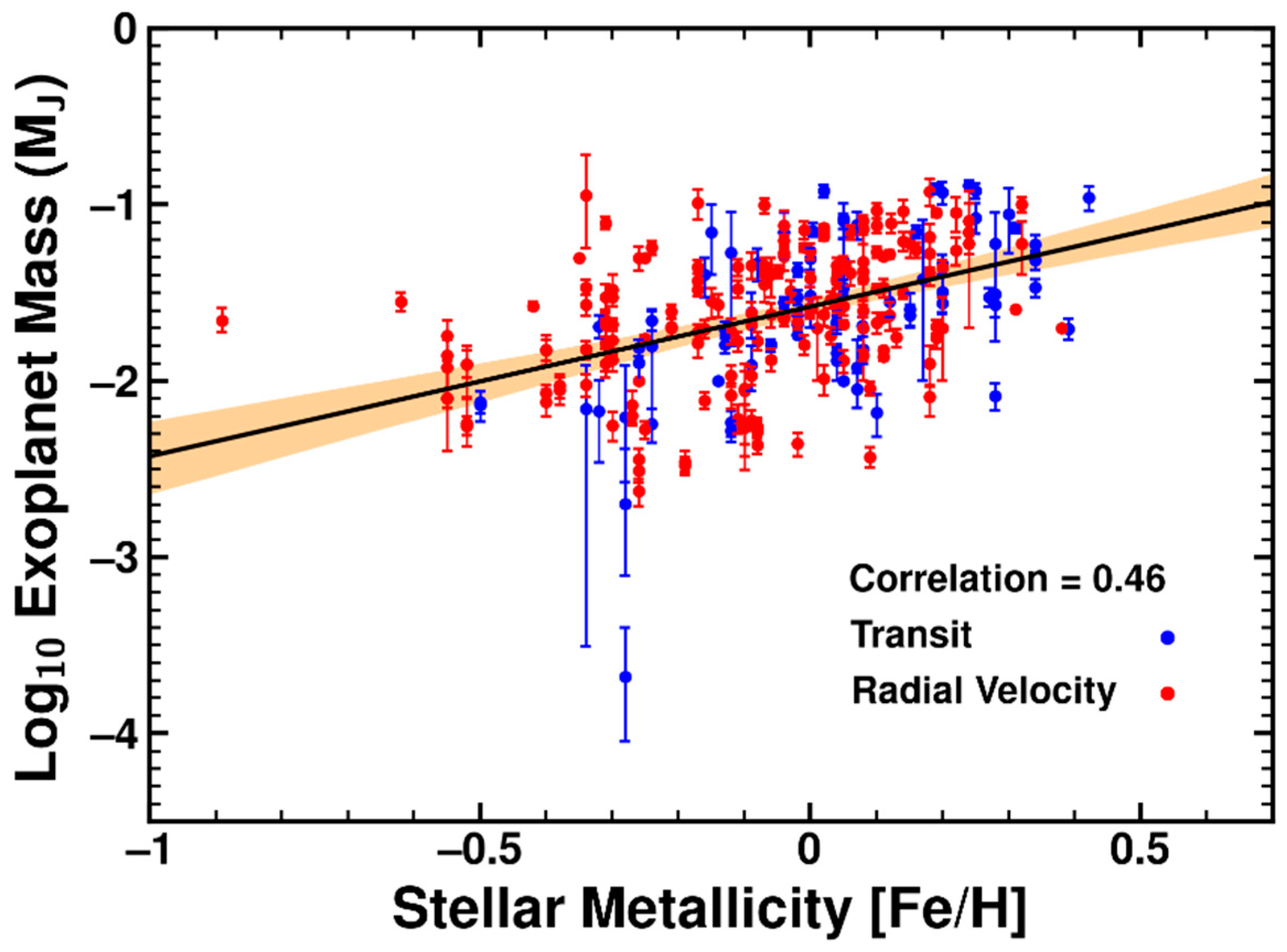
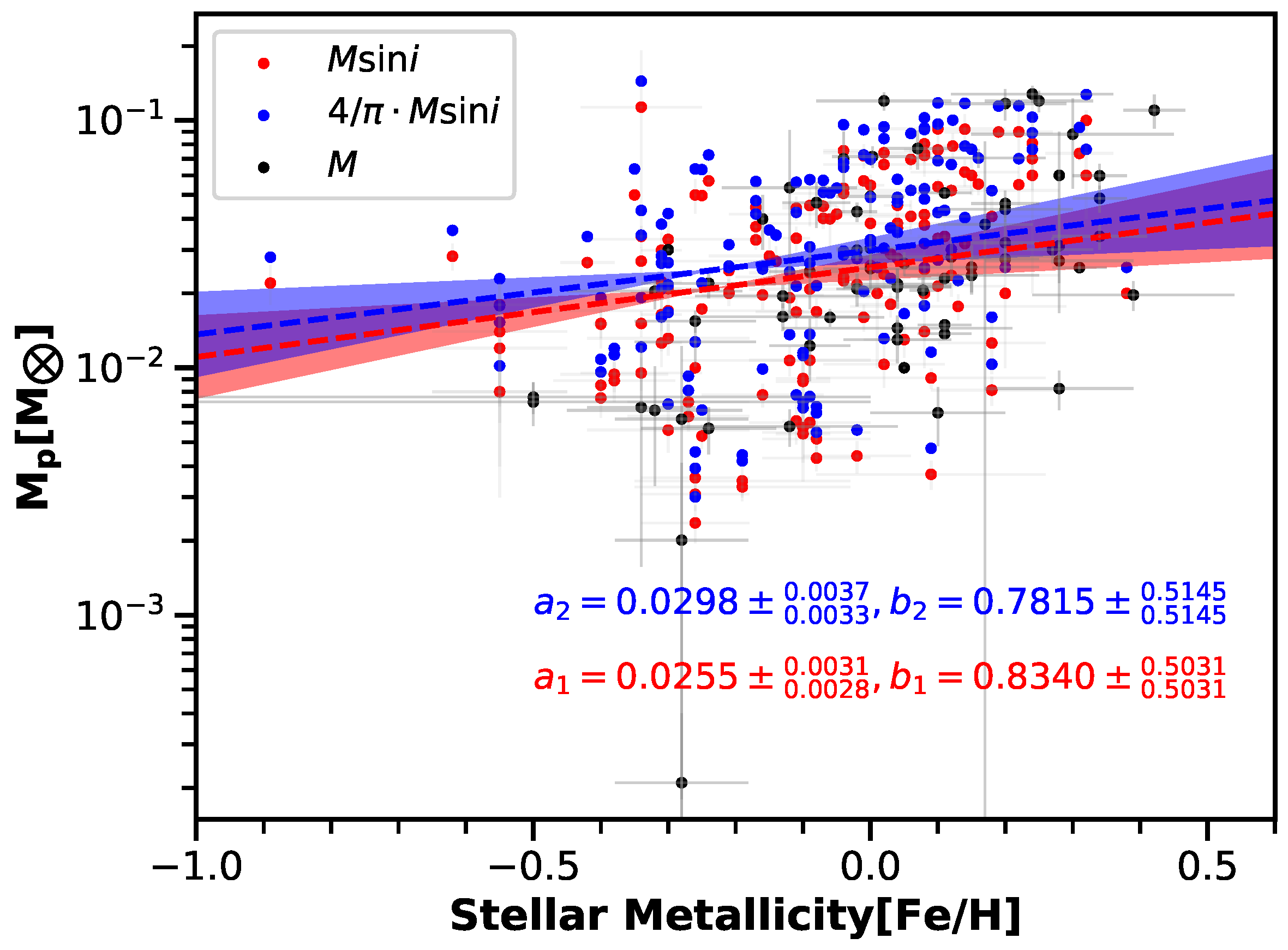
3.1. The Planet Mass–Stellar Metallicity Relation
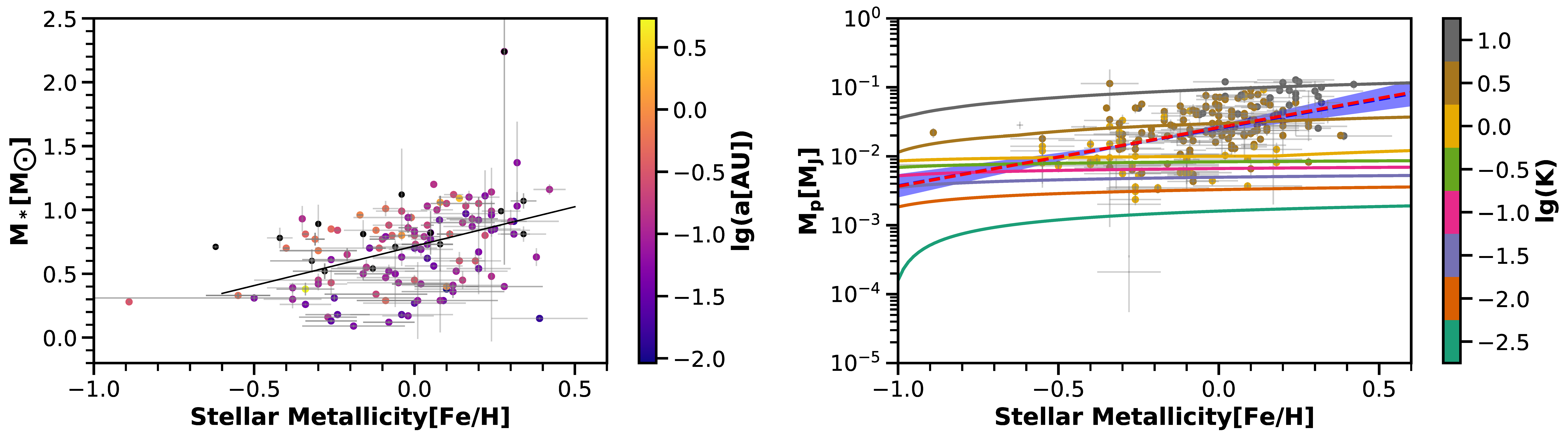
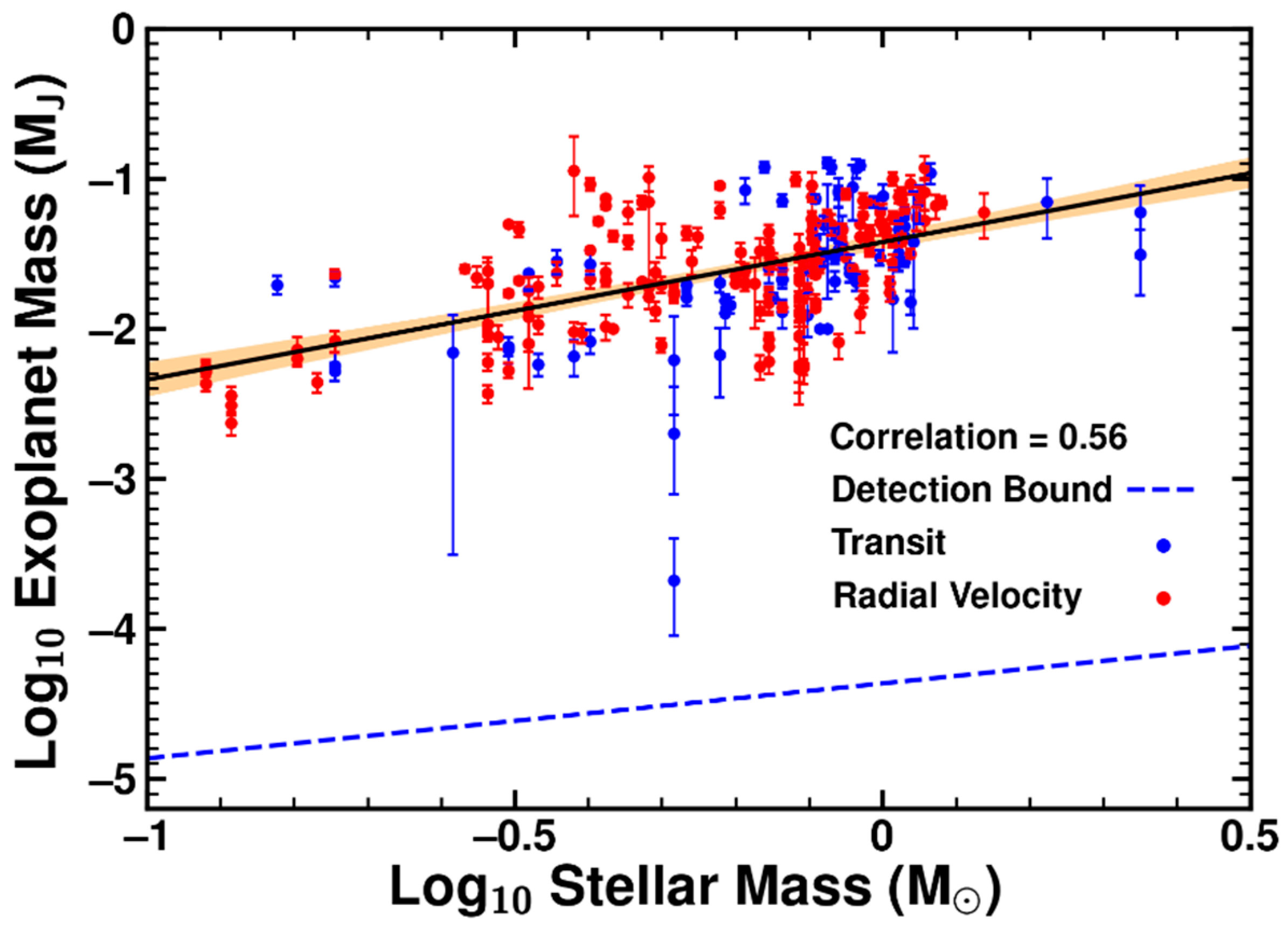
3.2. The Planet Mass–Stellar Mass Relation
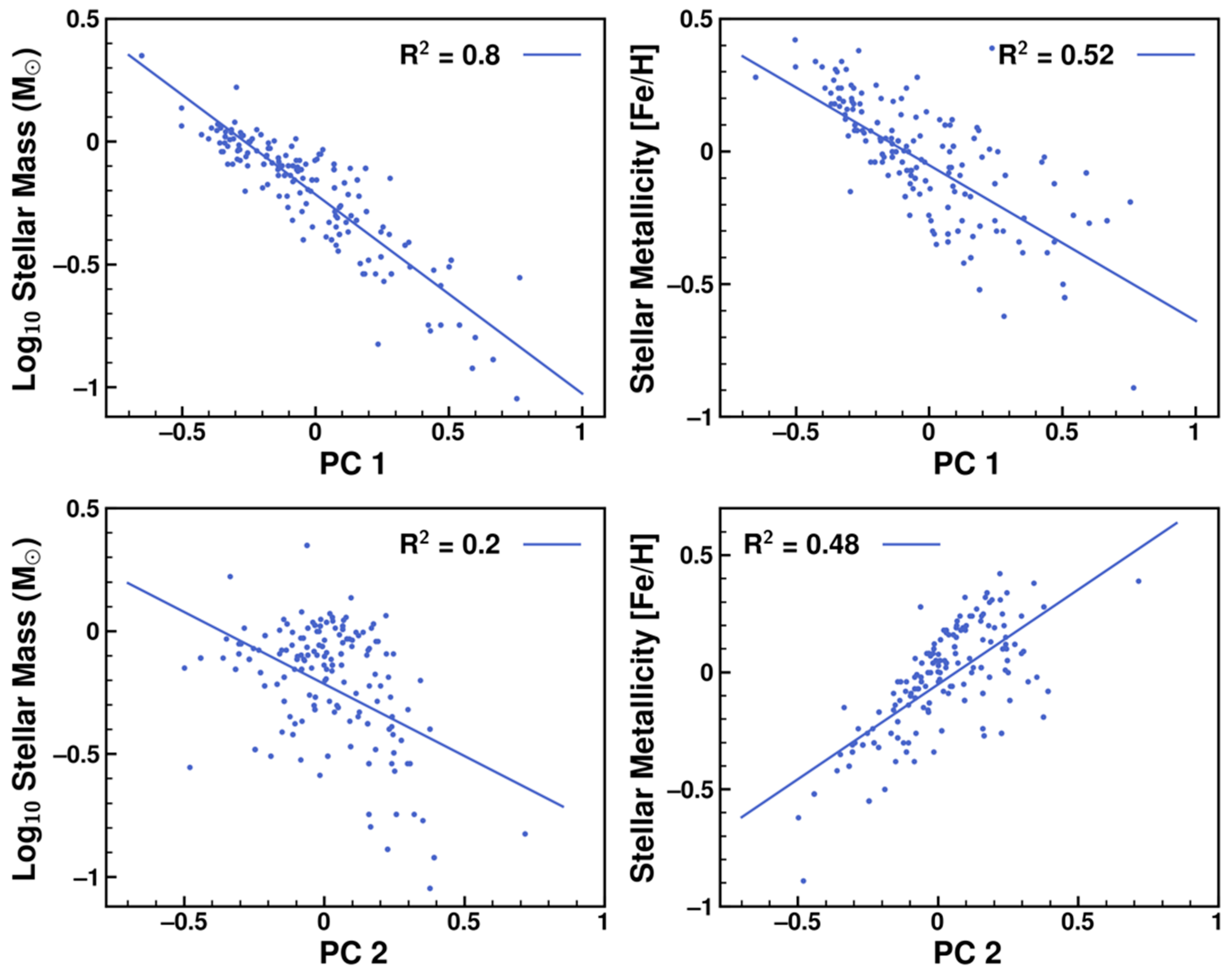
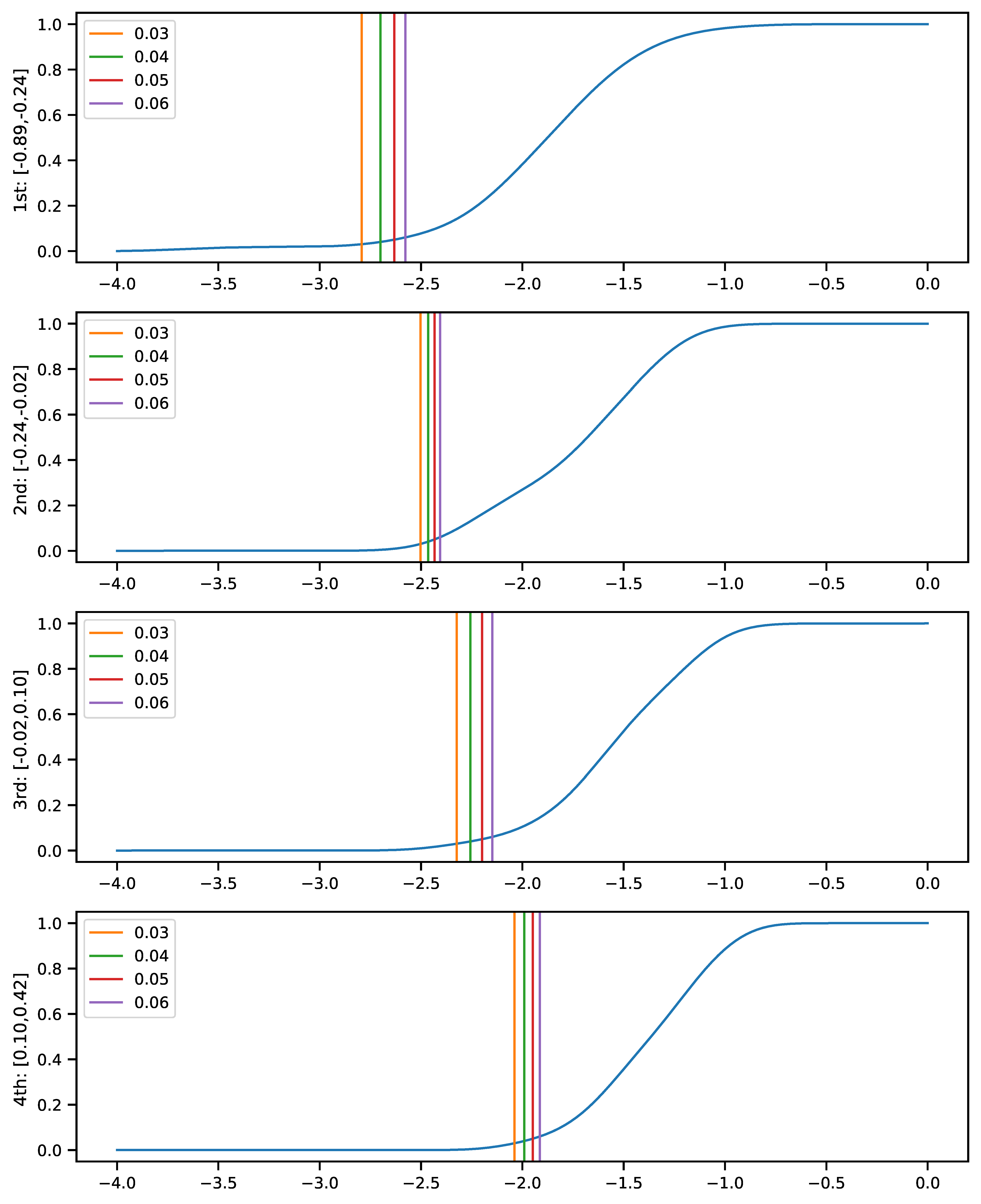
3.3. Uncertainty Analysis
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Sousa, S.G.; Adibekyan, V.; Santos, N.C.; Mortier, A.; Barros, S.C.C.; Delgado-Mena, E.; Demangeon, O.; Israelian, G.; Faria, J.P.; Figueira, P.; et al. The metallicity–period–mass diagram of low-mass exoplanets. Mon. Not. R. Astron. Soc. 2019, 485, 3981–3990. [Google Scholar] [CrossRef]
- Santos, N.C.; Israelian, G.; Mayor, M. Spectroscopic [Fe/H] for 98 extra-solar planet-host stars. Astron. Astrophys. 2004, 415, 1153–1166. [Google Scholar] [CrossRef]
- Hansen, J.T.; Casagrande, L.; Ireland, M.J.; Lin, J. Confirming known planetary trends using a photometrically selected Kepler sample. Mon. Not. R. Astron. Soc. 2020, 501, 5309–5318. [Google Scholar] [CrossRef]
- Zhu, W. Influence of Stellar Metallicity on Occurrence Rates of Planets and Planetary Systems. Astrophys. J. 2019, 873. [Google Scholar] [CrossRef]
- Santos, N.C. Confirming the Metal-Rich Nature of Stars with Giant Planets. arXiv 2001, arXiv:astro-ph/0109018v1. [Google Scholar]
- Pollack, J.B.; Hubickyj, O.; Bodenheimer, P.; Lissauer, J.J.; Podolak, M.; Greenzweig, Y. Formation of the Giant Planets by Concurrent Accretion of Solids and Gas. ICARUS 1996, 124, 62–85. [Google Scholar] [CrossRef]
- Mordanisi, C. Extrasolar planet population synthesis -IV. Correlations with disk metallicity, mass, and lifetime. Astron. Astrophys. 2012, 541, A97. [Google Scholar] [CrossRef]
- Thorngren, D.P.; Fortney, J.J.; Murray-Clay, R.A.; Lopez, E.D. The Mass–Metallicity Relation for Giant Planets. Astrophys. J. 2016, 831, 64. [Google Scholar] [CrossRef]
- Udry, S.; Santos, N.C. Statistical Properties of Exoplanets. Annu. Rev. Astron. Astrophys. 2007, 45, 397–439. [Google Scholar] [CrossRef]
- Ghezzi, L.; Cunha, K.; Smith, V.V.; De Araújo, F.X.; Schuler, S.C.; De La Reza, R. Stellar Parameters and Metallicities of Stars Hosting Jovian and Neptunian Mass Planets: A Possible Dependence of Planetary Mass on Metallicity. Astrophys. J. 2010, 720, 1290–1302. [Google Scholar] [CrossRef]
- Wu, Y. Mass and mass scalings of super-Earths. Astrophys. J. 2019, 874, 91. [Google Scholar] [CrossRef]
- Fulton, B.J.; Petigura, E.A. The California-Kepler Survey. VII. Precise Planet Radii Leveraging Gaia DR2 Reveal the Stellar Mass Dependence of the Planet Radius Gap. Astron. J. 2018, 156, 264. [Google Scholar] [CrossRef]
- Berger, T.A.; Huber, D.; Gaidos, E.; Van Saders, J.L.; Weiss, L.M. The Gaia–Kepler Stellar Properties Catalog. II. Planet Radius Demographics as a Function of Stellar Mass and Age. Astron. J. 2020, 160, 108. [Google Scholar] [CrossRef]
- Plavchan, P. Radial Velocity Prospects Current and Future: A White Paper Report prepared by the Study Analysis Group 8 for the Exoplanet Program Analysis Group (ExoPAG). arXiv 2015, arXiv:1503.01770. [Google Scholar]
- Fasano, G.; Franceschini, A. A multidimensional version of the Kolmogorov–Smirnov test. Mon. Not. R. Astron. Soc. 1987, 225, 155–170. [Google Scholar] [CrossRef]
- Paddock, G.F. The Derivation of the Radial Velocity Equation. Publ. Astron. Soc. Pac. 1913, 25, 208. [Google Scholar] [CrossRef]
- Leethochawalit, N.; Kirby, E.N.; Moran, S.M.; Ellis, R.S.; Treu, T. Evolution of the Stellar Mass–Metallicity Relation. I. Galaxies in thez∼ 0.4 Cluster Cl0024. Astrophys. J. 2018, 856. [Google Scholar] [CrossRef]
- Yates, R.M.; Kauffmann, G.; Guo, Q. The relation between metallicity, stellar mass and star formation in galaxies: An analysis of observational and model data. Mon. Not. R. Astron. Soc. 2012, 422, 215–231. [Google Scholar] [CrossRef]
- Lovis, C.; Fischer, D. Radial Velocity Techniques for Exoplanets; University of Arizona Press: Tucson, AZ, USA, 2010. [Google Scholar]
- Courcol, B.; Bouchy, F.; Deleuil, M. An upper boundary in the mass-metallicity plane of exo-Neptunes. Mon. Not. R. Astron. Soc. 2016, 461, 1841–1849. [Google Scholar] [CrossRef]
- Lammer, H.; Stökl, A.; Erkaev, N.V.; Dorfi, E.A.; Odert, P.; Güdel, M.; Kulikov, Y.N.; Kislyakova, K.G.; Leitzinger, M. Origin and loss of nebula-captured hydrogen envelopes from ‘sub’- to ‘super-Earths’ in the habitable zone of Sun-like stars. Mon. Not. R. Astron. Soc. 2014, 439, 3225–3238. [Google Scholar] [CrossRef]
- Armitage, P.J. A Brief Overview of Planet Formation. In Handbook of Exoplanets; Deeg, H., Belmonte, J., Eds.; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Andrews, S.M.; Rosenfeld, K.A.; Kraus, A.L.; Wilner, D.J. The mass dependence between protoplanetary disks and their stellar hosts. Astrophys. J. 2013, 771. [Google Scholar] [CrossRef]
- Mulders, G.D. Planet Populations as a Function of Stellar Properties. In Handbook of Exoplanets; Springer: Cham, Switzerland, 2018. [Google Scholar]
- Montmerle, T.; Augereau, J.-C.; Chaussidon, M.; Gounelle, M.; Marty, B.; Morbidelli, A. Solar System Formation and Early Evolution: The First 100 Million Years. In From Suns to Life: A Chronological Approach to the History of Life on Earth; Springer: Cham, Switzerland, 2007. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, J.H.; Zhao, D.; Ji, X.; Xie, B.; Fahy, K.A. Revisiting the Planet Mass and Stellar Metallicity Relation for Low-Mass Exoplanets Orbiting GKM Class Stars. Universe 2021, 7, 88. https://doi.org/10.3390/universe7040088
Jiang JH, Zhao D, Ji X, Xie B, Fahy KA. Revisiting the Planet Mass and Stellar Metallicity Relation for Low-Mass Exoplanets Orbiting GKM Class Stars. Universe. 2021; 7(4):88. https://doi.org/10.3390/universe7040088
Chicago/Turabian StyleJiang, Jonathan H., Daniel Zhao, Xuan Ji, Bohan Xie, and Kristen A. Fahy. 2021. "Revisiting the Planet Mass and Stellar Metallicity Relation for Low-Mass Exoplanets Orbiting GKM Class Stars" Universe 7, no. 4: 88. https://doi.org/10.3390/universe7040088
APA StyleJiang, J. H., Zhao, D., Ji, X., Xie, B., & Fahy, K. A. (2021). Revisiting the Planet Mass and Stellar Metallicity Relation for Low-Mass Exoplanets Orbiting GKM Class Stars. Universe, 7(4), 88. https://doi.org/10.3390/universe7040088





