The Low-Temperature Expansion of the Casimir-Polder Free Energy of an Atom with Graphene
Abstract
:1. Introduction
2. The Casimir-Polder Free Energy
2.1. Expansion of the
2.2. Expansion of the
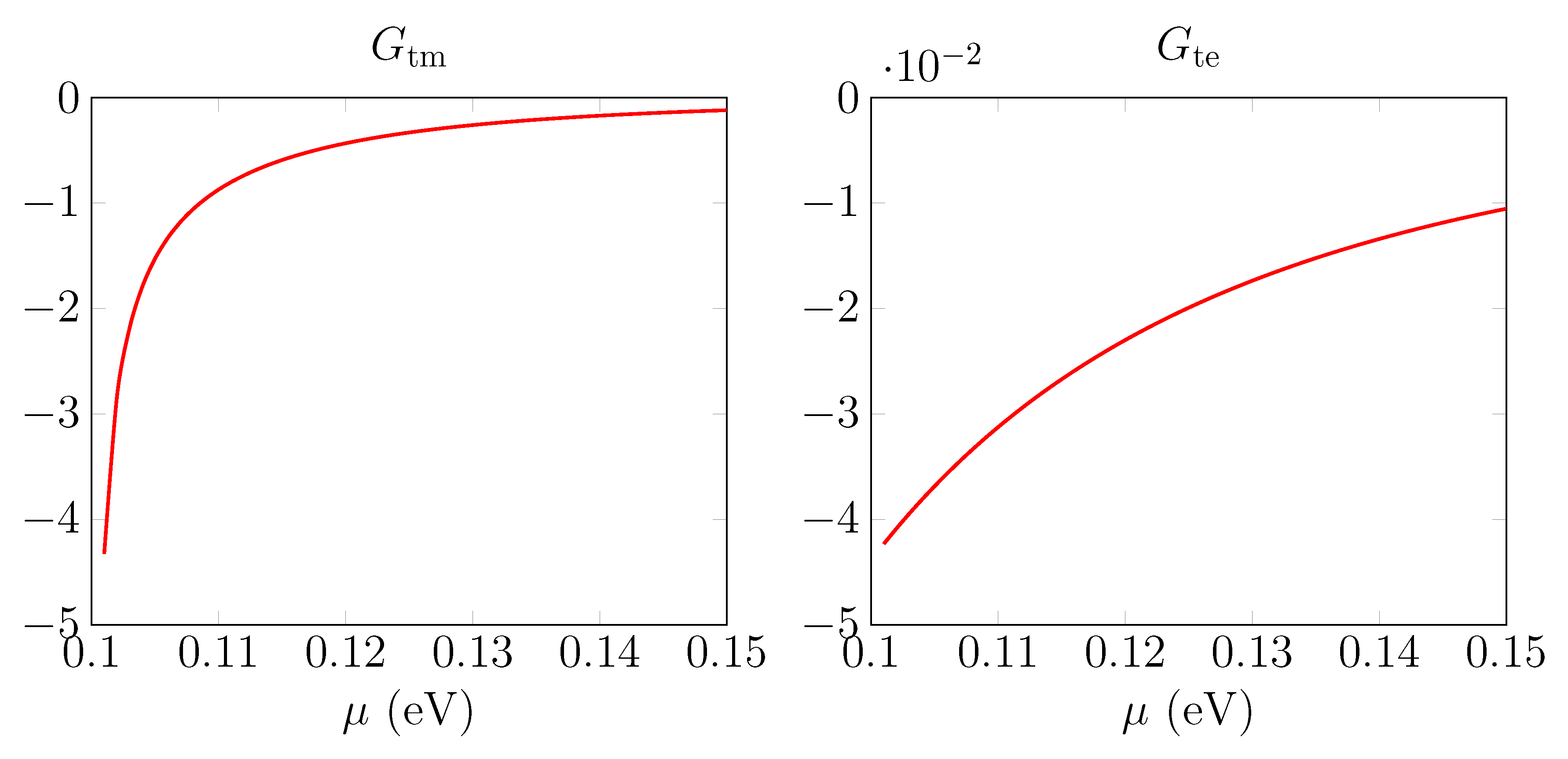
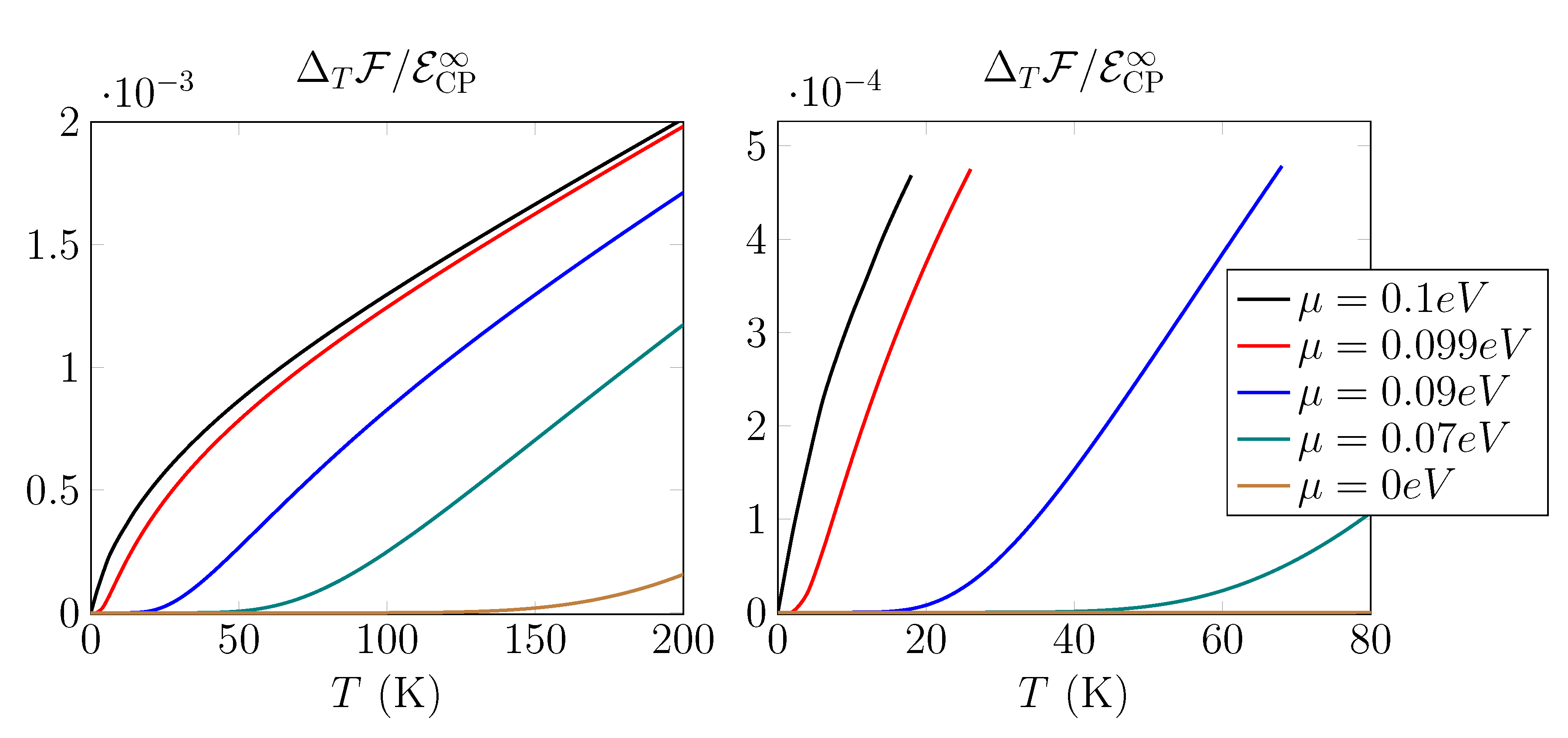
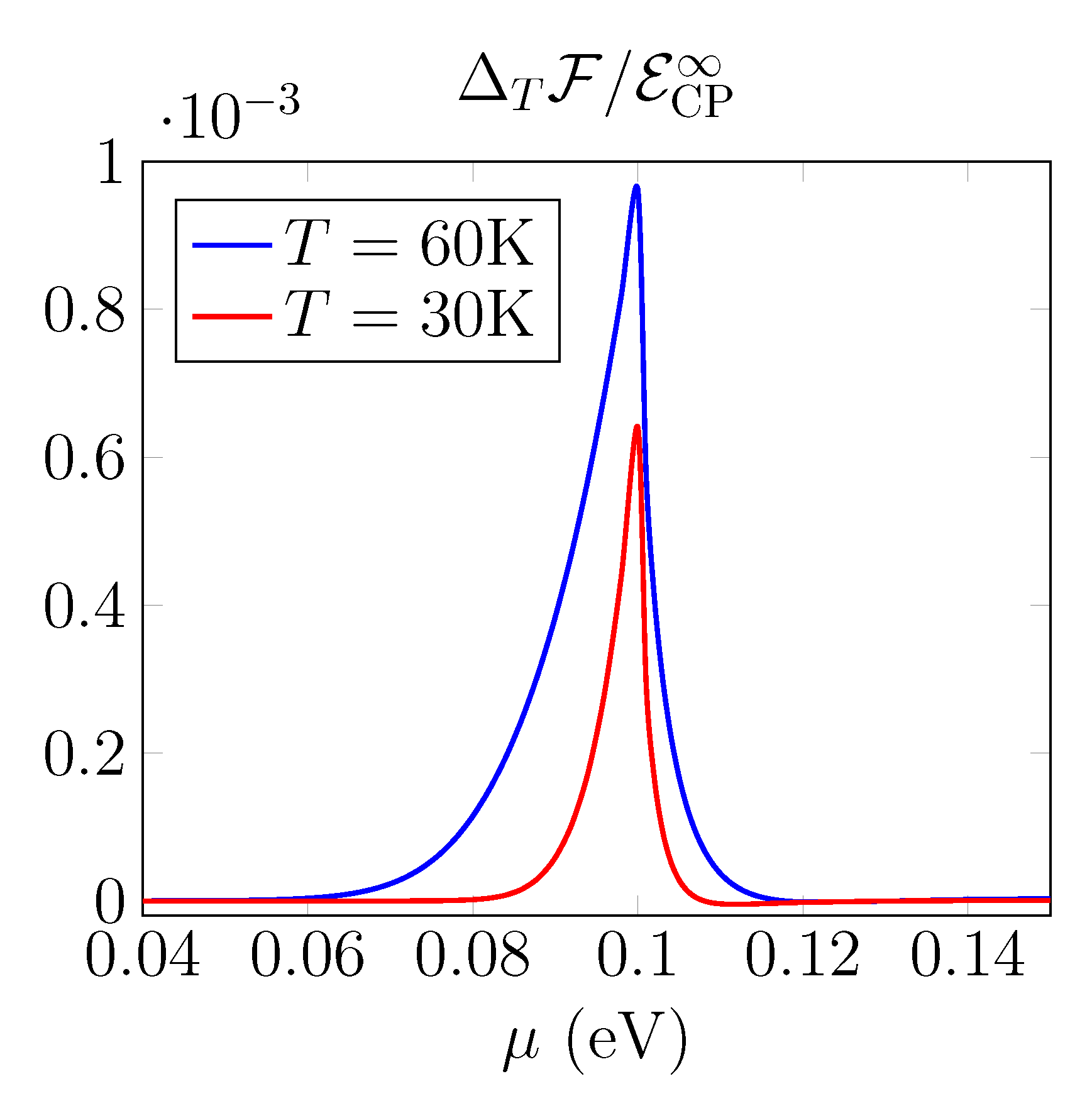
3. Numerical Evaluations
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Casimir, H.B.G. On the attraction between two perfectly conducting plates. Kon. Ned. Akad. Wetensch. Proc. 1948, 51, 793–795. [Google Scholar]
- Casimir, H.B.G.; Polder, D. The influence of retardation on the London-van der Waals forces. Phys. Rev. 1948, 73, 360–372. [Google Scholar] [CrossRef]
- Parsegian, A.V. Van der Waals Forces. A Handbook for Biologists, Chemists, Engineers, and Physicists; Cambridge University Press: Cambridge, UK, 2006; p. 380. [Google Scholar]
- Bordag, M.; Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. Advances in the Casimir Effect; Cambridge University Press: Cambridge, UK, 2009; pp. 1–768. [Google Scholar] [CrossRef]
- Bondarev, I.V.; Lambin, P. Van der Waals coupling in atomically doped carbon nanotubes. Phys. Rev. B 2005, 72, 35451. [Google Scholar] [CrossRef] [Green Version]
- Blagov, E.V.; Klimchitskaya, G.L.; Mostepanenko, V.M. Van der Waals interaction between a microparticle and a single-wall carbon nanotube. Phys. Rev. B 2007, 75, 235413. [Google Scholar] [CrossRef] [Green Version]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Casimir and Casimir-Polder Forces in Graphene Systems: Quantum Field Theoretical Description and Thermodynamics. Universe 2020, 6, 150. [Google Scholar] [CrossRef]
- Khusnutdinov, N.; Kashapov, R.; Woods, L.M. Casimir-Polder effect for a stack of conductive planes. Phys. Rev. A 2016, 94, 12513. [Google Scholar] [CrossRef] [Green Version]
- Khusnutdinov, N.; Woods, L.M. Casimir Effects in 2D Dirac Materials (Mini-review). JETP Lett. 2019, 110, 1–10. [Google Scholar] [CrossRef]
- Babb, J.F. Long-range atom-surface interactions for cold atoms. J. Phys. Conf. Ser. 2005, 19, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Marachevsky, V.N.; Pis’mak, Y.M. Casimir-Polder effect for a plane with Chern-Simons interaction. Phys. Rev. D 2010, 81, 65005. [Google Scholar] [CrossRef] [Green Version]
- Shajesh, K.V.; Schaden, M. Repulsive long-range forces between anisotropic atoms and dielectrics. Phys. Rev. A 2012, 85, 012523. [Google Scholar] [CrossRef] [Green Version]
- Thiyam, P.; Parashar, P.; Shajesh, K.V.; Persson, C.; Schaden, M.; Brevik, I.; Parsons, D.F.; Milton, K.A.; Malyi, O.I.; Boström, M. Anisotropic contribution to the van der Waals and the Casimir-Polder energies for CO2 and CH4 molecules near surfaces and thin films. Phys. Rev. A 2015, 92, 052704. [Google Scholar] [CrossRef] [Green Version]
- Antezza, M.; Fialkovsky, I.; Khusnutdinov, N. Casimir-Polder force and torque for anisotropic molecules close to conducting planes and their effect on CO2. Phys. Rev. B 2020, 102, 195422. [Google Scholar] [CrossRef]
- Obrecht, J.M.; Wild, R.J.; Antezza, M.; Pitaevskii, L.P.; Stringari, S.; Cornell, E.A. Measurement of the Temperature Dependence of the Casimir-Polder Force. Phys. Rev. Lett. 2007, 98, 063201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Laliotis, A.; de Silans, T.P.; Maurin, I.; Ducloy, M.; Bloch, D. Casimir-Polder interactions in the presence of thermally excited surface modes. Nat. Commun. 2014, 5. [Google Scholar] [CrossRef] [Green Version]
- Wylie, J.M.; Sipe, J.E. Quantum electrodynamics near an interface. II. Phys. Rev. A 1985, 32, 2030–2043. [Google Scholar] [CrossRef] [PubMed]
- Buhmann, S.; Welsch, D. Dispersion forces in macroscopic quantum electrodynamics. Prog. Quantum Electron. 2007, 31, 51–130. [Google Scholar] [CrossRef] [Green Version]
- Bezerra, V.B.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Romero, C. Lifshitz theory of atom-wall interaction with applications to quantum reflection. Phys. Rev. A 2008, 78, 042901. [Google Scholar] [CrossRef] [Green Version]
- Chaichian, M.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Tureanu, A. Thermal Casimir-Polder interaction of different atoms with graphene. Phys. Rev. A 2012, 86, 12515. [Google Scholar] [CrossRef] [Green Version]
- Bordag, M. Low Temperature Expansion in the Lifshitz Formula. Adv. Math. Phys. 2014, 2014, 1–34. [Google Scholar] [CrossRef]
- Khusnutdinov, N.; Kashapov, R.; Woods, L.M. Thermal Casimir and Casimir–Polder interactions in N parallel 2D Dirac materials. 2D Mater. 2018, 5, 35032. [Google Scholar] [CrossRef] [Green Version]
- Khusnutdinov, N.; Emelianova, N. Low-temperature expansion of the Casimir-Polder free energy for an atom interacting with a conductive plane. Int. J. Mod. Phys. A 2019, 34, 1950008. [Google Scholar] [CrossRef] [Green Version]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Nernst heat theorem for an atom interacting with graphene: Dirac model with nonzero energy gap and chemical potential. Phys. Rev. D 2020, 101, 116003. [Google Scholar] [CrossRef]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Quantum field theoretical description of the Casimir effect between two real graphene sheets and thermodynamics. Phys. Rev. D 2020, 102, 016006. [Google Scholar] [CrossRef]
- Bordag, M.; Fialkovsky, I.V.; Gitman, D.M.; Vassilevich, D.V. Casimir interaction between a perfect conductor and graphene described by the Dirac model. Phys. Rev. B 2009, 80, 245406. [Google Scholar] [CrossRef] [Green Version]
- Fialkovsky, I.V.; Marachevsky, V.N.; Vassilevich, D.V. Finite-temperature Casimir effect for graphene. Phys. Rev. B 2011, 84, 35446. [Google Scholar] [CrossRef] [Green Version]
- Bordag, M.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Petrov, V.M. Quantum field theoretical description for the reflectivity of graphene. Phys. Rev. D 2015, 91, 045037. [Google Scholar] [CrossRef] [Green Version]
- Bordag, M.; Fialkovskiy, I.; Vassilevich, D. Enhanced Casimir effect for doped graphene. Phys. Rev. B 2016, 93, 075414, Erratum in Phys. Rev. B 2017, 95, 119905. doi:10.1103/physrevb.95.119905. [Google Scholar] [CrossRef] [Green Version]
- Falkovsky, L.A.; Varlamov, A.A. Space-time dispersion of graphene conductivity. Eur. Phys. J. B 2007, 56, 281–284. [Google Scholar] [CrossRef] [Green Version]
- Gusynin, V.P.; Sharapov, S.G.; Carbotte, J.P. Magneto-optical conductivity in Graphene. J. Phys. Condens. Matter 2007, 19, 26222. [Google Scholar] [CrossRef] [Green Version]
- Fedoryuk, M.V. The Saddle-Point Method; Nauka: Moscow, Russia, 1977. (In Russian) [Google Scholar]
- Khusnutdinov, N.R. The thermal Casimir–Polder interaction of an atom with a spherical plasma shell. J. Phys. A Math. Theor. 2012, 45, 265301. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Milton, K.; Parashar, P.; Hong, L. Negativity of the Casimir Self-Entropy in Spherical Geometries. Entropy 2021, 23, 214. [Google Scholar] [CrossRef] [PubMed]
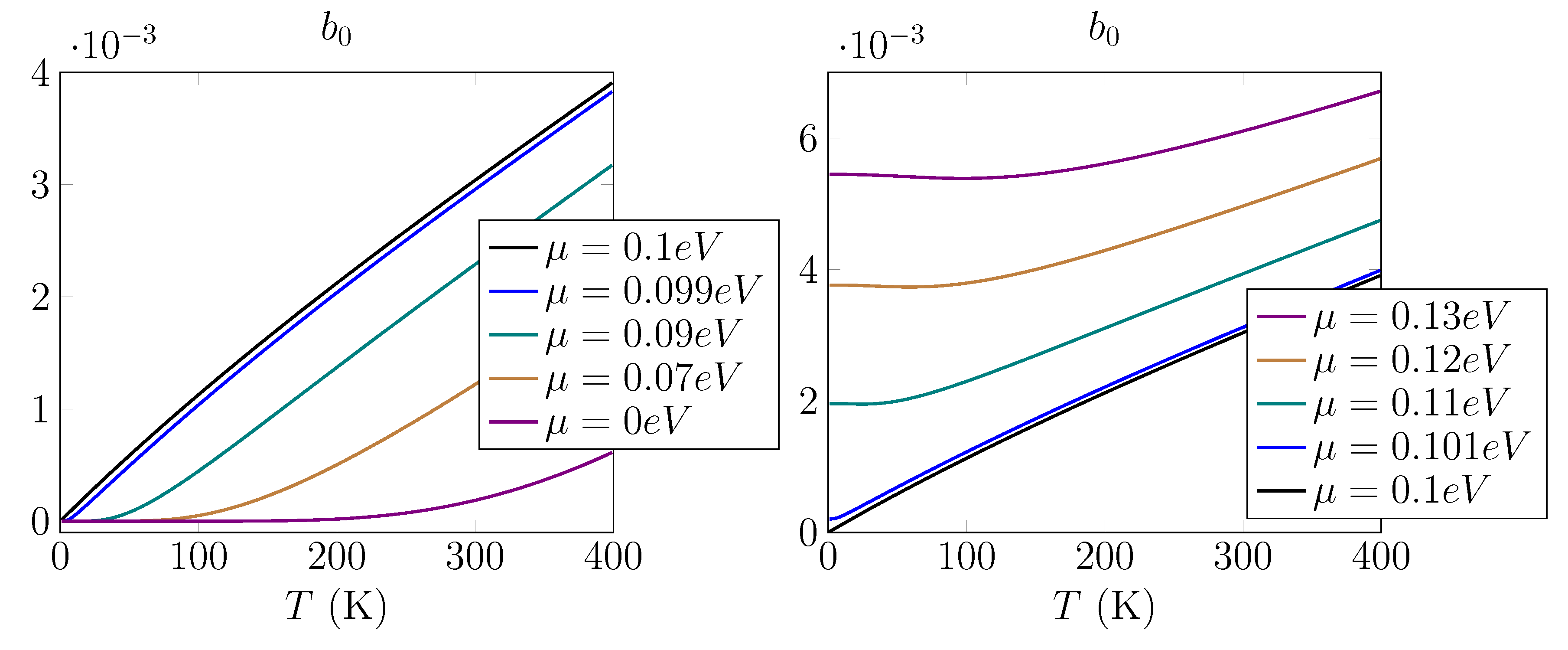


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khusnutdinov, N.; Emelianova, N. The Low-Temperature Expansion of the Casimir-Polder Free Energy of an Atom with Graphene. Universe 2021, 7, 70. https://doi.org/10.3390/universe7030070
Khusnutdinov N, Emelianova N. The Low-Temperature Expansion of the Casimir-Polder Free Energy of an Atom with Graphene. Universe. 2021; 7(3):70. https://doi.org/10.3390/universe7030070
Chicago/Turabian StyleKhusnutdinov, Nail, and Natalia Emelianova. 2021. "The Low-Temperature Expansion of the Casimir-Polder Free Energy of an Atom with Graphene" Universe 7, no. 3: 70. https://doi.org/10.3390/universe7030070
APA StyleKhusnutdinov, N., & Emelianova, N. (2021). The Low-Temperature Expansion of the Casimir-Polder Free Energy of an Atom with Graphene. Universe, 7(3), 70. https://doi.org/10.3390/universe7030070





