Torsion in String-Inspired Cosmologies and the Universe Dark Sector
Abstract
:1. Introduction: A Case for Going beyond GR and Riemannian Geometry
2. Review of the Running Vacuum Model (RVM) Framework
3. Review of the String-Inspired Gravitational Effective Theory
3.1. String-Inspired Effective Gravitational Action with Torsion
3.2. Connection with Torsional Topological Invariants and Axions
3.3. Gravitational Anomalies, Axions, and the Role of the Cotton Tensor
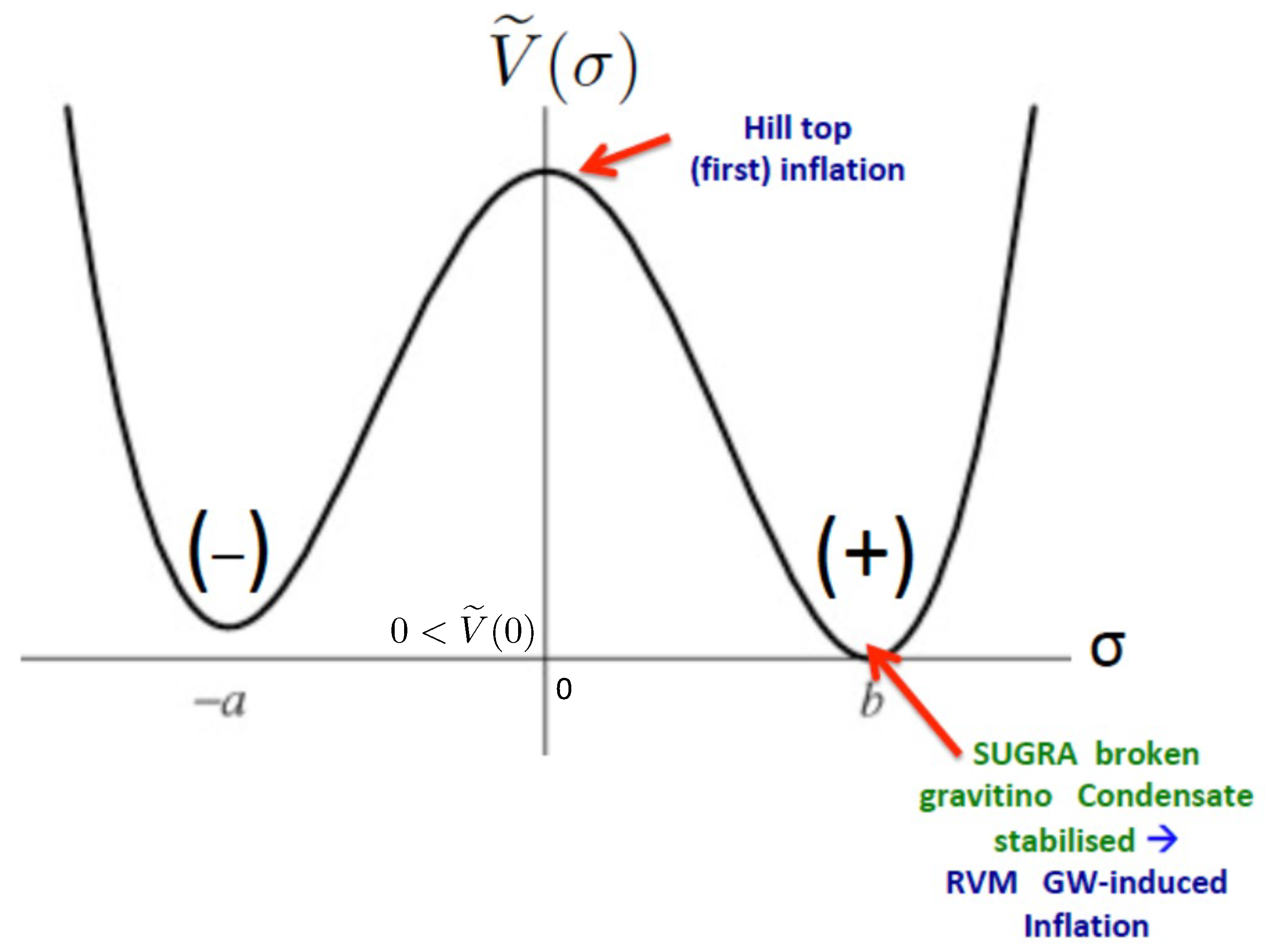
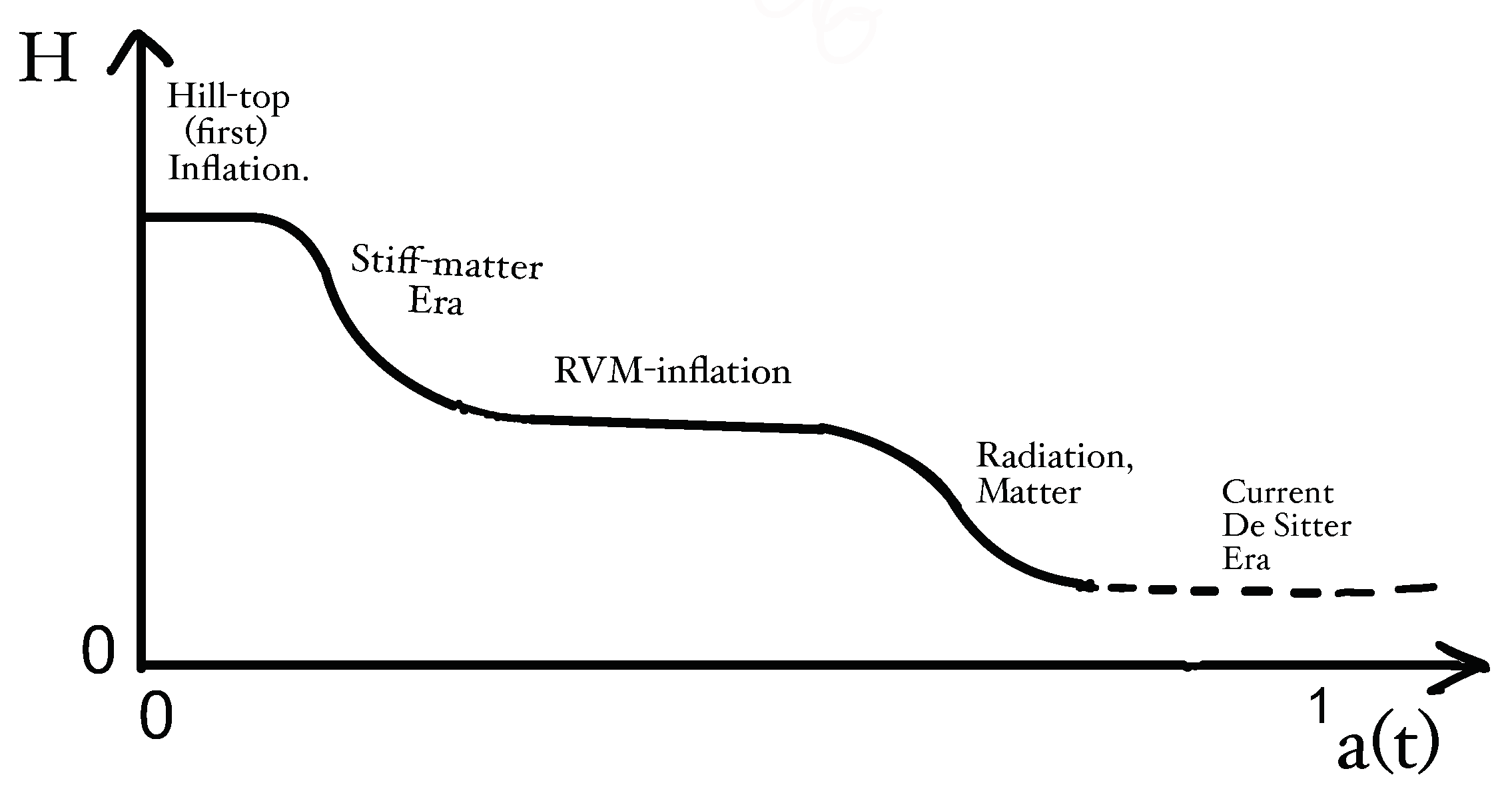
4. Primordial Gravitational Waves, Anomalies, and an RVM-like Inflation without Inflatons
4.1. On the Origin of Primordial Gravitational Waves
4.2. Gravitational-Wave Condensation, Gravitational Chern–Simons Terms, and RVM Inflation
4.3. On Potential Primordial Black Hole Effects on GW during RVM Inflation
5. Post-Inflationary Era, Kalb–Ramond Axions as Dark Matter and Leptogenesis
5.1. KR Axion Mass Generation and Dark Matter
5.2. Leptogenesis
6. Modern Era: RVM-like Deviations from CDM and Alleviation of Cosmological Data Tensions
6.1. Highlights of the RVM Phenomenology in the Current Era
6.2. Modified Stringy RVM Due to Quantum-Gravity Corrections?
6.3. Brief Comparison of the Stringy-RVM with Other Theories in Contorted Geometries
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Torsion Formalism in an Instructive Example: Quantum Electrodynamics in a Contorted Geometry
- (i)
- Additional terms of interaction of the fermions (F) with the derivative of the BI field :with the axial current (61), and:the vector current.
- (ii)
- Interaction terms of fermions with non-derivative terms (a different fermionic action, using non-minimal coupling of fermions with , has been proposed in [117] as a way to resolve an inconsistency of the Holst action, when coupled to fermions, in the case of constant . In that proposal, the factor in (A41) below, is replaced by the Dirac-self-conjugate quantity . The decomposition of the torsion into its irreducible components in the presence of the Holst action with an arbitrary (constant) BI parameter, leads to an inconsistency, implying that the vector component of the torsion is proportional to the axial fermion current, and hence this does not transform properly under improper Lorentz transformations. With the aforementioned modification of the fermion action, the problem is solved, as demonstrated in [117], upon choosing , which eliminates the vector component of the torsion. However this inconsistency is valid only if is considered as a constant. Promotion of the BI parameter to a pseudoscalar field, , resolves this issue, as discussed in [171], given that one obtains in that case consistent results, in the sense that the vector component of the torsion transforms correctly under parity, as a vector, since it contains now, apart from terms proportional to the vector fermionic current (A40), also terms proportional to the product of the BI pseudoscalar with the axial fermionic current (61), as well as terms of the form , all transforming properly as vectors under improper Lorentz transformations):
References
- Iorio, L. Editorial for the Special Issue 100 Years of Chronogeometrodynamics: The Status of the Einstein’s Theory of Gravitation in Its Centennial Year. Universe 2015, 1, 38–81. [Google Scholar] [CrossRef] [Green Version]
- Will, C.M. The Confrontation between General Relativity and Experiment. Living Rev. Rel. 2014, 4, 17. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Perlmutter, S. Measurements of Ω and Λ from 42 high redshift supernovae. Astrophys. J. 1999, 517, 565–586. [Google Scholar] [CrossRef]
- Riess, A.G. Observational evidence from supernovae for an accelerating universe and a cosmological constant. Astron. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef] [Green Version]
- Aghanim, N. Planck 2018 results. VI. Cosmological parameters Astron. Astrophys. 2020, 641, A6. [Google Scholar]
- Milgrom, M. A Modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. Astrophys. J. 1983, 270, 365–370. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Relativistic gravitation theory for the MOND paradigm. Phys. Rev. D 2004, 70, 083509. [Google Scholar] [CrossRef]
- Harvey, D.; Massey, R.; Kitching, T.; Taylor, A.; Tittley, E. The non-gravitational interactions of dark matter in colliding galaxy clusters. Science 2015, 347, 1462–1465. [Google Scholar] [CrossRef] [Green Version]
- Abbott, B.P. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef] [PubMed]
- Akiyama, K. First M87 Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole. Astrophys. J. 2019, 875, L1. [Google Scholar]
- Psaltis, D. Gravitational Test Beyond the First Post-Newtonian Order with the Shadow of the M87 Black Hole. Phys. Rev. Lett. 2020, 125, 141104. [Google Scholar] [CrossRef] [PubMed]
- Kanti, P.; Mavromatos, N.E.; Rizos, J.; Tamvakis, K.; Winstanley, E. Dilatonic black holes in higher curvature string gravity. Phys. Rev. D 1996, 54, 5049–5058. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zumalacárregui, M.; García-Bellido, J. Transforming gravity: From derivative couplings to matter to second-order scalar-tensor theories beyond the Horndeski Lagrangian. Phys. Rev. D 2014, 89, 064046. [Google Scholar] [CrossRef] [Green Version]
- Gleyzes, J.; Langlois, D.; Piazza, F.; Vernizzi, F. Exploring gravitational theories beyond Horndeski. JCAP 2015, 2, 18. [Google Scholar] [CrossRef] [Green Version]
- Yunes, N.; Pretorius, F. Dynamical Chern–Simons Modified Gravity. I. Spinning Black Holes in the Slow-Rotation Approximation. Phys. Rev. D 2009, 79, 084043. [Google Scholar] [CrossRef] [Green Version]
- Ayzenberg, D.; Yunes, N. Slowly-Rotating Black Holes in Einstein-Dilaton-Gauss-Bonnet Gravity: Quadratic Order in Spin Solutions. Phys. Rev. D 2014, 90, 044066. [Google Scholar] [CrossRef] [Green Version]
- Sotiriou, T.P.; Zhou, S.Y. Black hole hair in generalized scalar-tensor gravity. Phys. Rev. Lett. 2014, 112, 251102. [Google Scholar] [CrossRef] [Green Version]
- Sotiriou, T.P.; Zhou, S.Y. Black hole hair in generalized scalar-tensor gravity: An explicit example. Phys. Rev. D 2014, 90, 124063. [Google Scholar] [CrossRef] [Green Version]
- Sotiriou, T.P.; Faraoni, V. Black holes in scalar-tensor gravity. Phys. Rev. Lett. 2012, 108, 081103. [Google Scholar] [CrossRef] [Green Version]
- Jackiw, R.; Pi, S.Y. Chern–Simons modification of general relativity. Phys. Rev. D 2003, 68, 104012. [Google Scholar] [CrossRef] [Green Version]
- Alexander, S.; Yune, N.S. Chern–Simons Modified General Relativity. Phys. Rept. 2009, 480, 1–55. [Google Scholar] [CrossRef] [Green Version]
- Kiefer, C. Quantum gravity. Int. Ser. Monogr. Phys. 2004, 124, 1–308. [Google Scholar]
- Verlinde, E.P. On the Origin of Gravity and the Laws of Newton. JHEP 2011, 4, 29. [Google Scholar] [CrossRef] [Green Version]
- Niedermaier, M.; Reuter, M. The Asymptotic Safety Scenario in Quantum Gravity. Living Rev. Rel. 2006, 9, 5–173. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Green, M.B.; Schwarz, J.H.; Witten, E. Superstring Theory Vols. 1 and 2: 25th Anniversary Edition; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar] [CrossRef]
- Polchinski, J. String Theory. Vol. 1: An Introduction to the Bosonic String; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Susskind, L. The Anthropic landscape of string theory. arXiv 2003, arXiv:hep-th/0302219. [Google Scholar]
- Rovelli, C. Loop quantum gravity. Living Rev. Rel. 1998, 1, 1. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Lewandowski, J. Background independent quantum gravity: A Status report. Class. Quant. Grav. 2004, 21, R53. [Google Scholar] [CrossRef]
- Bojowald, M. Loop quantum cosmology. Living Rev. Rel. 2005, 8, 11. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ashtekar, A.; Singh, P. Loop Quantum Cosmology: A Status Report. Class. Quant. Grav. 2011, 28, 213001. [Google Scholar] [CrossRef] [Green Version]
- Oriti, D. Space-time geometry from algebra: Spin foam models for nonperturbative quantum gravity. Rept. Prog. Phys. 2001, 64, 1703–1756. [Google Scholar] [CrossRef]
- Perez, A. The Spin Foam Approach to Quantum Gravity. Living Rev. Rel. 2013, 16, 3. [Google Scholar] [CrossRef] [Green Version]
- Oriti, D. The Group field theory approach to quantum gravity. arXiv 2006, arXiv:gr-qc/0607032. [Google Scholar]
- Bahr, B.; Dittrich, B.; Ryan, J.P. Spin foam models with finite groups. J. Grav. 2013, 2013, 549824. [Google Scholar] [CrossRef] [Green Version]
- Riess, A.G.; Macri, L.M.; Hoffmann, S.L.; Scolnic, D.; Casertano, S.; Filippenko, A.V.; Tucker, B.E.; Reid, M.J.; Jones, D.O.; Silverman, J.M. A 2.4% Determination of the Local Value of the Hubble Constant. Astrophys. J. 2016, 826, 56. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.; Bucciarelli, B.; Lattanzi, M.G.; MacKenty, J.W.; Bowers, J.B.; Zheng, W.; Filippenko, A.V. Milky Way Cepheid Standards for Measuring Cosmic Distances and Application to Gaia DR2: Implications for the Hubble Constant. Astrophys. J. 2018, 861, 126. [Google Scholar] [CrossRef]
- Bonvin, V.; Courbin, F.; Suyu, S.H.; Marshall, P.J.; Rusu, C.E.; Sluse, D.; Tewes, M.; Wong, K.C.; Collett, T.; Fassnacht, C.D. H0LiCOW–V. New COSMOGRAIL time delays of HE 0435−1223: H0 to 3.8 per cent precision from strong lensing in a flat ΛCDM model. Mon. Not. Roy. Astron. Soc. 2017, 465, 4914–4930. [Google Scholar] [CrossRef] [Green Version]
- Macaulay, E.; Wehus, I.K.; Eriksen, H.K. Lower Growth Rate from Recent Redshift Space Distortion Measurements than Expected from Planck. Phys. Rev. Lett. 2013, 111, 161301. [Google Scholar] [CrossRef] [PubMed]
- Nesseris, S.; Pantazis, G.; Perivolaropoulos, L. Tension and constraints on modified gravity parametrizations of Geff(z) from growth rate and Planck data. Phys. Rev. D 2017, 96, 023542. [Google Scholar] [CrossRef] [Green Version]
- Valentino, E.D.; Anchordoqui, L.A.; Akarsu, O.; Ali-Haimoud, Y.; Amendola, L.; Arendse, N.; Asgari, M.; Ballardini, M.; Basilakos, S.; Battistelli, E. Cosmology Intertwined III: fσ8 and S8. arXiv 2008, arXiv:2008.11285. [Google Scholar] [CrossRef]
- Valentino, E.D.; Mena, O.; Pan, S.; Visinelli, L.; Yang, W.; Melchiorri, A.; Mota, D.F.; Riess, A.G.; Silk, J. In the realm of the Hubble tension—A review of solutions. Class. Quant. Grav. 2021, 38, 153001. [Google Scholar] [CrossRef]
- Perivolaropoulos, L.; Skara, F. Challenges for ΛCDM: An update. arXiv 2021, arXiv:2105.05208. [Google Scholar]
- Shapiro, I.L.; Solà, J. On the scaling behavior of the cosmological constant and the possible existence of new forces and new light degrees of freedom. Phys. Lett. B 2000, 475, 236–246. [Google Scholar] [CrossRef] [Green Version]
- Shapiro, I.L.; Solà, J. Cosmological constant, renormalization group and Planck scale physics. Nucl. Phys. B Proc. Suppl. 2004, 127, 71–76. [Google Scholar] [CrossRef] [Green Version]
- Solà, J. Cosmological constant and vacuum energy: Old and new ideas. J. Phys. Conf. Ser. 2013, 453, 012015. [Google Scholar] [CrossRef]
- Lima, J.A.S.; Basilakos, S.; Solà, J. Expansion History with Decaying Vacuum: A Complete Cosmological Scenario. Mon. Not. Roy. Astron. Soc. 2013, 431, 923–929. [Google Scholar] [CrossRef] [Green Version]
- Perico, E.L.D.; Lima, J.A.S.; Basilakos, S.; Solà, J. Complete Cosmic History with a dynamical Λ = Λ(H) term. Phys. Rev. D 2013, 88, 063531. [Google Scholar] [CrossRef] [Green Version]
- Basilakos, S.; Lima, J.A.S.; Solà, J. From inflation to dark energy through a dynamical Lambda: An attempt at alleviating fundamental cosmic puzzles. Int. J. Mod. Phys. D 2013, 22, 1342008. [Google Scholar] [CrossRef] [Green Version]
- Solà Peracaula, J.; Yu, H. Particle and entropy production in the Running Vacuum Universe. Gen. Rel. Grav. 2020, 52, 17. [Google Scholar] [CrossRef] [Green Version]
- Gómez-Valent, A.; Solà, J.; Basilakos, S. Dynamical vacuum energy in the expanding Universe confronted with observations: A dedicated study. JCAP 2015, 1, 4. [Google Scholar] [CrossRef] [Green Version]
- Solà, J.; Gómez-Valent, A.; Pérez, J.D. First evidence of running cosmic vacuum: Challenging the concordance model. Astrophys. J. 2017, 836, 43. [Google Scholar] [CrossRef] [Green Version]
- Solà, J.; Gómez-Valent, A.; Pérez, J.D. The H0 tension in light of vacuum dynamics in the Universe. Phys. Lett. B 2017, 774, 317–324. [Google Scholar] [CrossRef]
- Gomez-Valent, A.; Solà, J. Relaxing the σ8-tension through running vacuum in the Universe. EPL 2017, 120, 39001. [Google Scholar] [CrossRef] [Green Version]
- Solà Peracaula, J. Tensions in the ΛCDM and vacuum dynamics. Int. J. Mod. Phys. A 2018, 33, 1844009. [Google Scholar] [CrossRef]
- Tsiapi, P.; Basilakos, S. Testing dynamical vacuum models with CMB power spectrum from Planck. Mon. Not. Roy. Astron. Soc. 2019, 485, 2505–2510. [Google Scholar] [CrossRef] [Green Version]
- Papagiannopoulos, G.; Tsiapi, P.; Basilakos, S.; Paliathanasis, A. Dynamics and cosmological evolution in Λ-varying cosmology. Eur. Phys. J. C 2020, 80, 55. [Google Scholar] [CrossRef] [Green Version]
- Solà Peracaula, J.; Gómez-Valent, A.; Perez, J.D.; Moreno-Pulido, C. Running vacuum against the H0 and σ8 tensions. EPL 2021, 134, 19001. [Google Scholar] [CrossRef]
- Solà Peracaula, J. Running vacuum interacting with dark matter or with running gravitational coupling. Phenomenological implications. arXiv 2021, arXiv:2109.12086. [Google Scholar]
- Anagnostopoulos, F.K.; Benisty, D.; Basilakos, S.; Guendelman, E.I. Dark energy and dark matter unification from dynamical space time: Observational constraints and cosmological implications. JCAP 2019, 6, 003. [Google Scholar] [CrossRef] [Green Version]
- Banerjee, S.; Benisty, D.; Guendelman, E.I. Running Dark Energy and Dark Matter from Dynamical Spacetime. Bulg. J. Phys. 2021, 48, 117–137. [Google Scholar]
- Basilakos, S.; Mavromatos, N.E.; Solà Peracaula, J. Gravitational and Chiral Anomalies in the Running Vacuum Universe and Matter-Antimatter Asymmetry. Phys. Rev. D 2020, 101, 045001. [Google Scholar] [CrossRef] [Green Version]
- Mavromatos, N.E.; Solà Peracaula, J. Inflationary physics and transplanckian conjecture in the Stringy Running-Vacuum-Model: From the phantom vacuum to the true vacuum. arXiv 2021, arXiv:2105.02659. [Google Scholar]
- Mavromatos, N.E.; Solà Peracaula, J. Stringy-running-vacuum-model inflation: From primordial gravitational waves and stiff axion matter to dynamical dark energy. Eur. Phys. J. ST 2021, 230, 2077–2110. [Google Scholar] [CrossRef]
- Basilakos, S.; Mavromatos, N.E.; Solà Peracaula, J. Quantum Anomalies in String-Inspired Running Vacuum Universe: Inflation and Axion Dark Matter. Phys. Lett. B 2020, 803, 135342. [Google Scholar] [CrossRef]
- Hehl, F.W.; Heyde, P.V.D.; Kerlick, G.D.; Nester, J.M. General Relativity with Spin and Torsion: Foundations and Prospects. Rev. Mod. Phys. 1976, 48, 393. [Google Scholar] [CrossRef] [Green Version]
- Duncan, M.J.; Kaloper, N.; Olive, K.A. Axion hair and dynamical torsion from anomalies. Nucl. Phys. B 1992, 387, 215. [Google Scholar] [CrossRef]
- Svrcek, P.; Witten, E. Axions In String Theory. JHEP 2006, 6, 051. [Google Scholar] [CrossRef] [Green Version]
- Elizalde, E.; Odintsov, S.D.; Paul, T.; Gómez, D.S. Inflationary universe in F(R) gravity with antisymmetric tensor fields and their suppression during its evolution. Phys. Rev. D 2019, 063506. [Google Scholar] [CrossRef] [Green Version]
- Paul, T.; Banerjee, N. Cosmological quantum entanglement: A possible testbed for the existence of Kalb–Ramond field. Class. Quant. Grav. 2020, 37, 135013. [Google Scholar] [CrossRef]
- Paul, T. Antisymmetric tensor fields in modified gravity: A summary. Symmetry 2020, 12, 1573. [Google Scholar] [CrossRef]
- Elizalde, E.; Odintsov, S.D.; Oikonomou, V.K.; Paul, T. Logarithmic-corrected R2 Gravity Inflation in the Presence of Kalb-Ramond Fields. JCAP 2019, 2, 17. [Google Scholar] [CrossRef] [Green Version]
- Chakraborty, S.; SenGupta, S. Solutions on a brane in a bulk spacetime with Kalb-Ramond field. Ann. Phys. 2016, 367, 258–279. [Google Scholar] [CrossRef] [Green Version]
- Chakraborty, S.; Pal, S.; SenGupta, S. Inflationary Magnetogenesis and Anomaly Cancellation in Electrodynamics. arXiv 2018, arXiv:1810.03478. [Google Scholar]
- Barbero, J.F. Real Ashtekar variables for Lorentzian signature space times. Phys. Rev. D 1995, 51, 5507–5510. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Immirzi, G. Quantum gravity and Regge calculus. Nucl. Phys. B Proc. Suppl. 1997, 57, 65–72. [Google Scholar] [CrossRef] [Green Version]
- De Berredo-Peixoto, G.; Freidel, L.; Shapiro, I.L.; Souza, C.A.D. Dirac fields, torsion and Barbero-Immirzi parameter in Cosmology. JCAP 2012, 1206, 17. [Google Scholar] [CrossRef]
- Asante, S.K.; Dittrich, B.; Padua-Arguelles, J. Effective spin foam models for Lorentzian quantum gravity. Class. Quant. Grav. 2021, 38, 195002. [Google Scholar] [CrossRef]
- Shapiro, I.L. Physical aspects of the space-time torsion. Phys. Rept. 2002, 357, 113. [Google Scholar] [CrossRef] [Green Version]
- De Andrade, V.C.; Pereira, J.G. Torsion and the electromagnetic field. Int. J. Mod. Phys. D 1999, 8, 141–151. [Google Scholar] [CrossRef]
- Puetzfeld, D. Status of non-Riemannian cosmology. New Astron. Rev. 2005, 49, 59–64. [Google Scholar] [CrossRef] [Green Version]
- Magueijo, J.; Zlosnik, T.G.; Kibble, T.W.B. Cosmology with a spin. Phys. Rev. D 2013, 87, 063504. [Google Scholar] [CrossRef] [Green Version]
- Capozziello, S.; Cianci, R.; C, C.S.; Vignolo, S. f(R) cosmology with torsion. Phys. Scr. 2008, 78, 065010. [Google Scholar] [CrossRef]
- Poplawski, N.J. Matter-antimatter asymmetry and dark matter from torsion. Phys. Rev. D 2011, 83, 084033. [Google Scholar] [CrossRef] [Green Version]
- Poplawski, N.J. Four-fermion interaction from torsion as dark energy. Gen. Rel. Grav. 2012, 44, 491–499. [Google Scholar] [CrossRef] [Green Version]
- Cai, Y.F.; Laurentis, S.C.S.M.D.; Saridakis, E.N. f(T) teleparallel gravity and cosmology. Rept. Prog. Phys. 2016, 79, 106901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bahamonde, S.; Dialektopoulos, K.F.; Escamilla-Rivera, C.; Farrugia, G.; Gakis, V.; Hendry, M.; Hohmann, M.; Said, J.L.; Mifsud, J.; Valentino, E.D. Teleparallel Gravity: From Theory to Cosmology. arXiv 2021, arXiv:2106.13793. [Google Scholar]
- Jiménez, J.B.; Heisenberg, L.; Koivisto, T. Coincident General Relativity. Phys. Rev. D 2018, 98, 044048. [Google Scholar] [CrossRef] [Green Version]
- Heisenberg, L. A systematic approach to generalisations of General Relativity and their cosmological implications. Phys. Rept. 2019, 796, 1–113. [Google Scholar] [CrossRef] [Green Version]
- Jiménez, J.B.; Heisenberg, L.; Koivisto, T.S. The Geometrical Trinity of Gravity. Universe 2019, 5, 173. [Google Scholar] [CrossRef] [Green Version]
- De Andrade, L.C.G. A left-chiral Goedel-like teleparallel universe with gravitational anomalies and dynamo action with torsional sources. Eur. Phys. J. Plus 2021, 136, 956. [Google Scholar] [CrossRef]
- De Andrade, L.C.G. Cosmological and astrophysical consequences from the magnetic dynamo equation in torsioned spacetime and teleparallel gravity. Class. Quant. Grav. 2016, 33, 025006. [Google Scholar] [CrossRef]
- D’Ambrosio, F.; Heisenberg, L.; Kuhn, S. Revisiting Cosmologies in Teleparallelism. arXiv 2021, arXiv:2109.04209. [Google Scholar]
- D’Ambrosio, F.; Fell, S.D.B.; Heisenberg, L.; Kuhn, S. Black holes in f(ℚ) Gravity. arXiv 2021, arXiv:2109.03174. [Google Scholar]
- Witten, E. Gravitational Anomalies. Nucl. Phys. B 1984, 234, 269. [Google Scholar]
- Alexander, S.H.S.; Peskin, M.E.; Sheikh-Jabbari, M.M. Leptogenesis from gravity waves in models of inflation. Phys. Rev. Lett. 2006, 96, 081301. [Google Scholar] [CrossRef] [Green Version]
- De Cesare, M.; Mavromatos, N.E.; Sarkar, S. On the possibility of tree-level leptogenesis from Kalb–Ramond torsion background. Eur. Phys. J. C 2015, 75, 514. [Google Scholar] [CrossRef]
- Bossingham, T.; Mavromatos, N.E.; Sarkar, S. Leptogenesis from Heavy Right-Handed Neutrinos in CPT Violating Backgrounds. Eur. Phys. J. C 2018, 78, 113. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bossingham, T.; Mavromatos, N.E.; Sarkar, S. The role of temperature dependent string-inspired CPT violating backgrounds in leptogenesis and the chiral magnetic effect. Eur. Phys. J. C 2019, 79, 50. [Google Scholar] [CrossRef]
- Mavromatos, N.E.; Sarkar, S. Spontaneous CPT Violation and Quantum Anomalies in a Model for Matter–Antimatter Asymmetry in the Cosmos. Universe 2018, 5, 5. [Google Scholar] [CrossRef] [Green Version]
- Mavromatos, N.E.; Sarkar, S. Curvature and thermal corrections in tree-level CPT-Violating Leptogenesis. Eur. Phys. J. C 2020, 80, 558. [Google Scholar] [CrossRef]
- Moreno-Pulido, C.; Sola, J. Running vacuum in quantum field theory in curved spacetime: Renormalizing ρvac without ∼m4 terms. Eur. Phys. J. C 2020, 80, 692. [Google Scholar] [CrossRef]
- Moreno-Pulido, C.; Sola, J. Renormalized ρvac without m4 terms. arXiv 2021, arXiv:2110.08070. [Google Scholar]
- Metsaev, R.R.; Tseytlin, A.A. Order alpha-prime (Two Loop) Equivalence of the String Equations of Motion and the Sigma Model Weyl Invariance Conditions: Dependence on the Dilaton and the Antisymmetric Tensor. Nucl. Phys. B 1987, 293, 385–419. [Google Scholar] [CrossRef]
- Gross, D.J.; Sloan, J.H. The Quartic Effective Action for the Heterotic String. Nucl. Phys. B 1987, 291, 41–89. [Google Scholar] [CrossRef]
- Bento, M.C.; Mavromatos, N.E. Ambiguities in the Low-energy Effective Actions of String Theories With the Inclusion of Antisymmetric Tensor and Dilaton Fields. Phys. Lett. B 1987, 190, 105–109. [Google Scholar] [CrossRef]
- Benisty, D.; Guendelman, E.I.; Venn, A.v.; Vasak, D.; Struckmeier, J.; Stoecker, H. The Dark side of the torsion: Dark Energy from kinetic torsion. arXiv 2021, arXiv:2109.01052. [Google Scholar]
- Antoniadis, I.; Bachas, C.; Ellis, J.R.; Nanopoulos, D.V. Cosmological String Theories and Discrete Inflation. Phys. Lett. B 1988, 211, 393–399. [Google Scholar] [CrossRef] [Green Version]
- Ellis, J.R.; Mavromatos, N.E.; Nanopoulos, D.V.; Westmuckett, M. Liouville cosmology at zero and finite temperatures. Int. J. Mod. Phys. A 2006, 21, 1379–1444. [Google Scholar] [CrossRef] [Green Version]
- Kallosh, R.E.; Tyutin, I.V. The Equivalence theorem and gauge invariance in renormalizable theories. Yad. Fiz. 1973, 17, 190–209. [Google Scholar]
- Bergere, M.C.; Lam, Y.M.P. Equivalence Theorem and Faddeev-Popov Ghosts. Phys. Rev. D 1976, 13, 3247–3255. [Google Scholar] [CrossRef]
- Nieh, H.T.; Yan, M.L. An Identity in Riemann-cartan Geometry. J. Math. Phys. 1982, 23, 373. [Google Scholar] [CrossRef]
- Nieh, H.T.; Yang, C.N. A torsional topological invariant. Int. J. Mod. Phys. A 2007, 22, 5237–5244. [Google Scholar] [CrossRef]
- Nieh, H.T. Torsional Topological Invariants. Phys. Rev. D 2018, 98, 104045. [Google Scholar] [CrossRef] [Green Version]
- Chandia, O.; Zanelli, J. Topological invariants, instantons and chiral anomaly on spaces with torsion. Phys. Rev. D 1997, 55, 7580. [Google Scholar] [CrossRef] [Green Version]
- Banerjee, K. Some Aspects of Holst and Nieh-Yan Terms in General Relativity with Torsion. Class. Quant. Grav. 2010, 27, 135012. [Google Scholar] [CrossRef] [Green Version]
- Mercuri, S. Fermions in Ashtekar-Barbero connections formalism for arbitrary values of the Immirzi parameter. Phys. Rev. D 2006, 73, 084016. [Google Scholar] [CrossRef] [Green Version]
- Mercuri, S. From the Einstein-Cartan to the Ashtekar-Barbero canonical constraints, passing through the Nieh-Yan functional. Phys. Rev. D 2008, 77, 024036. [Google Scholar] [CrossRef] [Green Version]
- Castellani, L.; D’Auria, R.; Frè, P. Supergravity and Superstrings—A Geometric Perspective; World Scientific: Singapore, 1991; Volume 1, pp. 1–603. [Google Scholar]
- Taveras, V.; Yunes, N. The Barbero-Immirzi Parameter as a Scalar Field: K-Inflation from Loop Quantum Gravity? Phys. Rev. D 2008, 78, 064070. [Google Scholar] [CrossRef] [Green Version]
- Calcagni, G.; Mercuri, S. The Barbero-Immirzi field in canonical formalism of pure gravity. Phys. Rev. D 2009, 79, 084004. [Google Scholar] [CrossRef] [Green Version]
- Lattanzi, M.; Mercuri, S. A solution of the strong CP problem via the Peccei-Quinn mechanism through the Nieh-Yan modified gravity and cosmological implications. Phys. Rev. D 2010, 81, 125015. [Google Scholar] [CrossRef] [Green Version]
- Mavromatos, N.E. Geometrical origins of the Universe dark sector: String-inspired torsion and anomalies as seeds for inflation and dark matter. arXiv 2021, arXiv:2108.02152. [Google Scholar]
- Arvanitaki, A.; Dimopoulos, S.; Dubovsky, S.; Kaloper, N.; March-Russell, J. String Axiverse. Phys. Rev. D 2010, 81, 123530. [Google Scholar] [CrossRef] [Green Version]
- Marsh, D.J.E. Axion Cosmology. Phys. Rept. 2016, 643, 1–79. [Google Scholar] [CrossRef] [Green Version]
- Mavromatos, N.E.; Mitsou, V.A.; Sarkar, S.; Vergou, A. Implications of a Stochastic Microscopic Finsler Cosmology. Eur. Phys. J. C 2012, 72, 1956. [Google Scholar] [CrossRef]
- Ellis, J.R.; Mavromatos, N.E.; Westmuckett, M. Potentials between D-branes in a supersymmetric model of space-time foam. Phys. Rev. D 2005, 71, 106006. [Google Scholar] [CrossRef] [Green Version]
- Ellis, J.R.; Mavromatos, N.E.; Westmuckett, M. A Supersymmetric D-brane model of space-time foam. Phys. Rev. D 2004, 70, 044036. [Google Scholar] [CrossRef] [Green Version]
- Alexandre, J.; Houston, N.; Mavromatos, N.E. Dynamical Supergravity Breaking via the Super-Higgs Effect Revisited. Phys. Rev. D 2013, 88, 125017. [Google Scholar] [CrossRef] [Green Version]
- Alexandre, J.; Houston, N.; Mavromatos, N.E. Inflation via Gravitino Condensation in Dynamically Broken Supergravity. Int. J. Mod. Phys. D 2015, 24, 1541004. [Google Scholar] [CrossRef] [Green Version]
- Lalak, Z.; Lola, S.; Ovrut, B.A.; Ross, G.G. Large scale structure from biased nonequilibrium phase transitions: Percolation theory picture. Nucl. Phys. B 1995, 434, 675–696. [Google Scholar] [CrossRef] [Green Version]
- Lalak, D.C.D.Z.; Ovrut, B.A. Biased domain walls. Phys. Rev. D 1996, 53, 4237–4246. [Google Scholar]
- Ellis, J.; Mavromatos, N.E. Inflation induced by gravitino condensation in supergravity. Phys. Rev. D 2013, 88, 085029. [Google Scholar] [CrossRef] [Green Version]
- Antoniadis, I.; Rizos, J.; Tamvakis, K. Singularity - free cosmological solutions of the superstring effective action. Nucl. Phys. B 1994, 415, 497. [Google Scholar] [CrossRef] [Green Version]
- Bedroya, A.; Vafa, C. Trans-Planckian Censorship and the Swampland. JHEP 2020, 9, 123. [Google Scholar] [CrossRef]
- Bedroya, A.; Brandenberger, R.; Loverde, M.; Vafa, C. Trans-Planckian Censorship and Inflationary Cosmology. Phys. Rev. D 2020, 101, 103502. [Google Scholar] [CrossRef]
- Mizuno, S.; Mukohyama, S.; Pi, S.; Zhang, Y.L. Universal Upper Bound on the Inflationary Energy Scale from the Trans-Planckian Censorship Conjecture. Phys. Rev. D 2020, 102, 021301. [Google Scholar] [CrossRef]
- Ringeval, J.M.J.C.; Vennin, V. Encyclopædia Inflationaris. Phys. Dark Univ. 2014, 5–6, 75–235. [Google Scholar]
- Grande, J.; J, J.S.; Stefancic, H. LXCDM: A Cosmon model solution to the cosmological coincidence problem? JCAP 2006, 8, 011. [Google Scholar] [CrossRef] [Green Version]
- Pelinson, J.G.J.A.; Solà, J. Dark energy perturbations and cosmic coincidenc. Phys. Rev. D 2009, 79, 043006. [Google Scholar]
- Zel’dovich, Y.B. The equation of state at ultrahigh densities and its relativistic limitations. Zh. Eksp. Teor. Fiz. 1961, 41, 1609–1615. [Google Scholar]
- Chavanis, P.H. Cosmology with a stiff matter era. Phys. Rev. D 2015, 92, 103004. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Z.; Jiang, J.; Cai, Y.F.; Sasaki, M.; Pi, S. Primordial black holes and gravitational waves from resonant amplification during inflation. Phys. Rev. D 2020, 102, 103527. [Google Scholar] [CrossRef]
- Fu, C.; Liu, J.; Zhu, T.; Yu, H.; Wu, P. Resonance instability of primordial gravitational waves during inflation in Chern–Simons gravity. Eur. Phys. J. C 2021, 81, 204. [Google Scholar] [CrossRef]
- Gibbons, G.W.; Hawking, S.W. Cosmological Event Horizons, Thermodynamics, and Particle Creation. Phys. Rev. D 1977, 15, 2738. [Google Scholar] [CrossRef] [Green Version]
- Gell-Mann, M.; Ramond, P.; Slansky, R. Supergravity; Freedman, D.Z., van Nieuwenhuizen, P., Eds.; Springer: North-Holland, Amsterdam, 1979. [Google Scholar]
- Yanagida, T. Workshop on the Unified Theory and the Baryon Number in the Universe; Springer: Tsukuba, Japan, 1979. [Google Scholar]
- RMohapatra, N.; Senjanovic, G. Neutrino Mass and Spontaneous Parity Violation. Phys. Rev. Lett. 1980, 44, 912. [Google Scholar] [CrossRef] [Green Version]
- Schechter, J.; Valle, J.W.F. Neutrino Masses in SU(2) x U(1) Theories. Phys. Rev. D 1980, 22, 2227. [Google Scholar] [CrossRef]
- Lazarides, G.; Shafi, Q.; Wetterich, C. Proton Lifetime and Fermion Masses in an SO(10) Model. Nucl. Phys. B 1981, 181, 287. [Google Scholar] [CrossRef]
- Colladay, D.; Kostelecky, V.A. Lorentz violating extension of the standard model. Phys. Rev. D 1998, 58, 116002. [Google Scholar] [CrossRef] [Green Version]
- Kostelecky, V.A.; Russell, N. Data Tables for Lorentz and CPT Violation. Rev. Mod. Phys. 2011, 83, 11. [Google Scholar] [CrossRef] [Green Version]
- Kuzmin, V.A.; Rubakov, V.A.; Shaposhnikov, M.E. On the Anomalous Electroweak Baryon Number Nonconservation in the Early Universe. Phys. Lett. B 1985, 155, 36. [Google Scholar] [CrossRef]
- Gavela, M.B.; Hernandez, P.; Orloff, J.; Pene, P. Standard model CP violation and baryon asymmetry. Mod. Phys. Lett. A 1994, 9, 795–810. [Google Scholar] [CrossRef] [Green Version]
- Gavela, M.B.; Hernandez, P.; Orloff, J.; Quimbay, P.P.a.C. Standard model CP violation and baryon asymmetry. Part 2: Finite temperature. Nucl. Phys. B 1994, 430, 382–426. [Google Scholar] [CrossRef] [Green Version]
- Vissani, F. Do experiments suggest a hierarchy problem? Phys. Rev. D 1998, 57, 7027. [Google Scholar] [CrossRef] [Green Version]
- Bambhaniya, G.; Dev, P.S.B.; Goswami, S.; Khan, S.; Rodejohann, W. Naturalness, Vacuum Stability and Leptogenesis in the Minimal Seesaw Model. Phys. Rev. D 2017, 95, 095016. [Google Scholar] [CrossRef] [Green Version]
- Mavromatos, N.E.; Rizos, J. Exact solutions and the cosmological constant problem in dilatonic domain wall higher curvature string gravity. Int. J. Mod. Phys. A 2003, 18, 57. [Google Scholar] [CrossRef] [Green Version]
- Mavromatos, N.E.; Rizos, J. String inspired higher curvature terms and the Randall-Sundrum scenario. Phys. Rev. D 2000, 62, 124004. [Google Scholar] [CrossRef] [Green Version]
- Mavromatos, N.E. Gravitational anomalies, axions and a string-inspired running vacuum model in Cosmology. arXiv 2021, arXiv:2108.03998. [Google Scholar]
- Nieuwenhuizen, P.V. Supergravity. Phys. Rept. 1981, 68, 189. [Google Scholar] [CrossRef]
- Fradkin, E.S.; Tseytlin, A.A. One Loop Effective Potential in Gauged O(4) Supergravity. Nucl. Phys. B 1984, 234, 472. [Google Scholar] [CrossRef]
- Lanczos, L. Bemerkung zur de Sitterschen Welt. Phys. Z. 1922, 23, 539. [Google Scholar]
- Batalin, I.A.; Vilkovisky, G.A. Quantization of Gauge Theories with Linearly Dependent Generators. Phys. Rev. D 1983, 28, 2567–2582. [Google Scholar] [CrossRef]
- Witten, E. Dynamical Breaking of Supersymmetry. Nucl. Phys. B 1981, 188, 513. [Google Scholar] [CrossRef]
- Basilakos, S.; Mavromatos, N.E.; Solà, J. Starobinsky-like inflation and running vacuum in the context of Supergravity. Universe 2016, 2, 14. [Google Scholar] [CrossRef] [Green Version]
- Nojiri, S.; Odintsov, S.D. Modified gravity with ln R terms and cosmic acceleration. Gen. Rel. Grav. 2004, 1765–1780. [Google Scholar] [CrossRef] [Green Version]
- Eguchi, T.; Gilkey, P.B.; Hanson, A.J. Gravitation, Gauge Theories and Differential Geometry. Phys. Rept. 1980, 66, 213. [Google Scholar] [CrossRef]
- Hull, C.M. Anomalies, Ambiguities and Superstrings. Phys. Lett. B 1986, 167, 51–55. [Google Scholar] [CrossRef]
- Mavromatos, N.E. A Note on the Atiyah-singer Index Theorem for Manifolds With Totally Antisymmetric H Torsion. J. Phys. A 1988, 21, 2279. [Google Scholar] [CrossRef]
- Torres-Gomez, A.; Krasnov, K. Remarks on Barbero-Immirzi parameter as a field. Phys. Rev. D 2009, 79, 104014. [Google Scholar] [CrossRef] [Green Version]
- Freidel, L.; Minic, D.; Takeuchi, T. Quantum gravity, torsion, parity violation and all that. Phys. Rev. D 2005, 72, 104002. [Google Scholar] [CrossRef] [Green Version]
- Perez, A.; Rovelli, C. Physical effects of the Immirzi parameter. Phys. Rev. D 2006, 73, 044013. [Google Scholar] [CrossRef] [Green Version]
- Giacosa, F.; Hofmann, R.; Neubert, M. A model for the very early Universe. JHEP 2008, 2, 077. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mavromatos, N.E. Torsion in String-Inspired Cosmologies and the Universe Dark Sector. Universe 2021, 7, 480. https://doi.org/10.3390/universe7120480
Mavromatos NE. Torsion in String-Inspired Cosmologies and the Universe Dark Sector. Universe. 2021; 7(12):480. https://doi.org/10.3390/universe7120480
Chicago/Turabian StyleMavromatos, Nick E. 2021. "Torsion in String-Inspired Cosmologies and the Universe Dark Sector" Universe 7, no. 12: 480. https://doi.org/10.3390/universe7120480
APA StyleMavromatos, N. E. (2021). Torsion in String-Inspired Cosmologies and the Universe Dark Sector. Universe, 7(12), 480. https://doi.org/10.3390/universe7120480





