Grand Unified Origin of Gauge Interactions and Families Replication in the Standard Model
Abstract
1. Introduction
2. Gauge and Yukawa Structure
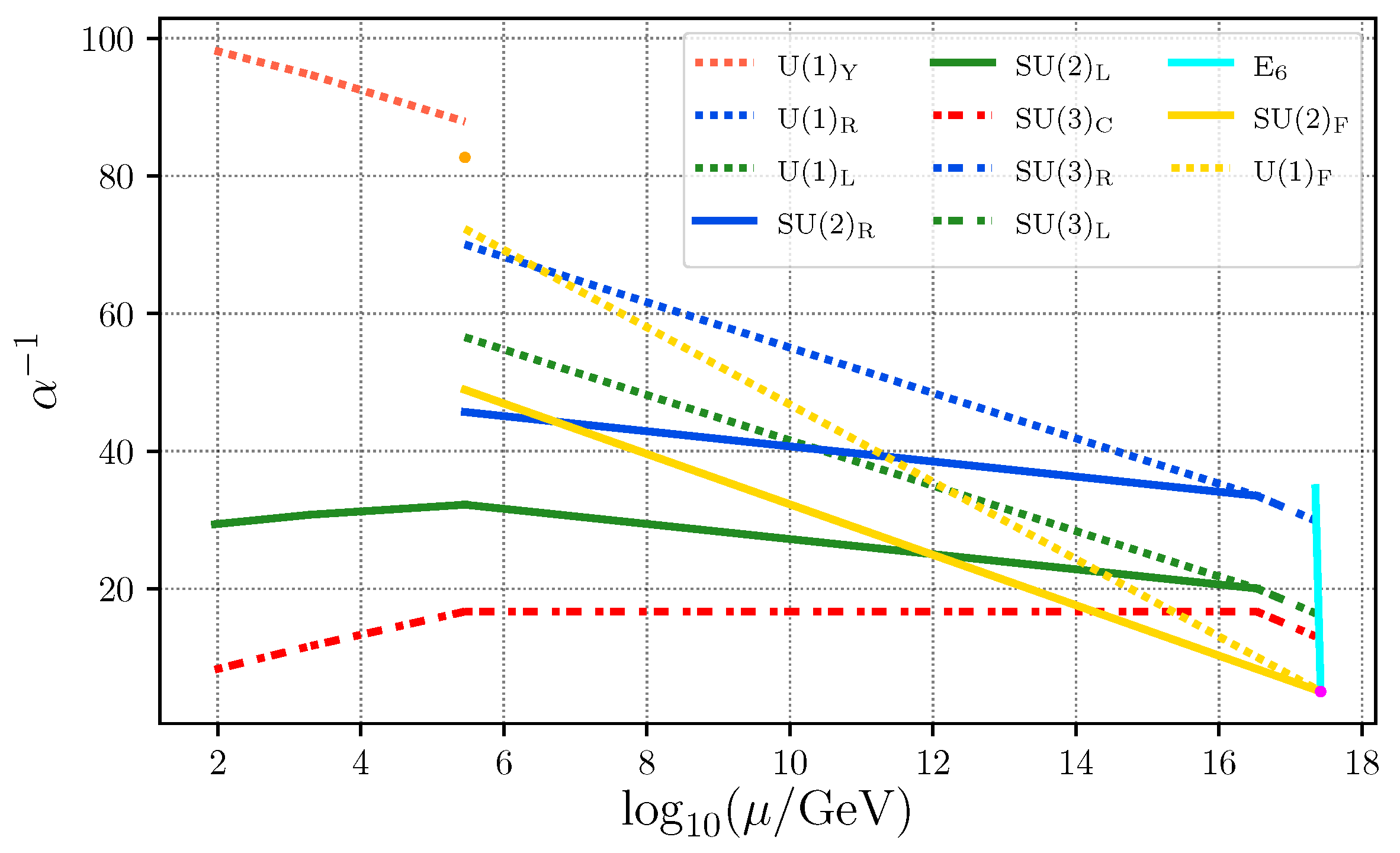
3. The Unification Picture
4. Quark Masses and Mixing
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
| 1 |
References
- Calibbi, L.; Ferretti, L.; Romanino, A.; Ziegler, R. Gauge coupling unification, the GUT scale, and magic fields. Phys. Lett. B 2009, 672, 152–157. [Google Scholar] [CrossRef]
- Martin, S.P. A Supersymmetry primer. Adv. Ser. Direct. High Energy Phys. 1998, 18, 1–98. [Google Scholar] [CrossRef]
- Georgi, H.; Glashow, S.L. Unity of All Elementary Particle Forces. Phys. Rev. Lett. 1974, 32, 438–441. [Google Scholar] [CrossRef]
- Fritzsch, H.; Minkowski, P. Unified Interactions of Leptons and Hadrons. Ann. Phys. 1975, 93, 193–266. [Google Scholar] [CrossRef]
- Chanowitz, M.S.; Ellis, J.R.; Gaillard, M.K. The Price of Natural Flavor Conservation in Neutral Weak Interactions. Nucl. Phys. B 1977, 128, 506–536. [Google Scholar] [CrossRef][Green Version]
- Georgi, H.; Nanopoulos, D.V. T Quark Mass in a Superunified Theory. Phys. Lett. B 1979, 82, 392–394. [Google Scholar] [CrossRef]
- Georgi, H.; Nanopoulos, D.V. Ordinary Predictions from Grand Principles: T Quark Mass in O(10). Nucl. Phys. B 1979, 155, 52–74. [Google Scholar] [CrossRef]
- Georgi, H.; Nanopoulos, D.V. Masses and Mixing in Unified Theories. Nucl. Phys. B 1979, 159, 16–28. [Google Scholar] [CrossRef]
- Georgi, H. Lie algebras in particle physics. From isospin to unified theories. Front. Phys. 1982, 54, 1–255. [Google Scholar]
- Gursey, F.; Ramond, P.; Sikivie, P. A Universal Gauge Theory Model Based on E6. Phys. Lett. B 1976, 60, 177–180. [Google Scholar] [CrossRef]
- Gursey, F.; Serdaroglu, M. E6 gauge field theory model revisited. Nuovo Cim. A 1981, 65, 337. [Google Scholar] [CrossRef]
- Achiman, Y.; Stech, B. Quark Lepton Symmetry and Mass Scales in an E6 Unified Gauge Model. Phys. Lett. B 1978, 77, 389–393. [Google Scholar] [CrossRef]
- Pati, J.C.; Salam, A. Lepton Number as the Fourth Color. Phys. Rev. D 1974, 10, 275–289, Erratum in 1975, 11, 703. [Google Scholar] [CrossRef]
- Athron, P.; King, S.F.; Miller, D.J.; Moretti, S.; Nevzorov, R.; King, S.F.; Miller, D.J.; Moretti, S.; Nevzorov, R. The Constrained E6SSM. 2008. Available online: https://arxiv.org/abs/0810.0617 (accessed on 22 November 2021).
- Hall, J.P.; King, S.F.; Nevzorov, R.; Pakvasa, S.; Sher, M.; Nevzorov, R.; Pakvasa, S.; Sher, M. Novel Higgs Decays and Dark Matter in the E(6)SSM. Phys. Rev. D 2011, 83, 075013. [Google Scholar] [CrossRef]
- Rizzo, T.G. Gauge Kinetic Mixing in the E6SSM. Phys. Rev. D 2012, 85, 055010. [Google Scholar] [CrossRef]
- Nevzorov, R. E6 inspired supersymmetric models with exact custodial symmetry. Phys. Rev. D 2013, 87, 015029. [Google Scholar] [CrossRef]
- Nevzorov, R.; Pakvasa, S. Exotic Higgs decays in the E6 inspired SUSY models. Phys. Lett. B 2014, 728, 210–215. [Google Scholar] [CrossRef]
- Nevzorov, R.; Thomas, A.W. E6 inspired composite Higgs model. Phys. Rev. D 2015, 92, 075007. [Google Scholar] [CrossRef]
- Athron, P.; Harries, D.; Nevzorov, R.; Williams, A.G. E6 Inspired SUSY Benchmarks, Dark Matter Relic Density and a 125 GeV Higgs. Phys. Lett. B 2016, 760, 19–25. [Google Scholar] [CrossRef]
- Ishihara, T.; Maekawa, N.; Takegawa, M.; Yamanaka, M. Leptogenesis in E6×U(1)A SUSY GUT model. JHEP 2016, 2, 108. [Google Scholar] [CrossRef][Green Version]
- Berezhiani, Z.G.; Dvali, G.R. Possible solution of the hierarchy problem in supersymmetrical grand unification theories. Bull. Lebedev Phys. Inst. 1989, 5, 55–59. [Google Scholar]
- Berezhiani, Z.; Csaki, C.; Randall, L. Could the supersymmetric Higgs particles naturally be pseudoGoldstone bosons? Nucl. Phys. B 1995, 444, 61–91. [Google Scholar] [CrossRef]
- Dvali, G.R.; Pokorski, S. The Role of the anomalous U(1)-A for the solution of the doublet - triplet splitting problem. Phys. Rev. Lett. 1997, 78, 807–810. [Google Scholar] [CrossRef]
- Barbieri, R.; Dvali, G.R.; Strumia, A.; Berezhiani, Z.; Hall, L.J. Flavor in supersymmetric grand unification: A Democratic approach. Nucl. Phys. B 1994, 432, 49–67. [Google Scholar] [CrossRef]
- Ordell, A.; Pasechnik, R.; Serôdio, H.; Nottensteiner, F. Classification of anomaly-free 2HDMs with a gauged U(1)’ symmetry. Phys. Rev. D 2019, 100, 115038. [Google Scholar] [CrossRef]
- Cárcamo Hernández, A.E.; Kovalenko, S.; Pasechnik, R.; Schmidt, I. Sequentially loop-generated quark and lepton mass hierarchies in an extended Inert Higgs Doublet model. JHEP 2019, 6, 056. [Google Scholar] [CrossRef]
- Vien, V.V.; Long, H.N.; Cárcamo Hernández, A.E. Fermion Mass and Mixing in a Low-Scale Seesaw Model based on the S4 Flavor Symmetry. Prog. Theor. Exp. Phys. 2019, 11, 113. [Google Scholar] [CrossRef]
- Cárcamo Hernández, A.E.; Hidalgo Velásquez, Y.; Pérez-Julve, N.A. A 3-3-1 model with low scale seesaw mechanisms. Eur. Phys. J. C 2019, 79, 828. [Google Scholar] [CrossRef]
- Cárcamo Hernández, A.E.; Long, H.N.; Vien, V.V. The first Δ(27) flavor 3-3-1 model with low scale seesaw mechanism. Eur. Phys. J. C 2018, 78, 804. [Google Scholar] [CrossRef]
- Björkeroth, F.; de Medeiros Varzielas, I.; López-Ibáñez, M.L.; Melis, A.; Vives, O. Leptogenesis in Δ(27) with a Universal Texture Zero. JHEP 2019, 09, 050. [Google Scholar] [CrossRef]
- Ding, G.J.; King, S.F.; Liu, X.G.; Lu, J.N. Modular S4 and A4 Symmetries and Their Fixed Points: New Predictive Examples of Lepton Mixing. JHEP 2019, 12, 030. [Google Scholar] [CrossRef]
- Berezhiani, Z.G.; Chkareuli, J.L. Quark-leptonic families in a model with su(5) × su(3) symmetry. Sov. J. Nucl. Phys. 1983, 37, 618–626. (In Russian) [Google Scholar]
- Berezhiani, Z.G. Horizontal Symmetry and Quark-Lepton Mass Spectrum: The SU(5) × SU(3)-h Model. Phys. Lett. B 1985, 150, 177–181. [Google Scholar] [CrossRef]
- Berezhiani, Z.G.; Khlopov, M.Y. The Theory of broken gauge symmetry of families. Sov. J. Nucl. Phys. 1990, 51, 739–746. (In Russian) [Google Scholar]
- Berezhiani, Z. Unified picture of the particle and sparticle masses in SUSY GUT. Phys. Lett. B 1998, 417, 287–296. [Google Scholar] [CrossRef]
- Ross, G.G.; Vives, O. Yukawa structure, flavor and CP violation in supergravity. Phys. Rev. D 2003, 67, 095013. [Google Scholar] [CrossRef]
- Ross, G.G.; Velasco-Sevilla, L.; Vives, O. Spontaneous CP violation and nonAbelian family symmetry in SUSY. Nucl. Phys. B 2004, 692, 50–82. [Google Scholar] [CrossRef]
- Camargo-Molina, J.E.; Morais, A.P.; Ordell, A.; Pasechnik, R.; Sampaio, M.O.; Wessén, J. Reviving trinification models through an E6 -extended supersymmetric GUT. Phys. Rev. D 2017, 95, 075031. [Google Scholar] [CrossRef]
- Camargo-Molina, J.E.; Morais, A.P.; Ordell, A.; Pasechnik, R.; Wessén, J. Scale hierarchies, symmetry breaking and particle spectra in SU(3)-family extended SUSY trinification. Phys. Rev. D 2019, 99, 035041. [Google Scholar] [CrossRef]
- Witten, E. Dynamical Breaking of Supersymmetry. Nucl. Phys. B 1981, 188, 513. [Google Scholar] [CrossRef]
- Slansky, R. Group Theory for Unified Model Building. Phys. Rep. 1981, 79, 1–128. [Google Scholar] [CrossRef]
- Kephart, T.W.; Vaughn, M.T. Tensor Methods for the Exceptional Group E6. Ann. Phys. 1983, 145, 162. [Google Scholar] [CrossRef]
- Deppisch, T. E6Tensors: A Mathematica Package for E6 Tensors. Comput. Phys. Commun. 2017, 213, 130–135. [Google Scholar] [CrossRef]
- Gursey, F. Symmetry Breaking Patterns in E6. In First Workshop on Grand Unification. Birkhäuser Boston: Boston, MA, USA, 1980; p. 0039. [Google Scholar]
- Chakrabortty, J.; Raychaudhuri, A. A Note on dimension-5 operators in GUTs and their impact. Phys. Lett. B 2009, 673, 57–62. [Google Scholar] [CrossRef]
- Morais, A.P.; Pasechnik, R.; Porod, W. Prospects for new physics from gauge left-right-colour-family grand unification hypothesis. Eur. Phys. J. C 2020, 80, 1162. [Google Scholar] [CrossRef]
- Glashow, S. Fifth Workshop on Grand Unification; Kang, K., Fried, H., Frampton, F., Eds.; World Scientific: Singapore, 1984. [Google Scholar]
| -Even | -Odd |
|---|---|
| U(1)F | U(1)W | U(1)B | |||||
|---|---|---|---|---|---|---|---|
| 1 | 1 | 0 | |||||
| 1 | 0 | ||||||
| 1 | |||||||
| 1 | |||||||
| 0 | 0 | 0 | |||||
| 0 | 0 | 0 | |||||
| 0 | 0 | 0 | |||||
| 0 | 0 | 0 | |||||
| 0 | 0 | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morais, A.P.; Pasechnik, R.; Porod, W. Grand Unified Origin of Gauge Interactions and Families Replication in the Standard Model. Universe 2021, 7, 461. https://doi.org/10.3390/universe7120461
Morais AP, Pasechnik R, Porod W. Grand Unified Origin of Gauge Interactions and Families Replication in the Standard Model. Universe. 2021; 7(12):461. https://doi.org/10.3390/universe7120461
Chicago/Turabian StyleMorais, António P., Roman Pasechnik, and Werner Porod. 2021. "Grand Unified Origin of Gauge Interactions and Families Replication in the Standard Model" Universe 7, no. 12: 461. https://doi.org/10.3390/universe7120461
APA StyleMorais, A. P., Pasechnik, R., & Porod, W. (2021). Grand Unified Origin of Gauge Interactions and Families Replication in the Standard Model. Universe, 7(12), 461. https://doi.org/10.3390/universe7120461







