Does the Loop Quantum μo Scheme Permit Black Hole Formation?
Abstract
:1. Introduction
2. Preliminaries: The Classical Dust Shell Model
3. Loop Quantizations of the Dust Shell Model
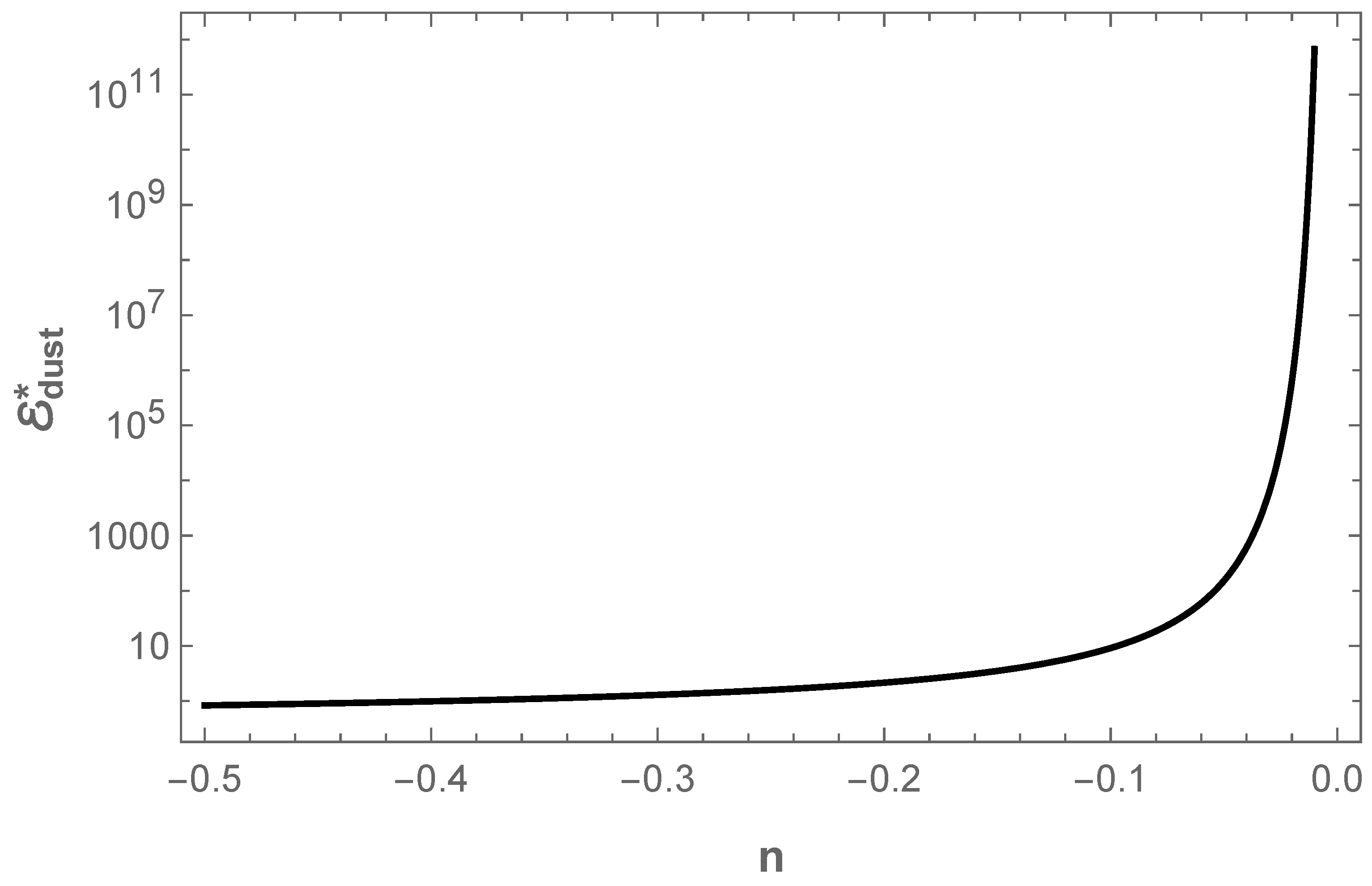
3.1. The Scheme
3.2. The Scheme
3.3. Other Quantizations with Different Kinds of Lattice Refinement
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
| 1 | Recently, a question on similar lines was asked regarding a homogeneous collapse to understand the role of triad vs. gauge-covariant fluxes, but only for a specific quantization prescription (the scheme) [15]. |
| 2 |
References
- Ashtekar, A.; Pawlowski, T.; Singh, P. Quantum nature of the Big Bang. Phys. Rev. Lett. 2006, 96, 141301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ashtekar, A.; Pawlowski, T.; Singh, P. Quantum nature of the Big Bang: An analytical and numerical investigation. I. Phys. Rev. D 2006, 73, 124038. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Pawlowski, T.; Singh, P. Quantum nature of the Big Bang: Improved dynamics. Phys. Rev. D 2006, 74, 084003. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Corichi, A.; Singh, P. Robustness of key features of loop quantum cosmology. Phys. Rev. D 2010, 77, 024046. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Singh, P. Loop Quantum Cosmology: A Status Report. Class. Quant. Grav. 2011, 28, 213001. [Google Scholar] [CrossRef] [Green Version]
- Singh, P. Are loop quantum cosmos never singular? Class. Quant. Grav. 2009, 26, 125005. [Google Scholar] [CrossRef]
- Singh, P. Curvature invariants, geodesics and the strength of singularities in Bianchi-I loop quantum cosmology. Phys. Rev. 2012, 85, 104011. [Google Scholar] [CrossRef] [Green Version]
- Singh, P. Loop quantum cosmology and the fate of cosmological singularities. arXiv 2014, arXiv:1509.09182. [Google Scholar]
- Saini, S.; Singh, P. Resolution of strong singularities and geodesic completeness in loop quantum Bianchi-II spacetimes. Class. Quant. Grav. 2017, 34, 235006. [Google Scholar] [CrossRef] [Green Version]
- Saini, S.; Singh, P. Generic absence of strong singularities in loop quantum Bianchi-IX spacetimes. Class. Quant. Grav. 2018, 35, 065014. [Google Scholar] [CrossRef] [Green Version]
- Corichi, A.; Singh, P. Is loop quantization in cosmology unique? Phys. Rev. D 2008, 78, 024034. [Google Scholar] [CrossRef] [Green Version]
- Corichi, A.; Singh, P. Geometric perspective on singularity resolution and uniqueness in loop quantum cosmology. Phys. Rev. D 2009, 80, 044024. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Olmedo, J.; Singh, P. Quantum transfiguration of Kruskal black holes. Phys. Rev. Lett. 2018, 121, 241301. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Olmedo, J.; Singh, P. Quantum extension of the Kruskal spacetime. Phys. Rev. D 2018, 98, 126003. [Google Scholar] [CrossRef] [Green Version]
- Giesel, K.; Li, B.-F.; Singh, P. Non-singular quantum gravitational dynamics of an LTB dust shell model: The role of quantization prescriptions. arXiv 2021, arXiv:2107.05797. [Google Scholar]
- Ashtekar, A.; Bojowald, M.; Lewandowski, J. Mathematical structure of loop quantum cosmology. Adv. Theor. Math. Phys. 2003, 7, 233–268. [Google Scholar] [CrossRef]
- Ashtekar, A.; Bojowald, M. Quantum geometry and the Schwarzschild singularity. Class. Quant. Grav. 2005, 23, 391–411. [Google Scholar] [CrossRef] [Green Version]
- Bohmer, C.G.; Vandersloot, K. Loop quantum dynamics of the Schwarzschild interior. Phys. Rev. D 2007, 76, 104030. [Google Scholar] [CrossRef] [Green Version]
- Campiglia, M.; Gambini, R.; Pullin, J. Loop quantization of spherically symmetric midi-superspaces: The interior problem. AIP Conf. Proc. 2008, 977, 52–63. [Google Scholar]
- Gambini, R.; Pullin, J. Black holes in Loop Quantum Gravity: The complete space-time. Phys. Rev. Lett. 2008, 101, 161301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gambini, R.; Pullin, J. Loop quantization of the Schwarzschild black hole. Phys. Rev. Lett. 2013, 110, 211301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gambini, R.; Olmedo, J.; Pullin, J. Quantum black holes in loop quantum gravity. Class. Quant. Grav. 2014, 31, 095009. [Google Scholar] [CrossRef]
- Corichi, A.; Singh, P. Loop quantization of the Schwarzschild interior revisited. Class. Quant. Grav. 2016, 33, 055006. [Google Scholar] [CrossRef]
- Olmedo, J.; Saini, S.; Singh, P. From black holes to white holes: A quantum gravitational, symmetric bounce. Class. Quant. Grav. 2017, 34, 225011. [Google Scholar] [CrossRef]
- Gambini, R.; Olmedo, J.; Pullin, J. Spherically symmetric loop quantum gravity: Analysis of improved dynamics. Class. Quant. Grav. 2020, 37, 205012. [Google Scholar] [CrossRef]
- Bojowald, M.; Goswami, R.; Maartens, R.; Singh, P. Black hole mass threshold from nonsingular quantum gravitational collapse. Phys. Rev. Lett. 2005, 95, 091302. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Goswami, R.; Joshi, P.S.; Singh, P. Quantum evaporation of a naked singularity. Phys. Rev. Lett. 2006, 96, 031302. [Google Scholar] [CrossRef] [Green Version]
- Gambini, R.; Pullin, J.; Rastgoo, S. Quantum scalar field in quantum gravity: The vacuum in the spherically symmetric case. Class. Quant. Grav. 2009, 26, 215011. [Google Scholar] [CrossRef]
- Bambi, C.; Malafarina, D.; Modesto, L. Non-singular quantum-inspired gravitational collapse. Phys. Rev. 2013, 88, 044009. [Google Scholar] [CrossRef] [Green Version]
- Tavakoli, Y.; Marto, J.; Dapor, A. Semiclassical dynamics of horizons in spherically symmetric collapse. Int. J. Mod. Phys. D 2014, 23, 1450061. [Google Scholar] [CrossRef]
- Benitez, F.; Gambini, R.; Lehner, L.; Liebling, S.; Pullin, J. Critical collapse of a scalar field in semiclassical Loop Quantum Gravity. Phys. Rev. Lett. 2020, 124, 071301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Han, M.; Liu, H. Improved effective dynamics of Loop-Quantum-Gravity black hole and Nariai limit. arXiv 2020, arXiv:2012.05729. [Google Scholar]
- Kelly, J.G.; Santacruz, R.; Wilson-Ewing, E. Black hole collapse and bounce in effective loop quantum gravity. Class. Quant. Grav. 2020, 38, 04LT01. [Google Scholar] [CrossRef]
- Bojowald, M.; Harada, T.; Tibrewala, R. Lemaître-Tolman-Bondi collapse from the perspective of loop quantum gravity. Phys. Rev. 2008, 78, 064057. [Google Scholar] [CrossRef] [Green Version]
- Barrabès, C.; Israel, W. Thin shells in general relativity and cosmology: The lightlike limit. Phys. Rev. 1991, 43, 1129. [Google Scholar] [CrossRef]
- Goswami, R.; Joshi, P.S. Naked Singularity formation in scalar field collapse. arXiv 2004, arXiv:gr-qc/0410144. [Google Scholar]
- Singh, P. Glimpses of spacetime beyond the singularities using supercomputers. Comput. Sci. Eng. 2018, 20, 26–38. [Google Scholar] [CrossRef] [Green Version]
- Diener, P.; Gupt, B.; Singh, P. Numerical simulations of a loop quantum cosmos: Robustness of the quantum bounce and the validity of effective dynamics. Class. Quant. Grav. 2014, 31, 105015. [Google Scholar] [CrossRef]
- Diener, P.; Gupt, B.; Megevand, M.; Singh, P. Numerical evolution of squeezed and non-Gaussian states in loop quantum cosmology. Class. Quant. Grav. 2014, 31, 165006. [Google Scholar] [CrossRef] [Green Version]
- Meissner, K.A. Black-hole entropy in loop quantum gravity. Class. Quant. Grav. 2004, 21, 5245–5251. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Baez, J.; Corichi, A.; Krasnov, K. Quantum Geometry and Black Hole Entropy. Phys. Rev. Lett. 1998, 80, 904–907. [Google Scholar] [CrossRef] [Green Version]
- Dreyer, O. Quasinormal Modes, the Area Spectrum, and Black Hole Entropy. Phys. Rev. Lett. 2003, 90, 081301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Domagala, M.; Lewandowski, J. Black-hole entropy from quantum geometry. Class. Quant. Grav. 2004, 21, 5233–5243. [Google Scholar] [CrossRef] [Green Version]
- Ansari, M.H. Generic degeneracy and entropy in loop quantum gravity. Nucl. Phys. B 2008, 795, 635–644. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A. Loop quantum cosmology: An overview. Gen. Rel. Grav. 2009, 41, 707–741. [Google Scholar] [CrossRef]
- Ashtekar, A.; Wilson-Ewing, E. Covariant entropy bound and loop quantum cosmology. Phys. Rev. 2008, 78, 064047. [Google Scholar] [CrossRef] [Green Version]
- Bojowald, M. Loop quantum cosmology and inhomogeneities. Gen. Rel. Grav. 2006, 38, 1771–1795. [Google Scholar] [CrossRef] [Green Version]
- Ashtekar, A.; Pawlowski, T.; Singh, P.; Vandersloot, K. Loop quantum cosmology of k = 1 FRW models. Phys. Rev. D 2007, 75, 024035. [Google Scholar] [CrossRef] [Green Version]
- Corichi, A.; Karami, A. Loop quantum cosmology of k = 1 FRW: A tale of two bounces. Phys. Rev. D 2011, 84, 044003. [Google Scholar] [CrossRef] [Green Version]
- Dupuy, J.L.; Singh, P. Implications of quantum ambiguities in k = 1 loop quantum cosmology: Distinct quantum turnarounds and the super-Planckian regime. Phys. Rev. D 2017, 95, 023510. [Google Scholar] [CrossRef] [Green Version]
- Li, B.-F.; Singh, P. Loop quantization prescriptions and black hole formation in the bound case. 2021; in preparation. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, B.-F.; Singh, P. Does the Loop Quantum μo Scheme Permit Black Hole Formation? Universe 2021, 7, 406. https://doi.org/10.3390/universe7110406
Li B-F, Singh P. Does the Loop Quantum μo Scheme Permit Black Hole Formation? Universe. 2021; 7(11):406. https://doi.org/10.3390/universe7110406
Chicago/Turabian StyleLi, Bao-Fei, and Parampreet Singh. 2021. "Does the Loop Quantum μo Scheme Permit Black Hole Formation?" Universe 7, no. 11: 406. https://doi.org/10.3390/universe7110406
APA StyleLi, B.-F., & Singh, P. (2021). Does the Loop Quantum μo Scheme Permit Black Hole Formation? Universe, 7(11), 406. https://doi.org/10.3390/universe7110406






