Holographic Interpretation of Relative State Complexity
Abstract
1. Introduction
2. Complexity and Chaos
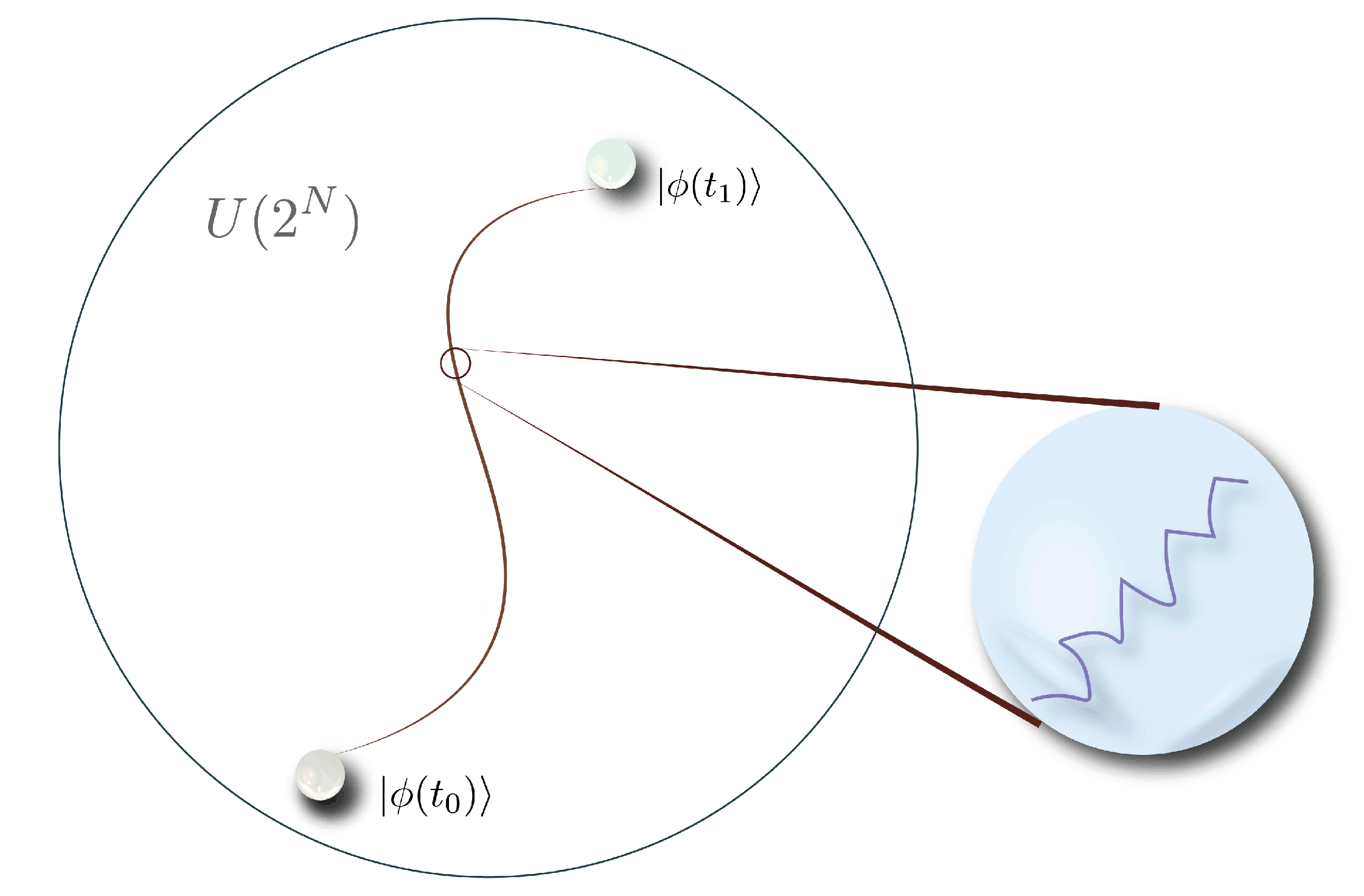
2.1. Complexity
2.1.1. Complexity = Action
2.1.2. Complexity = Volume
2.2. Chaos
3. Geometric Interpretation
3.1. Complexity & Chaos
3.2. Sachdev–Ye–Kitaev
4. Relative State Complexity & Pseudorandomness
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Couch, J.; Eccles, S.; Jacobson, T.; Nguyen, P. Holographic Complexity and Volume. J. High Energy Phys. 2018, 11, 044. [Google Scholar] [CrossRef]
- Stanford, D.; Susskind, L. Complexity and Shock Wave Geometries. Phys. Rev. D 2014, 90, 126007. [Google Scholar] [CrossRef]
- Christodoulou, M.; Rovelli, C. How Big is a Black Hole? Phys. Rev. D 2015, 91, 064046. [Google Scholar] [CrossRef]
- Brown, A.; Roberts, D.; Susskind, L.; Swingle, B.; Zhao, Y. Complexity, Action, and Black Holes. Phys. Rev. D 2016, 93, 086006. [Google Scholar] [CrossRef]
- Brown, A.; Roberts, D.; Susskind, L.; Swingle, B.; Zhao, Y. Complexity Equals Action. Phys. Rev. Lett. 2016, 116, 191301. [Google Scholar] [CrossRef]
- Brown, A.; Susskind, L. The Second Law of Quantum Complexity. Phys. Rev. D 2018, 97, 086015. [Google Scholar] [CrossRef]
- Nielsen, M.; Dowling, M.; Gu, M.; Doherty, A. Optimal Control, Geometry, and Quantum Computing. Phys. Rev. A 2006, 73, 062323. [Google Scholar] [CrossRef]
- Dowling, M.; Nielsen, M. The Geometry of Quantum Computation. arXiv 2007, arXiv:quant-ph/0701004. [Google Scholar]
- Nielsen, M. A Geometric Approach to Quantum Circuit Lower Bounds. arXiv 2005, arXiv:quant-ph/0502070. [Google Scholar]
- Maldacena, J.; Shenker, S.; Stanford, D. A Bound on Chaos. J. High Energy Phys. 2016, 08, 106. [Google Scholar] [CrossRef]
- Bohigas, O.; Giannoni, M.J.; Schmit, C. Characterization of Chaotic Quantum Spectra and Universality of Level Fluctuation Laws. Phys. Rev. Lett. 1984, 52, 1. [Google Scholar] [CrossRef]
- Bueno, P.; Magan, J.; Shahbazi, C. Complexity Measures in QFT and Constrained Geometric Actions. arXiv 2019, arXiv:1908.03577. [Google Scholar]
- Yosifov, A.; Filipov, L. Quantum Complexity and Chaos in Young Black Holes. Universe 2019, 5, 93. [Google Scholar] [CrossRef]
- Yang, R.Q.; Kim, K.Y. Time Evolution of the Complexity in Chaotic Systems: Concrete Examples. J. High Energy Phys. 2020, 05, 045. [Google Scholar] [CrossRef]
- Miyaji, M. Butterflies from Information Metric. J. High Energy Phys. 2016, 09, 002. [Google Scholar] [CrossRef]
- Van Raamsdonk, M. Evaporating Firewalls. J. High Energy Phys. 2014, 11, 038. [Google Scholar] [CrossRef]
- Kim, I.; Tang, E.; Preskill, J. The Ghost in the Radiation: Robust Encodings of the Black Hole Interior. arXiv 2020, arXiv:2003.05451. [Google Scholar] [CrossRef]
- Harlow, D.; Hayden, P. Quantum Computation vs. Firewalls. J. High Energy Phys. 2013, 06, 085. [Google Scholar] [CrossRef]
- Emerson, J.; Livine, E.; Lloyd, S. Convergence Conditions for Random Quantum Circuits. Phys. Rev. A 2005, 72, 060302. [Google Scholar] [CrossRef]
- Harrow, A.; Low, R. Random Quantum Circuits are Approximate 2-designs. Comm. Math. Phys. 2009, 291, 257–302. [Google Scholar] [CrossRef]
- Magan, J. Black holes, Complexity and Quantum Chaos. J. High Energy Phys. 2018, 09, 043. [Google Scholar] [CrossRef]
- Lloyd, S. Ultimate Physical Limits to Computation. Nature 2000, 406, 1047–1057. [Google Scholar] [CrossRef] [PubMed]
- Casini, H.; Huerta, M.; Myers, R. Towards a Derivation of Holographic Entanglement Entropy. J. High Energy Phys. 2011, 05, 036. [Google Scholar] [CrossRef]
- Carmi, D.; Chapman, S.; Marrochio, H.; Myers, R.C.; Sugishita, S. On the Time Dependence of Holographic Complexity. J. High Energy Phys. 2017, 188, 1711. [Google Scholar] [CrossRef]
- Kim, K.Y.; Niu, C.; Yang, R.Q.; Zhang, C.Y. Comparison of holographic and field theoretic complexities by time dependent thermofield double states. arXiv 2018, arXiv:1710.00600v2. [Google Scholar]
- Susskind, L. Entanglement is Not Enough. arXiv 2014, arXiv:1411.0690. [Google Scholar] [CrossRef]
- Ali, T.; Bhattacharyya, A.; Shajidul Haque, S.; Kim, E.; Moynihan, N.; Murugan, J. Chaos and Complexity in Quantum Mechanics. Phys. Rev. D 2020, 101, 026021. [Google Scholar] [CrossRef]
- Facchi, P.; Kulkarni, R.; Manko, V.; Marmo, G.; Sudarshan, E.; Ventriglia, F. Classical and Quantum Fisher Information in the Geometrical Formulation of Quantum Mechanics. Phys. Lett. A 2010, 374, 4801. [Google Scholar] [CrossRef]
- Balasubramanian, V.; DeCross, M.; Kar, A.; Parrikar, O. Quantum Complexity of Time Evolution with Chaotic Hamiltonians. J. High Energy Phys. 2020, 01, 134. [Google Scholar] [CrossRef]
- Polchinski, J.; Rosenhaus, V. The Spectrum in the Sachdev-Ye-Kitaev Model. J. High Energy Phys. 2016, 04, 001. [Google Scholar] [CrossRef]
- Maldacena, J.; Stanford, D. Comments on the Sachdev-Ye-Kitaev Model. Phys. Rev. D 2016, 94, 106002. [Google Scholar] [CrossRef]
- Maldacena, J.; Susskind, L. Cool Horizons for Entangled Black Holes. Fortschr. Phys. 2013, 61, 781–811. [Google Scholar] [CrossRef]
- Hartman, T.; Maldacena, J. Time Evolution of Entanglement Entropy from Black Hole Interiors. J. High Energy Phys. 2013, 05, 014. [Google Scholar] [CrossRef]
- Harlow, D. Aspects of the Papadodimas-Raju Proposal for the Black Hole Interior. J. High Energy Phys. 2014, 55, 2014. [Google Scholar] [CrossRef]
- Jalabert, R.; Pastawski, H. Environment-Independent Decoherence Rate in Classically Chaotic Systems. Phys. Rev. Lett. 2001, 86, 2490. [Google Scholar] [CrossRef]
- Hayden, P.; Leung, D.; Winter, A. Aspects of Generic Entanglement. Comm. Math. Phys. 2006, 265, 95. [Google Scholar] [CrossRef]
- Brown, A.; Susskind, L. The Complexity Geometry of a Single Qubit. Phys. Rev. D 2019, 100, 046020. [Google Scholar] [CrossRef]
- Nally, R. Stringy Effects and the Role of the Singularity in Holographic Complexity. J. High Energy Phys. 2019, 09, 094. [Google Scholar] [CrossRef]
- Susskind, L. Butterflies on the Stretched Horizon. arXiv 2013, arXiv:1311.7379v2. [Google Scholar]
- Susskind, L. Computational Complexity and Black Hole Horizons. arXiv 2014, arXiv:1402.5674v2. [Google Scholar] [CrossRef]
| 1. | We should note that, classically, the interior black hole volume continues to grow indefinitely, see Christodoulou–Rovelli [3]. |
| 2. | Using the classical action associated with the WDW patch in AdS spacetimes eliminates the ambiguities related to the size of the horizon relative to the AdS radius. |
| 3. | Note that the entropic behavior of complexity [6] suggests the relative state complexity for any and increases linearly in time and with the number of degrees of freedom. |
| 4. | |
| 5. | |
| 6. | Throughout the paper we will work under the assumption that a black hole (holographically dual to a strongly-coupled large-N CFT) is represented as a collection of N qubits, where . |
| 7. | For similar setup, see [13]. Note that for a time interval , where we can divide into j steps, where each is of order . |
| 8. | Being the fastest scramblers and the most chaotic objects in nature, black holes saturate the Lloyd’s bound. |
| 9. | |
| 10. | The authors of [27] propose a new diagnostic for quantum chaos, where by tuning the interaction Hamiltonian, a timescale, associated with the transition between classical and chaotic dynamics, is derived. |
| 11. | It was demonstrated in [29] that the geodesic remains locally minimal for at least exponential time. |
| 12. | For a detailed description of the instantaneous Hamiltonian, see [21]. |
| 13. | For a detailed take on this approach, see [29] and the references therein. |
| 14. | It should be noted that the dual AdS black hole is “young,” meaning it has evaporated much less than half of its initial degrees of freedom. |
| 15. | The scrambling time is well-motivated even from a purely classical general relativity perspective as it is related to the relaxation time. |
| 16. | Note that if the auxiliary CFT is an exact copy of the original CFT, the two boundary theories are initially weakly correlated, and adiabatically evolve toward a TFD state (9). |
| 17. | Using the minimal depth of a quantum circuit as a measure of the computational cost associated with executing a task, e.g., (47), resembles the Hartman–Maldacena tensor networks [33]. Tensor networks, like quantum circuits, have width and depth, and their evolution is very similar to that of circuits. |
| 18. | Effectively, the interaction Hamiltonian plays the role of an encoding map , where denotes an interior subregion which, assuming the validity of the equivalence principle, is purified by the late-time Hawking radiation. |
| 19. | Harlow–Hayden [18] argued that AMPS’ conjectured violation of the equivalence principle after Page time is computationally unrealizable for astrophysical black holes since it requires complicated measurements with superpolynomial-size quantum circuits on the emitted Hawking quanta the execution of which would take time exponential in the entropy. That is, no reasonable outside observer can verify that is purified by a subsystem M. |
| 20. | We should note, however, that if Alice had access to all the relevant degrees of freedom, e.g., she waits for the black hole to evaporate completely, she would be able to efficiently decode M. |
| 21. |

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yosifov, A.; Iyer, A.; Filipov, L. Holographic Interpretation of Relative State Complexity. Universe 2020, 6, 100. https://doi.org/10.3390/universe6080100
Yosifov A, Iyer A, Filipov L. Holographic Interpretation of Relative State Complexity. Universe. 2020; 6(8):100. https://doi.org/10.3390/universe6080100
Chicago/Turabian StyleYosifov, Alexander, Aditya Iyer, and Lachezar Filipov. 2020. "Holographic Interpretation of Relative State Complexity" Universe 6, no. 8: 100. https://doi.org/10.3390/universe6080100
APA StyleYosifov, A., Iyer, A., & Filipov, L. (2020). Holographic Interpretation of Relative State Complexity. Universe, 6(8), 100. https://doi.org/10.3390/universe6080100





