The Scale-Invariant Vacuum (SIV) Theory: A Possible Origin of Dark Matter and Dark Energy
Abstract
1. Introduction
2. The Basic Equations and Properties of the SIV Theory
2.1. Integrable Weyl Geometry, Cotensor Expressions, and the Scale Invariant Field Equations
2.2. The General Field Equation from an Action Principle and the Lagrangian
2.3. Fixing the Gauge
- Since represents the energy of the vacuum, it also establishes a relation between the energy density of the vacuum and the scale factor and its possible variations.
- As noted by Durrer and Maartens [29], “Instead of a cosmological constant, one may also introduce a scalar field or some other contribution to the energy-momentum tensor...”, this is just what is happening here. The field as defined in Equation (3) is therefore a scalar field associated with the vacuum properties at macroscopic scales in the Universe.
- In GR, and therefore the properties of the empty space associated with it are considered to not depend on the matter content of the Universe. This is evident upon considering the Einstein gauge () in (21) along with the fact that radiation does not contribute to the trace and the matter contribution vanishes since in the limit of ever increasing scale factor a. Thus, in standard GR, one is forced to accept . We adopt the same assumption here. This means that the above expressions of (27) are valid whatever the matter content of the Universe. In particular, if the IWG with satisfying (27) is adopted, then the corresponding equations (15) would imply local no-cosmological constant Einstein GR equations for the matter fields (see (30) and (31)).
- The solution of the above two equations (27) is of the form: . Both Equations (27) imply , while follows from the first equation. Any value of the parameter b would satisfy the equations. However, the value of b has to be consistent with the cosmological equations for the expansion factor when applied to the considered case of an empty space. The results below and in Maeder [15] show that has to be taken in this case.
- The expression of in current units (in calculations is usually taken). The value at the present time is then (with ).
3. Accelerating Cosmological Models
3.1. The Basic Equations of SIV Cosmology
3.2. Density, Geometry Parameters, and Conservation Laws
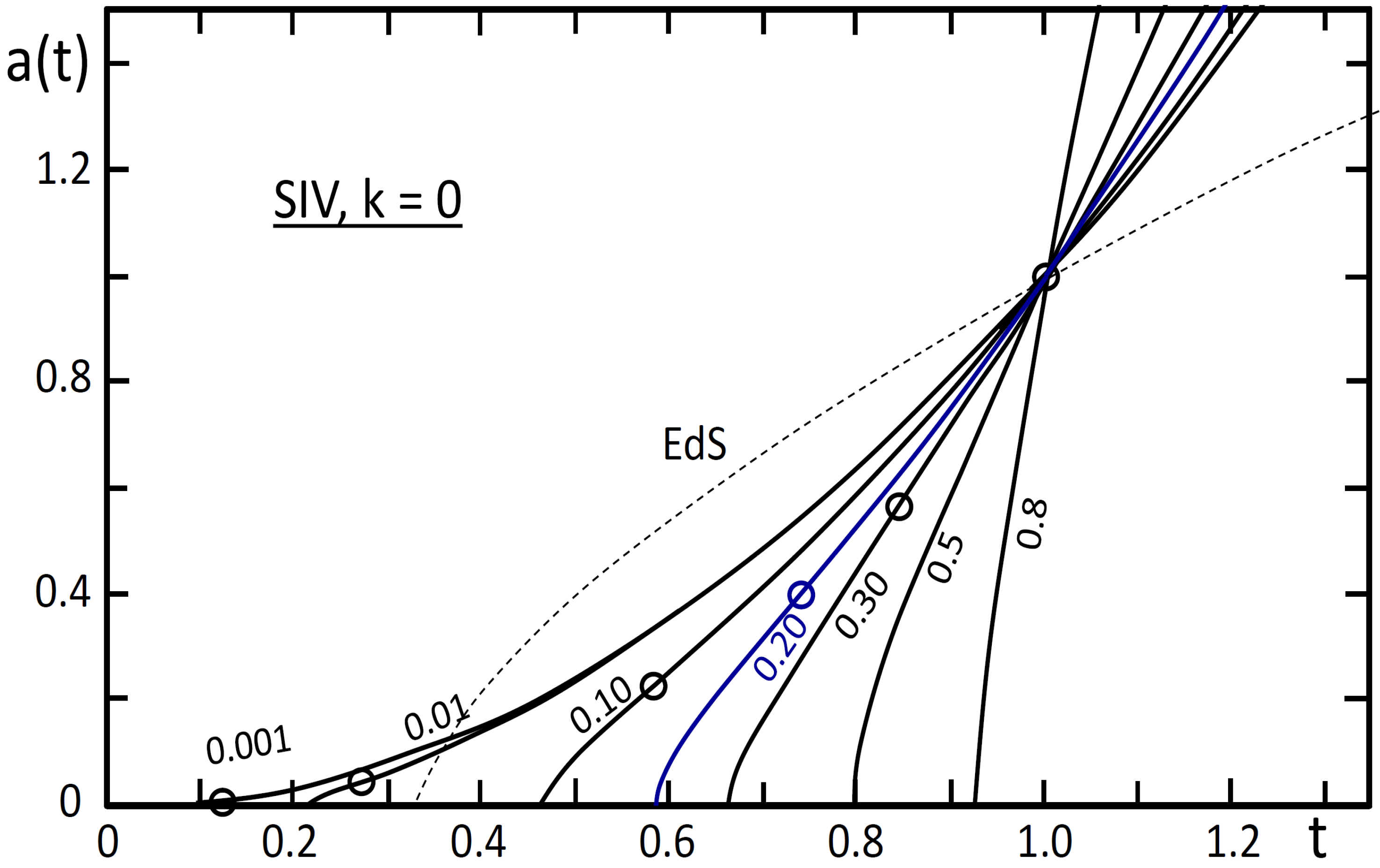
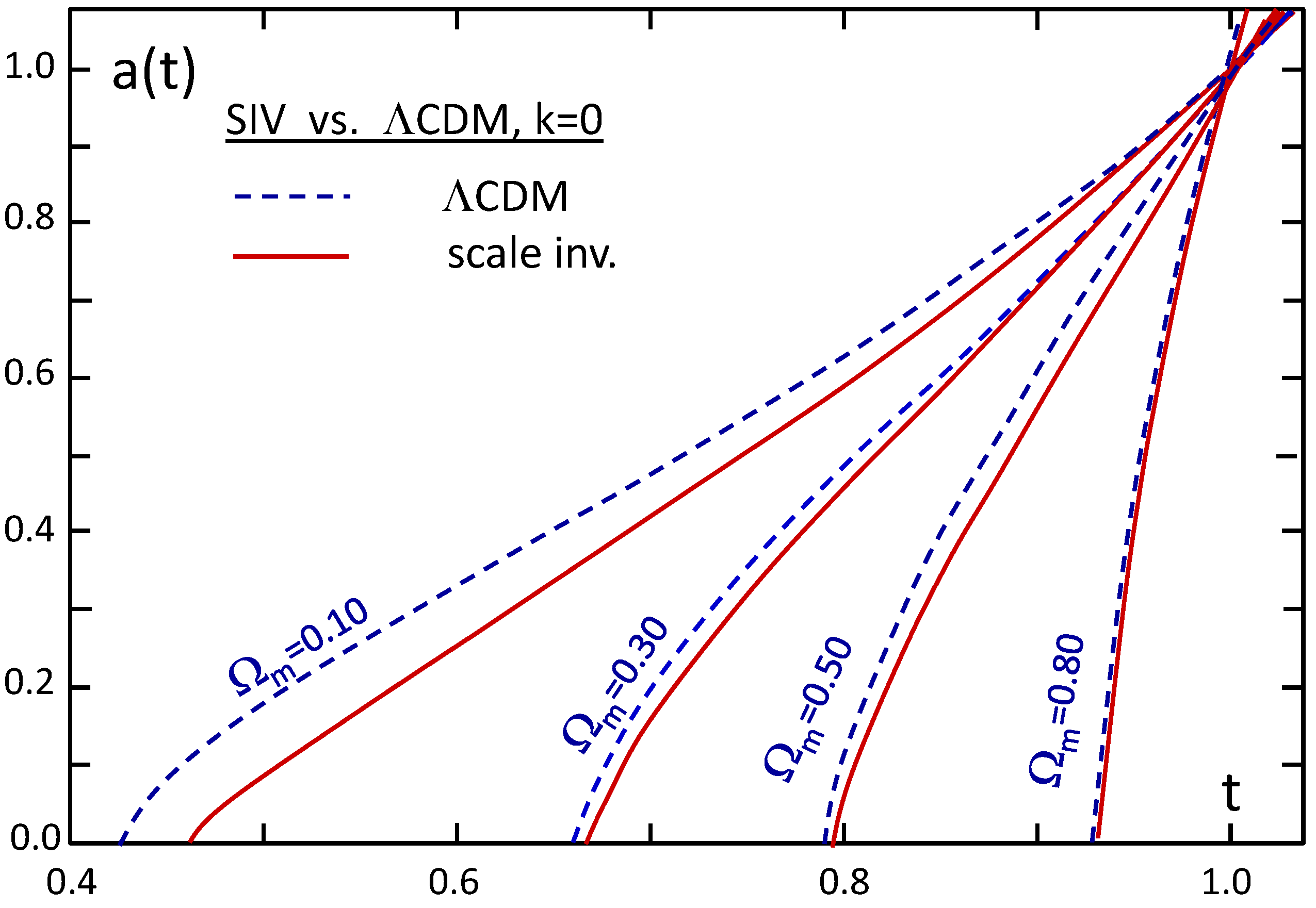
3.3. Results of Cosmological Models
4. Relation between the Hubble Constant and the Age of the Universe in the SIV Cosmology
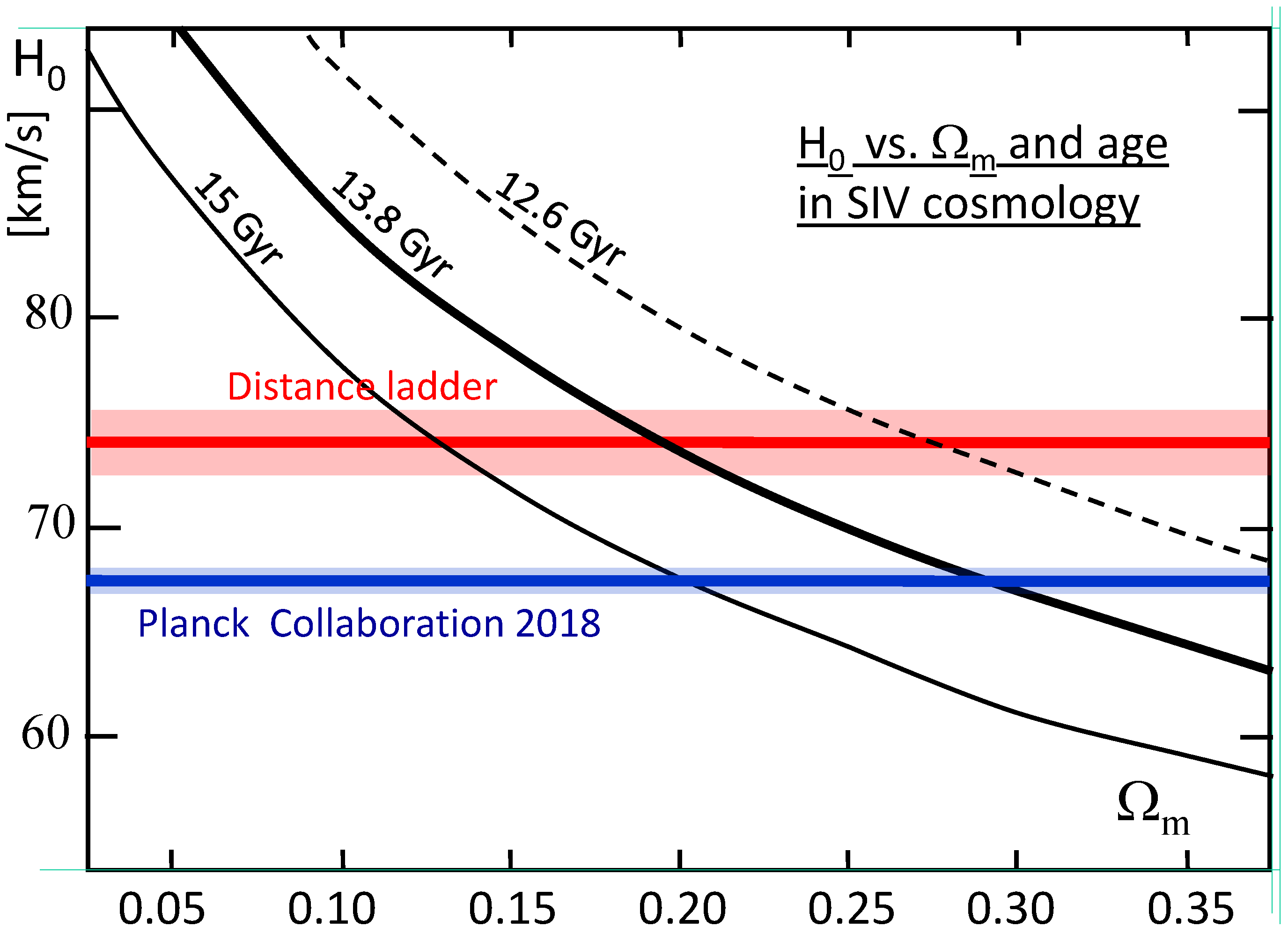
4.1. Theoretical Predictions
4.2. Comparisons with Observational Data
5. Basic Cosmological Tests of the SIV Cosmology
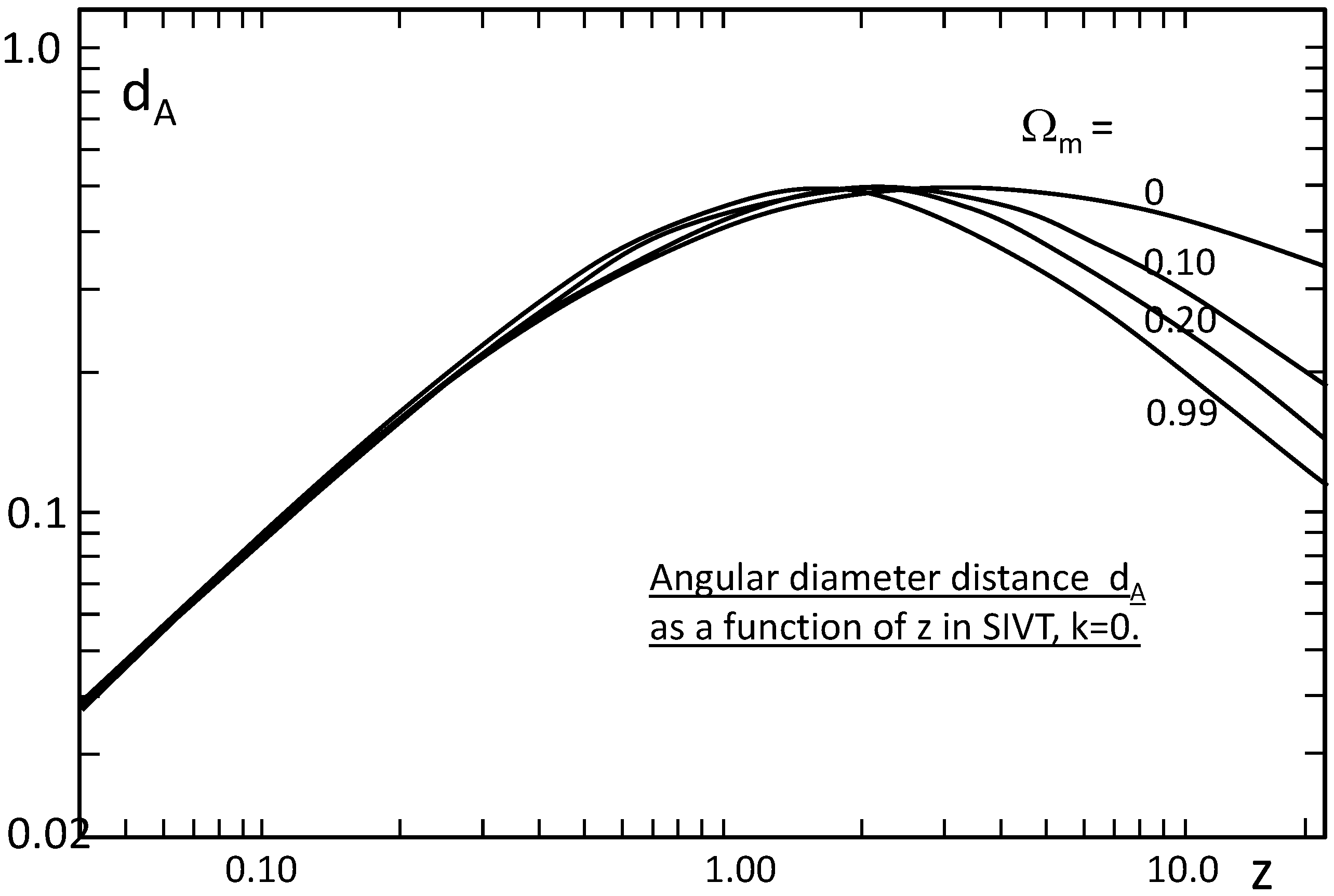
5.1. Distances
5.2. The Magnitude-Redshift Diagram of Distant Standard Candles
5.3. The Past Expansion Rates H(z) versus Redshift
5.4. The Redshift Drift in the SIV Theory
6. Properties and Tests in the Newton Like Approximation
6.1. The Basic Dynamical Equation and the Two-Body Problem
6.2. Tests on Stellar Dynamics and Galaxies
6.2.1. Clusters of Galaxies
6.2.2. Galaxy Rotation and the RAR
6.2.3. The Vertical Dispersion of Stellar Velocities in the Galaxy
6.3. The Growth of the Density Fluctuations
7. Conclusions and Perspectives
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. The Integrable Weyl Geometry
References
- Bertone, G.; Hooper, D. Dark-Matter History: How dark matter became a particle. CERN Courier 2017, 57, 27. [Google Scholar]
- De Swart, J.G.; Bertone, G.; Hooper, D. How dark matter came to matter. Nat. Astronomy 2017, 1, 0059. [Google Scholar] [CrossRef]
- Milgrom, M. A modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. Astrophys. J. 1983, 270, 365. [Google Scholar] [CrossRef]
- Milgrom, M. The Mond Limit from Spacetime Scale Invariance. Astrophys. J. 2009, 698, 1630. [Google Scholar] [CrossRef]
- Milgrom, M. MOND in galaxy groups: A superior sample. Phys. Rev. D 2019, 99, 044041. [Google Scholar] [CrossRef]
- Famaey, B.; McGaugh, S.S. Modified Newtonian Dynamics (MOND): Observational Phenomenology and Relativistic Extensions. Living Rev. Relativ. 2012, 15, 10. [Google Scholar] [CrossRef] [PubMed]
- Kroupa, P. The Dark Matter Crisis: Falsification of the Current Standard Model of Cosmology. Publ. Astron. Soc. Aust. 2012, 29, 395. [Google Scholar] [CrossRef]
- Kroupa, P. Galaxies as simple dynamical systems: Observational data disfavor dark matter and stochastic star formation. Can. J. Phys. 2015, 93, 169. [Google Scholar] [CrossRef]
- McGaugh, S.S.; Lelli, F.; Schombert, J.M. Radial Acceleration Relation in Rotationally Supported Galaxies. Phys. Rev. L 2016, 117, 201101. [Google Scholar] [CrossRef]
- Dirac, P.A.M. Long Range Forces and Broken Symmetries. Proc. R. Soc. Lond. Ser. A 1973, 333, 403. [Google Scholar]
- Weyl, H. Raum, Zeit, Materie. Vorlesungen Über Allgemeine Relativitätstheorie; Springer: Berlin, Germany, 1970. [Google Scholar]
- Canuto, V.; Adams, P.J.; Hsieh, S.-H.; Tsiang, E. Scale-covariant theory of gravitation and astrophysical applications. PHys. Rev. D 1977, 16, 1643. [Google Scholar] [CrossRef]
- Feynman, R.P. Mainly Mechanics, Radiation, and Heat Feynman Lectures on Physics; Addison-Wesley: Boston, MA, USA, 1963; Volume 1. [Google Scholar]
- Bondi, H. The cosmological scene 1945-1952 Modern Cosmology in Retrospect; Bertotti, B., Balbinot, R., Bergia, S., Eds.; Cambridge University Press: Cambridge, UK, 1990; p. 189. [Google Scholar]
- Maeder, A. An Alternative to the LambdaCDM Model: The Case of Scale Invariance. Astrophys. J. 2017, 834, 194. [Google Scholar] [CrossRef]
- Maeder, A.; Gueorguiev, V.G. The growth of the density fluctuations in the scale-invariant vacuum theory. Phys. Dark Universe 2019, 25, 100315. [Google Scholar] [CrossRef]
- Maeder, A. Dynamical Effects of the Scale Invariance of the Empty Space: The Fall of Dark Matter? Astrophys. J. 2017, 849, 158. [Google Scholar] [CrossRef]
- Maeder, A.; Gueorguiev, V.G. Scale-invariant dynamics of galaxies, MOND, dark matter, and the dwarf spheroidals. Mon. Not. R. Astron. Soc. 2019, 492, 2698. [Google Scholar] [CrossRef]
- Betoule, M.; Kessler, R.; Guy, J.; Mosher, J.; Hardin, D.; Biswas, R.; Astier, P.; El-Hage, P.; Konig, M.; Kuhlmann, S.; et al. Improved cosmological constraints from a joint analysis of the SDSS-II and SNLS supernova samples. Astron. Astrophys. 2014, 568, A22. [Google Scholar] [CrossRef]
- Lusso, E.; Piedipalumbo, E.; Risaliti, G.; Paolillo, M.; Bisogni, S.; Nardini, E.; Amati, L. Tension with the flat LambdaCDM model from a high-redshift Hubble diagram of supernovae, quasars, and gamma-ray bursts. Astron. Astrophys. 2019, 628, L4. [Google Scholar] [CrossRef]
- de Jesus, J.F.; Gregorio, M.T.; Andrade-Oliveira, F.; Valentim, R.; Matos, C.A.O. Bayesian correction of H(z) data uncertainties. Mon. Not. R. Astron. Soc. 2018, 477, 2867. [Google Scholar] [CrossRef]
- Capozziello, S.; Stornaiolo, C. Space-Time Deformations as Extended Conformal Transformations. Int. J. Geom. Methods Mod. Phys. 2008, 5, 185. [Google Scholar] [CrossRef]
- Capozziello, S.; de Laurentis, M. Space-Time Deformations as Extended Conformal Transformations. Phys. Rep. 2011, 509, 167. [Google Scholar] [CrossRef]
- Bouvier, P.; Maeder, A. Consistency of Weyl’s Geometry as a Framework for Gravitation. Astrophys. Space Sci. 1978, 54, 497. [Google Scholar] [CrossRef]
- Meierovich, B. Towards the theory of the evolution of the Universe. Phys. Rev. D 2012, 85, 123544. [Google Scholar] [CrossRef]
- Eddington, A.S. The Mathematical Theory of Relativity; Chelsea Publ. Co.: New York, NY, USA, 1923; 270p. [Google Scholar]
- Carroll, S.M.; Press, W.H.; Turner, E.L. The cosmological constant. Ann. Rev. Astron. Astrophys. 1992, 30, 499. [Google Scholar] [CrossRef]
- De Sitter, W. Einstein’s theory of gravitation. Mon. Not. R. Astron. Soc. 1917, 78, 3. [Google Scholar] [CrossRef]
- Durrer, R.; Maartens, R. Dark energy and dark gravity: Theory overview. Gen. Relativ. Gravit. 2008, 40, 301. [Google Scholar] [CrossRef]
- Sahni, V.; Shafieloo, A.; Starobinsky, A.A. Model-independent Evidence for Dark Energy Evolution from Baryon Acoustic Oscillations. Asrophys. J. 2014, 793, L40. [Google Scholar] [CrossRef]
- Sola, J.; Gomez-Valent, A.; de Cruz Perez, J. Hints of Dynamical Vacuum Energy in the Expanding Universe. Asrophys. J. 2015, 811, L14. [Google Scholar] [CrossRef]
- Ding, X.; Biesiada, M.; Cao, S.; Li, Z.; Zhu, Z. Is There Evidence for Dark Energy Evolution? Astrophys. J. 2014, 803, L22. [Google Scholar] [CrossRef]
- Sola, J.; de Cruz Perez, J.; Gomez-Valent, A.; Nunes, R.C. Dynamical Vacuum against a rigid Cosmological Constant. arXiv 2016, arXiv:1606.00450v2. [Google Scholar]
- Maeder, A. Evolution of the early Universe in the scale invariant theory. arXiv 2019, arXiv:1902.10115. [Google Scholar]
- Englert, F. Nobel Lecture: The BEH mechanism and its scalar boson*. Rev. Mod. Phys. 2014, 86, 843. [Google Scholar] [CrossRef]
- Higgs, P.W. Nobel Lecture: Evading the Goldstone theorem. Rev. Mod. Phys. 2014, 86, 851. [Google Scholar] [CrossRef]
- Krizek, M.; Somer, L. Anthropic principle and local Hubble expansion. In Cosmology on Small Scales 2016; Krizek, M., Dumin, Y., Eds.; Institute of Mathematics: Prague, Czech Republic, 2016; p. 65. [Google Scholar]
- Ade, P.A.R.; Aghanim, N.; Armitage-Caplan, C.; Arnaud, M.; Ashdown, M.; Atrio-Barandela, F.; Aumont, J.; Baccigalupi, C.; Banday, A.J.; Barreiro, R.B.; et al. [Planck Collaboration] Planck 2013 results. XVI. Cosmological parameters. Astron. Astrophys. 2014, 571, A16. [Google Scholar]
- de Jesus, J.F. Exact solution for flat scale-invariant cosmology. Rev. Mex. Astron. Astrophys. 2018, 55, 17. [Google Scholar] [CrossRef]
- Frieman, J.A.; Turner, M.S.; Huterer, D. Dark energy and the accelerating universe. Ann. Rev. Astron. Astrophys. 2008, 46, 385. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.M.; Scolnic, D. Large Magellanic Cloud Cepheid Standards Provide a 1% Foundation for the Determination of the Hubble Constant and Stronger Evidence for Physics beyond LambdaCDM. Astrophys. J. 2019, 876, 85. [Google Scholar] [CrossRef]
- Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. [Planck Collaboration] Planck 2018 results. VI. Cosmological parameters. arXiv 2018, arXiv:1807.06209. [Google Scholar]
- Freedman, W.L.; Madore, B.F. The Hubble Constant. Ann. Rev. Astron. Astrophys. 2010, 48, 673. [Google Scholar] [CrossRef]
- Riess, A.G.; Macri, L.M.; Hoffmann, L.M. A 2.4% Determination of the Local Value of the Hubble Constant. Astrophys. J. 2016, 826, 56. [Google Scholar] [CrossRef]
- Tully, B.R.; Courtois, H.M.; Sorce, J.H. Cosmicflows-3. Astron. J. 2016, 152, 50. [Google Scholar] [CrossRef]
- Shajib, A.J.; Birrer, S.; Treu, T.; Agnello, A.; Buckley-Geer, E.J.; Chan, J.H.H.; Christensen, L.; Lemon, C.; Lin, H.; Millon, M.; et al. STRIDES: A 3.9 per cent measurement of the Hubble constant from the strong lens system DES J0408-5354. arXiv 2019, arXiv:1910.06306. [Google Scholar]
- Chen, G.C.-F.; Fassnacht, C.D.; Suyu, S.H.; Rusu, C.E.; Chan, J.H.H.; Auger, M.W.; Hilbert, S.; Bonvin, V.; Birrer, S.; Millon, M.; et al. A SHARP view of H0LiCOW: H 0 from three time-delay gravitational lens systems with adaptive optics imaging. arXiv 2019, arXiv:1907.02533. [Google Scholar] [CrossRef]
- Aubourg, E.; Bailey, S.; Bautista, J.E.; Beutler, F.; Bhardwaj, V.; Bizyaev, D.; Blanton, M.; Blomqvist, M.; Bolton, A.S.; Bovy, J.; et al. Cosmological implications of baryon acoustic oscillation measurements. Phys. Rev. D 2015, 92, 123516. [Google Scholar] [CrossRef]
- L’Huillier, B.; Shafieloo, A. Model-independent test of the FLRW metric, the flatness of the Universe, and non-local estimation of H0 rd. JCAP 2017, 1, 15L. [Google Scholar] [CrossRef]
- VandenBerg, D.A.; Bond, H.E.; Nelan, E.P.; Nissen, P.E.; Schaefer, G.H.; Harmer, D. Three Ancient Halo Subgiants: Precise Parallaxes, Compositions, Ages, and Implications for Globular Clusters. Astrophys. J. 2014, 792, 110. [Google Scholar] [CrossRef][Green Version]
- Roukema, B.F.; Mourier, P.; Buchert, T.; Ostrowski, J.J. The background Friedmannian Hubble constant in relativistic inhomogeneous cosmology and the age of the Universe. Astron. Astrophys. 2018, 598, A111. [Google Scholar] [CrossRef]
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Castro, P.G.; Deustua, S.; Fabbro, S.; Goobar, A.; Groom, D.E.; et al. Measurements of Omega and Lambda from 42 High-Redshift Supernovae. Astrophys. J. 1999, 517, 565. [Google Scholar] [CrossRef]
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational Evidence from Supernovae for an Accelerating Universe and a Cosmological Constant. Astron. J. 1998, 116, 1009. [Google Scholar] [CrossRef]
- Scolnic, D.M.; Jones, D.O.; Rest, A.; Pan, Y.C.; Chornock, R.; Foley, R.J.; Huber, M.E.; Kessler, R.; Narayan, G.; Riess, A.G.; et al. The Complete Light-curve Sample of Spectroscopically Confirmed SNe Ia from Pan-STARRS1 and Cosmological Constraints from the Combined Pantheon Sample. Astrophys. J. 2018, 859, 101. [Google Scholar] [CrossRef]
- Risaliti, G.; Lusso, E. Cosmological constraints from the Hubble diagram of quasars at high redshifts. Nat. Astron. 2019, 3, 272. [Google Scholar] [CrossRef]
- Demianski, M.; Piedipalumbo, E.; Sawant, D.; Amati, L. Cosmology with gamma-ray bursts - II. Cosmography challenges and cosmological scenarios for the accelerated Universe. Astron. Astrophys. 2017, 598, A112. [Google Scholar] [CrossRef]
- Jimenez, R.; Loeb, A. Constraining Cosmological Parameters Based on Relative Galaxy Ages. Astrophys. J. 2002, 573, 37. [Google Scholar]
- Moresco, M.; Cimatti, A.; Jimenez, R.; Pozzetti, L.; Zamorani, G.; Bolzonella, M.; Dunlop, J.; Lamareille, F.; Mignoli, M.; Pearce, H.; et al. Improved constraints on the expansion rate of the Universe up to z 1.1 from the spectroscopic evolution of cosmic chronometers. J. Cosmol. Astropart. Phys. 2012, 8, 6. [Google Scholar] [CrossRef]
- Moresco, M. Raising the bar: New constraints on the Hubble parameter with cosmic chronometers at z 2. Mon. Not. R. Astron. Soc. 2015, 450, L16. [Google Scholar] [CrossRef]
- Moresco, M.; Pozzetti, L.; Cimatti, A.; Jimenez, R.; Maraston, C.; Verde, L.; Thomas, D.; Citro, A.; Tojeiro, R.; Wilkinson, D. A 6% measurement of the Hubble parameter at z 0.45: Direct evidence of the epoch of cosmic re-acceleration. J. Cosmol. Astropart. Phys. 2016, 5, 14. [Google Scholar] [CrossRef]
- Simon, J.; Verde, L.; Jimenez, R. Constraints on the redshift dependence of the dark energy potential. Phys. Rev. D 2005, 71, 123001. [Google Scholar] [CrossRef]
- Stern, D.; Jimenez, R.; Verde, L.; Kamionkowski, M.; Stanford, S.A. Cosmic chronometers: Constraining the equation of state of dark energy. I: H(z) measurements. J. Cosmol. Astropart. Phys. 2010, 2, 8. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, H.; Yuan, S.; Liu, S.; Zhang, T.-J.; Sun, Y.-C. Four new observational H(z) data from luminous red galaxies in the Sloan Digital Sky Survey data release seven. Res. Astron. Astrophys. 2014, 14, 1221. [Google Scholar] [CrossRef]
- Cole, S.; Percival, W.J.; Peacock, J.A.; Norberg, P.; Baugh, C.M.; Frenk, C.S.; Baldry, I.; Bland-Hawthorn, J.; Bridges, T.; Cannon, R.; et al. The 2dF Galaxy Redshift Survey: Power-spectrum analysis of the final data set and cosmological implications. Mon. Not. R. Astron. Soc. 2005, 362, 505. [Google Scholar] [CrossRef]
- Eisenstein, D.J.; Zehavi, I.; Hogg, D.W.; Scoccimarro, R.; Blanton, M.R.; Nichol, R.C.; Scranton, R.; Seo, H.-J.; Tegmark, M.; Zheng, Z.; et al. Detection of the Baryon Acoustic Peak in the Large-Scale Correlation Function of SDSS Luminous Red Galaxies. Astrophys. J. 2005, 633, 560. [Google Scholar] [CrossRef]
- Blomqvist, M.; du Mas des Bourboux, H.; Busca, N.G.; de Sainte Agathe, V.; Rich, J.; Balland, C.; Bautista, J.E.; Dawson, K.; Font-Ribera, A.; Guy, J.; et al. Baryon acoustic oscillations from the cross-correlation of Ly-alpha absorption and quasars in eBOSS DR14. Astron. Astrophys. 2019, 629, A86. [Google Scholar] [CrossRef]
- Delubac, T.; Bautista, J.E.; Busca, N.G.; Rich, J.; Kirkby, D.; Bailey, S.; Font-Ribera, A.; Slosar, A.; Lee, K.-G.; Pieri, M.M.; et al. Baryon acoustic oscillations in the Ly-alpha forest of BOSS DR11 quasars. Astron. Astrophys. 2015, 574, A59. [Google Scholar] [CrossRef]
- Font-Ribera, A.; Kirkby, D.; Busca, N.J. Quasar-Lyman alpha forest cross-correlation from BOSS DR11: Baryon Acoustic Oscillations. Cosmol. Astropart. Phys. 2014, 5, 27. [Google Scholar] [CrossRef]
- Ryan, J.; Doshi, S.; Ratra, B. Constraints on dark energy dynamics and spatial curvature from Hubble parameter and baryon acoustic oscillation data. Mon. Not. R. Astron. Soc. 2018, 480, 759. [Google Scholar] [CrossRef]
- Ryan, J.; Chen, Y.; Ratra, B. Baryon acoustic oscillation, Hubble parameter, and angular size measurement constraints on the Hubble constant, dark energy dynamics, and spatial curvature. Mon. Not. R. Astron. Soc. 2018, 488, 3844. [Google Scholar] [CrossRef]
- Sola, J.; Gomez-Valent, A.; de Cruz Perez, J. First Evidence of Running Cosmic Vacuum: Challenging the Concordance Model. Asrophys. J. 2017, 836, 43. [Google Scholar] [CrossRef]
- Pepe, F.A.; Cristiani, S.; Rebolo, L.R.; Santos, N.C.; Amorim, A.; Avila, G.; Benz, W.; Bonifacio, P.; Cabral, A.; Carvas, P.; et al. ESPRESSO: The Echelle spectrograph for rocky exoplanets and stable spectroscopic observations. Proc. SPIE 2010, 7735, 77350F. [Google Scholar]
- McVittie, G.C. Appendix to The Change of Redshift and Apparent Luminosity of Galaxies due to the Deceleration of Selected Expanding Universes. Astrophys. J. 1962, 136, 334. [Google Scholar]
- Liske, J.; Grazian, A.; Vanzella, E.; Dessauges, M.; Viel, M.; Pasquini, L.; Haehnelt, M.; Cristiani, S.; Pepe, F.; Avila, G.; et al. Cosmic dynamics in the era of Extremely Large Telescopes. Mon. Not. R. Astron. Soc. 2008, 386, 1192. [Google Scholar] [CrossRef]
- Maeder, A.; Bouvier, P. Scale invariance, metrical connection and the motions of astronomical bodies. Astron. Astrophys. 1979, 73, 82. [Google Scholar]
- Proctor, R.N.; Mendes de Oliveira, C.; Azanha, L.; Dupke, R.; Overzier, R. A derivation of masses and total luminosities of galaxy groups and clusters in the maxBCG catalogue. Mon. Not. R. Astron. Soc. 2015, 449, 234. [Google Scholar] [CrossRef]
- Sofue, Y.; Rubin, V. Rotation Curves of Spiral Galaxies. Ann. Rev. Astron. Astrophys. 2001, 39, 137. [Google Scholar] [CrossRef]
- Lelli, F.; McGaugh, S.S.; Schombert, J.M.; Pawlowski, M.S. One Law to Rule Them All: The Radial Acceleration Relation of Galaxies. Astrophys. J. 2017, 836, 152. [Google Scholar] [CrossRef]
- Dutton, A.A.; Maccio, A.V.; Obrera, A.; Buck, T. NIHAO - XVIII. Origin of the MOND phenomenology of galactic rotation curves in a Lambda CDM universe. Mon. Not. R. Astron. Soc. 2019, 485, 1886. [Google Scholar] [CrossRef]
- Milgrom, M. MOND impact on and of the recently updated mass-discrepancy-acceleration relation. arXiv 2016, arXiv:1609.06642. [Google Scholar]
- Banik, I.; Kroupa, P. Title not communicated. Mon. Not. R. Astron. Soc. 2020. in prep. [Google Scholar]
- Hees, A.; Folkner, W.M.; Jacobson, R.A.; Park, R.S. Constraints on modified Newtonian dynamics theories from radio tracking data of the Cassini spacecraft. Phys. Rev. D 2014, 89, 102002. [Google Scholar] [CrossRef]
- Meierovich, B. Galaxy rotation curves driven by massive vector fields: Key to the theory of the dark sector. Phys. Rev. D 2013, 87, 103510. [Google Scholar] [CrossRef]
- Meierovich, B. Static State of a Black Hole Supported by Dark Matter. Universe 2019, 5, 198. [Google Scholar] [CrossRef]
- Seabroke, G.M.; Gilmore, G. Revisiting the relations: Galactic thin disc age-velocity dispersion relation. Mon. Not. R. Astron. Soc. 2007, 380, 1348. [Google Scholar] [CrossRef]
- Spitzer, L.; Schwarzschild, M. The Possible Influence of Interstellar Clouds on Stellar Velocities. Astrophys. J. 1951, 118, 106. [Google Scholar] [CrossRef]
- Kumamoto, J.; Baba, J.; Saitoh, T.R. Imprints of zero-age velocity dispersions and dynamical heating on the age-velocity dispersion relation. Publ. Astron. Soc. Jpn. 2017, 69, 32. [Google Scholar] [CrossRef]
- Magnenat, P.; Martinet, L.; Maeder, A. The age dependence of stellar velocity dispersion in a scale-covariant (theory of) gravitation. Astron. Astrophys. 1978, 67, 51. [Google Scholar]
- Nordstrom, B.; Mayor, M.; Andersen, J.; Holmberg, J.; Pont, F.; Jorgensen, B.R.; Olsen, E.H.; Udry, S.; Mowlavi, N. The Geneva-Copenhagen survey of the Solar neighbourhood. Ages, metallicities, and kinematic properties of 14 000 F and G dwarfs. Astron. Astrophys. 2004, 418, 989. [Google Scholar] [CrossRef]
- Coles, P.; Lucchin, F. Cosmology. The Origin and Evolution of Cosmic Structure; Wiley & Sons Ltd.: Chichester, UK, 2002; 512p. [Google Scholar]
- Peebles, P.J.E. The Large-Scale Structure of the Universe; Princeton Univ. Press: Princeton, NJ, USA, 1980; 440p. [Google Scholar]
- Ostriker, J.P. Astronomical tests of the cold dark matter scenario. Ann. Rev. Astron. Astrophys. 1993, 31, 689. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. Simulations of X-ray clusters. Mon. Not. R. Astron. Soc. 1995, 275, 720. [Google Scholar] [CrossRef]
- Hashimoto, T.; Laporte, N.; Mawatari, K.; Ellis, R.S.; Inoue, A.K.; Zackrisson, E.; Roberts-Borsani, G.; Zheng, W.; Tamura, Y.; Bauer, F.E.; et al. The onset of star formation 250 million years after the Big Bang. Nature 2018, 557, 392. [Google Scholar] [CrossRef]
| 1. | We use the French spelling for metric connection to emphasize its relation to is construction within the Integrable Weyl Geometry (IWG) framework since within the standard Einstein GR such fields are zero by construction. |
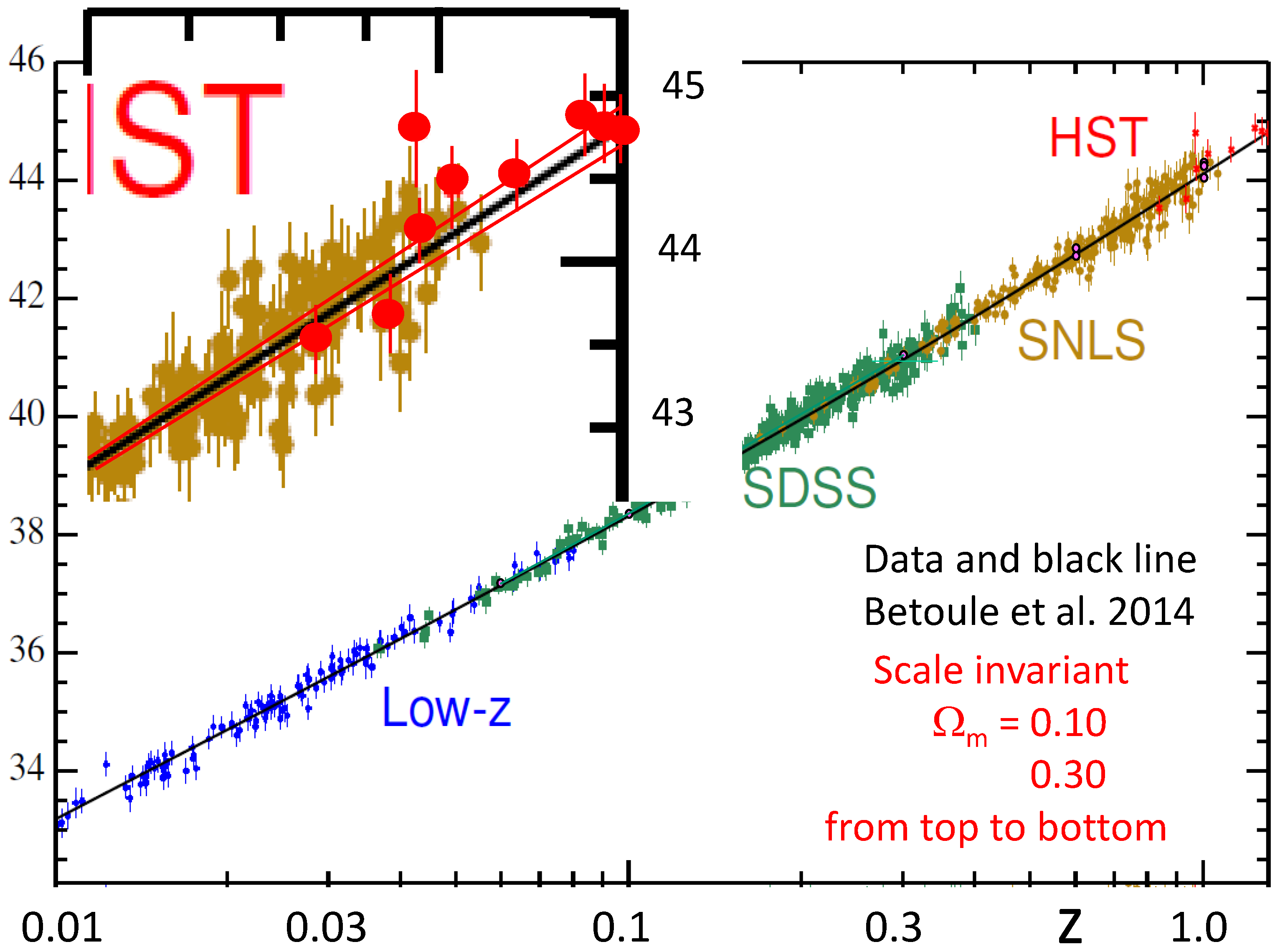
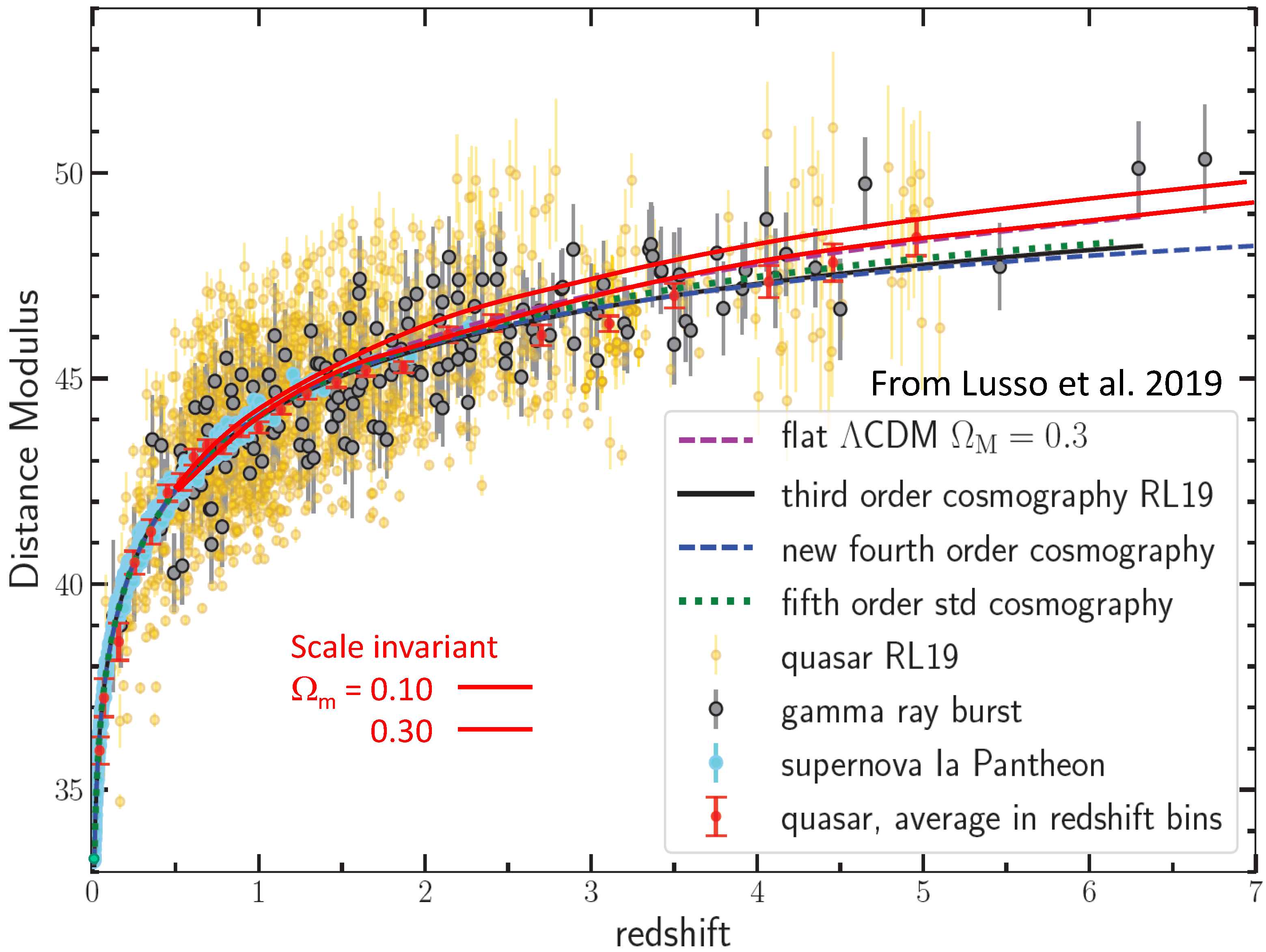
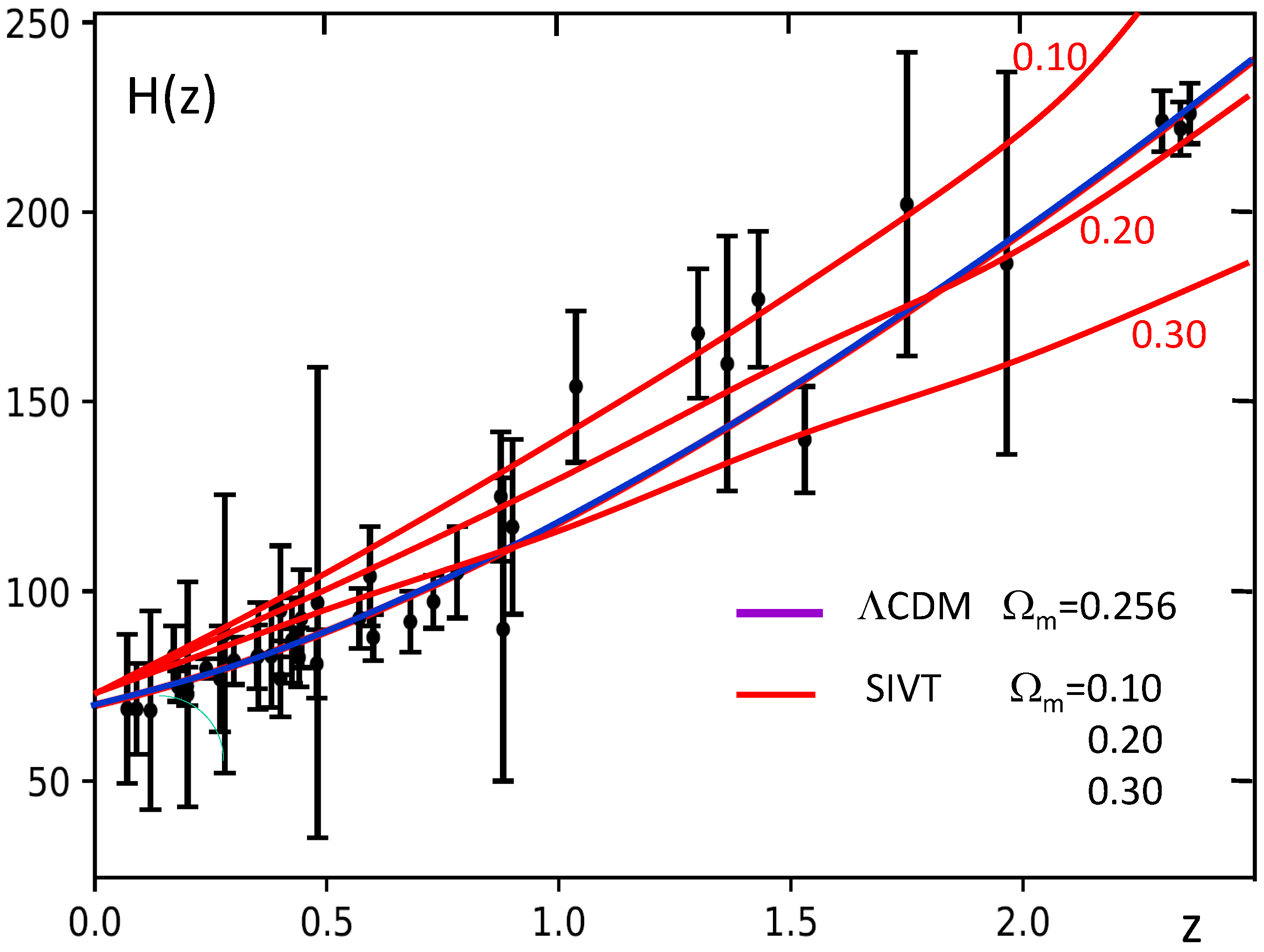

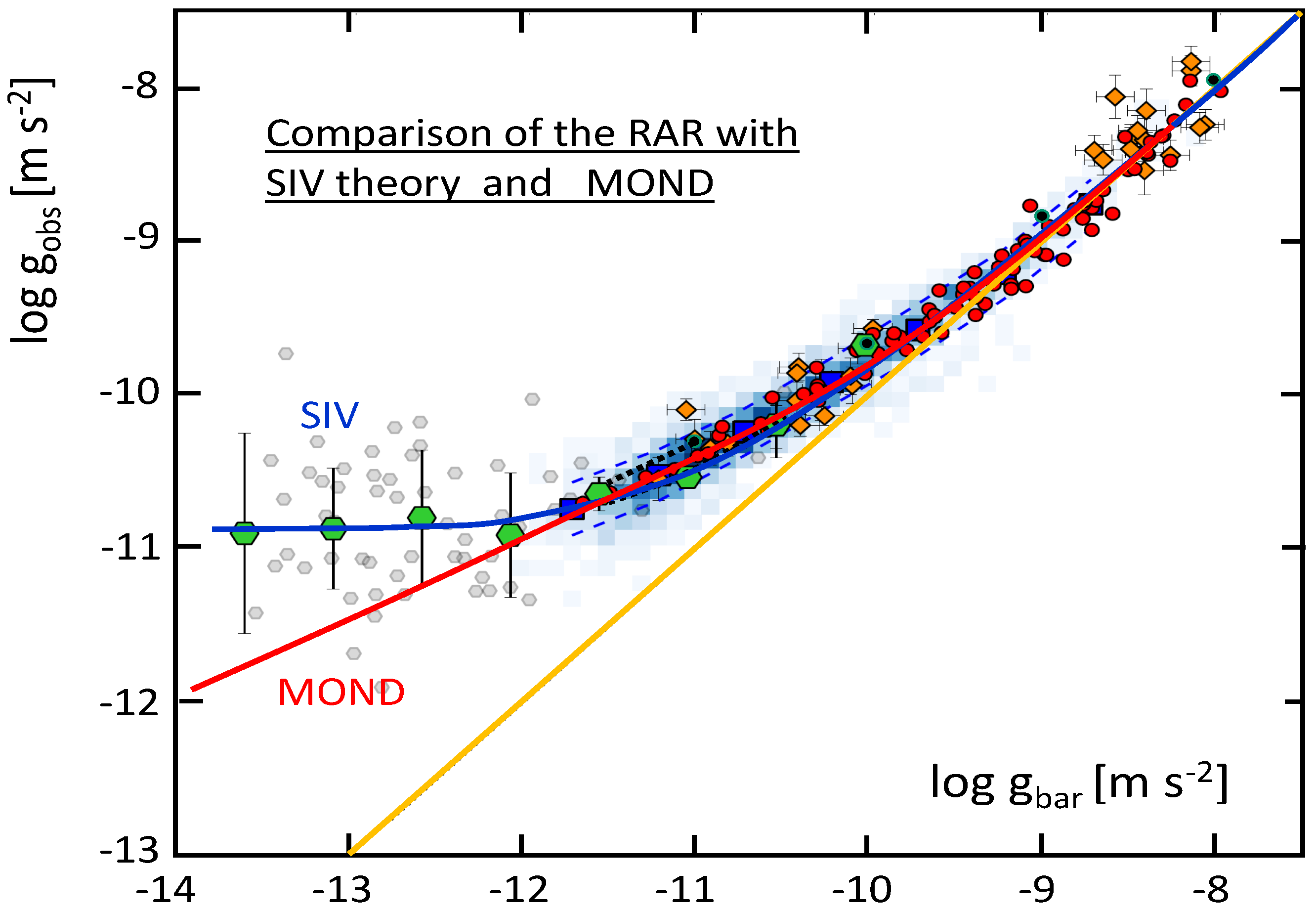


| z | |||||
|---|---|---|---|---|---|
| 0.10 | 38.35 | 38.35 | 38.34 | 38.32 | 38.31 |
| 0.30 | 40.99 | 40.98 | 40.95 | 40.92 | 40.89 |
| 0.70 | 43.23 | 43.20 | 43.13 | 43.06 | 43.00 |
| 1.00 | 44.24 | 44.19 | 44.09 | 44.00 | 43.92 |
| 2.00 | 46.30 | 46.20 | 46.02 | 45.88 | 45.75 |
| 3.00 | 47.54 | 47.40 | 47.16 | 46.98 | 46.83 |
| 4.00 | 48.43 | 48.24 | 47.97 | 47.76 | 47.58 |
| 5.00 | 49.11 | 48.89 | 48.58 | 48.35 | 48.16 |
| 6.00 | 49.66 | 49.42 | 49.08 | 48.83 | 48.63 |
| 7.00 | 50.13 | 49.86 | 49.49 | 49.23 | 49.03 |
| 8.00 | 50.52 | 50.23 | 49.85 | 49.58 | 49.37 |
| 9.00 | 50.87 | 50.56 | 50.16 | 49.88 | 49.66 |
| 10.0 | 51.18 | 50.85 | 50.44 | 50.15 | 49.93 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maeder, A.; Gueorguiev, V.G. The Scale-Invariant Vacuum (SIV) Theory: A Possible Origin of Dark Matter and Dark Energy. Universe 2020, 6, 46. https://doi.org/10.3390/universe6030046
Maeder A, Gueorguiev VG. The Scale-Invariant Vacuum (SIV) Theory: A Possible Origin of Dark Matter and Dark Energy. Universe. 2020; 6(3):46. https://doi.org/10.3390/universe6030046
Chicago/Turabian StyleMaeder, Andre, and Vesselin G. Gueorguiev. 2020. "The Scale-Invariant Vacuum (SIV) Theory: A Possible Origin of Dark Matter and Dark Energy" Universe 6, no. 3: 46. https://doi.org/10.3390/universe6030046
APA StyleMaeder, A., & Gueorguiev, V. G. (2020). The Scale-Invariant Vacuum (SIV) Theory: A Possible Origin of Dark Matter and Dark Energy. Universe, 6(3), 46. https://doi.org/10.3390/universe6030046






