Gravitational Interaction of Cosmic String with Spinless Particle

Abstract

We consider the gravitational interaction of spinless relativistic particle and infinitely thin cosmic string within the classical linearized-theory framework. We compute the particle’s motion in the transverse (to the unperturbed string) plane. The reciprocal action of the particle on the cosmic string is also investigated. We derive the retarded solution which includes the longitudinal (with respect to the unperturbed-particle motion) and totally-transverse string perturbations.

1. Introduction

Over recent decades, scientific interest has shifted from the microscopic theory to the problems of the Universe’s global evolution. Some cosmological scenarios, which pretended to be the proper description of evolution in the past, were proposed. One might say that at small length scales, General Relativity works well, while the gravitation on the cosmic scales is of basic interest.

The most of contemporary theories of the Universe’s evolution imply inflation at early stages [1,2]. In addition, the spontaneous symmetry breaking was proposed to be accompanied with phase transitions [3,4,5], where some topological defects may be created. The cosmic string is an example of topological defects, which, being appeared in the phase transitions of the Early Universe, may well survive during the evolution [6,7].

The standard problems of research within the theory of cosmic strings are related with the field effects of the curved background (vacuum polarization, self-action, gravitational radiation [8,9] etc.) and with the dynamics of the strings themselves. This work is devoted to the consideration of the elastic encounter of spinless particles with cosmic strings and thus involves both these problems.

In the literature it was considered the interaction of cosmic strings [10,11,12,13], including the self-action [14,15] the propagation of strings in the expanding Universe ([6,7], and refs. therein), the scattering on the string [16], etc. For the scattering problem, the back-effect of the particle on the string was neglected, assuming the smallness of the effect. Amongst the variants of mutual location of the string and particle’s trajectory, we consider the particle’s scattering in the transverse-to-string plane, with finite impact parameter b.

The curved space generated by the string aligned with the cartesian x-axis, represents the ultra-static spacetime, whose metric in cylindrical coordinates takes the form

$$\begin{array}{c}\hfill d{s}^2=d{t}^2-d{x}^2-d{\varrho }^2-{\beta }^2{\varrho }^{2}d{\phi }^2,\end{array}$$

(1)

where $0<\beta <1$. The quantity $\delta \phi =2\pi (1-\beta )$ defines the angular deficit [17]. It is proposed to be extremely small (for $\eta ={\eta }_{\mathrm{GUT}}\sim {10}^{16}\mathrm{GeV}$ it is of order ${10}^{-5}$) [18,19]. Hence we introduce the complement

$$\beta {}^{\prime }\equiv 1-\beta =\frac{\delta \phi }{2\pi },$$

which plays the role of the relative angle deficit.

For description of the scattered particle we shall introduce the cartesian coordinates. The radial variable change $\varrho \to r$ according to relation $\beta \varrho =R_0{(r/R_0)}^{\beta },$ where $R_0$ is an arbitrary lengthy scale, allows to reduce the metric (1) into conformally-Euclidean form (on the hyperplane $x=\mathrm{const}$):

$$\begin{array}{cc}\hfill & d{s}^2=d{t}^2-d{x}^2-{\mathrm{e}}^{-2(1-\beta )ln(r/R_0)}(d{y}^2+d{z}^2),\hfill \end{array}$$

(2)

where $r^2=y^2+z^2$.

In a series of works [16,20,21] the strings with finite width were of consideration. However, practically, the width’s contribution into the effects under our interest is insufficient. Indeed, the string’s tension (linear energy density) is constant in the thin-string limit, the exterior metric coincides with the conical metric (1), while the string’s width is estimated as ${\eta }^{-1}$ [20]. For $\eta ={\eta }_{\mathrm{GUT}}$ the string’s diameter is of order $d\sim {10}^{-29}\mathrm{cm}$, which is much less than impact parameters supposed for the scattering. Therefore we consider infinitely-thin cosmic string.

The paper is organized as follows: after the introduction, in the Section 2 we consider the setup of the gravitational-interaction problem for the particle-string system and introduce the iterational scheme to determine the string’s and particle’s dynamics to the first order. The particle’s motion is computed. In the Section 3 we consider the cosmic-string’s dynamics, the retarded solutions to the string’s equations-of-motion will be found. In the Section 4 we analyze that string’s excitation which is transverse to the particle’s trajectory. The Section 5 is devoted to the qualitative estimates of the restrictions related with the Perturbation Theory under usage. That string’s excitation, which is transverse to the string but longitudinal with respect to the particle’s trajectory, is investigated in detail. Finally, in the conclusion, we will discuss the results and point out some prospects of the presented work.

We use $\hslash =c=1$ units and the spacetime metric $g_{\mu \nu }$ with signature $(+---)$. The Greek indices $\mu ,\nu ,\cdots$ run over values 0, 1, 2, 3. The Riemann and Ricci tensors are defined as $R^{\mu }{}_{\nu \lambda \rho }\equiv {\partial }_{\lambda }{\mathrm{\Gamma }}_{\nu \rho }^{\mu }-\cdots$, $R_{\mu \nu }\equiv R^{\lambda }{}_{\mu \lambda \nu }.$

2. Setup

The pointlike spinless particle with mass m moves across the woldline with coordinates $Z^{\mu }\left(s\right)$ parametrized by the affine parameter s. The motion is described by the Polyakov’s action with the einbein $e\left(s\right)$; ${\dot{Z}}^{\mu }\equiv \partial Z^{\mu }/\partial s$ stands for the tangent vector to the worldline. The string propagates by its worldsheet ${\mathcal{V}}_2\subset {\mathcal{M}}_4$ with inner coordinates ${\sigma }^a=(\tau ,\sigma )$, which define the induced metric ${\gamma }_{ab}$ with signature ($+-$). Particle and string interact by gravity by with no cosmological constant.

Full action for the interacting particle-string system reads

$$\begin{array}{cc}\hfill S=-& \frac{\mu }{2}\int X_a^{\mu }{X}_b^{\nu }{g}_{\mu \nu }{\gamma }^{ab}\sqrt{\tilde{\gamma }}{d}^2\sigma -\frac{1}{2}\int \left(e{g}_{\mu \nu }{\dot{Z}}^{\mu }{\dot{Z}}^{\nu }+\frac{m^2}{e}\right)ds-\frac{1}{{\varkappa }^2}\int R\sqrt{\left|g\right|}{d}^{4}x.\hfill \end{array}$$

(3)

Here $\mu$ is the string tension, ${\displaystyle X^{\mu }}$—the worldsheet embedding coordinates, ${\displaystyle X_a^{\mu }\equiv \partial X^{\mu }/\partial {\sigma }^a}$—tangent vectors to the worldsheet, ${\gamma }^{ab}$—reverse metric on ${\mathcal{V}}_2$. Also ${\displaystyle \tilde{\gamma }\equiv |det\parallel {\gamma }_{ab}\parallel |}$ and ${\varkappa }^2=16$$\pi$ G. The string tension is related with the angular deficit as $\beta {}^{\prime }=4$ G$\mathsf{\mu }$.

Varying (3) over ${\displaystyle X^{\mu }}$, one obtains the string’s equation of motion in covariant form:

$$\begin{array}{c}\hfill {\partial }_a\left(X_b^{\nu }{g}_{\mu \nu }{\gamma }^{ab}\sqrt{\tilde{\gamma }}\right)=\frac{1}{2}{g}_{\nu \lambda ,\mu }{X}_a^{\nu }{X}_b^{\lambda }{\gamma }^{ab}\sqrt{\tilde{\gamma }},\end{array}$$

(4)

while variation with respect to ${\gamma }^{ab}$ yields the constraint with solution ${\gamma }_{ab}$ as induced metric on ${\mathcal{V}}_2$: ${\gamma }_{ab}=X_a^{\mu }{X}_b^{\nu }{g}_{\mu \nu }{|}_{x=X}.$

Varying S over e and coordinates $Z^{\mu }$, we get the constraint and particle’s equation of motion:

$$\begin{array}{c}\hfill e^2{g}_{\mu \nu }{\dot{Z}}^{\mu }{\dot{Z}}^{\nu }=m^2\frac{d}{ds}\left(e{\dot{Z}}^{\nu }{g}_{\mu \nu }\right)=\frac{e}{2}{g}_{\nu \lambda ,\mu }{\dot{Z}}^{\nu }{\dot{Z}}^{\lambda },\end{array}$$

(5)

and, finally, the variation over the full metric is the Einstein field equation

$$\begin{array}{c}\hfill R^{\mu \nu }-\frac{R}{2}{g}^{\mu \nu }=\frac{1}{2}{\varkappa }^2\left[T^{\mu \nu }+{\overline{T}}^{\mu \nu }\right],\end{array}$$

(6)

where

$$\begin{array}{c}\hfill T^{\mu \nu }=\mu \int X_a^{\mu }{X}_b^{\nu }{\gamma }^{ab}{\delta }^4\left(x-X\left(\sigma \right)\right)\sqrt{\tilde{\gamma }}{d}^2\sigma ,{\overline{T}}^{\mu \nu }=e\int \frac{{\dot{Z}}^{\mu }{\dot{Z}}^{\nu }{\delta }^4\left(x-Z\left(s\right)\right)}{{\left(g_{\lambda \rho }{\dot{Z}}^{\lambda }{\dot{Z}}^{\rho }\right)}^{1/2}\sqrt{\left|g\right|}}ds\end{array}$$

represent the energy-momentum tensor of the string and the particle, respectively.

The string’s metric (2) and the Schwarzschild solution for spinless particle represent the exact solution of non-linear gravity separately. However, the exact solution of the full system of the ponderomotive Equation (6) seems to be hard to find analytically. Furthermore, if we look forward by one step and think about the gravitational radiation due to collision, the necessity of the usage of flat background arises. Thus we shall solve the problem with help of the Perturbation Theory over the gravitational constant (G or $\varkappa$), excluding self-action: $g_{\mu \nu }={\eta }_{\mu \nu }+\varkappa {\mathfrak{h}}_{\mu \nu }$.

To the zeroth order, we have free string (aligned with the x-axis) and free particle, which moves to the positive direction of the z-axis with velocity v with impact parameter b. The mutual perpendicular of the string and the particle’s trajectory (the impact-parameter vector) will be the y-axis; the intersection of x-, y- and z-axes will be the coordinate-system origin. The $t=0$ and $s=0$ moment is the closest proximity of the unperturbed particle’s trajectory to the string (when the particle passes the y-axis). Thus

$$\begin{array}{c}\hfill {}^0{Z}^{\mu }\left(s\right)=u^{\mu }s+b^{\mu }{u}^{\mu }=\gamma (1,0,0,v)b^{\mu }=(0,0,b,0),\end{array}$$

where $\gamma \equiv {(1-v^2)}^{-1/2}$ is a Lorentz factor of the particle.

Therefore, the unperturbed string’s world-sheet is a plane spanned by t- and x-axes: ${\sigma }^0\equiv \tau =t$, ${\sigma }^1\equiv \sigma =x$, thus

$$\begin{array}{c}\hfill {}^0{X}^{\mu }={\delta }_a^{\mu }{\sigma }^a{}^0{\gamma }_{ab}={\eta }_{ab}{}^0{T}^{\mu \nu }=\mu {\delta }_a^{\mu }{\delta }_b^{\nu }{\eta }^{ab}\delta \left(y\right)\delta \left(z\right).\end{array}$$

To the first perturbation order, the Einstein equations are linear, hence ${}^0{T}^{\mu \nu }$ and ${}^0{\overline{T}}^{\mu \nu }$ are separate source of own linearized fields, which are denoted as $h^{\mu \nu }$ and ${\overline{h}}^{\mu \nu }$, respectively (${}^1{\mathfrak{h}}_{\mu \nu }=h_{\mu \nu }+{\overline{h}}_{\mu \nu }$):

$$\begin{array}{c}\hfill {\eta }^{\lambda \rho }\frac{{\partial }^2}{\partial x^{\lambda }\partial x^{\rho }}{h}_{\mu \nu }=-\varkappa \left({}^0{T}_{\mu \nu }-\frac{{}^{0}T}{2}{\eta }_{\mu \nu }\right),{}^{0}T\equiv {}^0{T}^{\sigma \tau }{\eta }_{\sigma \tau },\end{array}$$

(7)

and same for ${\overline{h}}_{\mu \nu }$. Both equations are given in the Lorentz gauge (${\partial }_{\nu }{h}^{\mu \nu }={\eta }^{\mu \nu }{\partial }_{\nu }h/2$), all tensor indices are raised with help of the Minkowski metric.

The solution (7) takes apparently simple form in the Fourier space:

$$\begin{array}{c}\hfill h_{\mu \nu }\left(q\right)=-\frac{{\left(2\pi \right)}^2\varkappa \mu \delta \left(q^0\right)\delta \left(q^1\right)}{{\delta }_{\alpha \beta }{q}^{\alpha }{q}^{\beta }}{\mathsf{\Sigma }}_{\mu \nu }{\mathsf{\Sigma }}_{\mu \nu }\equiv \mathrm{diag}(0,0,1,1),\end{array}$$

where the initial Greek letters take the value 1, 2 and correspond to the transverse (with respect to string) cartesian coordinates (y- and z-axes). The corresponding coordinate solutions of the linearized field created by string and particle, are given by

$$\begin{array}{c}\hfill h_{\mu \nu }\left(x\right)=\frac{\varkappa \mu }{2\pi }{\mathsf{\Sigma }}_{\mu \nu }ln\frac{r}{R_0},{\overline{h}}_{\mu \nu }\left(x\right)=-\frac{\varkappa m}{4\pi }\left(u_{\mu }{u}_{\nu }-\frac{1}{2}{\eta }_{\mu \nu }\right){\left[{\gamma }^2{(z-vt)}^2+x^2+y^2\right]}^{-1/2}.\end{array}$$

Particle’s scattering. To the zeroth order the einbein reads: ${}^{0}e=m$. The first order is determined by expressions

$$\begin{array}{c}\hfill {}^{1}e=-\frac{m}{2}\left(\varkappa h_{\mu \nu }{u}^{\mu }{u}^{\nu }+2{\eta }_{\mu \nu }{}^1{\dot{Z}}^{\mu }{u}^{\nu }\right),{}^1{\ddot{Z}}_{\mu }=-\varkappa \left(h_{\mu \nu ,\lambda }-\frac{1}{2}{h}_{\nu \lambda ,\mu }\right)u^{\nu }{u}^{\lambda }.\end{array}$$

(8)

Such a gauge corresponds to ${}^{1}e=0$, what, in turn, corresponds to the conservation of the natural parametrization of the proper time to the first order with the presence of curved metric.

In components, the particle’s acceleration is given by

$$\begin{array}{c}\hfill {}^1{\ddot{Z}}^0={}^1{\ddot{Z}}^x=0{}^1{\ddot{Z}}^y=-\frac{4G\mu {\gamma }^2{v}^{2}b}{b^2+{\gamma }^2{v}^2{s}^2}{}^1{\ddot{Z}}^z=\frac{4G\mu {\gamma }^3{v}^{3}s}{b^2+{\gamma }^2{v}^2{s}^2}.\end{array}$$

(9)

Since ${\ddot{Z}}^y<0$, the moving particle is attracted by the string. For comparison, we remind that the pointlike particle is attracted by another particle [22,23] (in any spacetime dimension), while is repelled by domain wall [24,25,26].

Integration of (9) with initial condition ${}^1{\dot{Z}}^{\mu }(s=0)=0$ yields

$$\begin{array}{c}\hfill {}^1{\dot{Z}}^0={}^1{\dot{Z}}^x=0{}^1{\dot{Z}}^y=-4G\mu \gamma varctan\frac{\gamma vs}{b}{}^1{\dot{Z}}^z=2G\mu \gamma vln\frac{b^2+{\gamma }^2{v}^2{s}^2}{b^2}.\end{array}$$

(10)

Substituting these corrections into the gauge (8), we notice that for the self-consistency one demands $R_0=b.$ Thus the scattering angle equals

$$\begin{array}{c}\hfill {\alpha }_{\mathrm{sc}}=\pi \beta {}^{\prime }.\end{array}$$

(11)

Finally, integrating (10) with initial condition ${}^1{Z}^{\mu }=0$, one obtains

$$\begin{array}{cccc}\hfill & {}^1{Z}^0=0\hfill & \hfill & {}^1{Z}^y=-\beta {}^{\prime }\left[\gamma vsarctan\frac{\gamma vs}{b}-\frac{b}{2}ln\frac{b^2+{\gamma }^2{v}^2{s}^2}{b^2}\right]\hfill \\ \hfill & {}^1{Z}^x=0\hfill & \hfill & {}^1{Z}^z=\beta {}^{\prime }\left[\frac{\gamma vs}{2}\left(ln\frac{b^2+{\gamma }^2{v}^2{s}^2}{b^2}-2\right)+barctan\frac{\gamma vs}{b}\right].\hfill \end{array}$$

(12)

The particle’s dynamics may be well investigated in the framework of full gravitational field of the cosmic string. The exact geodesics of the cosmic string geometry were considered in [16,20]. The direct comparison of these approaches shows that usage of the linearized metric instead of full cosmic-string metric is justified up to

$$\begin{array}{c}\hfill \left|s\right|⩽s_{\infty }=\frac{b}{\gamma v}exp\frac{1}{\sqrt{\beta {}^{\prime }}},\left|t\right|⩽t_{\infty }=\frac{b}{v}exp\frac{1}{\sqrt{\beta {}^{\prime }}}.\end{array}$$

(13)

3. Seeking the Excitations of the Cosmic String

The own particle’s field interacts with the string and deforms it. The linear part of this field, ${\overline{h}}_{\mu \nu }$, causes the following correction to the induced metric: ${}^1{\gamma }_{ab}=2{\delta }_{(a}^{\mu }{}^1{X}_{b)}^{\nu }{\eta }_{\mu \nu }+\varkappa {\overline{h}}_{\mu \nu }{\delta }_a^{\mu }{\delta }_b^{\nu },$ which, being plugged into (4), yields the following equation of motion:

$$\begin{array}{c}\hfill 2{\eta }^{a[b}{\partial }_a{\partial }_b{}^1{X}^{\mu ]}=\varkappa {\delta }_c^{\mu }{\delta }_d^{\nu }{\eta }^{cd}{\delta }_a^{\lambda }{\delta }_b^{\rho }{\eta }^{ab}{\left(\frac{1}{2}{\overline{h}}_{\lambda \rho ,\nu }-{\overline{h}}_{\nu \lambda ,\rho }\right)}_{y=z=0},\end{array}$$

(14)

We fix the gauge ${}^1{X}^a=0,$ what means that only the transverse string excitations are “physical”. It is well-known that transverse excitations of branes are considered as Nambu–Goldstone bosons. They appeared due to the spontaneous breaking the symmetry [27], and thus may be detected. In various brane models, such bosons are coupled with the induced metric [28,29].

As result, we have two wave equations for y- and z-components of the free-string excitation:

$$\begin{array}{c}\hfill {\square }_2{}^1{X}^{\alpha }={\delta }_a^{\lambda }{\delta }_b^{\rho }{\eta }^{ab}{\left(\frac{1}{2}{\overline{h}}_{\lambda \rho }{}^{,\alpha }-{\overline{h}}_{\lambda ,\rho }^{\alpha }\right)}_{y=z=0},\end{array}$$

(15)

where ($\alpha =y,z$). Hereafter the fields ${}^1{X}^{\alpha }$ will be denoted as ${\mathrm{\Phi }}^{\alpha }$:

$$\begin{array}{c}\hfill {\square }_2{\mathrm{\Phi }}^{\alpha }(t,x)=j^{\alpha }(t,x),\end{array}$$

(16)

where the sources are determined by the right-hand-side of Equation (15).

Due to the source’s dynamics, we will be interested in the retarded solutions to the wave equation. Introducing the retarded Green’s function, the formal expression for the retarded solution of (15) reads:

$$\begin{array}{c}\hfill {\mathrm{\Phi }}^{\alpha }(t,x)=-\frac{1}{{\left(2\pi \right)}^2}\int \frac{{\mathrm{e}}^{i(qx-\omega t)}{j}^{\alpha }(\omega ,q)}{{\omega }^2-q^2+2iϵ\omega }d\omega dq,\end{array}$$

(17)

where $j(\omega ,q)$ is a Fourier-transform of sources.

To resume, with respect to the string, both excitations ${\mathrm{\Phi }}^{\alpha }$ are transverse. With respect to the unperturbed particle’s trajectory, the y-deflection is transverse, while the z-deflection is longitudinal. Hence for brevity the mutually-transverse field (${\mathrm{\Phi }}^y$) will be called just “transverse”, and the transverse-longitudinal field (${\mathrm{\Phi }}^z$) will be called just “longitudinal”.

For the seeking the retarded solution to (15), we are interested in the Fourier-transforms of the sources $j^{\alpha }$ in (17). In the coordinate representation, they equal

$$\begin{array}{c}\hfill j^y(t,x)=-\frac{\varkappa }{2}{\partial }_y{\overline{h}}_a^a{|}_{y=z=0},j^z(t,x)=-\frac{\varkappa }{2}{\left(2{\partial }_t{\overline{h}}^{z0}+{\partial }_z{\overline{h}}_a^a\right)}_{y=z=0}.\end{array}$$

(18)

By the reasons defined below, it is necessary for us to compute these transforms for arbitrary spacetime dimensionality D. Omitting the computational routines, the Fourier-space expressions for two sources are given by

$$\begin{array}{cc}\hfill & j^y(\omega ,q)=\frac{\pi {\lambda }_y}{\mathrm{\Gamma }\left(\frac{D-1}{2}\right){\left(2b\right)}^{\frac{D-5}{2}}\gamma v}\frac{K_{\frac{D-3}{2}}\left(b\sqrt{q^2+{(\omega /\gamma v)}^2}\right)}{{(q^2+{\gamma }^{-2}{v}^{-2}{\omega }^2)}^{-\frac{D-3}{4}}}\hfill \\ \hfill & j^z(\omega ,q)=-\frac{\pi {\lambda }_{z}i\omega }{\mathrm{\Gamma }\left(\frac{D-1}{2}\right){\left(2b\right)}^{\frac{D-5}{2}}\gamma v^2}\frac{K_{\frac{D-5}{2}}\left(b\sqrt{q^2+{(\omega /\gamma v)}^2}\right)}{{(q^2+{\gamma }^{-2}{v}^{-2}{\omega }^2)}^{-\frac{D-5}{4}}},\hfill \end{array}$$

(19)

where $K_{\nu }\left(z\right)$ stands for the Macdonald function of index $\nu$ and

$${\lambda }_{y,z}=\frac{{\varkappa }_D^{2}m\mathrm{\Gamma }\left(\frac{D-1}{2}\right)}{4{\pi }^{\frac{D-1}{2}}}\left({\gamma }^2{v}^2\pm \frac{D-4}{D-2}\right).$$

With the propagator’s poles account, the integration over frequencies reduces to the proper contour closure in the complex plane of $\omega$. The integrand (17) contains two branching points $\omega =\pm iq\gamma v$, due to the Macdonald function argument $j^{y,z}(\omega ,q)$. Hence, it is necessary to make a cut from $\pm iq\gamma v$ to $\pm i\infty$ in the upper (lower) half-plane. Bypassing the branching point along the cut, we get the meromorphic integrand inside each contour, hence the initial integral over $\mathbb{R}$ splits onto the contribution due to the pole residuals and contribution due to the branching point bypass along the corresponding cut. It may be done with help of the rule

$${\left(iz\right)}^n{K}_n\left(iz\right)-(-i{z}^n)K_n(-iz)=-i\pi z^n{J}_n\left(z\right),z>0,$$

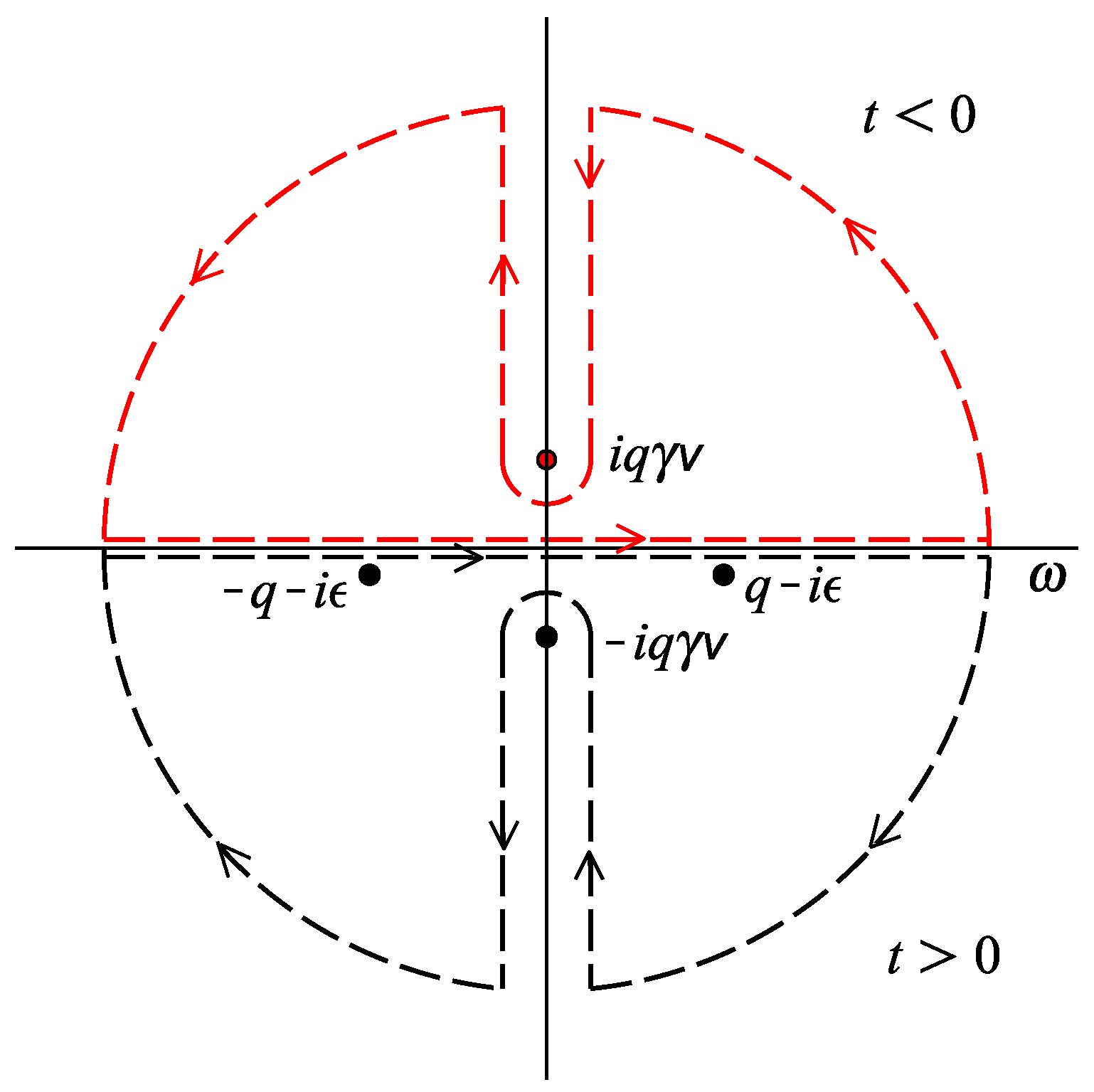
where $J_n\left(z\right)$ stands for the Bessel function of 1st kind of index n. The integration contours over the frequency, corresponding to the cases $t<0$ and $t>0$, respectively, are shown on the Figure 1.

The complete retarded solution, thereby, is given by sum ${\mathrm{\Phi }}^{y,z}={\mathrm{\Phi }}_{\mathrm{cut}}^{y,z}+{\mathrm{\Phi }}_{\mathrm{res}}^{y,z}\phantom{\mathrm{t}},$ where

$${\mathrm{\Phi }}_{\mathrm{cut}}^z=-\mathrm{\Lambda }\mathrm{sgn}t{I}_{\mathrm{cut}}^z{\mathrm{\Phi }}_{\mathrm{res}}^z\phantom{\mathrm{t}}=2\mathrm{\Lambda }\theta \left(t\right)I_{\mathrm{res}}^z\phantom{\mathrm{t}}{\mathrm{\Phi }}_{\mathrm{cut}}^y=\mathrm{\Lambda }v{I}_{\mathrm{cut}}^y{\mathrm{\Phi }}_{\mathrm{res}}^y\phantom{\mathrm{t}}=2\mathrm{\Lambda }v\theta \left(t\right)I_{\mathrm{res}}^y\phantom{\mathrm{t}}$$

($\mathrm{\Lambda }=2Gm\gamma$) (In fact, $\mathrm{\Lambda }$ depends upon D but, as it will be shown below, this dependence does not change the final answer.) and the four introduced amplitudes $I_{\mathrm{cut},\mathrm{res}\phantom{\mathrm{t}}}^{y,z}$ are defined as

$$\begin{array}{cc}\hfill & I_{\mathrm{cut}}^z\equiv \frac{\sqrt{2/\pi }}{b^{\frac{D-5}{2}}}\underset{0}{\overset{\infty }{\int }}dq\underset{q\gamma v}{\overset{\infty }{\int }}du\frac{u{\mathrm{e}}^{-u\left|t\right|}cosqx}{u^2+q^2}\frac{J_{\frac{D-5}{2}}\left(b\sqrt{{(u/\gamma v)}^2-q^2}\right)}{{({\gamma }^{-2}{v}^{-2}{u}^2-q^2)}^{-\frac{D-5}{4}}}\hfill \end{array}$$

(20)

$$\begin{array}{c}{I}_{\mathrm{res}}^z\phantom{\mathrm{t}}\equiv \frac{\sqrt{2/\pi }}{{\left(bv\right)}^{\frac{D-5}{2}}}\underset{0}{\overset{\infty }{\int }}dqcosqtcosqx{q}^{\frac{D-5}{2}}{K}_{\frac{D-5}{2}}\left(bq/v\right)\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hfill \end{array}$$

(21)

$$\begin{array}{cc}\hfill & I_{\mathrm{cut}}^y\equiv \frac{\sqrt{2/\pi }}{b^{\frac{D-5}{2}}}\underset{0}{\overset{\infty }{\int }}dq\underset{q\gamma v}{\overset{\infty }{\int }}du\frac{{\mathrm{e}}^{-u\left|t\right|}cosqx}{u^2+q^2}\frac{J_{\frac{D-3}{2}}\left(b\sqrt{{(u/\gamma v)}^2-q^2}\right)}{{({\gamma }^{-2}{v}^{-2}{u}^2-q^2)}^{-\frac{D-3}{4}}}\hfill \end{array}$$

(22)

$$\begin{array}{c}{I}_{\mathrm{res}}^y\phantom{\mathrm{t}}\equiv \frac{\sqrt{2/\pi }v}{{\left(bv\right)}^{\frac{D-5}{2}}}\underset{0}{\overset{\infty }{\int }}dqsinqtcosqx{q}^{\frac{D-5}{2}}{K}_{\frac{D-3}{2}}\left(bq/v\right).\hspace{1em}\hspace{1em}\hspace{1em}\hfill \end{array}$$

(23)

Thus, both excitations ${\mathrm{\Phi }}^y$ and ${\mathrm{\Phi }}^z$ are split onto two components: apart from the different time indicators, the corresponding amplitudes originate from different mathematical factors. $I_{\mathrm{cut}}^{y,z}$ originates from the integral over bypass contour around the cut to the branching points, hence this partial solution will be called as “cut”-amplitude (with index cut indication). $I_{\mathrm{res}\phantom{\mathrm{t}}}^{y,z}$ comes from the residuals (with index res) in the propagator’s poles, hence this partial solution will be called as “pole”-amplitude. Thus the full dynamics of the two retarded solutions consists in the four amplitudes $I_{\mathrm{cut},\mathrm{res}\phantom{\mathrm{t}}}^{y,z}$.

Direct substitution $D=4$ into the formal solutions for the amplitudes shows that the transverse integrals $I_{\mathrm{cut},\mathrm{res}\phantom{\mathrm{t}}}^y$ converge, while the longitudinal ones $I_{\mathrm{cut},\mathrm{res}\phantom{\mathrm{t}}}^z$ diverge logarithmically at small-q limit.

Let us start with the transverse amplitudes: the polar one is given by

$$\begin{array}{c}\hfill I_{\mathrm{res}}^y\phantom{\mathrm{t}}=\underset{0}{\overset{\infty }{\int }}\frac{sinqt}{q}cosqx{\mathrm{e}}^{-bq/v}dq.\end{array}$$

(24)

Computing it with help of the table integral (formula [30], f. 947-3), we get

$$\begin{array}{c}\hfill I_{\mathrm{res}}^y\phantom{\mathrm{t}}=\frac{1}{2}\left[arctan\frac{t^2-x^2-b^2/v^2}{2bt/v}+\frac{\pi }{2}\right],(t>0).\end{array}$$

(25)

The double-integral of the cut-amplitude $I_{\mathrm{cut}}^y$

$$\begin{array}{c}\hfill I_{\mathrm{cut}}^y\left(\right|t|,r)=\frac{2}{\pi }\underset{0}{\overset{\infty }{\int }}dq\underset{q\gamma v}{\overset{\infty }{\int }}du\frac{{\mathrm{e}}^{-u\left|t\right|}cosqx}{u^2+q^2}sin\left(b\sqrt{{(u/\gamma v)}^2-q^2}\right)\end{array}$$

(26)

can not be computed by the table integrals. Let us differentiate it with respect to t (for $t>0$) and change the integration variable $u=q\gamma v\sqrt{{\tilde{u}}^2+1}$:

$$\begin{array}{c}\hfill {\dot{I}}_{\mathrm{cut}}^y=\frac{2}{\pi }\underset{0}{\overset{\infty }{\int }}dq\underset{0}{\overset{\infty }{\int }}d\tilde{u}\frac{\tilde{u}{\mathrm{e}}^{-q\gamma v\left|t\right|\sqrt{{\tilde{u}}^2+1}}}{{\tilde{u}}^2+v^{-2}}cosqxsinbq\tilde{u}\end{array}$$

(27)

(hereafter, the dot will denote the derivative with respect to t). Integrating (27) consecutively over q and $\tilde{u}$, we obtain the following expression for ${\dot{I}}_{\mathrm{cut}}^y$:

$$\begin{array}{cc}\hfill & {\dot{I}}_{\mathrm{cut}}^y=\frac{b}{Q}\left[t^2+x^2+\frac{b^2}{v^2}-\gamma t\frac{b^2+v^2{t}^2+(2-v^2)x^2}{\sqrt{{\gamma }^2{v}^2{t}^2+b^2+x^2}}\right]\hfill \\ \hfill & Q\equiv {\left(t^2+b^2/v^2-x^2\right)}^2+4b^2{x}^2/v^2.\hfill \end{array}$$

Finally, integrating over t with boundary condition $I_{\mathrm{cut}}^y(t=\infty )=0$ (what follows from the exponential factor in the initial expression (26)), we conclude that $I_{\mathrm{cut}}^y(t,x)$ in a closed form equals

$$\begin{array}{c}\hfill I_{\mathrm{cut}}^y=\frac{1}{2}\left[arctan\frac{v^2{t}^2-v^2{x}^2-(2v^2-1)b^2}{2bv\sqrt{v^2{t}^2+(b^2+x^2)/{\gamma }^2}}-arctan\frac{t^2-x^2-b^2/v^2}{2b\left|t\right|/v}\right].\end{array}$$

(28)

The longitudinal pole-amplitude is given by the following expression:

$$\begin{array}{c}\hfill I_{\mathrm{res}\phantom{\mathrm{t}}}^z=\underset{0}{\overset{\infty }{\int }}\frac{cosqtcosqx}{q}{\mathrm{e}}^{-bq/v}dq,\end{array}$$

which diverges logarithmically. For the regularization we apply the dimensional-regularization method, well-known in Quantum theory. It was pointed out by Hawking [31], that in the case of non-trivial codimensionality of embedding, the dimensional regularization should be applied to the total space-time dimensionality and not to the dimensionality of the sub-manifold (It was successfully confirmed in [32,33,34]).

To do it, we restore $I_{\mathrm{res}\phantom{\mathrm{t}}}^z$ in the form of (21); in our case we have $D=4+2ϵ$ ($ϵ⩾0$):

$$\begin{array}{c}\hfill \mathrm{reg}{I}_{\mathrm{res}\phantom{\mathrm{t}}}^z=\sqrt{\frac{2bv}{\pi }}{\left({\mu }_{\mathrm{res}\phantom{\mathrm{t}}}^{2}bv\right)}^{-ϵ}\underset{0}{\overset{\infty }{\int }}dq\frac{cosqtcosqx}{q^{1/2-ϵ}}{K}_{ϵ-1/2}\left(bq/v\right),\end{array}$$

(29)

where ${\mu }_{\mathrm{res}}\phantom{\mathrm{t}}$ is an arbitrary mass constant introduced for dimensional reasons. For any $ϵ>0$ the integral converges. With the help of table integral ([35], f. 2.16.14-4), the expression (29) reduces to

$$\mathrm{reg}{I}_{\mathrm{res}\phantom{\mathrm{t}}}^z=\frac{\mathrm{\Gamma }\left(ϵ\right)v}{4}{\left(\frac{{\mu }_{\mathrm{res}\phantom{\mathrm{t}}}^2{v}^2}{2}\right)}^{-ϵ}\left[{\left({(t+x)}^2+\frac{b^2}{v^2}\right)}^{-ϵ}+{\left({(t-x)}^2+\frac{b^2}{v^2}\right)}^{-ϵ}\right].$$

Expanding $\mathrm{\Gamma }\left(z\right)$ in the pole $z=0$ and neglecting the infinite (in the limit $ϵ\to 0^{+}$) term ${ϵ}^{-1}$, the renormalized value of the amplitude $I_{\mathrm{res}\phantom{\mathrm{t}}}^z$ reads:

$$\begin{array}{c}\hfill \mathrm{ren}{I}_{\mathrm{res}\phantom{\mathrm{t}}}^z=-\frac{v}{4}ln\frac{[v^2{(t+x)}^2+b^2][v^2{(t-x)}^2+b^2]}{4r_{\mathrm{res}\phantom{\mathrm{t}}}^4},\end{array}$$

(30)

where $r_{\mathrm{res}\phantom{\mathrm{t}}}\propto 1/{\mu }_{\mathrm{res}\phantom{\mathrm{t}}}$ is an arbitrary lengthy scale.

In fact, in view of dimensional regularization, into the definition of $I_{\mathrm{res}\phantom{\mathrm{t}}}^z$ (20) one should add the contributions from all dimension-dependent factors in the pre-factor $\mathrm{\Lambda }$. However, due to the arbitrariness of the constant $r_{\mathrm{res}\phantom{\mathrm{t}}}$, such contributions can just redefine it.

The longitudinal cut-amplitude is given by the following formal expression:

$$\begin{array}{c}\hfill I_{\mathrm{cut}}^z\equiv \frac{2}{\pi }\underset{0}{\overset{\infty }{\int }}dq\underset{\gamma vq}{\overset{\infty }{\int }}du\frac{u{\mathrm{e}}^{-u\left|t\right|}cosqx}{u^2+q^2}\frac{cos\left(b\sqrt{{(u/\gamma v)}^2-q^2}\right)}{\sqrt{{(u/\gamma v)}^2-q^2}}.\end{array}$$

For the regularization of it we restore $I_{\mathrm{cut}}^z$ in the form (20). Putting $D=4+2ϵ$, for any $ϵ>0$ the integral over the infinitesimal semi-circle around each branching point vanishes in the zero-radius limit. The double-integral turns out to be finite, hence we change the integration variable u like in (27):

$$\begin{array}{c}\hfill \mathrm{reg}{I}_{\mathrm{cut}}^z=\sqrt{\frac{2b}{\pi }}{\left({\mu }_{\mathrm{cut}}^{2}b\right)}^{-ϵ}\underset{0}{\overset{\infty }{\int }}d\tilde{u}\underset{0}{\overset{\infty }{\int }}dq\frac{\tilde{u}{\mathrm{e}}^{-q\gamma v\left|t\right|\sqrt{{\tilde{u}}^2+1}}}{u^2+v^{-2}}\frac{J_{ϵ-1/2}\left(bq\tilde{u}\right)}{{\left(q\tilde{u}\right)}^{1/2-ϵ}}cosqx,\end{array}$$

where ${\mu }_{\mathrm{cut}}$—also some arbitrary positive parameter with mass dimension. Taking the inner integral with help of table one ([35], f. 2.12.8-4) we arrive at the following dimensionally-regularized expression:

$$\begin{array}{c}\hfill \mathrm{reg}{I}_{\mathrm{cut}}^z=\frac{\mathrm{\Gamma }\left(ϵ\right)}{2\pi }\sum _{\pm }\underset{0}{\overset{\infty }{\int }}d\tilde{u}\frac{{\mu }_{\mathrm{cut}}^{-2ϵ}{\tilde{u}}^{2ϵ}}{{\tilde{u}}^2+v^{-2}}{\left[{\left(\gamma v\left|t\right|\sqrt{{\tilde{u}}^2+1}\pm ix\right)}^2-b^2{\tilde{u}}^2\right]}^{-ϵ}.\end{array}$$

(31)

Performing the renormalization directly inside the integral and dropping the ${ϵ}^{-1}$-term from the Gamma-function’s Laurent expansion, we find after simplifications:

$$\begin{array}{c}\hfill \mathrm{ren}{I}_{\mathrm{cut}}^z=-\frac{1}{2\pi }\sum _{\pm }\underset{0}{\overset{\infty }{\int }}\frac{d\tilde{u}}{u^2+v^{-2}}ln\frac{({\gamma }^2{v}^2{t}^2+b^2){\tilde{u}}^2\pm 2bx+x^2+{\gamma }^2{v}^2{t}^2}{r_{\mathrm{cut}}^2{\tilde{u}}^2},\end{array}$$

where $r_{\mathrm{cut}}$ stands for the arbitrary length parameter. Now the integral over $\tilde{u}$ converges and equals

$$\begin{array}{c}\hfill \mathrm{ren}{I}_{\mathrm{cut}}^z=-\frac{v}{2}ln\frac{v^2{b}^2{x}^2+{\left({\gamma }^2{v}^2{t}^2+b^2+\gamma v^2\left|t\right|\sqrt{{\gamma }^2{v}^2{t}^2+b^2+x^2}\right)}^2}{r_{\mathrm{cut}}^2({\gamma }^2{v}^2{t}^2+b^2)}.\end{array}$$

(32)

Since we have used the regularization (and moreover inside the improper integral for $I_{\mathrm{cut}}^z$), we must convince ourselves that the found renormalized expressions ${\mathrm{\Phi }}^{y,z}$ do represent the solution to the initial wave equation (16). First we consider the dalembertian action on the symmetric partial amplitudes $I_{\mathrm{cut}}$ and $I_{\mathrm{res}}\phantom{\mathrm{t}}$:

$$\begin{array}{c}\hfill {\square }_2{I}_{\mathrm{res}}^y\phantom{\mathrm{t}}={\square }_2{I}_{\mathrm{res}}^z\phantom{\mathrm{t}}=0.\end{array}$$

(33)

Direct computation for the cut-amplitudes (28) yields

$$\begin{array}{c}\hfill {\square }_2{I}_{\mathrm{cut}}^y=\frac{\gamma vb}{{({\gamma }^2{v}^2{t}^2+b^2+x^2)}^{3/2}}-\frac{2bv}{v^2{x}^2+b^2}\delta \left(t\right),{\square }_2{I}_{\mathrm{cut}}^z=\frac{{\gamma }^3{v}^3\left|t\right|}{{({\gamma }^2{v}^2{t}^2+b^2+x^2)}^{3/2}}.\end{array}$$

(34)

Now we can switch an attention to the full solutions. The dalembertian of full solution for ${\mathrm{\Phi }}^z$ takes the form:

$$\begin{array}{c}\hfill {\square }_2{\mathrm{\Phi }}^z=j^z+2\mathrm{\Lambda }{\delta }^{\prime }\left(t\right){\left(I_{\mathrm{res}\phantom{\mathrm{t}}}^z-I_{\mathrm{cut}}^z\right)}_{t=0},\end{array}$$

where we imply $\delta \left(t\right){\dot{I}}_{\mathrm{cut},\mathrm{res}\phantom{\mathrm{t}}}^z=0$ in sense of distributions, since $I_{\mathrm{cut},\mathrm{res}\phantom{\mathrm{t}}}^z$ are time-symmetric by construction [36]. Therefore, it is necessary to satisfy the constraint $I_{\mathrm{res}\phantom{\mathrm{t}}}^z(0,x)=I_{\mathrm{cut}}^z(0,x),$ thus we find the relation of two length scales: $r_{\mathrm{cut}}=\sqrt{2}{r}_{\mathrm{res}\phantom{\mathrm{t}}}\equiv r_0.$ Substituting all expressions and constants, the full solution for ${\mathrm{\Phi }}^z$ reads

$$\begin{array}{cc}\hfill {\mathrm{\Phi }}^z=Gm\gamma v& [\mathrm{sgn}\left(t\right)ln\frac{v^2{b}^2{x}^2+{\left({\gamma }^2{v}^2{t}^2+b^2+\gamma v^2\left|t\right|\sqrt{{\gamma }^2{v}^2{t}^2+b^2+x^2}\right)}^2}{r_0^2({\gamma }^2{v}^2{t}^2+b^2)}-\hfill \\ & -\theta \left(t\right)ln\frac{[v^2{(t+x)}^2+b^2][v^2{(t-x)}^2+b^2]}{r_0^4}].\hfill \end{array}$$

(35)

For the dalembertian of full transverse solution we take the account of (33) and (34), and the boundary condition $I_{\mathrm{res}\phantom{\mathrm{t}}}^y(0,x)=0$; thus the dalembertian equals

$${\square }_2{\mathrm{\Phi }}^y=j^y+2\mathrm{\Lambda }v\delta \left(t\right){\left({\dot{I}}_{\mathrm{cut}}^y+{\dot{I}}_{\mathrm{res}\phantom{\mathrm{t}}}^y\right)}_{t=0^{+}}.$$

Comparing (25) with computed above ${\dot{I}}_{\mathrm{cut}}^y(0^{+},t)$, we convince ourselves that ${\dot{I}}_{\mathrm{cut}}^y(0^{+},t)=-{\dot{I}}_{\mathrm{res}\phantom{\mathrm{t}}}^y(0^{+},t)$ holds, hence the found ${\mathrm{\Phi }}^y$ does represent the solution of (16).

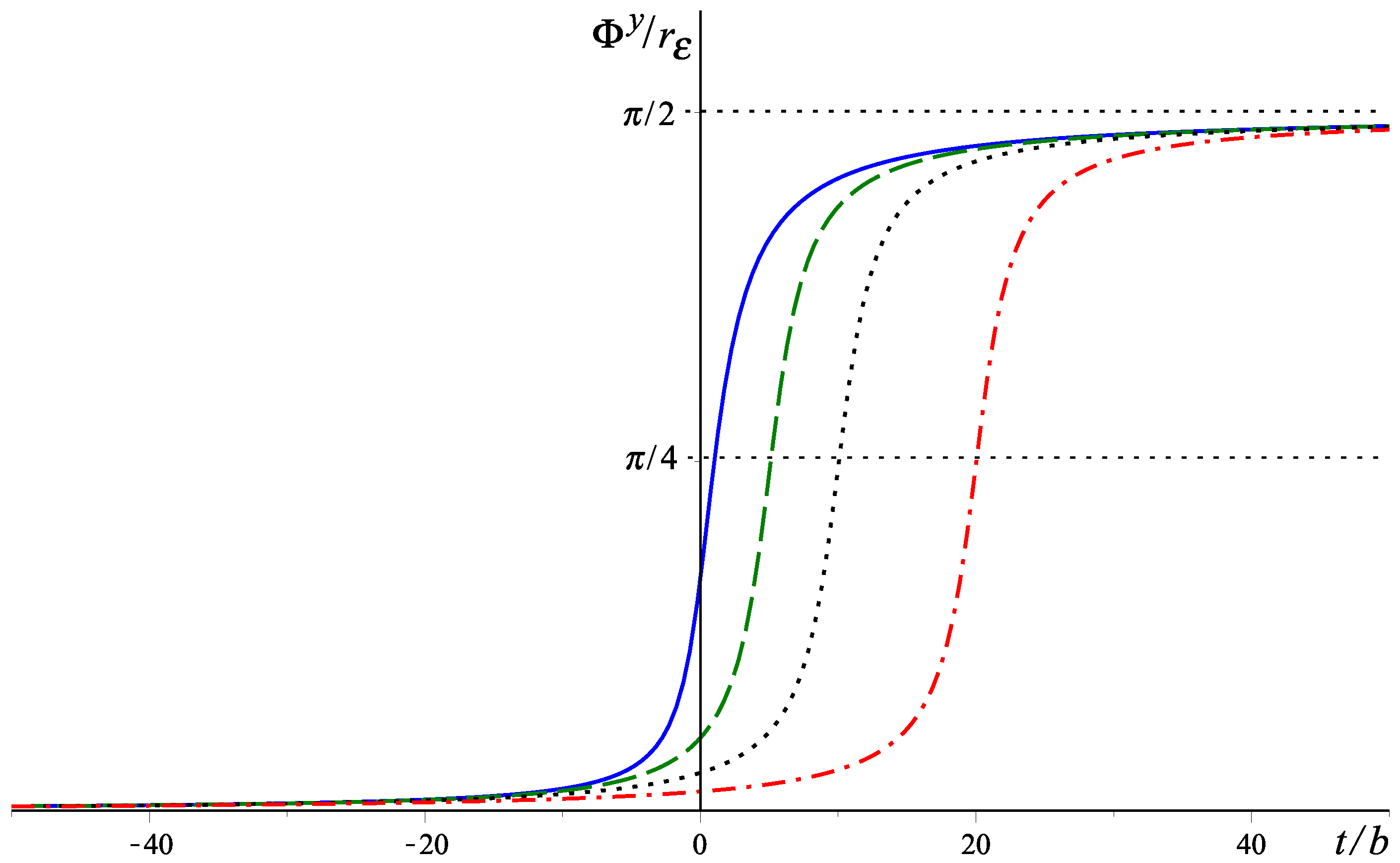
Combining all the contributions, the total transverse solution takes the form:

$$\begin{array}{c}\hfill {\mathrm{\Phi }}^y=Gm\gamma v\left[arctan\frac{v^2{t}^2-v^2{x}^2-(2v^2-1)b^2}{2bv\sqrt{v^2{t}^2+(b^2+x^2)/{\gamma }^2}}+arctan\frac{t^2-x^2-b^2/v^2}{2bt/v}+\pi \theta \left(t\right)\right].\end{array}$$

(36)

Therefore, the constructed functions ${\mathrm{\Phi }}^y$ and ${\mathrm{\Phi }}^z$ have the following properties:

• They are continuous with respect to t and x;
• They satisfy the equation of motion (16);
• They preserve the causality, that is, they represent the retarded solutions;
• They vanish in the limit $v\to 0$;
• Their values have characteristic length scale $2Gm\gamma =r_{\mathcal{E}}$ which is Schwarzschild radius associated with energy $\mathcal{E}$;
• ${\mathrm{\Phi }}^z$ depends logarithmically upon the single length factor $r_0$.

4. Transverse String Perturbation

In the absolute Past and Future, for fixed $0<v<1$ and x ($\left|t\right|\gg max(x,b/v))$${\mathrm{\Phi }}^y$ (36) behaves as

$$\begin{array}{c}\hfill {\mathrm{\Phi }}^y{|}_{t\to -\infty }\simeq \frac{r_{\mathcal{E}}b}{\left|t\right|}(1-v)\to 0{\mathrm{\Phi }}^y{|}_{t\to +\infty }\simeq r_{\mathcal{E}}\left[\pi v-\frac{b}{t}(1+v)+\mathcal{O}\left(t^{-2}\right)\to \pi v{r}_{\mathcal{E}}\right],\end{array}$$

(37)

hence the string’s final (as $t\to +\infty$) $y-$deflection equals $\pi v{r}_{\mathcal{E}}$ and does not depend upon x.

At any fixed point x both ${\mathrm{\Phi }}^y$ and ${\dot{\mathrm{\Phi }}}^y$ are positive. Therefore, the characteristic factor of the transverse string’s excitation is $r_{\mathcal{E}}$ in y-direction, and b along the string. The characteristic time factor is the position of half-asymptote of the plot ${\mathrm{\Phi }}^y\left(t\right)$ (Figure 2); this time equals

$$t=\sqrt{x^2+b^2}\equiv t_x$$

and does not depend on the particle’s velocity. Respectively, the doubled time $2t_x$ is a characteristic relaxation time of the string’s piece with coordinate x with respect to the final asymptotic.

Thus, the string’s y-deflection carries the properties of the transverse wave which propagates from the apex to the periphery. The wavefront propagates with superluminal speed

$$\begin{array}{c}\hfill V=\frac{\partial x_{1/2}}{\partial t}=\frac{t}{\sqrt{t^2-b^2}},\end{array}$$

(38)

which asymptotically tends to the speed-of-light.

In the ultrarelativistic case ($x\ll \gamma t$)

$$\begin{array}{c}\hfill {\mathrm{\Phi }}^y{|}_{b\ll x\ll \gamma \left|t\right|}\simeq \frac{2r_{\mathcal{E}}b\left|t\right|}{x^2}.\end{array}$$

We can expand (37) with respect to $1/\gamma$, to obtain:

$$\begin{array}{c}\hfill {\mathrm{\Phi }}^y{|}_{t\to -\infty }\simeq \frac{r_{\mathcal{E}}b}{2\left|t\right|{\gamma }^2},{\mathrm{\Phi }}^y{|}_{t\to +\infty }\simeq r_{\mathcal{E}}\left[\pi -\frac{2b}{t}+\mathcal{O}\left(\frac{x^2+b^2}{t^2}\right)\right].\end{array}$$

(39)

Therefore for $t⩽0$ the transverse excitation is suppressed as $1/\gamma$ and vanishes in the massless limit, which implies the finite particle’s energy [37]

$$m\to 0,\gamma \to \infty ,m\gamma \equiv \mathcal{E}=\mathrm{const}.$$

Hence the complete string’s transverse perturbation takes place at $t>0$ and is determined by expression

$$\begin{array}{c}\hfill {\mathrm{\Phi }}^y{|}_{v=1}=\frac{r_{\mathcal{E}}}{2}\left[2arctan\frac{t^2-x^2-b^2}{2bt}+\pi \right]\theta \left(t\right).\end{array}$$

(40)

Thus for the fixed particle’s energy the maximal attraction towards the particle is achieved in the massless limit; the string’s y-shift equals $\pi r_{\mathcal{E}}$ precisely.

5. Longitudinal String’s Excitation

In contrast with the transverse excitation, the longitudinal solution (35) depends upon the arbitrary scale factor $r_0$. Such a constant appearing in the dimensional regularization technique, is usually declared as “to be determined in the experiment” in the literature. However, we try to give some sense to it from some theoretical-speculation framework.

In the pure case of Minkowski metric the physically-motivated requirement is an absence of the perturbation at $t\to -\infty$. In our case, we are convinced that it is natural to impose a condition, that interaction starts at $t=-t_{\infty }$, where $t_{\infty }$ is deduced in formula (13).

For $t<0$ the longitudinal solution is governed by the first logarithm in (35), it originates from the cut-term of the total solution. One observes that ${\dot{\mathrm{\Phi }}}^z$ increases as function of t at any $t<0$. Thus at $t=-t_{\infty }$ with our hypothesis of no interaction, we require the disappearance of ${\mathrm{\Phi }}^z$ or its smallness with respect to the maximum (which happens at $t\sim b$). From some symmetry speculations, it is clear that for qualitative estimates it is enough to consider the string’s apex $x=0$. Fixing it in (35), we get

$$\begin{array}{c}\hfill |{\mathrm{\Phi }}^z(t<0,x)|<2Gm\gamma v|ln\frac{\sqrt{{\gamma }^2{v}^2{t}^2+b^2}+\gamma v^2\left|t\right|}{r_0}|.\end{array}$$

(41)

Fixing $t=-t_{\infty }$ here, we infer that within this hypothesis, the scale factor should be equal

$$r_0=\gamma v(1+v)t_{\infty }=\gamma (1+v)b{\mathrm{e}}^{1/\sqrt{\beta {}^{\prime }}}.$$

With this identification, the maximal z-deflections are given by

$$|{\mathrm{\Phi }}^z(-t_{\infty },0)|\simeq \frac{r_{\mathcal{E}}v}{2(1+v){\gamma }^2}{\mathrm{e}}^{-2/\sqrt{\beta {}^{\prime }}},\left|{\mathrm{\Phi }}^z(0,0)\right|\simeq r_{\mathcal{E}}vln\left(\gamma (1+v){\mathrm{e}}^{1/\sqrt{\beta {}^{\prime }}}\right),$$

so that the latter is exponentially large with respect to the first, to confirm the idea that the string was “almost at rest” in the effective past.

Due to logarithms, the same restriction concerns the maximal string’s coordinate x where it can receive the effective particle’s linearized-gravity field with no distortion:

$$x_{max}=v{t}_{\infty }=b{\mathrm{e}}^{1/\sqrt{\beta {}^{\prime }}}.$$

Furthermore, the consideration of Newtonian limit implies the large impact parameters with respect to the particle’s gravitational radius; the same note concerns the real (finite) width of a string:

$$b\gg r_g=2Gm,b\gg d={\eta }^{-1}.$$

Now we transit to the qualitative analysis of the longitudinal solution. At $t<0$${\mathrm{\Phi }}^z$ as a function of time, increases monotonously, reaching at $t=0$ the value

$${\mathrm{\Phi }}^z(0,x)=\frac{r_{\mathcal{E}}v}{2}ln\frac{{(1+v)}^2{b}^2{\gamma }^2{\mathrm{e}}^{2/\sqrt{\beta {}^{\prime }}}}{v^2{x}^2+b^2}=r_{\mathcal{E}}v\left(\frac{1}{\sqrt{\beta {}^{\prime }}}+ln\left[\gamma (1+v)\right]-\frac{1}{2}ln\frac{v^2{x}^2+b^2}{b^2}\right).$$

Therefore, the relatively large values $r_{\mathcal{E}}/\sqrt{\beta {}^{\prime }}$ and $r_{\mathcal{E}}ln2\gamma$ (the latter for ultrarelativistic particles) define some characteristic background value of the string’s longitudinal excitation:

$${\mathrm{\Phi }}_{\mathrm{bg}}^z=r_{\mathcal{E}}v\left(\frac{1}{\sqrt{\beta {}^{\prime }}}+ln\left[\gamma (1+v)\right]\right),$$

while the scale $r_{\mathcal{E}}$ defines the characteristic dynamical part on this background.

For the positive maximal time $t=t_{\infty }$ the value of ${\mathrm{\Phi }}^z$ will be determined by the pole-term of the total solution:

$${\mathrm{\Phi }}^z(t_{\infty },x)\simeq 2r_{\mathcal{E}}vln\left[\gamma (1+v)\right]\equiv {\mathrm{\Phi }}_{\infty }^z.$$

Since, according to our assumption, there is no effective interaction at $t>t_{\infty }$, then the value ${\mathrm{\Phi }}_{\infty }^z$ defines the final z-shift of the string. As we see, it does not depend upon the string’s angular defect and thus upon the identification of arbitrary constant $r_0$. Furthermore, this value is independent of x, that is, in its final state the string is shifted in z-direction parallel to its original position.

The relaxation time to the final deflection is $b{\mathrm{e}}^{1/\sqrt{\beta {}^{\prime }}}/v$ and too large. However, the dynamical part of the solution has own characteristic lengthy and time scales, related with b and x, as well as the transverse solution has.

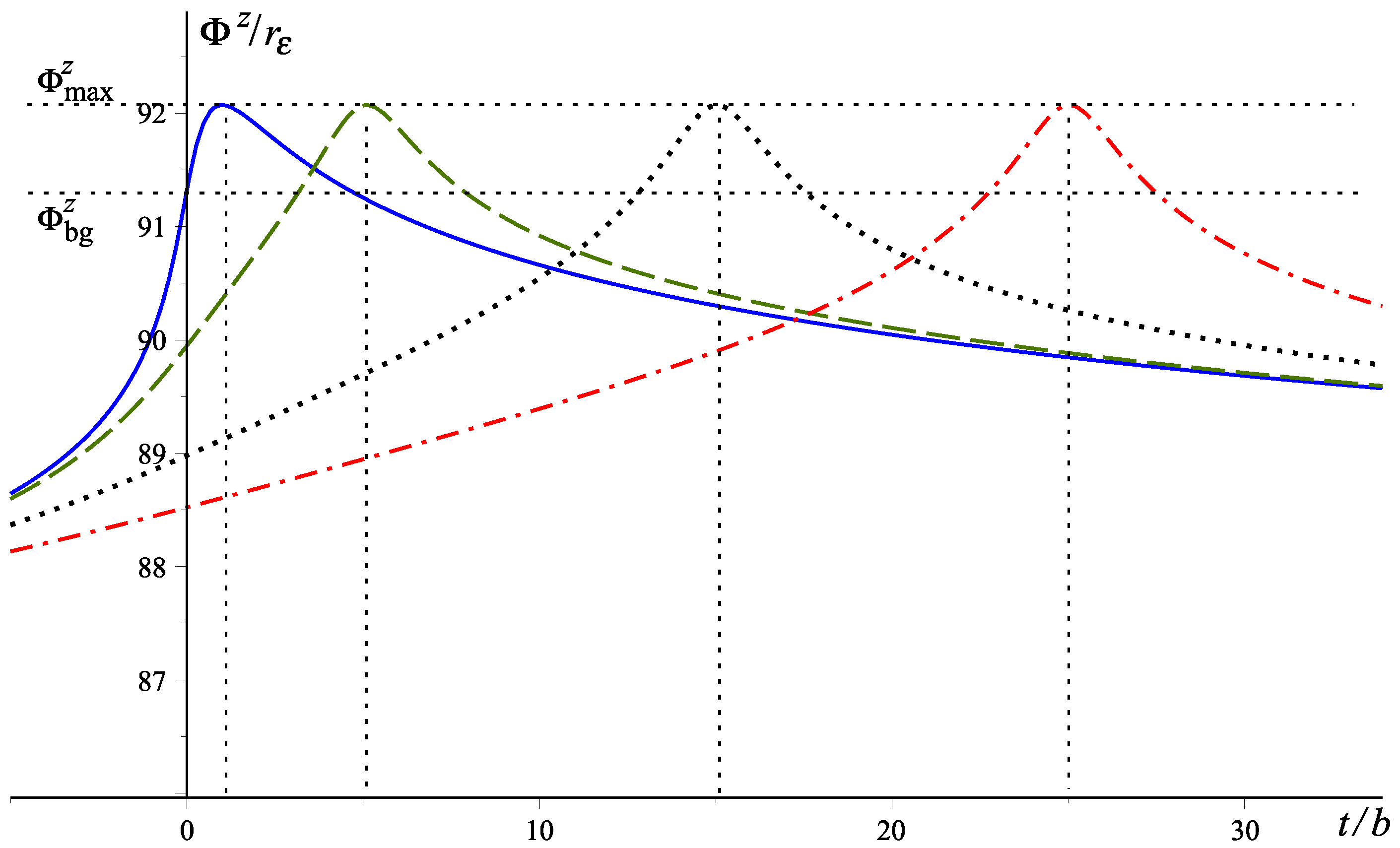
On the Figure 3 we plot the dependence of ${\mathrm{\Phi }}^z$ upon time.

The maxima correspond to $t=t_x.$ It is precisely the distance from the particle’s position at the moment of “collision” ($t=0$), to the observation point on string. The value at maximum

$$\begin{array}{c}\hfill {\mathrm{\Phi }}^z(t_x,x)=r_{\mathcal{E}}v\left(\frac{1}{\sqrt{\beta {}^{\prime }}}+2ln\gamma +ln(1+v)\right)\equiv {\mathrm{\Phi }}_{max}^z\end{array}$$

(42)

does not depend on x. Hence the excitation has a form of the wave (with constant amplitude) which propagates from the apex to periphery with the same velocity (38) as the transverse wave. The difference between two waves consists in the wavefront notion and in the character of residual phenomenon after the wavefront passage. In the transverse-perturbation case, the wavefront forms an apparent transverse (with respect to direction of propagation) rectangular frame in orthogonal to z-axis direction. On the wavefront, a piece of the string is attracted abruptly towards the particle’s trajectory. After the wavefront passage, that piece does not change the direction of motion and drifts slowly to the final shift value ${\mathrm{\Phi }}_{\infty }^y$. In the longitudinal-perturbation case as a wavefront we regard the position of the deflection maximum (42); after the wavefront passage, that piece of the string changes the direction of motion and goes towards the initial z-position.

For fixed x the equation ${\mathrm{\Phi }}^z\left(t\right)={\mathrm{\Phi }}_{\mathrm{bg}}^z$ has two roots

$$t_{\pm }\left(x\right)=\sqrt{b^2{\gamma }^2+x^2}\pm b\gamma ,0⩽t_{-}\left(x\right)<t_x<t_{+}\left(x\right).$$

Thus we can regard

$$\begin{array}{c}\hfill \delta t\simeq |t_{+}-t_{-}|=2\gamma b\end{array}$$

(43)

as a relaxation time of the wave’s dynamical part with respect to background. During this time the wavefront passes the observation point. At $t>t_{+}\left(x\right)$ the corresponding string’s piece (with coordinate $\pm x$) slowly decays to ${\mathrm{\Phi }}_{\infty }^z$ (with characteristic time $t_{\infty }$).

Since ${\dot{\mathrm{\Phi }}}^z>0$ at $t<t_x$ and ${\dot{\mathrm{\Phi }}}^z<0$ at $t>t_x$, one may say that the moving particle repels the string along the direction of own motion. Note that in the lateral direction the moving particle attracts the string.

It is clear that the wave-part of the longitudinal solution corresponds to the term $ln(v^2{(t\mp x)}^2+b^2)$ from Expression (35) for the wave running in the positive (negative) direction. These terms originate from the pole-part of the total solution which satisfies the homogeneous wave equation; the relatively surprising fact is that the maximal value (42) does not change in time, that is the slowly decaying cut-term (satisfying the homogeneous equation) also takes place in the keeping the maximal amplitude of pure wave nature.

From the formulae presented above, it is clear that the presence of factor $ln\gamma$ implies that we can not use massless limit for the longitudinal excitation. Indeed, due to the construction, the timescale of the dynamical-part-relaxation (43) is restricted by the timescale (13), related with the angular conical defect when the conformally-Euclidean coordinated are of usage. In what follows

$$\begin{array}{c}\hfill \gamma <{\gamma }_{max}={\mathrm{e}}^{1/\sqrt{\beta {}^{\prime }}}.\end{array}$$

(44)

Therefore we avoid the requirement of vanishing the cut-part for the Aichelburg–Sexl shockwave, as physical reasons demand. Nevertheless, for contemporary restrictions on the possible values of the angular conical defect, Expression (44) is a huge quantity much larger than possible Lorentz factors available now.

6. Conclusions

Effects of the gravitational interaction under elastic encounter of the cosmic string and spinless particle are considered. We have used iteration scheme with the Perturbation theory over gravitational constant (G or $\varkappa$), with flat space as background. The particle is scattered in the plane transverse to the unperturbed string, attracting to it by angle proportional to the conical angular deficit only and independent of the impact parameter b and particle’s energy $\mathcal{E}$.

The basic result of the work is a construction of the exact retarded solutions to the equations of motion for the cosmic string. They are obtained as inverse elementary functions. The solutions are continuous, vanish in the zero-speed limit and possess a series of other interesting analytical properties.

The characteristic interaction time is $b/v$, but the additional lengthy scalefactor $r_0\gg b$ arises. The proportionality coefficient is related with the angular defect $\beta {}^{\prime }$ of the background conical space.

In turn, the particle’s motion causes the string’s excitation: it represents deflections in transverse (to the unperturbed string) directions: the first deflection is longitudinal with respect to the particle’s motion, the second one is purely lateral. After the moment $t=b$ both excitations represent a single wave along the string.

The characteristic length parameter, which determines the value of transverse string deformations, is a particle’s gravitational radius $r_{\mathcal{E}}$ associated with energy $\mathcal{E}$. Thus we expect that the tiny a priori effect can be amplified if we consider the scattering of transplanckian particles or scattering of a beam one-sided from the string; this can yield a multiplicity.

Single waves running along the string, are transverse with respect to the direction of propagation. Both waves are superluminal, that is nothing but the light-spot effect. Effectively, as a source of waves one can consider the particle at the moment of collision. The the signal passes the distant to the observation point on the string ($t_x=\sqrt{b^2+x^2}$) with speed-of-light. Respectively, the minimal time to achieve the string ($t_0=b$) corresponds to apex; at this moment the wave appears in the apex and then propagates to periphery.

The mutually-transverse deflection (${\mathrm{\Phi }}^y$) has characteristic timescale of order of impact parameter. The wave caused by the particle’s attraction, after passage leaves the string in shifted state; the shift is of order of $r_{\mathcal{E}}$ in the direction towards the particle.

The longitudinal (with respect to the particle-motion direction) shift is directed outwards the particle: at $t<t_x$ the string’s piece x goes along the particle, and then after the wavefront passage it undergoes the backward recess. Therefore, in its longitudinal direction, the particle’s gravitational field repels the string. Such a non-trivial interaction was proper to the Particle–Domain wall system [24,25,26,38]. The interesting technical aspect is a usage of the dimensional-regularization method to preserve the causal structure of the longitudinal solution. A payment for this is the appearance of the extra arbitrary constant and, consequently, the appearance of extra scale factor. Within our Perturbation-theory framework we associate it with a combination of the impact parameter and the string’s conical angular deficit.

In conclusion, we notice that the approach allows to go beyond the elastic scattering and consider the gravitational radiation which arises in collision. Such inelastic processes, in particular, might change the cosmic-string energy at early stages of the Universe evolution, and change the energy balance within various cosmological scenaria. This kind of particle-string bremsstrahlung differs from the string radiation considered before (e.g., the radiation from cosmic-string loops [8,9]).

Naively, for string bremsstrahlung one might observe, that though the string’s deflection, found in the paper, is small, the total emitting energy comes from the whole string. If so, the real (Regarding the string’s length, the “real” means not that the cosmic string is real (observable now or active in the Past), but just “the actual string’s length in the assumption that the theoretical models, predicting the cosmic string’s existence, are adequate.”) string’s length is not infinite (as we have considered for simplicity), but extremely large, being of order of the Universe’s actual size. Such a computation is a natural task for the subsequent work.

In addition, the effects of string’s interaction with neutral particles with non-zero spin represents the particular interest and the prospect of further theoretical issues.