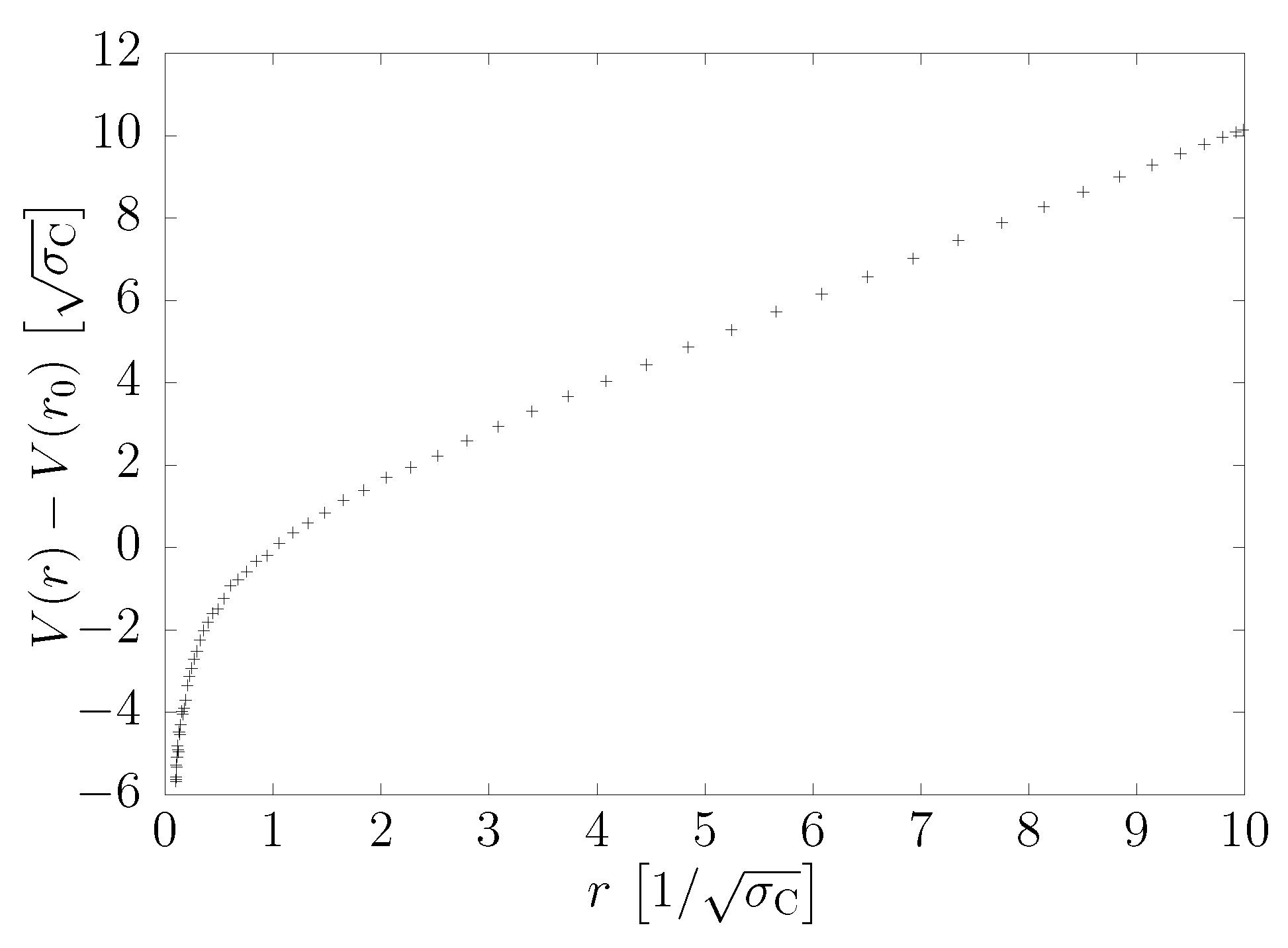Hamiltonian Approach to QCD at Finite Temperature †
Abstract
1. Introduction
2. Finite Temperature from Compactification of a Spatial Dimension
- invariance of the Euclidean Lagrange density, which should hold for any relativistically invariant theory. It does, however, not hold for a non-relativistic many-body system.
- The absence of massless modes, i.e., the existence of a mass gap on the manifold . This is the generic case. For instance, QCD in the confining phase is known to develop such a gap, and this follows also self-consistently from the results in the next section. Extra caution may be required at temperatures above the deconfinement phase transition, where we can check our findings against thermal perturbation theory.
3. Hamiltonian Approach to QCD in Coulomb Gauge
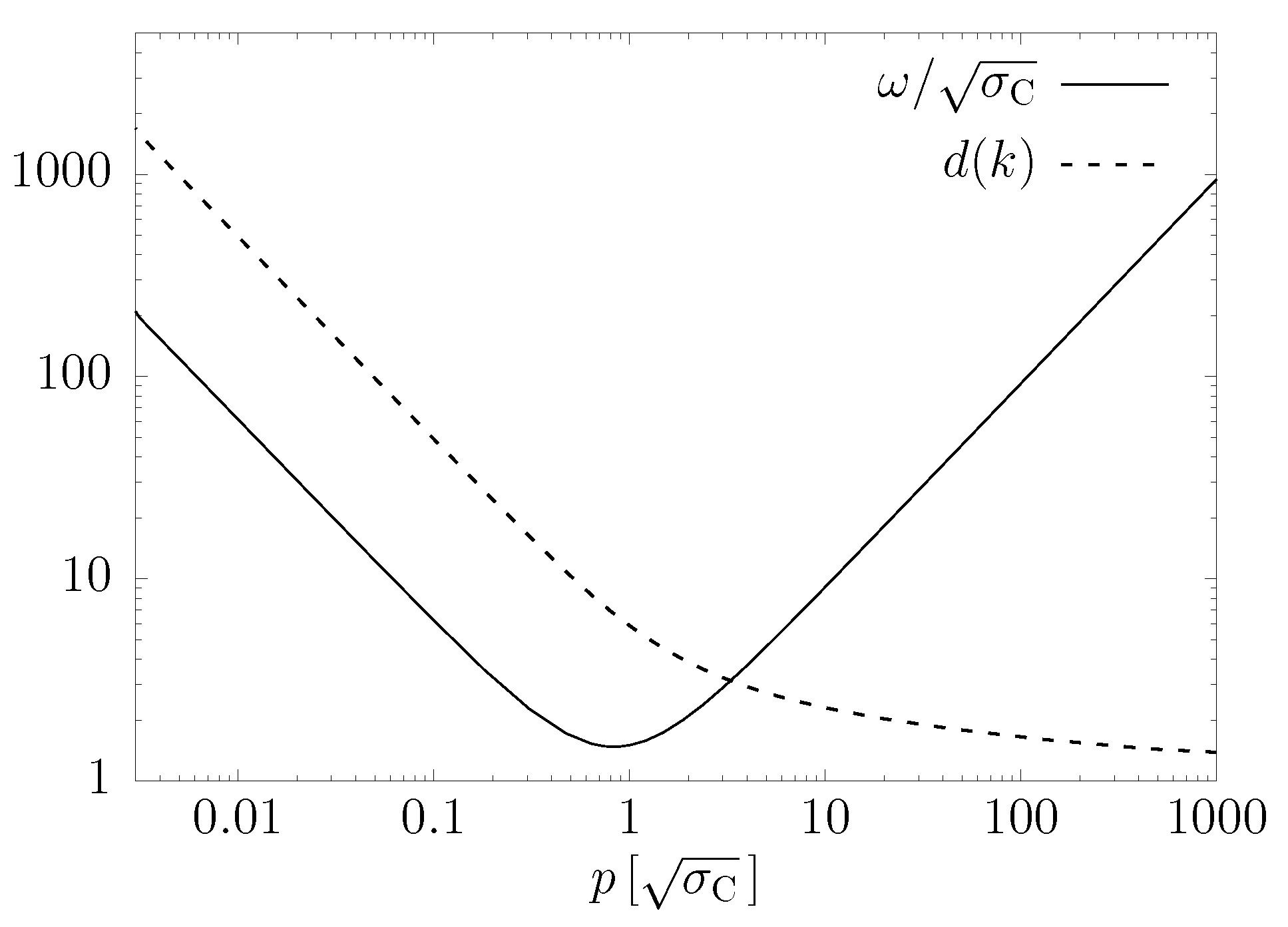
3.1. Yang–Mills Sector
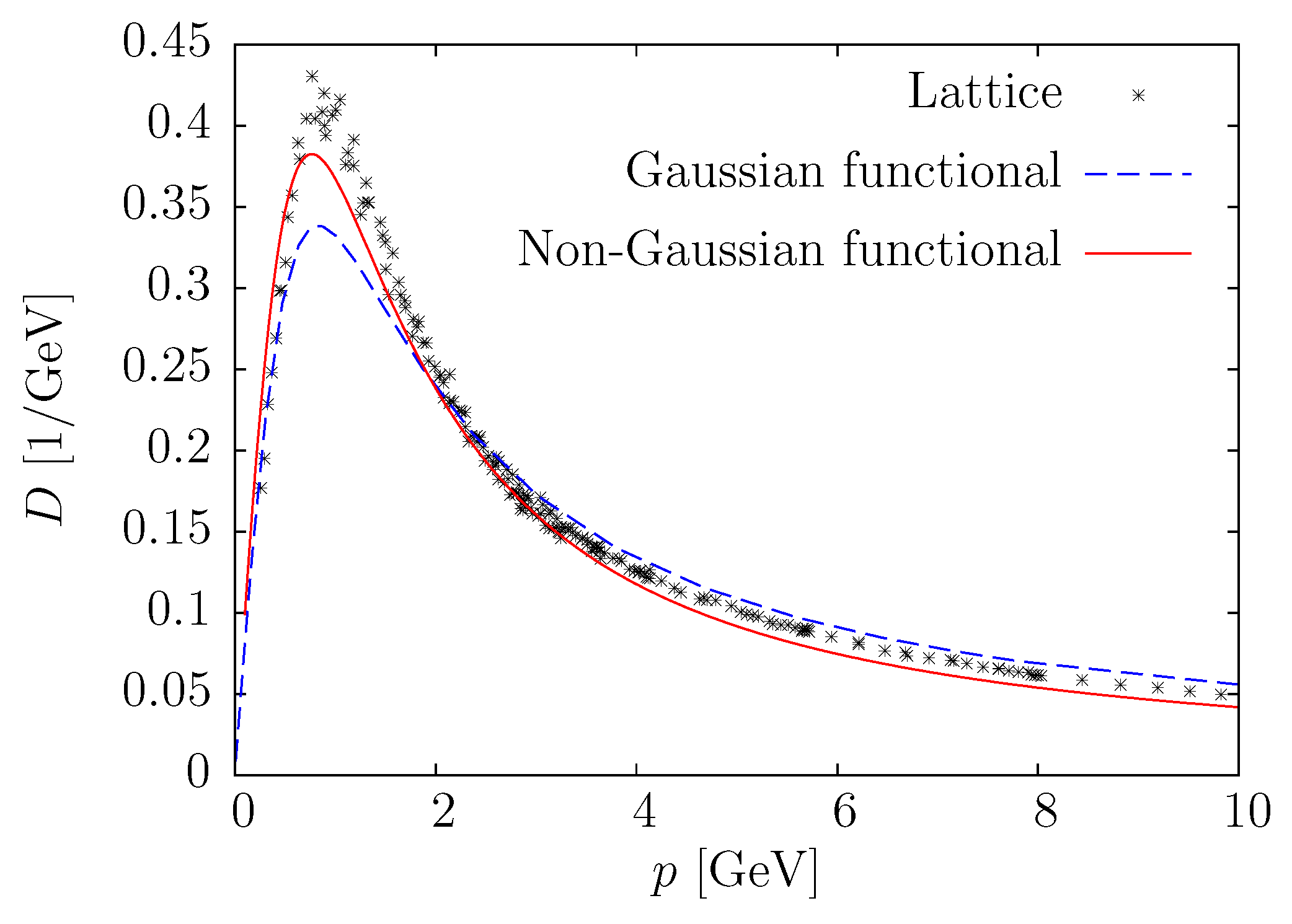
3.2. Quark Sector
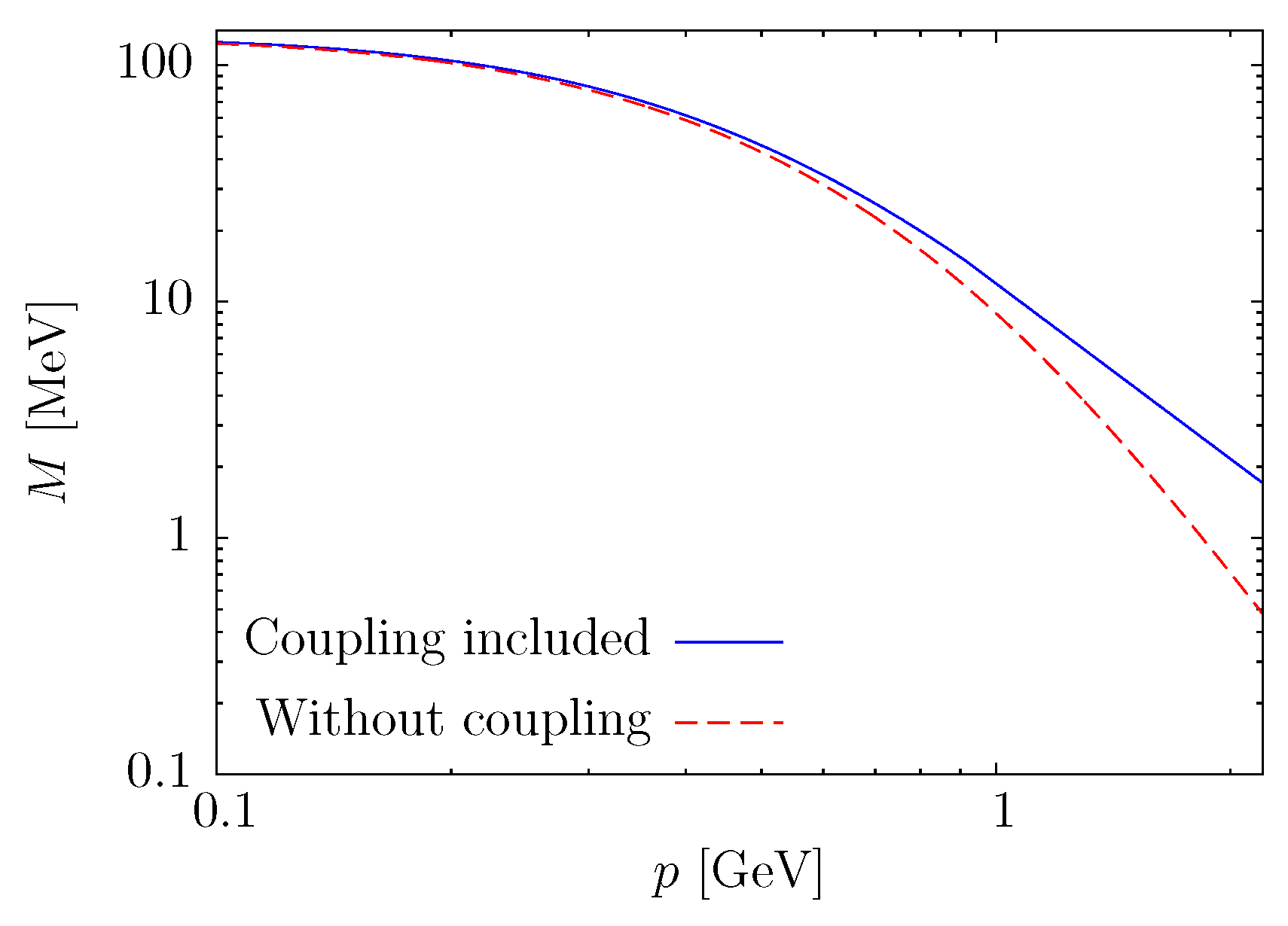
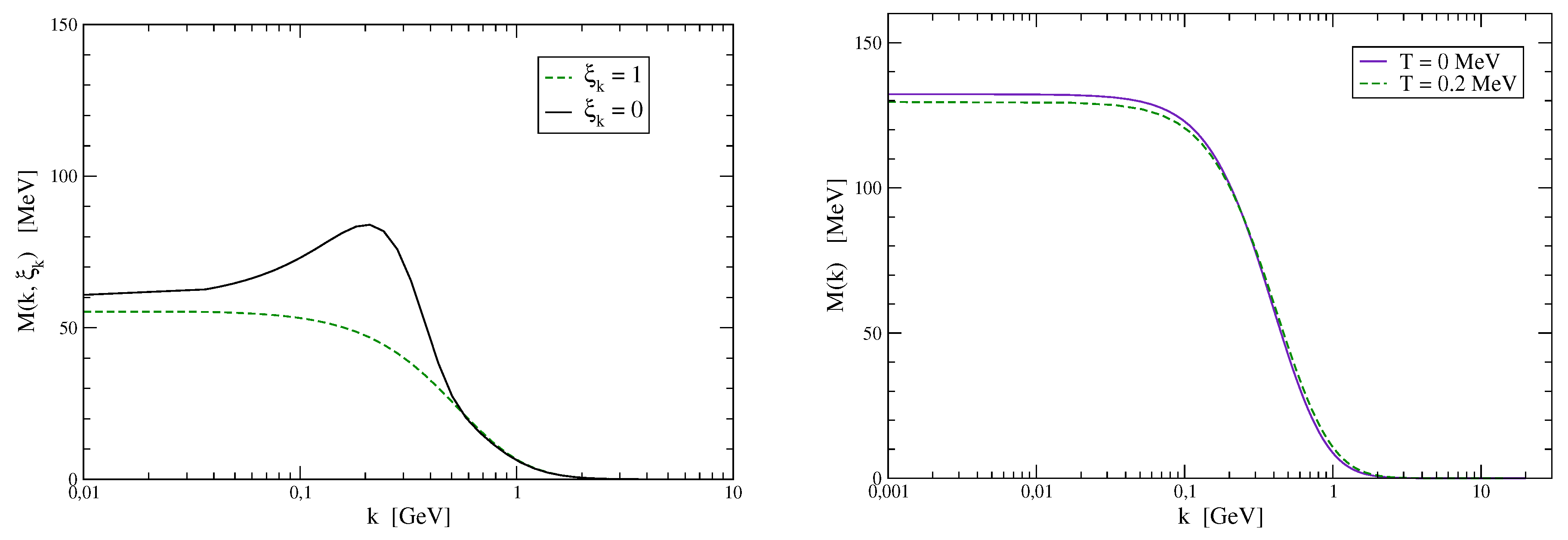
4. QCD at Finite Temperatures
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Karsch, F. Lattice QCD at high temperature and density. Lect. Notes Phys. 2002, 583, 209–249. [Google Scholar]
- Fukushima, K.; Hatsuda, T. The phase diagram of dense QCD. Rep. Prog. Phys. 2011, 74, 014001. [Google Scholar] [CrossRef]
- Gattringer, C.; Langfeld, K. Approaches to the sign problem in lattice field theory. Int. J. Mod. Phys. 2016, A31, 1643007. [Google Scholar] [CrossRef]
- Fischer, C.S. Infrared properties of QCD from Dyson-Schwinger equations. J. Phys. 2006, G32, R253–R291. [Google Scholar] [CrossRef]
- Alkofer, R.; von Smekal, L. The Infrared behavior of QCD Green’s functions: Confinement dynamical symmetry breaking, and hadrons as relativistic bound states. Phys. Rep. 2001, 353, 281. [Google Scholar] [CrossRef]
- Binosi, D.; Papavassiliou, J. Pinch Technique: Theory and Applications. Phys. Rep. 2009, 479, 1–152. [Google Scholar] [CrossRef]
- Watson, P.; Reinhardt, H. Propagator Dyson-Schwinger Equations of Coulomb Gauge Yang-Mills Theory Within the First Order Formalism. Phys. Rev. D 2007, 75, 045021. [Google Scholar] [CrossRef]
- Watson, P.; Reinhardt, H. Two-point functions of Coulomb gauge Yang-Mills theory. Phys. Rev. D 2008, 77, 025030. [Google Scholar] [CrossRef]
- Watson, P.; Reinhardt, H. Slavnov-Taylor identities in Coulomb gauge Yang-Mills theory. Eur. Phys. J. 2010, C65, 567–585. [Google Scholar] [CrossRef]
- Pawlowski, J.M. Aspects of the functional renormalisation group. Ann. Phys. 2007, 322, 2831–2915. [Google Scholar] [CrossRef]
- Gies, H. Introduction to the functional RG and applications to gauge theories. Lect. Notes Phys. 2012, 852, 287–348. [Google Scholar]
- Quandt, M.; Reinhardt, H.; Heffner, J. Covariant variational approach to Yang-Mills theory. Phys. Rev. D 2014, 89, 065037. [Google Scholar] [CrossRef]
- Quandt, M.; Reinhardt, H. A covariant variational approach to Yang-Mills Theory at finite temperatures. Phys. Rev. D 2015, 92, 025051. [Google Scholar] [CrossRef]
- Feuchter, C.; Reinhardt, H. Variational solution of the Yang-Mills Schrödinger equation in Coulomb gauge. Phys. Rev. D 2004, 70, 105021. [Google Scholar] [CrossRef]
- Reinhardt, H.; Feuchter, C. Yang-Mills wave functional in Coulomb gauge. Phys. Rev. D 2005, 71, 105002. [Google Scholar] [CrossRef]
- Reinosa, U.; Serreau, J.; Tissier, M.; Wschebor, N. Deconfinement transition in SU(2) Yang-Mills theory: A two-loop study. Phys. Rev. D 2015, 91, 045035. [Google Scholar] [CrossRef]
- Gribov, V. Quantization of non-Abelian gauge theories. Nucl. Phys. B 1978, 139, 1–19. [Google Scholar] [CrossRef]
- Canfora, F.E.; Dudal, D.; Justo, I.F.; Pais, P.; Rosa, L.; Vercauteren, D. Effect of the Gribov horizon on the Polyakov loop and vice versa. Eur. Phys. J. 2015, C75, 326. [Google Scholar] [CrossRef]
- Reinhardt, H. Hamiltonian finite-temperature quantum field theory from its vacuum on partially compactified space. Phys. Rev. D 2016, 94, 045016. [Google Scholar] [CrossRef]
- Christ, N.H.; Lee, T.D. Operator ordering and Feynman rules in gauge theories. Phys. Rev. D 1980, 22, 939–958. [Google Scholar] [CrossRef]
- Burgio, G.; Quandt, M.; Reinhardt, H. Coulomb-Gauge Gluon Propagator and the Gribov Formula. Phys. Rev. Lett. 2009, 102, 032002. [Google Scholar] [CrossRef]
- Campagnari, D.R.; Reinhardt, H. Non-Gaussian wave functionals in Coulomb gauge Yang-Mills theory. Phys. Rev. D 2010, 82, 105021. [Google Scholar] [CrossRef]
- Reinhardt, H. Dielectric Function of the QCD Vacuum. Phys. Rev. Lett. 2008, 101, 061602. [Google Scholar] [CrossRef] [PubMed]
- Hooft, G. Magnetic Monopoles in Unified Gauge Theories. Nucl. Phys. 1974, B79, 276–284. [Google Scholar] [CrossRef]
- Mandelstam, S. Vortices and Quark Confinement in Nonabelian Gauge Theories. Phys. Rep. 1976, 23, 245–249. [Google Scholar] [CrossRef]
- Zwanziger, D. No Confinement without Coulomb Confinement. Phys. Rev. Lett. 2003, 90, 102001. [Google Scholar] [CrossRef] [PubMed]
- Burgio, G.; Quandt, M.; Reinhardt, H.; Vogt, H. Coulomb versus physical string tension on the lattice. Phys. Rev. D 2015, 92, 034518. [Google Scholar] [CrossRef]
- Epple, D.; Reinhardt, H.; Schleifenbaum, W. Confining solution of the Dyson-Schwinger equations in Coulomb gauge. Phys. Rev. D 2007, 75, 045011. [Google Scholar] [CrossRef]
- Finger, J.R.; Mandula, J.E. Quark pair condensation and chiral symmetry breaking in QCD. Nucl. Phys. B 1982, 199, 168–188. [Google Scholar] [CrossRef]
- Adler, S.; Davis, A. Chiral symmetry breaking in Coulomb gauge QCD. Nucl. Phys. B 1984, 244, 469–491. [Google Scholar] [CrossRef]
- Alkofer, R.; Amundsen, P. Chiral symmetry breaking in an instantaneous approximation to Coulomb gauge QCD. Nucl. Phys. B 1988, 306, 305–342. [Google Scholar] [CrossRef]
- Pak, M.; Reinhardt, H. Quark sector of the QCD groundstate in Coulomb gauge. Phys. Rev. D 2013, 88, 125021. [Google Scholar] [CrossRef]
- Vastag, P.; Reinhardt, H.; Campagnari, D. Improved variational approach to QCD in Coulomb gauge. Phys. Rev. D 2016, 93, 065003. [Google Scholar] [CrossRef]
- Campagnari, D.R.; Ebadati, E.; Reinhardt, H.; Vastag, P. Revised variational approach to QCD in Coulomb gauge. Phys. Rev. D 2016, 94, 074027. [Google Scholar] [CrossRef]
- Campagnari, D.; Reinhardt, H. Variational and Dyson–Schwinger Equations of Hamiltonian Quantum Chromodynamics. Phys. Rev. D 2018, 97, 054027. [Google Scholar] [CrossRef]
- Quandt, M.; Ebadati, E.; Reinhardt, H.; Vastag, P. Chiral symmetry restoration at finite temperature within the Hamiltonian approach to QCD in Coulomb gauge. arXiv, 2018; arXiv:1806.04493. [Google Scholar]
- Reinhardt, H.; Heffner, J. Effective potential of the confinement order parameter in the Hamiltonian approach. Phys. Rev. D 2013, 88, 045024. [Google Scholar] [CrossRef]
| 1. | We thank the anonymous referee for pointing out this implicit assumption. |
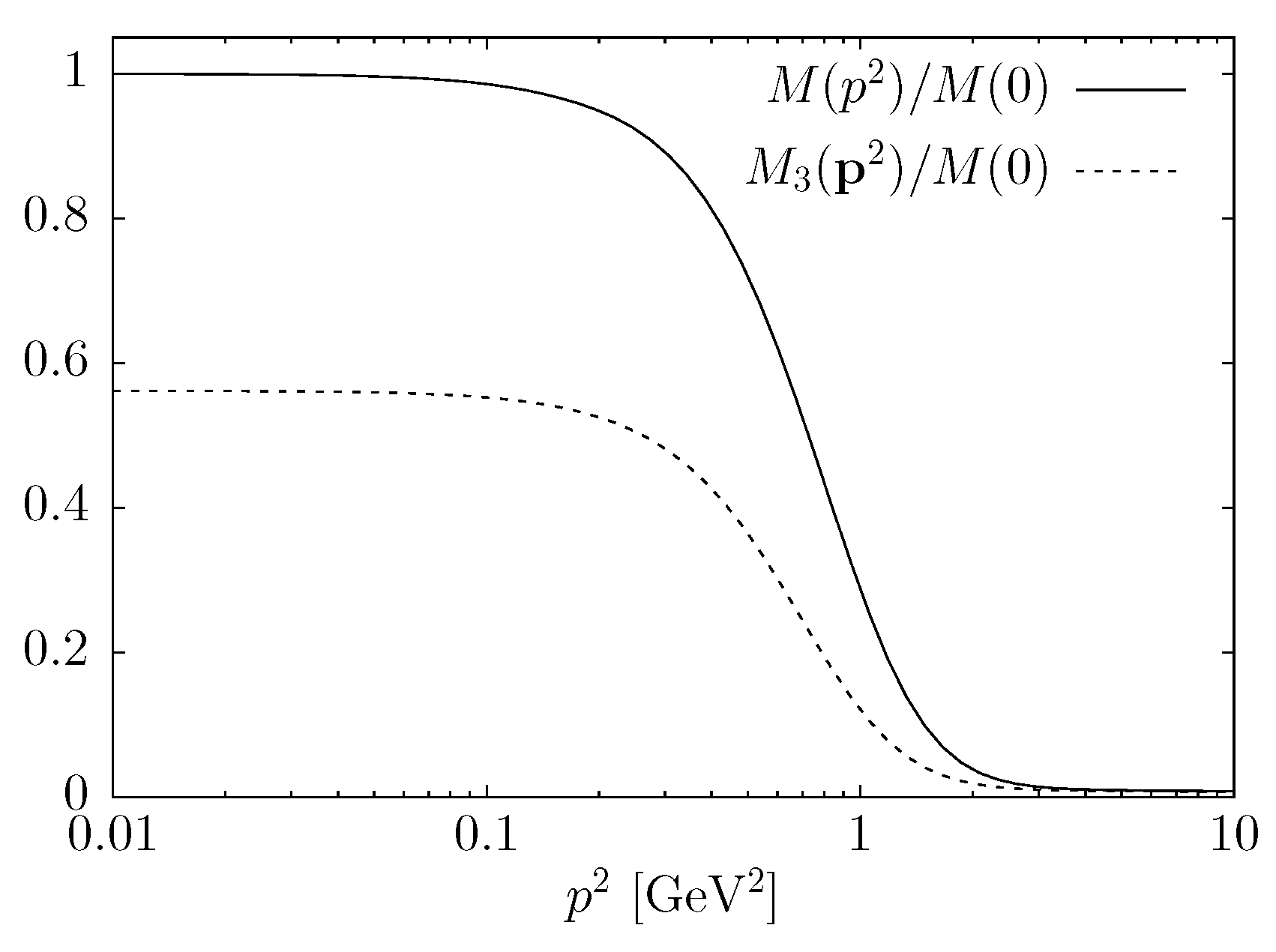
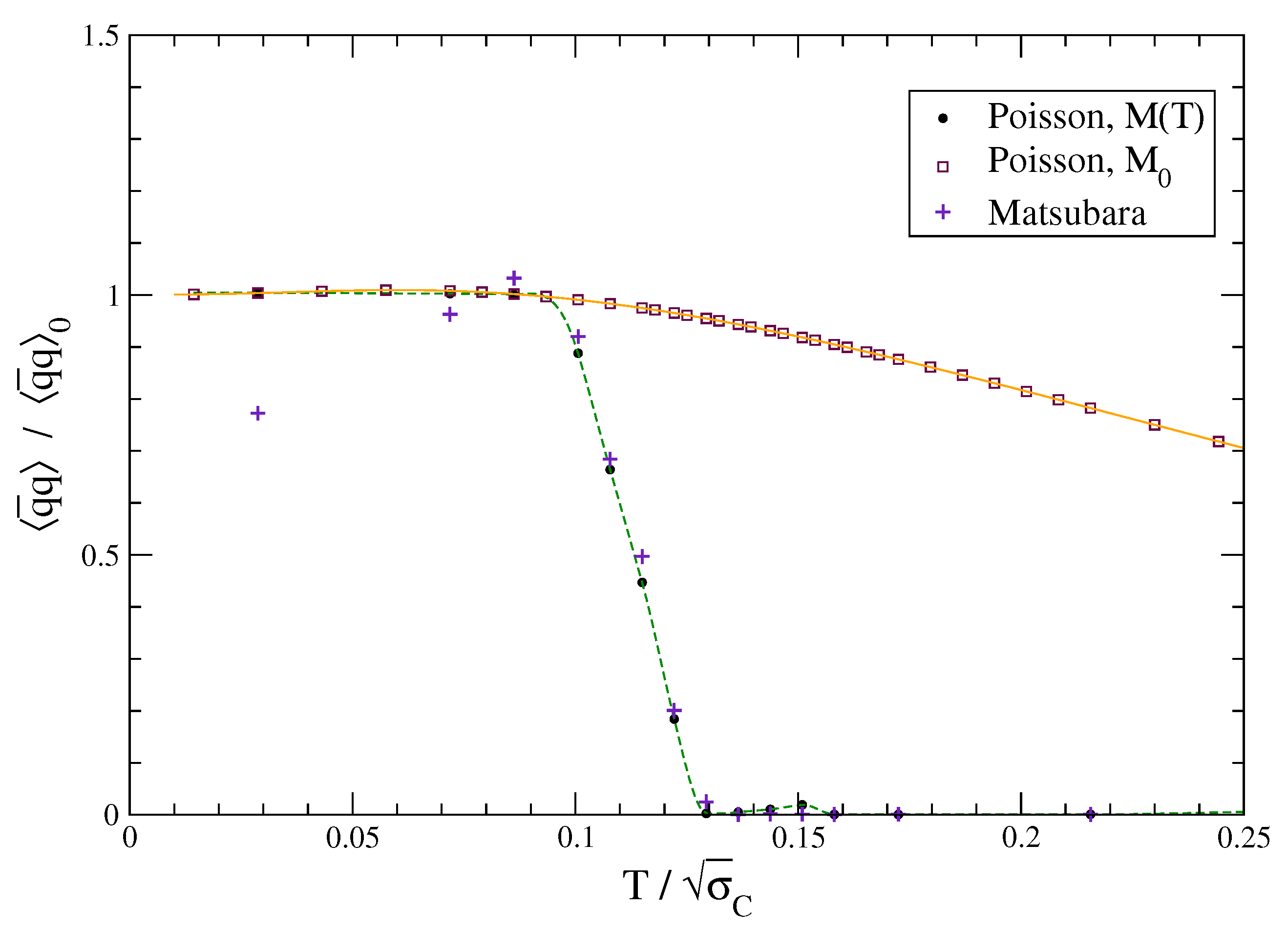
| 2. | Although we only consider a single massless quark flavor in this section, the results easily generalize to the case of massless flavors. In fact, the only change in this case would be an additional factor in the chiral condensate, which drops out when forming the ratios in Figure 7 and Figure 8. The results of this section are therefore independent of (as long as the quark flavors are massless), and it is sufficient to study . |

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reinhardt, H.; Campagnari, D.; Quandt, M. Hamiltonian Approach to QCD at Finite Temperature. Universe 2019, 5, 40. https://doi.org/10.3390/universe5020040
Reinhardt H, Campagnari D, Quandt M. Hamiltonian Approach to QCD at Finite Temperature. Universe. 2019; 5(2):40. https://doi.org/10.3390/universe5020040
Chicago/Turabian StyleReinhardt, Hugo, Davide Campagnari, and Markus Quandt. 2019. "Hamiltonian Approach to QCD at Finite Temperature" Universe 5, no. 2: 40. https://doi.org/10.3390/universe5020040
APA StyleReinhardt, H., Campagnari, D., & Quandt, M. (2019). Hamiltonian Approach to QCD at Finite Temperature. Universe, 5(2), 40. https://doi.org/10.3390/universe5020040