Entanglement and Disordered-Enhanced Topological Phase in the Kitaev Chain †
Abstract
1. Introduction
2. Model and Method
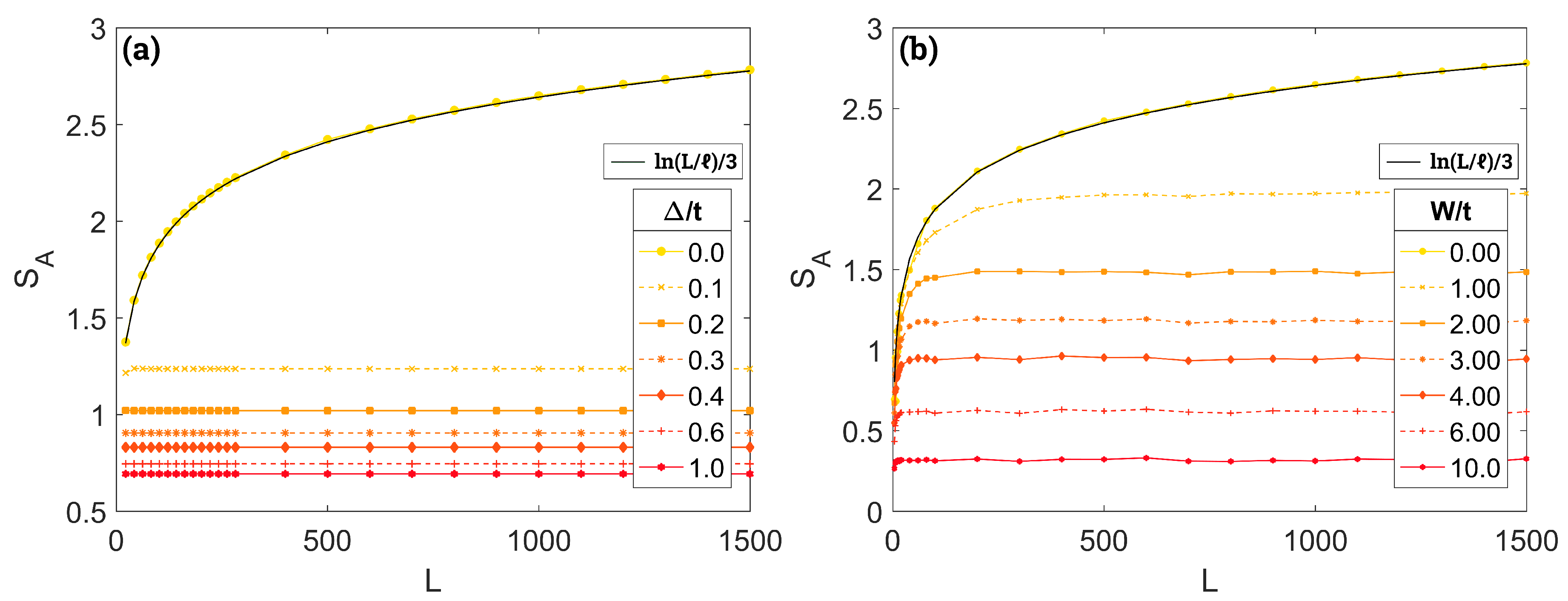
3. Entanglement Entropy
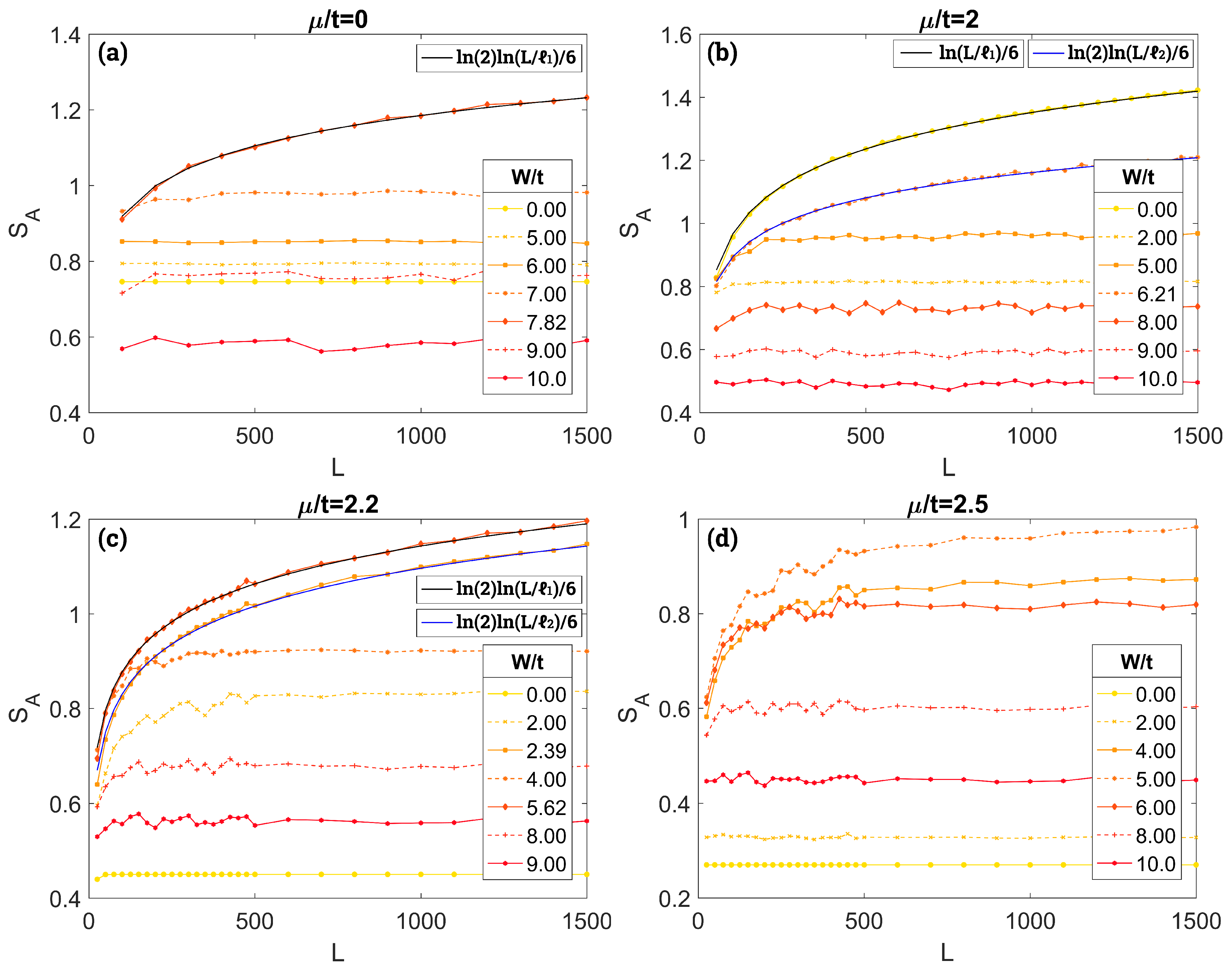
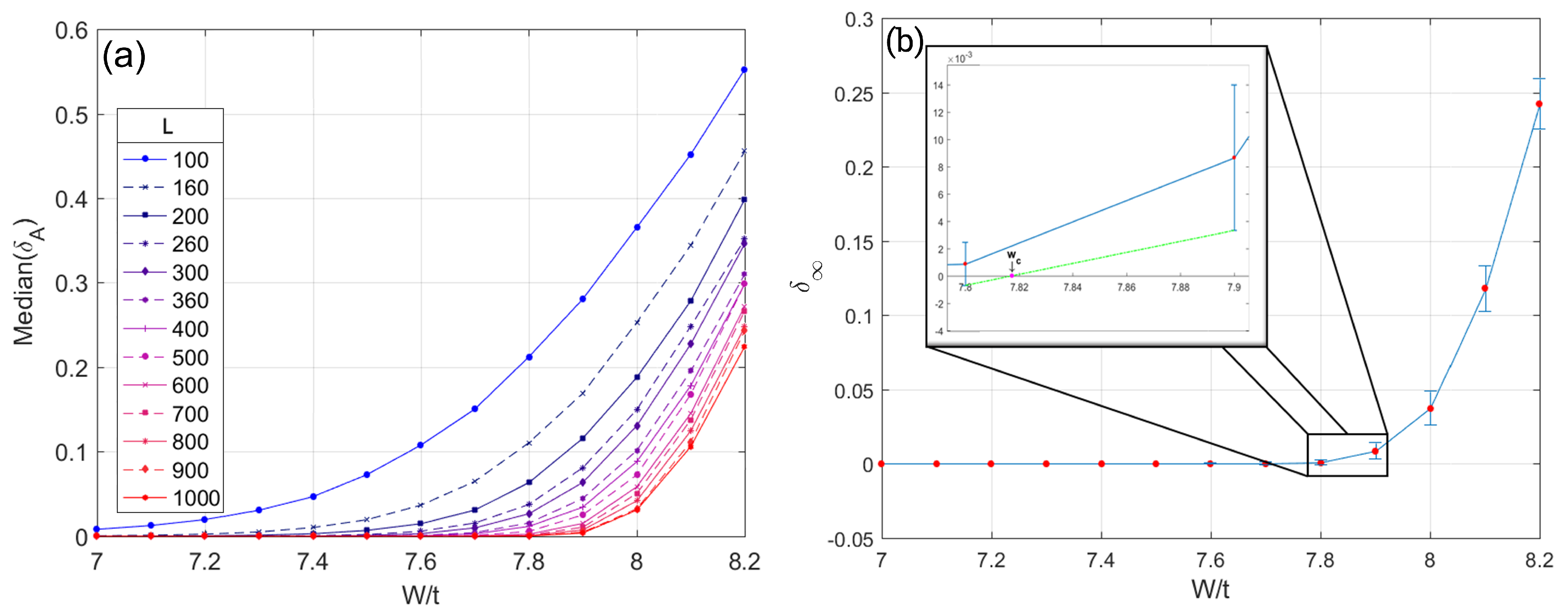
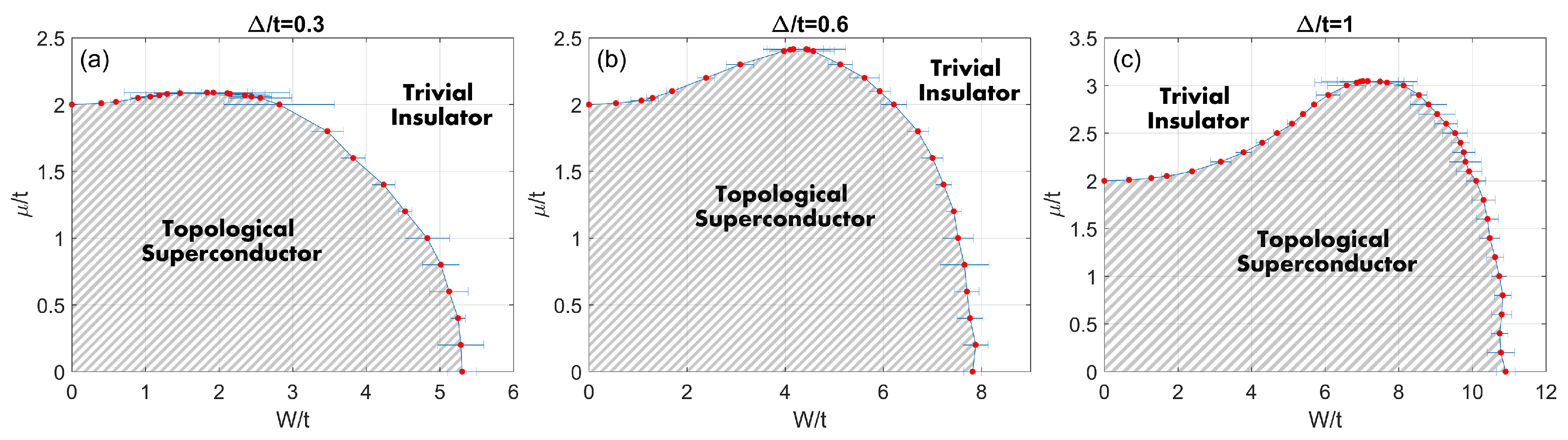
4. Phase Diagram from Entanglement
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Amico, L.; Fazio, R.; Osterloh, A.; Vedral, V. Entanglement in many-body systems. Rev. Mod. Phys. 2008, 80, 517–576. [Google Scholar] [CrossRef]
- Laflorencie, N. Quantum entanglement in condensed matter systems. Phys. Rep. 2016, 646, 1–59. [Google Scholar] [CrossRef]
- Kitaev, A.Y. Unpaired Majorana fermions in quantum wires. Physics-Uspekhi 2001, 44, 131. [Google Scholar] [CrossRef]
- Alicea, J. New directions in the pursuit of Majorana fermions in solid state systems. Rep. Prog. Phys. 2012, 75, 076501. [Google Scholar] [CrossRef] [PubMed]
- Sato, M.; Fujimoto, S. Majorana Fermions and Topology in Superconductors. J. Phys. Soc. Jpn. 2016, 85, 072001. [Google Scholar] [CrossRef]
- Aguado, R. Majorana quasiparticles in condensed matter. J. Phys. Soc. Jpn. 2017, 40, 523–593. [Google Scholar]
- Alicea, J.; Fendley, P. Topological Phases with Parafermions: Theory and Blueprints. Annu. Rev. Condens. Matter Phys. 2016, 7, 119–139. [Google Scholar] [CrossRef]
- Sarma, S.D.; Freedman, M.; Nayak, C. Majorana zero modes and topological quantum computation. Npj Quantum Inf. 2015, 1, 15001. [Google Scholar] [CrossRef]
- Lutchyn, R.M.; Sau, J.D.; Das Sarma, S. Majorana Fermions and a Topological Phase Transition in Semiconductor-Superconductor Heterostructures. Phys. Rev. Lett. 2010, 105, 077001. [Google Scholar] [CrossRef] [PubMed]
- Oreg, Y.; Refael, G.; von Oppen, F. Helical Liquids and Majorana Bound States in Quantum Wires. Phys. Rev. Lett. 2010, 105, 177002. [Google Scholar] [CrossRef] [PubMed]
- Mourik, V.; Zuo, K.; Frolov, S.M.; Plissard, S.R.; Bakkers, E.P.A.M.; Kouwenhoven, L.P. Signatures of Majorana Fermions in Hybrid Superconductor-Semiconductor Nanowire Devices. Science 2012, 336, 1003–1007. [Google Scholar] [CrossRef] [PubMed]
- Deng, M.T.; Yu, C.L.; Huang, G.Y.; Larsson, M.; Caroff, P.; Xu, H.Q. Anomalous Zero-Bias Conductance Peak in a Nb–InSb Nanowire–Nb Hybrid Device. Nano Lett. 2012, 12, 6414–6419. [Google Scholar] [CrossRef]
- Das, A.; Ronen, Y.; Most, Y.; Oreg, Y.; Heiblum, M.; Shtrikman, H. Zero-bias peaks and splitting in an Al–InAs nanowire topological superconductor as a signature of Majorana fermions. Nat. Phys. 2012, 8, 887–895. [Google Scholar] [CrossRef]
- Finck, A.D.K.; Van Harlingen, D.J.; Mohseni, P.K.; Jung, K.; Li, X. Anomalous Modulation of a Zero-Bias Peak in a Hybrid Nanowire-Superconductor Device. Phys. Rev. Lett. 2013, 110, 126406. [Google Scholar] [CrossRef] [PubMed]
- Churchill, H.O.H.; Fatemi, V.; Grove-Rasmussen, K.; Deng, M.T.; Caroff, P.; Xu, H.Q.; Marcus, C.M. Superconductor-nanowire devices from tunneling to the multichannel regime: Zero-bias oscillations and magnetoconductance crossover. Phys. Rev. B 2013, 87, 241401. [Google Scholar] [CrossRef]
- Li, H.; Haldane, F.D.M. Entanglement Spectrum as a Generalization of Entanglement Entropy: Identification of Topological Order in Non-Abelian Fractional Quantum Hall Effect States. Phys. Rev. Lett. 2008, 101, 010504. [Google Scholar] [CrossRef] [PubMed]
- Motrunich, O.; Damle, K.; Huse, D.A. Griffiths effects and quantum critical points in dirty superconductors without spin-rotation invariance: One-dimensional examples. Phys. Rev. B 2001, 63, 224204. [Google Scholar] [CrossRef]
- Gruzberg, I.A.; Read, N.; Vishveshwara, S. Localization in disordered superconducting wires with broken spin-rotation symmetry. Phys. Rev. B 2005, 71, 245124. [Google Scholar] [CrossRef]
- Akhmerov, A.R.; Dahlhaus, J.P.; Hassler, F.; Wimmer, M.; Beenakker, C.W.J. Quantized Conductance at the Majorana Phase Transition in a Disordered Superconducting Wire. Phys. Rev. Lett. 2011, 106, 057001. [Google Scholar] [CrossRef]
- Fulga, I.C.; Hassler, F.; Akhmerov, A.R.; Beenakker, C.W.J. Scattering formula for the topological quantum number of a disordered multimode wire. Phys. Rev. B 2011, 83, 155429. [Google Scholar] [CrossRef]
- Potter, A.C.; Lee, P.A. Engineering a p + ip superconductor: Comparison of topological insulator and Rashba spin-orbit-coupled materials. Phys. Rev. B 2011, 83, 184520. [Google Scholar] [CrossRef]
- Stanescu, T.D.; Lutchyn, R.M.; Das Sarma, S. Majorana fermions in semiconductor nanowires. Phys. Rev. B 2011, 84, 144522. [Google Scholar] [CrossRef]
- Brouwer, P.W.; Duckheim, M.; Romito, A.; von Oppen, F. Topological superconducting phases in disordered quantum wires with strong spin-orbit coupling. Phys. Rev. B 2011, 84, 144526. [Google Scholar] [CrossRef]
- Brouwer, P.W.; Duckheim, M.; Romito, A.; von Oppen, F. Probability Distribution of Majorana End-State Energies in Disordered Wires. Phys. Rev. Lett. 2011, 107, 196804. [Google Scholar] [CrossRef] [PubMed]
- Sau, J.D.; Tewari, S.; Das Sarma, S. Experimental and materials considerations for the topological superconducting state in electron- and hole-doped semiconductors: Searching for non-Abelian Majorana modes in 1D nanowires and 2D heterostructures. Phys. Rev. B 2012, 85, 064512. [Google Scholar] [CrossRef]
- Lobos, A.M.; Lutchyn, R.M.; Das Sarma, S. Interplay of Disorder and Interaction in Majorana Quantum Wires. Phys. Rev. Lett. 2012, 109, 146403. [Google Scholar] [CrossRef]
- Pientka, F.; Kells, G.; Romito, A.; Brouwer, P.W.; von Oppen, F. Enhanced Zero-Bias Majorana Peak in the Differential Tunneling Conductance of Disordered Multisubband Quantum-Wire/Superconductor Junctions. Phys. Rev. Lett. 2012, 109, 227006. [Google Scholar] [CrossRef]
- Pientka, F.; Romito, A.; Duckheim, M.; Oreg, Y.; von Oppen, F. Signatures of topological phase transitions in mesoscopic superconducting rings. New J. Phys. 2013, 15, 025001. [Google Scholar] [CrossRef]
- DeGottardi, W.; Sen, D.; Vishveshwara, S. Majorana Fermions in Superconducting 1D Systems Having Periodic, Quasiperiodic, and Disordered Potentials. Phys. Rev. Lett. 2013, 110, 146404. [Google Scholar] [CrossRef]
- Neven, P.; Bagrets, D.; Altland, A. Quasiclassical theory of disordered multi-channel Majorana quantum wires. New J. Phys. 2013, 15, 055019. [Google Scholar] [CrossRef]
- Rieder, M.T.; Brouwer, P.W.; Adagideli, I. Reentrant topological phase transitions in a disordered spinless superconducting wire. Phys. Rev. B 2013, 88, 060509. [Google Scholar] [CrossRef]
- Sau, J.D.; Das Sarma, S. Density of states of disordered topological superconductor-semiconductor hybrid nanowires. Phys. Rev. B 2013, 88, 064506. [Google Scholar] [CrossRef]
- DeGottardi, W.; Thakurathi, M.; Vishveshwara, S.; Sen, D. Majorana fermions in superconducting wires: Effects of long-range hopping, broken time-reversal symmetry, and potential landscapes. Phys. Rev. B 2013, 88, 165111. [Google Scholar] [CrossRef]
- Chevallier, D.; Simon, P.; Bena, C. From Andreev bound states to Majorana fermions in topological wires on superconducting substrates: A story of mutation. Phys. Rev. B 2013, 88, 165401. [Google Scholar] [CrossRef]
- Jacquod, P.; Büttiker, M. Signatures of Majorana fermions in hybrid normal-superconducting rings. Phys. Rev. B 2013, 88, 241409. [Google Scholar] [CrossRef]
- Adagideli, I.; Wimmer, M.; Teker, A. Effects of electron scattering on the topological properties of nanowires: Majorana fermions from disorder and superlattices. Phys. Rev. B 2014, 89, 144506. [Google Scholar] [CrossRef]
- Hui, H.Y.; Sau, J.D.; Das Sarma, S. Generalized Eilenberger theory for Majorana zero-mode-carrying disordered p-wave superconductors. Phys. Rev. B 2014, 90, 064516. [Google Scholar] [CrossRef]
- Crépin, F.M.C.; Zaránd, G.; Simon, P. Nonperturbative phase diagram of interacting disordered Majorana nanowires. Phys. Rev. B 2014, 90, 121407. [Google Scholar] [CrossRef]
- Gergs, N.M.; Fritz, L.; Schuricht, D. Topological order in the Kitaev/Majorana chain in the presence of disorder and interactions. Phys. Rev. B 2016, 93, 075129. [Google Scholar] [CrossRef]
- Hegde, S.S.; Vishveshwara, S. Majorana wave-function oscillations, fermion parity switches, and disorder in Kitaev chains. Phys. Rev. B 2016, 94, 115166. [Google Scholar] [CrossRef]
- Bagrets, D.; Altland, A.; Kamenev, A. Sinai Diffusion at Quasi-1D Topological Phase Transitions. Phys. Rev. Lett. 2016, 117, 196801. [Google Scholar] [CrossRef] [PubMed]
- Grabsch, A.; Texier, C. Topological phase transitions in the 1D multichannel Dirac equation with random mass and a random matrix model. Europhys. Lett. 2016, 116, 17004. [Google Scholar] [CrossRef]
- Pekerten, B.; Teker, A.; Bozat, O.; Wimmer, M.; Adagideli, I. Disorder-induced topological transitions in multichannel Majorana wires. Phys. Rev. B 2017, 95, 064507. [Google Scholar] [CrossRef]
- Brzezicki, W.; Oleś, A.M.; Cuoco, M. Driving topological phases by spatially inhomogeneous pairing centers. Phys. Rev. B 2017, 95, 140506. [Google Scholar] [CrossRef]
- McGinley, M.; Knolle, J.; Nunnenkamp, A. Robustness of Majorana edge modes and topological order: Exact results for the symmetric interacting Kitaev chain with disorder. Phys. Rev. B 2017, 96, 241113. [Google Scholar] [CrossRef]
- Lieu, S.; Lee, D.K.K.; Knolle, J. Disorder protected and induced local zero-modes in longer-range Kitaev chains. Phys. Rev. B 2018, 98, 134507. [Google Scholar] [CrossRef]
- Monthus, C. Topological phase transitions in random Kitaev α-chains. J. Phys. A Math. Theor. 2018, 51, 465301. [Google Scholar] [CrossRef]
- Wang, J.; Chakravarty, S. Binary disorder in quantum Ising chains and induced Majorana zero modes. arXiv, 2018; arXiv:1808.04481. [Google Scholar]
- Mishra, U.; Jafari, R.; Akbari, A. Disordered Kitaev chain with long-range pairing: Loschimdt echo revivals and dynamical phase transitions. arXiv, 2018; arXiv:1810.06236. [Google Scholar]
- Peschel, I. Calculation of reduced density matrices from correlation functions. J. Phys. A Math. Gen. 2003, 36, L205. [Google Scholar] [CrossRef]
- Holzhey, C.; Larsen, F.; Wilczek, F. Geometric and renormalized entropy in conformal field theory. Nucl. Phys. B 1994, 424, 443–467. [Google Scholar] [CrossRef]
- Vidal, G.; Latorre, J.I.; Rico, E.; Kitaev, A. Entanglement in Quantum Critical Phenomena. Phys. Rev. Lett. 2003, 90, 227902. [Google Scholar] [CrossRef] [PubMed]
- Calabrese, P.; Cardy, J. Entanglement entropy and quantum field theory. J. Stat. Mech. Theory Exp. 2004, 2004, P06002. [Google Scholar] [CrossRef]
- Calabrese, P.; Cardy, J. Entanglement entropy and conformal field theory. J. Phys. A Math. Theor. 2009, 42, 504005. [Google Scholar] [CrossRef]
- Ma, S.k.; Dasgupta, C.; Hu, C.k. Random Antiferromagnetic Chain. Phys. Rev. Lett. 1979, 43, 1434–1437. [Google Scholar] [CrossRef]
- Dasgupta, C.; Ma, S.K. Low-temperature properties of the random Heisenberg antiferromagnetic chain. Phys. Rev. B 1980, 22, 1305–1319. [Google Scholar] [CrossRef]
- Fisher, D.S. Random antiferromagnetic quantum spin chains. Phys. Rev. B 1994, 50, 3799–3821. [Google Scholar] [CrossRef]
- Fisher, D.S. Critical behavior of random transverse-field Ising spin chains. Phys. Rev. B 1995, 51, 6411–6461. [Google Scholar] [CrossRef]
- Refael, G.; Moore, J.E. Entanglement Entropy of Random Quantum Critical Points in One Dimension. Phys. Rev. Lett. 2004, 93, 260602. [Google Scholar] [CrossRef]
- Refael, G.; Moore, J.E. Criticality and entanglement in random quantum systems. J. Phys. A Math. Theor. 2009, 42, 504010. [Google Scholar] [CrossRef]
- Torlai, G.; McAlpine, K.D.; De Chiara, G. Schmidt gap in random spin chains. Phys. Rev. B 2018, 98, 085153. [Google Scholar] [CrossRef]
- Berkovits, R. Entanglement Properties and Quantum Phases for a Fermionic Disordered One-Dimensional Wire with Attractive Interactions. Phys. Rev. Lett. 2015, 115, 206401. [Google Scholar] [CrossRef] [PubMed]
- Leiman, S.; Eisenbach, A.; Berkovits, R. Correspondence between many-particle excitations and the entanglement spectrum of disordered ballistic one-dimensional systems. Europhys. Lett. 2015, 112, 46003. [Google Scholar] [CrossRef]
- Berkovits, R. Low eigenvalues of the entanglement Hamiltonian, localization length, and rare regions in one-dimensional disordered interacting systems. Phys. Rev. B 2018, 97, 115408. [Google Scholar] [CrossRef]
- Friedman, B.; Berkovits, R. Entanglement entropy distribution in the strongly disordered one-dimensional Anderson model. arXiv, 2018; arXiv:0812.0006. [Google Scholar]
- Berkovits, R. Extracting many-particle entanglement entropy from observables using supervised machine learning. arXiv, 2018; arXiv:1810.00346. [Google Scholar] [CrossRef]
- White, S.R. Density matrix formulation for quantum renormalization groups. Phys. Rev. Lett. 1992, 69, 2863–2866. [Google Scholar] [CrossRef]
- Schollwöck, U. The density-matrix renormalization group in the age of matrix product states. Ann. Phys. 2011, 326, 96–192. [Google Scholar] [CrossRef]
- Goldstein, M.; Berkovits, R. On-site interaction effects on localization: Dominance of nonuniversal contributions. Phys. Rev. B 2003, 68, 245116. [Google Scholar] [CrossRef]
- Lian, B.; Wang, J.; Sun, X.Q.; Vaezi, A.; Zhang, S.C. Quantum phase transition of chiral Majorana fermions in the presence of disorder. Phys. Rev. B 2018, 97, 125408. [Google Scholar] [CrossRef]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Levy, L.; Goldstein, M. Entanglement and Disordered-Enhanced Topological Phase in the Kitaev Chain. Universe 2019, 5, 33. https://doi.org/10.3390/universe5010033
Levy L, Goldstein M. Entanglement and Disordered-Enhanced Topological Phase in the Kitaev Chain. Universe. 2019; 5(1):33. https://doi.org/10.3390/universe5010033
Chicago/Turabian StyleLevy, Liron, and Moshe Goldstein. 2019. "Entanglement and Disordered-Enhanced Topological Phase in the Kitaev Chain" Universe 5, no. 1: 33. https://doi.org/10.3390/universe5010033
APA StyleLevy, L., & Goldstein, M. (2019). Entanglement and Disordered-Enhanced Topological Phase in the Kitaev Chain. Universe, 5(1), 33. https://doi.org/10.3390/universe5010033



