PHENIX Results of Three-Particle Bose-Einstein Correlations in \({\sqrt{s_{NN}}}\) = 200 GeV Au+Au Collisions
Abstract
:1. Introduction
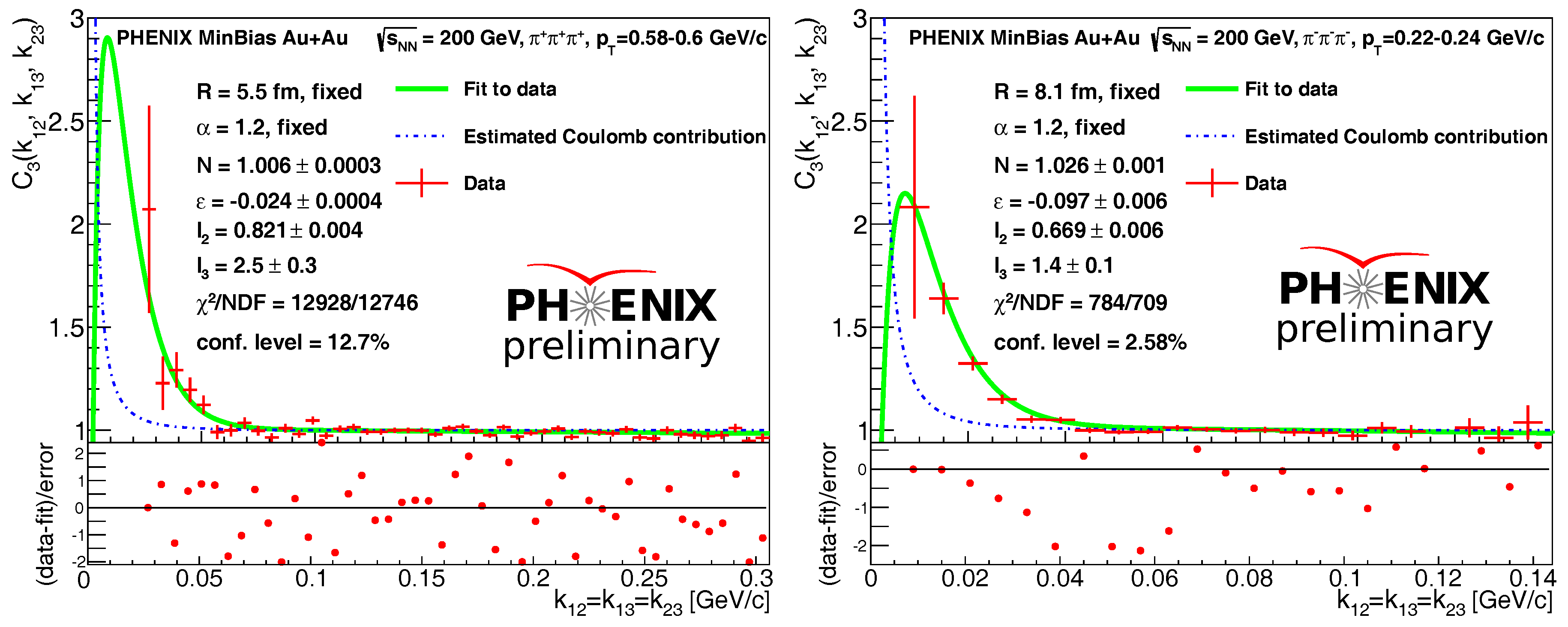
2. Results
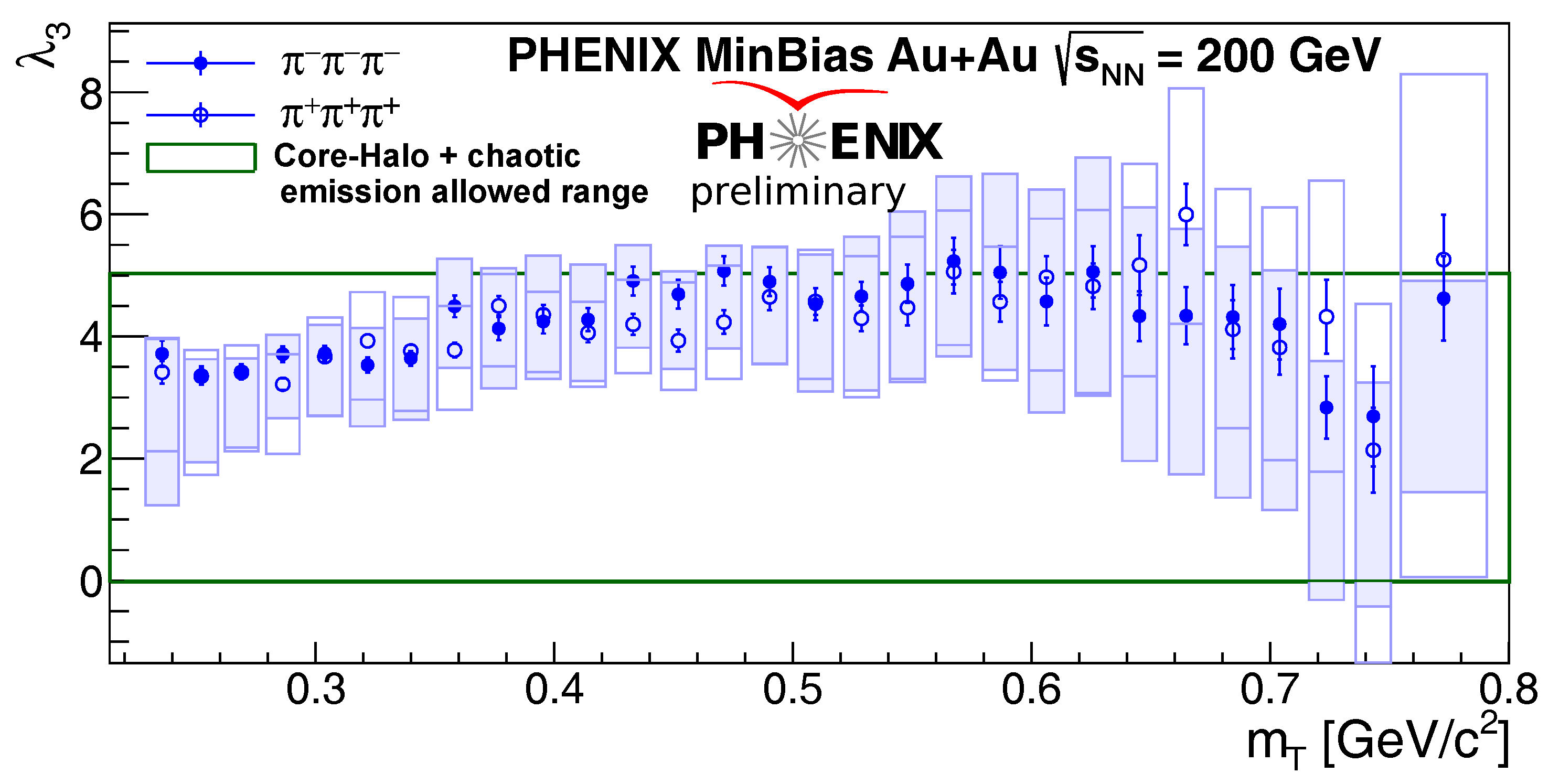
2.1. Three-Particle Correlation Strength
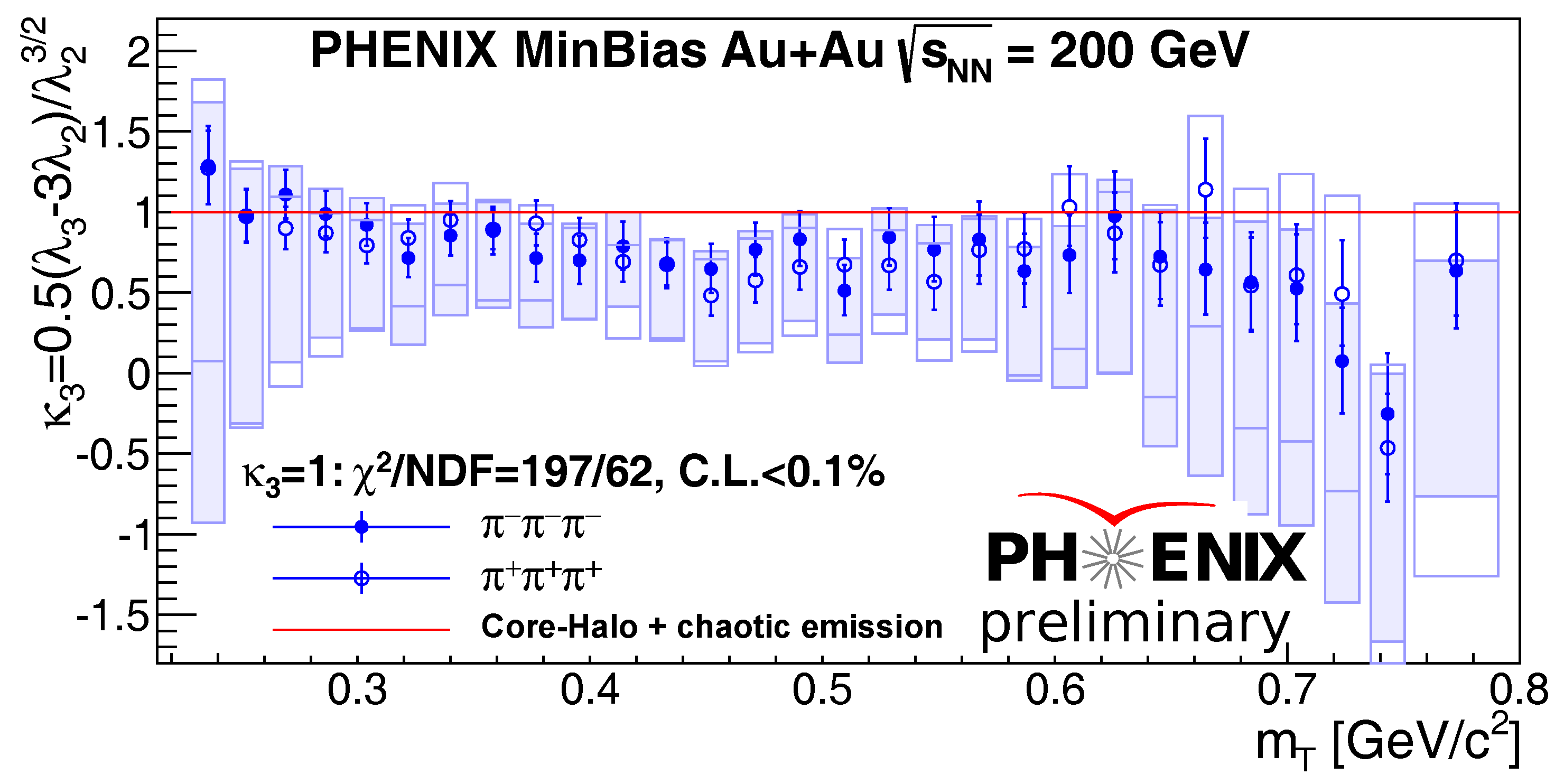
2.2. core-halo Independent Parameter
3. Conclusions
Acknowledgments
Conflicts of Interest
References
- Gyulassy, M.; Kauffmann, S.K.; Wilson, L.W. Pion interferometry of nuclear collisions. Phys. Rev. C 1979, 20, 2267–2292. [Google Scholar] [CrossRef]
- Boal, D.H.; Gelbke, C.K.; Jennings, B.K. Intensity interferometry in subatomic physics. Rev. Mod. Phys. 1990, 62, 553–602. [Google Scholar] [CrossRef]
- Baym, G. The physics of Hanbury Brown-Twiss intensity interferometry: From stars to nuclear collisions. Acta Phys. Pol. B 1998, 29, 1839–1884. [Google Scholar]
- Kittel, W. Bose-Einstein Correlations in Z Fragmentation and Other Reactions. Acta Phys. Pol. B 2001, 32, 3927–3972. [Google Scholar]
- Csörgő, T. Particle Interferometry from 40 MeV to 40 TeV. Heavy Ion Phys. 2002, 15, 1–80. [Google Scholar] [CrossRef]
- Wiedemann, U.; Scotto, P.; Heinz, U. Transverse momentum dependence of Hanbury-Brown-Twiss correlation radii. Phys. Rev. C 1996, 53, 918–931. [Google Scholar] [CrossRef]
- Csörgő, T.; Lörstad, B. Bose-Einstein correlations for three-dimensionally expanding, cylindrically symmetric, finite systems. Phys. Rev. C 1996, 54, 1390–1403. [Google Scholar] [CrossRef]
- Adare, A.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Akimoto, R.; Alexander, J.; Alfred, M.; Al-Ta’ani, H.; Angerami, A.; Aoki, K.; et al. Lévy-stable two-pion Bose-Einstein correlations in = 200 GeV Au+Au collisions. arXiv, 2017; arXiv:nucl-ex/1709.05649. [Google Scholar]
- Bolz, J.; Ornik, U.; Plumer, M.; Schlei, B.R.; Weiner, R.M. Resonance decays and partial coherence in Bose-Einstein correlations. Phys. Rev. D 1993, 47, 3860–3870. [Google Scholar] [CrossRef]
- Csörgő, T.; Lörstad, B.; Zimányi, J. Bose-Einstein correlations for systems with large halo. Z. Phys. C 1996, 71, 491–497. [Google Scholar] [Green Version]
- Csanád, M. Measurement and analysis of two- and three-particle correlations. Nucl. Phys. A 2006, 774, 611–614. [Google Scholar] [CrossRef]
- Nolan, J.P. Univariate Stable Distributions. In Stable Distributions—Models for Heavy Tailed Data; Publishing House: Boston, MA, USA, 2018. [Google Scholar]
- Metzler, R.; Barkai, E.; Klafter, J. Anomalous Diffusion and Relaxation Close to Thermal Equilibrium: A Fractional Fokker-Planck Equation Approach. Phys. Rev. Lett. 1999, 82, 3563–3567. [Google Scholar] [CrossRef]
- Csörgő, T.; Hegyi, S.; Zajc, W.A. Bose-Einstein Correlations for Levy stable source distributions. Eur. Phys. J. C 2004, 36, 67–78. [Google Scholar] [CrossRef]
- Csanád, M.; Csörgő, T.; Nagy, M. Anomalous diffusion of pions at RHIC. Braz. J. Phys. 2007, 37, 1002–1013. [Google Scholar] [CrossRef]
- Csanád, M. Lévy femtoscopy with PHENIX at RHIC. Universe 2017, 3, 85. [Google Scholar] [CrossRef]
- Gangadharan, D. Techniques for multiboson interferometry. Phys. Rev. C 2015, 92, 014902. [Google Scholar] [CrossRef]
- Alt, E.O.; Csörgo, T.; Lörstad, B.; Schmidt-Sorensen, J. Coulomb corrections to the three-body correlation function in high-energy heavy ion reactions. Phys. Lett. B 1999, 458, 407–414. [Google Scholar] [CrossRef]
- Boggild, H.; Boissevainb, J.; Doddc, J.; Esumid, S.; Fabjane, C.W.; Ferencef, D.; Franzg, A.; Hardtkeh, D.; van Heckeb, H.; Humanich, T.J.; et al. Three pion correlations in sulphur lead collisions at the CERN SPS. Phys. Lett. B 1999, 455, 77–83. [Google Scholar] [CrossRef]
- Csörgo, T.; Lörstad, B.; Schmid-Sørensen, J.; Ster, A. Partial coherence in the core-halo picture of Bose– Einstein n-particle correlations. Eur. Phys. J. C 1999, 9, 275–281. [Google Scholar] [CrossRef]
- Kincses, D.; PHENIX Collaboration. PHENIX results on Lévy analysis of Bose-Einstein correlation functions. Acta Phys. Polon. Supp. 2017, 10, 627. [Google Scholar] [CrossRef]

© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Novák, T. PHENIX Results of Three-Particle Bose-Einstein Correlations in \({\sqrt{s_{NN}}}\) = 200 GeV Au+Au Collisions. Universe 2018, 4, 57. https://doi.org/10.3390/universe4030057
Novák T. PHENIX Results of Three-Particle Bose-Einstein Correlations in \({\sqrt{s_{NN}}}\) = 200 GeV Au+Au Collisions. Universe. 2018; 4(3):57. https://doi.org/10.3390/universe4030057
Chicago/Turabian StyleNovák, Tamás. 2018. "PHENIX Results of Three-Particle Bose-Einstein Correlations in \({\sqrt{s_{NN}}}\) = 200 GeV Au+Au Collisions" Universe 4, no. 3: 57. https://doi.org/10.3390/universe4030057
APA StyleNovák, T. (2018). PHENIX Results of Three-Particle Bose-Einstein Correlations in \({\sqrt{s_{NN}}}\) = 200 GeV Au+Au Collisions. Universe, 4(3), 57. https://doi.org/10.3390/universe4030057



